Simulation and Experimental Study of Gas Turbine Blade Tenon-Root Detachment on Spin Test
Abstract
1. Introduction
2. Numerical Simulation
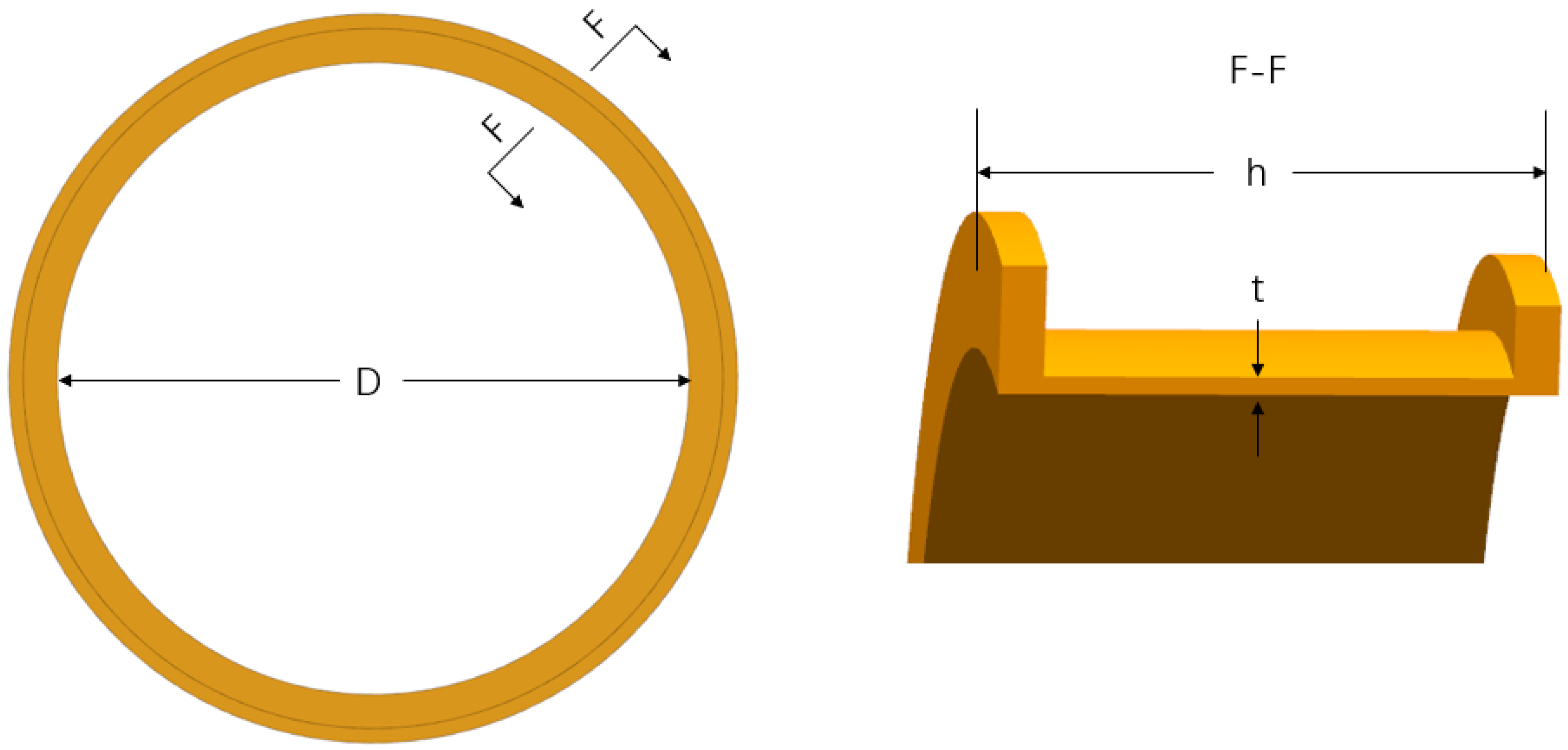
2.1. Containment-Coefficient Methods
2.2. Containment Simulation Analysis
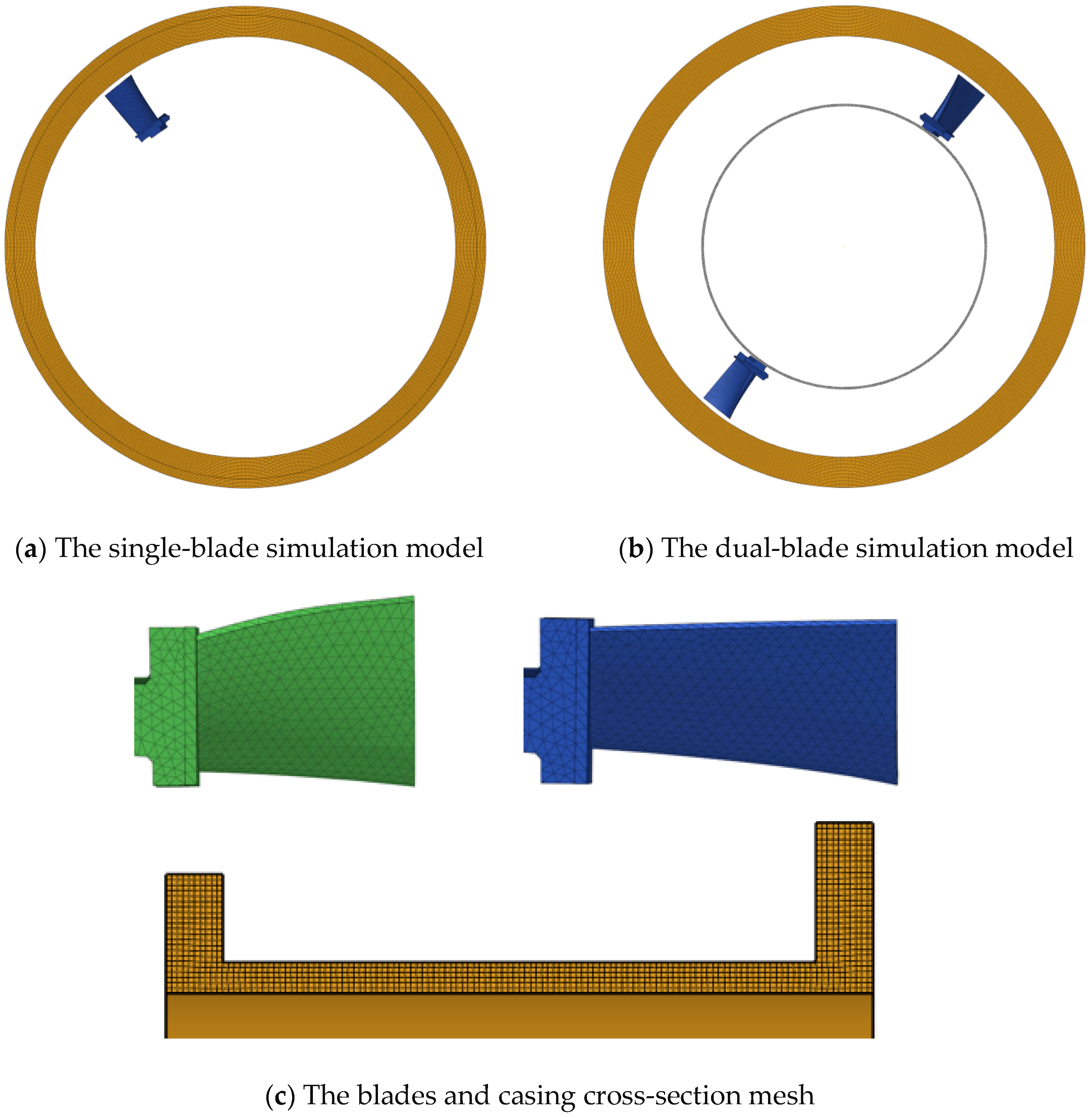
2.2.1. The Single-Blade Containment Numerical Simulation

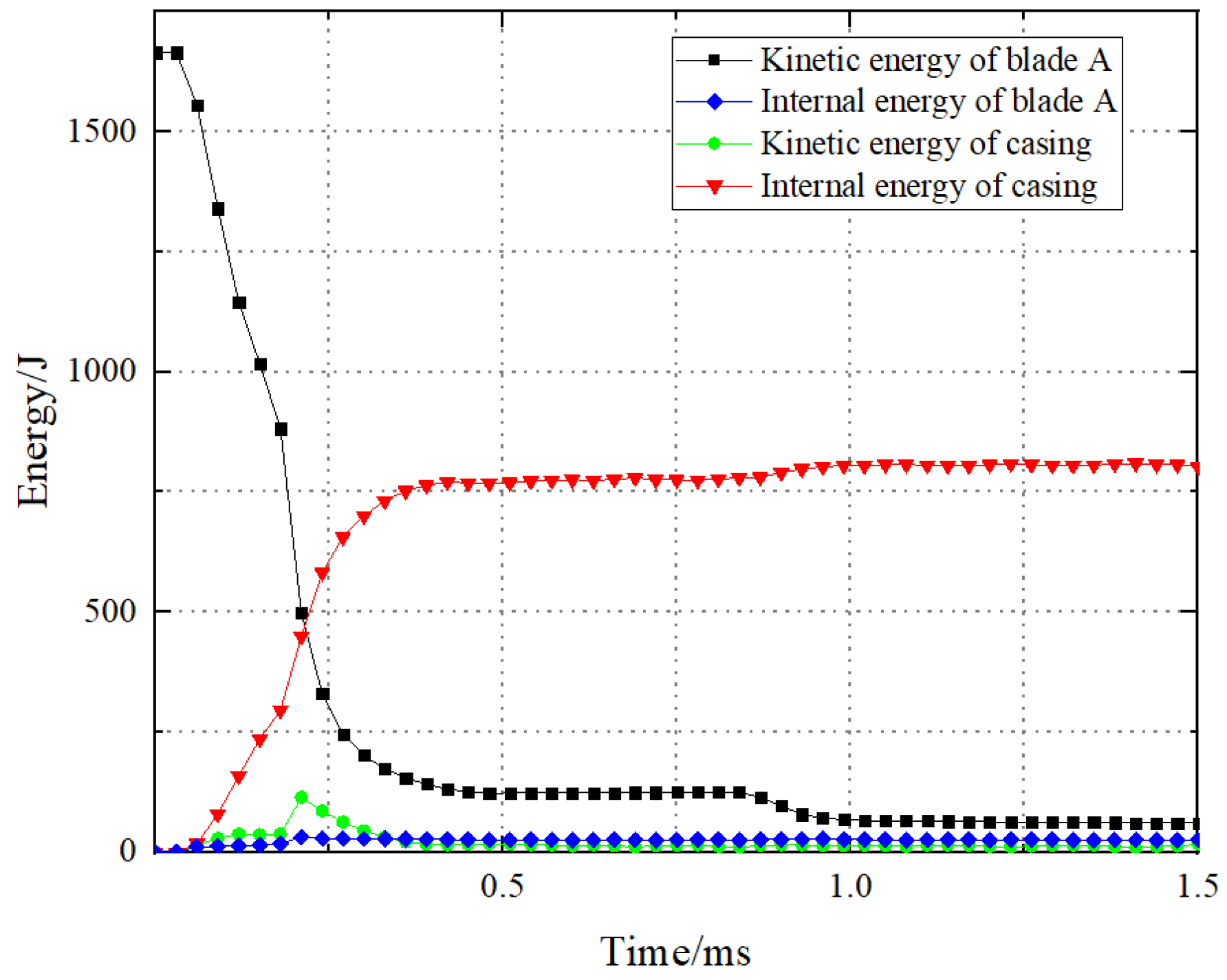
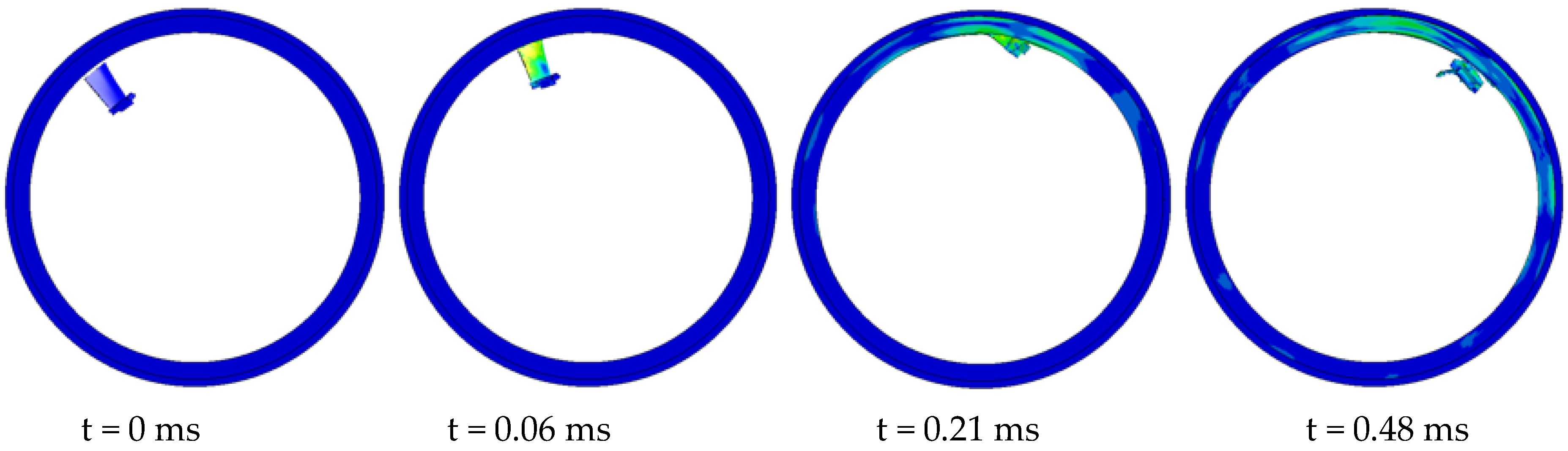
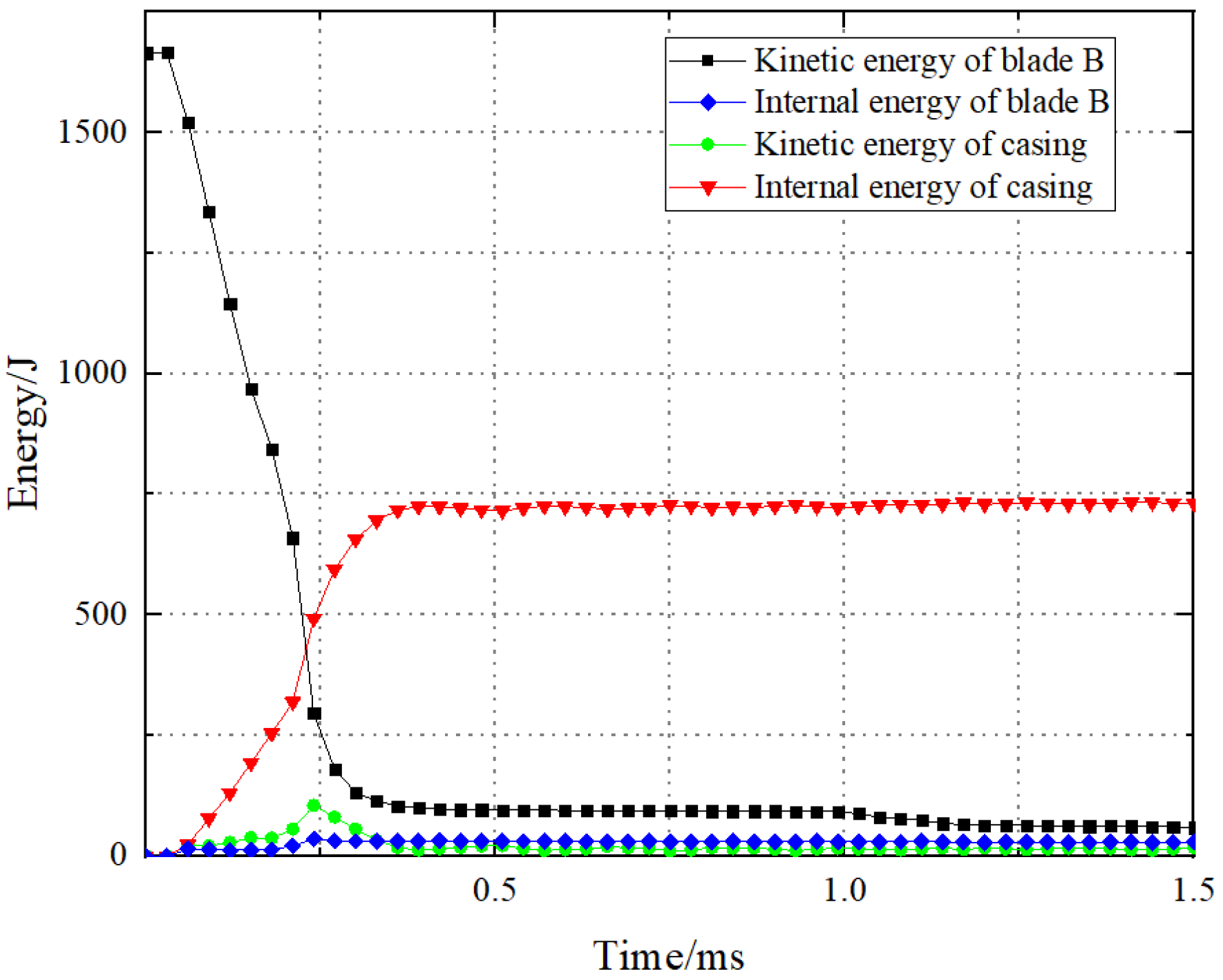
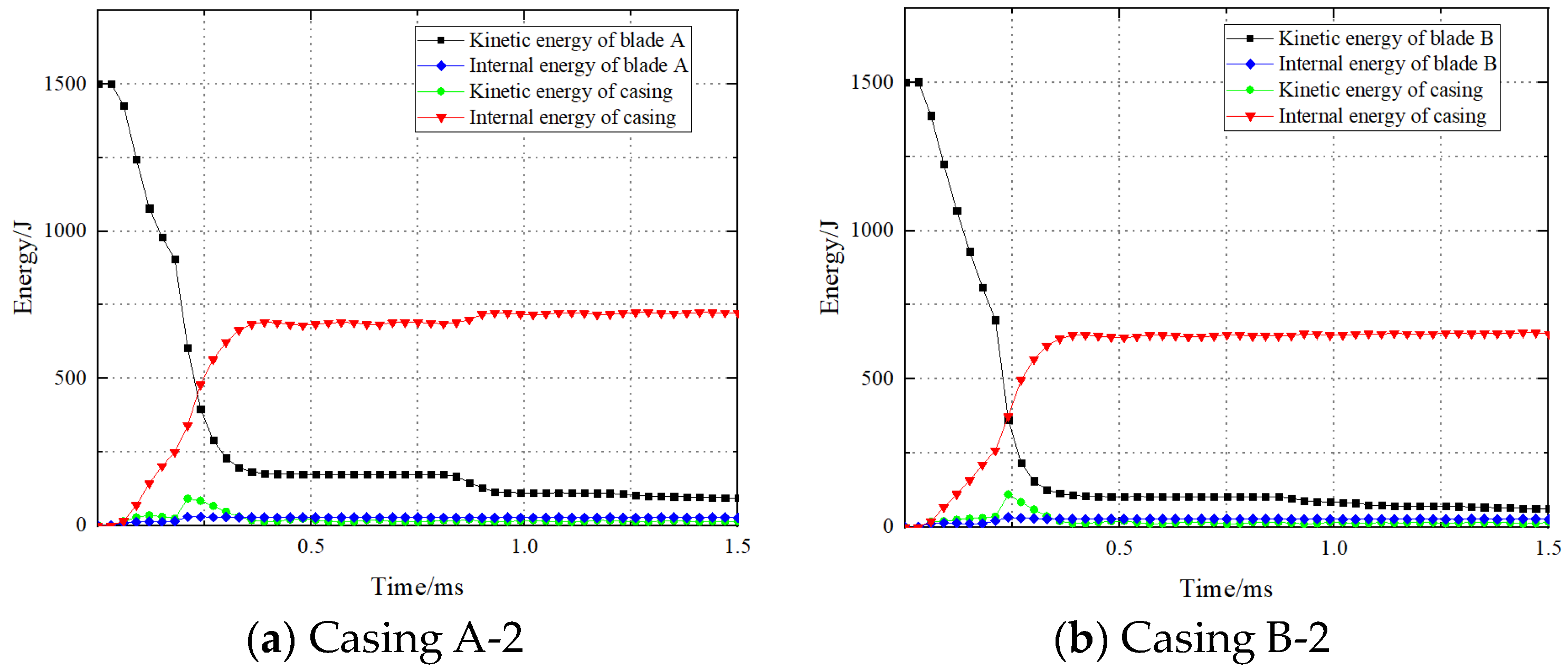
2.2.2. The Dual-Blade Containment Numerical Simulation
3. Test Verification
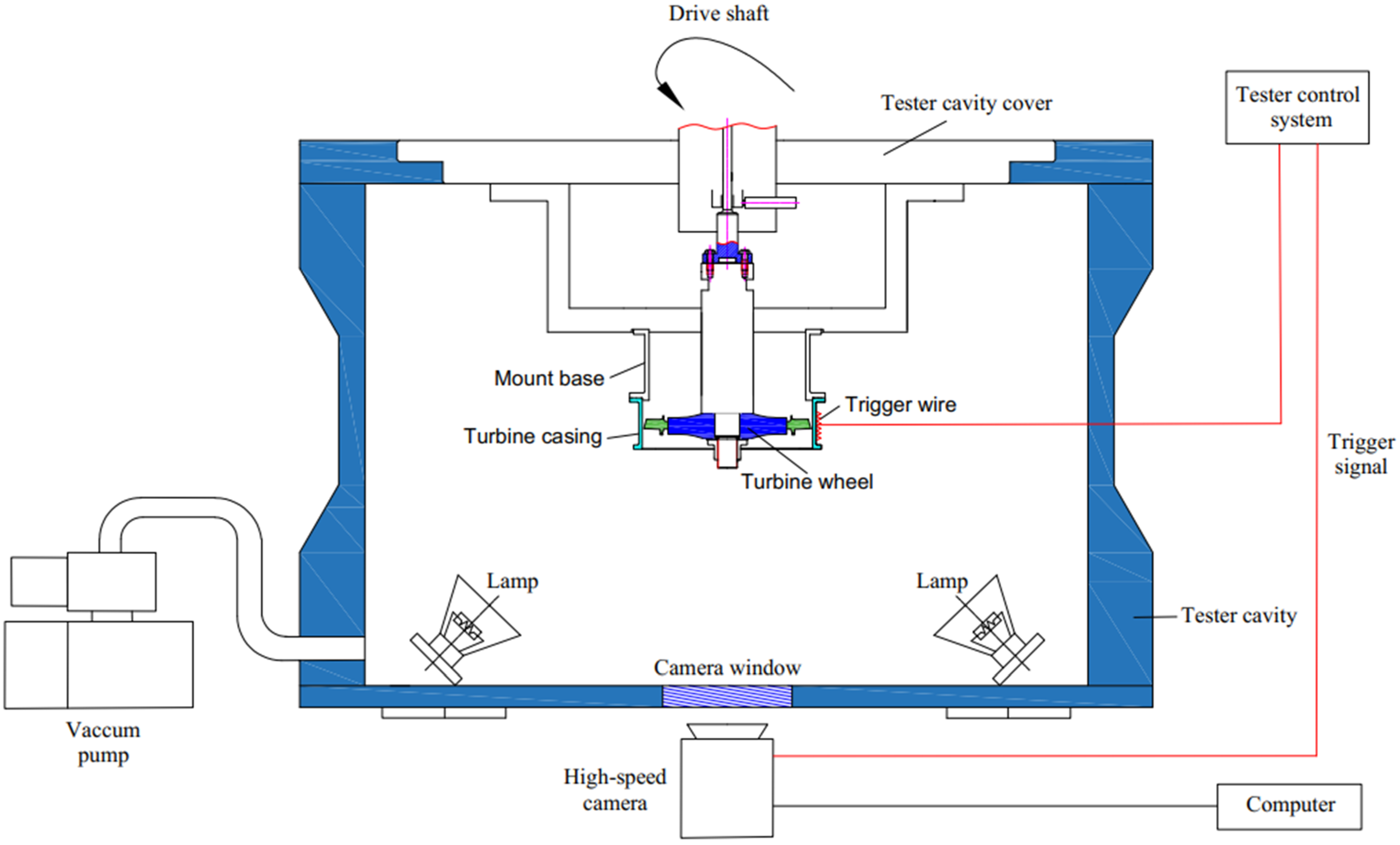
3.1. Containment Test Set-Up
3.2. Experimental Results
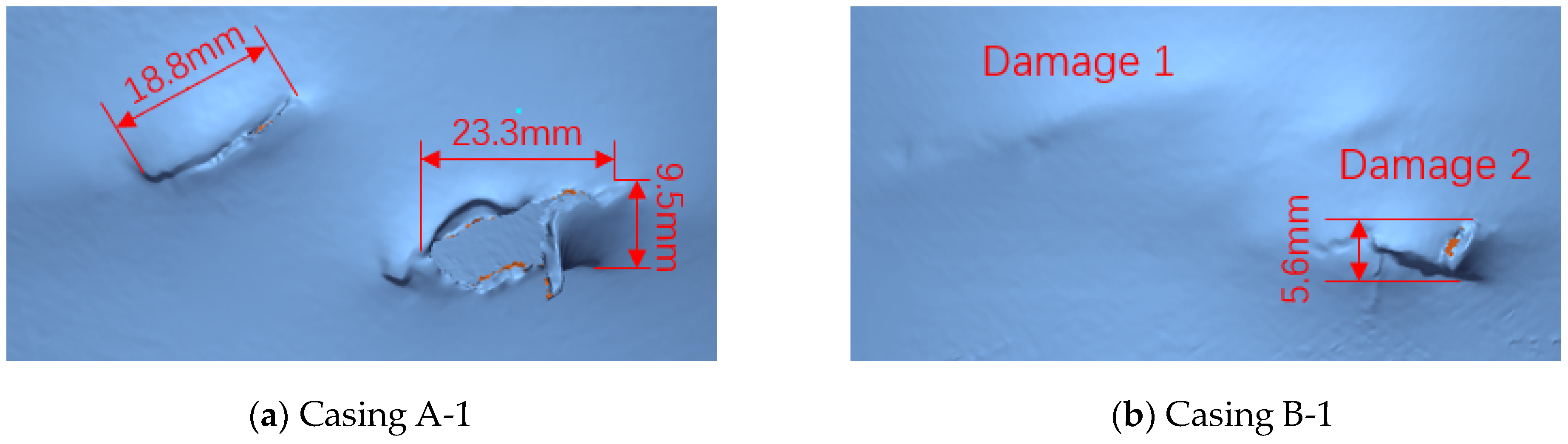
3.2.1. The Single-Blade Containment Test Results
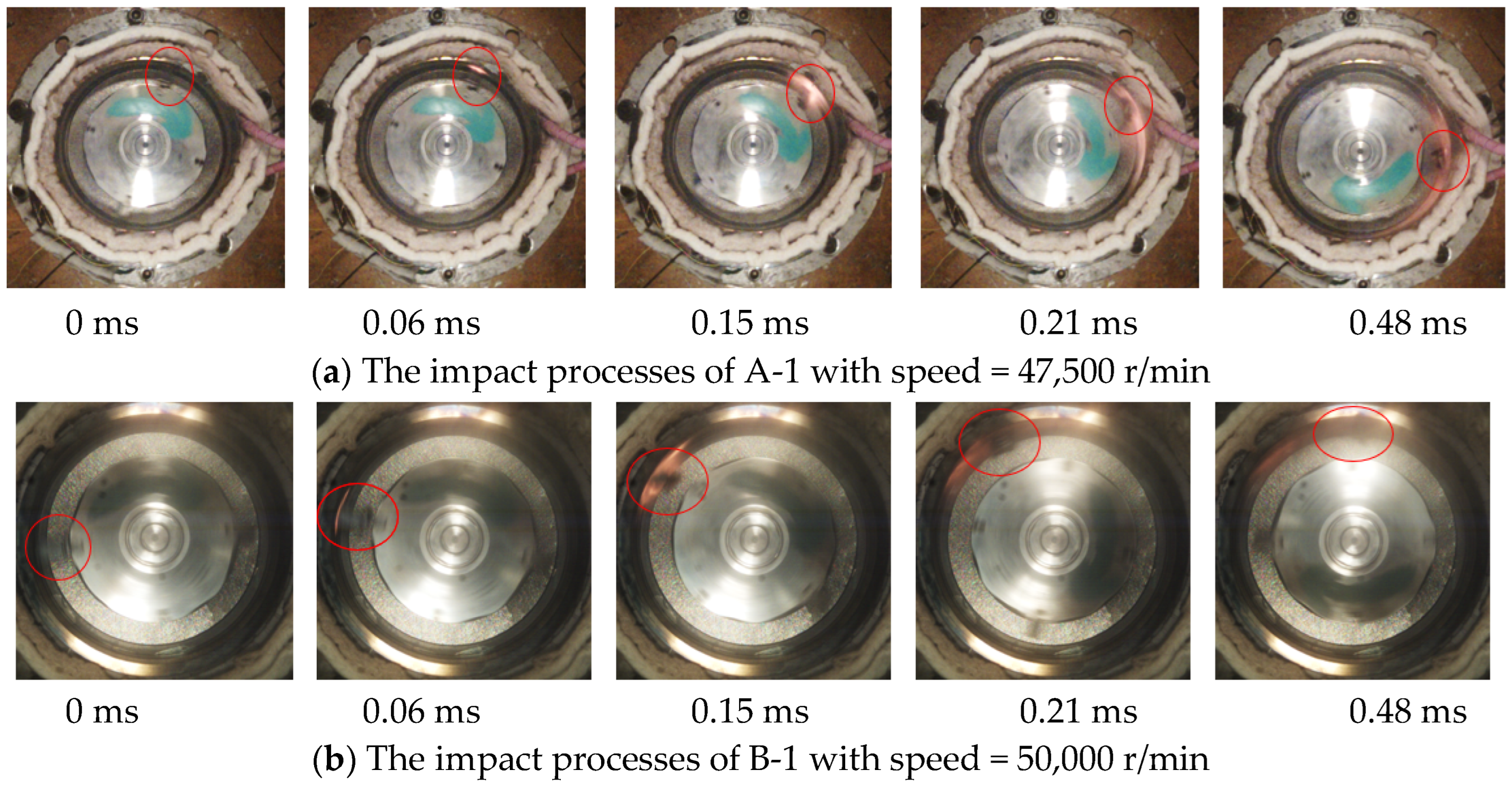

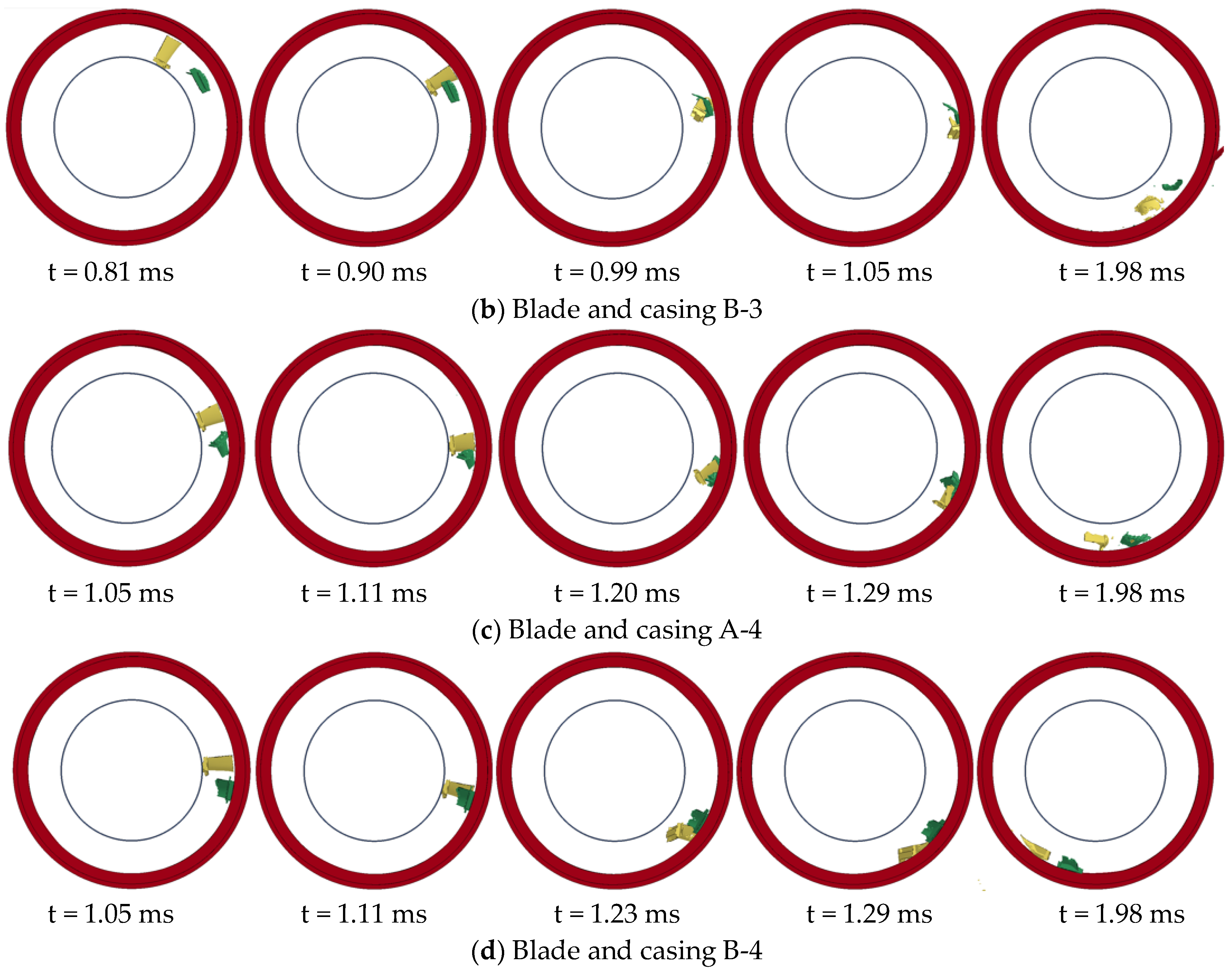
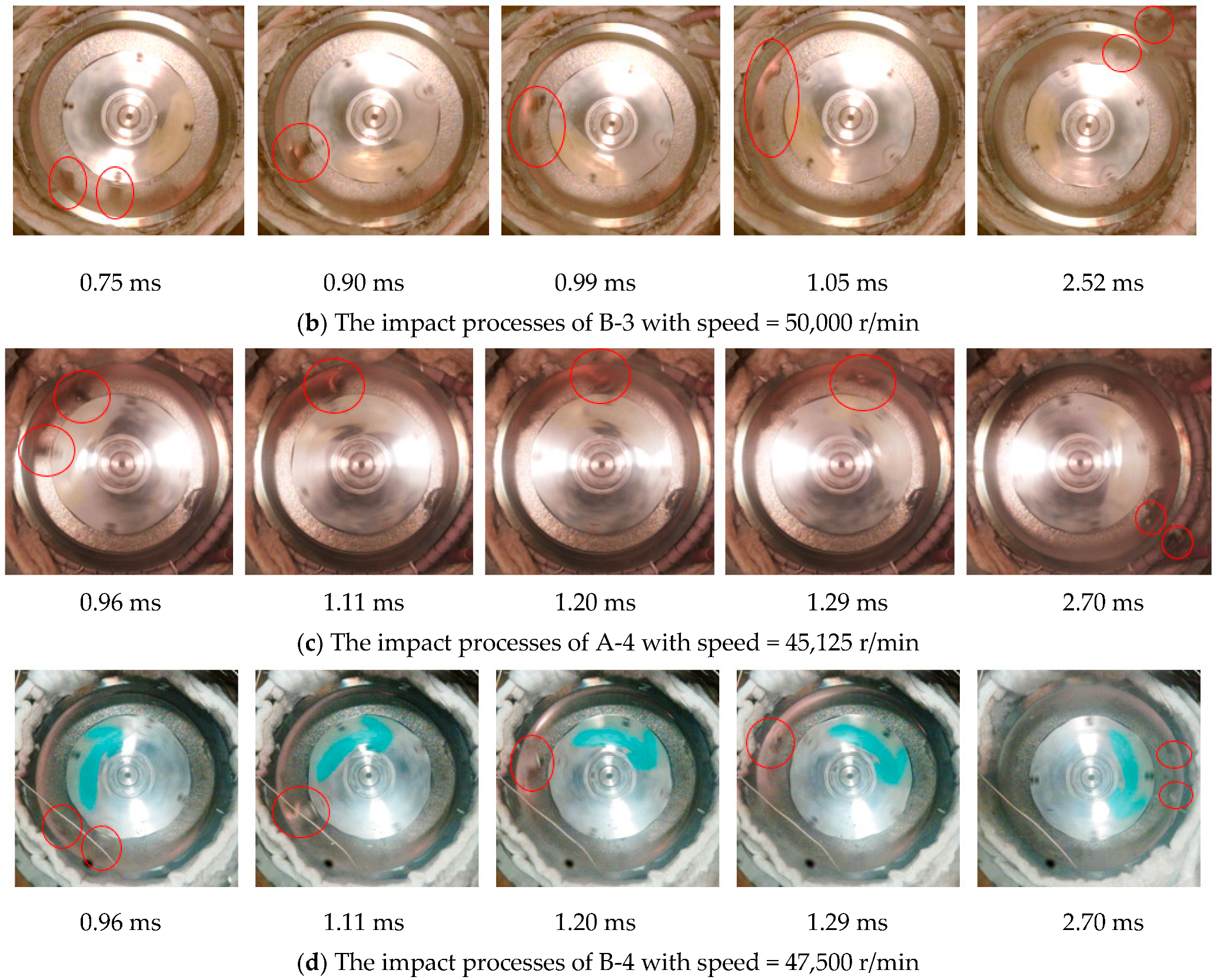
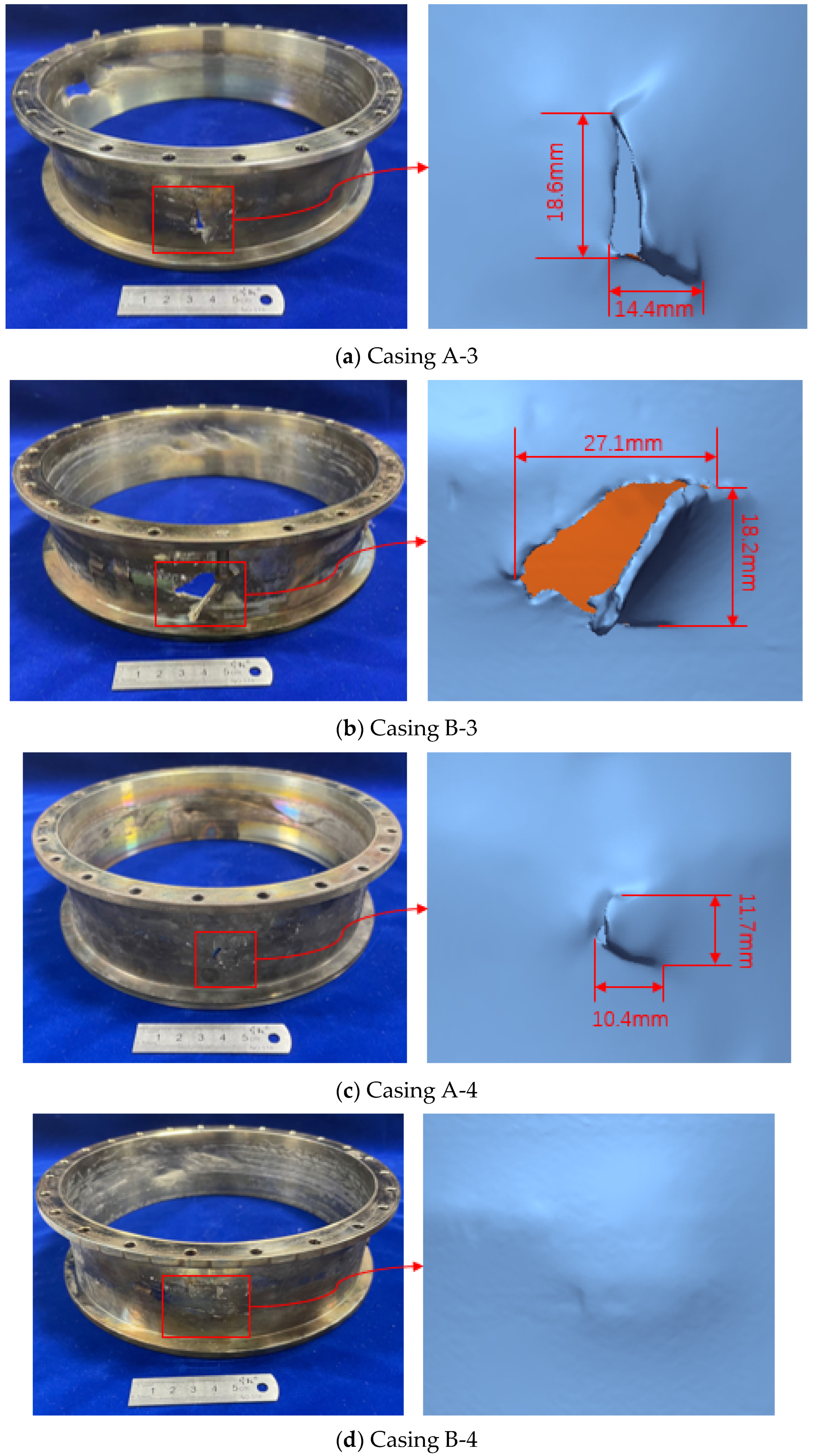
3.2.2. The Dual-Blade Containment Test Results
4. Discussion
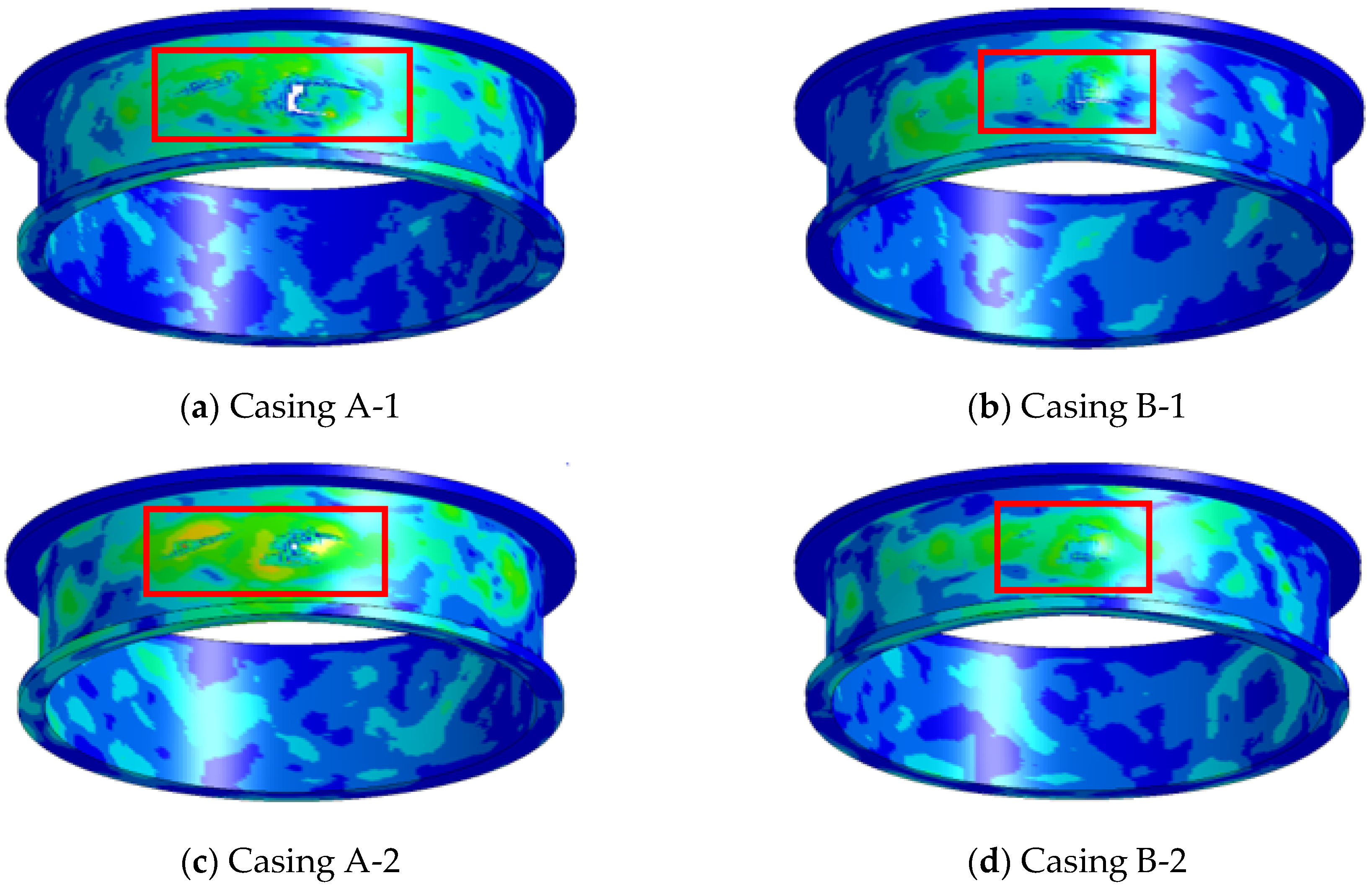
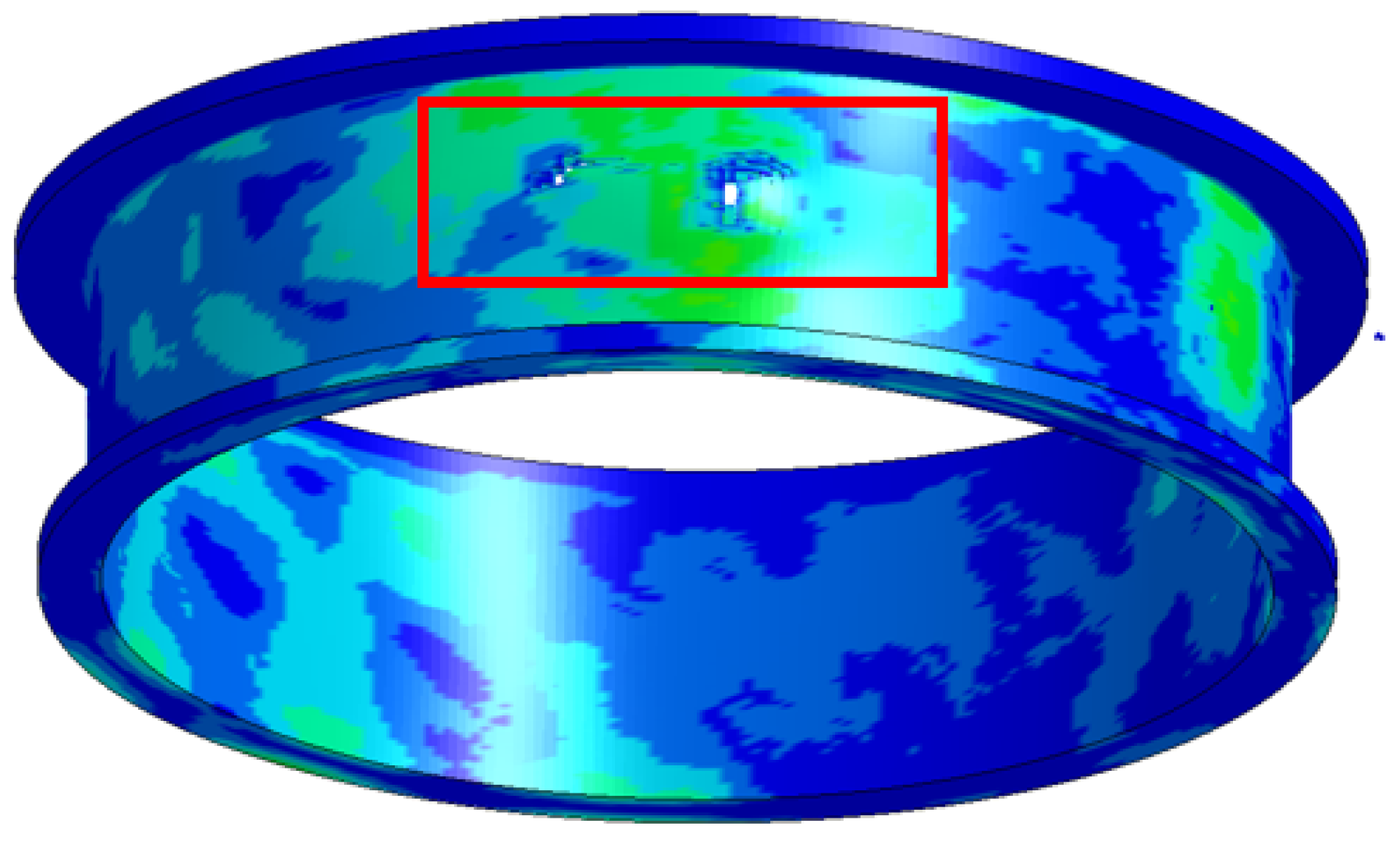
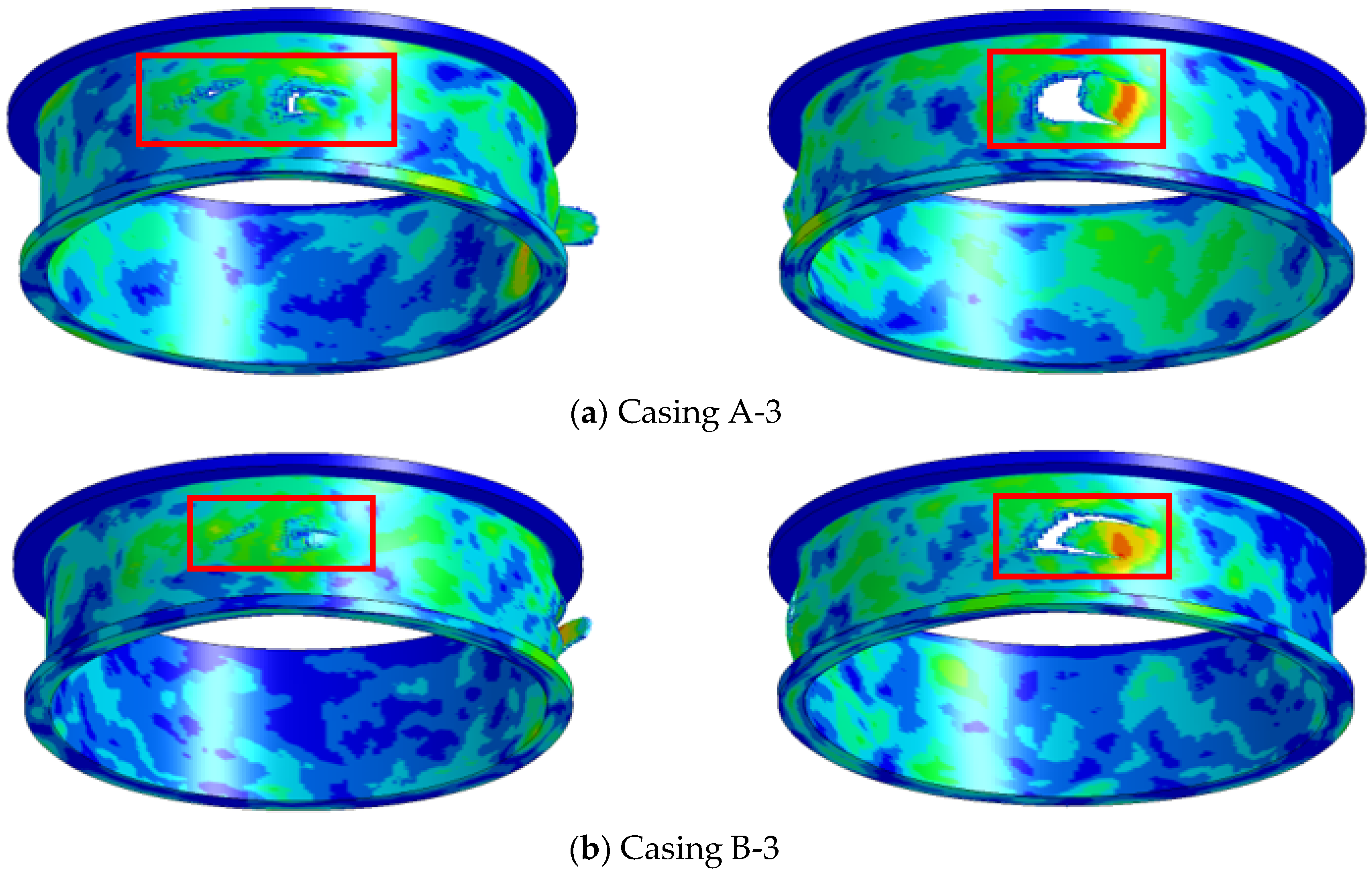
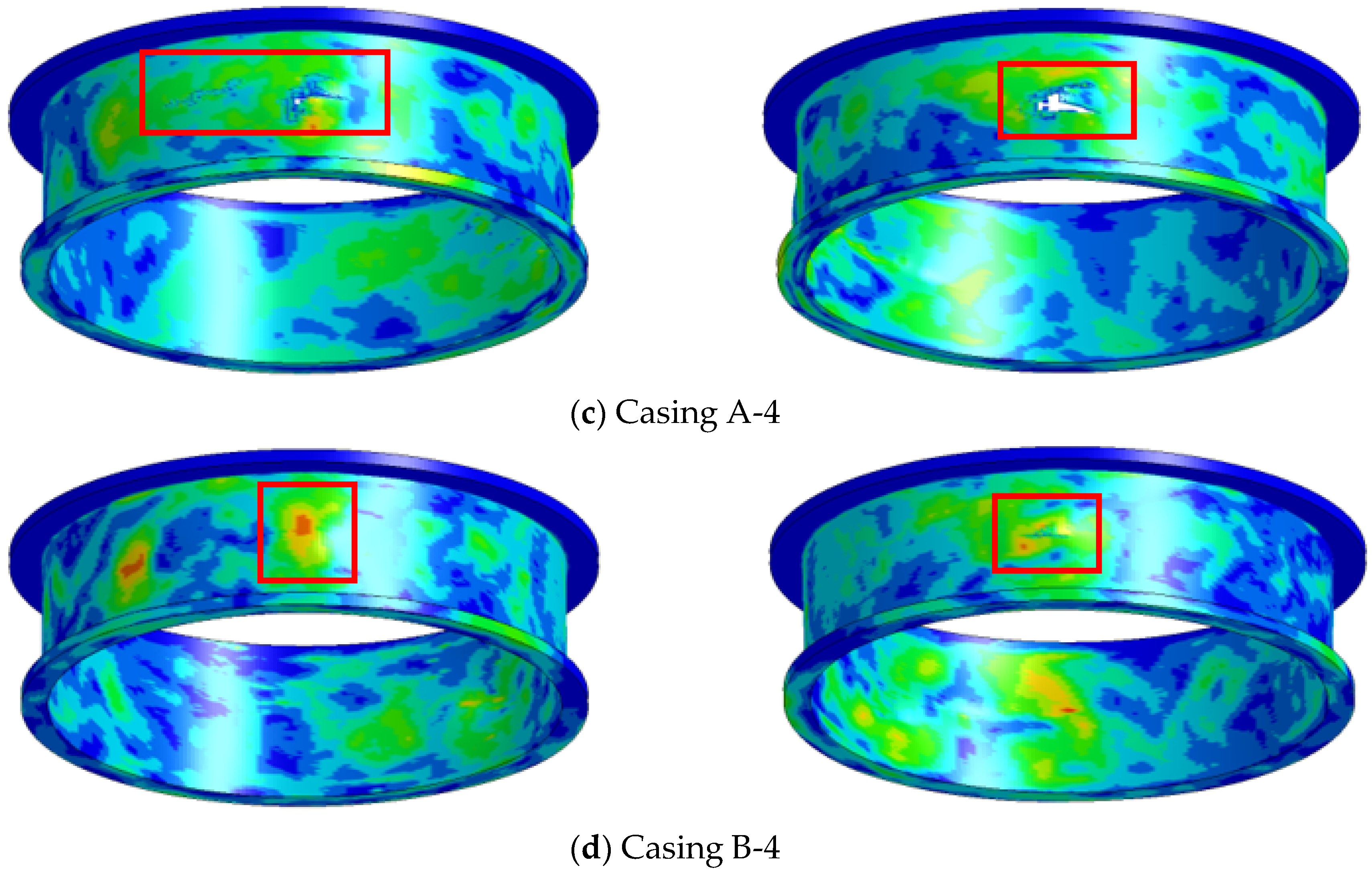
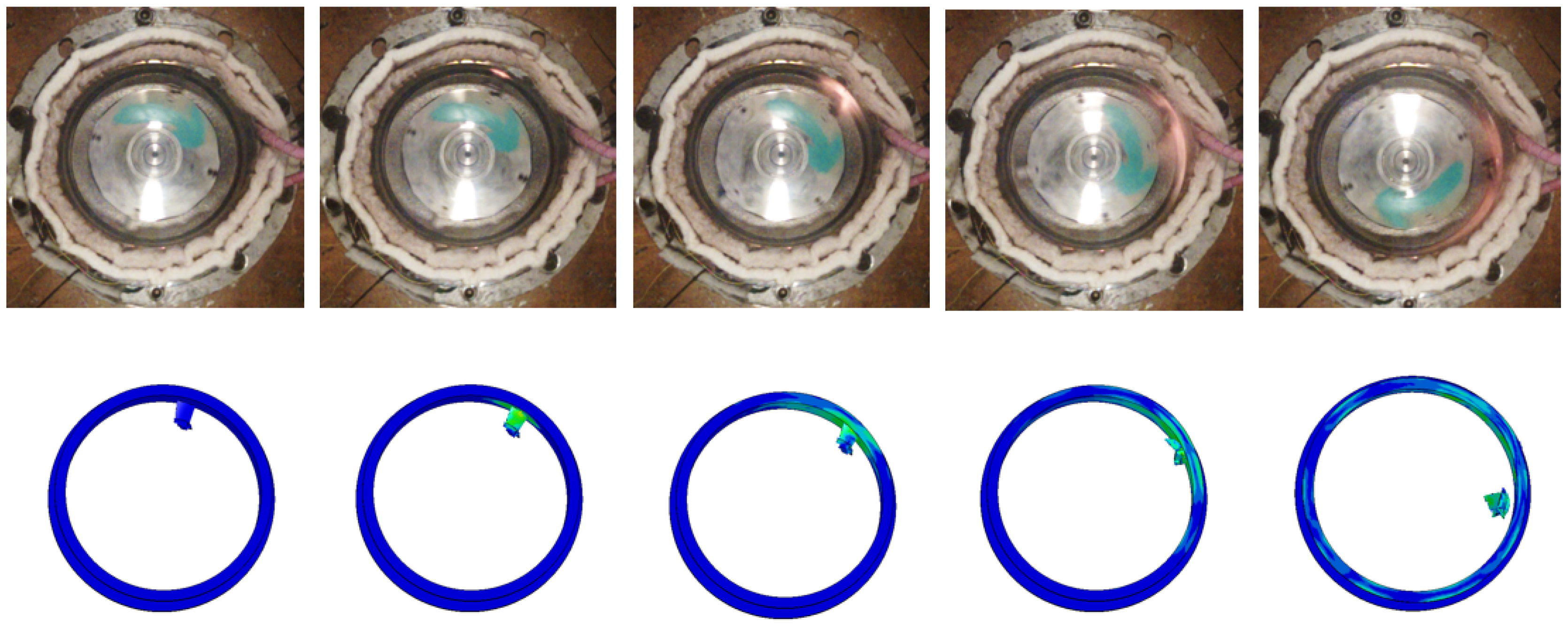
4.1. Simulation and Experimentation Comparison
4.2. Influence of Blade Shape in Casing Containment
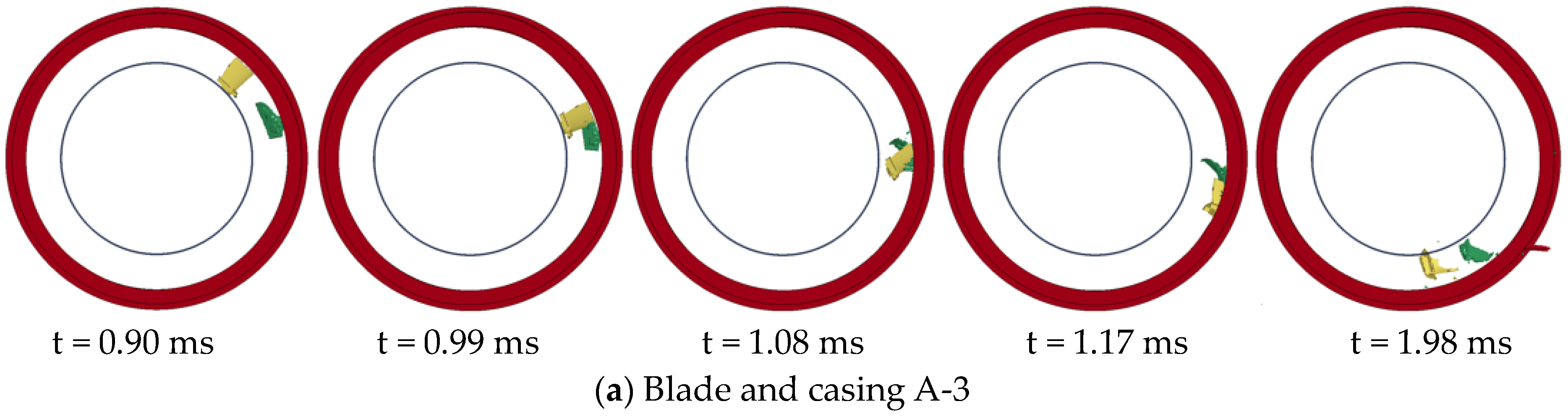
4.3. Influence of Dual-Blade Collision in Casing Containment
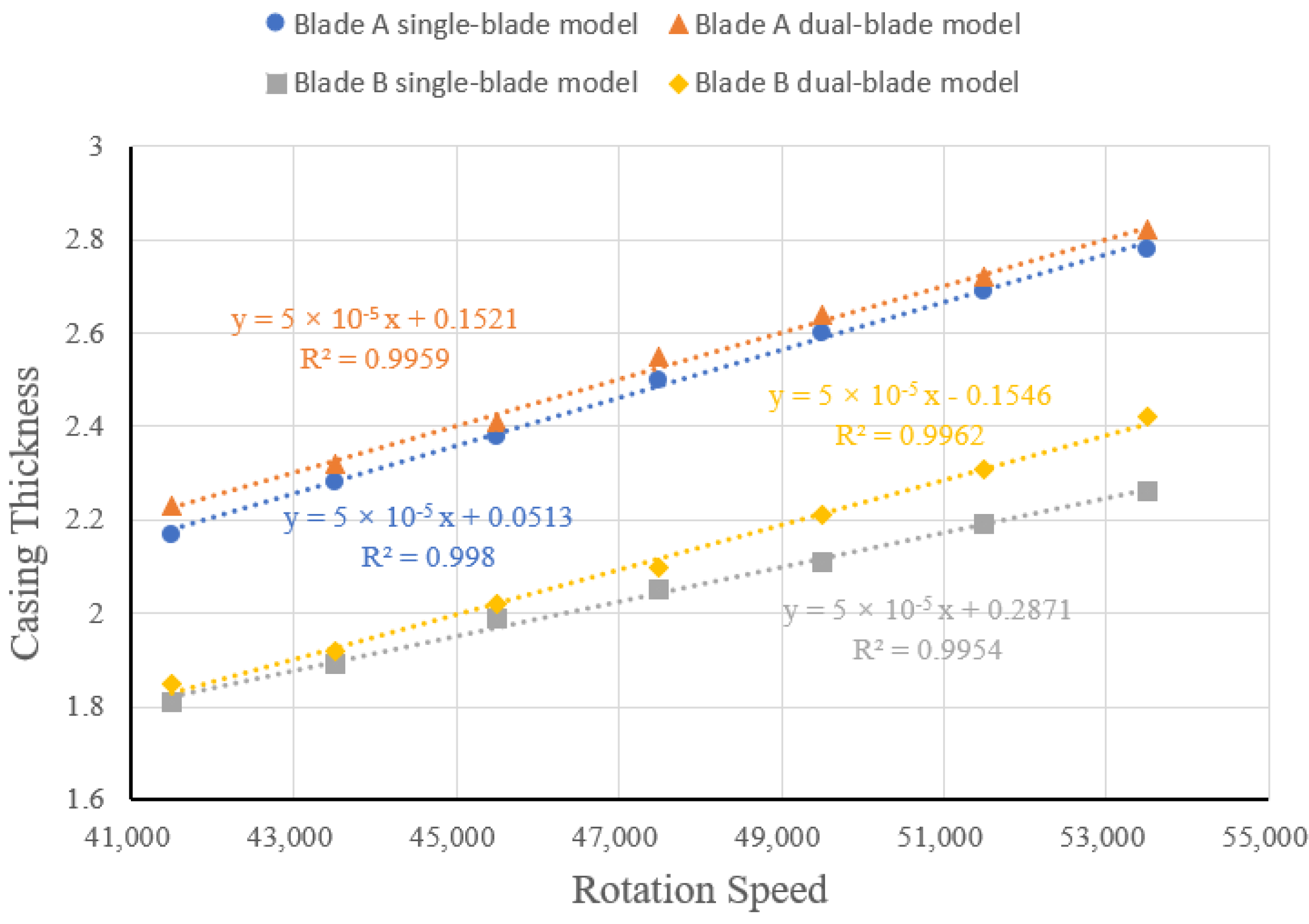
4.4. GasTurbine Single-Blade Containment Curve
| Critical Speed (r/min) | 41,500 | 43,500 | 45,500 | 47,500 | 49,500 | 51,500 | 53,500 | |
| Casing Thinkness (mm) | Single blade | 2.17 | 2.28 | 2.38 | 2.50 | 2.60 | 2.69 | 2.78 |
| Dual blade | 2.23 | 2.32 | 2.41 | 2.55 | 2.64 | 2.72 | 2.82 | |
| Critical Speed (r/min) | 41,500 | 43,500 | 45,500 | 47,500 | 49,500 | 51,500 | 53,500 | |
| Casing Thinkness (mm) | Single blade | 1.81 | 1.89 | 1.99 | 2.05 | 2.11 | 2.19 | 2.26 |
| Dual blade | 1.85 | 1.92 | 2.02 | 2.10 | 2.21 | 2.31 | 2.42 | |
5. Conclusions
- (1)
- The impact response process of a single gas turbine blade detachment mainly consists of three stages: the tip of the blade hitting the casing, the root of the blade hitting the casing, and the blade sliding along the inner wall of the casing. The maximum damage to the casing is mainly caused by the impact of the blade root in the second stage.
- (2)
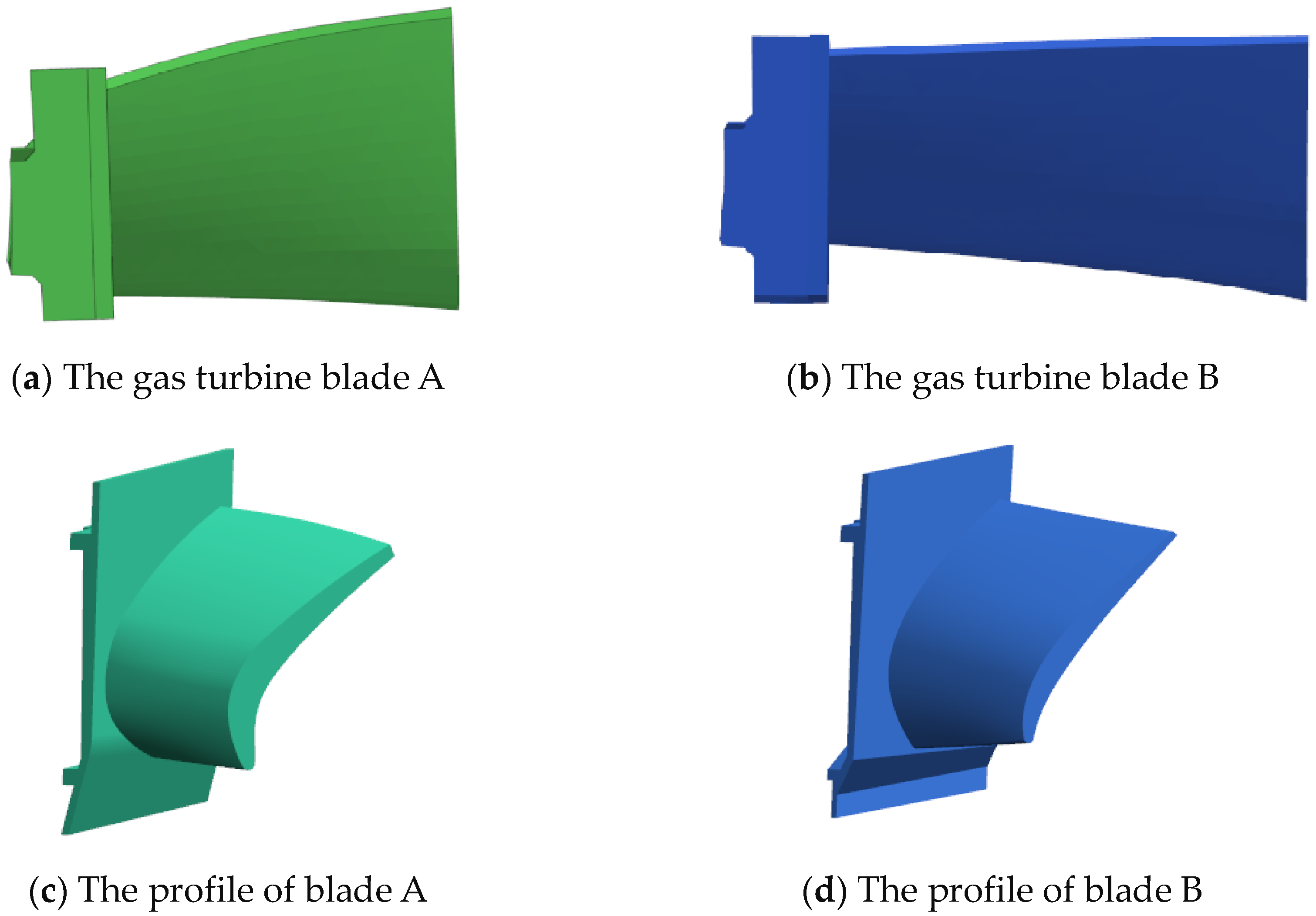
- Due to the longer blade structure of the gas turbine blade B compared to the blade A, the energy of the blade root impacting the casing is relatively lower. Therefore, the safety factor of the gas turbine blade B is higher than that of the blade A.
- (3)
- It was observed that dual-blade containment tests resulted in more severe damage compared to single-blade tests. The simulations showed that in dual-blade tests, the impact energy was higher due to the influence of the first blade, leading to larger damage. This finding highlights the critical role of interaction effects between successive blades in determining containment outcomes, shedding light on nuanced failure mechanisms critical for engine safety assessments.
- (4)
- Based on the numerical simulation results, curves of the containment speed for gas turbine blade detachment under different casing thicknesses are proposed. The fitting degrees of the containment curves for the blade A and B are 0.994 and 0.997, respectively. This contributes to further optimizing shell design, improving the safety and reliability of aircraft engines, and providing new ideas and methods for the development of the aviation engineering field.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Meher-Homji, C.B. Blading Vibration and Failures in Gas Turbines: Part B—Compressor and Turbine Airfoil Distress. In Proceedings of the ASME International Gas Turbine and Aeroengine Congress and Exposition, Houston, TX, USA, 5–8 June 1995. [Google Scholar]
- Australian Transport Safety Bureau; Canberra ACT. Examination of a Failed Rolls-Royce RB211-524 Turbofan Engine—Boeing Commercial Aircraft Group, 747-436, G-BNLD; Technical Analysis Rep. No. 20/02, Occurrence File No. BO/200200646; Australian Transport Safety Bureau: Canberra, Australia, 2002.
- Federal Aviation Administratio. Design Considerations for Minimizing Hazards Caused by Uncontained Turbine Engine and Auxiliary Power Unit Rotor Failure; FAA Advisory Circular No. 20-128A, 25 March; Federal Aviation Administration: Washington, DC, USA, 1997.
- Sarkar, S.; Atluri, S. Effects of multiple blade interaction on the containment of blade fragments during a rotor failure. J. Finite Elem. Anal. Des. 1996, 23, 211–223. [Google Scholar] [CrossRef]
- Federal Aviation Administration. Federal Aviation Administration Regulations, Part 33, Airworthiness Standards: Aircraft Engines; Federal Aviation Administration: Washington, DC, USA, 1984.
- MIL-STD-1783B; Engine Structural Integrity Program. Department of Defense: Washington, DC, USA, 2002.
- CS-E 810; Certification Specifications and Acceptable Means of Compliance for Engines: Compressor and Turbine Blade Failure. European Aviation Safety Agency: Cologne, Germany, 2015.
- CARR-33; Airworthiness Standards for Aircraft Engines. Civil Aviation Administration of China: Beijing, China, 2005.
- The Aviation Herald. Incident: Korean A333 at Seoul on 26 May 2011, Engine Shut Down in Flight Following Uncontained Failure. Available online: http://avherald.com/h?article=43da45e4&opt=0 (accessed on 4 June 2011).
- The Aviation Herald. Incident: Skywest CRJ7 at Chicago on 10 June 2017, Uncontained Engine Failure. Available online: http://avherald.com/h?article=4aa3ba31&opt=0 (accessed on 12 June 2017).
- Teng, X.; Wierzbicki, T. Gouging and fracture of engine containment structure under fragment impact. J. Aerosp. Eng. 2008, 21, 174–186. [Google Scholar] [CrossRef]
- Qiu, J.; Shi, J.; Su, H.; Zhang, J.; Feng, J.; Shi, Q.; Tian, X. Fatigue lifespan of engine box influenced by fan blade out. IOP Conf. Ser. Mater. Sci. Eng. 2017, 265, 012026. [Google Scholar] [CrossRef]
- He, Z.; Guo, X.; Xuan, H.; Shan, X.; Fan, X.; Chen, C.; Hong, W. Characteristics and Mechanisms of Turboshaft Engine Axial Compressor Casing Containment. Chin. J. Aeronaut. 2021, 34, 171–180. [Google Scholar] [CrossRef]
- Jiang, X.; Liu, S.; Li, S. Analysis of engine containment test method of turbofan engine with high by-pass ratio. J. Aeroengine 2021, 47, 60–63. [Google Scholar]
- Xuan, H.; Hu, Y.; Wu, Y.; He, Z. Containment Ability of Kevlar 49 Composite Case under Spinning Impact. J. Aerosp. Eng. 2018, 31, 04017096. [Google Scholar] [CrossRef]
- He, Z.; Xuan, H.; Bai, C. Containment of soft wall casing wrapped with Kevlar fabric. Chin. J. Aeronaut. 2019, 32, 954–966. [Google Scholar] [CrossRef]
- Bai, C.; Xuan, H.; Huang, X. Containment ability and groove depth design of U type protection ring. Chin. J. Aeronaut. 2016, 29, 395–402. [Google Scholar] [CrossRef][Green Version]
- Sengoz, K.; Kan, S.; Eskandarian, A. Development of a Generic Gas-Turbine Engine Fan Blade-Out Full-Fan Rig Model; The George Washington FHWA/NHTSA National Crash Analysis Center: Washington, DC, USA, 2015.
- Tuninetti, V.; Sepúlveda, H. Computational Mechanics for Turbofan Engine Blade Containment Testing: Fan Case Design and Blade Impact Dynamics by Finite Element Simulations. Aerospace 2024, 11, 333. [Google Scholar] [CrossRef]
- Yang, S.; Chen, C.; Liu, G. An Experimental and Simulation Study of Impact Resistance in Sandwich Structures Casing. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2019, 233, 3635–3648. [Google Scholar] [CrossRef]
- He, Q.; Xie, Z.; Xuan, H. Multi-blade effects on aero-engine blade containment. J. Aerosp. Sci. Technol. 2016, 49, 101–111. [Google Scholar] [CrossRef]
- Sinha, S.K.; Dorbala, S. Dynamic loads in the fan containment structure of a turbofan engine. J. Aerosp. Eng. 2009, 22, 260–269. [Google Scholar] [CrossRef]
- Eryilmaz, I.; Guenchi, B.; Pachidis, V. Multi-blade shedding in turbines with different casing and blade tip architectures. J. Aerosp. Sci. Technol. 2019, 87, 300–310. [Google Scholar] [CrossRef]
- VanderKlok, A.; Stamm, A.; Xiao, X. Fan-blade-out experiment at small scale. J. Exp. Tech. 2016, 40, 1479–1484. [Google Scholar] [CrossRef]
- Kraus, A.; Frischbier, J. Containment and penetration simulation in case of blade loss in a low pressure turbine. In DYNAmore LS-DYNA Forum; DYNAmore GmbH: Stuttgart, Germany, 2002. [Google Scholar]
- Roy, P.A.; Meguid, S.A. Containment and arrest of blade shedding in gas turbine engines using novel dual-ring design. J. Eng. Gas Turbines Power 2021, 143, 071015. [Google Scholar] [CrossRef]
- International Aviation Editorial Board. Stress Standards for the Spey MK202 Engine (EGD-3); International Aviation Editorial Board: London, UK, 1979. [Google Scholar]
- Johnson, G.R.; Cook, W.H. A constitutive model and data for metals subjected to large strains, high rates and high temperatures. In Proceedings of the 7th International Symposium on Ballistics, The Hague, The Netherlands, 19–21 April 1983; pp. 541–547. [Google Scholar]
- Johnson, G.R.; Cook, W.H. Fracture characteristics of three metals subjected to various strains, strain rates, temperatures and pressures. Eng. Fract. Mech. 1985, 21, 31–48. [Google Scholar] [CrossRef]
- Chen, L.; Xuan, H.; Jia, W.; Liu, J.; Fang, Z.; Zheng, Y. Neck Structure Optimal Design of the Turbine Wheel for Containment Design of the Air Turbine Starter. Aerospace 2023, 10, 802. [Google Scholar] [CrossRef]
- Fan, X. Simulation and Structural Optimization Study of Containment Ring Containment Capability with U-Shaped Section. Master’s Thesis, Zhejiang University, Hangzhou, China, 2021. [Google Scholar]



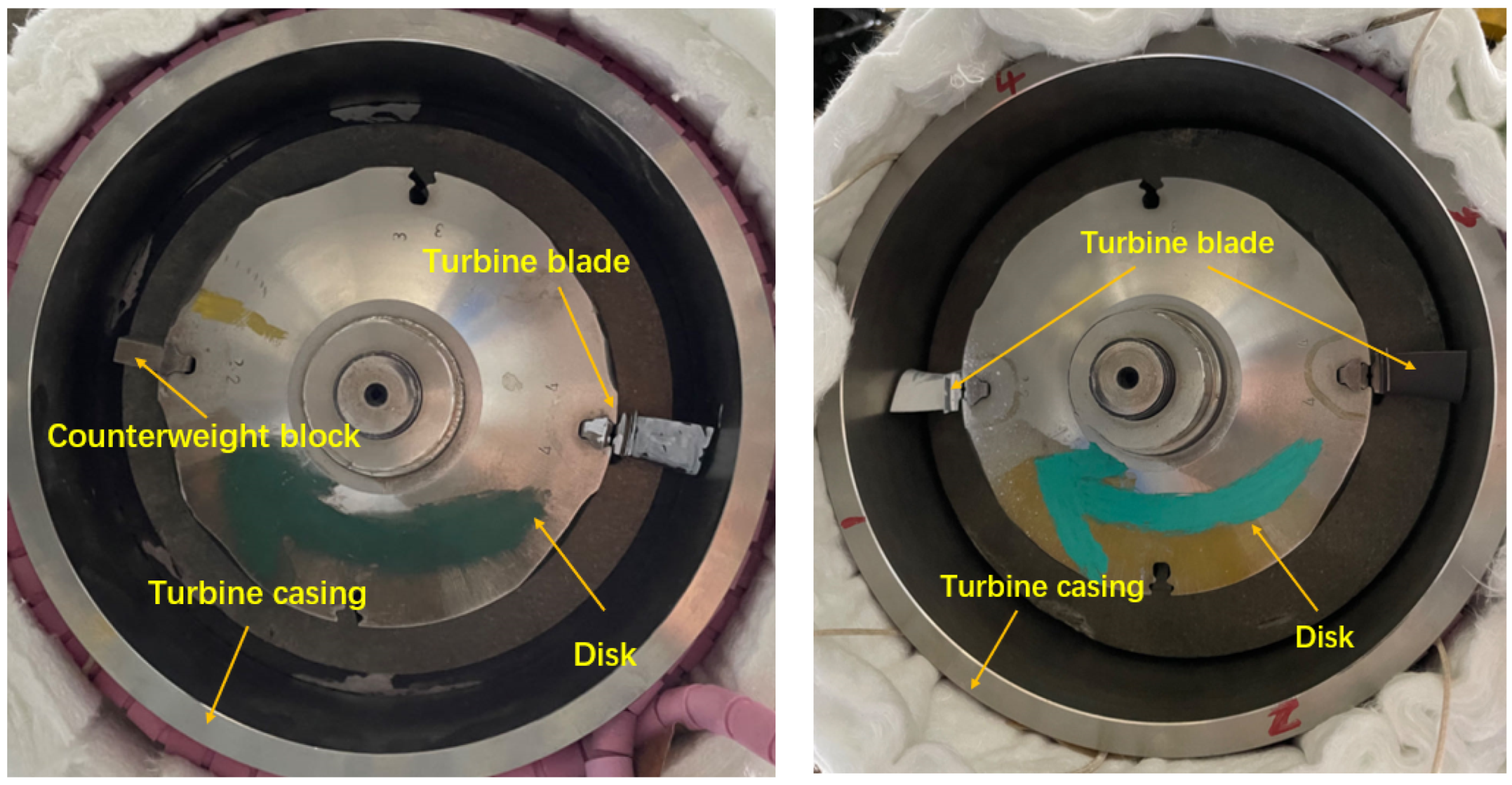
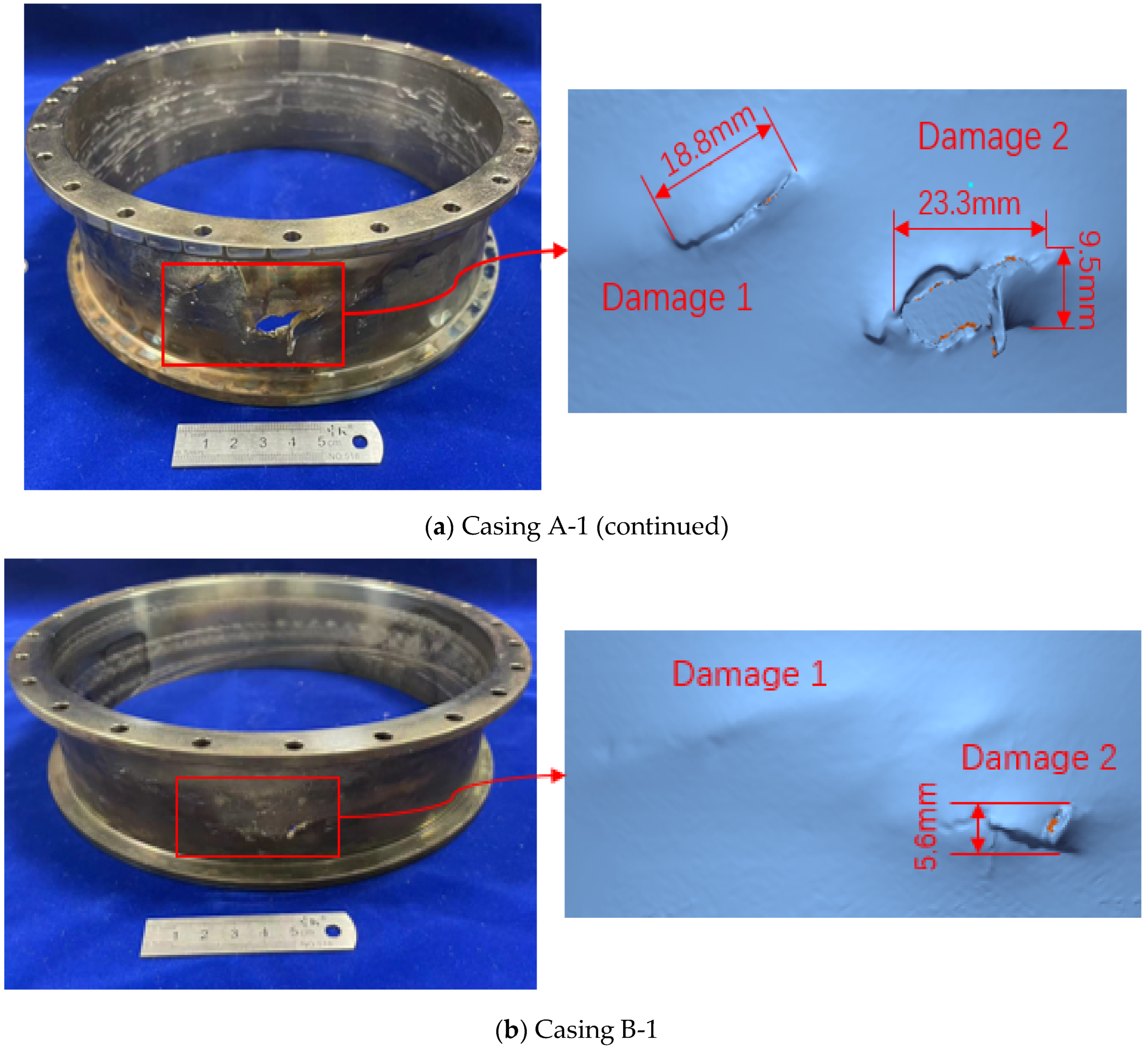
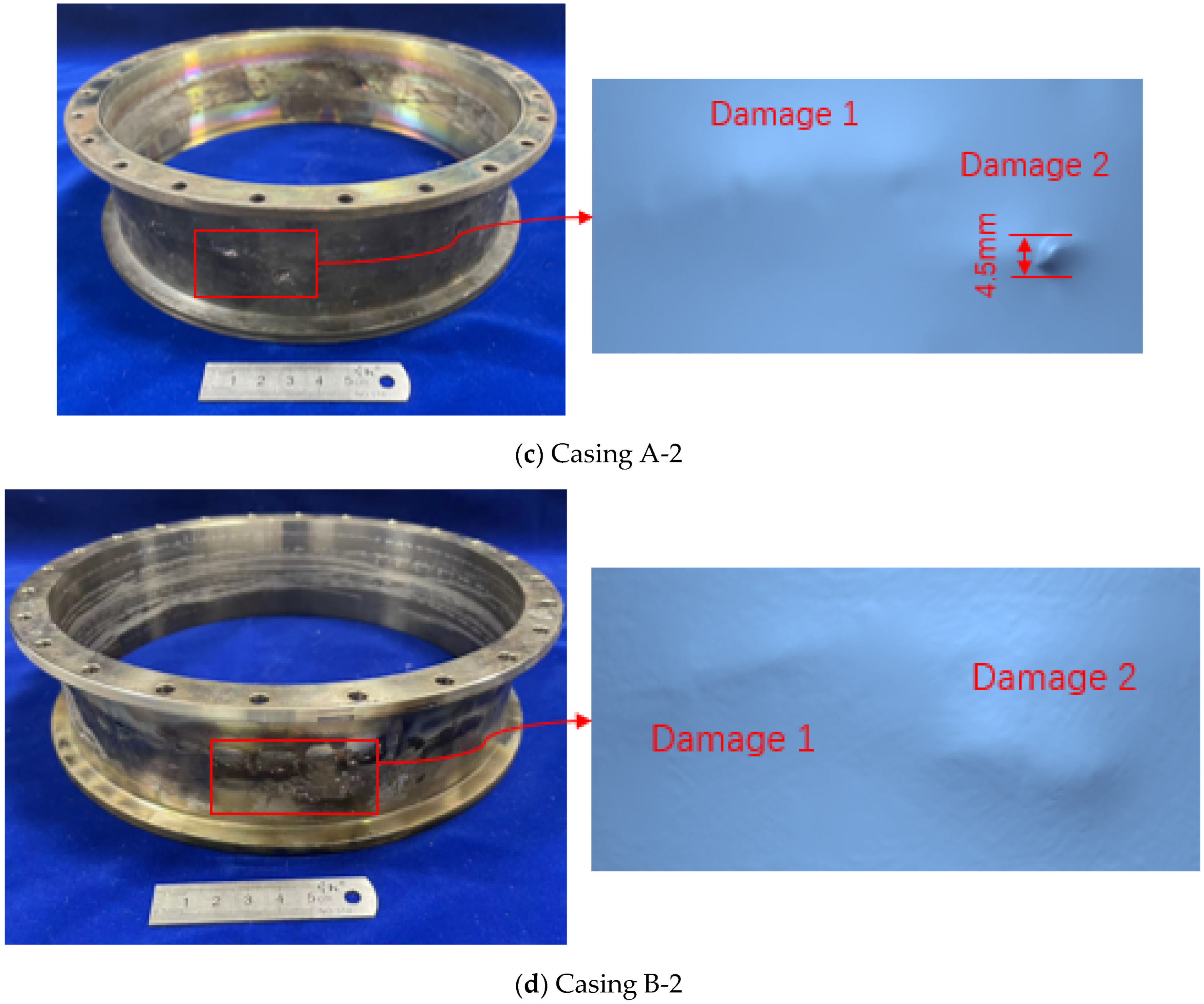
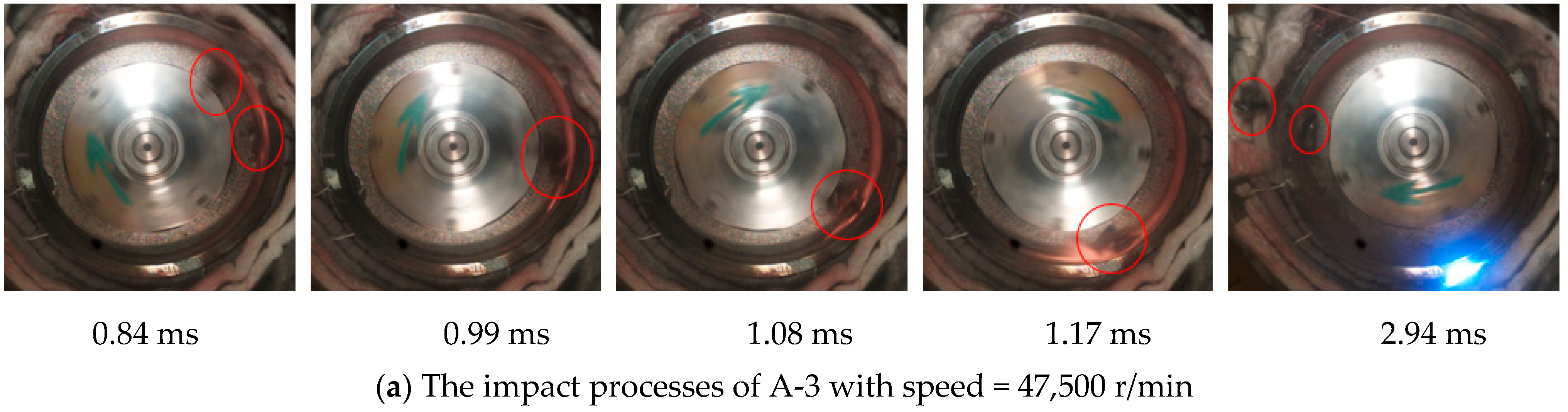

| Material | J-C Constitutive Model Parameters | ||||
| A/MPa | B/MPa | n | C | M | |
| GH4169 | 872 | 1084 | 0.545 | 0.0134 | 1.3 |
| K417 | 850 | 1090 | 0.65 | 0.014 | 1.1 |
| Material | J-C Failure Model Parameters | ||||
| D1 | D2 | D3 | D4 | D5 | |
| GH4169 | 0.11 | 0.24 | 1.92 | 2.12 × 10−5 | 0.65 |
| K417 | −0.1 | 0.24 | 0.45 | 0.011 | 3.84 |
| Simulation ID | Simulation Model | Thickness (mm) | Spin Speed (r/min) | Results |
|---|---|---|---|---|
| A-1 | Single-blade model | 2.37 | 47,500 | Non-containment |
| B-1 | 2.12 | 50,000 | Critical containment | |
| A-2 | 2.37 | 45,125 | Critical containment | |
| B-2 | 2.12 | 47,500 | Containment | |
| A-3 | Dual-blade model | 2.37 | 47,500 | Non-containment |
| B-3 | 2.12 | 50,000 | Non-containment | |
| A-4 | 2.37 | 45,125 | Non-containment | |
| B-4 | 2.12 | 47,500 | Containment |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, M.; Wang, J.; Xuan, H.; Xiong, W.; He, Z.; Qu, M. Simulation and Experimental Study of Gas Turbine Blade Tenon-Root Detachment on Spin Test. Aerospace 2024, 11, 629. https://doi.org/10.3390/aerospace11080629
Yu M, Wang J, Xuan H, Xiong W, He Z, Qu M. Simulation and Experimental Study of Gas Turbine Blade Tenon-Root Detachment on Spin Test. Aerospace. 2024; 11(8):629. https://doi.org/10.3390/aerospace11080629
Chicago/Turabian StyleYu, Maoyu, Jianfang Wang, Haijun Xuan, Wangjiao Xiong, Zekan He, and Mingmin Qu. 2024. "Simulation and Experimental Study of Gas Turbine Blade Tenon-Root Detachment on Spin Test" Aerospace 11, no. 8: 629. https://doi.org/10.3390/aerospace11080629
APA StyleYu, M., Wang, J., Xuan, H., Xiong, W., He, Z., & Qu, M. (2024). Simulation and Experimental Study of Gas Turbine Blade Tenon-Root Detachment on Spin Test. Aerospace, 11(8), 629. https://doi.org/10.3390/aerospace11080629





