Comparative Study on Mechanical Response in Rigid Pavement Structures of Static and Dynamic Finite Element Models
Abstract
1. Introduction
2. Methodology
2.1. Finite Element Analysis in ANSYS (ANSYS 2021 R1)
2.2. Significance Analysis in SPSS
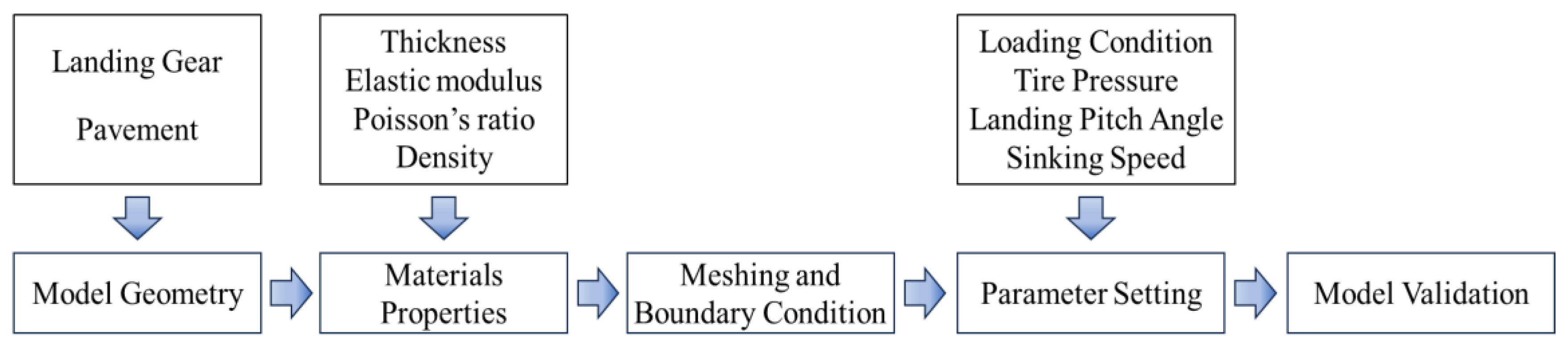
2.3. Developing a Finite Element Model
2.3.1. Model Geometry
2.3.2. Material Properties
2.3.3. Meshing and Boundary Conditions
2.3.4. Parameter Setting and Vibration Frequency
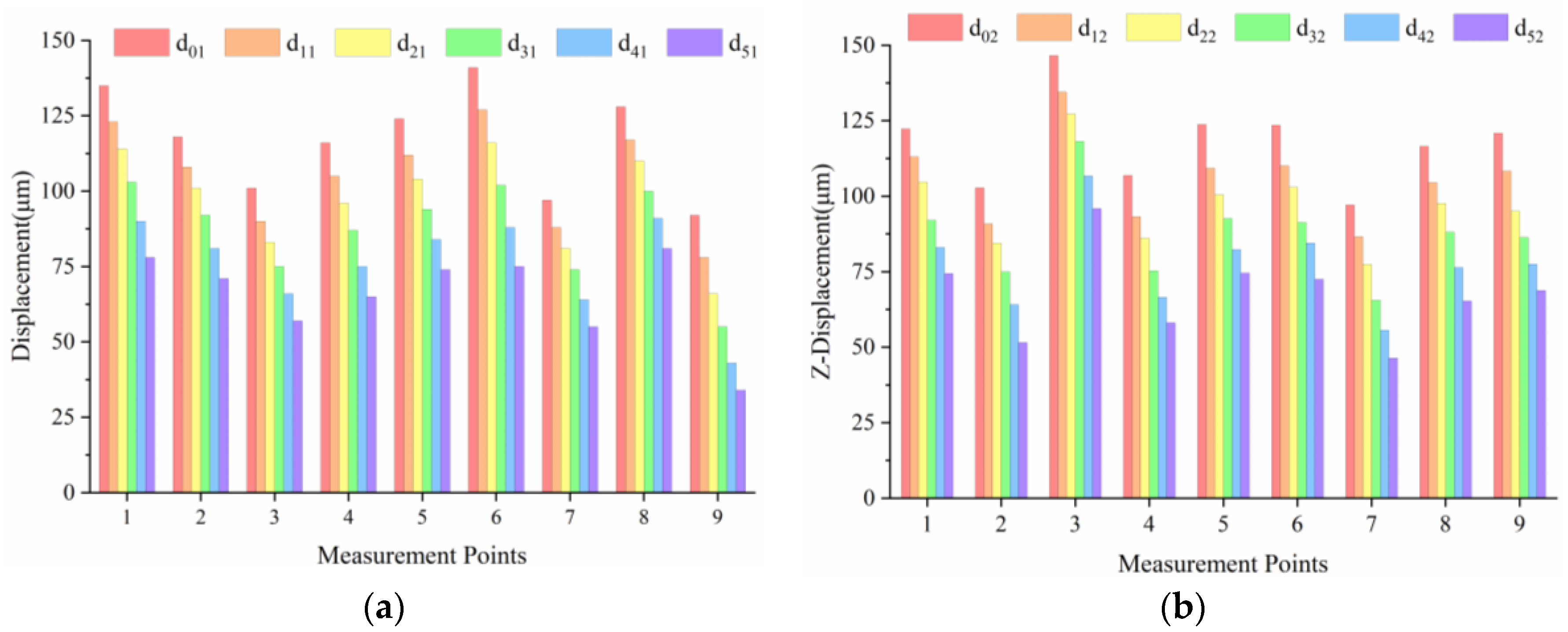
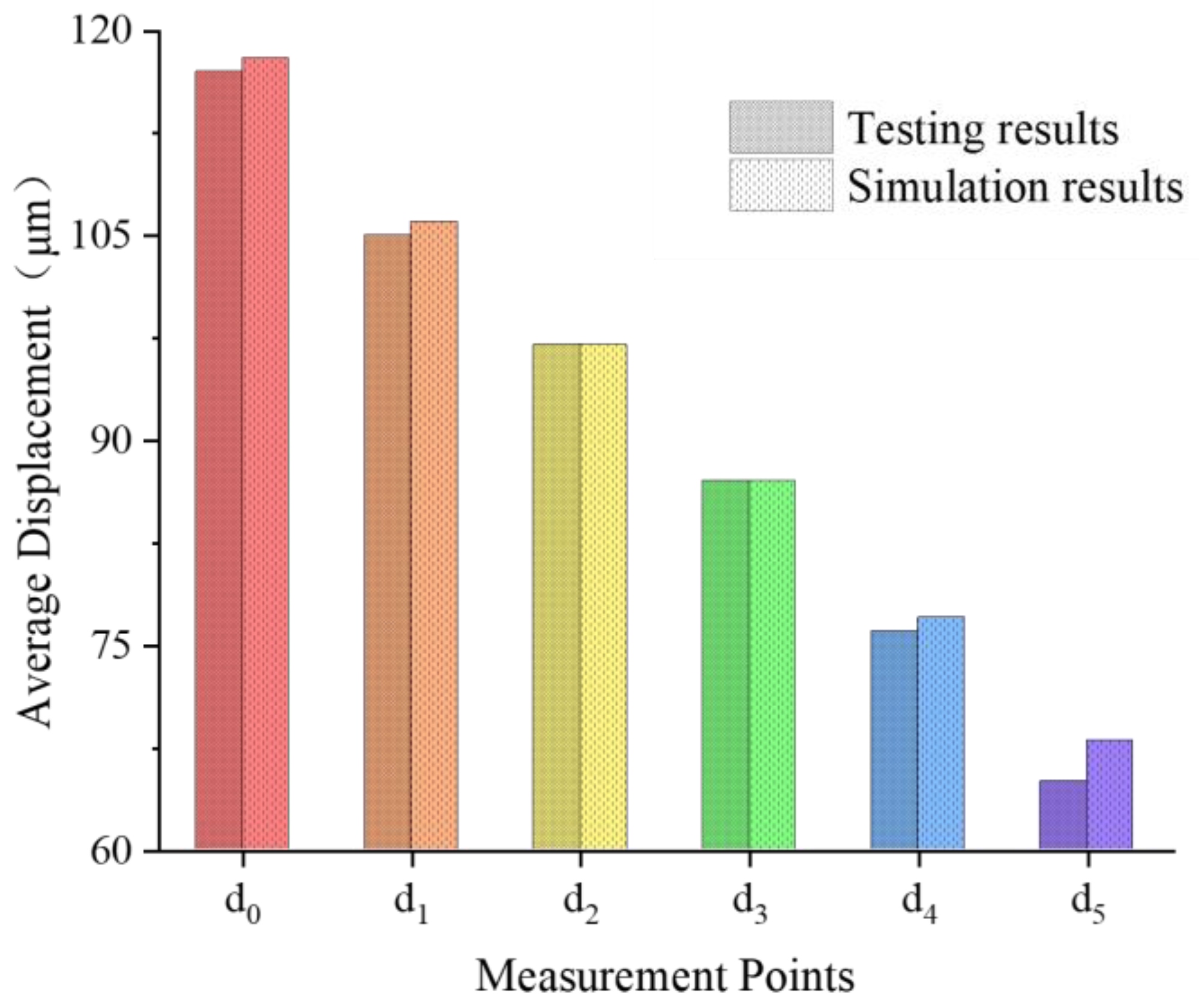
2.3.5. Model Validation
3. Results and Discussion
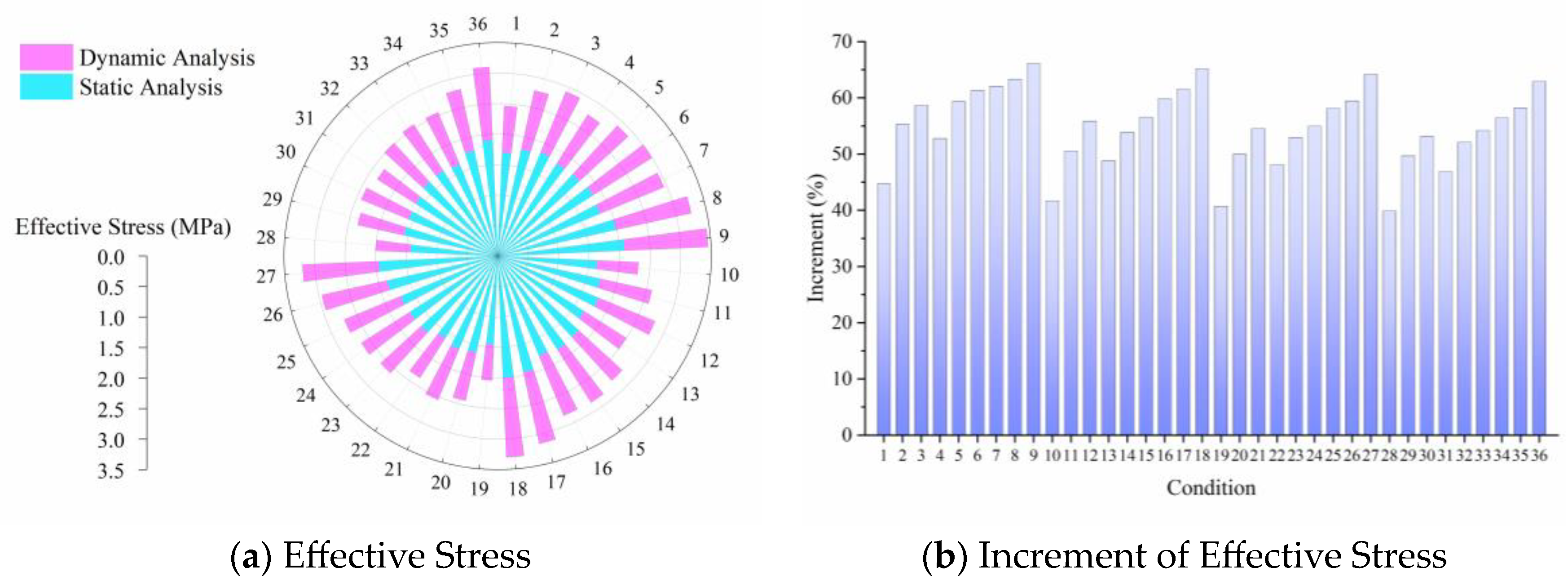
3.1. Dynamic and Static Analysis
3.2. Significance Analysis
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Li, T.; Wu, Y.; Wu, H. A Study on Impact of Different Surface Treatment Agents on the Durability of Airport Pavement Concrete. Coatings 2022, 12, 162. [Google Scholar] [CrossRef]
- Gu, G.; Chen, F.; Ma, T.; Xu, F.; Yang, D. Electromagnetic and mechanical properties of soft magnetic cement composite for airport runway induction heating: Experimental and simulation analyses. J. Clean. Prod. 2022, 332, 130141. [Google Scholar] [CrossRef]
- Miah, M.T.; Oh, E.; Chai, G.; Bell, P. An overview of the airport pavement management systems (APMS). Int. J. Pavement Res. Technol. 2021, 13, 581–590. [Google Scholar] [CrossRef]
- Dong, Z.; Wang, T.; Ma, X.; Cao, C.; Sun, J. Structural performance evaluation of airport asphalt pavement based on field data measurement and finite element simulation. Measurement 2023, 210, 112553. [Google Scholar] [CrossRef]
- Senthil, K.; Pelecanos, L.; Rupali, S.; Sharma, R.; Saini, K.; Iqbal, M.A.; Gupta, N.K. Experimental and numerical investigation of transient dynamic response on reinforced concrete tunnels against repeated impact loads. Int. J. Impact Eng. 2023, 178, 104604. [Google Scholar] [CrossRef]
- Sun, J.; Chai, G.; Oh, E.; Bell, P. A Review of PCN Determination of Airport Pavements Using FWD/HWD Test. Int. J. Pavement Res. Technol. 2022, 16, 908–926. [Google Scholar] [CrossRef]
- Zegeye, E.; Le, J.; Turos, M.; Marasteanu, M. Investigation of size effect in asphalt mixture fracture testing at low temperature. Road Mater. Pavement Des. 2012, 13, 88–101. [Google Scholar] [CrossRef]
- Zhu, X.; Zhang, Q.; Chen, L.; Du, Z. Mechanical response of hydronic asphalt pavement under temperature–vehicle coupled load: A finite element simulation and accelerated pavement testing study. Constr. Build. Mater. 2021, 272, 121884. [Google Scholar] [CrossRef]
- Li, Q.; Meng, H. About the dynamic strength enhancement of concrete-like materials in a split Hopkinson pressure bar test. Int. J. Solids Struct. 2003, 40, 343–360. [Google Scholar] [CrossRef]
- Dong, Z.; Ma, X. Analytical solutions of asphalt pavement responses under moving loads with arbitrary non-uniform tire contact pressure and irregular tire imprint. Road Mater. Pavement Des. 2017, 19, 1887–1903. [Google Scholar] [CrossRef]
- JTG D40-2011; Specifications for Design of Highway Cement Concrete Pavement. Ministry of Transport of the People’s Republic of China: Beijing, China, 2011.
- JTG D50-2017; Specifications for Design of Highway Asphalt Pavement. Ministry of Transport of the People’s Republic of China: Beijing, China, 2017.
- MH/T 5004-2010; Specifications for Airport Cement Concrete Pavement Design. CAAC: Beijing, China, 2010.
- Cao, D.; Zhao, Y.; Liu, W.; Li, Y.; Ouyang, J. Comparisons of asphalt pavement responses computed using layer properties backcalculated from dynamic and static approaches. Road Mater. Pavement Des. 2018, 20, 1114–1130. [Google Scholar] [CrossRef]
- Du, L. Simulation Analysis on Performance Degradation of Airfield Pavement Structure under Aircraft Load. Master’s Thesis, Southeast University, Nanjing, China, 2021. [Google Scholar]
- Huang, Y. Pavement Analysis and Design; China Communication Press: Beijing, China, 1998. [Google Scholar]
- Kim, S. Influence of horizontal resistance at plate bottom on vibration of plates on elastic foundation under moving loads. Eng. Struct. 2004, 26, 519–529. [Google Scholar] [CrossRef]
- Wang, J.; Dou, Y.; Li, J.; Wei, M.; Zhai, Y. Dynamic Response Analysis of Jointed Concrete Pavements Under Moving Load Factors. Constr. Technol. 2018, 47, 93–97. [Google Scholar]
- Saad, B.; Mitri, H.; Poorooshasb, H. Three-dimentional dynamic analysis of flexible conventional pavement foundation. J. Transp. Eng. 2005, 131, 460–469. [Google Scholar] [CrossRef]
- Zeng, J. Research on Key Performance of Airprot Runway Pavement with Epoxy Asphalt Concrete. Master’s Thesis, Southeast University, Nanjing, China, 2013. [Google Scholar]
- Liang, L.; Gu, Q.; Liu, G.; Zhang, R.; Jiang, L. Using ADAMS to Assess Dynamic Load of Pavement during Aircraft Landing. J. Southwest Jiaotong Univ. 2012, 47, 502–508. [Google Scholar]
- Qian, J.; Pan, X.; Cen, Y.; Liu, D. Aircraft Taxiing Dynamic Load Induced by Runway Roughness. J. Vib. Shock 2022, 41, 176–184, 269. [Google Scholar] [CrossRef]
- Zhao, M.; Zhao, H.; Wu, D. Identification of Cavities Underneath Concrete Pavement Based on Pavement vibration. China J. Highw.Transp. 2020, 33, 42–52. [Google Scholar] [CrossRef]
- Fu, G.; Wang, H.; Zhao, Y.; Yu, Z.; Li, Q. Non-destructive evaluation of longitudinal cracking in semi-rigid asphalt pavements using FWD deflection data. Struct. Control Health Monit. 2022, 29, e3050. [Google Scholar] [CrossRef]
- Qin, F. Analytical Dynamic Responses of Aeroplane in Landing and Taxiing. Master’s Thesis, Nanjing University of Aeronautics and Astronautics, Nanjing, China, 2011. [Google Scholar]
- Cui, Y.; Cen, G.; Liang, L. Study on the Characteristics Dynamic Load of New Aircraft Landing. Comput. Integr. Manuf. Syst. 2020, 37, 15–21. [Google Scholar]
- Bai, Z.; Liu, Y.; Yang, J.; He, S. A constitutive model for concrete subjected to extreme dynamic loadings. Int. J. Impact Eng. 2020, 138, 103483. [Google Scholar] [CrossRef]
- LeMinh, H.; Khatir, S.; AbdelWahab, M.; CuongLe, T. A concrete damage plasticity model for predicting the effects of compressive high-strength concrete under static and dynamic loads. J. Build. Eng. 2021, 44, 103239. [Google Scholar] [CrossRef]
- Shi, B.; Cao, B.; Zhang, Y.; Xu, W.; Ren, W. Test and Analysis on Bearing Capacity of Highway Runway Asphalt Pavement Based on HWD. Sci. Technol. Eng. 2015, 15, 134–140. [Google Scholar]
- Mourlas, C.; Markou, G.; Papadrakakis, M. Accurate and computationally efficient nonlinear static and dynamic analysis of reinforced concrete structures considering damage factors. Eng. Struct. 2019, 178, 258–285. [Google Scholar] [CrossRef]
- Li, J.; Yang, J. Refinement of Structural Statical Mathematical Model. ACTA Aeronaut. ET Astronaut. Sin. 1993, 14, 13–18. [Google Scholar]
- Xing, Y.; Yang, R. Application of Euler Midpoint Symplectic Integration Method for the Solution of Dynamic Equilibrium Equations. Chin. J. Theor. Appl. Mech. 2007, 39, 100–105. [Google Scholar]
- Chatterjee, S. Regression Analysis by Example; Wiley-Interscience: Hoboken, NJ, USA, 2006. [Google Scholar]
- Aldaco, J.R.; Gibert, O.; Giraldo, D.M.; Barbat, A.H. Seismic vulnerability assessment of urban areas using multi-criteria analysis and geographic information systems: A case study in Manizales, Colombia. Nat. Hazards 2020, 104, 1323–1350. [Google Scholar]
- Xue, H. Analysis on Vibration Frequency Response of Rigid Pavement in Conditions of Aircraft Taxing. Master’s Thesis, Civil Aviation University of China, Tianjin, China, 2014. [Google Scholar]
- Luo, R. Nonlinear Finite Element Analysis of 34*10.75-16 Tire of Aircraft in ABAOUS. Master’s Thesis, Guilin University of Electronic Technology, Guilin, China, 2015. [Google Scholar]
- HB 8498-2014; Test Requirement for Landing Gear System Performance of Civil Aircraft. Avic China Aero-Polytechnology Establishment: Beijing, China, 2014.
- Weng, X. Airport Pavement Design; China Communication Press: Beijing, China, 2017. [Google Scholar]
- Meng, Q.; Zhong, K.; Sun, M. Dynamic Response Analysis of Airport Pavement under Impact Loading. Appl. Sci. 2023, 13, 5723. [Google Scholar] [CrossRef]
- Zhu, L. Simulation and Expression of Dynamic Behavior of Rigid Pavement Based on Large Aircraft Virtual Prototype. Doctor’s Thesis, Tongji University, Shanghai, China, 2017. [Google Scholar]
- JTG E60—2008; Field Test Methods of Subgrade and Pavement for Highway Engineering. China Communications Press: Beijing, China, 2008.
- MH/T 5110-2015; In-situ Measurement Specification for Pavement and Subgrade of Civil Airports. Civil Aviation Administration of China: Beijing, China, 2015.
- Zhao, J.; Wang, H. Dynamic pavement response analysis under wide-base tyre considering vehicle-tyre–pavement interaction. Road Mater. Pavement Des. 2021, 23, 1650–1666. [Google Scholar] [CrossRef]
- Xie, N.; Lv, S.; He, Y.; Lei, W.; Pu, C.; Meng, H.; Ma, H.; Peng, X. Load response and fatigue life of cement-stabilized macadam base structure considering dynamic and static load differences and tension-compression modulus differences. Constr. Build. Mater. 2023, 394, 132060. [Google Scholar] [CrossRef]
- Ameen, M. Computational Elasticity. Theory of Elasticity and Finite and Boundary Element Methods; Alpha Science International Ltd.: Oxford, UK, 2005. [Google Scholar]


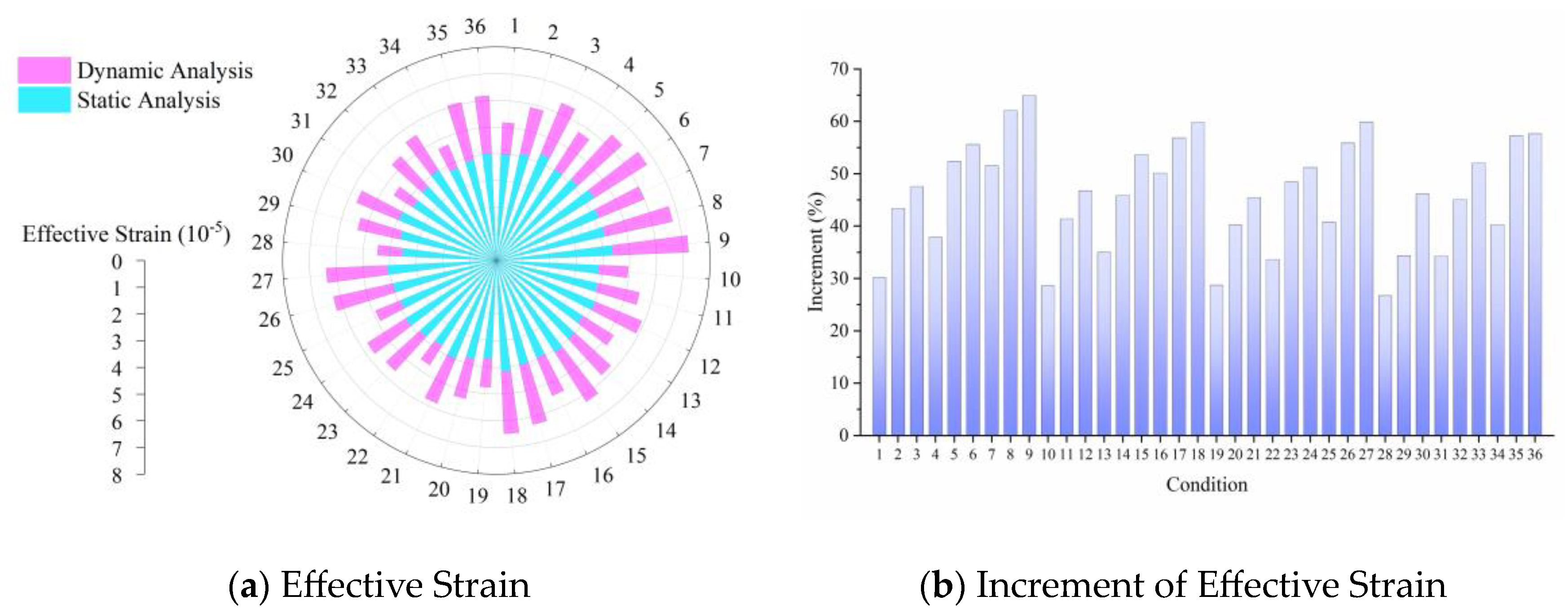
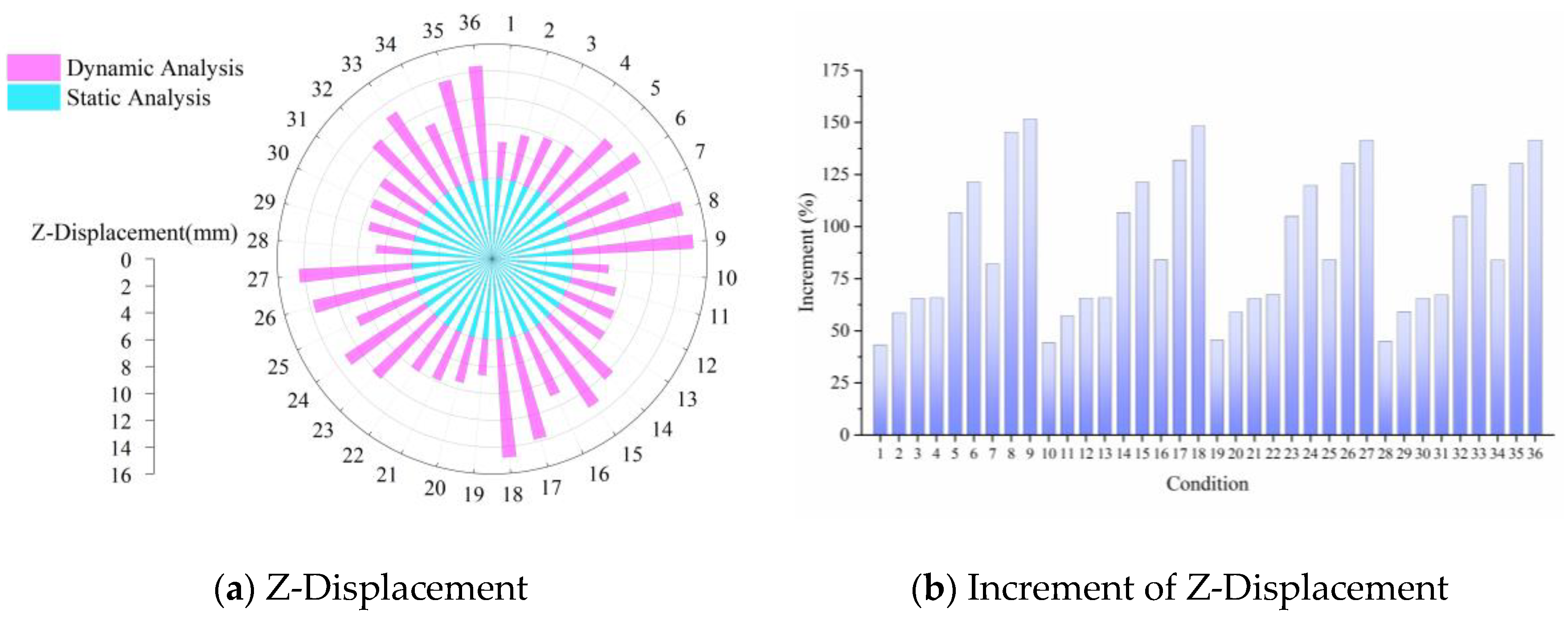

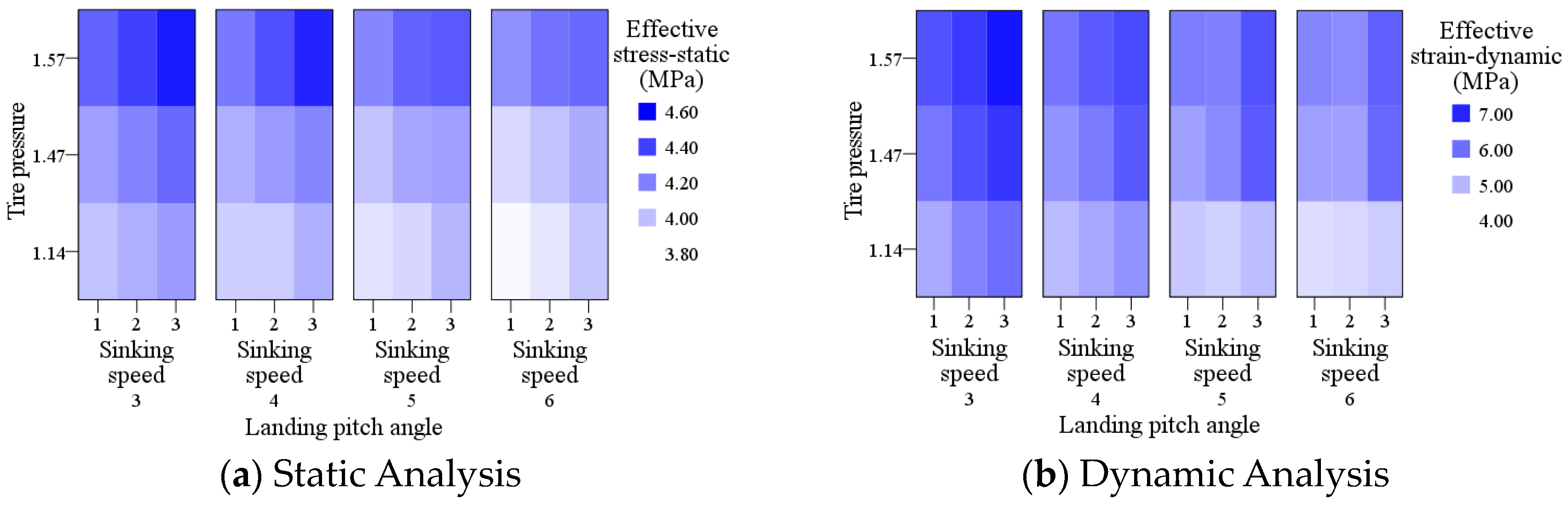

| Part | C10 [MPa] | C20 [MPa] | C30 [MPa] | Density [g/cm3] |
|---|---|---|---|---|
| Tread compound rubber | 0.534 | −0.051 | 0.01880 | 1.217 |
| Sidewall rubber | 0.413 | −0.041 | 0.01742 | 1.151 |
| Carcass rubber | 0.574 | −0.055 | 0.01961 | 1.158 |
| Tread bead rubber | 0.347 | −0.033 | 0.01553 | 1.144 |
| Tread rubber | 0.526 | −0.050 | 0.01852 | 1.176 |
| Structural Layer | Thickness [m] | Elastic Modulus [MPa] | Poisson’s Ratio | Density [kg/m3] |
|---|---|---|---|---|
| Cement concrete layer | 0.42 | 32,500 | 0.15 | 2500 |
| Cement stabilized base (upper) | 0.2 | 1700 | 0.25 | 2100 |
| Cement stabilized base (lower) | 0.2 | 1700 | 0.25 | 2100 |
| Soil subgrade | 4.12 | 70 | 0.4 | 1800 |
| No. | Condition | No. | Condition | No. | Condition |
|---|---|---|---|---|---|
| 1 | 3°-1 m/s-1.14 MPa | 13 | 4°-2 m/s-1.14 MPa | 25 | 5°-3 m/s-1.14 MPa |
| 2 | 3°-1 m/s-1.47 MPa | 14 | 4°-2 m/s-1.47 MPa | 26 | 5°-3 m/s-1.47 MPa |
| 3 | 3°-1 m/s-1.57 MPa | 15 | 4°-2 m/s-1.57 MPa | 27 | 5°-3 m/s-1.57 MPa |
| 4 | 3°-2 m/s-1.14 MPa | 16 | 4°-3 m/s-1.14 MPa | 28 | 6°-1 m/s-1.14 MPa |
| 5 | 3°-2 m/s-1.47 MPa | 17 | 4°-3 m/s-1.47 MPa | 29 | 6°-1 m/s-1.47 MPa |
| 6 | 3°-2 m/s-1.57 MPa | 18 | 4°-3 m/s-1.57 MPa | 30 | 6°-1 m/s-1.57 MPa |
| 7 | 3°-3 m/s-1.14 MPa | 19 | 5°-1 m/s-1.14 MPa | 31 | 6°-2 m/s-1.14 MPa |
| 8 | 3°-3 m/s-1.47 MPa | 20 | 5°-1 m/s-1.47 MPa | 32 | 6°-2 m/s-1.47 MPa |
| 9 | 3°-3 m/s-1.57 MPa | 21 | 5°-1 m/s-1.57 MPa | 33 | 6°-2 m/s-1.57 MPa |
| 10 | 4°-1 m/s-1.14 MPa | 22 | 5°-2 m/s-1.14 MPa | 34 | 6°-3 m/s-1.14 MPa |
| 11 | 4°-1 m/s-1.47 MPa | 23 | 5°-2 m/s-1.47 MPa | 35 | 6°-3 m/s-1.47 MPa |
| 12 | 4°-1 m/s-1.57 MPa | 24 | 5°-2 m/s-1.57 MPa | 36 | 6°-3 m/s-1.57 MPa |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meng, Q.; Zhong, K.; Li, Y.; Sun, M. Comparative Study on Mechanical Response in Rigid Pavement Structures of Static and Dynamic Finite Element Models. Aerospace 2024, 11, 596. https://doi.org/10.3390/aerospace11070596
Meng Q, Zhong K, Li Y, Sun M. Comparative Study on Mechanical Response in Rigid Pavement Structures of Static and Dynamic Finite Element Models. Aerospace. 2024; 11(7):596. https://doi.org/10.3390/aerospace11070596
Chicago/Turabian StyleMeng, Qiao, Ke Zhong, Yuchun Li, and Mingzhi Sun. 2024. "Comparative Study on Mechanical Response in Rigid Pavement Structures of Static and Dynamic Finite Element Models" Aerospace 11, no. 7: 596. https://doi.org/10.3390/aerospace11070596
APA StyleMeng, Q., Zhong, K., Li, Y., & Sun, M. (2024). Comparative Study on Mechanical Response in Rigid Pavement Structures of Static and Dynamic Finite Element Models. Aerospace, 11(7), 596. https://doi.org/10.3390/aerospace11070596






