Study on Heat Transfer Characteristics of Jet Impingement of Turbine Bending Surface
Abstract
1. Introduction
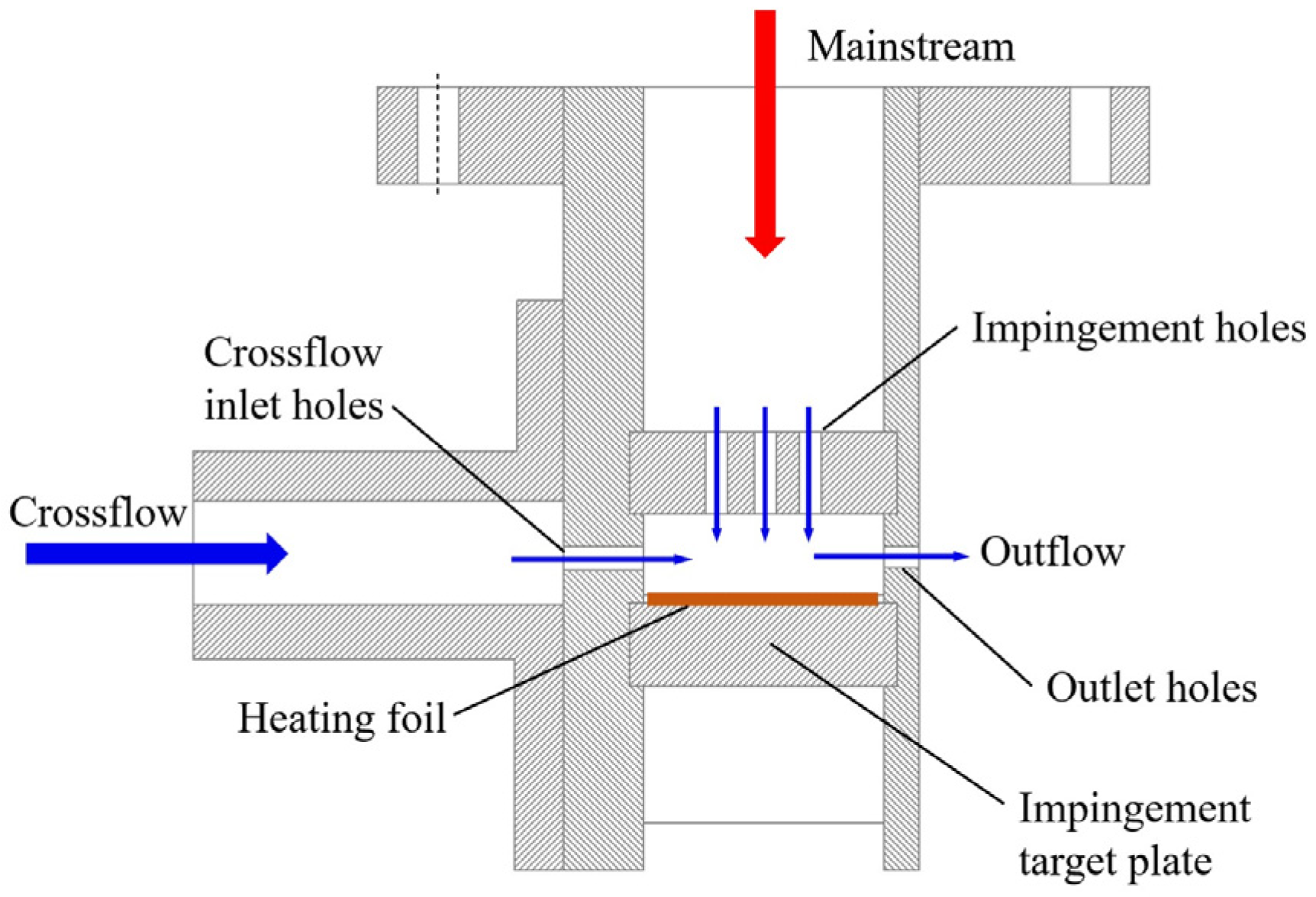
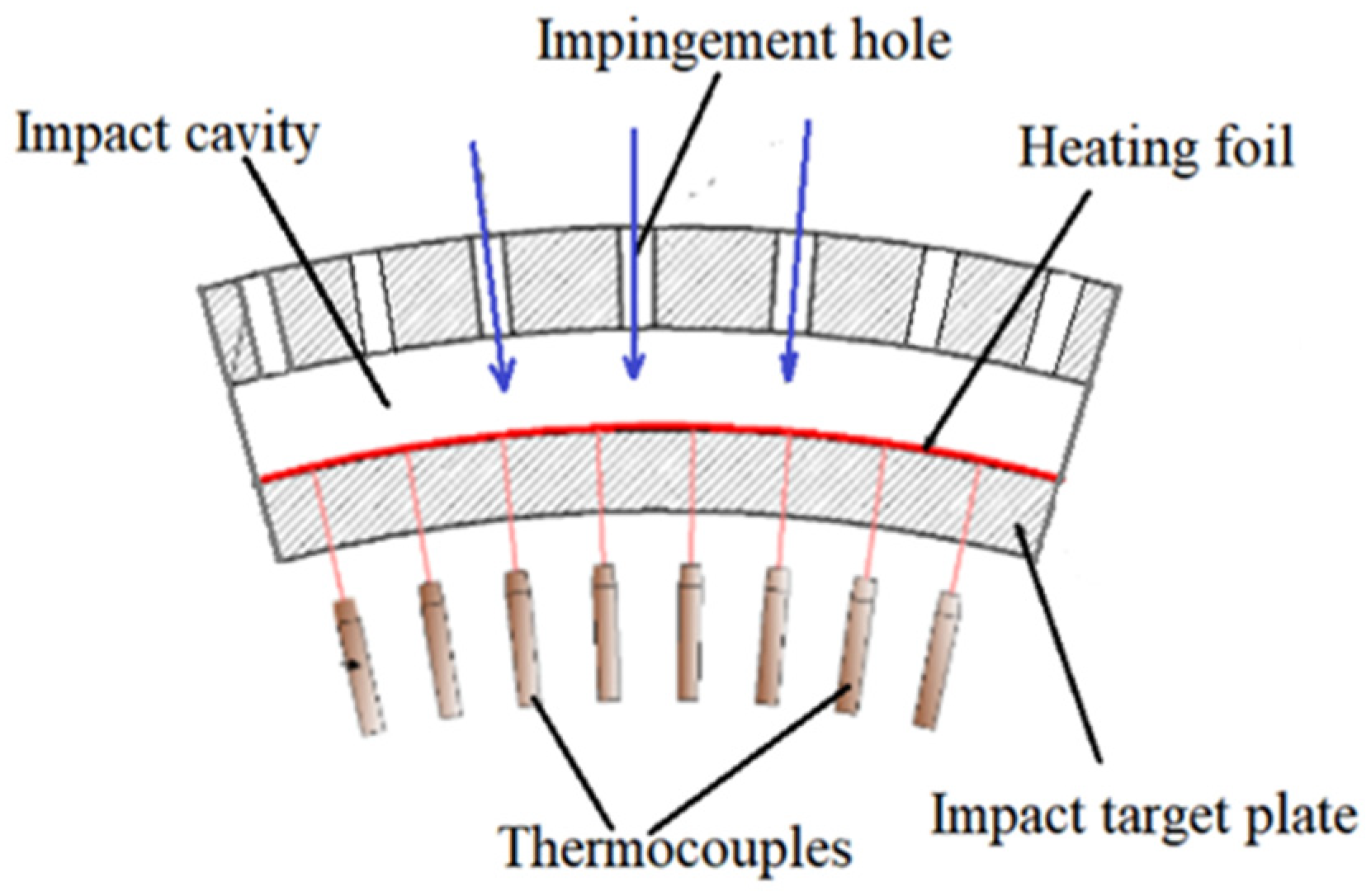
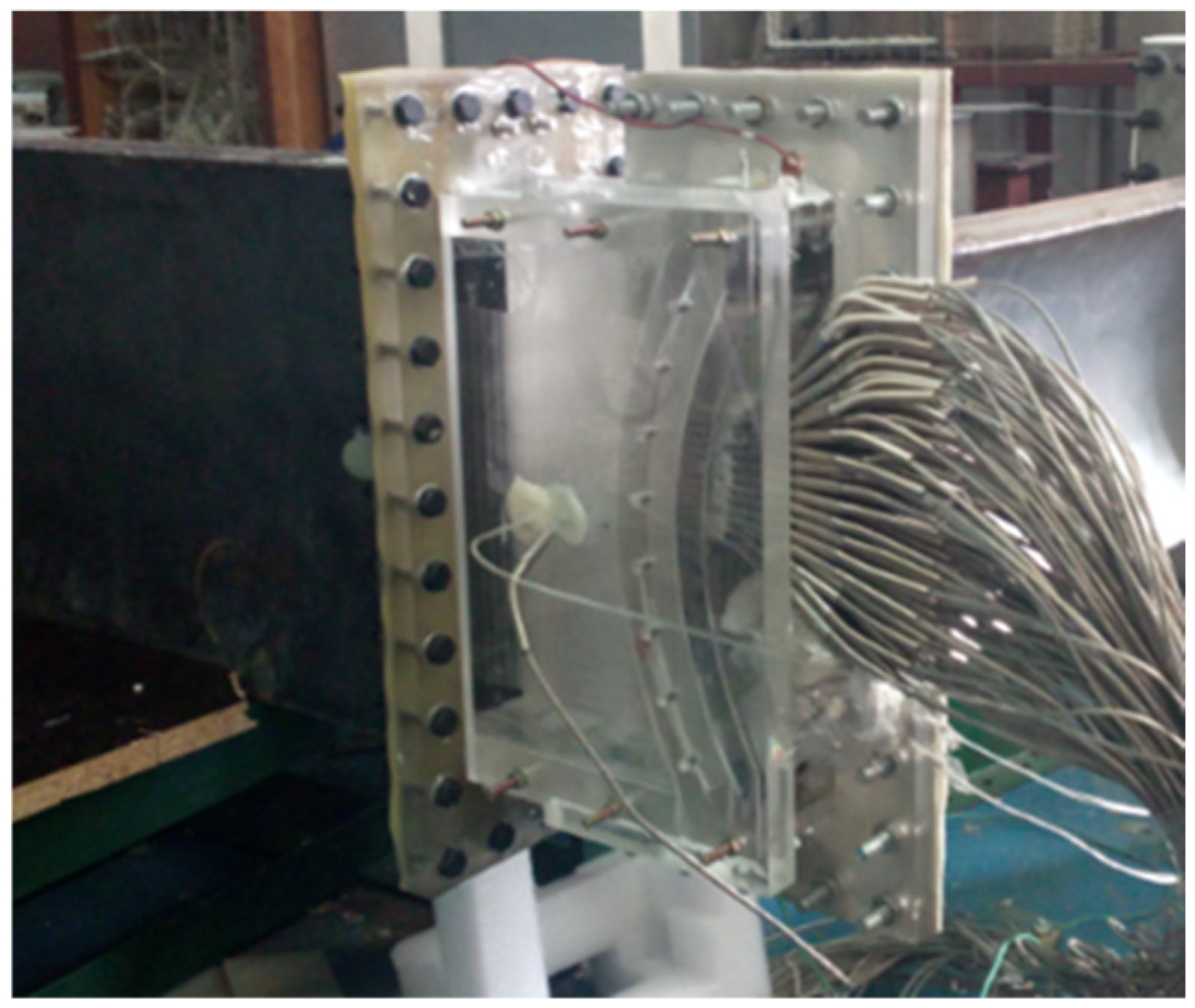
2. Experimental Setup and Test Facility
Test System
3. Definition of Parameters
4. Analysis of Test Results
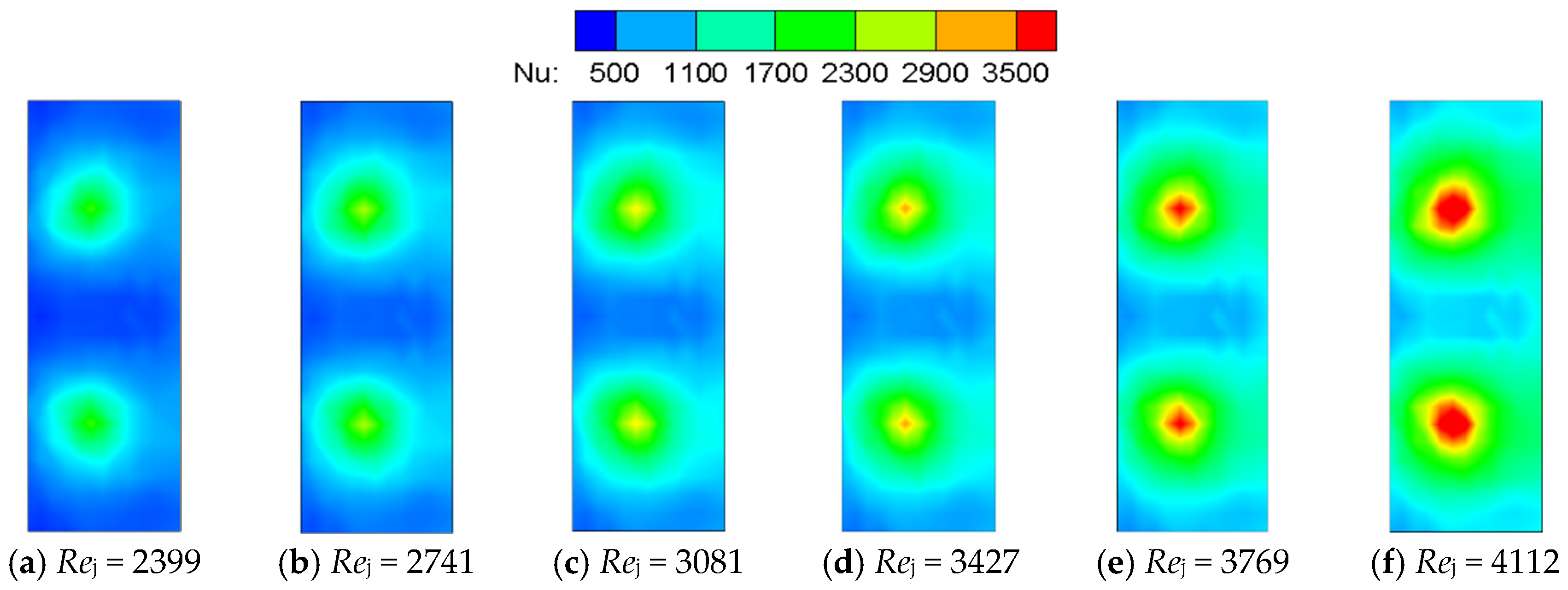
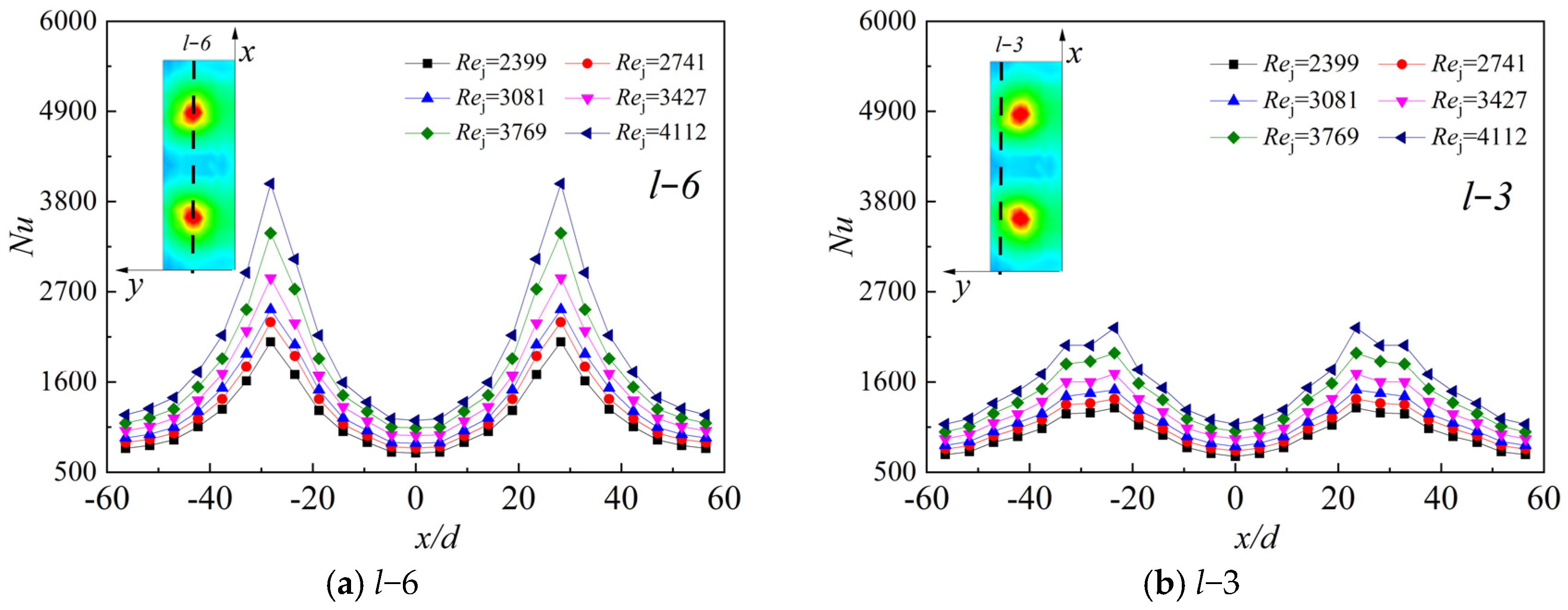
4.1. The Effect of Rej on Nu in Typical Structure
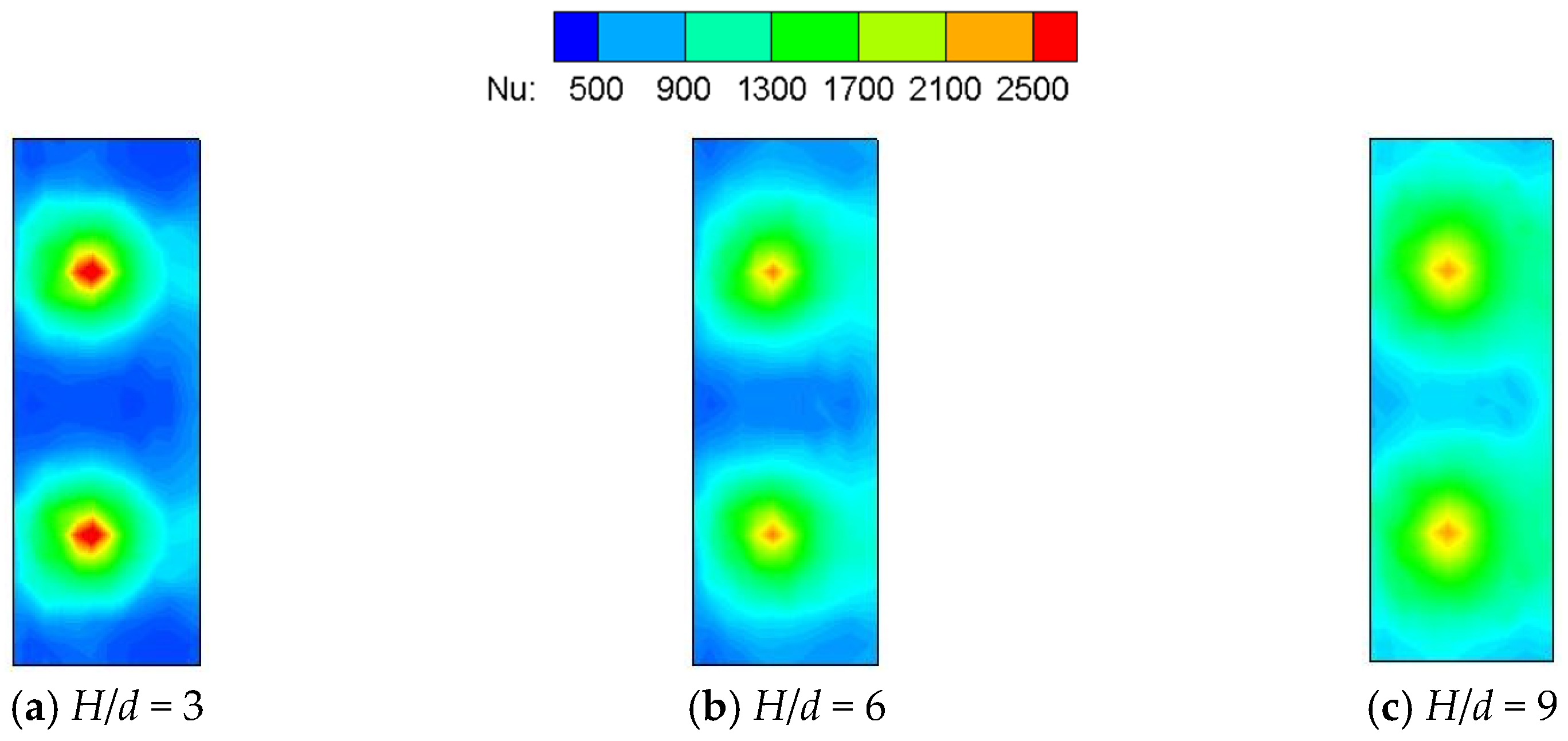
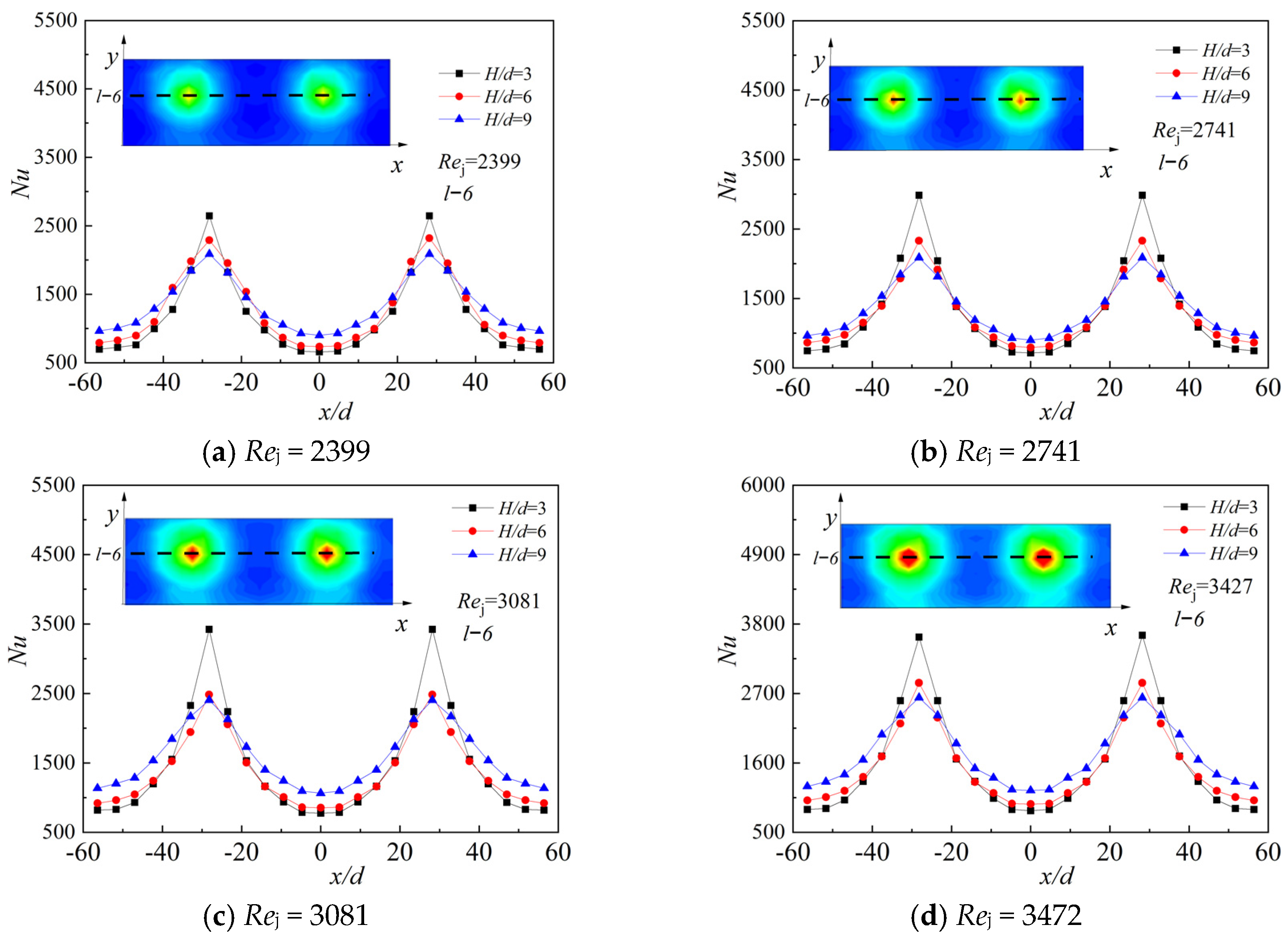
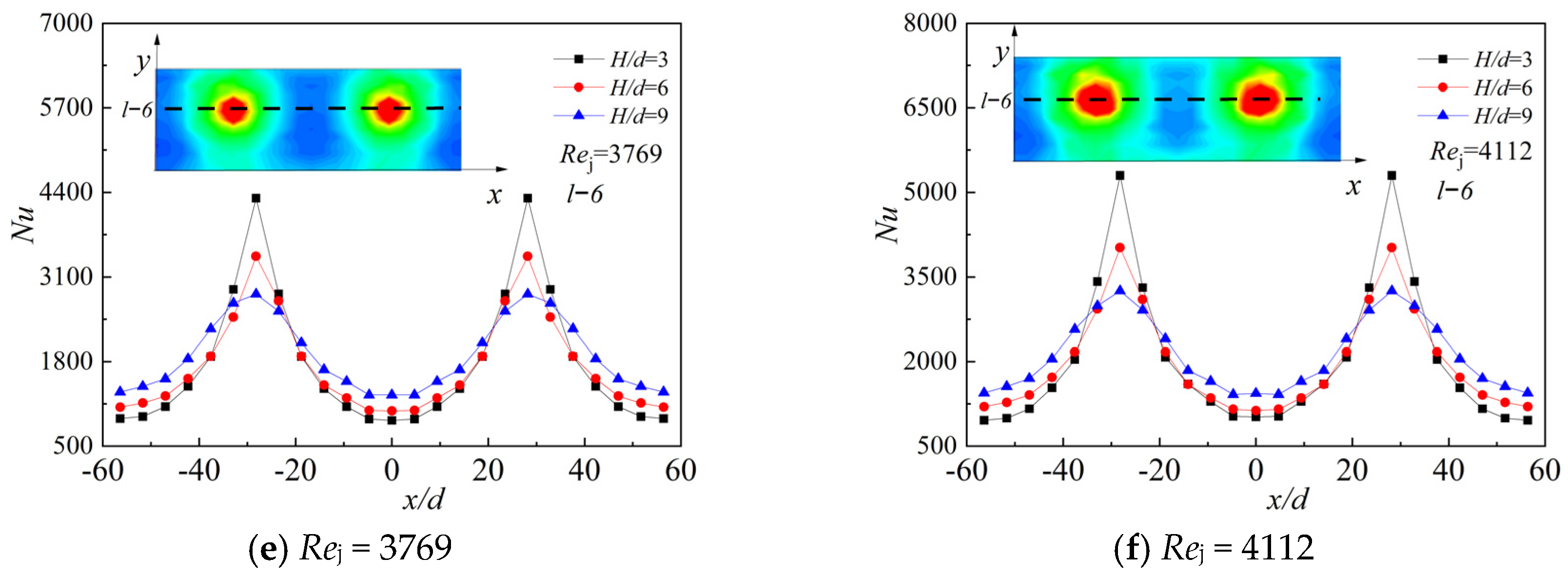
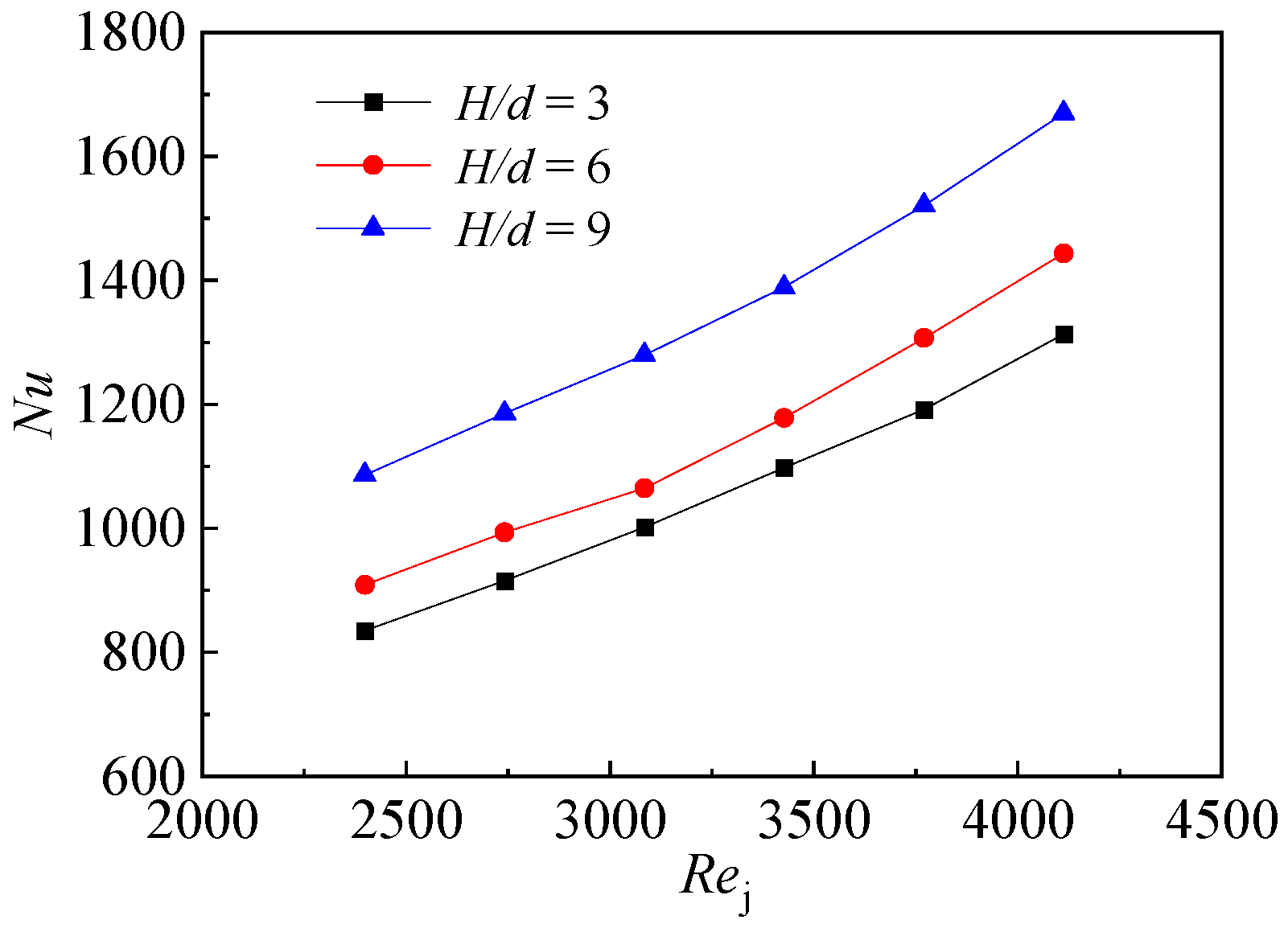
4.2. Effect of Relative Impingement Distance H/d on Nu
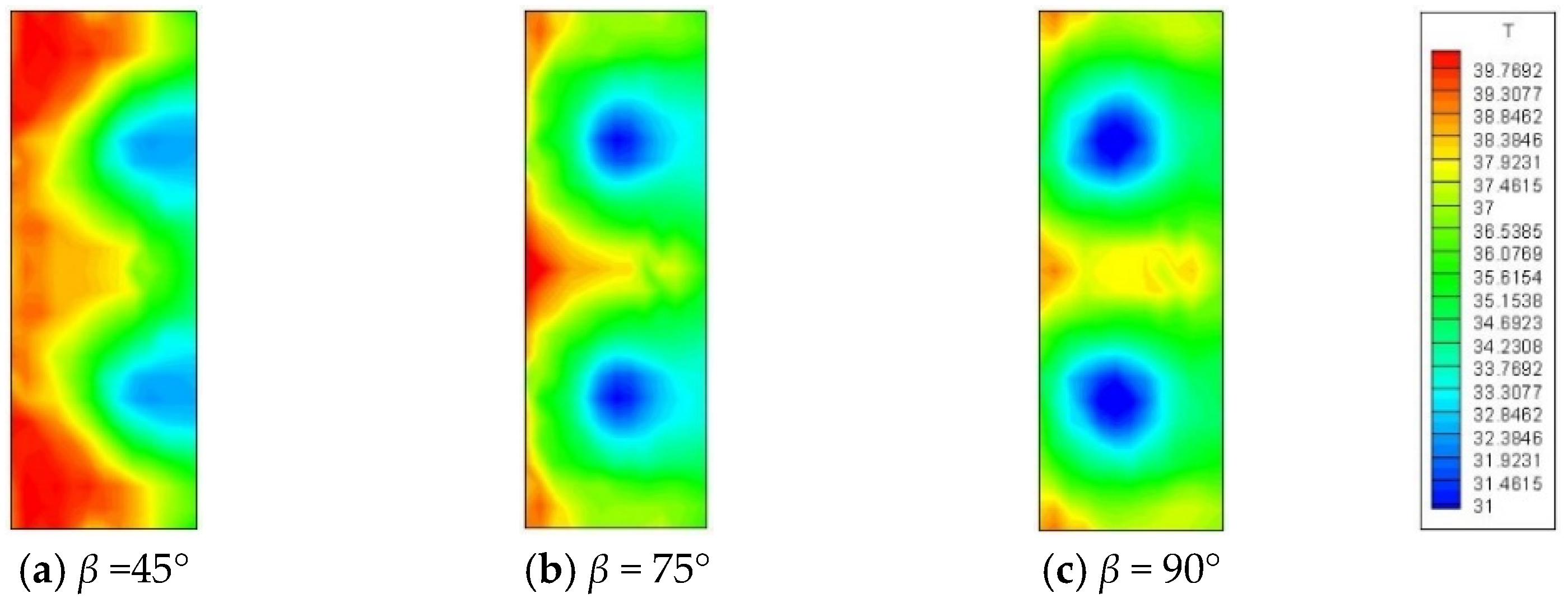
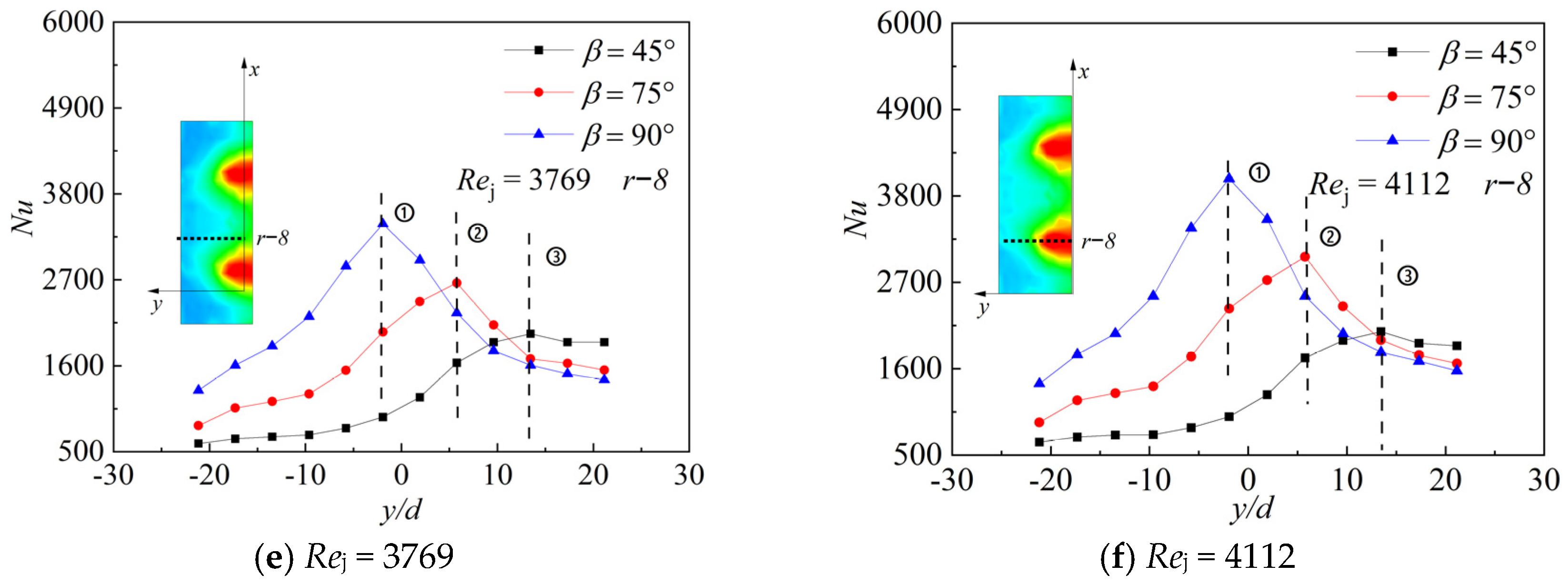
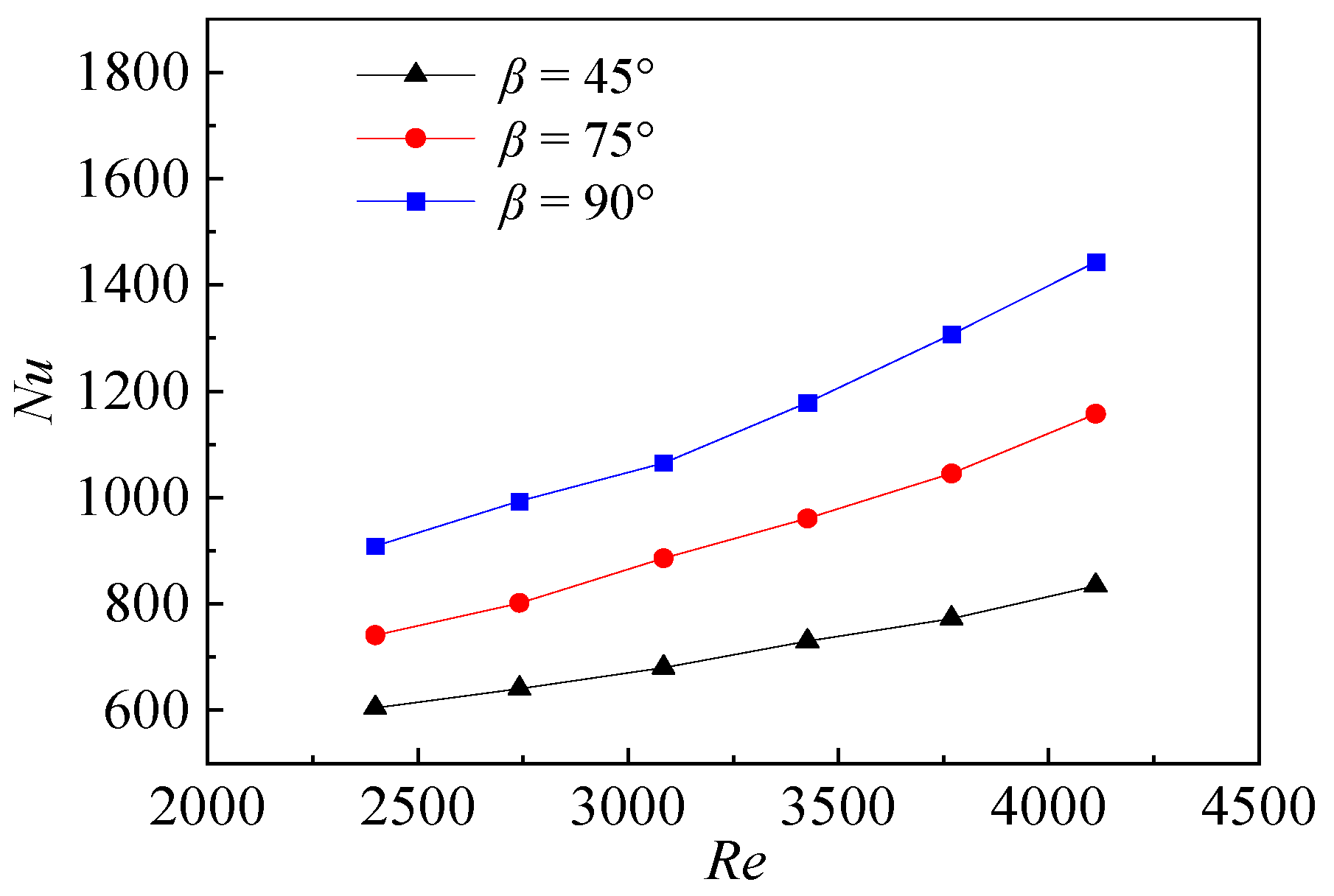
4.3. Effect of Impinging Angle (β) on Nu
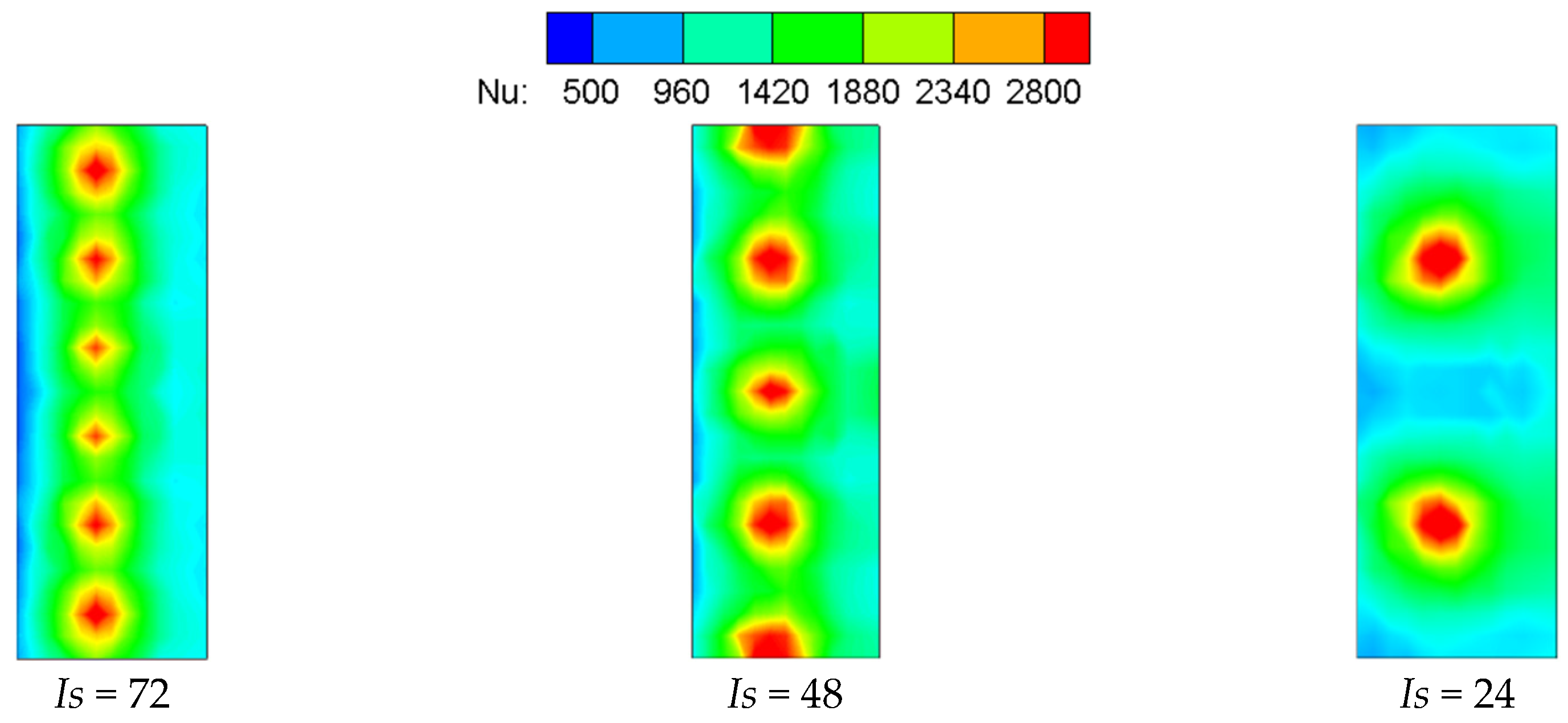
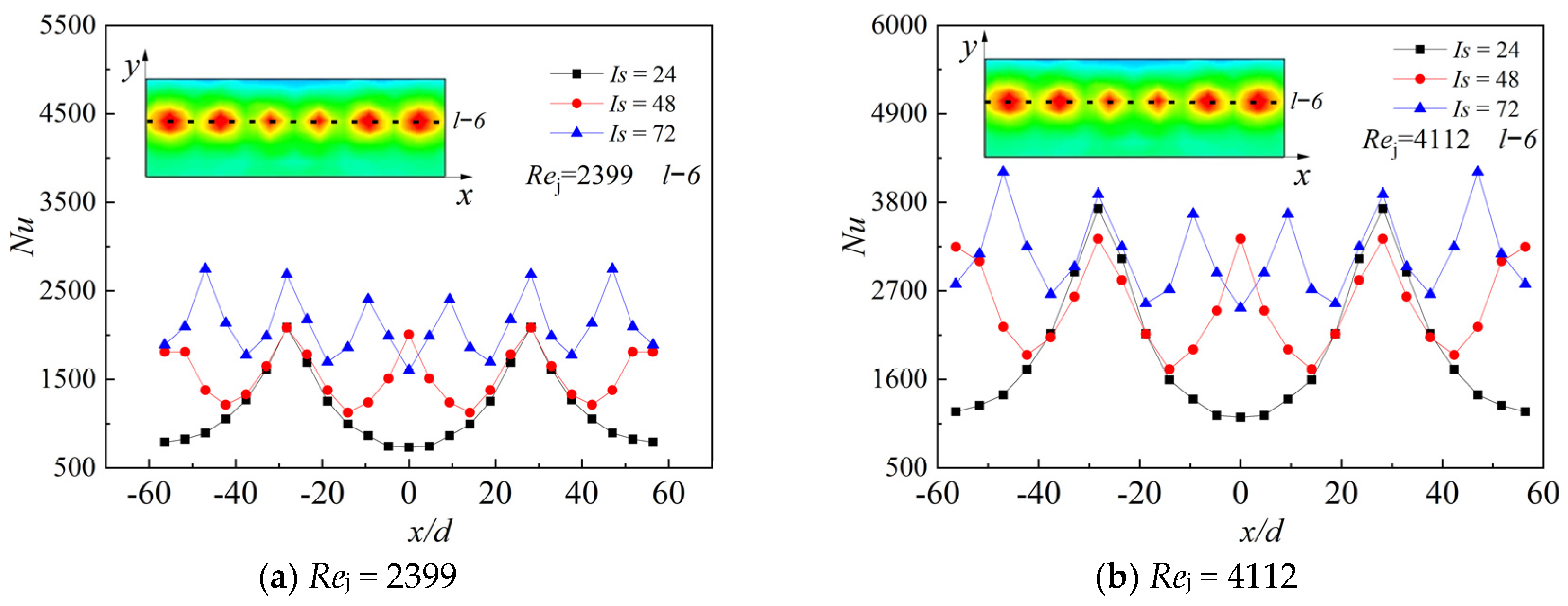
4.4. The Effect of Consistency (Is) on Nu
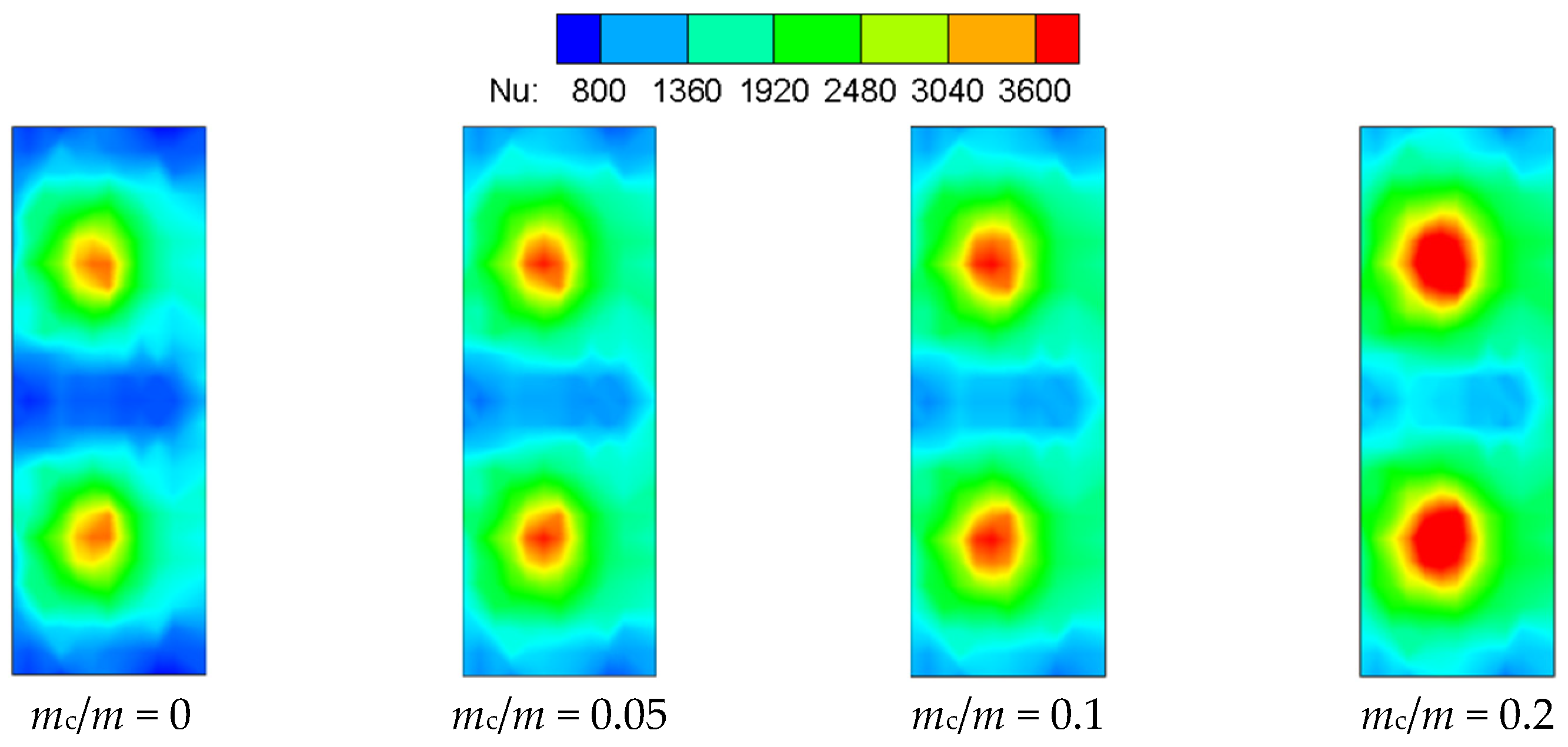
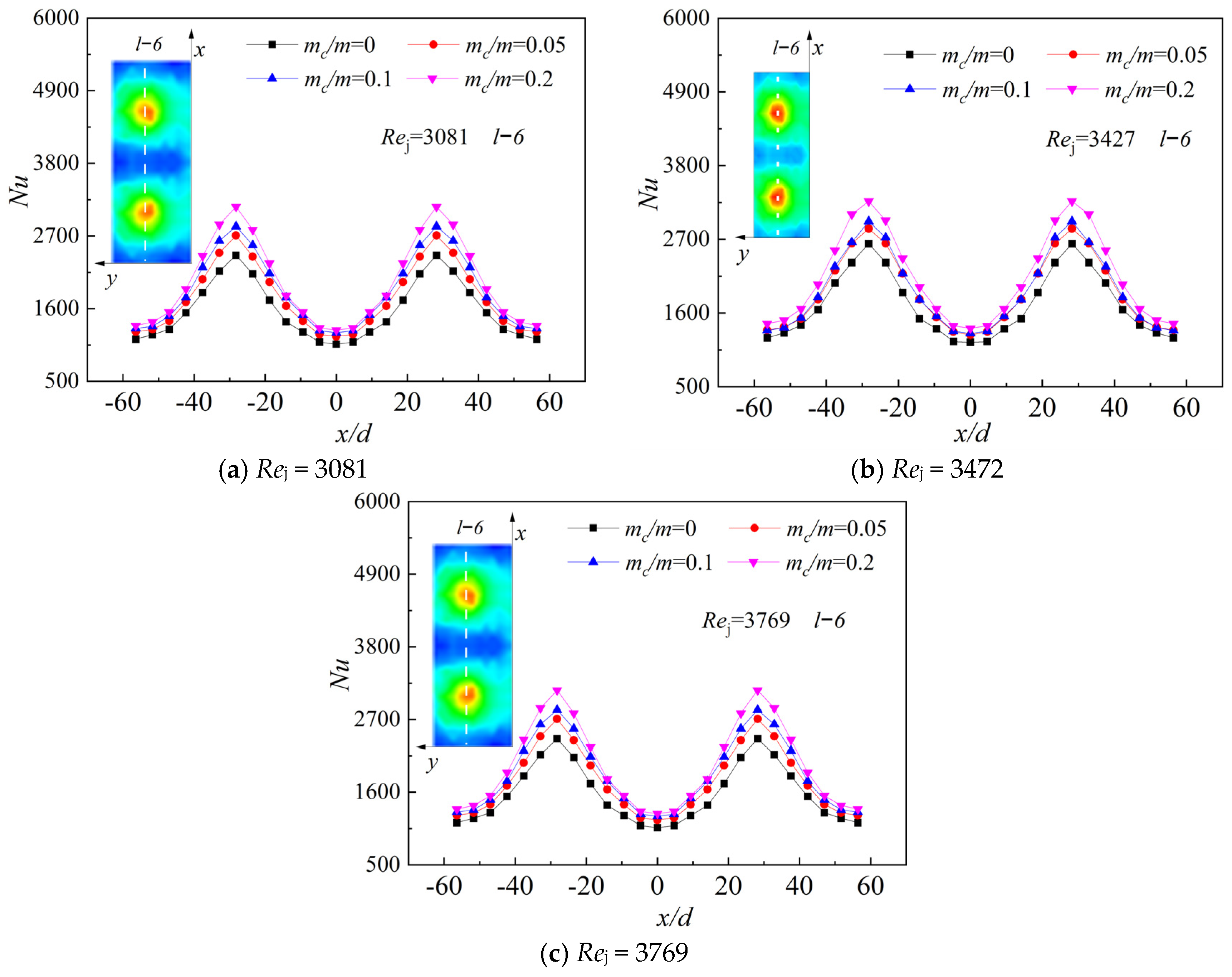
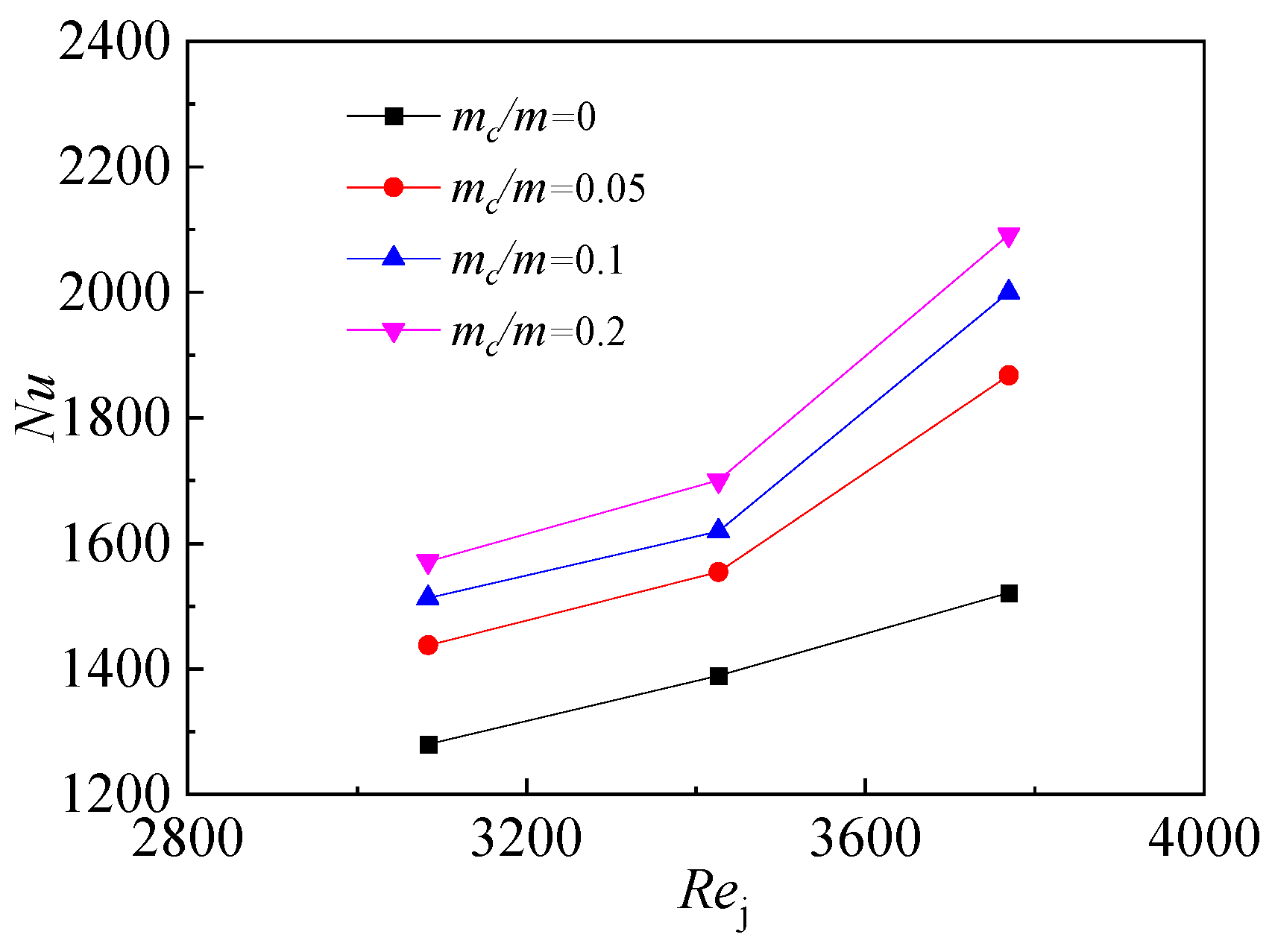
4.5. The Effect of Initial Crossflow Ratio (mc/m) on Nu
5. Conclusions
- (1)
- Effect of the equivalent Reynolds number: The effectiveness of heat transfer on the impingement plate enhances with an increase in the Reynolds number (Rej). Specifically, the peak heat transfer efficiency occurs at a Rej of 4112, showcasing significant improvements in local and average heat transfer performance.
- (2)
- Effect of elative impingement distance: The distance between the jet and the target plate plays a crucial role in heat transfer efficiency. For a shorter distance (H/d = 3), we observe a peak in local heat transfer rates, whereas, for a larger distance (H/d = 9), the average heat transfer rate is more favorable, especially at an Rej of 4112.
- (3)
- Effect of impingement angle: Changing the angle at which the jet hits the target surface affects heat transfer, with a 90° angle offering the most significant benefits. This configuration achieves the highest local and average heat transfer rates across all tested Reynolds numbers, peaking at a Rej of 4112.
- (4)
- Effect of hole consistency: Increasing the number of holes around the circumference of the impingement jet enhances the uniformity of the jet’s coverage and, consequently, the overall heat transfer efficiency. The best performance is noted with the highest consistency level (Is = 72) at all Reynolds numbers, showing a peak in both local and average heat transfer at an Rej of 4112.
- (5)
- Effect of initial crossflow: Introducing a crossflow at the inlet significantly benefits the heat transfer by enhancing the removal of boundary layers near the plate’s surface. As the ratio of initial crossflow increases, so does the heat transfer effectiveness, with the most notable improvements observed at an Rej of 3769, with an initial crossflow ratio of 0.2.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | Heating area |
| A0 | Area of the target plate |
| dc | Diameter of impingement hole |
| d | Diameter of crossflow inlet hole |
| do | Diameter of crossflow outlet hole |
| H | Impingement distance |
| H/d | Relative impingement distance |
| h | Convective heat transfer coefficient |
| I | Current flowing through the heating film |
| Is | Impingement hole consistency |
| l | Arc length of the periodic target plate |
| m | Equivalent mass flow through the target surface |
| mc | Mass flow of the crossflow |
| mc/m | Initial crossflow ratio |
| Nu | Nusselt number |
| Q | Heat generated by heating film |
| Qloss | Heat loss due to conduction |
| Rej | Impingement Reynolds number |
| T0 | Wall temperature when the heating film is not powered on |
| Tw | Temperature of the heating film |
| Toutside | Temperature of the outer wall of the impingement target plate |
| U | Voltage value at the ends of the heating film |
| Greek alphabets | |
| α | Circumferential spacing angle of the impingement hole |
| β | Impingement direction angle of the impingement hole |
| δ | Thickness of the target plate |
| λ | Thermal conductivity of the impingement target plate |
| λair | Thermal conductivity of the airflow |
| μ | Dynamic viscosity of the mainstream |
| φ | Circumferential spacing angle of the crossflow inlet and outlet hole |
References
- Yang, J.; Wang, X.J.; Yang, Q. Advances in jet impinging heat transfer. Vacuum Cryog. 2018, 24, 217–222. [Google Scholar]
- Xie, G.; Liu, C.L.; Ye, L.; Wang, R.; Niu, J.J.; Zhai, Y.N. Effects of impingement gap and hole arrangement on overall cooling effectiveness for impingement/effusion cooling. Int. J. Heat Mass Transf. 2020, 152, 119449. [Google Scholar] [CrossRef]
- Tepe, A.Ü.; Yetişken, Y.; Uysal, Ü.; Arslan, K. Experimental and numerical investigation of jet impingement cooling using extended jet holes. Int. J. Heat Mass Transf. 2020, 158, 119945. [Google Scholar] [CrossRef]
- Metzger, D.E.; Florschuetz, L.W.; Takeuchi, D.I.; Behee, R.D.; Berry, R.A. Heat transfer characteristics for inline and staggered arrays of circular jets with crossflow of spent air. J. Heat Transf. 1979, 101, 526–531. [Google Scholar] [CrossRef]
- Florschuetz, L.W.; Metzger, D.E.; Su, C.C. Heat Transfer Characteristics for Jet Array Impingement with Initial Crossflow. In Proceedings of the ASME 1983 International Gas Turbine Conference and Exhibit, Phoenix, AZ, USA, 27–31 March 1983. [Google Scholar]
- Florschuetz, L.W.; Su, C.C. Effects of Crossflow Temperature on Heat Transfer Within an Array of Impinging Jets. J. Heat Transf. 1987, 109, 74–82. [Google Scholar] [CrossRef]
- Zhang, Z.Y.; Zhang, J.Z.; Yang, W.H. Convective heat transfer of jet impingement inside semi-confined channel. J. Aeronaut. Power 2006, 4, 626–630. [Google Scholar]
- León De Paz, M.; Jubran, B.A. Numerical modeling of multi micro jet impingement cooling of a three-dimensional turbine vane. Heat Mass Transf. 2011, 47, 1561–1579. [Google Scholar] [CrossRef]
- Attalla, M.; Salem, M. Experimental Investigation of Heat Transfer for a Jet Impinging Obliquely on a Flat Surface. Exp. Heat Transf. 2014, 28, 378–391. [Google Scholar] [CrossRef]
- Wang, K.; Li, H.; Zhu, J. Experimental study of heat transfer characteristic on jet impingement cooling with film extraction flow. Appl. Therm. Eng. 2014, 70, 620–629. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, J.Z.; Yang, W.H. Experimental research on heat transfer characteristics of denser jet array impingement. Hangkong Dongli Xuebao/J. Aerosp. Power 2009, 24, 1264–1269. [Google Scholar]
- Shin, M.; Senguttuvan, S.; Kim, S. Investigations of Flow and Heat Transfer Characteristics in a Channel Impingement Cooling Configuration with a Single Row of Water Jets. Energies 2021, 14, 4327. [Google Scholar] [CrossRef]
- Otero Perez, J.J.; Sandberg, R.D.; Mizukami, S.; Tanimoto, K. High-fidelity simulations of multi-jet impingement cooling flows. J. Turbomach. 2021, 143, 081011. [Google Scholar] [CrossRef]
- Forster, M.; Weigand, B. Experimental and numerical investigation of jet impingement cooling onto a concave leading edge of a generic gas turbine blade. Int. J. Therm. Sci. 2021, 164, 106862. [Google Scholar] [CrossRef]
- Mao, J.K.; Miao, G.J.; Liu, Z.X. Experimental study of the flow structures of the concave surface with inclined impingement in limited space. J. Aeronaut. Power 2010, 25, 11–17. [Google Scholar]
- Waehayee, M.; Tekasakul, P.; Eiamsaard, S.; Nuntadusit, C. Flow and Heat Transfer Characteristics of in-Line Impinging Jets With Crossflow At Short Jet-To-Plate Distance. Exp. Heat Transf. 2015, 28, 511–530. [Google Scholar] [CrossRef]
- Lu, Q.; Muthukumar, R.; Ge, H.; Parameswaran, S. Numerical study of a rotating liquid jet impingement cooling system. Int. J. Heat Mass Transf. 2020, 163, 120. [Google Scholar] [CrossRef]
- Qiu, D.; Luo, L.; Zhao, Z.; Wang, S.; Wang, Z.; Sunden, B. On heat transfer and flow characteristics of jets impingement on a concave surface with varying pin-fin arrangements. Int. J. Therm. Sci. 2021, 170, 107163. [Google Scholar] [CrossRef]
- He, W.; Deng, Q.; Yang, G.; Feng, Z. Effects of Turning Angle and Turning Internal Radius on Channel Impingement Cooling for a Novel Internal Cooling Structure. J. Turbomach. 2021, 143, 091005. [Google Scholar] [CrossRef]
- Ahmed, F.B.; Tucholke, R.; Weigand, B.; Meier, K. Numerical Investigation of Heat Transfer and Pressure Drop Characteristics for Different Hole Geometries of a Turbine Case Impingement Cooling System. In Proceedings of the ASME Turbo Expo: Turbine Technical Conference and Exposition, Vancouver, BC, Canada, 6–10 June 2011. [Google Scholar]
- Ma, L. Study on the Impact Heat Transfer Characteristics of Internal Array of Turbines. Master’s Thesis, Nanjing University of Aeronautics and Astronautics, Nanjing, China, 2014. [Google Scholar]
- Ortega-Casanova, J.; Granados-Ortiz, F.J. Numerical simulation of the heat transfer from a heated plate with surface variations to an impinging jet. Int. J. Heat Mass Transf. 2014, 76, 128–143. [Google Scholar] [CrossRef]
- Jia, Y.; Long, P.; Xueqin, B.; Shen, X.; Lin, G.; Bai, L. Experimental investigation and correlation development of jet impingement heat transfer with two rows of aligned jet holes on an internal surface of a wing leading edge. Chin. J. Aeronaut. 2018, 31, 1962–1972. [Google Scholar]
- Yamagami, S. Surface Orientation Effect on Local Heat Transfer by Round Water Jet Impingement. Flow Turbul. Combust. 2019, 102, 485–496. [Google Scholar] [CrossRef]
- Sriromreun, P.; Sriromreun, P. A Numerical and Experimental Investigation of Dimple Effects on Heat Transfer Enhancement with Impinging Jets. Energies 2019, 12, 813. [Google Scholar] [CrossRef]
- Yan, J.K.; Guo, T.; Zhu, H.R.; Zheng, J.; Guo, W.; Su, Y.L. Experimental investigation of heat transfer characteristics on micro-scale array jet impingement. J. Propul. Technol. 2016, 37, 1681–1687. [Google Scholar]
- Haji Hosseinloo, A.; Tan, S.P.; Yap, F.F.; Toh, K.C. Shock and vibration protection of submerged jet impingement cooling systems: Theory and experiment. Appl. Therm. Eng. 2014, 73, 1076–1086. [Google Scholar] [CrossRef]
- Joshi, S.N.; Dede, E.M. Two-phase jet impingement cooling for high heat flux wide band-gap devices using multi-scale porous surfaces. Appl. Therm. Eng. 2017, 110, 10–17. [Google Scholar] [CrossRef]


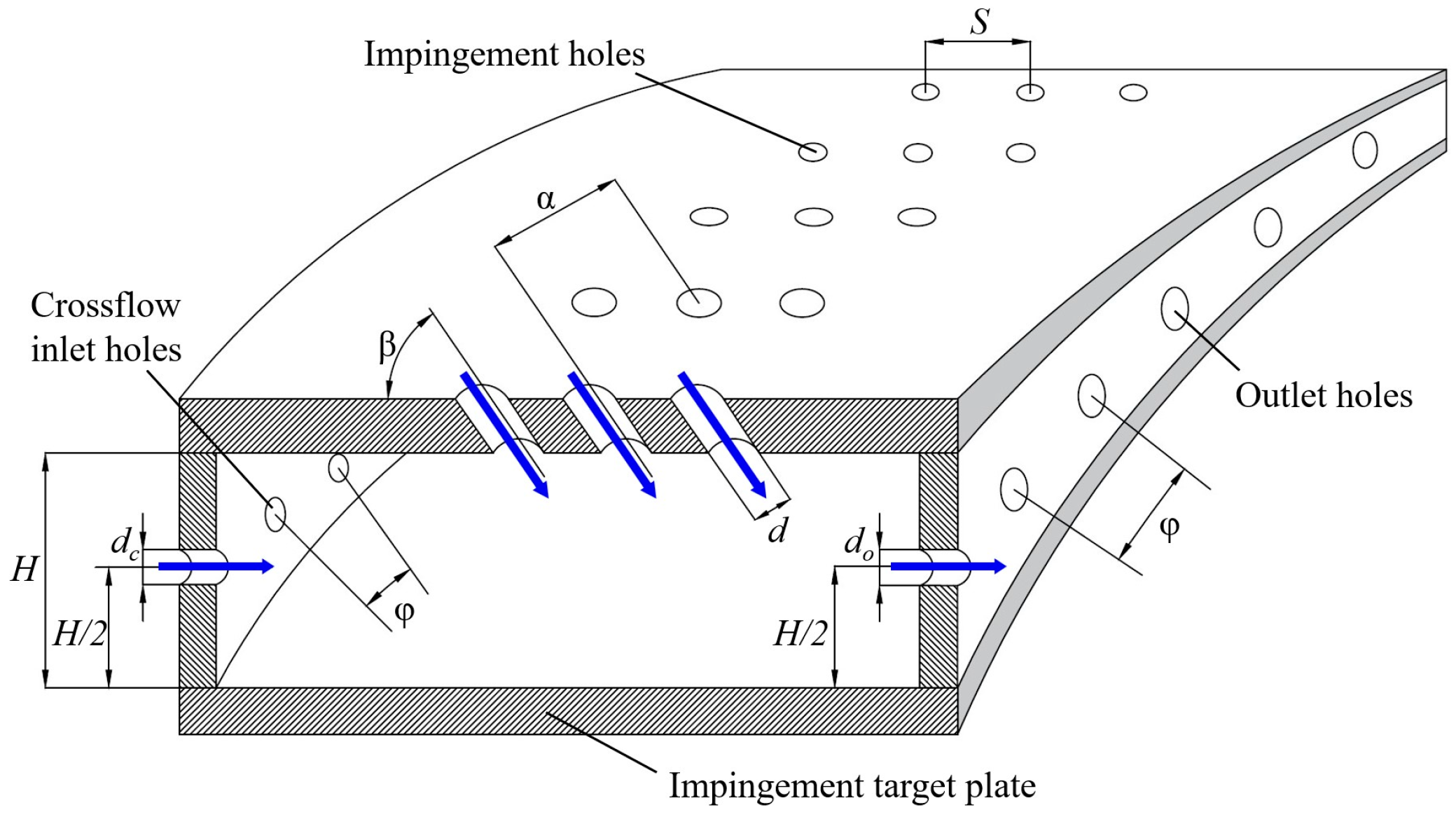



| Geometry | H/d | β/° | Is |
|---|---|---|---|
| No. 1 | 3 | 90 | 24 |
| No. 2 | 6 | 90 | 24 |
| No. 3 | 9 | 90 | 24 |
| No. 4 | 6 | 45 | 24 |
| No. 5 | 6 | 75 | 24 |
| No. 6 | 6 | 90 | 48 |
| No. 7 | 6 | 90 | 72 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zeng, F.; Sun, R.; Chen, Z.; Liu, Y.; Yang, W. Study on Heat Transfer Characteristics of Jet Impingement of Turbine Bending Surface. Aerospace 2024, 11, 554. https://doi.org/10.3390/aerospace11070554
Zeng F, Sun R, Chen Z, Liu Y, Yang W. Study on Heat Transfer Characteristics of Jet Impingement of Turbine Bending Surface. Aerospace. 2024; 11(7):554. https://doi.org/10.3390/aerospace11070554
Chicago/Turabian StyleZeng, Fei, Ruijia Sun, Zhenhua Chen, Yuang Liu, and Weihua Yang. 2024. "Study on Heat Transfer Characteristics of Jet Impingement of Turbine Bending Surface" Aerospace 11, no. 7: 554. https://doi.org/10.3390/aerospace11070554
APA StyleZeng, F., Sun, R., Chen, Z., Liu, Y., & Yang, W. (2024). Study on Heat Transfer Characteristics of Jet Impingement of Turbine Bending Surface. Aerospace, 11(7), 554. https://doi.org/10.3390/aerospace11070554




