Improved Multi-Body Dynamic Simulation of Landing Gear Drop Test Incorporating Structural Flexibility and Bearing Contact
Abstract
1. Introduction
2. Materials and Methods
2.1. Structural Parts
2.2. Dynamic Properties
2.2.1. Vertical Properties
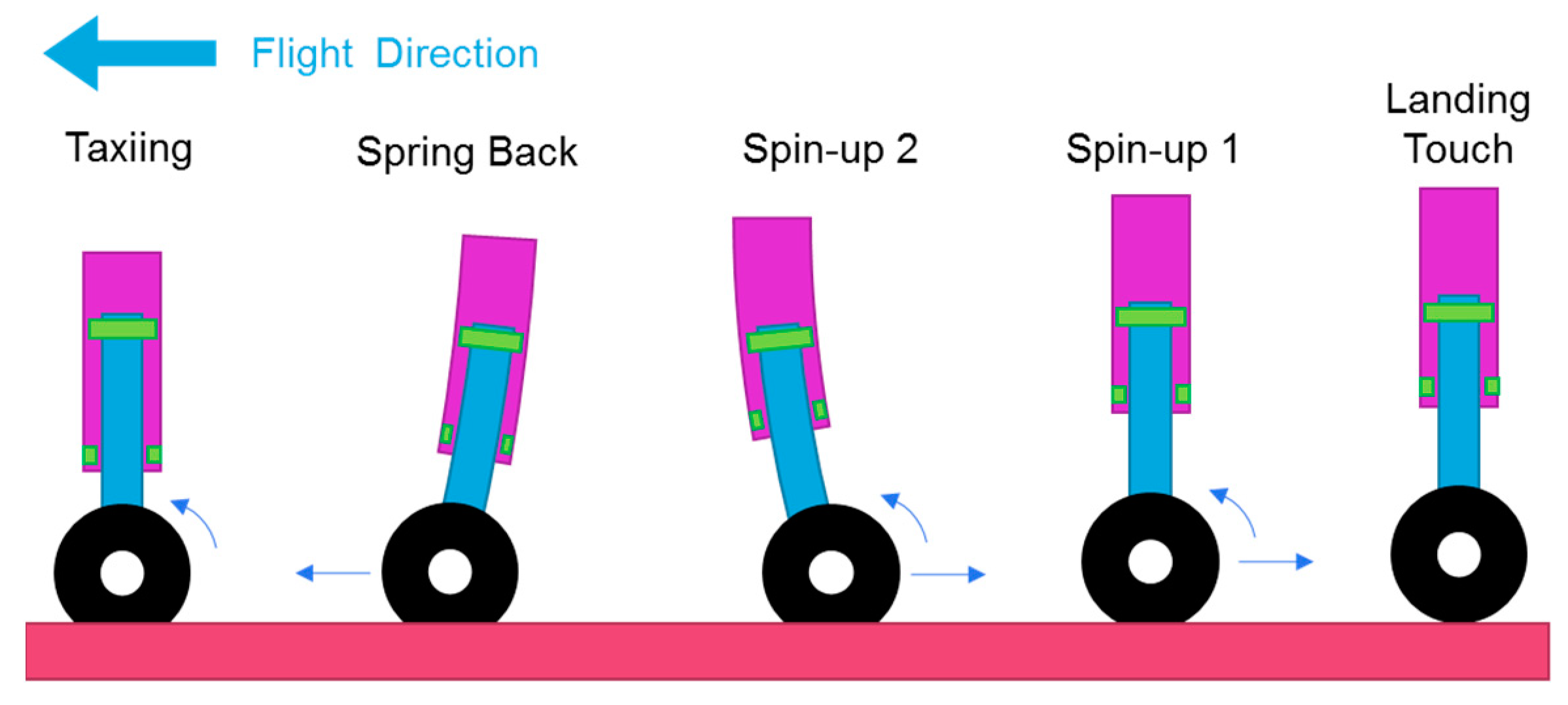
2.2.2. Longitudinal Properties
2.3. Experimental Drop Test
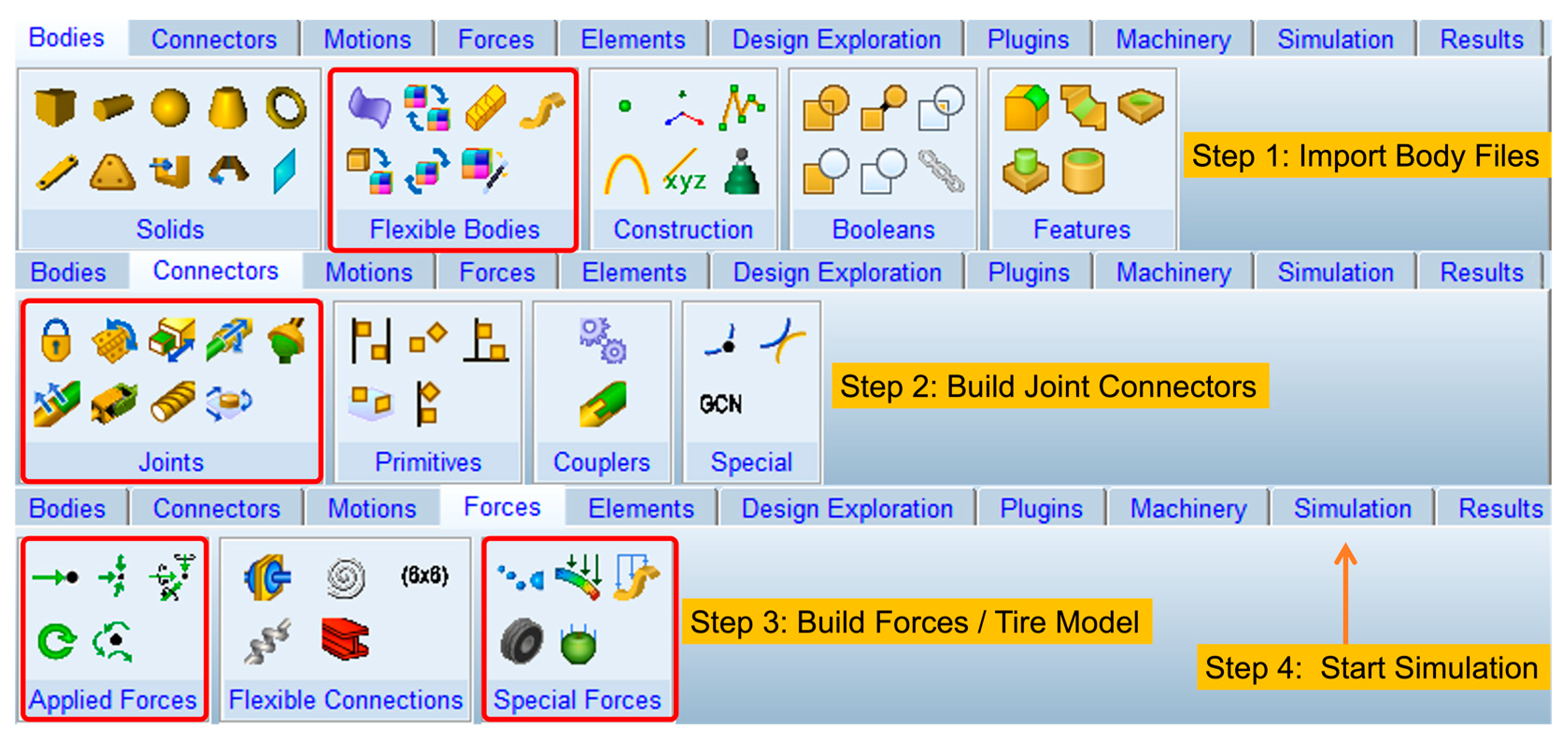
2.4. Multi-Body Dynamics Modeling
2.4.1. Theoretical Basis
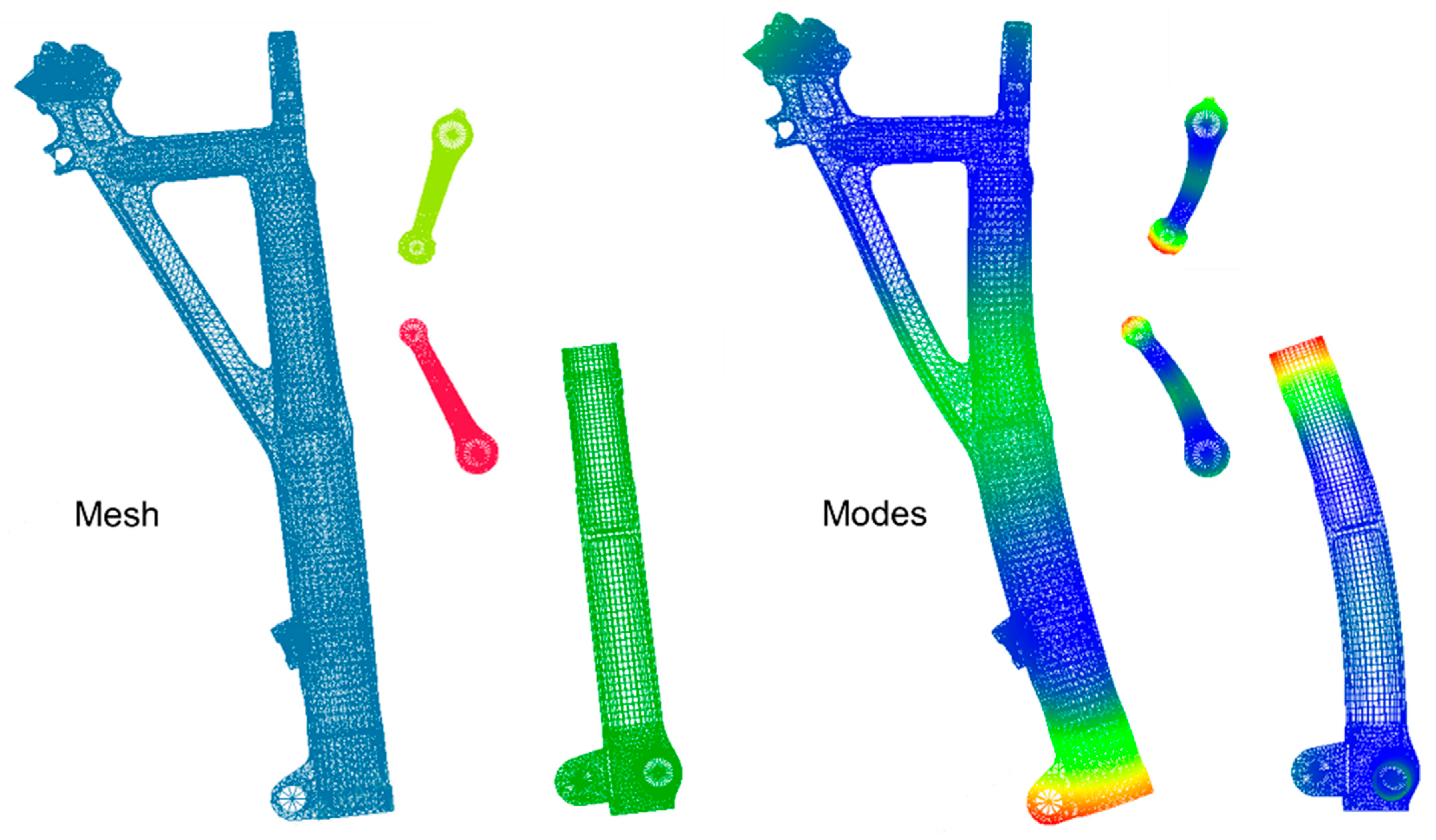
2.4.2. Flexible Bodies Modeling
2.4.3. Joint Connector
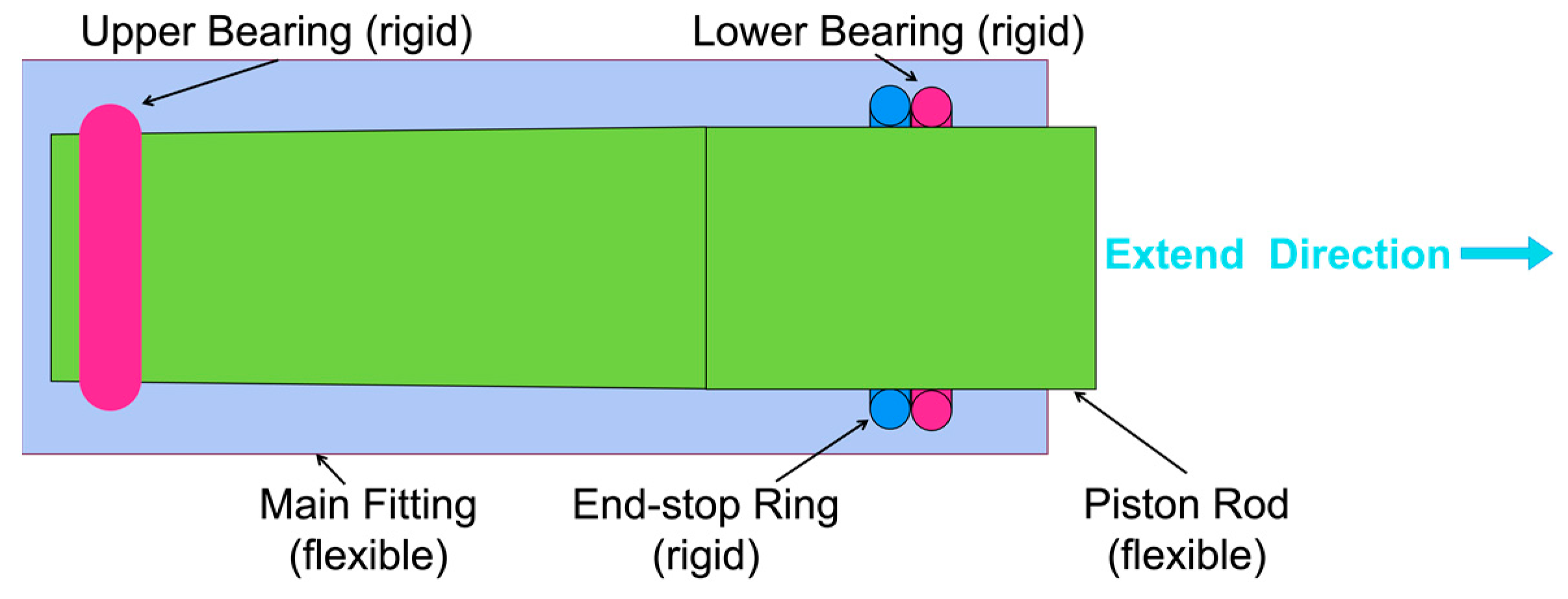
2.4.4. Bearing Contact
2.4.5. Others
3. Results and Discussion
3.1. MBD Model and Simlation Case
3.2. Simulation Results
3.3. Parameter Influence Study
3.3.1. Structure Flexibility Influence
3.3.2. Bearing Contact Influence
4. Future Direction
4.1. MBD Modeling
4.2. Landing Loads Design
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Suresh, P.; Sura, N.K.; Shankar, K.; Radhakrishnan, G. Synthesis of landing dynamics on land-base high performance aircraft considering multi-variate landing conditions. Mech. Based Des. Struct. Mach. 2023, 51, 3945–3964. [Google Scholar] [CrossRef]
- Schmidt, R.K. The Design of Aircraft Landing Gear; SAE International: Warrendale, PA, USA, 2021. [Google Scholar]
- Pritchard, J. Overview of landing gear dynamics. J. Aircr. 2001, 38, 130–137. [Google Scholar] [CrossRef]
- Fang, W.; Zhu, L.; Wang, Y. Landing Performance Study for Four Wheels Twin Tandem Landing Gear Based on Drop Test. Aerospace 2022, 9, 334. [Google Scholar] [CrossRef]
- EASA. Certification Specifications and Acceptable Means of Compliance for Large Aeroplanes, CS-25 Amendment 27; EASA: Cologne, Germany, 2023.
- Krüger, W.R.; Morandini, M. Recent developments at the numerical simulation of landing gear dynamics. CEAS Aeronaut. J. 2011, 1, 55–68. [Google Scholar] [CrossRef]
- Correia, D.; Ferreira, A. Aircrafts on-ground dynamics models and simulation software: State-of-the-art. Sustainability 2021, 13, 9147. [Google Scholar] [CrossRef]
- Taylor, P.; Hanson, L.; Barnes, T. A Brief History of Aircraft Loads Analysis Methods. In Proceedings of the 44th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Norfolk, VA, USA, 7–10 April 2003. [Google Scholar] [CrossRef]
- Norwood, D.S.; Chichester, R.H. Full scale aircraft drop test program for the F-35C carrier variant. In Proceedings of the 56th AIAA/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Kissimmee, FL, USA, 5–9 January 2015. [Google Scholar] [CrossRef]
- Caijun, X.; Xiuli, X.; Wengang, Q. Research on the performance of buffer for landing gear based on the drop test. J. Vibroeng. 2012, 14, 794–804. [Google Scholar]
- Wang, H.; Wu, D.; Wang, F.; Ren, H. A method for determining the horizontal impact load based on the rotational speed of the aircraft’s wheel in a landing gear drop test. Int. J. Crashworthiness 2018, 23, 627–634. [Google Scholar] [CrossRef]
- Gan, S.; Fang, X.; Wei, X. Parametric Analysis on Landing Gear Strut Friction of Light Aircraft for Touchdown Performance. Appl. Sci. 2021, 11, 5445. [Google Scholar] [CrossRef]
- Kang, B.H.; Kim, B.G.; Choi, S.B. Aircraft landing gear system with magnetorheological shock strut: Performance evaluation via drop test. J. Intell. Mater. Syst. Struct. 2023, 34, 1471–1485. [Google Scholar] [CrossRef]
- Currey, N.S. Aircraft Landing Gear Design: Principles and Practices; American Institute of Aeronautics and Astronautics: Washington, DC, USA, 1988. [Google Scholar]
- Lernbeiss, R.; Ploechl, M. Simulation model of an aircraft landing gear considering elastic properties of the shock absorber. Proc. Inst. Mech. Eng. Part K J. Multi-Body Dyn. 2007, 221, 77–86. [Google Scholar] [CrossRef]
- Zhang, Z.; Wei, X.; Ye, Q. Heading Load Dynamic Simulation of Landing Gear Test. In The Proceedings of the 2018 Asia-Pacific International Symposium on Aerospace Technology (APISAT 2018); Springer: Singapore, 2019; pp. 1469–1476. [Google Scholar]
- Richards, P.W.; Erickson, A. Dynamic Ground Loads Analysis Using Detailed Modeling of Landing Gear and Aircraft Aeroservoelastics. In Proceedings of the AIAA SciTech 2019 Forum, San Diego, CA, USA, 7–11 January 2019. [Google Scholar] [CrossRef]
- McDonald, M.; Richards, P.W.; Walker, M.; Erickson, A.J. Carrier Landing Simulation using Detailed Aircraft and Landing. In Proceedings of the AIAA SciTech 2020 Forum, Orlando, FL, USA, 6–10 January 2020. [Google Scholar] [CrossRef]
- Orlandea, N.V. Multibody systems history of ADAMS. J. Comput. Nonlinear Dyn. 2016, 11, 060301. [Google Scholar] [CrossRef]
- Zhang, L.; Xue, C.J. A Landing Gear Drop Dynamic Simulation based on the LMS. Virtual. Lab. Appl. Mech. Mater. 2011, 55–57, 684–687. [Google Scholar] [CrossRef]
- Hewlett, J. Methods for Real-Time Simulation of Systems of Rigid and Flexible Bodies with Unilateral Contact and Friction; McGill University: Montreal, QC, Canada, 2020. [Google Scholar]
- Kadam, M.; Sathish, S.; Bujurke, A.; Joshi, K.; Gopalsamy, B. Dynamics of Articulated Landing Gear in Tail-Down Landing Condition. In Machines, Mechanism and Robotics: Proceedings of iNaCoMM 2017; Springer: Singapore, 2019; pp. 195–206. [Google Scholar]
- Huang, M. Research on loads applied on powered wheels and struts of civil aircraft at touchdown. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2022, 236, 840–851. [Google Scholar] [CrossRef]
- Stachiw, T.; Khouli, F.; Langlois, R.G.; Afagh, F.F. Landing gear mechanical network synthesis for improving comfort at landing considering aircraft flexibility. J. Aircr. 2021, 58, 1242–1253. [Google Scholar] [CrossRef]
- Zhang, M.; Wei, X.; Yang, Z.; Shi, X. Six-wheel trolley type landing gear ground load analysis study. Xibei Gongye Daxue Xuebao/J. Northwestern Polytech. Univ. 2022, 40, 1090–1099. [Google Scholar] [CrossRef]
- Baskaran, S.; Sivaprakasam, S. Friction analysis of aircraft landing gears due to landing impact. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2022, 236, 274–283. [Google Scholar] [CrossRef]
- Gao, Z. Aircraft Design Manual: Volume 14, Take-Off and Landing System Design; Aviation Industry Press: Beijing, China, 2001. [Google Scholar]
- Pecora, R. A Rational Numerical Method for Simulation of Drop-Impact Dynamics of Oleo-Pneumatic Landing Gear. Appl. Sci. 2021, 11, 4136. [Google Scholar] [CrossRef]




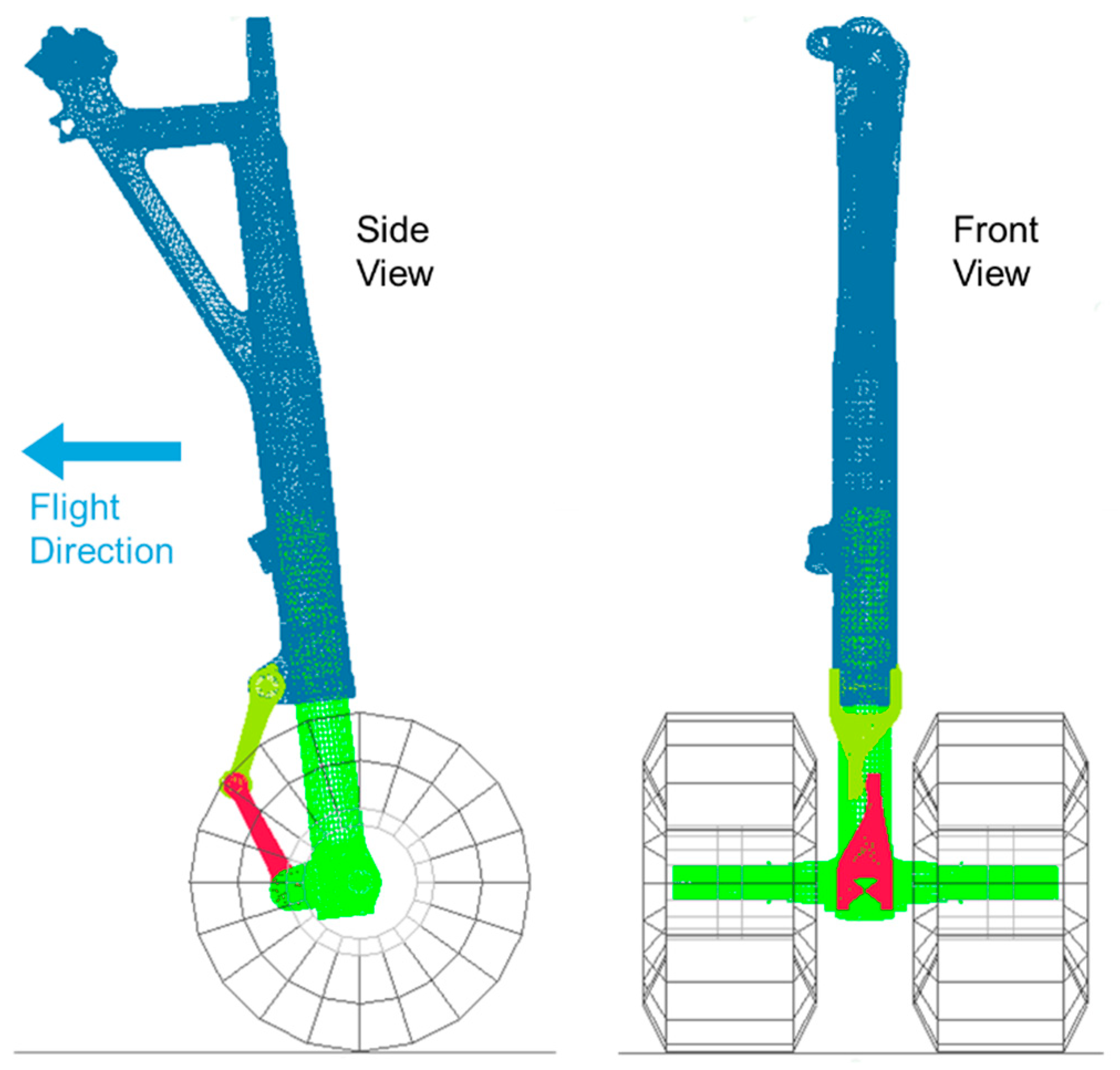
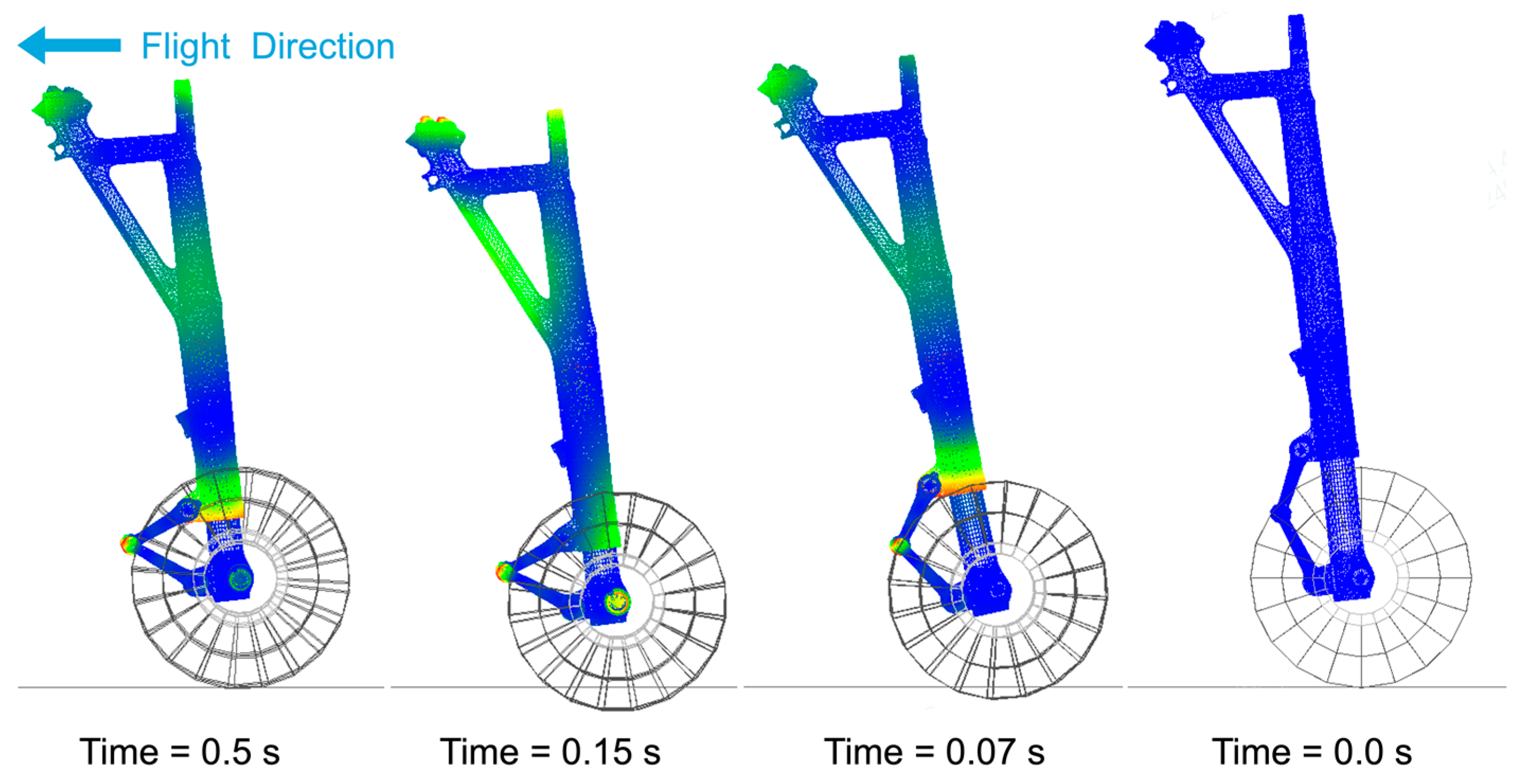
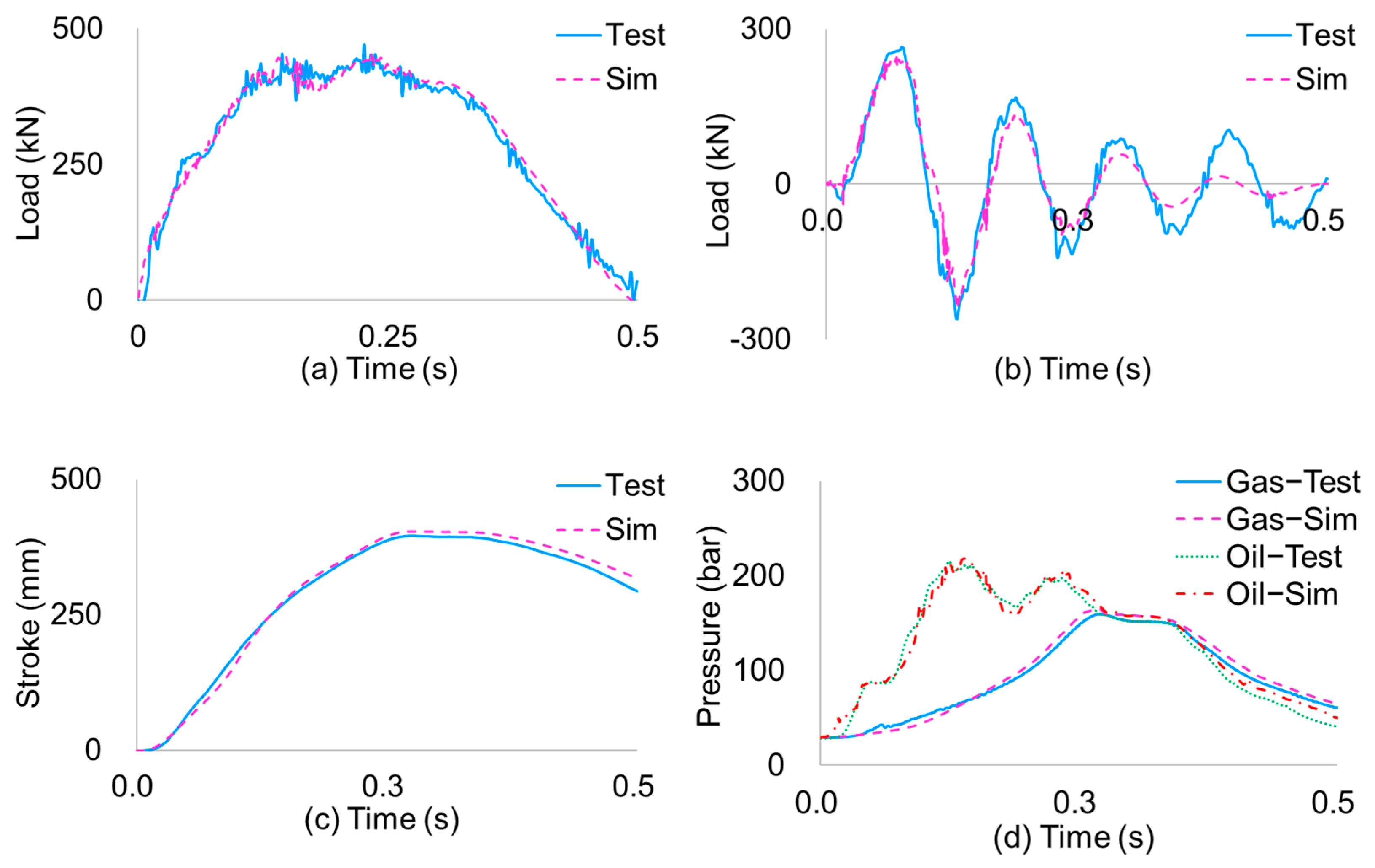


| Material Name | Elastic Modus (GPa) | Poisson’s Ratio (Dimensionless) | Density (kg/m3) |
|---|---|---|---|
| 300M Steel | 205 | 0.27 | 7850 |
| Aluminum Alloy | 70 | 0.33 | 2810 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, W.; Wang, Y. Improved Multi-Body Dynamic Simulation of Landing Gear Drop Test Incorporating Structural Flexibility and Bearing Contact. Aerospace 2024, 11, 543. https://doi.org/10.3390/aerospace11070543
Liu W, Wang Y. Improved Multi-Body Dynamic Simulation of Landing Gear Drop Test Incorporating Structural Flexibility and Bearing Contact. Aerospace. 2024; 11(7):543. https://doi.org/10.3390/aerospace11070543
Chicago/Turabian StyleLiu, Wenbin, and Youshan Wang. 2024. "Improved Multi-Body Dynamic Simulation of Landing Gear Drop Test Incorporating Structural Flexibility and Bearing Contact" Aerospace 11, no. 7: 543. https://doi.org/10.3390/aerospace11070543
APA StyleLiu, W., & Wang, Y. (2024). Improved Multi-Body Dynamic Simulation of Landing Gear Drop Test Incorporating Structural Flexibility and Bearing Contact. Aerospace, 11(7), 543. https://doi.org/10.3390/aerospace11070543






