Numerical and Experimental Analyses of the Effect of Water Injection on Combustion of Mg-Based Hydroreactive Fuels
Abstract
1. Introduction
2. Experimental Methods
2.1. Experimental Sample
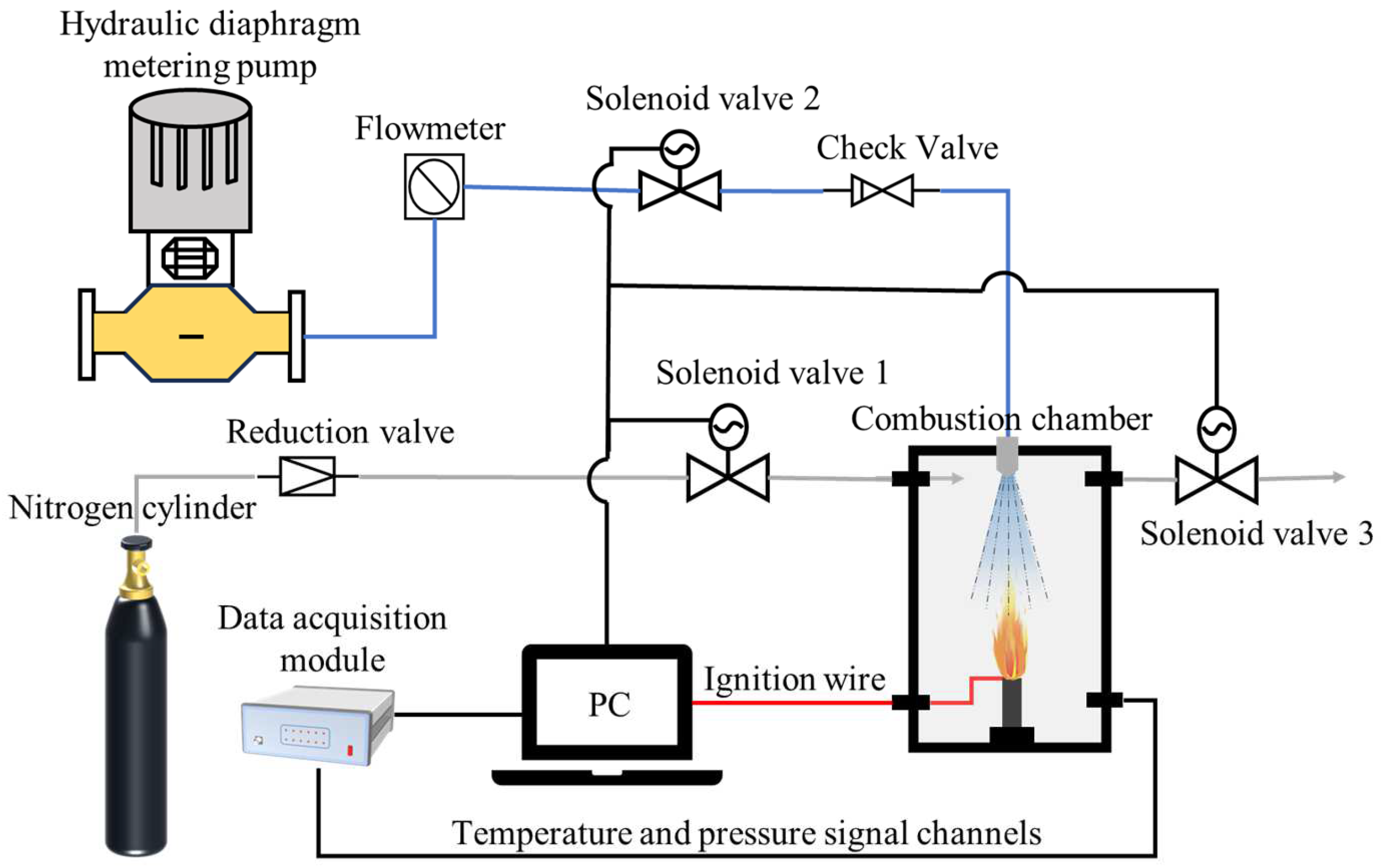
2.2. Experimental Setup
3. Numerical Simulation
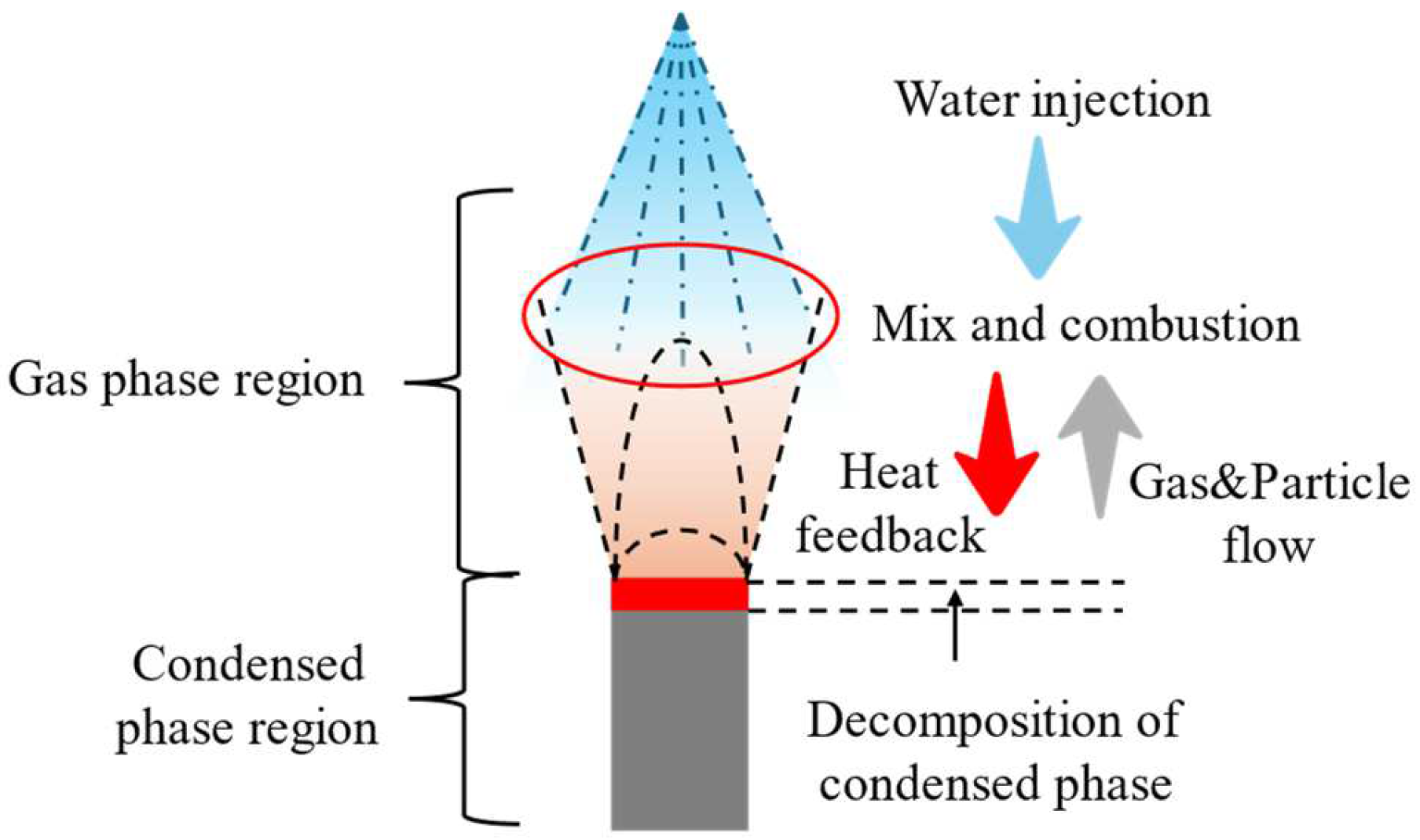
3.1. Physical Model
- (1)
- The oxidation of the Mg particles occurring at the burning surface was ignored.
- (2)
- The products in the gas phase were considered ideal gases.
- (3)
- The reaction of Mg with the water in the gas phase was homogeneous.
- (4)
- The reaction rate obeyed the Arrhenius Law.
3.2. Mathematical Model
3.2.1. Governing Equations for the Continuous Phase
3.2.2. Governing Equations for Discrete Particles
3.2.3. Combustion Kinetic Model
3.2.4. Coupling between the Gas and Condensed Phases
3.2.5. Turbulent Governing Equation
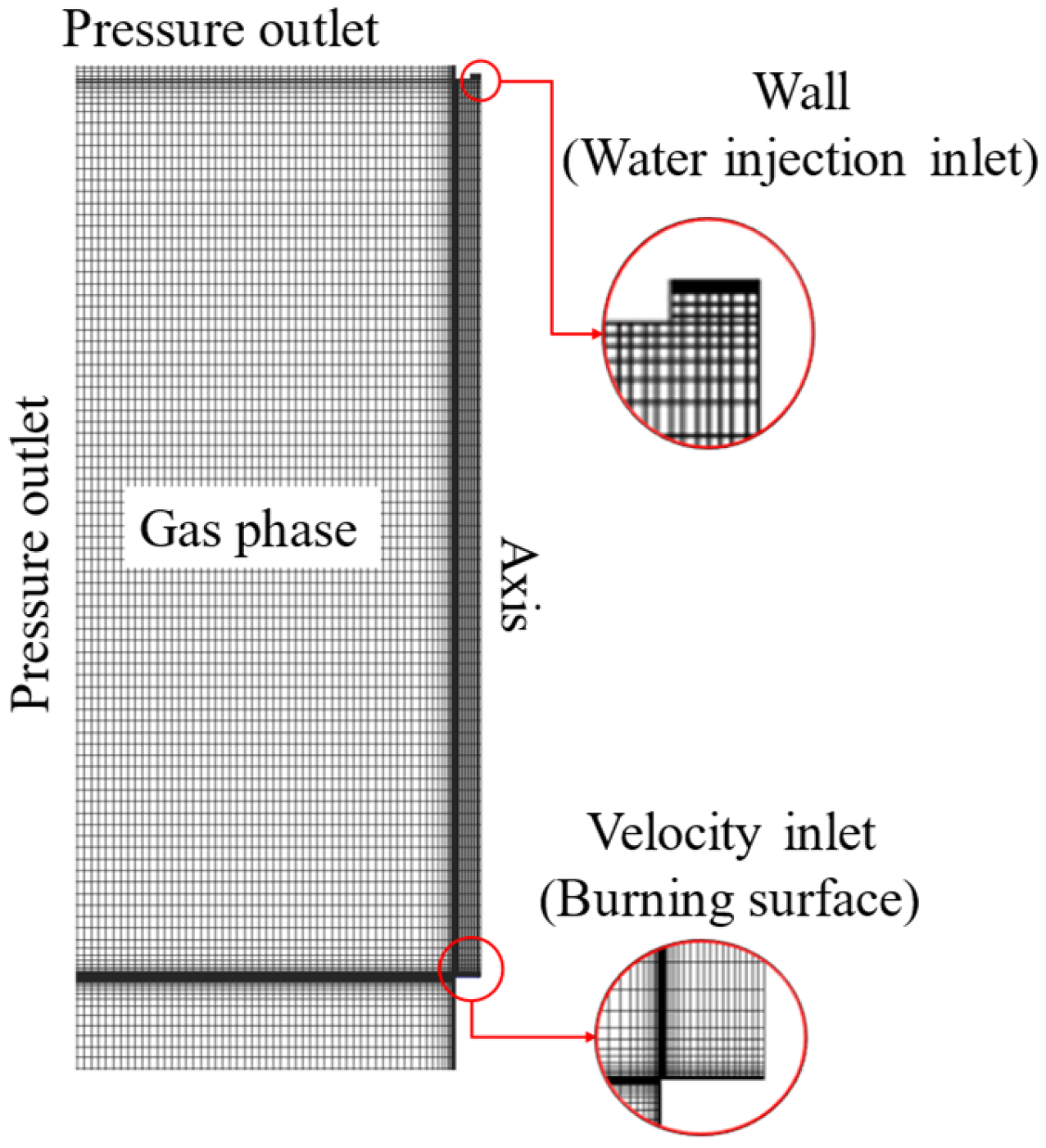
3.2.6. Computational Domain and Boundary Conditions
4. Results and Discussion
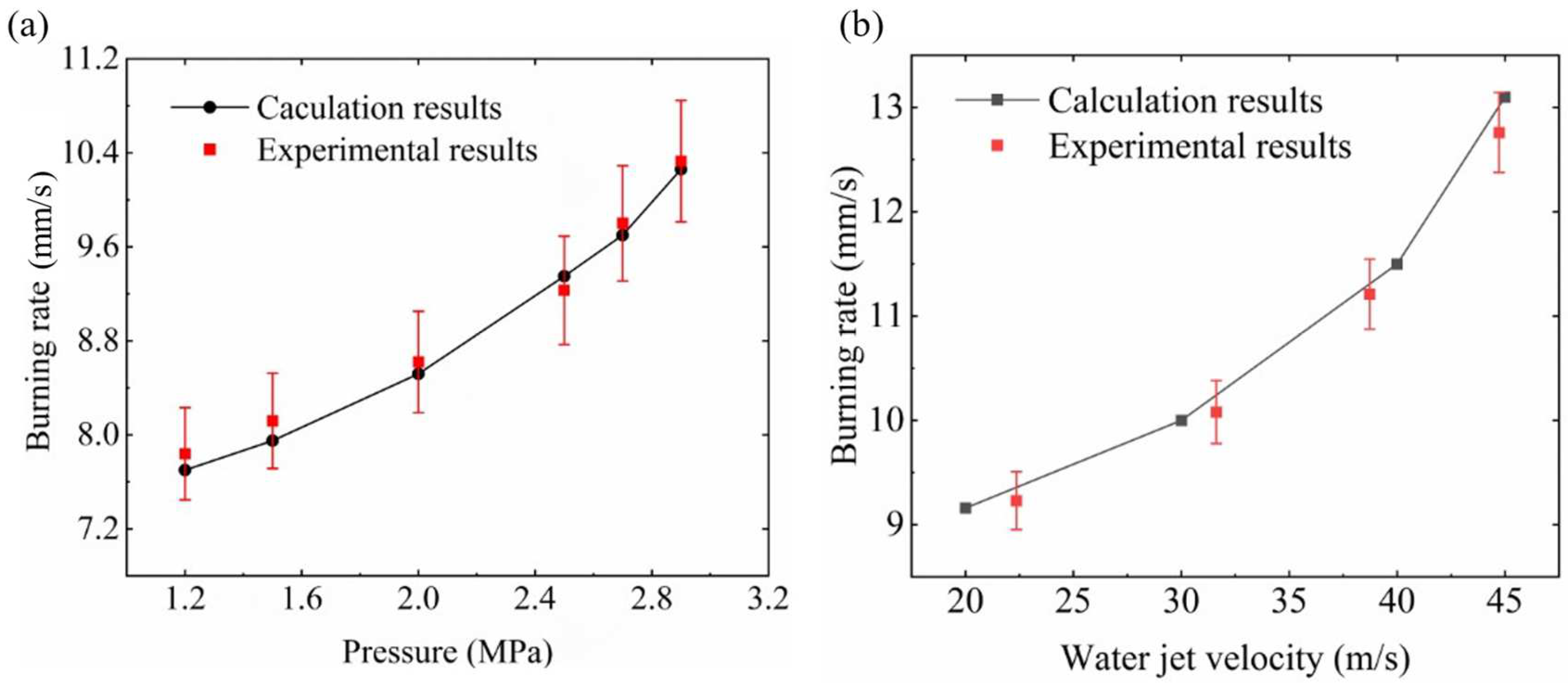
4.1. Model Verification and Validation
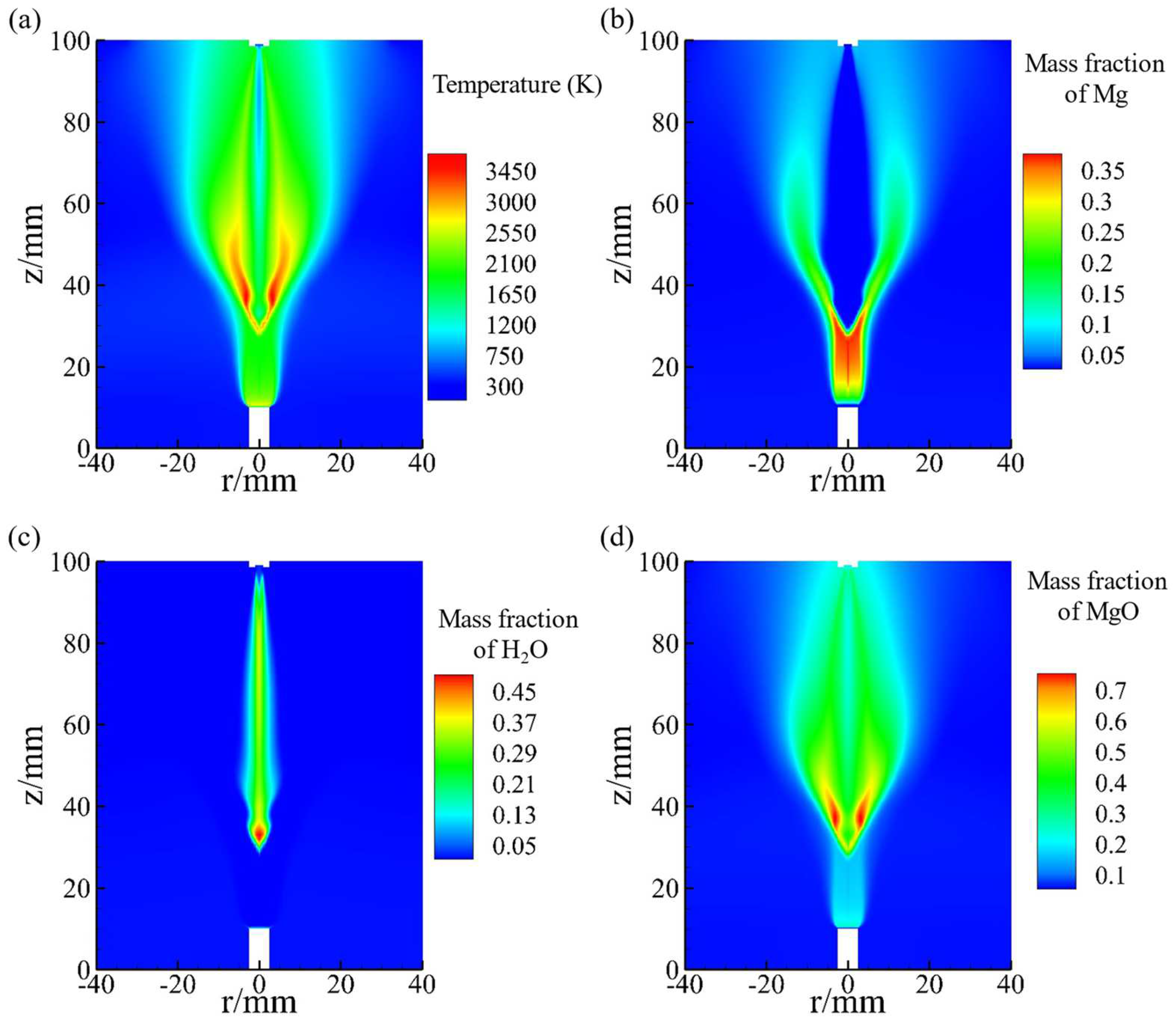
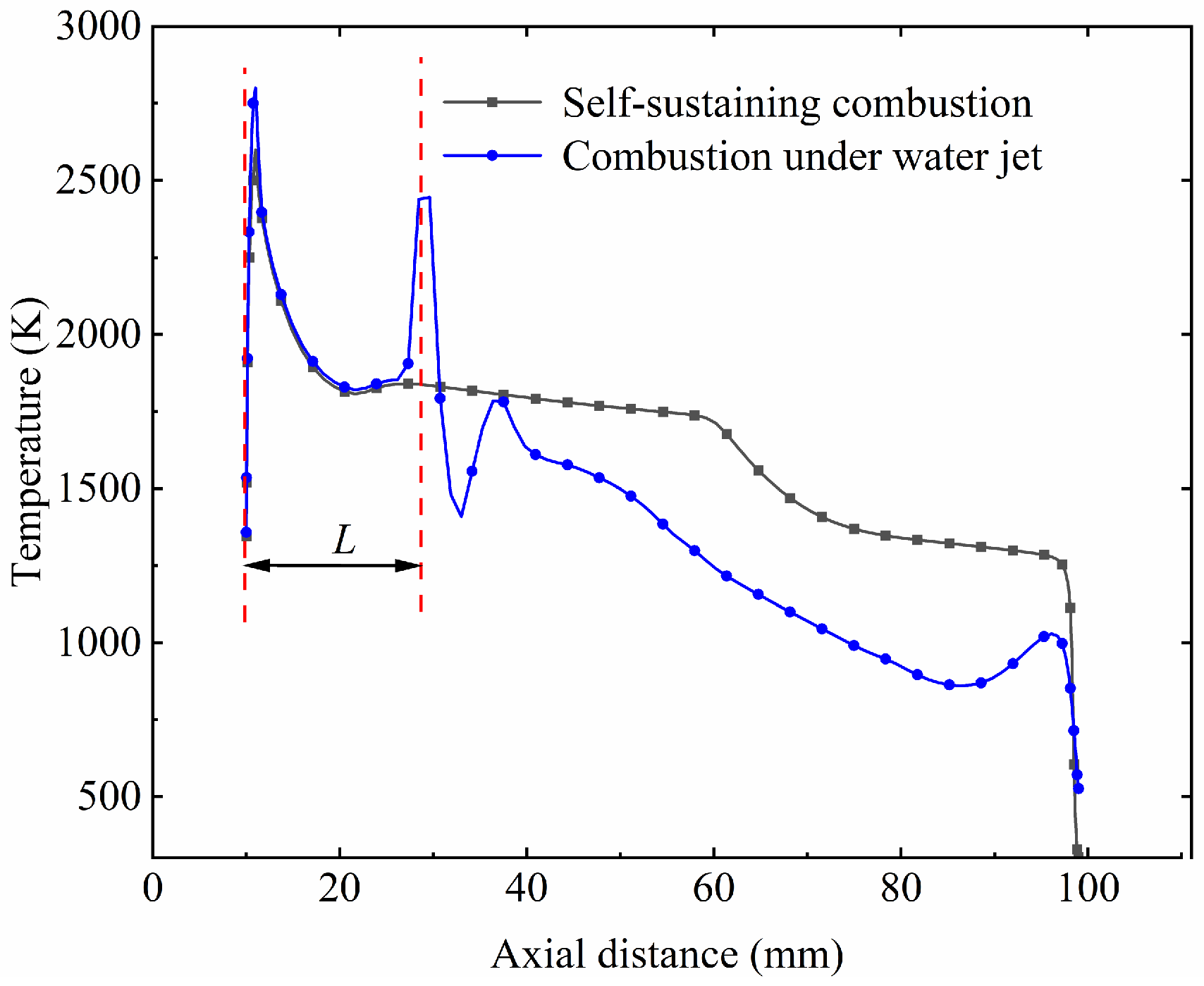
4.2. Flame Structure and Temperature
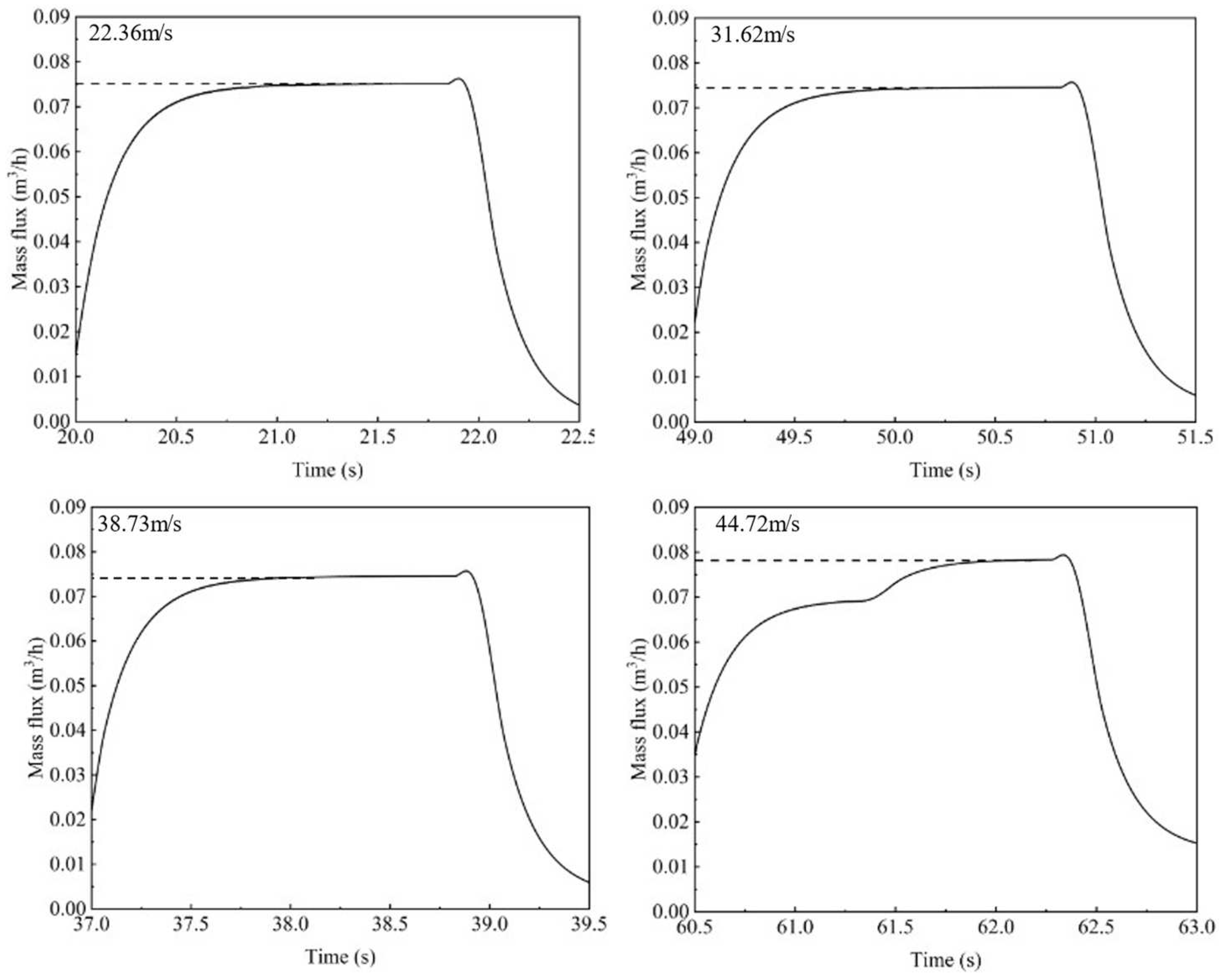
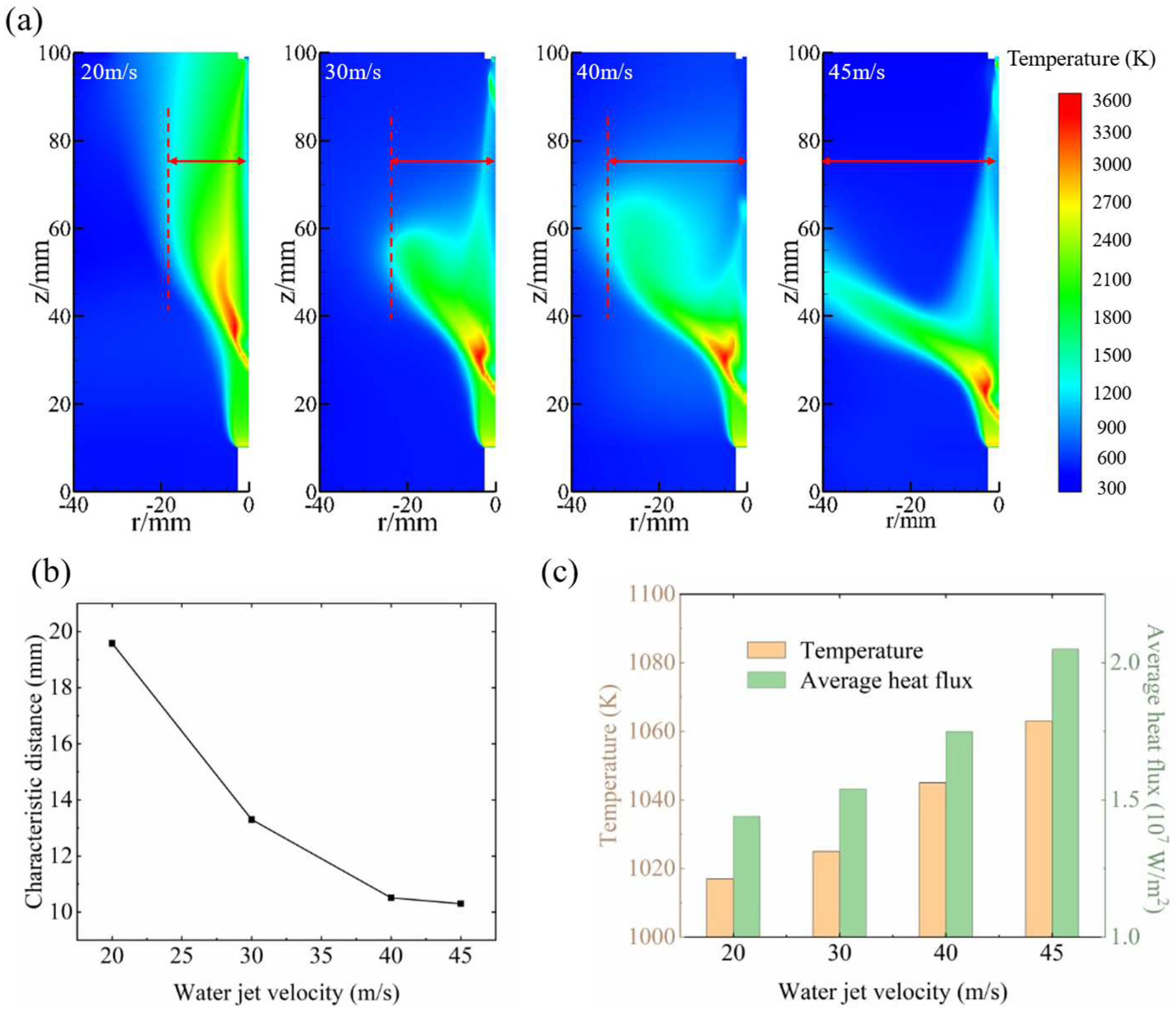
4.3. Effect of the Water Jet Velocity
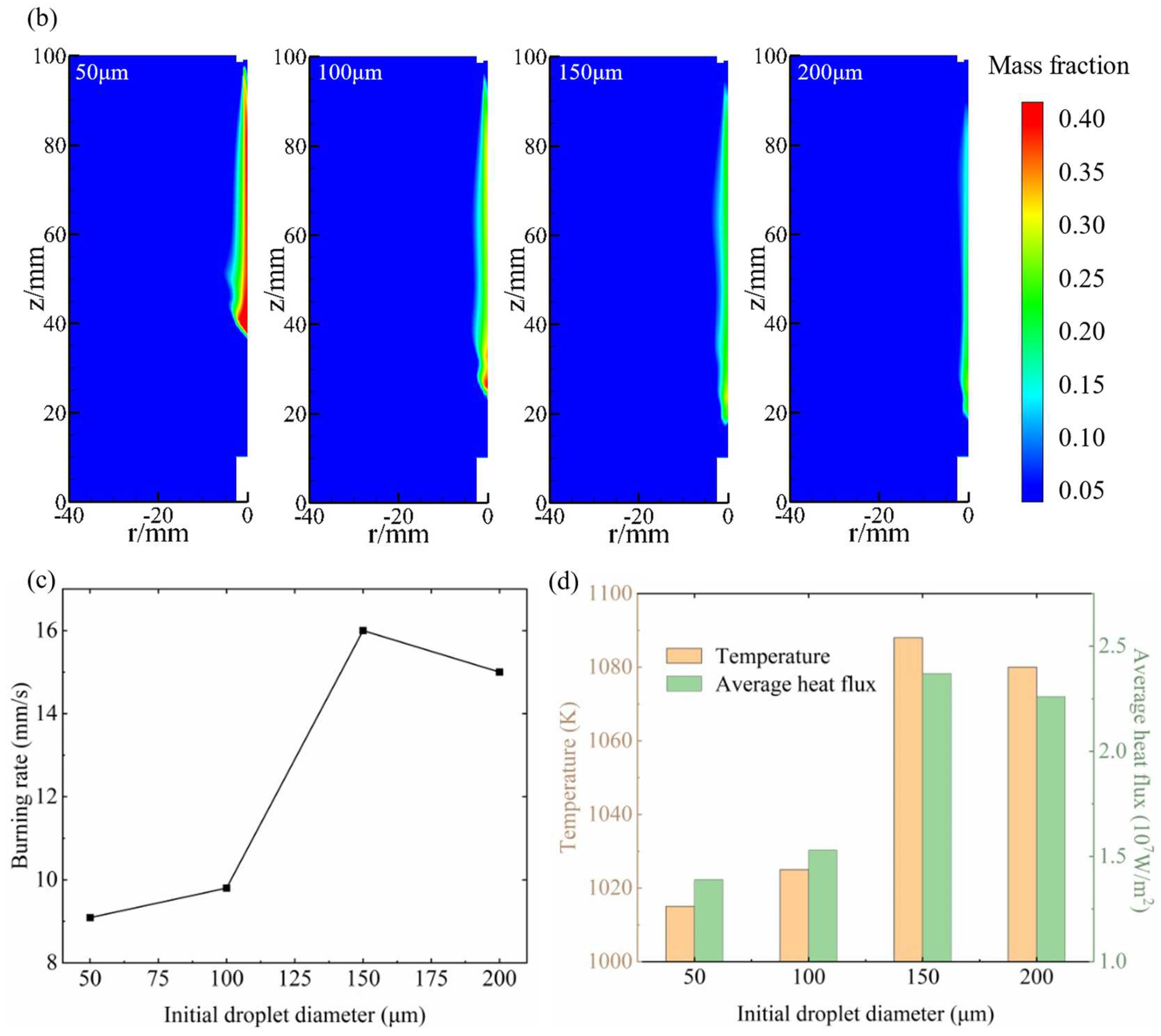
4.4. Effect of Water Droplet Particles
5. Conclusions
- (1)
- Compared to the self-sustained combustion, the addition of water injection formed a new Mg/H2O non-premixed combustion region in the gas phase.
- (2)
- Additionally, the initial momentum of the droplet affects the mixing degree of the water injection and the fuel-rich gas, which is related to the initial velocity and the initial droplet size (mass). The higher the velocity of the water injection, the stronger the penetration ability of the water droplets into the fuel-rich gas, bringing the Mg/H2O non-premixed combustion region towards the burning surface, which increased the heat flux, resulting in a positive correlation between the fuel burning rate and injection velocity.
- (3)
- To a certain extent, the correlation between the droplet diameter and burning rate also followed this pattern; however, an excessive droplet diameter may cause incomplete evaporation, leading to a minor reduction in the burning rate.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Boryaev, A.A. Calculation and experimental estimation of the efficiency of using lithium, sodium, magnesium, and aluminum as fuels in hydro-reactive propellants. Therm. Sci. Eng. Prog. 2021, 23, 100881. [Google Scholar] [CrossRef]
- Zhang, J.; Xia, Z.; Huang, L.; Ma, L. Power cycle analysis of two-phase underwater ramjet. Appl. Ocean Res. 2018, 71, 69–76. [Google Scholar] [CrossRef]
- Shi, H.; Ren, J.; Tang, W.; Li, J.; Yang, R. Effect of fast-burning compound ACP and catalyst on the combustion performance of Al/Mg-based fuel-rich HTPB propellants. FirePhysChem 2022, 2, 28–35. [Google Scholar] [CrossRef]
- Ao, W.; Zhang, Y.; Liu, L.; Huo, C.; Liu, P.; Li, L.K.B. Ignition and combustion experiments on Mg/AP composite fuels in different reaction environments. Combust. Flame 2023, 247, 112505. [Google Scholar] [CrossRef]
- Ghassemi, H.; Farshi Fasih, H. Investigation of interior ballistics and performance analysis of hydro-reactive motors. Aerosp. Sci. Technol. 2015, 41, 99–105. [Google Scholar] [CrossRef]
- Ghassemi, H.; Farshi Fasih, H. Propulsive characteristics of metal fuel-rich pyrotechnics in hydro-reactive motors. Aerosp. Sci. Technol. 2013, 28, 1–8. [Google Scholar] [CrossRef]
- Zou, M.; Yang, R.; Guo, X.; Huang, H.; He, J.; Zhang, P. The preparation of Mg-based hydro-reactive materials and their reactive properties in seawater. Int. J. Hydrogen Energy 2011, 36, 6478–6483. [Google Scholar] [CrossRef]
- Liu, J.; Wang, Y.; Li, X.; Cong, J. Using the Impulse Method to Determine High-Pressure Dynamic Burning Rate of Solid Propellants. Aerospace 2023, 10, 818. [Google Scholar] [CrossRef]
- Zhang, K.; Han, Y.; Ren, D.; Zhu, C. Experimental and numerical investigations of the effect of pressure on combustion characteristics of Mg-based solid fuels. Fuel 2021, 305, 121529. [Google Scholar] [CrossRef]
- Wang, Z.; Yu, K.; Liu, Y. A Discontinuous Galerkin–Finite Element Method for the Nonlinear Unsteady Burning Rate Responses of Solid Propellants. Aerospace 2024, 11, 97. [Google Scholar] [CrossRef]
- Ao, W.; Wen, Z.; Liu, L.; Liu, P.; Gan, Y.; Wang, L.; Li, L.K.B. Controlling the combustion and agglomeration characteristics of a solid composite propellant via a DC electric field. Aerosp. Sci. Technol. 2022, 128, 107766. [Google Scholar] [CrossRef]
- Hu, J.; Han, C.; Xia, Z.; Huang, L.; Huang, X. Experimental Investigation on Combustion of High-Metal Magnesium-Based Hydroreactive Fuels. J. Propuls. Power 2013, 29, 692–698. [Google Scholar] [CrossRef]
- Huang, H.; Zou, M.; Guo, X.; Yang, R.; Li, Y. Analysis of the aluminum reaction efficiency in a hydro-reactive fuel propellant used for a water ramjet. Combust. Explos. Shock Waves 2013, 49, 541–547. [Google Scholar] [CrossRef]
- Huang, H.; Zou, M.; Guo, X.; Yang, R.; Zhang, P. Analysis of the Solid Combustion Products of a Mg-Based Fuel-Rich Propellant used for Water Ramjet Engines. Propellants Explos. Pyrotech. 2012, 37, 407–412. [Google Scholar] [CrossRef]
- Huang, H.; Zou, M.; Guo, X.; Yang, R.; Li, Y.; Jiang, E.; Li, Z. Study of Different Al/Mg Powders in Hydroreactive Fuel Propellant Used for Water Ramjet. J. Energetic Mater. 2014, 32, S83–S93. [Google Scholar] [CrossRef]
- Huang, L.; Zhang, W.; Xia, Z.; Hu, J.X. Experimental Study on Ignition Process of a Magnesium-Based Water Ramjet Engine. J. Propuls. Power 2014, 30, 857–862. [Google Scholar] [CrossRef]
- Huang, L.; Xia, Z.; Hu, J.; Zhu, Q. Performance study of a water ramjet engine. Sci. China Technol. Sci. 2011, 54, 877–882. [Google Scholar] [CrossRef]
- Sun, D.; Li, Y.; Liu, P.; Chen, B.; Fan, W. Numerical Investigation on the Effect of Ammonium Perchlorate Content and Position on the Combustion Characteristics of an Ammonium Perchlorate/Hydroxyl-Terminated Polybutadiene Propellant. Aerospace 2023, 10, 692. [Google Scholar] [CrossRef]
- Yue, S.; Liu, L.; Liu, H.; Jiang, Y.; Liu, P.; Pang, A.; Zhang, G.; Ao, W. Agglomerate Size Evolution in Solid Propellant Combustion under High Pressure. Aerospace 2023, 10, 515. [Google Scholar] [CrossRef]
- Martinez-Sanchis, D.; Sternin, A.; Haidn, O.; Tajmar, M. Combustion Regimes in Turbulent Non-Premixed Flames for Space Propulsion. Aerospace 2023, 10, 671. [Google Scholar] [CrossRef]
- Hu, J.; Han, C.; Xia, Z.; Huang, L.; Huang, X. Statistical model for combustion of high-metal magnesium-based hydro-reactive fuel. Chin. Phys. B 2012, 21, 124501. [Google Scholar] [CrossRef]
- Beckstead, M.W.; Derr, R.L.; Price, C.F. A model of composite solid-propellant combustion based on multiple flames. AIAA J. 1970, 8, 2200–2207. [Google Scholar] [CrossRef]
- Gross, M.L.; Hedman, T.D.; Son, S.F.; Jackson, T.L.; Beckstead, M.W. Coupling micro and meso-scale combustion models of AP/HTPB propellants. Combust. Flame 2013, 160, 982–992. [Google Scholar] [CrossRef]
- Gross, M.L.; Beckstead, M.W. Diffusion flame calculations for composite propellants predicting particle-size effects. Combust. Flame 2010, 157, 864–873. [Google Scholar] [CrossRef]
- Choi, H.; Yoh, J.J. A grain-scale modeling on the surface burning and agglomeration of the metalized solid propellants. Combust. Flame 2024, 261, 113284. [Google Scholar] [CrossRef]
- Zhu, M.; Chen, X.; Zhou, C.; Xu, J.; Musa, O.; Xiang, H. Experimental and numerical investigations on the decomposition and combustion characteristics of composite propellant with Mg/Al particles additives. Appl. Therm. Eng. 2017, 111, 863–875. [Google Scholar] [CrossRef]
- Zhang, K.; Han, Y.; Ren, D.; Zhu, C. Experimental and numerical analyses of the combustion characteristics of Mg/PTFE/Viton fuel-rich pyrolants in the atmospheric environment. Numer. Heat Transf. Part A Appl. 2020, 78, 180–198. [Google Scholar] [CrossRef]
- Zhang, K.; Han, Y.; Ren, D.; Zhu, C. Experimental and numerical investigations on the condensed combustion products of Mg-based solid fuels. Fuel 2022, 329, 125423. [Google Scholar] [CrossRef]
- Zou, X.; Wang, N.; Wang, C.; Wang, J.; Tang, Y.; Shi, B. Investigation on the microscale combustion characteristics of AP/HTPB propellant under wide pressure range. Fuel 2021, 306, 121652. [Google Scholar] [CrossRef]
- Morsi, S.A.; Alexander, A.J. An investigation of particle trajectories in two-phase flow systems. J. Fluid Mech. 1972, 55, 193–208. [Google Scholar] [CrossRef]
- Ramakrishna, P.A.; Paul, P.J.; Mukunda, H.S. Sandwich propellant combustion: Modeling and experimental comparison. Proc. Combust. Inst. 2002, 29, 2963–2973. [Google Scholar] [CrossRef]
- Liu, L.; Zhang, G.; Wen, Z.; Li, S.; Liu, P.; He, G.; Ao, W. Numerical investigation and experimental validation of aluminized propellant combustion under high pressures: Critical effects of heat feedback. Aerosp. Sci. Technol. 2024, 147, 109032. [Google Scholar] [CrossRef]
- Yang, W.; Han, Z.; Zhou, J.; Liu, J.; Cen, K. Theoretical study on the reaction of magnesium with water in the gas-phase. Int. J. Hydrogen Energy 2011, 36, 10608–10613. [Google Scholar] [CrossRef]
- Analytis, G.T. Implementation of the renormalization group (RNG) k–ε turbulence model in GOTHIC/6.lb: Solution methods and assessment. Ann. Nucl. Energy 2003, 30, 349–387. [Google Scholar] [CrossRef]

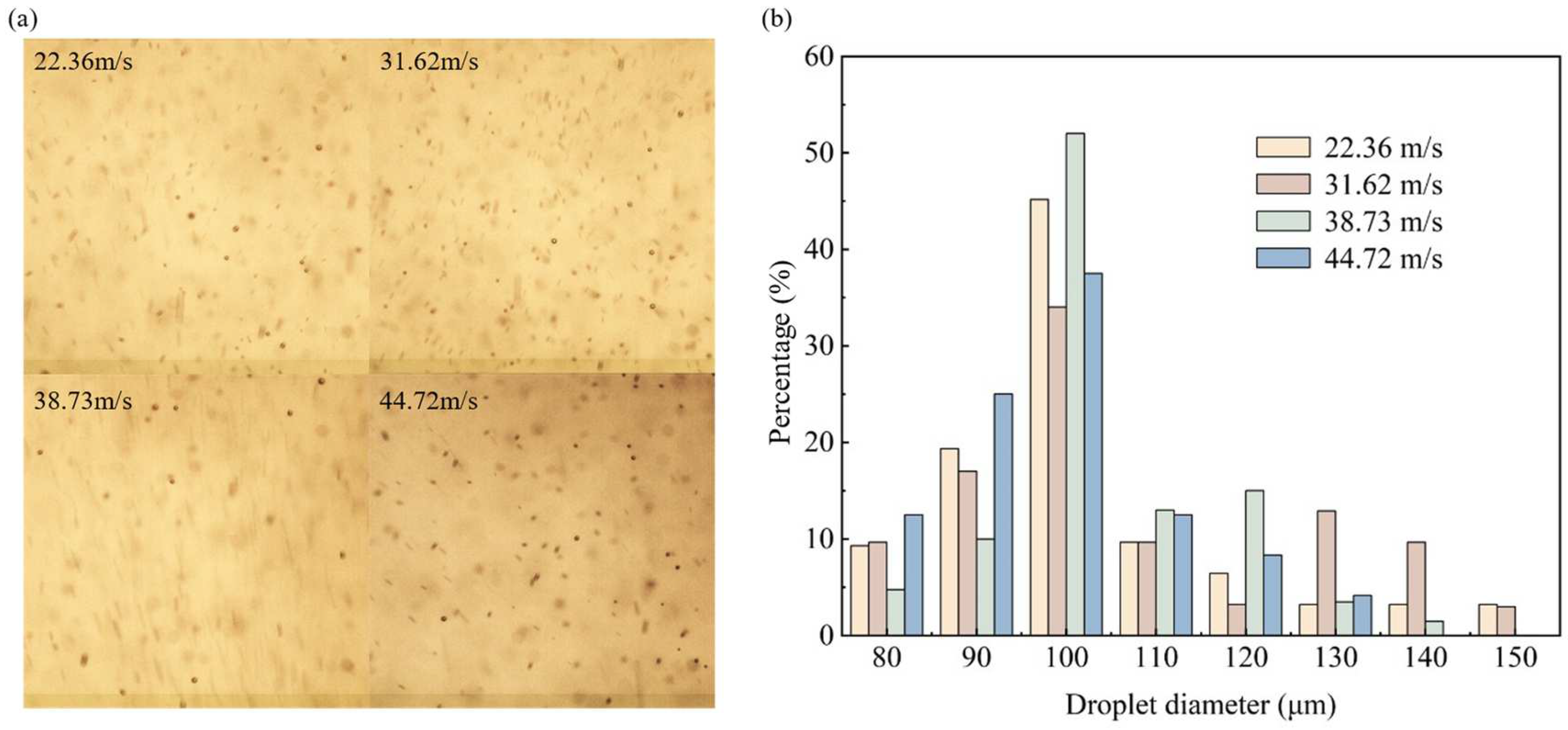


| Name | Branding | Model | Measurement Range | Accuracy |
|---|---|---|---|---|
| Pressure sensors | Xing Hang | PTA-10G21G102 | 0–10 MPa | 0.25% F.S |
| Flowmeter | Xi Chuang | LWG-Y-4 | 0–0.25 m3/h | Repeatability: ≤0.2% |
| Thermocouple | Cheng Lin | TT-K-30 | 73–1673 K | ±2.5 K |
| Data Acquisition Module | DeweSoft | SIRIUS® XHS | - | - |
| Data Acquisition Software | DeweSoft | DeweSoftX-64bit | - | - |
| Pressure (MPa) | 1.2 | 1.5 | 2.0 | 2.5 | 2.7 | 2.9 |
| Burning rate (mm/s) | 7.84 | 8.12 | 8.62 | 9.23 | 9.80 | 10.33 |
| Water injection velocity (m/s) | 22.36 | 31.62 | 38.73 | 44.72 |
| Burning rate (mm/s) | 7.84 | 8.12 | 8.62 | 9.23 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shao, S.; Yue, S.; Qiao, H.; Liu, P.; Ao, W. Numerical and Experimental Analyses of the Effect of Water Injection on Combustion of Mg-Based Hydroreactive Fuels. Aerospace 2024, 11, 542. https://doi.org/10.3390/aerospace11070542
Shao S, Yue S, Qiao H, Liu P, Ao W. Numerical and Experimental Analyses of the Effect of Water Injection on Combustion of Mg-Based Hydroreactive Fuels. Aerospace. 2024; 11(7):542. https://doi.org/10.3390/aerospace11070542
Chicago/Turabian StyleShao, Shiyao, Songchen Yue, Hong Qiao, Peijin Liu, and Wen Ao. 2024. "Numerical and Experimental Analyses of the Effect of Water Injection on Combustion of Mg-Based Hydroreactive Fuels" Aerospace 11, no. 7: 542. https://doi.org/10.3390/aerospace11070542
APA StyleShao, S., Yue, S., Qiao, H., Liu, P., & Ao, W. (2024). Numerical and Experimental Analyses of the Effect of Water Injection on Combustion of Mg-Based Hydroreactive Fuels. Aerospace, 11(7), 542. https://doi.org/10.3390/aerospace11070542







