RANS-Based Aerodynamic Shape Optimization of a Wing with a Propeller in Front of the Wingtip
Abstract
1. Introduction
2. Computational Tools
2.1. Flow Solver
2.2. Geometry Parameterization
2.3. Mesh Movement
2.4. Optimizer
2.5. Propeller Model
3. Validation Cases
3.1. Geometry and Specifications
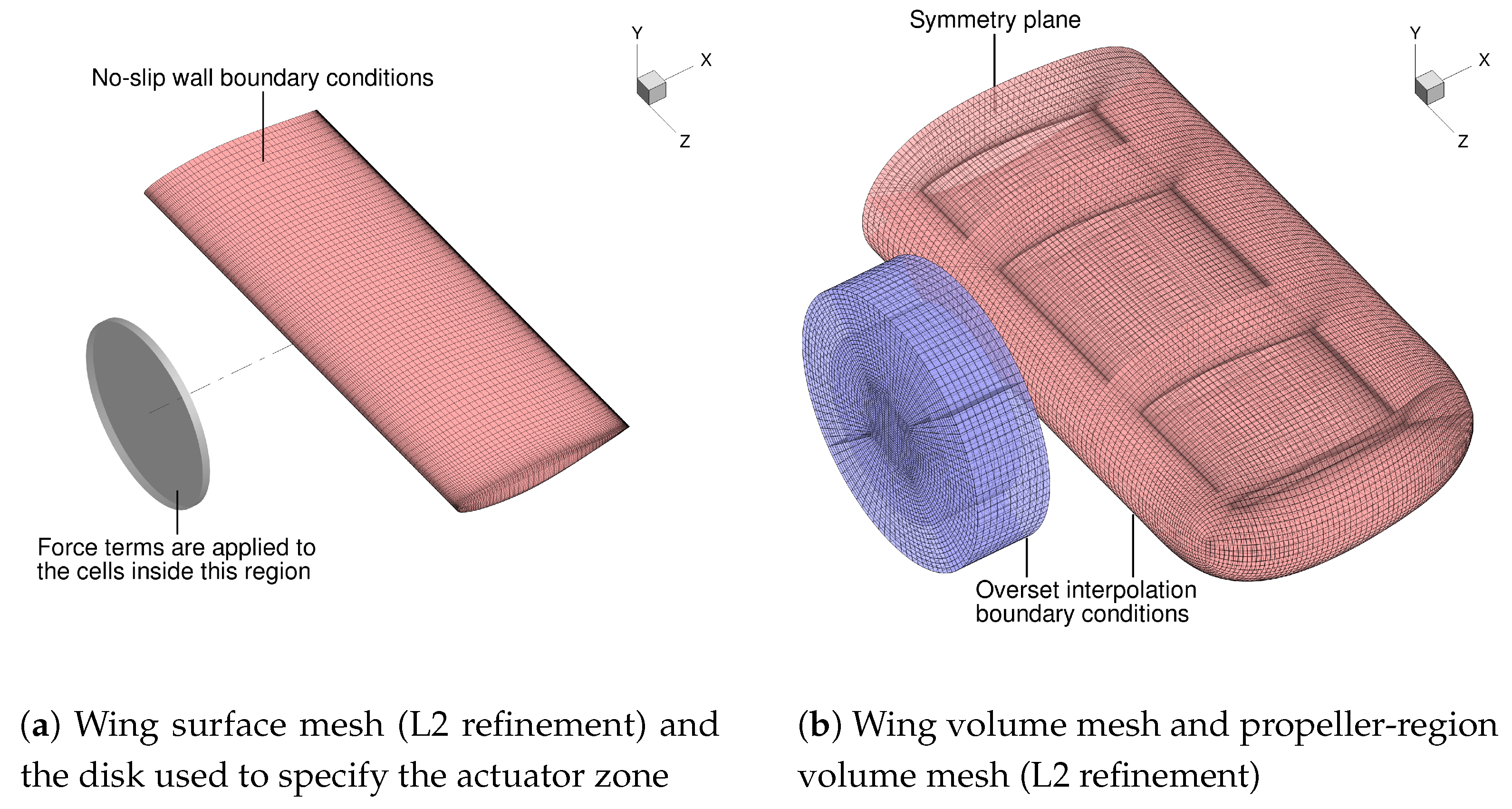
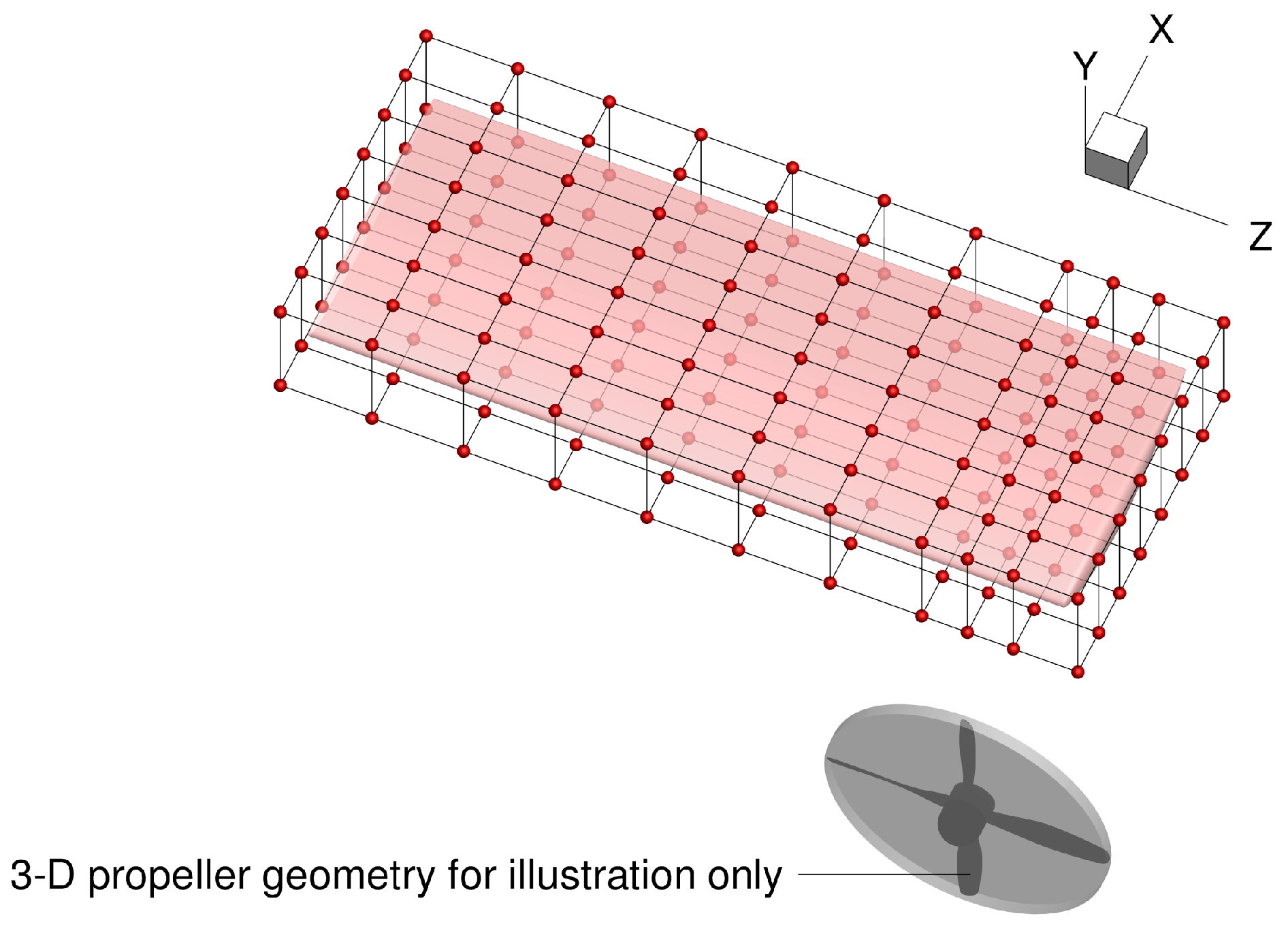
3.2. CFD Volume Meshes
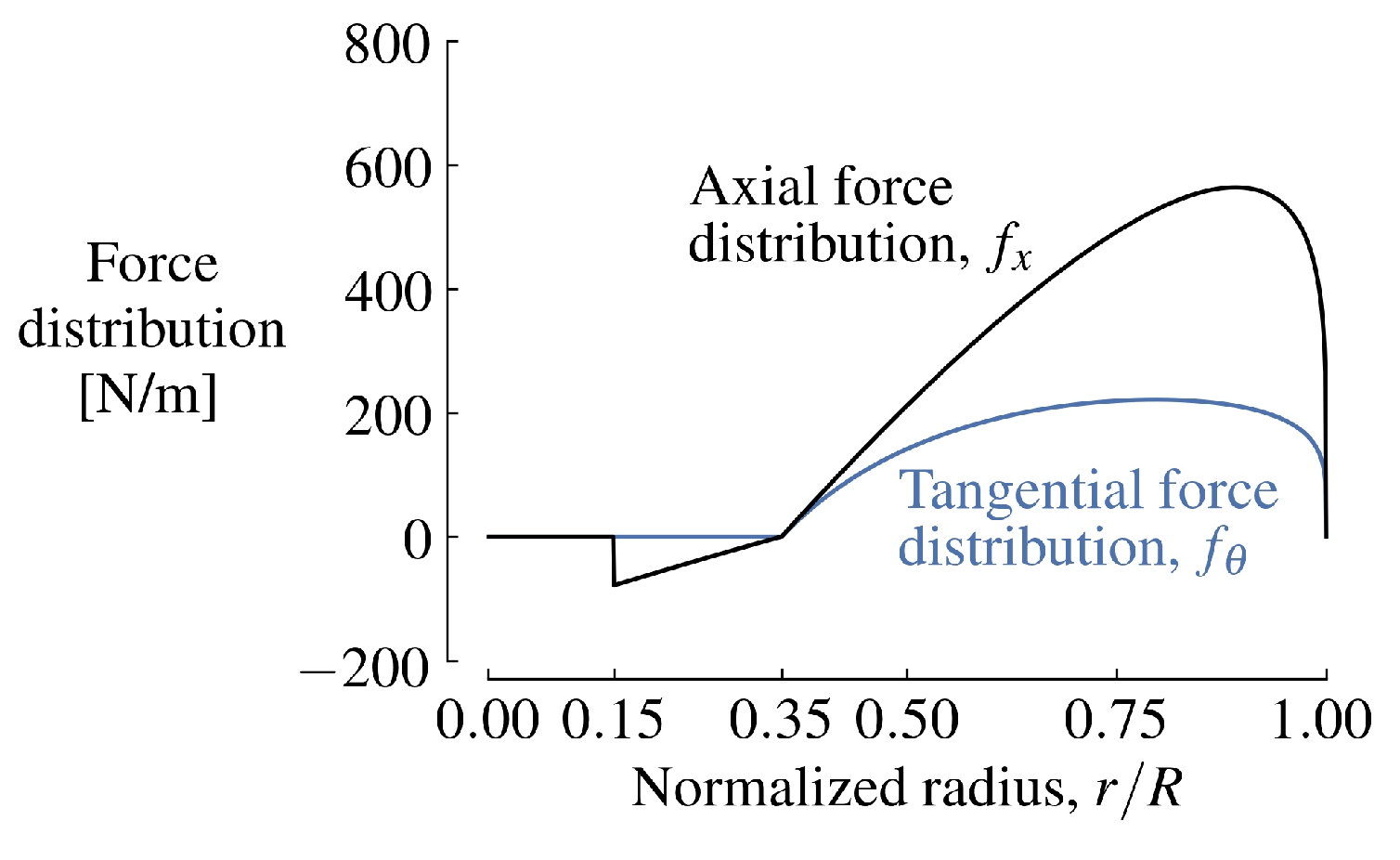
3.3. Propeller Model Inputs
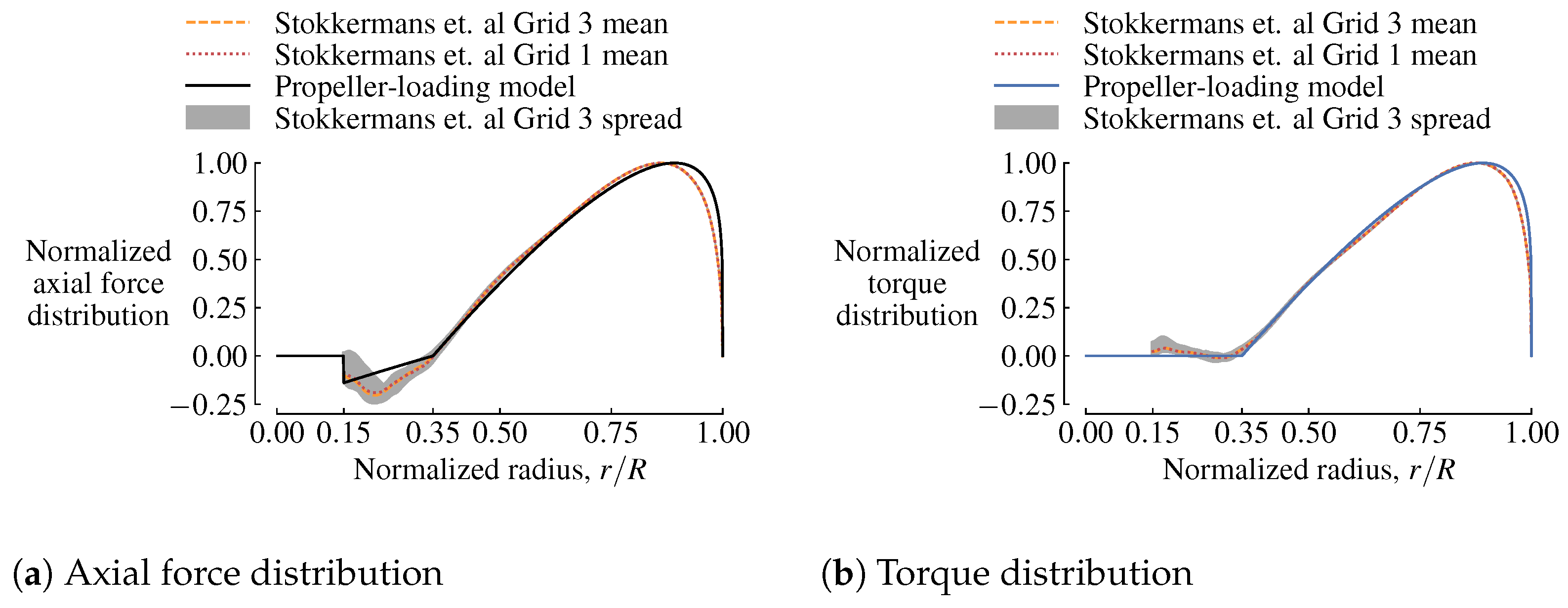
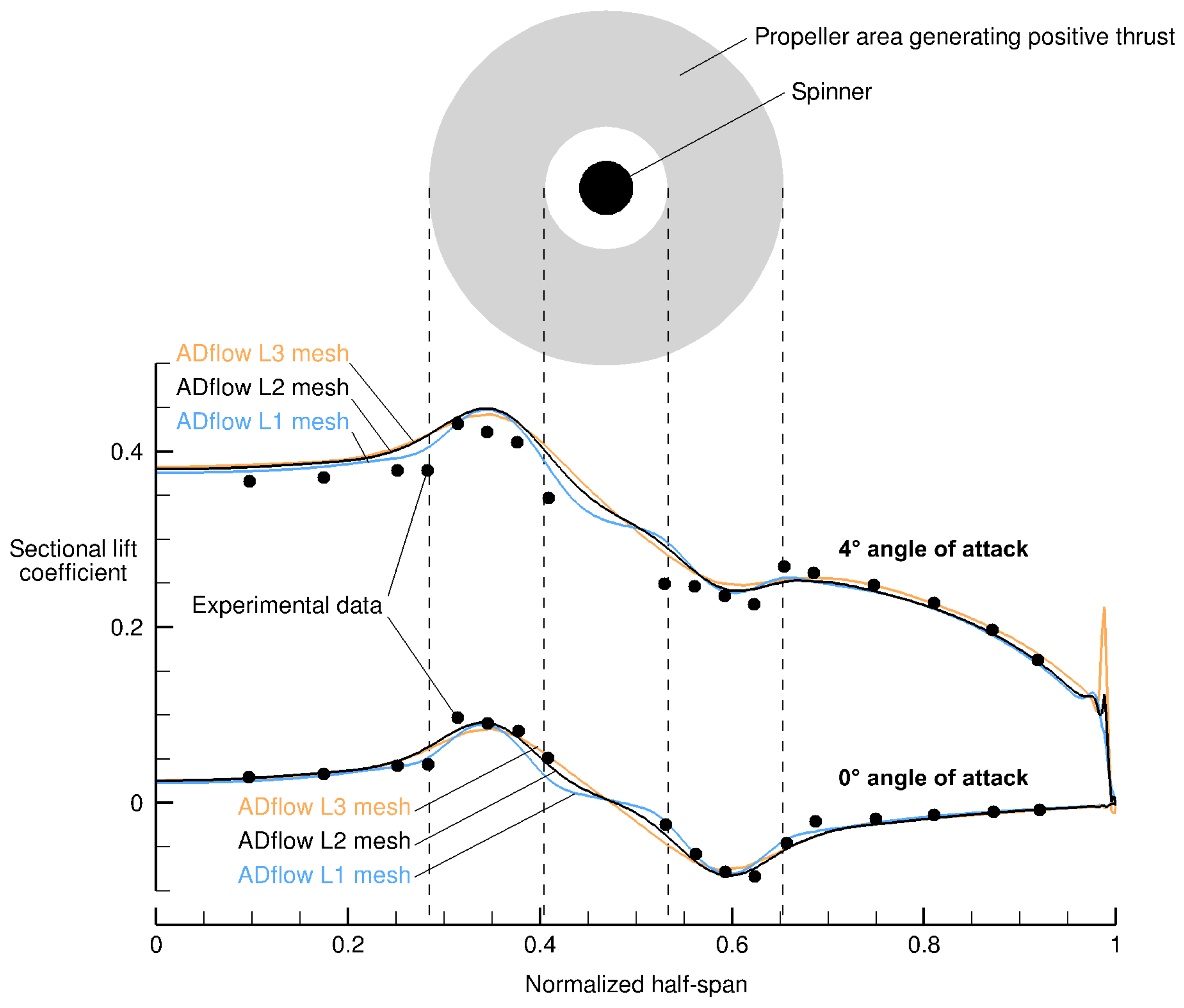
3.4. Validation Results
4. Optimization Problem Descriptions
4.1. Geometry and Parameterization
4.2. Flight Conditions
4.3. Optimization Problem Formulations
4.4. Baseline Optimization Cases for Comparison
5. Optimization Results
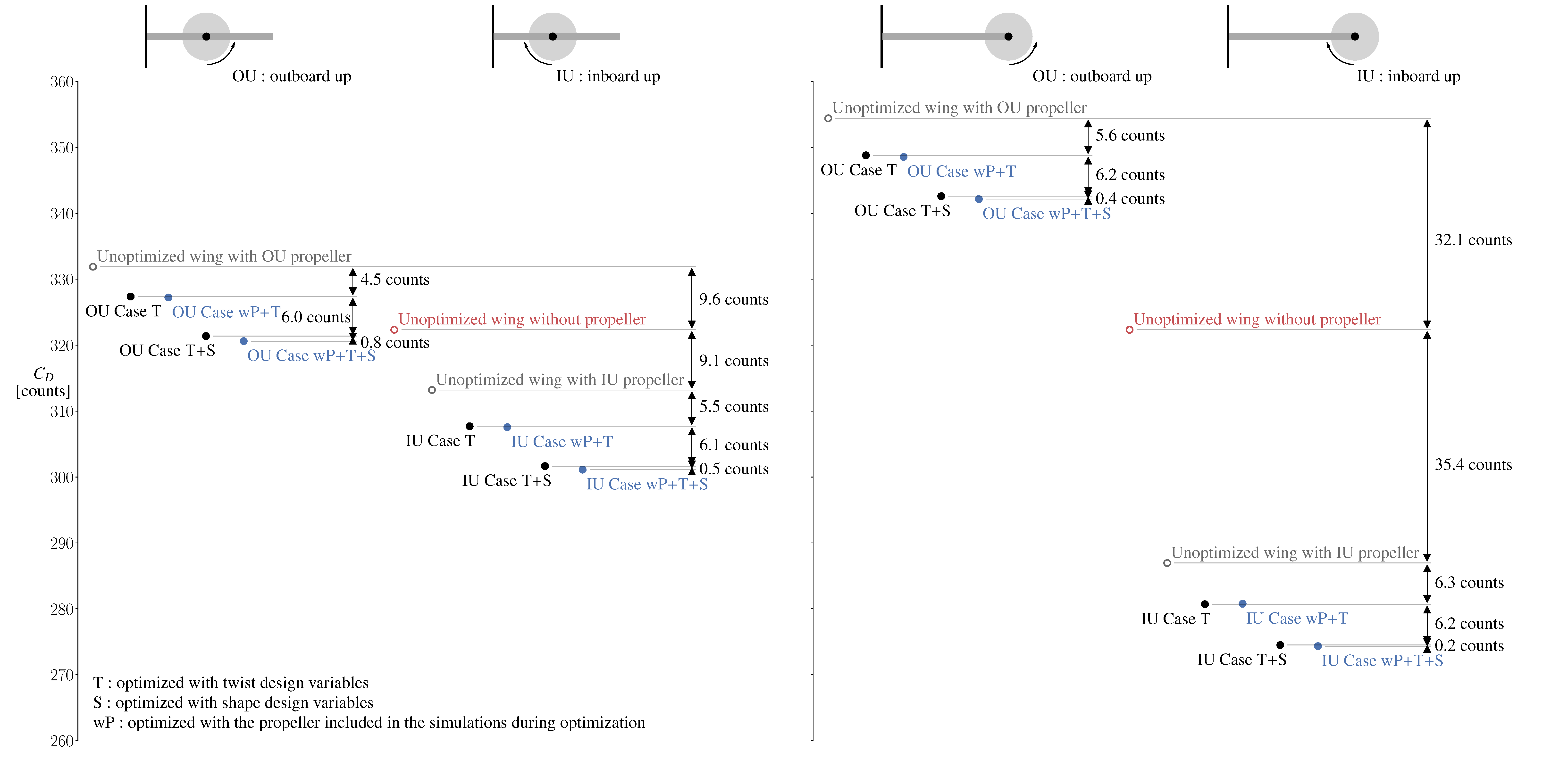
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Mesh Refinement Study
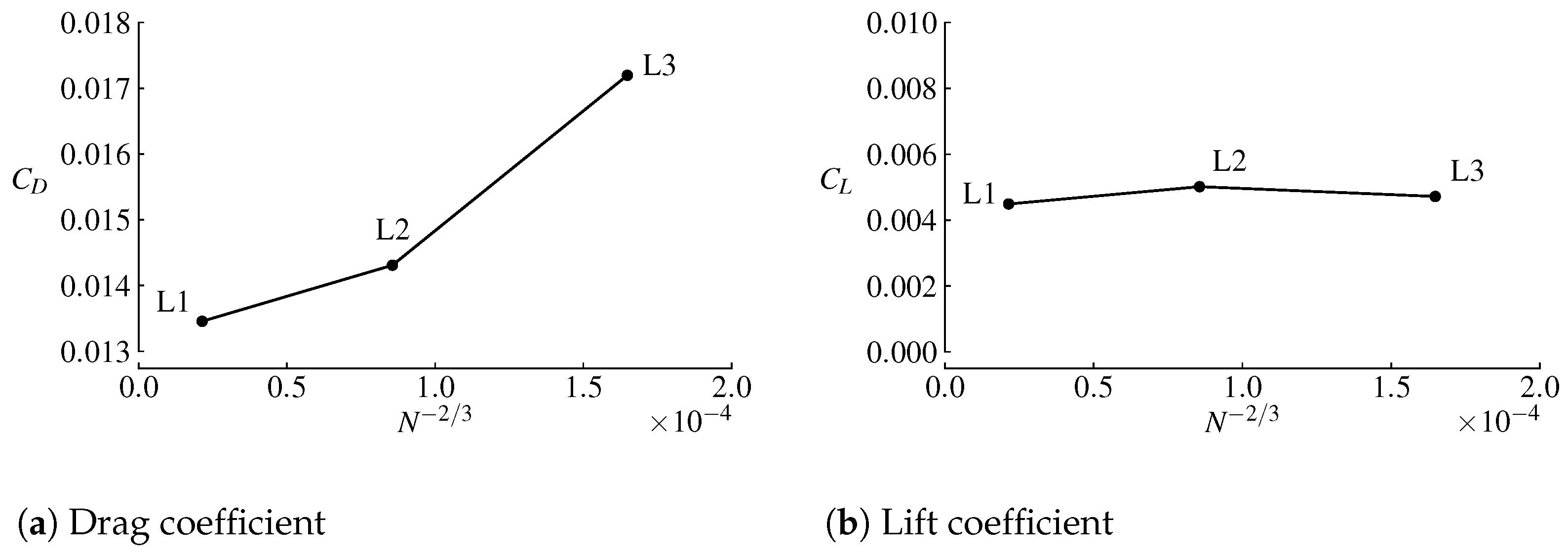

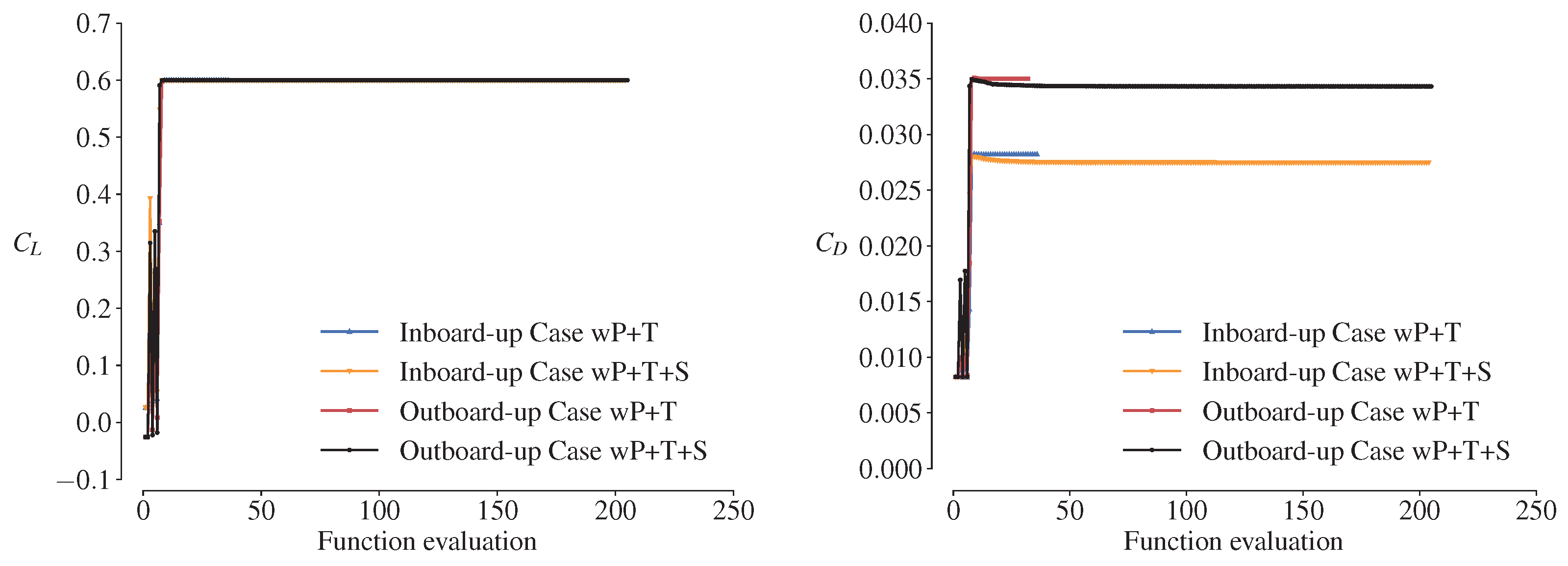
Appendix B. Optimization Convergence Plots
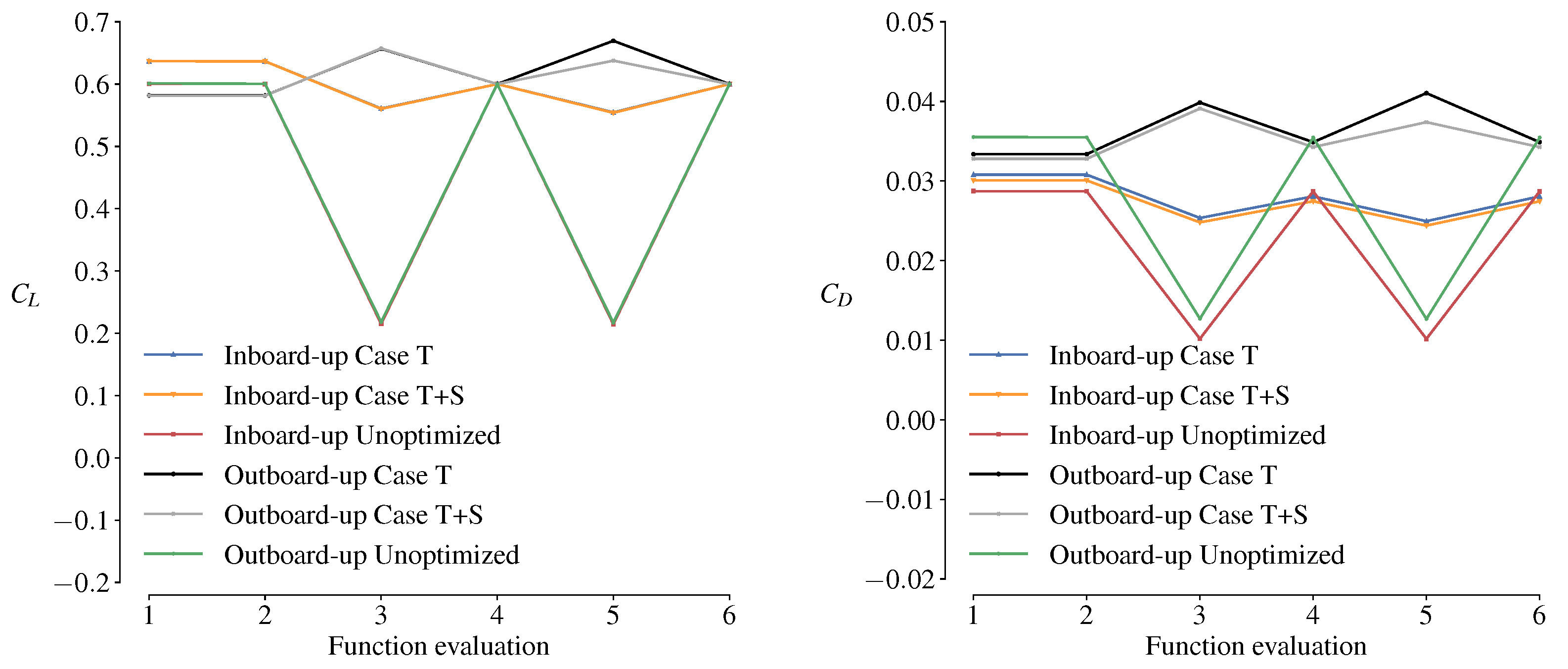
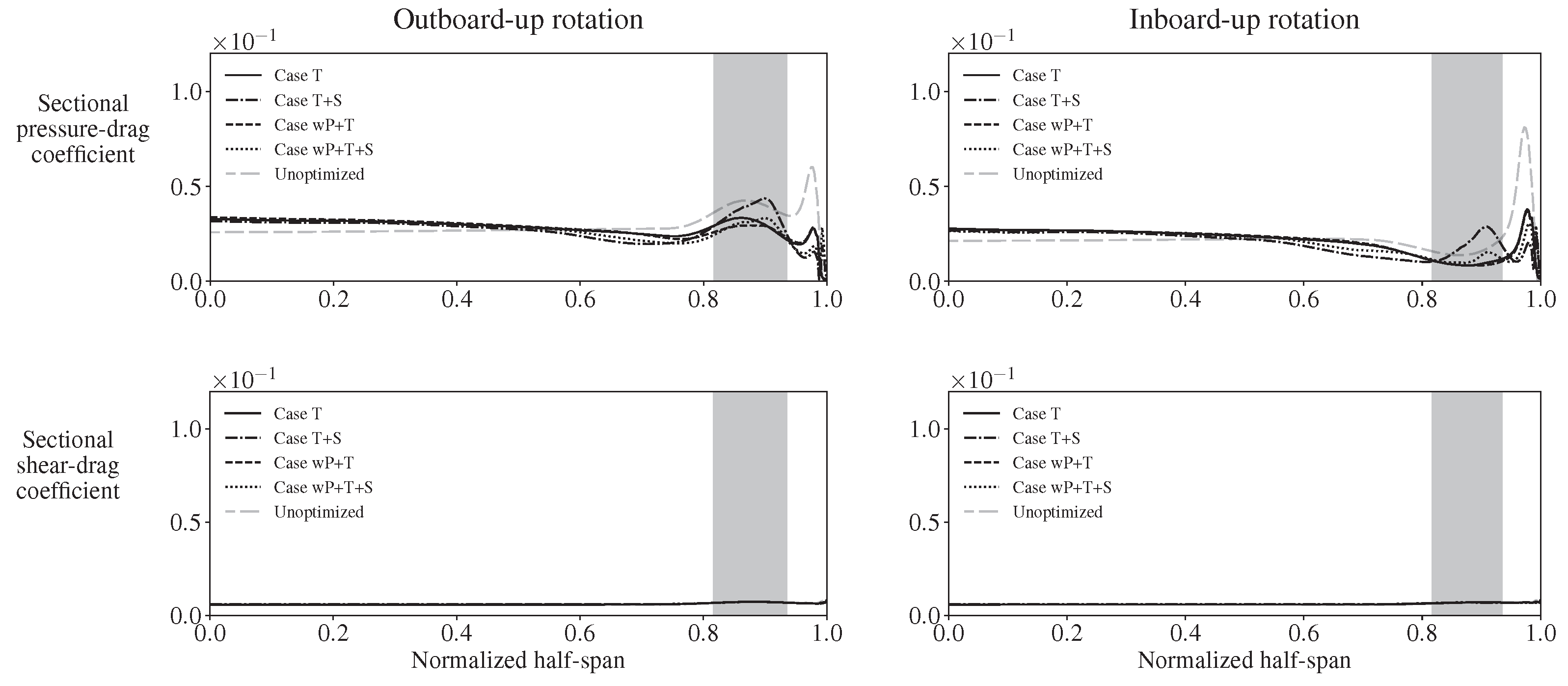
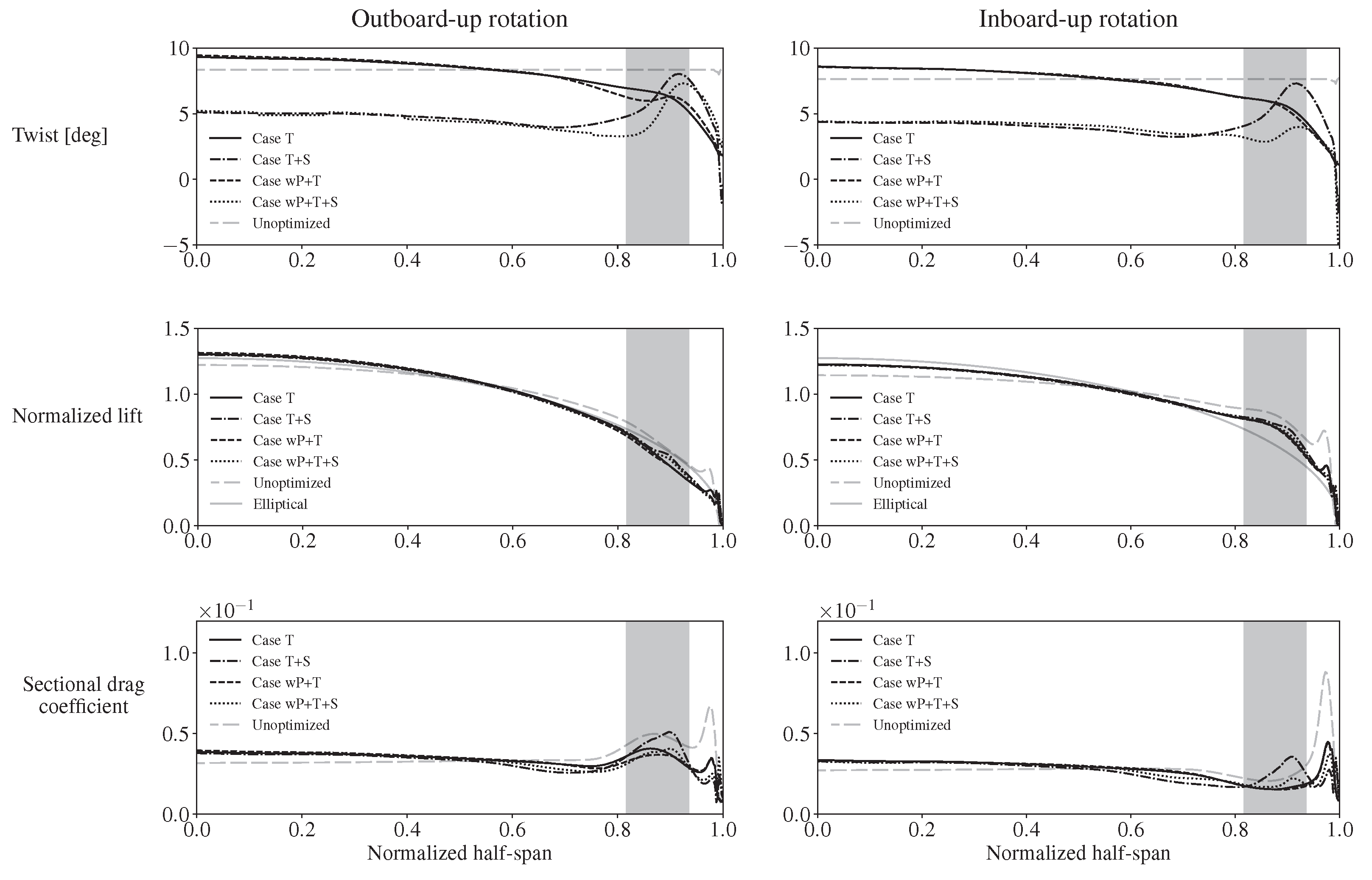
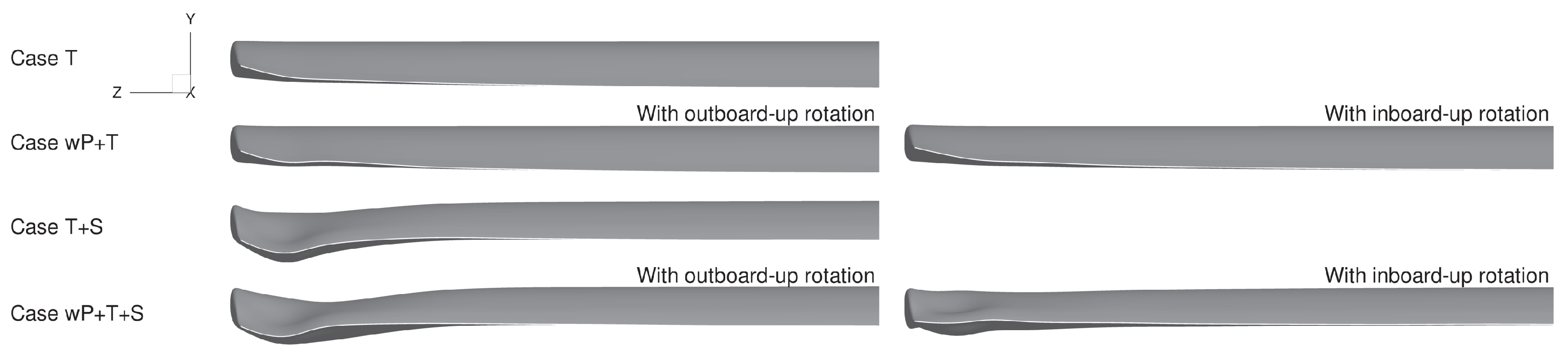
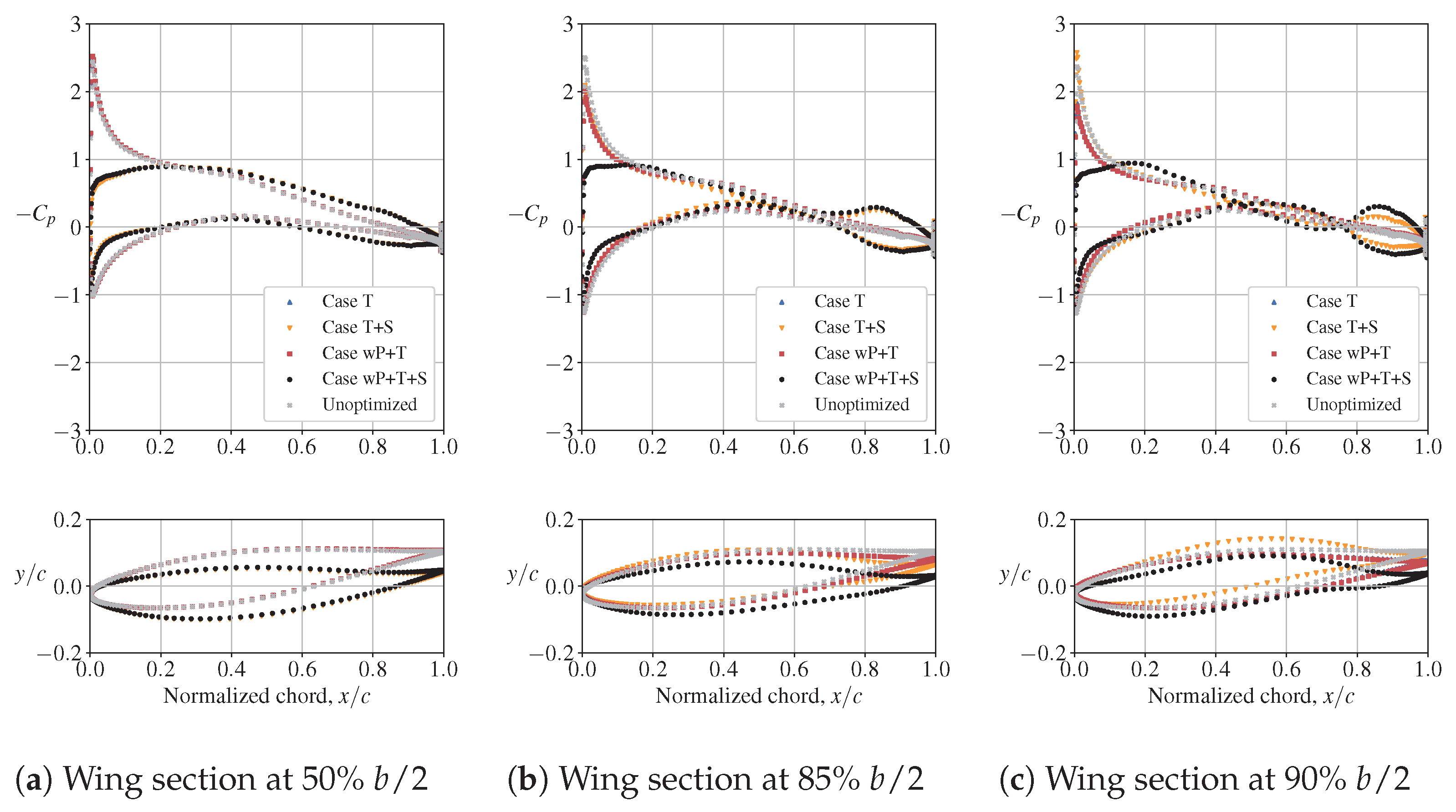
Appendix C. Spanwise Drag-Coefficient Breakdowns for Optimization Results
References
- Moore, M.D.; Fredericks, B. Misconceptions of Electric Aircraft and their Emerging Aviation Markets. In Proceedings of the 52nd Aerospace Sciences Meeting, National Harbor, MD, USA, 13–17 January 2014; Number AIAA 2014-0534. AIAA: Reston, VA, USA, 2014; p. 2014-0535. [Google Scholar] [CrossRef]
- Patterson, M.D.; German, B. Conceptual Design of Electric Aircraft with Distributed Propellers: Multidisciplinary Analysis Needs and Aerodynamic Modeling Development. In Proceedings of the 52nd Aerospace Sciences Meeting, National Harbor, MD, USA, 13–17 January 2014; Number AIAA 2014-0534. AIAA: Reston, VA, USA, 2014. [Google Scholar] [CrossRef]
- Stoll, A.M.; Bevirt, J.; Moore, M.D.; Fredericks, W.J.; Borer, N.K. Drag Reduction Through Distributed Electric Propulsion. In Proceedings of the 14th AIAA Aviation Technology, Integration, and Operations Conference, Atlanta, GA, USA, 16–20 June 2014; Number AIAA 2014-2851. AIAA: Reston, VA, USA, 2014. [Google Scholar] [CrossRef]
- Stoll, A.M.; Bevirt, J.; Pei, P.P.; Stilson, E.V. Conceptual Design of the Joby S2 Electric VTOL PAV. In Proceedings of the 14th AIAA Aviation Technology, Integration, and Operations Conference, Atlanta, GA, USA, 16–20 June 2014; Number AIAA 2014-2407. AIAA: Reston, VA, USA, 2014. [Google Scholar] [CrossRef]
- Duffy, M.J.; Wakayama, S.R.; Hupp, R.; Lacy, R.; Stauffer, M. A Study in Reducing the Cost of Vertical Flight with Electric Propulsion. In Proceedings of the 17th AIAA Aviation Technology, Integration, and Operations Conference, Denver, CO, USA, 5–9 June 2017; Number AIAA 2017-3442. AIAA: Reston, VA, USA, 2017. [Google Scholar] [CrossRef]
- Deere, K.A.; Viken, S.A.; Carter, M.B.; Viken, J.K.; Derlaga, J.M.; Stoll, A.M. Comparison of High-Fidelity Computational Tools for Wing Design of a Distributed Electric Propulsion Aircraft. In Proceedings of the 35th AIAA Applied Aerodynamics Conference, Denver, CO, USA, 5–9 June 2017; Number AIAA 2017-3925. AIAA: Reston, VA, USA, 2017. [Google Scholar] [CrossRef]
- Fredericks, W.J.; McSwain, R.G.; Beaton, B.F.; Klassman, D.W.; Theodore, C.R. Greased Lightning (GL-10) Flight Testing Campaign; Technical Memorandum NASA-TM-2017-219643; NASA: Washington, DC, USA, 2017. [Google Scholar]
- Antcliff, K.R.; Capristan, F.M. Conceptual Design of the Parallel Electric-Gas Architecture with Synergistic Utilization Scheme (PEGASUS) Concept. In Proceedings of the 18th AIAA/ISSMO Multidisciplinary Analysis and Optimization Conference, Denver, CO, USA, 5–9 June 2017; Number AIAA 2017-4001. AIAA: Reston, VA, USA, 2017. [Google Scholar] [CrossRef]
- Alba, C.; Elham, A.; German, B.J.; Veldhuis, L.L.M. A surrogate-based multi-disciplinary design optimization framework modeling wing-propeller interaction. Aerosp. Sci. Technol. 2018, 78, 721–733. [Google Scholar] [CrossRef]
- Hwang, J.T.; Ning, A. Large-scale multidisciplinary optimization of an electric aircraft for on-demand mobility. In Proceedings of the 2018 AIAA/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Kissimmee, FL, USA, 8–12 January 2018; Number AIAA 2018-1384. AIAA: Reston, VA, USA, 2018. [Google Scholar] [CrossRef]
- Droandi, G.; Syal, M.; Bower, G. Tiltwing Multi-Rotor Aerodynamic Modeling in Hover, Transition and Cruise Flight Conditions. In Proceedings of the AHS International Forum 74, Phoenix, AZ, USA, 14–17 May 2018; Paper 74-2018-1267. VFS: Fairfax, VA, USA, 2018. [Google Scholar]
- Brelje, B.J.; Martins, J.R.R.A. Electric, Hybrid, and Turboelectric Fixed-Wing Aircraft: A Review of Concepts, Models, and Design Approaches. Prog. Aerosp. Sci. 2019, 104, 1–19. [Google Scholar] [CrossRef]
- Moore, K.R.; Ning, A. Takeoff and Performance Trade-Offs of Retrofit Distributed Electric Propulsion for Urban Transport. J. Aircr. 2019, 56, 1880–1892. [Google Scholar] [CrossRef]
- De Vries, R.; Brown, M.; Vos, R. Preliminary Sizing Method for Hybrid-Electric Distributed-Propulsion Aircraft. J. Aircr. 2019, 56, 2172–2188. [Google Scholar] [CrossRef]
- Chauhan, S.S.; Martins, J.R.R.A. Tilt-Wing eVTOL Takeoff Trajectory Optimization. J. Aircr. 2020, 57, 93–112. [Google Scholar] [CrossRef]
- Hoogreef, M.; de Vries, R.; Sinnige, T.; Vos, R. Synthesis of Aero-Propulsive Interaction Studies Applied to Conceptual Hybrid-Electric Aircraft Design. In Proceedings of the AIAA Scitech 2020 Forum, Orlando, FL, USA, 6–10 January 2020; Number AIAA 2020-0503. AIAA: Reston, VA, USA, 2020. [Google Scholar] [CrossRef]
- Chauhan, S.S.; Martins, J.R.R.A. RANS-Based Aerodynamic Shape Optimization of a Wing Considering Propeller-Wing Interaction. J. Aircr. 2021, 58, 497–513. [Google Scholar] [CrossRef]
- Prandtl, L. Mutual Influence of Wings and Propeller; Technical Note NACA-TN-74, NACA; An English translation of an extract from the First Report of the Göttingen Aerodynamic Laboratory; NACA: Hampton, VA, USA, 1921. [Google Scholar]
- Kuhn, R.E.; Draper, J.W. An Investigation of a Wing-Propeller Configuration Employing Large-Chord Plain Flaps and Large-Diameter Propellers for Low-Speed Flight and Vertical Take-Off; Technical Note NACA-TN-3307; NACA: Hampton, VA, USA, 1954. [Google Scholar]
- Kuhn, R.E.; Draper, J.W. Investigation of the Aerodynamic Characteristics of a Model Wing-Propeller Combination and of the Wing and Propeller Separately at Angles of Attack up to 90°; Technical Report NACA-TR-1263; NACA: Hampton, VA, USA, 1956. [Google Scholar]
- Snyder, M.H., Jr.; Zumwalt, G.W. Effects of Wingtip-Mounted Propellers on Wing Lift and Induced Drag. J. Aircr. 1969, 6, 392–397. [Google Scholar] [CrossRef]
- Kroo, I. Propeller-Wing Integration For Minimum Induced Loss. J. Aircr. 1986, 23, 561–565. [Google Scholar] [CrossRef]
- Miranda, L.R.; Brennan, J.E. Aerodynamic Effects of Eingtip-Mounted Propellers and Turbines. In Proceedings of the 4th Applied Aerodynamics Conference, San Diego, CA, USA, 9–11 June 1986; Number AIAA 1986-1802. AIAA: Reston, VA, USA, 1986. [Google Scholar] [CrossRef]
- Veldhuis, L.L.M. Propeller Wing Aerodynamic Interference. Ph.D Thesis, Delft University of Technology, Delft, The Netherlands, 2005. [Google Scholar]
- Sinnige, T.; van Arnhem, N.; Stokkermans, T.C.A.; Eitelberg, G.; Veldhuis, L.L.M. Wingtip-Mounted Propellers: Aerodynamic Analysis of Interaction Effects and Comparison with Conventional Layout. J. Aircr. 2019, 56, 295–312. [Google Scholar] [CrossRef]
- Miley, S.J.; Howard, R.M.; Holmes, B.J. Wing Laminar Boundary Layer in the Presence of a Propeller Slipstream. J. Aircr. 1988, 25, 606–611. [Google Scholar] [CrossRef]
- Stokkermans, T.; Veldhuis, L.; Soemarwoto, B.; Fukari, R.; Eglin, P. Breakdown of aerodynamic interactions for the lateral rotors on a compound helicopter. Aerosp. Sci. Technol. 2020, 101, 105845. [Google Scholar] [CrossRef]
- Whitfield, D.L.; Jameson, A. Euler Equation Simulation of Propeller-Wing Interaction in Transonic Flow. J. Aircr. 1984, 21, 835–839. [Google Scholar] [CrossRef]
- Witkowski, D.P.; Lee, A.K.H.; Sullivan, J.P. Aerodynamic Interaction Between Propellers and Wings. J. Aircr. 1989, 26, 829–836. [Google Scholar] [CrossRef]
- Ardito Marretta, R.M.; Davi, G.; Milazzo, A.; Lombardi, G. Wing Pitching and Loading with Propeller Interference. J. Aircr. 1999, 36, 468–471. [Google Scholar] [CrossRef]
- Veldhuis, L.L.M.; Heyma, P.M. Aerodynamic optimisation of wings in multi-engined tractor propeller arrangements. Aircr. Des. 2000, 3, 129–149. [Google Scholar] [CrossRef]
- Moens, F.; Gardarein, P. Numerical Simulation of the Propeller/Wing Interactions for Transport Aircraft. In Proceedings of the 19th AIAA Applied Aerodynamics Conference, Anaheim, CA, USA, 11–14 June 2001; Number AIAA 2001-2404. AIAA: Reston, VA, USA, 2001. [Google Scholar] [CrossRef]
- Roosenboom, E.W.M.; Stürmer, A.; Schröder, A. Advanced Experimental and Numerical Validation and Analysis of Propeller Slipstream Flows. J. Aircr. 2010, 47, 284–291. [Google Scholar] [CrossRef]
- Gomariz-Sancha, A.; Maina, M.; Peace, A.J. Analysis of propeller-airframe interaction effects through a combined numerical simulation and wind-tunnel testing approach. In Proceedings of the 53rd AIAA Aerospace Sciences Meeting, Kissimmee, FL, USA, 5–9 January 2015; Number AIAA 2015-1026. AIAA: Reston, VA, USA, 2015. [Google Scholar] [CrossRef]
- Rakshith, B.R.; Deshpande, S.M.; Narasimha, R.; Praveen, C. Optimal Low-Drag Wing Planforms for Tractor-Configuration Propeller-Driven Aircraft. J. Aircr. 2015, 52, 1791–1801. [Google Scholar] [CrossRef]
- Epema, K. Wing Optimisation for Tractor Propeller Configurations. Master’s Thesis, Delft University of Technology, Delft, The Netherlands, 2017. [Google Scholar]
- Stokkermans, T.C.A.; van Arnhem, N.; Sinnige, T.; Veldhuis, L.L.M. Validation and Comparison of RANS Propeller Modeling Methods for Tip-Mounted Applications. AIAA J. 2019, 57, 566–580. [Google Scholar] [CrossRef]
- Van Arnhem, N.; de Vries, R.; Sinnige, T.; Vos, R.; Veldhuis, L.L.M. Aerodynamic Performance and Static Stability Characteristics of Aircraft with Tail-Mounted Propellers. J. Aircr. 2022, 59, 415–432. [Google Scholar] [CrossRef]
- Koyuncuoglu, H.U.; He, P. Simultaneous wing shape and actuator parameter optimization using the adjoint method. Aerosp. Sci. Technol. 2022, 130, 107876. [Google Scholar] [CrossRef]
- Russo, O.; Aprovitola, A.; de Rosa, D.; Pezzella, G.; Viviani, A. Computational Fluid Dynamics Analyses of a Wing with Distributed Electric Propulsion. Aerospace 2023, 10, 64. [Google Scholar] [CrossRef]
- Alvarez, E.J.; Ning, A. Meshless Large-Eddy Simulation of Propeller–Wing Interactions with Reformulated Vortex Particle Method. J. Aircr. 2024, 61. [Google Scholar] [CrossRef]
- Pedreiro, L.N. Estudo e otimização de uma asa sob efeito de hélice na configuração tractor para redução de arrasto. Master’s Thesis, Universidade Federal de Minas Gerais, Belo Horizonte, MG, Brazil, 2017. [Google Scholar]
- Martins, J.R.R.A. Aerodynamic Design Optimization: Challenges and Perspectives. Comput. Fluids 2022, 239, 105391. [Google Scholar] [CrossRef]
- Yildirim, A.; Kenway, G.K.W.; Mader, C.A.; Martins, J.R.R.A. A Jacobian-free approximate Newton–Krylov startup strategy for RANS simulations. J. Comput. Phys. 2019, 397, 108741. [Google Scholar] [CrossRef]
- Mader, C.A.; Kenway, G.K.W.; Yildirim, A.; Martins, J.R.R.A. ADflow: An Open-Source Computational Fluid Dynamics Solver for Aerodynamic and Multidisciplinary Optimization. J. Aerosp. Inf. Syst. 2020, 17, 508–527. [Google Scholar] [CrossRef]
- Lee, Y.; Baeder, J. Implicit hole cutting—A new approach to overset grid connectivity. In Proceedings of the 16th AIAA Computational Fluid Dynamics Conference, Orlando, FL, USA, 23–26 June 2003; Number AIAA 2003-4128. AIAA: Reston, VA, USA, 2003. [Google Scholar] [CrossRef]
- Landmann, B.; Montagnac, M. A highly automated parallel Chimera method for overset grids based on the implicit hole cutting technique. Int. J. Numer. Methods Fluids 2011, 66, 778–804. [Google Scholar] [CrossRef]
- Kenway, G.K.W.; Secco, N.; Martins, J.R.R.A.; Mishra, A.; Duraisamy, K. An Efficient Parallel Overset Method for Aerodynamic Shape Optimization. In Proceedings of the 58th AIAA/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, AIAA SciTech Forum, Grapevine, TX, USA, 9–13 January 2017; Number AIAA 2017-0357. AIAA: Reston, VA, USA, 2017. [Google Scholar] [CrossRef]
- Jameson, A.; Schmidt, W.; Turkel, E. Numerical Solution of the Euler Equations by Finite Volume Methods Using Runge–Kutta Time Stepping Schemes. In Proceedings of the 14th Fluid and Plasma Dynamics Conference, Palo Alto, CA, USA, 23–25 June 1981; Number AIAA 1981-1259. AIAA: Reston, VA, USA, 1981. [Google Scholar] [CrossRef]
- Spalart, P.; Allmaras, S. A One-Equation Turbulence Model for Aerodynamic Flows. In Proceedings of the 30th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 6–9 January 1992; Number AIAA 1992-439. AIAA: Reston, VA, USA, 1992. [Google Scholar] [CrossRef]
- Kenway, G.K.W.; Mader, C.A.; He, P.; Martins, J.R.R.A. Effective Adjoint Approaches for Computational Fluid Dynamics. Prog. Aerosp. Sci. 2019, 110, 100542. [Google Scholar] [CrossRef]
- Secco, N.; Kenway, G.K.W.; He, P.; Mader, C.A.; Martins, J.R.R.A. Efficient Mesh Generation and Deformation for Aerodynamic Shape Optimization. AIAA J. 2021, 59, 1151–1168. [Google Scholar] [CrossRef]
- Hascoët, L. TAPENADE: A tool for Automatic Differentiation of programs. In Proceedings of the 4th European Congress on Computational Methods, ECCOMAS’2004, Jyvaskyla, Finland, 24–28 July 2004. [Google Scholar]
- Hascoët, L.; Pascual, V. The Tapenade automatic differentiation tool: Principles, model, and specification. Acm Trans. Math. Softw. 2013, 39, 1–43. [Google Scholar] [CrossRef]
- Kenway, G.K.W.; Kennedy, G.J.; Martins, J.R.R.A. A CAD-Free Approach to High-Fidelity Aerostructural Optimization. In Proceedings of the 13th AIAA/ISSMO Multidisciplinary Analysis Optimization Conference, Fort Worth, TX, USA, 13–15 September 2010; Number AIAA 2010-9231. AIAA: Reston, VA, USA, 2010. [Google Scholar] [CrossRef]
- Luke, E.; Collins, E.; Blades, E. A Fast Mesh Deformation Method Using Explicit Interpolation. J. Comput. Phys. 2012, 231, 586–601. [Google Scholar] [CrossRef]
- Gill, P.E.; Murray, W.; Saunders, M.A. SNOPT: An SQP Algorithm for Large-Scale Constrained Optimization. Siam Rev. 2005, 47, 99–131. [Google Scholar] [CrossRef]
- Osusky, L.; Buckley, H.; Reist, T.; Zingg, D.W. Drag Minimization Based on the Navier–Stokes Equations Using a Newton–Krylov Approach. AIAA J. 2015, 53, 1555–1577. [Google Scholar] [CrossRef]
- Perez, R.E.; Jansen, P.W.; Martins, J.R.R.A. pyOpt: A Python-Based Object-Oriented Framework for Nonlinear Constrained Optimization. Struct. Multidiscip. Optim. 2012, 45, 101–118. [Google Scholar] [CrossRef]
- Wu, N.; Kenway, G.; Mader, C.A.; Jasa, J.; Martins, J.R.R.A. pyOptSparse: A Python framework for large-scale constrained nonlinear optimization of sparse systems. J. Open Source Softw. 2020, 5, 2564. [Google Scholar] [CrossRef]
- Hoekstra, M. A RANS-based analysis tool for ducted propeller systems in open water condition. Int. Shipbuild. Prog. 2006, 53, 205–227. [Google Scholar]
- Chauhan, S.S. Estimating propeller-induced tangential velocities by hand. 2023. preprint. Available online: http://doi.org/10.13140/RG.2.2.26021.88801 (accessed on 1 January 2024).
- Sinnige, T.; de Vries, R.; Corte, B.D.; Avallone, F.; Ragni, D.; Eitelberg, G.; Veldhuis, L.L.M. Unsteady Pylon Loading Caused by Propeller-Slipstream Impingement for Tip-Mounted Propellers. J. Aircr. 2018, 55, 1605–1618. [Google Scholar] [CrossRef]
- Biermann, D.; Hartman, E.P. Full-Scale Tests of 4- and 6-Blade, Single- and Dual-Rotating Propellers; Special Report NACA-SR-157; NACA: Hampton, VA, USA, 1940. [Google Scholar]
- Anonymous. Generalized Method of Propeller Performance Estimation; Technical Report PDB 6101 Revision A; Hamilton Standard: Windsor Locks, CT, USA, 1963. [Google Scholar]
- Raymer, D.P. Aircraft Design: A Conceptual Approach, 5th ed.; AIAA: Reston, VA, USA, 2012. [Google Scholar]
- McCormick, B.W. Aerodynamics of V/STOL Flight, 1st ed.; Academic Press: San Diego, CA, USA, 1967. [Google Scholar]
- Kroo, I.M. Drag due to Lift: Concepts for Prediction and Reduction. Annu. Rev. Fluid Mech. 2000, 33, 587–617. [Google Scholar] [CrossRef]
- Bons, N.P.; He, X.; Mader, C.A.; Martins, J.R.R.A. Multimodality in Aerodynamic Wing Design Optimization. AIAA J. 2019, 57, 1004–1018. [Google Scholar] [CrossRef]
- Reist, T.A.; Koo, D.; Zingg, D.W.; Bochud, P.; Castonguay, P.; Leblond, D. Cross Validation of Aerodynamic Shape Optimization Methodologies for Aircraft Wing-Body Optimization. AIAA J. 2020, 58. [Google Scholar] [CrossRef]


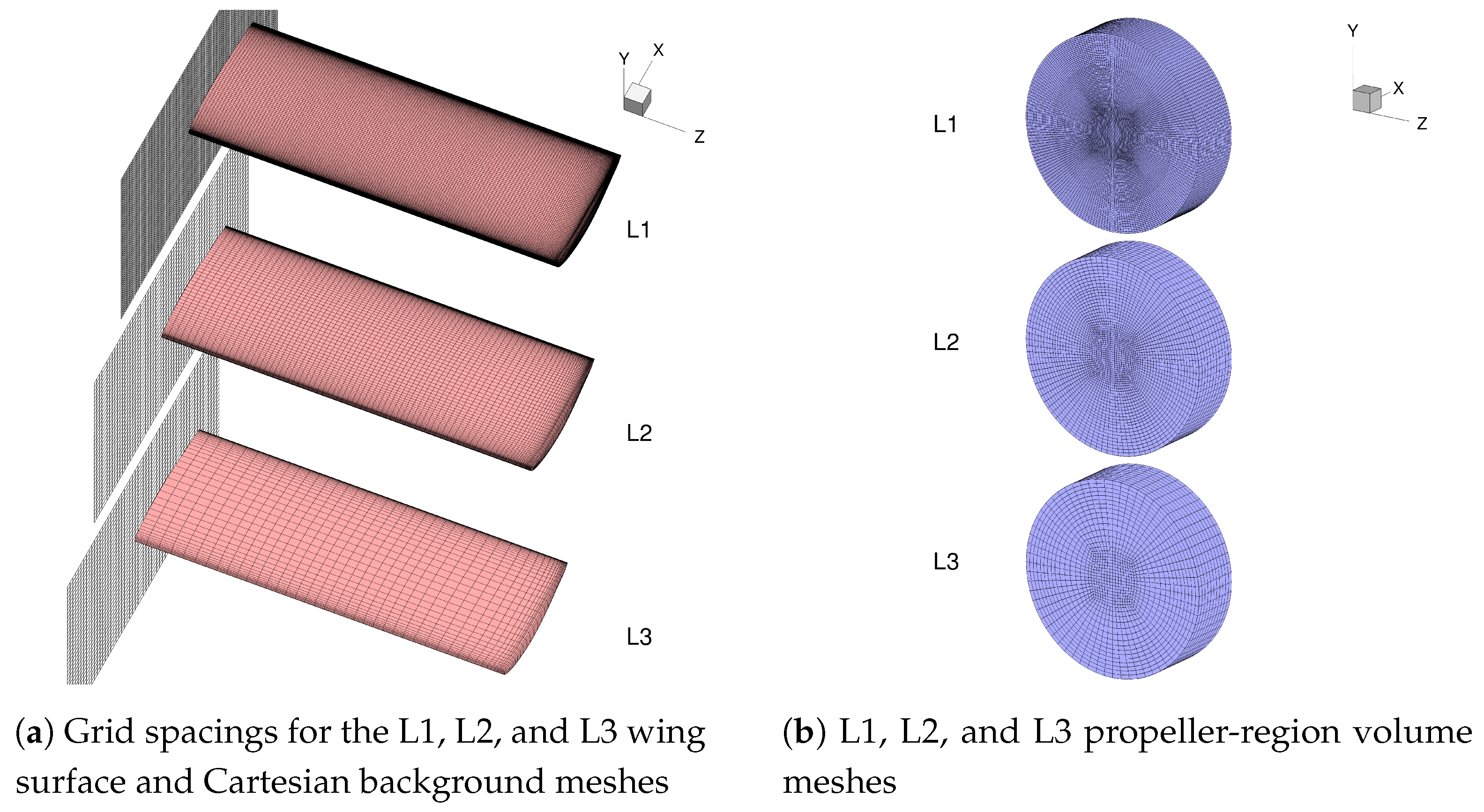





| Mesh | Total Number of Cells | Total Number of Computation Cells, N |
|---|---|---|
| L1 | 10,621,440 | 10,085,277 |
| L2 | 1,343,520 | 1,263,010 |
| L3 | 509,152 | 472,605 |
| Mach Number | Altitude | Assumed Aircraft Lift-to-Drag Ratio | Propeller Tip Mach Number | |
|---|---|---|---|---|
| 0.6 | 0.3 | 1500 ft | 10 | 0.6 |
| Advance Ratio, | Thrust Coefficient, | Thrust, T | Pitch-to-Diameter Ratio, |
|---|---|---|---|
| 1.6 | 0.20 | 5.5 kN | 1.7 |
| Function or Variable | Description | Quantity | |
|---|---|---|---|
| Minimize | Drag coefficient | ||
| by varying | Twist of each FFD section [] | 11 | |
| Total design variables | 11 | ||
| subject to | Lift constraint | 1 | |
| Total constraint functions | 1 |
| Function or Variable | Description | Quantity | |
|---|---|---|---|
| Minimize | Drag coefficient | ||
| by varying | Twist of each FFD section [] | 11 | |
| Vertical displacements of the FFD control points for airfoil-shape | |||
| control ( of the airfoil maximum thickness) [cm] | 176 | ||
| Total design variables | 187 | ||
| subject to | Lift constraint | 1 | |
| Constraints to prevent airfoil thicknesses at locations on a uniform | |||
| grid from decreasing | 100 | ||
| Constraints to prevent the airfoil-shape design variables from | |||
| vertically displacing the leading edge | 11 | ||
| Constraints to prevent the airfoil-shape design variables from | |||
| vertically displacing the trailing edge | 11 | ||
| Total constraint functions | 123 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chauhan, S.S.; Martins, J.R.R.A. RANS-Based Aerodynamic Shape Optimization of a Wing with a Propeller in Front of the Wingtip. Aerospace 2024, 11, 512. https://doi.org/10.3390/aerospace11070512
Chauhan SS, Martins JRRA. RANS-Based Aerodynamic Shape Optimization of a Wing with a Propeller in Front of the Wingtip. Aerospace. 2024; 11(7):512. https://doi.org/10.3390/aerospace11070512
Chicago/Turabian StyleChauhan, Shamsheer S., and Joaquim R. R. A. Martins. 2024. "RANS-Based Aerodynamic Shape Optimization of a Wing with a Propeller in Front of the Wingtip" Aerospace 11, no. 7: 512. https://doi.org/10.3390/aerospace11070512
APA StyleChauhan, S. S., & Martins, J. R. R. A. (2024). RANS-Based Aerodynamic Shape Optimization of a Wing with a Propeller in Front of the Wingtip. Aerospace, 11(7), 512. https://doi.org/10.3390/aerospace11070512





