Heat Transfer Models and Measurements of Brushless DC Motors for Small UASs
Abstract
1. Introduction
1.1. Motivation
1.2. Literature Review
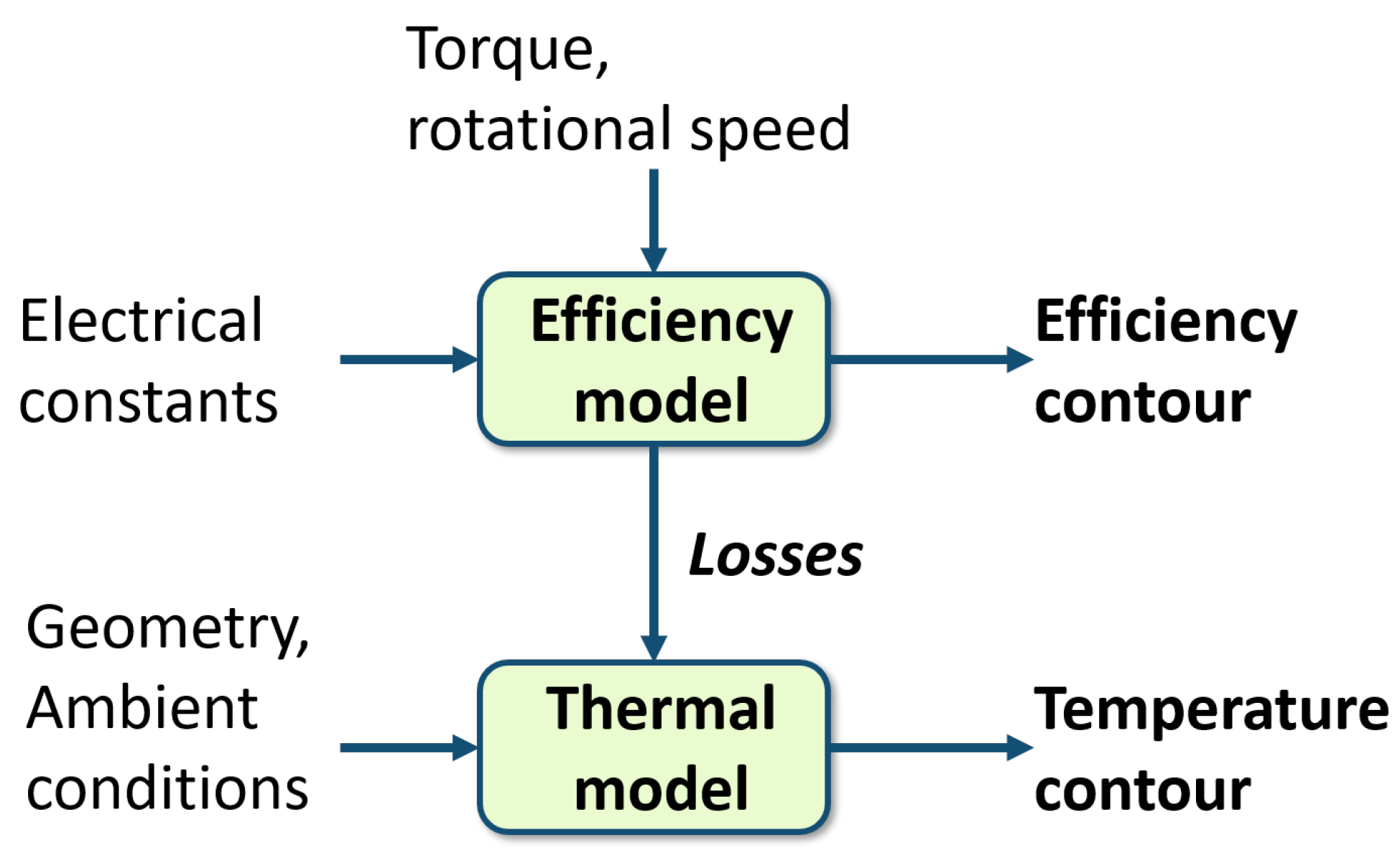
2. Methodology
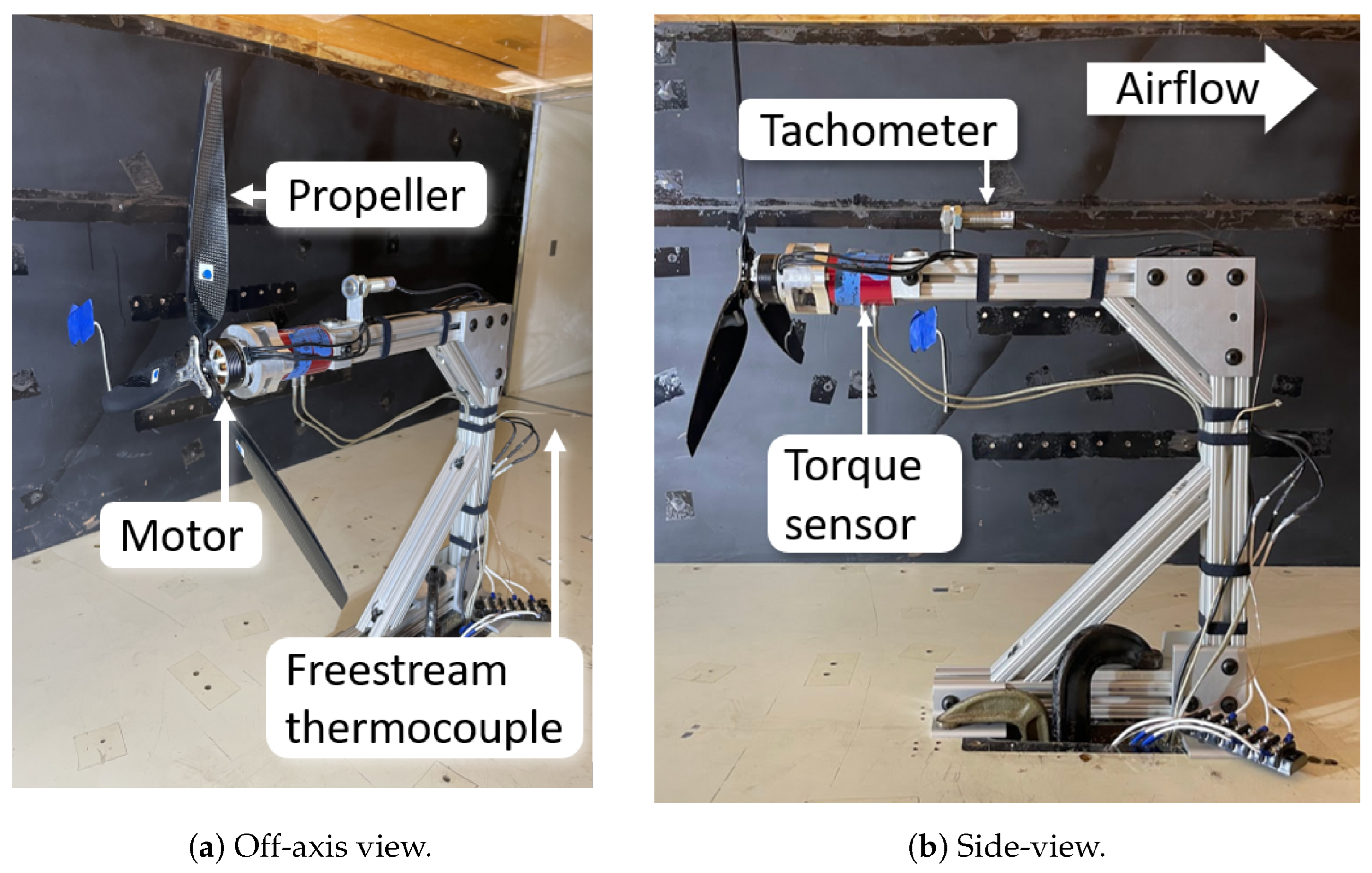
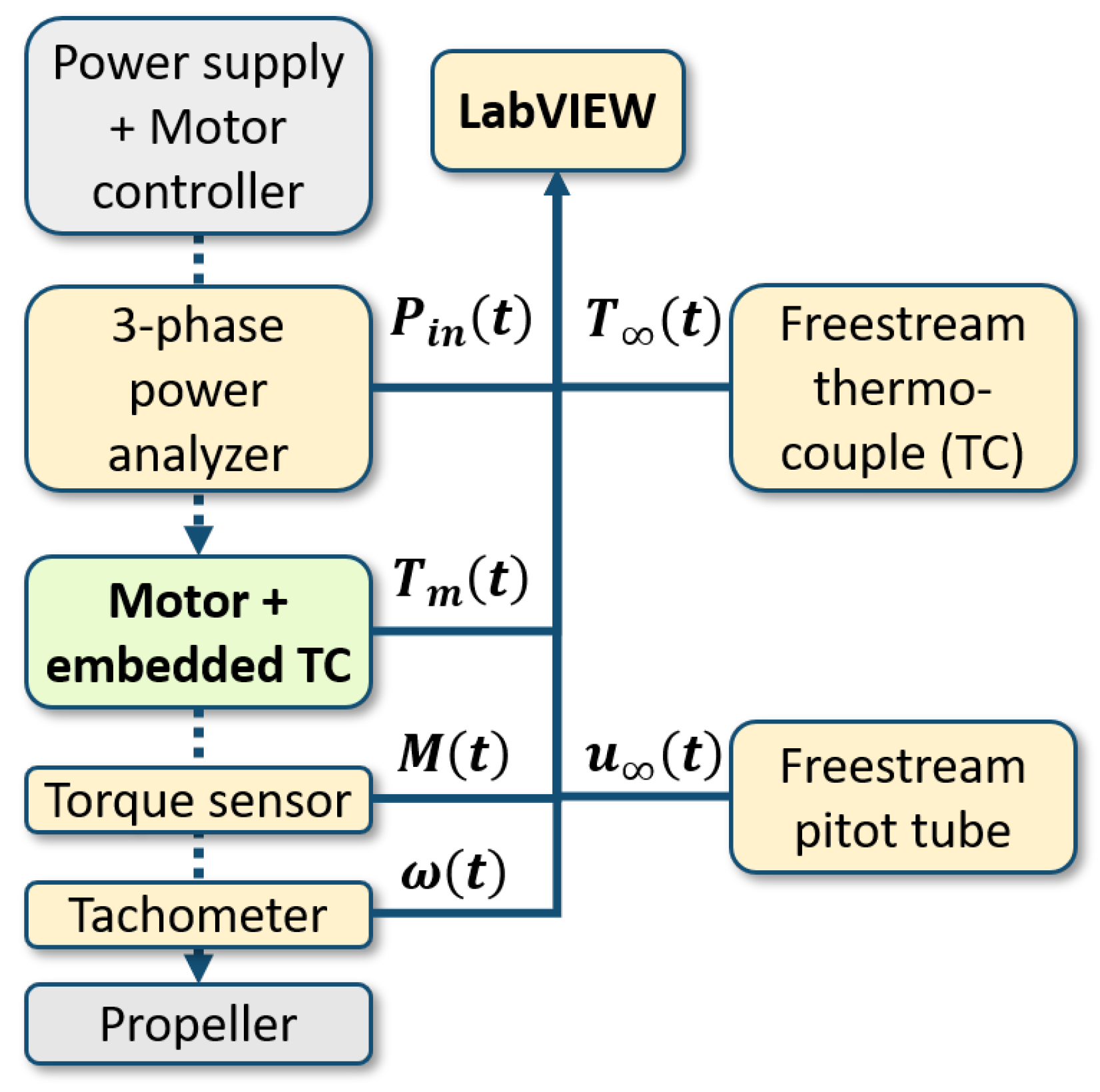
2.1. Wind Tunnel Stand
2.2. Tested Motors
2.3. Instrumentation
2.4. Control Variables
2.5. Assumptions
- Convection was the motor’s sole mode of heat transfer. To enforce this assumption, we installed a layer of thermally non-conductive plastic (Delrin) between the aluminum motor housing and the aluminum test stand. Delrin’s thermal conductivity is 1000× smaller than aluminum’s (0.210 vs. 237 W/(m·K), respectively [40]). We ignored radiation;
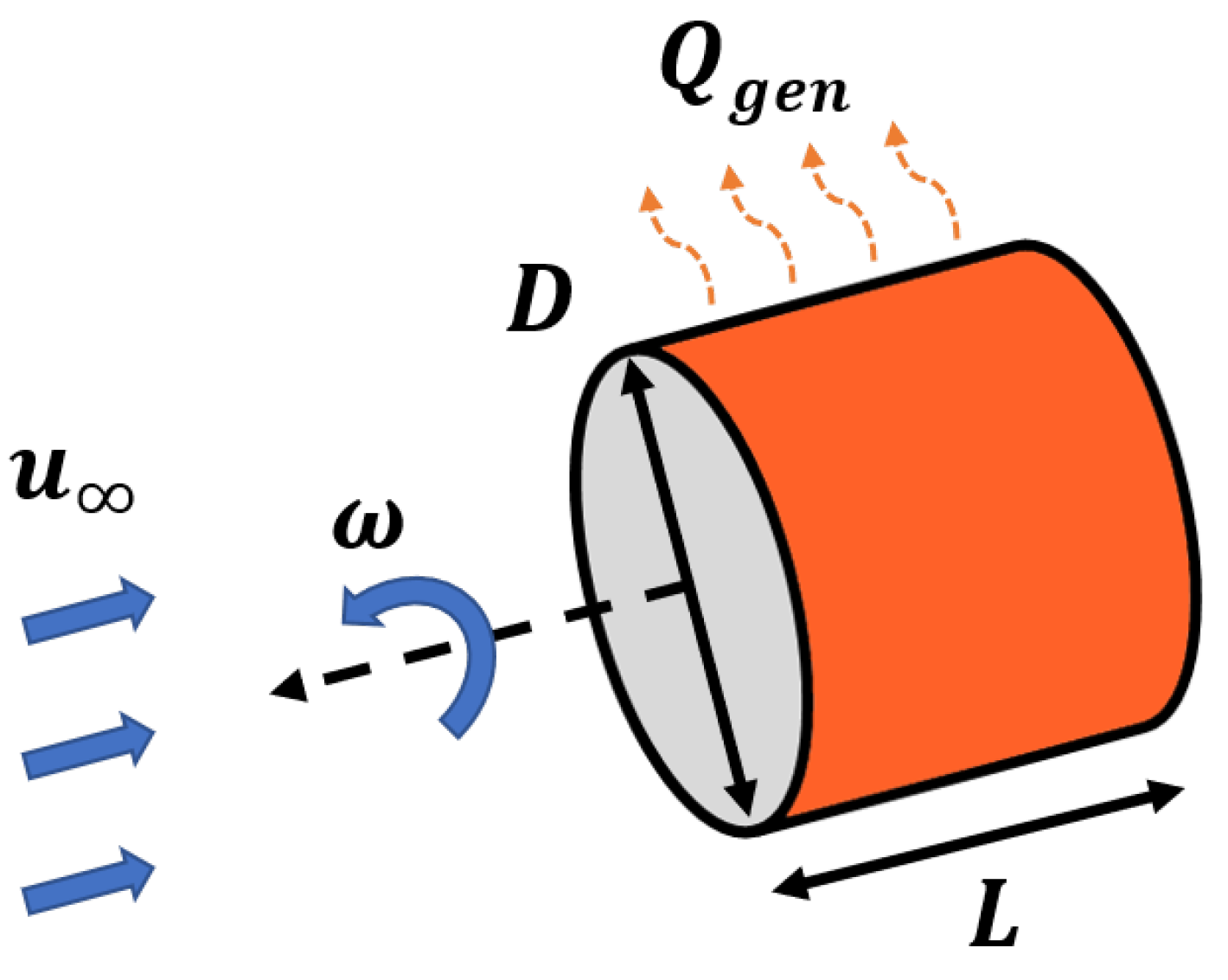
- Convection only occurred along the motor’s lateral surface area. The propeller and propeller adapter impede airflow onto the front of the motor. Forced convection due to the freestream velocity and rotational speed dissipated losses along the lateral surface area as depicted in Figure 7;
- The fluid characteristic length was the motor’s diameter (). The heat transfer literature suggests setting the characteristic length as the volume-to-surface area ratio. The motor is approximately a cylinder, and its volume/surface area ratio is proportional to diameter: . Additionally, the electrical literature shows that a motor’s diameter drives torque more than its length (Equation (4)).
2.6. Limiting Trade-Off
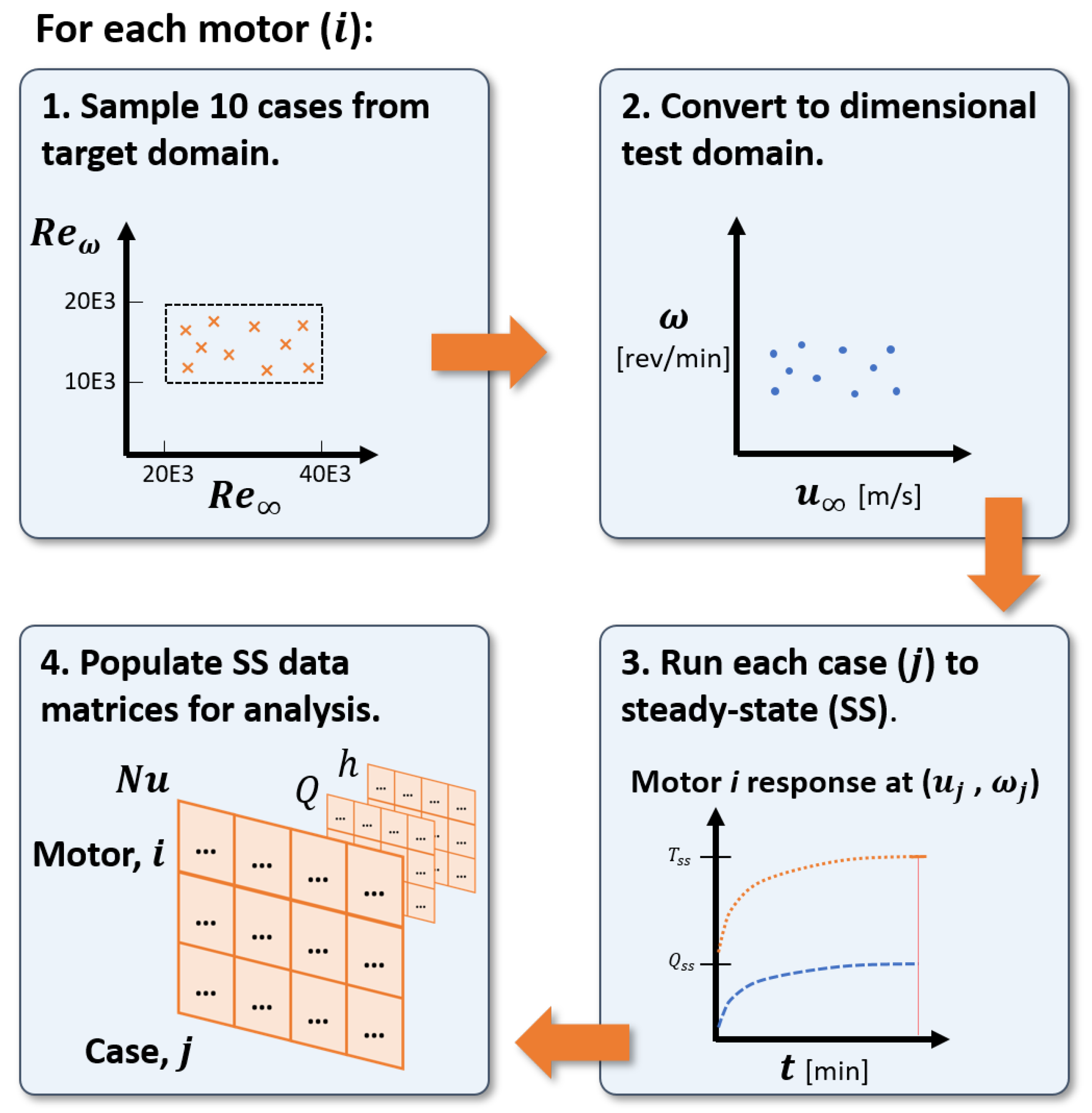
2.7. Steady-State Analysis
2.8. Numerical Correlation
2.9. Uncertainty Propagation
3. Results
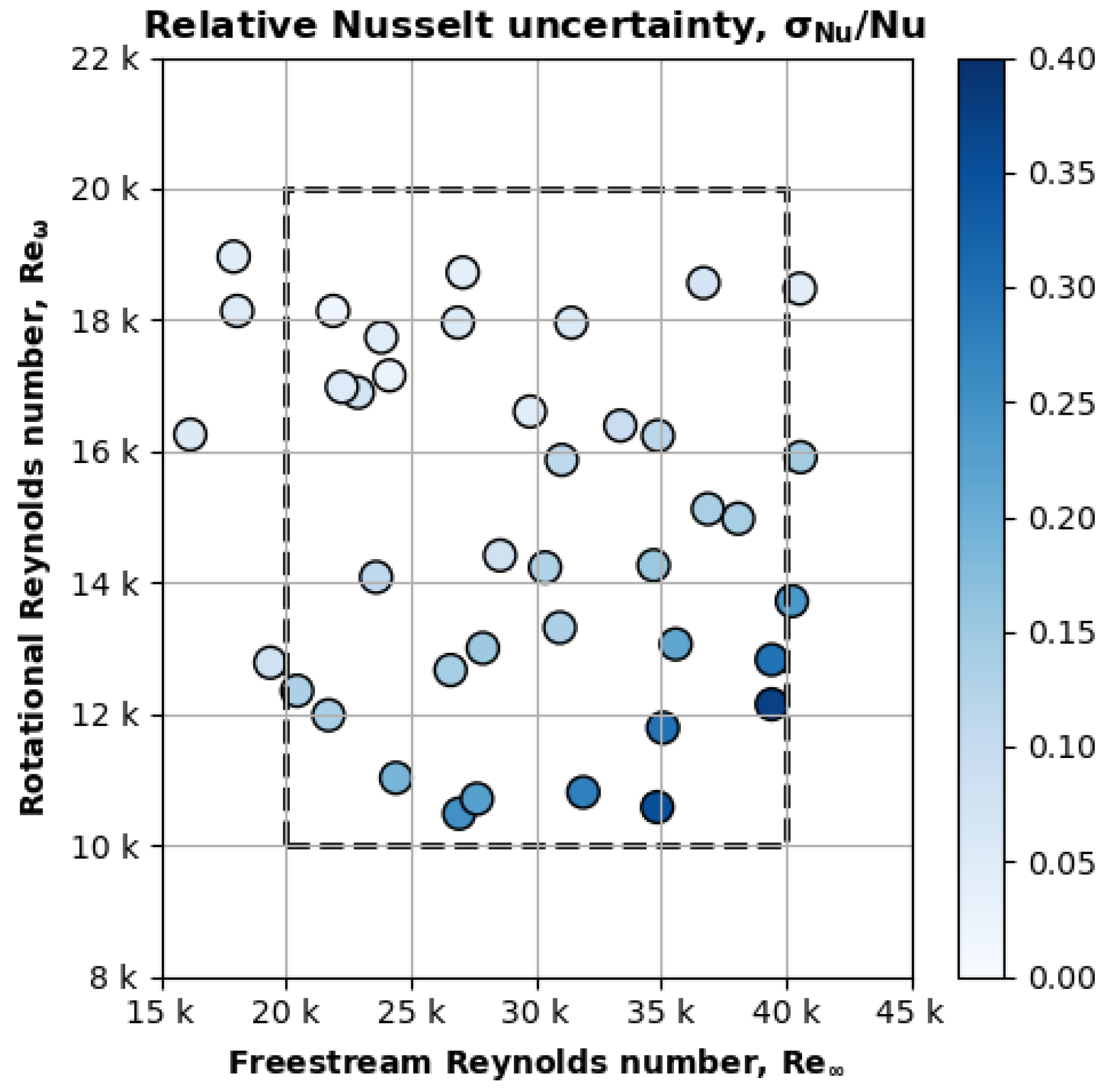
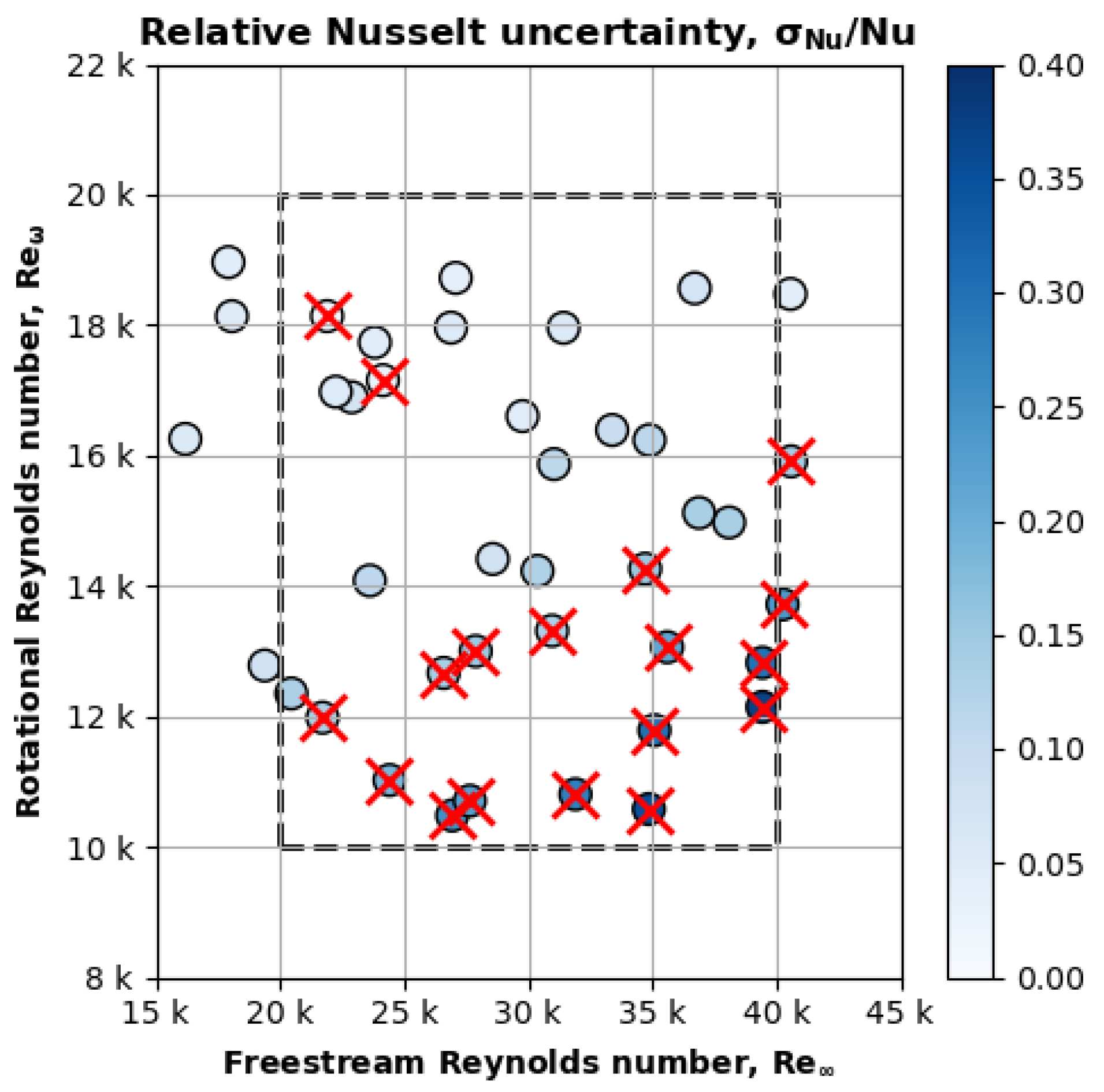
3.1. Reducing Uncertainty
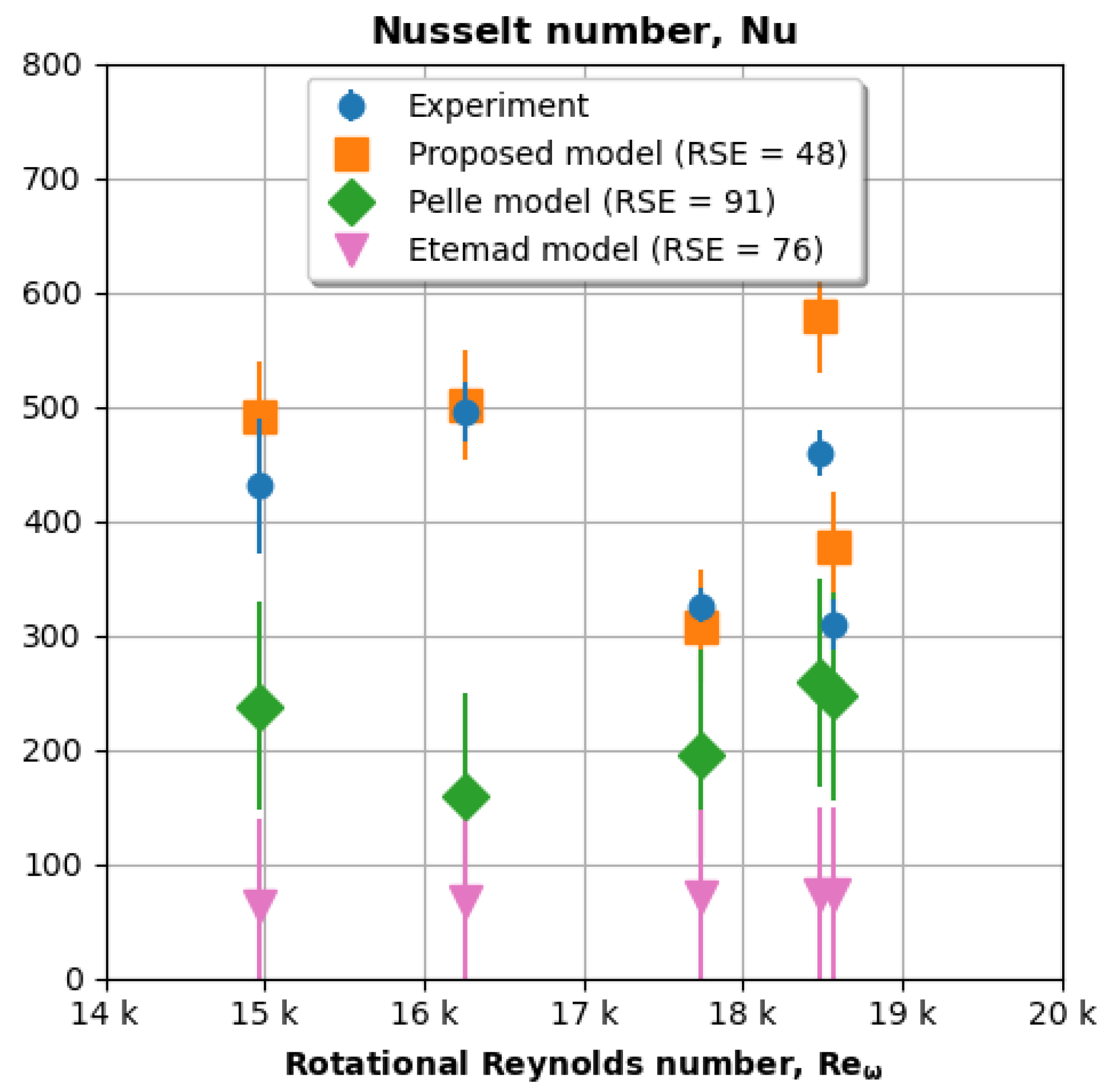
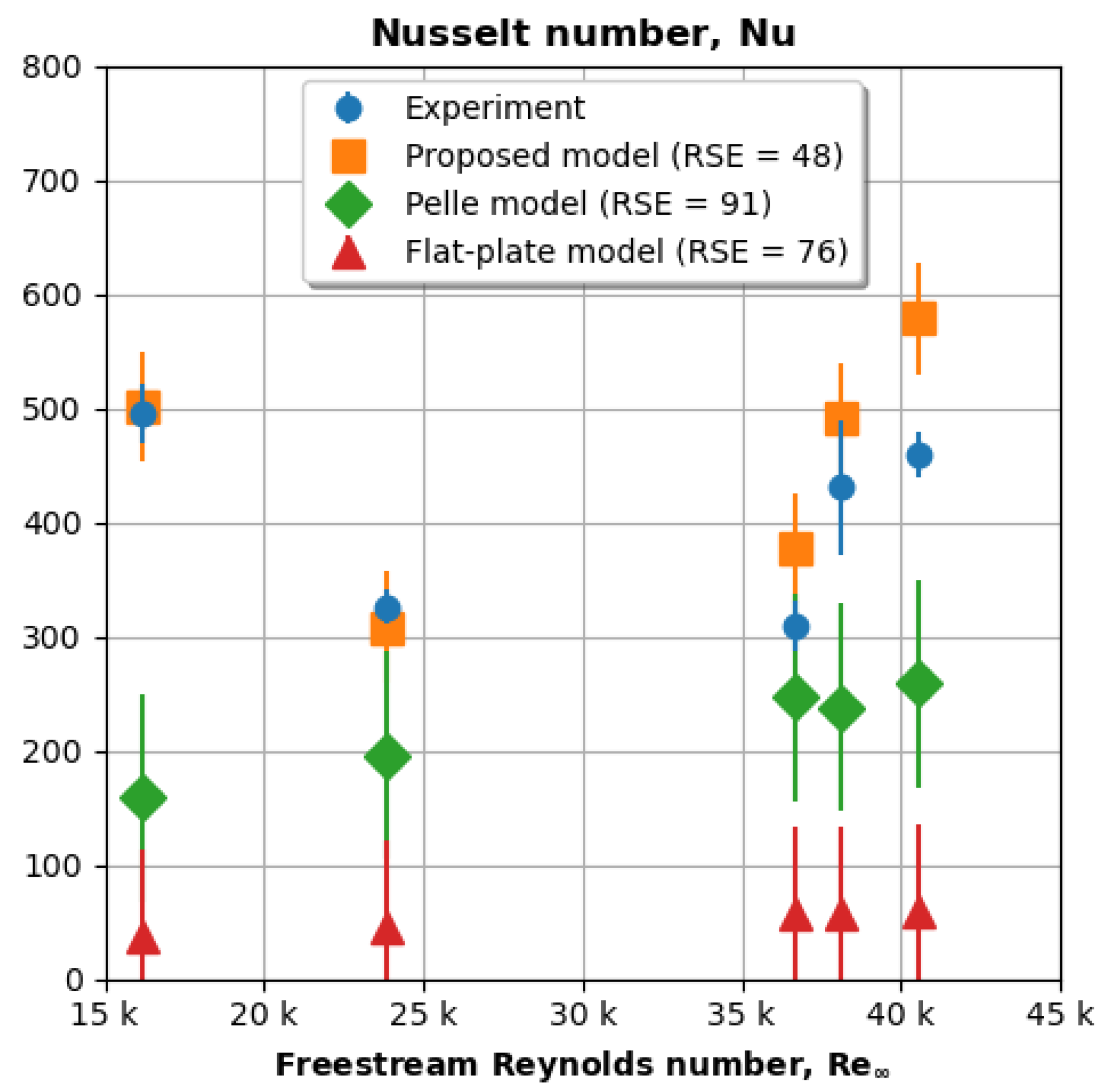
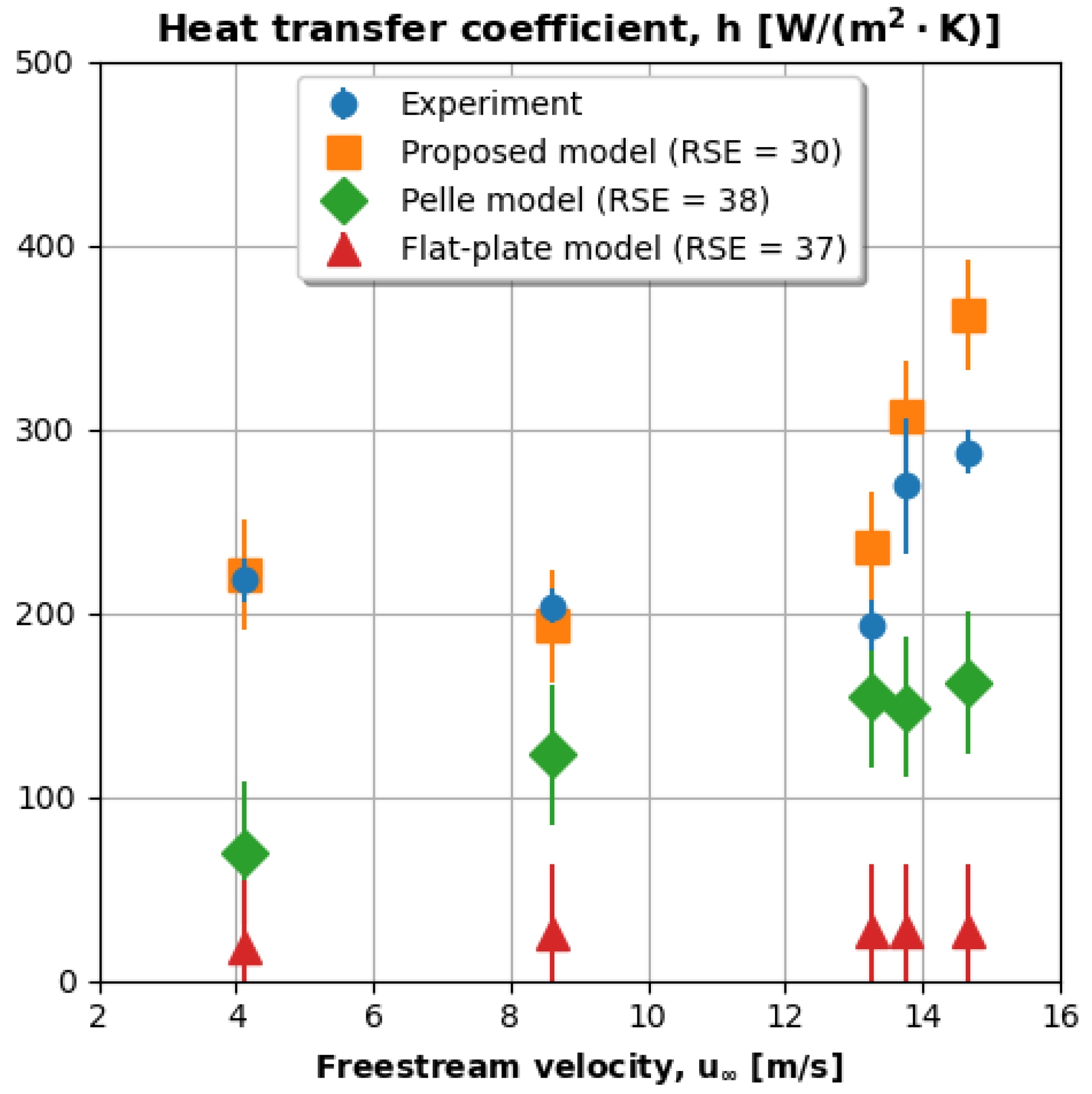
3.2. Nusselt Correlation
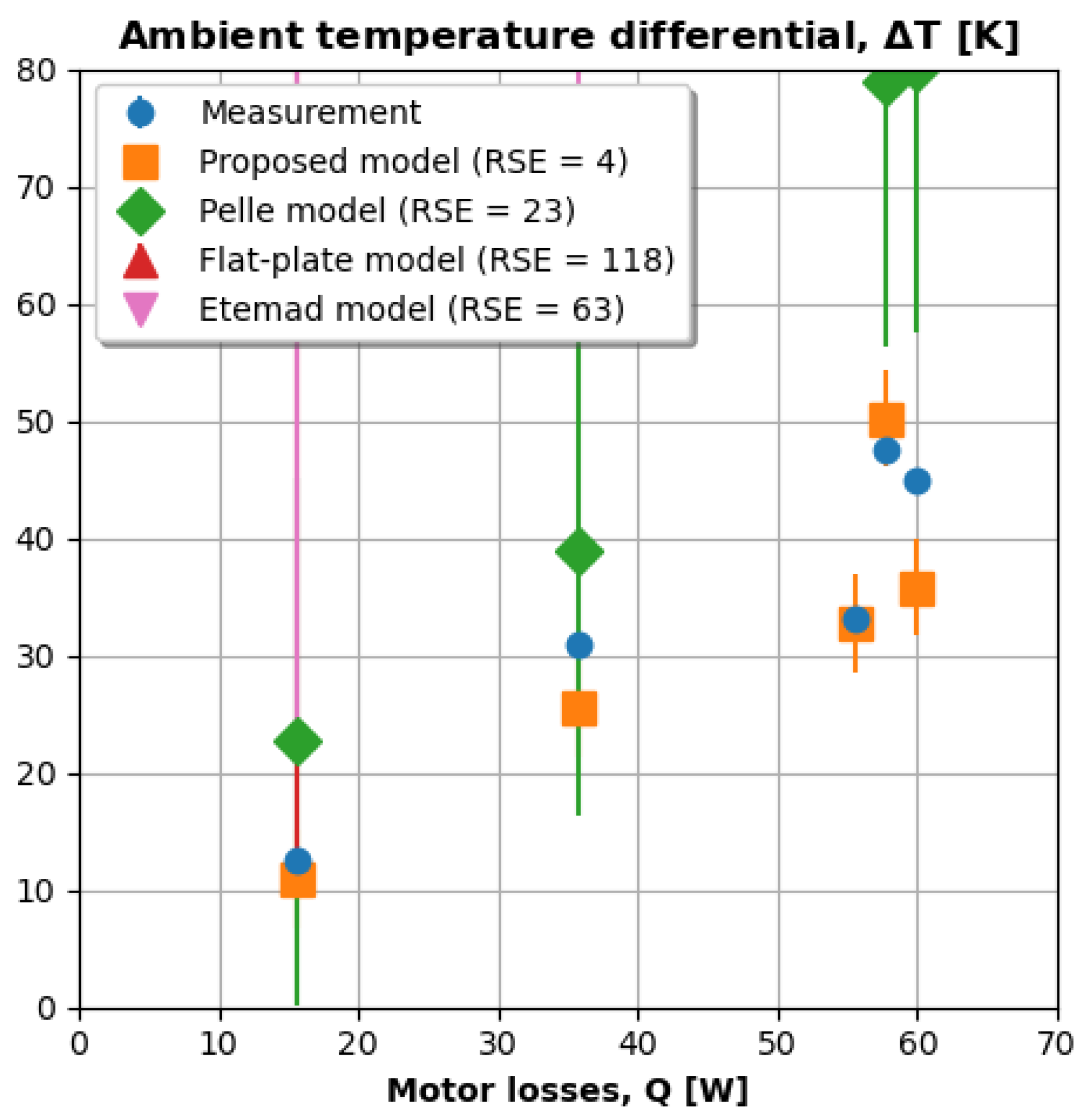
3.3. Validation
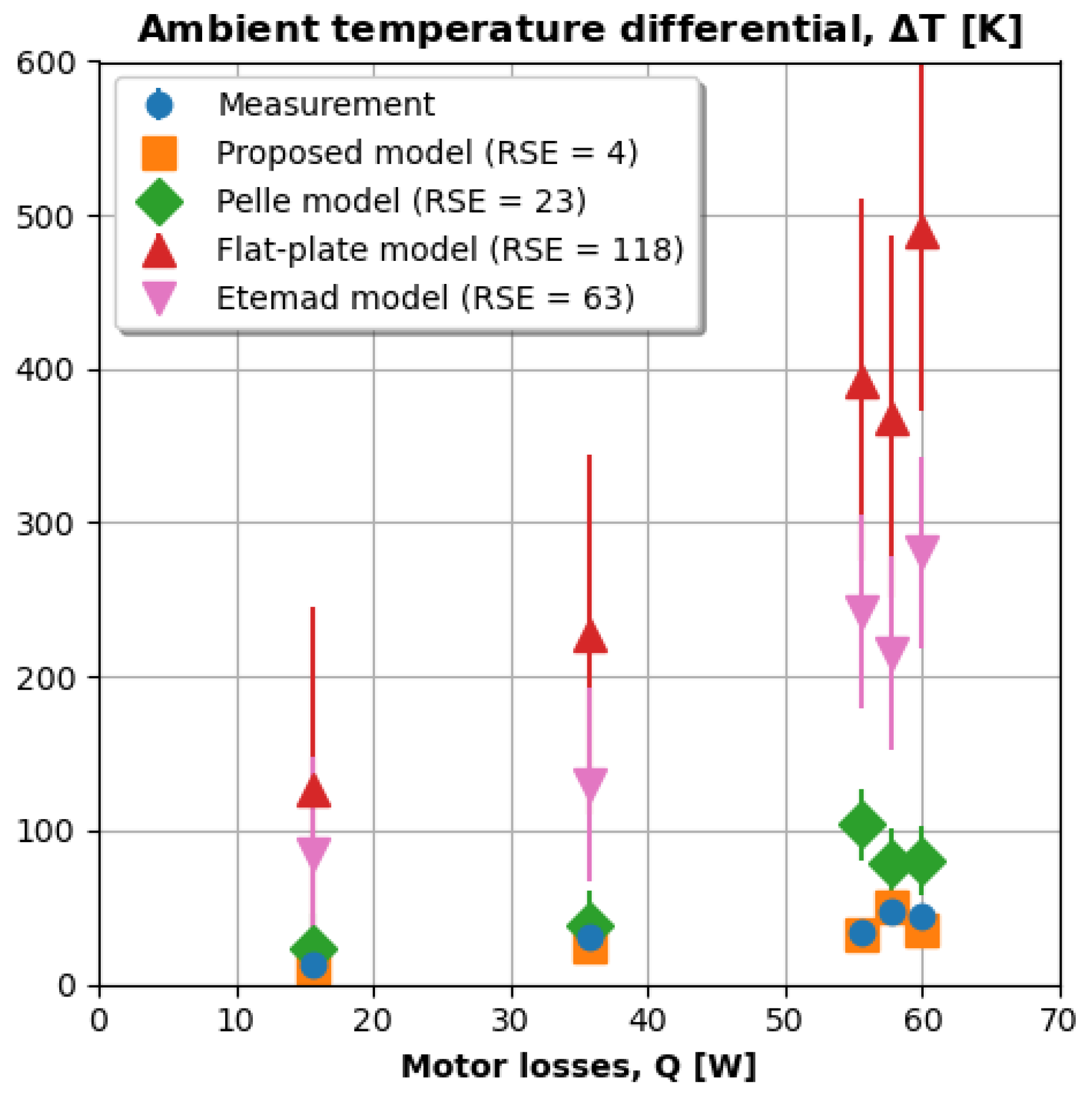
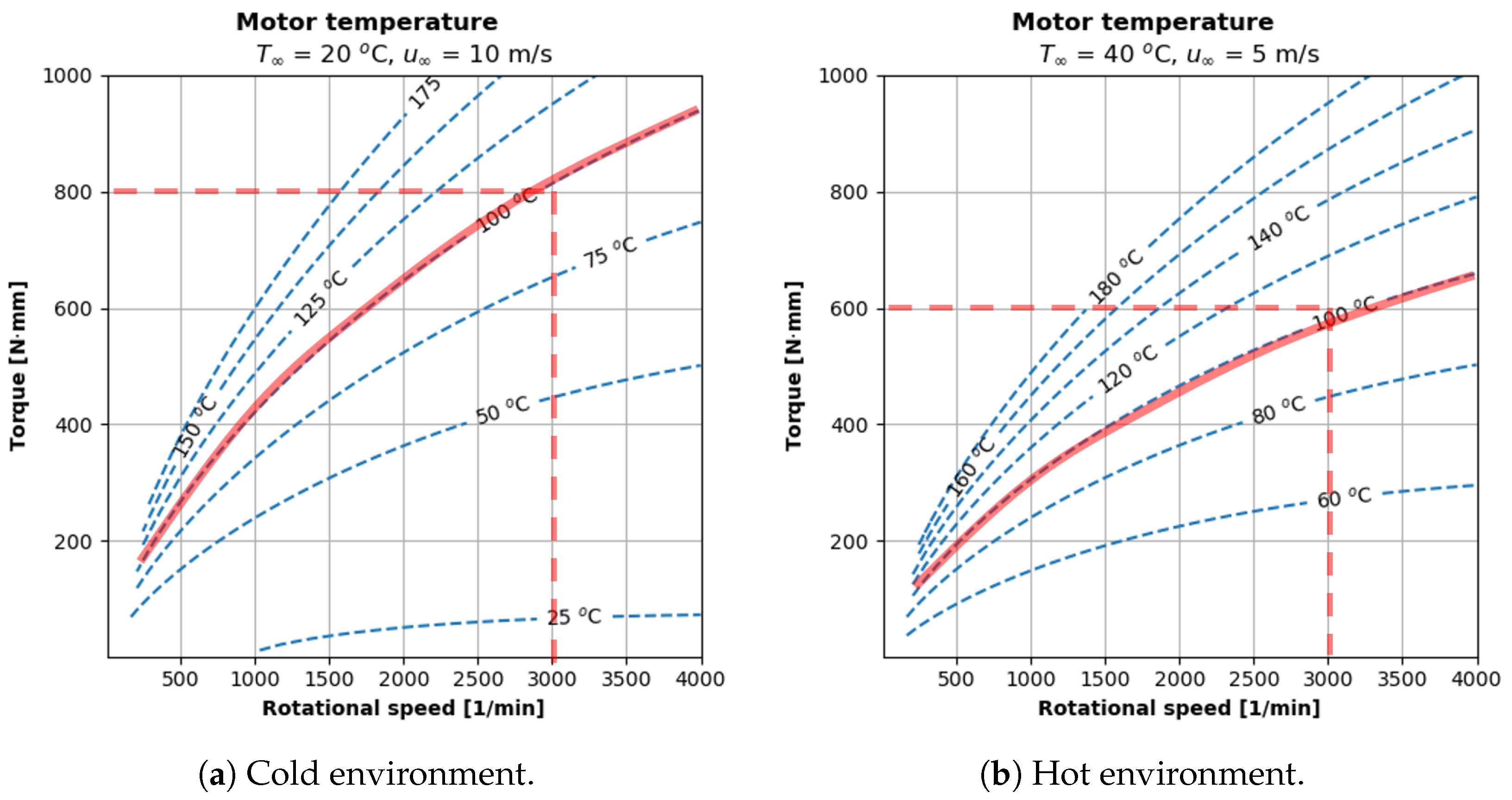
4. Case Study
- Cold environment with 10 m/s airflow at 20 °C;
- Hot environment with 5 m/s airflow at 40 °C.
5. Discussion
5.1. Key Takeaways
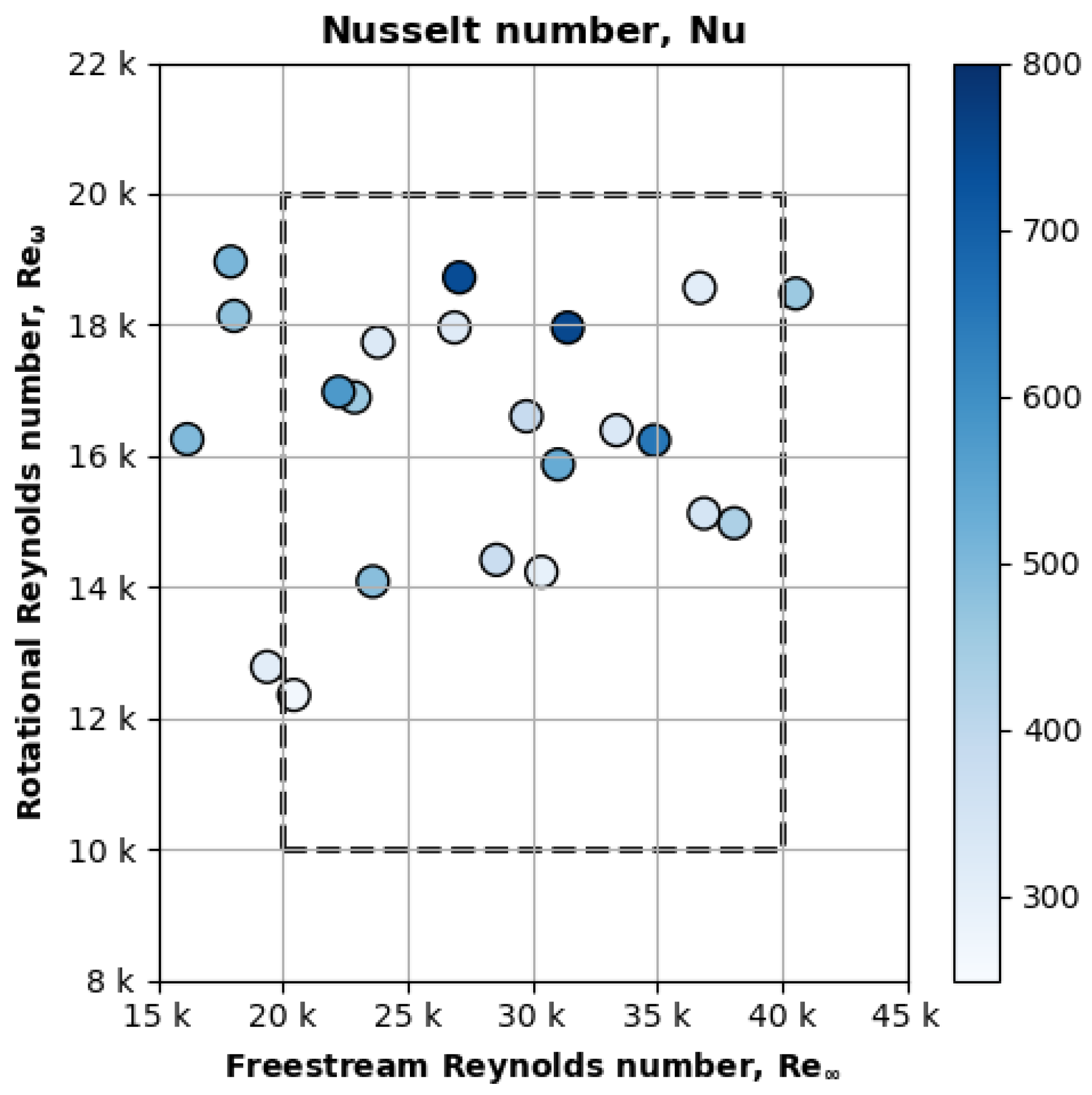
- Each motor’s thermal response is unique. Cooling is sensitive to flow conditions, motor rotational speed, and geometry. For example, Figure 11 shows two cases, both at roughly 25,000, 18,000. One case has a 300 while the other has 700, which is almost a twofold increase. These two points with nearly identical flow conditions vary in aspect ratio, as seen in Figure 8. Therefore, the dimensional heat transfer coefficient of the two motors of different sizes in similar flows will differ;
- Engineers should use Nusselt correlations carefully. Correlations are highly empirical, and a correlation developed for a body in one flow may not be appropriate for the same body in a different flow. Outrunner motors are rotating cylinders, yet Etemad’s correlation for such shapes (Equation (10)) significantly overestimates motor temperature in Figure 16. Engineers should strive to find a correlation that is based on a configuration that closely matches the configuration under consideration;
- Nameplate ratings are variable, not constant. “Continuous-”, “rated-”, and “limit-” torque ratings are not static parameters. A motor’s output torque is limited by its temperature, and the case study showed that the motor temperature varies with torque and rotational speed. Engineers can estimate the rated torque outside the manufacturer’s test points using our thermal and efficiency models;
- Electric motors suffer in hot environments just like combustion engines. In the case study, the motor reached its limit temperature at a lower torque in the hot environment than in the cold environment. The rotational speed was constant in both cases; therefore, the motor lost power in the hot case. This is analogous to how combustion engines develop less power in hot air. Designers can predict these changes using our models;
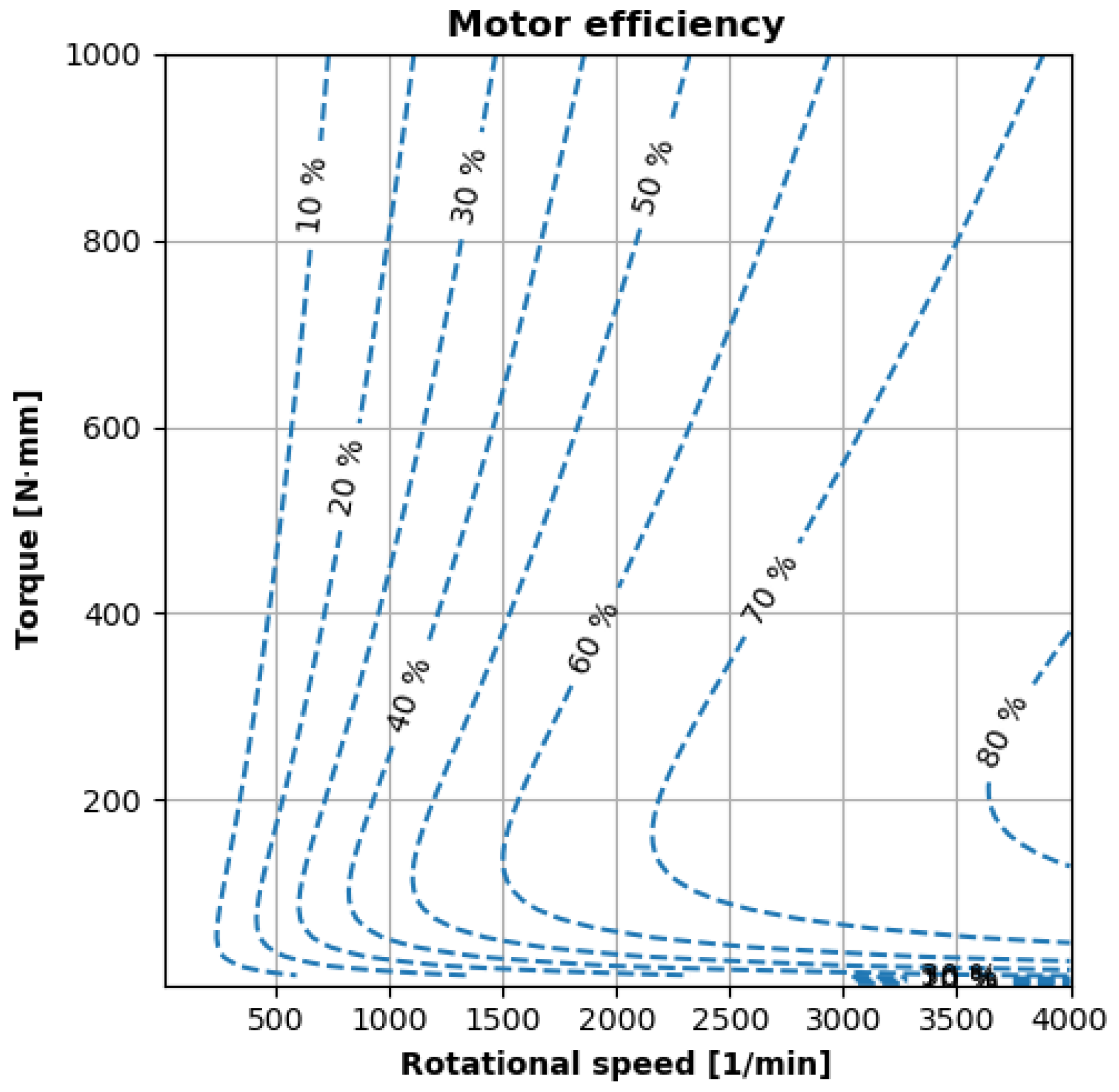
- Gearing can increase efficiency, improve cooling, and reduce weight. The case study showed how low torque and high speed increase efficiency and cooling. Torque is also the motor’s principle size driver [8], so trading torque for speed at a constant power also reduces size. Our thermal models can help engineers predict these improvements at the conceptual design stage.
5.2. Novel Contributions
- Novel methodology to characterize electric motor heat transfer in different freestream and rotational flow conditions. The approach allowed us to study motors of various sizes and match the freestream Reynolds and rotational Reynolds numbers. Our approach could not match heat flux across cases like traditional heat transfer studies. Nonetheless, the tested conditions reflect realistic loads and flow regimes and improve the literature. Reference [2] used a spinning cylinder containing a heating coil to simulate a motor. The study approximated motor losses from the current delivered to the coils. Our approach uses actual motors under load and directly measures the motor’s operating losses. Practitioners can use our methodology to test powertrains before flight tests and characterize the powertrain’s temperature response. Researchers can use the method to replicate and validate our results or develop new correlations;
- Novel experimental data on outrunner brushless DC motors’ heat transfer. We characterized the steady-state heat transfer of four different motors with unique aspect ratios under Reynolds-matched flows ( ranging from 20,000 to 40,000, ranging from 10,000 to 20,000). Our data improve on the literature, which only contains studies on single motors. For example, reference [43] conducted detailed experimental Particle Image Velocimetry (PIV) and computational fluid studies on the thermal and flow processes of a single fixed-size brushless DC motor. However, these results are of little use for conceptual designers who seek heat transfer data for a broad range of devices, which could vary in size and aspect ratio. Our experimental data sacrifice some fidelity for robustness to fill this gap in the literature. Practitioners can interpolate within our data and extrapolate our data’s trends to estimate the heat transfer of their motors without having to conduct any experiments. Researchers can use our data to validate other heat transfer models or evaluate the utility of other literature correlations;
- Novel Nusselt number correlations for the external heat transfer of brushless DC motors in rotational and axial (freestream) flow. Nusselt correlations are highly empirical, and literature correlations examine the internal heat transfer of brushless DC motors. For example, Ref. [30] derives a Nusselt correlation for heat transfer across the internal airgap of brushless DC motors of varying inner and outer radii. The correlation is derived from computational data, which studied a wide range of Reynolds numbers ( ranging from 1750 to 40,000 and ranging from 1750 to 35,000). Conceptual designers cannot apply this correlation to study a motor’s steady-state response since the airflow inside a brushless DC motor differs from the external airflow, which dissipates the motor’s heat to the environment. Internally, the flow regime is like Taylor–Couette flow: an external cylinder (rotor) spinning around an internal cylinder (stator) with some axial flow. Externally, the flow regime is a single cylinder spinning inside a more pronounced axial (freestream) flow. We empirically derived a new correlation to address this gap in the literature. We then applied this correlation in two case studies to solve a problem faced by conceptual designers: predicting a motor’s continuous torque. Practitioners and researchers can use our correlation to estimate heat transfer for similar motors. Moreover, these users need not be limited to aerospace engineers. Heat transfer is critical to rating motors, and motor designers could benefit from a reliable and validated correlation to predict heat transfer.
5.3. Future Work
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| A | Area [m2] |
| Aspect ratio | |
| D | Diameter [m] |
| d | Throttle setting (duty ratio) |
| h | Convective heat transfer coefficient [W/(m2·K)] |
| Motor no-load current [A] | |
| i | Motor index (1–4) |
| j | Test-case index (1–10) |
| , | Air (fluid) thermal conductivity [W/(m·K)] |
| Motor torque constant [N·m/A] | |
| L | Length [m] |
| M | Torque [N·m] |
| m | Mass [kg] |
| Nusselt number | |
| P | Power [W] |
| Prandtl number | |
| Q | Heat or losses [W] |
| q | Heat flux [W/m2] |
| Reynolds number | |
| Motor winding resistance [] | |
| T | Temperature [°C or K] |
| U | Volume [m3] |
| DC voltage [V] | |
| Efficiency | |
| Dynamic viscosity [m2/s] | |
| Uncertainty [per unit] | |
| Electromagnetic loading [Pa] | |
| Rotational speed [min−1] |
References
- Anderson, K.R.; Lin, J.; McNamara, C.; Magri, V. CFD study of forced air cooling and windage losses in a high speed electric motor. J. Electron. Cool. Therm. Control 2015, 5, 27. [Google Scholar] [CrossRef]
- Markovic, M.; Saunders, L.; Perriard, Y. Determination of the thermal convection coefficient for a small electric motor. In Proceedings of the Conference Record of the 2006 IEEE Industry Applications Conference Forty-first IAS Annual Meeting, IEEE, Tampa, FL, USA, 8–12 October 2006; Volume 1, pp. 58–61. [Google Scholar] [CrossRef]
- Rahman, S.; Hasanpour, S.; Khan, I.; Toliyat, H.A.; Hussain, H.A. Power Dense High-Speed Motor-Generator System for Powering Futuristic Unmanned Aircraft System (UAS). In Proceedings of the IECON 2022—48th Annual Conference of the IEEE Industrial Electronics Society, Brussels, Belgium, 17–20 October 2022; pp. 1–6. [Google Scholar] [CrossRef]
- Hughes, A.; Drury, W. Electric Motors and Drives: Fundamentals, Types and Applications; Newnes: Oxford, UK, 2013. [Google Scholar] [CrossRef]
- Mohan, N. Electric Machines and Drives, 1st ed.; Wiley: Hoboken, NJ, USA, 2012; Available online: https://bit.ly/3xLA98A (accessed on 20 April 2024).
- Mevey, J. Sensorless Field Oriented Control of Brushless Permanent Magnet Synchronous Motors. Master’s Thesis, Kansas State University, Manhattan, KS, USA, 2009. Available online: http://hdl.handle.net/2097/1507 (accessed on 20 April 2024).
- Hendershot, J.; Miller, T. Design of Brushless Permanent-Magnet Machines; Oxford Academic: Oxford, UK, 2010. [Google Scholar] [CrossRef]
- Hanselman, D. Brushless Permanent Magnet Motor Design; The Writers’ Collective: Cranston, RI, USA, 2003; Available online: https://digitalcommons.library.umaine.edu/fac_monographs/231 (accessed on 20 April 2024).
- Dilevrano, G.; Ragazzo, P.; Ferrari, S.; Pellegrino, G.; Burress, T. Magnetic, thermal and structural scaling of synchronous machines. In Proceedings of the 2022 IEEE Energy Conversion Congress and Exposition (ECCE), IEEE, Detroit, MI, USA, 9–13 October 2022; pp. 1–8. [Google Scholar] [CrossRef]
- Saemi, F.; Benedict, M. Flight-Validated Electric Powertrain Efficiency Models for Small UASs. Aerospace 2023, 11, 16. [Google Scholar] [CrossRef]
- Motor Design Ltd. Motor-CAD v14.1 Manual (11/01/2021); Motor Design Ltd.: Wrexham, UK, 2021; Available online: https://bit.ly/3w5bknB (accessed on 20 April 2024).
- Koegler, L. KDE 10218XF-105 Brushless DC Motor; KDE Direct, LLC.: Bend, OR, USA, 2024; Available online: https://bit.ly/412Ggjw (accessed on 20 April 2024).
- Scorpion Power System, Ltd. Scorpion M-3011-760kv; Scorpion Power System, Ltd.: Hong Kong, China, 2024; Available online: https://bit.ly/3SKrppX (accessed on 20 April 2024).
- Vegh, J.M.; Botero, E.; Clark, M.; Smart, J.; Alonso, J.J. Current capabilities and challenges of NDARC and SUAVE for eVTOL aircraft design and analysis. In Proceedings of the 2019 AIAA/IEEE Electric Aircraft Technologies Symposium (EATS), IEEE, Indianapolis, IN, USA, 22–24 August 2019; pp. 1–19. [Google Scholar] [CrossRef]
- Johnson, W.; Silva, C.; Solis, E. Concept Vehicles for VTOL Air Taxi Operations; Technical Report ARC-E-DAA-TN50731; National Aeronautics and Space Administration: Washington, DC, USA, 2018. Available online: https://ntrs.nasa.gov/citations/20180003381 (accessed on 20 April 2024)Technical Report ARC-E-DAA-TN50731.
- Ugwueze, O.; Statheros, T.; Horri, N.; Bromfield, M.A.; Simo, J. An Efficient and Robust Sizing Method for eVTOL Aircraft Configurations in Conceptual Design. Aerospace 2023, 10, 311. [Google Scholar] [CrossRef]
- Sridharan, A.; Govindarajan, B.; Chopra, I. A scalability study of the multirotor biplane tailsitter using conceptual sizing. J. Am. Helicopter Soc. 2020, 65, 1–18. [Google Scholar] [CrossRef]
- Lienhard, V.J.H.; Lienhard, J.H., IV. A Heat Transfer Textbook, 6th ed.; Phlogiston Press: Cambridge, MA, USA, 2024. [Google Scholar]
- Hadi, K.; Setiyoso, A.; Hindersah, H. Heat Transfer Model on 120 kW BLDC’s Motor Integrated Controller. In Proceedings of the 2022 7th International Conference on Electric Vehicular Technology (ICEVT), IEEE, Bali, Indonesia, 14–16 September 2022; pp. 181–185. [Google Scholar] [CrossRef]
- Cengel, Y.; Ghajar, A. Heat and Mass Transfer, 5th ed.; McGraw Hill Education: New York, NY, USA, 2014; Available online: https://bit.ly/4aPsJ2Y (accessed on 20 April 2024).
- Dennis, S.; Smith, N. Forced convection from a heated flat plate. J. Fluid Mech. 1966, 24, 509–519. [Google Scholar] [CrossRef]
- Grosh, R.; Cess, R. Heat transfer to fluids with low Prandtl numbers for flow across plates and cylinders of various cross section. Trans. Am. Soc. Mech. Eng. 1958, 80, 667–676. [Google Scholar] [CrossRef]
- Etemad, G.A. Free-Convection Heat Transfer From a Rotating Horizontal Cylinder to Ambient Air With Interferometric Study of Flow. J. Fluids Eng. 1955, 77, 1283–1289. [Google Scholar] [CrossRef]
- Pellé, J.; Harmand, S. Heat transfer study in a discoidal system: The influence of an impinging jet and rotation. Exp. Heat Transf. 2007, 20, 337–358. [Google Scholar] [CrossRef]
- Harmand, S.; Pellé, J.; Poncet, S.; Shevchuk, I.V. Review of fluid flow and convective heat transfer within rotating disk cavities with impinging jet. Int. J. Therm. Sci. 2013, 67, 1–30. [Google Scholar] [CrossRef]
- Liang, D.; Zhu, Z.; Feng, J.; Guo, S.; Li, Y.; Wu, J.; Zhao, A. Influence of critical parameters in lumped-parameter thermal models for electrical machines. In Proceedings of the 2019 22nd International Conference on Electrical Machines and Systems (ICEMS), IEEE, Harbin, China, 11–14 August 2019; pp. 1–6. [Google Scholar] [CrossRef]
- Bilgin, B.; Liang, J.; Terzic, M.V.; Dong, J.; Rodriguez, R.; Trickett, E.; Emadi, A. Modeling and analysis of electric motors: State-of-the-art review. IEEE Trans. Transp. Electrif. 2019, 5, 602–617. [Google Scholar] [CrossRef]
- Demetriades, G.D.; De La Parra, H.Z.; Andersson, E.; Olsson, H. A real-time thermal model of a permanent-magnet synchronous motor. IEEE Trans. Power Electron. 2009, 25, 463–474. [Google Scholar] [CrossRef]
- Howey, D.A.; Childs, P.R.; Holmes, A.S. Air-gap convection in rotating electrical machines. IEEE Trans. Ind. Electron. 2010, 59, 1367–1375. [Google Scholar] [CrossRef]
- Teamah, A.M.; Hamed, M.S. Cooling of a synchronous electric motor using an axial air flow. Int. J. 2023, 20, 100525. [Google Scholar] [CrossRef]
- Fénot, M.; Dorignac, E.; Giret, A.; Lalizel, G. Convective heat transfer in the entry region of an annular channel with slotted rotating inner cylinder. Appl. Therm. Eng. 2013, 54, 345–358. [Google Scholar] [CrossRef]
- Childs, P.R.; Turner, A. Heat’Transfer on the Surface of a Cylinder Rotating in an Annulus at High Axial and Rotational Reynolds Numbers. In Proceedings of the International Heat Transfer Conference Digital Library, Brighton, UK, 14–18 August 1994; Begel House Inc.: Danbury, CT, USA, 1994. [Google Scholar] [CrossRef]
- Wu, P.S.; Hsieh, M.F.; Cai, W.L.; Liu, J.H.; Huang, Y.T.; Caceres, J.F.; Chang, S.W. Heat transfer and thermal management of interior permanent magnet synchronous electric motor. Inventions 2019, 4, 69. [Google Scholar] [CrossRef]
- Cavazzuti, M.; Gaspari, G.; Pasquale, S.; Stalio, E. Thermal management of a Formula E electric motor: Analysis and optimization. Appl. Therm. Eng. 2019, 157, 113733. [Google Scholar] [CrossRef]
- Castle Creations, Inc. Phoenix Edge HV 60 AMP ESC, 12S/50.4V, no BEC; Castle Creations, Inc.: Olathe, KS, USA, 2024; Available online: https://bit.ly/4aZ4VsS (accessed on 20 April 2024).
- Koegler, L. KDE4215XF-465 Brushless DC Motor; KDE Direct, LLC.: Bend, OR, USA, 2024; Available online: https://bit.ly/4b6Hcar (accessed on 20 April 2024).
- Koegler, L. KDE5215XF-330 Brushless DC Motor; KDE Direct, LLC.: Bend, OR, USA, 2024; Available online: https://bit.ly/44ayoy3 (accessed on 20 April 2024).
- Koegler, L. KDE3510XF-475 Brushless DC Motor; KDE Direct, LLC.: Bend, OR, USA, 2024; Available online: https://bit.ly/3U73zWi (accessed on 20 April 2024).
- Koegler, L. KDE3520XF-400 Brushless DC Motor; KDE Direct, LLC.: Bend, OR, USA, 2024; Available online: https://bit.ly/3xSG6R2 (accessed on 20 April 2024).
- Bergman, T.L.; Lavine, A.S.; Incropera, F.P.; DeWitt, D.P. Introduction to Heat Transfer; Wiley: Hoboken, NJ, USA, 2011; Available online: https://bit.ly/44cWWWX (accessed on 20 April 2024).
- Lebigot, E. Uncertainties: A Python Package for Calculations with Uncertainties. Available online: https://bit.ly/3uzum4T (accessed on 20 April 2024).
- Guo, Y.; Liu, L.; Ba, X.; Lu, H.; Lei, G.; Yin, W.; Zhu, J. Designing High-Power-Density Electric Motors for Electric Vehicles with Advanced Magnetic Materials. World Electr. Veh. J. 2023, 14, 114. [Google Scholar] [CrossRef]
- Melka, B.; Smolka, J.; Hetmanczyk, J.; Bulinski, Z.; Makiela, D.; Ryfa, A. Experimentally validated numerical model of thermal and flow processes within the permanent magnet brushless direct current motor. Int. J. Therm. Sci. 2018, 130, 406–415. [Google Scholar] [CrossRef]

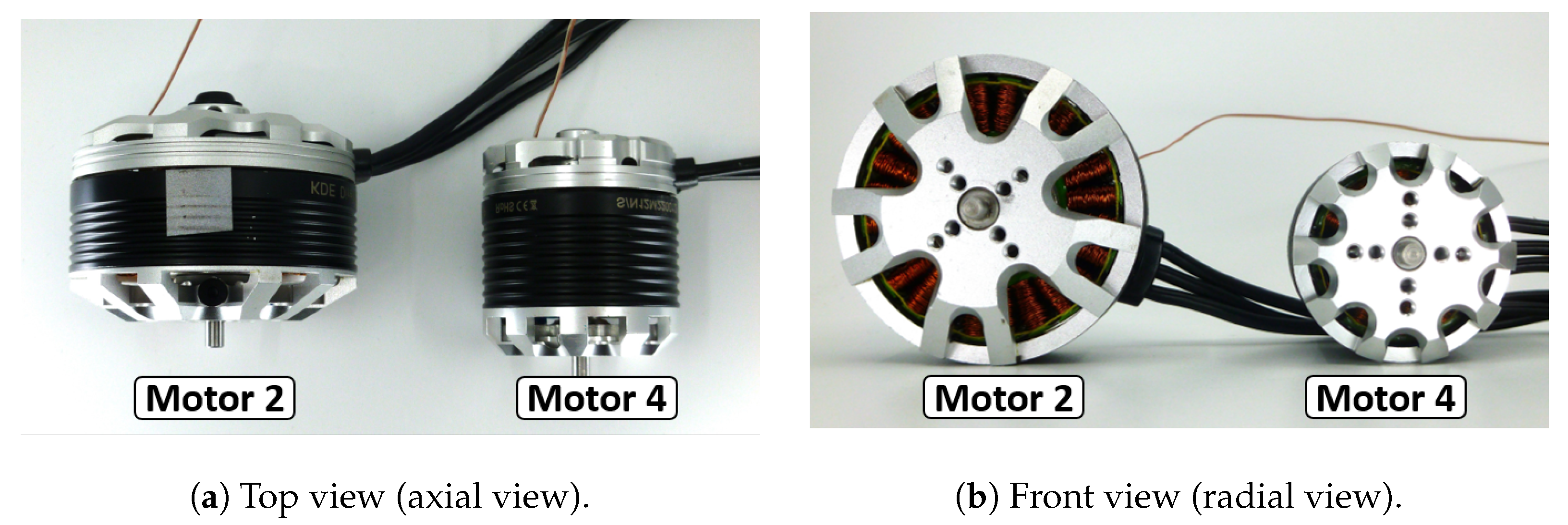



| Motor | 1 | 2 | 3 | 4 | ||
|---|---|---|---|---|---|---|
| Diameter | D | [mm] | 48 | 60 | 42 | 42 |
| Length | L | [mm] | 36 | 41 | 35 | 45 |
| Lateral surface area | [cm2] | 55 | 77 | 46 | 60 | |
| Aspect ratio | 1.3 | 1.5 | 1.2 | 0.9 | ||
| Full specifications | Ref. [36] | Ref. [37] | Ref. [38] | Ref. [39] |
| Torque | 0.4 N·mm | |
| Rotational speed | 2 rad/s | |
| Electric power | 0.5 W | |
| Temperature differential | 1 K |
| Torque constant | 20.5 N·mm/A | |
| Winding resistance | 52 m | |
| No-load current | 0.7 A | |
| DC voltage | 16 V | |
| Diameter | D | 48.2 mm |
| Length | L | 36.0 mm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Saemi, F.; Whitson, A.; Benedict, M. Heat Transfer Models and Measurements of Brushless DC Motors for Small UASs. Aerospace 2024, 11, 401. https://doi.org/10.3390/aerospace11050401
Saemi F, Whitson A, Benedict M. Heat Transfer Models and Measurements of Brushless DC Motors for Small UASs. Aerospace. 2024; 11(5):401. https://doi.org/10.3390/aerospace11050401
Chicago/Turabian StyleSaemi, Farid, Annalaine Whitson, and Moble Benedict. 2024. "Heat Transfer Models and Measurements of Brushless DC Motors for Small UASs" Aerospace 11, no. 5: 401. https://doi.org/10.3390/aerospace11050401
APA StyleSaemi, F., Whitson, A., & Benedict, M. (2024). Heat Transfer Models and Measurements of Brushless DC Motors for Small UASs. Aerospace, 11(5), 401. https://doi.org/10.3390/aerospace11050401








