Electric Sail Test Cube–Lunar Nanospacecraft, ESTCube-LuNa: Solar Wind Propulsion Demonstration Mission Concept
Abstract
1. Introduction
1.1. Electric Solar Wind Sail and Plasma Brake in the Context of Propellantless Propulsion
1.2. E-Sail and Plasma Brake Demonstration Missions
1.3. Interplanetary and Lunar Nanospacecraft
1.4. ESTCube-Luna Consortium and Paper Structure
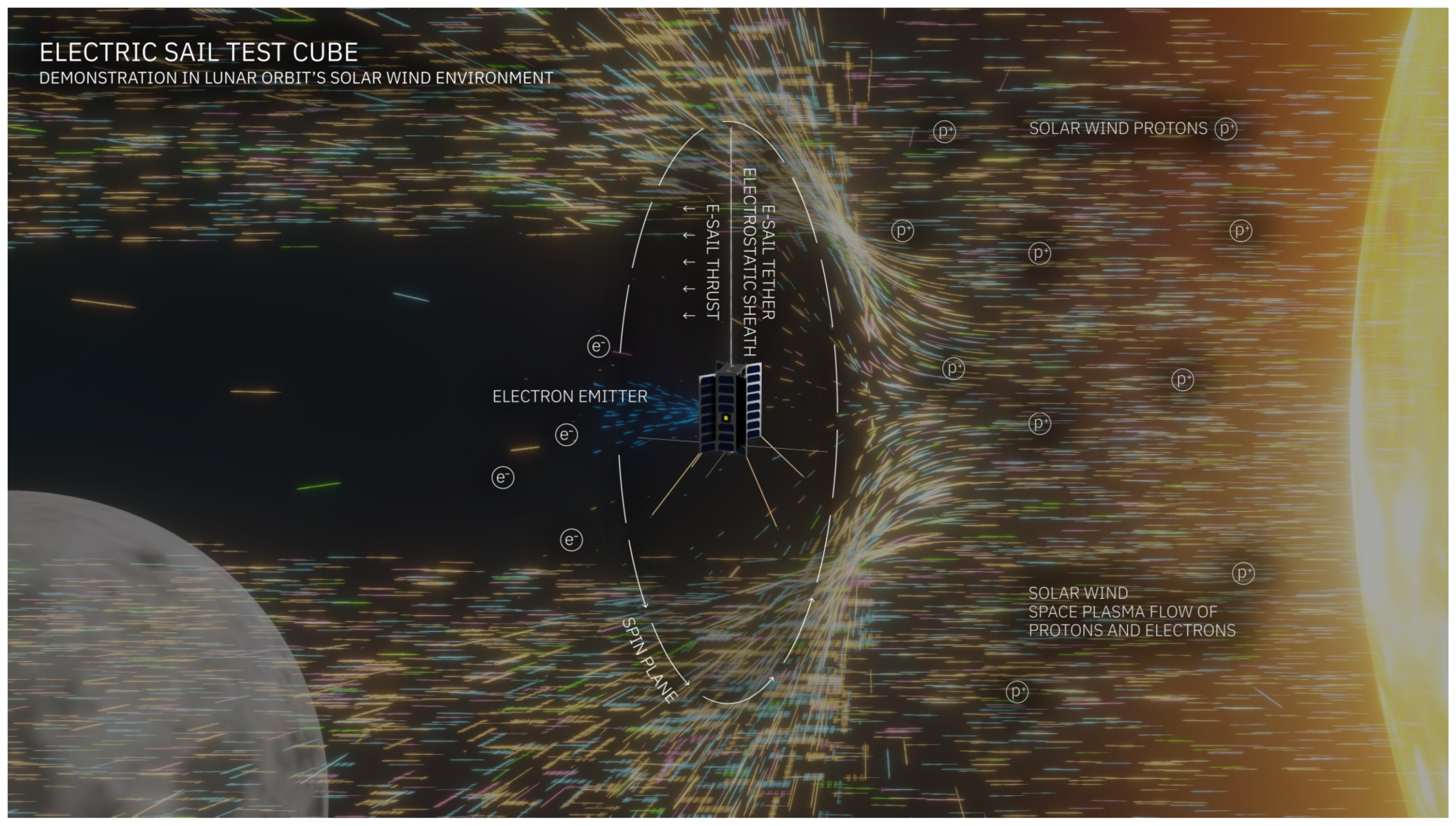
2. E-Sail Experiment Design for Solar Wind in Lunar Orbit
2.1. ESTCube-Luna E-sail Experiment Requirements
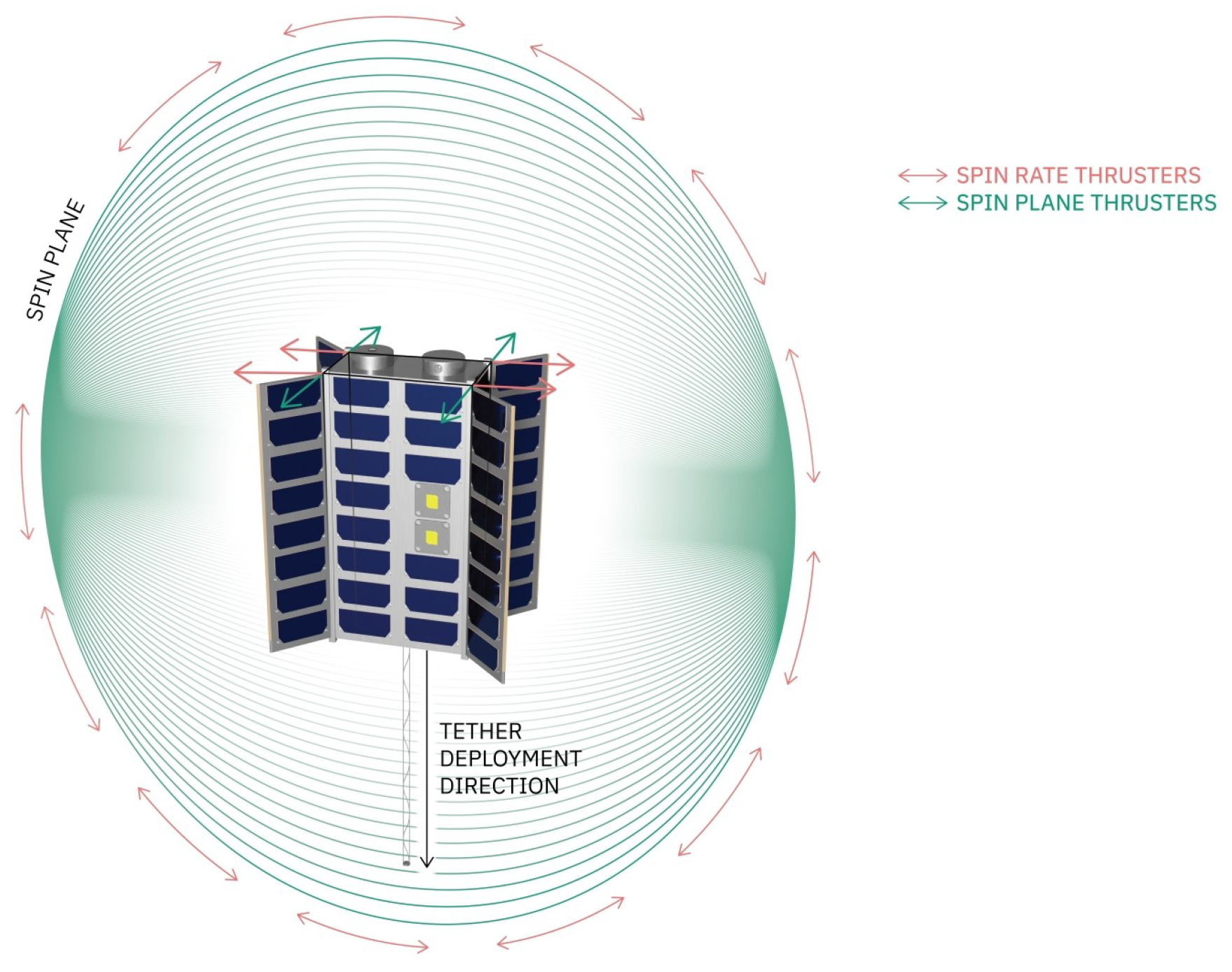
2.2. Spin Rate Modification with E-Sail Torque
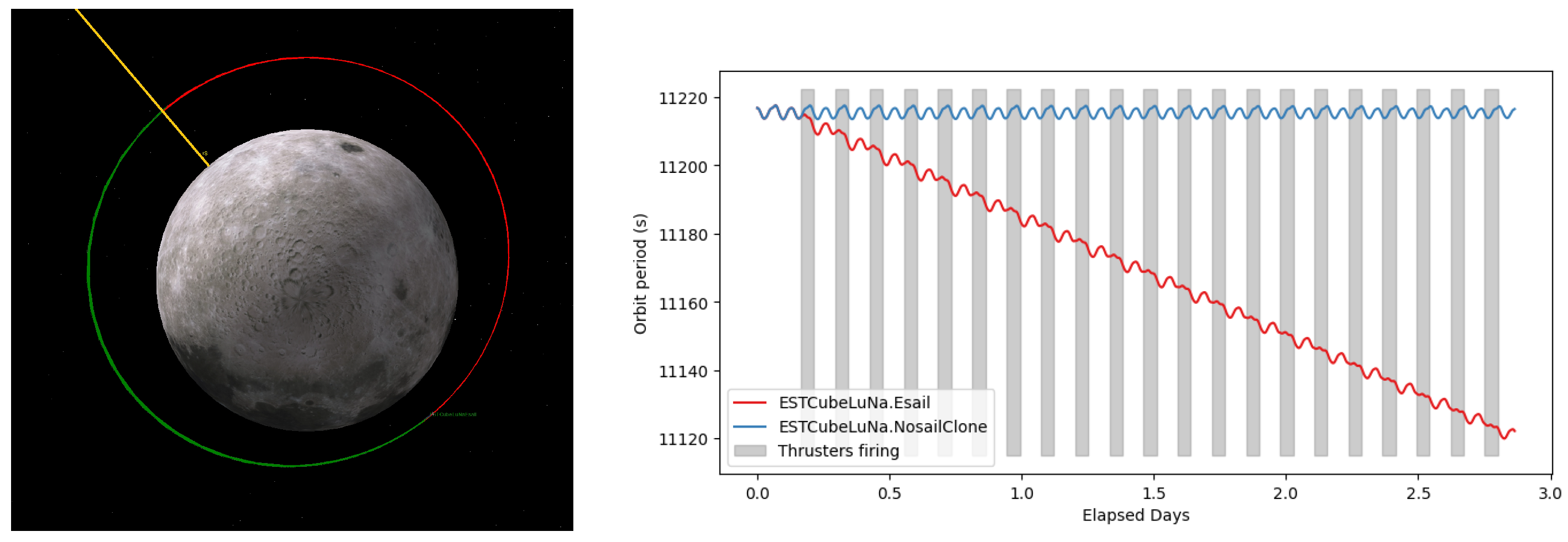
2.3. Orbit Modification with E-Sail Thrust
2.4. E-Sail Tether Deflection
3. ESTCube-Luna E-Sail Payload and Experiment Operations
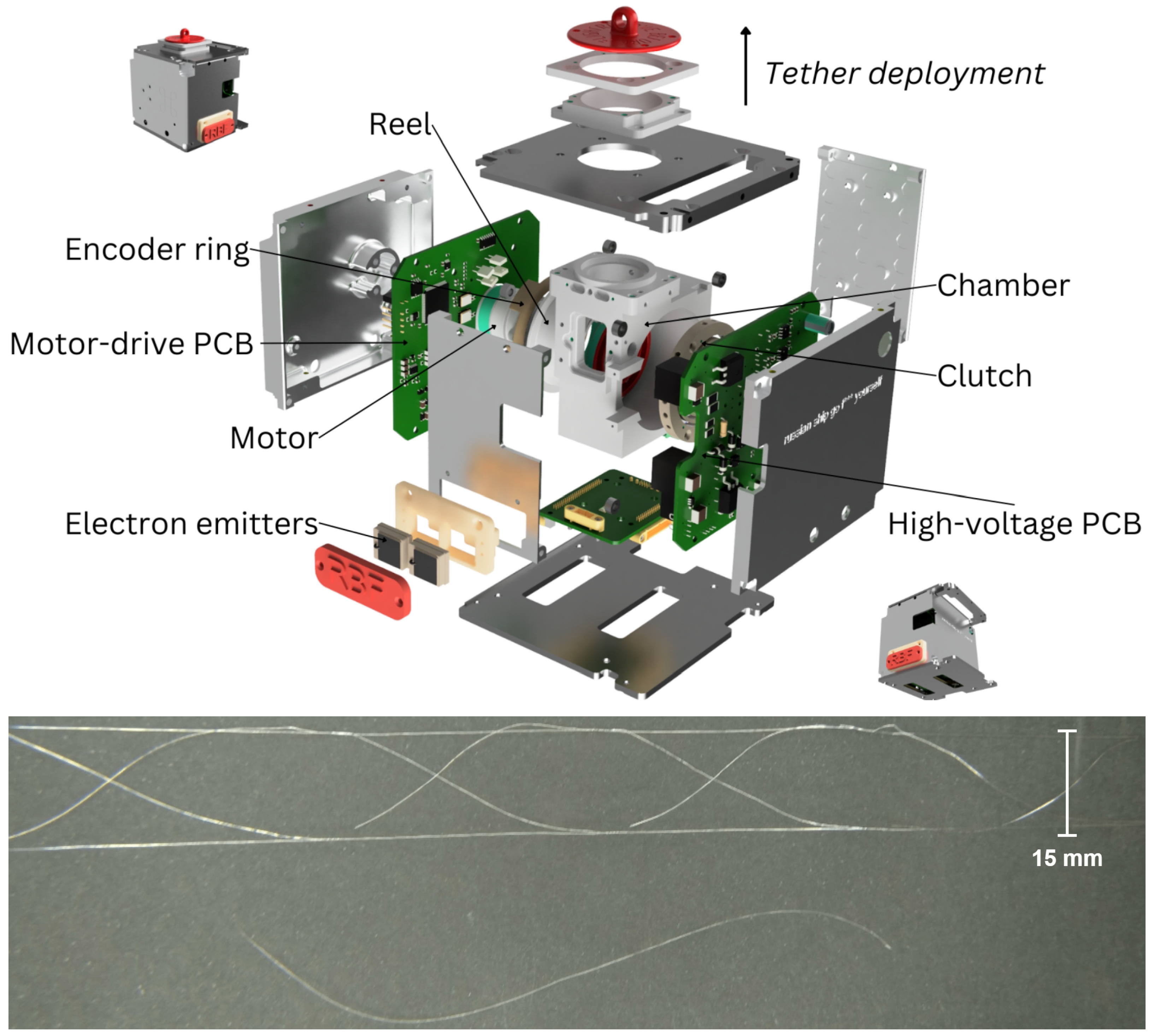
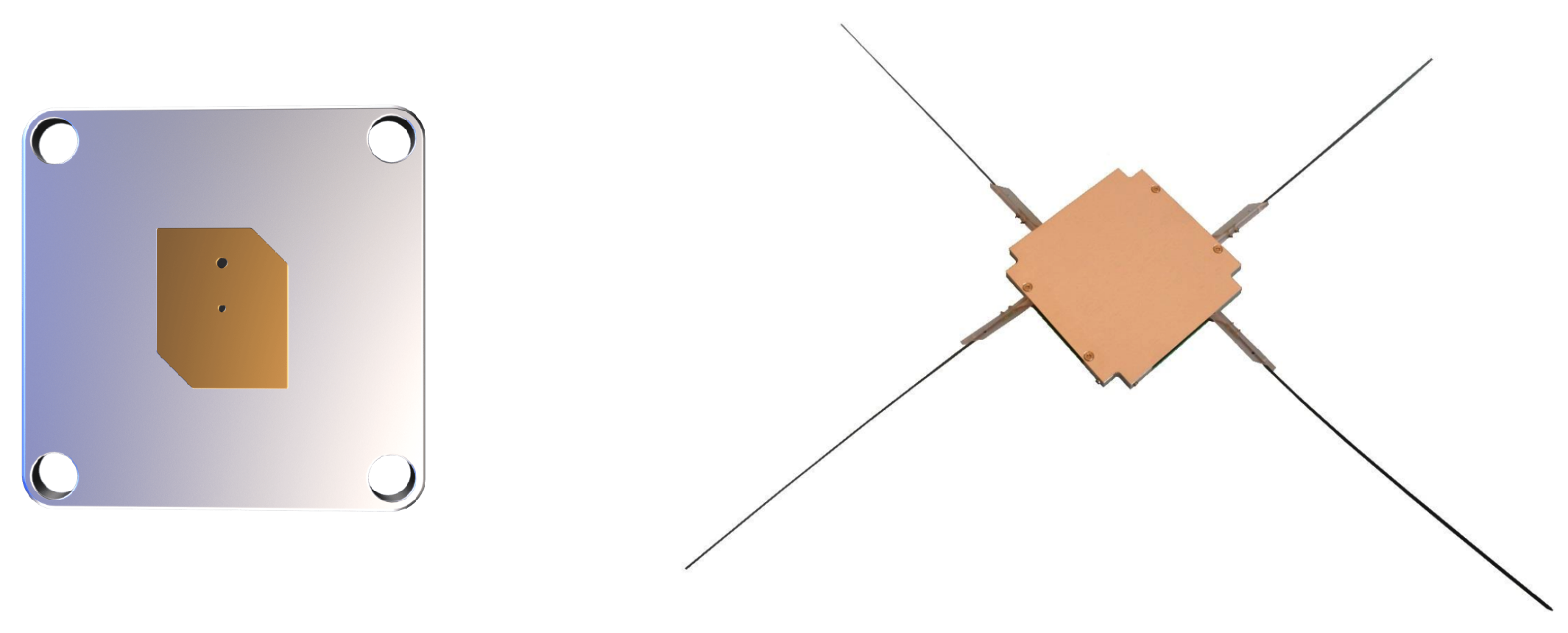
3.1. E-Sail Hardware
3.2. E-Sail Deployment
3.3. E-Sail Environment Characterisation
4. ESTCube-Luna Stretch Goal: Lunar Escape Manoeuvre
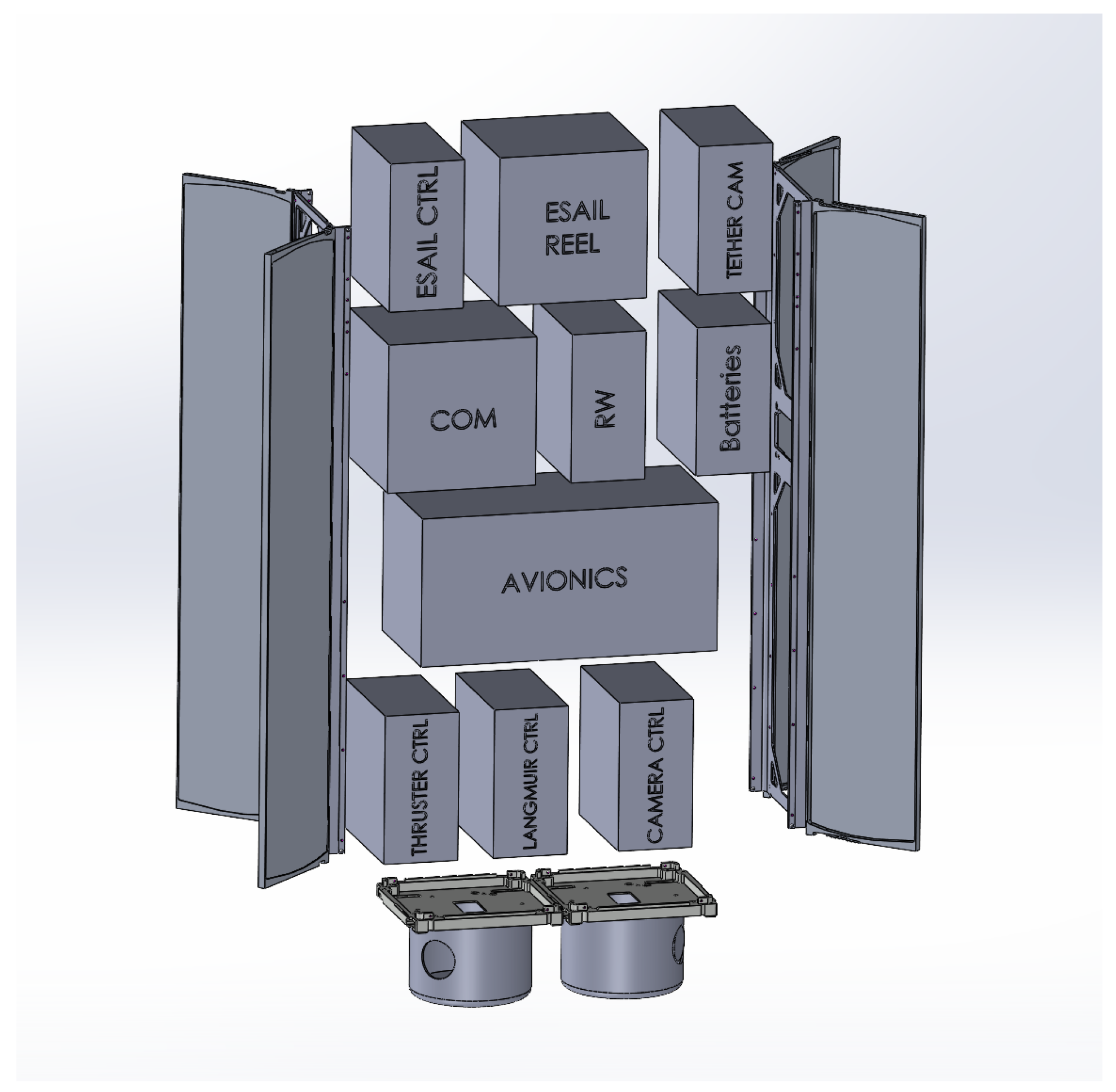
5. ESTCube-Luna Preliminary Mission Requirements and Platform Design Solutions
5.1. System Engineering
- Launch and operate in lunar orbit.
- Operate DSN-free by developing alternative solutions for communications and navigation without the access to space agency DSNs.
- Withstand three years of operations in the lunar orbit environment: solar and cosmic particles, radiation and large temperature fluctuations between sunlit and shadowed sides.
- Perform and monitor the spin manoeuvres and E-sail tether deployment using reaction wheels, attitude thrusters and sensors as well as the tether camera.
- Operate and characterise the E-sail performance by charging the tether and measuring changes in orbit, spin rate, tether deflection angle and surrounding plasma environment.
- Ensure sufficient power production during E-sail tether deployment and operations.
- Estimate the ESTCube-LuNa position using optical navigation and triangulation of the Moon, the Earth and the Sun.
- Estimate the ESTCube-LuNa attitude by combining the navigation solution with Sun sensors, inertial measurement unit (IMU) and star tracker (the tether camera can double as a star tracker when the spin rate is low, such as after tether deployment).
- Transmit telemetry and commands via a wide-beam antenna.
- Transmit data and perform ranging with narrow-beam antennas.
5.2. Electrical Power System (EPS)
5.3. On-Board Computer (OBC)
5.4. Communication (COM)
5.4.1. Low-Speed communication (LSCOM)
5.4.2. High-Speed Communication (HSCOM)
5.5. Attitude and Navigation System (ANS)
5.5.1. Attitude Thrusters (ATs)
5.5.2. Triangulated Celestial Navigation (TCN)
5.5.3. Real-Time 3D (RT3D)
6. Conclusions and Future Work
- Integrate the ESME thrust and spacecraft attitude dynamics with DOCKS in order to simulate generic E-sail experiments and missions with a high-fidelity numerical orbit propagator.
- Integrate the ESME tether dynamics with high-fidelity attitude control for tether deployment.
- Refine the escape manoeuvre and develop other trajectory options by integrating realistic spin plane change abilities: either with E-sail or with thrusters on a remote unit.
- Develop ESTCube-LuNa trajectory options with a fixed spin plane.
- Refine the design of experiments, payloads and estimate the E-sail tether length with real-life experiments: how much tether can be hosted on a reel with an automated tether factory?
- Develop deep space communication and ranging nanospacecraft solutions for which there are no European transponders.
- Develop navigation and attitude determination systems and algorithms.
- Develop attitude control systems and algorithms.
- Continue developing the real-time 3D modelling environment.
- Develop a remote unit technology concept for a potential ESTCube lunar escape mission concept with optimal thruster placement for E-sail spin plane control. We see this as the primary showstopper for implementing the lunar escape manoeuvre, as proposed in Section 4 with modelling details described in the work of Palos et al. [42].
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Janhunen, P. Electric Sail for Spacecraft Propulsion. J. Propuls. Power 2004, 20, 763–764. [Google Scholar] [CrossRef]
- Janhunen, P.; Sandroos, A. Simulation study of solar wind push on a charged wire: Basis of solar wind electric sail propulsion. Ann. Geophys. 2007, 25, 755–767. [Google Scholar] [CrossRef]
- Bassetto, M.; Niccolai, L.; Quarta, A.A.; Mengali, G. A comprehensive review of Electric Solar Wind Sail concept and its applications. Prog. Aerosp. Sci. 2022, 128, 100768. [Google Scholar] [CrossRef]
- Janhunen, P.; Toivanen, P.; Envall, J.; Merikallio, S.; Montesanti, G.; Gonzalez Del Amo, J.; Kvell, U.; Noorma, M.; Lätt, S. Overview of electric solar wind sail applications. Proc. Est. Acad. Sci. 2014, 63, 267–278. [Google Scholar] [CrossRef]
- Seppänen, H.; Rauhala, T.; Kiprich, S.; Ukkonen, J.; Simonsson, M.; Kurppa, R.; Janhunen, P.; Hæggström, E. One kilometer (1 km) electric solar wind sail tether produced automatically. Rev. Sci. Instrum. 2013, 84, 095102. [Google Scholar] [CrossRef] [PubMed]
- Wiegmann, B.M. NASA Innovative Advanced Concepts (NIAC): Heliopause Electrostatic Rapid Transit System (HERTS), Final Report; NASA: Washington, DC, USA, 2015. [Google Scholar]
- Janhunen, P.; Toivanen, P.K.; Polkko, J.; Merikallio, S.; Salminen, P.; Haeggström, E.; Seppänen, H.; Kurppa, R.; Ukkonen, J.; Kiprich, S.; et al. Invited Article: Electric solar wind sail: Toward test missions. Rev. Sci. Instrum. 2010, 81, 111301. [Google Scholar] [CrossRef] [PubMed]
- Toivanen, P.K.; Janhunen, P. Spin Plane Control and Thrust Vectoring of Electric Solar Wind Sail. J. Propuls. Power 2013, 29, 178–185. [Google Scholar] [CrossRef]
- Toivanen, P.; Janhunen, P. Thrust vectoring of an electric solar wind sail with a realistic sail shape. Acta Astronaut. 2017, 131, 145–151. [Google Scholar] [CrossRef]
- Toivanen, P.; Janhunen, P.; Envall, J. Electric sail control mode for amplified transverse thrust. Acta Astronaut. 2015, 106, 111–119. [Google Scholar] [CrossRef][Green Version]
- Bassetto, M.; Quarta, A.A.; Mengali, G. Thrust model and guidance scheme for single-tether E-sail with constant attitude. Aerosp. Sci. Technol. 2023, 142, 108618. [Google Scholar] [CrossRef]
- Janhunen, P. Electrostatic Plasma Brake for Deorbiting a Satellite. J. Propuls. Power 2010, 26, 370–372. [Google Scholar] [CrossRef]
- Janhunen, P. Simulation study of the plasma-brake effect. Ann. Geophys. 2014, 32, 1207–1216. [Google Scholar] [CrossRef]
- Iakubivskyi, I.; Janhunen, P.; Praks, J.; Allik, V.; Bussov, K.; Clayhills, B.; Dalbins, J.; Eenmäe, T.; Ehrpais, H.; Envall, J.; et al. Coulomb drag propulsion experiments of ESTCube-2 and FORESAIL-1. Acta Astronaut. 2020, 177, 771–783. [Google Scholar] [CrossRef]
- Wiegmann, B.M.; Stone, N.; Wright, K. The Heliopause Electrostatic Rapid Transit System (HERTS)-Design, Trades, and Analyses Performed in a Two Year NASA Investigation of Electric Sail Propulsion Systems. In Proceedings of the 53rd AIAA/SAE/ASEE Joint Propulsion Conference, Atlanta, GA, USA, 10–12 July 2017; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2017. [Google Scholar] [CrossRef][Green Version]
- Johnson, L.; Polzin, K. Electric Sail Propulsion for Deep Space Missions. In Proceedings of the 70th International Astronautical Congress (IAC), Washington, DC, USA, 21–25 October 2019. [Google Scholar]
- Lafleur, T. Charged aerodynamics: Ionospheric plasma drag on objects in low-Earth orbit. Acta Astronaut. 2023, 212, 370–386. [Google Scholar] [CrossRef]
- Aurora Propulsion Technologies Receives EIC Grant and Equity Funding-AURORA—Propulsion Technologies. Available online: https://aurorapt.fi/news/aurora-propulsion-technologies-receives-eic-grant-and-equity-funding/ (accessed on 28 November 2023).
- Peitso, P.; Janhunen, P.; Genzer, M.; Yli-Opas, P.; Laurila, H.; Hieta, M.; Haukka, H.; Macieira, D.; Toivanen, P.; Polkko, J.; et al. ESA Dragliner-Coulomb drag based telecommunication satellite deorbiting device. In Proceedings of the Winter Satellite Workshop, Espoo, Finland, 17–19 January 2024; Aalto University: Espoo, Finland, 2024. [Google Scholar]
- Mori, O.; Shirasawa, Y.; Mimasu, Y.; Tsuda, Y.; Sawada, H.; Saiki, T.; Yamamoto, T.; Yonekura, K.; Hoshino, H.; Kawaguchi, J.; et al. Overview of IKAROS Mission. In Advances in Solar Sailing; Springer: Berlin/Heidelberg, Germany, 2014; pp. 25–43. [Google Scholar] [CrossRef]
- Vulpetti, G.; Johnson, L.; Matloff, G.L. The NanoSAIL-D2 NASA Mission. In Solar Sails; Springer: Berlin/Heidelberg, Germany, 2015; pp. 173–178. [Google Scholar] [CrossRef]
- Betts, B.; Nye, B.; Vaughn, J.; Greeson, E.; Chute, R.; Spencer, D.A.; Ridenoure, R.W.; Munakata, R.; Wong, S.D.; Diaz, A.; et al. LightSail 1 mission results and public outreach strategies. In Proceedings of the 4th International Symposium on Solar Sailing, Japan Space Forum, Tokyo, Japan, 17–20 January 2017. [Google Scholar]
- Sanchez-Arriaga, G.; del Pino, A.; Sharifi, G.; Tarabini Castellani, L.; García-Gonzaléz, S.; Ortega, A.; Cruces, D.; Velasco, A.; Orte, S.; Ruiz, A.; et al. The E.T.PACK-F Project: Towards a flight-ready deorbit device based on electrodynamic tether technology. In Proceedings of the AIAA SCITECH 2024 Forum, Orlando, FL, USA, 8–12 January 2024. [Google Scholar] [CrossRef]
- Lätt, S.; Slavinskis, A.; Ilbis, E.; Kvell, U.; Voormansik, K.; Kulu, E.; Pajusalu, M.; Kuuste, H.; Sünter, I.; Eenmäe, T.; et al. ESTCube-1 nanosatellite for electric solar wind sail in-orbit technology demonstration. Proc. Est. Acad. Sci. 2014, 63, 200–209. [Google Scholar] [CrossRef]
- Envall, J.; Janhunen, P.; Toivanen, P.; Pajusalu, M.; Ilbis, E.; Kalde, J.; Averin, M.; Kuuste, H.; Laizans, K.; Allik, V.; et al. E-sail test payload of ESTCube-1 nanosatellite. Proc. Est. Acad. Sci. 2014, 63, 210–221. [Google Scholar] [CrossRef]
- Khurshid, O.; Tikka, T.; Praks, J.; Hallikainen, M. Accommodating the plasma brake experiment on-board the Aalto-1 satellite. Proc. Est. Acad. Sci. 2014, 63, 258–266. [Google Scholar] [CrossRef]
- Praks, J.; Mughal, M.R.; Vainio, R.; Janhunen, P.; Envall, J.; Oleynik, P.; Näsilä, A.; Leppinen, H.; Niemelä, P.; Slavinskis, A.; et al. Aalto-1, multi-payload CubeSat: Design, integration and launch. Acta Astronaut. 2021, 187, 370–383. [Google Scholar] [CrossRef]
- Slavinskis, A.; Pajusalu, M.; Kuuste, H.; Ilbis, E.; Eenmae, T.; Sunter, I.; Laizans, K.; Ehrpais, H.; Liias, P.; Kulu, E.; et al. ESTCube-1 in-orbit experience and lessons learned. IEEE Aerosp. Electron. Syst. Mag. 2015, 30, 12–22. [Google Scholar] [CrossRef]
- Mughal, M.R.; Praks, J.; Vainio, R.; Janhunen, P.; Envall, J.; Näsilä, A.; Oleynik, P.; Niemelä, P.; Nyman, S.; Slavinskis, A.; et al. Aalto-1, multi-payload CubeSat: In-orbit results and lessons learned. Acta Astronaut. 2021, 187, 557–568. [Google Scholar] [CrossRef]
- Palmroth, M.; Praks, J.; Vainio, R.; Janhunen, P.; Kilpua, E.K.; Afanasiev, A.; Ala-Lahti, M.; Alho, A.; Asikainen, T.; Asvestari, E.; et al. FORESAIL-1 CubeSat Mission to Measure Radiation Belt Losses and Demonstrate Deorbiting. J. Geophys. Res. Space Phys. 2019, 124, 5783–5799. [Google Scholar] [CrossRef]
- Anger, M.; Niemelä, P.; Cheremetiev, K.; Clayhills, B.; Fetzer, A.; Lundén, V.; Hiltunen, M.; Kärkkäinen, T.; Mayank, M.; Turc, L.; et al. Foresail-2: Space Physics Mission in a Challenging Environment. Space Sci. Rev. 2023, 219, 66. [Google Scholar] [CrossRef]
- Anger, M.; Niemelä, P.; Fetzer, A.; Lundén, V.; Räty, T.; Turc, L.; Osmane, A.; Grandin, M.; Palmroth, M.; Kilpua, E.; et al. Descoping Options for Foresail-2 Radiation Belt CubeSat Mission. In Proceedings of the Winter Satellite Workshop, Espoo, Finland, 17–19 January 2024; Aalto University: Espoo, Finland, 2024. [Google Scholar]
- Klesh, A.; Baker, J.; Krajewski, J. MarCO: Flight Review and Lessons Learned. In Proceedings of the 33rd Annual AIAA/USU Conference on Small Satellite, Logan, UT, USA, 3–8 August 2019. [Google Scholar]
- Rivkin, A.S.; Cheng, A.F. Planetary defense with the Double Asteroid Redirection Test (DART) mission and prospects. Nat. Commun. 2023, 14, 1003. [Google Scholar] [CrossRef]
- Andrews, D.; Wahlund, J.E.; Kohout, T.; Penttilä, A.; Andrews, D.; Wahlund, J.E.; Kohout, T.; Penttilä, A. Asteroid Prospection Explorer (APEX) Cubesat For the ESA Hera Mission. EPSC 2019, 2019, EPSC-DPS2019-1287. [Google Scholar]
- Goldberg, H.; Karatekin, Ö.; Ritter, B.; Herique, A.; Tortora, P.; Prioroc, C.; Gutierrez, B.; Martino, P.; Carnelli, I. The Juventas CubeSat in Support of ESA’s Hera Mission to the Asteroid Didymos. In Proceedings of the 33rd Annual AIAA/USU Small Satellite Conference, Logan, UT, USA, 3–8 August 2019. [Google Scholar]
- Kohout, T.; Cardi, M.; Näsilä, A.; Palomba, E.; Topputo, F. Milani CubeSat for ESA Hera mission. In Proceedings of the European Planetary Science Congress 2021 (EPSC 2021), Virtual, 13–24 September 2021. [Google Scholar] [CrossRef]
- MINERVA-II1: Successful Image Capture, Landing on Ryugu and Hop!|Topics|JAXA Hayabusa2 Project. Available online: https://www.hayabusa2.jaxa.jp/en/topics/20180922e/ (accessed on 10 March 2024).
- Thompson, M.R.; Rosen, M. Utilization and Validation of DSS-17 on the CAPSTONE Lunar Mission. In Proceedings of the 33rd AAS/AIAA Space Flight Mechanics Conference, Austin, TX, USA, 14–19 January 2023. [Google Scholar]
- Cervone, A.; Topputo, F.; Speretta, S.; Menicucci, A.; Turan, E.; Di Lizia, P.; Massari, M.; Franzese, V.; Giordano, C.; Merisio, G.; et al. LUMIO: A CubeSat for observing and characterizing micro-meteoroid impacts on the Lunar far side. Acta Astronaut. 2022, 195, 309–317. [Google Scholar] [CrossRef]
- Kramer, A.; Bangert, P.; Schilling, K. UWE-4: First Electric Propulsion on a 1U CubeSat—In-Orbit Experiments and Characterization. Aerospace 2020, 7, 98. [Google Scholar] [CrossRef]
- Palos, M.F.; Janhunen, P.; Toivanen, P.; Tajmar, M.; Iakubivskyi, I.; Micciani, A.; Orsini, N.; Kütt, J.; Rohtsalu, A.; Dalbins, J.; et al. Electric Sail Mission Expeditor, ESME: Software Architecture and Initial ESTCube Lunar Cubesat E-Sail Experiment Design. Aerospace 2023, 10, 694. [Google Scholar] [CrossRef]
- Iakubivskyi, I.; Mačiulis, L.; Janhunen, P.; Dalbins, J.; Noorma, M.; Slavinskis, A. Aspects of nanospacecraft design for main-belt sailing voyage. Adv. Space Res. 2021, 67, 2957–2980. [Google Scholar] [CrossRef]
- Janhunen, P. Increased electric sail thrust through removal of trapped shielding electrons by orbit chaotisation due to spacecraft body. Ann. Geophys. 2009, 27, 3089–3100. [Google Scholar] [CrossRef]
- Monitoring Camera Crystalspace “Suupistri”. Available online: https://crystalspace.eu/monitoring-camera-suupistri/ (accessed on 10 March 2024).
- Dalbins, J.; Allaje, K.; Iakubivskyi, I.; Kivastik, J.; Komarovskis, R.O.; Plans, M.; Sunter, I.; Teras, H.; Ehrpais, H.; Ilbis, E.; et al. ESTCube-2: The Experience of Developing a Highly Integrated CubeSat Platform. In Proceedings of the IEEE Aerospace Conference Proceedings, Big Sky, MT, USA, 5–12 March 2022. [Google Scholar] [CrossRef]
- Sakamoto, H.; Mughal, M.R.; Slavinskis, A.; Praks, J.; Toivanen, P.; Janhunen, P.; Palmroth, M.; Kilpua, E.; Vainio, R. Verification of Tether Deployment System aboard CubeSat through Dynamics Simulations and Tests. In Proceedings of the IEEE Aerospace Conference Proceedings, Big Sky, MT, USA, 6–13 March 2021. [Google Scholar] [CrossRef]
- Janhunen, P.; Toivanen, P. Safety criteria for flying E-sail through solar eclipse. Acta Astronaut. 2015, 114, 1–5. [Google Scholar] [CrossRef]
- Spellman. UM8-40 Series DC-DC High Voltage Power Supplies|SpellmanHV. Available online: https://www.spellmanhv.com/en/high-voltage-power-supplies/UM8-40 (accessed on 10 March 2024).
- Laframboise, J.G.; Parker, L.W.; Laframboise, J.G.; Parker, L.W. Probe design for orbit-limited current collection. PhFl 1973, 16, 629–636. [Google Scholar] [CrossRef]
- Kobayashi, M.M.; Holmes, S.; Yarlagadda, A.; Aguirre, F.; Chase, M.; Angkasa, K.; Burgett, B.; Mcnally, L.; Dobreva, T.; Satorius, E. The Iris Deep-Space Transponder for the SLS EM-1 Secondary Payloads. IEEE Aerosp. Electron. Syst. Mag. 2019, 34, 34–44. [Google Scholar] [CrossRef]
- Andrew O’Dea. Deep Space Network, 203 Sequential Ranging; Technical Report; Jet Propulsion Laboratory, California Institute of Technology: Pasadena, CA, USA, 2019.
- Pajusalu, M.; Iakubivskyi, I.; Schwarzkopf, G.J.; Knuuttila, O.; Väisänen, T.; Bührer, M.; Palos, M.F.; Teras, H.; Le Bonhomme, G.; Praks, J.; et al. SISPO: Space Imaging Simulator for Proximity Operations. PLoS ONE 2022, 17, e0263882. [Google Scholar] [CrossRef] [PubMed]
- GitHub-RicDen/FlyByGen: Automatic Fly-by Data Generation Pipeline. Available online: https://github.com/RicDen/FlyByGen (accessed on 10 March 2024).
- Planetary System Research|Asteroid Image Simulator—Bitbucket. Available online: https://bitbucket.org/planetarysystemresearch/asteroid-image-simulator/src/master/ (accessed on 10 March 2024).
- Unreal Engine 5. Available online: https://www.unrealengine.com/en-US/unreal-engine-5 (accessed on 10 March 2024).
- Teras, H.; Islam, Q.S.; Kruuse, K.; Pajusalu, M. ULYSSES-A State of the Art Sandbox Simulator for Planetary Surfaces. In Proceedings of the 73rd International Astronautical Congress, Paris, France, 18–22 September 2022. [Google Scholar]
- Bock, D.; Tajmar, M. Highly miniaturized FEEP propulsion system (NanoFEEP) for attitude and orbit control of CubeSats. Acta Astronaut. 2018, 144, 422–428. [Google Scholar] [CrossRef]
- Kuuste, H.; Eenmäe, T.; Allik, V.; Agu, A.; Vendt, R.; Ansko, I.; Laizans, K.; Sünter, I.; Lätt, S.; Noorma, M. Imaging system for nanosatellite proximity operations. Proc. Est. Acad. Sci. 2014, 63, 250–257. [Google Scholar] [CrossRef]
- Henry, S.; Christian, J.A. Absolute Triangulation Algorithms for Space Exploration. J. Guid. Control. Dyn. 2022, 46, 21–46. [Google Scholar] [CrossRef]
- Solar Textures|Solar System Scope. Available online: https://www.solarsystemscope.com/textures/ (accessed on 10 March 2024).
- Jain, R.; Sharma, H.; Segret, B. DOCKS Propagator: An Open-source Adaptive Time-step Trajectory Propagator for CubeSat Missions. In Proceedings of the IEEE Aerospace Conference Proceedings, Big Sky, MT, USA, 5–12 March 2022. [Google Scholar] [CrossRef]

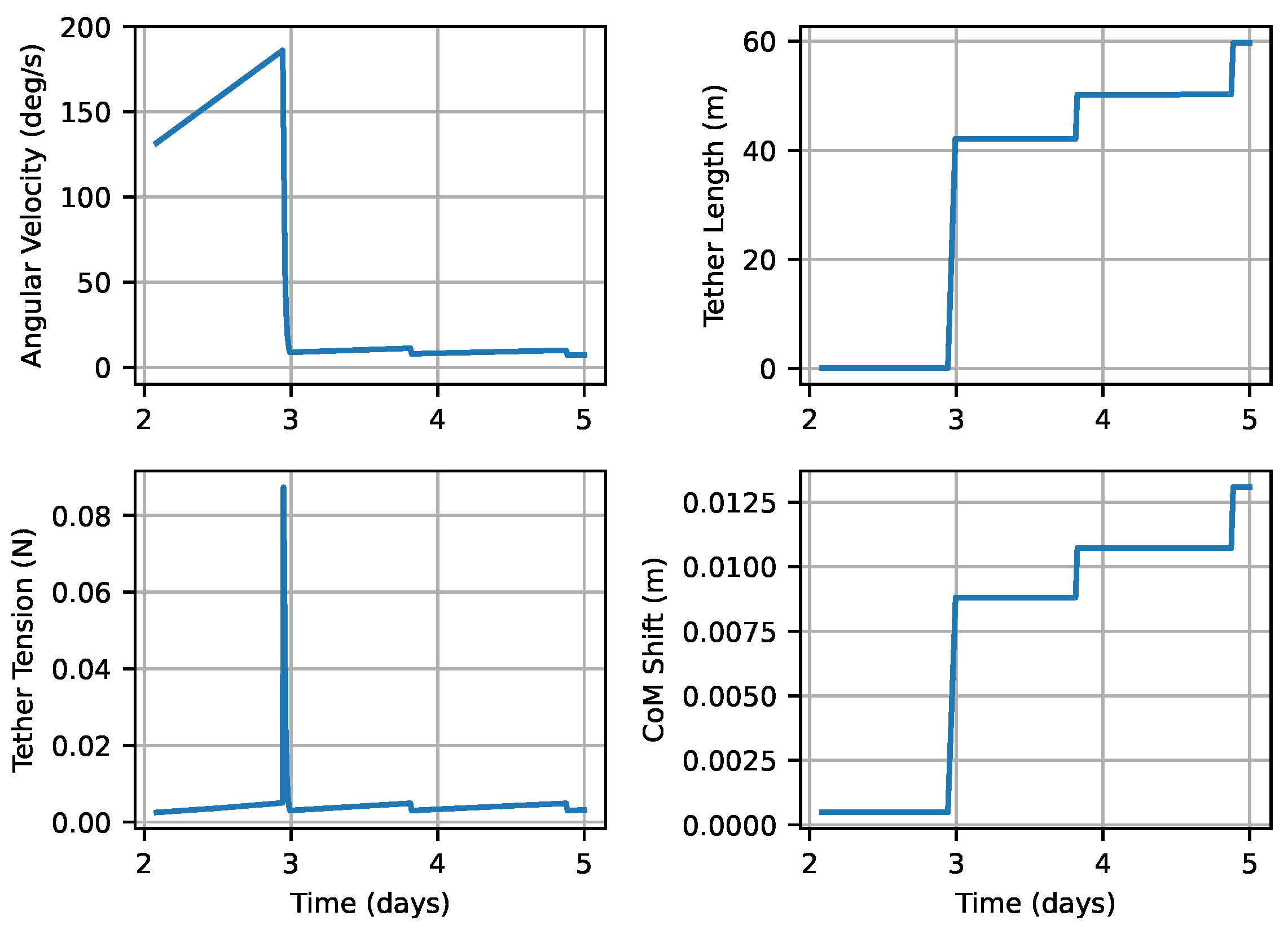
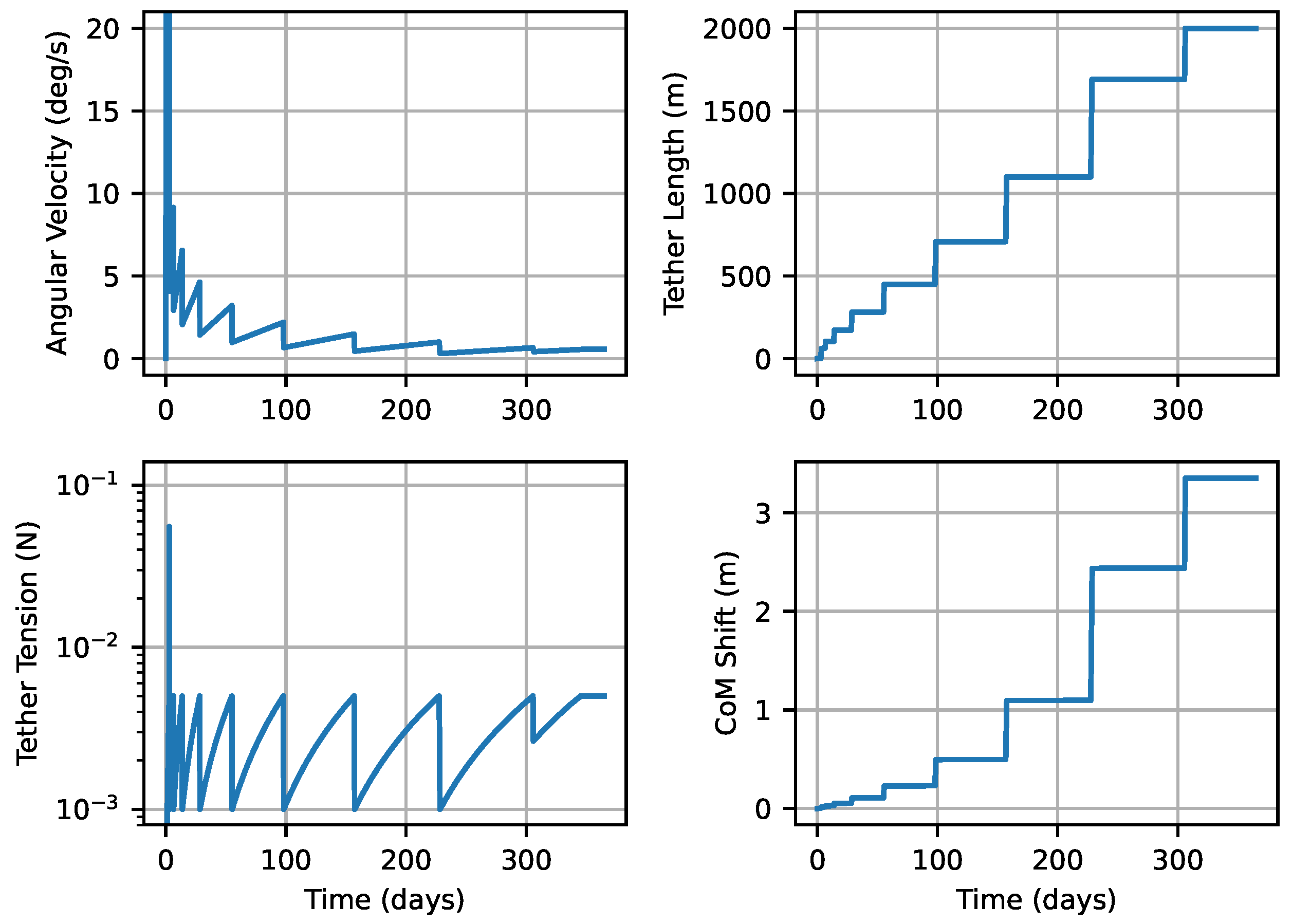
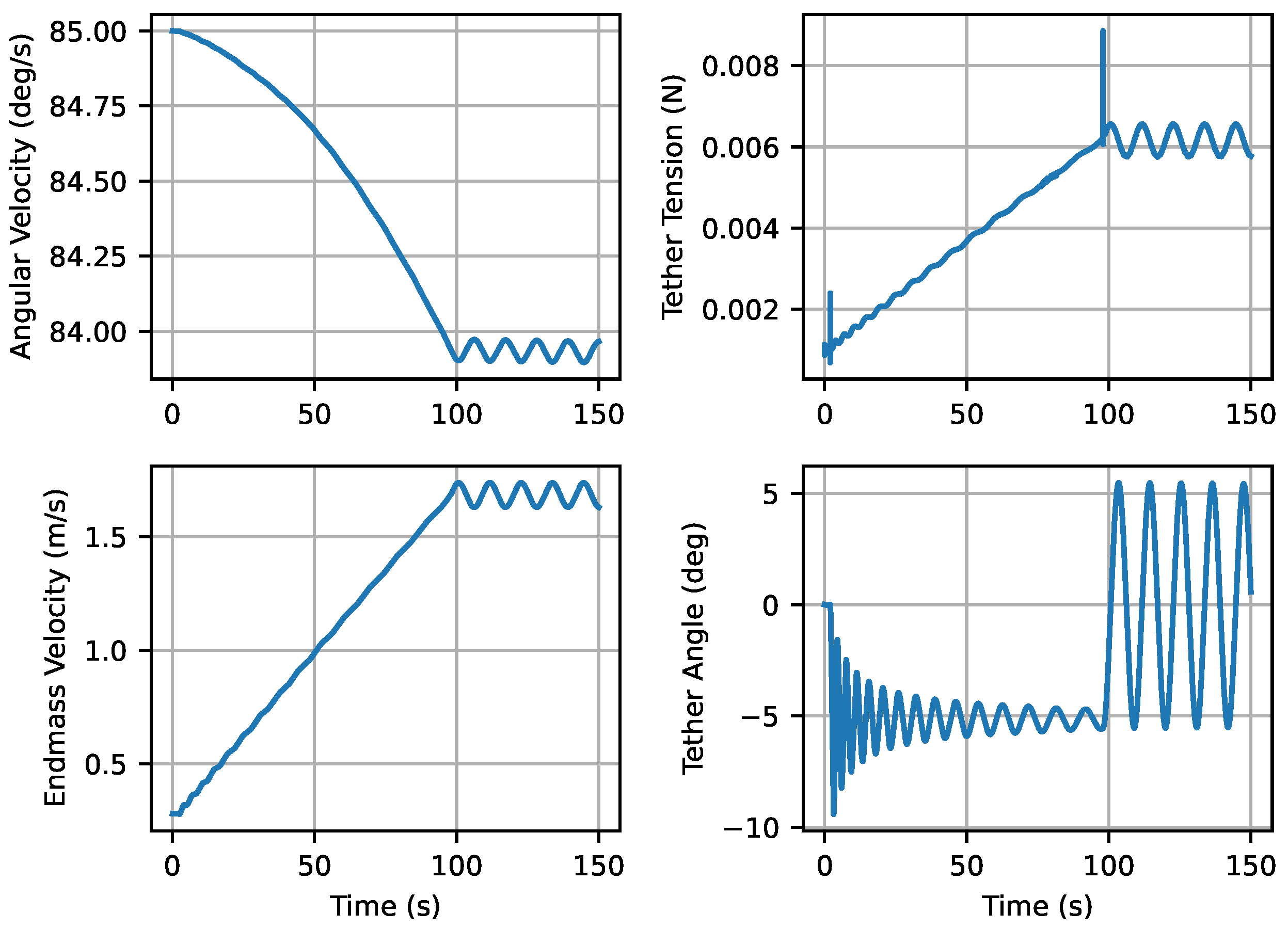
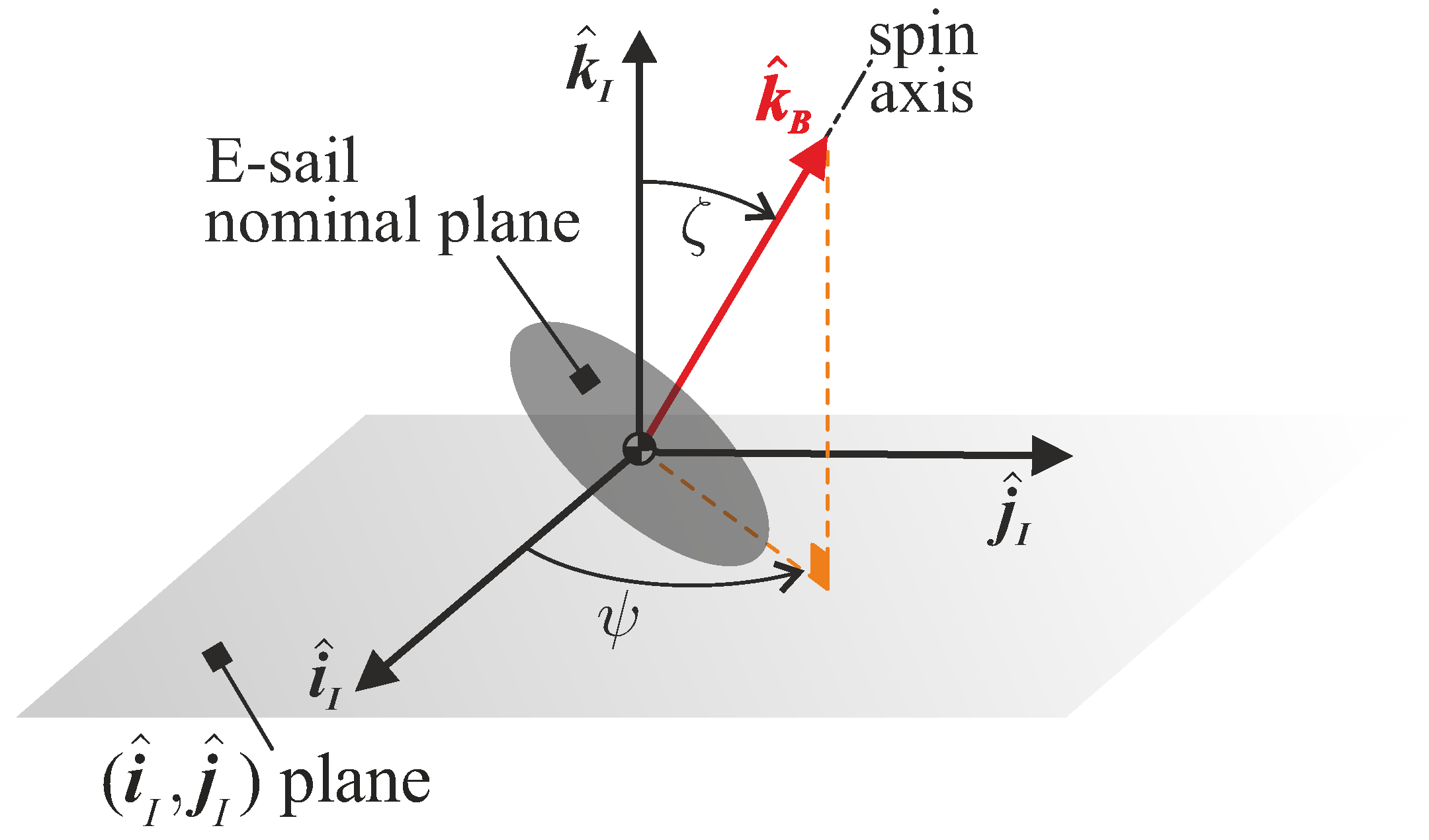



| Tether Length (km) | Rot. Rate (rad/s) | Rot. Period (s) | Ang. Momentum (N m s) |
|---|---|---|---|
| 0.1 | 0.117 | 53.6 | 3.86 |
| 0.5 | 0.0350 | 180 | 55.0 |
| 1.0 | 0.0190 | 330 | 191 |
| 2.0 | 0.00998 | 630 | 702 |
| Initial Pericentre Altitude [km] | Initial Semimajor Axis [km] | Escape Time [days] | [deg/day] | [deg/day] | Sail-On Time |
|---|---|---|---|---|---|
| 500 | 5593.5 | 485 | 275 | 71 | 33.26% |
| 1500 | 8093.5 | 327 | 194 | 51 | 28.07% |
| 3000 | 11843.5 | 269 | 118 | 33 | 20.29% |
| Component | Mass (g) |
|---|---|
| Structure and wings | 4000 |
| E-sail | 2200 |
| Tether camera | 500 |
| Langmuir probes | 400 |
| Avionics | 1000 |
| HSCOM | 1000 |
| RW | 700 |
| TNC | 1000 |
| Solar cells | 300 |
| Attitude thrusters | 200 |
| Sum: | 11,300 |
| Total with 30% margin: | 14,690 |
| Part | Power (mW) |
|---|---|
| E-sail | 2000 |
| Tether camera | 1000 |
| Langmuir probe | 4000 |
| HSCOM transmission | 7000 |
| HSCOM reception | 1000 |
| Reaction wheels | 6000 |
| Two attitude thrusters | 2500 |
| Triangulated celestial navigation | 4500 |
| On-board computer | 600 |
| Electrical power system | 400 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Slavinskis, A.; Palos, M.F.; Dalbins, J.; Janhunen, P.; Tajmar, M.; Ivchenko, N.; Rohtsalu, A.; Micciani, A.; Orsini, N.; Moor, K.M.; et al. Electric Sail Test Cube–Lunar Nanospacecraft, ESTCube-LuNa: Solar Wind Propulsion Demonstration Mission Concept. Aerospace 2024, 11, 230. https://doi.org/10.3390/aerospace11030230
Slavinskis A, Palos MF, Dalbins J, Janhunen P, Tajmar M, Ivchenko N, Rohtsalu A, Micciani A, Orsini N, Moor KM, et al. Electric Sail Test Cube–Lunar Nanospacecraft, ESTCube-LuNa: Solar Wind Propulsion Demonstration Mission Concept. Aerospace. 2024; 11(3):230. https://doi.org/10.3390/aerospace11030230
Chicago/Turabian StyleSlavinskis, Andris, Mario F. Palos, Janis Dalbins, Pekka Janhunen, Martin Tajmar, Nickolay Ivchenko, Agnes Rohtsalu, Aldo Micciani, Nicola Orsini, Karl Mattias Moor, and et al. 2024. "Electric Sail Test Cube–Lunar Nanospacecraft, ESTCube-LuNa: Solar Wind Propulsion Demonstration Mission Concept" Aerospace 11, no. 3: 230. https://doi.org/10.3390/aerospace11030230
APA StyleSlavinskis, A., Palos, M. F., Dalbins, J., Janhunen, P., Tajmar, M., Ivchenko, N., Rohtsalu, A., Micciani, A., Orsini, N., Moor, K. M., Kuzmin, S., Bleiders, M., Donerblics, M., Ofodile, I., Kütt, J., Eenmäe, T., Allik, V., Viru, J., Halapuu, P., ... Tamm, A. (2024). Electric Sail Test Cube–Lunar Nanospacecraft, ESTCube-LuNa: Solar Wind Propulsion Demonstration Mission Concept. Aerospace, 11(3), 230. https://doi.org/10.3390/aerospace11030230











