Numerical Investigation on the Mechanism of Solid Rocket Motor Instability Induced by Differences between On-Ground and In-Flight Conditions
Abstract
1. Introduction
2. Numerical Methods
2.1. Physical Model and Initial Settings
2.2. Material Properties and Boundary Conditions
2.3. Governing Equations and Numerical Methods
2.3.1. Governing Equations for Structures
2.3.2. Governing Equations for Flow Field
2.3.3. Governing Equations for Fluid–Structure Interaction
2.3.4. Numerical Methods
2.4. Grid Size Independence Test
2.5. Numerical Method Validation
3. Results and Discussion
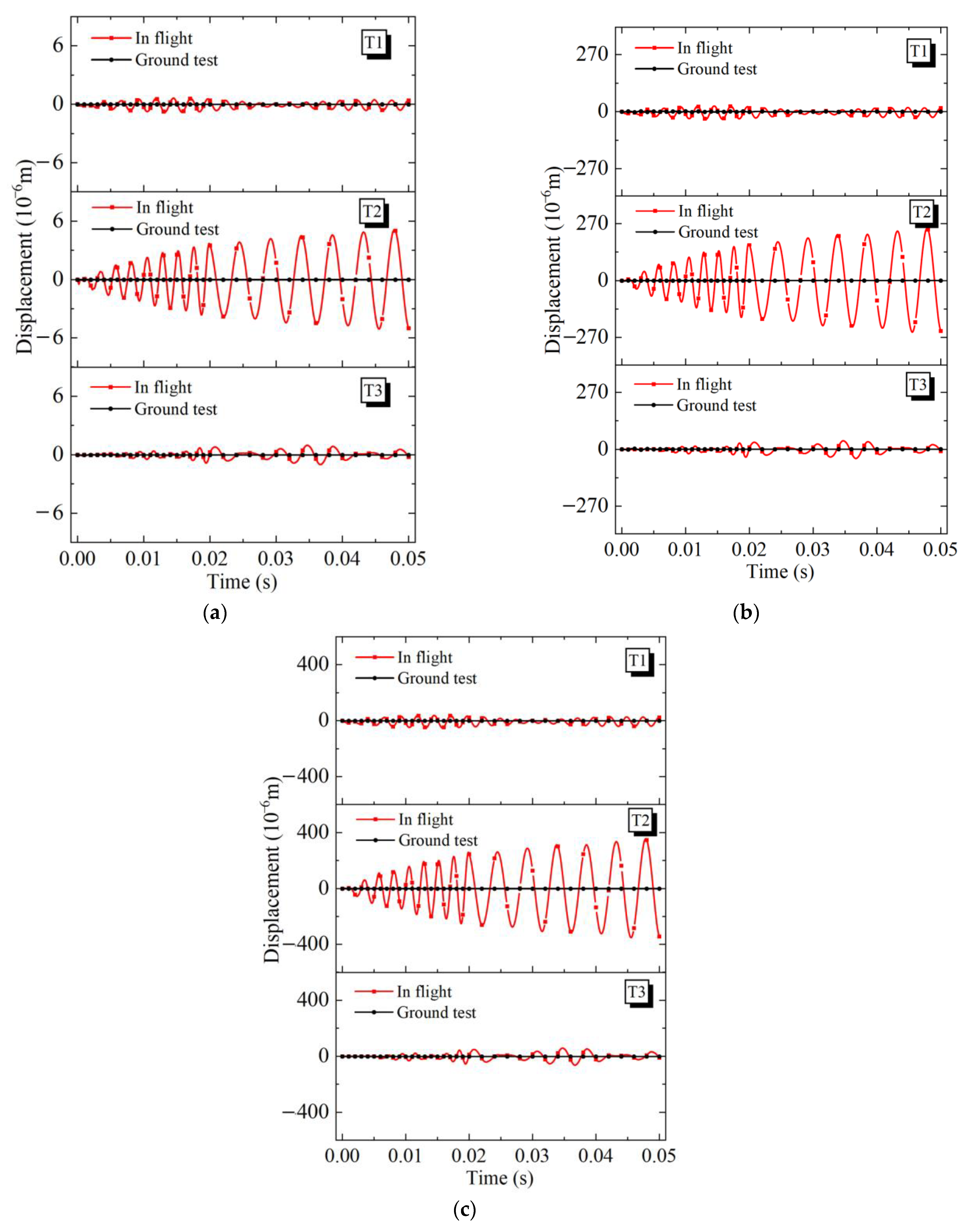
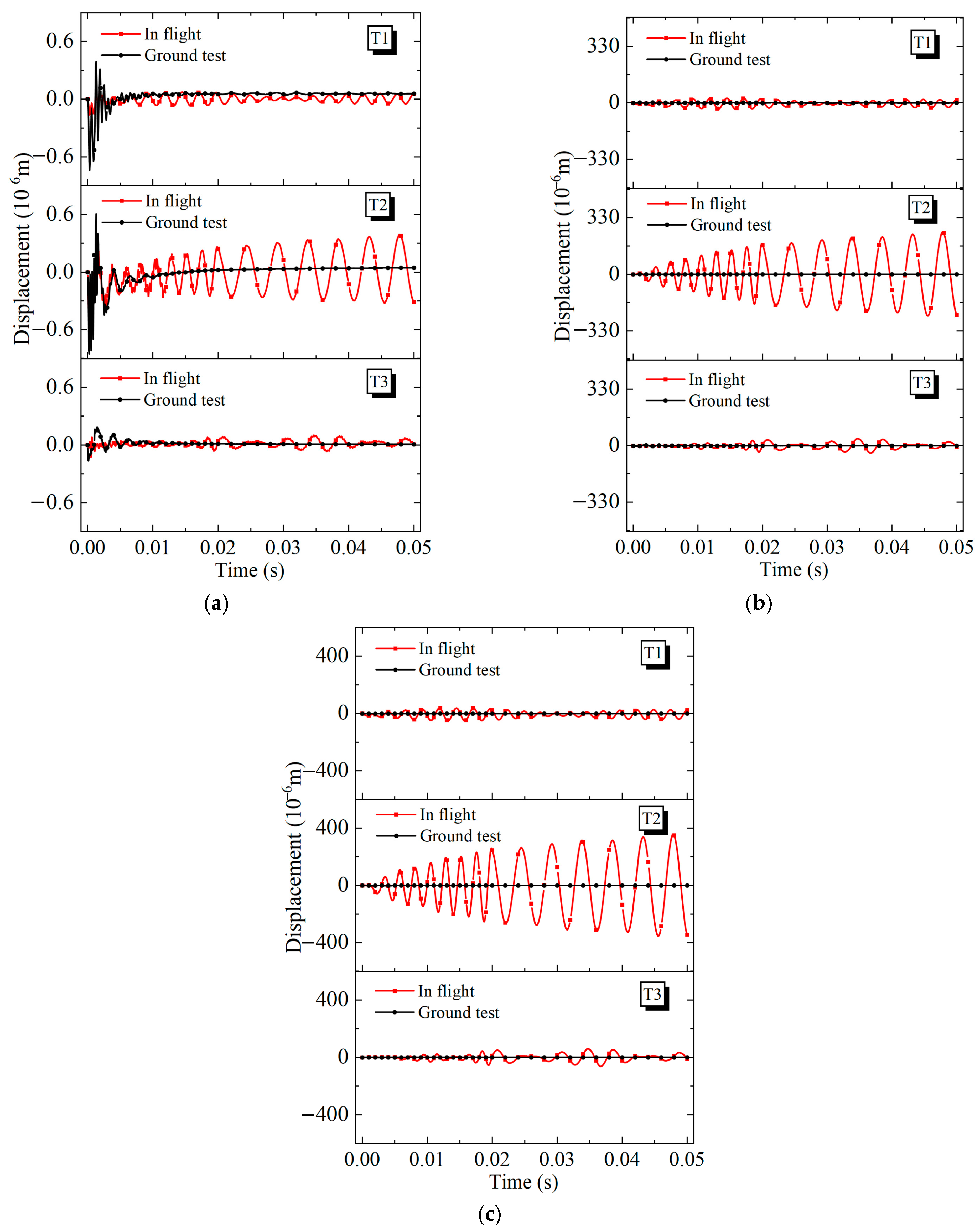
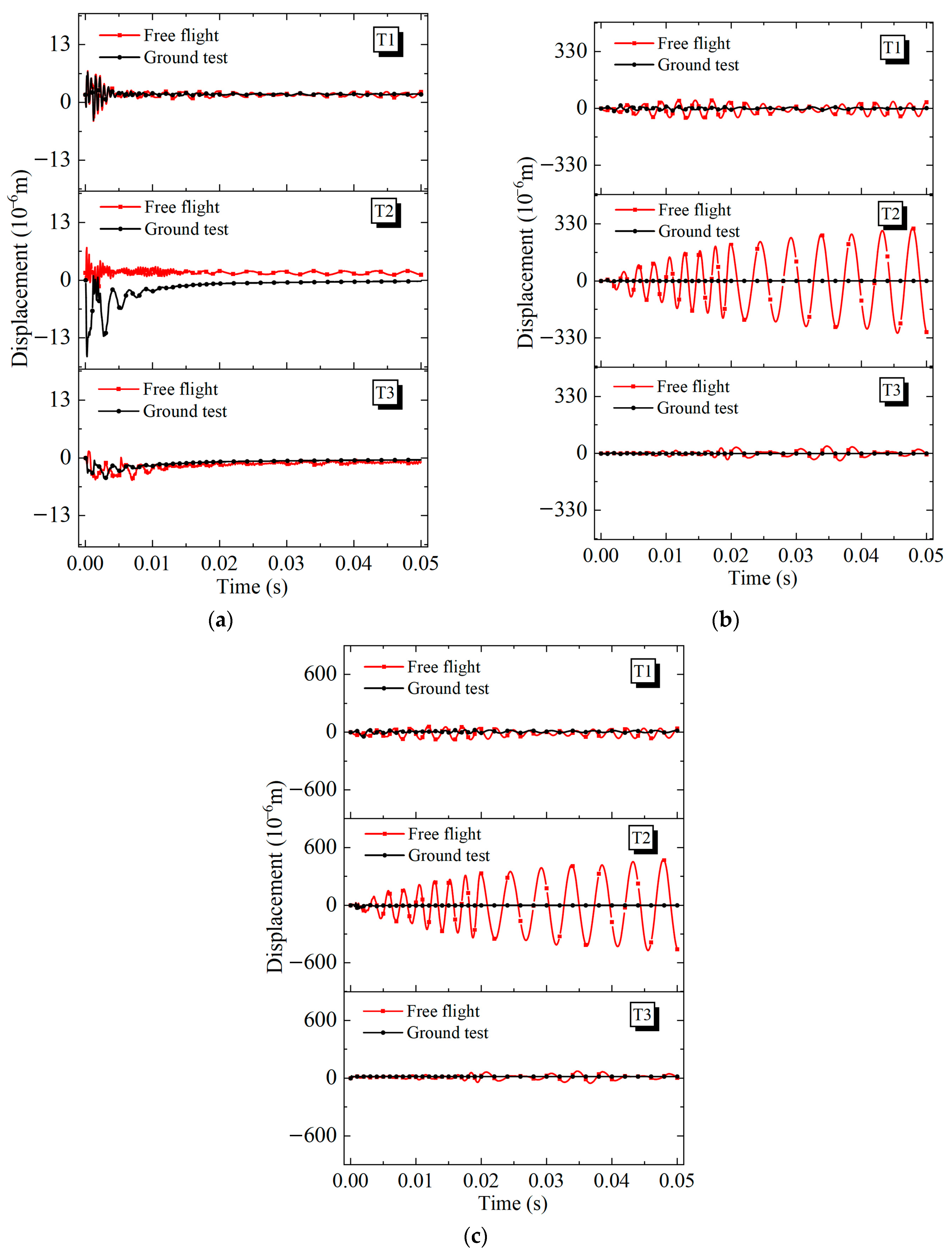
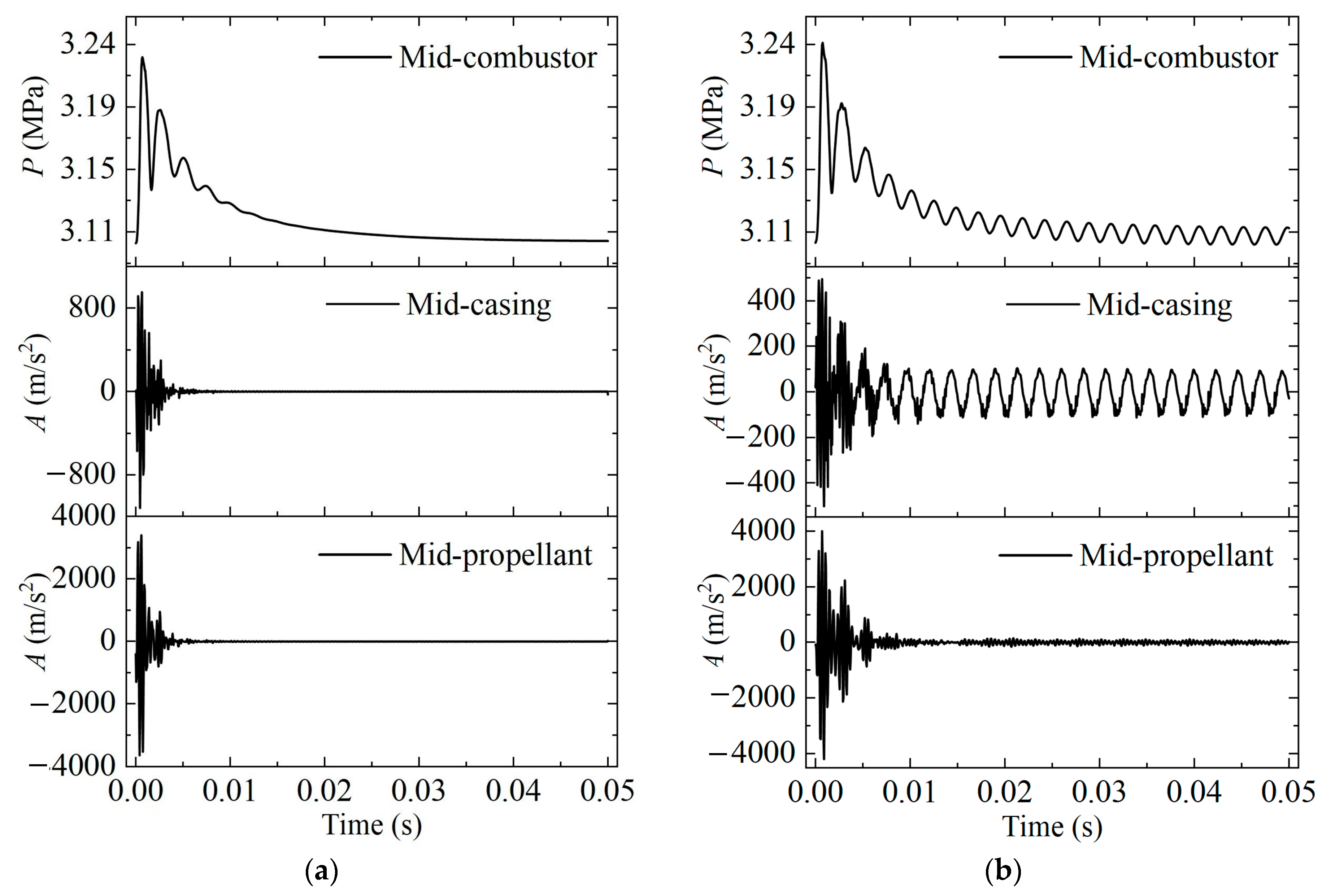
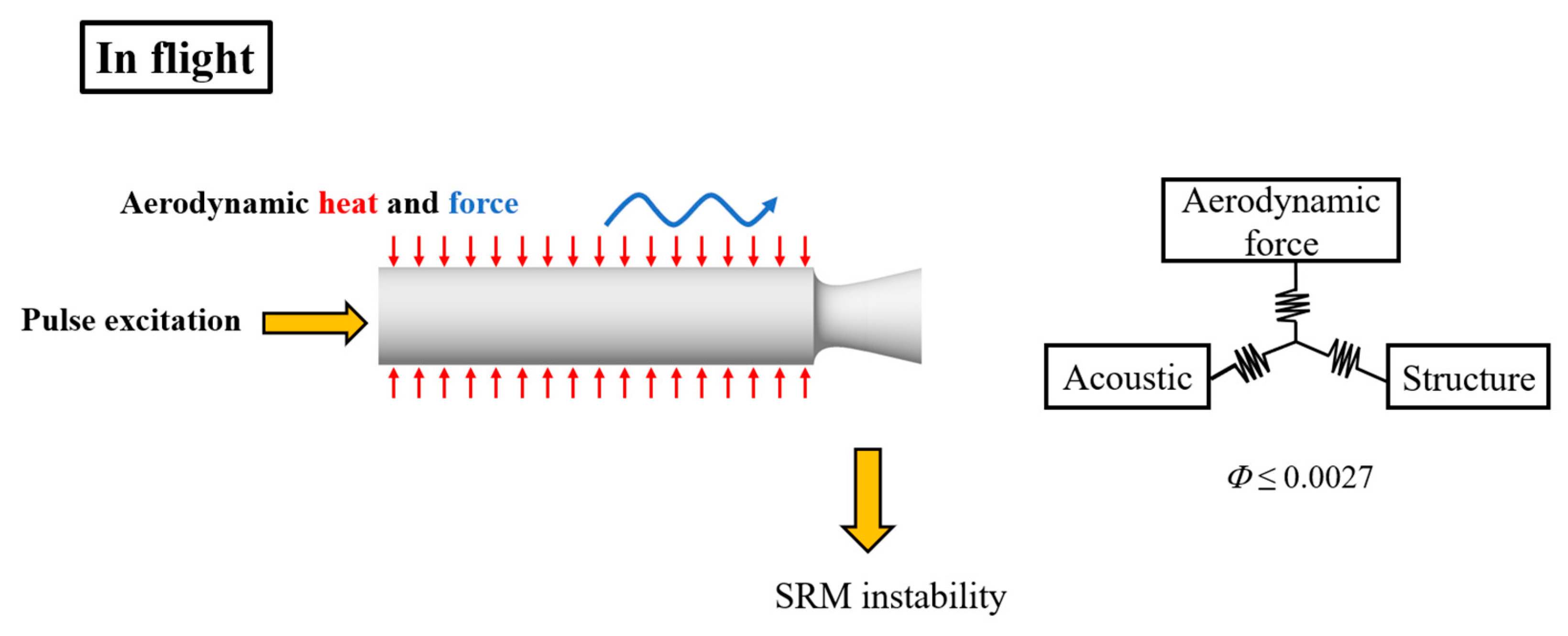
3.1. Characteristics of SRM Instability
3.2. Coupling Characteristics of Pressure Oscillation and Structural Vibration
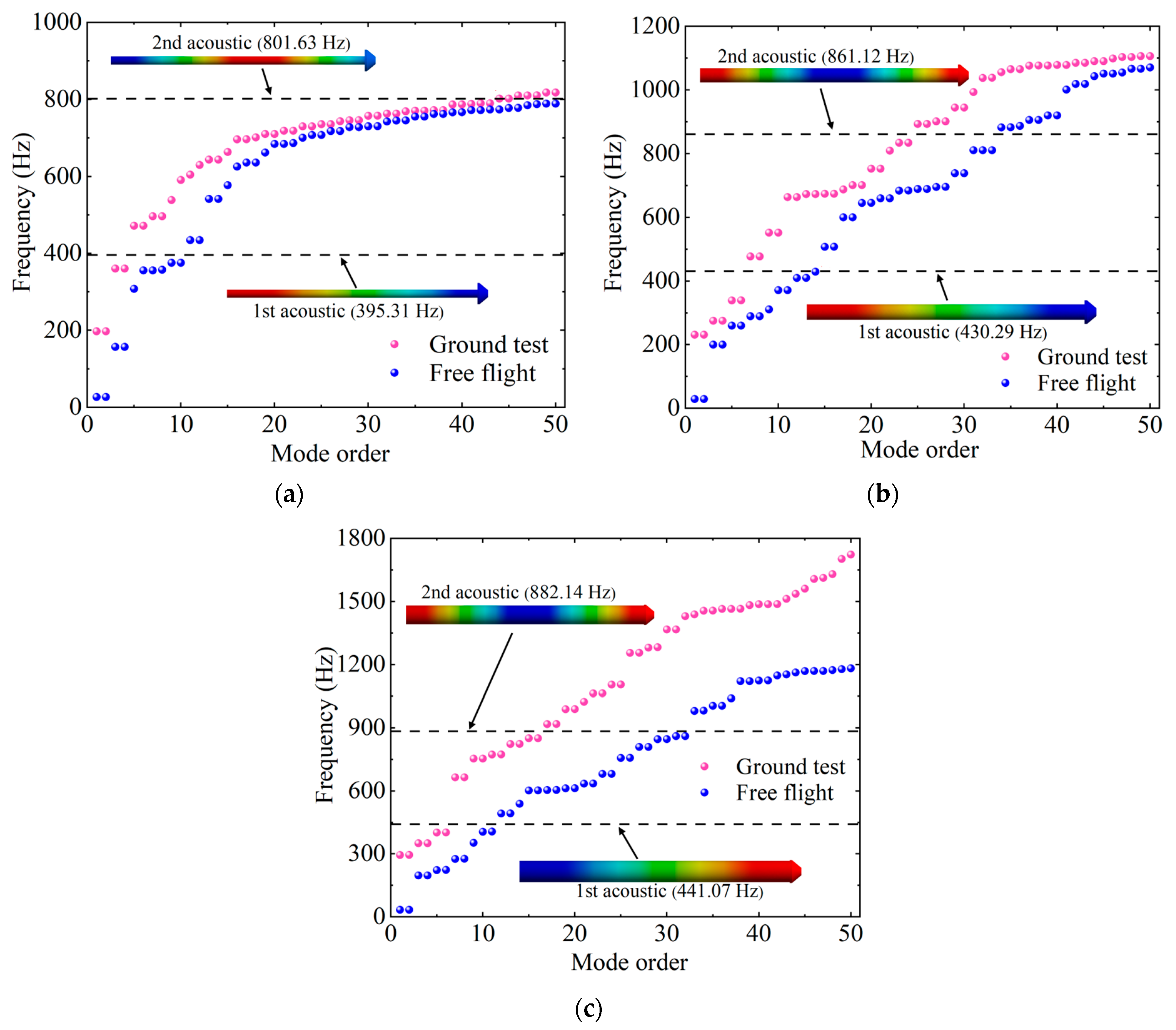
3.3. Effects of Aerodynamic Heating and Fixed Constraints on Structural Natural Frequency
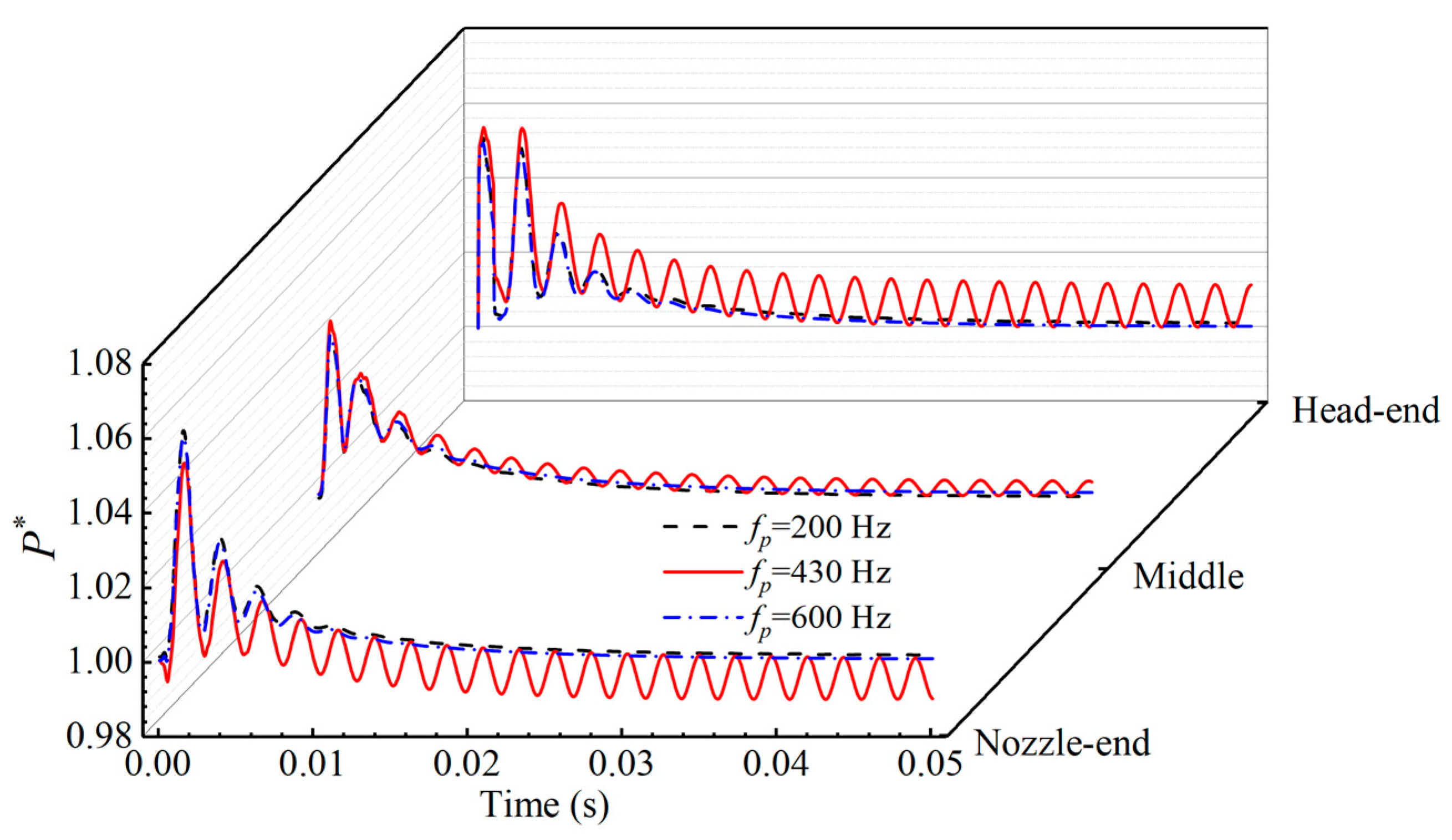
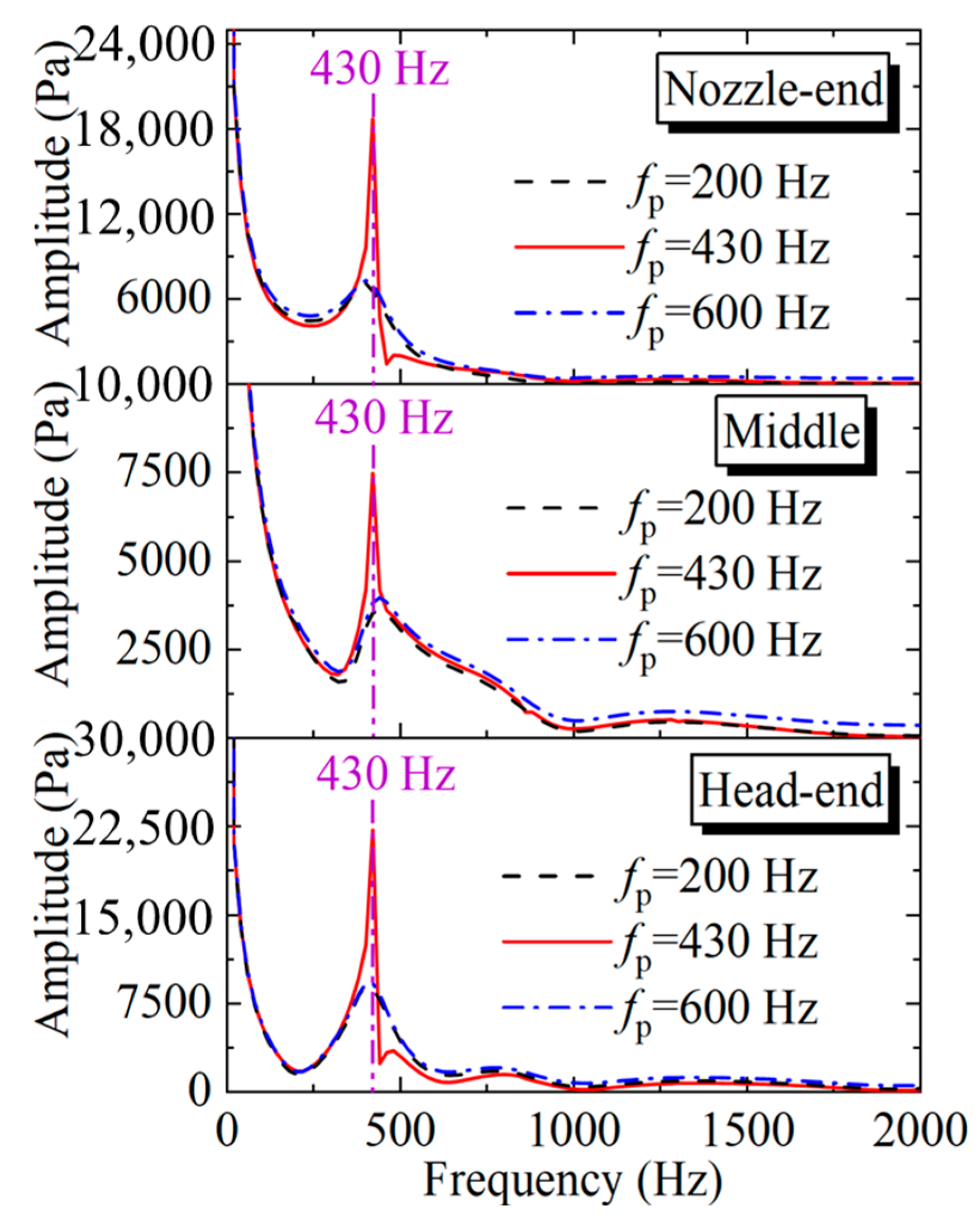
3.4. Effect of Aerodynamic Force Frequency on SRM Instability
4. Conclusions
- (1)
- The distinctions between the ground-test and in-flight conditions lie in the stronger constraints imposed, as well as the absence of aerodynamic forces and aerodynamic heat in ground tests. This leads to significant differences in the response of an SRM to impulse excitation under in-flight and ground-test conditions. After pulse excitation, compared to ground testing, the combustion chamber pressure, thrust, and structural oscillations of an SRM are significantly amplified during in-flight conditions, eventually leading to limit cycle behaviors.
- (2)
- Under in-flight conditions, the SRM is subjected to weaker constraints and thus structural vibrations are not effectively suppressed. Furthermore, when the natural frequencies of the structure, which are reduced by aerodynamic heating, and the first-order acoustic frequency are increased via a propellant regression approach and reach resonance conditions, the aerodynamic forces caused by airflow resistance are transmitted to the internal flow field through structural vibrations, resulting in the sustained excitation of pressure oscillations in the internal flow field.
- (3)
- The instability factor Φ is proposed to represent the coupling relationship between the structural natural frequency and the first-order acoustic frequency of an SRM. When Φ is within the range 0–0.0027, the SRM is in an unstable state that is highly susceptible to pulse excitation.
- (4)
- The airflow resistance can be decomposed into aerodynamic forces at different frequencies; when the frequencies of aerodynamic forces, first-order acoustic frequency, and structural natural frequency are particularly close, the pressure oscillations and structural vibrations resonate with each other, thus the initial pulse will lead to SRM instability.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Blomshield, F.S. Lessons learned in solid rocket combustion instability. In Proceedings of the 43rd AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Cincinnati, OH, USA, 8–11 July 2007. [Google Scholar]
- Zhang, Q.; Wei, Z.J.; Su, W.X.; Li, J.W.; Wang, N.F. Theoretical modeling and numerical study for thrust-oscillation characteristics in solid rocket motors. J. Propuls. Power 2012, 28, 312–322. [Google Scholar] [CrossRef]
- Fabignon, Y.; Dupays, J.; Avalon, G.; Vuillot, F.; Lupoglazoff, N.; Casalis, G.; Prévost, M. Instabilities and pressure oscillations in solid rocket motors. J. Aerosp. Sci. Technol. 2003, 7, 191–200. [Google Scholar] [CrossRef]
- Blomshield, F.S. Historical perspective of combustion instability in motors: Case studies. In Proceedings of the 37th Joint Propulsion Conference and Exhibit, Salt Lake City, UT, USA, 8–11 July 2001. [Google Scholar]
- Blomshield, F.S.; Crump, J.E.; Mathes, H.B.; Stalnaker, R.A.; Beckstead, M.W. Stability testing of full-scale tactical motors. J. Propuls. Power 1997, 13, 349–355. [Google Scholar] [CrossRef]
- Blomshield, F.S.; Stalnaker, R. Pulsed motor firings-pulse amplitude; formulation; enhanced instrumentation. In Proceedings of the 34th AIAA/ASME/SAE/ASEE Joint Propulsion Conference and Exhibit, Cleveland, OH, USA, 13–15 July 1998. [Google Scholar]
- Culick, F.E.C.; Yang, V. Prediction of the stability of unsteady motions in solid propellant rocket motors. AIAA Prog. Astronaut. Aeronaut. 1992, 143, 719–779. [Google Scholar]
- Culick, F.E.C. Nonlinear behavior of acoustic waves in combustion chambers-II. Acta Astronaut. 1976, 3, 735–757. [Google Scholar] [CrossRef]
- Emelyanov, V.N.; Teterina, I.V.; Volkov, K.N.; Garkushev, A.U. Pressure oscillations and instability of working processes in the combustion chambers of solid rocket motors. Acta Astronaut. 2017, 135, 161–171. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, P.; Wang, Z.; Xu, G.; Jin, B. Numerical investigation of mode competition and cooperation on the combustion instability in a non-premixed combustor. Acta Astronaut. 2022, 198, 271–285. [Google Scholar] [CrossRef]
- Ji, S.; Wang, B.; Zhao, D. Numerical analysis on combustion instabilities in end-burning-grain solid rocket motors utilizing pressure-coupled response functions. Aerosp. Sci. Technol. 2020, 98, 105701. [Google Scholar] [CrossRef]
- Narayanaswarni, L.L.; Zinn, B.T.; Daniel, B.R. Investigation of the characteristics of the velocity-coupled response functions of solid propellants. In Proceedings of the 23rd Aerospace Sciences Meeting, Reno, NV, USA, 14–17 January 1985. [Google Scholar]
- Bernardini, M.; Cimini, M.; Stella, F.; Cavallini, E.; Di Mascio, A.; Neri, A.; Martelli, E. Large-eddy simulation of vortex shedding and pressure oscillations in solid rocket motors. AIAA J. 2020, 58, 5191–5201. [Google Scholar] [CrossRef]
- Flandro, G.A.; Jacobs, H.R. Vortex generated sound in cavities. In Proceedings of the Aeroacoustics Conference, Seattle, WA, USA, 15–17 October 1973. [Google Scholar]
- Sun, B.B.; Li, S.P.; Su, W.X.; Li, J.W.; Wang, N.F. Effects of gas temperature on nozzle damping experiments on cold-flow rocket motors. Acta Astronaut. 2016, 126, 18–26. [Google Scholar] [CrossRef]
- Temkin, S.; Dobbins, R.A. Attenuation and dispersion of sound by particulate-relaxation processes. J. Acoust. Soc. Am. 1966, 40, 317–324. [Google Scholar] [CrossRef]
- Fabignon, Y.; Anthoine, J.; Davidenko, D.; Devillers, R.; Dupays, J.; Gueyffier, D.; Hijlkema, J.; Lupoglazoff, N.; Lamet, J.M.; Tessé, L.; et al. Recent advances in research on solid rocket propulsion. J. Aerosp. Lab. 2016, 30, 1–14. [Google Scholar]
- Fowler, J.; Rosenthal, J. Missile vibration environment for solid propellant oscillatory burning. In Proceedings of the 7th Propulsion Joint Specialist Conference, Salt Lake City, UT, USA, 14–18 June 1971. [Google Scholar]
- Hughes, P.M. The Influence of the Elasticity of the Casing and Grain on the Combustion Instability of a Solid Rocket Motor. DREV Memo. 1981, 2588, 81. [Google Scholar]
- Beal, T.R. Dynamic stability of a flexible missile under constant and pulsating thrusts. AIAA J. 1965, 3, 486–494. [Google Scholar] [CrossRef]
- Wu, J.J. On the stability of a free-free beam under axial thrust subjected to directional control. J. Sound Vib. 1975, 43, 45–52. [Google Scholar] [CrossRef]
- Greatrix, D.R. Parametric analysis of combined acceleration effects on solid-propellant combustion. Can. Aeronaut. Space J. 1994, 40, 68–73. [Google Scholar]
- Greatrix, D.R. Combined structural oscillation effects on solid rocket internal ballistics. In Proceedings of the 35th Joint Propulsion Conference and Exhibit, Los Angeles, CA, USA, 20–24 June 1999. [Google Scholar]
- Greatrix, D.R.; Harris, P.G. Structural vibration considerations for solid rocket internal ballistics modeling. In Proceedings of the 36th AIAA/ASME/SAE/ASEE Joint Propulsion Conference and Exhibit, Las Vegas, NV, USA, 24–28 July 2000. [Google Scholar]
- Loncaric, S.; Greatrix, D.R.; Fawaz, Z. Star-grain rocket motor-nonsteady internal ballistics. Aerosp. Sci. Technol. 2004, 8, 47–55. [Google Scholar] [CrossRef]
- Montesano, J.; Behdinan, K.; Greatrix, D.R.; Fawaz, Z. Internal chamber modeling of a solid rocket motor: Effects of coupled structural and acoustic oscillations on combustion. J. Sound Vib. 2008, 311, 20–38. [Google Scholar] [CrossRef]
- Zhang, D.; Cheng, S.; Xu, F.; Hu, Y.; Li, H. Study on nonlinear vibration of simplified solid rocket motor model. Aerosp. Sci. Technol. 2019, 93, 105302. [Google Scholar] [CrossRef]
- Mason, T.D.; Folkman, S.; Behring, M. Thrust oscillations of the Space Shuttle solid rocket Booster motor during static tests. In Proceedings of the 15th Joint Propulsion Conference, Las Vegas, NV, USA, 18–20 June 1979. [Google Scholar]
- Gori, F.; De Stefanis, M.; Worek, W.M.; Minkowycz, W.J. Transient thermal analysis of Vega launcher structures. Appl. Therm. Eng. 2008, 28, 2159–2166. [Google Scholar] [CrossRef]
- Ji, S.; Wang, B. Modeling and analysis of triggering pulse to thermoacoustic instability in an end-burning-grain model solid rocket motor. Aerosp. Sci. Technol. 2019, 95, 105409. [Google Scholar] [CrossRef]
- Version, A.N.Y. 2020R2 User’s Guide; ANSYS, Inc.: Pittsburgh, PA, USA, 2020. [Google Scholar]
- Li, Q.; Liu, P.; He, G. Fluid-solid coupled simulation of the ignition transient of solid rocket motor. Acta Astronaut. 2015, 110, 180–190. [Google Scholar] [CrossRef]
- Bathe, K.J.; Zhang, H.; Wang, M.H. Finite element analysis of incompressible and compressible fluid flows with free surfaces and structural interactions. J. Comput. Struct. 1995, 56, 193–213. [Google Scholar] [CrossRef]
- Xu, G.; Liu, P.; Ao, W.; Wang, Z.; Jin, B. Numerical investigation of thermoacoustic instability caused by small disturbance in a solid rocket motor. Aerosp. Sci. Technol. 2021, 113, 106678. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Dowell, E.H.; Hall, K.C. Modeling of fluid-structure interaction. Annu. Rev. Fluid Mech. 2001, 33, 445–490. [Google Scholar] [CrossRef]
- Cook, R.D.; Saunders, H. Concepts and Applications of Finite Element Analysis, 2nd ed.; John Wiley & Sons: New York, NY, USA, 1984. [Google Scholar]
- Giordano, J.; Jourdan, G.; Burtschell, Y.; Medale, M.; Zeitoun, D.E.; Houas, L. Shock wave impacts on deforming panel, an application of fluid-structure interaction. Shock Waves 2005, 14, 103–110. [Google Scholar] [CrossRef]
- Li, S.B.; Wang, Z.G.; Huang, W.; Lei, J.; Xu, S.R. Design and investigation on variable Mach number waverider for a wide-speed range. Aerosp. Sci. Technol. 2018, 76, 291–302. [Google Scholar] [CrossRef]
- Zhang, T.T.; Wang, Z.G.; Huang, W.; Yan, L. Parameterization and optimization of hypersonic-gliding vehicle configurations during conceptual design. Aerosp. Sci. Technol. 2016, 58, 225–234. [Google Scholar] [CrossRef]
- Levine, J.N.; Baum, J.D. A numerical study of nonlinear instability phenomena in solid rocket motors. AIAA J. 1983, 21, 557–564. [Google Scholar] [CrossRef]
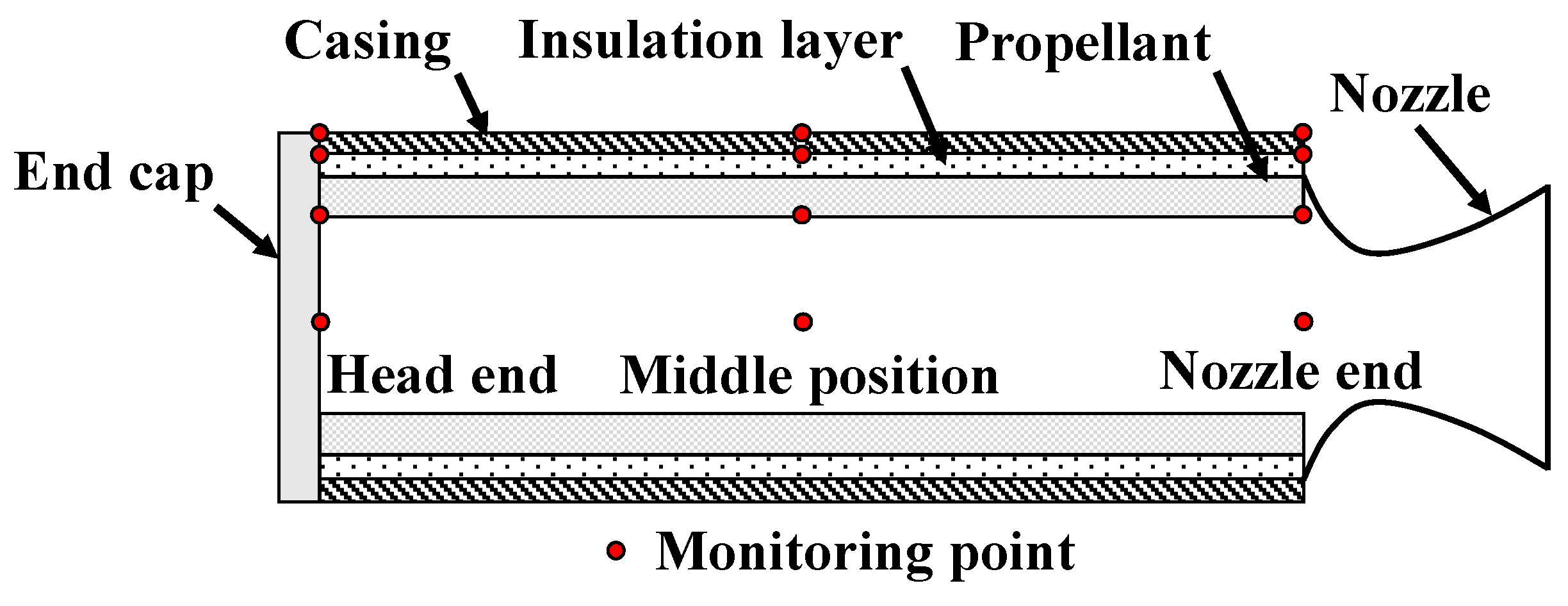

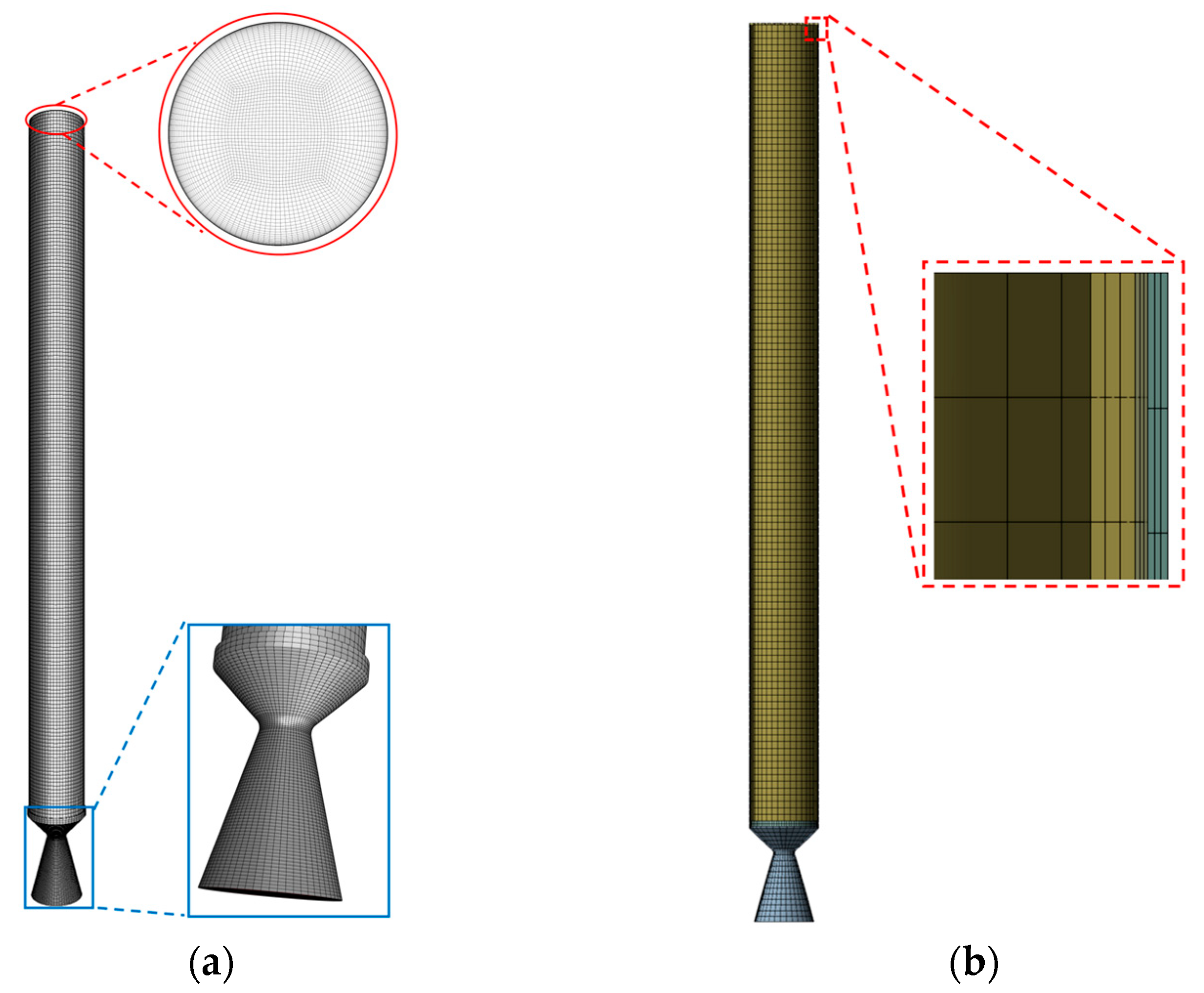

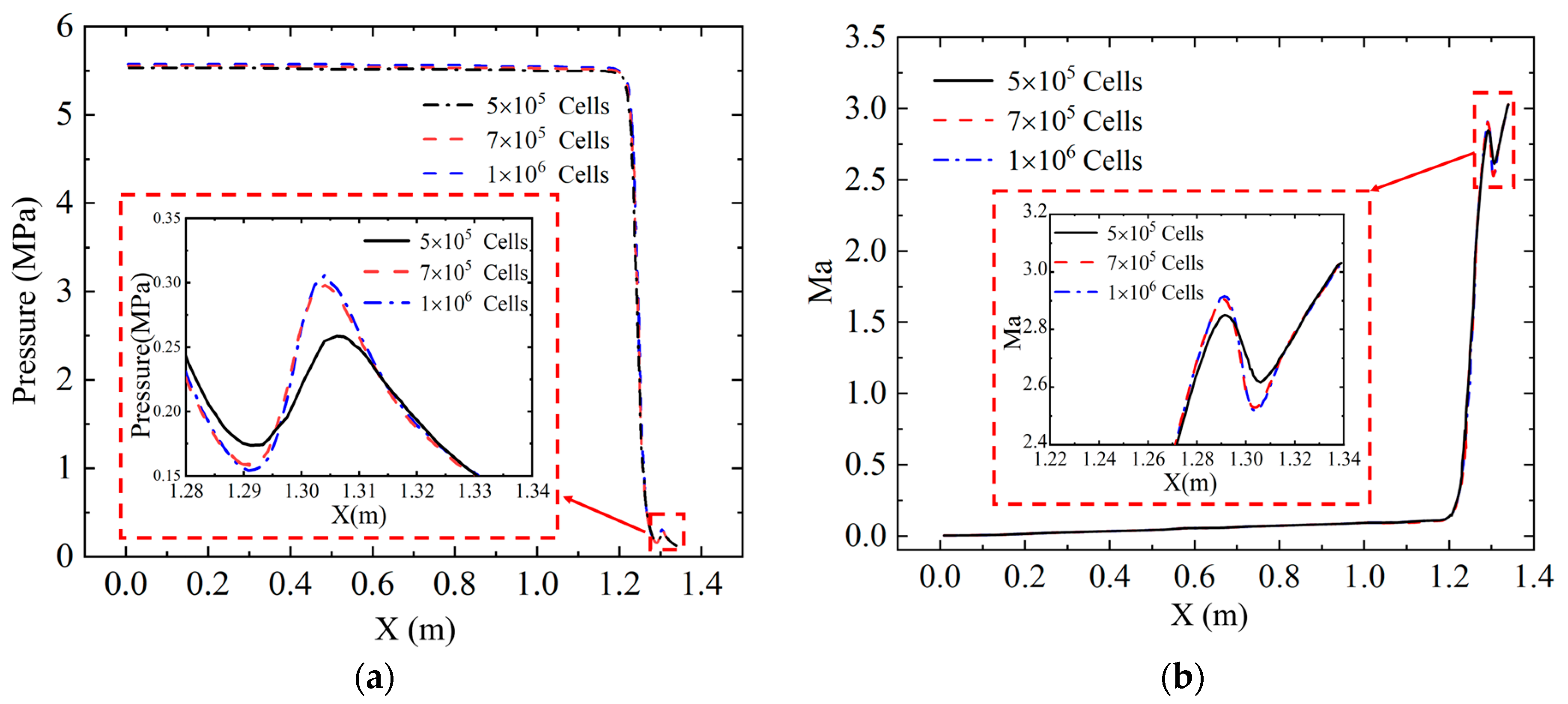



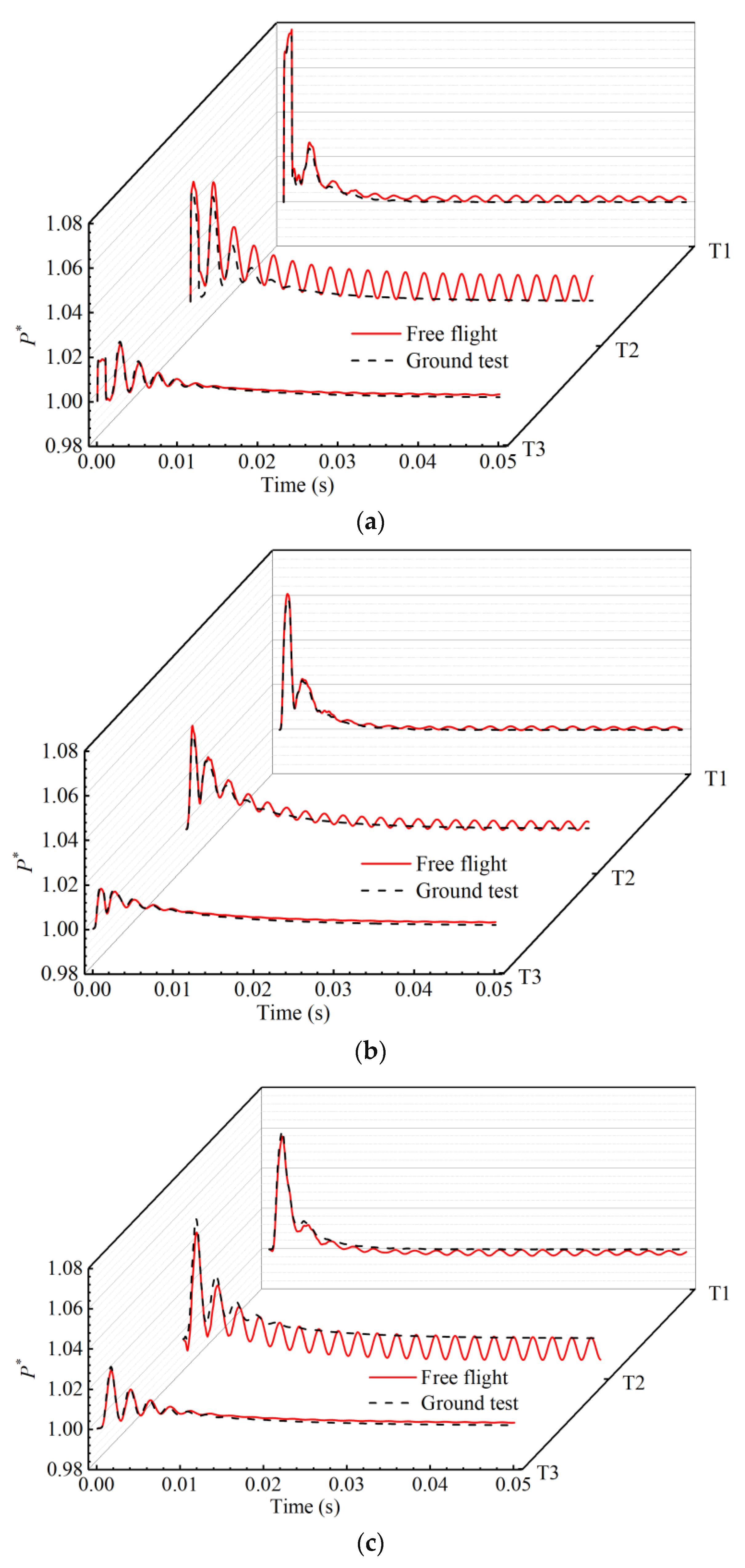




| The SRM length (mm) | 1300 |
| The SRM diameter (mm) | 105 |
| Initial length-to-diameter ratio of the combustor | 11 |
| Initial propellant thickness (mm) | 35 |
| Insulation layer thickness (mm) | 1 |
| Casing thickness (mm) | 1.5 |
| Casing inner wall radius (mm) | 50 |
| Nozzle throat radius (mm) | 16 |
| Nozzle expansion ratio | 7.5 |
| γ | 1.27 |
| Cp (J/(kg·K)) | 1675 |
| Rg (J/(kg·K)) | 360.03 |
| M (g/mol) | 23.09 |
| T (K) | 2500 |
| Casing density (kg/m3) | 4500 |
| Casing elastic modulus (GPa) | 110 |
| Casing Poisson’s ratio | 0.33 |
| Insulation layer density (kg/m3) | 1240 |
| Insulation layer elastic modulus (MPa) | 12.2 |
| Insulation layer Poisson’s ratio | 0.498 |
| Propellant density (kg/m3) | 1600 |
| Propellant elastic modulus (MPa) | 21.7 |
| Propellant Poisson’s ratio | 0.496 |
| Burning Instants | Casing Average Temperature (K) | Elastic Modulus (GPa) |
|---|---|---|
| T1 | Tc = 340.68 | 103.64 |
| T2 | Tc = 453.78 | 95.89 |
| T3 | Tc = 907.96 | 67.18 |
| Burning Instants | 1st Order (Hz) | 2nd Order (Hz) |
|---|---|---|
| T1 | 395.31 | 801.63 |
| T2 | 430.29 | 861.12 |
| T3 | 441.07 | 882.14 |
| Cases | Aerodynamic Force Frequencies (Hz) | Casing Temperature (K) | Pulse Intensities (kg/(m2·s)) | Pulse Duration (s) |
|---|---|---|---|---|
| CaseT1-1 | 395.31 | 340.68 | 100 | 0.001 |
| CaseT1-2 | - | 298.15 | 100 | 0.001 |
| CaseT2-1 | 430.29 | 453.78 | 100 | 0.001 |
| CaseT2-2 | - | 298.15 | 100 | 0.001 |
| CaseT3-1 | 441.07 | 907.96 | 100 | 0.001 |
| CaseT3-2 | - | 298.15 | 100 | 0.001 |
| Cases | Aerodynamic Force Frequency (Hz) | Casing Temperature (K) |
|---|---|---|
| CaseT2-1 | 430 | 453.78 |
| CaseT2-3 | 200 | 453.78 |
| CaseT2-4 | 600 | 453.78 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, G.; Li, C.; Pu, W.; Zhou, B.; Yang, H.; Yang, Z. Numerical Investigation on the Mechanism of Solid Rocket Motor Instability Induced by Differences between On-Ground and In-Flight Conditions. Aerospace 2024, 11, 215. https://doi.org/10.3390/aerospace11030215
Wang G, Li C, Pu W, Zhou B, Yang H, Yang Z. Numerical Investigation on the Mechanism of Solid Rocket Motor Instability Induced by Differences between On-Ground and In-Flight Conditions. Aerospace. 2024; 11(3):215. https://doi.org/10.3390/aerospace11030215
Chicago/Turabian StyleWang, Ge, Chengke Li, Weiqiang Pu, Bocheng Zhou, Haiwei Yang, and Zenan Yang. 2024. "Numerical Investigation on the Mechanism of Solid Rocket Motor Instability Induced by Differences between On-Ground and In-Flight Conditions" Aerospace 11, no. 3: 215. https://doi.org/10.3390/aerospace11030215
APA StyleWang, G., Li, C., Pu, W., Zhou, B., Yang, H., & Yang, Z. (2024). Numerical Investigation on the Mechanism of Solid Rocket Motor Instability Induced by Differences between On-Ground and In-Flight Conditions. Aerospace, 11(3), 215. https://doi.org/10.3390/aerospace11030215





