A Road Map to the Structural Optimization of a Type-C Composite UAV

Abstract

The present paper investigates the design process and the dimensioning of a tailless type-C composite sandwich unmanned aerial vehicle (UAV). The objective is to investigate an innovative aircraft configuration which exceeds the standard approach of ribs and spars and replaces them with a sandwich structure for future unmanned aerial systems. The necessity of carbon fiber-reinforced materials arose due to the weight constraint of a Class C UAV, i.e., the whole vehicle must be under 25 kg, which limits the mass of the structure to 9 kg. The structural design of composite structures differs from the one of traditional isotropic structures. The number of holes should be limited, as drilling down the composite aerostructure would conclude to the generation of delaminations. In addition, the joints between sections with different thicknesses could lead to stress concentrations and disbands. Therefore, the present report is crucial for the continuance of the present project as it has contributed both to the structural design and assessment of the UAV. This work focusses on the computation of loads, the process of structural sizing through a multi-disciplinary optimization approach, and the simulation-based structural proof. Particular attention is paid to the specifically developed semi-analytical method for predicting the aero-elastic load. Based on the detailed finite element model of the global structure, the applicability of the minimum number of bolts as a major structural joining variant is proven. The design process from single components to the assembly of the overall aircraft results in the realization of the demonstrator structure.

1. Introduction

In the realm of aircraft design, numerous optimization efforts have been undertaken in the past three decades. These endeavors primarily concentrate on achieving optimal aerodynamic or structural designs [1,2]. Sobieski and Haftka’s analysis of advancements [1] reveals that the multi-disciplinary optimization methodology (MDO) has evolved beyond its origins in structural optimization, expanding in both breadth and depth to encompass the complete array of disciplines necessary for each specific application. As per the findings of this survey, the predominant challenges hindering the realization of MDO technology’s full potential seem to revolve around the demanding computational requirements and complexities arising from the organization of MDO tasks.

Bartholomew [2] offers a definition of multidisciplinary optimization (MDO) that integrates cutting-edge analysis tools and discusses its role as a pivotal tool within the framework of concurrent engineering. The discussion emphasizes that MDO allows for the addressing of constraints across a diverse range of disciplines from the early stages of the design process. Kroo [3] underscores crucial aspects of MDO applications during the preliminary design phase and provides an overview of the field’s evolution, covering computational tools, strategies, and challenges. While many researchers primarily concentrate on applying MDO to the overall aircraft design [4,5] during the conceptual design stage as well as the wing design [6,7] during the preliminary design phase, Sobester and Keane [4] conduct a multidisciplinary analysis for UAV airframes, focusing on a blended wing body design and optimizing geometry through constraint analysis. Rajagopal et al. [5] approach the conceptual design of a UAV as an optimization problem, addressing initial aircraft sizing via this method. In the conceptual design phase, disciplines typically utilize low-fidelity analysis tools, such as empirical relations, making the MDO approach less demanding in terms of computing power and time. Conversely, during the preliminary design phase, as the design matures, disciplines employ high-fidelity analysis tools such as computational fluid dynamics (CFD) and the finite element method (FEM), necessitating substantial computing resources. Additionally, in this stage, the coupling between disciplines intensifies, leading to increased memory and computing power requirements.

Numerous research endeavors in the realm of aircraft design employing the multidisciplinary optimization (MDO) approach have concentrated on its application to traditional commercial transport aircrafts and contemporary fighter aircrafts. In the study by Grossman et al. [6], the integration of aerodynamic and structural design for a subsonic transport wing was pursued in order to achieve the minimum weight while adhering to range constraints. Two recommended methods aimed at mitigating the computational load were presented. The modular sensitivity method sought to reduce the expense of sensitivity derivatives, thus enabling the utilization of black box disciplinary software packages. The study demonstrated that derivatives of aeroelastic responses and divergence speed could be computed without the costly calculation of the derivatives of aerodynamic influence coefficients and structural stiffness matrices. Additionally, to curtail computational costs, a sequential approximate optimization approach was implemented. Dovi and Wrenn [7] introduced a novel technique called KSOPT, utilizing an envelope function formulation to transform a constrained optimization problem into an unconstrained one. The primary advantage of this method in multi-objective optimization lies in eliminating the need for the separate optimization for each objective, a requirement of certain optimization methods. Comparative studies were conducted using a typical wide-body transport aircraft, evaluating this method against two classic multi-objective optimization techniques, namely the penalty function method and global criterion method.

Wakayama et al. [8] report fundamental findings from wing planform optimization, aiming for the minimum drag while adhering to the constraints on structural weight and maximum lift. The study explicitly highlights that highly tapered wings, favored for achieving large spans, are strongly supported by considerations of induced drag and structural factors. Parasite and compressibility drag exhibit limited impact on wing taper, necessitating the imposition of maximum lift constraints in order to ensure the generation of realistic tip chords. Martins et al. [9] concentrate on showcasing a novel integrated aerodynamic-structural design methodology for aerospace vehicles. Utilizing high-fidelity models for both aerodynamics and structure, they employ a high-fidelity coupling procedure, employing Euler equations for aerodynamic analysis and a detailed finite element method (FEM) model for the primary structure. Carrier [10] delineates the multidisciplinary optimization (MDO) system implemented at ONERA, encompassing various optimization algorithms, including a gradient-based optimizer and a genetic algorithm (GA). While aerodynamic and structural disciplines are analyzed with high-fidelity methods, other disciplines, such as engine performance and flight mechanics, are assessed using simpler methods. This methodology is applied to optimize the performance of a high-speed civil transport aircraft, with the overarching objective of maximizing the aircraft range, while considering multiple design constraints. Kumano et al. [11] introduce the MDO system for small jet aircraft design via the integration of computational fluid dynamics (CFD) codes and a NASTRAN-based aeroelastic structural interface code. They employ a kriging model to reduce the computational time for evaluating the objective function in the multi-objective genetic algorithm (MOGA). Multiple non-dominated solutions, reflecting trade-offs among drag, structural weight, drag divergence, and pitching moment, are identified. Kim et al. [12] present a multidisciplinary design for a supersonic fighter wing with multiple objectives using response surface methodology. They select nine wing and airfoil parameters as aerodynamic design variables, and also add four structural variables to determine wing skin thickness. Multipoint design optimization is conducted for three representative design points, accounting for various flight conditions. In [13], the authors propose a multi-fidelity optimization approach for a composite wing, considering both structural and aeroelastic constraints. Kilimtzidis et al. [14] suggest an optimization framework for composite aircraft wings based on the equivalent plate method, achieving significant computational gains while maintaining high accuracy. Additionally, Imumbhon et al. [15] explore the design and structural analysis of a reciprocating wing for a vertical take-off and landing (VTOL) UAV. Bartoli et al. [16] introduce a surrogate-based optimization algorithm, which demonstrates more robust results than conventional methods. Finally, Sekar et al. [17] present an optimization study of a UAV wing, focusing on weight reduction while preserving a high strength/weight ratio.

In recent times, unmanned aerial vehicles (UAVs) have captured the interest of aerospace engineers due to their potential applications in reconnaissance, including firefighting, surface reconnaissance, and search and rescue operations. Consequently, there has been extensive research focused on UAV applications. Hutagalung et al. [18] performed a structural analysis of a composite UAV, but did so without using any optimization algorithms. Also, Blair and Takahashi [19] followed the traditional spar–rib approach for the design of the wings of a UAV. Gonzalez et al. [20] examine the application of evolutionary algorithms (EAs) for both single- and multi-objective airfoil optimization. They underscore the drawbacks of employing gradient-based approaches for problems featuring multi-objective, multi-modal, and non-differentiable functions. Additionally, they demonstrate that EAs possess the capability to identify global optima and can operate in parallel by adapting to various solver codes. In another study, Gonzalez et al. [21] emphasize the challenges in UAV design arising from the diverse and non-intuitive nature of configurations and missions that these vehicles can undertake. An MDO framework is employed in [22], where two case studies utilize high-fidelity analysis codes. The first case involves dual-objective optimization, focusing on the detailed design of a single-element airfoil for a small UAV application. The fitness functions aim to minimize the drag at two different flight conditions, with constraints on the maximum thickness, maximum thickness location, and pitching moment. In the second case study, multi-criteria wing design optimization for a UAV is conducted, with fitness functions targeting the minimization of wave drag and spanwise cap weight. Rayed et al. [23] undertake the topology optimization of a UAV structure to achieve mass reduction and stress minimization, evaluating the vibrational and fatigue behavior of the UAV. Kumar et al. [24] implement structural optimization for a multi-rotor UAV, exploring various conceptual design frames of a Quadcopter, and investigating the impact of different material configurations. Martinez et al. [25] study the multi-objective and multi-scale optimization of composite structures for an aircraft overhead locker, successfully applying the optimization approach at both micro and macro levels. The advantages of evolutionary methods over classical algorithms in single and multi-objective optimization problems are well elucidated by Goldberg [26] and Deb [27].

This paper investigates the structural design of a tailless UAV as an optimization problem by considering the aerodynamics and structural concepts. To achieve the weight limitation, which is 9 kg structural weight, topology optimization analysis was performed to minimize the weight of the UAV. To increase its toughness setting as an objective function, the generated stresses minimization was used instead of the commonly used compliance matrix. Furthermore, through computational fluid dynamic (CFD) analysis, the coefficients for the drag and the lift as well as the forces acting on the aircraft wings and structure were calculated. Regarding the loads, analytical methods were also used to validate the computational calculations, providing a simplified way to apply the loads into all structural calculations. Finally, composite optimization analysis was used to optimize and provide the final lamination for each structural part of the proposed design. Both composite laminates and sandwich structures have been utilized for the weight reduction. The UAV design involves a small number of parts and connections, pursuant to the integrated design philosophy. The proposed procedure has enabled the design of a composite aircraft shell under 9 kg, which is capable of withstanding all structural and aerodynamic loads.

2. Studies and Conceptual Design

2.1. Computational Fluid Dynamics

A CFD analysis was performed on the UAV to help reveal all crucial information on the lift (C_L) and drag (C_d) coefficients, as well as the applied bending moments and shear forces onto the wings and structure of the drone in flight. It is a fact that the tailless design helps the reduction of parasitic drag and weight, as well as improving the stealth characteristics of the UAV. As Figure 1 depicts, the airflow direction was placed in front of the aircraft at a magnitude of 33 m/s. The slip conditions were set on the top, bottom, and side of the aircraft, while the outlet was set at the back of the aircraft.

The mesh generated for the CFD analysis, as shown in Figure 2, was an unstructured mesh of tetras with 10 layers of hexas close to the UAV geometry. The purpose of this was to accurately capture the boundary layer phenomena close to the surface. The total size of the model was ~8,300,000 elements. The target of this analysis was to perform a quick analysis that will capture the basic phenomena around the UAV and provide a preliminary estimation of the loads on the UAV structure. For this reason, no complex turbulence models were used, other than the 1-equation Spallart-Almaras [28] turbulence model (a very robust model for aerospace and external aerodynamics applications). Furthermore, no sensitivity analysis was performed regarding the size and refinement of the mesh or the turbulence model (Figure 3).

The CFD analysis revealed larger applied pressures at the tip of the aircraft and the edges of the wings, as expected. In addition, increased pressure was observed around the inlet of the aircraft. The pressure magnitude was approximately 1 atm, as indicated in Figure 4.

To allow the validation of the CFD calculations while providing a simplified and accurate way to iterate and assess the loads, an analytical solution was developed. The latter facilitates the estimation of the pressure distribution along the wing based on several variables shown in

Table 1. The variables required for the calculation of the distributed pressure along the wing of the aircraft.

Root Chord, c [m]	0.586
Tip Chord [m]	0.241
Span, b [m]	2.800
Take-off Gross Weight, TOGW [N]	300.000
Load factor n = L/W	4.000
Wing Area, S [m2]	1.158
Aspect Ratio, AR	6.771
Wing Taper Ratio	0.411
Safety Factor	1.500
Lift, Lw [N]	900.000
Number of Wing Sections	10.000
y (spanwise interval) [m]	0.140
Lift factor [N/m2]	1615.633
Mean Aerodynamic Chord, MAC [m]	0.437
y MAC [m]	0.603

. The calculation is based on Schrenk’s method [29], which is one of the most popular methods used to perform a preliminary analysis of wing loads, along with the Diederich method [30] and the Fourier series method. Schrenk’s method is relatively accurate when the lift distribution on the wing is close to the elliptical distribution. The lift distribution on the wing is analogous to the shape of the wing (as one of the main drivers of lift generation is the area of the wing); therefore, Schrenk’s method can be used with acceptable results on the unswept and untwisted wings of rectangular shape or trapezoidal shape, similar to the proposed wing design. In very simple and concise steps, the chord of the wing is calculated as an average of the actual chord and an elliptical one, and the wing is discretized in a number of sub-areas with the lift being calculated on each of these sub-areas. For the lift generated by the wing, the total weight of the aircraft is considered through the load factor n = L/W, and, in most cases, a safety factor is applied on top of those loads. The shear forces and bending moments are calculated along the wing in the same philosophy as in a typical shear/moment diagram calculation of a cantilever problem in statics theory. The presented procedure is efficient, accurate, and correlates well with the CFD analysis. The largest benefit is that, in the case of redesign and modifications, it instantaneously provides distributed pressures on the aircraft fuselage and wings, thus saving a great amount of computational time which would usually be required to set up a new CFD analysis. Also, it provides a very accurate and simple method to apply the distributed pressures onto the FEA models, through a polynomial equation. The final distribution of the pressure is depicted in Figure 5.

2.2. Topology Optimization

Due to the entailed challenges associated with the proposed problem, especially the extremely challenging weight limitations, optimization studies were initially conducted in order to define the optimum and critical structural paths, allowing for the most efficient design. For better understanding and to facilitate the thorough examination of the different areas, the studies conducted were distinguished in two steps as follows: (a) the fuselage optimization and (b) the wings optimization. Both analyses were based on topology optimization and, specifically, the “Level Set” methodology, using an initial design space, with inertia relief [30] being used to account for the lack of boundary conditions (BC), therefore presenting a more realistic structural representation of the aircraft in flight. In addition, the objective function (OF) was set to minimize the maximum stresses which appear on the vehicle under loading, with the overall weight being constrained to less than 20% of the initial mass of the design space. The material for this analysis was selected to be isotropic with engineering constants being close to those for solid aeronautic aluminum (

Table 2. Homogenized material properties for the preliminary optimization analysis.

	Aluminum T6-7075
	Physical properties
E [MPa]	70,000
v	0.32
G [MPa]	26
ρ [ton/mm3]	3 × 10−9
	Strength properties
Xt [MPa]	480
S [MPa]	330

). It is worth noting that all the optimization procedures utilized in this work, using the Altair Optistruct software, are gradient-based and either sequential quadratic programming (SQP) or dual optimizer programming based on separable convex approximation (DUAL) have been utilized, depending on the number of design variables (https://2021.help.altair.com/2021/hwsolvers/os/topics/solvers/os/gradient_based_opt_method_intro_c.htm (accessed on 6 March 2024)).

For the analysis of the fuselage, several load cases were used, such as equivalent wing loads and the weight of the drone in parked position and landing. The results are depicted in Figure 6. As expected, considering the color contour from blue to red, an excess of material is required around the housings of the wings and on the top and rear of the aircraft in order to maintain its structural integrity. The material concentration around the wing housing facilitated sturdy support conditions for the wings, as they simulated the boundary conditions of a cantilever beam. Furthermore, the prediction of material continuity on the top and rear of the drone was required as it kept the entire structure together. The wing housings acted as a shear web for the fuselage, as they bore the shear stresses developed by the wings at the fuselage sides and the bending moment, while the top and the rear parts deliver the loads arising by the weight of the fuselage and the aerodynamic loads.

For the wings, where the pressure profile is qualitatively visualized in Figure 7, the same procedure was followed (Figure 8), with multiple load cases used, such as the distributed pressure (obtained from both the CFD and analytical studies presented in the previous section, Figure 5b) and the wings’ own weight. Finally, the boundary conditions used were as described above, through the use of the inertia relief computational technique.

The results highlighted as critical regions were the top and bottom faces of the wing, located close to the leading edge and up to the middle of its width. In addition, excess material was required on the top and bottom through the thickness of the cross section, rendering the entire wing hollow.

3. Structural Design

This section is dedicated to the conceptual design of the main structural components of the UAV, following the design ideas and results discussed in the previous section. Initially, the assembly of the UAV is explained and analyzed. Furthermore, the components are looked into in depth, based on manufacturing considerations. Finally, the findings of composite optimization analysis and stress analysis will produce the outcome as to whether the proposed UAV design is feasible, considering manufacturing constraints as well as weight and stress limitations.

3.1. Full Assembly Design of the UAV

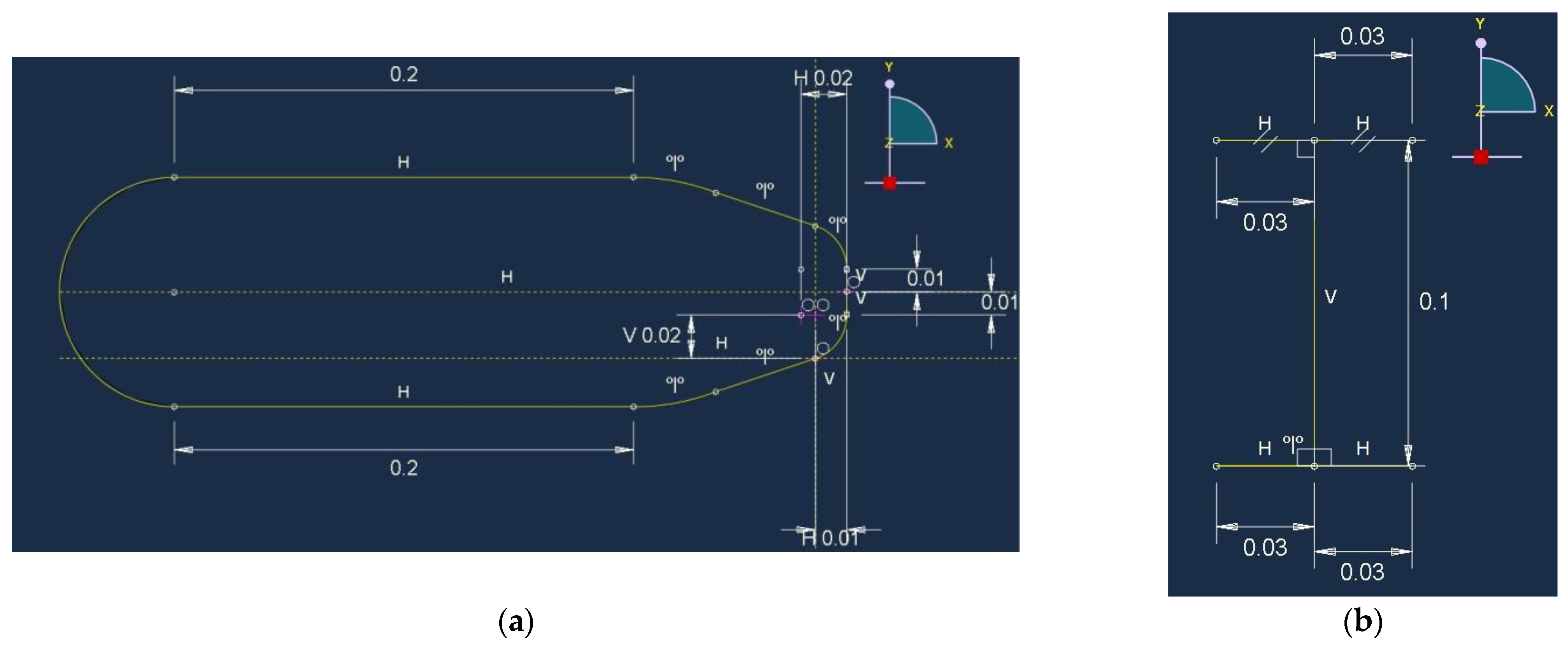
In this section the full assembly of the current drone design is demonstrated, and the structural components are listed (

Table 3. List of the structural components of the UAV.

Part Number	Part Name
001	Main cap
002	Cargo holder
003	Wings
004	Wing tips
005	Quick release cap
006	Bolts and bolt holders

) and explained. In Figure 9, the preliminary structural design of the UAV is depicted. More specifically, the design philosophy is based on a large container (002), which will host the energy system, the electronics, and the first-person-view (FPV) camera. The latter will be jointed with the wings (003) through the 001 component, an intermediate structural and sturdy component. The 001 component is the main cap of the UAV. It protects the cargo, provides fast and easy access to the cargo holder for maintenance, and is connected to the wings and the cargo holder with bolts (006). The quick release cap (005) completes the aerodynamic shape of the UAV as this helps with fast refueling.

Main cap—001

The main cap is a key structural component merging the wings and the cargo holder. More specifically, it is connected to the cargo holder with four M6 bolts, and it connected to the wings via three M6 bolts. This part contributes mainly to the structural integrity of the UAV, designed as a sandwich structure, and bears both the weight of the wings and the cargo holder through the bolt connections. Sandwich structures offer a very high bending stiffness/weight ratio, constituting a very good option in weight-sensitive applications.

Cargo holder—002

The cargo holder is the structural component containing and supporting all the mandatory apparatus for the drone operations, including electronics, the gas tank, fuel cells, batteries, the FPV camera, motors, and the landing system.

Wings—003

The wings are designed as a single unit component attached to the main cap. The wings are the main structural component responsible for bearing all flight loads, as well as for providing the aerodynamic lift. It should be noted that the wings are also responsible for connecting/supporting the vertical rotors and for transferring the loads through the whole structure, both in VTOL and normal flight mode.

Wing tips—004

The wing tips are responsible for the aerodynamic stability of the aircraft.

Quick release cap—005

The quick release cap contributes mostly to the aerodynamics of the UAV by fulfilling its aerodynamic shape. It is also expected to provide fast refueling access to the tank and hydrogen system.

Bolts and inserts—006

All bolted connections have been realized with the help of solid carbon patches (supporting and transmitting shear loads into the composite skin) and threaded inserts (keenserts) being inserted in the patches and skin during lamination. The low number of bolted connections would reduce the difficulties in the assembly of the whole UAV.

3.2. Material Selection and Manufacturing Considerations

In Figure 9, the conceptual design of the UAV is depicted. Several manufacturing considerations had to be considered in order to achieve both the desired weight limit (<9 kg) and the mandatory structural integrity. The manufacturing considerations include the following:

• Skin thickness on each structural component of the aircraft
• The skin lay-up on each structural component
• The distinction in regions entailing only composite laminates or sandwich laminates
• The composite ply thicknesses in each distinct laminate

For the time being, the materials used were picked based on (a) their physical properties; (b) their fracture properties; and, of course, (c) their density. The latter are distinguished in a UD carbon fiber composite material, a woven carbon fiber, and a polymeric foam used as the core material in selected areas. Their properties are shown in

Table 4. Material properties.

	UD Carbon Sigratex CE 1007-15038 [31]	Woven Carbon Fiber Fabric 24K 385 g/m2, Tenax IMS65 E23 [32]	Foam Core AIREX C70.48 [33]
	Physical properties
E1 [MPa]	121,000	48,700	35
E2 [MPa]	10,400	18,500	35
V12	0.325	0.32	0.25
G12 [MPa]	5100	4200
G23 [MPa]	4300	4200
ρ [kg/m3]	1580	1420	38
	Strength properties
Xt [MPa]	2537	1302	0.95
Xc [MPa]	1580	1092	0.6
Yt [MPa]	82	1305	0.55
Yc [MPa]	236	1087
S12 [MPa]	90	87.9

.

3.3. Composite Optimization

The optimization procedure is finalized with the composite optimization. The latter digs in the mechanics of composites, providing the optimum stack lay-up and the facilitation of composite manufacturing constraints. This is a two-step procedure. The first step provides general information regarding the composite lay-up thickness throughout the entire UAV. This is critical as it contributes to the redesign of the UAV. The second step optimizes the thickness and lay-up of each laminate separately, based on composite manufacturing constrains.

Free-size optimization

The procedure started with a free-size composite optimization analysis. The free-size optimization analysis facilitated the faster estimation of the entire UAV thickness. It also indicated which regions and which sandwich laminates of the UAV should entail only composite skin. Concerning the OF for this optimization study, the minimization of the overall stresses was set with the following constrains: (a) the mass to remain below 9 kg and (b) to maintain a symmetric lay-up throughout the thickness of the aircraft (a constraint that facilitates the manufacturability of each skin of the laminate). The loads input were as follows: (a) the final weight, namely 25 kg, (b) the distributed pressure calculated in Section 2.2 by hand calculations and CFD analysis, and finally (c) 3 g acceleration in all three directions (used separately). For optimization purposes (as a design space), the entire UAV structure was modelled as a single laminate, initially comprising several thick plies (super plies), one for each selected material, with different ply orientations. This procedure allows to identify the most appropriate plies in each area of the structure with their respective thicknesses. 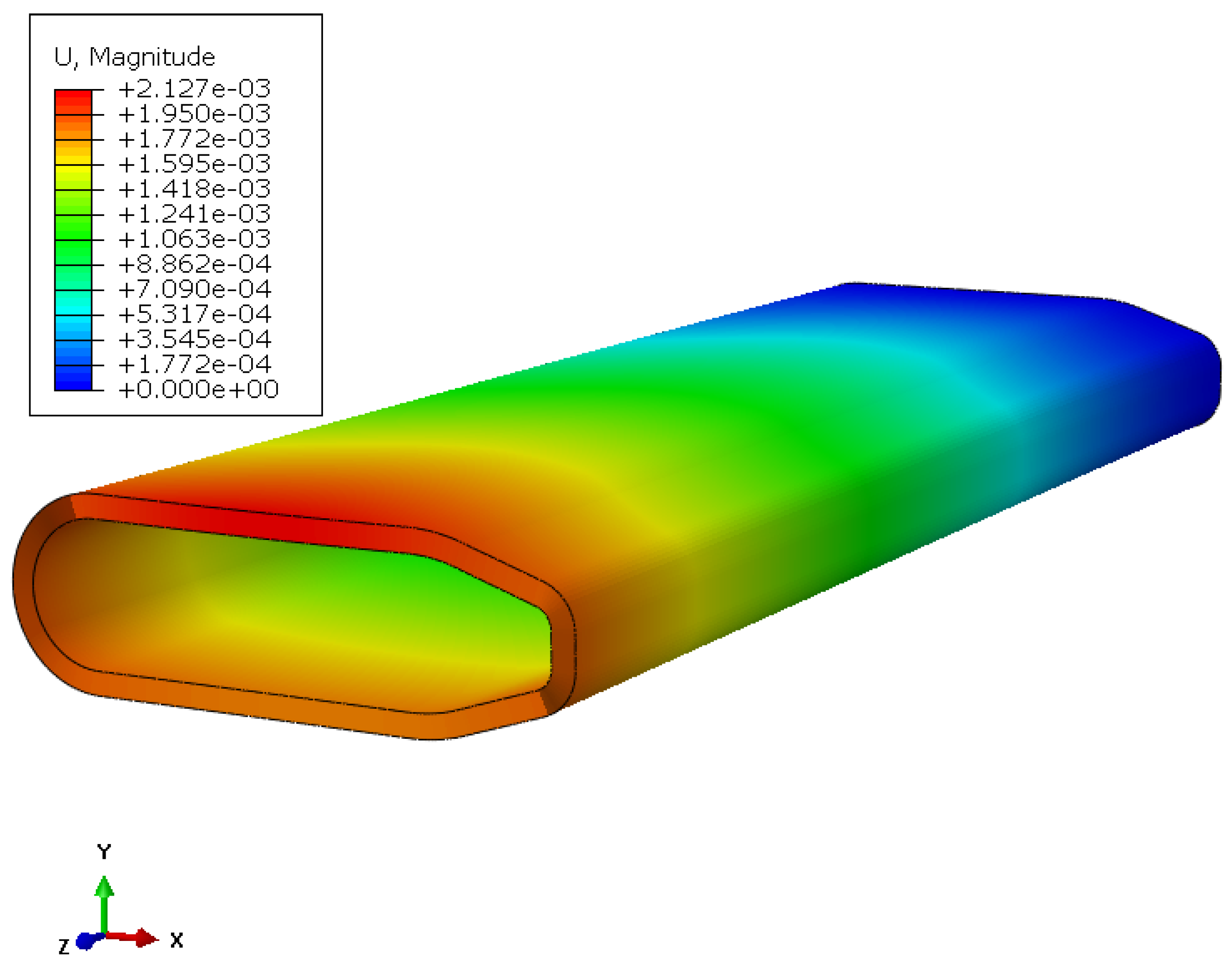Figure 10 depicts the initial calculated values of the thickness laminates throughout the whole aircraft.

Size optimization

The second step differs from the first step in terms of manufacturing constrains and design space. In this analysis, we focus on the optimization of each distinct laminate configuration, based on the ply selection of the previous step. The goal is to derive an optimal laminate configuration with the correct ply thickness based on all manufacturing constraints. The total thickness of each laminate will be equal to the one predicted by the free-size optimization step. 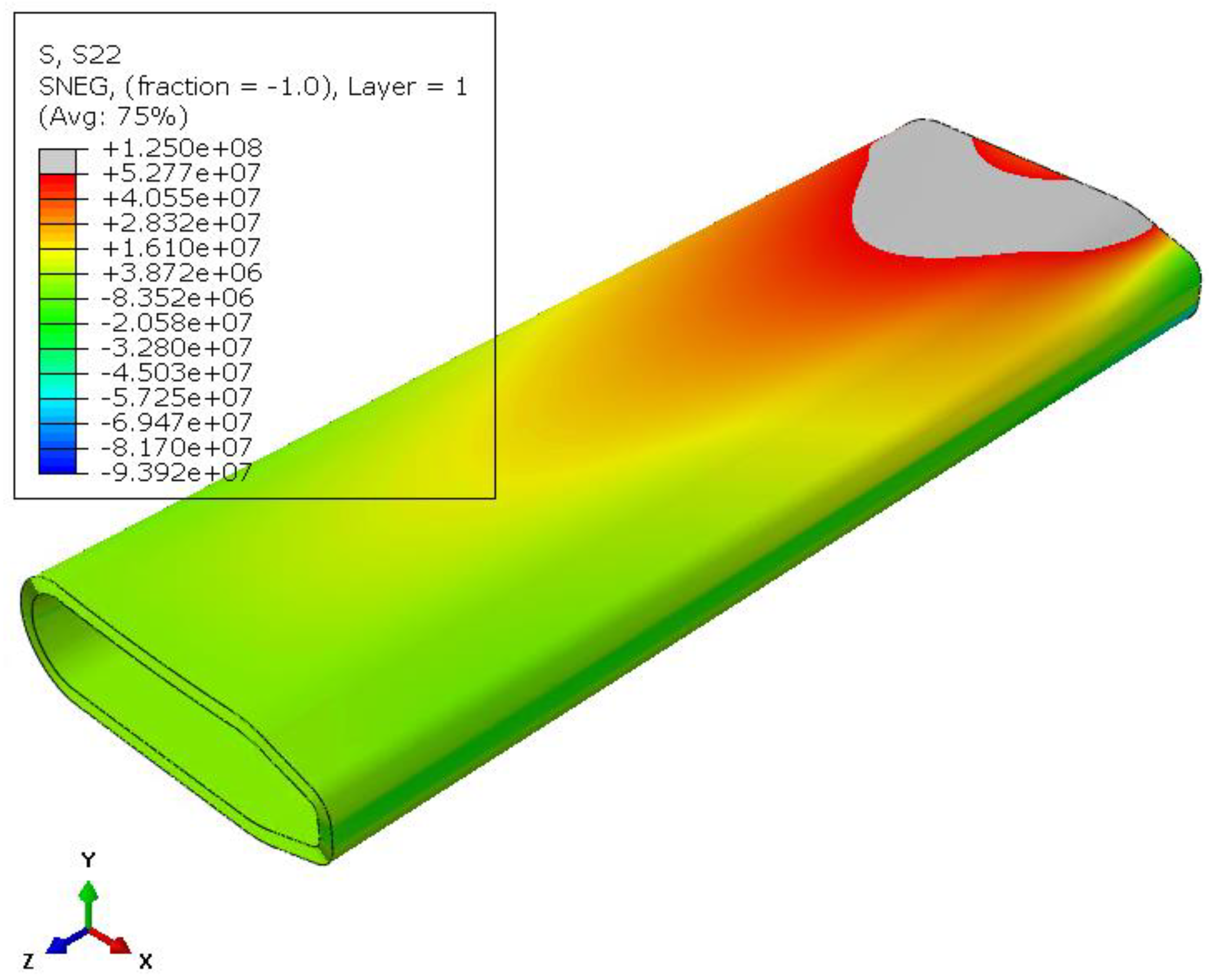In Figure 11, the updated design with the partitioned sub-components is illustrated. For this second step of the composite optimization, that is, the size optimization analysis, the same loads were applied as in the free-size optimization. The OF again was set to minimize the resulting stresses of the structure. The mass was constrained not to exceed 9 kg, while for each laminate, symmetry conditions regarding the lay-up were applied. The difference between this and the first step is that for each composite ply, a specific thickness was constrained. The thickness of each ply was set as the input based on the fabrication process of each composite material. Consequently, the UD ply thickness was set to 0.125 mm and the woven carbon to 0.25 mm. The optimizer utilizes this input value and results in a manufacturable lay-up for each composite part.

Following this step, the analytical design process presented in the next section was pursued, and the whole structure was partitioned into distinct thicknesses and components with ply transitions and shapes, taking into consideration both the manufacturability of the part and the aerospace standards.

4. Component Design

In this section, the final outcomes following the redesign of the structure and drone based on all the above studies are presented.

Table 5. Table of the weight of each component of the UAV.

Component	Weight [kg]
Main cap—001	1.545
Quick release cap—005	0.204
Cargo holder—002	2.877
Inserts—006	0.061
Wings—003a	0.952
Wings—003b and 003d	1.604
Wings—003c and 003e	1.00
Wings—003f (flaps)	0.255
Wing tips—004	0.275
Total	8.773

depicts all the parts of the aircraft and their respective weights.

Quick release cap—005

As already mentioned in Section 3.1, the quick release cap mostly contributes to the aerodynamics of the UAV (Figure 12). The laminate is comprised of woven carbon plies, and its total thickness is set 0.5 mm (

Table 6. Laminate properties of the 005 component.

Material	Thickness—h [mm]	Ply Orientation [deg]	Weight [kg]
Woven carbon	0.25	0	0.102
Woven carbon	0.25	0	0.102
	0.5		0.204

).

Main cap—001

The main cap is located under the quick release cap, and is a structural component of the drone. It protects the hydrogen supply, the fuel cells, the batteries, the electronics, and the FPV camera. It is connected to the cargo body and contributes significantly to the aerodynamics of the UAV. It is comprised of a sandwich composite laminate which entails both composite and foam core plies (

Table 7. Laminate properties of the 001 component.

Material	Thickness—h [mm]	Ply Orientation [deg]	Weight [kg]
Woven carbon	0.25	0	0.305
Woven carbon	0.25		0.305
Foam core	4–5	0	0.322
Woven carbon	0.25	0	0.305
Woven carbon	0.25		0.305
	5–6		1.545

), as depicted in Figure 13 and Figure 14.

Cargo holder—002

Inside the cargo holder, the fuel cells, the hydrogen system, the electronics, the batteries, and the FPV camera are located and supported. It is one of the main structural components of the drone, and the one the landing system is attached to. Similarly to the main cap, it is considered a key structural part, and is comprised of a sandwich composite laminate, as it is expected to anticipate greater loads than other parts. In Figure 15 and Figure 16, the skin and core of the sandwich laminate are depicted, and the material details are shown in

Table 8. Laminate properties of 002 component.

Material	Thickness—h [mm]	Ply Orientation [deg]	Weight [kg]
Woven carbon	0.25	45	0.612
Woven carbon	0.25	0	0.612
Foam core	3–7	0	0.428
Woven carbon	0.25	0	0.612
Woven carbon	0.25	45	0.612
	4–8		2877

.

Inserts—006

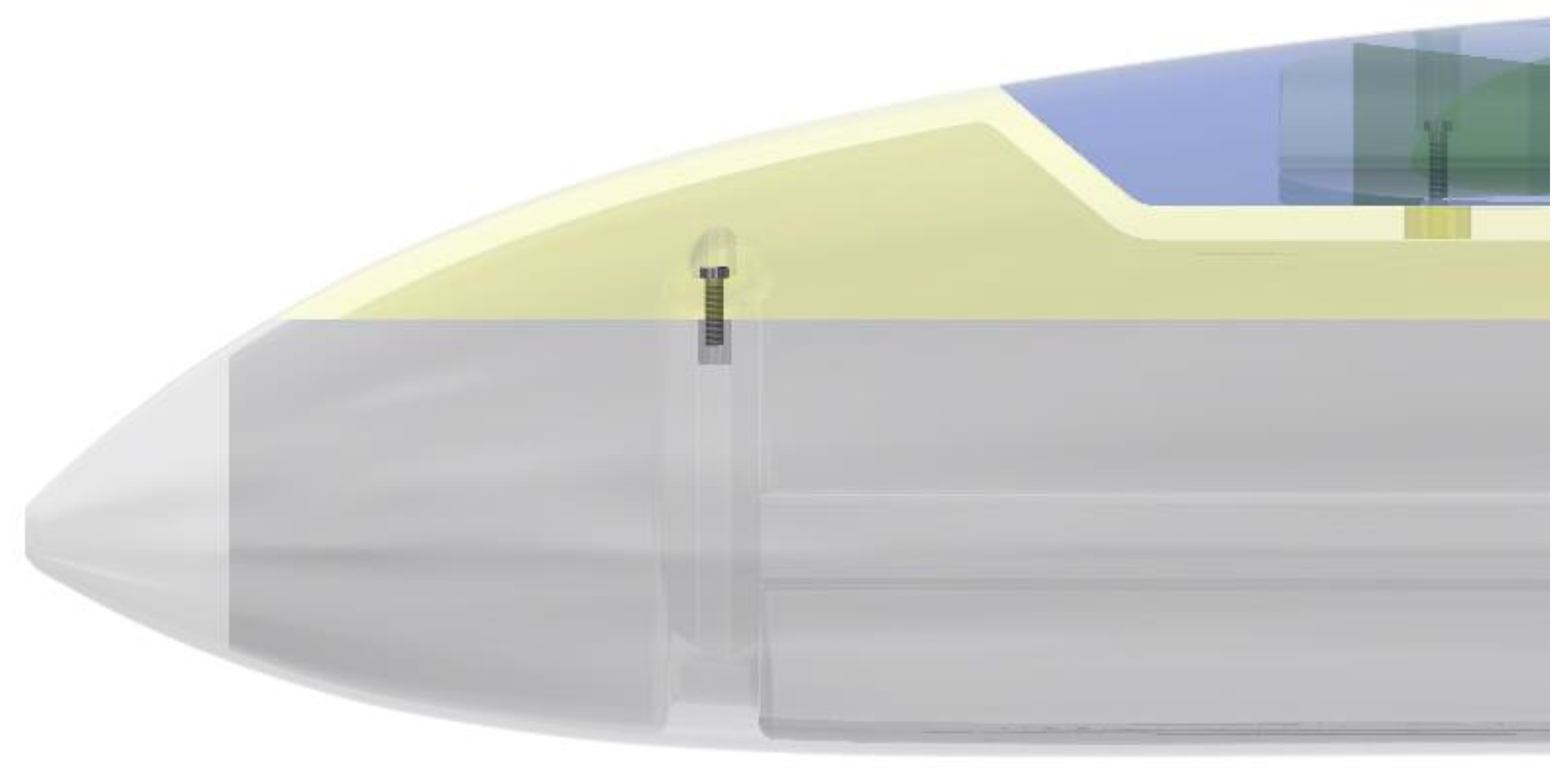
The bolt holders are designed to be fabricated with solid carbon fiber (Figure 17). Their presence protects the main cap, the wings, and the cargo holder from excessive stresses arising from the bolt forces (tension, shear-out, etc.) and pre-tensioning.

Figure 18 depicts the modelling methodology used for the connection of two parts, e.g., the main cap with the cargo holder by the presence of the bolts. All the nodes of the holes in the purple and grey regions, respectively, are connected via two distinct rigid connections; the latter are termed RBE2 with a yellow and light blue font colors, respectively (Figure 18).

The two rigid bodies (RBE2 elements) are connected through a C-bush 0D connector. The C-bush is in fact a spring with six degrees of freedom (DoFs), three displacements, and three rotations, used here as full rigids, thus allowing the export of all forces into the bolted elements to be used later in the calculation and assessment of all connectors.

Wings—003a

The presented structural component of the wing section (central wing section) connects the whole wing component with the fuselage. This is a key structural part, bearing significant loads in all mission phases of the UAV, and acting as a support for the wings, withstanding both the aerodynamic loads in normal flight mode and the VTOL loads. It is essentially a sandwich structure with areas entailing only composite skin, as well as parts with both skin and foam cores (

Table 9. Laminate breakdown of the 003a wing component.

Material	Thickness—h [mm]	Ply Orientation [deg]	Weight [kg]
Woven carbon	0.25	0	0.1675
Foam core	0.5–10	0	0.452
Woven carbon	0.25	0	0.1675
	0.75		0.787

). Figure 19 presents the anatomy of part 003a, with Figure 19a presenting the composite skin, and Figure 19b showing the core internal part.

Wings—003b and 003d

The main wing component is both a structural and aerodynamic component of the drone. It is a thin-walled sandwich structure, which incorporates both UD and woven composite fabrics. Specifically, it has been segregated into two separate laminates. The shear webs entail a cross-ply lamination (“0/90/Foam”) which is capable of bearing excessive shear loads and preventing the deformation of the foil shape during flight, while the top and bottom surfaces of the 003b part have been structured both with UD and woven fabrics in order to deal with greater bending loads, as shown in

Table 10. Laminate breakdown of the wing 003b and 003d components.

Shear Web Lamination			Top/Bottom Faces Lamination
Material	Thickness [mm]	Orientation [deg]	Material	Thickness [mm]	Orientation [deg]
UD	0.125	90	Woven	0.25	0
UD	0.125	0	UD	0.125	0
Foam	5	0	Foam	5	0
UD	0.125	0	UD	0.125	0
UD	0.125	90	Woven	0.25	0

. Figure 20 presents the geometry of wing 003b and d parts, along with the respective laminates of the shear webs and the top and bottom surfaces.

Wings—003c and 003e

These parts of the drone are attached to both wing components 003b and d and to the wing tip 004d part (Figure 21). They are responsible for holding the flaps while completing the airfoil shape of the wings. It is a thin carbon structure comprised of woven fabrics (

Table 11. Laminate breakdown of the wing 003c and 003e components.

Material	Thickness [mm]	Ply Orientation [deg]	Weight [kg]
Woven carbon	0.25	0	0.333
Woven carbon	0.25	0	0.333
Woven carbon	0.25	0	0.333
	0.75		1

). Its only structural requirement is to hold the flaps and flap mechanisms. On the other hand, it does not bear any significant loads, thus its small thickness is sufficient in keeping it sturdy.

Wings—003f (flaps)

Through the motion of the flaps, the operator manages to steer the UAV (Figure 22). Therefore, the flaps ought to be able to withstand the aerodynamic loads, while maintaining their shape. Therefore, their laminate is comprised of three layers of woven fabric (

Table 12. Laminate breakdown of the wing 003f component.

Material	Thickness [mm]	Ply Orientation [deg]	Weight [kg]
Woven carbon	0.25	0	0.085
Woven carbon	0.25	0	0.085
Woven carbon	0.25	0	0.085
	0.75		0.255

).

Wings tips—004

The wing tips (winglets) are responsible for the drone stabilization during normal flight operation (Figure 23). Their structure was designed as a sandwich structure (two layers filled with the core) in order to be able to maintain its structural rigidity against the aerodynamic loads. The core thickness is not mentioned in the below material as it is intended to fill the empty space between the skin lay-up (

Table 13. Laminate breakdown of the wing 004 component.

Material	Thickness [mm]	Ply Orientation [deg]	Weight [kg]
Woven carbon	0.25	0	0.1375
Woven carbon	0.25	0	0.1375
	0.5		0.275

).

5. Stress Analysis

This section outlines the structural studies of the described design. Starting with the description of the modelling methodology, the stress analysis of the UAV is conducted and explained with all results and failure indices for each structural component. Furthermore, all analytical studies helping to assess the structural viability of the concept are presented, including the strength viability of the bolted connections. Also, it should be highlighted that a comparative case study is presented in the Appendix A in order to manifest the greater effectiveness of the proposed sandwich-structured design approach when compared to traditional spar–rib approaches that use stiffeners.

5.1. FE Model

In order to acquire accurate results, a fine FE mesh was created with an element size for discretization in the range of 5 to 10 mm, optimized for use with the OptiStruct solver, with an element failure (elements out of range based on structural criteria) of less than 1%. The mesh was unstructured, while it was entailing mostly triangle and pyramid elements (Figure 24).

Different element types were used for the discretization of the UAV. More specifically, in

Table 14. The respective element type used for the discretization of each component of the UAV.

Component	Element Type
Main cap—001	Skin—shell elements, Figure 13 Core—solid elements, Figure 14
Quick release cap—005	Skin—shell elements, Figure 12
Cargo holder—002	Skin—shell elements, Figure 15 Core—solid elements, Figure 16
Inserts—006	Solid elements—Figure 17
Wings—003a	Skin—shell elements, Figure 19a Core—solid elements, Figure 19b
Wings—003b and d	Shell elements, Figure 20
Wings—003c and e	Shell elements, Figure 21
Wings—003f (flaps)	Shell elements, Figure 22
Wing tips—004	Skin—shell elements, Figure 23 Core—solid elements, Figure 23

, the element types used for the discretization of each component are listed. In most parts, both shell and solid elements were used. For the skin parts, CTRIA3 shell elements for the triangles and CQUAD4 for the rectangles were used, while for the solid regions tetrahedrons, pyramids and pentas (https://altairuniversity.com/wp-content/uploads/2014/02/meshing.pdf (accessed on 6 March 2024)) with four, five, and six nodes, respectively, were used (Figure 25).

Furthermore, great consideration was given to the lamination transition areas. Also, the artificial stress concentration had to be eliminated in regions where rigid connections were placed. The complexity of the problem, ranging from the anisotropy and inhomogeneity of the materials and the consideration of joints among the parts to the analysis itself, renders the mesh quality and FE discretization quite challenging. Taking into consideration all the above, a linear static analysis was performed for the estimation of critical stresses along the UAV shell. The composite failure criterion was set to be the maximum strain (

Table 15. Mathematical expression of the maximum strain criterion and failure strains of the involved materials.

$\begin{array}{l}{\epsilon }_1\ge {\epsilon }_{Lt} or \left|{\epsilon }_1\right|\ge {\epsilon }_{Lc}\\ {\epsilon }_2\ge {\epsilon }_{Tt} or \left|{\epsilon }_2\right|\ge {\epsilon }_{Tc}\\ {\epsilon }_3\ge {\epsilon }_{Tt} or \left|{\epsilon }_3\right|\ge {\epsilon }_{Tc}\\ \left|{\epsilon }_{12}\right|\ge {\epsilon }_L\end{array}$		Material
	Failure strains	UD carbon	Woven carbon fiber fabric	Foam core
	${\epsilon }_{Lt}$	0.015	0.014	0.013
	${\epsilon }_{Lc}$	0.055	0.003	0.016
	${\epsilon }_{Tt}$	0.003	0.0023	0.013
	${\epsilon }_{Tc}$	0.015	0.015	0.016
	${\epsilon }_L$	0.012	0.025	0.024

), as this takes into account that the composite fails when the strain exceeds the respective allowance, making it a simple and direct way to predict the failure of composites [34].

In Table 15, ${\epsilon }_{Lt}$ and ${\epsilon }_{Lc}$ are the tensile and compressive failure strains along the fiber direction, ${\epsilon }_{Tt}$ and ${\epsilon }_{Tc}$ are the tensile and compressive failure strains vertically to the fiber direction, and ${\epsilon }_L$ is the shear failure strain [34].

5.2. Static Analysis

A static analysis was performed to determine the maximum stresses on the UAV components. The following load cases were used:

• Its own total weight (25 kg) during levitation
• The discretized pressure calculated in Section 2
• Acceleration loads of 3 g in all directions.

No boundary conditions were used, as the drone’s most critical stress state takes place during levitation. Therefore, in order to eliminate any singularity effects resulting from the lack of boundary conditions, the static analysis was solved using the inertia relief computational technique. Figure 26 depicts the predicted displacement field. It is observed that the maximum displacement occurs at the tip of the wing tip 004 component. Vertical displacements of 7mm were observed. As will be further presented, no critical amount of stresses are developed in the structural components of the UAV. The aforementioned statement occurs taking into consideration the displacement prediction. The predicted displacement of 7 mm considering a 1500 mm wingspan is within the allowed limits, as it is permitted for the deflection to be 5% of the semi-wing span [34], which, in our case, is 0.05%.

5.3. Failure Index

The composite failure indices for most critical parts are presented hereafter. A safety factor (SF) of 2 was selected and therefore failure indices above 0.5 should be eliminated. A general rule for the safety index estimation is to derive the internal resulting stresses during loading from the materials strength. For our case though, a strain-based failure criterion was used, as already mentioned. In Figure 27 the failure index of the UAV is again presented, with the threshold of the results lowered to the maximum value for better visualization. The target is to demonstrate the regions of the structure that develop the higher stresses. Those are the regions inside the red circles. Regions (1) and (2) are located around the bolted connections and therefore transmit an amount of the developed force to the structure. Region (3) is on the mid-bottom of the wing, an area of transition from a thick to a thinner cross section, therefore some stress concentration is justified there. Region (4) is another minor stress concentration region around connections, However, significantly lower than the structural limits.

5.4. Maximum Stresses and Safety Factor

Figure 28 and Figure 29 depict the maximum stresses which occurred during the loading of the aircraft. For the skin, the maximum stresses reached up to 50 MPa, while for the foam, this was less than <1 MPa. This provides a uniform stress distribution on the structure, as the strain variation is almost 50 MPa, far from causing any structural damage to the UAV. In addition, the safety factor of each material is presented. For the skin (carbon laminate), the SF is over 5, while for the foam, this is over 4, indicating that the strength of each material is five and four times higher than the developed stresses within each material, respectively.

5.5. Bolted Connections

Within the design of the UAV, we tried to keep the number of bolts (Figure 30 and Figure 31) as low as possible in order to avoid stress concentrations, but also to limit the design complexity and reduce the assembly/disassembly time. Figure 31 depicts all the bolts along with their connections to the UAV parts.

Numerical bolt calculations were conducted to check the viability of all bolted connections together with their affecting parameters, such as bolt types, grades, pretension, etc.

Table 16. Bolt numbering and location.

	Shear [N]	Tension [N]	Fmax [N]	SF
Bolt 1	1.98	52.7	10,400	>>2
Bolt 2	76.93	2.9	10,400	>>2
Bolt 3	153.7	18.8	10,400	>>2
Bolt 4	2.5	9.1	10,400	>>2

lists the loads on each bolt (as retrieved from the analysis conducted) together with the resultants and the safety factors computed analytically for each connection. It is shown that the maximum shear load reported within the bolts is approximately 154 N, while the maximum load in tension is 53 N. The aforementioned values are far from the bolts’ limits, which are 10,400 N. (Table 16, Figure 32).

6. Discussion

Due to the multi-disciplinary character of this work, a lot of issues can be proposed and remarked. When it comes to dynamic loading like gusts or shock loads, it should be highlighted that the structural design was performed considering 3 g acceleration in all three directions, namely, a 750 N load in every direction, and 90 kPa pressure on top of the entire aircraft, for a velocity magnitude of 33 m/s. Considering that the missions of the UAV will take place in up to 10 m/s wind and an acceptable gust will potentially rise up to 20 m/s, the structural integrity of the UAV will withhold this. Also, regarding the manufacturing of the UAV and the subsequent environmental impact, it should be emphasized that the present design is quite flexible in terms of fabrication processes. Even if the study was performed with autoclave-based thermosets, the fabrication process would be performed either by resin infusion or out-of-autoclave prepregs. The first method would be easily utilized in bio-based resins, while, by employing the second method, the environmental impact is drastically reduced due to the absence of an autoclave furnace. In addition, concerning the usage of 3D-printed thermoplastics, in this UAV, the winglets, the flaps, and the quick release cap would be easily replaced by 3D-printed materials. The remaining parts are considered structural parts, and, without a reinforcement, they may not withstand the calculated forces, especially after many missions. Considering the scalability of the present UAV, by employing a liquid resin infusion, the scale-up process would be applicable (i.e., fabrication of large wind turbine blades). In terms of structural analysis, the scalability would pose several challenges, especially in the joint parts. However, taking into account the attained weight reduction achieved by the proposed methodology, critical points would be easily reinforced through increasing the composite’s thickness.

7. Conclusions

The present work gives an insight into the design process of a type C composite UAV, demonstrating all studies conducted to assess its viability. To reach a final design concept that covers all requirements, several complementing steps were performed. Initially, aerodynamic studies were carried out to determine and understand the loads subjected onto the UAV in different missions/scenarios. A comprehensive CFD analysis was executed, the findings of which were validated and enhanced with an analytical solution aiming to eliminate the discrepancies of pressure distributions along the aircraft between the two solutions. Consequently, topology optimization analysis was performed both on the body and the wings in order to determine the optimum material paths and establish a basis for the conceptual preliminary structural design. Composite optimization studies were also conducted, aiming to determine the minimum and maximum laminate thicknesses throughout the UAV, following both mass and ply thickness constraint rules. All these studies were needed to perform a structural design of the full drone assembly, which was then used for thorough structural examination via static analysis. The low stresses developed both in the composite fabrics and the foam core highlight the feasibility of the design, suggesting a successful design approach that will lead to the final detailed design of the drone.

Conclusively, the proposed road map for the optimization-based UAV design consists of the following steps:

• Aerodynamic analysis for the estimation of loads.
• Topology optimization to determine the material paths of the initial design.
• Free-size optimization to obtain the first estimation of the thicknesses of each material configuration.
• Size/Composite optimization to fully determine the thicknesses and lay-ups of each material region.
• Static and stress analysis to ensure the safe and successful UAV design.

The aforementioned design procedure is easily applicable to any type, size, and configuration of UAV since the whole design process is optimization based. Furthermore, the manufacturing constraints applied during the optimization procedures enhance the manufacturability of the proposed UAV. For future work, the incorporation of composite spring leaf landing gear and the utilization of different materials, such as high-performance 3D-printed thermoplastics, could be highly interesting focuses of future studies. Another compelling research direction could be towards the manufacturing processes and possible challenges of the proposed UAV.