Characterization of a Solar Sail Membrane for Abaqus-Based Simulations
Abstract
1. Introduction
2. Materials and Methods
2.1. Dimensions and Constituent Materials
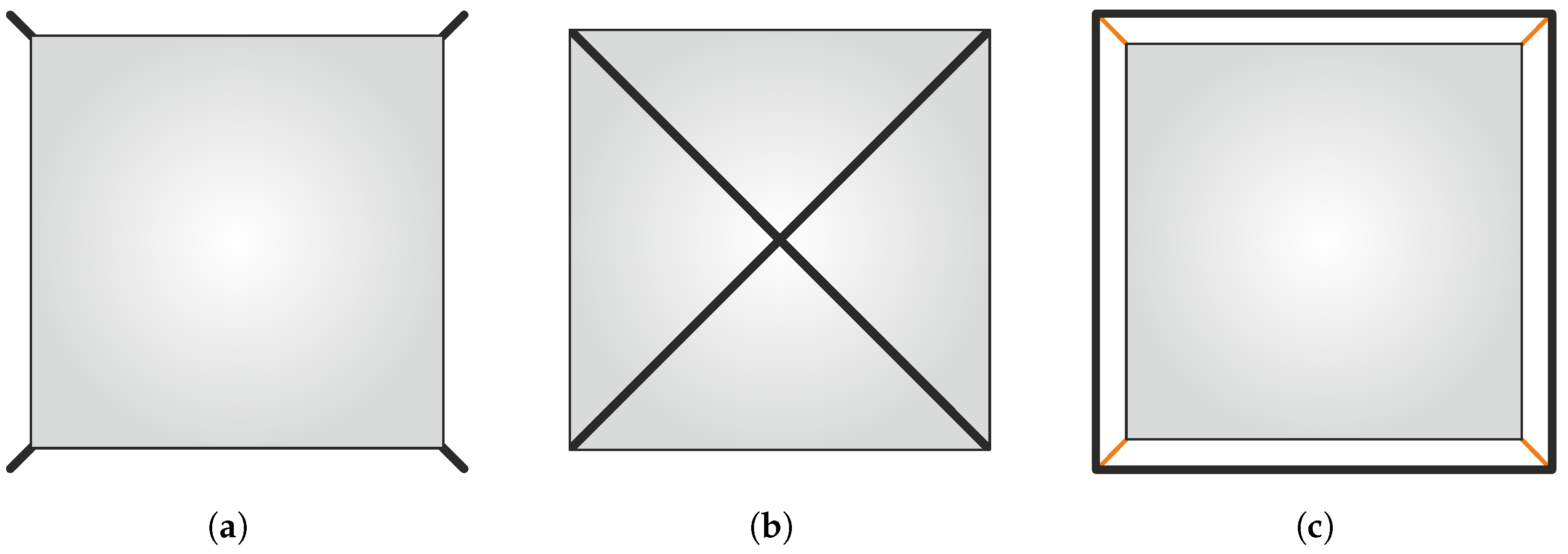
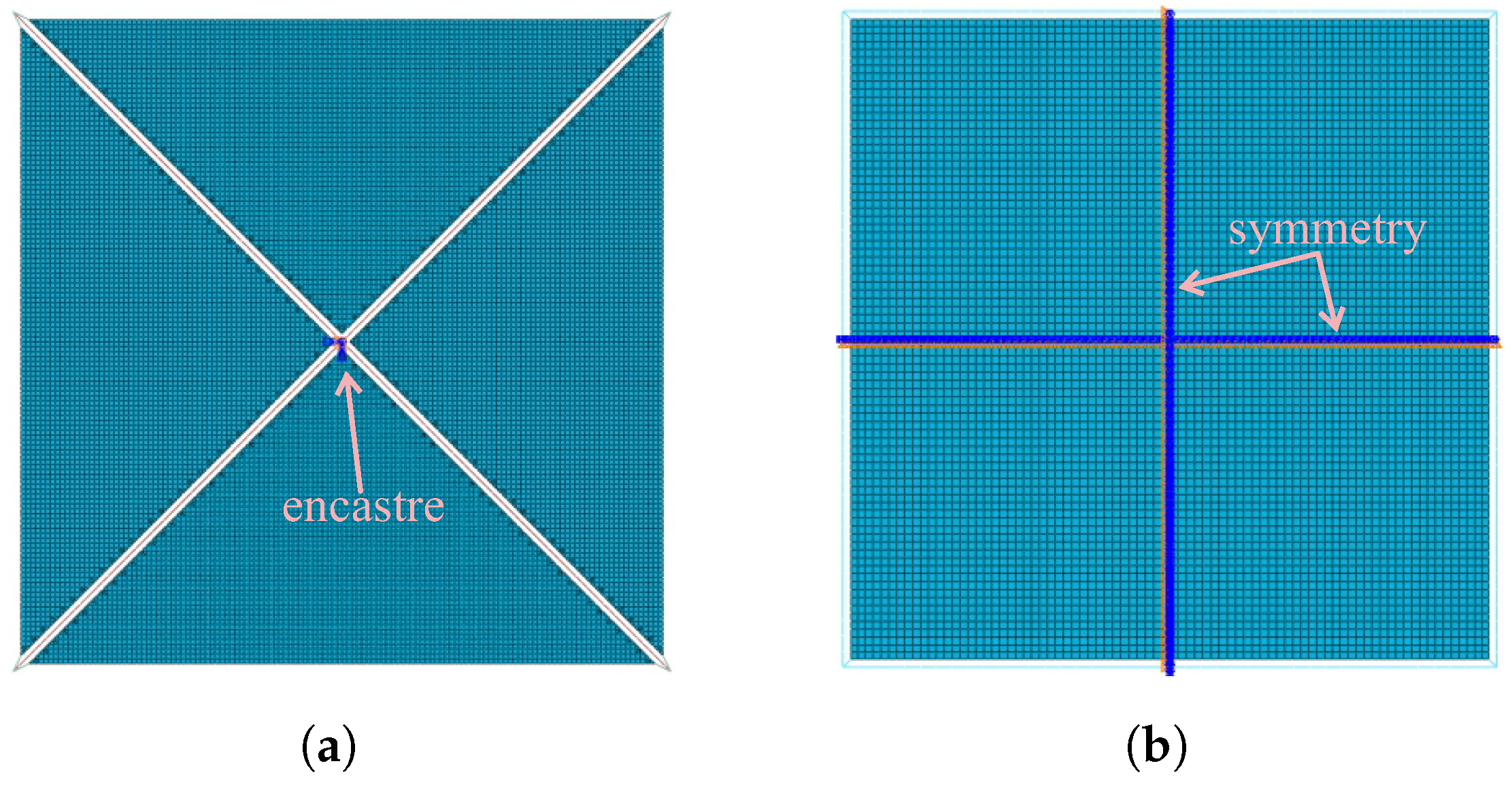
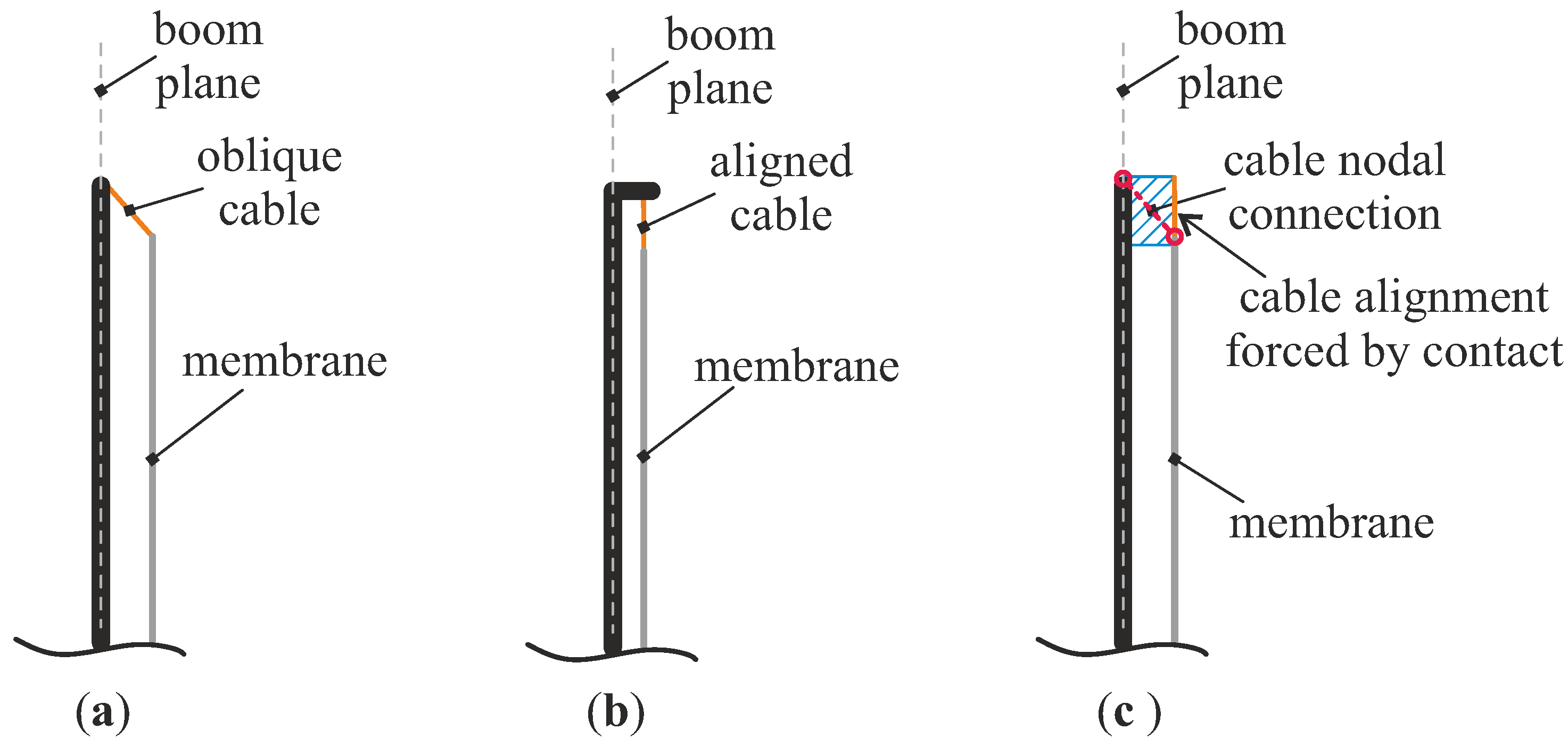
2.2. Finite Element Modeling Techniques
2.3. Pre-Tensioning Application
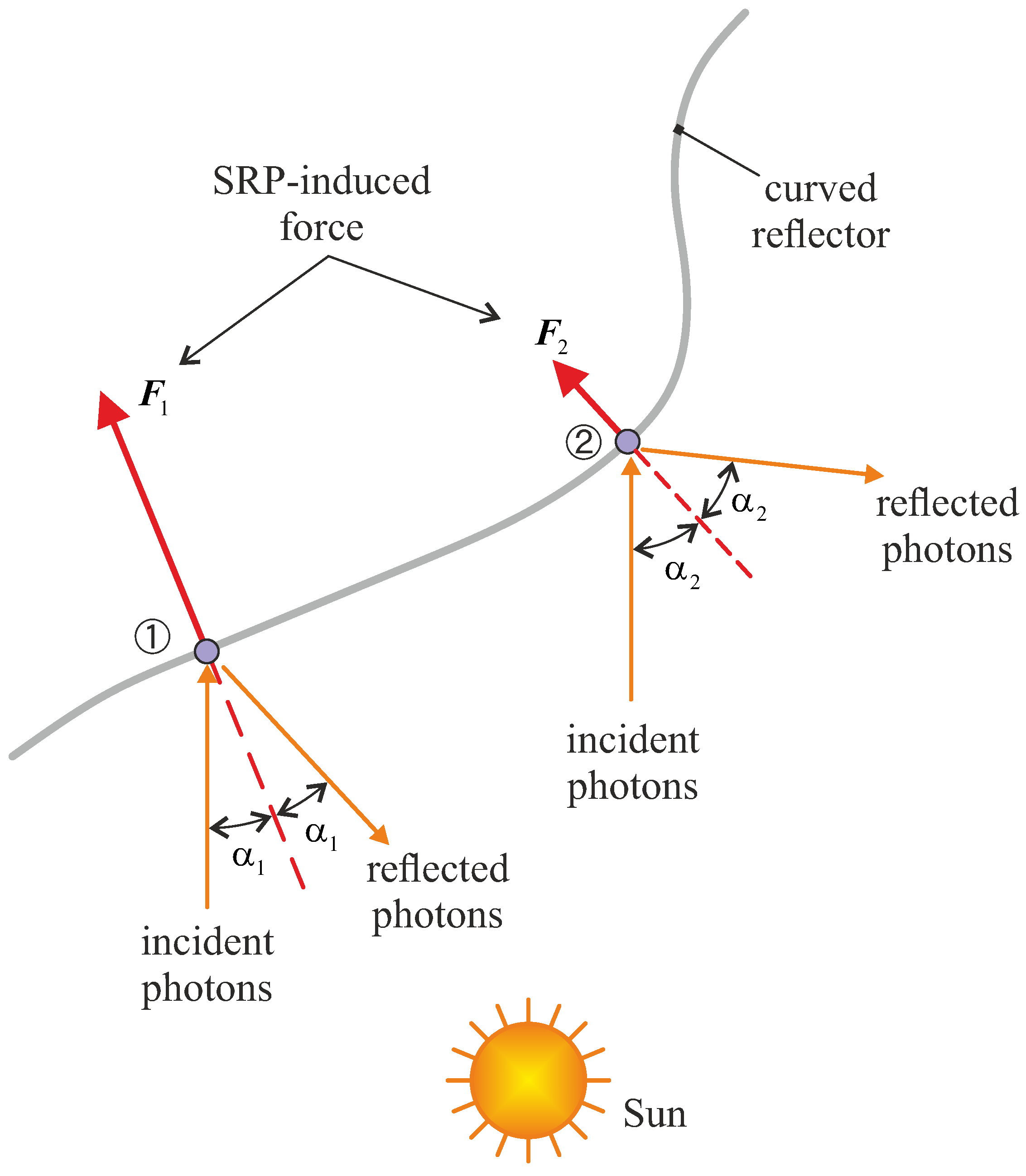
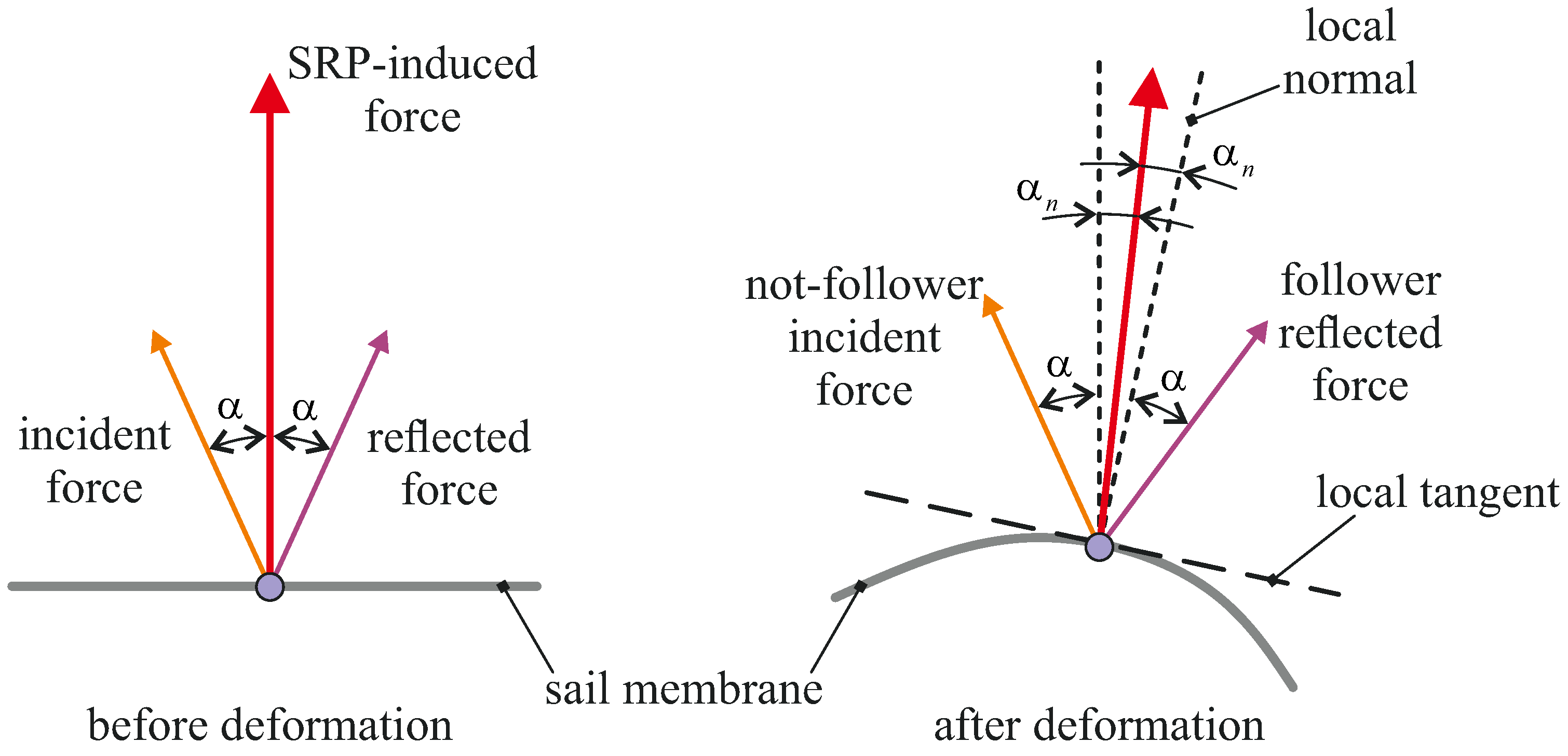
2.4. Solar Radiation Pressure Application
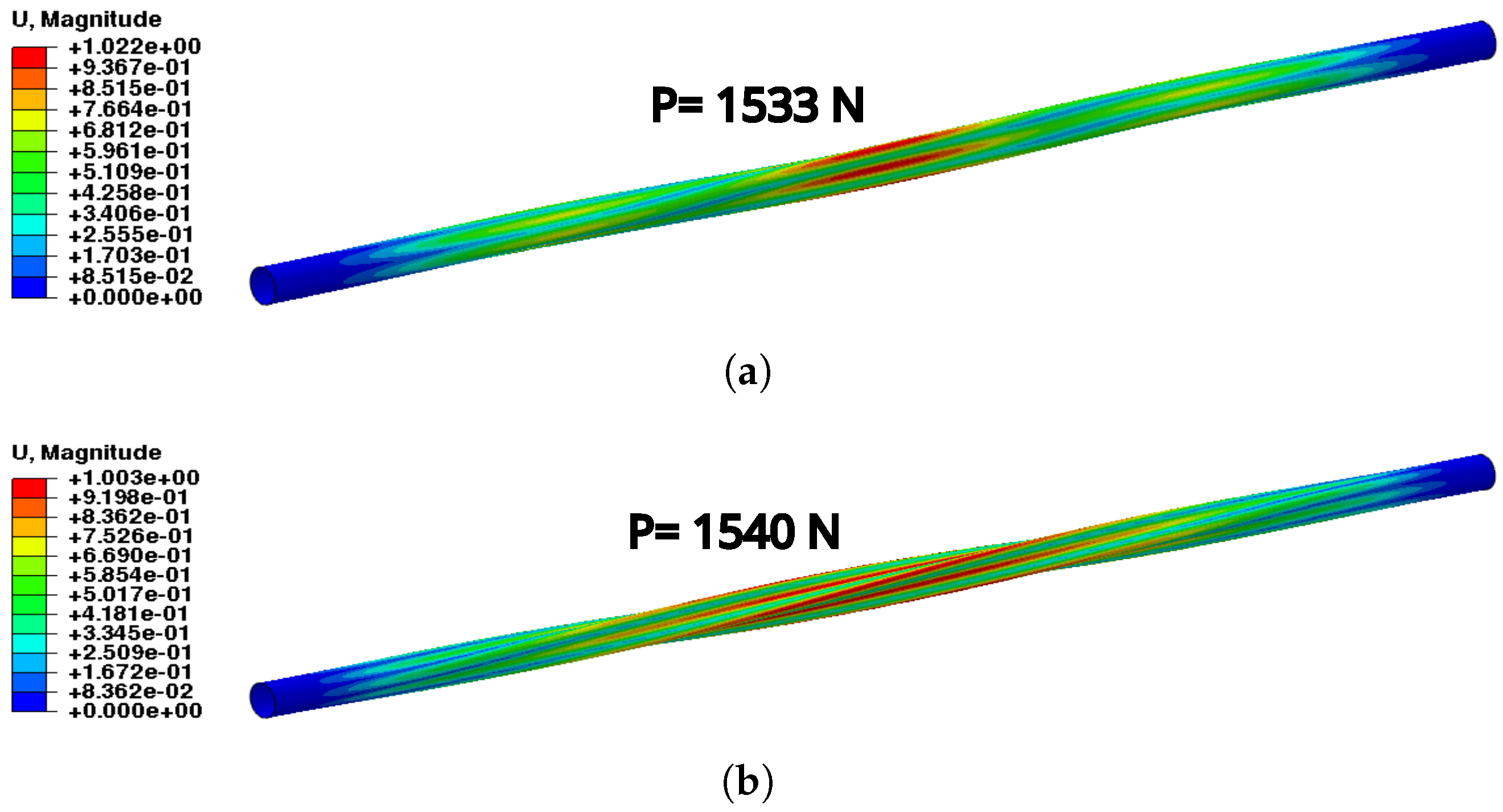
2.5. Boom Buckling Problem
3. Numerical Simulation Results
3.1. Pre-Tensioning
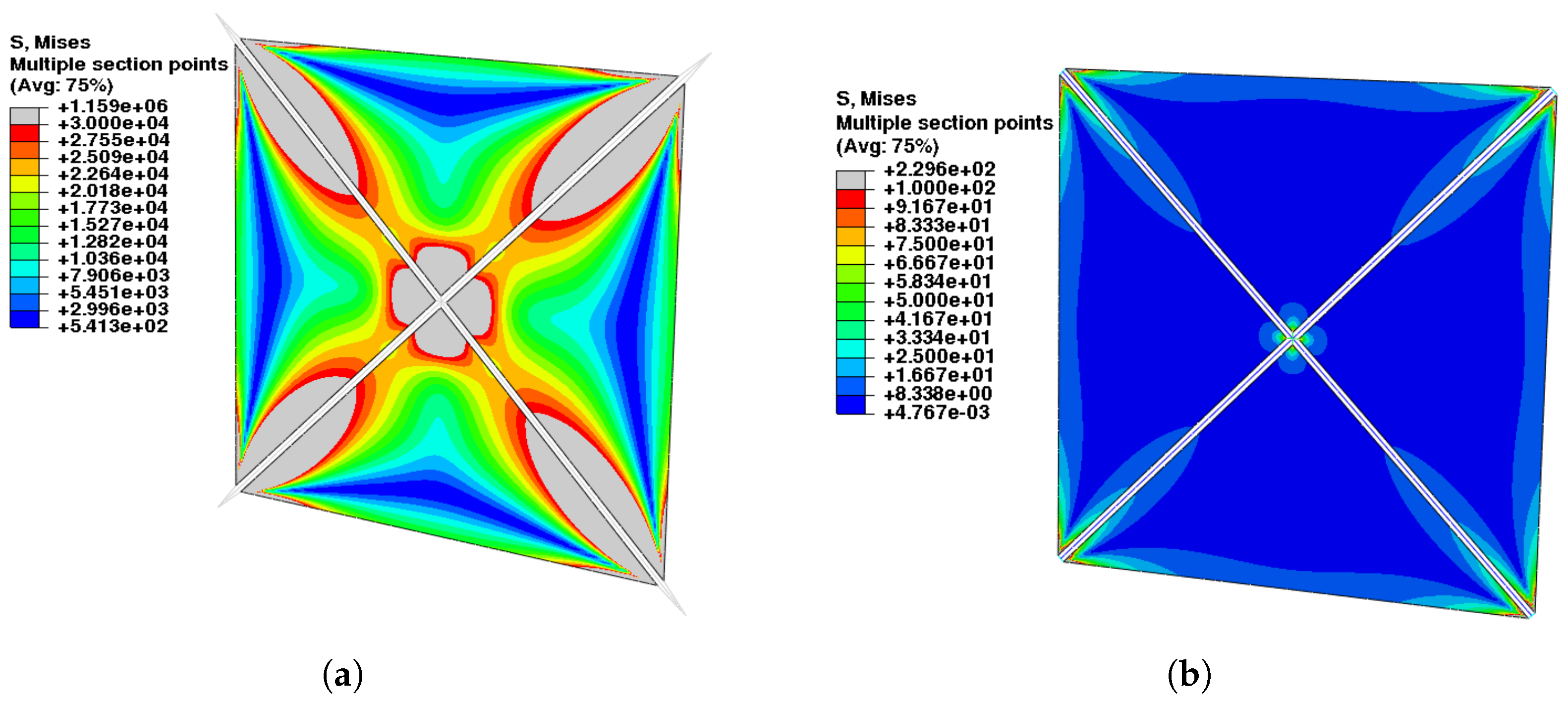
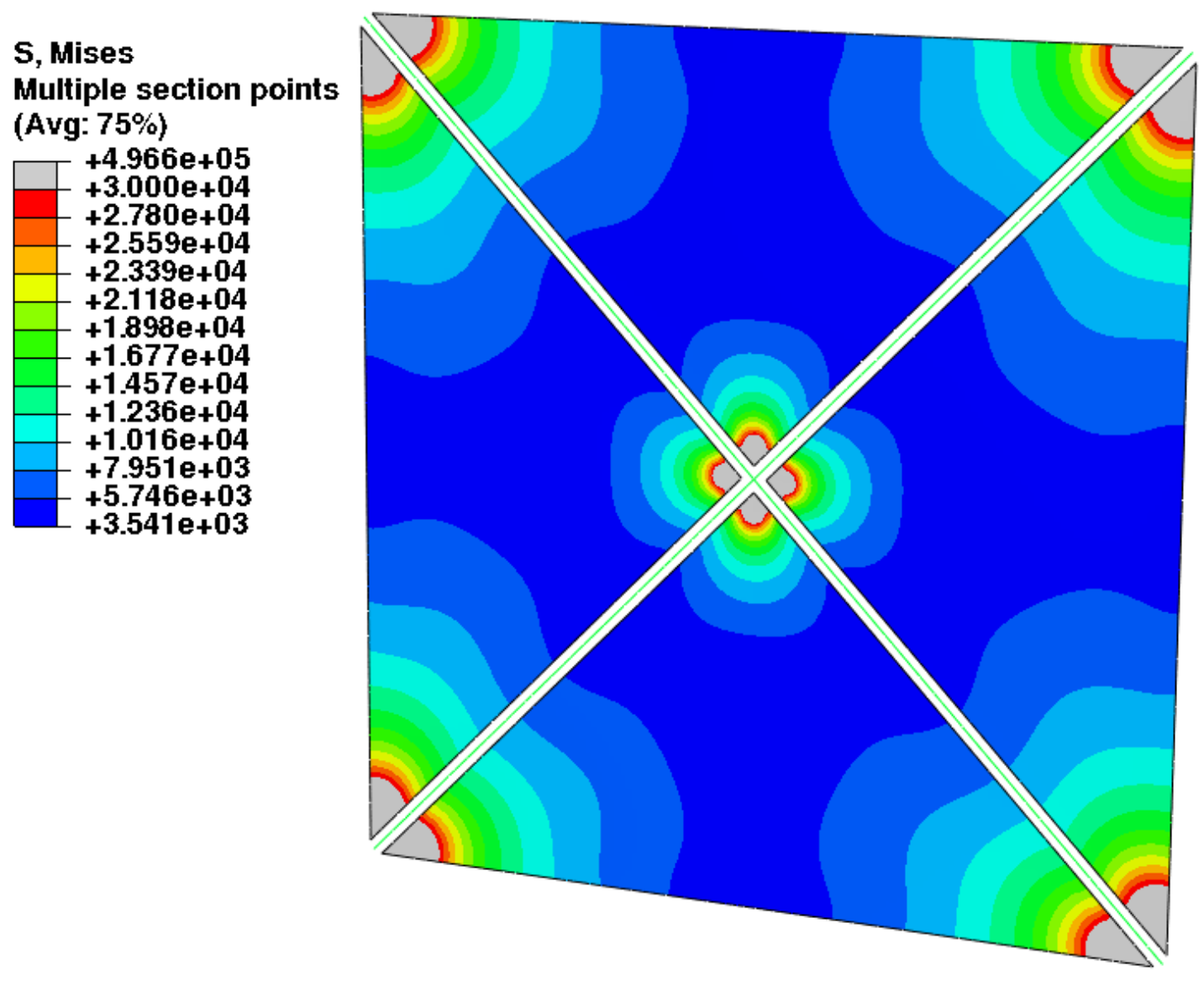
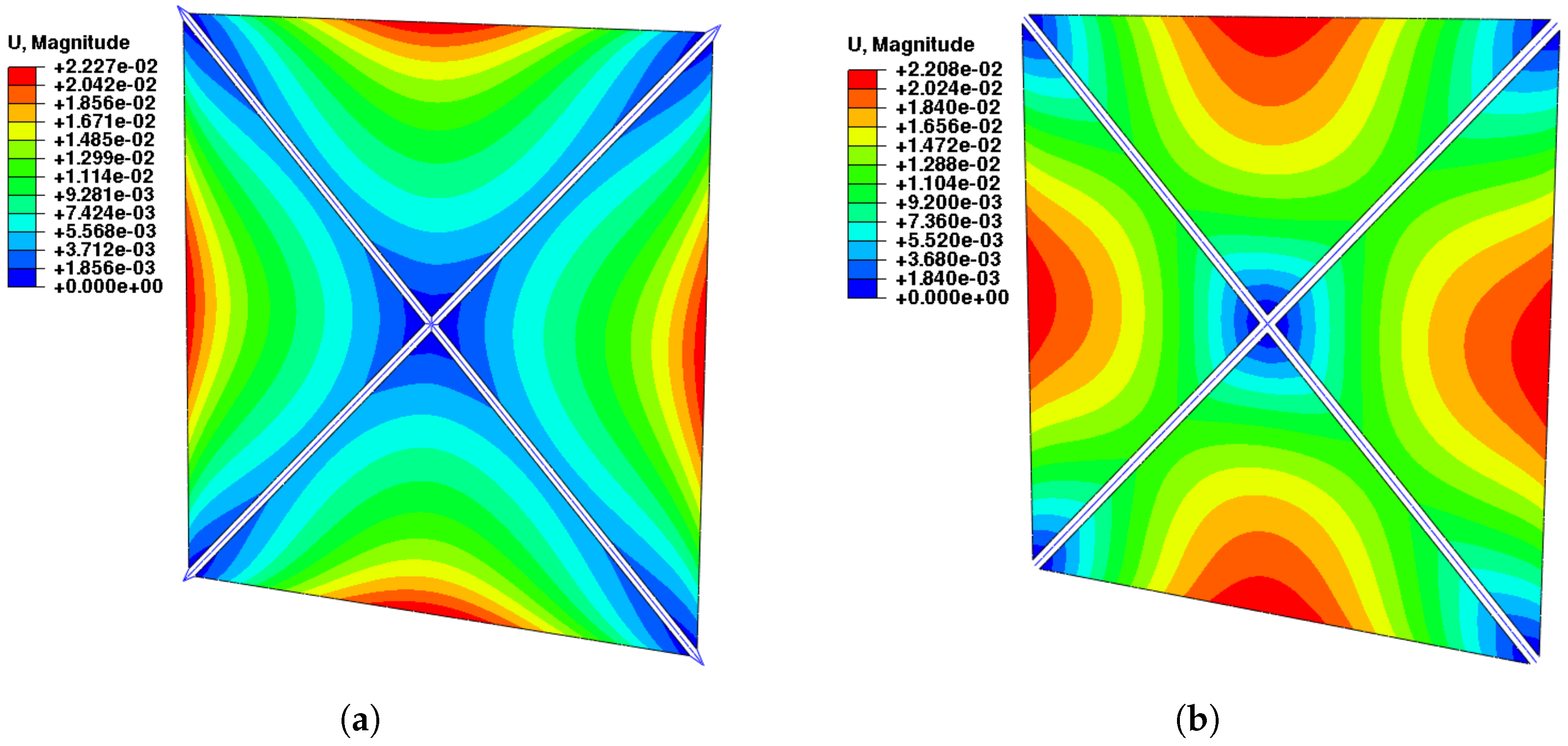
3.2. Solar Radiation Pressure
3.3. Boom Buckling
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gong, S.; Macdonald, M. Review on solar sail technology. Astrodynamics 2019, 3, 93–125. [Google Scholar] [CrossRef]
- Fu, B.; Sperber, E.; Eke, F. Solar sail technology—A state of the art review. Prog. Aerosp. Sci. 2016, 86, 1–19. [Google Scholar] [CrossRef]
- Johnson, L.; Young, R.; Barnes, N.; Friedman, L.; Lappas, V.; McInnes, C.R. Solar Sails: Technology Furthermore, Demonstration Status. Int. J. Aeronaut. Space Sci. 2012, 13, 421–427. [Google Scholar] [CrossRef][Green Version]
- Zhao, P.; Wu, C.; Li, Y. Design and application of solar sailing: A review on key technologies. Chin. J. Aeronaut. 2023, 36, 125–144. [Google Scholar] [CrossRef]
- Mori, O.; Shirasawa, Y.; Mimasu, Y.; Tsuda, Y.; Sawada, H.; Saiki, T.; Yamamoto, T.; Yonekura, K.; Hoshino, H.; Kawaguchi, J.; et al. Overview of IKAROS Mission. In Advances in Solar Sailing; Macdonald, M., Ed.; Springer: Berlin/Heidelberg, Germany, 2014; Chapter 3; pp. 25–43. [Google Scholar] [CrossRef]
- Umesato, M.; Okahashi, T. Development of small solar power sail demonstrator IKAROS. NEC Tech. J. 2011, 6, 52–56. [Google Scholar]
- Tanaka, Y.; Shibuya, K.; Negishi, M.; Fujino, H.; Mori, T. The great challenge of “IKAROS”: A space yacht flying with a solar sail. J. Inst. Electr. Eng. Jpn. 2011, 131, 336–340. [Google Scholar] [CrossRef]
- Tsuda, Y.; Mori, O.; Funase, R.; Sawada, H.; Yamamoto, T.; Saiki, T.; Endo, T.; Kawaguchi, J. Flight status of IKAROS deep space solar sail demonstrator. Acta Astronaut. 2011, 69, 833–840. [Google Scholar] [CrossRef]
- Matsushita, M.; Okuizumi, N.; Mori, O.; Satou, Y.; Iwasa, T.; Matunaga, S. Influence of curved thin-film device on deformation of a solar sail. Adv. Space Res. 2021, 67, 2628–2642. [Google Scholar] [CrossRef]
- Okuizumi, N.; Satou, Y.; Mori, O.; Sakamoto, H.; Furuya, H. Analytical investigation of global deployed shape of a spinning solar sail membrane. AIAA J. 2021, 59, 1075–1086. [Google Scholar] [CrossRef]
- Boden, R.C.; Hernando-Ayuso, J. Shape estimation of gossamer structures using distributed sun-angle measurements. J. Spacecr. Rocket. 2018, 55, 415–426. [Google Scholar] [CrossRef]
- Zhang, F.; Gong, S.; Baoyin, H. Three-Axes Attitude Control of Solar Sail Based on Shape Variation of Booms. Aerospace 2021, 8, 198. [Google Scholar] [CrossRef]
- Matsushita, M.; Okuizumi, N.; Satou, Y.; Mori, O.; Iwasa, T.; Matunaga, S. Influence of thin-film device with curvature on natural frequency of rectangle membrane under uniaxial tension. Astrodynamics 2019, 3, 257–272. [Google Scholar] [CrossRef]
- Sproewitz, T.; Grundmann, J.T.; Seefeldt, P.; Spietz, P.; Hillebrandt, M.; Jahnke, R.; Mikulz, E.; Renger, T.; Reershemius, S.; Sasaki, K.; et al. Membrane Deployment Technology Development at DLR for Solar Sails and Large-Scale Photovoltaics. In Proceedings of the IEEE Aerospace Conference, Big Sky, MT, USA, 2–9 March 2019. [Google Scholar] [CrossRef]
- Zhang, F.; Gong, S.; Gong, H.; Baoyin, H. Solar sail attitude control using shape variation of booms. Chin. J. Aeronaut. 2022, 35, 326–336. [Google Scholar] [CrossRef]
- Chen, T.Z.; Liu, X.; Cai, G.P.; You, C.L. Attitude and vibration control of a solar sail. Adv. Space Res. 2023, 71, 4557–4567. [Google Scholar] [CrossRef]
- Boni, L.; Bassetto, M.; Niccolai, L.; Mengali, G.; Quarta, A.A.; Circi, C.; Pellegrini, R.C.; Cavallini, E. Structural response of Helianthus solar sail during attitude maneuvers. Aerosp. Sci. Technol. 2023, 133, 1–9. [Google Scholar] [CrossRef]
- McInnes, C.R. Solar Sailing: Technology, Dynamics and Mission Applications; Springer-Praxis Series in Space Science and Technology; Springer: Berlin/Heidelberg, Germany, 1999; Chapter 2; pp. 38–40. [Google Scholar] [CrossRef]
- Boni, L.; Mengali, G.; Quarta, A.A. Finite Element Analysis of Solar Sail Force Model with Mission Application. Proc. Inst. Mech. Eng. Part G: J. Aerosp. Eng. 2019, 233, 1838–1846. [Google Scholar] [CrossRef]
- Seefeldt, P.; Grundmann, J.T.; Hillebrandt, M.; Zander, M. Performance analysis and mission applications of a new solar sail concept based on crossed booms with tip-deployed membranes. Adv. Space Res. 2021, 67, 2736–2745. [Google Scholar] [CrossRef]
- Bassetto, M.; Niccolai, L.; Boni, L.; Mengali, G.; Quarta, A.A.; Circi, C.; Pizzurro, S.; Pizzarelli, M.; Pellegrini, R.C.; Cavallini, E. Sliding mode control for attitude maneuvers of Helianthus solar sail. Acta Astronaut. 2022, 198, 100–110. [Google Scholar] [CrossRef]
- Landini, F.; Frassetto, F.; Zangrilli, L.; Caracci, V.; Riva, A.; Loreggia, D.; Redaelli, E.; Riva, M.; Pellegrini, R.; Cavallini, E.; et al. SailCor: Compact coronagraph for the Helianthus sub-L1 Mission with solar photonic propulsion. In Proceedings of the International Conference on Space Optics—ICSO 2022, Dubrovnik, Croatia, 3–7 October 2023; Minoglou, K., Karafolas, N., Cugny, B., Eds.; SPIE: Bellingham, WA, USA, 2022. [Google Scholar] [CrossRef]
- Aliasi, G.; Mengali, G.; Quarta, A.A. Artificial Lagrange points for solar sail with electrochromic material panels. J. Guid. Control. Dyn. 2013, 36, 1544–1550. [Google Scholar] [CrossRef]
- Mu, J.; Gong, S.; Ma, P.; Li, J. Dynamics and control of flexible spinning solar sails under reflectivity modulation. Adv. Space Res. 2015, 56, 1737–1751. [Google Scholar] [CrossRef]
- Anonymous. Abaqus Scripting User’s Guide; Dassault Systèmes: Paris, France, 2022. [Google Scholar]
- Anonymous. Abaqus User Subroutines Reference Guide; Dassault Systèmes: Paris, France, 2022. [Google Scholar]
- Anonymous. Abaqus Theory Manual; Dassault Systèmes: Paris, France, 2022. [Google Scholar]
- Satou, Y.; Okuizumi, N.; Sakamoto, H.; Furuya, H.; Ono, G.; Shirasawa, Y.; Mori, O. Nonflatness of Solar Sail Membrane Predicted by Nonlinear Finite Element Analyses. In Proceedings of the Spacecraft Structures Conference, National Harbor, MA, USA, 13–17 January 2014. [Google Scholar] [CrossRef]
- Satou, Y.; Mori, O.; Okuizumi, N.; Shirasawa, Y.; Furuya, H.; Sakamoto, H. Deformation Properties of Solar Sail IKAROS Membrane with Nonlinear Finite Element Analyses. In Proceedings of the 2nd AIAA Spacecraft Structures Conference, Kissimmee, FL, USA, 5–9 January 2015. Paper AIAA 2015-0436. [Google Scholar] [CrossRef]
- Wright, J.L. Space Sailing; Gordon and Breach Science: Langhorne, PA, USA, 1992; pp. 223–226. [Google Scholar]
- Sleight, D.; Muheim, D. Parametric studies of square solar sails using finite element analysis. In Proceedings of the 45th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics & Materials Conference, Palm Springs, CA, USA, 19–22 April 2004. [Google Scholar] [CrossRef]
- Boni, L.; Mengali, G.; Quarta, A.A. Solar sail structural analysis via improved finite element modeling. Proc. Inst. Mech. Eng. Part G: J. Aerosp. Eng. 2017, 231, 306–318. [Google Scholar] [CrossRef]
- Biddy, C.; Svitek, T. LightSail-1 solar sail design and qualification. In Proceedings of the 41st Aerospace Mechanisms Symposium, Pasadena, CA, USA, 16–18 May 2012. [Google Scholar]
- Banik, J.; Murphey, T. Performance validation of the triangular rollable and collapsible mast. In Proceedings of the 24th Annual AIAA/USU Conference on Small Satellites, Logan, UT, USA, 9–12 August 2010. [Google Scholar]
- Sickinger, C.; Herbeck, L.; Breitbach, E. Structural engineering on deployable CFRP booms for a solar propelled sailcraft. Acta Astronaut. 2006, 58, 185–196. [Google Scholar] [CrossRef]
- Almeida, J.H.S.; Bittrich, L.; Jansen, E.; Tita, V.; Spickenheuer, A. Buckling optimization of composite cylinders for axial compression: A design methodology considering a variable-axial fiber layout. Compos. Struct. 2019, 222, 110928. [Google Scholar] [CrossRef]









| Components | Material | Modulus (GPa) | Poisson’s Ratio | Density (kg/m3) |
|---|---|---|---|---|
| Sail | Aluminized Kapton | 1572 | ||
| Boom | Composite | 124 | 1600 | |
| Cable | Kevlar | 62 | 1440 |
| (GPa) | (GPa) | (GPa) | (GPa) | (GPa) | ||
|---|---|---|---|---|---|---|
| 124 | 51 | 22 | 57 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Boni, L.; Bassetto, M.; Quarta, A.A. Characterization of a Solar Sail Membrane for Abaqus-Based Simulations. Aerospace 2024, 11, 151. https://doi.org/10.3390/aerospace11020151
Boni L, Bassetto M, Quarta AA. Characterization of a Solar Sail Membrane for Abaqus-Based Simulations. Aerospace. 2024; 11(2):151. https://doi.org/10.3390/aerospace11020151
Chicago/Turabian StyleBoni, Luisa, Marco Bassetto, and Alessandro A. Quarta. 2024. "Characterization of a Solar Sail Membrane for Abaqus-Based Simulations" Aerospace 11, no. 2: 151. https://doi.org/10.3390/aerospace11020151
APA StyleBoni, L., Bassetto, M., & Quarta, A. A. (2024). Characterization of a Solar Sail Membrane for Abaqus-Based Simulations. Aerospace, 11(2), 151. https://doi.org/10.3390/aerospace11020151






