Mitigation of Shock-Induced Separation Using Square-Shaped Micro-Serrations—A Preliminary Study
Abstract
1. Introduction
2. Methodology
2.1. Description of the Computational Domain and Boundary Conditions
2.2. Numerical Method
2.3. Code Validation
3. Results and Discussion
3.1. Vorticity-Based Criterion for Separation Assessment
3.2. Effects of a Single Stair on Shock-Induced Separation
3.3. Effects of Serration Size on Shock-Induced Separation
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Huang, W.; Du, Z.B.; Yan, L.; Moradi, R. Flame propagation and stabilization in dual-mode scramjet combustors: A survey. Prog. Aerosp. Sci. 2018, 101, 13–30. [Google Scholar] [CrossRef]
- Pasquariello, V.; Grilli, M.; Hickel, S.; Adams, N.A. Large-eddy simulation of passive shock-wave/boundary-layer interaction control. Int. J. Heat Fluid Flow 2014, 49, 116–127. [Google Scholar] [CrossRef]
- Zhang, Y.; Tan, H.J.; Wang, Z.Y.; Li, X.; Guo, Y.J. Progress of shockwave/boundary layer interaction and its control in inlet. J. Propuls. Technol. 2020, 41, 241–259. (In Chinese) [Google Scholar] [CrossRef]
- Hadjadj, A.; Dussauge, J.P. Shock wave boundary layer interaction. Shock Waves 2009, 19, 449–452. [Google Scholar] [CrossRef]
- Meier, G.; Szumowski, A.P.; Selerowicz, W.C. Self-excited oscillations in internal transonic flows. Prog. Aerosp. Sci. 1990, 27, 145–200. [Google Scholar] [CrossRef]
- Spaid, F.W.; Frishett, J.C. Incipient separation of a supersonic, turbulent boundary layer, including effects of heat transfer. AIAA J. 1972, 10, 915–922. [Google Scholar] [CrossRef]
- Hou, W.T.; Qiao, W.Y.; Luo, H.L. Numerical simulation of effects of film-cooling on interaction between shock wave and boundary layer. J. Propuls. Technol. 2009, 30, 555–560. (In Chinese) [Google Scholar] [CrossRef]
- Huang, W.; Chen, Z.; Yan, L.; Yan, B.B.; Du, Z.B. Drag and heat flux reduction mechanism induced by the spike and its combinations in supersonic flows: A review. Prog. Aerosp. Sci. 2019, 105, 31–39. [Google Scholar] [CrossRef]
- Zhang, Q.F.; Tan, H.J.; Chen, H. Experimental study of unstart oscillatory flow control of hypersonic inlet with movable slot-plate. J. Propuls. Technol. 2017, 38, 1450–1458. (In Chinese) [Google Scholar] [CrossRef]
- He, L.H.; Chen, H.; Yue, L.J.; Zhang, Q.F. Interaction of a shock train with inherent isentropic waves in a curved isolator. Phys. Fluids 2022, 34, 066106. [Google Scholar] [CrossRef]
- Ferri, A. Experimental Results with Airfoils Tested in the High-Speed Tunnel at Guidonia. 1940, NACA-TM-946. Available online: https://ntrs.nasa.gov/citations/19930094471 (accessed on 6 September 2013).
- Panaras, A.G.; Lu, F.K. Micro-vortex generators for shock wave/boundary layer interactions. Prog. Aerosp. Sci. 2015, 74, 16–47. [Google Scholar] [CrossRef]
- Giepman, R.H.M.; Schrijer, F.F.J.; van Oudheusden, B.W. Flow control of an oblique shock wave reflection with micro-ramp vortex generators: Effects of location and size. Phys. Fluids 2014, 26, 066101. [Google Scholar] [CrossRef]
- Martis, R.R.; Misra, A. Separation attenuation in swept shock wave–boundary-layer interactions using different microvortex generator geometries. Shock Waves 2017, 27, 747–760. [Google Scholar] [CrossRef]
- Rybalko, M.; Babinsky, H.; Loth, E. Vortex generators for a normal shock/boundary layer interaction with a downstream diffuser. J. Propul. Power 2012, 28, 71–82. [Google Scholar] [CrossRef]
- Colliss, S.P.; Babinsky, H.; Nübler, K.; Lutz, T. Joint experimental and numerical approach to three-dimensional shock control bump research. AIAA J. 2014, 52, 436–446. [Google Scholar] [CrossRef]
- Bruce, P.J.K.; Colliss, S.P. Review of research into shock control bumps. Shock Waves 2015, 25, 451–471. [Google Scholar] [CrossRef]
- Li, W.P.; Liu, H. Large-eddy simulation of shock-wave/boundary-layer interaction control using a backward facing step. Aerosp. Sci. Technol. 2019, 84, 1011–1019. [Google Scholar] [CrossRef]
- Murugan, J.N.; Govardhan, R.N. Shock wave–boundary layer interaction in supersonic flow over a forward-facing step. J. Fluid Mech. 2016, 807, 258–302. [Google Scholar] [CrossRef]
- Slater, J.W. Improvements in modeling 90-degree bleed holes for supersonic inlets. J. Propul. Power 2012, 28, 773–781. [Google Scholar] [CrossRef]
- Li, J.; Tian, Y.; Zhong, F.Y.; Yang, S.H. Effects of boundary-layer bleeding on flow field in scramjet combustor. J. Propuls. Technol. 2019, 40, 2700–2707. (In Chinese) [Google Scholar] [CrossRef]
- Szwaba, R. Influence of air-jet vortex generator diameter on separation region. J. Therm. Sci. 2013, 22, 294–303. [Google Scholar] [CrossRef]
- Reese, B.M.; Collins, E.G., Jr.; Fernandez, E.; Alvi, F.S. Nonlinear adaptive approach to microjet-based flow separation control. AIAA J. 2016, 54, 3002–3014. [Google Scholar] [CrossRef][Green Version]
- Kinefuchi, K.; Starikovskiy, A.Y.; Miles, R.B. Numerical investigation of nanosecond pulsed plasma actuators for control of shock-wave/boundary-layer separation. Phys. Fluids 2018, 30, 106105. [Google Scholar] [CrossRef]
- Bisek, N.J.; Rizzetta, D.P.; Poggie, J. Plasma control of a turbulent shock boundary-layer interaction. AIAA J. 2013, 51, 1789–1804. [Google Scholar] [CrossRef]
- Yang, G.; Yao, Y.F.; Fang, J.; Gan, T.; Li, Q.S.; Lu, L.P. Large-eddy simulation of shock-wave/turbulent boundary layer interaction with and without SparkJet control. Chin. J. Aeronaut. 2016, 29, 617–629. [Google Scholar] [CrossRef]
- Herrmann, D.; Blem, S.; Gulhan, A. Experimental study of boundary-layer bleed impact on ramjet inlet performance. J. Propul. Power 2011, 27, 1186–1195. [Google Scholar] [CrossRef]
- Wang, B.; Liu, W.D.; Zhao, Y.X.; Fan, X.Q.; Wang, C. Experimental investigation of the micro-ramp based shock wave and turbulent boundary layer interaction control. Phys. Fluids 2012, 24, 055110. [Google Scholar] [CrossRef]
- Blinde, P.L.; Humble, R.A.; van Oudheusden, B.W.; Scarano, F. Effects of micro-ramps on a shock wave/turbulent boundary layer interaction. Shock Waves 2009, 19, 507–520. [Google Scholar] [CrossRef]
- Zhang, Y. Flow Control Methods Based on Shape Memory Alloy for Hypersonic Inlets. Ph.D. Thesis, Nanjing University of Aeronautics and Astronautics, Nanjing, China, 2015. (In Chinese). [Google Scholar]
- Lu, F.K.; Li, Q.; Liu, C.Q. Microvortex generators in high-speed flow. Prog. Aerosp. Sci. 2012, 53, 30–45. [Google Scholar] [CrossRef]
- Pitt Ford, C.; Babinsky, H. Micro-ramp control for oblique shock wave/boundary layer interactions. In Proceedings of the 37th AIAA Fluid Dynamics Conference and Exhibit, Miami, FL, USA, 25–28 June 2007; p. 4115. [Google Scholar] [CrossRef]
- Benek, J.A.; Suchyta, C.J.; Babinsky, H. Simulations of incident shock boundary layer interactions. In Proceedings of the 54th AIAA Aerospace Sciences Meeting, San Diego, CA, USA, 4–8 January 2016; p. 0352. [Google Scholar] [CrossRef]
- Benhachmi, D.; Greber, I.; Hingst, W.R. Experimental and numerical investigation of an oblique shock wave/turbulent boundary layer interaction with continuous suction. In Proceedings of the 27th Aerospace Sciences Meeting, Reno, NV, USA, 9–12 January 1989; p. 357. [Google Scholar] [CrossRef]
- Xie, W.Z.; Yang, S.Z.; Zeng, C.; Liao, K.; Ding, R.H.; Zhang, L.; Guo, S.M. Improvement of the free-interaction theory for shock wave/turbulent boundary layer interactions. Phys. Fluids 2021, 33, 075104. [Google Scholar] [CrossRef]
- Huang, H.X.; Tan, H.J.; Sun, S.; Ling, Y. Evolution of supersonic corner vortex in a hypersonic inlet/isolator model. Phys. Fluids 2016, 28, 126101. [Google Scholar] [CrossRef]
- Li, N.; Chang, J.T.; Xu, K.J.; Yu, D.R.; Bao, W.; Song, Y.P. Prediction dynamic model of shock train with complex background waves. Phys. Fluids 2017, 29, 116103. [Google Scholar] [CrossRef]
- Sekar, K.R.; Karthick, S.; Jegadheeswaran, S.; Kannan, R. On the unsteady throttling dynamics and scaling analysis in a typical hypersonic inlet–isolator flow. Phys. Fluids 2020, 32, 126104. [Google Scholar] [CrossRef]
- Tang, D.G.; Li, J.P.; Zeng, F.Z.; Li, Y.; Yan, C. Bayesian parameter estimation of SST model for shock wave-boundary layer interaction flows with different strengths. Chin. J. Aeronaut. 2023, 36, 217–236. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- ANSYS Inc. Fluent Theory Guide; ANSYS Inc.: Canonsburg, PA, USA, 2022. [Google Scholar]
- Grossman, I.J.; Bruce, P.J. Confinement effects on regular–irregular transition in shock-wave–boundary-layer interactions. J. Fluid Mech. 2018, 853, 171–204. [Google Scholar] [CrossRef]
- Matheis, J.; Hickel, S. On the transition between regular and irregular shock patterns of shock-wave/boundary-layer interactions. J. Fluid Mech. 2015, 776, 200–234. [Google Scholar] [CrossRef]
- Teramoto, S. Large eddy simulation of shock wave/boundary layer interaction. Trans. Jpn. Soc. Aeronaut. Space Sci. 2005, 47, 268–275. [Google Scholar] [CrossRef][Green Version]
- Wollblad, C.; Davidson, L.; Eriksson, L.-E. Large eddy simulation of transonic flow with shock wave/turbulent boundary layer interaction. AIAA J. 2006, 44, 2340–2353. [Google Scholar] [CrossRef]

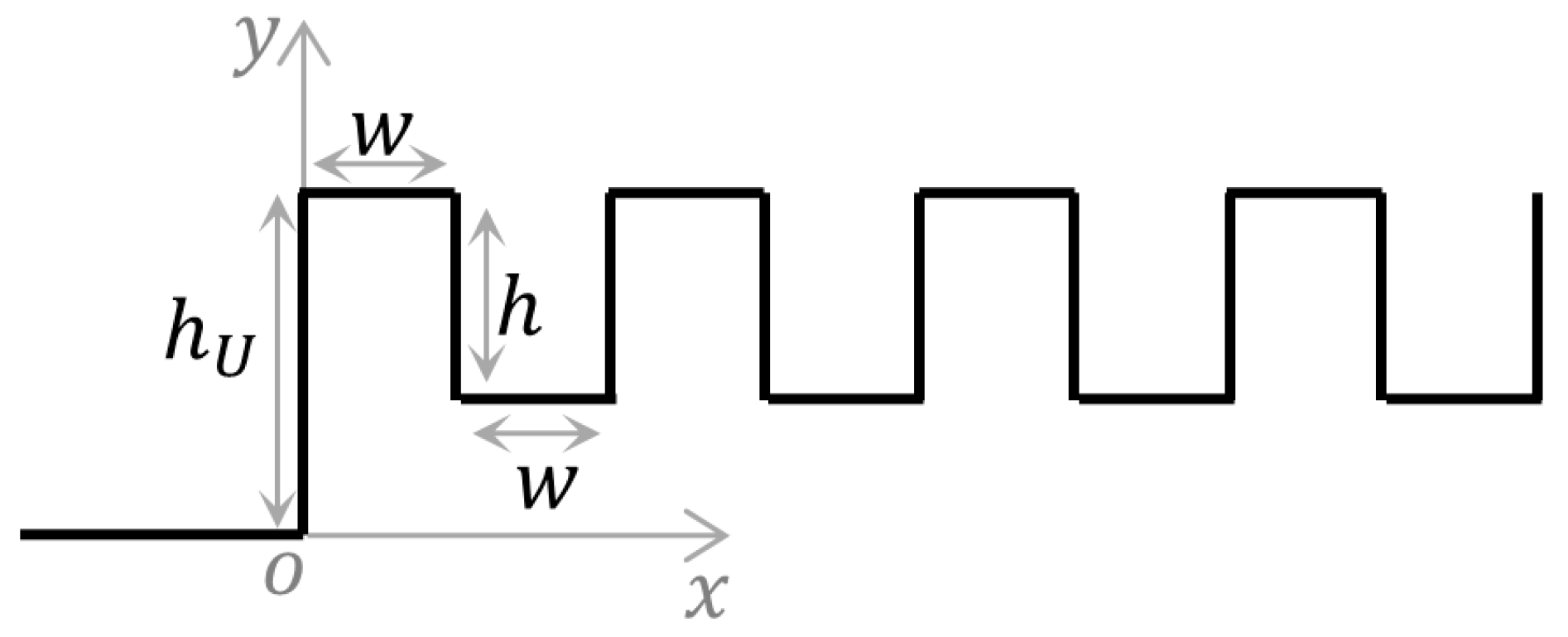


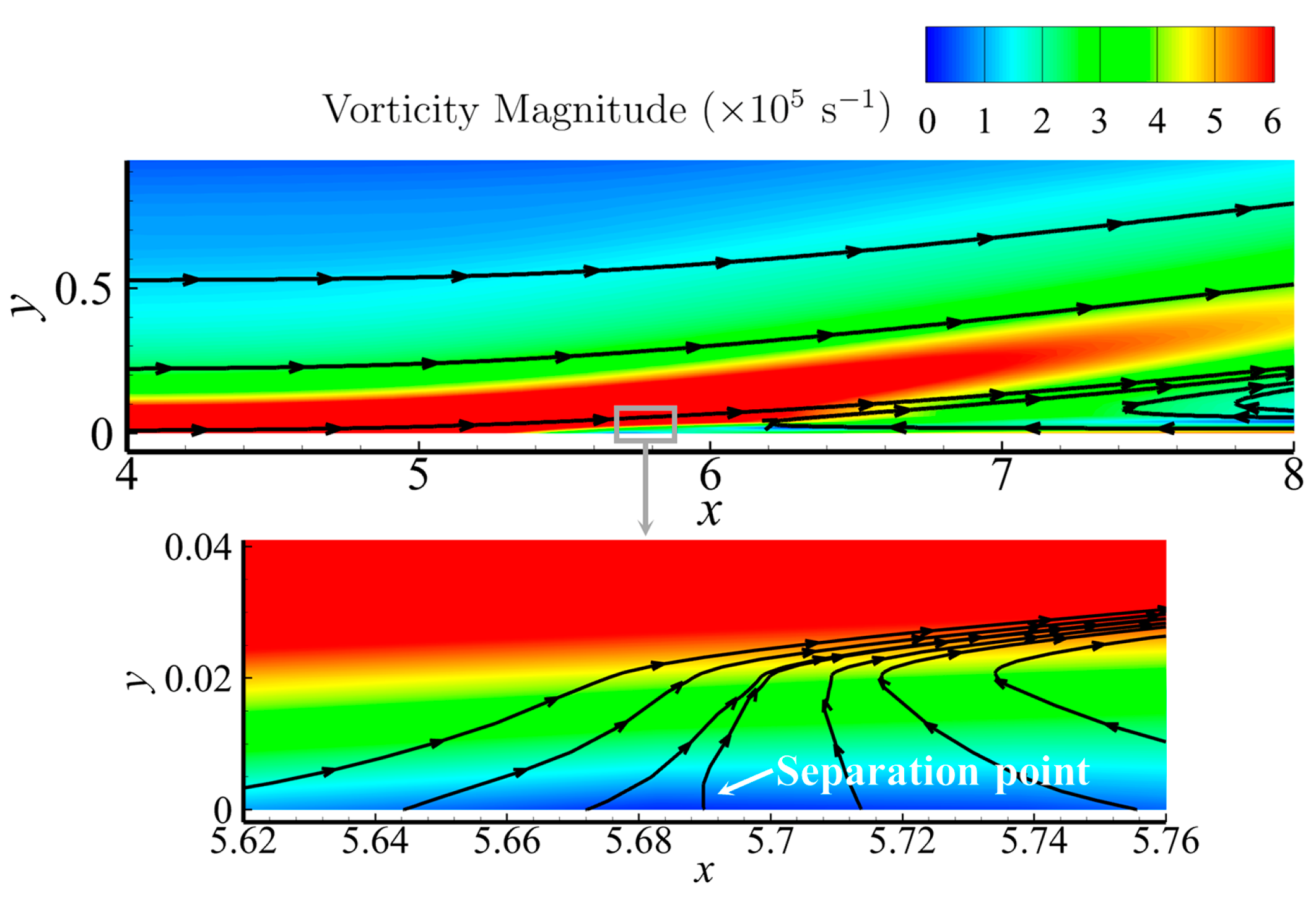

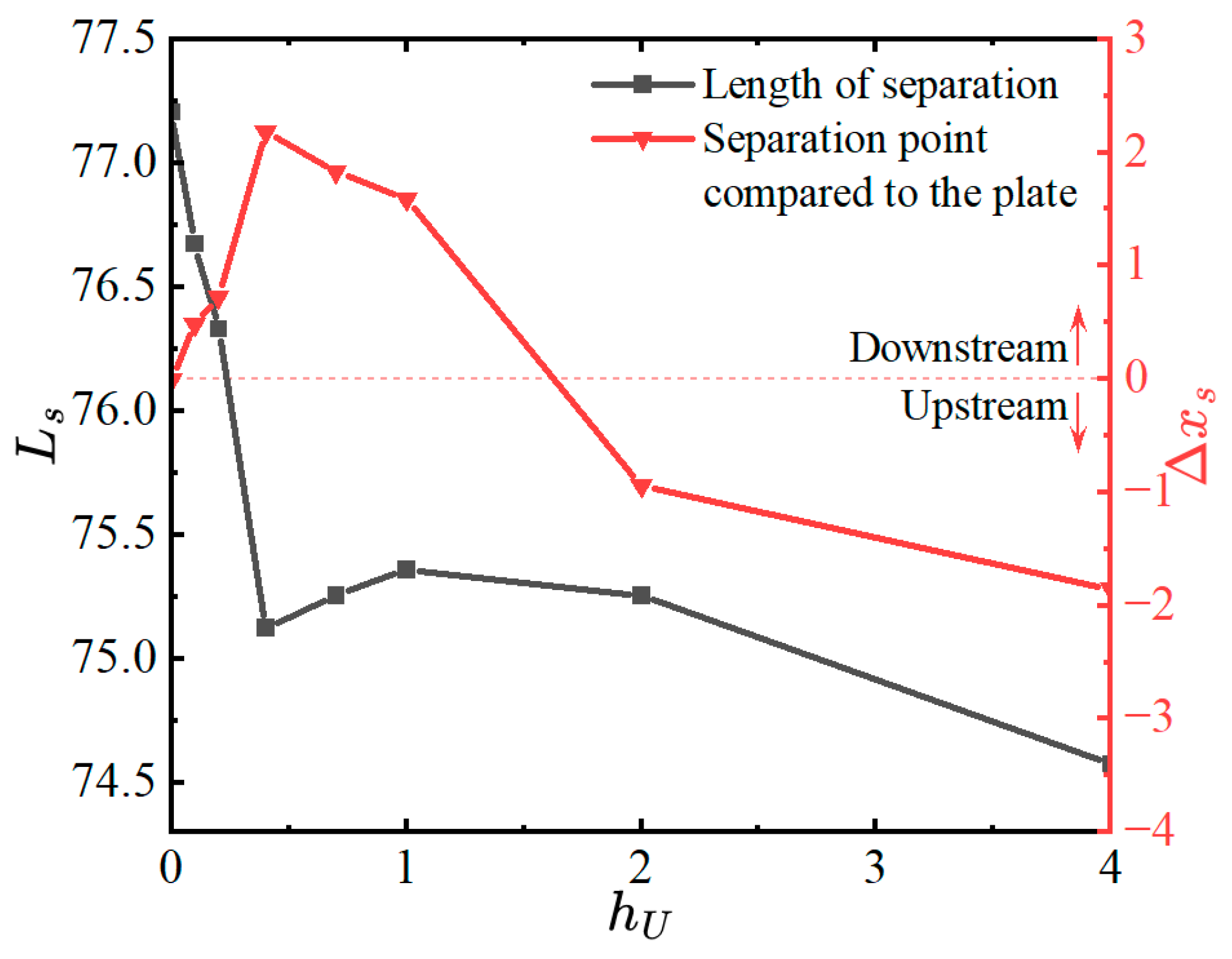
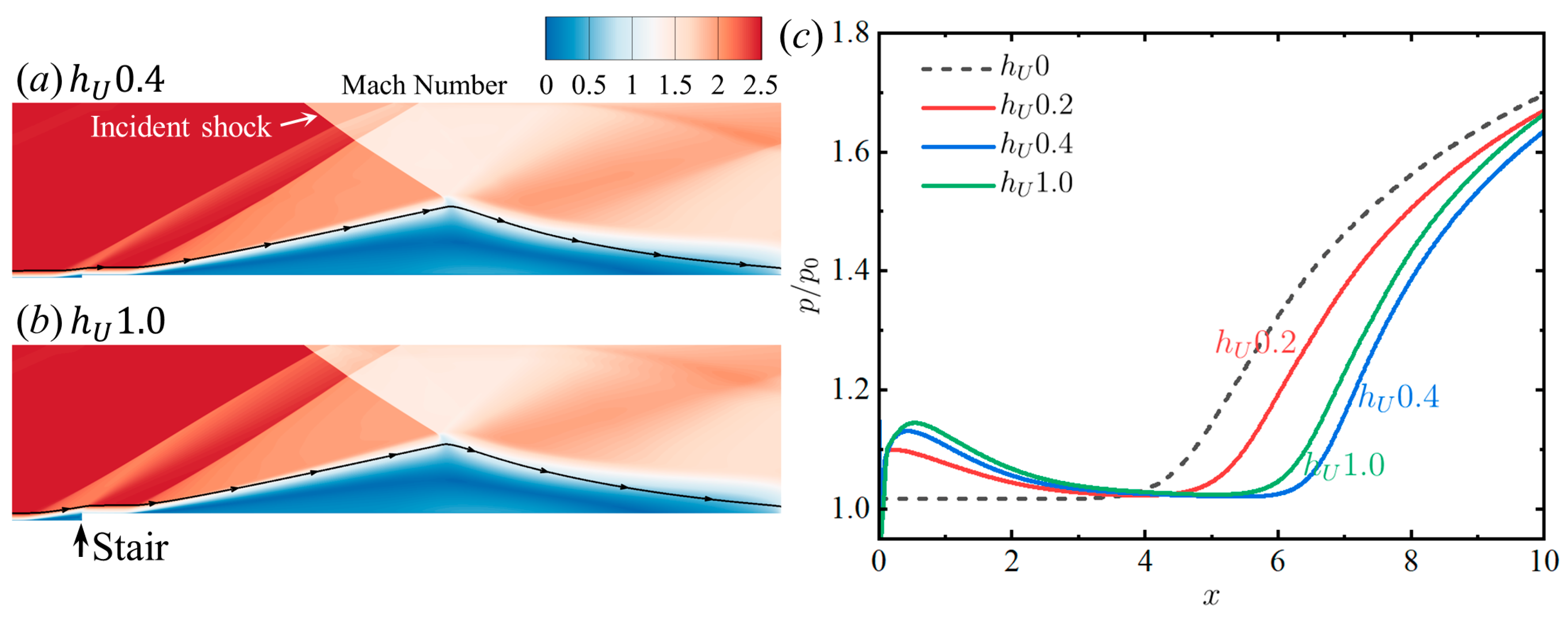
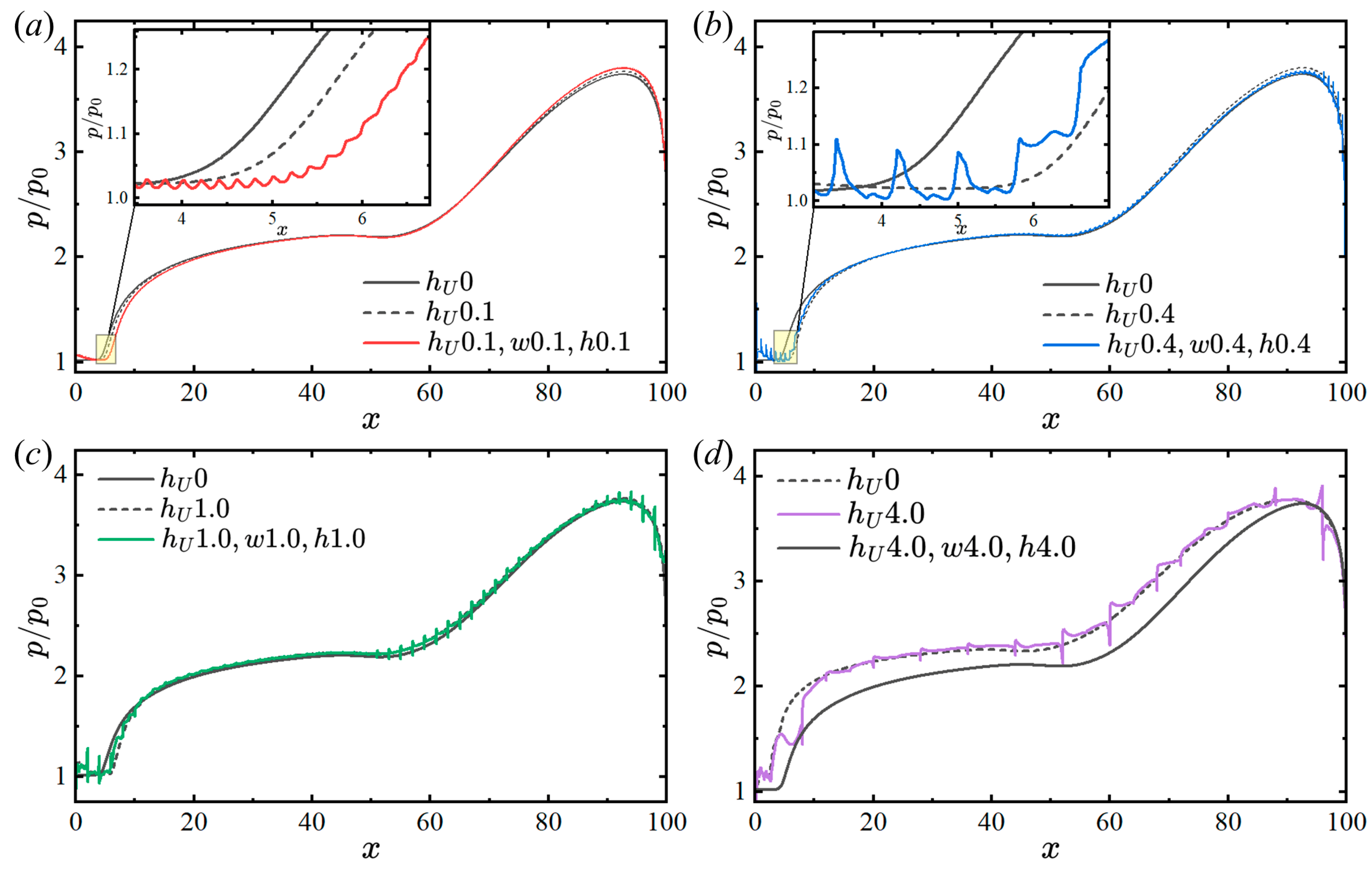
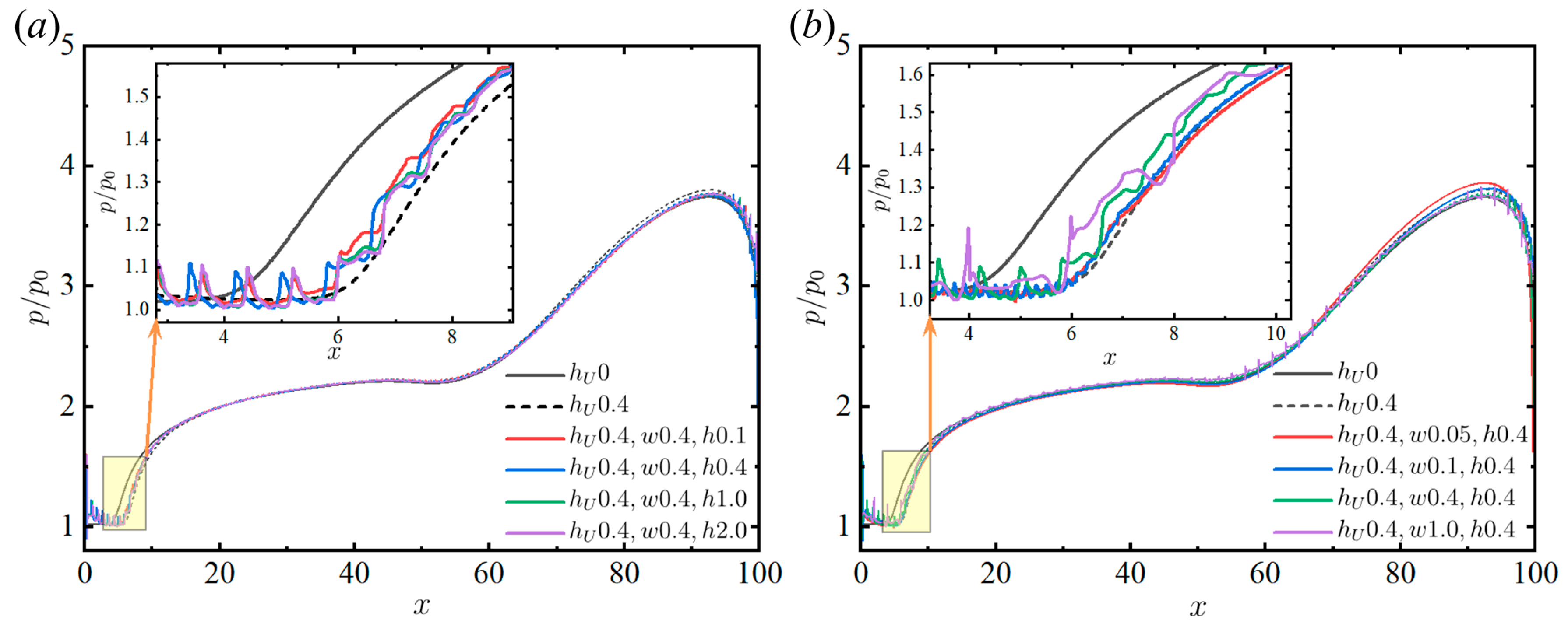
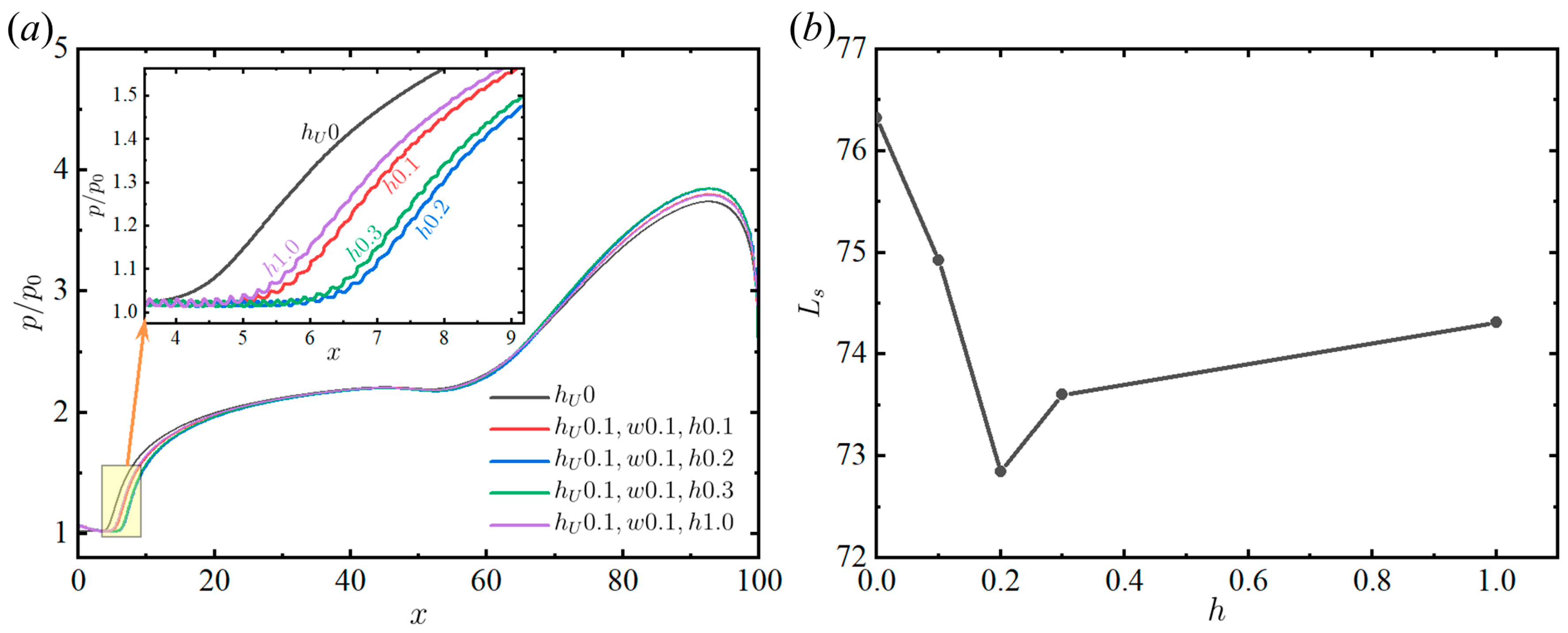
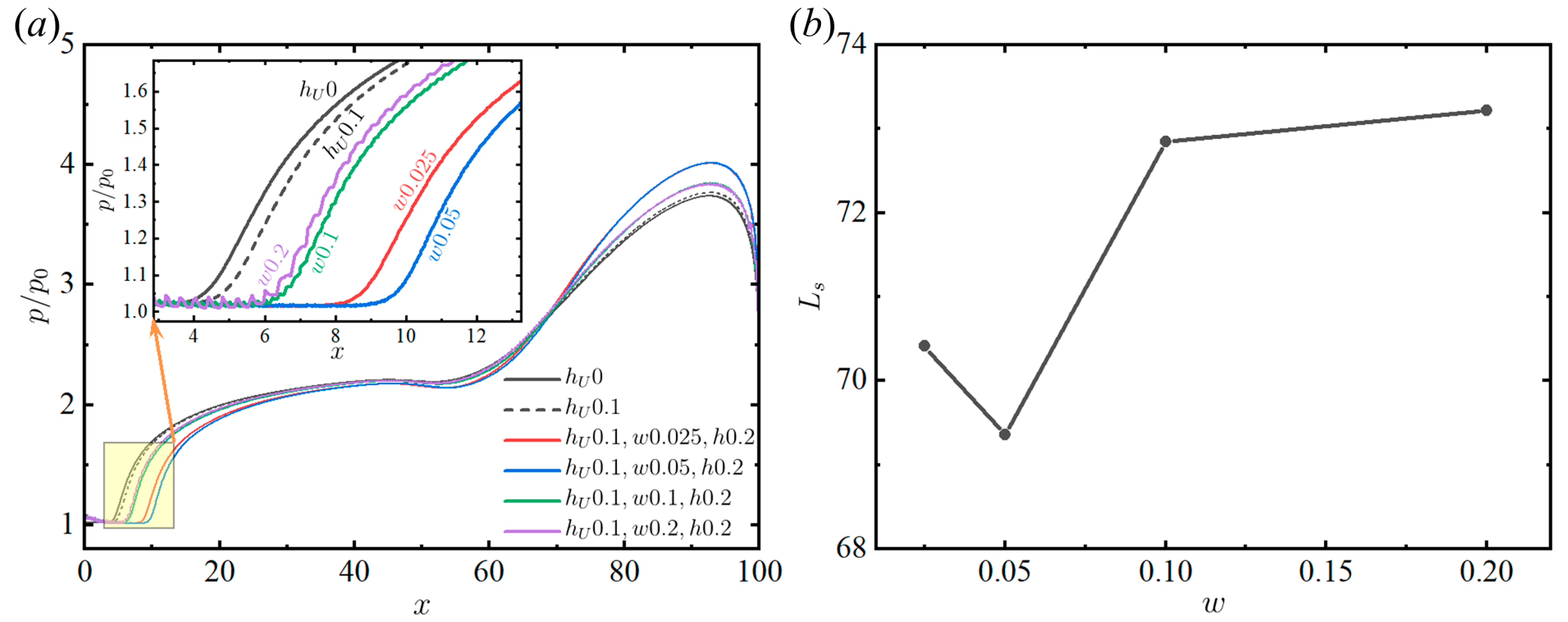
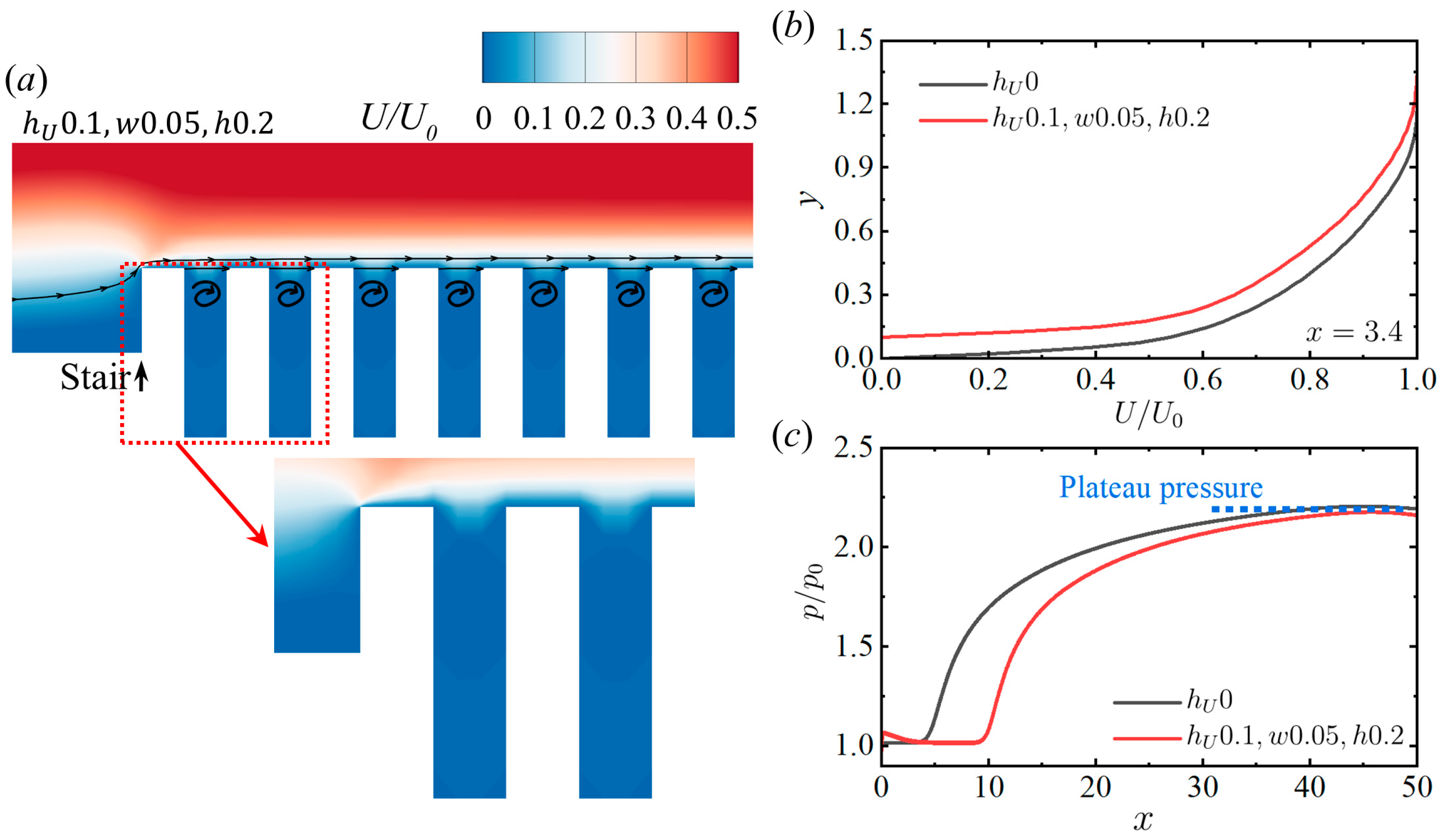

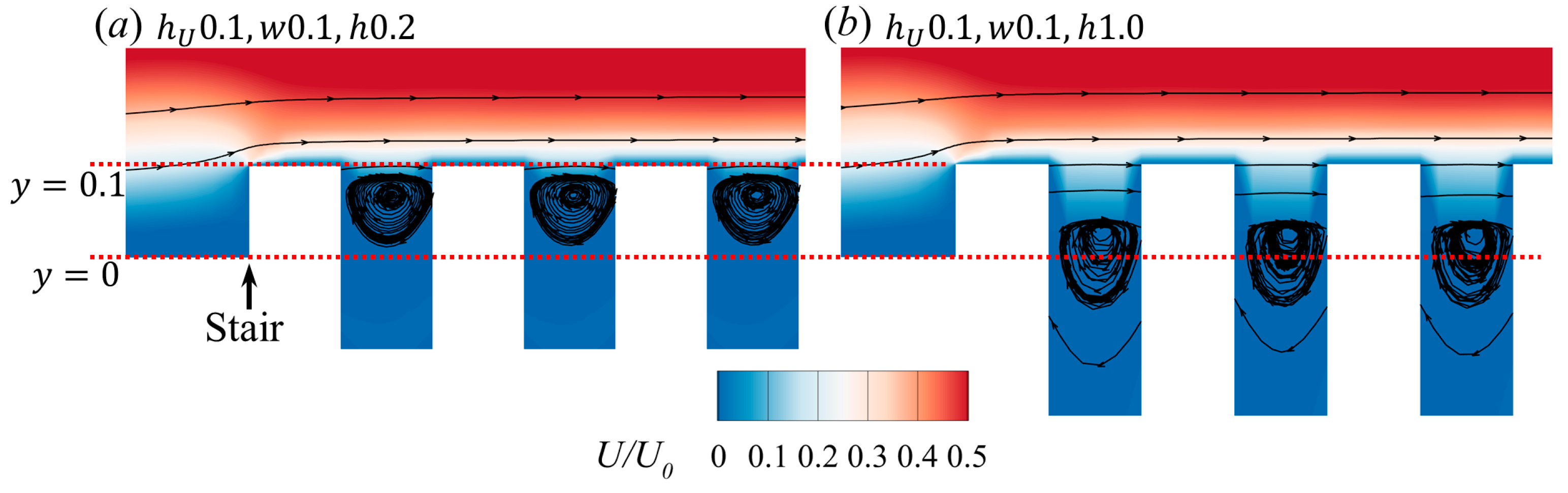
| Parameters | Explanations |
|---|---|
| Incoming Mach number | |
| Dimensionless height of the first windward stair at = 0 | |
| Dimensionless width of the micro-serration | |
| Dimensionless depth of the micro-serration | |
| The deflection angle of incident shock (deg.) | |
| The impinging point of the inviscid incident shock at the bottom wall (, 0) |
| Separation Point | Reattachment Point | Separation Length | |
|---|---|---|---|
| 5.32 | 82.53 | 77.21 | |
| Vorticity magnitude | 5.69 | 82.02 | 76.33 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, F.; Gao, Z.; Zhang, Q.; Yue, L.; Chen, H. Mitigation of Shock-Induced Separation Using Square-Shaped Micro-Serrations—A Preliminary Study. Aerospace 2024, 11, 148. https://doi.org/10.3390/aerospace11020148
Yu F, Gao Z, Zhang Q, Yue L, Chen H. Mitigation of Shock-Induced Separation Using Square-Shaped Micro-Serrations—A Preliminary Study. Aerospace. 2024; 11(2):148. https://doi.org/10.3390/aerospace11020148
Chicago/Turabian StyleYu, Fangyou, Zhanbiao Gao, Qifan Zhang, Lianjie Yue, and Hao Chen. 2024. "Mitigation of Shock-Induced Separation Using Square-Shaped Micro-Serrations—A Preliminary Study" Aerospace 11, no. 2: 148. https://doi.org/10.3390/aerospace11020148
APA StyleYu, F., Gao, Z., Zhang, Q., Yue, L., & Chen, H. (2024). Mitigation of Shock-Induced Separation Using Square-Shaped Micro-Serrations—A Preliminary Study. Aerospace, 11(2), 148. https://doi.org/10.3390/aerospace11020148






