Calculation and Selection of Airfoil for Flapping-Wing Aircraft Based on Integral Boundary Layer Equations
Abstract
:1. Introduction
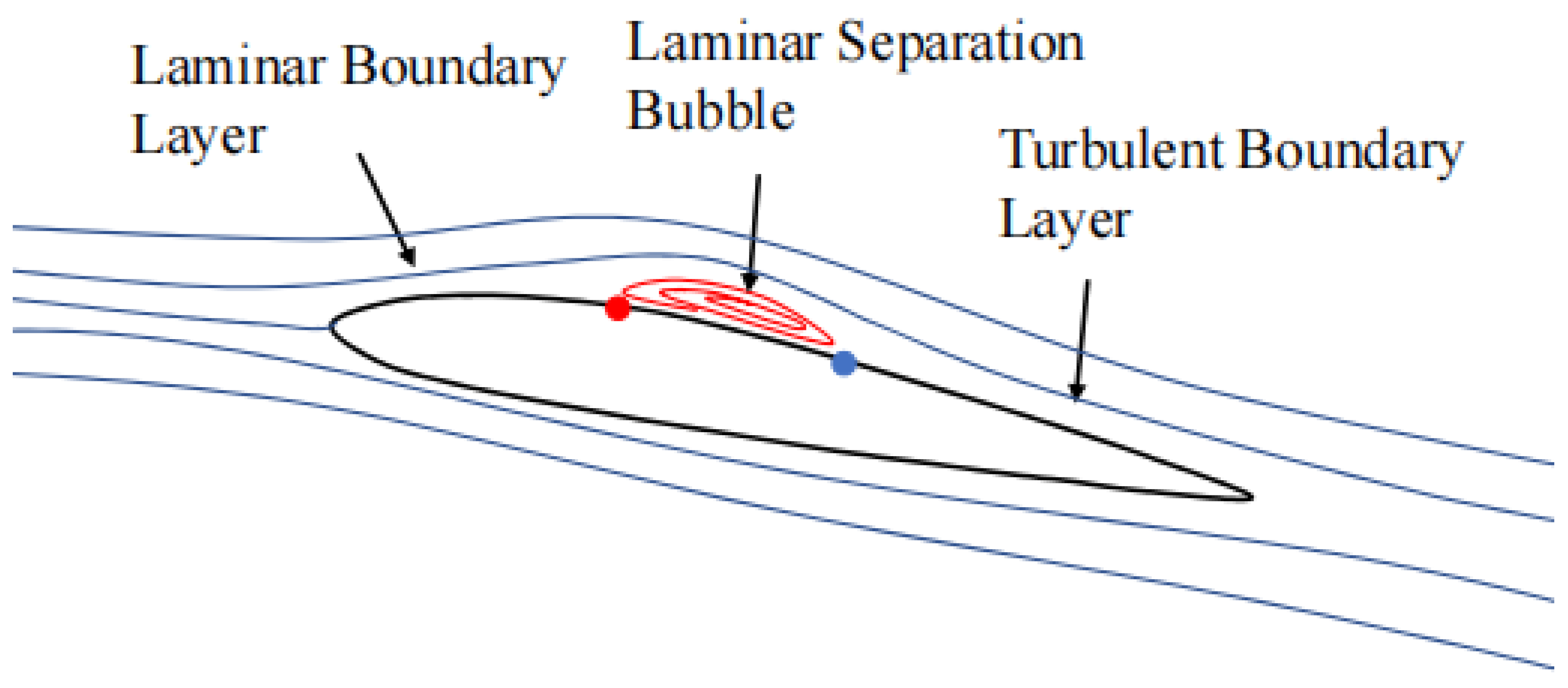
1.1. Characteristics of the Problem
1.2. Review of Literature
1.3. Shortcomings with Existing Research
1.4. The Innovations and Work of This Article
2. Theoretical Method
2.1. Boundary Layer Calculation
2.1.1. Momentum and Kinetic Energy Equations
2.1.2. Laminar Integral Equations
2.1.3. Turbulence Integral Equations
2.1.4. Transition
2.2. 2-D Unsteady Panel Method
2.2.1. Panel Method Model
2.2.2. Wake Shape
2.2.3. Boundary Layer Thickness Effect
2.3. Equation Creation
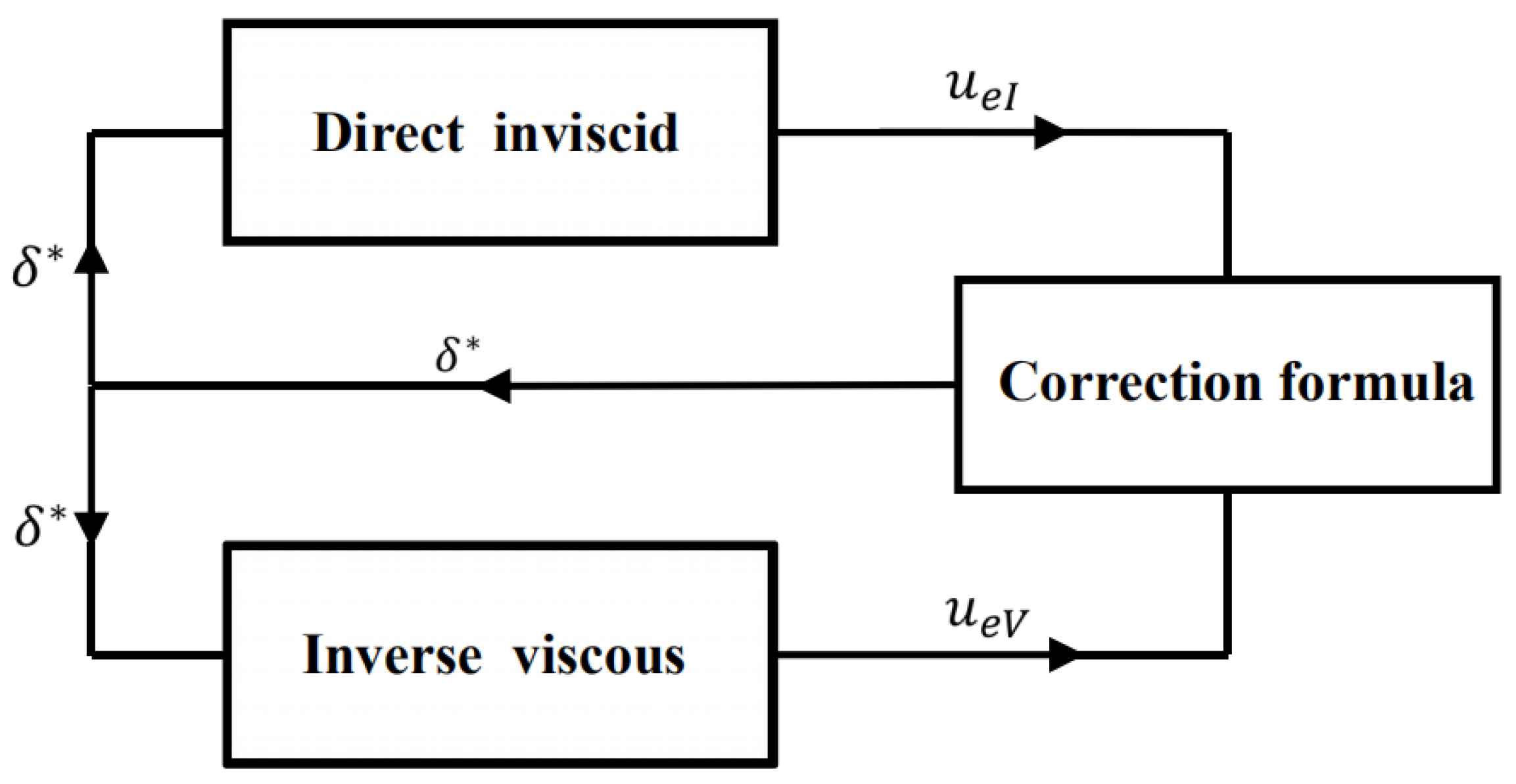
2.4. Viscous/Inviscid Interaction
2.5. Performance Parameter
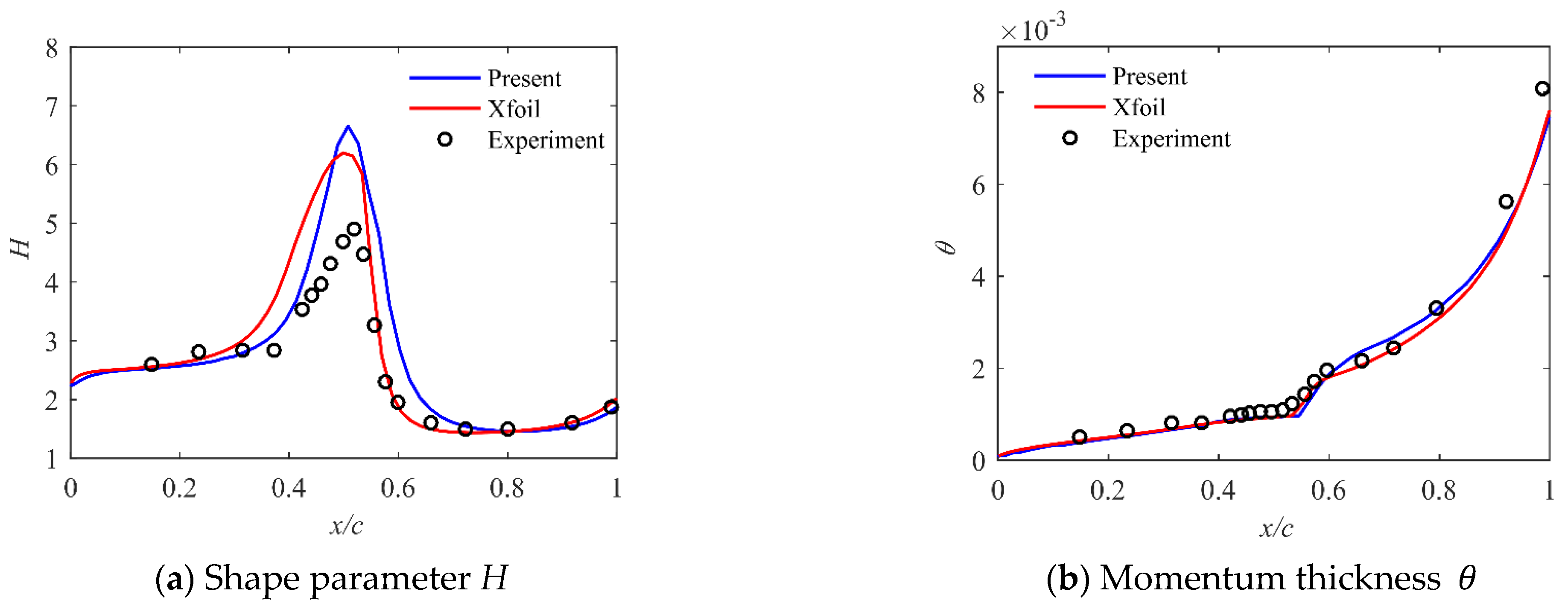
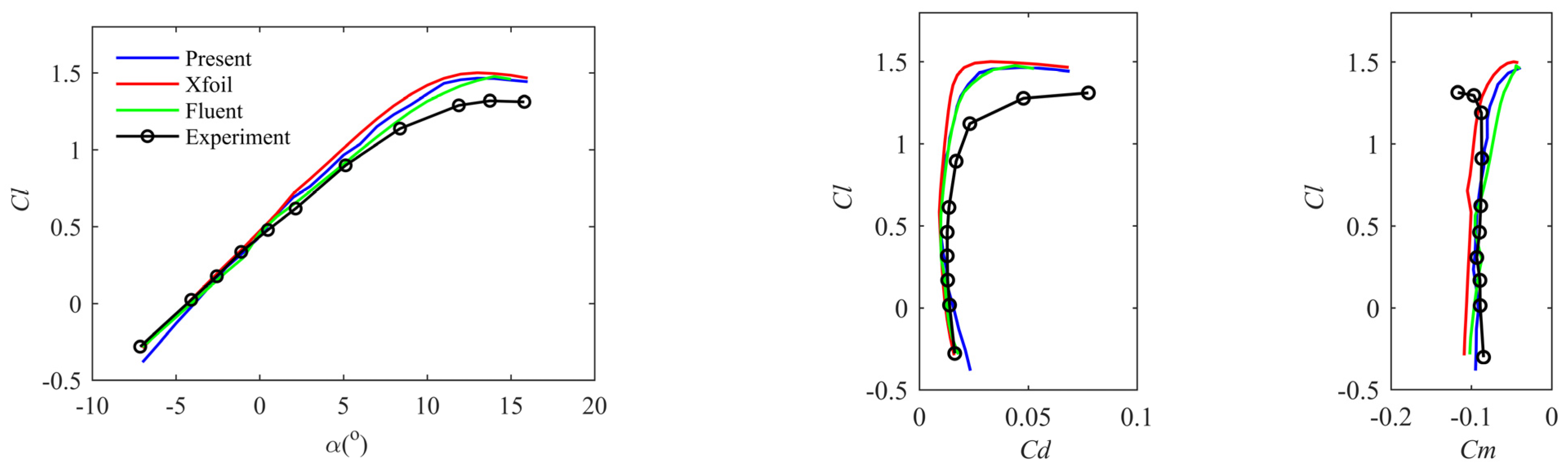
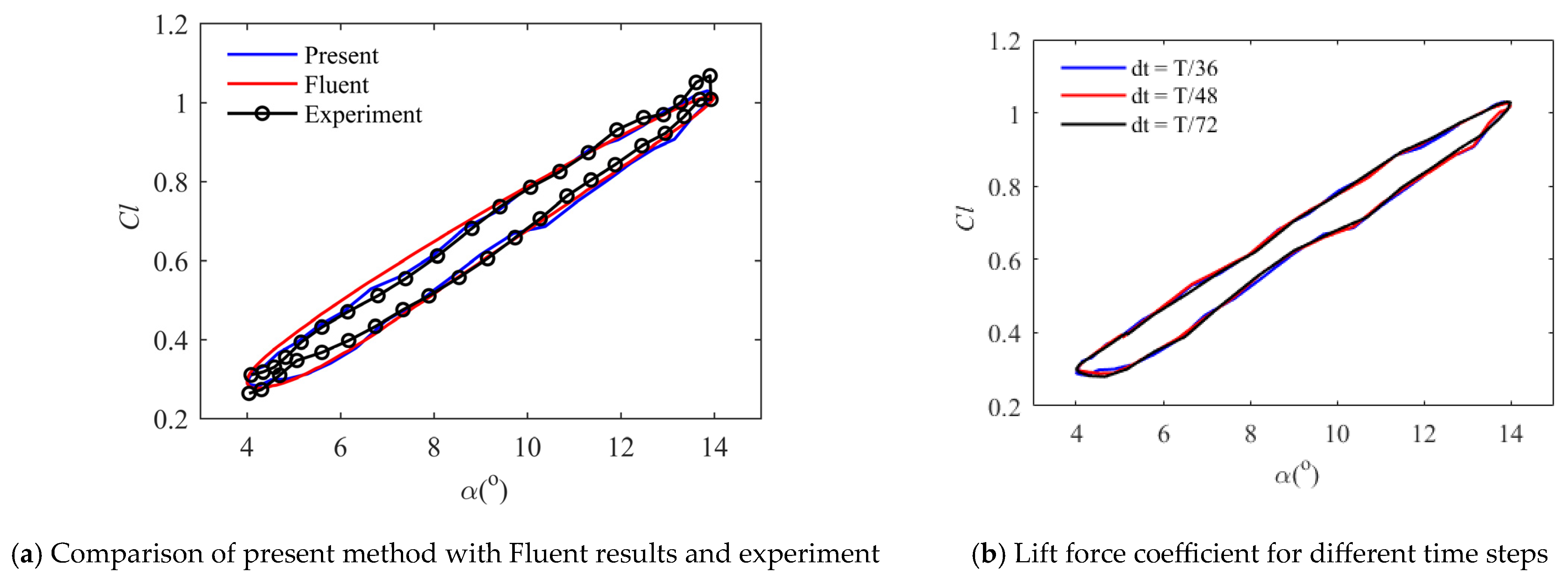
3. Method Validation
3.1. Steady State Situation
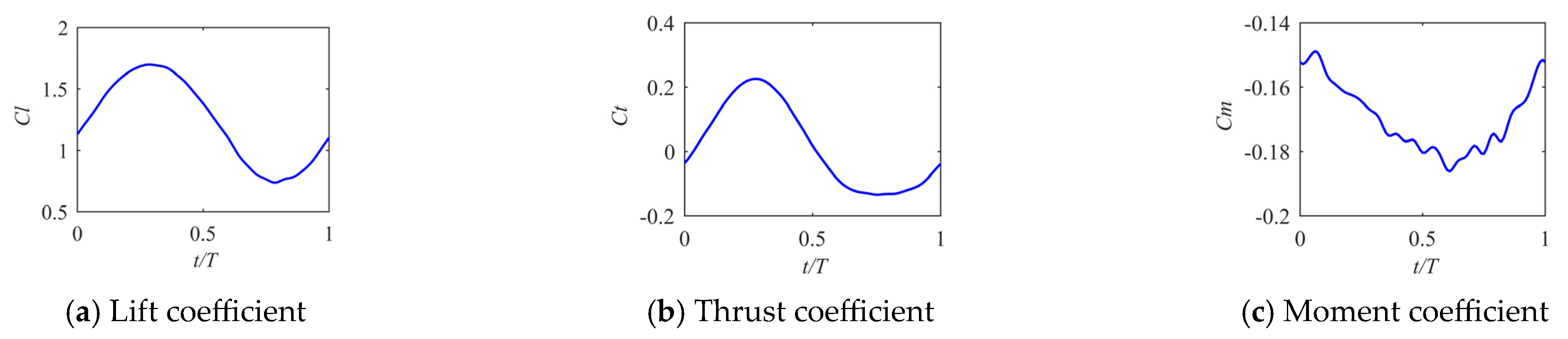
3.2. Unsteady State Situation
4. Results and Discussion
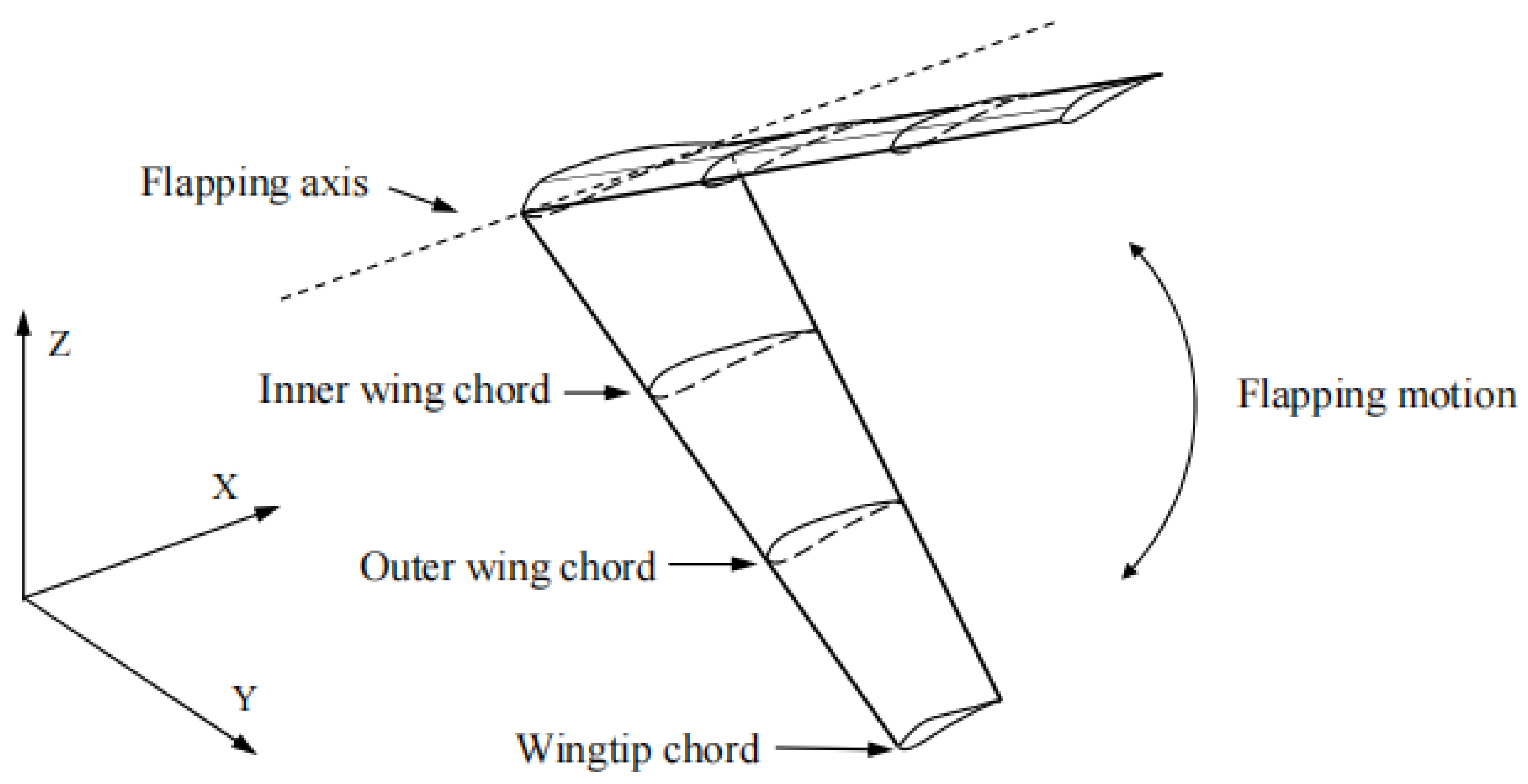
4.1. Wing Model
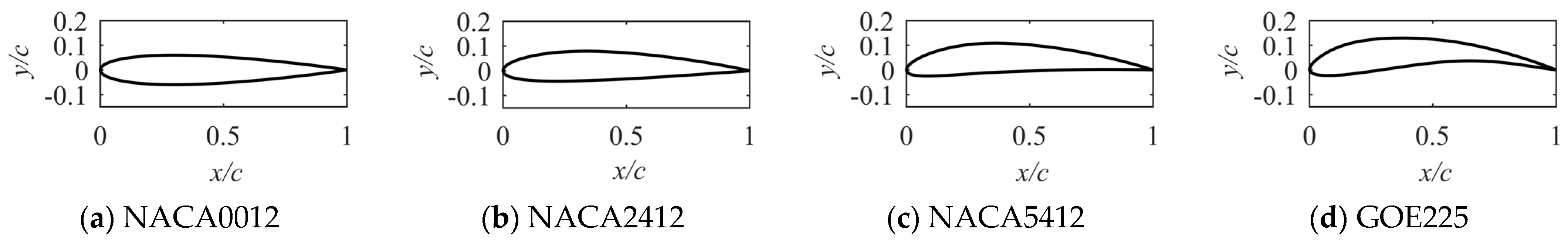
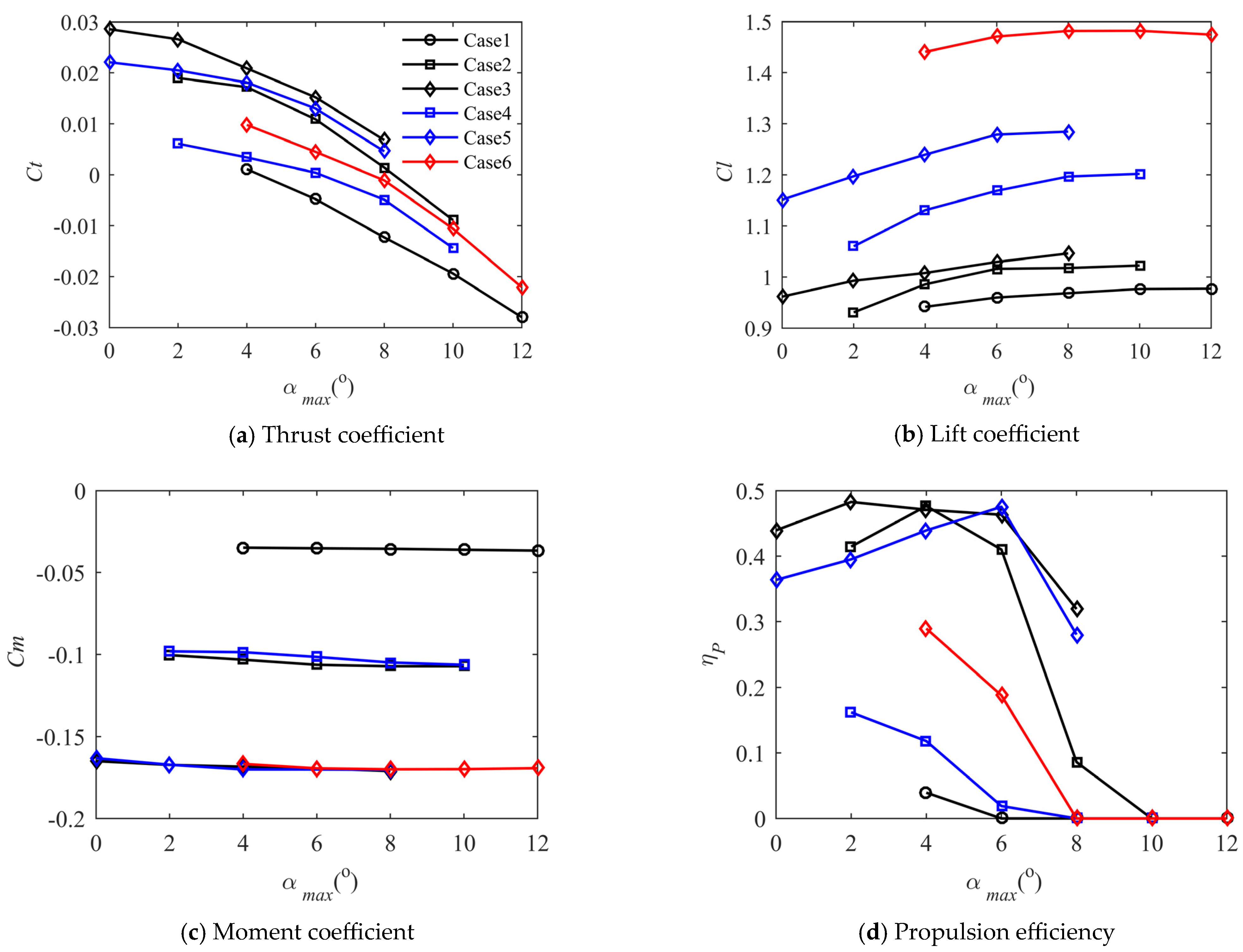
4.2. Wingtip Airfoil
4.3. Outer-Wing Airfoil
4.4. Inner-Wing Airfoil
5. Conclusions
- The method proposed in this article can quickly and accurately calculate the steady and unsteady aerodynamic forces of the airfoil in a Reynolds number range of 105. This method can exhibit typical low-Reynolds-number phenomena, such as laminar flow separation, transition, and turbulent separation at the trailing edge. Compared with the inviscid model, it can more accurately calculate the viscous aerodynamic force. Compared with the method of solving the global N–S equations, it saves a lot of time and is suitable for the early design stage.
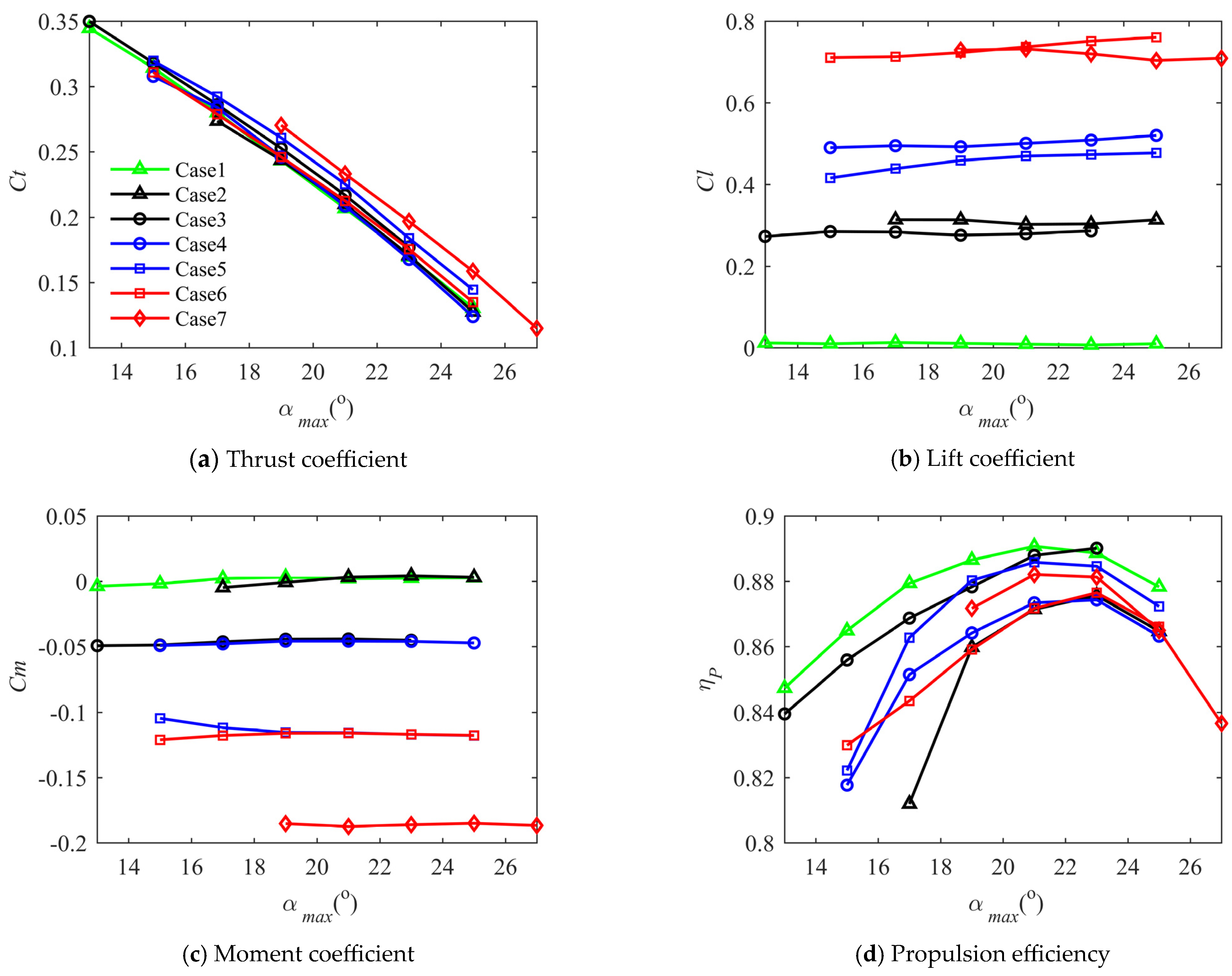
- The lift coefficient of the unsteady airfoil increases with the increase of the airfoil camber and average angle of attack, and the thrust coefficient decreases with the increase of the airfoil camber and average angle of attack. The propulsive efficiency of the same airfoil increases and then decreases with pitch amplitude, and airfoils with a small curvature and big plunge amplitude have a high propulsive efficiency. The moment coefficient only increases with the airfoil camber.
- The airfoil’s camber significantly impacts the lift coefficient, thrust coefficient, and propulsion efficiency. To optimize flight efficiency, it is essential to choose airfoils with varying cambers for different sections of the ornithopter’s wings, aligning with the distinct requirements of each wing section.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| dissipation coefficient | |
| skin friction coefficient | |
| shear stress coefficient | |
| shape parameter | |
| kinetic energy shape parameter | |
| momentum thickness Reynolds number | |
| boundary layer edge velocity | |
| boundary layer thickness | |
| displacement thickness | |
| thin shear layer coordinates | |
| momentum thickness | |
| kinetic energy thickness | |
| boundary layer edge viscosity | |
| density | |
| boundary layer density | |
| reduced frequency | |
| Strouhal number |
References
- Shyy, W.; Aono, H.; Kang, C. An Introduction to Flapping Wing Aerodynamics; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar]
- Winslow, J.; Otsuka, H.; Govindarajan, B.; Chopra, I. Basic understanding of airfoil characteristics at low Reynolds numbers (104–105). J. Aircr. 2018, 55, 1050–1061. [Google Scholar] [CrossRef]
- Badrya, C.; Govindarajan, B.; Chopra, I. Basic understanding of unsteady airfoil aerodynamics at low Reynolds numbers. In Proceedings of the AIAA Aerospace Sciences Meeting, Kissimmee, FL, USA, 8–12 January 2018. [Google Scholar]
- Shyy, W.; Klevebring, F.; Nilsson, M. Rigid and flexible low Reynolds number airfoils. J. Aircr. 1999, 36, 523–529. [Google Scholar] [CrossRef]
- Vest, M.S. Unsteady Aerodynamics and Propulsive Characteristics of Flapping Wings with Applications to Avian Flight. Ph.D. Thesis, University of California, San Diego, CA, USA, 1996. [Google Scholar]
- Carruthers, A.C.; Walker, S.M.; Thomas, A.L.R. Aerodynamics of aerofoil sections measured on a free-flying bird. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2009, 224, 855–864. [Google Scholar] [CrossRef]
- Lang, X.Y.; Song, B.F.; Yang, W.Q. Aerodynamic performance of owl-like airfoil undergoing bio-inspired flapping kinematics. Chin. J. Aeronaut. 2021, 34, 239–252. [Google Scholar] [CrossRef]
- Wu, T.; Song, B.F.; Song, W.P.; Yang, W.Q.; Xue, D.; Han, Z.H. Lift performance enhancement for flapping airfoils by considering surging motion. Chin. J. Aeronaut. 2022, 35, 194–207. [Google Scholar] [CrossRef]
- Ashraf, M.A.; Young, J.; Lai, J.C. Reynolds number, thickness and camber effects on flapping airfoil propulsion. J. Fluids Struct. 2011, 27, 145–160. [Google Scholar] [CrossRef]
- Chang, X.; Zhang, L.; Ma, R.; Wang, N. Numerical investigation on aerodynamic performance of a bionic flapping wing. Appl. Math. Mech. 2019, 40, 1625–1646. [Google Scholar] [CrossRef]
- DeLaurier, J.D. The development of an efficient ornithopter wing. Aeronaut. J. 1993, 97, 153–162. [Google Scholar] [CrossRef]
- Anderson, J.M.; Streitlien, K.; Barrett, D.S. Oscillating foils of high propulsive efficiency. J. Fluid Mech. 1998, 360, 41–72. [Google Scholar] [CrossRef]
- Young, J.; Lai, J.C. Oscillation frequency and amplitude effects on the wake of a plunging airfoil. AIAA J. 2004, 42, 2042–2052. [Google Scholar] [CrossRef]
- Tuncer, I.H.; Walz, R.; Platzer, M.F. A computational study on the dynamic stall of a flapping airfoil. In Proceedings of the 16th AIAA Applied Aerodynamics Conference, Albuquerque, NM, USA, 15–18 June 1998. [Google Scholar]
- Tuncer, I.H.; Kaya, M. Optimization of flapping airfoils for maximum thrust and propulsion efficiency. AIAA J. 2005, 43, 2329–2341. [Google Scholar] [CrossRef]
- Windte, J.; Radespiel, R. Propulsive efficiency of a moving airfoil at transitional low Reynolds numbers. AIAA J. 2008, 46, 2165–2177. [Google Scholar] [CrossRef]
- Radespiel, R.; Windte, J.; Scholz, U. Numerical and experimental flow analysis of moving airfoils with laminar separation bubbles. AIAA J. 2007, 45, 1346–1356. [Google Scholar] [CrossRef]
- Larijani, R.F. A Non-Linear Aeroelastic Model for the Study of Flapping-Wing Flight. Ph.D. Thesis, University of Toronto, Toronto, ON, Canada, 2001. [Google Scholar]
- Drela, M. XFOIL: An analysis and design system for low Reynolds number airfoils. In Low Reynolds Number Aerodynamics: Proceedings of the Conference Notre Dame, Indiana, USA, 5–7 June 1989; Springer: Berlin/Heidelberg, Germany, 1989. [Google Scholar]
- Ozdemir, H.; van Garrel, A.; Koodly Ravishankara, A. Unsteady interacting boundary layer method. In Proceedings of the 35th Wind Energy Symposium, Grapevine, TX, USA, 9–13 January 2017. [Google Scholar]
- García, N.R. Unsteady Viscous-Inviscid Interaction Technique for Wind Turbine Airfoils. Ph.D. Thesis, Technical University of Denmark, Lyngby, Denmark, 2011. [Google Scholar]
- Crimi, P.; Reeves, B.L. Analysis of leading edge separation bubbles on airfoils. AIAA J. 1976, 14, 1548–1555. [Google Scholar] [CrossRef]
- Horton, H.P. A Semi-Empirical Theory for the Growth and Bursting of Laminar Separation Bubbles; Technical Report No. 1073; Her Majesty’s Stationery Office: London, UK, 1949. [Google Scholar]
- Eppler, R.A.; Somers, D.M. A Computer Program for the Design and Analysis of Low-Speed Airfoils; Technical Report No. 80210; NASA: Washington, DC, USA, 1980.
- Ye, B. The Modeling of Laminar-to-Turbulent Transition for Unsteady Integral Boundary Layer Equations with High Order Discontinuous Galerkin Method. Master’s Thesis, Delft University of Technology, Delft, The Netherlands, 2015. [Google Scholar]
- Drela, M.; Giles, M.B. Viscous-inviscid analysis of transonic and low Reynolds number airfoils. AIAA J. 1987, 25, 1347–1355. [Google Scholar] [CrossRef]
- Schlichting, H.; Kestin, J. Boundary Layer Theory; McGraw-Hill: New York, NY, USA, 1961; pp. 195–207. [Google Scholar]
- Eppler, R. Practical Calculation of Laminar and Turbulent Bled-Off Boundary Layers; Technical Report No. NASA-TM-75328; NASA: Washington, DC, USA, 1978.
- Head, M.R.; Patel, V.C. Improved Entrainment Method for Calculating Turbulent Boundary Layer Development; Technical Report No. 3643; Her Majesty’s Stationery Office: London, UK, 1968. [Google Scholar]
- Green, J.E.; Weeks, D.J. Prediction of Turbulent Boundary Layers and Wakes in Incompressible Flow by a Lag Entrainment Method; Technical Report No. 3791; Her Majesty’s Stationery Office: London, UK, 1973. [Google Scholar]
- Swafford, T.W. Analytical approximation of two dimensional separated turbulent boundary layer velocity profiles. AIAA J. 1983, 21, 923–926. [Google Scholar] [CrossRef]
- Thomas, J.L. Integral boundary-layer models for turbulent separated flows. In Proceedings of the 17th Fluid Dynamics, Plasma Dynamics, and Lasers Conference, Snowmass, CO, USA, 25–27 June 1984. [Google Scholar]
- Smith, A.M.O. Transition, Pressure Gradient and Stability Theory; Technical Report No. ES 26388; Douglas Aircraft Company: Long Beach, NY, USA, 1956. [Google Scholar]
- Van Ingen, J.L. A Suggested Semi-Empirical Method for the Calculation of the Boundary-Layer Region; Technical Report No. VTH71; Delft University of Technology: Delft, The Netherlands, 1956. [Google Scholar]
- Obremski, H.J.; Morkovin, M.V.; Landahl, M. A Portfolio of Stability Characteristics of Incompressible Boundary Layers; Advisory Group for Aerospace Research and Development, NATO: Washington, DC, USA, 1969. [Google Scholar]
- Gleyzes, C.; Cousteix, J.; Bonnet, J. Theoretical and experimental study of low Reynolds number transitional separation bubbles. In Proceeding of the Conference on Low Reynolds Number Airfoil Aerodynamics, Notre Dame, IN, USA, 16–18 June 1985. [Google Scholar]
- Hess, J.L.; Smith, A.M.O. Calculation of potential flow about arbitrary bodies. Prog. Aeronaut. Sci. 1967, 8, 1–138. [Google Scholar] [CrossRef]
- Teng, N.H. The Development of a Computer Code (U2DIIF) for the Numerical Solution of Unsteady, Inviscid and Incompressible Flow Over an Airfoil. Ph.D Thesis, Naval Postgraduate School, Monterey, CA, USA, 1987. [Google Scholar]
- Lock, R.; Williams, B. Viscous-inviscid interactions in external aerodynamics. Prog. Aeronaut. Sci. 1987, 24, 51–171. [Google Scholar] [CrossRef]
- Carter, J. A new boundary-layer inviscid iteration technique for separated flow. In Proceedings of the 4th Computational Fluid Dynamics Conference, Williamsburg, VA, USA, 23–25 July 1979. [Google Scholar]
- Kwon, O.K.; Pletcher, R.H. Prediction of incompressible separated boundary layers including viscous-inviscid interaction. J. Fluids Eng. 1979, 101, 466–472. [Google Scholar] [CrossRef]
- Crank, J.; Nicolson, P. A practical method for numerical evaluation of solutions of partial differential equations of the heat-conduction type. In Mathematical Proceedings of the Cambridge Philosophical Society; Cambridge University Press: Cambridge, UK, 1947. [Google Scholar]
- LeBlanc, P.J. An Experimental Investigation of Transitional Instabilities in Laminar Separation Bubble Flows on Airfoils Operating at Low Reynolds numbers. Ph.D. Thesis, University of Southern California, Los Angeles, CA, USA, 1992. [Google Scholar]
- Jacobs, E.N.; Sherman, A. Airfoil Section Characteristics as Affected by Variations of the Reynolds Number; Technical Report No. 586; NACA: Washington, DC, USA, 1937. [Google Scholar]
- Carta, F.O. A Comparison of the Pitching and Plunging Response of an Oscillating Airfoil; Technical Report No. NASA CR 3172; NASA: Washington, DC, USA, 1979.
- Lang, X.; Song, B.; Yang, W.; Yang, X. Effect of spanwise folding on the aerodynamic performance of three dimensional flapping flat wing. Phys. Fluids 2022, 34, 021906. [Google Scholar] [CrossRef]




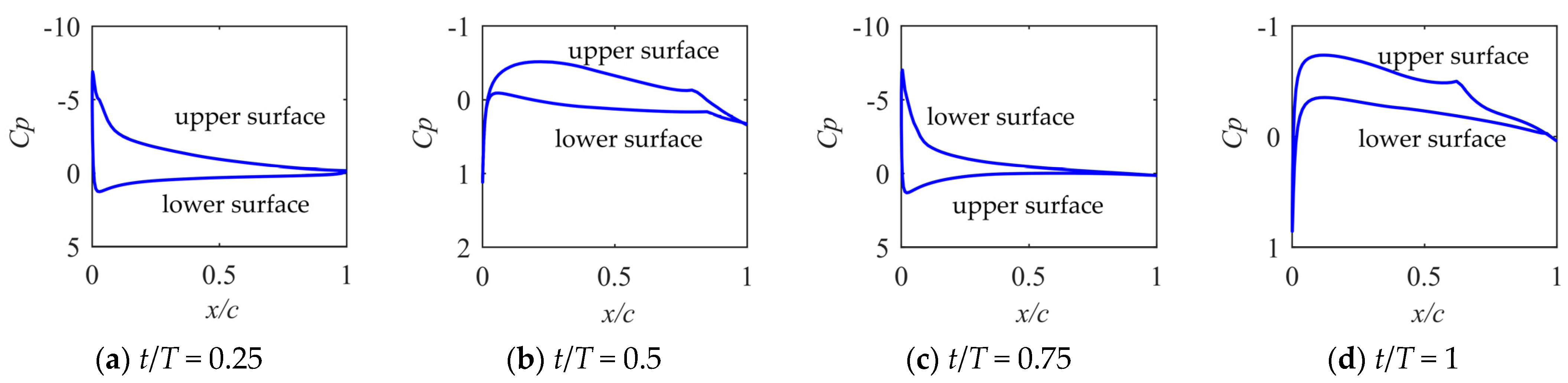
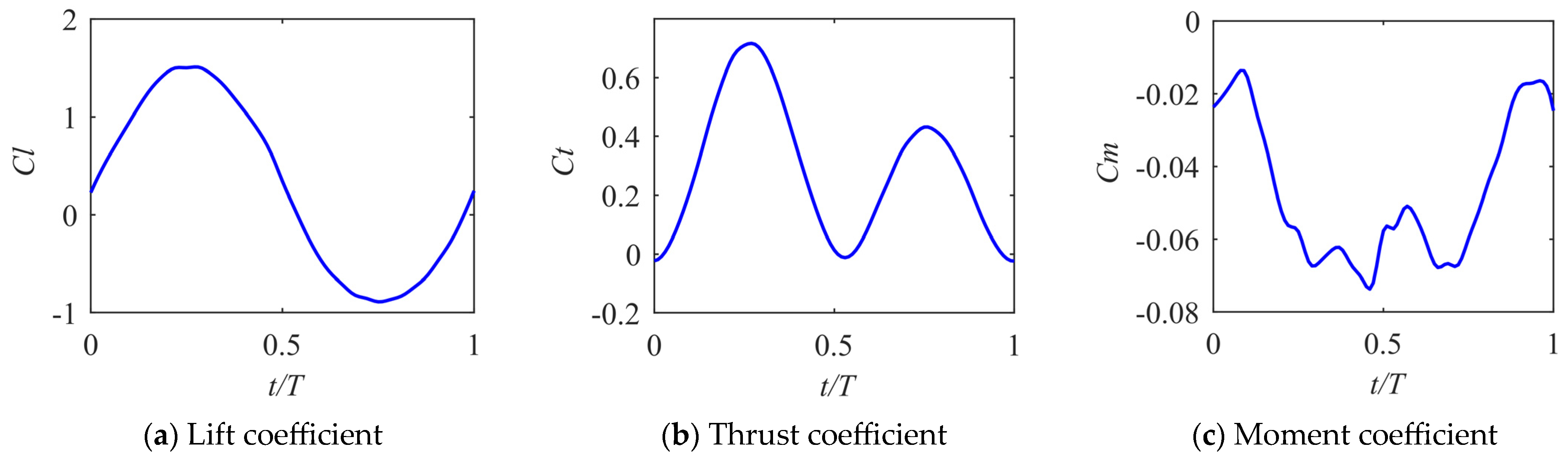
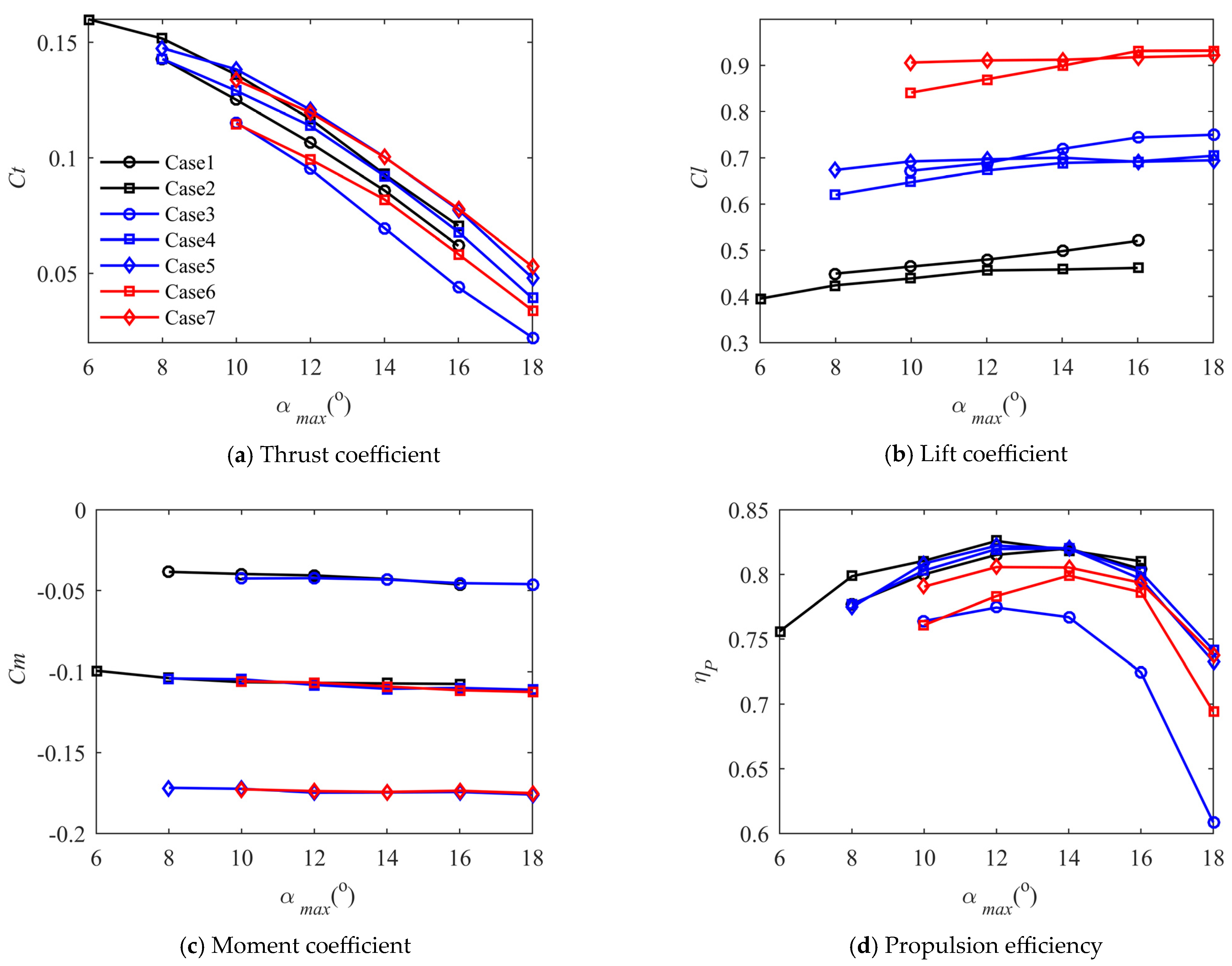
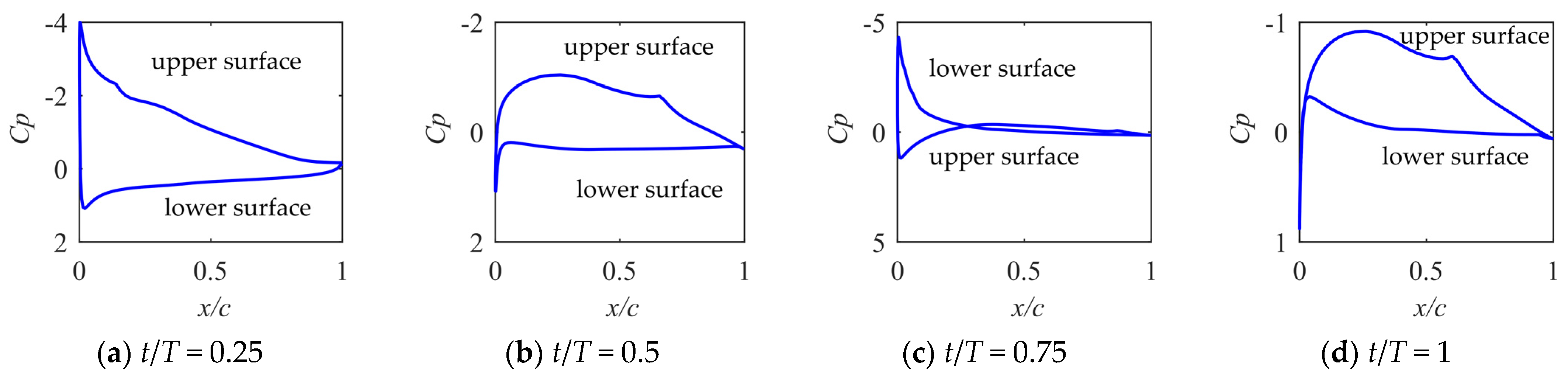


| Airfoil Panel Numbers | 48 | 96 | 144 |
|---|---|---|---|
| 0.835 | 0.848 | 0.850 | |
| 0.0136 | 0.0129 | 0.0127 |
| Airfoil | ||
|---|---|---|
| Case 1 | NACA0012 | |
| Case 2 | NACA0012 | |
| Case 3 | NACA2412 | |
| Case 4 | NACA2412 | |
| Case 5 | NACA5412 | |
| Case 6 | NACA5412 | |
| Case 7 | GOE225 |
| Airfoils | ||
|---|---|---|
| Case 1 | NACA2412 | |
| Case 2 | NACA5412 | |
| Case 3 | NACA2412 | |
| Case 4 | NACA5412 | |
| Case 5 | GOE225 | |
| Case 6 | NACA5412 | |
| Case 7 | GOE225 |
| Airfoil | ||
|---|---|---|
| Case 1 | NACA2412 | |
| Case 2 | NACA5412 | |
| Case 3 | GOE225 | |
| Case 4 | NACA5412 | |
| Case 5 | GOE225 | |
| Case 6 | GOE225 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qi, M.; Zhu, W.; Li, S. Calculation and Selection of Airfoil for Flapping-Wing Aircraft Based on Integral Boundary Layer Equations. Aerospace 2024, 11, 46. https://doi.org/10.3390/aerospace11010046
Qi M, Zhu W, Li S. Calculation and Selection of Airfoil for Flapping-Wing Aircraft Based on Integral Boundary Layer Equations. Aerospace. 2024; 11(1):46. https://doi.org/10.3390/aerospace11010046
Chicago/Turabian StyleQi, Ming, Wenguo Zhu, and Shu Li. 2024. "Calculation and Selection of Airfoil for Flapping-Wing Aircraft Based on Integral Boundary Layer Equations" Aerospace 11, no. 1: 46. https://doi.org/10.3390/aerospace11010046
APA StyleQi, M., Zhu, W., & Li, S. (2024). Calculation and Selection of Airfoil for Flapping-Wing Aircraft Based on Integral Boundary Layer Equations. Aerospace, 11(1), 46. https://doi.org/10.3390/aerospace11010046






