Numerical Investigation of a Vortex Diverter Designed for Improving the Performance of the Submerged Inlet
Abstract
:1. Introduction
- Install flow control devices near the entrance to displace the local boundary layer and prevent low-energy airflow from entering the intake duct. For example, Pérez installed a triangular wing vortex generator upstream of the entrance to reduce the boundary layer thickness, resulting in a 20% increase in the mass flow rate in the intake duct [19]. Sun placed a Bump-type vortex generator on the upstream surface, which effectively displaced the low-energy flow in the upstream boundary layer, improving the inlet flow quality. Under cruise conditions, the total pressure recovery coefficient at the exit increased by 3.7% [20]. Xie designed a boundary layer splitter that improved the quality of airflow inside the intake duct by displacing the low-energy flow in the boundary layer. The study results showed an improvement of 3.3% in the total pressure recovery coefficient of the intake duct and a 28.2% reduction in circumferential distortion DC60 [21]. Saheby designed a ridge-type vortex generator near the entrance, which can divert the boundary layer at the intake entrance. The total pressure recovery coefficient increased by 0.97%, 2.17%, and 4.33% at Mach numbers of 0.3, 0.5, and 0.8, respectively [22].
- Install flow control devices to promote the local beneficial mixing of airflow within the intake duct and facilitate energy exchange between the flows, thereby delaying flow separation. For example, Jirasek installed two sets of 64 micro-vane vortex generators inside the intake duct. The best control effect was achieved when the height of the vortex generators was approximately 50% of the local boundary layer thickness. This arrangement resulted in a 2% increase in the total pressure recovery coefficient and a nearly 50% reduction in the distortion index [23]. Micro-vane vortex generators are typically small in size and are proportionally related to the local boundary layer thickness. Each micro-vane vortex generator generates a small streamwise vortex, so an array of these generators is commonly used for flow control. Gissen studied the array configuration of micro-vane vortex generators and established a mapping relationship between the array parameters and the performance parameters of the intake duct using the response surface methodology. The results showed that the optimal control effect was achieved when the vane height was approximately 30% of the boundary layer thickness and the vane had a relative flow angle deviation of 13° [24].
- Direct the low energy flows within the intake duct to migrate towards designated locations and divert vortices and low energy flows out of the intake duct. For example, Cheng arranged a pair of vortex-excising slots on the sidewall of the intake duct. The exits of these slots led to the exterior surface of the body, resulting in two channels that could release vortices and low energy flows. After vortex discharged, the total pressure recovery coefficient increased by 2.8%, and the distortion index decreased by 51.0% [25]. Xie proposed a flow control method that combines boundary layer bleeding and ramp side-edge vortex diverting. During cruise flight, the total pressure recovery coefficient of the intake duct increased by 3.06%, and the DC60 value decreased by 72.57% [26].
2. Baseline Configuration of Submerged Inlet and Numerical Simulation Method
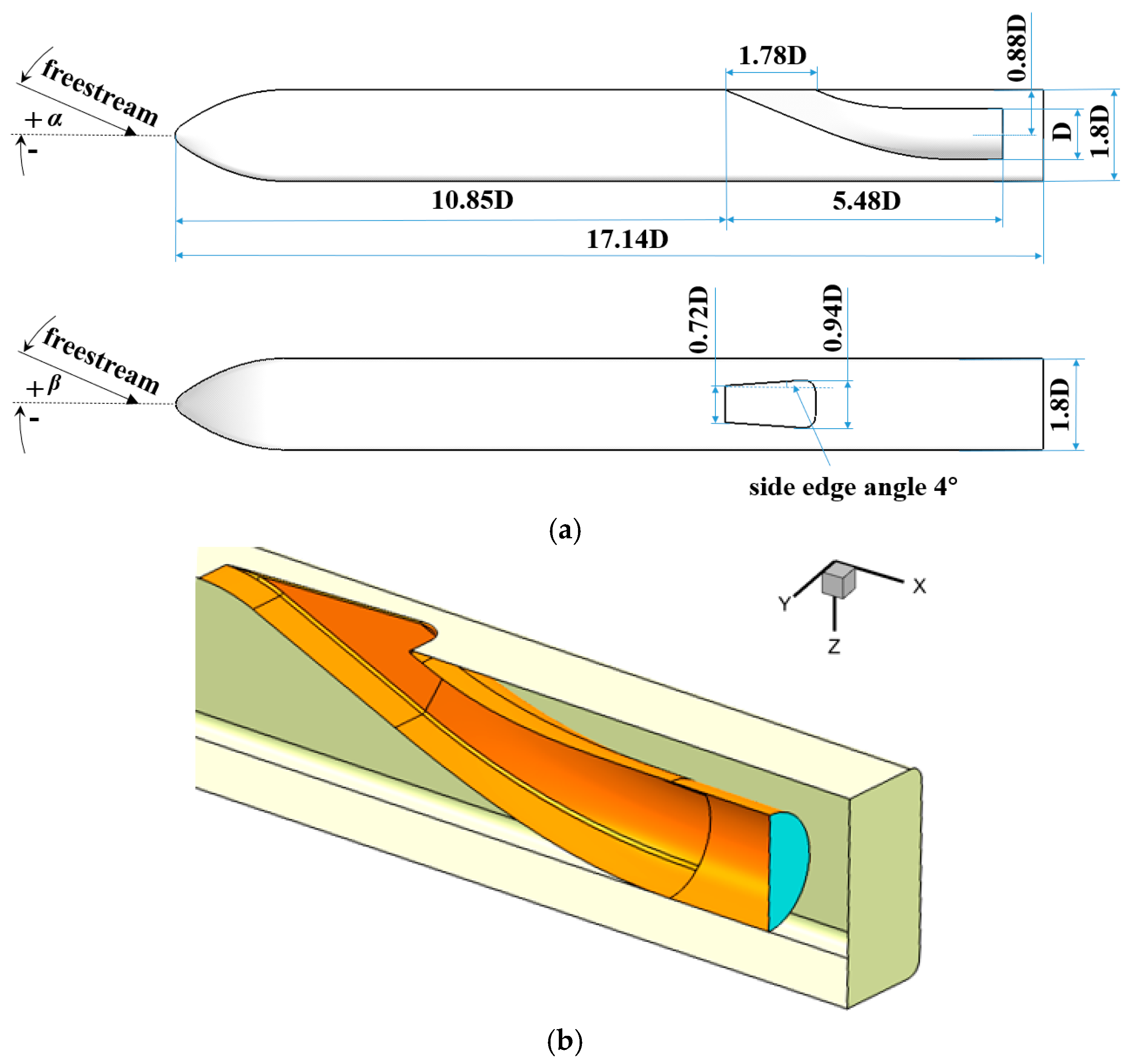
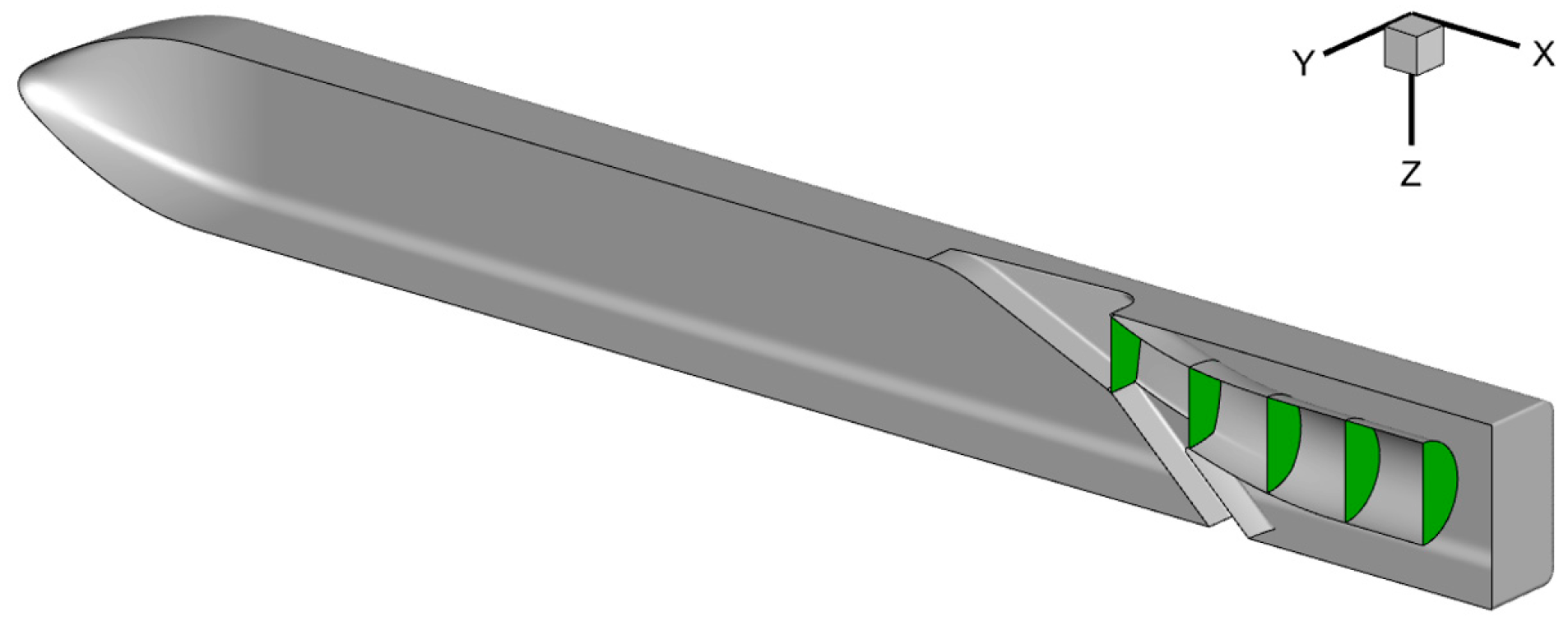
2.1. Baseline Configuration of Submerged Inlet
2.2. Numerical Simulation Method Validation
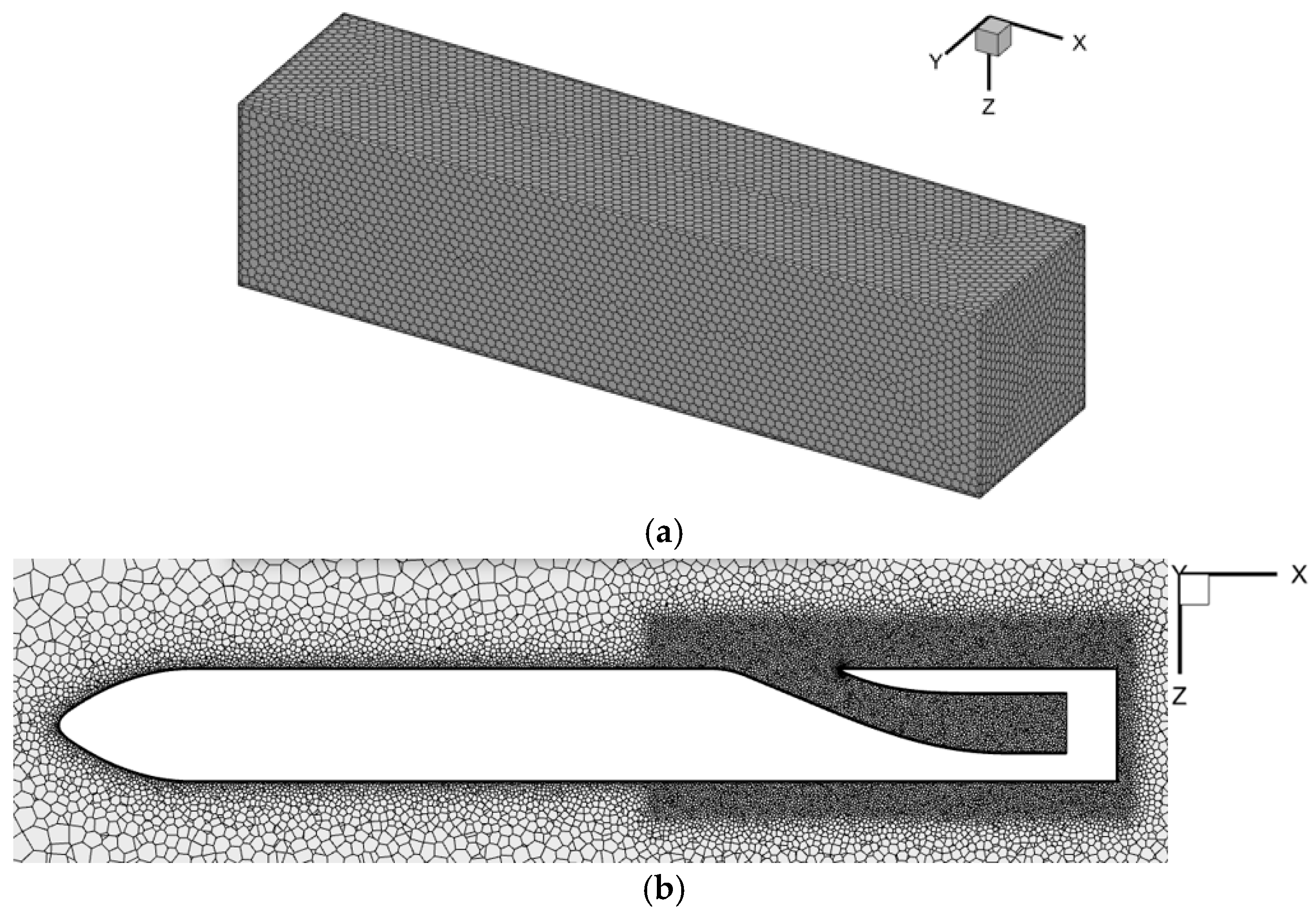
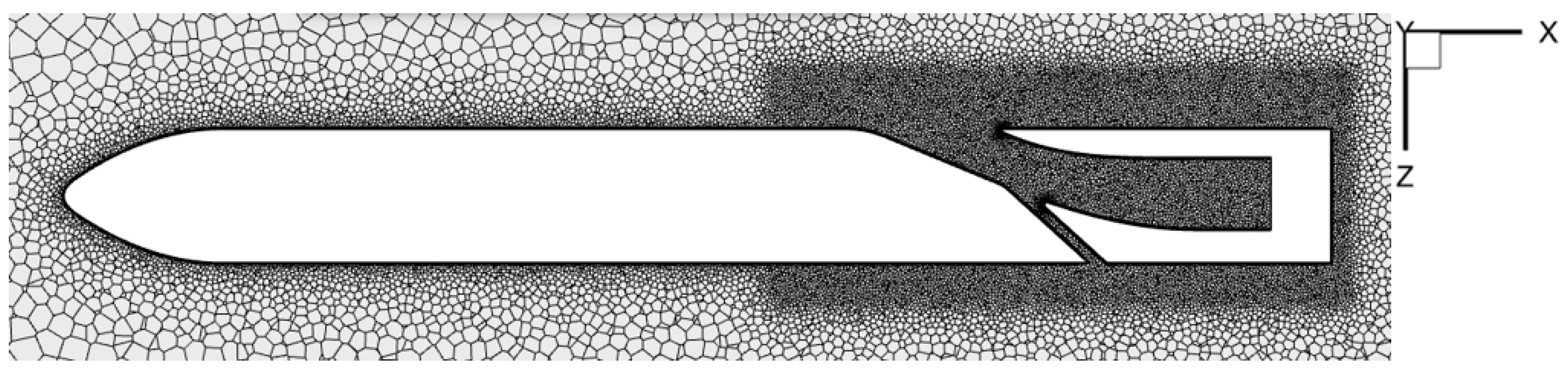
2.2.1. Calculation Method and Calculation Grid
2.2.2. Grid Independence Analysis
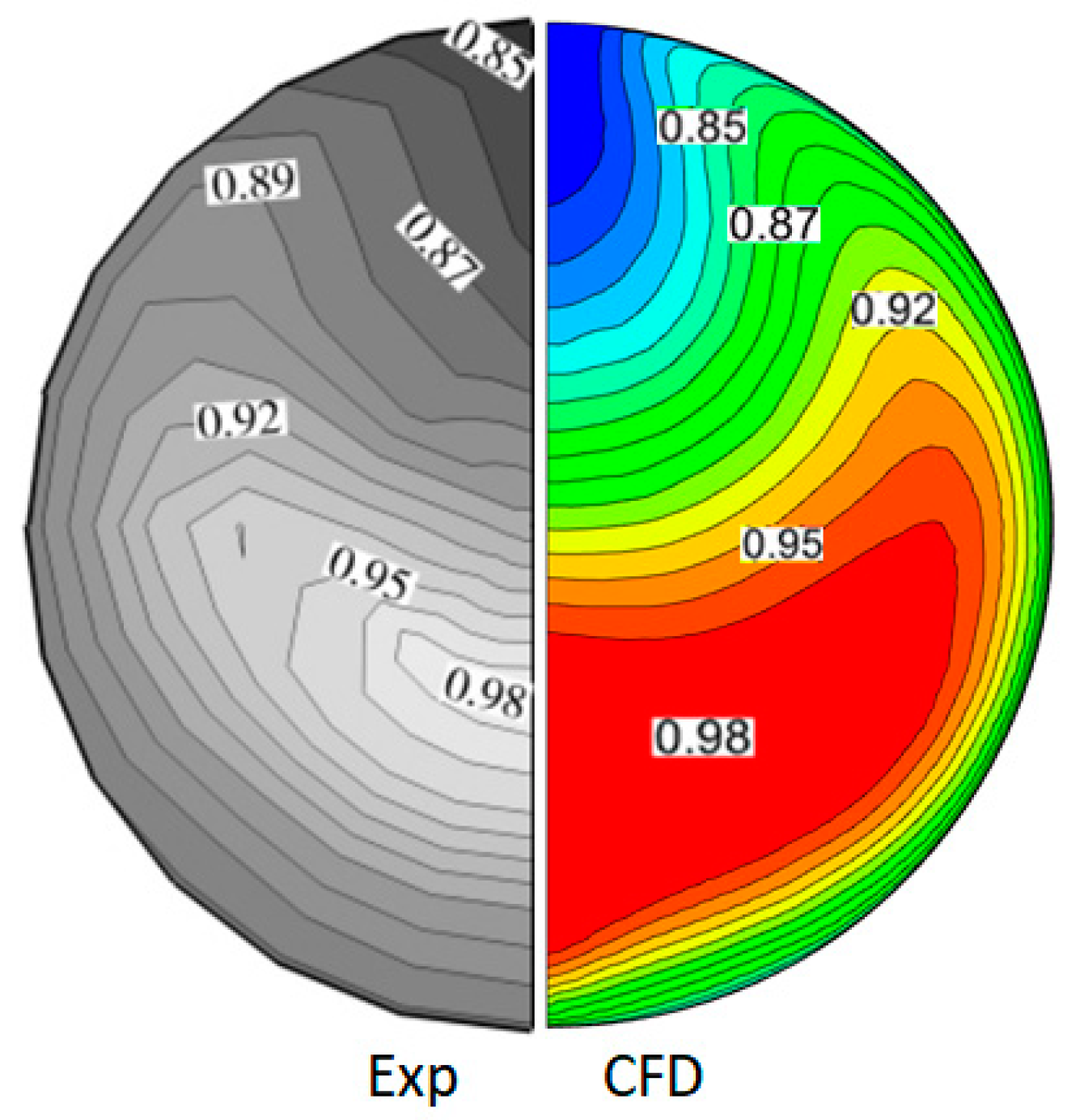
2.3. Example Verification
3. Simulation Analysis of Aerodynamic Characteristics of Baseline Configuration of Submerged Inlet
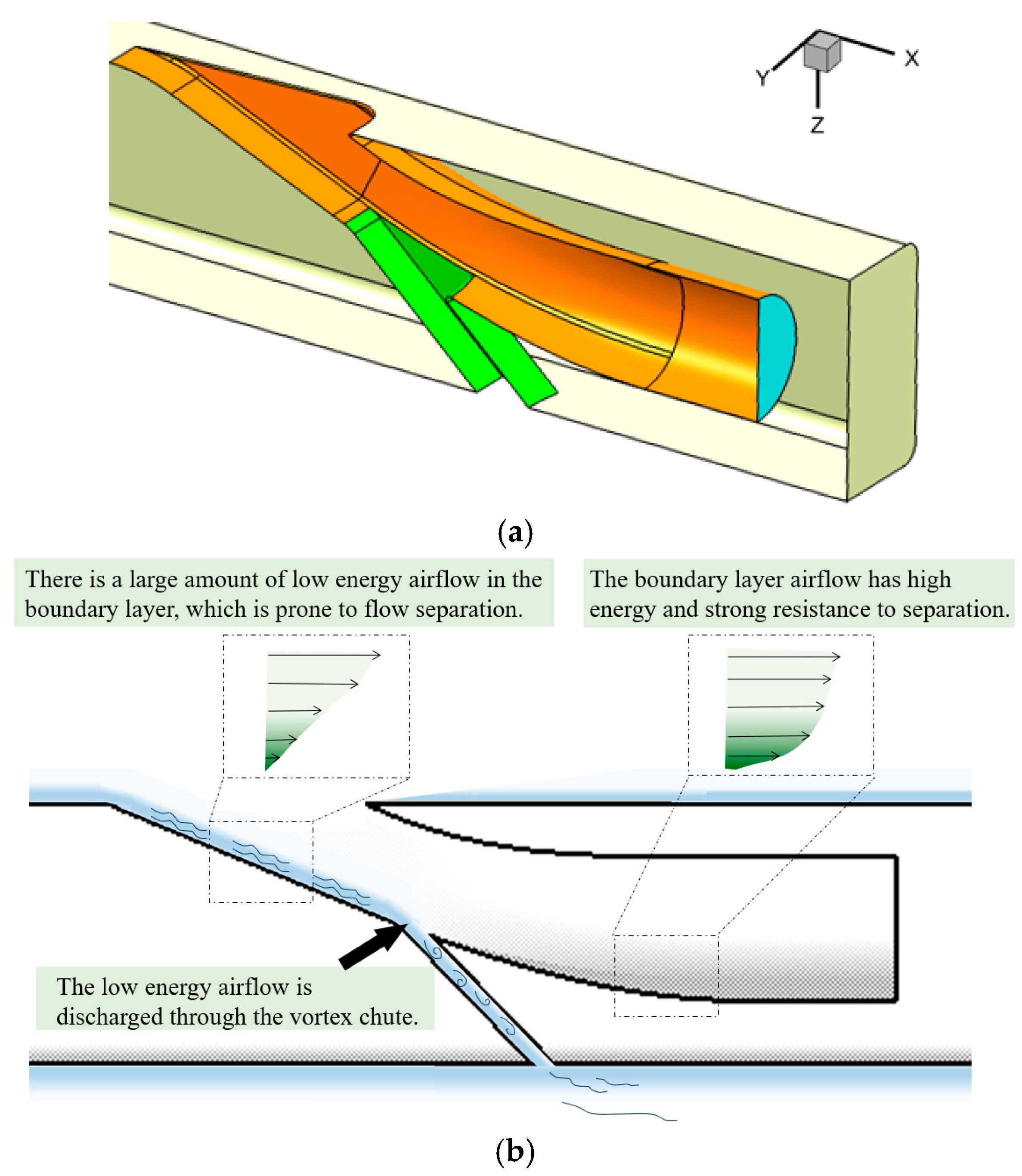
4. Vortex Diverter Design Method for Improving the Performance of Submerged Inlet
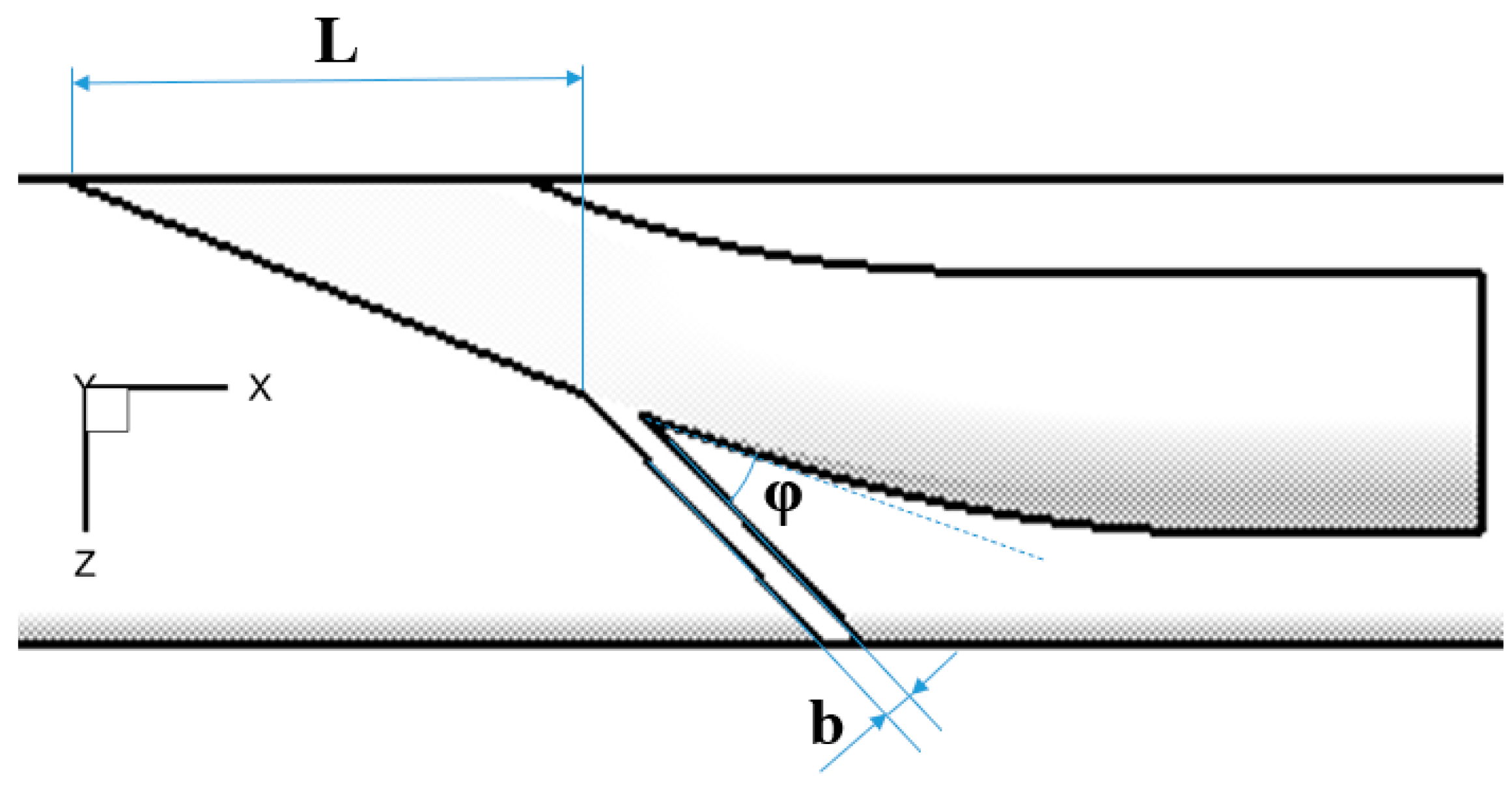
4.1. Design Principle
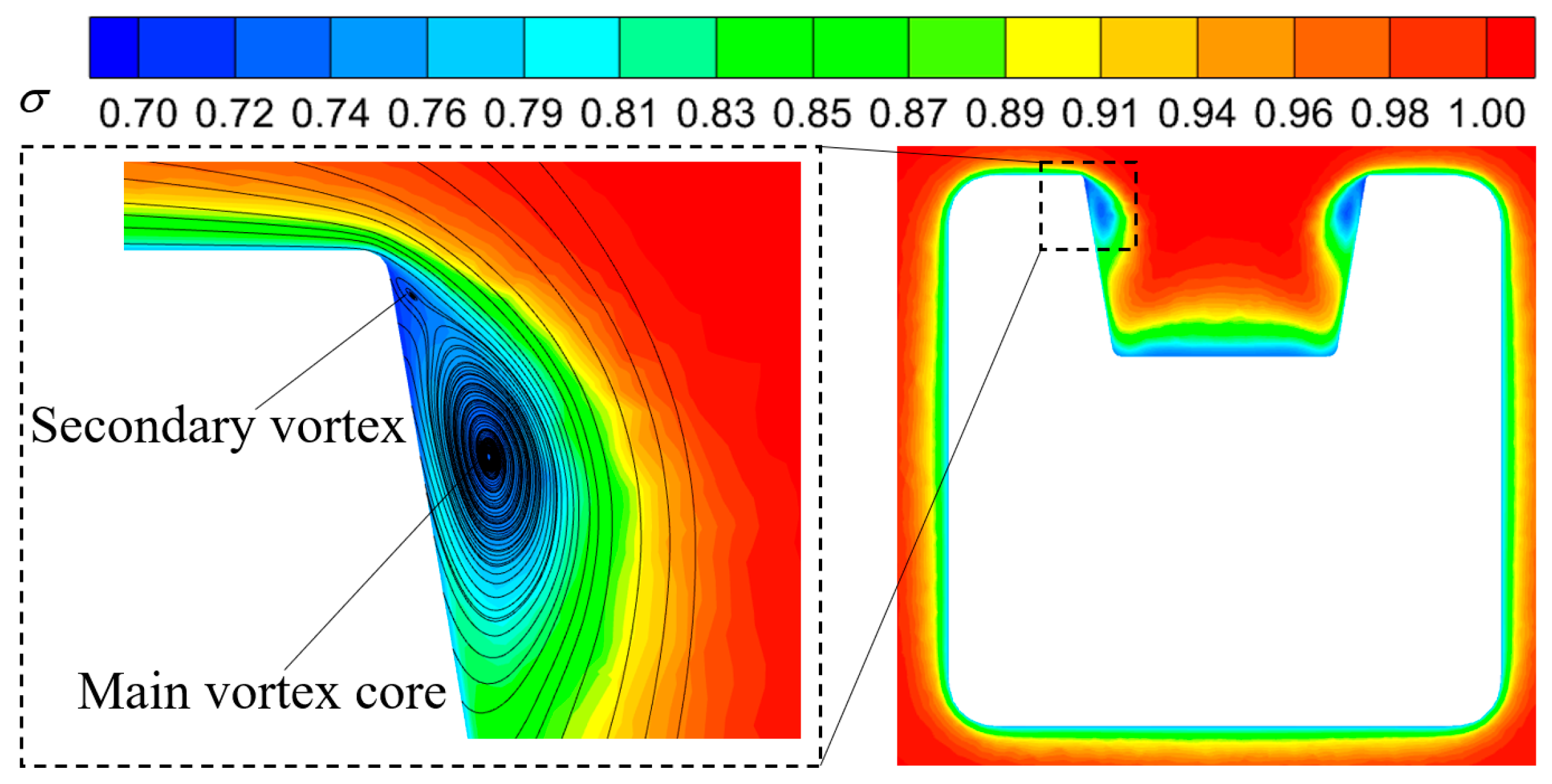
4.2. The Improvement Mechanism of Vortex Diverter on Submerged Inlet Performance
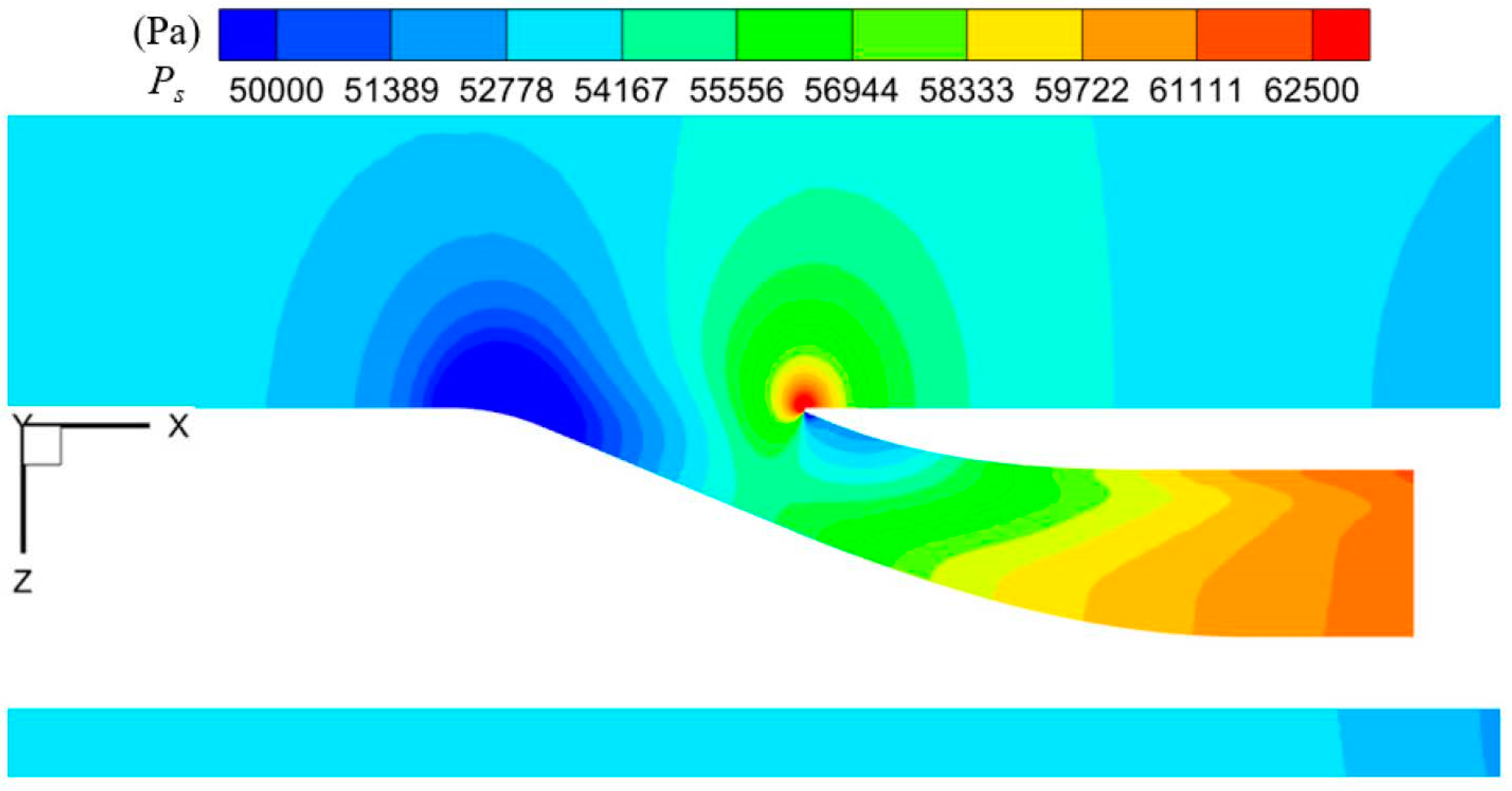
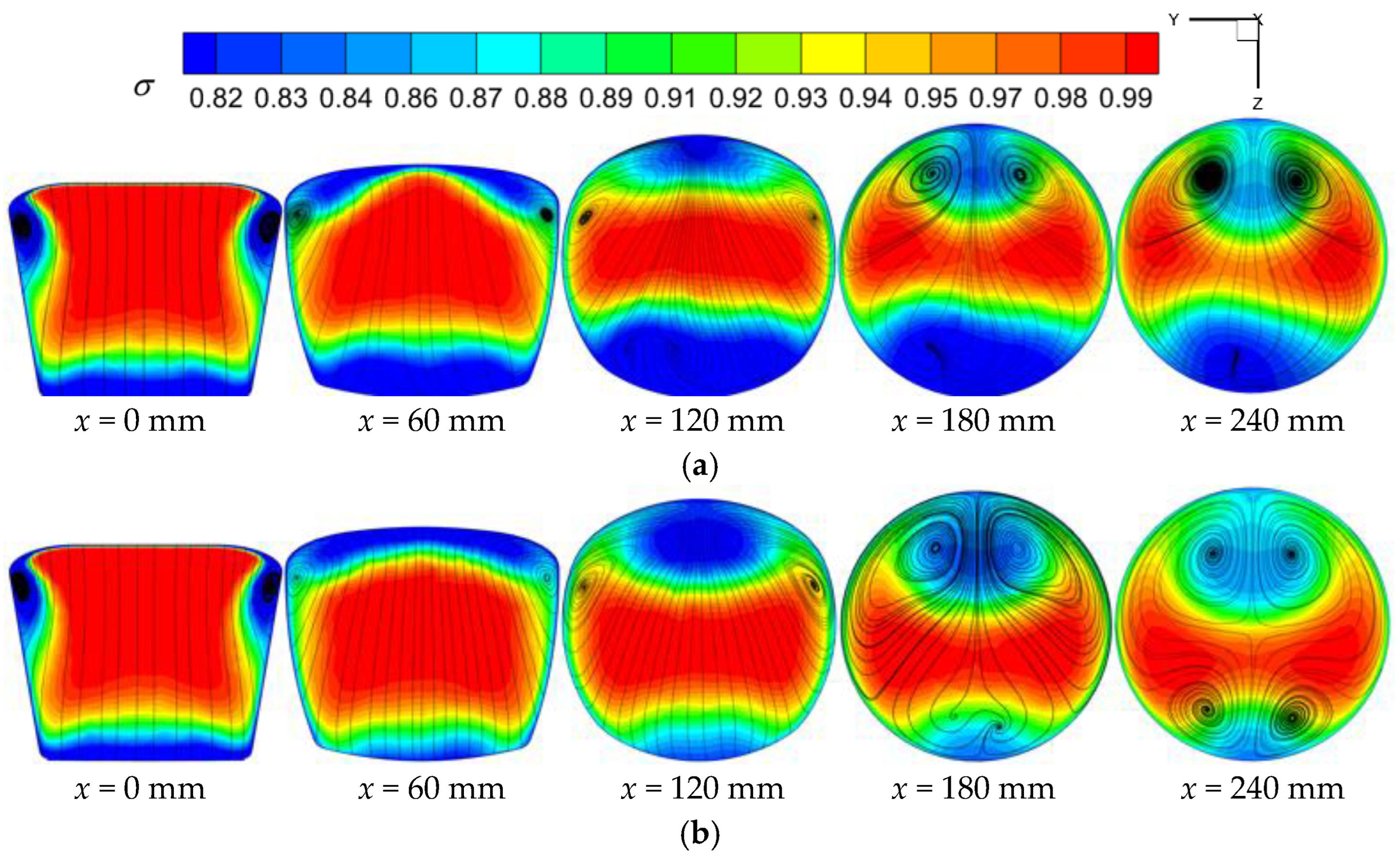
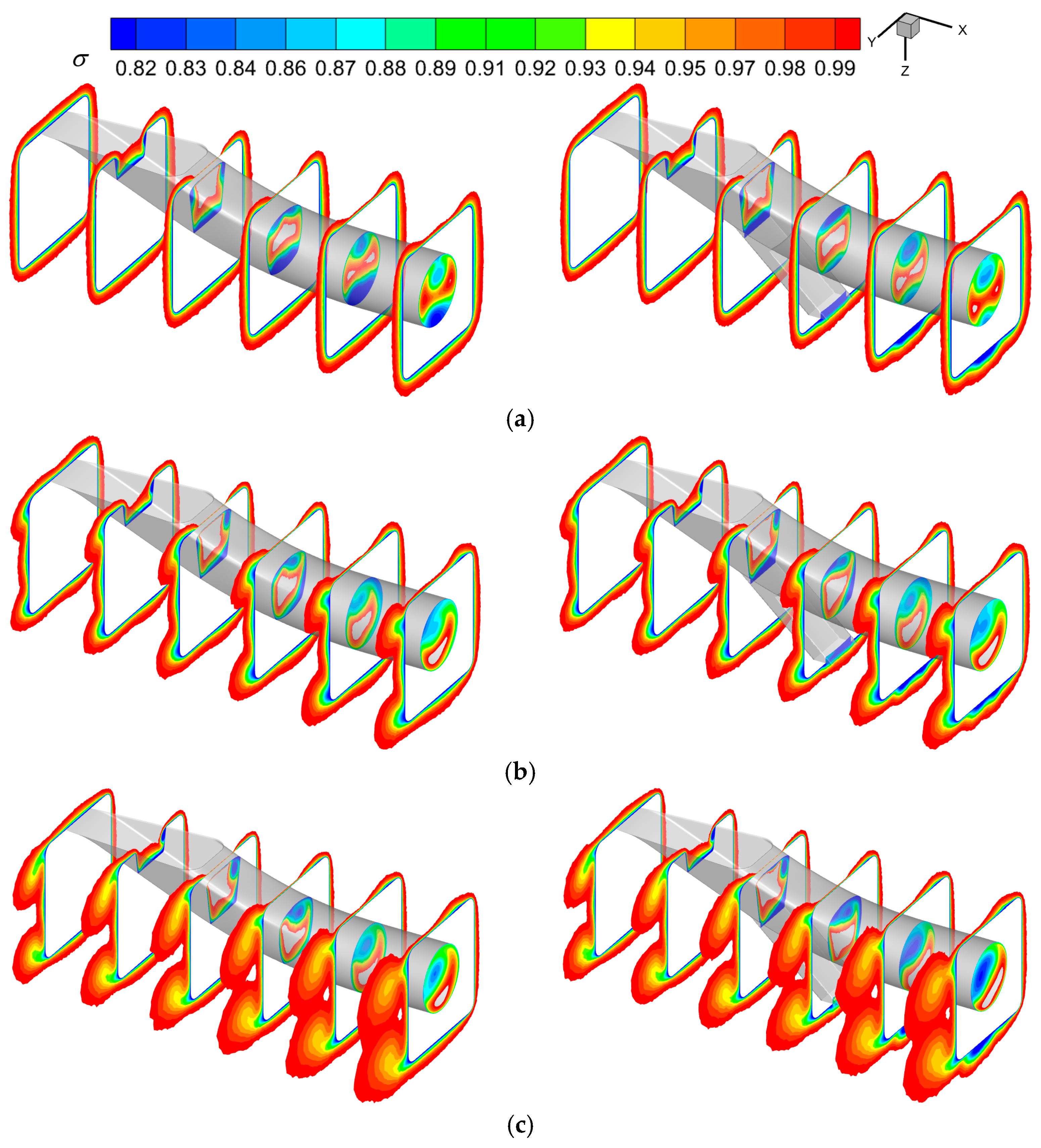
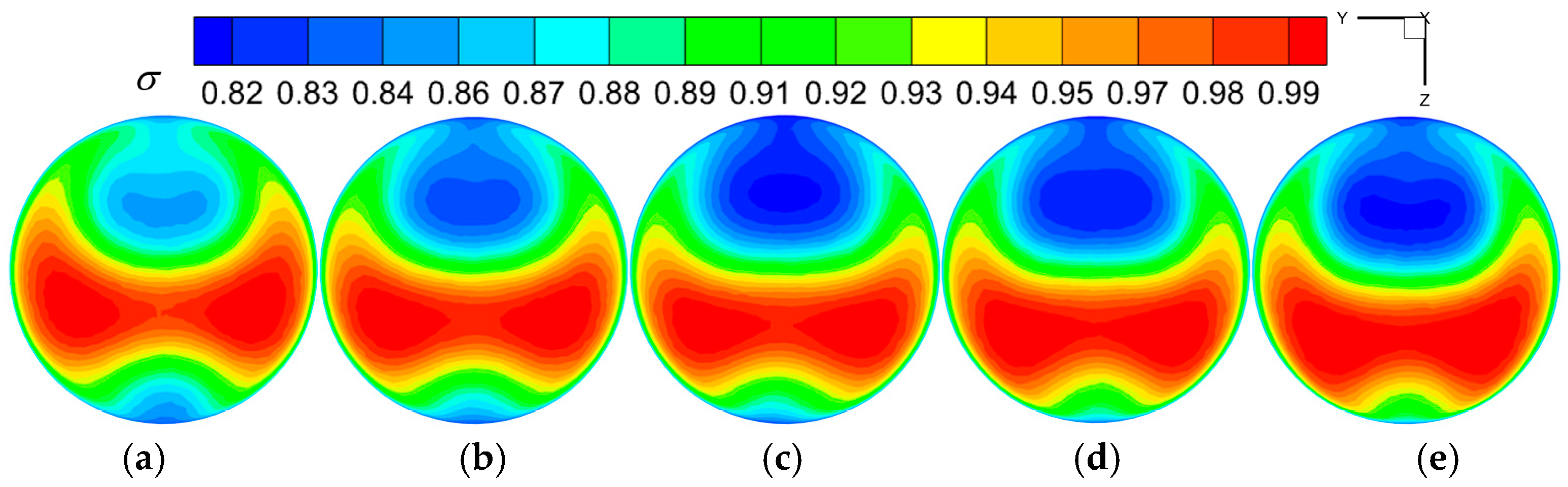
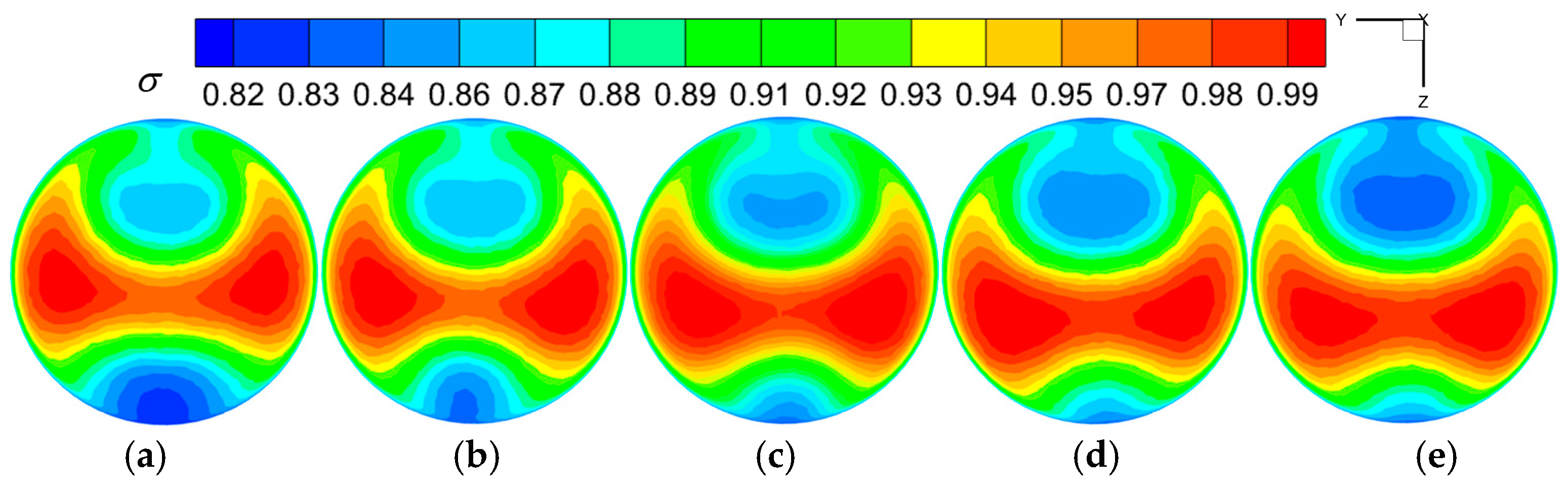
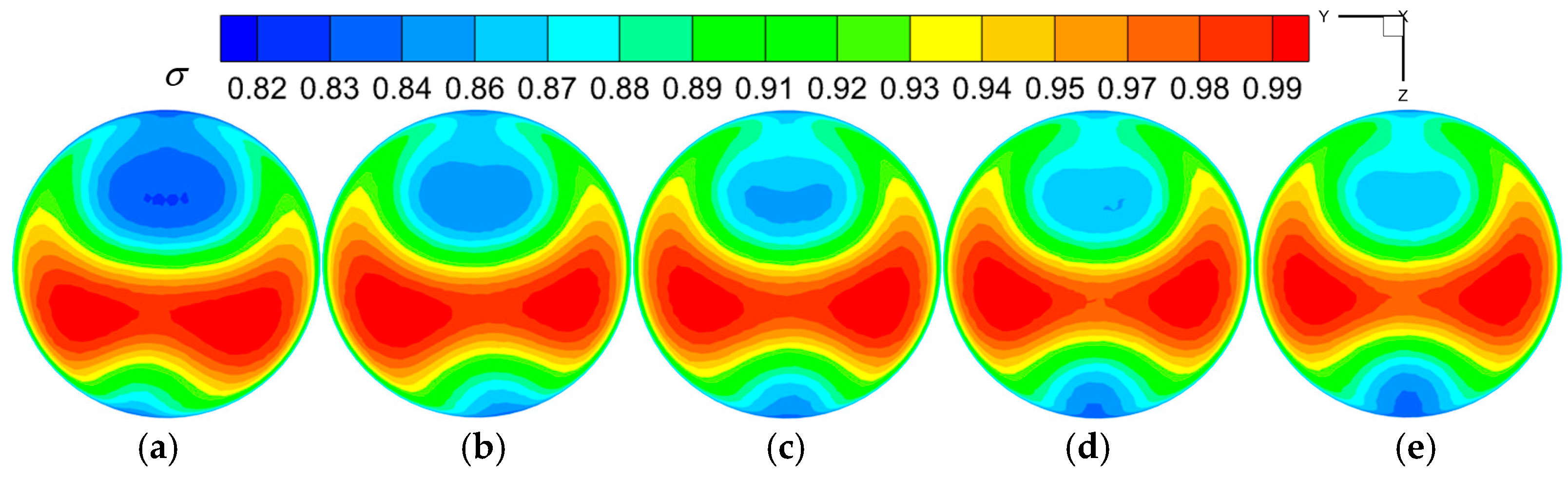
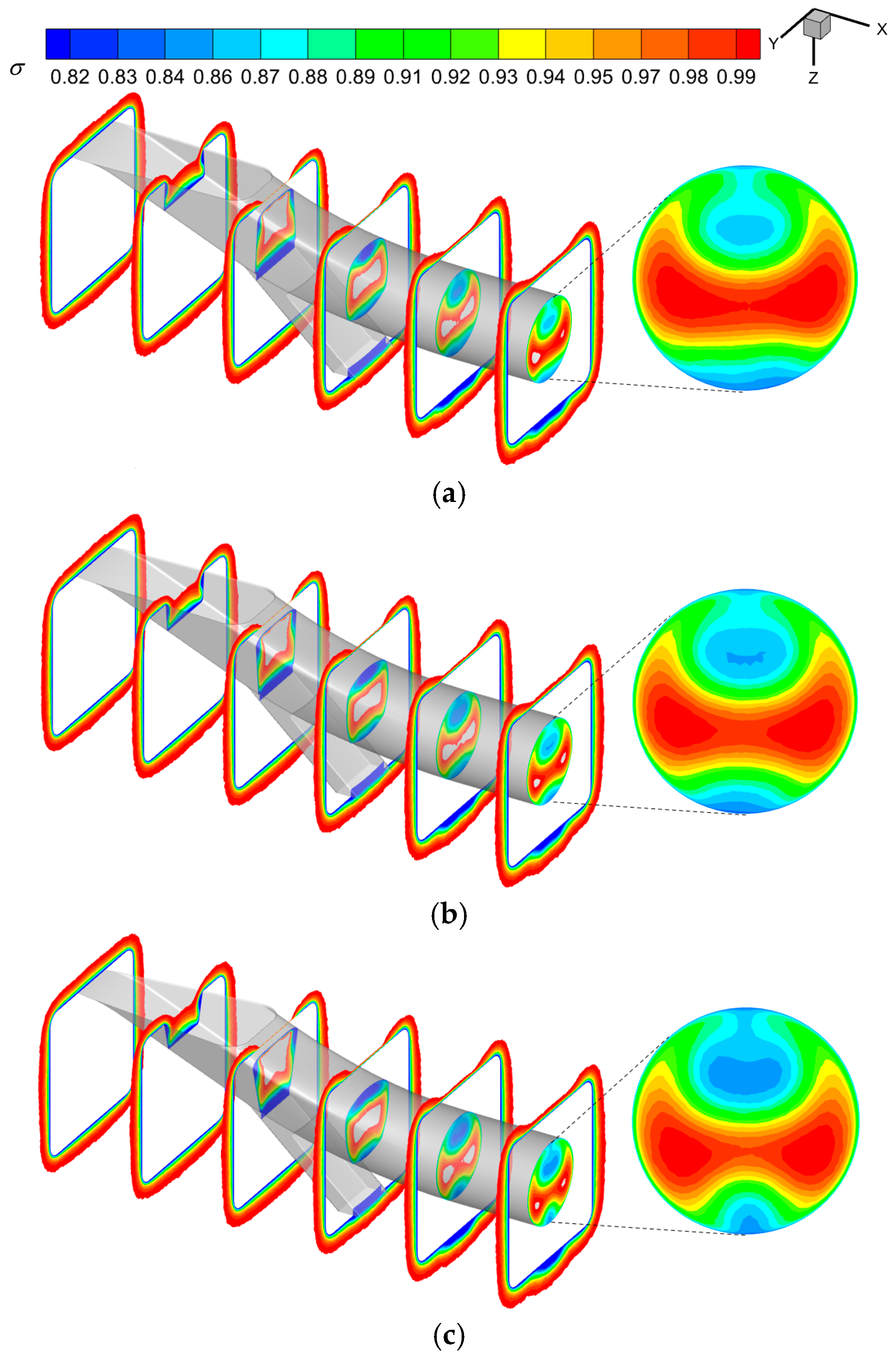
4.2.1. Distribution of Total Pressure Recovery Coefficient
- (1)
- Distribution of the total pressure recovery coefficient on the symmetry plane
- (2)
- Distribution of total pressure recovery coefficient on monitoring surface
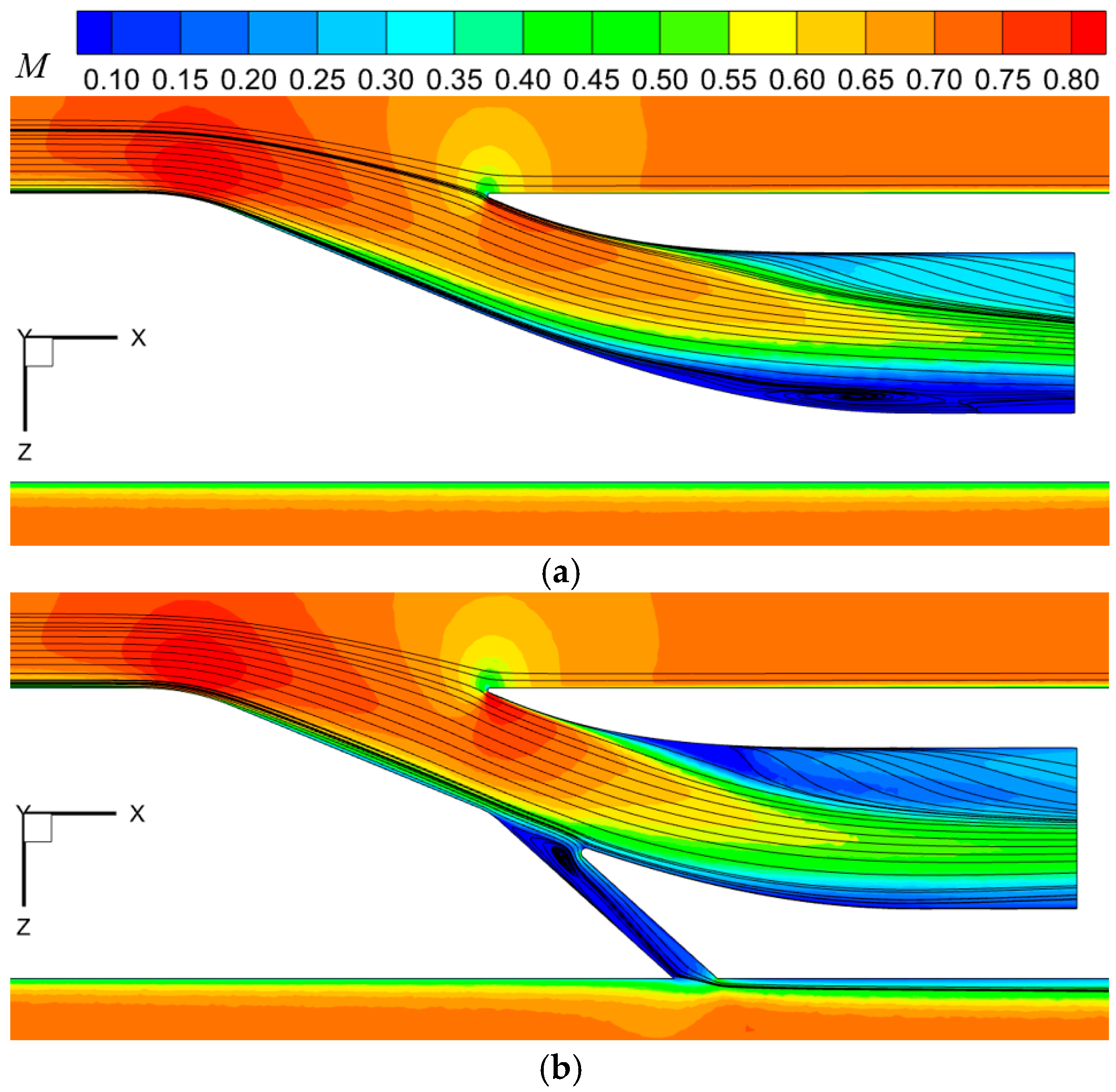
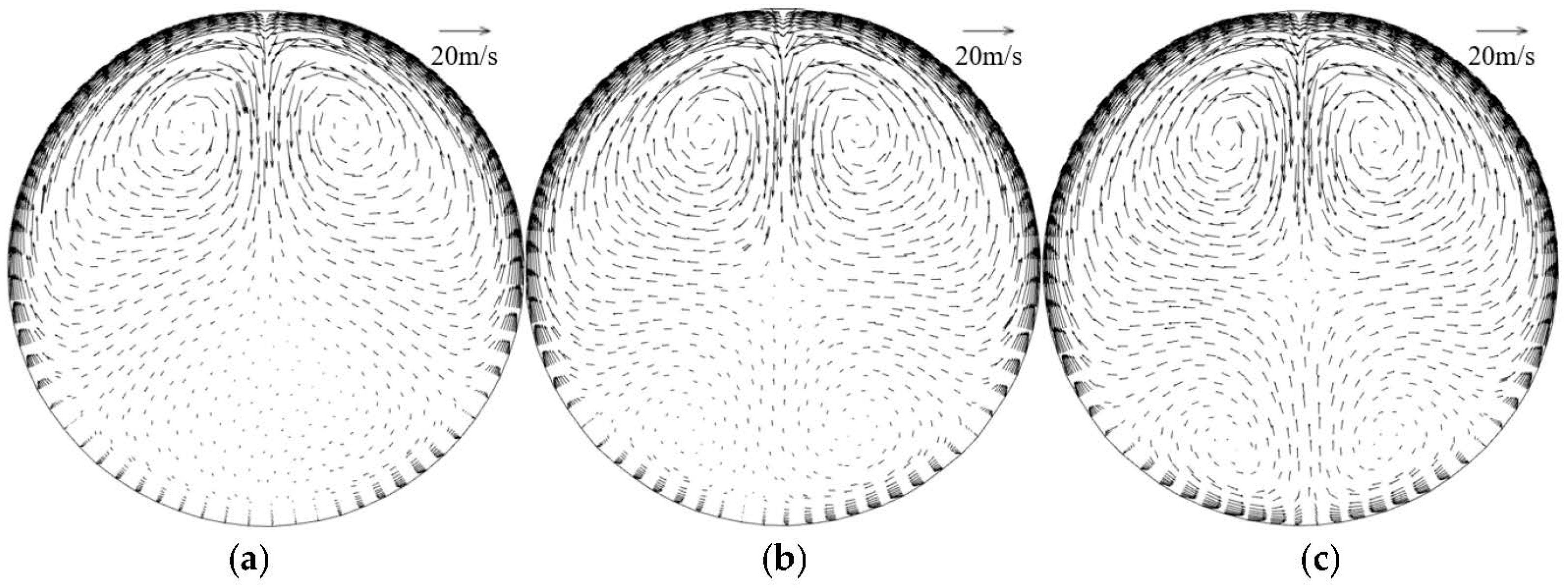
4.2.2. Mach Number Distribution
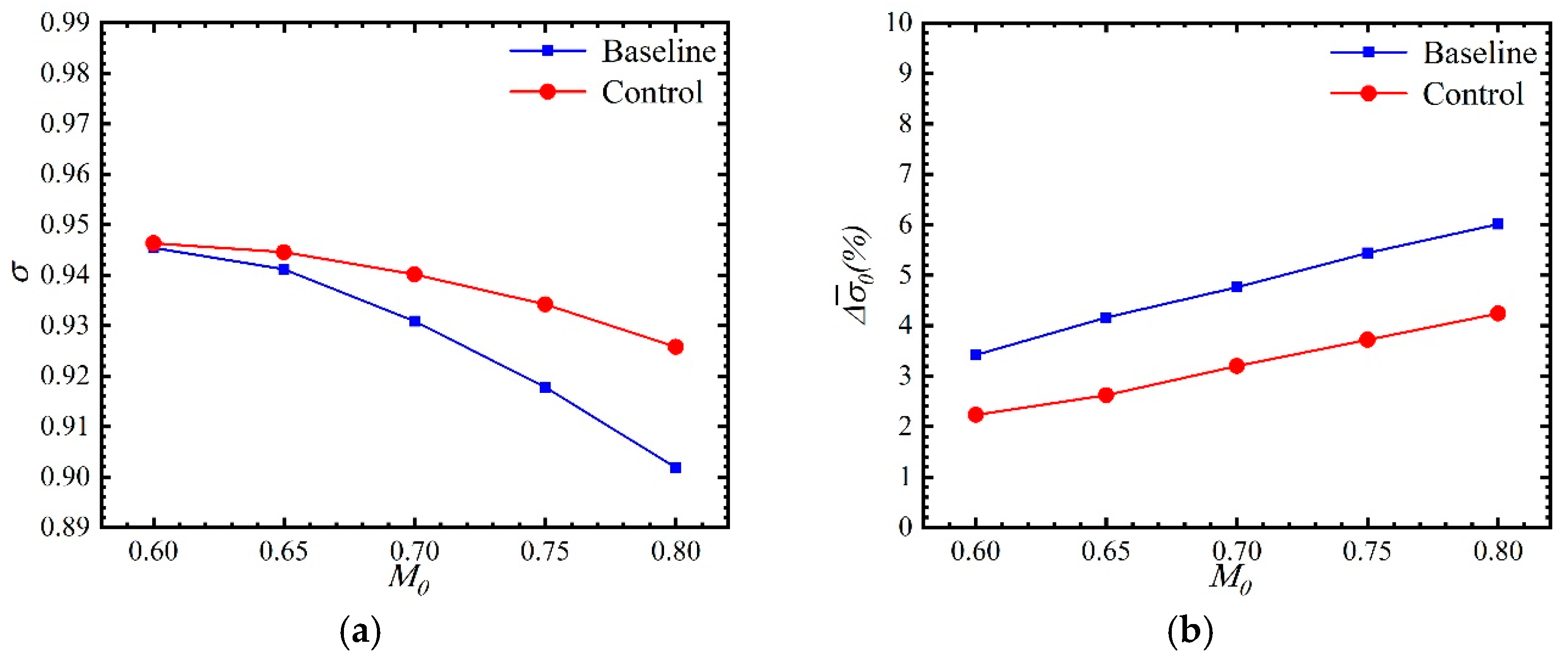
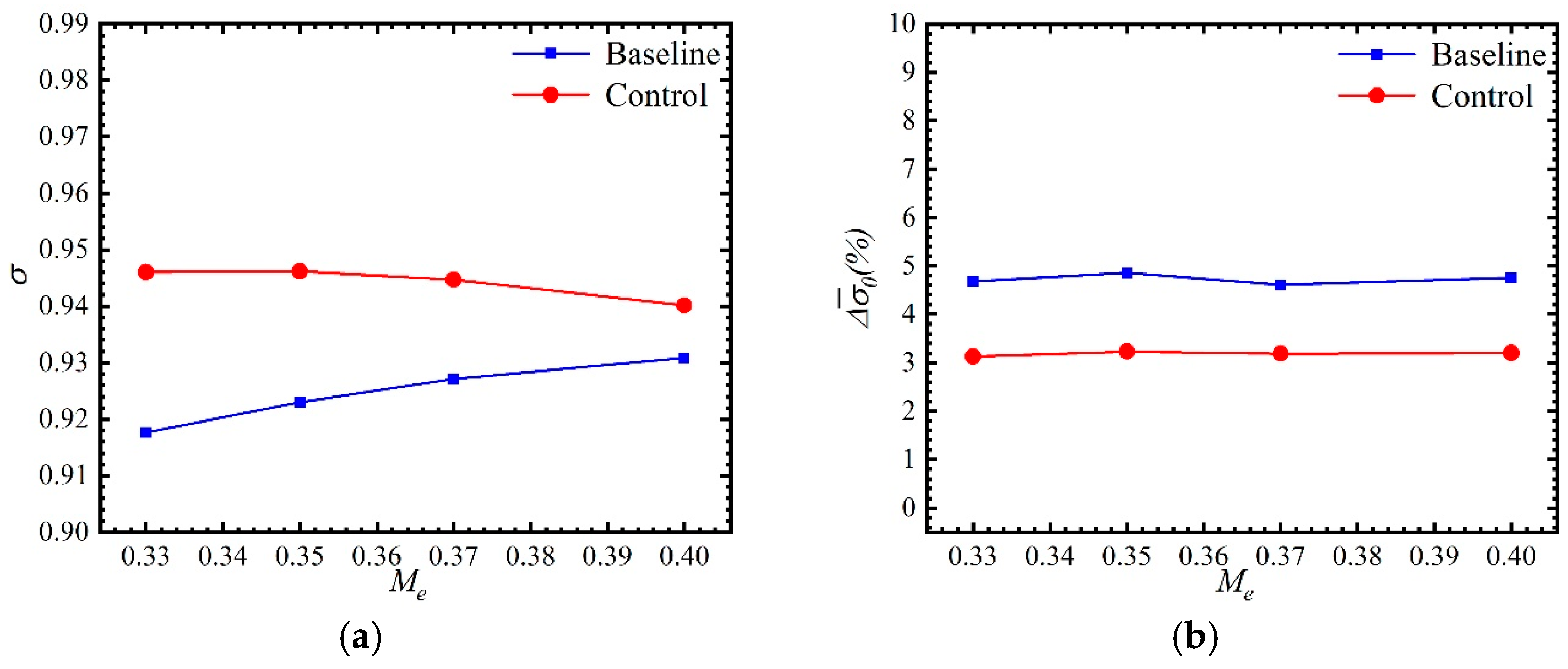
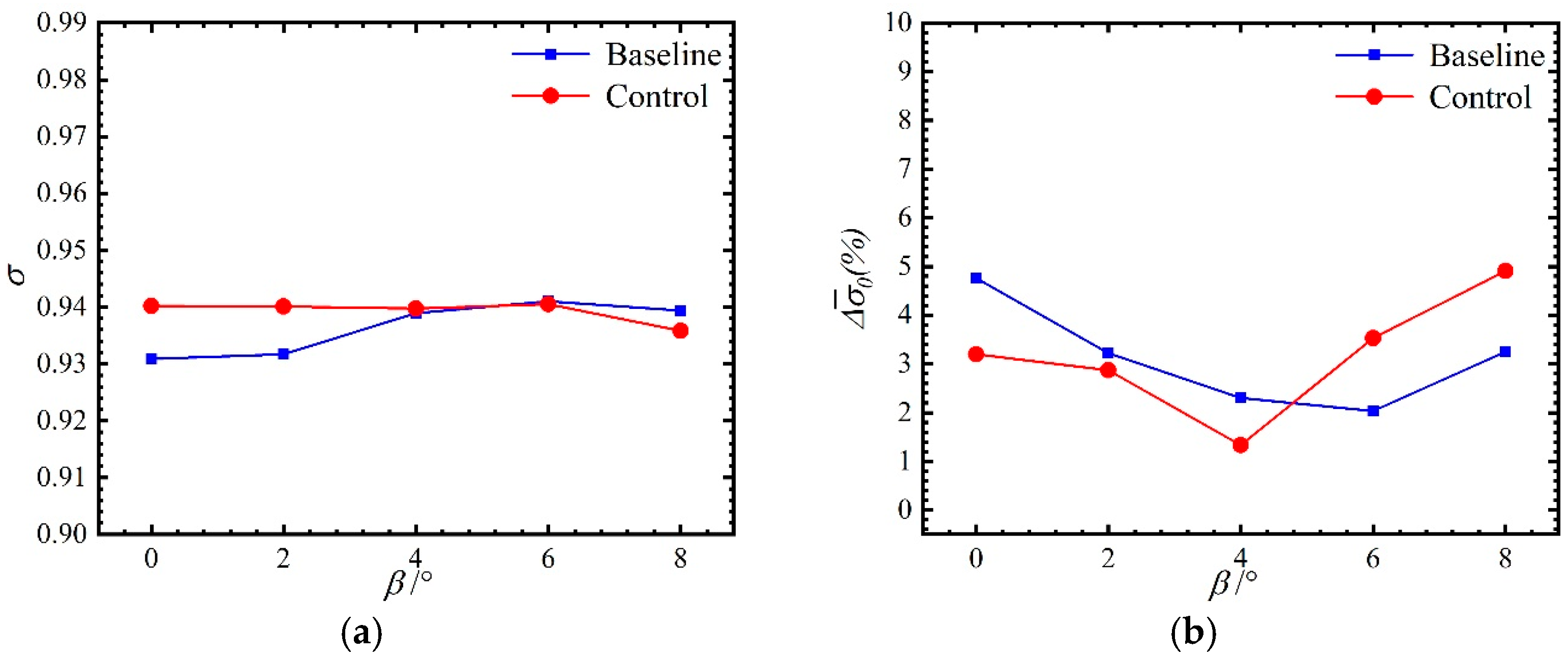
4.3. Study on the Adaptability of Vortex Diverter Design
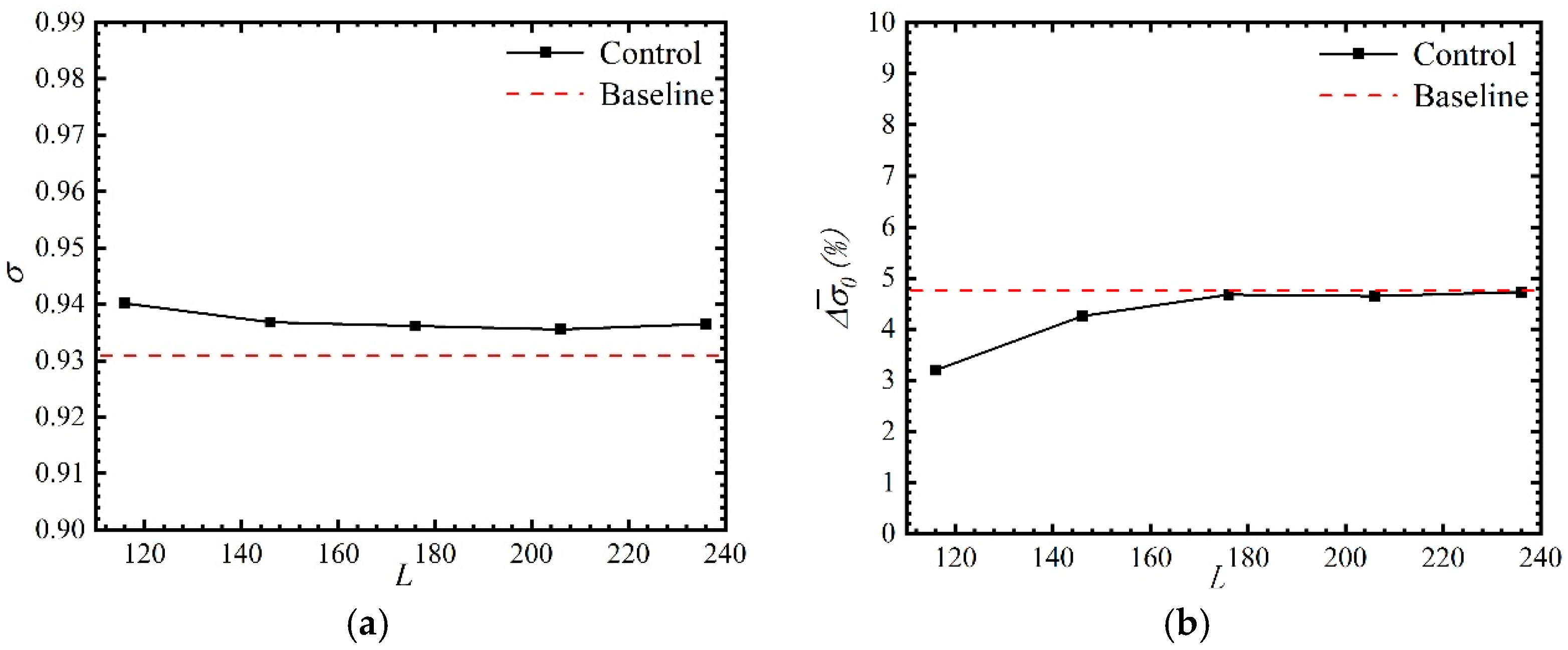
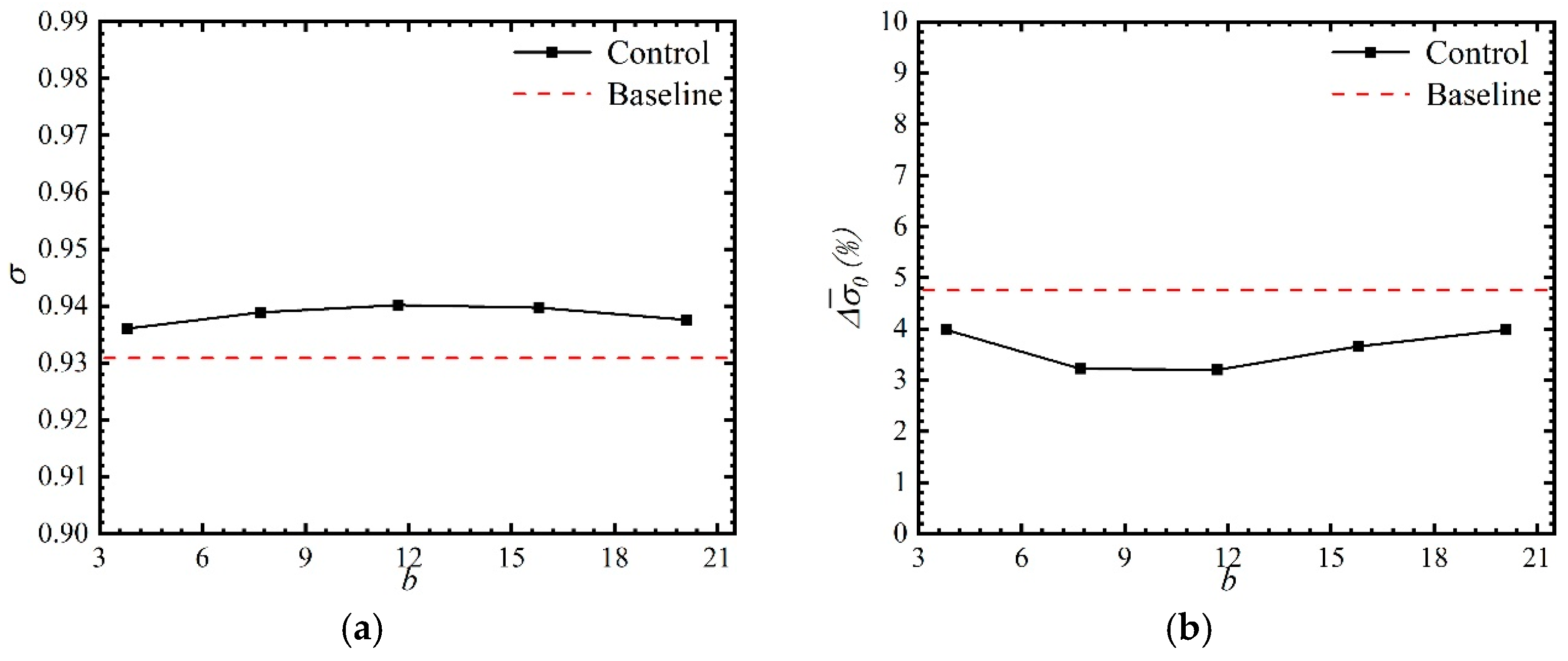
4.4. The Influence of Vortex Diverter Design Parameters on the Performance of the Submerged Inlet
4.5. The Matching Design of Vortex Diverter and Submerged Inlet
5. Conclusions
- (1)
- The vortex diverter exhausts the low-energy airflow on the side of the larger curvature radius of the inlet duct. As a result, the average kinetic energy of the airflow inside the inlet duct increases, the ability to resist separation strengthens, and the formation of separation vortices on the side of the larger curvature radius of the inlet duct is suppressed. This improves the airflow quality inside the submerged inlet and reduces the total pressure distortion. The maximum increase in the total pressure recovery coefficient is 3.1099%, and the maximum reduction in the circumferential total pressure distortion is 49.5207%.
- (2)
- The vortex diverter can enhance the intake performance of the submerged inlet under different flow Mach numbers, exit Mach numbers, and angles of attack, demonstrating its wide adaptability. However, when the sideslip angle is relatively large (greater than 8°), the performance of the control configuration is slightly inferior to that of the baseline configuration. This is because when the sideslip angle is too large, the low-energy airflow within the inlet is transferred from the bottom to the sidewalls of the duct. As a result, the efficiency of the vortex diverter in expelling low-energy airflow is reduced. However, considering that the sideslip angle variation is relatively small during the cruising of supersonic cruise missiles, it can be concluded that the vortex diverter exhibits good adaptability.
- (3)
- The vortex diverter has a relatively stable effect on improving the inlet performance of the submerged inlet. Among the key parameters of the vortex diverter: the distance L between the leading edge of the vortex diverter and the leading edge of the inlet determines the exhaust location of the vortex diverter; the thickness b of the vortex diverter determines the maximum exhaust flow rate of the vortex diverter; and the angle between the vortex diverter and the sidewall of the larger radius of curvature of the inlet determines the pressure gradient at the leading edge of the vortex diverter. Among these parameters, the distance L is the most influential on performance. The calculation results indicate that the installation of the vortex diverter near the throat region yields the optimal improvement in inlet performance.
- (4)
- The computational results indicate the presence of two distinct low-pressure regions at the exit of the baseline configuration. These low-pressure regions are caused by the accumulation of the boundary layer and the entrained vortices generated by the side edges. The vortex diverter can effectively expel the low-energy airflow from the accumulation of the boundary layer. Furthermore, by installing the vortex diverter and appropriately decreasing the side-edge angle at the entrance of the inlet, the intensity of the entrained vortices generated by the side edges can be significantly reduced, thereby improving the total pressure distribution at the exit of the inlet. Therefore, combining the installation of the vortex diverter with adjustments to the side-edge angle provides a more effective approach to enhancing the inlet performance.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| AIP | aerodynamic interface plane |
| b | the thickness of the vortex diverter, mm |
| D | exit diameter of inlet duct, mm |
| L | the distance from the leading edge of the vortex diverter to the front lip of the inlet, mm |
| M0 | freestream Mach number |
| Me | Mach number at the exit |
| P0 | the total pressure of the freestream flow, Pa |
| PAIP | mass-averaged total pressure over the aerodynamic interface plane, Pa |
| angle of attack, ° | |
| angle of sideslip, ° | |
| angle of side edge, ° | |
| total pressure recovery coefficient | |
| the total pressure recovery coefficient of the low-pressure region | |
| the circumferential total pressure distortion | |
| the angle between the vortex diverter and the wall with a larger curvature radius of the inlet on the symmetry plane, ° |
References
- Jia, G.W.; Yin, P.; Shao, S.; Wang, J. Review of RCS measurement and imaging methods of stealth aircraft. J. Natl. Univ. Def. Technol. 2022, 44, 93–103. [Google Scholar]
- Zheng, R.S.; Qi, K.N.; Zhang, Q.B.; Xiao, Z.; Liu, H.J. Integrated Investigation of Aerodynamic Shape and Stealth Performance for Supersonic Vehicle with “X” Sawtooth Lip Inlet. J. Propuls. Technol. 2017, 38, 9. [Google Scholar] [CrossRef]
- Yu, X.H.; Zhao, Y.M. A Study on Stealth Technology in UAV Inlet Design. Flight Dyn. 2007, 4, 69–72. [Google Scholar]
- Shi, L.; Guo, R. Electromagnetic Scattering of a Submerged Inlet. Acta Aeronaut. Astronaut. Sin. 2008, 29, 1098–1104. [Google Scholar]
- Frick, C.W.; Davis, W.F.; Randall, L.M.; Mossman, E.A. An Experimental Investigation of NACA Submerged Duct Entrances; Technical Report Archive & Image Library: Washington, DC, USA, 1945. [Google Scholar]
- Taylor, R.A. Some Effects of Side-Wall Modifications on the Drag and Pressure Recovery of an NACA Submerged Inlet at Transonic Speeds; Technical Report Archive & Image Library: Washington, DC, USA, 1952. [Google Scholar]
- Hall, C.F.; Frank, J.L. Ram-Recovery Characteristics of NACA Submerged Inlets at High Subsonic Speeds; Technical Report Archive & Image Library: Washington, DC, USA, 1948. [Google Scholar]
- Axelson, J.A.; Taylor, R.A. Preliminary Investigation of the Transonic Characteristics of an NACA Submerged Inlet; Technical Report Archive & Image Library: Washington, DC, USA, 1950. [Google Scholar]
- Mossman, E.A.; Randall, L.M. An Experimental Investigation of the Design Variables for NACA Submerged Duct Entrances; Technical Report Archive & Image Library: Washington, DC, USA, 1948. [Google Scholar] [CrossRef]
- Frank, J.L. Pressure-Distribution and Ram-Recovery Characteristics of NACA Submerged Inlets at High Subsonic Speeds; Technical Report Archive & Image Library: Washington, DC, USA, 1950. [Google Scholar]
- Anderson, W.E.; Frazer, A.C. Investigation of an NACA Submerged Inlet at Mach Numbers from 1.17 to 1.99; Technical Report Archive & Image Library: Washington, DC, USA, 1952. [Google Scholar]
- Sun, S.; Guo, R.R. Numerical Analysis and Experimental Validation of a Submerged Inlet on the Plane Surface. Chin. J. Aeronaut. 2005, 18, 199–205. [Google Scholar] [CrossRef]
- Sun, S.; Guo, R.R.; Wu, Y.Z. Characterization and Performance Enhancement of Submerged Inlet with Flush-Mounted Planar Side Entrance. J. Propuls. Power 1971, 23, 987–995. [Google Scholar] [CrossRef]
- Wang, Y.G.; Wang, C.H.; Xiao, Y.C.; Chen, B.; Zhou, S.; Guo, J.; Sun, M. Construction methodology for lip surface of a submerged inlet. Aerosp. Sci. Technol. 2016, 54, 340–352. [Google Scholar] [CrossRef]
- Huang, H.X. Recent Progress in Subsonic S-Shaped Inlets. J. Propuls. Technol. 2020, 41, 2641. [Google Scholar] [CrossRef]
- Harrison, N.A.; Anderson, J.; Fleming, J.L.; Ng, W.F. Active Flow Control of a Boundary Layer-Ingesting Serpentine Inlet Diffuser. J. Aircr. 2013, 50, 262–271. [Google Scholar] [CrossRef]
- Yadav, K.R.; Paul, A.R.; Hegde, N.; Jain, A. A Comparison of Circular and Slotted Synthetic Jets for Flow Control in a Twin Air Intake. Def. Sci. J. 2020, 70, 113–121. [Google Scholar] [CrossRef]
- Cheng, D.S.; Sun, S.; Wen, Y.F.; Tan, H.J. Submerged inlet performance improvement with blowing on fuselage. J. Aerosp. Power 2012, 27, 1131–1138. [Google Scholar]
- Perez, C.C.; Ferreira, S.B.; Silva, L.F.F.D.; Jesus, A.B.D.; Oliveira, G.L. Computational Study of Submerged Air Inlet Performance Improvement Using Vortex Generators. J. Aircr. 2007, 44, 1574–1587. [Google Scholar] [CrossRef]
- Sun, S.; Tan, H.-J.; Wang, C.-X. Submerged Inlet Performance Enhancement Using a Unique Bump-Shaped Vortex Generator. J. Propuls. Power 2016. [Google Scholar] [CrossRef]
- Xie, W.Z.; Yang, S.Z.; Zeng, C.; Guo, S. Effects of forebody boundary layer on the performance of a submerged inlet. Aeronaut. J. 2021, 125, 1260–1281. [Google Scholar] [CrossRef]
- Saheby, E.B.; Shen, X.; Huang, G.; Hays, A.P. Flow structure of the ridge integrated submerged inlet. Aerosp. Sci. Technol. 2021. [Google Scholar] [CrossRef]
- Jirasek, A. Design of Vortex Generator Flow Control in Inlets. J. Aircr. 2006, 43, 1886–1892. [Google Scholar] [CrossRef]
- Gissen, A.N.; Vukasinovic, B.; Mcmillan, M.L.; Glezer, A. Distortion Management in a Boundary Layer Ingestion Inlet Diffuser Using Hybrid Flow Control. J. Propuls. Power 2014, 30, 834–844. [Google Scholar] [CrossRef]
- Cheng, D.S.; Tan, H.J.; Sun, S.; Tong, Y. Computational Study of a High-Performance Submerged Inlet with Bleeding Vortex. J. Aircr. 2012, 49, 852–860. [Google Scholar] [CrossRef]
- Xie, W.Z.; Zeng, C.; Wang, Z.Z.; Guo, S.M. Flow Control for a Submerged Inlet. J. Fluids Eng. 2022, 144, 121202. [Google Scholar] [CrossRef]
- Akkermans, R.A.D.; Cieslik, A.R.; Kamp, L.P.J.; Trieling, R.R.; Clercx, H.J.H.; van-Heijst, G.J.F. The three-dimensional structure of an electromagnetically generated dipolar vortex in a shallow fluid layer. J. Phys. Fluids 2008, 20, 116601. [Google Scholar] [CrossRef]
- Duran-matute, M.; Kamp, L.P.J.; Trieling, R.R.; van-Heijst, G.J.F. Scaling of decaying shallow axisymmetric swirl flows. J. Fluid Mech. 2010, 648, 471. [Google Scholar] [CrossRef]
- Duran-Matute, M.; Albagnac, J.; Kamp, L.P.J.; van-Heijst, G.J.F. Dynamics and structure of decaying shallow dipolar vortices. J. Phys. Fluids 2010, 22, 116606. [Google Scholar] [CrossRef]
- Alfonsi, G. Reynolds-Averaged Navier–Stokes Equations for Turbulence Modeling. Appl Mech. Rev. 2009, 62, 933–944. [Google Scholar] [CrossRef]
- Deck, S.; Duveau, P.L.; D’Espiney, P. Development and application of Spalart—Allmaras one equation turbulence model to three-dimensional supersonic complex configurations. Aerosp. Sci. Technol. 2002, 6, 171–183. [Google Scholar] [CrossRef]
- Zhang, J.Y.; Zhan, H.; Mi, B.G. Study on Aerodynamic Design of the Front Auxiliary Inlet. Aerospace 2023, 10, 700. [Google Scholar] [CrossRef]
- Bai, X.; Mi, B.G. Prepositive Synergistic Bulge Design for Improving Aerodynamic Performance of Submerged Inlet. Aerospace 2023, 10, 649. [Google Scholar] [CrossRef]
- Zhang, J.Y.; Mi, B.G. Internal Aerodynamic Performance Enhancement for Aircraft with High Maneuver by Designing a Distributed Submerged Inlet. Appl. Sci. 2023, 13, 1459. [Google Scholar] [CrossRef]





| Grid Scheme | Number of Grids (Millions) | PAIP (Pa) | Relative Error (%) |
|---|---|---|---|
| 1 | 0.9 | 69,742.10 | 0.18 |
| 2 | 1.3 | 69,773.08 | 0.13 |
| 3 | 1.8 | 69,824.22 | 0.06 |
| 4 | 5.0 | 69,869.53 | / |
| Me | Pressure Recovery Coefficient Total (%) | Relative Error (%) | |
|---|---|---|---|
| Experimental Results [12] | Calculation Results | ||
| 0.33 | 90.37 | 91.25 | 0.97 |
| 0.35 | 91.04 | 91.45 | 0.45 |
| 0.37 | 91.62 | 91.73 | 0.12 |
| 0.40 | 92.20 | 92.44 | 0.26 |
| Design Parameter | Value 1 | Value 2 | Value 3 | Value 4 | Value 5 |
|---|---|---|---|---|---|
| L (mm) | 115.70 | 145.70 | 175.70 | 205.70 | 235.70 |
| b (mm) | 5.65 | 11.45 | 17.43 | 23.58 | 29.93 |
| (°) | 10 | 15 | 20 | 25 | 30 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Zhan, H.; Mi, B. Numerical Investigation of a Vortex Diverter Designed for Improving the Performance of the Submerged Inlet. Aerospace 2024, 11, 42. https://doi.org/10.3390/aerospace11010042
Zhang J, Zhan H, Mi B. Numerical Investigation of a Vortex Diverter Designed for Improving the Performance of the Submerged Inlet. Aerospace. 2024; 11(1):42. https://doi.org/10.3390/aerospace11010042
Chicago/Turabian StyleZhang, Junyao, Hao Zhan, and Baigang Mi. 2024. "Numerical Investigation of a Vortex Diverter Designed for Improving the Performance of the Submerged Inlet" Aerospace 11, no. 1: 42. https://doi.org/10.3390/aerospace11010042
APA StyleZhang, J., Zhan, H., & Mi, B. (2024). Numerical Investigation of a Vortex Diverter Designed for Improving the Performance of the Submerged Inlet. Aerospace, 11(1), 42. https://doi.org/10.3390/aerospace11010042





