Low-Thrust Transfer to Quasi-Synchronous Martian Elliptic Orbit via Nonlinear Feedback Control
Abstract
1. Introduction
2. Orbit Dynamics
2.1. Modified Equinoctial Elements
2.2. Orbit Perturbations
- (a)
- harmonics of the areopotential, with coefficient (i.e., , , , and ),
- (b)
- third body gravitational pull due to the Sun, and
- (c)
- solar radiation pressure.
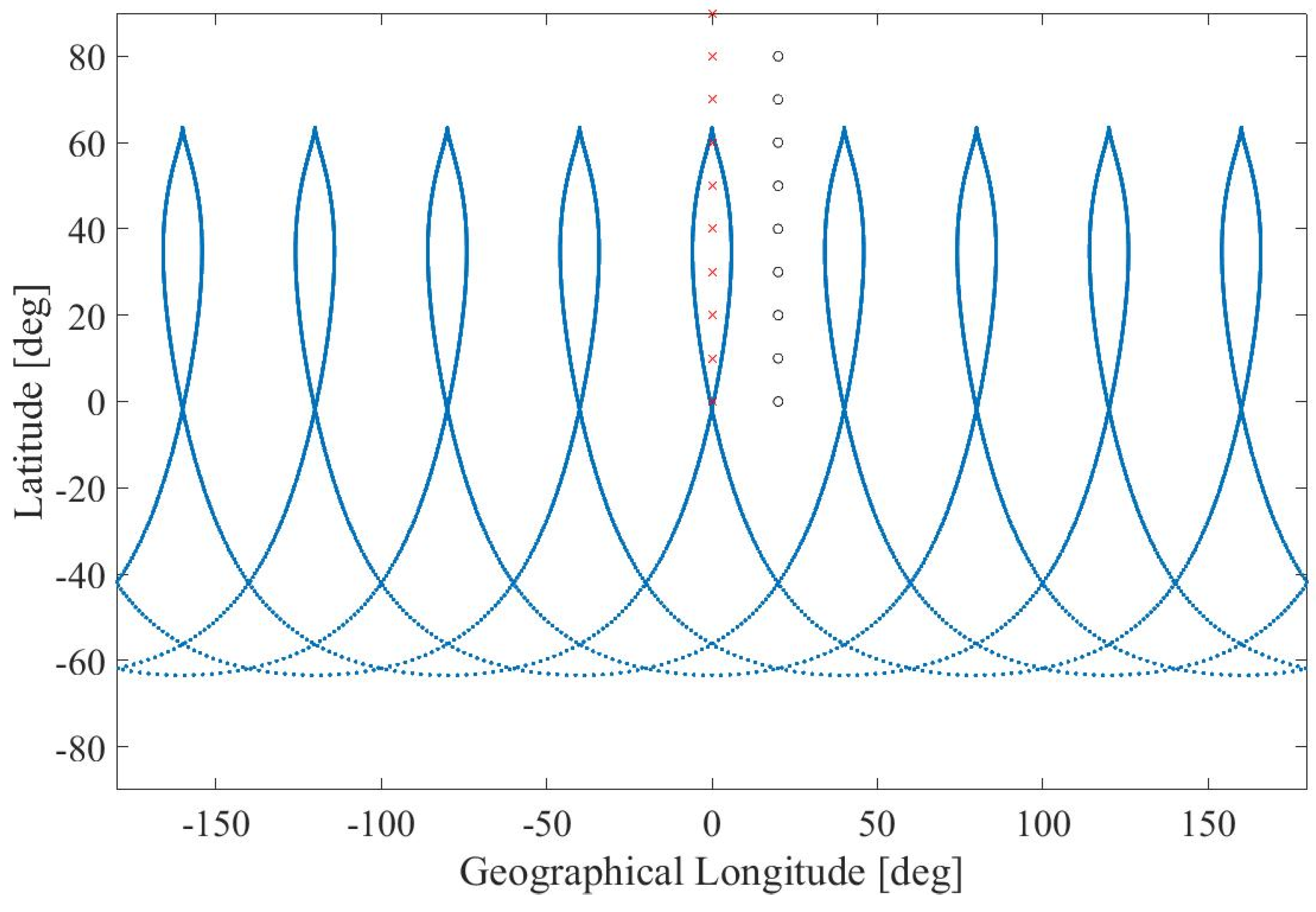
2.3. Operational Orbit and Coverage Analysis
3. Nonlinear Orbit Control
3.1. Operational Orbit
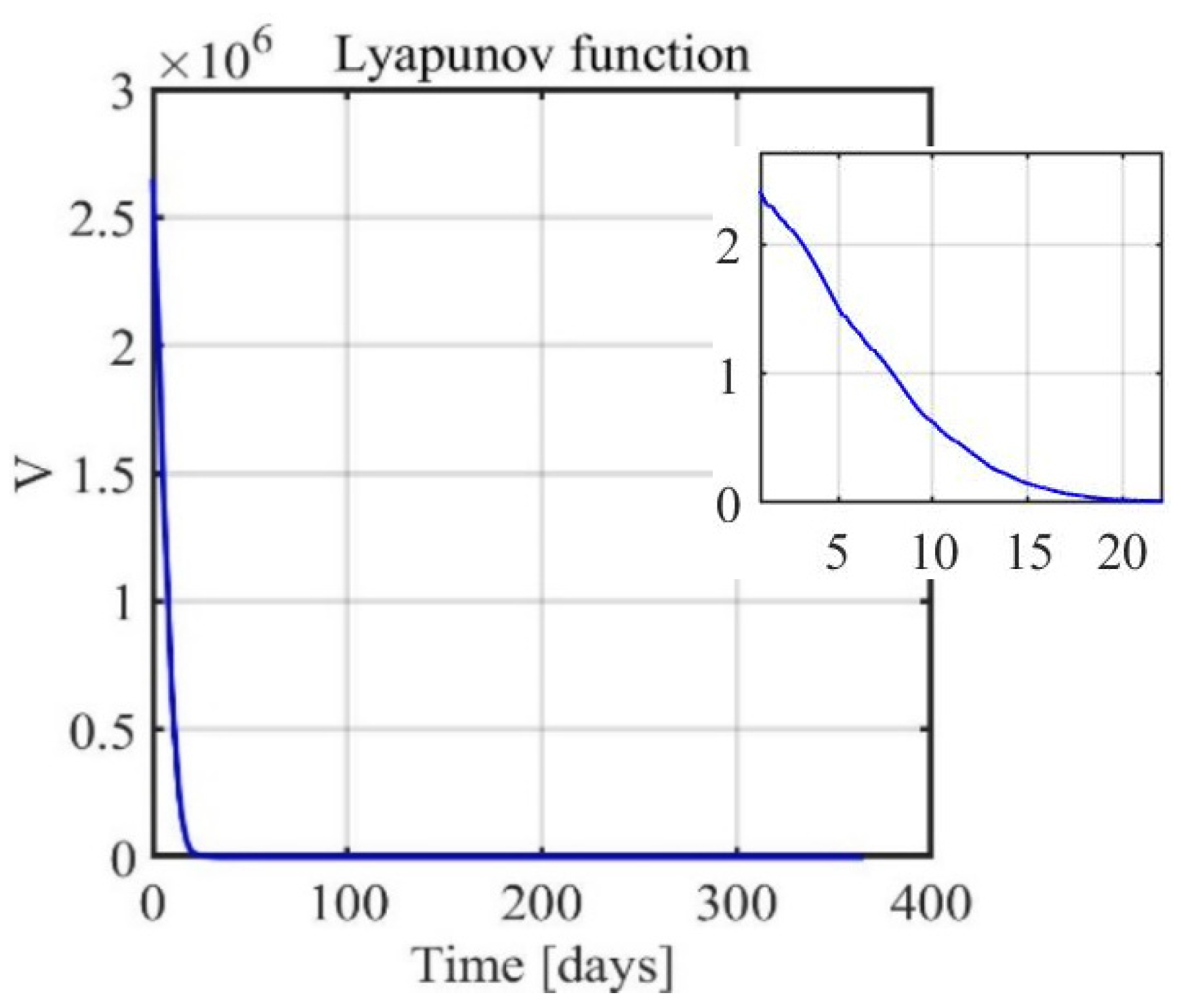
3.2. Feedback Law and Related Stability Analysis
- rectilinear trajectories
- target set, i.e.,
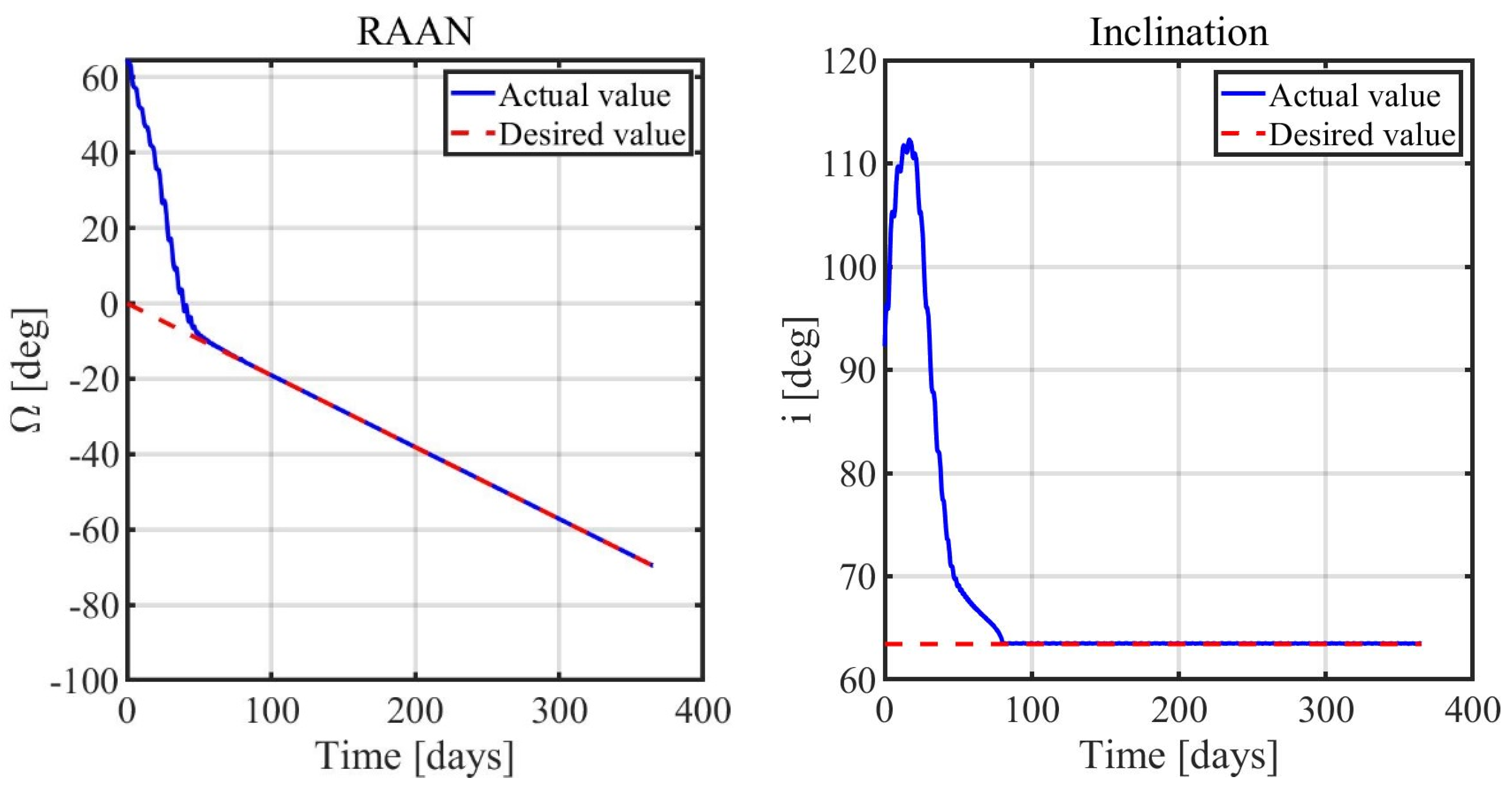
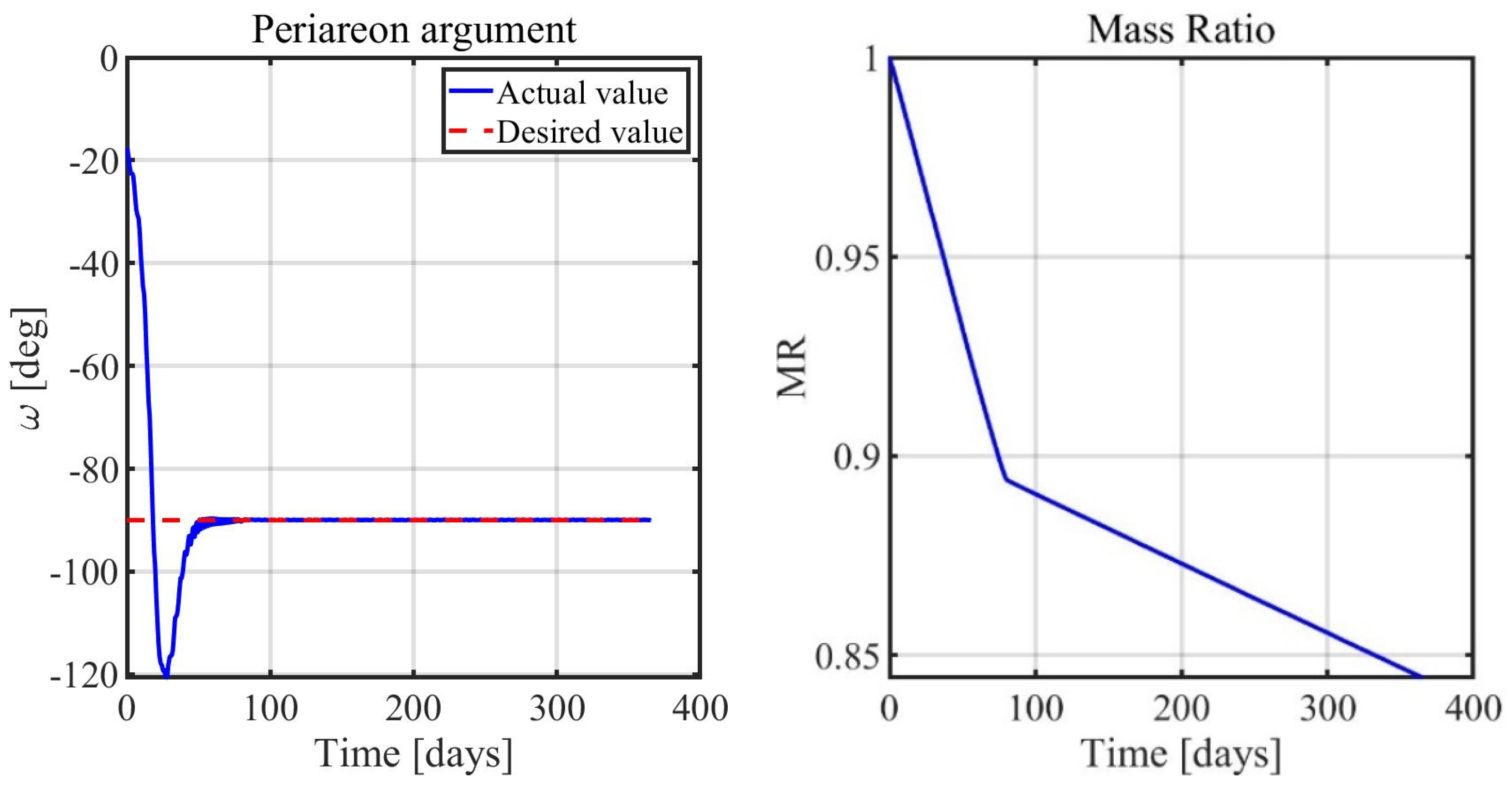
4. Numerical Simulations
- km/s, effective exhaust velocity
4.1. Orbit Injection with Temporary Propulsion Failure
4.2. Orbit Injection with Dispersed Initial Conditions and Errors in Perturbation Estimation
5. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| reference surface | |
| solar radiation pressure coefficient | |
| nodal day | |
| coefficients of gravitational harmonics | |
| mean anomaly | |
| solar radiation pressure | |
| Martian equatorial radius | |
| thrust | |
| maximum available thrust | |
| nodal orbital period | |
| Martian gravitational potential | |
| Lyapunov function | |
| non-Keplerian acceleration vector | |
| desired semimajor axis | |
| perturbation acceleration | |
| thrust acceleration | |
| osculating eccentricity | |
| desired eccentricity | |
| true anomaly | |
| osculating inclination | |
| desired inclination | |
| modified equinoctial elements (MEE) | |
| instantaneous mass of the vehicle | |
| initial spacecraft mass | |
| mass time rate | |
| osculating semilatus rectum | |
| desired semilatus rectum | |
| position vector | |
| radius | |
| apoapse radius | |
| periapse radius | |
| actual time | |
| time of flight | |
| osculating RAAN | |
| desired RAAN | |
| heading angle | |
| Martian gravitational parameter | |
| shadow function | |
| absolute longitude | |
| latitude | |
| vector of final conditions | |
| osculating argument of periapse | |
| desired argument of perigee | |
| Martian angular rate |
Appendix A. Planetary Parameters of Mars
References
- Ely, T.A.; Anderson, R.; Bar-Sever, Y.E.; Bell, D.; Guinn, J.; Jah, M.; Kallemeyn, P.; Levene, E.; Romans, L.; Wu, S.-C. Mars Network Constellation Design Drivers and Strategies. In Proceedings of the AAS/AIAA Astrodynamics Specialist Conference, Girdwood, AK, USA, 16–19 August 1999; pp. 99–301. [Google Scholar]
- Nann, I.; Izzo, D.; Walker, R. A Reconfigurable Mars Constellation for Radio Occultation Measurements and Navigation. In Proceedings of the 4th International Workshop on Satellite Constellation and Formation Flying, Sao José dos Campos, Brazil, 14—16 January 2005. [Google Scholar]
- Bell, D.J.; Cesarone, R.; Ely, T.; Edwards, C.; Townes, S. Mars Network: A Mars Orbiting Communications & Navigation Satellite Constellation. In Proceedings of the 2000 IEEE Aerospace Conference, Big Sky, MT, USA, 18—25 March 2000. [Google Scholar]
- Kelly, P.W.; Bevilacqua, R. Constellation Design for Mars Navigation using Small Satellites. In Proceedings of the 2018 AIAA Aerospace Sciences Meeting, Kissimmee, FL, USA, 8–12 January 2018; p. 1538. [Google Scholar]
- Pontani, M.; Pustorino, M.; Teofilatto, P. Mars Constellation Design and Low-Thrust Deployment Using Nonlinear Orbit Control. J. Astronaut. Sci. 2022, 69, 1691–1725. [Google Scholar] [CrossRef]
- Ulybyshev, Y.P.; Donianz, V.N. Elliptic Orbit Constellations for Regional Communication and Molniya–Zond Satellite Constellation. In Proceedings of the 53rd International Astronautical Congress, Houston, TX, USA, 10–19 October 2002. [Google Scholar]
- Ulybyshev, Y.P. Design of satellite constellations with continuous coverage on elliptic orbits of Molniya type. Cosm. Res. 2009, 47, 310–321. [Google Scholar] [CrossRef]
- Palmerini, G.B.; Graziani, F. Polar Elliptic Orbit for Global Coverage Constellations. In Proceedings of the AAS/AIAA Astrodynamics Conference, Scottsdale, AZ, USA, 1–3 August 1994; pp. 94–3720. [Google Scholar]
- Draim, J.E. Three- and Four-Satellite Continuous-Coverage Constellations. J. Guid. Control Dyn. 1985, 8, 725–730. [Google Scholar] [CrossRef]
- Draim, J.E. Design philosophy for the Ellipso satellite system. In Proceedings of the 17th AIAA International Communications Satellite Systems Conference and Exhibit, Yokohama, Japan, 23–27 February 1998. [Google Scholar]
- Pontani, M. Constellations of Repeating Satellites for Local Telecommunication and Monitoring Services. In Applied and Industrial Mathematics in Italy II; Series on Advances in Mathematics for Applied Sciences; World Scientific: Singapore, 2007; pp. 513–524. [Google Scholar]
- Cano, J.L.; Caramagno, A.; Catullo, V.; Cassi, C. ExoMars Mission Analysis and Design–Launch, Cruise and Arrival Phases. Adv. Astronaut. Sci. 2007, 127, 7–173. [Google Scholar]
- Pontani, M.; Pustorino, M. Nonlinear Earth orbit control using low-thrust propulsion. Acta Astronaut. 2021, 179, 296–310. [Google Scholar] [CrossRef]
- Pontani, M.; Teofilatto, P. Deployment strategies of a satellite constellation for polar ice monitoring. Acta Astronaut. 2022, 193, 346–356. [Google Scholar] [CrossRef]
- Gurfil, P. Nonlinear feedback control of low-thrust orbital transfer in a central gravitational field. Acta Astronaut. 2008, 60, 631–648. [Google Scholar] [CrossRef]
- Prussing, J.E.; Conway, B.A. Orbital Mechanics; Oxford University Press: Oxford, UK, 2013; pp. 46–54, 238–243. [Google Scholar]
- Schaub, H.; Junkins, J.L. Analytical Mechanics of Space Systems; AIAA Education Series; AIAA: Reston, VA, USA, 2009; pp. 574–597. [Google Scholar]
- Broucke, R.A.; Cefola, P.J. On the equinoctial orbit elements. Celest. Mech. 1972, 5, 303–310. [Google Scholar] [CrossRef]
- Battin, R.H. An Introduction to the Mathematics and Methods of Astrodynamics; AIAA Education Series; AIAA: Reston, VA, USA, 1987; pp. 484–503. [Google Scholar]
- Curtis, H.D. Orbital Mechanics for Engineering Students; Elsevier: Amsterdam, The Netherlands, 1987; pp. 479–541. [Google Scholar]
- Jurdjevic, V.; Quinn, J.P. Controllability and Stability. J. Differ. Equ. 1978, 28, 381–389. [Google Scholar] [CrossRef]
- MATLAB Symbolic Toolbox. Available online: https://it.mathworks.com/help/symbolic/ (accessed on 21 February 2023).
- Santoro, R.; Pontani, M. Orbit acquisition, rendezvous, and docking with a noncooperative capsule in a Mars sample return mission. Acta Astronaut. 2023; in press. [Google Scholar] [CrossRef]





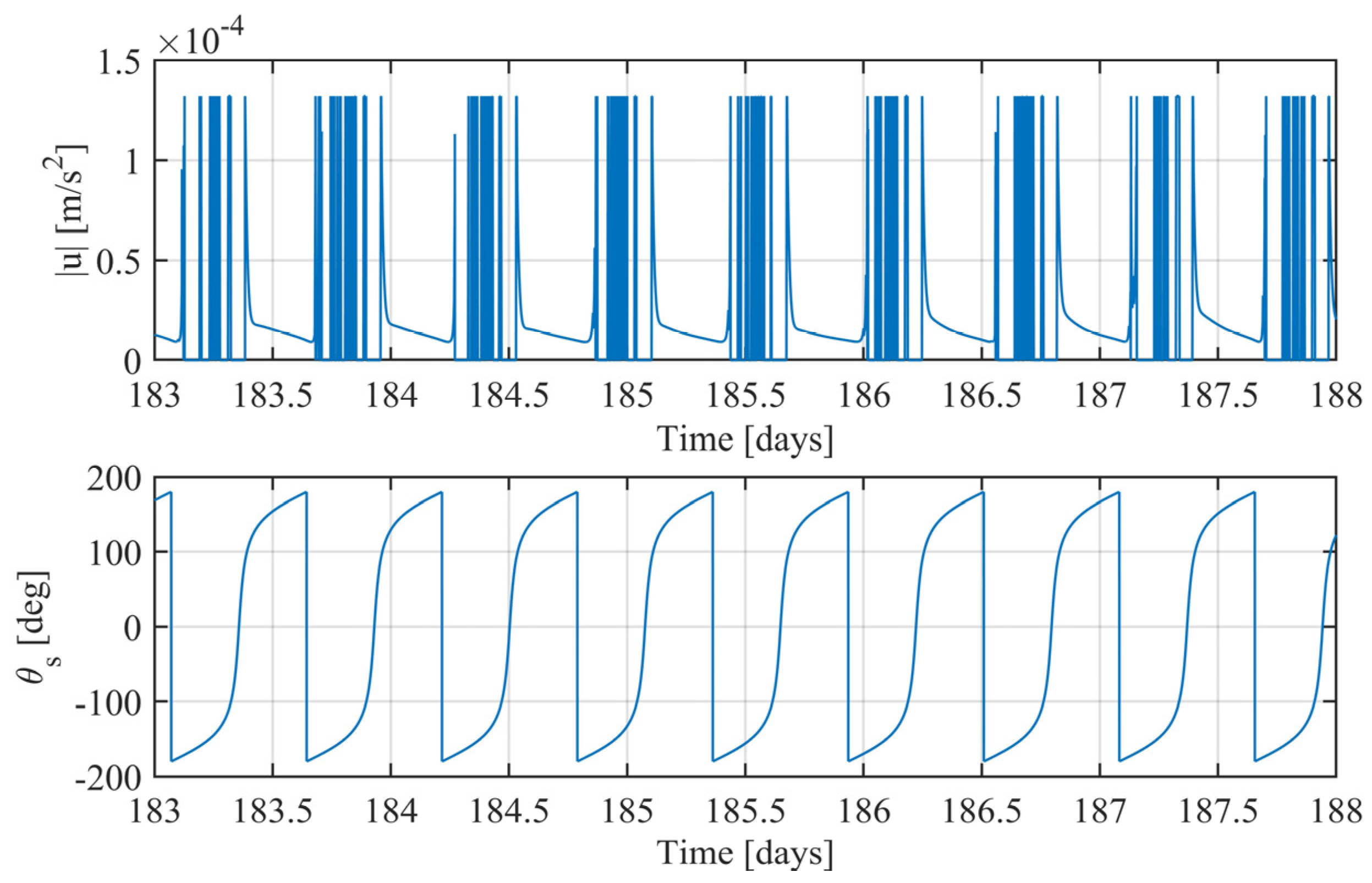

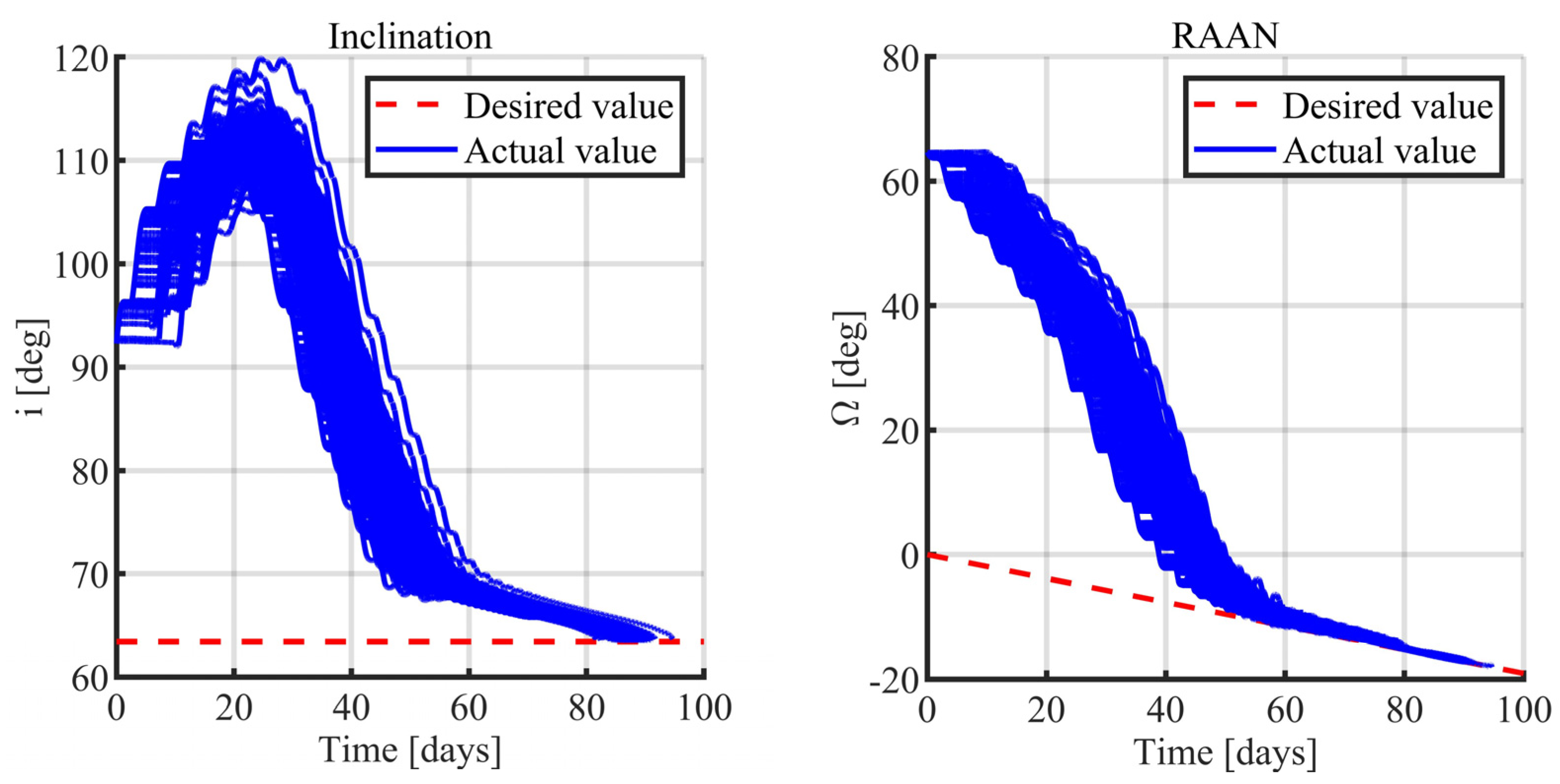
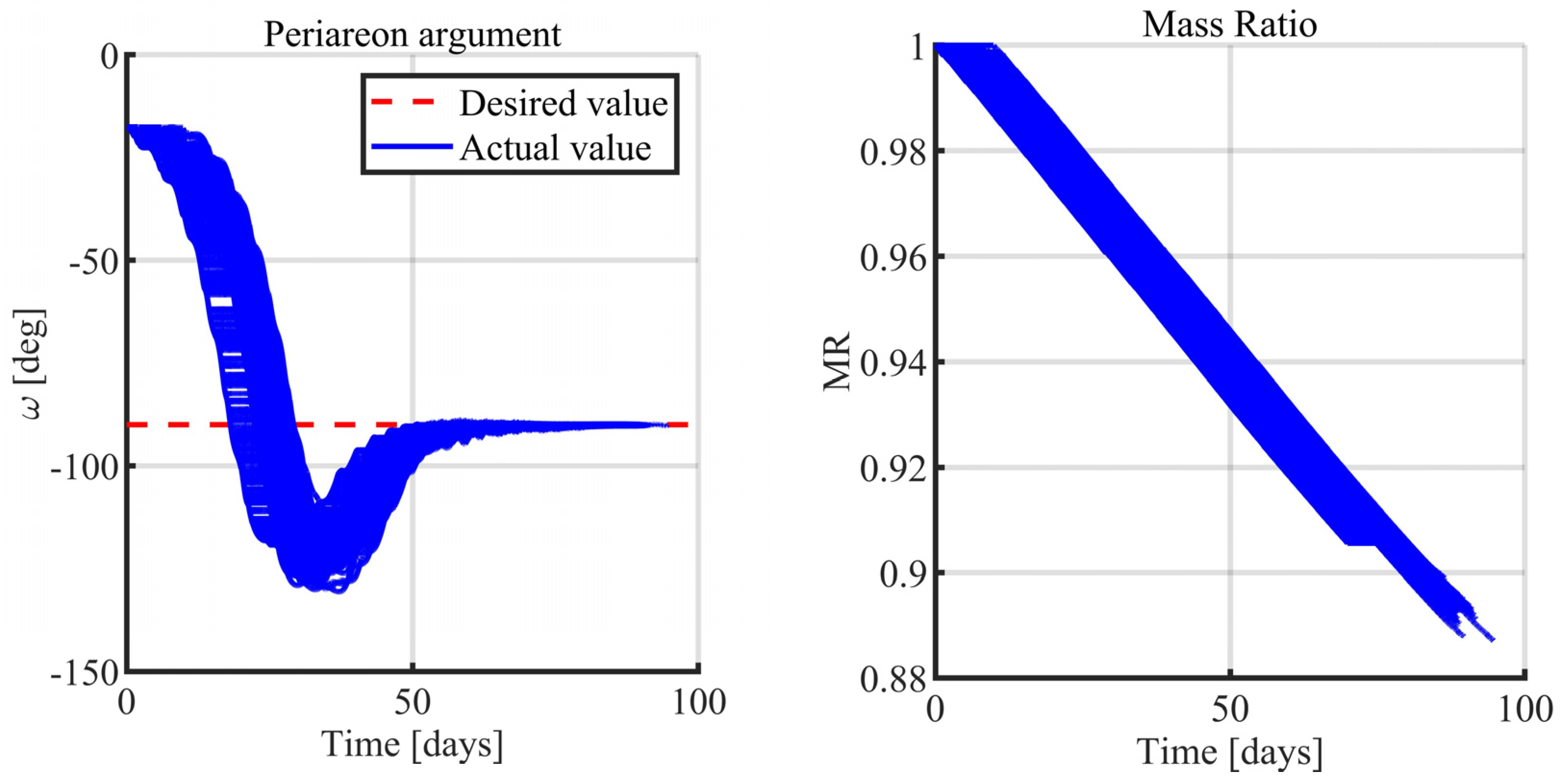
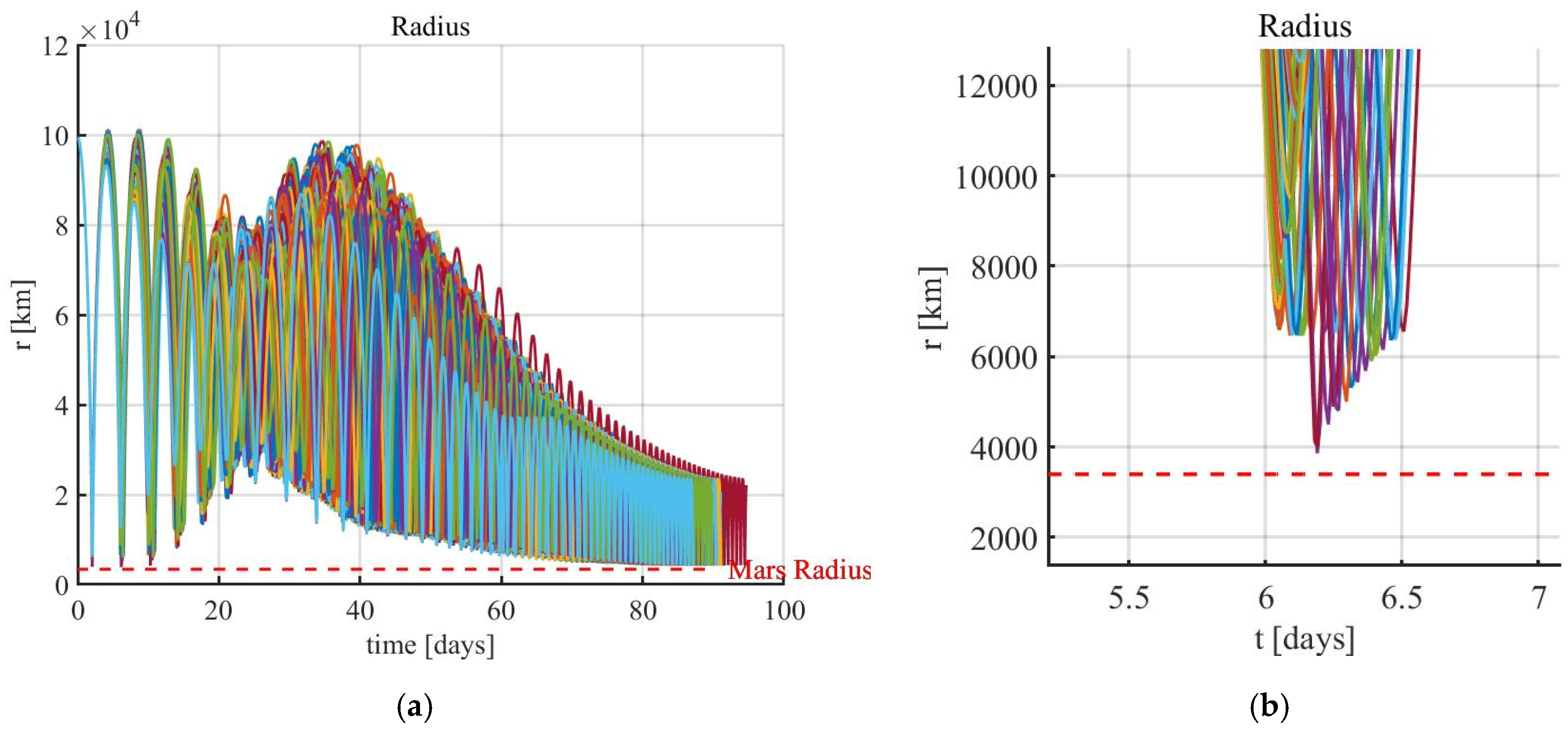


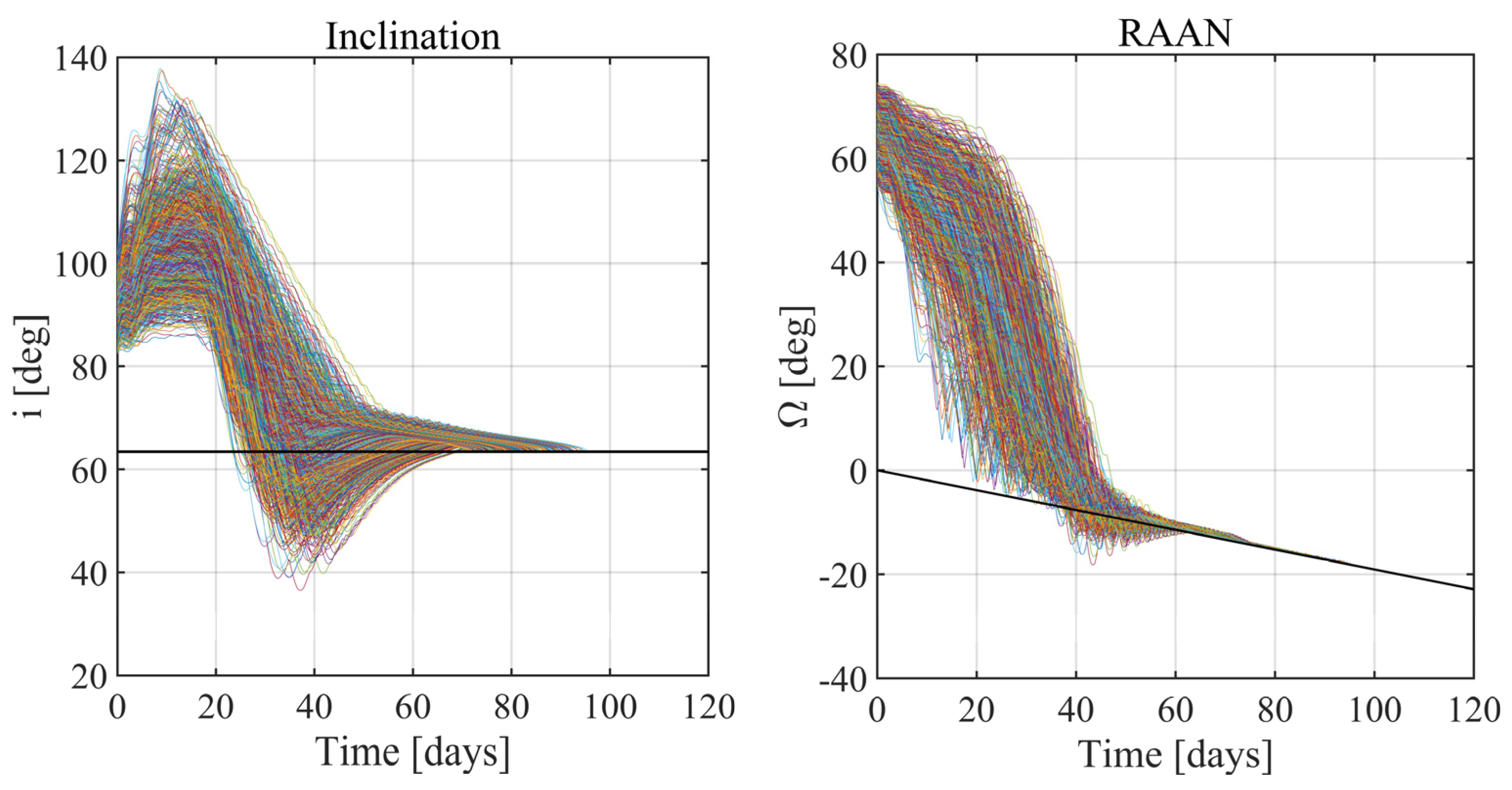

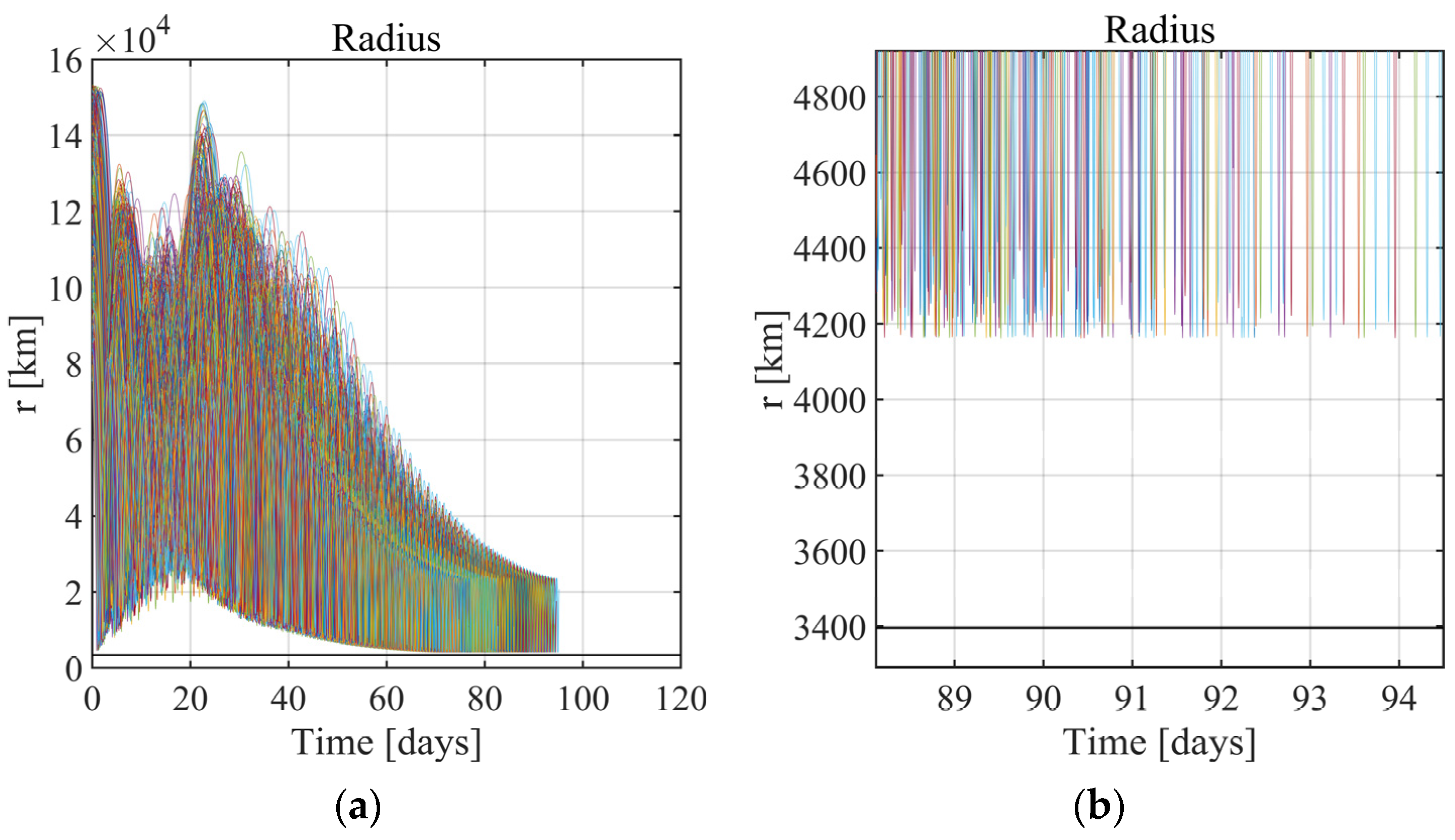

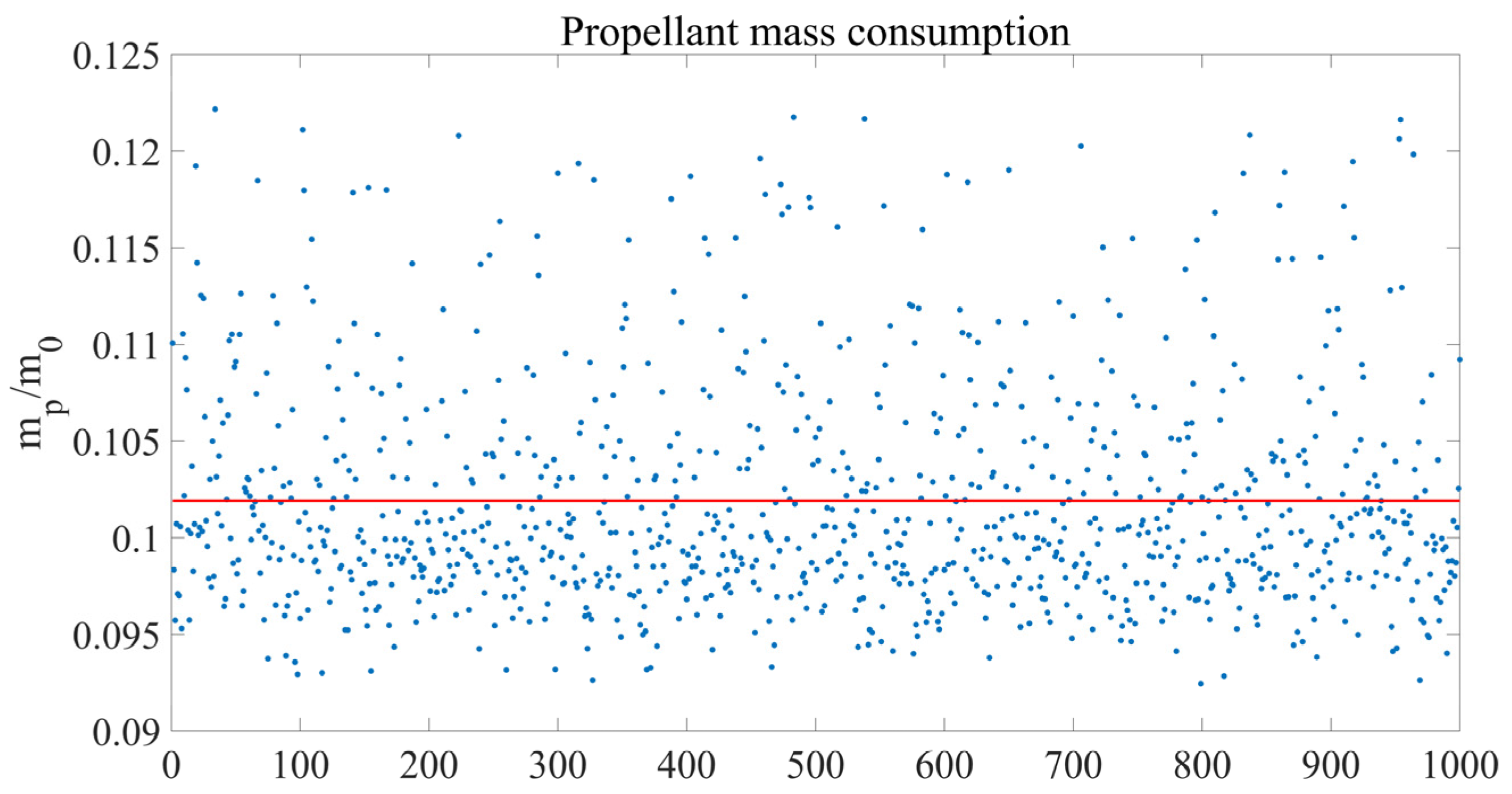
| Latitude (Deg) | 50 | 60 | 70 | 80 | 90 |
|---|---|---|---|---|---|
| (deg) | 0.4 | 9.7 | 19.2 | 29.0 | 38.7 |
| (deg) | 1.0 | 10.3 | 19.8 | 29.4 | 38.7 |
| Latitude (Deg) | 0 | 10 | 20 | 30 | 40 | 50 |
|---|---|---|---|---|---|---|
| 902.6 | 902.9 | 595.4 | 566.3 | 311.5 | / | |
| 909.6 | 908.1 | 699.1 | 592.8 | 394.6 | 71.6 |
| Mean Value | Standard Deviation | |
|---|---|---|
| Time of convergence [days] | 87.53 | 1.57 |
| Mass Ratio | 0.894 | 1.063 × 10−2 |
| Parameter | Unit | Mean Value | Std Deviation | Lower Bound | Upper Bound |
|---|---|---|---|---|---|
| Periares radius | [km] | - | - | 3596 | 10,000 |
| Apoares radius | [km] | - | - | 80,000 | 150,000 |
| Inclination | [deg] | 92.29 | 5.00 | 87.29 | 97.29 |
| RAAN | [deg] | 64.70 | 5.00 | 59.70 | 69.70 |
| Argument of periares | [deg] | 342.39 | 5.00 | 337.39 | 437.39 |
| True anomaly | [deg] | 180.00 | 5.00 | 175.00 | 185.00 |
| Mean Value | Standard Deviation | |
|---|---|---|
| Time of convergence [days] | 77.10 | 4.46 |
| Mass Ratio | 0.898 | 5.813 × 10−2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Santoro, R.; Pustorino, M.; Pontani, M. Low-Thrust Transfer to Quasi-Synchronous Martian Elliptic Orbit via Nonlinear Feedback Control. Aerospace 2023, 10, 670. https://doi.org/10.3390/aerospace10080670
Santoro R, Pustorino M, Pontani M. Low-Thrust Transfer to Quasi-Synchronous Martian Elliptic Orbit via Nonlinear Feedback Control. Aerospace. 2023; 10(8):670. https://doi.org/10.3390/aerospace10080670
Chicago/Turabian StyleSantoro, Riccardo, Marco Pustorino, and Mauro Pontani. 2023. "Low-Thrust Transfer to Quasi-Synchronous Martian Elliptic Orbit via Nonlinear Feedback Control" Aerospace 10, no. 8: 670. https://doi.org/10.3390/aerospace10080670
APA StyleSantoro, R., Pustorino, M., & Pontani, M. (2023). Low-Thrust Transfer to Quasi-Synchronous Martian Elliptic Orbit via Nonlinear Feedback Control. Aerospace, 10(8), 670. https://doi.org/10.3390/aerospace10080670






