Analysis and Suppression of Thrust Trap for Turbo-Ramjet Mode Transition with the Integrated Optimal Control Method

Abstract

An aircraft/engine integrated optimal control method is proposed for turbine-based combined cycle (TBCC) engines based on the Gauss pseudospectral method. The optimal flight trajectory and TBCC control law are obtained for a TBCC-powered aircraft, and the “thrust trap” that occurs during turbo-ramjet mode transition is further analyzed and suppressed. Results show that the aircraft goes through the mode transition phase using a “climb-dive” trajectory, which is a strategy of applying gravity-assist and temporarily reducing the drag. Furthermore, the TBCC engine adjusts at the quickest rate to minimize thrust loss. With the coupling of the trajectory and TBCC control law, the minimum thrust during the mode transition is only 23% of the thrust before the mode transition, suggesting the “thrust trap” phenomenon. By decreasing the mode transition time from 60 s to 15 s, the minimum thrust can only increase to 30%, and the “thrust trap” phenomenon cannot be effectively suppressed. When the operating speed range of the turbine engine increases from Ma2.5 to 2.9, the minimum thrust will reach 80%, and the “thrust trap” tends to level off.

1. Introduction

Aerospace hypersonic vehicles and combined-cycle engine technology are interdisciplinary engineering technologies that are rapidly developing and have significant national defense impact and economic value. Turbine-based combined cycle (TBCC) is a turbine engine-based, integrated ramjet engine, and it employs other forms of power to yield power with “wide speed range, high performance, and reusability”. Consequently, it has become the focus of research regarding high-speed maneuvering air and space equipment [1].

Compared to engines with a single power form, TBCC engines include mode transition between two or more sub-engines. The mode transition process faces the challenge of “thrust trap”, wherein the thrust of the combined-cycle engine makes it difficult to overcome the vehicle’s drag [2]. Mode transition involves synergistic control problems, such as inlet/nozzle flow channel switching, compression/expansion profile control, and fuel flow control. Therefore, most of the published studies on mode transition focus on TBCC control and thrust performance [3]. Chen et al. [4,5] established a component-based turbo-ramjet engine model and used a multivariate control law derived from the Newton–Raphson multi-objective planning algorithm to achieve stable mode transitions. Wang et al. [6] developed the performance simulation program of the airbreathing high-speed propulsion system HiMach. Based on its thrust continuity and flow continuity criteria, they studied the mode transition performance characteristics and transition strategy of the internal/external parallel TBCC engine. Chang et al. [7,8] established a control-oriented combined dynamic zero-dimensional propulsion model and a curve-fitting model of a supersonic vehicle. Additionally, they studied the control law of mode transition considering the aircraft/engine dynamic characteristics and analyzed the influence of the inlet splitter, nozzle splitter, and turbine windmill state on the control law under aircraft/engine aerodynamic coupling. Nie et al. [9] proposed a thrust control-based control law for a tandem turbo-ramjet combined engine based on an online engine real-time model based on EKF; Csank and Stueber et al. [10] simulated turbine/ramjet mode transition based on the High Mach Transient Engine Cycle Code (HiTECC) and used it for designing and testing control algorithms. A simulation framework based on the Memory-Mapped-Files technique was created to study the complex interactions between inlet flow dynamics, “variable geometry” actuation mechanisms, and flow controls in the transition from supersonic to hypersonic conditions and vice versa in Ref. [11].

The above studies are broadly divided into two categories: one decouples the flight motion and propulsion effect using a given trajectory or thrust demand to simplify the design of the mode transition control strategies, while analysis and suppression of mode transition “thrust trap” in conjunction with flight motion are not delved into [12,13,14]. The other, represented by Refs. [15,16], has researched mode transition from the perspective of flight/engine integration. However, these studies lack sufficient consideration of the component control law of the combined engine, making exploring the optimal control strategy to suppress “thrust trapping” quite challenging. Thus, it can be recognized that there is, so far, a scarcity of comprehensive research combining flight motion and combined dynamics control strategy that analyzes the mechanism of “thrust trap” and the means to suppress it. The core of integrated flight and engine study is a reasonable overall modeling technique that should fully consider the component control laws and flight motion characteristics. Specifically, the mode transition first involves the dynamic adjustment of the “variable geometry” inlet and nozzle system, including the fuel supply law of the turbine/ramjet combustion chamber. Moreover, different synergistic control strategies will affect the thrust, specific impulse, and stability of the mode transition process, and the overall modeling technique should consider the control laws of the components. Second, when the start Mach number of the mode transition and the transition time are specified, the thrust changes stemming from the different propulsion control strategies will affect the acceleration characteristics of the aircraft, resulting in different Mach numbers at the termination of the mode transition. In that situation, the flight motion shall be considered when conducting a quantitative analysis of the mode transition speed range and time. Generally, it is imperative to research the overall modeling techniques applicable to the mode transition based on the component control of the combined dynamics of the mode transition process, taking into account the flight motion characteristics at the aircraft/engine integration level. This research will help to improve the understanding of “thrust trap” characteristics of turbine-based combined power at the flight/engine level, and, thus, provide new methodological support for suppressing the “thrust trap”.

Therefore, this paper introduces a trajectory optimization method based on the Gaussian pseudospectrum for the turbine-ramjet mode transition process. It combines the working characteristics of the variable geometry inlet and nozzle system, turbine engine, and combustion chamber. It also established an overall modeling method of turbine-based combined dynamics based on the flight/engine optimal control law. Moreover, the optimal control law of components considering flight/engine integration is obtained and the mode transition characteristics of turbine-ramjet are elaborated upon. Last but not least, this paper gives an analysis of the suppression effect of the mode transition time and the maximum operating speed of the turbine on the “thrust trap”. Notably, the theoretical analysis in this paper is primarily based on overall modeling and simulation. Its objective is to propose a method for studying the suppression of ‘thrust trap’ during mode transition from the perspective of aircraft/engine synergistic optimal control. Therefore, the matching design for the turbine and ramjet can be achieved, which can be further analyzed in conjunction with more realistic models or data for the TBCC engine scheme of a specific configuration.

2. Method Description

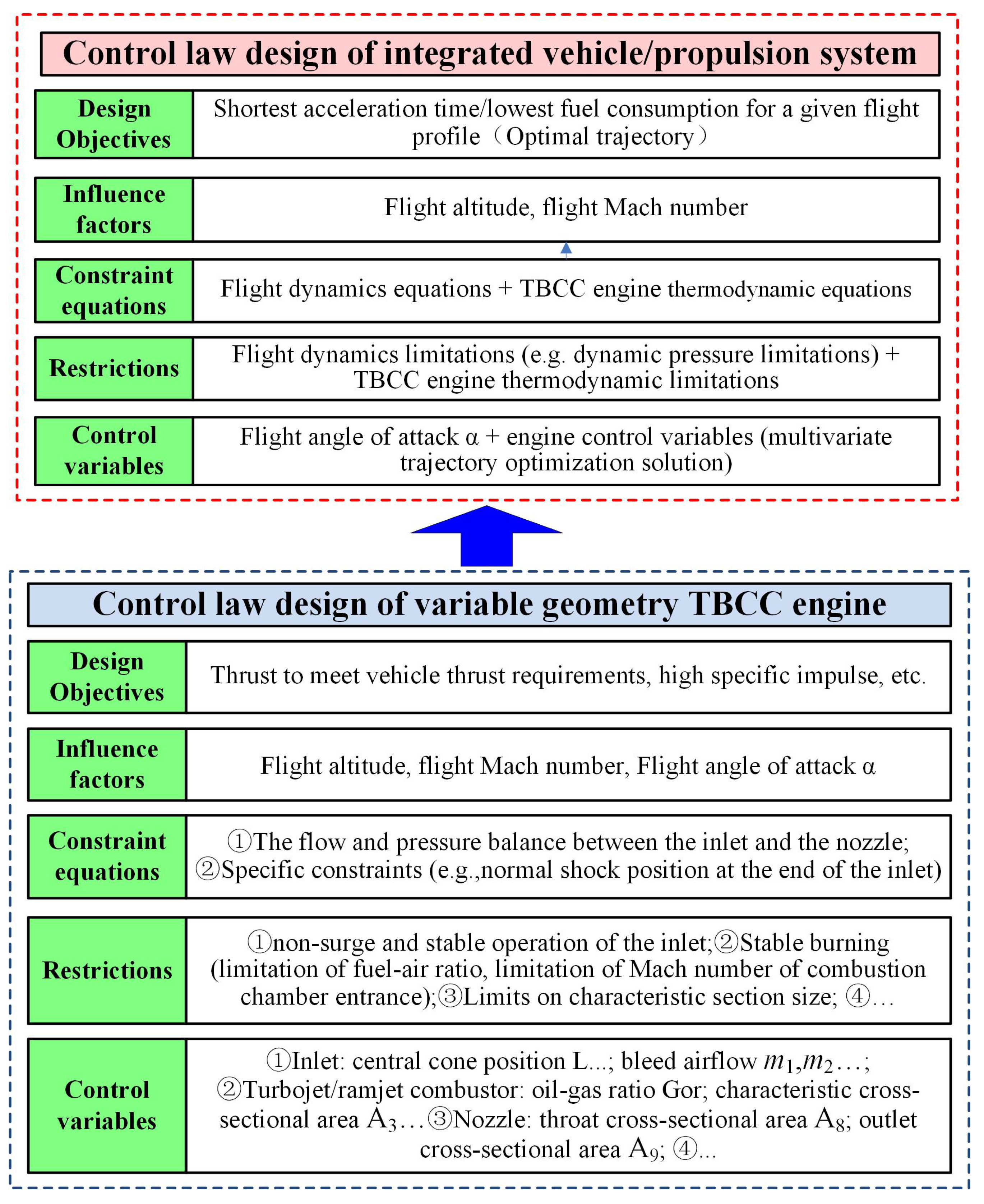
The integrated modeling method based on the optimal control law of aircraft/engine mainly comprises two levels. The first is the aircraft/engine modeling level, comprising the dynamic flight modeling in a vertical plane for “flight motion” and the TBCC modeling for “variable geometry”. The second is the optimization design level of multivariable control law, which is mainly based on the trajectory optimization method of the Gauss pseudospectral method. The optimization objective of this study is to minimize either the flight acceleration time or fuel consumption. The constraint equations consist of the superposition of flight dynamics and the TBCC engine thermodynamic equations. The limiting conditions involve the coupling of flight limitations, such as flight dynamic pressure, with TBCC engine thermodynamic limitations. The control variables are determined by the TBCC engine control variables based on the attack angle of the vehicle during flight, as shown in Figure 1, thereby transforming the propulsion system’s control law design problem into a multivariate optimal trajectory solution problem for a specific vehicle and mission. Herein, based on the analysis of the optimal trajectory of the vehicle and the optimal control law of the components of the TBCC engine, the overall performance of the TBCC engine during the mode transition process is quantitatively analyzed and the parameters are optimally designed.

2.1. Description of the Aircraft/Engine Model

At the aircraft/engine modeling level, only the effect of “variable trajectory” characteristics in the vertical plane is considered herein. Thus, the vehicle can be seen as a point mass model moving in the vertical plane [17], and its dynamics equations [18] are as follows:

$$\begin{array}{l}\dot{h}=V\sin \gamma \\ \dot{V}=\frac{T\cos \alpha -D}{m}-g\sin \gamma \\ \dot{\gamma }=\frac{T\sin \alpha +L}{mV}+(\frac{V}{r}-\frac{g}{V})\cos \gamma \\ \dot{m}=-\frac{T}{g\cdot Isp}\end{array}$$

(1)

where h and V denote the flight altitude and flight speed, respectively. γ and α denote climb angle and angle of attack, respectively. T and D denote the thrust and drag, respectively. L and Isp denote lift and specific impulse, respectively. m, r, and g denote vehicle mass, vehicle distance from the center of the earth, and gravitational acceleration. The superscript then represents the differential of the parameter, and the takeoff weight of the vehicle in this paper is 19,050 kg.

The lift L and drag D are defined as:

$$L=qS{C}_L(\alpha ,Ma)$$

(2)

$$D=qS{C}_D(\alpha ,Ma)$$

(3)

q is the flight dynamic pressure, S is the reference wing area of the vehicle, and the value taken herein is 41.4 m². Lift coefficient C_L and drag coefficient C_D are both functions of attack angle and Mach number Ma. Since there is no engineered vehicle performance data for the wide-speed range (Ma0–Ma4.0), the vehicle lift/drag coefficient for high Mach number (Ma > 1.5) in this paper is referenced to X–43 [19], while the data for low Mach number (Ma ≤ 1.5) data are obtained from Ref. [17]. A “variable geometry” TBCC engine is studied in the case of a turbine/ramjet combined engine with an operating speed range of Ma0.4–Ma4.0, wherein the inlet and nozzle have an axisymmetric structure. Moreover, the central cone of the inlet can be moved laterally along the axis and the de Laval nozzle throat and outlet area can be adjusted. The modeling method is shown in Refs. [20,21,22]. The turbine engine has a single rotor structure configuration, with a maximum state engine thrust of 153.39 kN, a flow rate of 121 kg/s, a pressure ratio of 15, a combustion chamber exit temperature of 1350 K, and a maximum afterburner temperature limit of 2200 K. Additionally, with a maximum temperature limit of 2200 K, the ramjet chamber is modeled following Refs. [23,24,25,26]. Other parts and complete engine modeling methods are discussed in Ref. [27].

For the turbine-ramjet mode transition process, the following simplifications are made: (i) the operating speed range of the turbine engine is set to Ma0–Ma2.5, i.e., when the flight Mach number is more significant than 2.5, the thrust of the turbine engine is set to 0; (ii) the indicated value dT describes the mode transition process, once the value dT is 0 (i.e., turbine mode) and dT is 1.0 (i.e., ram mode), the minimum time of the mode transition process is set to 60 s, i.e., the change rate dT′ ≤ 1.0/60 s; and (iii) the thrust calculation formula during the mode transition process is $T_{\mathrm{total}}=T_{\mathrm{turb}}+dT\cdot (T_{\mathrm{turb}}-T_{ram})$, which signifies that the TBCC thrust changes with the dT during mode transition.

Based on the above modeling method, firstly, based on the identified turbine engine, the inlet/nozzle capacity is clarified to take the inlet capture area of the turbine engine as a benchmark, and when the ramjet capture area is less than 150%, the combined turbine/ramjet power is not able to satisfy the wide-speed range climb demand. To meet the mode transition from turbine to ramjet and accelerate to the Ma4.0 within the performance parameter levels defined in this paper, Figure 2 illustrates the approximate sizing relationships of separate turbine and ramjet engines that are required when the turbine engine is operating in the Ma0–2.5 speed range. Notably, the modeling assumes that the turbine and ramjet engines have separate inlet and nozzle systems with the same configuration. That is, the one turbine engine and one ramjet engine in parallel layout form do not share the inlet and nozzle systems.

2.2. Multivariate Control Law Optimization Design

The principal of the integrated modeling method of TBCC based on optimal control laws lies in the trajectory optimization method based on the Gauss pseudospectral method. This modeling method converts the control law design problem of “variable geometry” components into a multivariate optimal trajectory solution problem for a specific vehicle and mission. The aircraft/engine integrated control vectors should be constructed first in the specific modeling process. In this study, seven controllable variables are included for the combined engine. They are the relative length of the central cone of the inlet L_in,r (i.e., ratio of the length of the central cone extending the lip to radius of the lip), the fuel-to-air ratio f_b of the combustion chamber, the fuel-to-air ratio f_ab of the afterburner, the fuel-to-air ratio f of the ramjet combustion chamber, the nozzle throat area A₈, the outlet area A₉, and the rate of change dT′ of the mode-transition marker. The abovementioned controllable variables and the flight angle of attack variation rate $\dot{\alpha }$ are used to construct the aircraft/engine integrated control vector $\overrightarrow{\beta }=[{\beta }_1,{\beta }_2,\dots {\beta }_8]$, where the angle of attack variation rate is used as the control quantity to ensure a smooth flight; the control vector variation range is shown in

Table 1. Control vectors $\overrightarrow{\beta }$ for aircraft/engine integration.

	Inlet	Turbine Combustion Chamber		Ramjet Combustion Chamber	Nozzle		Mode Transition	Aircraft
$\overrightarrow{\beta }$	β1	β2	β3	β4	β5	β6	β7	β8
Variables	Lin,r	fb	fab	f	A8	A9	dT′	$\dot{\alpha }$
Min	2	0	0	0	0	0	0	−0.5
Max	4	0.06	0.06	0.06	2.85	2.85	1/60	0.5

. Note that, when operating in subsonic and transonic regions (Ma < 1.1), the central cone of the inlet is fixed, and the L_in,r in the control vector becomes an invalid control variable, which is excluded in the control law optimization.

Based on the aircraft/engine system model, the flight altitude h, velocity V, climb angle γ, vehicle mass m, angle of attack α, and mode transition marker dT are set as state parameters, and the aircraft/engine control vector $\overrightarrow{\beta }=[{\beta }_1,{\beta }_2,\dots {\beta }_8]$ is set as the control variable. Subsequently, through the trajectory optimization solution, the optimal design of the multivariate control law at the aircraft/engine integration level can be achieved. Based on the vehicle control equations, the optimization problem of the climb trajectory can be formulated as the corresponding cost function and parameter restrictions. The performance index considered in this study is the minimum fuel consumption in the climb section. The boundary conditions and constraints are shown in

Table 2. Boundary conditions and constraints.

Parameters	Time	Control Variables	Status Parameters
	t/s	$\overrightarrow{\mathit{\beta }}$	h/km	V/(m/s)	$\mathit{\gamma }$/deg	m/kg	$\mathit{\alpha }$/deg	dT
Initial Value	0	Table 1	0	135	0	19,050	/	/
End value	/		22	1185.6	/	/	/	/
Minimum value	10		0	5	−45	11,430	−5	0
Maximum value	5000		26.4	1304	45	19,050	10	1

. The maximum and minimum limits of dynamic pressure are 75 and 10 kPa, respectively. The cost function is expressed as

$$J_{\min ,fuel}=-m\left(t_f\right)$$

(4)

where m(t_f) represents the weight of the vehicle after the climb.

The above optimization problem can be converted to a continuous Bolza problem using the Gauss pseudospectral method, which constructs Lagrange interpolation polynomials on a set of Legendre points to approximate a system’s state and control variables. Thus, the continuous-optimal control problem can be transformed to a nonlinear planning problem (NLP). Studies have shown that Karush–Kuhn–Tucker (KKT) multipliers of NLP can be used to accurately estimate the co-state at both the Legendre–Gauss points and the boundary points owing to the equivalence between the KKT conditions and the discretized first-order necessary conditions. The general-purpose package GPOPS is used herein to solve the trajectory optimization problem; more details about this algorithm are provided in Refs. [28,29].

3. Characteristics Analysis of Thrust Trap

Based on the integrated modeling method of the above optimal control law, the overall characteristics of aircraft/engine and component control laws are determined. The vehicle/combined engine system from Ma0.4 takeoff to Ma4.0 and the most fuel-efficient trajectory are studied. Furthermore, the mode transition process is emphatically analyzed.

3.1. Aircraft/Engine General Characteristics

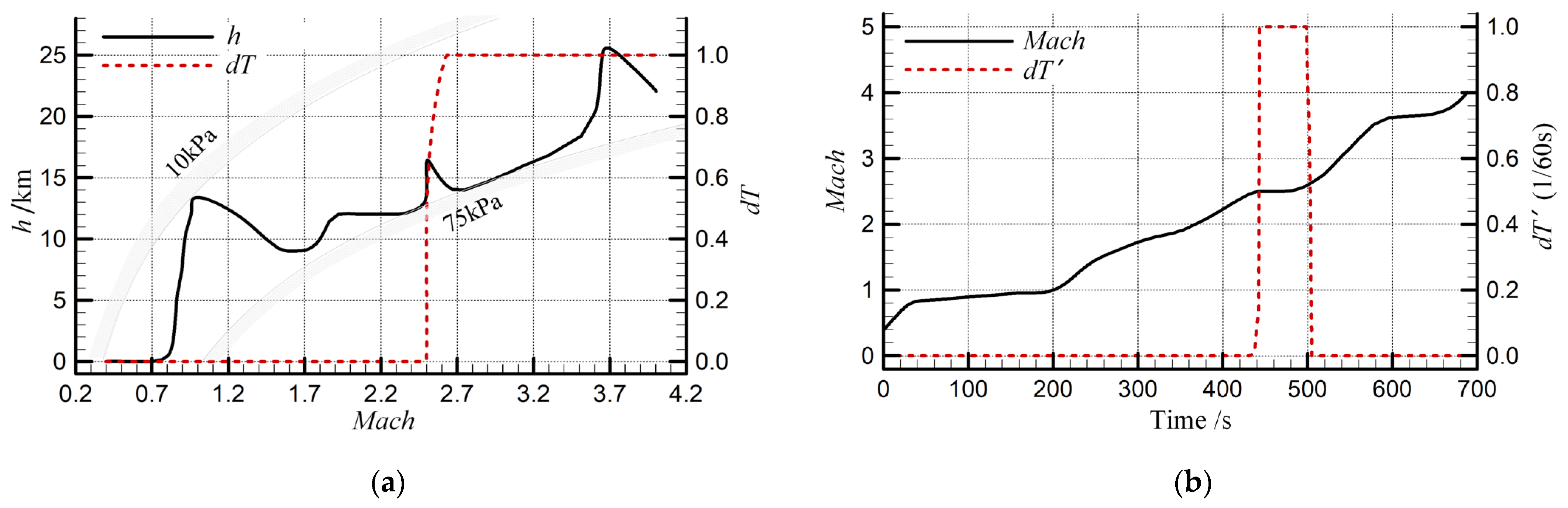
Figure 3a displays the variation of the most fuel-efficient climb flight path and the mode transition marker dT. The figure shows that the vehicle flies within a dynamic pressure corridor of minimum 10 kPa and maximum 75 kPa. Additionally, the vehicle may use a “climb-dive” strategy to improve the local economy across the transonic region in the mode transition region and at Mach numbers greater than 3.5. More details have been discussed in Refs. [30,31]. Specifically, the mode transition marker dT is zero until Mach 2.5, suggesting that the combined engine is in turbine mode; after Mach number exceeds 2.63, dT equals 1, suggesting that the combined engine is in the ramjet mode. When the flight Mach number is between 2.5 and 2.63, dT gradually increases from 0 to 1.0, suggesting that the mode transition from turbine mode to ramjet mode is realized.

The above mode transition process can be obtained from the curves of the flight Mach number and the rate of change of the mode transition marker dT′ with time, as shown in Figure 3b. Before the start of the mode transition, the flight Mach number stays at Ma2.5; it increases only when the mode transition progresses. Additionally, the mode transition process lasts 66 s, with the intermediate 55 s rate of change dT′ is maintained at the maximum limit value of 1.0/the 60 s. It indicates that, under the established simplified conditions, the mode transition adjustment mechanism mostly functions in the maximum change amplitude state. Thus, the intermediate amplitude state time is relatively small.

The combined engine’s thrust and specific impulse characteristics are presented in Figure 4. The figure has three prominent lower thrust regions corresponding to the transonic region, mode transition region, and high Mach number 3.6–4.0 range. Moreover, the local thrust drop in the above velocity range corresponds to the “climb-dive” acceleration strategy in Figure 3a. For the mode transition phase, the minimum thrust is only 21.9 kN, which is 18% of thrust before the mode transition and the concave phenomenon of the entire thrust curve is evident. Additionally, the thrust of the ramjet engine is a relative surplus between Mach 2.7 and 3.5, and its thrust decreases more significantly when the flight Mach number is less than 2.7 or more than 3.5. Compared to the change of thrust, the combined engine’s specific impulse exhibits a gradually decreasing trend. In the subsonic climb phase, the afterburner in the turbine engine is not functional and the specific impulse exceeds 2500 s. In the transonic phase, the afterburner ignites and the engine specific impulse sharply decreases to about 1800 s, and then slowly decreases with the gradually increasing Mach number. During the mode transition process, the specific impulse significantly decreases, and the minimum value of the specific impulse is only 675 s. After the completion of the mode transition, the ramjet engine gradually accelerates to its optimal working speed range of Ma2.7–4.0, and its specific impulse gradually increases and remains almost constant.

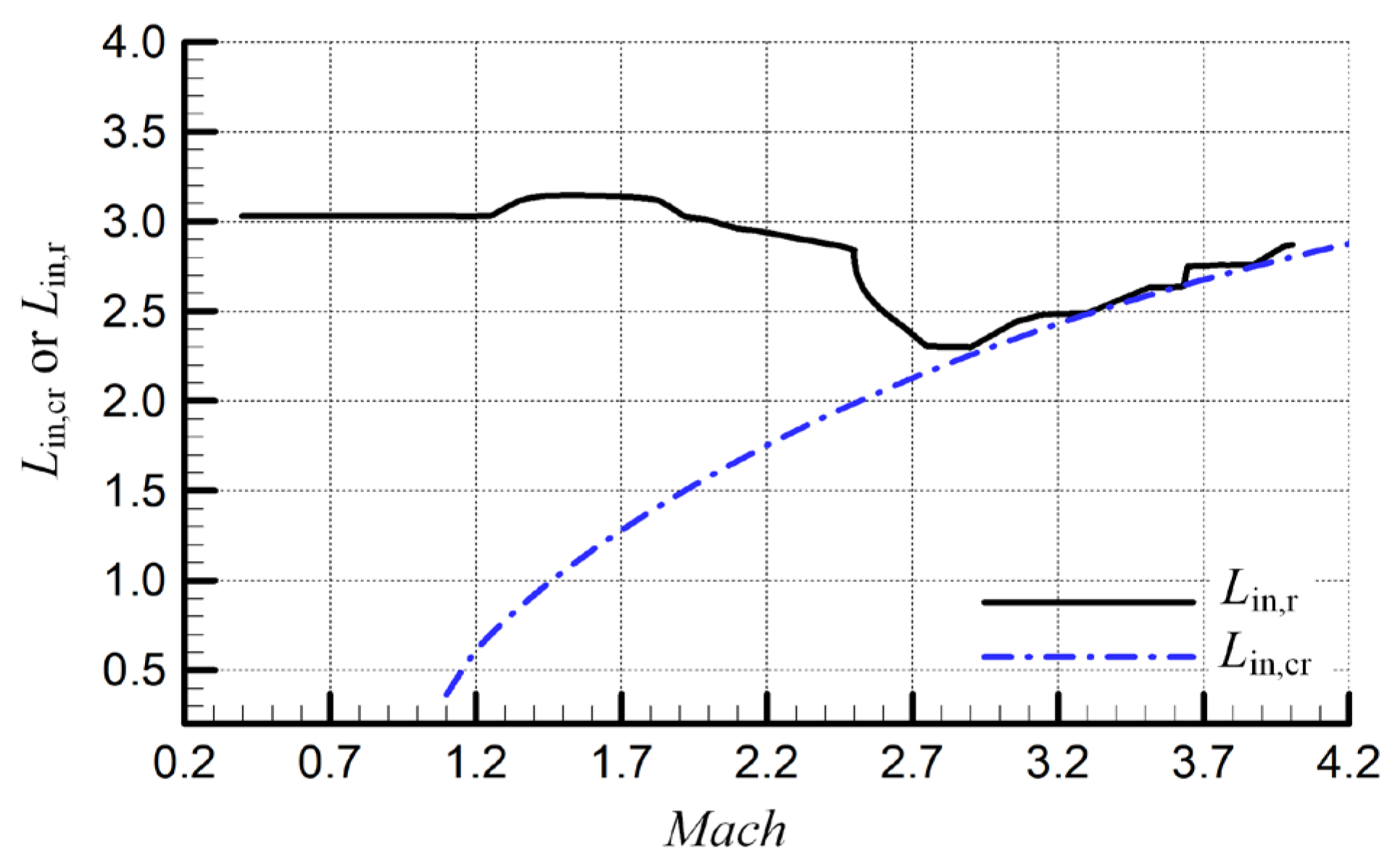
The component characteristics mainly influence the variation of the abovementioned overall parameters of the aircraft/engine model. Then, the following analysis is conducted for the control law of the components. Figure 5 shows the movement characteristics of the inlet center cone, wherein L_in,cr is the relative position of the ideal center cone corresponding to the critical state of the inlet, and L_in,r is the actual center cone position of the inlet. When the flight velocity is less than Ma1.1, the center cone is maintained at a relative length of 3.03. As the Mach number of flight increases, the center cone gradually moves forward to a relative length of 3.15 before gradually contracting due to the turbine engine flow characteristics. The maximum capture mass flow rate of the inlet in the turbine mode is significantly higher than the turbine engine flow capacity. Thus, the center cone needs to significantly move forward to overflow surplus air, allowing the inlet to capture the flow required by the turbine engine. In the mode transition, the center cone rapidly contracts according to the maximum limiting rate to achieve the shock-on-lip; in the ramjet mode, the center cone will be controlled basically according to the critical state. As the flight Mach number increases, the center cone gradually moves forward to maintain the shock-on-lip state. Note that a slight fluctuation difference exists between the actual position of the center cone and the ideal position in the ramming state, which slightly differs between the ramjet engine flow and the critical state. We believe that this difference stems from the accuracy problem of trajectory optimization solution. In addition, turbine engines, ramjet combustion chambers, and nozzle control laws are discussed in Ref. [27].

In summary, the integrated TBCC modeling method yields the optimal control law of the components considering aircraft/engine integration. In the turbine mode, the center cone of the inlet is in a sub-critical state to meet the flow demands of the turbine engine; in the ramjet mode, the center cone is in a critical state to achieve the optimal performance of the ramjet engine; during the mode transition, the center cone rapidly contracts according to the maximum change rate and the captured flow in the inlet significantly increases. The above component control law is basically in line with objective understandings. The quantitative result of the propulsion control laws derived from the modeling method is mostly in line with the objective understanding.

3.2. Mode Transition Characteristics

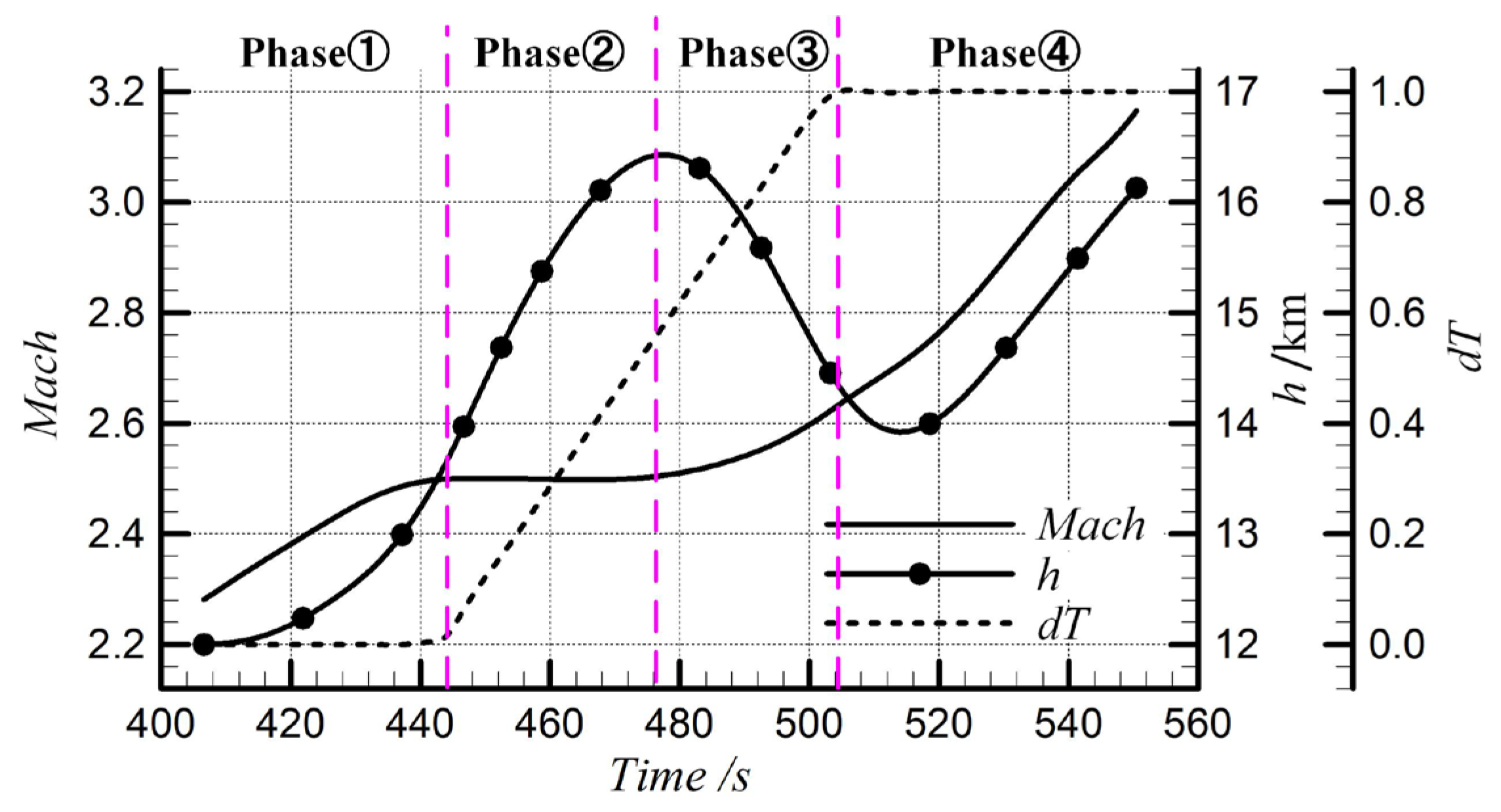
The integrated modeling method of TBCC can yield the component control law. Moreover, it can more comprehensively realize the in-depth analysis of “mode transition” characteristics from the perspective of aircraft/engine integration. Figure 6 shows the flight altitude, Mach number, and mode transition marker dT as a function of time during the mode transition process. The mode transition can now be roughly divided into four phases: ① Before the mode transition, the turbine engine alone pushes the aircraft to accelerate to Ma = 2.5 and h = 13.9 km. ② The vehicle maintains a constant Mach number of 2.5 to ensure that the flight altitude can climb from h = 13.9 km to the highest point of h = 16.4 km when the turbine engine operates normally. Then, mode transition commences in the climbing process. The process starts at 447 s and ends at 477 s, lasting 30 s. ③ The vehicle starts to dive, accelerating to Ma = 2.63 and h = 14.3 km with the assistance of gravity. The process lasts 27 s, and the mode transition ends at 504 s; the total time taken for the mode transition is 57 s. ④ After the mode transition ends, the ramjet engine works in a separate mode. The vehicle now climbs with the maximum dynamic pressure limit of 75 kPa with equal dynamic pressure. In summary, combined with the “climb-dive” acceleration strategy of the vehicle, the turbine-ramjet conversion process comprises four typical phases: a separate turbine climb phase at the maximum turbine operating speed before the mode transition, a turbine-ramjet co-working phase at the maximum turbine operating speed during the mode transition, a separate ramjet dive acceleration phase during the mode transition, and a separate ramjet climb phase after the mode transition.

Figure 7a shows the center cone’s position and the flow capture coefficient variation during the mode transition. The figure shows that, in the first two phases of the mode transition, since the turbine engine is the dominant power, its flow is mainly determined by the turbine gas generator. Furthermore, the turbine engine body flow is significantly less than the maximum ideal capture flow in the inlet, and since the flight Mach number is basically unchanged, as shown in Figure 6, the center cone position is maintained. When the mode transition enters phase 3, the turbine engine is turned off and the combined engine works in the ramjet mode. Since the inlet is in a critical state corresponding to the best performance of the ramjet engine, and the inlet is in a sub-critical state at the beginning of phase 3, the center cone rapidly contracts according to the maximum change rate of L_in,r of 0.01/s. Additionally, the inlet flow capture coefficient rapidly increases. After the mode transition, it enters phase 4. When the central cone position gradually converges to the ideal state, the flow capture coefficient increases to almost 100%.

To further analyze the sharp decrease of thrust during the turbine-ramjet mode transition, Figure 7b depicts the variation of the turbine/ramjet engine thrust and total thrust with time during the mode transition. As the vehicle adopts the “climb-dive” acceleration strategy during the mode transition, the turbine engine thrust gradually decreases as the vehicle climbs higher in the first stage of mode transition. When the mode transition commences at 447 s, the turbine engine thrust decreases by about 22% due to the altitude increase. Then, when the mode transition enters phase 2, the further increase in the flight altitude makes the turbine engine thrust decrease further. At this time, due to the smaller thrust per unit flow rate of the ramjet engine in this speed range and the influence of the inlet working in the sub-critical state, the ramjet engine thrust is relatively small and the total thrust decreases further. In phase 3 of the mode transition, the flight Mach number is greater than the maximum Mach number of 2.5 for the turbine engine, and the thrust of the turbine engine decreases to 0. Therefore, the minimal thrust value during the mode transition occurs at 477 s, which is only 23% of the thrust at 443 s. After phase 4 of the mode transition, the thrust of the ramjet engine gradually increases with the Mach number of flight. Moreover, the total engine thrust increases. Generally, the thrust during the mode transition is only 23% of the pre-modal transition thrust, which is the “thrust trap” phenomenon that occurs due to the comprehensive effects of four aspects: the “climb-dive” strategy, turbine engine shutdown, low thrust per unit flow rate of the ramjet engine, and thrust loss of the sub-critical ramjet engine.

4. Suppression Study of Thrust Trap

As mentioned above, the integrated modeling method of TBCC improves the understanding of the component control laws and “mode transitions” at the aircraft/engine level. However, we believe that this method also has the advantages of TBCC design optimization and comprehensive evaluation of critical parameters at the aircraft/engine level. Therefore, a parametric analysis of the mode transition Mach number and transition time is performed herein.

Table 3. Effect of different mode transition parameters on the minimum ramjet size.

	Turbine Maximum Operating Speed	Mode Transition Time	Turbine Size	Ramjet Size
Benchmark Case	Ma = 2.5	60 s	1.0	1.5−0.02
Mode transition time effect	Ma = 2.5	30 s	1.0	1.48−0.02
	Ma = 2.5	15 s	1.0	1.46−0.02
Effect of maximum turbine operating speed	Ma = 2.7	60 s	1.0	1.4−0.02
	Ma = 2.9	60 s	1.0	1.3−0.02
Optimal Case	Ma = 2.9	15 s	1.0	1.26−0.02

provides the effect of different mode transition parameters on the minimum ramjet size for the flight target speed range of Ma0.4–4.0. Using the cross-sectional area of the turbine engine as the benchmark size, the minimum ramjet size corresponding to the benchmark size is 1.5_−0.02. This means that, by designing the ramjet to be 1.5 times the size of the turbine engine, the TBCC can push the aircraft to complete the wide-speed climb mission. When less than 1.48 times the size of the turbine engine, the mission cannot be accomplished due to insufficient thrust. Therefore, the theoretical minimum ramjet size is between 1.5 and 1.48 times the turbine engine.

As the mode-transition time decreases, the minimum ramjet area at 30 and 15 s does not significantly decrease, which is 1.48_−0.02 and 1.46_−0.02, respectively. As can be shown, the turbine/ramjet matching characteristics do not significantly improve when the mode transition time is reduced. When the maximum turbine operating speed increases from Ma2.5 to 2.7 and 2.9, the minimum ramjet size significantly decreases to 1.4_−0.02 and 1.3_−0.02, respectively. Therefore, the turbine/ramjet matching characteristics can be effectively improved by increasing the turbine operating speed. When the maximum turbine operating speed reaches Ma2.9 and the mode transition time is reduced to 15 s, the integrated and optimized minimum ramjet size is 1.26_−0.02. Generally, a 50% reduction in the mode transition time achieves only 2% size optimization. Size optimization is more influenced by the maximum turbine operating speed: a Mach increase of 0.2 at the maximum turbine operating speed achieves 7% size optimization of the ramjet.

4.1. Mode Transition Time Effect

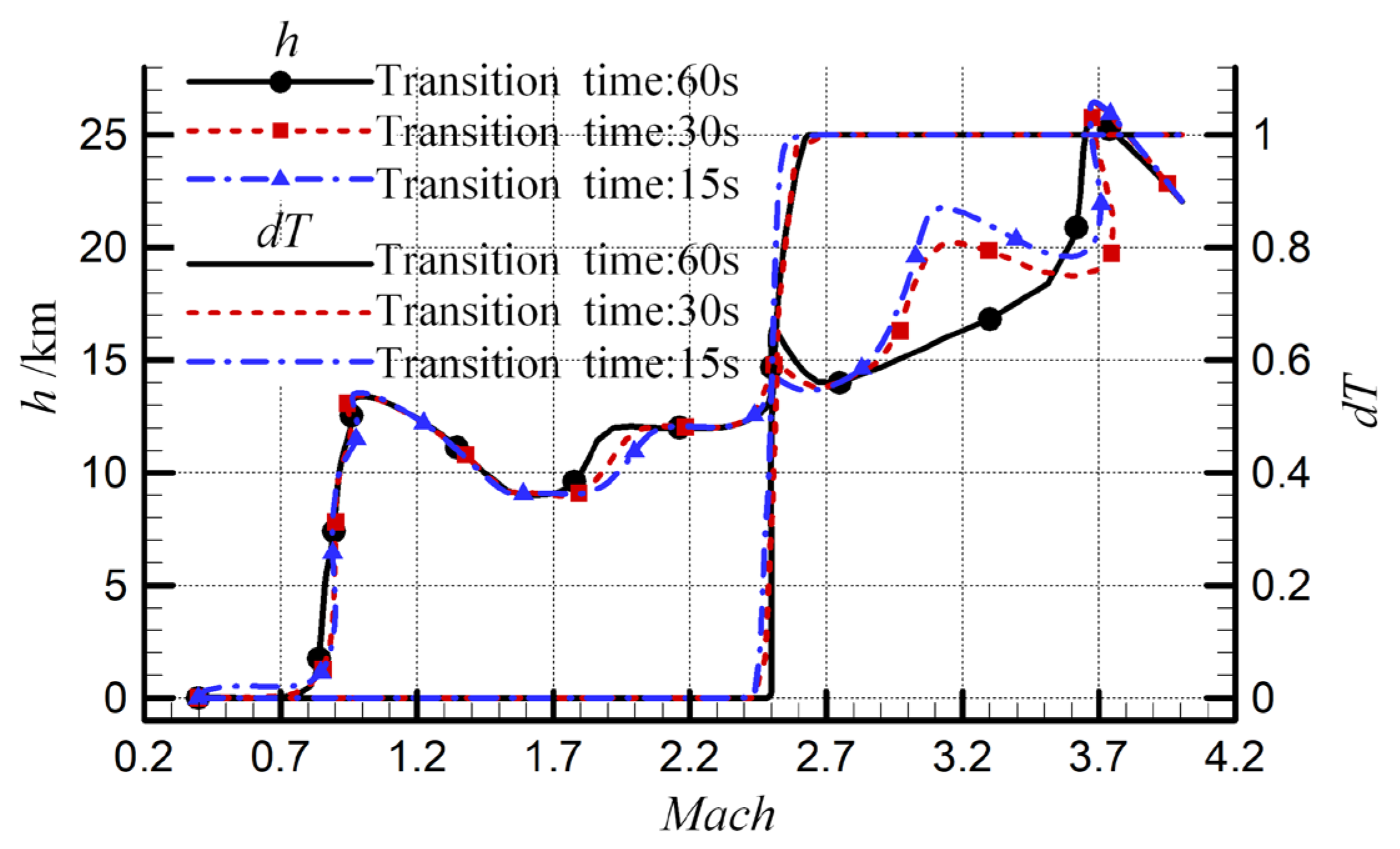
Based on the above minimum ramjet size scheme, comparative analysis is performed for the trajectory, mode transition, and thrust characteristics for different mode transition times, as shown in Figure 8, Figure 9 and Figure 10. It is worth noting that, as the mode transition time decreases, the maximum limiting speed of the center cone motion increases from 0.01/s to 0.02/s and 0.04/s. Figure 8 shows that, as the mode transition time decreases, the local climb characteristics near the supersonic Ma1.7 slightly shift toward the high Mach number. Additionally, the “climb-dive” mode is adopted near Ma3.2 for fuel-saving flights. Especially in the mode transition region, the center cone moves more rapidly from the sub-critical state of the turbine mode to the near-critical state of the ramjet mode due to the reduction of the mode transition time. Therefore, the “climb-dive” curve in the mode transition process is relatively flat. Moreover, the comparison of the indicated value of dT with the Mach number shows that it stays approximately constant with varying mode transition time.

The variation law of the center cone motion characteristics with time is given in Figure 9. Since the starting time of the mode transition is not the same for the three schemes, the relative time treatment is chosen herein. Figure 10 shows that, as the mode transition time decreases and the maximum rate of center cone motion increases, the center cone of the mode transition process moves from the sub-critical state of the turbine to the near-critical state of the press at a more significant rate. Additionally, the center cone rapidly contracts according to the maximum rate of change. Then, the mode transition time significantly decreases.

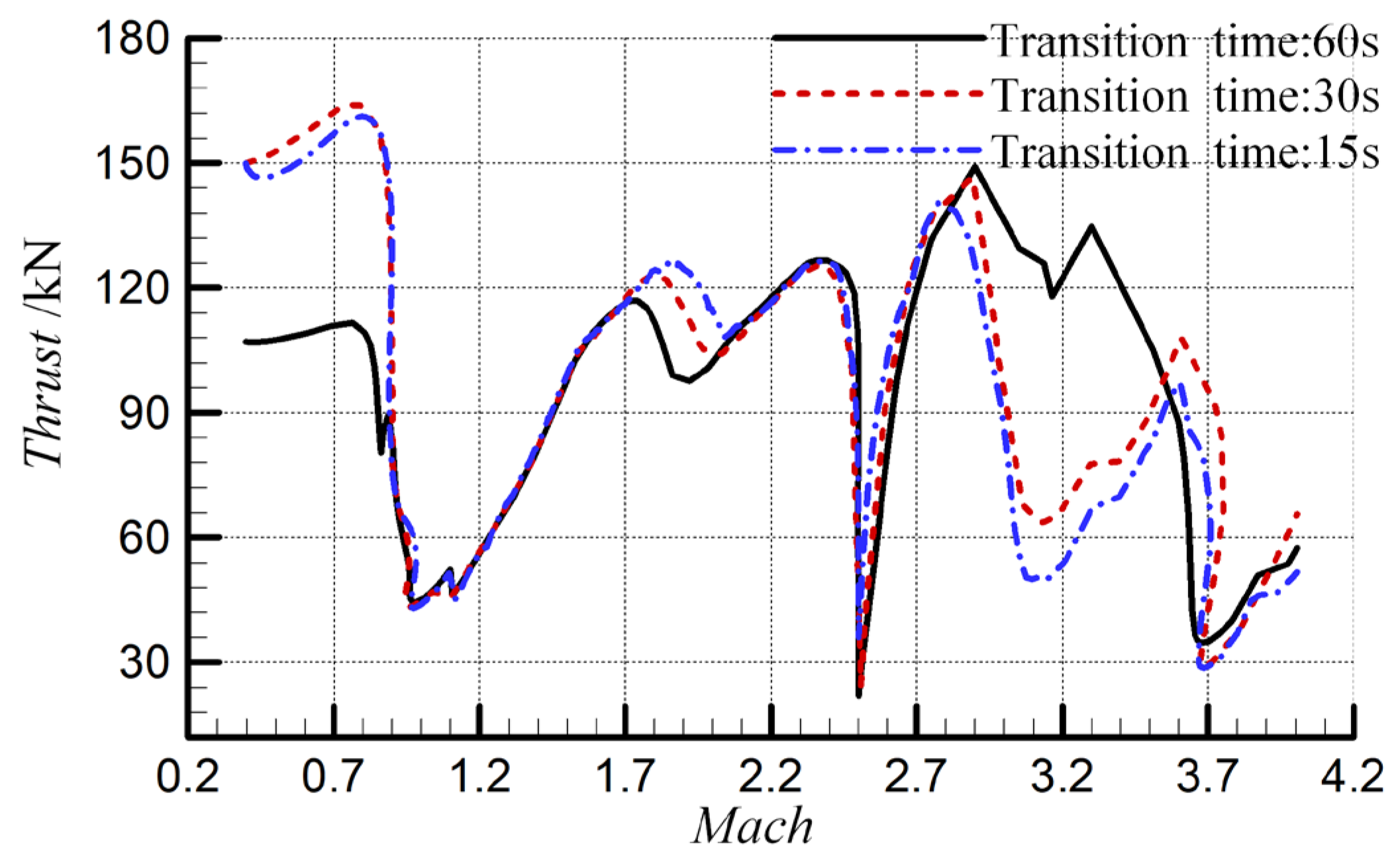
Figure 10 compares the thrust characteristics at the three mode-transition times noted above. The figure shows that the afterburner in the turbine engine works under subsonic flights for both the 30 and 15 s cases. Additionally, the thrust near subsonic and Ma3.2 exhibits a more noticeable difference due to the “climb-dive” strategy near Ma3.2. In the mode transition phase, the “thrust trap” phenomenon is apparent in all three cases. The minimum thrust could increase to 30% of the pre-modal transition by decreasing the mode transition time from 60 s to 15 s, and the “thrust trap” phenomenon alleviates with decreasing mode transition time.

4.2. Mode Transition Mach Number Effect

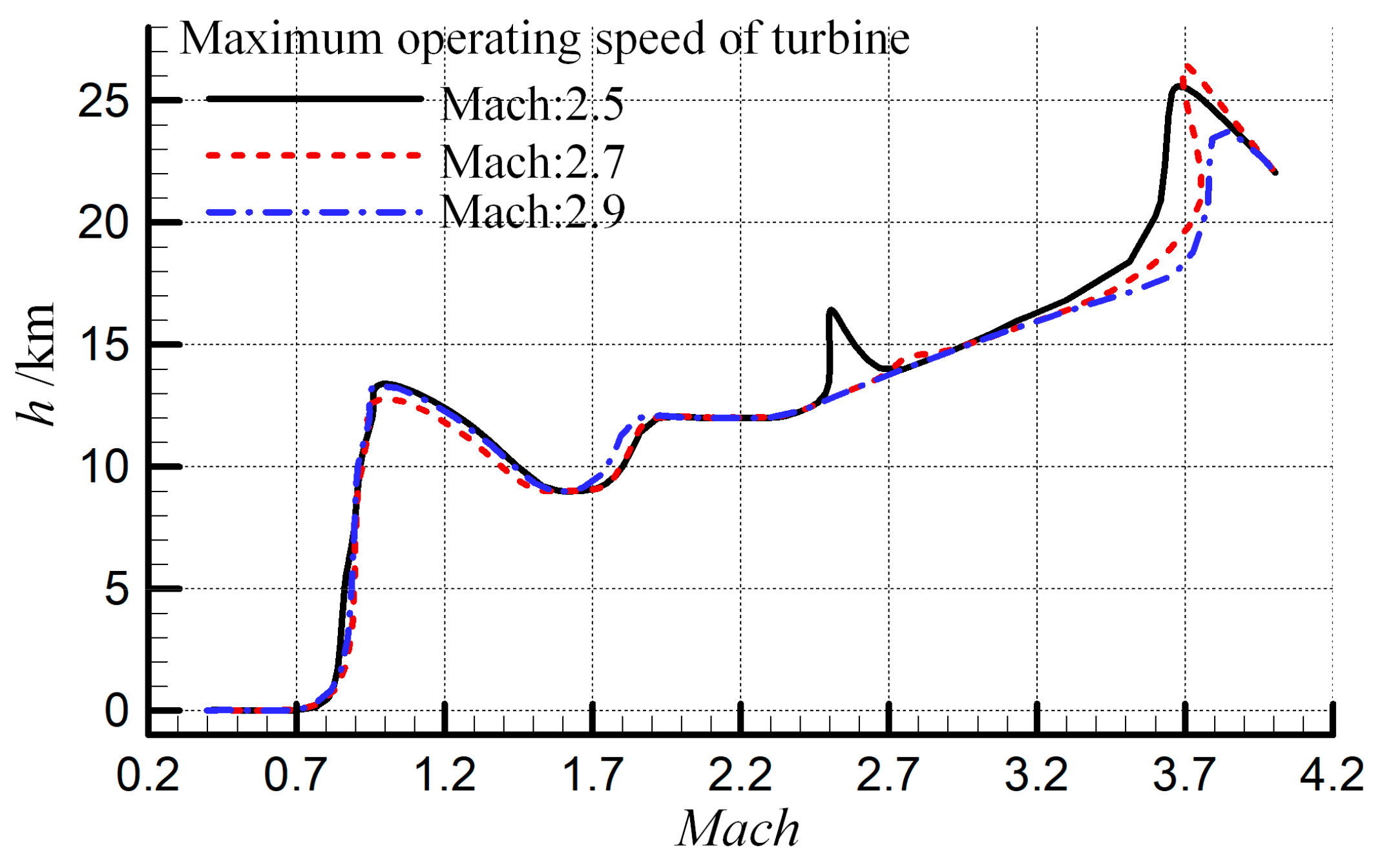
Based on the results of mode transition time effects, this section analyzes the effects of turbine maximum operating speed ranges. Figure 11 presents the comparison of the trajectories for the maximum operating speeds of Ma2.5, 2.7, and 2.9 states. The figure shows that the mode transition processes of the Ma2.7 and 2.9 states do not exhibit a significant “climb-dive” strategy compared to those of the Ma2.5 state. Moreover, the trajectory characteristics indicate that the mode transitions of the Ma2.7 and 2.9 state can generate enough thrust to accelerate the vehicle, and the “thrust trap” phenomenon during the mode transitions is significantly weakened.

Figure 12 shows the variation of the mode transition marker dT for the three turbines’ maximum operating speed range. The figure shows that the initial Mach number of the mode transition remains around Ma2.5 with increasing maximum operating speed of the turbine. Subject to the mode transition time limit of 60 s, the termination Mach number gradually increases with the maximum operating speed. The termination Mach numbers of mode transition are about 2.6, 2.9, and 3.1 for the Ma2.5, 2.7, and 2.9 states, respectively. The optimal case in the figure is the case of the turbine maximum operating speed of Mach 2.9 and time of 15 s. The figure shows that the start Mach number of the mode transition increases to Ma2.7, and the end Mach number is Ma2.9 due to the significant decrease in the mode transition time. Then, the turbine engine can function in the entire speed range, and the thrust loss during the mode transition will decrease.

The thrust characteristics of different turbine maximum operating speed are shown in Figure 13. The figure clearly shows the thrust trap phenomenon due to the combined effects of the following four aspects: “climb-dive” strategy, turbine shutdown during the mode transition, low thrust per unit flow of the ramjet engine, and thrust loss of the sub-critical ramjet engine. With the increasing turbine operating speed, the “thrust trap” phenomenon of the Ma2.7 scheme is significantly weakened. The “thrust trap” phenomenon of the Ma2.9 scheme is no longer apparent. The main reasons for the change of the “thrust trap” phenomenon are the increase in the ramjet engine thrust per unit flow during the high-Mach-number mode transition, which corresponds to the increase in the total thrust of the ramjet engine; additionally, the operating speed range expands in the high Mach number state and the thrust decrease caused by the turbine engine shutdown reduces. Therefore, the effective thrust during the mode transition allows the acceleration of the vehicle. The Ma2.7 and Ma2.9 scenarios do not adopt the “climb-dive” mode transition strategy but accelerate the climb along the 75 kPa dynamic pressure trajectory. Moreover, with increasing mode transition Mach number, the angle of the conical shock wave at the exact center cone position gradually decreases. The sub-critical overflow flow in the inlet at the beginning of the mode transition decreases, which corresponds to the decrease of the overflow loss.

In summary, when the turbine engine with a maximum operating Mach number of 2.9 undergoes mode transition, the thrust minima in the mode transition process increases to 80% of the pre-modal transition thrust. There are three reasons for this: combined effects of the constant dynamic pressure climb trajectory during the mode transition; increase in the thrust per unit flow of the ramjet engine; and the reduction of the sub-critical state ramjet engine overflow loss. Accordingly, the “thrust trap” phenomenon gradually tends to level off.

5. Conclusions

This study proposed an aircraft/engine integrated optimal control method of TBCC engines based on the Gauss pseudospectral method. The optimal flight trajectory and the TBCC control law were obtained for a TBCC-powered aircraft. The “thrust trap” during turbo-ramjet mode transition is further analyzed and suppressed. Several conclusions are summarized as follows.

(1) The thrust trap of the turbo-ramjet mode transition is affected by the flight motion and TBCC engine control law. Combined with the aircraft’s attack angle and the TBCC engine’s control variables, the aircraft/engine integrated control parameters can be established to solute the multivariate optimum trajectory. Then, the optimal control law for TBCC and the optimal trajectory can be simultaneously obtained via the Gauss pseudospectral method.

(2) The aircraft goes through the mode transition phase using a “climb-dive” trajectory, which is a strategy of applying gravity-assist and temporarily reducing the drag. Furthermore, the TBCC engine adjusts at the quickest rate to minimize thrust loss. For the aircraft to overcome the thrust trap, a TBCC with maximum turbine operating Mach 2.5 and 60 s of mode transition requires a ramjet engine with 150% turbine inlet/nozzle capacity. With the coupling of the trajectory and TBCC control law, the minimum thrust during the mode transition is only 23% of the thrust before the mode transition, suggesting the “thrust trap” phenomenon.

(3) By decreasing the mode transition time from 60 s to 15 s, the ramjet’s size in the TBCC was reduced by 2.7%. The minimum thrust can merely increase to 30%, and the “thrust trap” phenomenon cannot be effectively suppressed. When the operating speed range of the turbine engine increases from Ma2.5 to 2.9, the TBCC engine’s ramjet size may be improved by 13.3%, the minimum thrust will reach 80%, and the “thrust trap” tends to level off.