Improving the Aerodynamic Performance of WIG Aircraft with a Micro-Vortex Generator (MVG) in Low-Speed Condition
Abstract
1. Introduction
2. Methodology
2.1. Design and Fabrication Process of Fuselage
2.2. Micro-Vortex Generator (MVG) Device
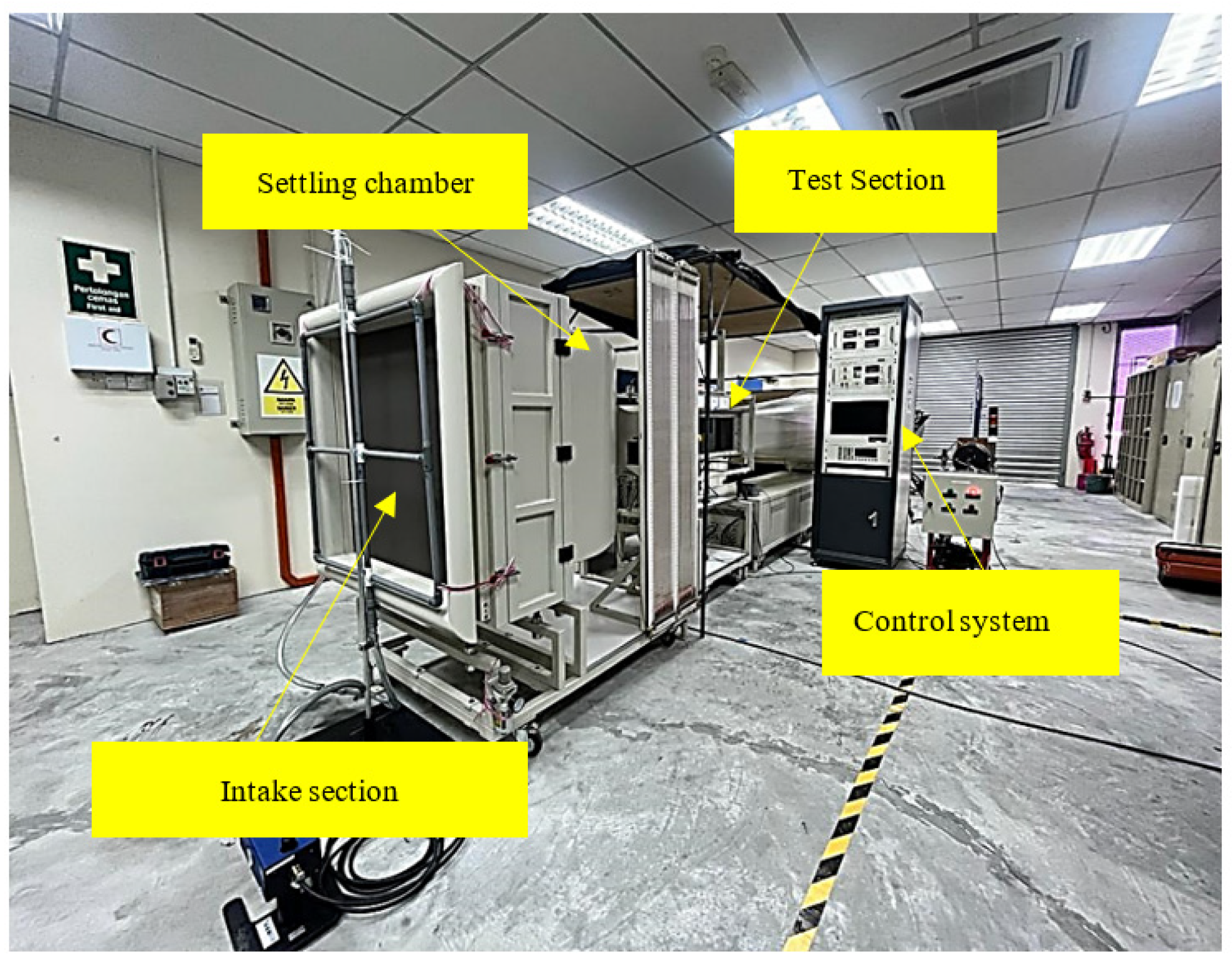
2.3. Experimental Set-Up
2.4. Aerodynamic Performance
2.5. Subsonic Wind Tunnel Facility
3. Results and Discussion
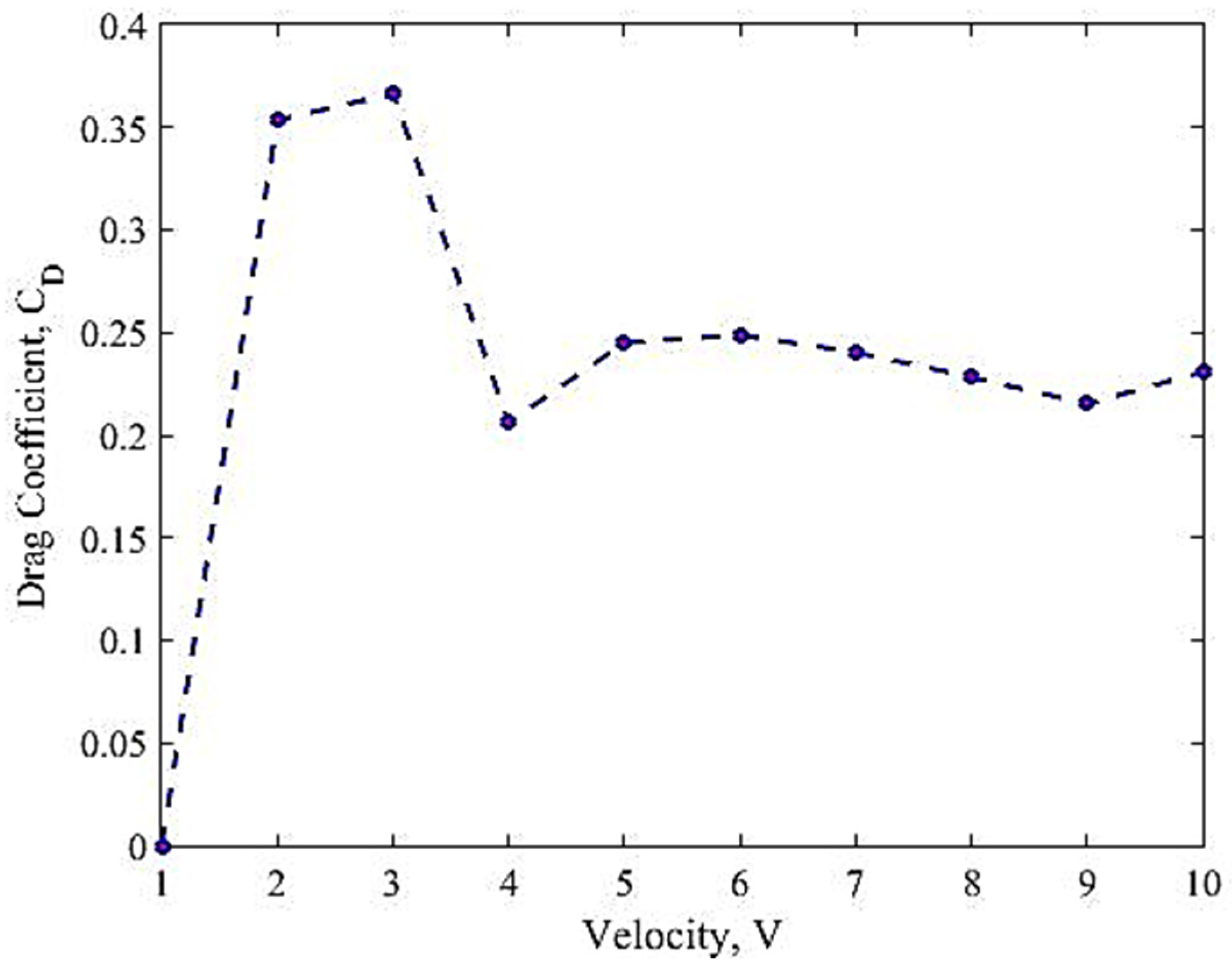
3.1. Uncontrolled Case of Aerodynamic Performance
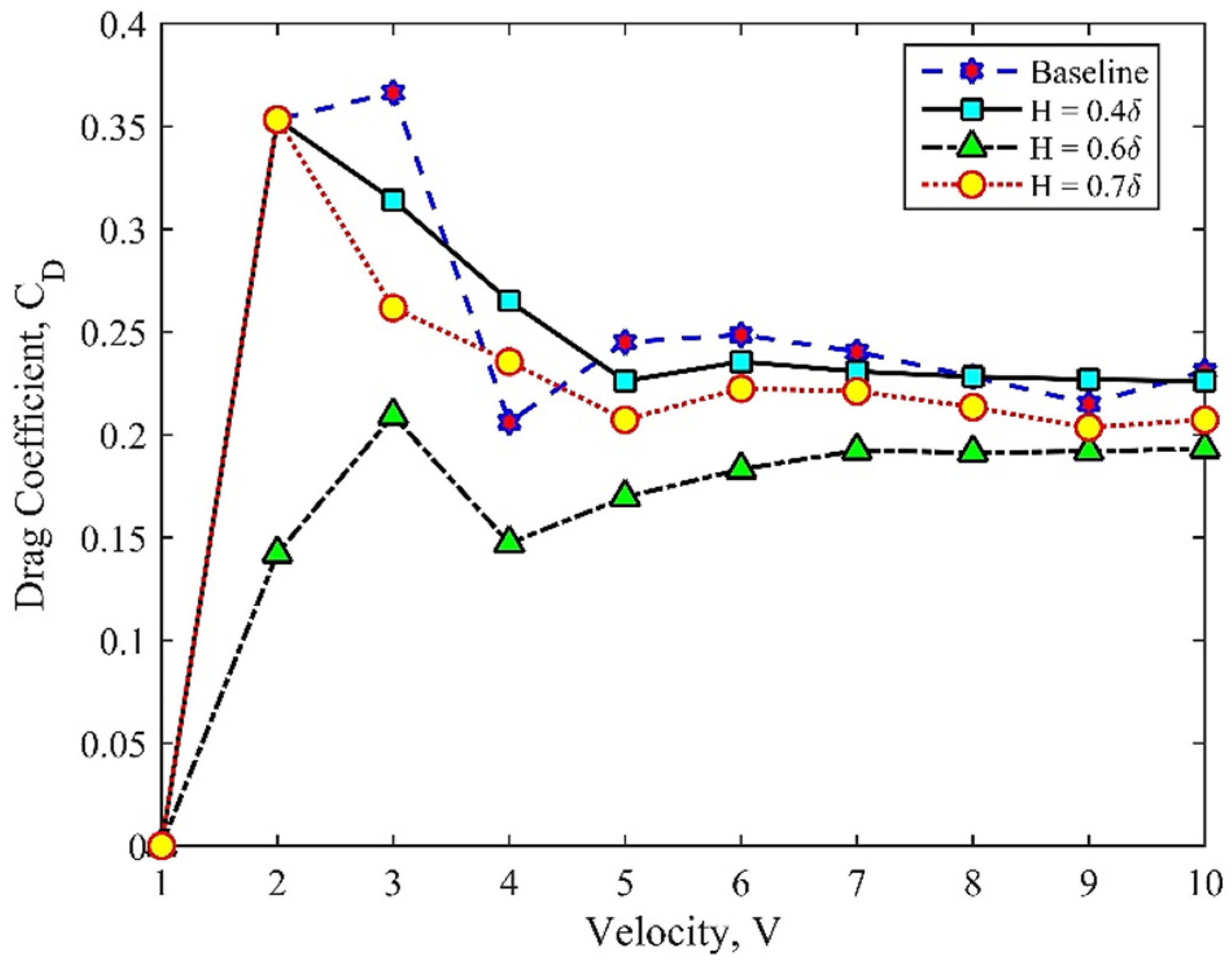
3.2. Effect of Micro-Ramp Angle towards Aerodynamic Performance
3.3. Effect of Micro-Ramp Height towards Aerodynamic Performance
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Halloran, M.; O’Meara, S. Wing in Ground Effect Craft Review; DSTO-GD-02; Defence Science and Technology Organisation: Canberra, Australia, 1999; p. 87. [Google Scholar]
- Mohamed, M.; Amin, I. Effect of Wing Geometrical Parameters on the Aerodynamic Performance of Wing in Ground Marine Craft. In Proceedings of the 3rd International Conference on Maritime Technology and Engineering, MARTECH 2016, Lisbon, Portugal, 4–6 July 2016; Volume 1, pp. 347–352. [Google Scholar] [CrossRef]
- Rozhdestvensky, K.V. Wing-in-Ground Effect Vehicles. Prog. Aerosp. Sci. 2006, 42, 211–283. [Google Scholar] [CrossRef]
- Knyazhskiy, A.; Nebylov, A.; Nebylov, V. Metho Ds for Signal Processing and Motion Control of Ground Effect Vehicle. In Proceedings of the 2017 IEEE International Workshop on Metrology for AeroSpace (MetroAeroSpace), Padua, Italy, 21–23 June 2017; pp. 307–311. [Google Scholar] [CrossRef]
- Syahin, A.; Zinnyrah, M.; Azfar, N.; Said, I.; Che Idris, A.; Rahman, M.; Saad, M. Effect of Micro-Ramp Vortex Generator in Improving Aerodynamics Performance of Wing-in-Ground Craft Fuselage. PERINTIS eJournal 2021, 11, 61–69. [Google Scholar]
- Said, I.; Abdul Rahman, M.R.; Che Idris, A.; Mohd Sakri, F.; Saad, M.R. The Effect of Flow Control on Wing-in-Ground Craft Hull-Fuselage for Improved Aerodynamics Performance. In Proceedings of the International Conference of Aerospace and Mechanical Engineering 2019: AeroMech 2019, Gelugor, Malaysia, 20–21 November 2019; Lecture Notes in Mechanical Engineering. Springer: Singapore; pp. 501–510. [Google Scholar] [CrossRef]
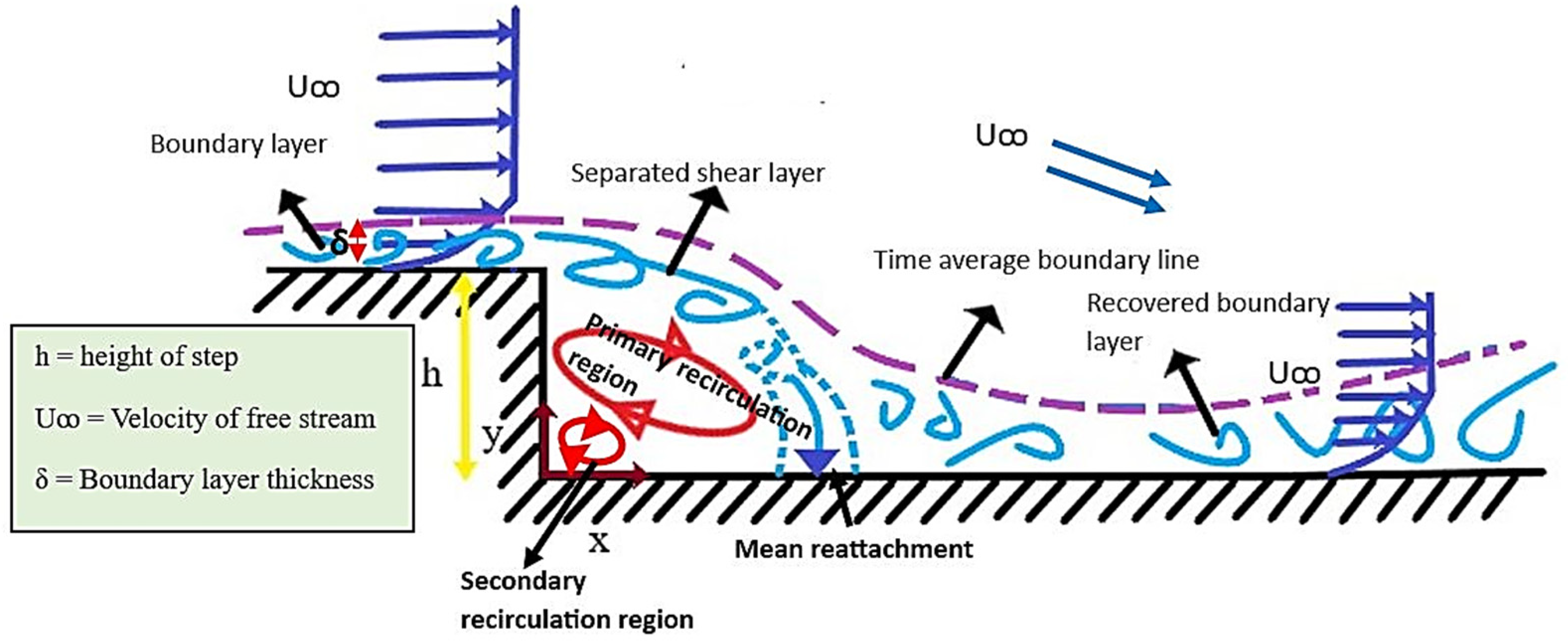
- Chen, L.; Asai, K.; Nonomura, T.; Xi, G.; Liu, T. A Review of Backward-Facing Step (BFS) Flow Mechanisms, Heat Transfer and Control. Therm. Sci. Eng. Prog. 2018, 6, 194–216. [Google Scholar] [CrossRef]
- Pont-Vílchez, A.; Trias, F.X.; Gorobets, A.; Oliva, A. Direct numerical simulation of backward-facing step flow at Reτ = 395 and expansion ratio. J. Fluid Mech. 2018, 863, 341–363. [Google Scholar] [CrossRef]
- Wang, F.; Gao, A.; Wu, S.; Zhu, S.; Dai, J.; Liao, Q. Experimental Investigation of Coherent Vortex Structures in a Backward-Facing Step Flow. Water 2019, 11, 2629. [Google Scholar] [CrossRef]
- Guo, G.M.; Liu, H.; Zhang, B. Numerical Study of Active Flow Control over a Hypersonic Backward-Facing Step Using Supersonic Jet in near Space. Acta Astronaut. 2017, 132, 256–267. [Google Scholar] [CrossRef]
- Pouryoussefi, S.G.; Mirzaei, M.; Hajipour, M. Experimental Study of Separation Bubble Control behind a Backward-Facing Step Using Plasma Actuators. Acta Mech. 2015, 226, 1153–1165. [Google Scholar] [CrossRef]
- Huang, W.; Yan, L. Numerical Investigation on the Ram-Scram Transition Mechanism in a Strut-Based Dual-Mode Scramjet Combustor. Int. J. Hydrogen Energy 2016, 41, 4799–4807. [Google Scholar] [CrossRef]
- Grandemange, M.; Cadot, O.; Courbois, A.; Herbert, V.; Ricot, D.; Ruiz, T.; Vigneron, R. A Study of Wake Effects on the Drag of Ahmed’s Squareback Model at the Industrial Scale. J. Wind Eng. Ind. Aerodyn. 2015, 145, 282–291. [Google Scholar] [CrossRef]
- Li, C.; Chen, X.; Li, Y.; Musa, O.; Zhu, L.; Li, W. Role of the Backward-Facing Steps at Two Struts on Mixing and Combustion Characteristics in a Typical Strut-Based Scramjet with Hydrogen Fuel. Int. J. Hydrogen Energy 2019, 44, 28371–28387. [Google Scholar] [CrossRef]
- D’Adamo, J.; Sosa, R.; Artana, G. Active Control of a Backward Facing Step Flow with Plasma Actuators. J. Fluids Eng. Trans. ASME 2014, 136, 121105. [Google Scholar] [CrossRef]
- Fatahian, E.; Lohrasbi Nichkoohi, A.; Salarian, H.; Khaleghinia, J. Comparative Study of Flow Separation Control Using Suction and Blowing over an Airfoil with/without Flap. Sadhana Acad. Proc. Eng. Sci. 2019, 44, 220. [Google Scholar] [CrossRef]
- Abed, N.K. Flow Separation Control of Backward-Facing Step Airfoil NACA0015 by Blowing Technique. DJES 2019, 12, 99–119. [Google Scholar] [CrossRef]
- Zhang, Z.; Li, D.; Ming, X. Active Control of Flow over Backward Facing Step by Synthetic Jets. In Proceedings of the 32nd AIAA Applied Aerodynamics Conference, Atlanta, GA, USA, 16–20 June 2014; pp. 1–10. [Google Scholar] [CrossRef]
- Dandois, J.; Garnier, E.; Sagaut, P. Numerical Simulation of Active Separation Control by a Synthetic Jet. J. Fluid Mech. 2007, 574, 25–58. [Google Scholar] [CrossRef]
- Ruisi, R.; Zare-Behtash, H.; Kontis, K.; Erfani, R. Active Flow Control over a Backward-Facing Step Using Plasma Actuation. Acta Astronaut. 2016, 126, 354–363. [Google Scholar] [CrossRef]
- Xu, H.Y.; Xing, S.L.; Ye, Z.Y. Numerical Study of the S809 Airfoil Aerodynamic Performance Using a Co-Flow Jet Active Control Concept. J. Renew. Sustain. Energy 2015, 7, 023131. [Google Scholar] [CrossRef]
- Zhu, Y.; Yi, S.; Ding, H.; Nie, W.; Zhang, Z. Structures and Aero-Optical Effects of Supersonic Flow over a Backward Facing Step with Vortex Generators. Eur. J. Mech. B Fluids 2019, 74, 302–311. [Google Scholar] [CrossRef]
- Lo, K.H.; Zare-Behtash, H.; Kontis, K. Control of Flow Separation on a Contour Bump by Jets in a Mach 1.9 Free-Stream: An Experimental Study. Acta Astronaut. 2016, 126, 229–242. [Google Scholar] [CrossRef]
- Amit, A.; Saraf, K.; Mahendra, B.; Singh, P.; Tej, C.; Chouhanr, S. Study of Flow Separation on Airfoil with Bump. Int. J. Appl. Eng. Res. 2018, 13, 12868–12872. [Google Scholar]
- Ramzi, M. Numerical Study of Long Separation Bubble on Slotted Thick Airfoil. PAMM 2018, 18, 98–99. [Google Scholar] [CrossRef]
- Shi, X.; Xu, S.; Ding, L.; Huang, D. Passive Flow Control of a Stalled Airfoil Using an Oscillating Micro-Cylinder. Comput. Fluids 2019, 178, 152–165. [Google Scholar] [CrossRef]
- Udroiu, R. New Methodology for Evaluating Surface Quality of Experimental Aerodynamic Models Manufactured by Polymer Jetting Additive Manufacturing. Polymers 2022, 14, 371. [Google Scholar] [CrossRef]
- Dong, X.; Chen, Y.; Dong, G.; Liu, Y. Study on Wake Structure Characteristics of a Slotted Micro-Ramp with Large-Eddy Simulation. Fluid Dyn. Res. 2017, 49, 035507. [Google Scholar] [CrossRef]
- Sun, Z. Micro Vortex Generators for Boundary Layer Control: Principles and Applications. Int. J. Flow Control 2015, 7, 67–86. [Google Scholar] [CrossRef]
- Ye, Q.; Schrijer, F.F.J.; Scarano, F. Boundary Layer Transition Mechanisms behind a Micro-Ramp. J. Fluid Mech. 2016, 793, 132–161. [Google Scholar] [CrossRef]
- Ford, C.W.P.; Babinsky, H. Micro-Ramp Control for Oblique Shock Wave/Boundary Layer Interactions. In Proceedings of the 37th AIAA Fluid Dynamics Conference and Exhibit, Miami, FL, USA, 25–27 June 2007; Volume 2, pp. 972–985. [Google Scholar] [CrossRef]
- Li, X.K.; Liu, W.; Zhang, T.J.; Wang, P.M.; Wang, X.D. Experimental and Numerical Analysis of the Effect of Vortex Generator Installation Angle on Flow Separation Control. Energies 2019, 12, 4583. [Google Scholar] [CrossRef]
- Fouatih, O.M.; Medale, M.; Imine, O.; Imine, B. Design Optimization of the Aerodynamic Passive Flow Control on NACA 4415 Airfoil Using Vortex Generators. Eur. J. Mech. B Fluids 2016, 56, 82–96. [Google Scholar] [CrossRef]
- Said, I.; Poonaesparan, M.K.; Bohari, B.; Idris, A.C.; Rahman, M.R.A.; Saad, M.R. The Effect of Streamwise Location of Micro Vortex Generator on Airfoil Aerodynamic Performance in Subsonic Flow. J. Aeronaut. Astronaut. Aviat. 2021, 53, 173–178. [Google Scholar] [CrossRef]
- Mushyam, A.; Bergada, J.M. Active Flow Control on Laminar Flow over a Backward Facing Step. J. Phys. Conf. Ser. 2015, 633, 012110. [Google Scholar] [CrossRef]
- van Sluis, M.; Nasrollahi, S.; Rao, A.G.; Eitelberg, G. Experimental and Numerical Analyses of a Novel Wing-In-Ground Vehicle. Energies 2022, 15, 1497. [Google Scholar] [CrossRef]
- He, W.; Yu, P.; Li, L.K.B. Ground Effects on the Stability of Separated Flow around a NACA 4415 Airfoil at Low Reynolds Numbers. Aerosp. Sci. Technol. 2018, 72, 63–76. [Google Scholar] [CrossRef]
- Kosasih, B.; Saleh Hudin, H. Influence of Inflow Turbulence Intensity on the Performance of Bare and Diffuser-Augmented Micro Wind Turbine Model. Renew. Energy 2016, 87, 154–167. [Google Scholar] [CrossRef]
- Yang, Z.; Haan, F.L.; Hu, H.; Ma, H. An Experimental Investigation on the Flow Separation on a Low-Reynolds-Number Airfoil. In Proceedings of the 45th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 8–11 January 2007; Volume 5, pp. 3421–3431. [Google Scholar] [CrossRef]
- Jehad, D.G.; Hashim, G.A.; Zarzoor, A.K.; Azwadi, C.S.N. Numerical Study of Turbulent Flow over Backward-Facing Step with Different Turbulence Models. Adv. Res. Des. 2015, 4, 20–27. [Google Scholar]
- Hilo, A.K.; Abu Talib, A.R.; Acosta Iborra, A.; Hameed Sultan, M.T.; Abdul Hamid, M.F. Effect of Corrugated Wall Combined with Backward-Facing Step Channel on Fluid Flow and Heat Transfer. Energy 2020, 190, 116294. [Google Scholar] [CrossRef]
- Giepman, R.H.M.; Schrijer, F.F.J.; Van Oudheusden, B.W. Flow Control of an Oblique Shock Wave Reflection with Micro-Ramp Vortex Generators: Effects of Location and Size. Phys. Fluids 2014, 26, 066101. [Google Scholar] [CrossRef]







| Parameter | Width, d (mm) | Length, ℓ (mm) | Height, h (mm) | Spacing, λ (mm) | Angle, β (°) |
|---|---|---|---|---|---|
| Measurement | 3.6δ | 4.5δ | 0.8δ | 4.7δ | 48 |
| Parameter | Ramp Angle, α(°) | h (mm) | ||||
|---|---|---|---|---|---|---|
| Ramp-type VG | 12 | 16 | 24 | 0.4 δ | 0.6 δ | 0.8 δ |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Methal, Z.; Talib, A.S.A.; Bakar, M.S.A.; Rahman, M.R.A.; Sulaiman, M.S.; Saad, M.R. Improving the Aerodynamic Performance of WIG Aircraft with a Micro-Vortex Generator (MVG) in Low-Speed Condition. Aerospace 2023, 10, 617. https://doi.org/10.3390/aerospace10070617
Methal Z, Talib ASA, Bakar MSA, Rahman MRA, Sulaiman MS, Saad MR. Improving the Aerodynamic Performance of WIG Aircraft with a Micro-Vortex Generator (MVG) in Low-Speed Condition. Aerospace. 2023; 10(7):617. https://doi.org/10.3390/aerospace10070617
Chicago/Turabian StyleMethal, Zinnyrah, Ahmad Syahin Abu Talib, Mohd Supian Abu Bakar, Mohd Rosdzimin Abdul Rahman, Mohamad Syafiq Sulaiman, and Mohd Rashdan Saad. 2023. "Improving the Aerodynamic Performance of WIG Aircraft with a Micro-Vortex Generator (MVG) in Low-Speed Condition" Aerospace 10, no. 7: 617. https://doi.org/10.3390/aerospace10070617
APA StyleMethal, Z., Talib, A. S. A., Bakar, M. S. A., Rahman, M. R. A., Sulaiman, M. S., & Saad, M. R. (2023). Improving the Aerodynamic Performance of WIG Aircraft with a Micro-Vortex Generator (MVG) in Low-Speed Condition. Aerospace, 10(7), 617. https://doi.org/10.3390/aerospace10070617








