Integrated Flight Control System Characterization Approach for Civil High-Speed Vehicles in Conceptual Design
Abstract
1. Introduction
2. Studies on Flight Control System Design for High-Speed Vehicles
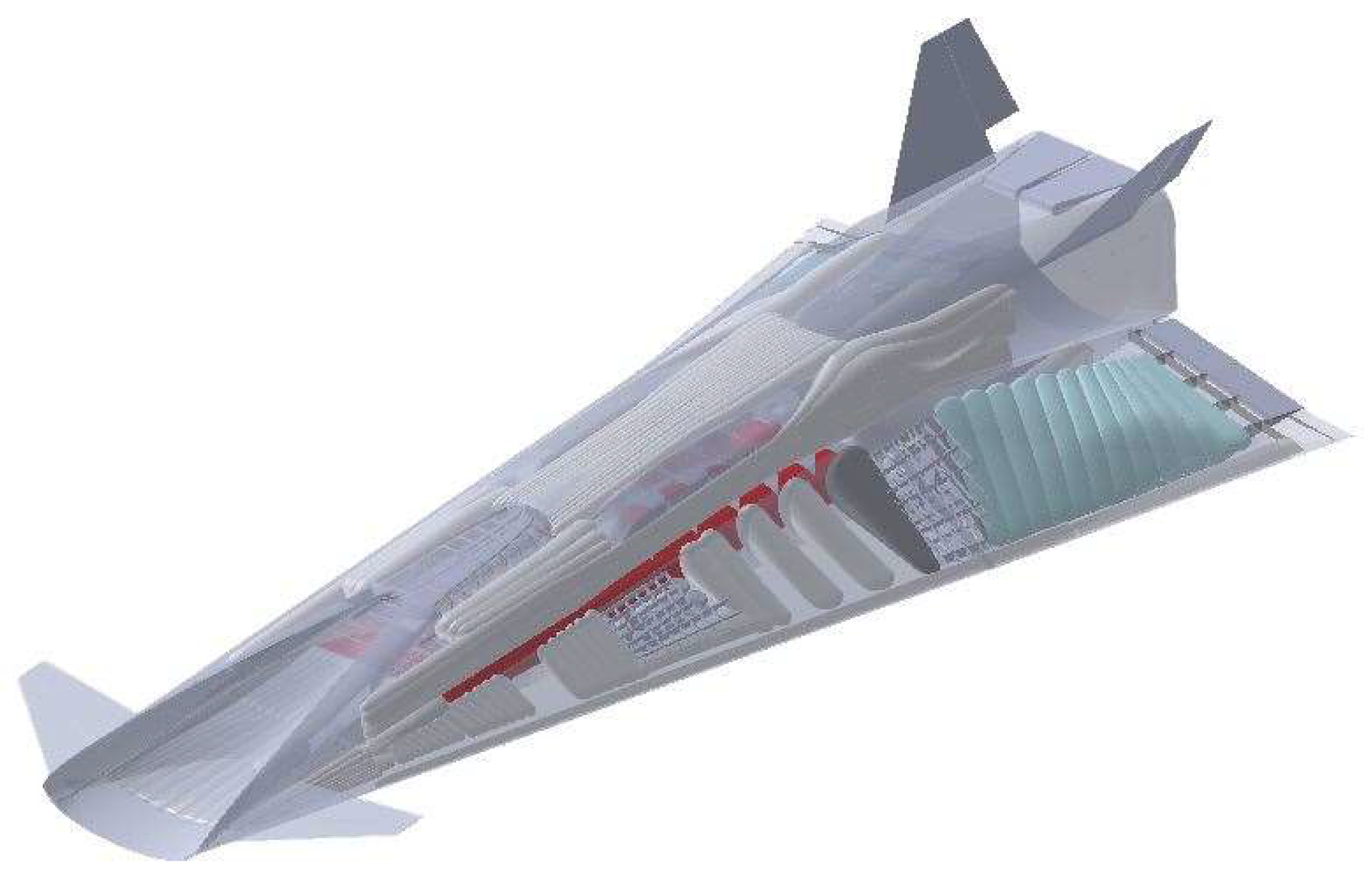
3. STRATOFLY MR3 Vehicle and Mission Concepts
4. Flight Control System Integrated Design Methodology
4.1. Methodology Overview
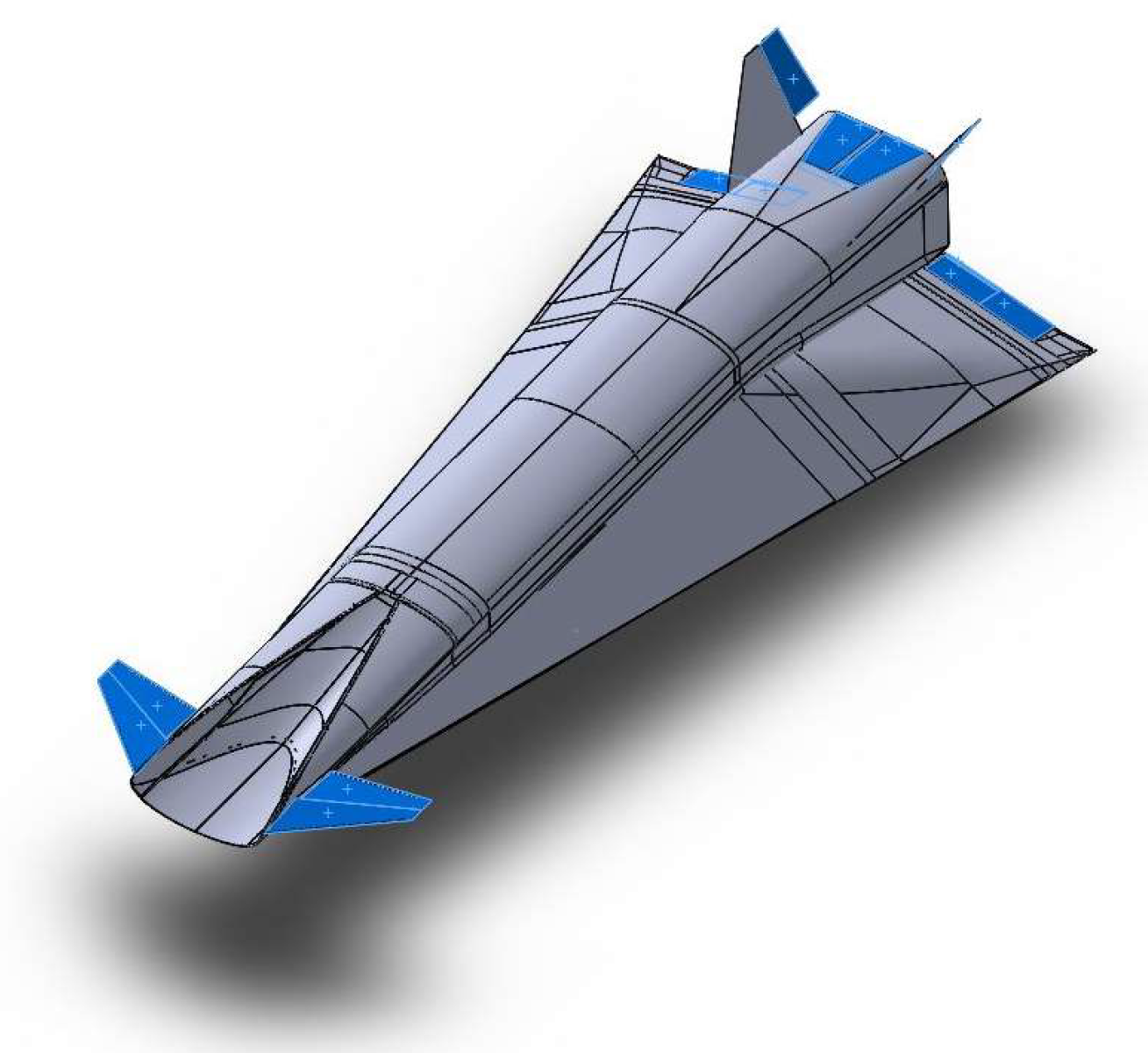
4.2. Step 1: Geometrical Definition of Flight Control Surfaces
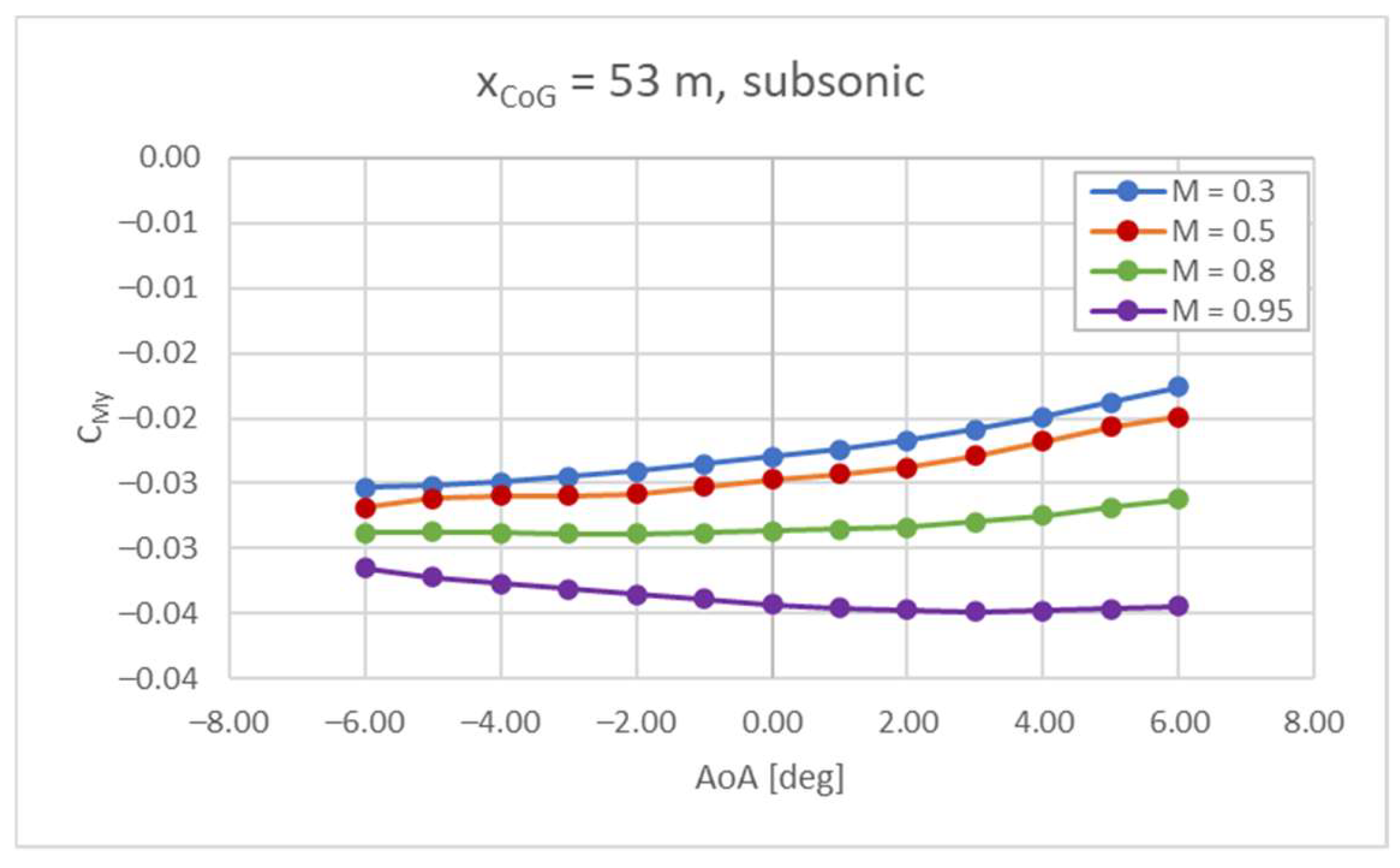
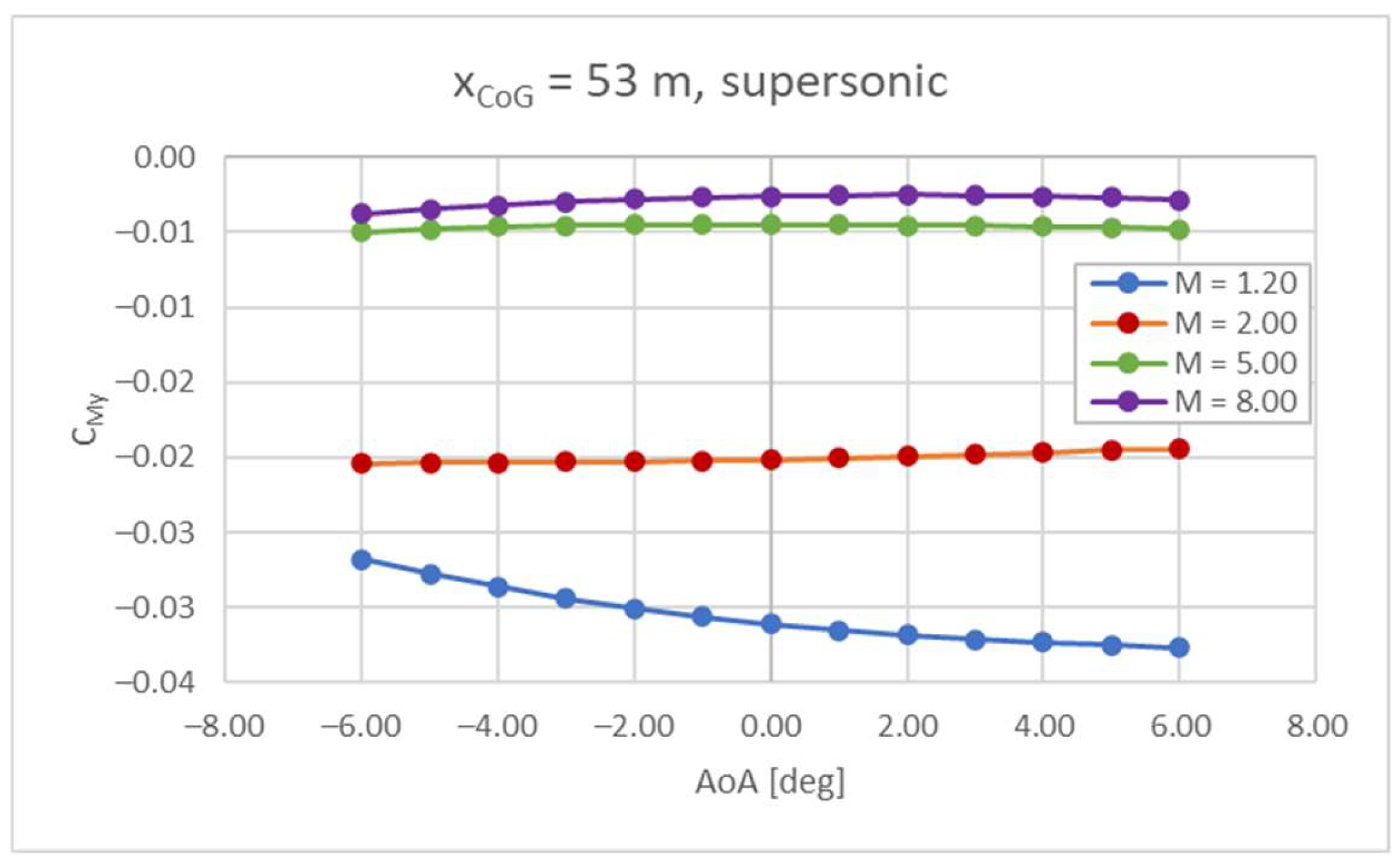
4.3. Step 2: Stability Analysis for the Clean Configuration
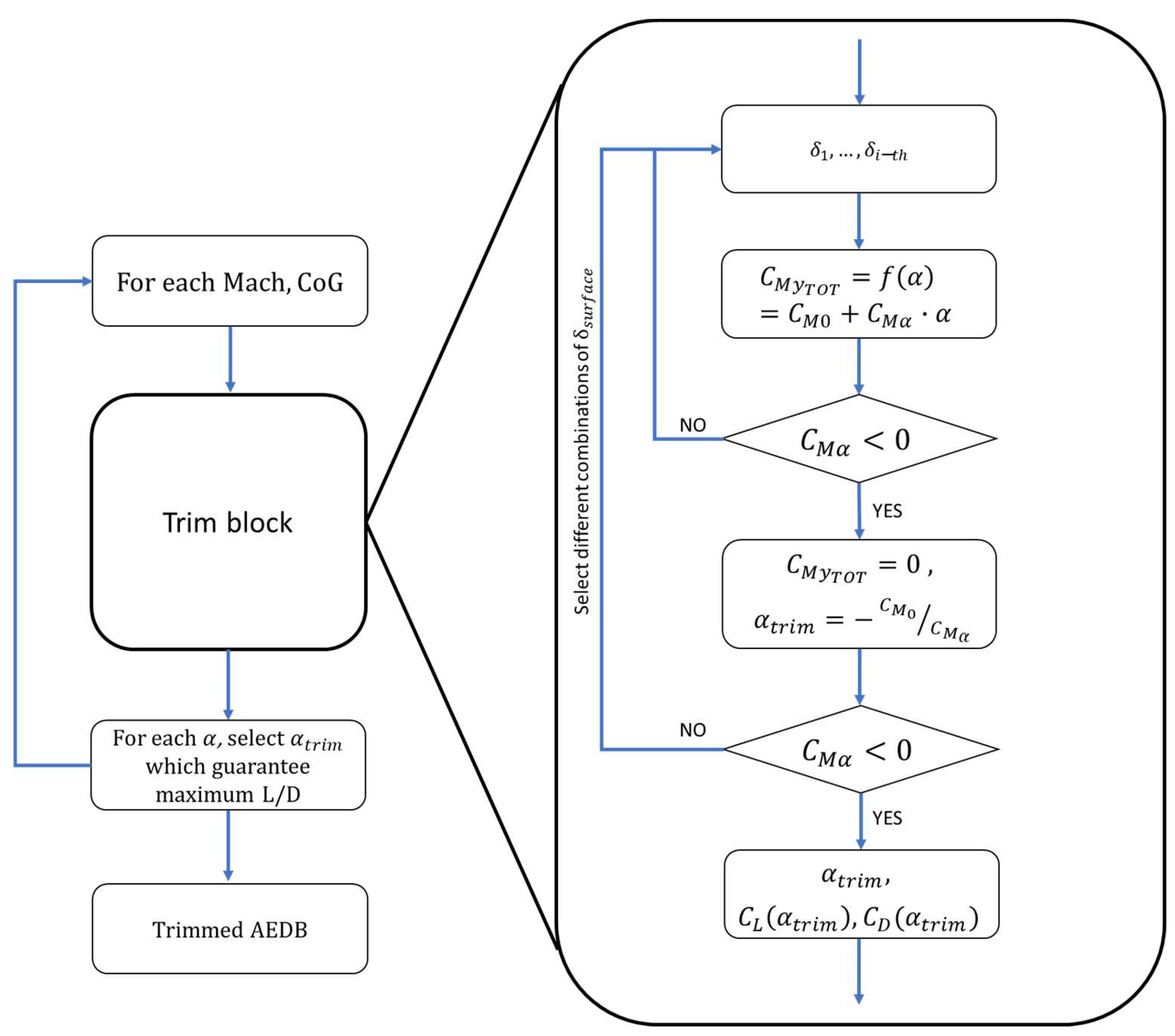
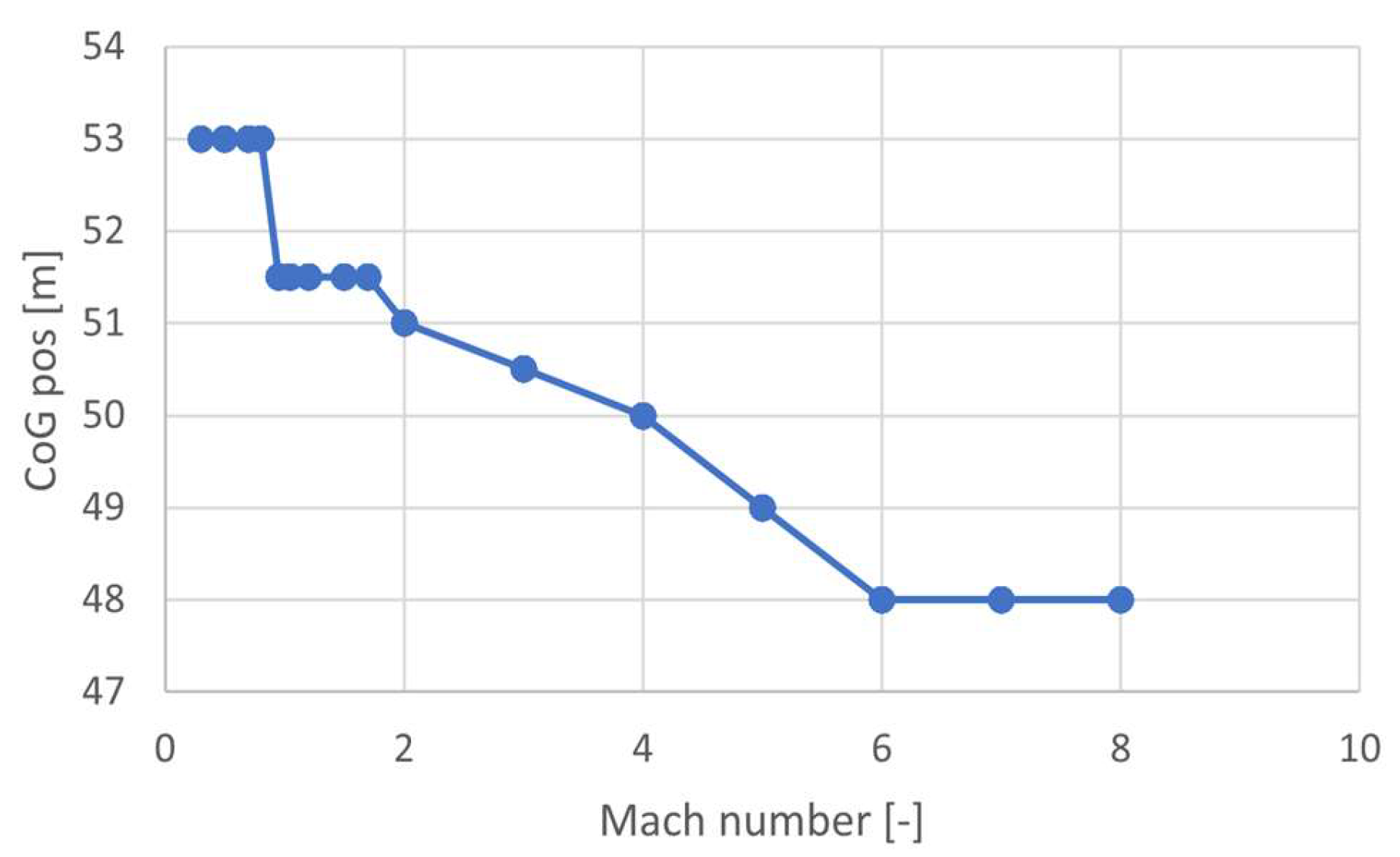
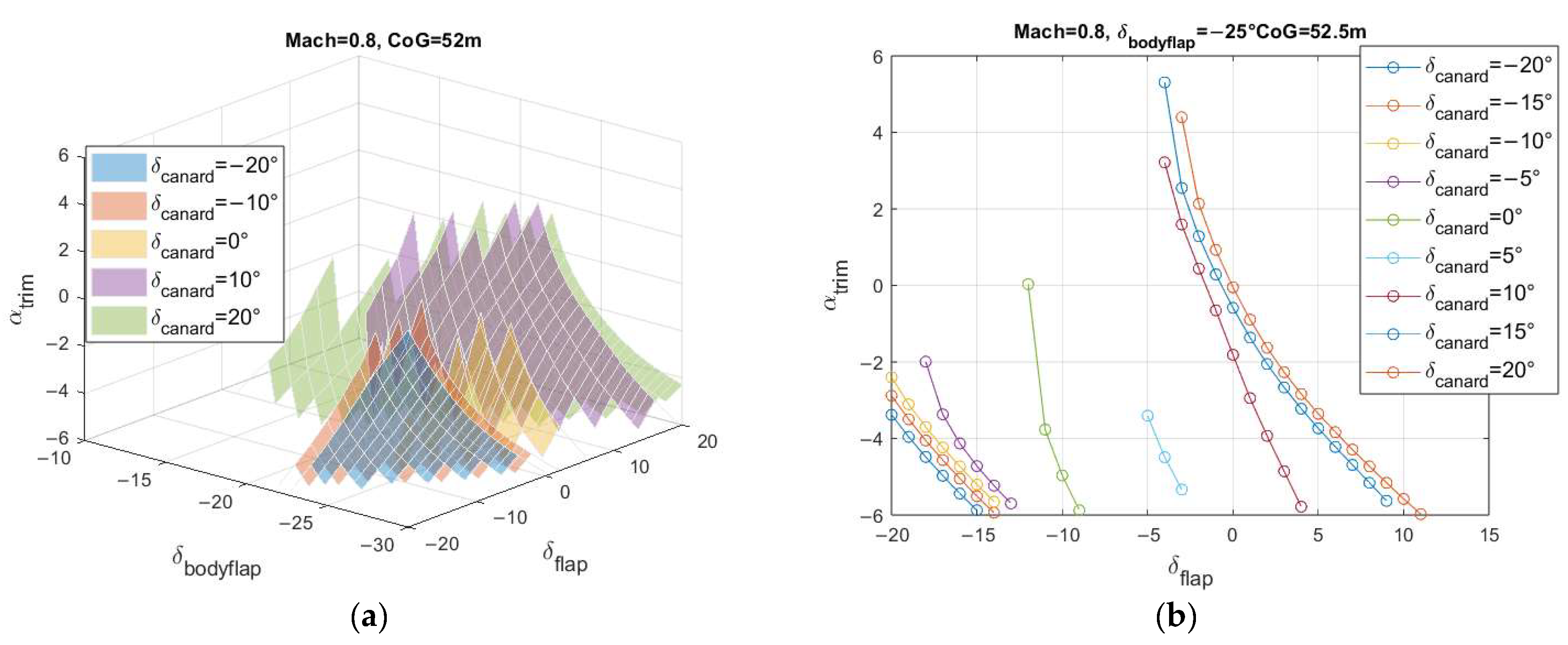
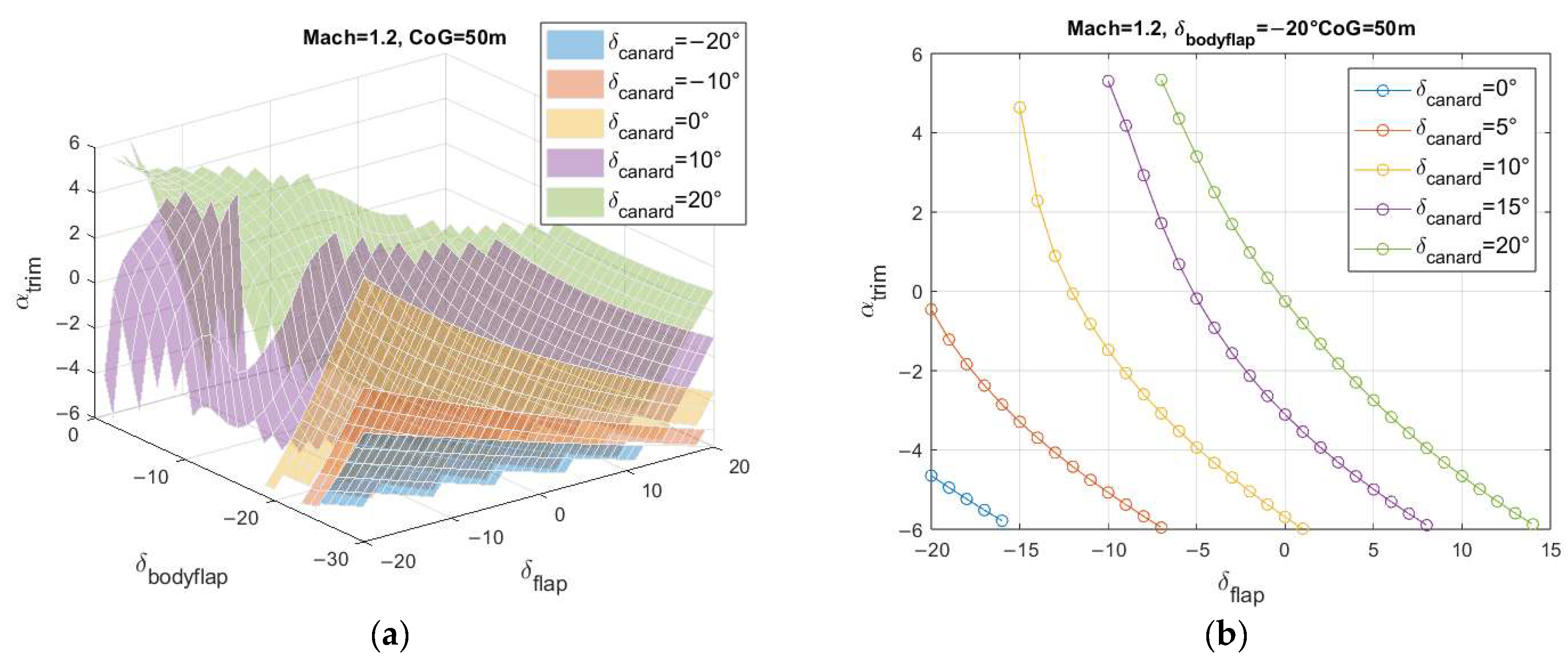
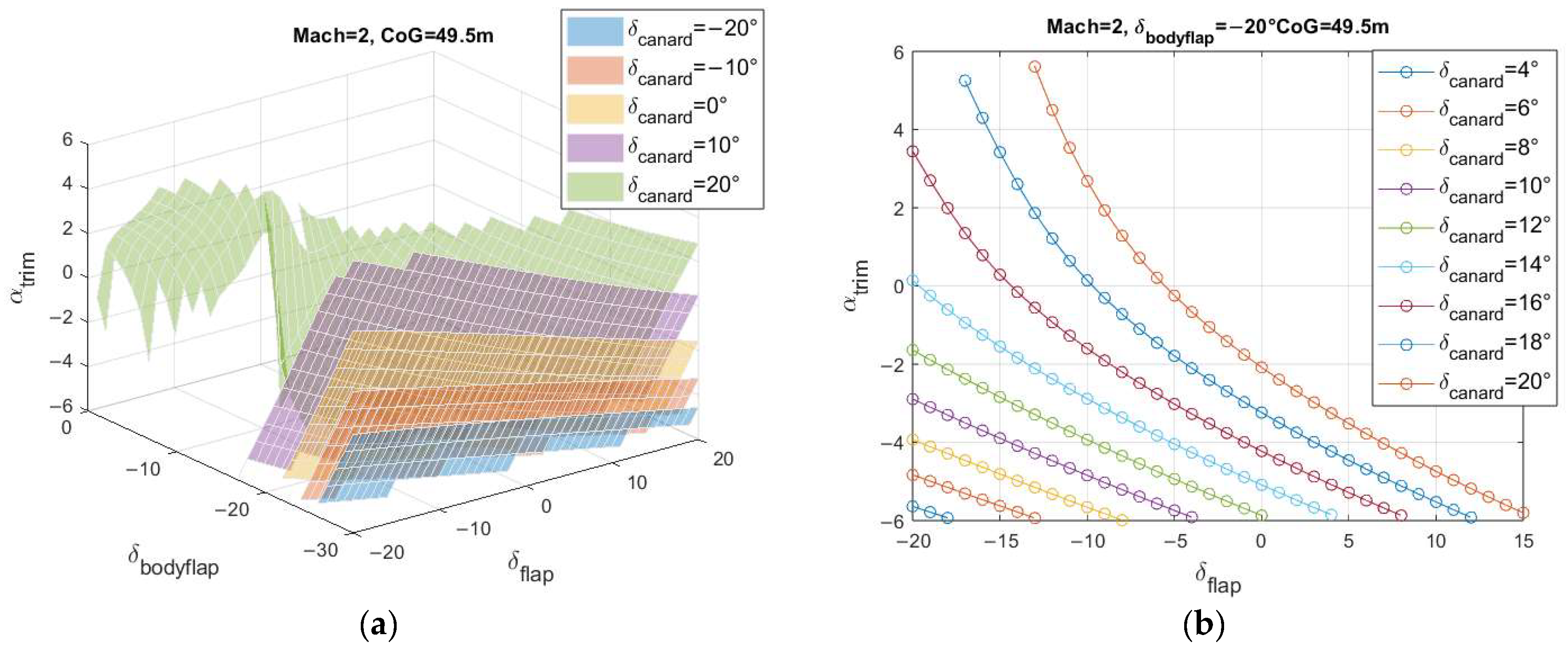
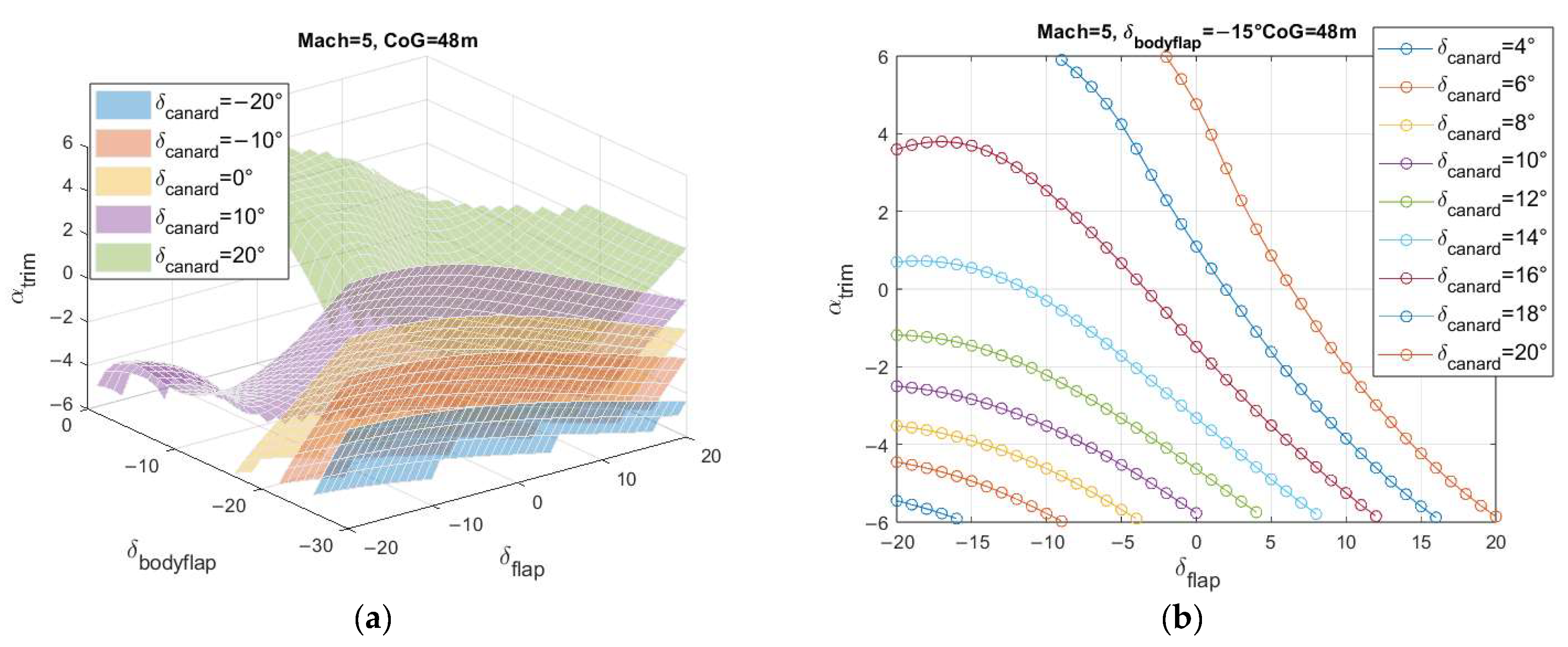
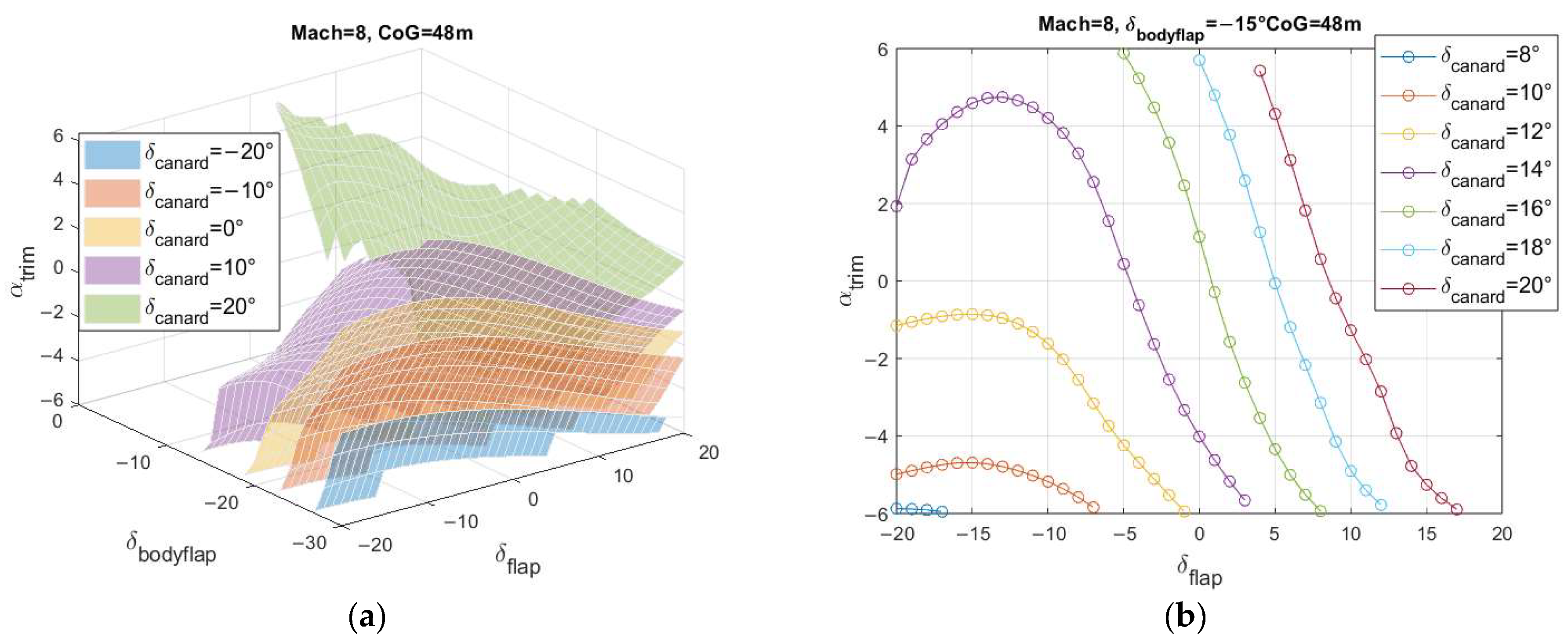
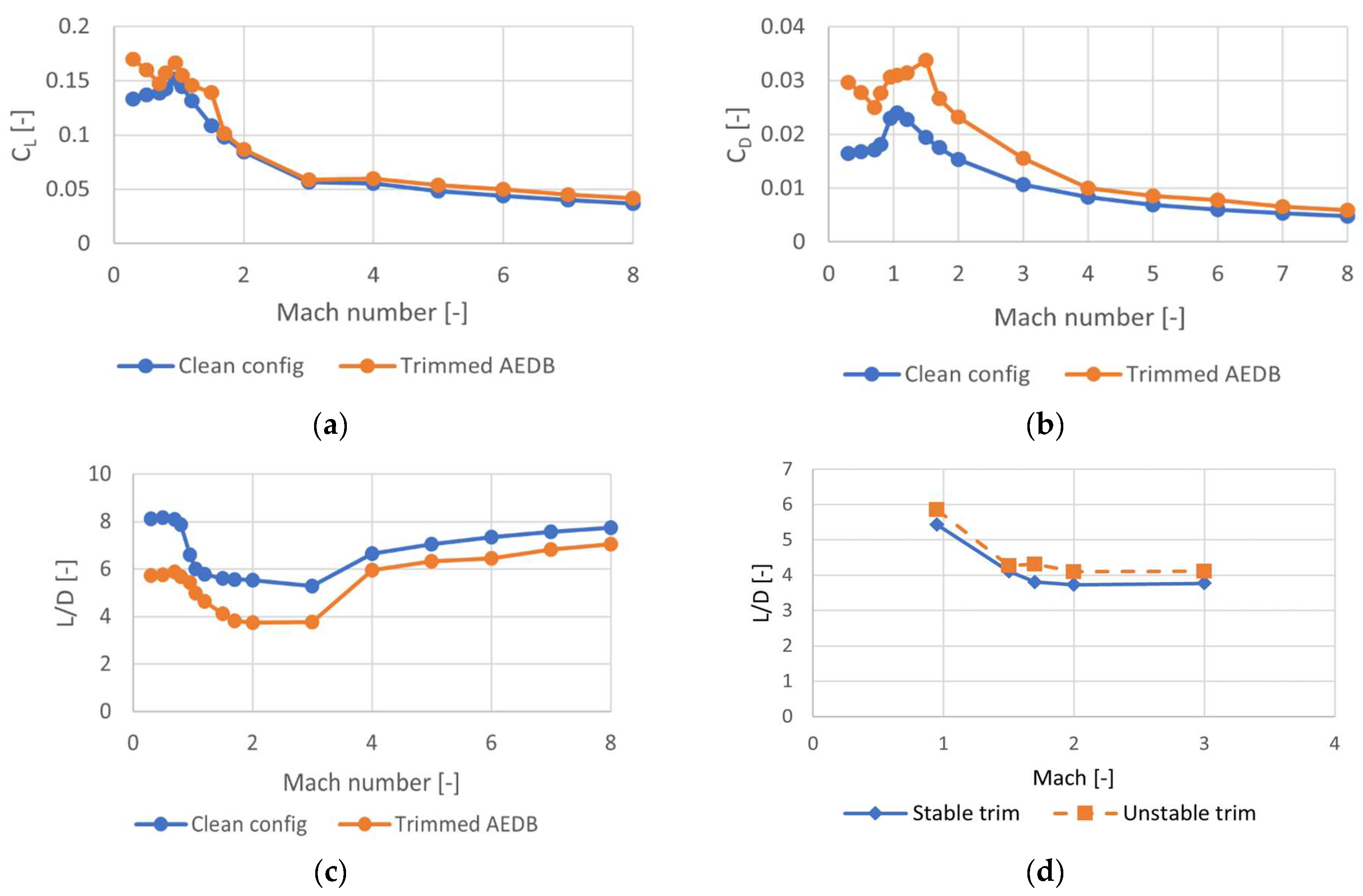
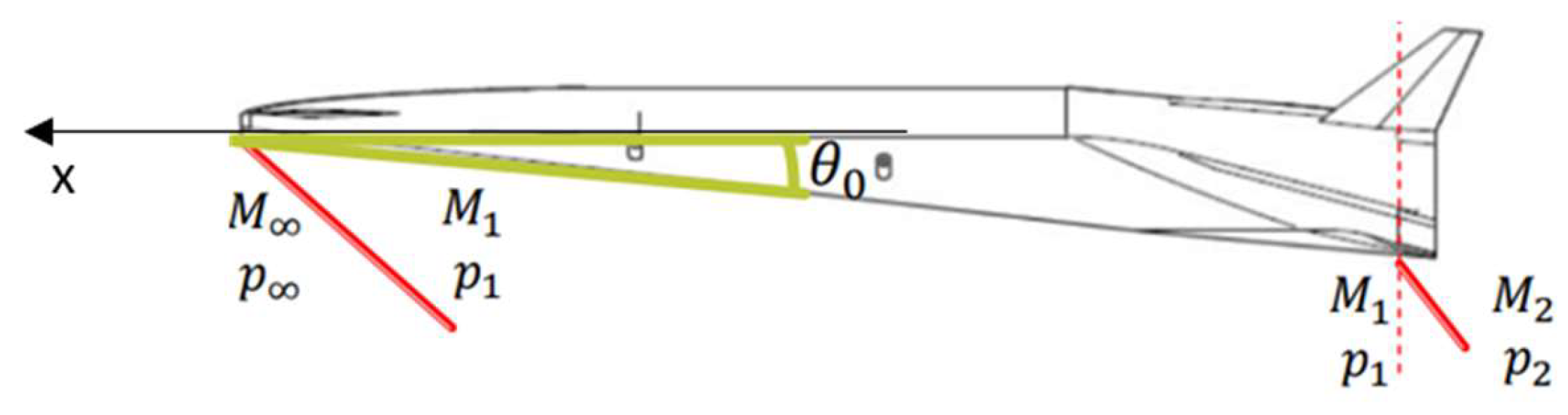
4.4. Step 3: Aerodynamic Characterization of Control Surfaces and Trim Analysis
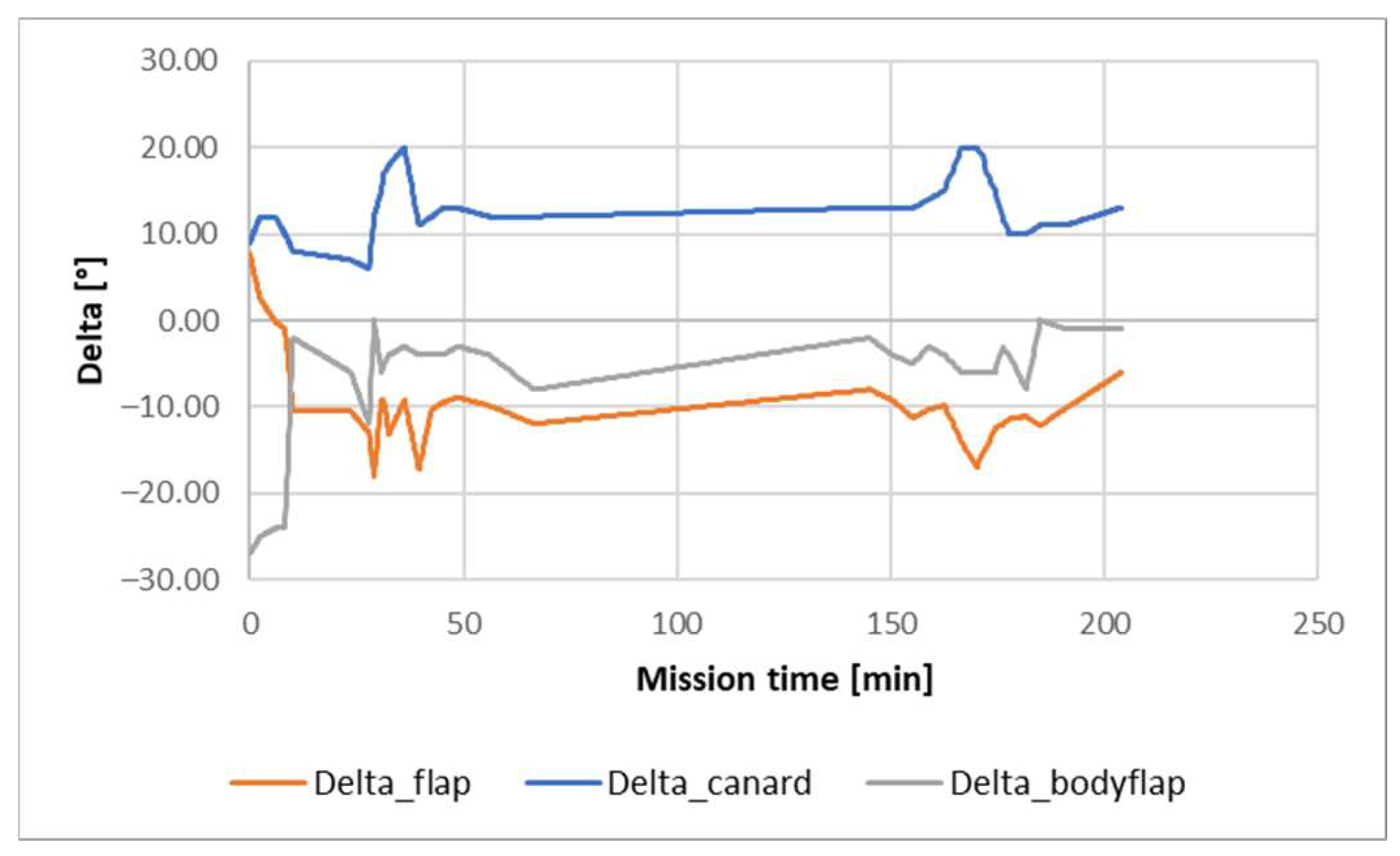
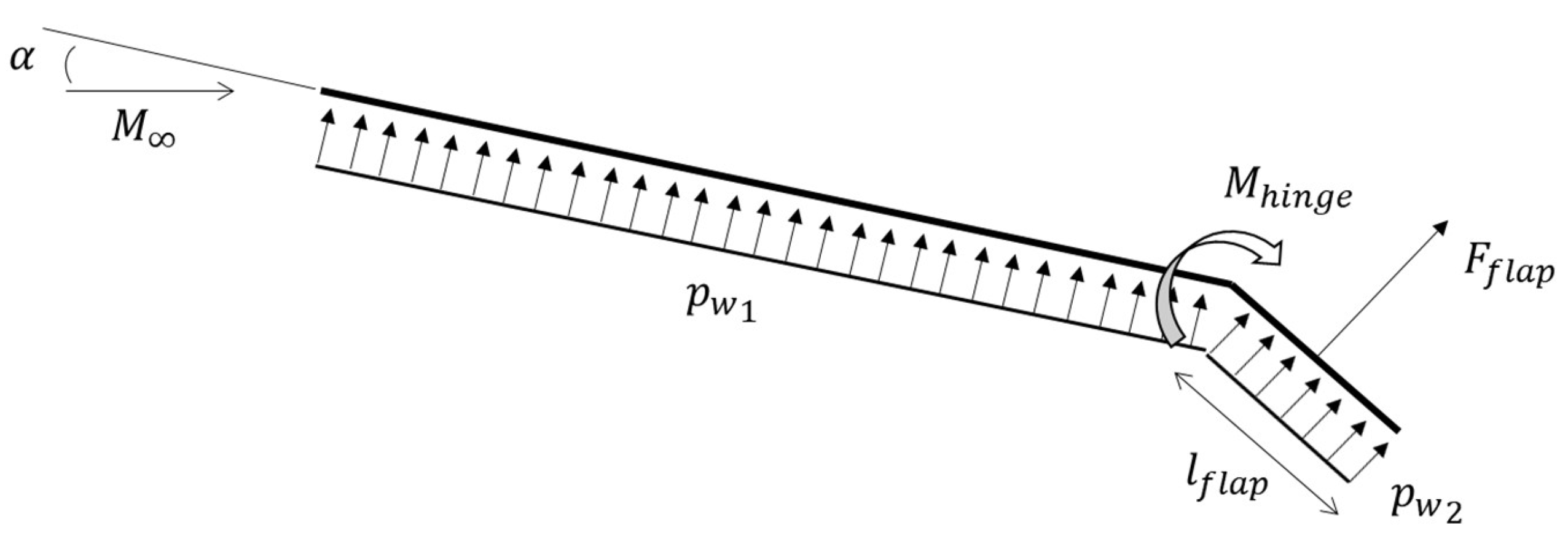
4.5. Step 4: Evaluation of Hinge Moment
4.6. Step 5: Detailed Estimation of Power Demand
5. Conclusions and Future Works
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| AoA | Angle of Attack |
| ATR | Air Turbo Rocket |
| CoG | Center of Gravity |
| DMR | Dual Mode Ramjet |
| EIS | Entry Into Service |
| FCS | Flight Control System |
| L/D | Lift-to-Drag ratio |
| MTOM | Maximum Take-Off Mass |
| selected mobile surface length [m] | |
| static pressure [Pa] | |
| static pressure on selected mobile surface [Pa] | |
| selected mobile surface width [m] | |
| drag coefficient | |
| lift coefficient | |
| hinge moment coefficient | |
| hinge moment coefficient at angle of attack equal to zero | |
| pitching moment coefficient for angle of attack equal to zero | |
| global pitching moment coefficient | |
| contribution to pitching moment coefficient due to elevons | |
| contribution to pitching moment coefficient due to canard | |
| contribution to pitching moment coefficient due to body flap | |
| contribution to pitching moment coefficient due to thrust | |
| global pitching moment coefficient for clean aircraft configuration only | |
| contribution to pitching moment coefficient due to angle of attack | |
| force acting on the selected mobile surface [N] | |
| moment generated by the actuator [Nm] | |
| hinge moment [Nm] | |
| Mach number | |
| P | power demand to the actuator [W] |
| angle of attack | |
| oblique shock wave angle | |
| ratio of specific heats of air | |
| deflection angle of the control surface | |
| efficiency of the transmission | |
| wedge angle of the lower part of the vehicle | |
| angular speed of the control surface [rad/s] | |
| variation of drag coefficient due to control surfaces | |
| variation of lift coefficient due to control surfaces | |
| global variation of pitching moment coefficient | |
| i-th effect of control surfaces on global pitching moment coefficient | |
| effect of thrust vector on global pitching moment coefficient |
References
- Andro, J.-Y.; Rotärmel, W.; Nebula, F.; Morani, G.; Steelant, J. Design of the Actuation System for the Hexafly-Int Hypersonic Glider. In Proceedings of the 1st International Conference on High-Speed Vehicle Science and Technology, Moscow, Russia, 26–29 November 2018. [Google Scholar]
- Viola, N.; Fusaro, R.; Gori, O.; Marini, M.; Roncioni, P.; Saccone, G.; Saracoglu, B.; Ispir, A.C.; Fureby, C.; Nilson, T.; et al. STRATOFLY MR3—How to reduce the environmental impact of high-speed transportation. In Proceedings of the AIAA Scitech 2021 Forum, Online, 11–15 and 19–21 January 2021. [Google Scholar] [CrossRef]
- Rufolo, G.C.; Roncioni, P.; Marini, M.; Votta, R.; Palazzo, S. Experimental and numerical aerodynamic data integration and aerodatabase development for the PRORA-USV-FTB_1 reusable vehicle. In Proceedings of the 14th AIAA/AHI International Space Planes and Hypersonic Systems and Technologies Conference, Canberra, Australia, 6–9 November 2006; pp. 1173–1212. [Google Scholar] [CrossRef]
- Viola, N.; Roncioni, P.; Gori, O.; Fusaro, R. Aerodynamic characterization of hypersonic transportation systems and its impact on mission analysis. Energies 2021, 14, 3580. [Google Scholar] [CrossRef]
- Fusaro, R.; Viola, N.; Ferretto, D.; Vercella, V.; Fernandez Villace, V.; Steelant, J. Life cycle cost estimation for high-speed transportation systems. CEAS Space J. 2020, 12, 213–233. [Google Scholar] [CrossRef]
- Rajaram, D.; Cai, Y.; Chakraborty, I.; Mavris, D.N. Integrated sizing and optimization of aircraft and subsystem architectures in early design. J. Aircr. 2018, 55, 1942–1954. [Google Scholar] [CrossRef]
- Javaid, K.H.; Serghides, V.C. Thrust-matching requirements for the conceptual design of hypersonic waverider vehicles. J. Aircr. 2005, 42, 1055–1064. [Google Scholar] [CrossRef]
- Ingenito, A. Design of Supersonic/Hypersonic Vehicles. In Subsonic Combustion Ramjet Design; Springer Briefs in Applied Science and Technology; Springer: Cham, Switzerland, 2021. [Google Scholar] [CrossRef]
- Ferretto, D.; Fusaro, R.; Viola, N. A conceptual design tool to support high-speed vehicle design. In Proceedings of the AIAA Aviation 2020 Forum, Online, 15–19 June 2020. [Google Scholar] [CrossRef]
- Bowcutt, K.G. Physics drivers of hypersonic vehicle design. In Proceedings of the 22nd AIAA International Space Planes and Hypersonics Systems and Technologies Conference, Orlando, FL, USA, 17–19 September 2018. [Google Scholar] [CrossRef]
- Ingenito, A.; Gulli, S.; Bruno, C.; Colemann, G.; Chudoba, B.; Czysz, P.A. Sizing of a fully integrated hypersonic commercial airliner. J. Aircr. 2011, 48, 2161–2164. [Google Scholar] [CrossRef]
- Roskam, J. Airplane Design; DARcorporation: Lawrence, KS, USA, 1985. [Google Scholar]
- Torenbeek, E. Synthesis of Subsonic Airplane Design: An Introduction to the Preliminary Design of Subsonic General Aviation and Transport Aircraft, with Emphasis on Layout, Aerodynamic Design, Propulsion and Performance; Springer Science & Business Media: Dordrecht, The Netherlands, 2013. [Google Scholar]
- Raymer, D. Aircraft Design: A Conceptual Approach; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2012. [Google Scholar]
- Sadraey, M.H. Aircraft Design: A Systems Engineering Approach; John Wiley & Sons: Hoboken, NJ, USA, 2012. [Google Scholar]
- Sitchin, E.V.; Smith, B.; Stahlschmidt, W.W.; Raz, A.K.; Delaurentis, D.A. Modeling Hypersonic Vehicle Interdependencies at the Subsystem Level. In Proceedings of the AIAA 2021 Aviation Forum, Virtual, 2–6 August 2021. [Google Scholar] [CrossRef]
- Scigliano, R.; Pezzella, G.; Marini, M.; Di Benedetto, S.; Steelant, J. Aerothermal design of the Hexafly-INT glider. In Proceedings of the 2016 AIAA Space and Astronautics Forum, Long Beach, CA, USA, 13–16 September 2016. [Google Scholar] [CrossRef]
- Scigliano, R.; Benedetto, S.D.; Marini, M.; Villace, V.; Steelant, J. Hexafly-INT hypersonic vehicle thermal protection system design. In Proceedings of the 71st International Astronautical Conference, Online, 12–14 October 2020. [Google Scholar]
- Ferretto, D.; Fusaro, R.; Viola, N. Propellant subsystem design for hypersonic cruiser exploiting liquid hydrogen. In Proceedings of the AIAA 2022 Aviation Forum, Chicago, IL, USA, 27 June–1 July 2022. [Google Scholar] [CrossRef]
- Steelant, J.; van Duijn, M. Structural analysis of the LAPCAT-MR2 waverider based vehicle. In Proceedings of the 17th AIAA International Space Planes and Hypersonic Systems and Technologies Conference, San Francisco, CA, USA, 11–14 April 2011. [Google Scholar] [CrossRef]
- Rodriguez-Segade, M.; Hernandez, S.; Diaz, J. Multi-bubble scheme and structural analysis of a hypersonic stratospheric flight vehicle. Aerosp. Sci. Technol. 2022, 124, 107514. [Google Scholar] [CrossRef]
- Ispir, A.C.; Goncalvers, P.M.; Saracoglu, B.H. Analysis of a combined cycle propulsion system for STRATOFLY hypersonic vehicle over and extended trajectory. In Proceedings of the 9th EASN International Conference on “Innovation in Aviation & Space”; MATEC Web of Conferences, Athens, Greece, 3–6 September 2019; EDP Sciences: Les Ulis, France, 2019; Volume 304. [Google Scholar] [CrossRef]
- Seebass, R. Sonic boom theory. J. Aircr. 1969, 6, 177–184. [Google Scholar] [CrossRef]
- Ispir, A.C.; Saracoglu, B.H. Development of a 1D dual mode ramjet model for a hypersonic civil aircraft. In Proceedings of the AIAA Propulsion and Energy 2019 Forum, Indianapolis, IN, USA, 19–22 August 2019. [Google Scholar] [CrossRef]
- Steelant, J.; Varvill, R.; Defoort, S.; Hanneman, K.; Marini, M. Achievements obtained for sustained hypersonic flight within the LAPCAT-II Project. In Proceedings of the 20th International Space Planes and Hypersonic Systems and Technologies Conference, Glasgow, UK, 6–9 July 2015. [Google Scholar] [CrossRef]
- Langener, T.; Erb, S.; Steelant, J. Trajectory simulation and optimization of the LAPCAT-MR2 hypersonic cruiser concept. In Proceedings of the 29th Congress of the International Council of the Aeronautical Sciences, St. Petersburg, Russia, 7–12 September 2014. [Google Scholar]
- Hirschel, E.H.; Weiland, C. Selected Aerothermodynamic Design Problems of Hypersonic Flight Vehicles; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2009; Volume 229. [Google Scholar]
- Koelle, D.E.; Kuczera, H. Sanger II, an advanced launcher system for Europe. Acta Astronaut. 1989, 19, 63–72. [Google Scholar] [CrossRef]
- Navò, A.; Bergada, J.M. Aerodynamic study of the NASA’s X-43A hypersonic aircraft. Appl. Sci. 2020, 10, 8211. [Google Scholar] [CrossRef]
- Chakraborty, I.; Mavris, D.N.; Emeneth, M.; Schneegans, A. A system and mission level analysis of electrically actuated flight control surfaces using Pacelab SysArc. In Proceedings of the 52nd Aerospace Sciences Meeting, National Harbor, MD, USA, 13–17 January 2014. [Google Scholar] [CrossRef]







| Surface Type | Ratio Movable Surface/Reference Surface [27] | Resulting Movable Surface (Total) [m2] |
|---|---|---|
| Pitch control devices | 0.048 | 120 |
| Roll control devices | 0.022 | 55 |
| Lateral control devices | 0.021 | 52.5 |
| Surface Type | LAPCAT MR2.4 Movable Surfaces (Total) [m2] |
|---|---|
| Wing trailing edge surfaces (roll) | 60 |
| Canard (pitch) | 100 |
| Rudders (yaw) | 32 |
| Surface Name | Chord (Mean) [m] | Span [m] | Deflection Limits [Deg] | Surface [m2] |
|---|---|---|---|---|
| External Elevon | 3.00 | 5.00 | +/−25 | 15.00 |
| Internal Elevon | 3.00 | 5.00 | +/−25 | 15.00 |
| Canard | 5.75 | 8.70 | +/−20 | 50.00 |
| Rudder | 3.05 | 6.50 | +/−20 | 19.80 |
| Body Flap | 7.14 | 4.05 | −30 | 23.70 |
| Single Surface Actuator Mass [kg] | |
|---|---|
| Elevon | 74.4 |
| Body Flap | 178.3 |
| Canard | 81.2 |
| Rudder | 17.7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ferretto, D.; Gori, O.; Fusaro, R.; Viola, N. Integrated Flight Control System Characterization Approach for Civil High-Speed Vehicles in Conceptual Design. Aerospace 2023, 10, 495. https://doi.org/10.3390/aerospace10060495
Ferretto D, Gori O, Fusaro R, Viola N. Integrated Flight Control System Characterization Approach for Civil High-Speed Vehicles in Conceptual Design. Aerospace. 2023; 10(6):495. https://doi.org/10.3390/aerospace10060495
Chicago/Turabian StyleFerretto, Davide, Oscar Gori, Roberta Fusaro, and Nicole Viola. 2023. "Integrated Flight Control System Characterization Approach for Civil High-Speed Vehicles in Conceptual Design" Aerospace 10, no. 6: 495. https://doi.org/10.3390/aerospace10060495
APA StyleFerretto, D., Gori, O., Fusaro, R., & Viola, N. (2023). Integrated Flight Control System Characterization Approach for Civil High-Speed Vehicles in Conceptual Design. Aerospace, 10(6), 495. https://doi.org/10.3390/aerospace10060495









