The Exact Solution of the Bending Moment in the Folding Process of Negative Poisson’s Ratio Honeycomb Tape Spring and Multi-Objective Optimization Design
Abstract
1. Introduction
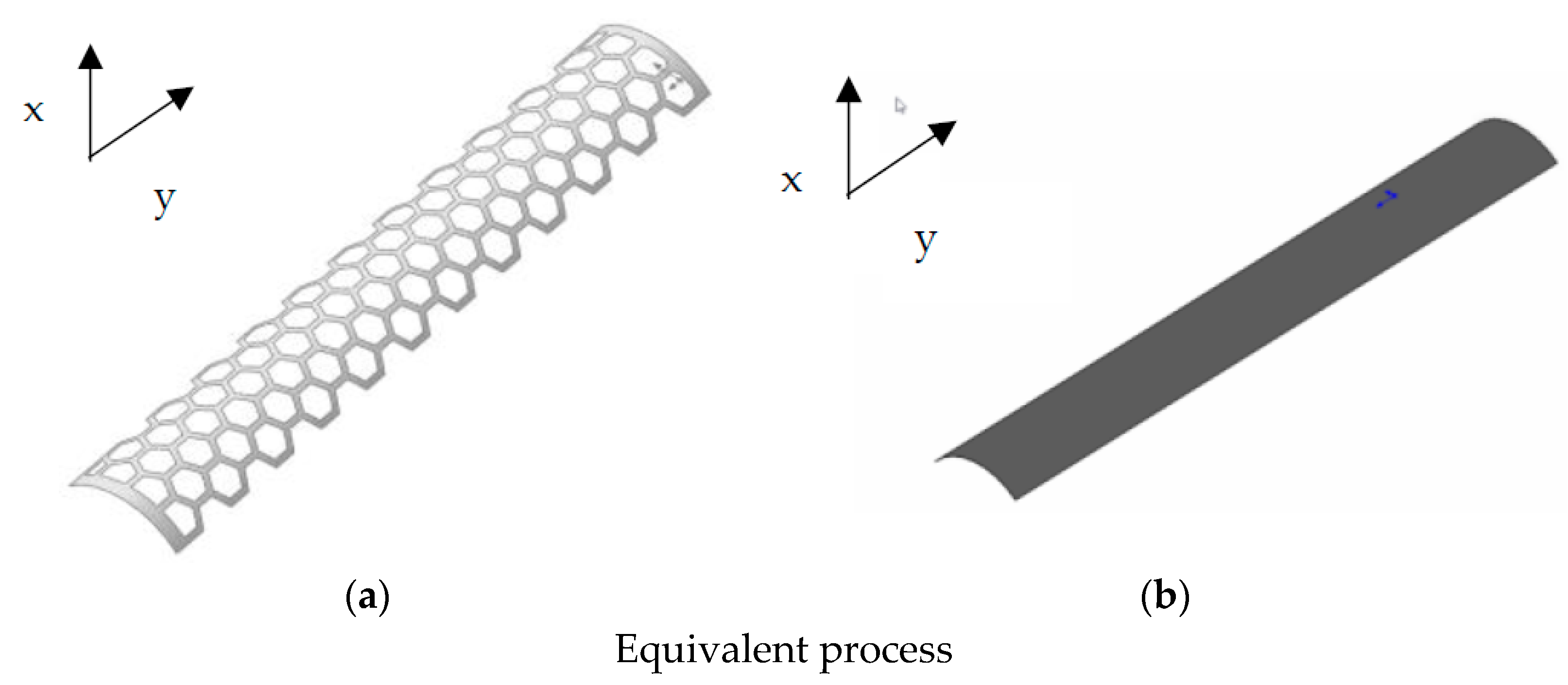
2. Theoretical Analysis of the Folding Process for Honeycomb Negative Poisson’s Ratio Honeycomb Tape Springs Considering Geometric Nonlinearity
2.1. Equivalent Parameters of the Negative Poisson’s Ratio Honeycomb Structure
2.2. Theoretical Analysis of a Moment in the Bending Process Considering Geometric Nonlinearity
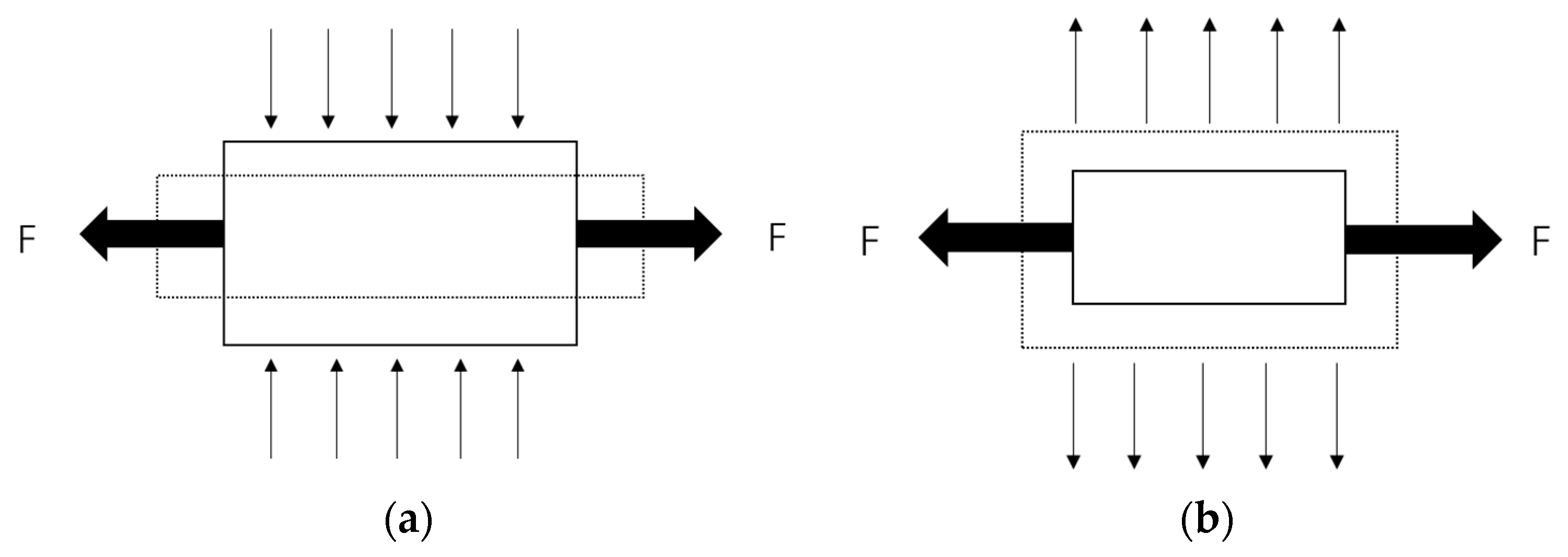
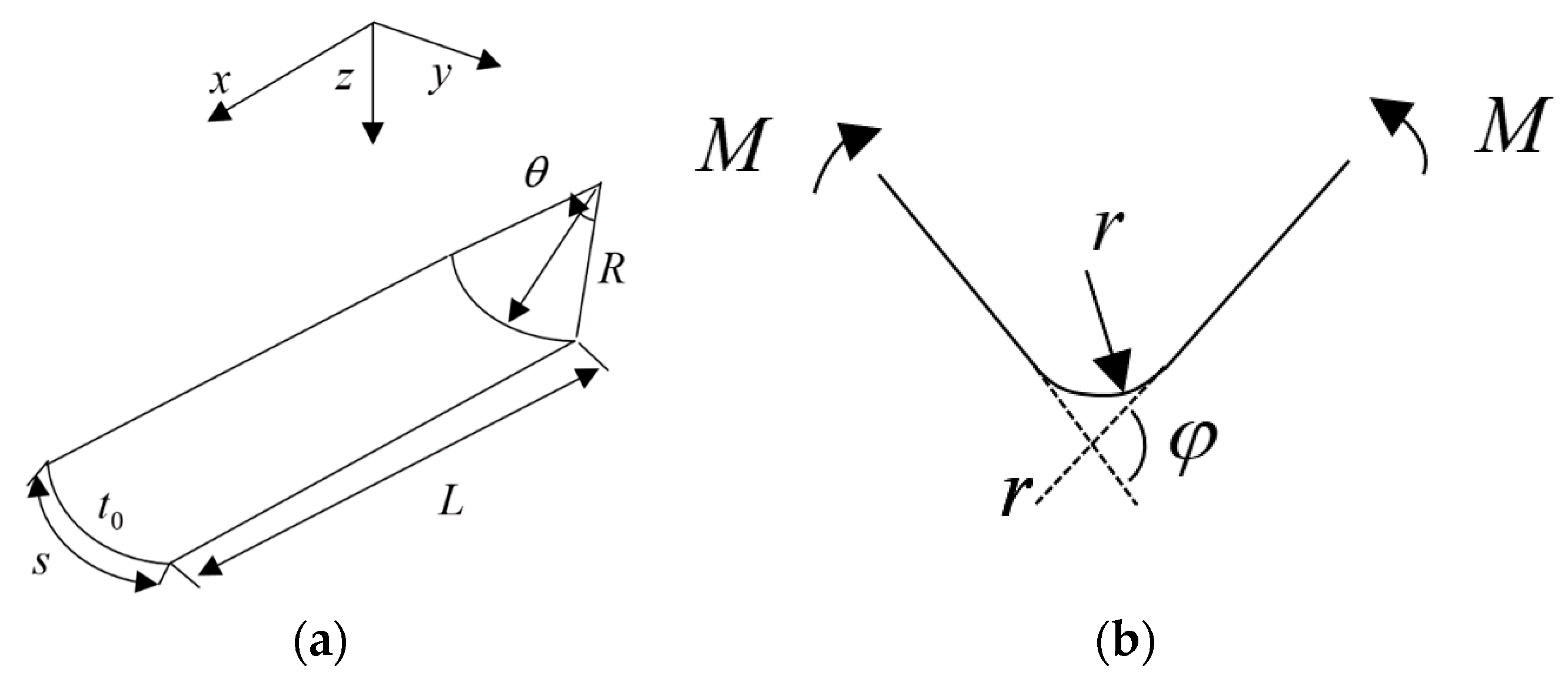
2.2.1. Bending Moment of Isotropic Tape Spring for Folding
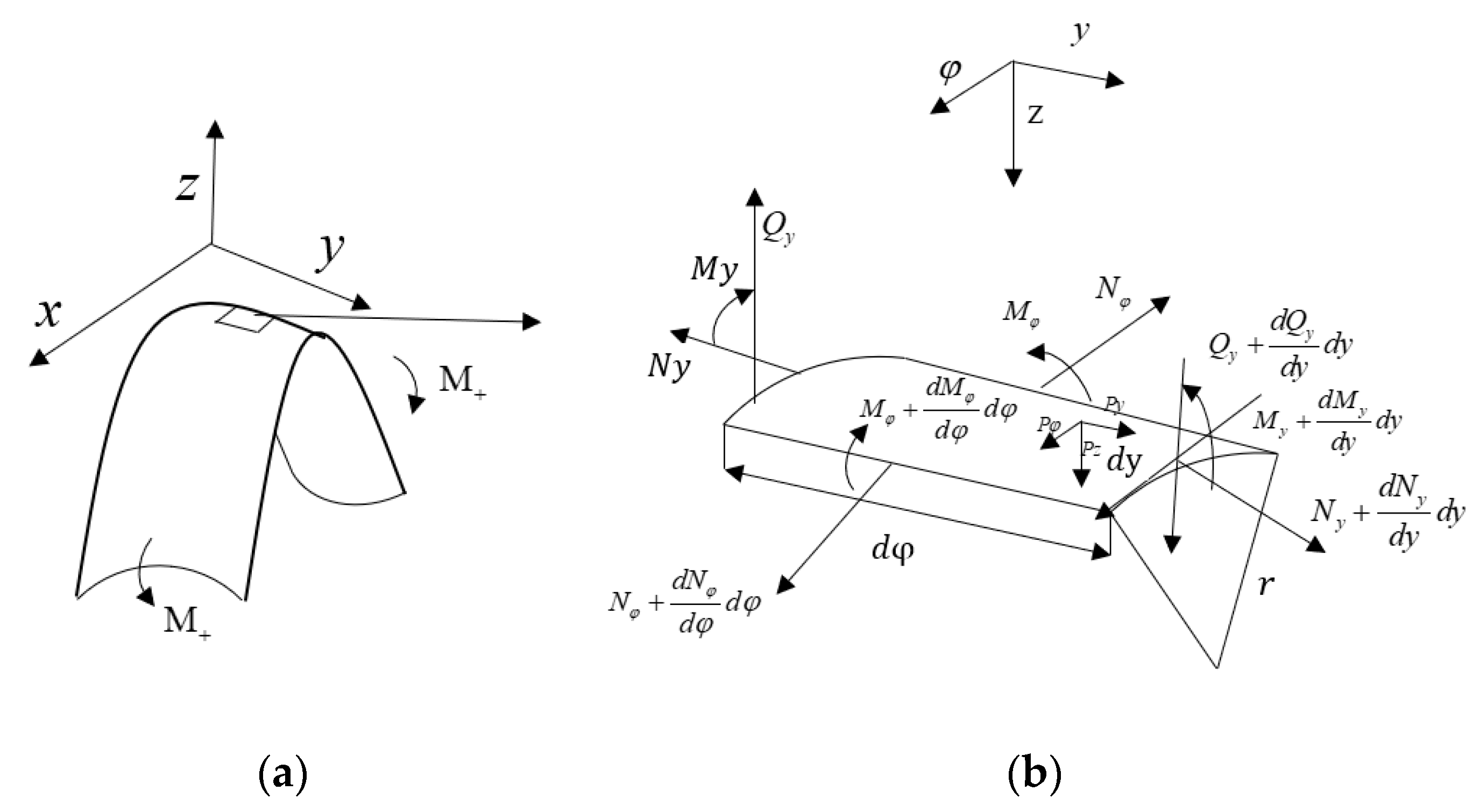
2.2.2. The Folding Moment of Negative Poisson’s Ratio Honeycomb Tape Spring
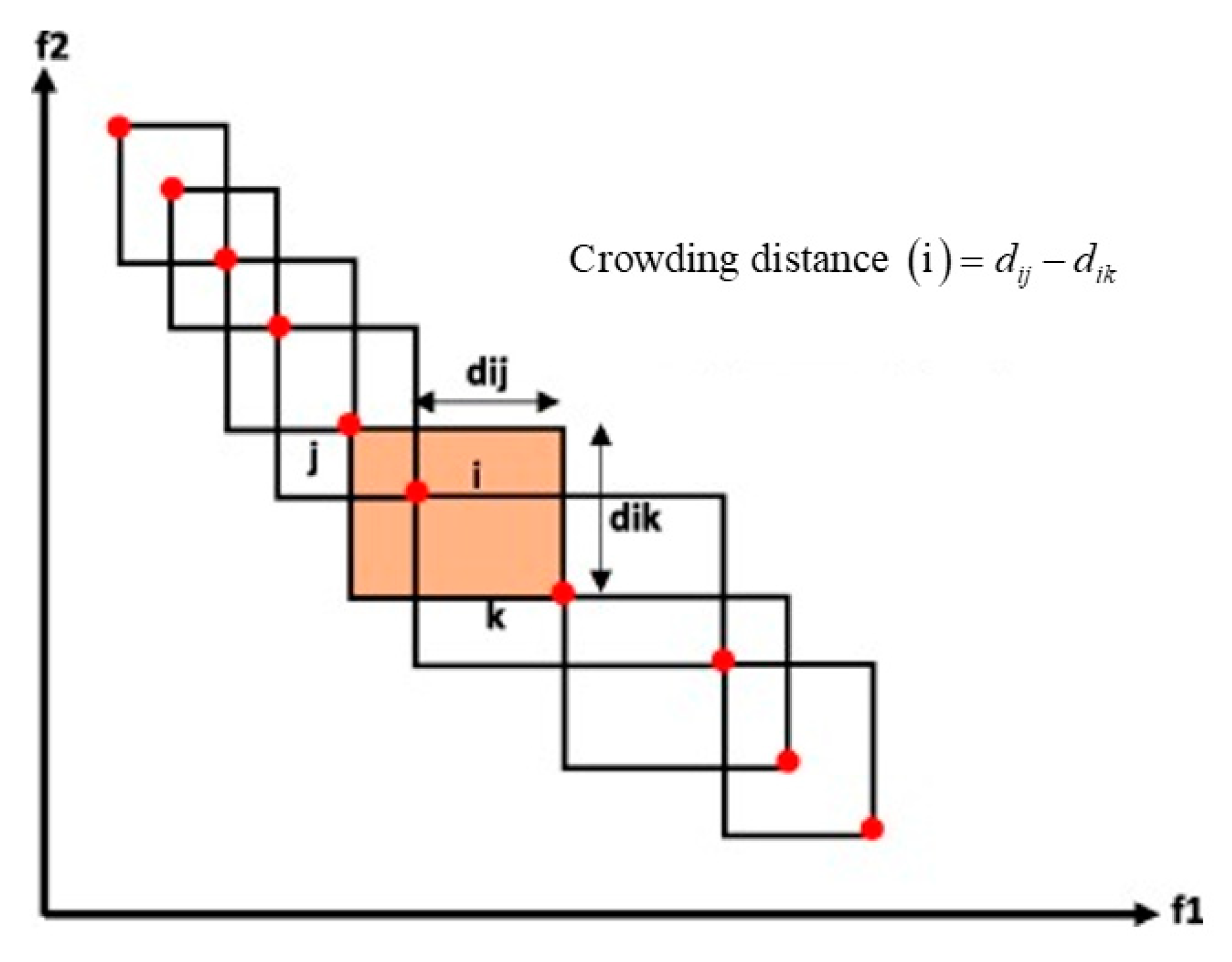
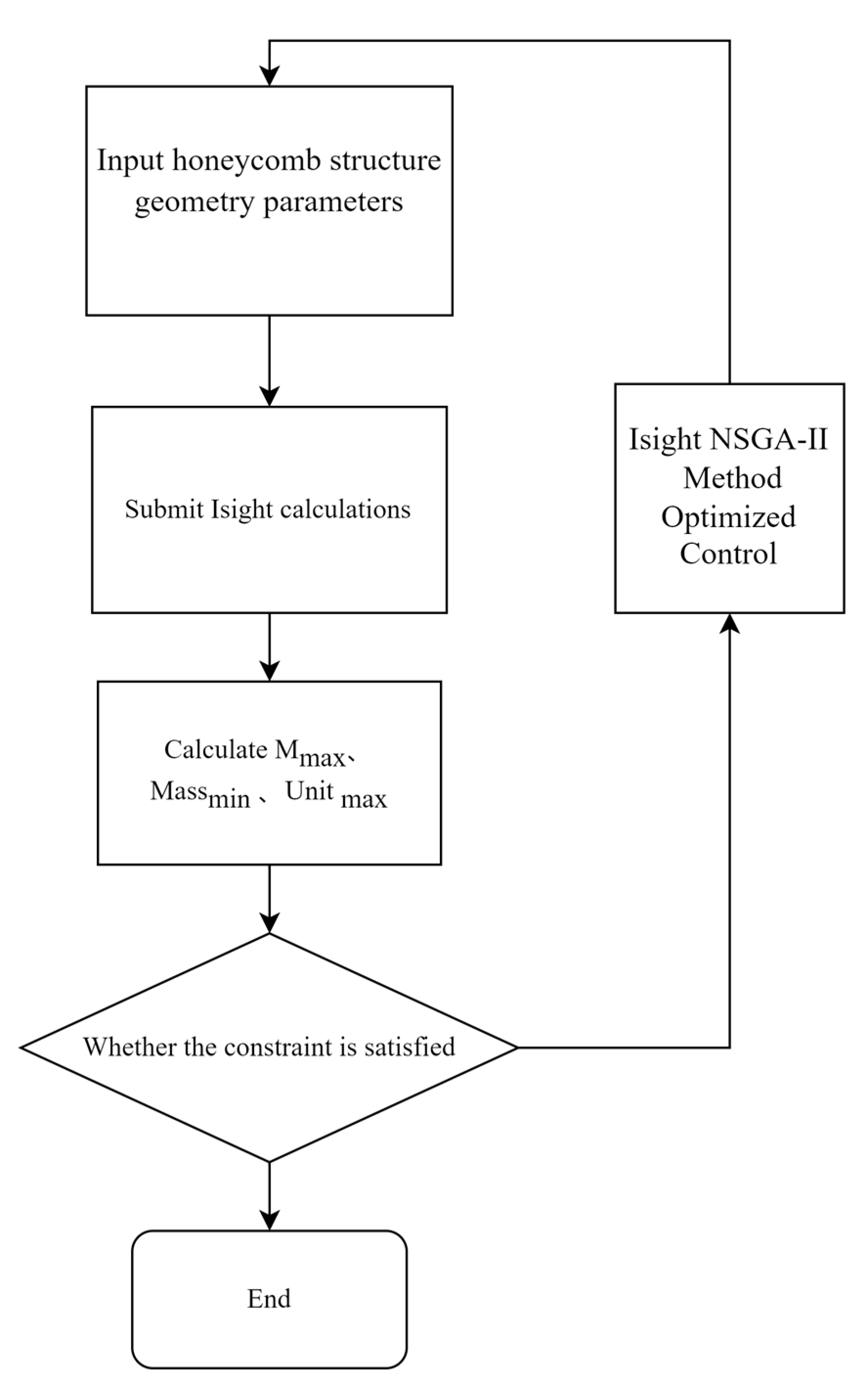
3. Multi-Objective Optimization Design
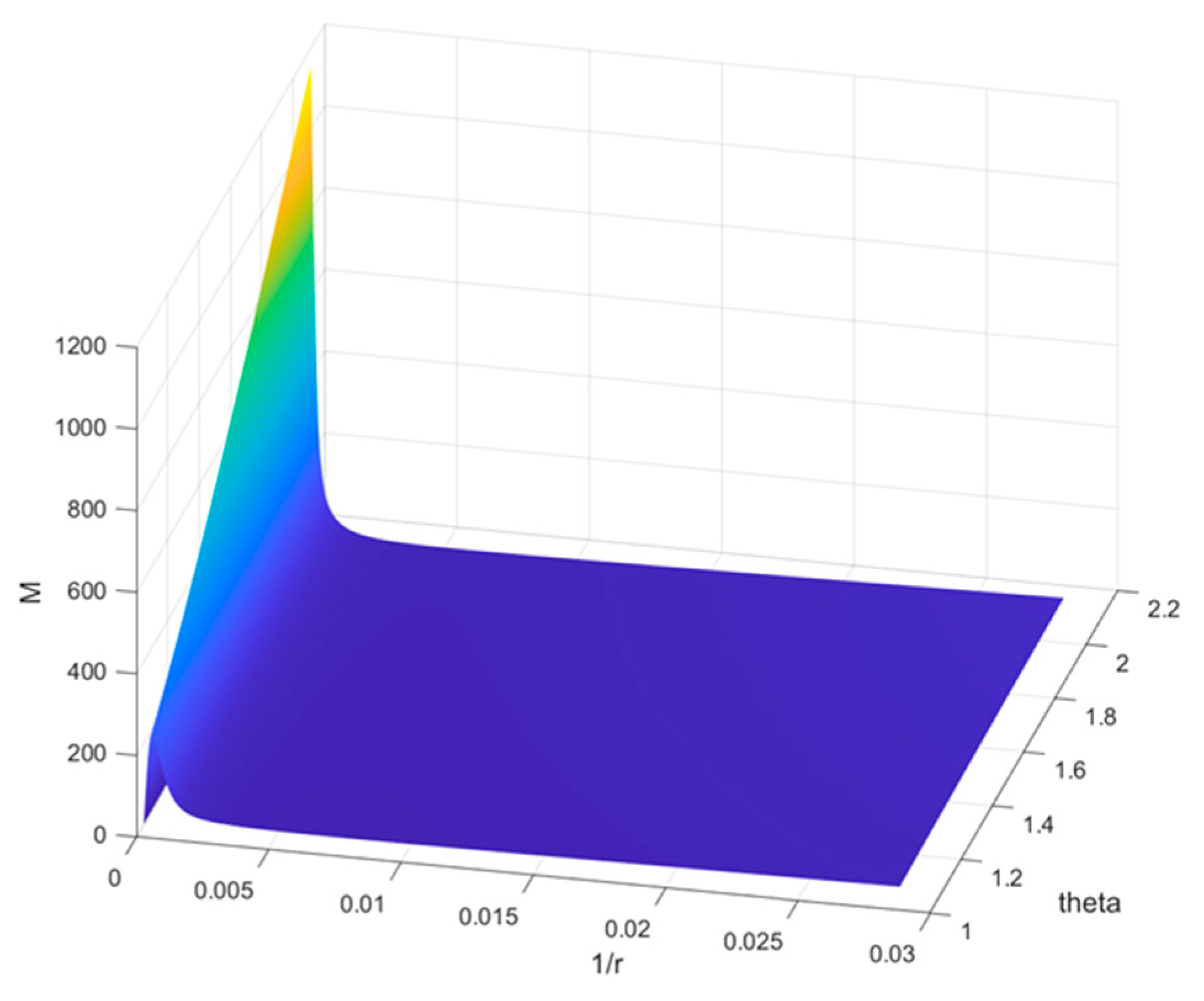
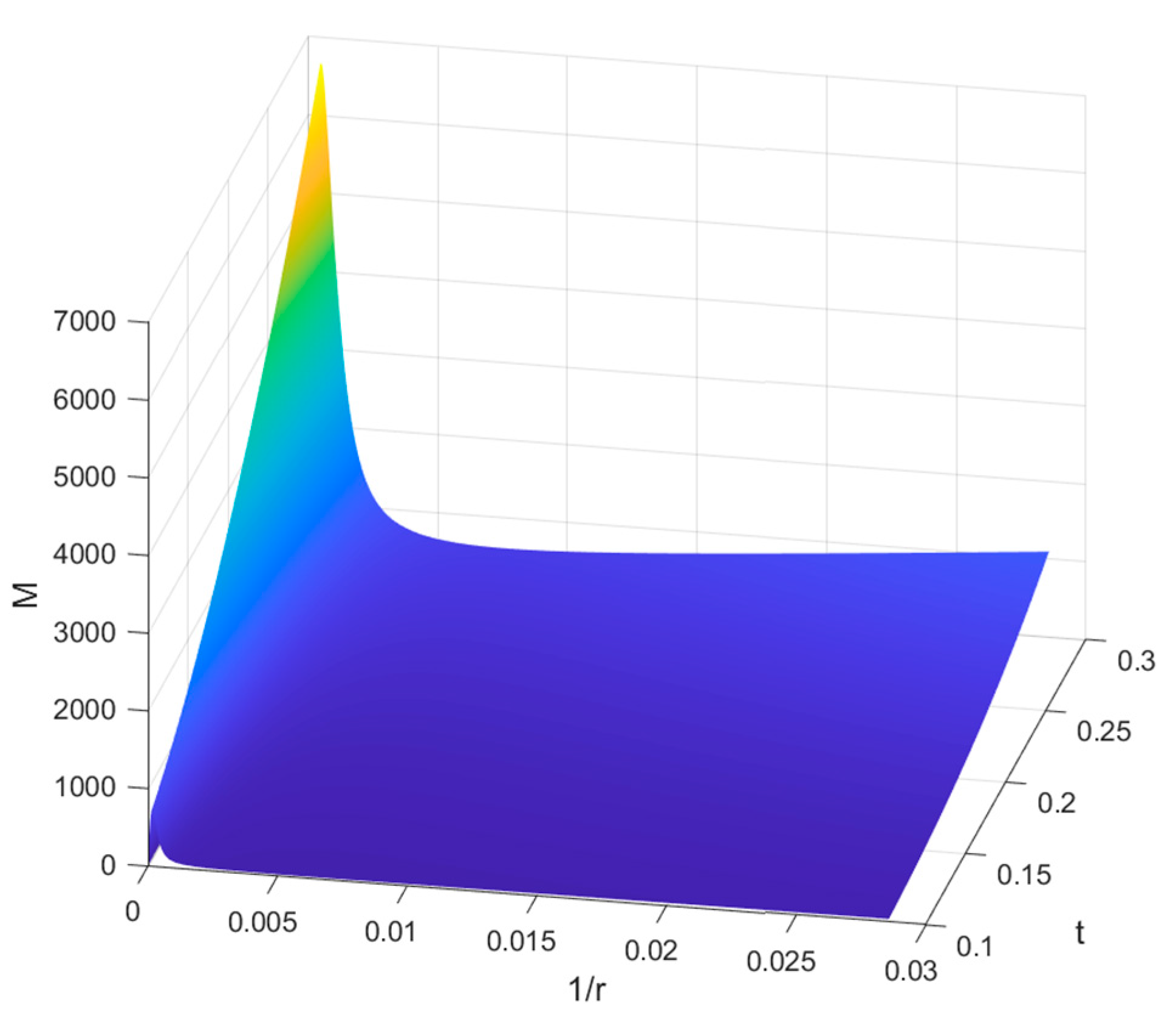
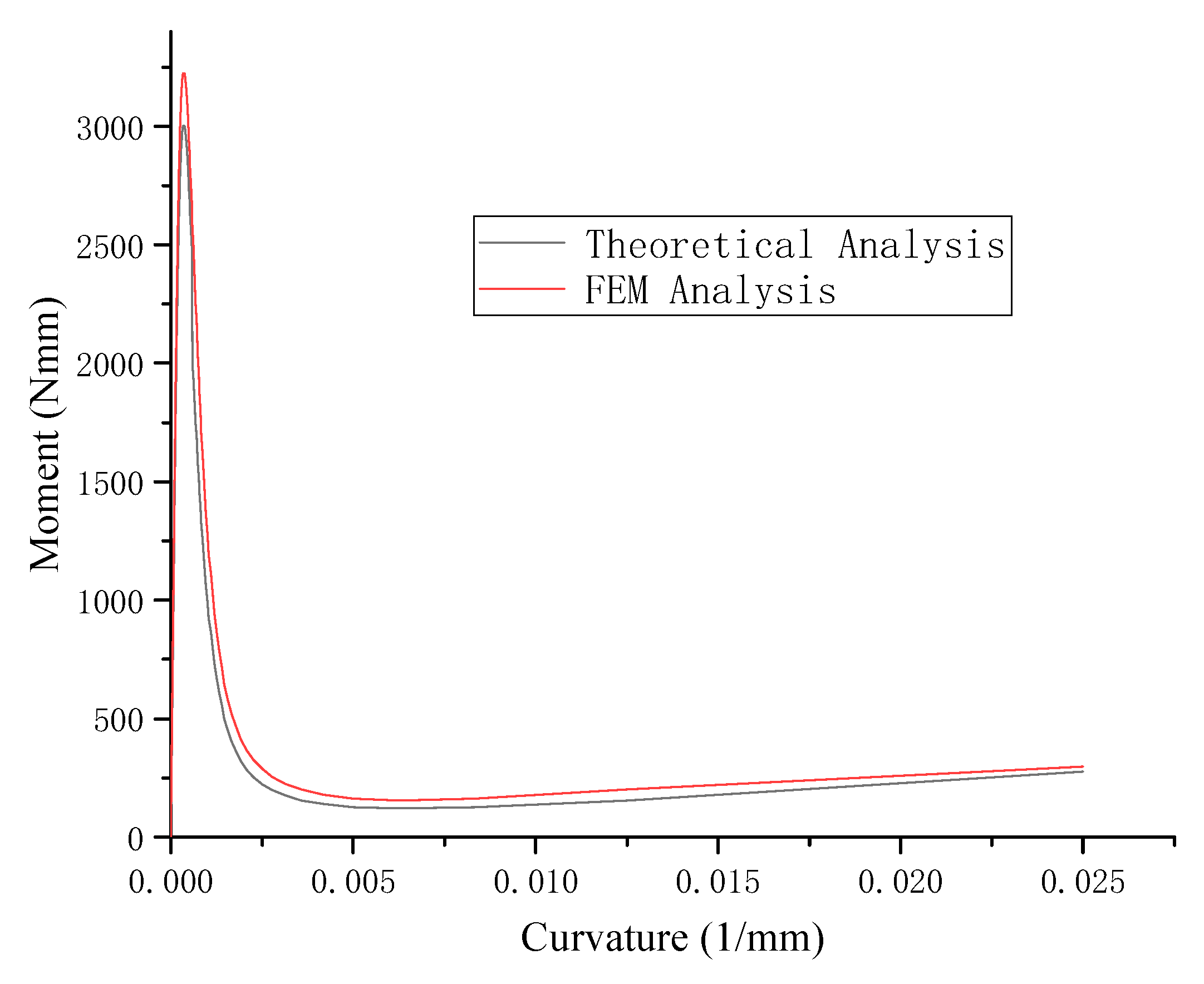
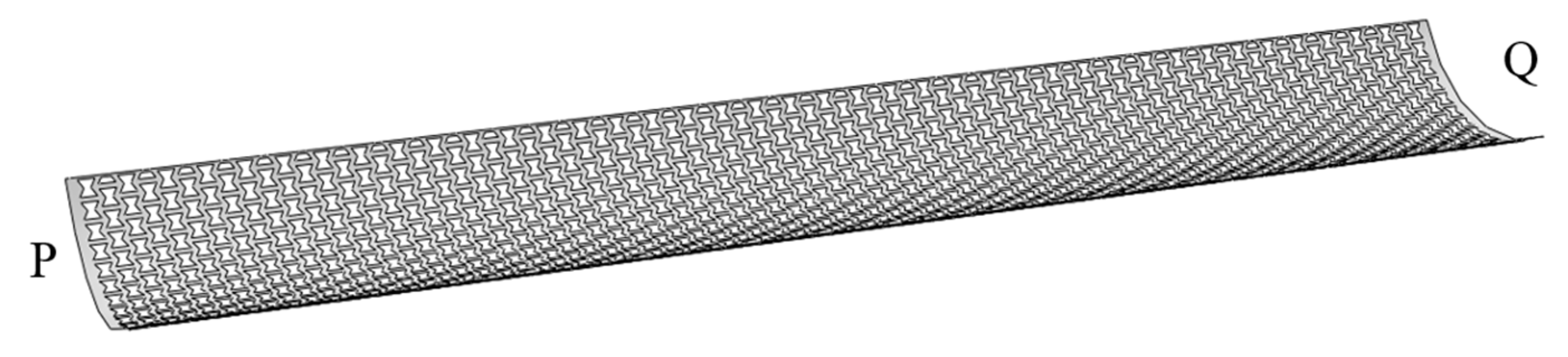
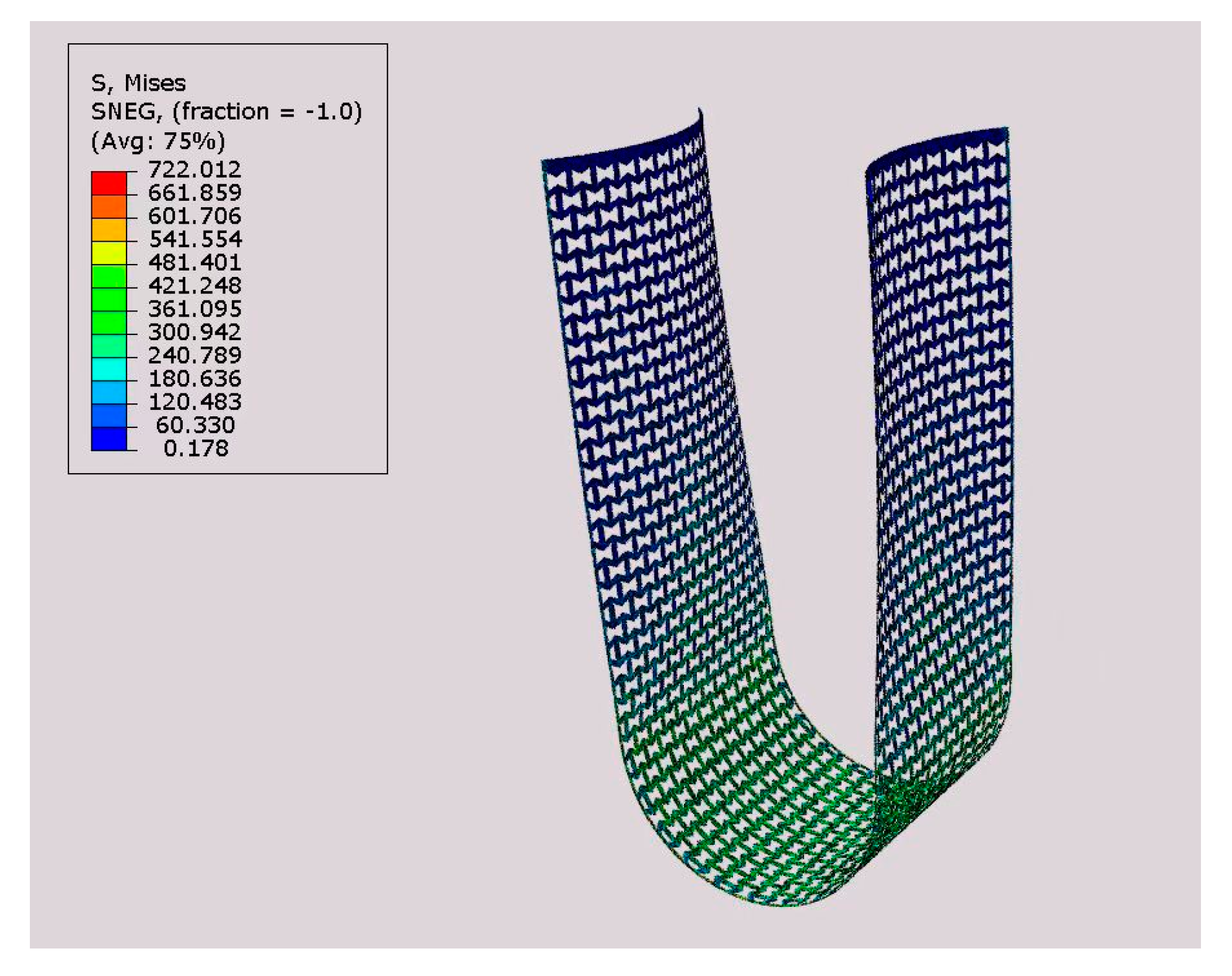
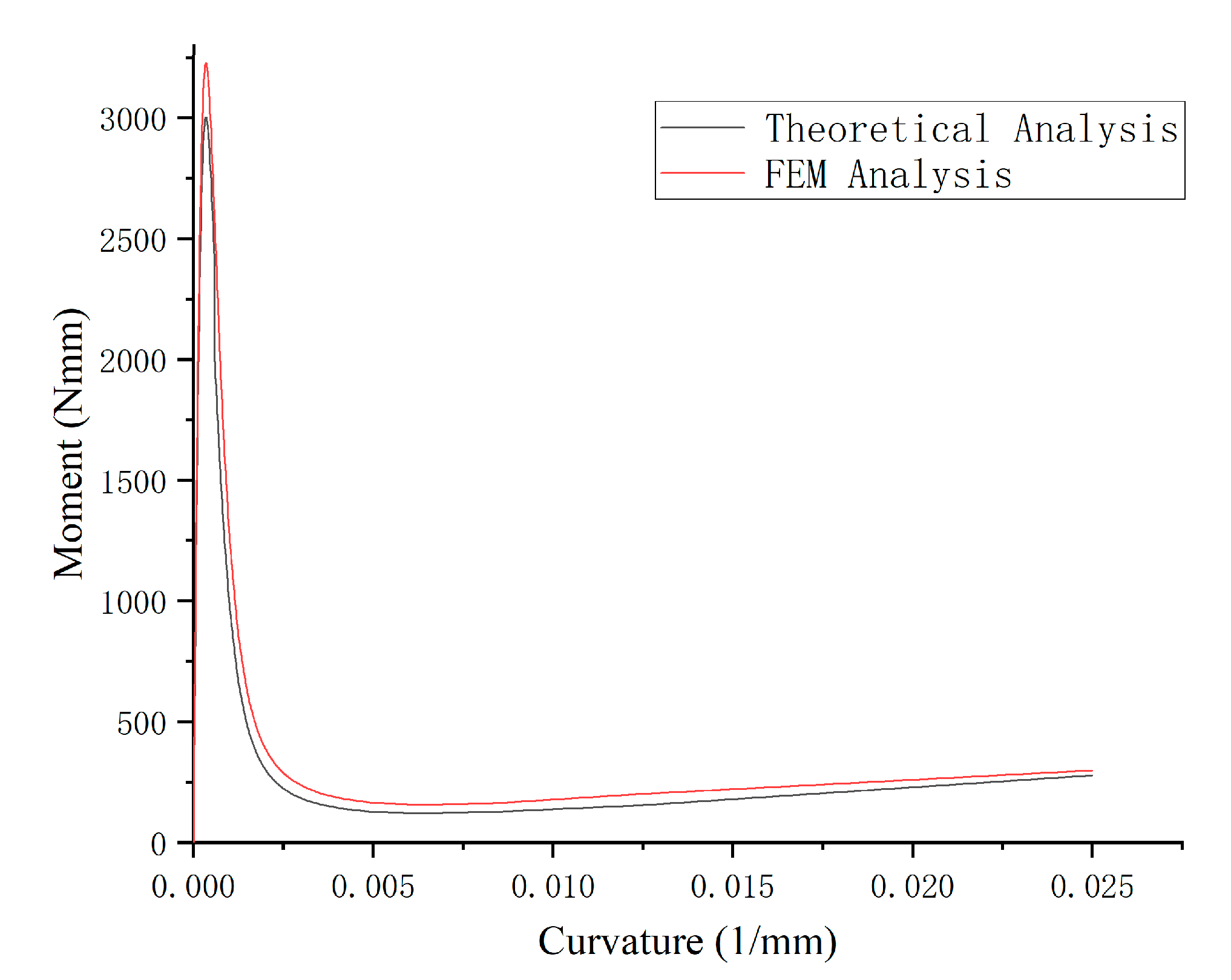
4. Numerical Argument Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Symbol | Description |
| E1, E2 | Elastic modulus |
| v12, v21 | Poisson’s Ratio |
| Nx, Ny, Nφ | Normal force |
| Mx, My, Mφ | Moment |
| Qx, Qy | Transverse force |
| εx, εy, εφ | Strain |
| σx, σy, σφ | Stress |
References
- Ma, X.; Li, T.; Ma, J.; Wang, Z.; Shi, C.; Zheng, S.; Cui, Q.; Li, X.; Liu, F.; Guo, H.; et al. Recent Advances in Space-Deployable Structures in China. Engineering 2022, 17, 207–219. [Google Scholar] [CrossRef]
- Yildiz, K. Cable Actuated Tensegrity Structures for Deployable Space Booms with Enhanced Stiffness; The Pennsylvania State University: State College, PA, USA, 2018. [Google Scholar]
- Lan, X.; Liu, L.; Zhang, F.; Liu, Z.X.; Wang, L.L.; Li, Q.F.; Peng, F.; Peng, F.; Hao, S.D.; Dai, W.X.; et al. World’s first spaceflight on-orbit demonstration of a flexible solar array system based on shape memory polymer composites. Sci. China Technol. Sci. 2020, 63, 1436–1451. [Google Scholar] [CrossRef]
- Ma, S.; Liang, B.; Wang, T. Dynamic analysis of a hyper-redundant space manipulator with a complex rope network. Aerosp. Sci. Technol. 2020, 100, 105768. [Google Scholar] [CrossRef]
- Li, C.; Wang, Z.; Xu, R.; Lv, G.; Yuan, L.; He, Z.; Wang, J. The Scientific Information Model of Chang’e-4 Visible and Near-IR Imaging Spectrometer (VNIS) and In-Flight Verification. Sensors 2019, 19, 2806. [Google Scholar] [CrossRef] [PubMed]
- Nie, R.; He, B.; Yan, S.; Ma, X. Design optimization of mesh antennas for on-orbit thermal effects. Int. J. Mech. Sci. 2020, 175, 105547. [Google Scholar] [CrossRef]
- Firth, J.A. Deployment Mechanisms for Rollable Spacecraft Booms; North Carolina State University: Raleigh, NC, USA, 2019. [Google Scholar]
- Arora, H.; Dangarwala, R.; Mukherjee, S.; Munjal, B.S. Investigation of tape flexures for space borne deployable structures. Int. J. Space Struct. 2022, 37, 37–48. [Google Scholar] [CrossRef]
- Seffen, K.; You, Z.; Pellegrino, S. Folding and deployment of curved tape springs. Int. J. Mech. Sci. 2000, 42, 2055–2073. [Google Scholar] [CrossRef]
- Mallikarachchi, H.M.Y.C.; Pellegrino, S.; Mallikarachchi, C. Quasi-Static Folding and Deployment of Ultrathin Composite Tape-Spring Hinges. J. Spacecr. Rocket. 2011, 48, 187–198. [Google Scholar] [CrossRef]
- Ye, H.; Zhang, Y.; Yang, Q.; Xiao, Y.; Grandhi, R.V.; Fischer, C.C. Optimal design of a three tape-spring hinge deployable space structure using an experimentally validated physics-based model. Struct. Multidiscip. Optim. 2017, 56, 973–989. [Google Scholar] [CrossRef]
- Ye, H.; Zhang, Y.; Yang, Q.; Zhang, B. Quasi-static analysis and multi-objective optimization for tape spring hinge. Struct. Multidiscip. Optim. 2019, 60, 2417–2430. [Google Scholar] [CrossRef]
- Yang, H.; Lu, F.; Guo, H.; Liu, R. Design of a New N-Shape Composite Ultra-Thin Deployable Boom in the Post-Buckling Range Using Response Surface Method and Optimization. IEEE Access 2019, 7, 129659–129665. [Google Scholar] [CrossRef]
- Yao, Y.; Li, G.; Ning, X. Ultra-thin, Ultra-lightweight Structural Electronic Skin for Measurements of Tape-spring Hinge Behavior. In Proceedings of the AIAA Scitech 2021 Forum, Virtual, 11–15 and 19–21 January 2021. [Google Scholar] [CrossRef]
- Chang, W.; Cao, D.; Lian, M. Simulation and analysis of tape spring for deployed space structures. In Proceedings of the Young Scientists Forum 2017, Shanghai, China, 24–26 November 2017. [Google Scholar] [CrossRef]
- Yang, Y.; Wang, F.; Liu, J. Application of Honeycomb Structures in Key Components of Space Deployable Structures. Adv. Mater. Sci. Eng. 2022, 2022, 4756272. [Google Scholar] [CrossRef]
- Kelkar, P.U.; Kim, H.S.; Cho, K.-H.; Kwak, J.Y.; Kang, C.-Y.; Song, H.-C. Cellular Auxetic Structures for Mechanical Metamaterials: A Review. Sensors 2020, 20, 3132. [Google Scholar] [CrossRef] [PubMed]
- Lakes, R.S.; Witt, R. Making and Characterizing Negative Poisson’s Ratio Materials. Int. J. Mech. Eng. Educ. 2002, 30, 50–58. [Google Scholar] [CrossRef]
- Balan, M.; Mertens, J.; Bahubalendruni, M.R. Auxetic mechanical metamaterials and their futuristic developments: A state-of-art review. Mater. Today Commun. 2022, 34, 105285. [Google Scholar] [CrossRef]
- Ren, X.; Das, R.; Tran, P.; Ngo, T.D.; Xie, Y.M. Auxetic metamaterials and structures: A review. Smart Mater. Struct. 2018, 27, 023001. [Google Scholar] [CrossRef]
- Coenen, V.L.; Alderson, K.L. Mechanisms of failure in the static indentation resistance of auxetic carbon fibre laminates. Phys. Status Solidi (B) 2011, 248, 66–72. [Google Scholar] [CrossRef]
- Argatov, I.I.; Guinovart-Díaz, R.; Sabina, F.J. On local indentation and impact compliance of isotropic auxetic materials from the continuum mechanics viewpoint. Int. J. Eng. Sci. 2012, 54, 42–57. [Google Scholar] [CrossRef]
- Novak, N.; Krstulović-Opara, L.; Ren, Z.; Vesenjak, M. Compression and shear behaviour of graded chiral auxetic structures. Mech. Mater. 2020, 148, 103524. [Google Scholar] [CrossRef]
- Henyš, P.; Vomáčko, V.; Ackermann, M.; Sobotka, J.; Solfronk, P.; Šafka, J.; Čapek, L. Normal and shear behaviours of the auxetic metamaterials: Homogenisation and experimental approaches. Meccanica 2019, 54, 831–839. [Google Scholar] [CrossRef]
- Choi, J.B.; Lakes, R.S. Non-linear properties of polymer cellular materials with a negative Poisson’s ratio. J. Mater. Sci. 1992, 27, 4678–4684. [Google Scholar] [CrossRef]
- Amin, F.; Ali, M.N.; Ansari, U.; Mir, M.; Minhas, M.A.; Shahid, W. Auxetic Coronary Stent Endoprosthesis: Fabrication and Structural Analysis. J. Appl. Biomater. Funct. Mater. 2015, 13, 127–135. [Google Scholar] [CrossRef] [PubMed]
- Lakes, R. Foam Structures with a Negative Poisson’s Ratio. Science 1987, 235, 1038–1040. [Google Scholar] [CrossRef] [PubMed]
- Brighenti, R. Smart behaviour of layered plates through the use of auxetic materials. Thin-Walled Struct. 2014, 84, 432–442. [Google Scholar] [CrossRef]
- Guo, M.-F.; Yang, H.; Ma, L. Design and analysis of 2D double-U auxetic honeycombs. Thin-Walled Struct. 2020, 155, 106915. [Google Scholar] [CrossRef]
- Simpson, J.; Kazancı, Z. Crushing investigation of crash boxes filled with honeycomb and re-entrant (auxetic) lattices. Thin-Walled Struct. 2020, 150, 106676. [Google Scholar] [CrossRef]
- Wei, L.; Zhao, X.; Yu, Q.; Zhu, G. A novel star auxetic honeycomb with enhanced in-plane crushing strength. Thin-Walled Struct. 2020, 149, 106623. [Google Scholar] [CrossRef]
- E Evans, K. Auxetic polymers: A new range of materials. Endeavour 1991, 15, 170–174. [Google Scholar] [CrossRef]
- Hou, S.; Liu, T.; Zhang, Z.; Han, X.; Li, Q. How does negative Poisson’s ratio of foam filler affect crashworthiness? Mater. Des. 2015, 82, 247–259. [Google Scholar] [CrossRef]
- Mohsenizadeh, S.; Alipour, R.; Rad, M.S.; Nejad, A.F.; Ahmad, Z. Crashworthiness assessment of auxetic foam-filled tube under quasi-static axial loading. Mater. Des. 2015, 88, 258–268. [Google Scholar] [CrossRef]
- Mazhnik, E.; Oganov, A.R. A model of hardness and fracture toughness of solids. J. Appl. Phys. 2019, 126, 125109. [Google Scholar] [CrossRef]
- Maruszewski, T.S.; Wojciechowski, K.W. Anomalous deformation of constrained auxetic square. Rev. Adv. Mater. Sci. 2010, 23, 169–174. [Google Scholar] [CrossRef]
- Harkati, A.; Boutagouga, D.; Bezazi, A.; Scarpa, F.; Ouisse, M. In-plane elastic constants of a new curved cell walls honeycomb concept. Thin-Walled Struct. 2020, 149, 106613. [Google Scholar] [CrossRef]
- Pasternak, E.; Dyskin, A. Apparent Negative Stiffness Induced by Constriction. Phys. Status Solidi (B) 2022, 259, 2200379. [Google Scholar] [CrossRef]
- Xie, Y.M.; Yang, X.; Shen, J.; Yan, X.; Ghaedizadeh, A.; Rong, J.; Huang, X.; Zhou, S. Designing orthotropic materials for negative or zero compressibility. Int. J. Solids Struct. 2014, 51, 4038–4051. [Google Scholar] [CrossRef]
- Grima, J.N.; Caruana-Gauci, R.; Wojciechowski, K.W.; E Evans, K. Smart hexagonal truss systems exhibiting negative compressibility through constrained angle stretching. Smart Mater. Struct. 2013, 22, 084015. [Google Scholar] [CrossRef]
- Chekkal, I.; Bianchi, M.; Remillat, C.; Bécot, F.-X.; Jaouen, L.; Scarpa, F. Vibro-Acoustic Properties of Auxetic Open Cell Foam: Model and Experimental Results. Acta Acust. United Acust. 2010, 96, 266–274. [Google Scholar] [CrossRef]
- Ye, H.F.; Tao, M.; Zhang, W.Z. Modeling and Sound Insulation Performance Analysis of Two Honeycomb-hole Coatings. J. Phys. Conf. Ser. 2018, 1016, 012001. [Google Scholar] [CrossRef]
- Eghbali, P.; Younesian, D.; Farhangdoust, S. Enhancement of the low-frequency acoustic energy harvesting with auxetic resonators. Appl. Energy 2020, 270, 115217. [Google Scholar] [CrossRef]
- Hosseinkhani, A.; Younesian, D.; Eghbali, P.; Moayedizadeh, A.; Fassih, A. Sound and vibration energy harvesting for railway applications: A review on linear and nonlinear techniques. Energy Rep. 2021, 7, 852–874. [Google Scholar] [CrossRef]
- Zhang, X.Y.; Ren, X.; Wang, X.Y.; Zhang, Y.; Xie, Y.M. A novel combined auxetic tubular structure with enhanced tunable stiffness. Compos. Part B Eng. 2021, 226, 109303. [Google Scholar] [CrossRef]
- Zhong, R.; Ren, X.; Zhang, X.Y.; Luo, C.; Zhang, Y.; Xie, Y.M. Mechanical properties of concrete composites with auxetic single and layered honeycomb structures. Constr. Build. Mater. 2022, 322, 126453. [Google Scholar] [CrossRef]
- Shen, L.; Wang, Z.; Wang, X.; Wei, K. Negative Poisson’s ratio and effective Young’s modulus of a vertex-based hierarchical re-entrant honeycomb structure. Int. J. Mech. Sci. 2021, 206, 106611. [Google Scholar] [CrossRef]
- Hector, K.W.; Restrepo, D.; Bonilla, C.T.; Hector, L.G.; Mankame, N.; Zavattieri, P.D. Mechanics of Chiral Honeycomb Architectures with Phase Transformations. J. Appl. Mech. 2019, 86, 111014. [Google Scholar] [CrossRef]
- Allen, H.G. Analysis and Design of Structural Sandwich Panels: The Commonwealth and International Library: Structures and Solid body Mechanics Division; Elsevier: Amsterdam, The Netherlands, 2013. [Google Scholar]
- Gibson, L.J.; Ashby, M.F.; Schajer, G.S.; Robertson, C.I. The mechanics of two-dimensional cellular materials. Proc. R. Soc. Lond. A Math. Phys. Sci. 1982, 382, 25–42. [Google Scholar] [CrossRef]
- Burton, W.; Noor, A. Assessment of continuum models for sandwich panel honeycomb cores. Comput. Methods Appl. Mech. Eng. 1997, 145, 341–360. [Google Scholar] [CrossRef]
- Fu, M.-H.; Yin, J. Equivalent elastic parameters of the honeycomb core. Chin. J. Theor. Appl. Mech. 1999, 31, 113. [Google Scholar] [CrossRef]
- Meng, J.; Deng, Z.; Zhang, K.; Xu, X.; Wen, F. Band gap analysis of star-shaped honeycombs with varied Poisson’s ratio. Smart Mater. Struct. 2015, 24, 095011. [Google Scholar] [CrossRef]
- Mingjin, L. Study of Mechanics Characteristics of Negative Poisson’s Ratio Honeycomb Core Structure; University of Science and Technology of China: Hefei, China, 2012. [Google Scholar]
- Cao, Y.; Khan, A.; Abdi, A.; Ghadiri, M. Combination of RSM and NSGA-II algorithm for optimization and prediction of thermal conductivity and viscosity of bioglycol/water mixture containing SiO2 nanoparticles. Arab. J. Chem. 2021, 14, 103204. [Google Scholar] [CrossRef]
- Aydar, A.Y. Utilization of Response Surface Methodology in Optimization of Extraction of Plant Materials. In Statistical Approaches with Emphasis on Design of Experiments Applied to Chemical Processes; IntechOpen: London, UK, 2018; pp. 157–169. [Google Scholar] [CrossRef]
- Liu, L.; Zhou, Y.; Xu, J.; Zheng, X.; Chang, K.-W.; Huang, X. Cross-Lingual Dependency Parsing by POS-Guided Word Reordering. In Proceedings of the Findings of the Association for Computational Linguistics: EMNLP 2020, Punta Cana, Dominican Republic, 8–12 November 2020. [Google Scholar] [CrossRef]
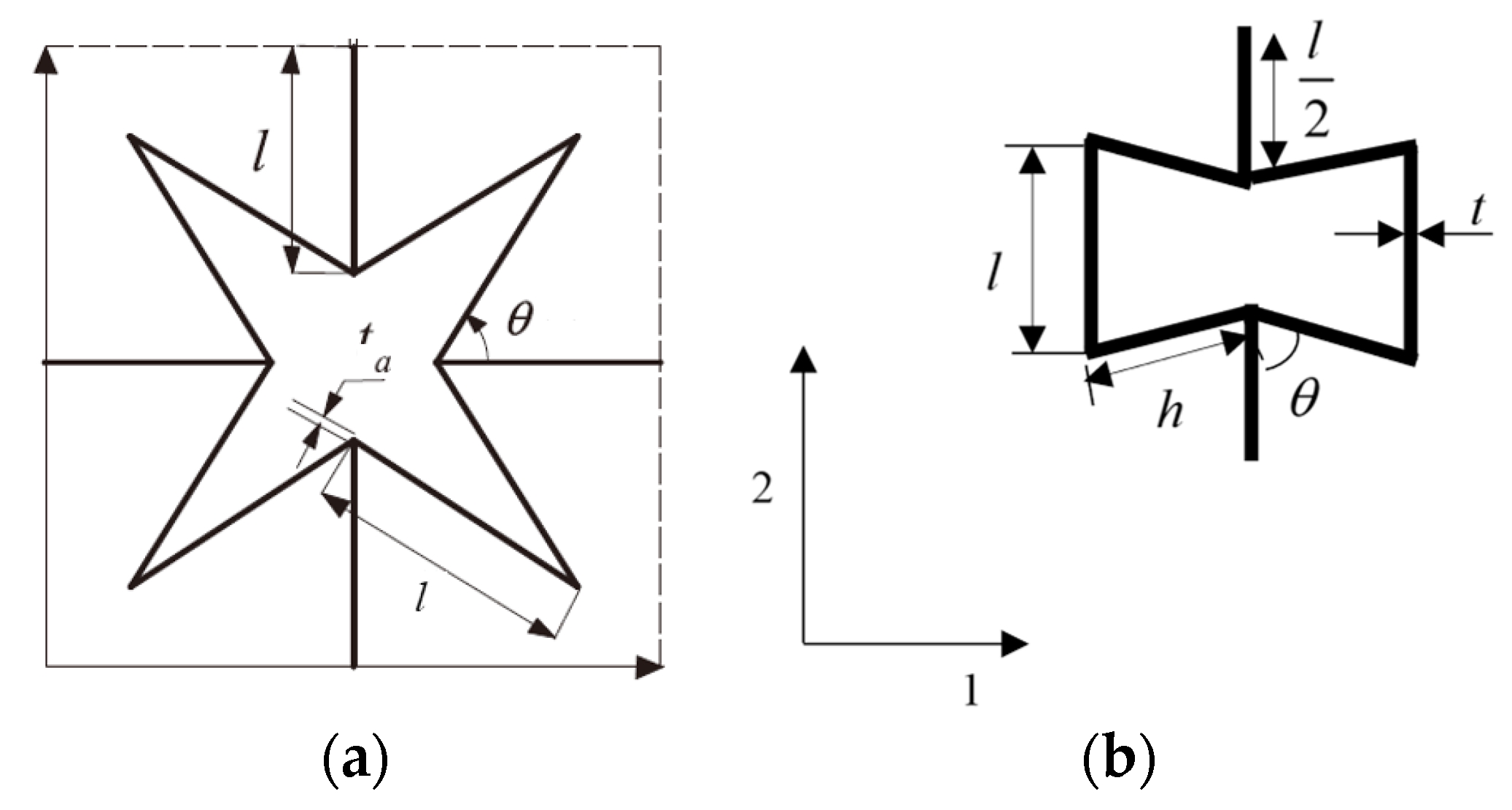



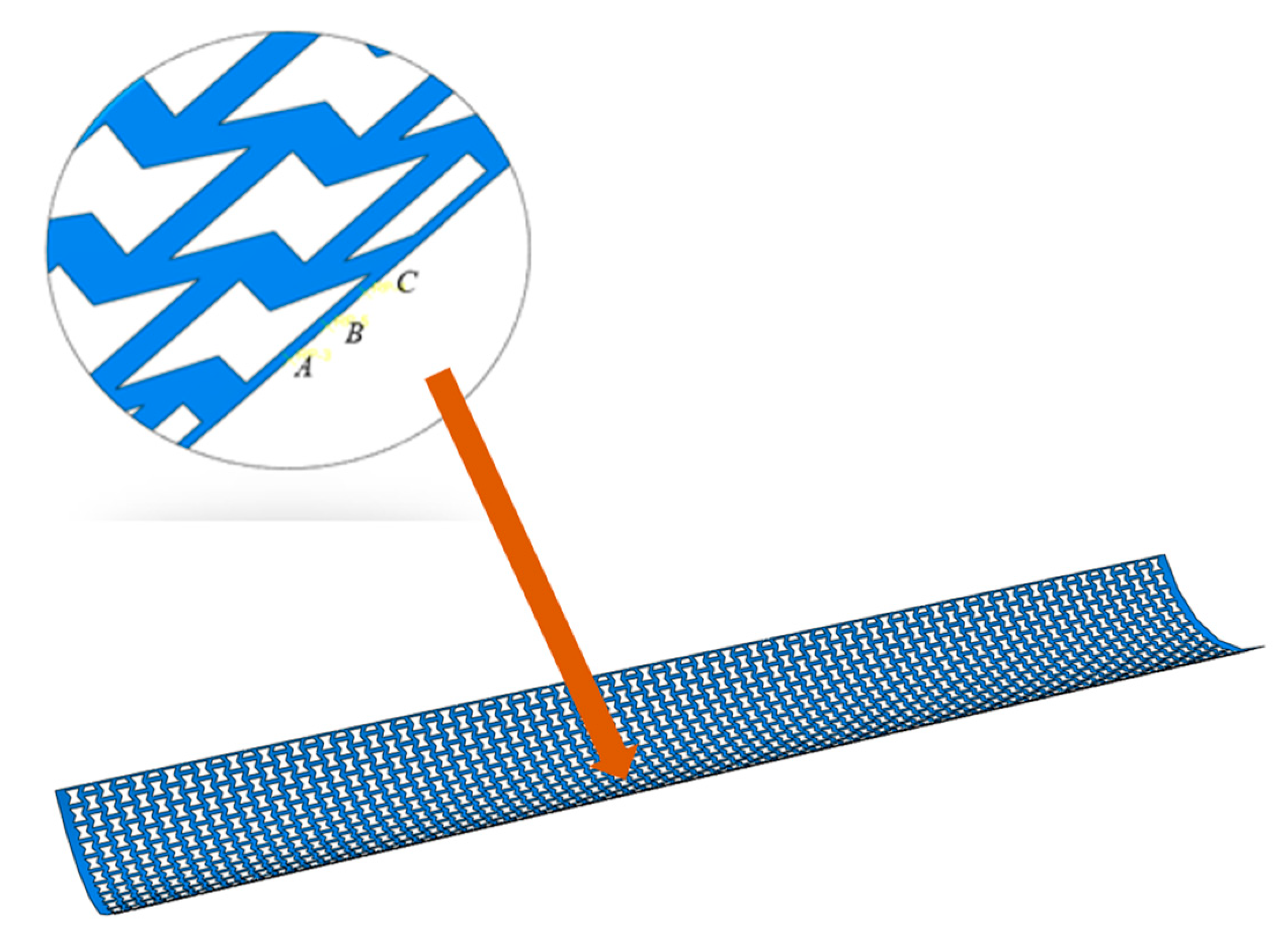
| Parameters | Star-Shaped Honeycomb | Auxetic Re-Entrant Honeycomb |
|---|---|---|
| L | 350 | 350 |
| R | 35 | 35 |
| ψ | π/2 | π/2 |
| θ | π/6 | π/6 |
| t0 | 0.3 | 0.3 |
| t | 2 | 2 |
| l | 10 | 10 |
| h | 10 | 8.88 |
| Young’s Modulus | 210 GPa | |
| Poisson’s ratio | 0.3 | |
| Parameter | t | to | M | Mass | Unit | ||
|---|---|---|---|---|---|---|---|
| Model | |||||||
| Tape spring | \ | \ | \ | 950.3 | 53.625 | 17.72 | |
| Star shaped honeycomb | 2 | 0.3 | 0.52 | 17.2 | 7.54 | 2.28 | |
| Auxetic re-entrant honeycomb | 2 | 0.3 | 1.046 | 305.7 | 12.09 | 25.29 | |
| Parameter | t | to | M | Mass | Unit | ||
|---|---|---|---|---|---|---|---|
| Model | |||||||
| Initial model | 2 | 0.3 | 1.046 | 305.7 | 12.09 | 25.29 | |
| Optimization model | 2.04 | 0.298 | 1.04 | 301.6 | 9.11 | 33.11 | |
| Parameters | Data |
|---|---|
| Length L/mm | 350 |
| The radius of the central corner R/mm | 35 |
| The central angle of cross section α (rad) | 1.57 |
| Depth t/(mm) | 0.3 |
| Location | Boundary Conditions |
|---|---|
| Centroid of the cross-section P | U1 = U2 = UR2 = UR3 = 0, UR1 = 1.57 |
| Centroid of the cross-section Q | U1 = U2 = U2 = UR2 = UR3 = 0, UR1 = −1.57 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Y.; Wang, F.; Liu, J. The Exact Solution of the Bending Moment in the Folding Process of Negative Poisson’s Ratio Honeycomb Tape Spring and Multi-Objective Optimization Design. Aerospace 2023, 10, 390. https://doi.org/10.3390/aerospace10050390
Yang Y, Wang F, Liu J. The Exact Solution of the Bending Moment in the Folding Process of Negative Poisson’s Ratio Honeycomb Tape Spring and Multi-Objective Optimization Design. Aerospace. 2023; 10(5):390. https://doi.org/10.3390/aerospace10050390
Chicago/Turabian StyleYang, Yang, Fan Wang, and Jieshan Liu. 2023. "The Exact Solution of the Bending Moment in the Folding Process of Negative Poisson’s Ratio Honeycomb Tape Spring and Multi-Objective Optimization Design" Aerospace 10, no. 5: 390. https://doi.org/10.3390/aerospace10050390
APA StyleYang, Y., Wang, F., & Liu, J. (2023). The Exact Solution of the Bending Moment in the Folding Process of Negative Poisson’s Ratio Honeycomb Tape Spring and Multi-Objective Optimization Design. Aerospace, 10(5), 390. https://doi.org/10.3390/aerospace10050390






