Investigation of Very Large Eddy Simulation Method for Applications of Supersonic Turbulent Combustion
Abstract
1. Introduction
2. Numerical Methodology
2.1. Governing Equations
2.2. Shear-Stress Transport Model
2.3. Very Large Eddy Simulation
2.4. Improved Delayed Detached Eddy Simulation
2.5. Hybrid Partially Stirred Reactor/Ingenito Supersonic Combustion Model
2.6. Discretization Method
3. Results and Discussion
3.1. Case 1: Settles Supersonic Ramped Cavity
3.1.1. Case Setup of the Supersonic Ramped Cavity
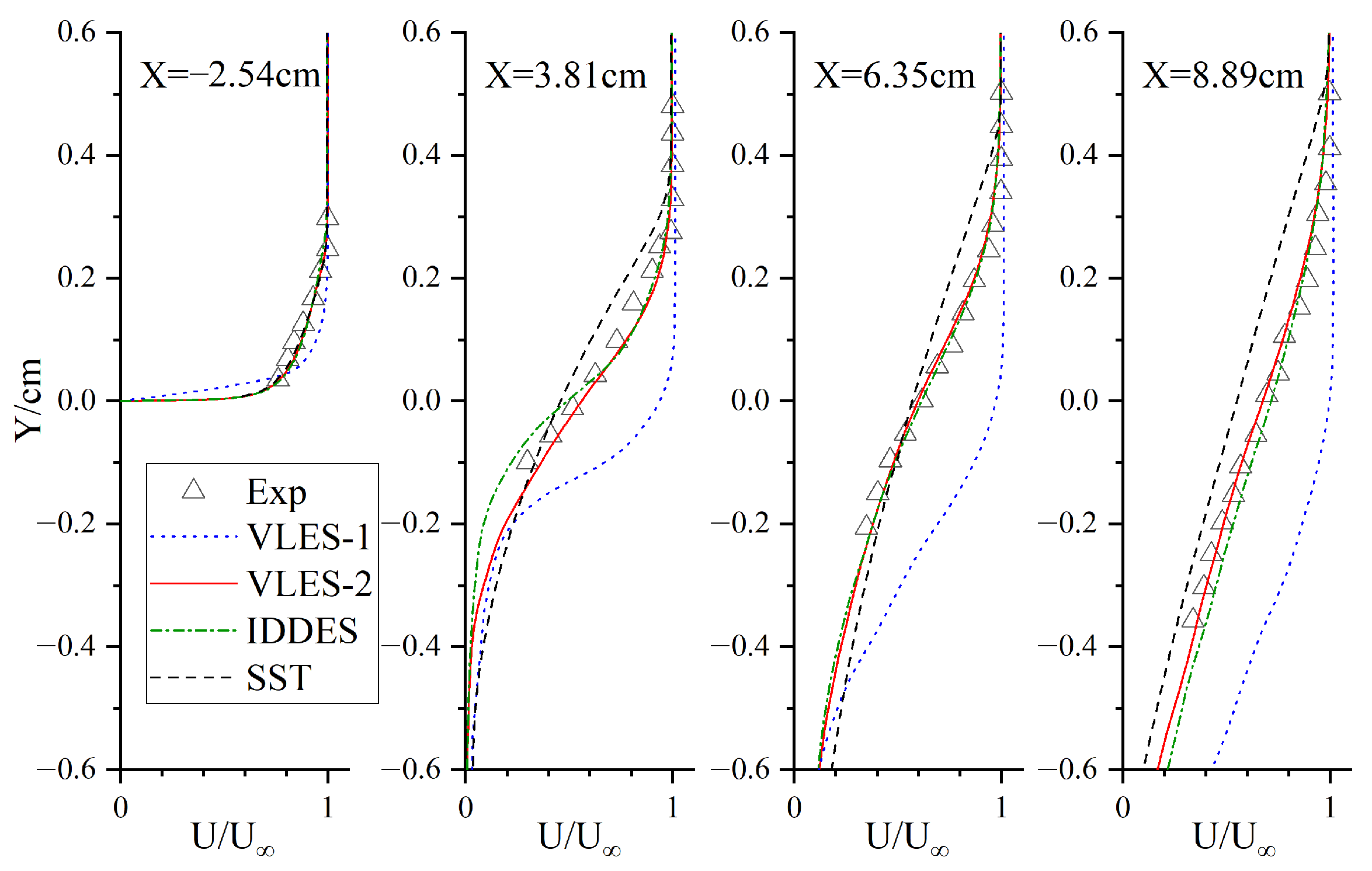
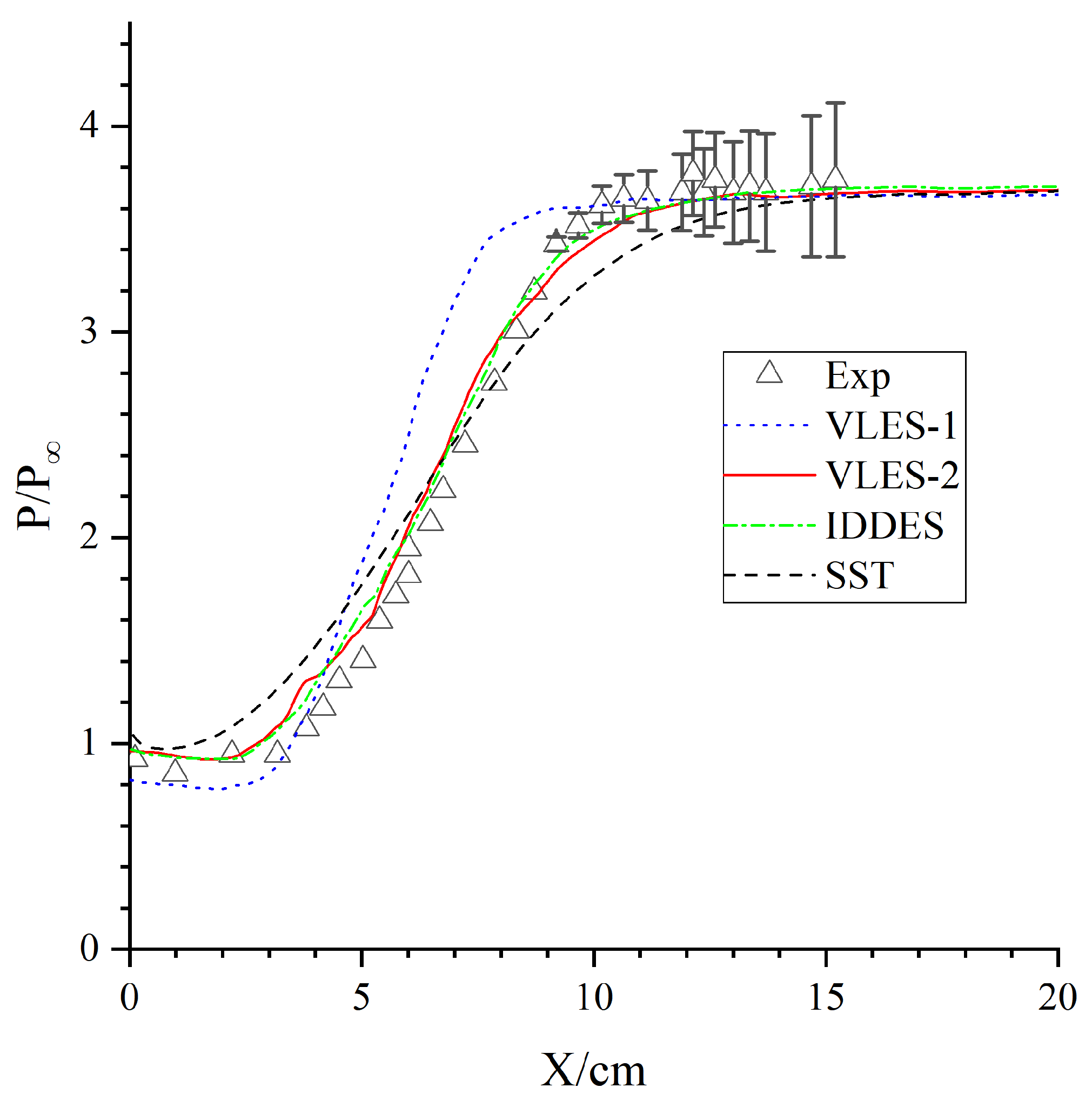
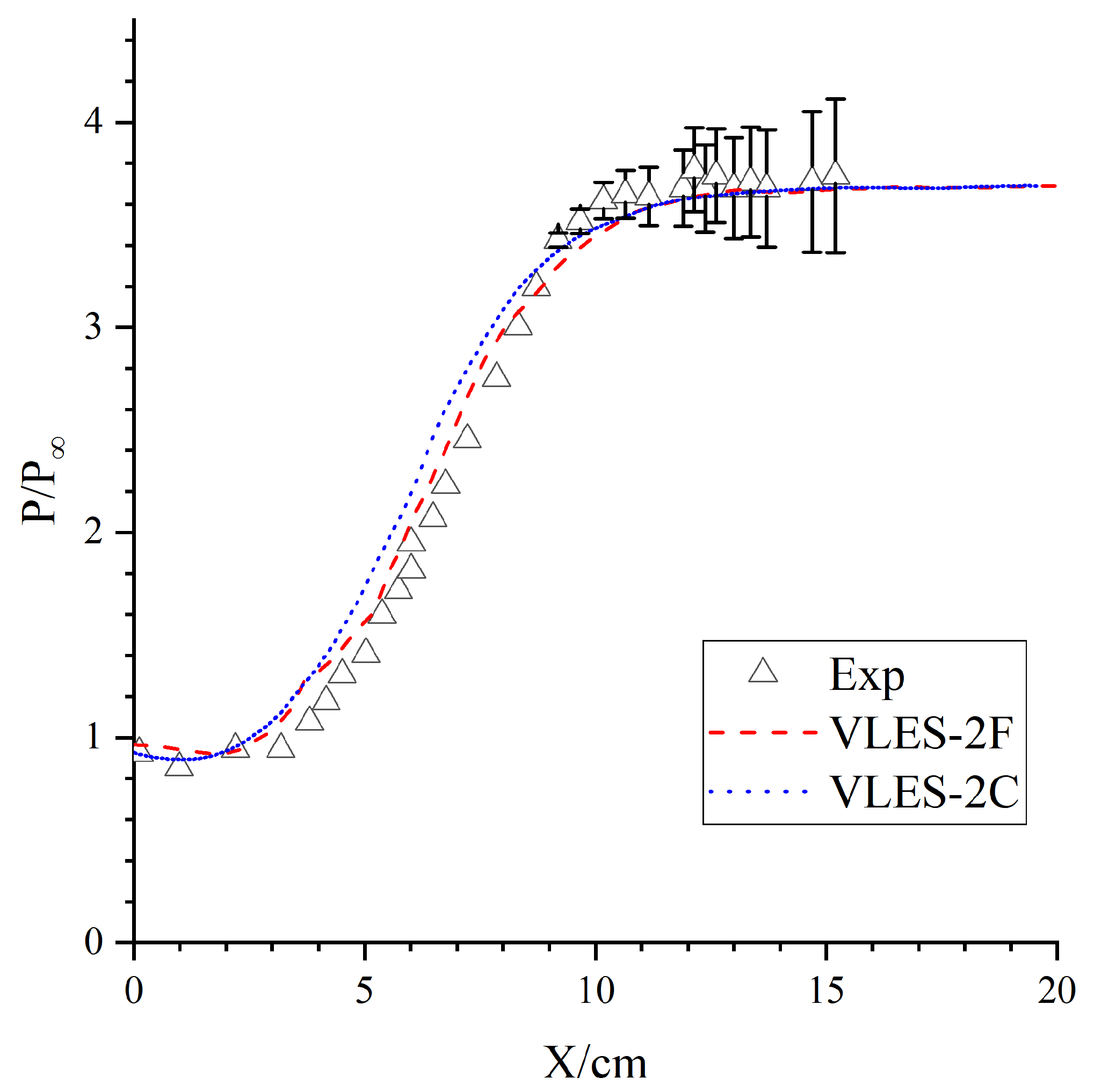
3.1.2. Validation with Experimental Data of the Supersonic Ramped Cavity
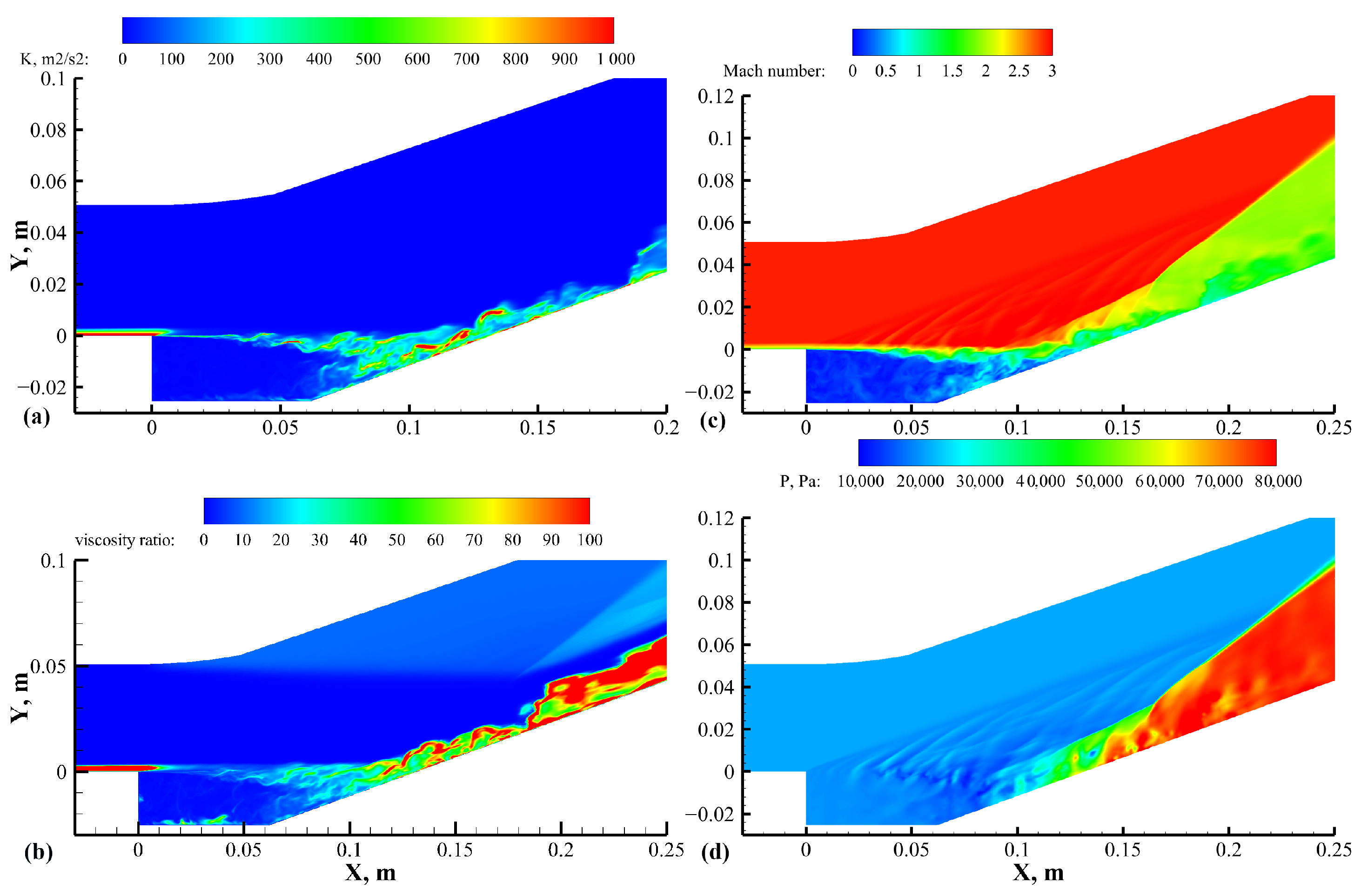
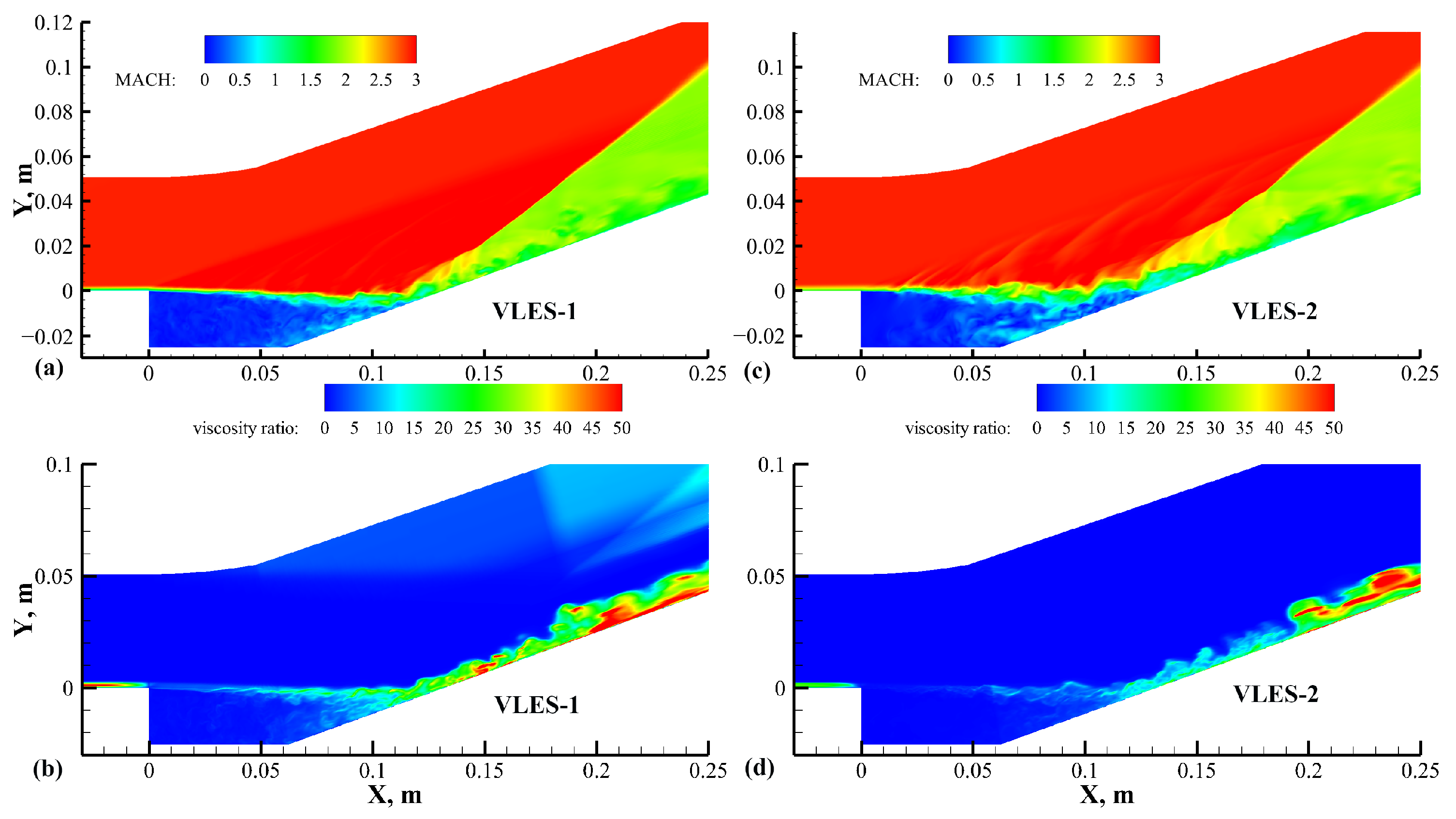
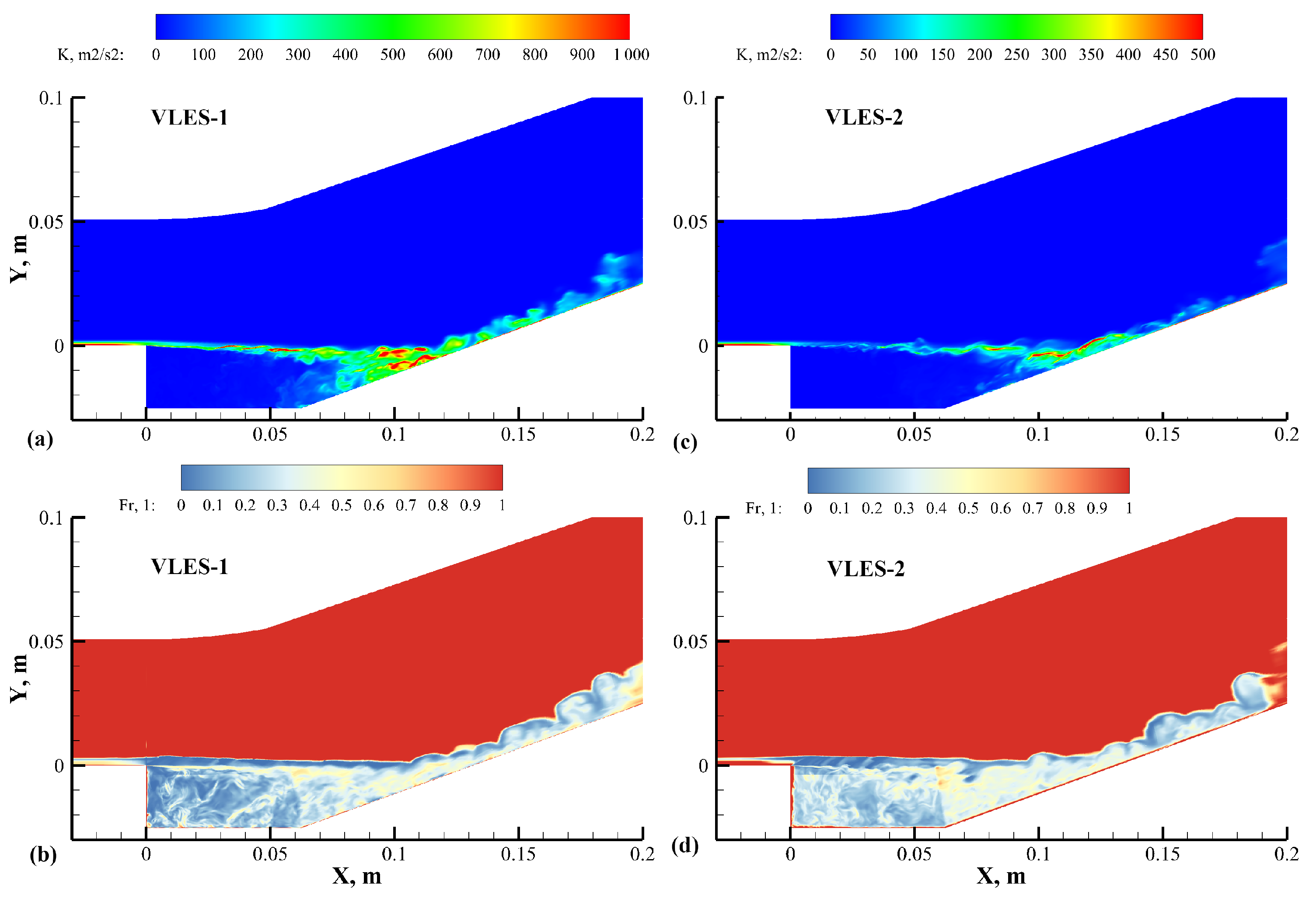
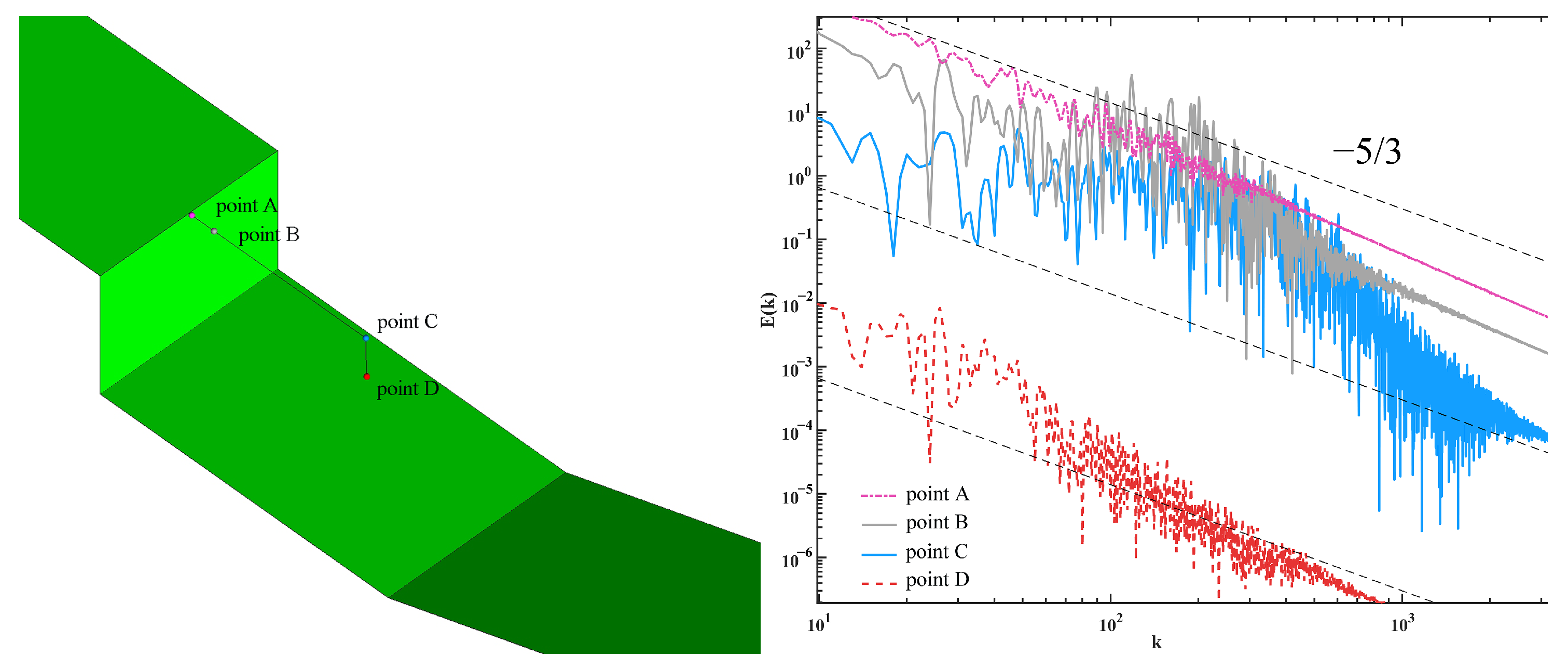
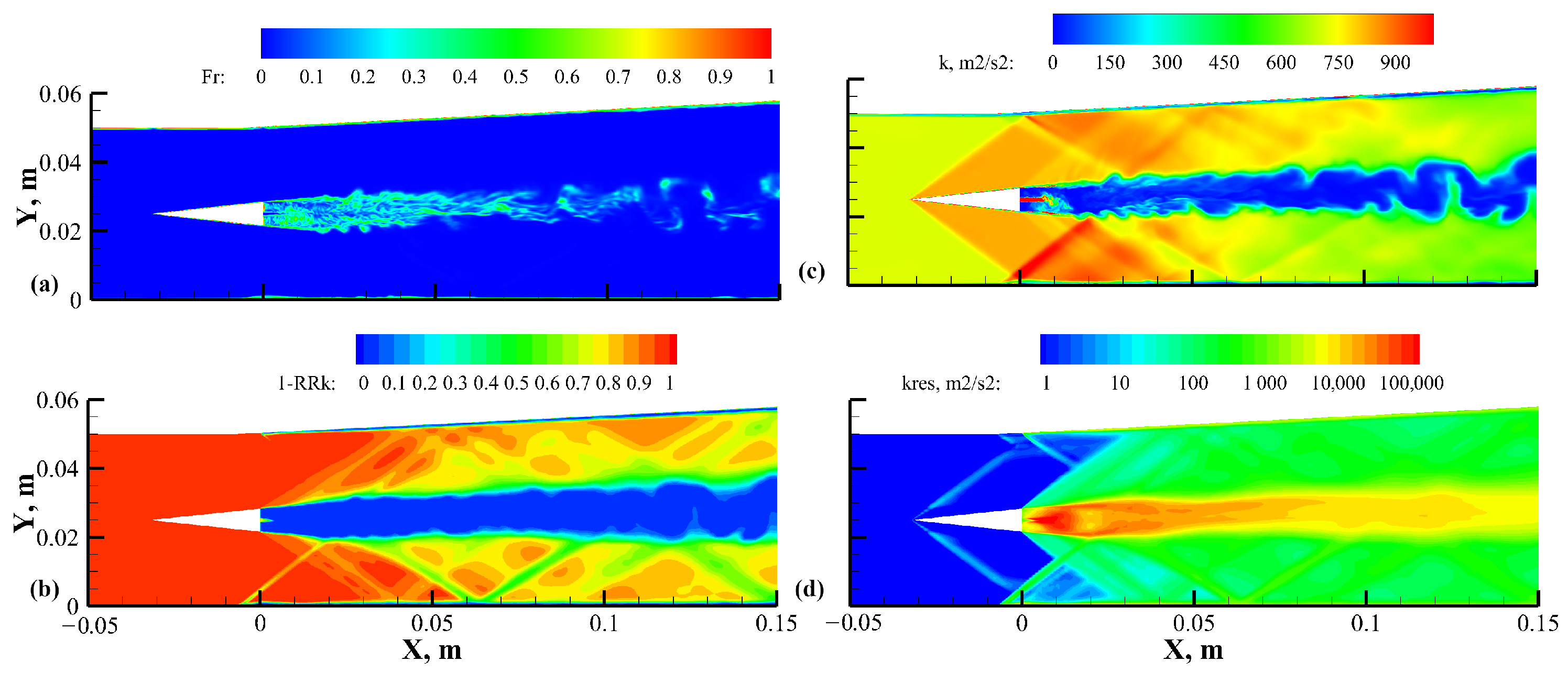
3.1.3. Analysis of Flow Features
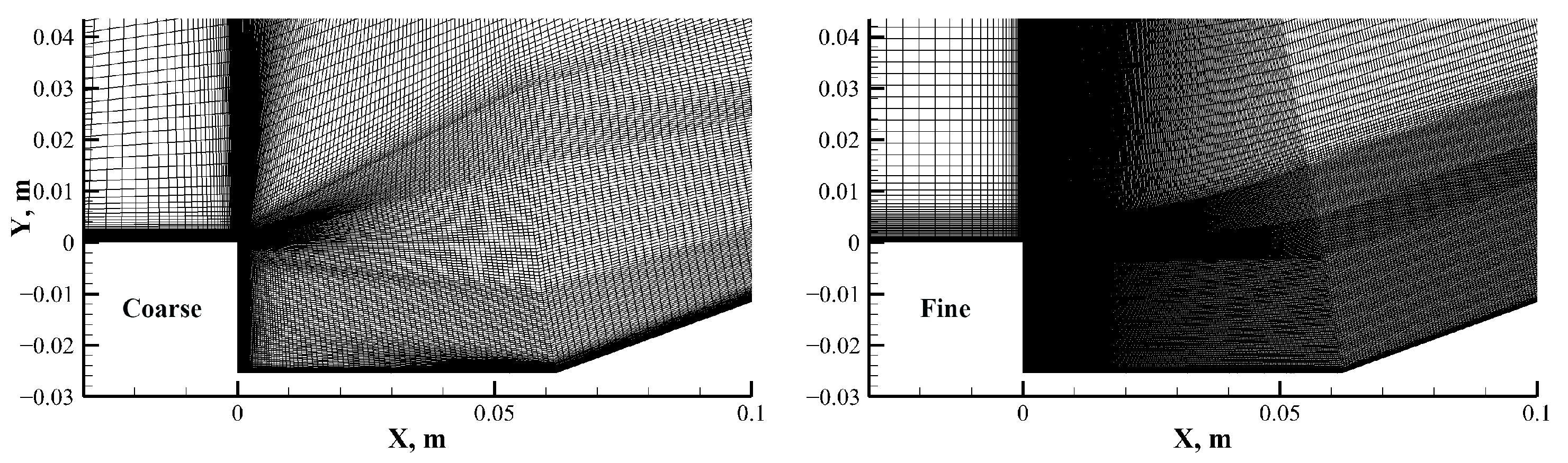
3.1.4. Grid Sensitivity Test
3.2. Case 2: DLR Supersonic Strut-Based Flame
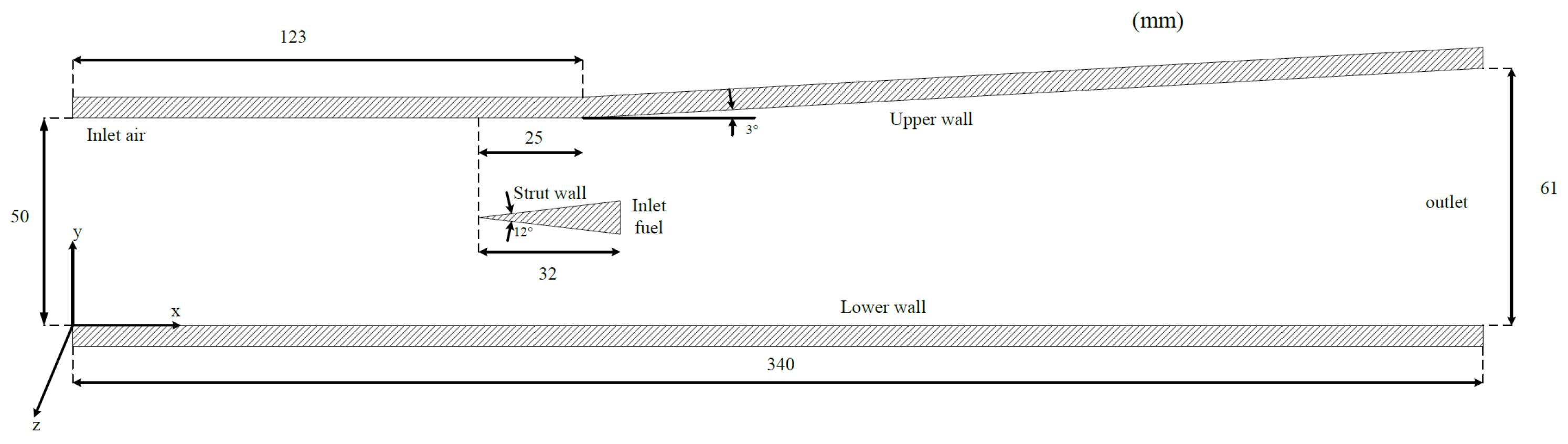
3.2.1. Case Setup of the Supersonic Strut-Based Flame
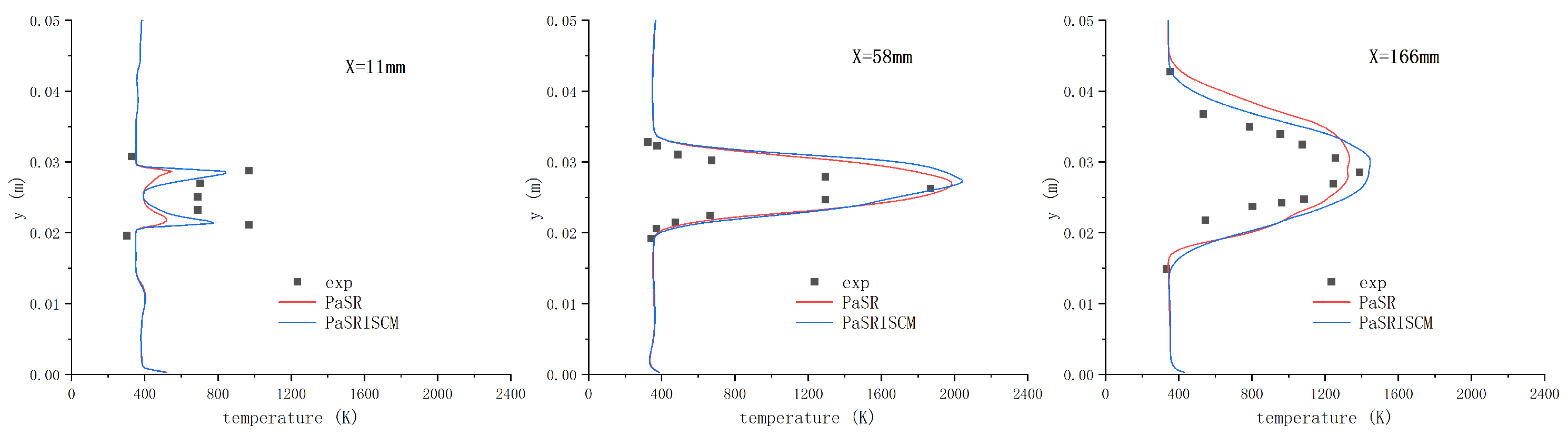
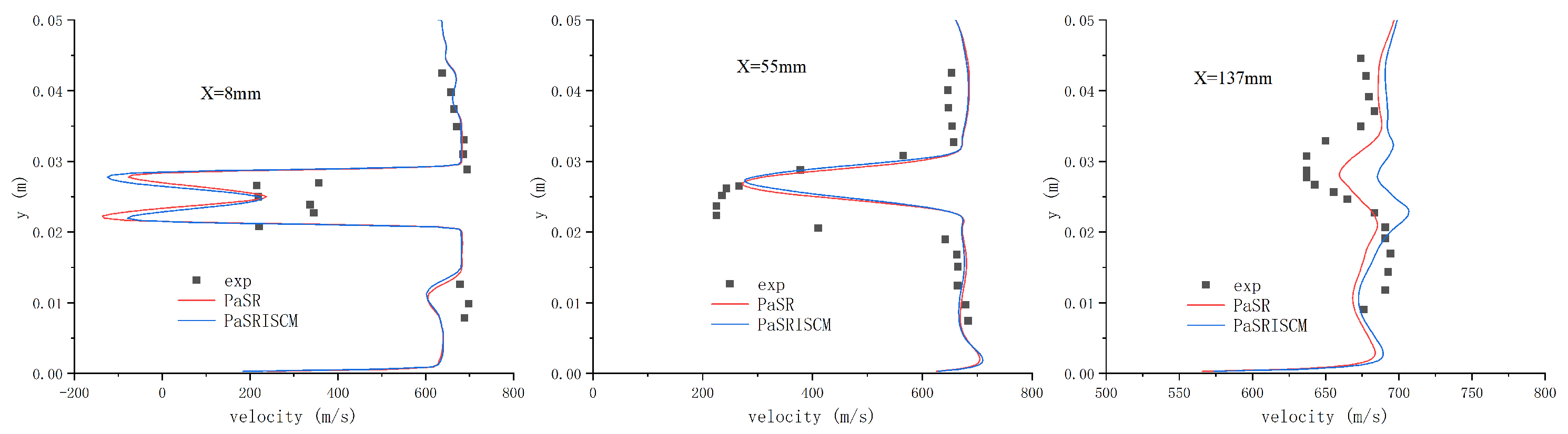
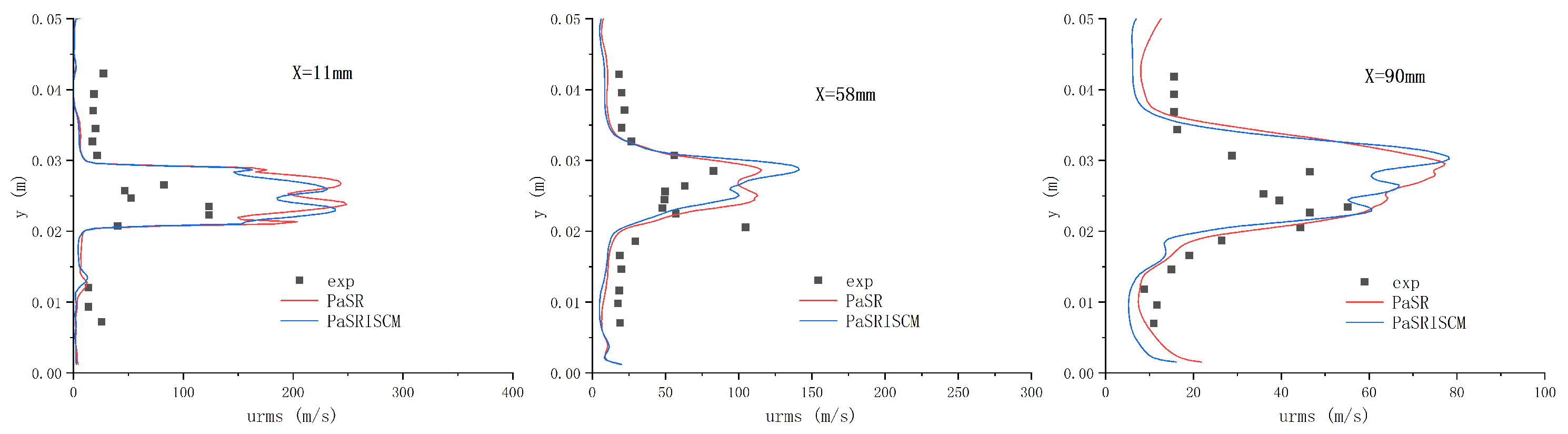
3.2.2. Validation with Experimental Data of the Supersonic Strut-Based Flame
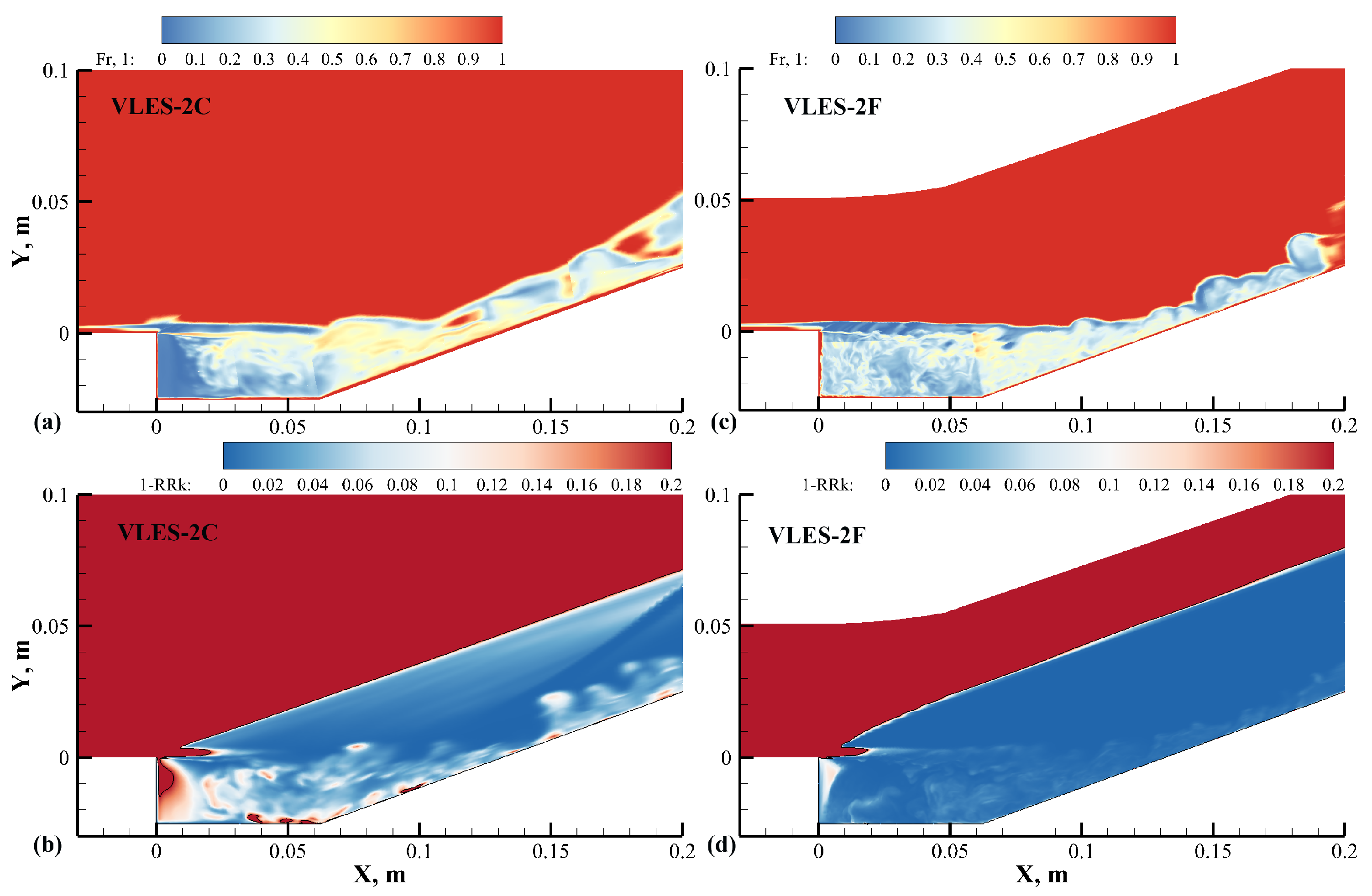
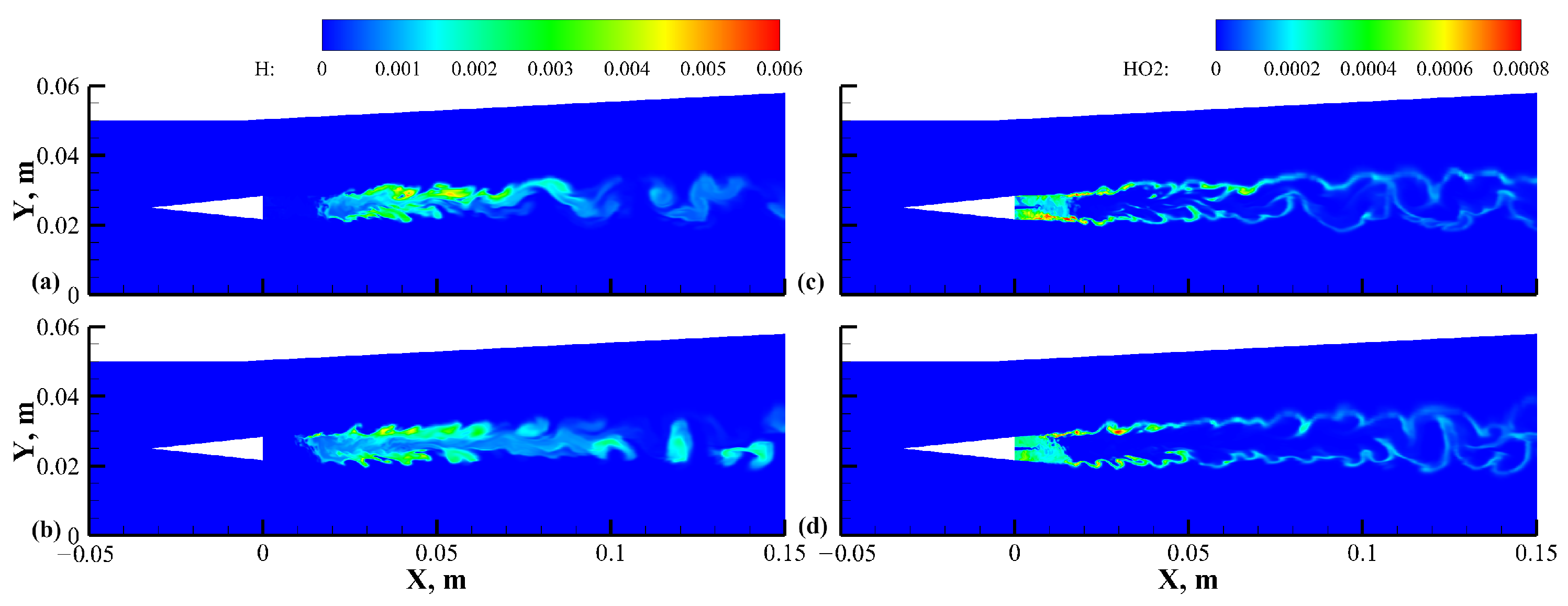
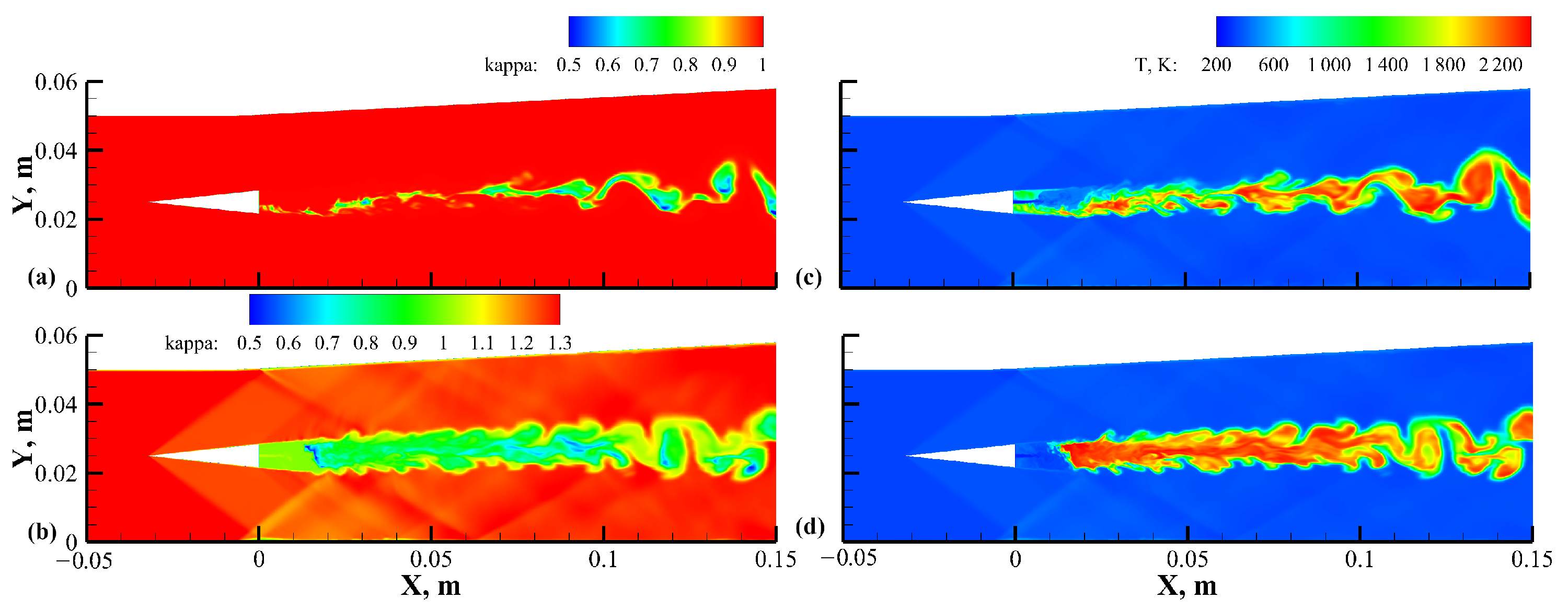
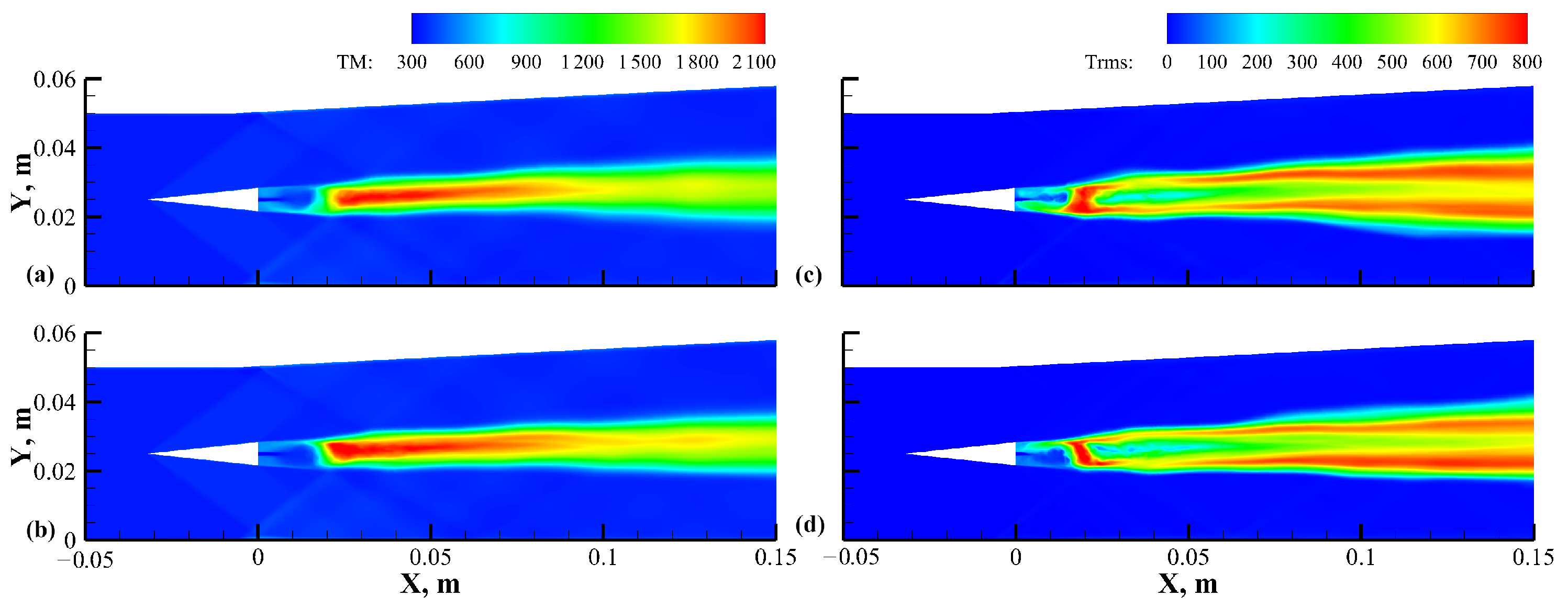
3.2.3. Analysis of Flame Features
3.2.4. Performance of the VLES Model
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations and Nomenclatures
| VLES | Very large eddy simulation |
| IDDES | Improved delayed detached eddy simulation |
| SLA | Shear layer adaptive |
| PaSR | Partially stirred reactor |
| ISCM | Ingenito supersonic combustion model |
| LES | Large eddy simulation |
| RANS | Reynolds-averaged Navier–Stokes simulation |
| DNS | Direct numerical simulation |
| DES | Detached eddy simulation |
| PANS | Partial averaged Navier–Stokes |
| WMLES | Wall-modeled LES |
| RSC-LES | Reynolds-stress-constrained large eddy simulation |
| MSD | Modeled stress diminishing |
| GIS | Grid-induced separation |
| TVD | Total variation diminishing |
| SST | Shear-stress transport |
| LLM | Logarithmic-law mismatch |
| HLLC | Harten, Lax, and van Leer Contact |
| LUSGS | Lower-upper symmetric Gauss-Seidel |
| ODEs | Ordinary differential equations |
| CFL | Courant–Friedrichs–Lewy |
| DLR | The German Aerospace Center |
| Mass density, kg/m3 | |
| u | Velocity, m/s |
| p | Pressure, kg/ |
| T | Temperature, K |
| E | Total energy, |
| e | Internal energy, |
| H | Total enthalpy, |
| h | Internal enthalpy, |
| K | Kinetic energy, |
| Viscous tensor, | |
| Mass production rate, | |
| Y | Mass fraction |
| D | Mass diffusion coefficient, |
| Dynamic viscosity, | |
| Kinematic viscosity, | |
| k | Turbulent kinetic energy, |
| Specific turbulent frequency, 1/s | |
| Turbulence dissipation rate, | |
| Prandtl number | |
| Schmidt number | |
| Thermal conductivity, | |
| Kronecker operator | |
| Strain rate tensor, 1/s | |
| a | Sound speed, m/s |
| Turbulent Mach number | |
| Resolution control function | |
| Volume-averaging length scale, m | |
| Maximum length scale, m | |
| Length scale in the vorticity direction, m | |
| IDDES grid length scale, m | |
| Wall distance, m | |
| Delaying factor | |
| Elevating factor | |
| Timescale, s | |
| Superscripts | |
| Reynolds average or filtered average | |
| Favre average or Favre filtered average | |
| Subscripts | |
| t | Turbulent |
| m | Specie |
| c | Cut-off |
| i | Integral |
| k | Kolmogorov |
| s | Smagorinsky |
Appendix A. Chemical Timescale
References
- Waidmann, W.; Alff, F.; Bohm, M.; Brummund, U.; Clauss, W.; Oschwald, M. Supersonic combustion of hydrogen air in a scramjet combustion chamber. Space Technol.-Ind. Commer. Appl. 1995, 15, 421–429. [Google Scholar]
- Oevermann, M. Numerical investigation of turbulent hydrogen combustion in a Scramjet using flamelet modeling. Aerosp. Sci. Technol. 2000, 4, 463–480. [Google Scholar] [CrossRef]
- Menon, S.; Genin, F.; Chernyavsky, B. Large Eddy Simulation of Scramjet Combustion Using a Subgrid Mixing/combustion Model. In Proceedings of the AIAA International Space Planes and Hypersonic Systems and Technologies, Norfolk, VA, USA, 15–19 December 2003. [Google Scholar] [CrossRef]
- Goyne, C.P.; Rodriguez, C.G.; Krauss, R.H.; McDaniel, J.C.; McClinton, C.R. Experimental and numerical study of a dual-mode scramjet combustor. J. Propuls. Power 2006, 22, 481–489. [Google Scholar] [CrossRef]
- Vanyai, T.; Grieve, S.; Street, O.; Denman, Z.; McIntyre, T.; Veeraragavan, A.; Wheatley, V.; Smart, M. Fundamental Scramjet Combustion Experiments Using Hydrocarbon Fuel. J. Propuls. Power 2019, 35, 953–963. [Google Scholar] [CrossRef]
- Wu, J.; Wang, Z.; Bai, X.; Sun, M.; Wang, H. The hybrid RANS/LES of partially premixed supersonic combustion using G/Z flamelet model. Acta Astronaut. 2016, 127, 375–383. [Google Scholar] [CrossRef]
- Bose, S.T.; Park, G.I. Wall-Modeled Large-Eddy Simulation for Complex Turbulent Flows. Annu. Rev. Fluid Mech. 2018, 50, 535–561. [Google Scholar] [CrossRef]
- Piomelli, U. Large-eddy simulation: Achievements and challenges. Prog. Aerosp. Sci. 1999, 35, 335–362. [Google Scholar] [CrossRef]
- Edwards, J.; Boles, J.; Baurle, R. Large-eddy/Reynolds-averaged Navier–Stokes simulation of a supersonic reacting wall jet. Combust. Flame 2012, 159, 1127–1138. [Google Scholar] [CrossRef]
- Fulton, J.A.; Edwards, J.R.; Hassan, H.A.; McDaniel, J.C.; Goyne, C.P.; Rockwell, R.D.; Cutler, A.D.; Johansen, C.T.; Danehy, P.M. Large-eddy/Reynolds-averaged Navier–Stokes simulations of reactive flow in dual-mode scramjet combustor. J. Propuls. Power 2014, 30, 558–575. [Google Scholar] [CrossRef]
- Peterson, D.M.; Hassan, E.; Bornhoft, B. Influence of Periodicity on Simulations of a Round Supersonic Combustor Using Hybrid RANS/LES. In Proceedings of the AIAA Scitech 2020 Forum, Orlando, FL, USA, 6–10 January 2020. [Google Scholar]
- Heinz, S. A review of hybrid RANS-LES methods for turbulent flows: Concepts and applications. Prog. Aerosp. Sci. 2020, 114, 100597. [Google Scholar] [CrossRef]
- Spalart, P.R.; Jou, W.H.; Strelets, M.; Allmaras, S.R. Comments on the feasibility of LES for winds, and on a hybrid RANS/LES approach. In Proceedings of the Advances in DNS/LES, Louisiana Tedti University, Ruston, LA, USA, 4–8 August 1997. [Google Scholar]
- Spalart, P.R.; Deck, S.; Shur, M.L.; Squires, K.D.; Strelets, M.K.; Travin, A. A new version of detached-eddy simulation, resistant to ambiguous grid densities. Theor. Comput. Fluid Dyn. 2006, 20, 181–195. [Google Scholar] [CrossRef]
- Shur, M.; Spalart, P.; Strelets, M.; Travin, A. A hybrid RANS-LES approach with delayed-DES and wall-modelled LES capabilities. Int. J. Heat Fluid Flow 2008, 29, 1638–1649. [Google Scholar] [CrossRef]
- Speziale, C.G. Turbulence Modeling for Time-Dependent RANS and VLES: A Review. AIAA J. 1998, 36, 173–184. [Google Scholar] [CrossRef]
- Girimaji, S.S. Partially-Averaged Navier-Stokes Model for Turbulence: A Reynolds-Averaged Navier-Stokes to Direct Numerical Simulation Bridging Method. J. Appl. Mech. 2006, 73, 413–421. [Google Scholar] [CrossRef]
- Dzanic, T.; Girimaji, S.S.; Witherden, F.D. Partially-averaged Navier–Stokes simulations of turbulence within a high-order flux reconstruction framework. J. Comput. Phys. 2022, 456, 110992. [Google Scholar] [CrossRef]
- Chen, S.; Xia, Z.; Pei, S.; Wang, J.; Yang, Y.; Xiao, Z.; Shi, Y. Reynolds-Stress-Constrained Large-Eddy Simulation of wall-bounded turbulent flows. J. Fluid Mech. 2012, 703, 1–28. [Google Scholar] [CrossRef]
- Fan, T.; Xiao, X.; Edwards, J.; Hassan, H.; Baurle, R. Hybrid LES/RANS simulation of a shock wave/boundary layer interaction. In Proceedings of the 41st Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 14–17 January 2002. [Google Scholar] [CrossRef]
- Sánchez-Rocha, M.; Menon, S. The compressible hybrid RANS/LES formulation using an additive operator. J. Comput. Phys. 2009, 228, 2037–2062. [Google Scholar] [CrossRef]
- Wang, H.; Shan, F.; Piao, Y.; Hou, L.; Niu, J. IDDES simulation of hydrogen-fueled supersonic combustion using flamelet modeling. Int. J. Hydrogen Energy 2015, 40, 683–691. [Google Scholar] [CrossRef]
- Won, S.H.; Jeung, I.S.; Parent, B.; Choi, J.Y. Numerical Investigation of Transverse Hydrogen Jet into Supersonic Crossflow Using Detached-Eddy Simulation. AIAA J. 2010, 48, 1047–1058. [Google Scholar] [CrossRef]
- Spalart, P.R.; Shur, M.L.; Strelets, M.K.; Travin, A.K. Initial noise predictions for rudimentary landing gear. J. Sound Vib. 2011, 330, 4180–4195. [Google Scholar] [CrossRef]
- Garbaruk, A.V.; Spalart, P.R.; Strelets, M.K.; Shur, M.L. Flow and noise prediction for tandem cylinder. Matem. Mod. 2014, 26, 119–136. [Google Scholar]
- Shur, M.L.; Spalart, P.R.; Strelets, M.K.; Travin, A.K. An Enhanced Version of DES with Rapid Transition from RANS to LES in Separated Flows. Flow Turbul. Combust. 2015, 95, 709–737. [Google Scholar] [CrossRef]
- Han, X.; Krajnovic, S. Very-large-eddy simulation based on k-omega model. AIAA J. 2015, 53, 1103-U8. [Google Scholar] [CrossRef]
- Launder, B.E.; Spalding, D.B. The numerical computation of turbulent flows. Comput. Methods Appl. Mech. Eng. 1974, 3, 269–289. [Google Scholar] [CrossRef]
- Wilcox, D.C. Reassessment of the scale-determining equation for advanced turbulence models. AIAA J. 1988, 26, 1299–1310. [Google Scholar] [CrossRef]
- Xia, Z.; Cheng, Z.; Han, X.; Mao, J. VLES turbulence modelling for separated flow simulation with OpenFOAM. J. Wind. Eng. Ind. Aerodyn. 2020, 198, 104077. [Google Scholar] [CrossRef]
- Huang, Z.; Guo, T.; Han, X.; Mao, J. Very Large Eddy Simulation of Lean Premixed Flames to Imposed Inlet Velocity Oscillations. Combust. Sci. Technol. 2022, 194, 2933–2956. [Google Scholar] [CrossRef]
- Jin, Y.; Lu, L.; Huang, Z.; Han, X. Numerical investigation of flat-plate film cooling using Very-Large Eddy Simulation method. Int. J. Therm. Sci. 2022, 171, 107263. [Google Scholar] [CrossRef]
- Wan, P.; Han, X.; Mao, J. Very Large Eddy Simulation of turbulent flow and heat transfer for single cylinder and cylindrical pin matrix. Appl. Therm. Eng. 2020, 169, 114972. [Google Scholar] [CrossRef]
- Xia, Z.; Han, X.; Mao, J. Assessment and Validation of Very-Large-Eddy Simulation Turbulence Modeling for Strongly Swirling Turbulent Flow. AIAA J. 2019, 58, 148–163. [Google Scholar] [CrossRef]
- Tiwari, P.; Xia, Z.; Han, X. Comparison of VLES and LES Turbulence Modeling for Swirling Turbulent Flow. J. Appl. Fluid Mech. 2020, 13, 1107–1116. [Google Scholar] [CrossRef]
- Harten, A. The artificial compression method for computation of shocks and contact discontinuities. III—Self-adjusting hybrid schemes. Math. Comput. 1978, 32, 363–389. [Google Scholar]
- Pirozzoli, S. Conservative Hybrid Compact-WENO Schemes for Shock-Turbulence Interaction. J. Comput. Phys. 2002, 178, 81–117. [Google Scholar] [CrossRef]
- Ducros, F.; Ferrand, V.; Nicoud, F.; Weber, C.; Darracq, D.; Gacherieu, C.; Poinsot, T. Large-eddy simulation of the shock turbulence interaction. J. Comput. Phys. 1999, 152, 517–549. [Google Scholar] [CrossRef]
- Hill, D.J.; Pullin, D.I. Hybrid tuned center-difference-WENO method for large eddy simulations in the presence of strong shocks. J. Comput. Phys. 2004, 194, 435–450. [Google Scholar] [CrossRef]
- Menter, F. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Heinz, S. A model for the reduction of the turbulent energy redistribution by compressibility. Phys. Fluids 2003, 15, 3580–3583. [Google Scholar] [CrossRef]
- Sarkar, S. The pressure–dilatation correlation in compressible flows. Phys. Fluids A Fluid Dyn. 1992, 4, 2674–2682. [Google Scholar] [CrossRef]
- Horstman, C.C.; Settles, G.S.; Williams, D.R.; Bogdonoff, S.M. A Reattaching Free Shear Layer in Compressible Turbulent Flow. AIAA J. 1982, 20, 79–85. [Google Scholar] [CrossRef]
- Wu, W.; Piao, Y.; Xie, Q.; Ren, Z. Flame diagnostics with a conservative representation of chemical explosive mode analysis. AIAA J. 2019, 57, 1355–1363. [Google Scholar] [CrossRef]
- Wu, W.; Piao, Y.; Liu, H. Analysis of flame stabilization mechanism in a hydrogen-fueled reacting wall-jet flame. Int. J. Hydrogen Energy 2019, 44, 26609–26623. [Google Scholar] [CrossRef]
- Kee, R.J.; Rupley, F.M.; Miller, J.A. CHEMKIN-III: A FORTRAN chemical kinetics package for the analysis of gas-phase chemical and plasma kinetics. Sandia Rep. 1996, sand96, 142–146. [Google Scholar]
- Argyropoulos, C.D.; Markatos, N.C. Recent advances on the numerical modelling of turbulent flows. Appl. Math. Model. 2015, 39, 693–732. [Google Scholar] [CrossRef]
- Meyers, J.; Sagaut, P. On the model coefficients for the standard and the variational multi-scale Smagorinsky model. J. Fluid Mech. 2006, 569, 287–319. [Google Scholar] [CrossRef]
- Chauvet, N.; Deck, S.; Jacquin, L. Zonal Detached Eddy Simulation of a Controlled Propulsive Jet. AIAA J. 2007, 45, 2458–2473. [Google Scholar] [CrossRef]
- Deck, S. Recent improvements in the Zonal Detached Eddy Simulation (ZDES) formulation. Theor. Comput. Fluid Dyn. 2012, 26, 523–550. [Google Scholar] [CrossRef]
- Chaouat, B. The State of the Art of Hybrid RANS/LES Modeling for the Simulation of Turbulent Flows. Flow Turbul. Combust. 2017, 99, 279–327. [Google Scholar] [CrossRef]
- Sabelnikov, V.; Fureby, C. LES combustion modeling for high Re flames using a multi-phase analogy. Combust. Flame 2013, 160, 83–96. [Google Scholar] [CrossRef]
- Ingenito, A.; Bruno, C. Physics and Regimes of Supersonic Combustion. AIAA J. 2010, 48, 515–525. [Google Scholar] [CrossRef]
- Li, Z.; Ferrarotti, M.; Cuoci, A.; Parente, A. Finite-rate chemistry modelling of non-conventional combustion regimes using a Partially-Stirred Reactor closure: Combustion model formulation and implementation details. Appl. Energy 2018, 225, 637–655. [Google Scholar] [CrossRef]
- Wartha, E.M.; Bösenhofer, M.; Harasek, M. Characteristic Chemical Time Scales for Reactive Flow Modeling. Combust. Sci. Technol. 2020, 193, 2807–2832. [Google Scholar] [CrossRef]
- Golovitchev, V.; Chomiak, J. Numerical modeling of high temperature air flameless combustion. In Proceedings of the the 4th International Symposium on High Temperature Air Combustion and Gasification (HiTACG), Rome, Italy, 26–30 November 2001; pp. 27–30. [Google Scholar]
- Ó Conaire, M.; Curran, H.J.; Simmie, J.; Pitz, W.; Westbrook, C.K. A comprehensive modeling study of hydrogen oxidation. Int. J. Chem. Kinet. 2004, 36, 603–622. [Google Scholar] [CrossRef]
- Toro, E.F. The HLLC Riemann solver. Shock Waves 2019, 29, 1065–1082. [Google Scholar] [CrossRef]
- Ren, Z.; Xu, C.; Lu, T.; Singer, M.A. Dynamic Adaptive Chemistry with Operator Splitting Schemes for Reactive Flow Simulations. J. Comput. Phys. 2014, 263, 19–36. [Google Scholar] [CrossRef]
- Yoon, S.; Jameson, A. Lower-Upper Symmetric-Gauss-Seidel method for the Euler and Navier-Stokes equations. AIAA J. 1988, 26, 1025–1026. [Google Scholar] [CrossRef]
- Xie, Q.; Xiao, Z.; Ren, Z. A spectral radius scaling semi-implicit iterative time stepping method for reactive flow simulations with detailed chemistry. J. Comput. Phys. 2018, 368, 47–68. [Google Scholar] [CrossRef]
- Brown, P.N.; Byrne, G.D.; Hindmarsh, A.C. VODE: A Variable-Coefficient ODE Solver. SIAM J. Sci. Stat. Comput. 1989, 10, 1038–1051. [Google Scholar] [CrossRef]
- Padway, E.; Nishikawa, H. Resolving Confusions over Third-Order Accuracy of Unstructured MUSCL. AIAA J. 2022, 60, 1415–1439. [Google Scholar] [CrossRef]
- Venkatakrishnan, V. Convergence to Steady State Solutions of the Euler Equations on Unstructured Grids with Limiters. J. Comput. Phys. 1995, 118, 120–130. [Google Scholar] [CrossRef]
- Settles, G.S.; Baca, B.K.; Bogdonoff, S.M.; Williams, D.R. Reattachment of a Compressible Turbulent Free Shear Layer. AIAA J. 1982, 20, 60–67. [Google Scholar] [CrossRef]
- Luo, D.; Yan, C.; Wang, X. Computational study of supersonic turbulent-separated flows using partially averaged Navier-stokes method. Acta Astronaut. 2015, 107, 234–246. [Google Scholar] [CrossRef]
- White, J.A.; Baurle, R.A.; Fisher, T.C.; Quinlan, J.R.; Black, W.S. Low-Dissipation Advection Schemes Designed for Large Eddy Simulations of Hypersonic Propulsion Systems. In Proceedings of the 48th AIAA/ASME/SAE/ASEE Joint Propulsion Conference and Exhibit, Atlanta, GA, USA, 30 July–1 August 2012. [Google Scholar] [CrossRef]
- Hunt, J.C.; Wray, A.A.; Moin, P. Eddies, streams, and convergence zones in turbulent flows. In Proceedings of the Summer Programm (Center for Turbulence Research, Stanford University), Stanford, CA, USA, 1 December 1988; pp. 193–208. [Google Scholar]
- Adalsteinsson, G.F.; Kevlahan, N.K.-R. Compressive Sampling for Energy Spectrum Estimation of Turbulent Flows. SIAM J. Sci. Comput. 2015, 37, B452–B472. [Google Scholar] [CrossRef]







| Air | Fuel | |
|---|---|---|
| U (m/s) | 730 | 1200 |
| T (K) | 340 | 250 |
| P (Pa) | 101,325 | 101,325 |
| 0.232 | 0 | |
| 0.736 | 0 | |
| 0 | 1 | |
| 0.032 | 0 |
| Case Name | Turbulence Model | Combustion Model | Reconstruction |
|---|---|---|---|
| M0 | VLES-2 | PaSR | Modified Ducros |
| M1 | VLES-2 | PaSR-ISCM | Original Ducros |
| M2 | VLES-2 | PaSR-ISCM | Modified Ducros |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yan, C.; Xu, Y.; Cao, R.; Piao, Y. Investigation of Very Large Eddy Simulation Method for Applications of Supersonic Turbulent Combustion. Aerospace 2023, 10, 384. https://doi.org/10.3390/aerospace10040384
Yan C, Xu Y, Cao R, Piao Y. Investigation of Very Large Eddy Simulation Method for Applications of Supersonic Turbulent Combustion. Aerospace. 2023; 10(4):384. https://doi.org/10.3390/aerospace10040384
Chicago/Turabian StyleYan, Chong, Yibing Xu, Ruizhe Cao, and Ying Piao. 2023. "Investigation of Very Large Eddy Simulation Method for Applications of Supersonic Turbulent Combustion" Aerospace 10, no. 4: 384. https://doi.org/10.3390/aerospace10040384
APA StyleYan, C., Xu, Y., Cao, R., & Piao, Y. (2023). Investigation of Very Large Eddy Simulation Method for Applications of Supersonic Turbulent Combustion. Aerospace, 10(4), 384. https://doi.org/10.3390/aerospace10040384






