Multi-Objective, Multi-Disciplinary Design Optimization and Multi-Attribute Evaluation of Hybrid Rocket Motors Used for Manned Lunar Lander
Abstract
1. Introduction
2. A Detailed Description of the Problem and Model
2.1. Problem under Study
- (1)
- The diameter and height of the outer envelope of the descent stage shall not exceed 4.2 m and 3.2 m, respectively;
- (2)
- The mass of the descent motor and the complete lunar lander shall not exceed 10,282 kg and 16,000 kg, respectively;
- (3)
- The total impulse and total velocity increment of the descent motor shall not be less than 2.48 × 107 N·s and 2261 m/s, respectively;
- (4)
- During descent and landing, the propulsion system hovering in the weak lunar gravitational field shall meet the requirements on a wider range of thrust regulation.
2.2. Modeling of HRM/LRE-Propelled Lunar Lander
2.2.1. Propulsion
- Hybrid rocket motor
2.2.2. Structure
2.2.3. Trajectory
2.3. Multi-Attribute Evaluation Method
2.3.1. Technical Performance
2.3.2. Non-Technical Performance
- Continuous non-technical attributes: cost
- Liquid rocket engine
- Hybrid rocket motor
- Discrete non-technical attribute modeling analysis method
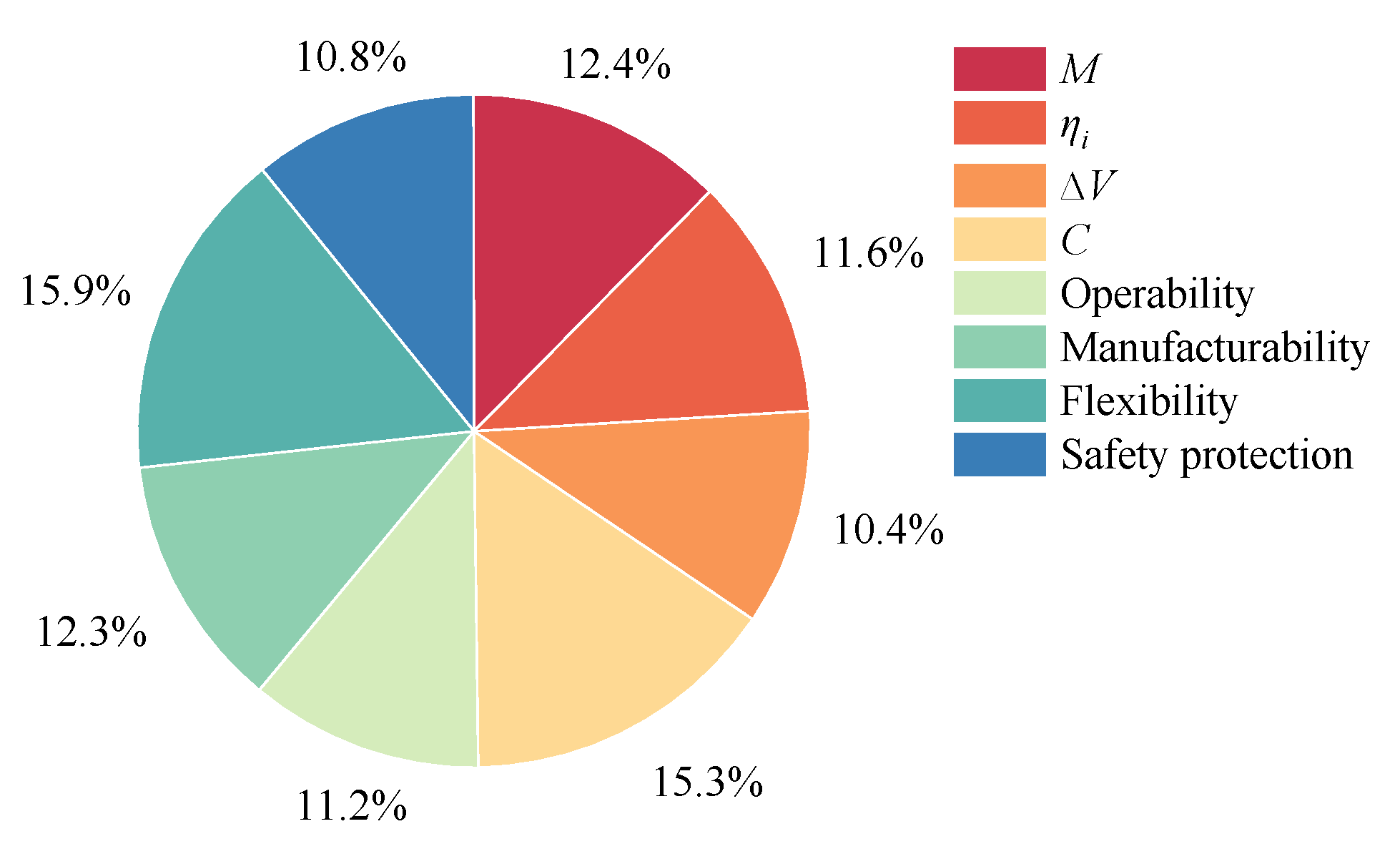
2.3.3. Multi-Attribute Evaluation Strategy Based on Entropy Weight Method-TOPSIS
3. Design Optimization and MAE
3.1. MODO of Manned Lunar Lander
3.2. MAE Process
4. Results and Discussions
4.1. MODO Results
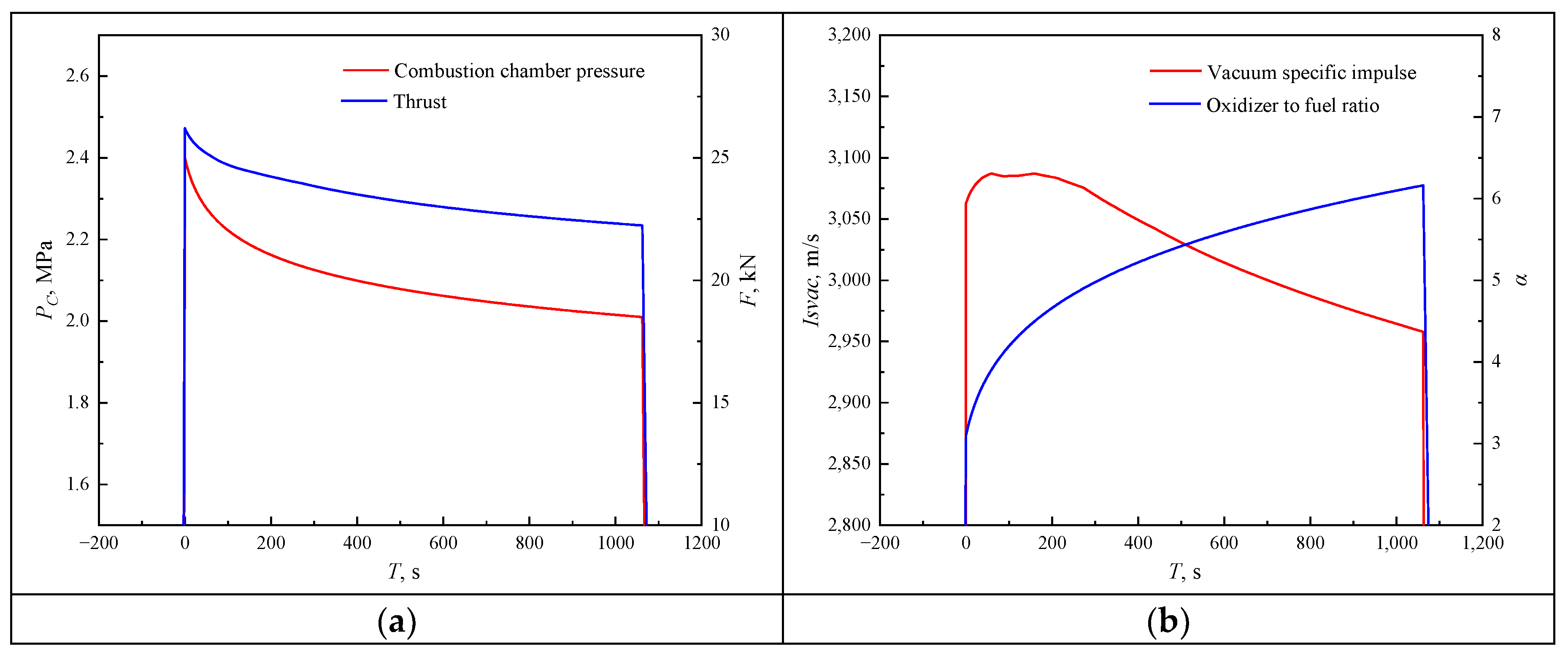
4.1.1. Hybrid Scheme Optimization Results
4.1.2. Liquid Scheme Optimization Results
4.2. Analysis of MAE Results
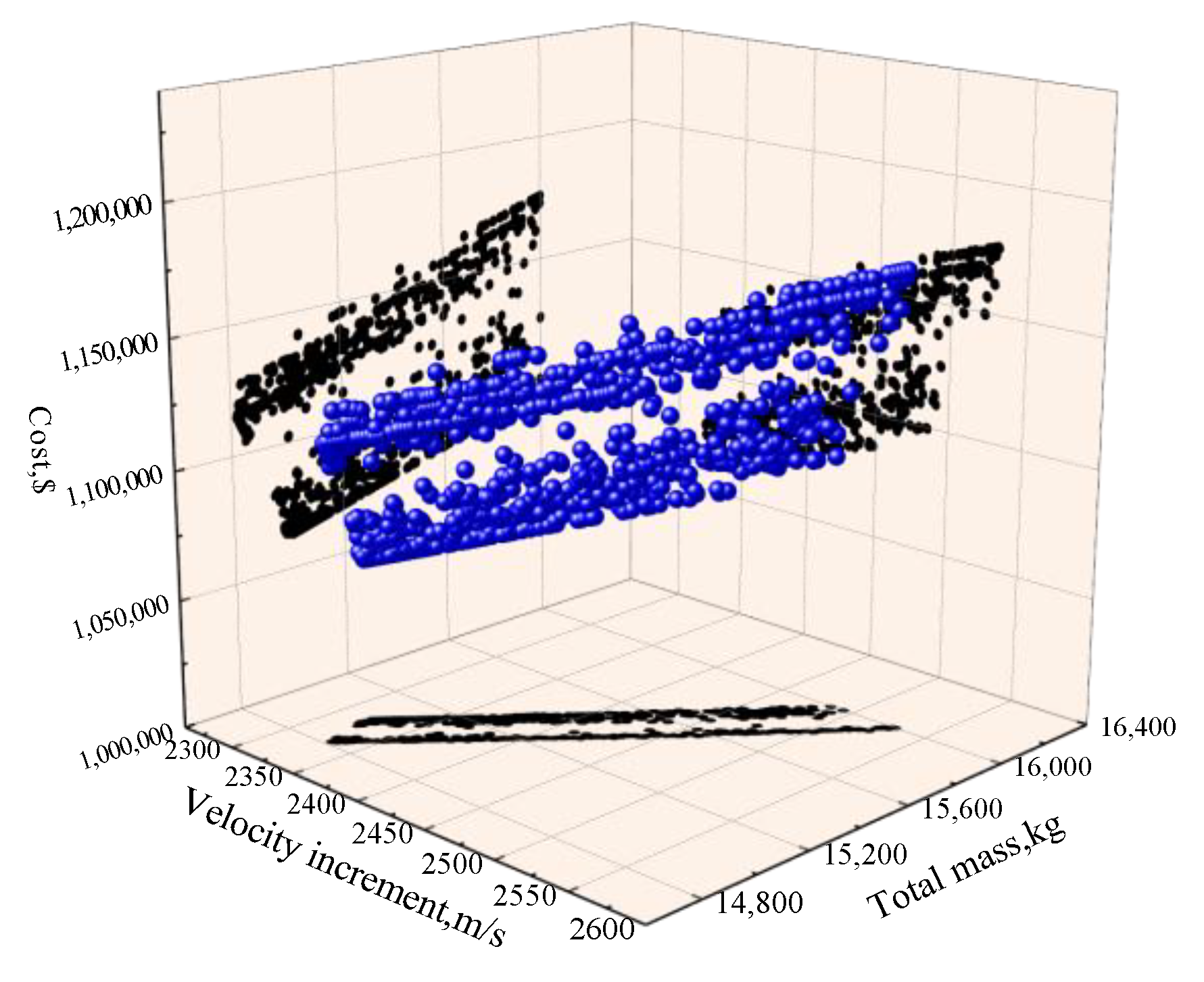
4.2.1. Results of Continuous Attributes Evaluation for All Pareto Front Solutions
4.2.2. The MAE Results
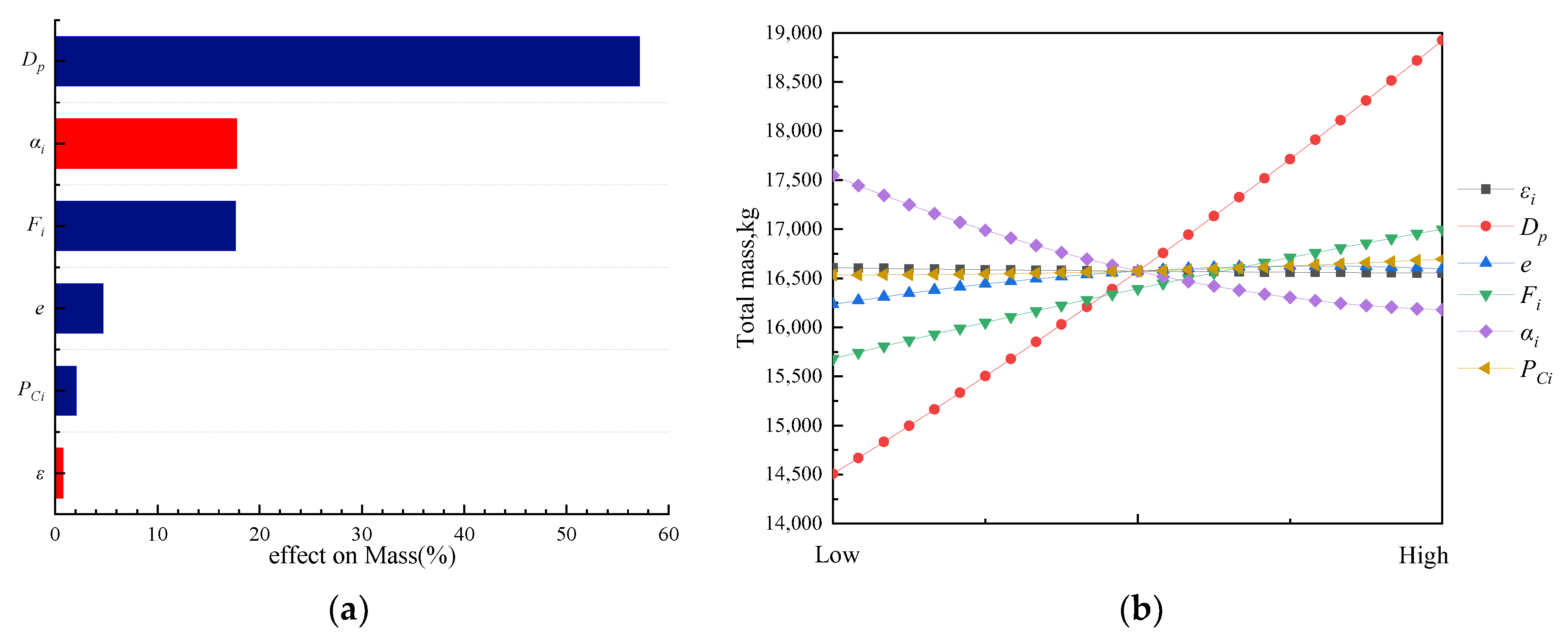
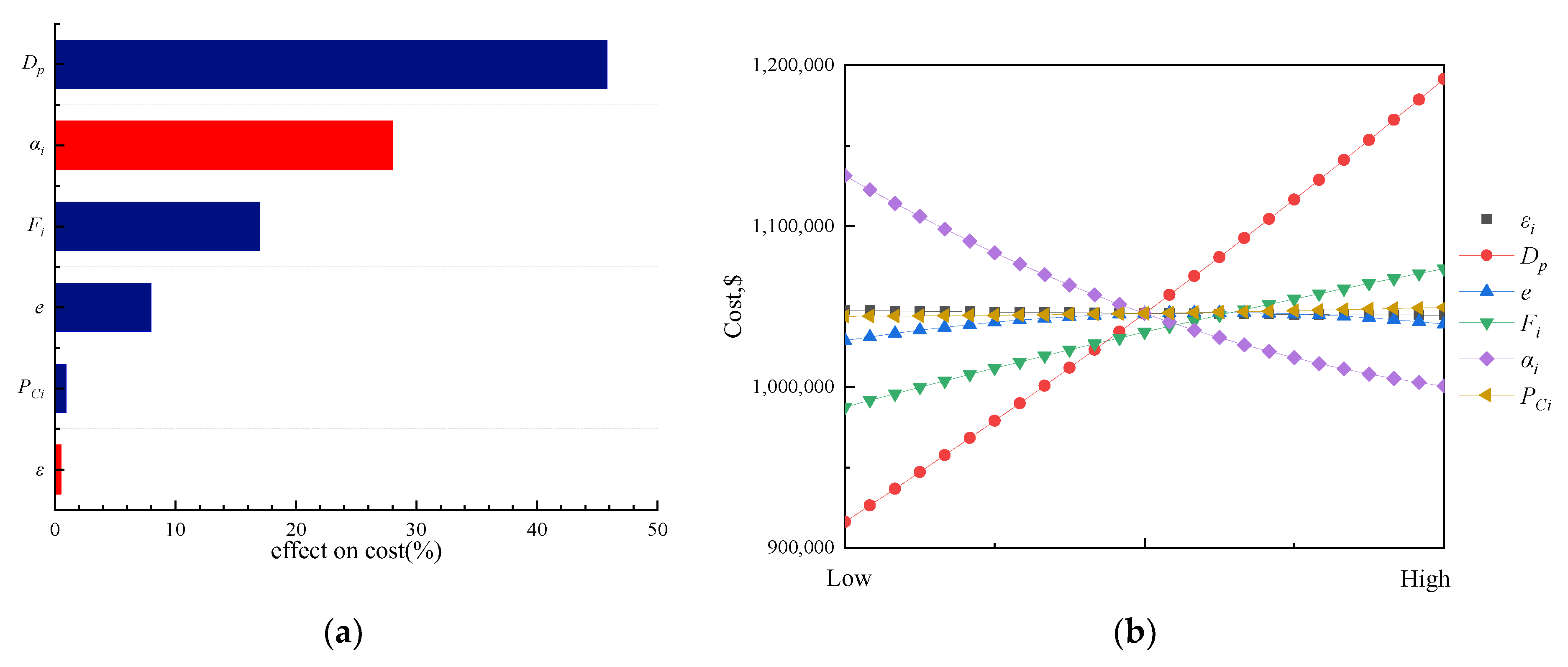
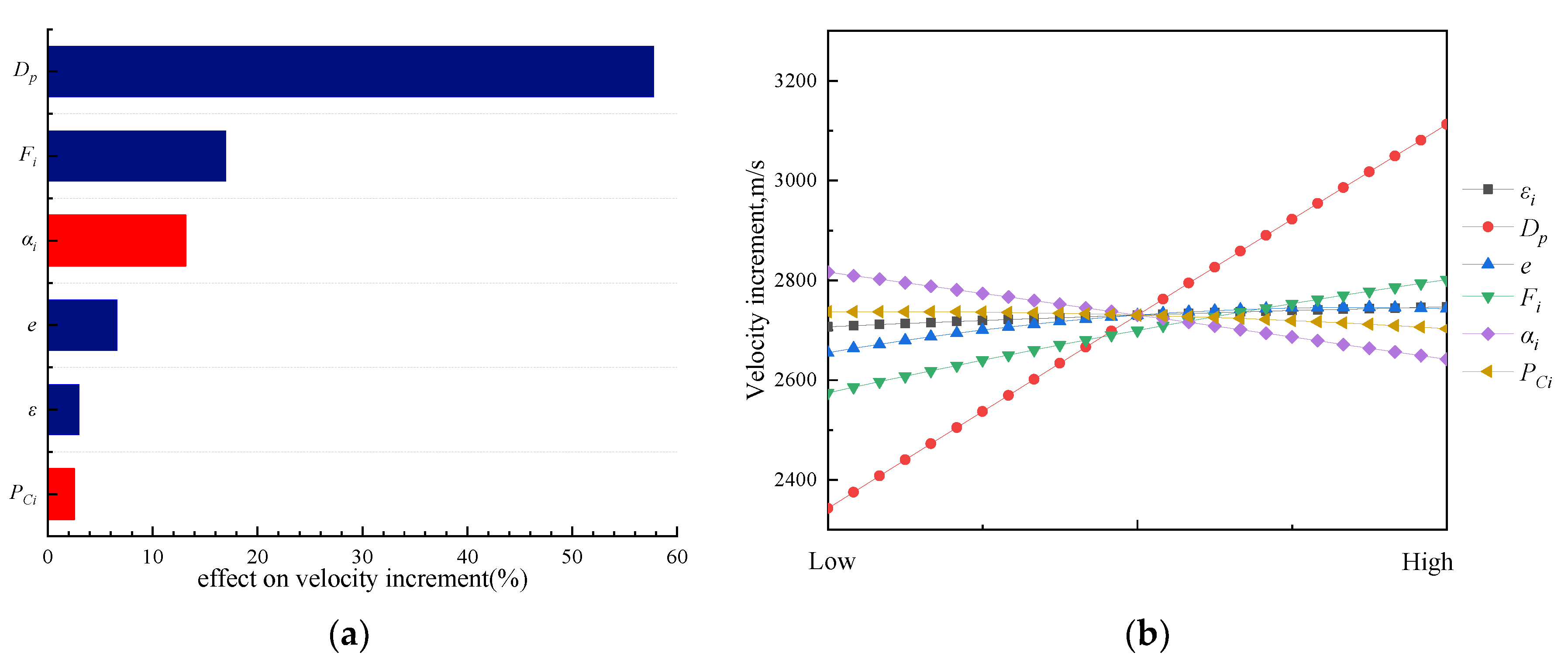
4.3. Parametric Analysis of HRM
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Cohen, M.M. From Apollo LM to Altair: Design, Environments, Infrastructure, Missions, and Operations. In Proceedings of the AIAA SPACE 2009 Conference & Exposition, Pasadena, CA, USA, 14–17 September 2009. [Google Scholar] [CrossRef]
- Meyer, C.; Maul, W. The Application of Neural Networks to the SSME Startup Transient. In Proceedings of the 27th Joint Propulsion Conference, Sacramento, CA, USA, 24–26 June 1991. [Google Scholar] [CrossRef]
- Dinesh, M.; Rajput, S.S.; Kumar, R. Protrusion Effect on the Performance of Hybrid Rocket with Liquefying and Non-Liquefying Fuels. Acta Astronaut. 2021, 178, 536–547. [Google Scholar] [CrossRef]
- Kuo, K.K.; Chiaverini, M.J. (Eds.) Fundamentals of Hybrid Rocket Combustion and Propulsion; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2007. [Google Scholar]
- Schmierer, C.; Kobald, M.; Tomilin, K.; Fischer, U.; Schlechtriem, S. Low Cost Small-Satellite Access to Space Using Hybrid Rocket Propulsion. Acta Astronaut. 2019, 159, 578–583. [Google Scholar] [CrossRef]
- Jens, E.T.; Cantwell, B.J.; Hubbard, G.S. Hybrid Rocket Propulsion Systems for Outer Planet Exploration Missions. Acta Astronaut. 2016, 128, 119–130. [Google Scholar] [CrossRef]
- Virgin Galactic Successfully Completes First Fully Crewed Spaceflight. Vayu Aerosp. Def. Rev. 2021, 4, 82–84. Available online: https://www.proquest.com/scholarly-journals/virgin-galactic-successfully-completes-first/docview/2580731010/se-2 (accessed on 9 August 2022).
- Wei, S.S.; Lee, M.C.; Huang, J.W.; Lu, Y.; Kang, C.H.; Kao, S.T.; Lu, S.J.; Huang, C.H.; Zhan, J.J.; Chen, Z.R.; et al. Demonstration of Tethered Hovering Flight of HTTP-3AT Hybrid Rocket. Acta Astronaut. 2022, 191, 279–292. [Google Scholar] [CrossRef]
- Faenza, M.G.; Boiron, A.J.; Haemmerli, B.; Verberne, O. The Nammo Nucleus Launch: Norwegian Hybrid Sounding Rocket over 100km. In Proceedings of the AIAA Propulsion and Energy 2019 Forum, Indianapolis, IN, USA, 19–22 August 2019. [Google Scholar] [CrossRef]
- DAUNTLESS 2021 Vaya Space SmallSat Launch Company and Hybrid Engine Sales. Available online: https://www.vayaspace.com/post/vaya-space-awarded-multiple-patents-for-vortex-hybrid-engine-technology (accessed on 9 August 2022).
- GILMOUR. Available online: https://www.gspace.com/post/gilmour-space-completes-final-qualification-test-of-sirius-rocket-engine (accessed on 8 November 2022).
- Martin, F.; Chapelle, A.; Orlandi, O.; Yvart, P. Hybrid Propulsion Systems for Future Space Applications. In Proceedings of the 46th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Nashville, TN, USA, 25–28 July 2010. [Google Scholar]
- Casalino, L.; Masseni, F.; Pastrone, D. Optimal Design of Electrically Fed Hybrid Mars Ascent Vehicle. Aerospace 2021, 8, 181. [Google Scholar] [CrossRef]
- Yelken, U.; Yalcintas, A.; Kara, O.; Baysal, M.; Karabeyoglu, M.A. Experimental Study of Lunar-Based Hybrid Rocket Engine. In Proceedings of the AIAA Propulsion and Energy 2021 Forum, Virtual Online, 9–11 August 2021. [Google Scholar]
- Lee, D.; Han, S.; Moon, H. Development of 200 N-Class Throttleable Hybrid Rocket Motor for Lunar Module Application. FirePhysChem 2021, 1, 251–259. [Google Scholar] [CrossRef]
- Ullah, R.; Zhou, D.Q.; Zhou, P.; Hussain, M.; Amjad Sohail, M. An Approach for Space Launch Vehicle Conceptual Design and Multi-Attribute Evaluation. Aerosp. Sci. Technol. 2013, 25, 65–74. [Google Scholar] [CrossRef]
- Ullah, R.; Zhou, D.Q.; Zhou, P.; Baseer, M. A Novel Weight Allocation and Decision Making Method for Space Launch Vehicle Design Concept Selection. Int. J. Ind. Syst. Eng. 2015, 19, 155–168. [Google Scholar] [CrossRef]
- Wang, P.; Zhu, H.; Xie, L.; Xiao, M.; Tian, H.; Cai, G. Multi-attribute evaluation approach for small launch vehicle with multi-objective multi-discipline design optimization. In Proceedings of the International Astronautical Congress, IAC, Virtual Online, 12–14 October 2020; Agenzia Spaziale Italiana (ASI): Rome, Italy, 2020. [Google Scholar]
- Moon, Y.; Jang, T.S.; Park, C.; Kwon, S. Requirements Analysis of Propulsion Systems for Lunar-Exploration Mission. J. Spacecr. Rockets 2013, 50, 620–631. [Google Scholar] [CrossRef]
- Zhu, H.; Tian, H.; Cai, G.B.; Bao, W.M. Uncertainty Analysis and Probabilistic Design Optimization of Hybrid Rocket Motors for Manned Lunar Landing. Sci. China Technol. Sci. 2015, 58, 1234–1241. [Google Scholar] [CrossRef]
- Kelly, T.J. Manned Lunar Lander Design—The Project Apollo Lunar Module (LM). In Proceedings of the Space Programs and Technologies Conference, Huntsville, AL, USA, 24–27 March 1992. [Google Scholar] [CrossRef]
- Apollo 11 Lunar Module/EASEP. Available online: https://nssdc.gsfc.nasa.gov/nmc/spacecraft/display.action?id=1969-059C (accessed on 28 October 2022).
- Smith, P.D. Apollo Experience Report: Spacecraft Structure Subsystem; National Aeronautics and Space Administration: Washington, DC, USA, 1974. [Google Scholar]
- Lunar Module. Available online: https://history.nasa.gov/alsj/CSM08_LM_&_SLA_Overview_pp61-68.pdf (accessed on 5 June 2018).
- Wilhite, A.W.; Wagner, J.; Tolson, R.; Moen, M.M. Lunar Module Descent Mission Design. In Proceedings of the AIAA/AAS Astrodynamics Specialist Conference and Exhibit, Honolulu, Hawaii, 18–21 August 2008. [Google Scholar] [CrossRef]
- Bennett, F.V. Apollo Experience Report: Mission Planning for Lunar Module Descent and Ascent; National Aeronautics and Space Administration: Washington, DC, USA, 1972. [Google Scholar]
- Hammock, W.R.; Currie, E.C.; Fisher, A.E. Apollo Experience Report: Descent Propulsion System; National Aeronautics and Space Administration: Washington, DC, USA, 1973. [Google Scholar]
- Wang, P.; Xu, W.; Zhu, H.; Tian, H.; Cai, G. An Application of Analytical Target Cascading for a Hierarchical Multidisciplinary System: The Preliminary Design of a Launch Vehicle Powered by Hybrid Rocket Motors. Aerospace 2022, 9, 778. [Google Scholar] [CrossRef]
- Ahn, B.; Kang, H.; Lee, E.; Yun, Y.; Kwon, S. Design of Multiport Grain with Hydrogen Peroxide Hybrid Rocket. J. Propuls. Power 2018, 34, 1189–1197. [Google Scholar] [CrossRef]
- Okninski, A. On Use of Hybrid Rocket Propulsion for Suborbital Vehicles. Acta Astronaut. 2018, 145, 1–10. [Google Scholar] [CrossRef]
- Casalino, L.; Masseni, F.; Pastrone, D. Robust Design Approaches for Hybrid Rocket Upper Stage. J. Aerosp. Eng. 2019, 32, 1–13. [Google Scholar] [CrossRef]
- Rao, D.; Cai, G.; Zhu, H.; Tian, H. Design and Optimization of Variable Thrust Hybrid Rocket Motors for Sounding Rockets. Sci. China Technol. Sci. 2012, 55, 125–135. [Google Scholar] [CrossRef]
- Zhu, H.; Wang, P.; Xu, W.; Zhang, Y.; Tian, H.; Cai, G. Design Optimization and Parameter Analysis of a Hybrid Rocket Motor-Powered Small LEO Launch Vehicle. Int. J. Aerosp. Eng. 2021, 2021, 5574436. [Google Scholar] [CrossRef]
- Lobanoff, V.S.; Ross, R.R. Centrifugal Pumps: Design and Application; Elsevier: Amsterdam, The Netherlands, 2013; ISBN 0080500854. [Google Scholar]
- Jamilnia, R.; Naghash, A. Simultaneous Optimization of Staging and Trajectory of Launch Vehicles Using Two Different Approaches. Aerosp. Sci. Technol. 2012, 23, 85–92. [Google Scholar] [CrossRef]
- Frank, C.P.; Atanian, M.F.; Pinon-Fischer, O.J.; Mavris, D.N. A conceptual design framework for performance, life-cycle cost, and safety evaluation of suborbital vehicles. In Proceedings of the 54th AIAA Aerospace Sciences Meeting, San Diego, CA, USA, 4–8 January 2016. [Google Scholar]
- Friedland, E.I.; Nieroski, J.S. Liquid Rocket Engine Cost Estimating Relationships. In Proceedings of the 2nd Annual Meeting, San Francisco, CA, USA, 26–29 July 1965. [Google Scholar]
- Frank, C.P.; Pinon-Fischer, O.J.; Mavris, D.N. A Flexible Multi-Disciplinary Environment for Performance, Life-Cycle Cost, and Safety Evaluation of Suborbital Vehicles. Aerosp. Sci. Technol. 2018, 77, 555–562. [Google Scholar] [CrossRef]
- Deb, K.; Pratap, A.; Agarwal, S.; Meyarivan, T. A Fast and Elitist Multiobjective Genetic Algorithm: NSGA-II. IEEE Trans. Evol. Comput. 2002, 6, 182–197. [Google Scholar] [CrossRef]
- Verma, S.; Pant, M.; Snasel, V. A Comprehensive Review on NSGA-II for Multi-Objective Combinatorial Optimization Problems. IEEE Access 2021, 9, 57757–57791. [Google Scholar] [CrossRef]



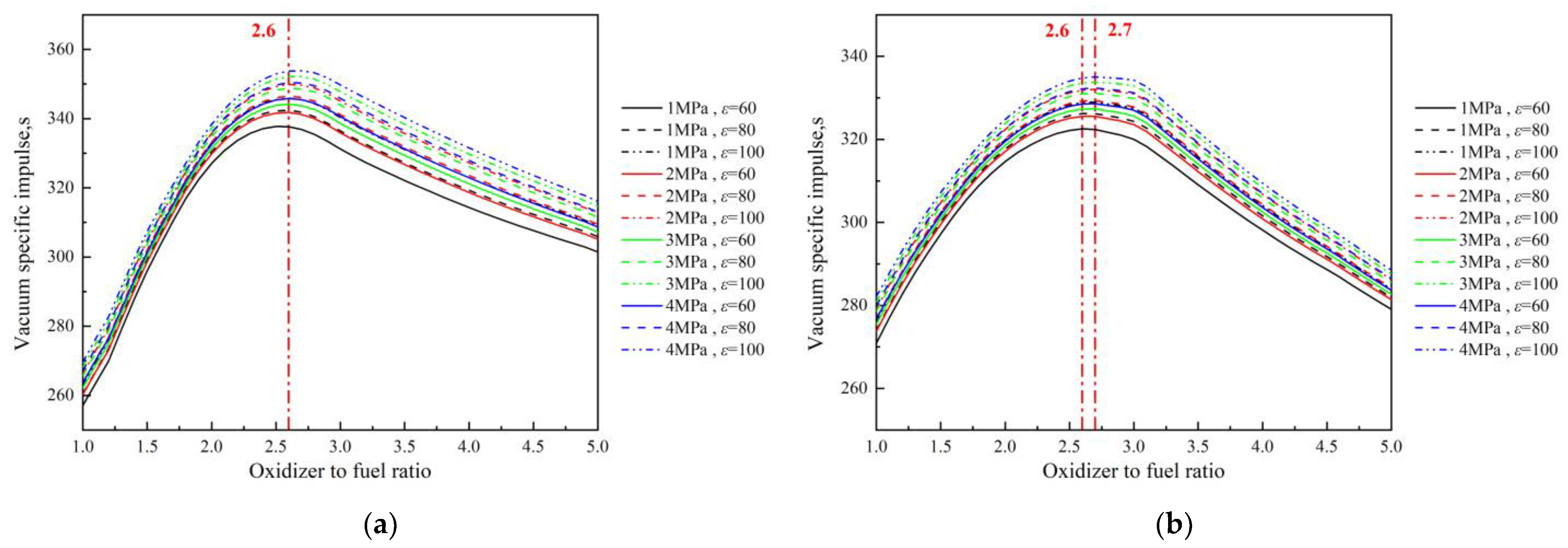

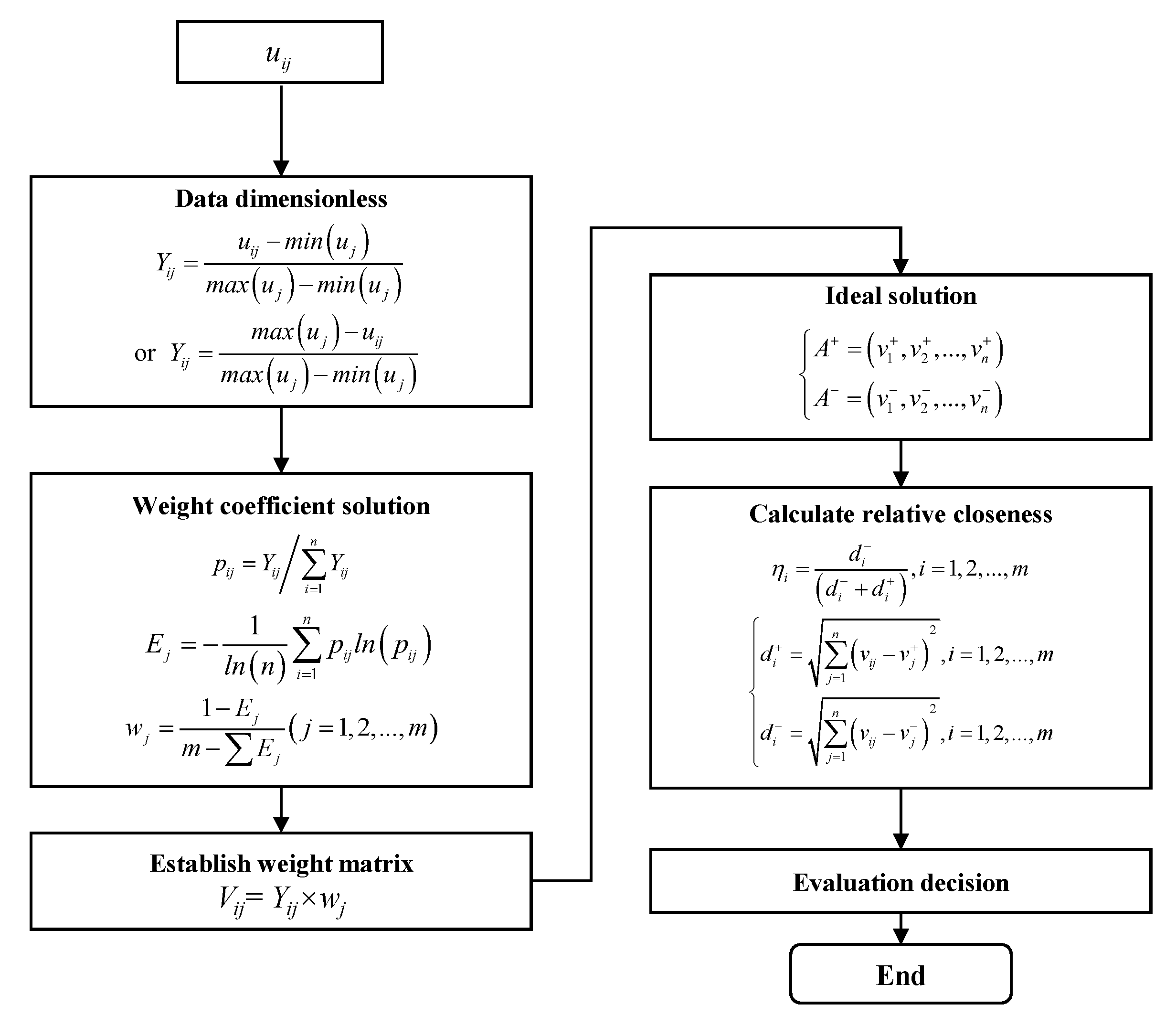


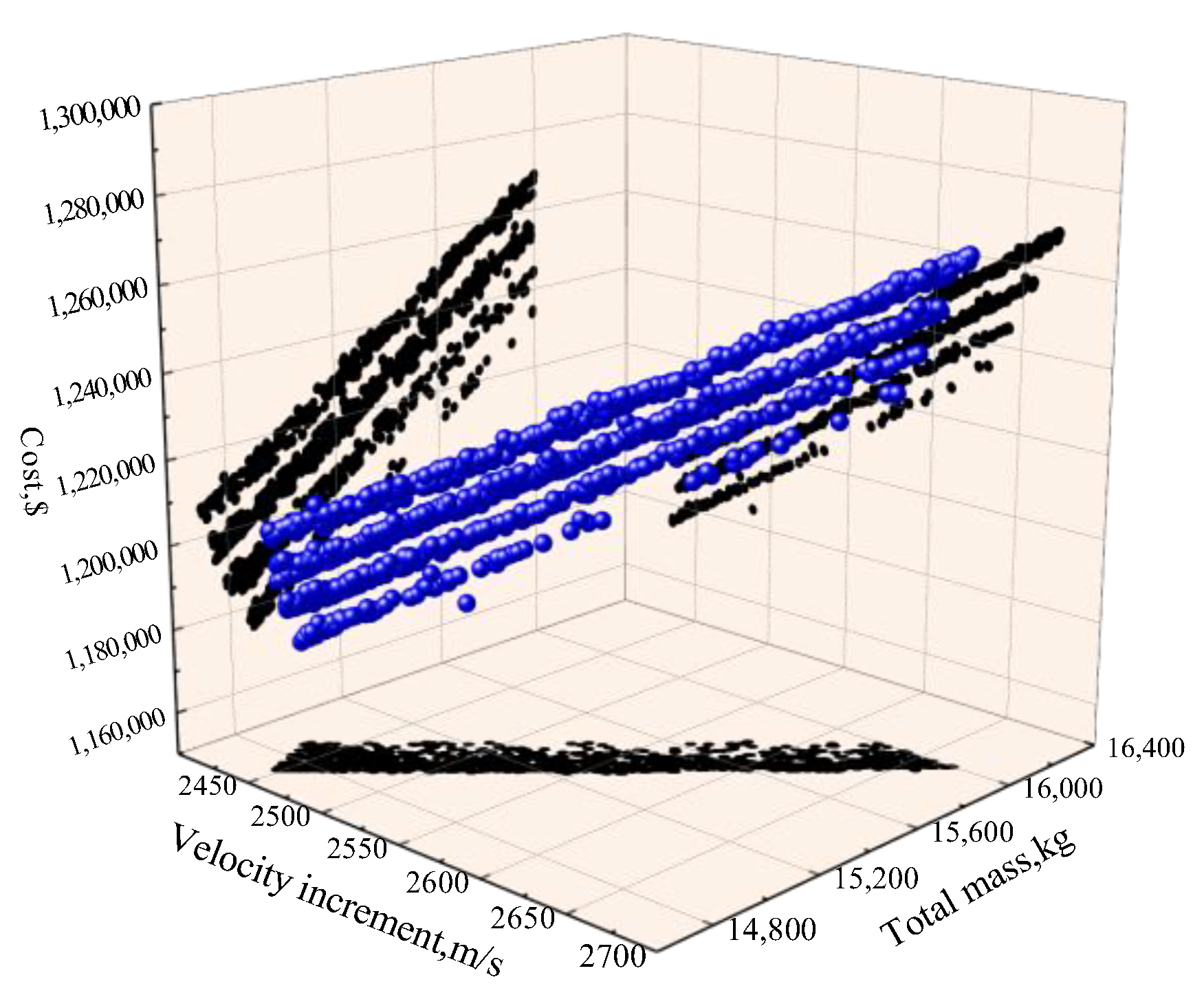
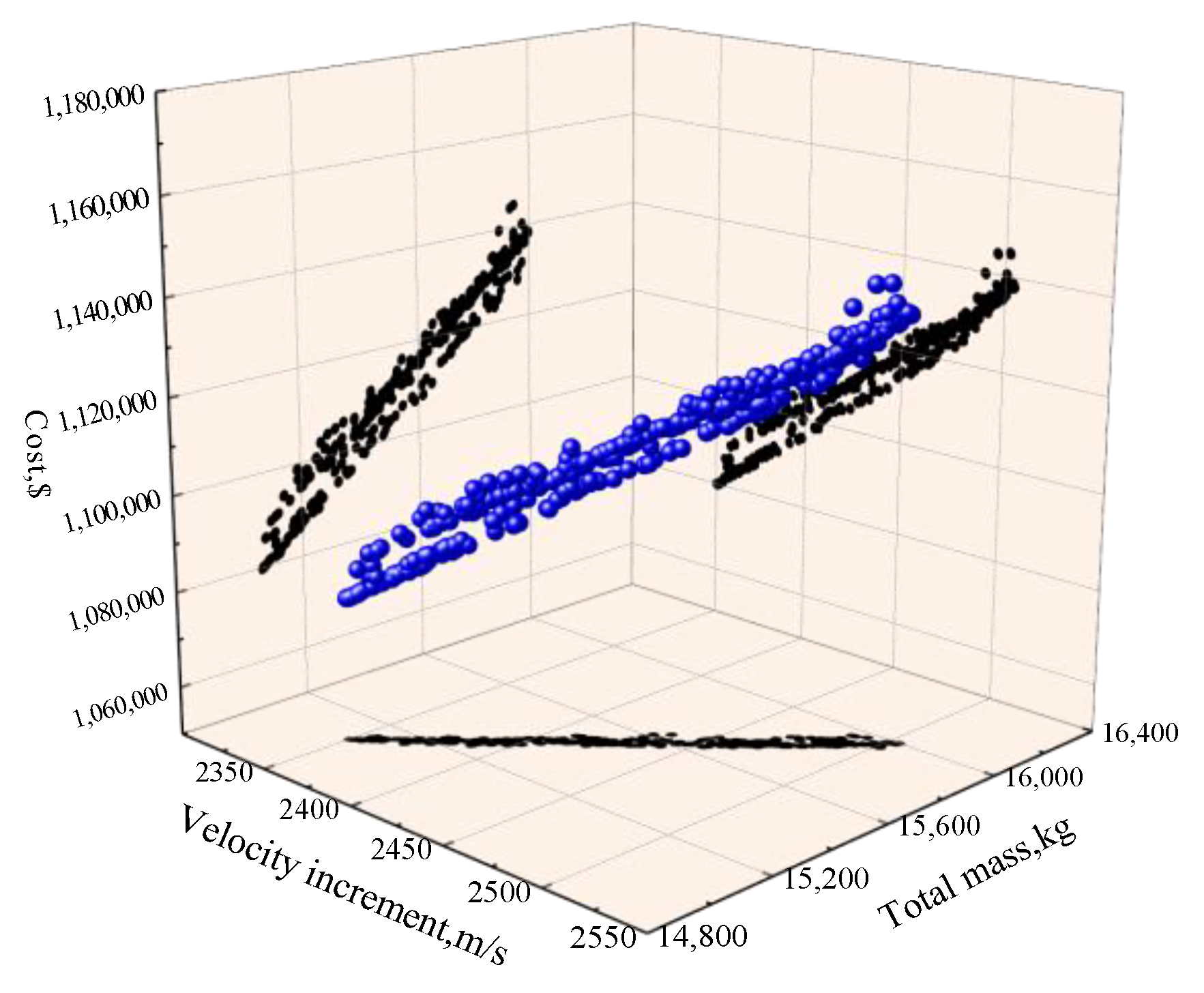




| Main Structure | LRE-ND | LRE-LK | HRM-TGGF | HRM-TGPF | HRM-WGGF | HRM-WGPF |
|---|---|---|---|---|---|---|
| Liquid oxidizer | Liquid oxygen | N2O4 | 98%H2O2 | 98%H2O2 | 98%H2O2 | 98%H2O2 |
| Liquid fuel | Kerosene | UDMH | —— | —— | —— | —— |
| Solid fuel | —— | —— | HTPB | HTPB | HTPB | HTPB |
| Gas bottle | CF wound aluminum liner | |||||
| Tank | CF wound aluminum liner and fiberglass insulation | CF wound aluminum liner | CF wound aluminum liner and rubber oxidizer sac | |||
| Chamber and nozzle | CF wound shell and high silica insulation | |||||
| Electric pump | —— | —— | Alloy steel | —— | Alloy steel | |
| Pipe/valve | Mainly aluminum alloy | |||||
| Skin and skeleton | CF | |||||
| LRE-ND | LRE-LK | HRM-TGGF | |
| Preparation duration | (0.5,0.6,0.7) 0.6 | (0.5,0.5,0.7) 0.55 | (0.6,0.7,0.7) 0.675 |
| General assembly | (0.5,0.6,0.7) 0.6 | (0.4,0.6,0.7) 0.575 | (0.8,0.8,0.8) 0.8 |
| General test | (0.3,0.5,0.5) 0.45 | (0.3,0.5,0.7) 0.5 | (0.5,0.6,0.8) 0.625 |
| Propellant storage | (0.6,0.7,0.7) 0.675 | (0.5,0.5,0.6) 0.525 | (0.6,0.7,0.8) 0.7 |
| Propellant transfer | (0.4,0.5,0.6) 0.5 | (0.7,0.7,0.9) 0.75 | (0.7,0.8,0.9) 0.8 |
| Propellant filling | (0.4,0.6,0.6) 0.55 | (0.5,0.6,0.7) 0.6 | (0.7,0.7,0.8) 0.725 |
| Propellant pressurization | (0.7,0.7,0.8) 0.725 | (0.7,0.7,0.8) 0.725 | (0.8,0.9,0.9) 0.875 |
| Operability average score | 0.586 | 0.604 | 0.743 |
| HRM-TGPF | HRM-WGGF | HRM-WGPF | |
| Preparation duration | (0.6,0.7,0.7) 0.675 | (0.6,0.7,0.7) 0.675 | (0.6,0.7,0.7) 0.675 |
| General assembly | (0.8,0.8,0.8) 0.8 | (0.8,0.8,0.8) 0.8 | (0.8,0.8,0.8) 0.8 |
| General test | (0.3,0.3,0.5) 0.35 | (0.5,0.6,0.8) 0.625 | (0.3,0.3,0.5) 0.35 |
| Propellant storage | (0.6,0.7,0.8) 0.7 | (0.6,0.7,0.8) 0.7 | (0.6,0.7,0.8) 0.7 |
| Propellant transfer | (0.7,0.8,0.9) 0.8 | (0.7,0.8,0.9) 0.8 | (0.7,0.8,0.9) 0.8 |
| Propellant fill | (0.7,0.7,0.8) 0.725 | (0.7,0.7,0.8) 0.725 | (0.7,0.7,0.8) 0.725 |
| Propellant pressurization | (0.8,0.9,0.9) 0.875 | (0.8,0.9,0.9) 0.875 | (0.8,0.9,0.9) 0.875 |
| Operability average score | 0.704 | 0.743 | 0.704 |
| LRE-ND | LRE-LK | HRM-TGGF | |
| Solid grain | (——) 1 | (——) 1 | (0.8,0.8,0.8) 0.8 |
| Tank | (0.4,0.5,0.6) 0.5 | (0.5,0.5,0.7) 0.55 | (0.6,0.6,0.6) 0.6 |
| Feed system | (0.6,0.6,0.8) 0.65 | (0.6,0.6,0.7) 0.625 | (0.6,0.6,0.8) 0.65 |
| Chamber | (0.6,0.6,0.7) 0.625 | (0.6,0.6,0.7) 0.625 | (0.5,0.7,0.7) 0.65 |
| Nozzle | (0.5,0.5,0.7) 0.55 | (0.5,0.5,0.7) 0.55 | (0.4,0.4,0.5) 0.425 |
| Manufacturability average score | 0.665 | 0.67 | 0.625 |
| HRM-TGPF | HRM-WGGF | HRM-WGPF | |
| Solid grain | (0.8,0.8,0.8) 0.8 | (0.6,0.7,0.7) 0.675 | (0.6,0.7,0.7) 0.675 |
| Tank | (0.4,0.6,0.6) 0.55 | (0.4,0.6,0.6) 0.55 | (0.4,0.6,0.6) 0.55 |
| Feed system | (0.4,0.6,0.6) 0.55 | (0.6,0.6,0.8) 0.65 | (0.4,0.6,0.6) 0.55 |
| Chamber | (0.6,0.7,0.7) 0.675 | (0.6,0.7,0.7) 0.675 | (0.6,0.7,0.7) 0.675 |
| Nozzle | (0.4,0.4,0.5) 0.425 | (0.4,0.4,0.5) 0.425 | (0.4,0.4,0.5) 0.425 |
| Manufacturability average score | 0.6 | 0.595 | 0.575 |
| LRE-ND | LRE-LK | HRM-TGGF | |
| Throttle/variable thrust | (0.6,0.7,0.8) 0.7 | (0.6,0.7,0.8) 0.7 | (0.6,0.7,0.7) 0.675 |
| Restart and multi-ignition | (0.6,0.8,0.8) 0.75 | (0.6,0.7,0.7) 0.675 | (0.6,0.8,0.8) 0.75 |
| Flexibility average score | 0.725 | 0.6875 | 0.7125 |
| HRM-TGPF | HRM-WGGF | HRM-WGPF | |
| Throttle/variable thrust | (0.7,0.7,0.8) 0.725 | (0.6,0.7,0.7) 0.675 | (0.7,0.7,0.8) 0.725 |
| Restart and multi-ignition | (0.6,0.6,0.6) 0.6 | (0.6,0.8,0.8) 0.75 | (0.6,0.6,0.6) 0.6 |
| Flexibility average score | 0.6625 | 0.7125 | 0.6625 |
| LRE-ND | LRE-LK | HRM-TGGF | |
| Environmental protection | (0.5,0.5,0.7) 0.55 | (0.6,0.7,0.9) 0.725 | (0.7,0.8,0.9) 0.8 |
| Safety of production | (0.5,0.6,0.7) 0.6 | (0.5,0.6,0.7) 0.6 | (0.6,0.8,0.9) 0.775 |
| Safety of storage | (0.4,0.5,0.5) 0.475 | (0.4,0.5,0.6) 0.5 | (0.6,0.6,0.8) 0.65 |
| Safety of usage | (0.5,0.6,0.8) 0.625 | (0.5,0.5,0.6) 0.525 | (0.6,0.8,0.9) 0.775 |
| Safety/environmental-friendliness average score | 0.5625 | 0.5875 | 0.75 |
| HRM-TGPF | HRM-WGGF | HRM-WGPF | |
| Environmental protection | (0.7,0.8,0.9) 0.8 | (0.7,0.8,0.9) 0.8 | (0.7,0.8,0.9) 0.8 |
| Safety of production | (0.6,0.8,0.9) 0.775 | (0.6,0.8,0.9) 0.775 | (0.6,0.8,0.9) 0.775 |
| Safety of storage | (0.6,0.6,0.8) 0.65 | (0.6,0.6,0.8) 0.65 | (0.6,0.6,0.8) 0.65 |
| Safety of usage | (0.6,0.6,0.6) 0.6 | (0.6,0.8,0.9) 0.775 | (0.6,0.6,0.6) 0.6 |
| Safety/environmental-friendliness average score | 0.706 | 0.75 | 0.706 |
| LRE-ND | LRE-LK | HRM-TGGF | HRM-TGPF | HRM-WGGF | HRM-WGPF | |
|---|---|---|---|---|---|---|
| Pcave/Mpa | 1.4 | 1.8 | 2 | 2.3 | 2.2 | 2.4 |
| Fave/kN | 29 | 26.2 | 23.37 | 23.5 | 24.5 | 24.7 |
| ε | 60 | 90 | 66 | 69 | 81 | 80 |
| 2.7 | 2.6 | 5.18 | 5.32 | 4.79 | 4.55 | |
| e/Dp | —— | —— | 0.4 | 0.39 | 0.1 | 0.095 |
| Dp/m | —— | —— | 1.074 | 1.077 | 1.75 | 1.748 |
| Tmotor/s | 854 | 945 | 1062 | 1055 | 1019 | 1005 |
| I/×103 kNs | 24.8 | 24.8 | 24.8 | 24.8 | 24.9 | 24.8 |
| Total mass M/kg | 15,092 | 14,936 | 15,010 | 14,810 | 15,130 | 14,847 |
| Descent motor mass/kg | 9292 | 9136 | 9210 | 9010 | 9330 | 9047 |
| Descent propellant mass/kg | 7900 | 7650 | 8250 | 8230 | 8130 | 8010 |
| Motor effective propellant mass fraction ηi | 0.84 | 0.83 | 0.89 | 0.91 | 0.87 | 0.88 |
| Isave/s | 320 | 332 | 309 | 308.8 | 315 | 317 |
| ΔV/m/s | 2319 | 2321 | 2391 | 2440 | 2363 | 2402 |
| C/ thousand dollars | 1127 | 1066 | 1104 | 1179 | 1085 | 1165 |
| Relative closeness | 0.60935 | 0.73999 | 0.72062 | 0.67692 | 0.70474 | 0.68938 |
| Rank | 1022 | 1 | 110 | 278 | 151 | 225 |
| Index | LRE-ND | LRE-LK | HRM-TGGF | HRM-TGPF | HRM-WGGF | HRM-WGPF |
|---|---|---|---|---|---|---|
| Total Mass/kg | 15,092 | 14,936 | 15,010 | 14,810 | 15,130 | 14,847 |
| C/ thousand dollars | 1127 | 1066 | 1104 | 1179 | 1085 | 1165 |
| ηi | 0.844 | 0.83 | 0.89 | 0.91 | 0.87 | 0.88 |
| ΔV/m/s | 2319 | 2321 | 2391 | 2440 | 2363 | 2402 |
| Operability | 0.586 | 0.604 | 0.743 | 0.704 | 0.743 | 0.704 |
| Manufacturability | 0.665 | 0.670 | 0.625 | 0.6 | 0.595 | 0.575 |
| Flexibility | 0.725 | 0.688 | 0.713 | 0.663 | 0.713 | 0.663 |
| Safety/environmental protection | 0.5625 | 0.5875 | 0.75 | 0.706 | 0.75 | 0.706 |
| Relative closeness | 0.434398 | 0.437641 | 0.667505 | 0.536286 | 0.547130 | 0.462153 |
| Rank | 6 | 5 | 1 | 3 | 2 | 4 |
| Value | |
|---|---|
| Descent Propellant mass/kg | 8250 |
| Effective propellant mass/kg | 8197 |
| Oxidizer mass/kg | 6925 |
| Grain mass/kg | 1325 |
| Thrust chamber mass/kg | 151 |
| Single tank mass/kg | 52 |
| Gas bottle mass/kg | 42 |
| Pipeline valves mass/kg | 234 |
| Skin mass/kg | 25 |
| Residual mass/kg | 300 |
| Descent motor mass/kg | 9210 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Li, X.; Wang, P.; Zhang, X.; Zhu, H.; Cai, G. Multi-Objective, Multi-Disciplinary Design Optimization and Multi-Attribute Evaluation of Hybrid Rocket Motors Used for Manned Lunar Lander. Aerospace 2023, 10, 272. https://doi.org/10.3390/aerospace10030272
Liu Y, Li X, Wang P, Zhang X, Zhu H, Cai G. Multi-Objective, Multi-Disciplinary Design Optimization and Multi-Attribute Evaluation of Hybrid Rocket Motors Used for Manned Lunar Lander. Aerospace. 2023; 10(3):272. https://doi.org/10.3390/aerospace10030272
Chicago/Turabian StyleLiu, Yang, Xintong Li, Pengcheng Wang, Xiaotian Zhang, Hao Zhu, and Guobiao Cai. 2023. "Multi-Objective, Multi-Disciplinary Design Optimization and Multi-Attribute Evaluation of Hybrid Rocket Motors Used for Manned Lunar Lander" Aerospace 10, no. 3: 272. https://doi.org/10.3390/aerospace10030272
APA StyleLiu, Y., Li, X., Wang, P., Zhang, X., Zhu, H., & Cai, G. (2023). Multi-Objective, Multi-Disciplinary Design Optimization and Multi-Attribute Evaluation of Hybrid Rocket Motors Used for Manned Lunar Lander. Aerospace, 10(3), 272. https://doi.org/10.3390/aerospace10030272






