Figure 1.
Orbit insertion as a function of the apoapsis altitude at Mars and Venus from trajectories with = 2.5 and 3.5 km/s, respectively, periapsis altitude = 200 km.
Figure 1.
Orbit insertion as a function of the apoapsis altitude at Mars and Venus from trajectories with = 2.5 and 3.5 km/s, respectively, periapsis altitude = 200 km.
Figure 2.
Dry-to-wet mass ratio as a function of from the rocket equation. The dotted horizontal line demarcates the region below which the propellant mass fraction exceeds 60%.
Figure 2.
Dry-to-wet mass ratio as a function of from the rocket equation. The dotted horizontal line demarcates the region below which the propellant mass fraction exceeds 60%.
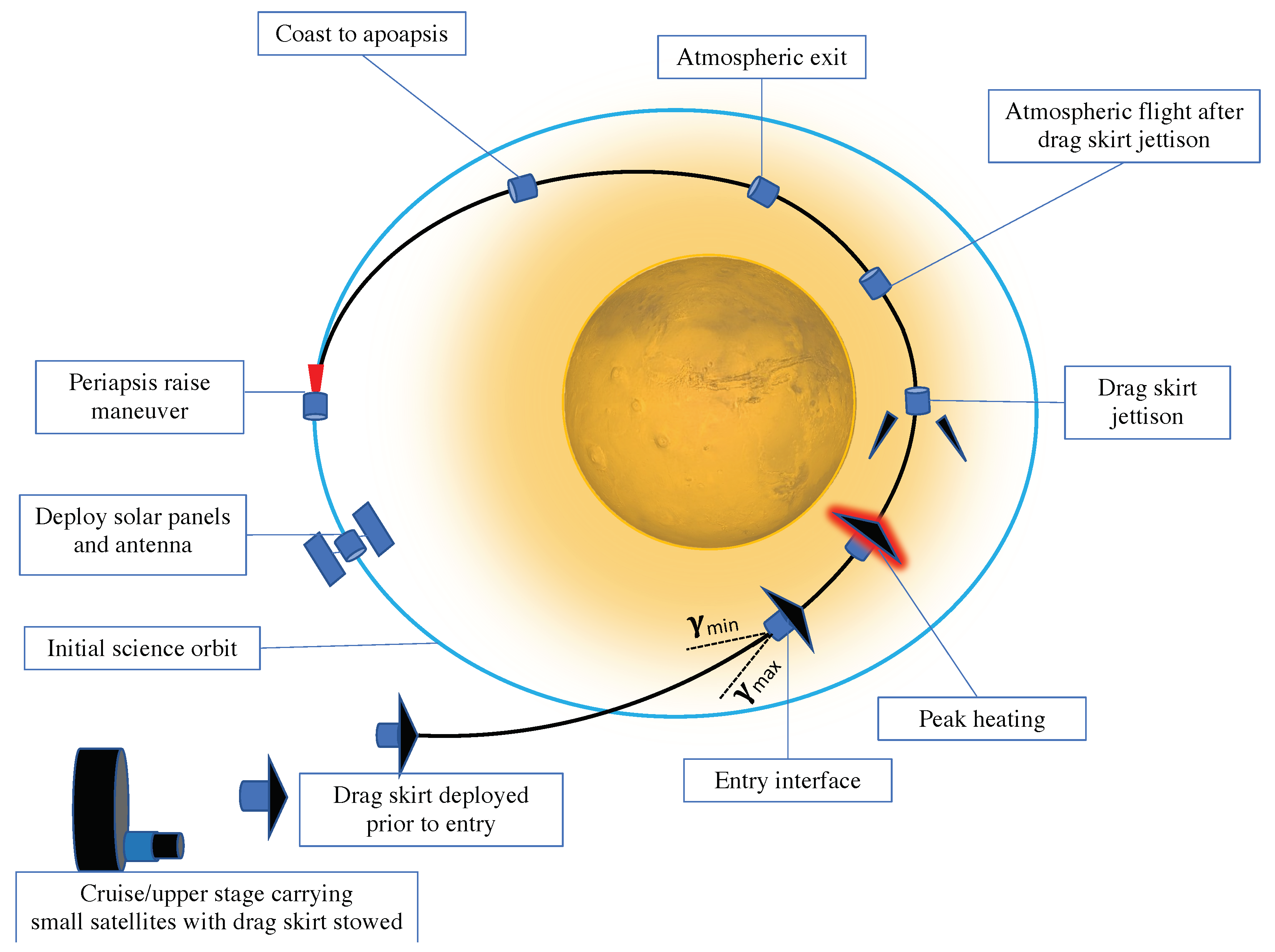
Figure 3.
Schematic showing the key events in drag-modulation aerocapture for small satellite orbit insertion. Distances are not to scale, and atmospheric extent is greatly exaggerated for clarity.
Figure 3.
Schematic showing the key events in drag-modulation aerocapture for small satellite orbit insertion. Distances are not to scale, and atmospheric extent is greatly exaggerated for clarity.
Figure 4.
Stowed and deployed configurations of the ADEPT SR-1 flight test article. Reproduced from the work by Dutta et al./NASA [
34]. The scaled-up version of the SR-1 article used in this study has a stowed diameter of 0.5 m and a deployed diameter of 1.5 m.
Figure 4.
Stowed and deployed configurations of the ADEPT SR-1 flight test article. Reproduced from the work by Dutta et al./NASA [
34]. The scaled-up version of the SR-1 article used in this study has a stowed diameter of 0.5 m and a deployed diameter of 1.5 m.
Figure 5.
Mars drag-modulation aerocapture feasibility chart with = 20 kg/m2, = 0.235 m, target apoapsis = 2000 km. The star indicates the selected baseline = 7.5 and = 2.65 km/s.
Figure 5.
Mars drag-modulation aerocapture feasibility chart with = 20 kg/m2, = 0.235 m, target apoapsis = 2000 km. The star indicates the selected baseline = 7.5 and = 2.65 km/s.
Figure 6.
Approach trajectory of the Mars small satellite aerocapture vehicle. The white ring and the solid line indicate the equatorial plane and the direction of the north pole, respectively.
Figure 6.
Approach trajectory of the Mars small satellite aerocapture vehicle. The white ring and the solid line indicate the equatorial plane and the direction of the north pole, respectively.
Figure 7.
Nominal Mars small satellite aerocapture trajectory for a vehicle with = 20 kg/m2, = 7.5, = 0.235 m entering at atm. relative EFPA = −9.25 deg. Target apoapsis = 2000 km.
Figure 7.
Nominal Mars small satellite aerocapture trajectory for a vehicle with = 20 kg/m2, = 7.5, = 0.235 m entering at atm. relative EFPA = −9.25 deg. Target apoapsis = 2000 km.
Figure 8.
Effect of density variation on Mars aerocapture corridor bounds. The black dash-dot line indicates the selected EFPA, and the black dotted lines indicate the assumed delivery error.
Figure 8.
Effect of density variation on Mars aerocapture corridor bounds. The black dash-dot line indicates the selected EFPA, and the black dotted lines indicate the assumed delivery error.
Figure 9.
Evolution of altitude rate and altitude during Mars aerocapture. The green diamond indicates the time when a preset altitude rate threshold (−200 m/s) is exceeded, at which point density estimation is cut off and apoapsis prediction is initiated. The red circle indicates the jettison event.
Figure 9.
Evolution of altitude rate and altitude during Mars aerocapture. The green diamond indicates the time when a preset altitude rate threshold (−200 m/s) is exceeded, at which point density estimation is cut off and apoapsis prediction is initiated. The red circle indicates the jettison event.
Figure 10.
Histogram of the apoapsis altitude (left) and the apoapsis altitude and periapsis altitude (right) for aerocapture at Mars. The dashed line indicates the target apoapsis altitude of 2000 km.
Figure 10.
Histogram of the apoapsis altitude (left) and the apoapsis altitude and periapsis altitude (right) for aerocapture at Mars. The dashed line indicates the target apoapsis altitude of 2000 km.
Figure 11.
Scatter plots of the peak deceleration, peak stagnation-point heat rate and the total heat load for aerocapture at Mars. The dotted lines indicate the 99.87 percentile values.
Figure 11.
Scatter plots of the peak deceleration, peak stagnation-point heat rate and the total heat load for aerocapture at Mars. The dotted lines indicate the 99.87 percentile values.
Figure 12.
Small satellite and host orbiter aim points on the Mars B-plane. After releasing the SmallSat, the host orbiter performs a deflection maneuver to target its aim point.
Figure 12.
Small satellite and host orbiter aim points on the Mars B-plane. After releasing the SmallSat, the host orbiter performs a deflection maneuver to target its aim point.
Figure 13.
Orbiter deflection maneuver
as a function of time from SmallSat release until entry, and the angular position
on the host aim-point
circle shown in
Figure 12.
Figure 13.
Orbiter deflection maneuver
as a function of time from SmallSat release until entry, and the angular position
on the host aim-point
circle shown in
Figure 12.
Figure 14.
Hyperbolic near-equatorial approach trajectory of the Mars SmallSat (green), and the polar approach trajectory of the host orbiter spacecraft (magenta) until periapsis.
Figure 14.
Hyperbolic near-equatorial approach trajectory of the Mars SmallSat (green), and the polar approach trajectory of the host orbiter spacecraft (magenta) until periapsis.
Figure 15.
Mars small satellite in 200 × 2000 km near-equatorial orbit post aerocapture (green), and the host orbiter in 250 × 70,000 km polar orbit after propulsive orbit insertion.
Figure 15.
Mars small satellite in 200 × 2000 km near-equatorial orbit post aerocapture (green), and the host orbiter in 250 × 70,000 km polar orbit after propulsive orbit insertion.
Figure 16.
Venus drag-modulation aerocapture feasibility chart with = 20 kg/m2, = 0.235 m, target apoapsis = 2000 km. The star indicates the selected baseline = 7.5 and = 3.51 km/s.
Figure 16.
Venus drag-modulation aerocapture feasibility chart with = 20 kg/m2, = 0.235 m, target apoapsis = 2000 km. The star indicates the selected baseline = 7.5 and = 3.51 km/s.
Figure 17.
Approach trajectory of the Venus small satellite aerocapture vehicle. The white ring and the solid line indicate the equatorial plane and the direction of the north pole, respectively.
Figure 17.
Approach trajectory of the Venus small satellite aerocapture vehicle. The white ring and the solid line indicate the equatorial plane and the direction of the north pole, respectively.
Figure 18.
Nominal Venus small satellite aerocapture trajectory for a vehicle with = 20 kg/m2, = 7.5, = 0.235 m entering at atm. relative to EFPA = −5.20 deg. Target apoapsis = 2000 km.
Figure 18.
Nominal Venus small satellite aerocapture trajectory for a vehicle with = 20 kg/m2, = 7.5, = 0.235 m entering at atm. relative to EFPA = −5.20 deg. Target apoapsis = 2000 km.
Figure 19.
Effect of density variation on Venus aerocapture corridor. The black dash-dot line indicates the selected EFPA, and the black dotted lines indicate the assumed error.
Figure 19.
Effect of density variation on Venus aerocapture corridor. The black dash-dot line indicates the selected EFPA, and the black dotted lines indicate the assumed error.
Figure 20.
Evolution of altitude rate and altitude during Venus aerocapture. The red circle indicates the time at which drag skirt jettison is commanded.
Figure 20.
Evolution of altitude rate and altitude during Venus aerocapture. The red circle indicates the time at which drag skirt jettison is commanded.
Figure 21.
Histogram of the apoapsis altitude (left) and the apoapsis altitude and periapsis altitude (right) for Venus aerocapture. The black dashed line indicates the target apoapsis altitude of 2000 km.
Figure 21.
Histogram of the apoapsis altitude (left) and the apoapsis altitude and periapsis altitude (right) for Venus aerocapture. The black dashed line indicates the target apoapsis altitude of 2000 km.
Figure 22.
Scatter plots of the peak deceleration, peak stagnation-point heat rate and the total heat load for Venus aerocapture. The black dotted line indicates the 99.87 percentile values.
Figure 22.
Scatter plots of the peak deceleration, peak stagnation-point heat rate and the total heat load for Venus aerocapture. The black dotted line indicates the 99.87 percentile values.
Figure 23.
Small satellite and host orbiter aim points on the Venus B-plane. The inset shows a magnified view of the two aim points, both of which have = .
Figure 23.
Small satellite and host orbiter aim points on the Venus B-plane. The inset shows a magnified view of the two aim points, both of which have = .
Figure 24.
Hyperbolic approach trajectory of the Venus SmallSat (green) until entry interface and the approach trajectory of the host orbiter spacecraft (magenta) until periapsis.
Figure 24.
Hyperbolic approach trajectory of the Venus SmallSat (green) until entry interface and the approach trajectory of the host orbiter spacecraft (magenta) until periapsis.
Figure 25.
Venus small satellite in 200 × 2000 km polar orbit post aerocapture (green), and the host orbiter in a 400 × 150,000 km polar orbit after propulsive orbit insertion (magenta).
Figure 25.
Venus small satellite in 200 × 2000 km polar orbit post aerocapture (green), and the host orbiter in a 400 × 150,000 km polar orbit after propulsive orbit insertion (magenta).
Figure 26.
SmallSat and host orbiter aim points on the Mars B-plane. After releasing each SmallSat, the host orbiter performs a small maneuver to target the aim point for the next one.
Figure 26.
SmallSat and host orbiter aim points on the Mars B-plane. After releasing each SmallSat, the host orbiter performs a small maneuver to target the aim point for the next one.
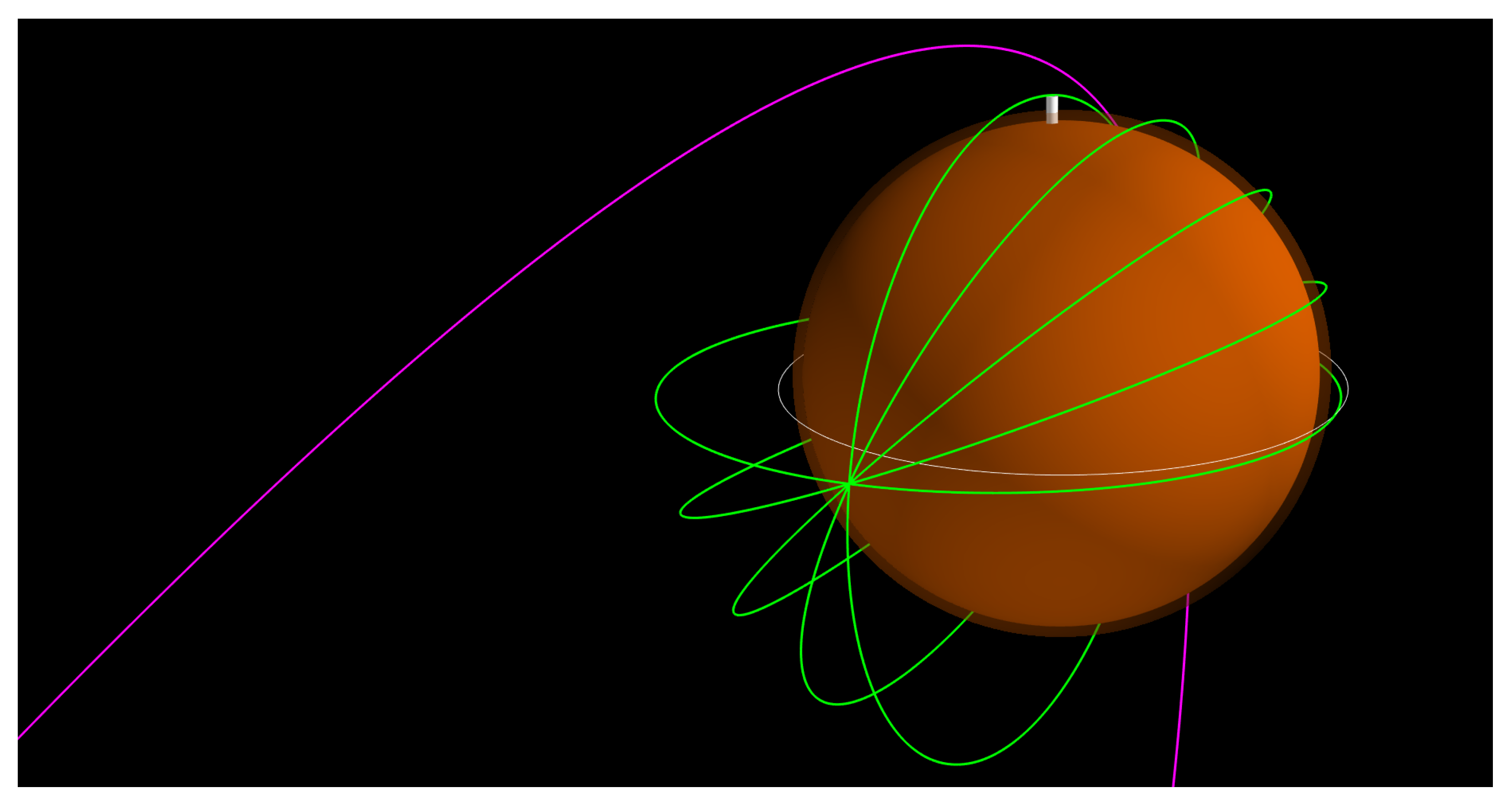
Figure 27.
Approach trajectory of the five Mars small satellites with various inclinations (green) until atmospheric entry and the host orbiter (magenta) until periapsis.
Figure 27.
Approach trajectory of the five Mars small satellites with various inclinations (green) until atmospheric entry and the host orbiter (magenta) until periapsis.
Figure 28.
Mars small satellite constellation in 200 × 2000 km orbits with various inclinations from equatorial to polar (green), and the host orbiter in 250 × 70,000 km polar orbit.
Figure 28.
Mars small satellite constellation in 200 × 2000 km orbits with various inclinations from equatorial to polar (green), and the host orbiter in 250 × 70,000 km polar orbit.
Figure 29.
SmallSat and host orbiter aim points on the Venus B-plane. After releasing each SmallSat, the host orbiter performs a maneuver to target the aim point for the next one.
Figure 29.
SmallSat and host orbiter aim points on the Venus B-plane. After releasing each SmallSat, the host orbiter performs a maneuver to target the aim point for the next one.
Figure 30.
Approach trajectory of the five Venus small satellites with various inclinations (green) until atmospheric entry and the host orbiter (magenta) until periapsis.
Figure 30.
Approach trajectory of the five Venus small satellites with various inclinations (green) until atmospheric entry and the host orbiter (magenta) until periapsis.
Figure 31.
Venus small satellite constellation in 200 × 2000 km near-polar inclination orbits (green), and the host orbiter in a 250 × 150,000 km polar orbit (magenta).
Figure 31.
Venus small satellite constellation in 200 × 2000 km near-polar inclination orbits (green), and the host orbiter in a 250 × 150,000 km polar orbit (magenta).
Figure 32.
Mission cost as a function of
for a chemical propulsion system.
refers to the science payload (instrument mass). Derived using data from Edwards et al. [
7].
Figure 32.
Mission cost as a function of
for a chemical propulsion system.
refers to the science payload (instrument mass). Derived using data from Edwards et al. [
7].
Table 1.
Mars aerocapture vehicle atmospheric entry state.
Table 1.
Mars aerocapture vehicle atmospheric entry state.
| Parameter | Value |
|---|
| Entry altitude, km | 120 |
| Entry longitude 1, deg. | −89.76 |
| Entry latitude, deg. | −0.71 |
| Atm. relative entry speed, km/s | 5.36 |
| Atm. relative heading angle 2, deg. | 9.38 |
| Atm. relative EFPA, deg. | −9.25 |
Table 2.
Mars SmallSat aerocapture entry corridor.
Table 2.
Mars SmallSat aerocapture entry corridor.
| Parameter | Value |
|---|
| Overshoot limit, deg. | −8.78 |
| Undershoot limit, deg. | −9.86 |
| TCW, deg. | 1.08 |
Table 3.
Effect of density variations on Mars aerocapture corridor.
Table 3.
Effect of density variations on Mars aerocapture corridor.
| Parameter | Min. Density | Average | Max. Density |
|---|
| Overshoot, deg. | −9.035 | −8.839 | −8.651 |
| Undershoot, deg. | −10.082 | −9.992 | −9.903 |
| TCW, deg. | 1.014 | 1.018 | 1.022 |
Table 4.
Mars aerocapture Monte Carlo uncertainties.
Table 4.
Mars aerocapture Monte Carlo uncertainties.
| Category | Variable | Nominal | Uncertainty | Distribution |
|---|
| Navigation | EFPA | −9.25 deg. | ±0.20 deg. (3) | Normal |
| Atmosphere | Mean density | - | ±3 | Normal |
| | Random | - | rpscale = 1 | Uniform |
| Aerodynamics | | 7.5 | ±10% (3) | Normal |
Table 5.
Statistics for Mars aerocapture orbit apoapsis altitude.
Table 5.
Statistics for Mars aerocapture orbit apoapsis altitude.
| Achieved Apoapsis Altitude Bin | Percentage |
|---|
| ±400 km of target | 88.1% |
| ±600 km of target | 96.8% |
| ±800 km of target | 99.5% |
| ±1000 km of target | 99.9% |
Table 6.
Statistics from Mars aerocapture Monte Carlo simulations.
Table 6.
Statistics from Mars aerocapture Monte Carlo simulations.
| Parameter | Min. | 5%-ile | Avg. | 95%-ile | Max. |
|---|
| Apoapsis alt., km | 1295 | 1685 | 2086 | 2481 | 3210 |
| Periapsis alt., km | 38.0 | 43.9 | 47.1 | 50.1 | 52.0 |
| Deceleration, g | 1.34 | 1.78 | 2.11 | 2.41 | 2.64 |
| Heat rate, W/cm2 | 32 | 35 | 37 | 39 | 47 |
| Heat load, kJ/cm2 | 5.3 | 7.0 | 8.2 | 9.4 | 11.2 |
| PRM , m/s | 30.4 | 31.5 | 32.8 | 34.4 | 37.0 |
| ACM , m/s | −132.9 | −49.7 | 2.9 | 59.9 | 122.8 |
Table 7.
Venus aerocapture vehicle atmospheric entry state.
Table 7.
Venus aerocapture vehicle atmospheric entry state.
| Parameter | Value |
|---|
| Entry altitude, km | 150 |
| Entry longitude, deg. | −6.22 |
| Entry latitude, deg. | 23.28 |
| Atm. relative entry speed, km/s | 10.82 |
| Atm. relative heading angle, deg. | 89.99 |
| Atm. relative EFPA, deg. | −5.20 |
Table 8.
Venus SmallSat aerocapture entry corridor.
Table 8.
Venus SmallSat aerocapture entry corridor.
| Parameter | Value |
|---|
| Overshoot limit EFPA, deg. | −5.10 |
| Undershoot limit EFPA, deg. | −5.53 |
| TCW, deg. | 0.43 |
Table 9.
Effect of density variations on Venus aerocapture corridor.
Table 9.
Effect of density variations on Venus aerocapture corridor.
| Parameter | Min. Density | Average | Max. Density |
|---|
| Overshoot, deg. | −5.171 | −5.109 | −5.057 |
| Undershoot, deg. | −5.582 | −5.530 | −5.483 |
| TCW, deg. | 0.410 | 0.421 | 0.427 |
Table 10.
Venus aerocapture Monte Carlo uncertainties.
Table 10.
Venus aerocapture Monte Carlo uncertainties.
| Category | Variable | Nominal | Uncertainty | Distribution |
|---|
| Navigation | EFPA | −5.20 deg. | ±0.10 deg. (3) | Normal |
| Atmosphere | Mean density | - | ±3 | Normal |
| | Random | - | rpscale = 1 | Uniform |
| Aerodynamics | | 7.5 | ±10% (3) | Normal |
Table 11.
Statistics for Venus aerocapture orbit apoapsis altitude.
Table 11.
Statistics for Venus aerocapture orbit apoapsis altitude.
| Achieved Apoapsis Altitude Bin | Percentage |
|---|
| ±400 km of target | 91.4% |
| ±600 km of target | 96.2% |
| ±800 km of target | 98.4% |
| ±1000 km of target | 99.0% |
Table 12.
Statistics from Venus aerocapture Monte Carlo simulations.
Table 12.
Statistics from Venus aerocapture Monte Carlo simulations.
| Parameter | Min. | 5%-ile | Avg. | 95%-ile | Max. |
|---|
| Apoapsis alt., km | 1399 | 1865 | 2107 | 2510 | 5418 |
| Periapsis alt., km | 97.5 | 99.3 | 101.2 | 102.6 | 103.4 |
| Deceleration, g | 4.13 | 5.47 | 7.11 | 8.81 | 9.51 |
| Heat rate, W/cm2 | 129 | 144 | 157 | 168 | 191 |
| Heat load, kJ/cm2 | 10.4 | 12.3 | 16.1 | 19.7 | 22.2 |
| PRM , m/s | 22.9 | 26.1 | 26.5 | 27.1 | 28.2 |
| ACM , m/s | −552.7 | −101.5 | −21.0 | 28.2 | 131.4 |
Table 13.
Mars small satellite constellation targeting and orbiter deflection maneuvers.
Table 13.
Mars small satellite constellation targeting and orbiter deflection maneuvers.
| Maneuver | Type | Time, Days | , m/s |
|---|
| Targeting | E-7 | 4.63 |
| Targeting | E-6 | 5.38 |
| Targeting | E-5 | 6.43 |
| Targeting | E-4 | 7.99 |
| Orbiter deflection | E-3 | 0.95 |
Table 14.
Venus small satellite constellation targeting and orbiter deflection maneuvers.
Table 14.
Venus small satellite constellation targeting and orbiter deflection maneuvers.
| Maneuver | Type | Time, Days | , m/s |
|---|
| Targeting | E-4 | 4.71 |
| Targeting | E-3.5 | 5.37 |
| Targeting | E-3 | 6.18 |
| Targeting | E-2.5 | 7.29 |
| Orbiter deflection | E-2 | 18.2 |