Performance Analysis and Optimization of Fuel Tank Ground-Based Washing Inerting on Unmanned Aerial Vehicles

Abstract

In this study, a ground-based washing inerting (GBWI) method was proposed, and the application of GBWI in the field of fire prevention and explosion suppression of unmanned aerial vehicle (UAV) fuel tanks was studied using a volume of fluid (VOF) two-phase flow model at a given typical flight envelope. The variation in oxygen concentration in tank ullage during flight was calculated considering the entrance of ambient air and the escape of dissolved oxygen from the fuel. The results indicate that the oxygen concentration in the ullage increases with increasing initial oxygen concentration in the ullage and the initial fuel load after GBWI. In the climb and cruise stages, the oxygen concentration in the ullage increases slowly, while in the tactical descent stage, the oxygen concentration in the ullage increases rapidly, easily exceeding the limiting oxygen concentration (LOC) of aviation fuel combustion. To expand the application range of GBWI, an optimization scheme of nitrogen filling protection was proposed so that the GBWI could meet the requirements of an inert tank at different fuel loads and initial oxygen concentrations in the ullage. Compared with a traditional on-board inert gas generation system (OBIGGS), the optimized GBWI method could greatly reduce the fuel compensation loss and improve the maneuverability of UAVs at the same time.

1. Introduction

The combustion and explosion of aircraft fuel tanks are major reasons for aircraft crashes. For military aircraft, flight conditions can be severe, and when an aircraft is attacked by artillery shells, fuel tank explosions can easily occur, resulting in the destruction of the aircraft [1]. During the Vietnam War, the USA Air Force lost thousands of planes because of small anti-aircraft weapon attacks that caused fuel tanks to explode [2].

When the oxygen concentration in tank ullage is higher than the LOC required for fuel combustion, a combustion explosion can easily occur when an external ignitor is present; the LOC is 12% for civilian aircraft and 9% for military aircraft, respectively [3,4,5]. Therefore, reducing the oxygen concentration in the ullage to a level lower than the LOC can effectively reduce fuel tank flammability and improve aircraft safety performance.

Inerting is a fuel tank protection technology that can effectively reduce the oxygen concentration in ullage in real time. It injects inert gases such as N₂, halon or CO₂ into the ullage to replace oxygen and enhance fuel tank safety [6,7]. Fuel tank inerting technology was developed in the 1960s to prevent military aircraft from burning and exploding when attacked by 23 mm shells [8]. With the further development of tank inerting technology, the OBIGGS has become the most economical and mature tank inerting technology at present. It uses a hollow fiber membrane to separate air to form nitrogen-enriched air, thus inerting the fuel tank. The OBIGGS has been widely used in the Boeing series, Airbus series, large aircraft such as the C919 and F-22, AH-64 attack helicopters and Y20 military transport aircraft [9,10].

Several problems have been exposed in the OBIGGS in terms of inerting technology. For example, it needs to bleed air from the high-pressure section of the engine, which reduces dynamic engine performance. Therefore, it is not suitable for helicopters, small transport aircraft and other models without high-pressure air [11,12]. The heavy weight and large volume of hollow fiber membrane air separation components increase aircraft weight and reduce the available cabin space [13].

To reduce the additional weight of aircraft and the adverse effects of OBIGGS on aircraft engine performance, the FAA proposed a theoretical method named GBWI [14]. GBWI refers to a fuel tank protection technology that washes the fuel tank with nitrogen-enriched air generated by the ground air separation assembly when the aircraft is shut down on the ground, in order to reduce the oxygen concentration in the ullage and keep the tank in an inerted state on the ground and in flight. The American Aviation Regulatory Advisory Committee has noted that GBWI is a fuel tank inerting method with considerable advantages and development potential in terms of the economic cost [15].

The performance of GBWI is related to the initial oxygen concentration, fuel load and flight status. An experimental study on a Boeing 737 showed that after washing the ullage of the central wing tank to an oxygen concentration of 8%, the oxygen concentration in the ullage remained at 10–12% during flight, lower than the LOC. When the fuel load was 20%, the oxygen concentration in the ullage could be kept below 10% during take-off and climbing; however, when the fuel load was 80%, this level could only be maintained for 15 min after take-off because when the fuel load increased, the amount of escaped dissolved oxygen from the fuel increased. The larger the fuel load, the smaller the ullage volume, so the influence of the ambient air entering became greater [16].

GBWI eliminates the need to carry an OBIGGS, greatly reducing aircraft weight and increasing the available cabin volume. Because there is no pilot directly involved in UAV flight, the structural weight related to ensuring pilot safety can be greatly reduced. Moreover, the compact structure of UAVs requires higher mobility performance. Therefore, the application of GBWI in UAVs is of great significance in reducing weight, lowering fuel compensation loss and enhancing maneuverability [17,18,19].

In this paper, the CFD method was used to analyze the application performance of GBWI in UAVs under different conditions, and then GBWI was optimized to increase the applicable boundaries. This study provides a reference for the application and design of GBWI in UAVs.

2. Computational Conditions

2.1. Flight Envelope

The main threat of ignition of military aircraft comes from enemy fire, and thus aircraft are in a relatively safe area when descending and landing as the possibility of attack is very small. During the descent and return flight of UAV, with the increase in environmental pressure, a large amount of air enters the ullage of the tank, resulting in the increase in oxygen concentration in the ullage, which easily exceeds the LOC. At present, even if a large OBIGGS is used in the fuel tank inert system of passenger aircraft and military aircraft, in practice, it cannot guarantee that the oxygen concentration is lower than the LOC and comes at great cost. Therefore, the variation in oxygen concentration in the fuel tank is not an indicator which is able to evaluate the performance of the inert system during the descent and return stage. Due to the limitations of UAV structure, weight, size and other factors, it is difficult to guarantee the inert state of the fuel tank during the whole time and there is no mandatory requirement for the oxygen concentration level in ullage in the descent and return stage of the design index requirements. Therefore, in this paper, the descent and return stage is not considered in the flight envelope. According to the actual UAV production requirements from aircraft manufacturers, a typical flight envelope design is shown in Figure 1.

The flight envelope of the UAV mainly includes the stages of climb–cruise–climb–cruise–tactical descent–cruise. The external environmental pressure and temperature of the UAV fuel tank change with the change in flight altitude and can be calculated according to the USA Standard Atmosphere [20], which can be expressed as:

$$p=\{\begin{array}{l}1.01325\times {10}^{{}^5}{(1-0.225577\times {10}^{-4}H)}^{5.25588}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}H\le 11000 \mathrm{m}\\ 2.263204\times {10}^{-4}\exp [-1.576885\times {10}^{-4}(H-11000)] 11000 \mathrm{m} < H < 12000 \mathrm{m}\end{array}$$

(1)

$$T=\{\begin{array}{l}283.15-\xi H\hspace{1em}0<H<11000 \mathrm{m}\\ 216.65\hspace{1em}11000 \mathrm{m}<H<20000 \mathrm{m}\end{array}$$

(2)

where p is pressure, Pa; H is altitude, m; T is temperature, K; and ξ is the annual average temperature lapse rate, 0.0065 K/m.

2.2. Fuel Tank Model

Since the fuel consumption sequence in the tank compartment during flight is not considered, the tank can be assumed to be singular. The tank volume is 7.5 m³ with a length of 3 m, width of 2.5 m and height of 1 m, as shown in Figure 2. The fuel outlet boundary is used to simulate fuel consumption during flight and is 100 mm in diameter. The vent is used to maintain pressure balance with the ambient air and is 50 mm in diameter.

2.3. Mathematical Model

There is an obvious gas–liquid interface in the fuel tank, and the VOF two-phase flow model can reflect the dynamic change in the free liquid level in real time. The VOF model uses a set of momentum equations in the simulation and tracks the volume fraction of each phase fluid in the region [21,22].

Mass conservation equation:

$$\frac{\partial \rho }{\partial t}+\nabla \cdot (\rho \mathit{u})=S_{\mathrm{m}}$$

(3)

where ρ is density, kg/m³; t is time, s; u is the velocity vector, m/s; and S_m is the mass source term, kg/m³.

Momentum conservation equation:

$$\frac{\partial (\rho \mathit{u})}{\partial t}+\nabla \cdot (\rho \mathit{u}\mathit{u})=-\nabla p+\nabla \cdot (\mu (\nabla \mathit{u}+\nabla {\mathit{u}}^{\mathrm{T}}\left)\right)+\rho \mathit{g}+\mathit{F}$$

(4)

where p is pressure, Pa; g is gravitational acceleration, m/s²; and F is the momentum source term, N/m³.

Energy conservation equation:

$$\frac{\partial }{\partial t}(\rho E)+\nabla \cdot (\mathit{u}(\rho E+p))=\nabla \cdot [k_{eff}\nabla T-{\displaystyle \sum h_{\mathrm{q}}{J}_{\mathrm{q}}]+S_{\mathrm{E}}}$$

(5)

where E is energy, J/kg; k_eff is the effective thermal conductivity, W/(m∙K); T is temperature, K; h_q is the enthalpy of phase q, J/kg; J_q is the flux of phase q, kg/(m³∙s); and S_E is the energy source term, W/m³.

Turbulence equation.

The k-ε model is used to describe the gas–liquid flow characteristics in the fuel tank, which can be expressed as:

$$\frac{\partial }{\partial t}(\rho k)+\frac{\partial }{\partial x_i}(\rho k{u}_i)=\frac{\partial }{\partial x_j}(P_k{\mu }_{\mathrm{eff}}\frac{\partial k}{\partial x_j})+G_k+G_b-\rho \epsilon -Y_M+S_k$$

(6)

$$\frac{\partial }{\partial t}(\rho \epsilon )+\frac{\partial }{\partial x_i}(\rho \epsilon u_{\mathrm{i}})=\frac{\partial }{\partial x_j}(P_{\epsilon }{\mu }_{\mathrm{eff}}\frac{\partial \epsilon }{\partial x_j})+C_{\epsilon 1}\frac{\epsilon }{k}(G_k+C_{\epsilon 3}{G}_b)-C_{\epsilon 2}\rho \frac{{\epsilon }^2}{k}-R_{\epsilon }+S_{\epsilon }$$

(7)

where k is the turbulence kinetic energy, kg/(m∙s³); ε is the dissipation rate of turbulence kinetic energy; u_i is the velocity, m/s; P_k and P_ε are the inverse effective Prandtl numbers; G_k is the generation of turbulence kinetic energy due to the mean velocity gradients, kg/(m∙s³); G_b is the generation of turbulence kinetic energy due to buoyancy, kg/(m∙s³); Y_M is the contribution of the fluctuating dilatation in compressible turbulence to the overall dissipation rate, kg/(m∙s³); S_k and Sε are user-defined source terms, kg/(m∙s³); C_ε1, C_ε2 and C_ε3 are constants; and Rε is an additional term, kg/(m∙s³).

Species transport equation:

$$\frac{\partial }{\partial t}({\alpha }_q{\rho }_q{Y}_{n,q})+\nabla \cdot ({\alpha }_q{\rho }_q\mathit{u}{Y}_{n,q}-{\alpha }_{q}D\nabla Y_{n,q})=S_{\lg ,\mathrm{n}}$$

(8)

where Y_n,q is the mass fraction of species n in phase q; D is the mass diffusion coefficient, m²/s; and S_lg,n is the source term of mass transfer, kg/m³.

VOF two-phase flow model.

The density and viscosity of the gas and liquid mixture can be expressed as:

$$\rho ={\alpha }_l{\rho }_l+{\alpha }_g{\rho }_g$$

(9)

$$\mu ={\alpha }_l{\mu }_l+{\alpha }_g{\mu }_g$$

(10)

where α_l and α_g are the volume fractions of liquid and gas in the cell, respectively; ρ_l and ρ_g are the densities of liquid and gas in the cell, respectively, kg/s; and μL and μ_g are the viscosities of liquid and gas in the cell, Pa∙s.

The gas and liquid volume fractions range from 0 to 1, and the total volume of the gas and liquid is 1.

$${\alpha }_{\mathrm{g}}+{\alpha }_{\mathrm{l}}=1$$

(11)

The gas–liquid interface can be tracked in real time by solving the continuity equation of the volume fraction [23]:

$$\frac{\partial {\alpha }_{\mathrm{l}}}{\partial t}+\mathit{u}\cdot \nabla {\alpha }_{\mathrm{l}}=0$$

(12)

Interfacial mass transfer model.

Dissolved oxygen in fuel escapes during flight due to changes in the internal and external environmental conditions of the tank. The gas mass transfer between the ullage and fuel can be expressed as:

$$S=Ka(c*-c)$$

(13)

where S is the gas mass transfer source, kg/(m³∙s); K is the gas mass transfer rate, m/s; a is the interfacial area between the gas and liquid, m²/m³; c* is the instantaneous dissolved gas concentration at the gas–fuel interface, kg/m³; and c is the dissolved gas concentration in the fuel, kg/m³.

The gas mass transfer coefficient K can be calculated according to the classic penetration mass transfer theory:

$$K=2\sqrt{\frac{D_{\mathrm{g}}}{\pi t}}$$

(14)

where D_g is the mass diffusion coefficient of O₂ or N₂ in jet fuel, m²/s, as can be inferred from the experimental values measured by the authors [12,24].

2.4. Assumptions

Since there is no violent shaking in the fuel tank and the temperature and pressure do not change during ground washing, the gas–liquid interfacial mass transfer rate is low. Therefore, it can be considered that the concentration of dissolved oxygen and nitrogen in the fuel always remains at the saturated concentration of dissolved oxygen and nitrogen at room temperature and pressure. The influence of UAV acceleration on oxygen concentration change is not considered for the time being, and the fuel–gas interface is assumed to remain stable in the calculation for the entire flight envelope.

2.5. Boundary Settings

Nitrogen-enriched air is used to wash the fuel tank ullage, where the gas components in the ullage are O₂ and N₂. Regardless of the change in the fuel consumption rate when the aircraft accelerates, the fuel consumption rate during flight can be set as 1.6 × 10⁻⁴ m³/s.

The fuel tank wall is set as a “no-slip wall”; the fuel outlet at the bottom is the velocity outlet boundary, and the vent at the right top is the pressure outlet boundary. Gravitational acceleration is taken as the same for the entire flight envelope, extending in the negative direction of the Y-axis with a magnitude of −9.8 m/s². The fuel outlet is the velocity outlet, and the velocity is 0.0016 m/s. In the calculation process, the component transport equation and energy equation are opened, the standard k-ε model is selected for the turbulence model, and the PISO model is selected for pressure and velocity coupling [25]. To reduce the calculation cost, a first-order upwind model can be used to calculate the energy equation, turbulence equation, momentum equation and component transport equation under the premise of ensuring the accuracy of the calculation.

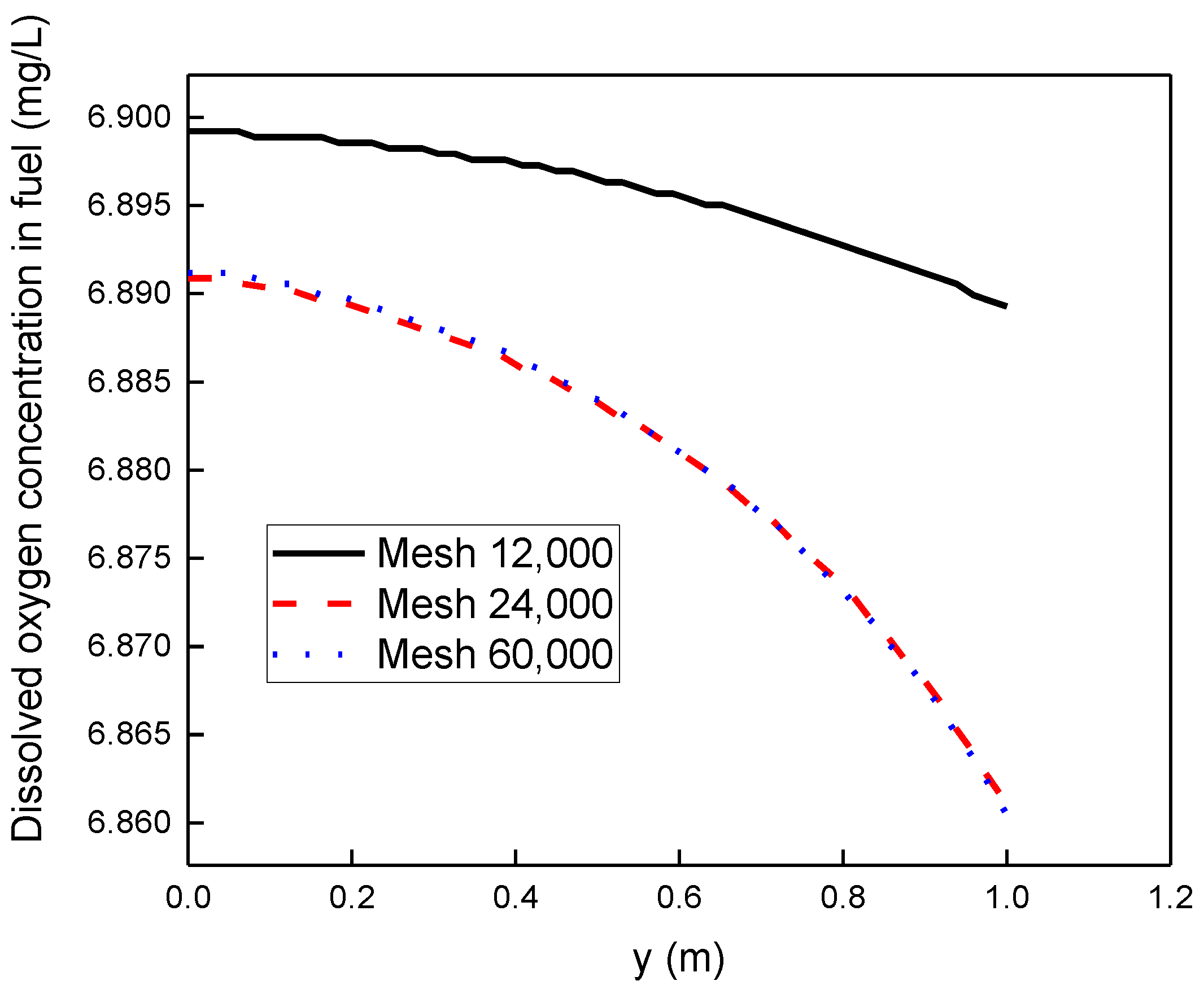
2.6. Validation of the CFD Calculation Model

Since the tank studied in this paper is small and simple in structure, the two-dimensional model can be used for calculation. In order to eliminate the influence of mesh quality on the calculation results, three different quantities of mesh were considered, 12,000, 24,000 and 60,000, and these three grids were used to simulate the variation in oxygen concentration in the fuel tank during the climbing stage of the UAV after ground washing. The initial fuel load was 50%, and the initial oxygen concentration in the ullage of the fuel tank was 2%. According to the flight envelope, the dissolved oxygen concentration in the fuel was calculated when the UAV climbed for 120 s. The distribution of the dissolved oxygen concentration in fuel on the line segment (x = 1500 mm, 0 < y < 1000 mm) is shown in Figure 3. When the number of grids is 24,000, the calculation result is very close to that when the number of grids is 60,000. Therefore, considering the calculation time and cost, on the basis of ensuring accuracy, 24,000 grids were selected for simulation calculation.

The CFD calculation model and gas mass transfer model adopted have been verified by the authors’ previous experimental studies. Reference [26] reports the dissolved oxygen evolution of an inert aircraft fuel tank under different pressure conditions using the volume of fluid model in the CFD software: the maximum relative differences of oxygen concentration in ullage and the dissolved oxygen concentration between the CFD calculation results and experimental measurements are all less than 8%. Therefore, the CFD calculation model adopted in this paper has high accuracy and can be used to study the performance of GBWI on UAVs.

3. Results and Discussion

The variation in oxygen concentration in the ullage at a given flight envelope is calculated after ground washing. The initial fuel loads are 30%, 50% and 70%, and the initial oxygen concentrations in the ullage are 1%, 2% and 3%. The variations in the oxygen concentration in the ullage at different stages of the flight envelope are shown in Figure 4. Taking the initial fuel load of 70% and initial oxygen concentration in the ullage of 3% as an example, the distribution of dissolved oxygen concentration in the fuel at different flight times is shown in Figure 5. Since no fuel is present in the ullage, the dissolved oxygen concentration in fuel is zero and is shown in blue. The higher the concentration of dissolved oxygen in fuel, the redder the cloud image color in Figure 5.

Combining Figure 3 and Figure 4, it can be seen that the oxygen concentration in the ullage gradually increases after the aircraft takes off, which is due to the combined effect of the entry of external air and the escape of dissolved oxygen in the fuel. The oxygen concentration in the ullage increases with increasing initial oxygen concentration at the same fuel load. The higher the fuel load, the higher the oxygen concentration in the ullage at the same initial oxygen concentration in the ullage. This is because the higher the fuel load, the more dissolved oxygen escapes, and the smaller the volume of the ullage, the greater the influence on the oxygen concentration change in the ullage [27].

During the climb and cruise stages, the oxygen concentration in the ullage increases slowly, while during the tactical descent stage, due to the increase in external environmental pressure, a large amount of air enters the ullage, resulting in a sharp increase in the oxygen concentration.

Taking the initial fuel load of 70% and the initial oxygen concentration in the ullage of 3% as an example, the growth rate of the oxygen concentration in the climbing and cruising stages is less than 0.18%/s, and it is 2.9%/s in the tactical descent stage. When the initial fuel load is 30% and the initial oxygen concentration in the ullage is 1%, the oxygen concentration in the ullage is 8.88% at the end of flight, which is less than the LOC and meets the inert requirements of military aircraft in the whole flight envelope. Under other conditions, at the end of the flight, the oxygen concentration in the ullage is more than 9%, and the maximum oxygen concentration reaches 12.31%.

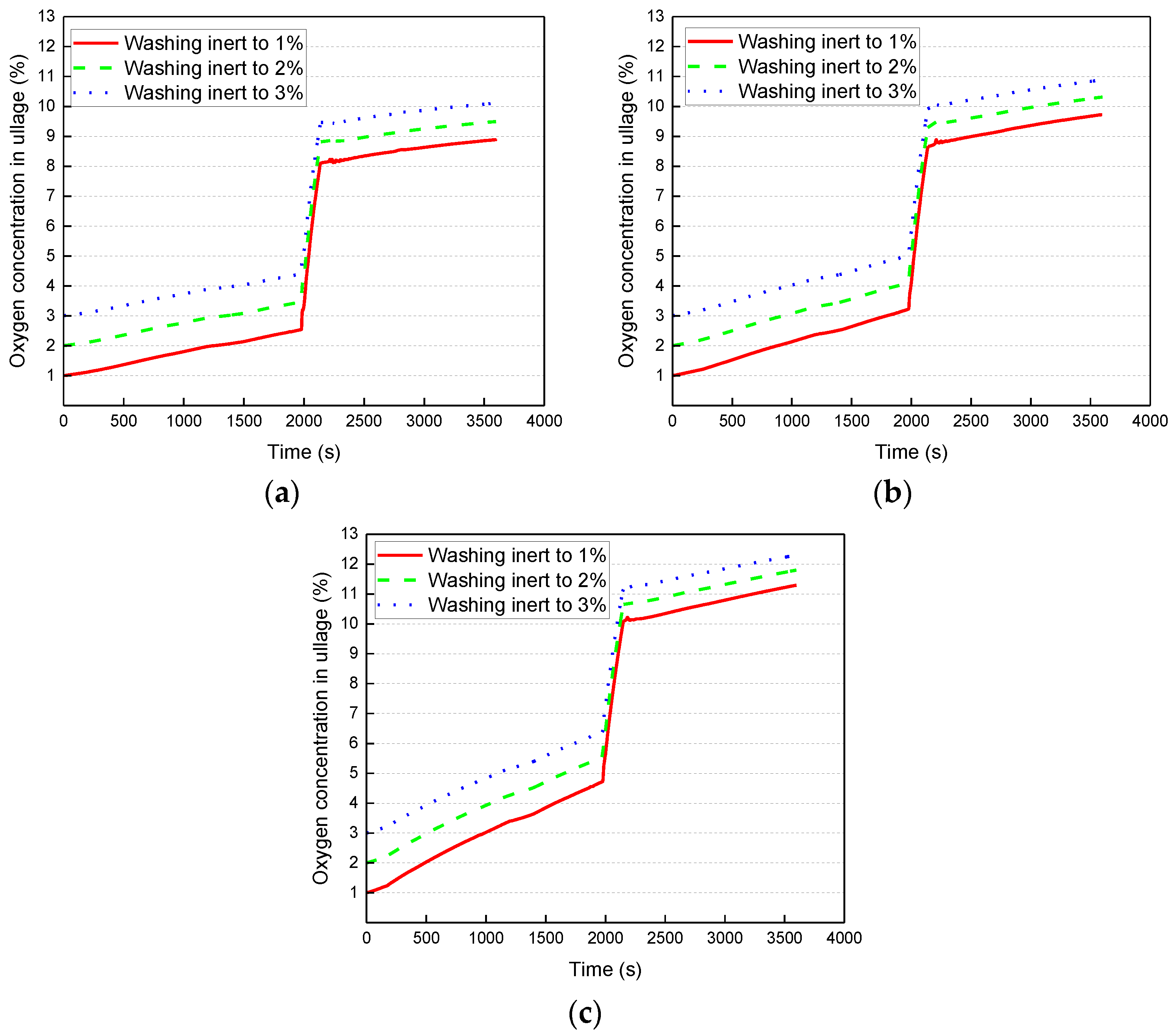
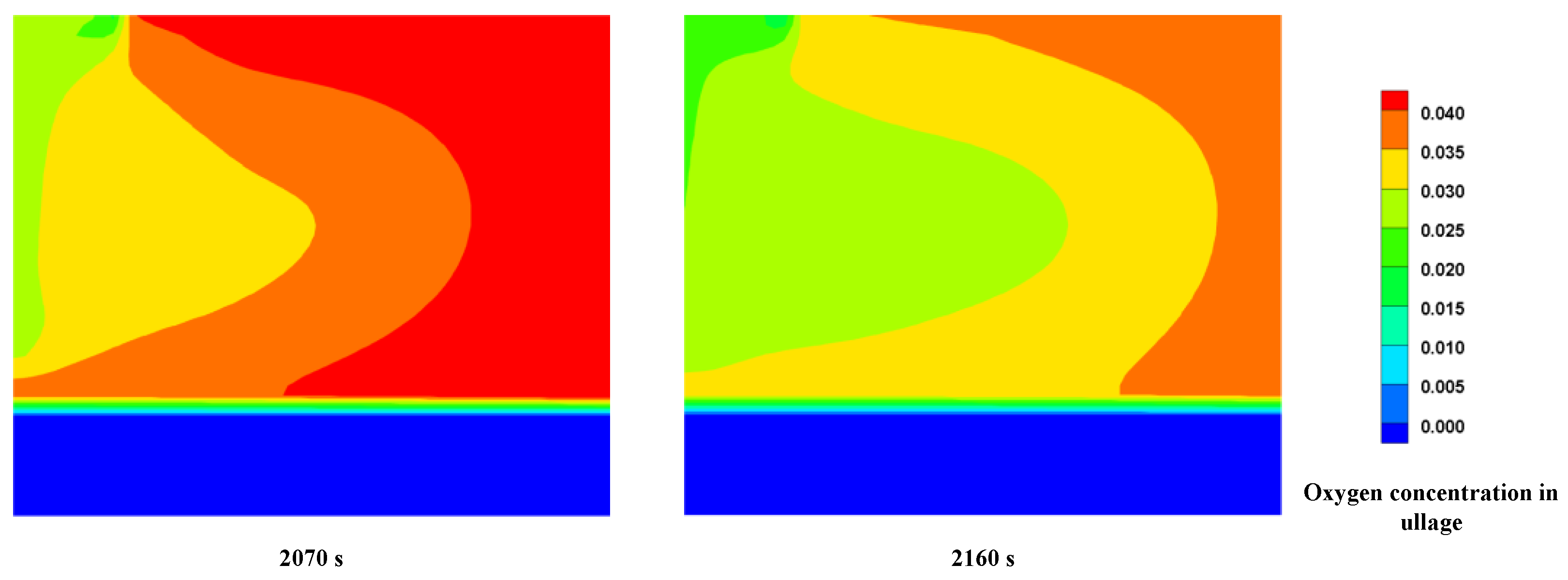
4. Optimization of GBWI

According to the calculation results above, the oxygen concentration in the ullage may exceed the LOC at different initial fuel loads and initial oxygen concentrations in the ullage, so GBWI cannot fully meet the requirements of UAV inertness. The rapid increase in oxygen concentration is mainly caused by the large amount of outside air entering the tank during tactical descent. Due to the short time of the tactical descent stage, nitrogen can be stored in cylinders and injected into the ullage during the tactical descent stage in order to reduce the increase rate of oxygen concentration and fulfill the tank’s inerting requirements. This is called nitrogen filling protection technology.

The nitrogen inlet is arranged on the top left of the tank, and the boundary condition is set at the mass inlet. According to the calculation results above, it is only necessary to study the inerting effect of nitrogen filling protection technology with different fuel loads when the initial oxygen concentration in the ullage is 3%. To reduce the quality of nitrogen carried, the nitrogen flow rate should be consistent with the flow rate of external air into the tank. The air mass flow of the vent in the tactical descent stage can be monitored from the CFD calculation, as shown in Figure 6.

Nitrogen is injected into the ullage during the entire period of tactical descent, and the oxygen concentration decreases. Taking the initial fuel load of 30% as an example, the oxygen concentration distribution in the ullage at different times in the tactical descent stage is shown in Figure 7, and the oxygen concentration in ullage is expressed as a volume fraction. The lower part of the tank is fuel, which is in the liquid state. Therefore, the oxygen concentration in ullage is zero, which is shown in blue. The higher the concentration in ullage, the redder the cloud image color in Figure 7.

The variation in oxygen concentration in the ullage from the beginning of the tactical descent to the end of flight with nitrogen filling protection at different fuel loads is shown in Figure 8.

It can be seen from the calculation that the oxygen concentration in the ullage is always less than 9% at the flight envelope with nitrogen filling protection. The higher the initial fuel load, the higher the oxygen concentration in the ullage. This is because the higher the fuel load, the higher the oxygen concentration will be at the beginning of the tactical descent. Moreover, the higher the fuel load, the smaller the mass flow of the nitrogen inlet. Therefore, the tank inerting of UAVs can be realized by combining GBWI and nitrogen filling protection.

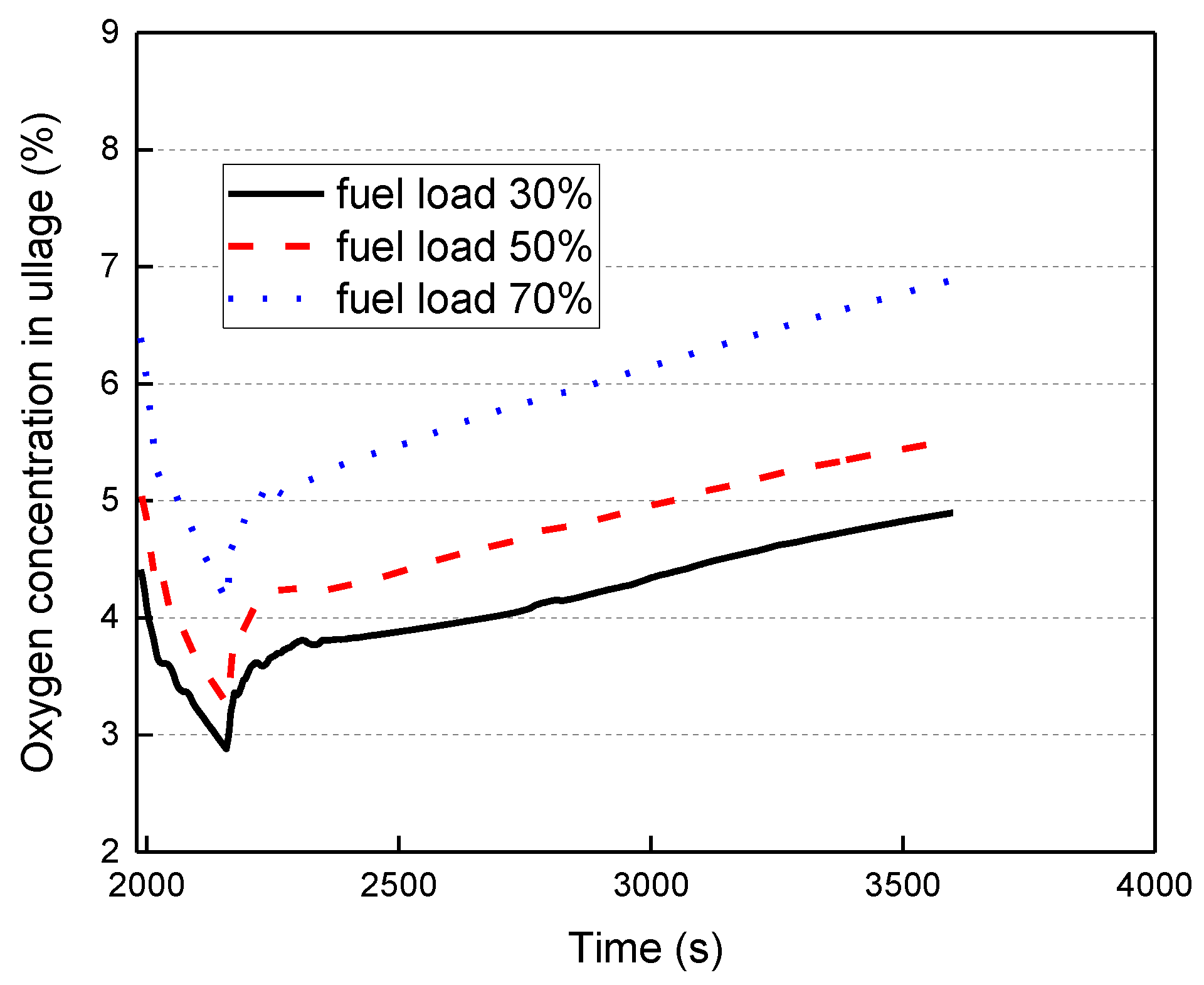
5. Inerting System Performance Comparison

According to the analysis above, the optimized GBWI can meet the inert requirements of UAVs, but the UAV needs to carry cylinders to store nitrogen, which increases the weight of the aircraft. To study the superiority of the optimized GBWI, the take-off total mass method is used to calculate the fuel compensation loss and compare it with the OBIGGS. The take-off total mass method is a compensatory loss evaluation method that combines the mass, drag and power required to compensate for the loss of aircraft with fuel consumption [28]. In the GBWI and OBIGGS, the items that increase the total take-off mass mainly include fixed system device mass and engine exhaust.

In the actual OBIGGS, the mass of a single hollow fiber membrane assembly is 8.25 kg, and in the optimized GBWI, the mass of the nitrogen storage cylinder is 2 kg. Due to the more economical and convenient source of nitrogen on the ground, the cost of nitrogen production on the ground is not considered. The comparison of fuel compensation loss between the optimized GBWI and OBIGGS is shown in

Table 1. Fuel compensatory loss in different inerting systems.

	Fuel Compensation Loss/kg
	Fuel Load 30%	Fuel Load 50%	Fuel Load 70%
Optimized GBWI	2.144	2.108	2.072
OBIGGS	8.340	8.325	8.312

.

It is clear that on the premise of meeting the inerting requirements, the fuel compensation loss caused by the optimized GBWI is much lower than that of the OBIGGS. This is due to the large mass of the hollow fiber membrane air separation module, while the influence of the volume of the air separation module on the aircraft structure is not taken into account. Therefore, the optimized GBWI can be applied to UAV fuel tank inerting with low fuel compensation loss.

6. Conclusions

In this paper, the application of GBWI in UAVs is analyzed and optimized using the CFD method. At a given flight envelope, the oxygen concentration in the ullage increases gradually after inert ground washing. During the climbing and cruising stages, the oxygen concentration in the ullage increases slowly, with a growth rate of less than 0.18%/s. During the tactical descent stage, the oxygen concentration in the ullage increases sharply, with a growth rate of 2.9%/s, due to the large amount of outside air entering the tank.

When the initial fuel load of the tank is 30% and the initial oxygen concentration in the ullage is 1%, the gas oxygen concentration in the ullage is always lower than the LOC during flight, which can meet the tank inerting requirements. The lower the initial oxygen concentration in the ullage and the smaller the initial fuel load, the more conducive it is to the application of GBWI.

Nitrogen filling protection technology is proposed after the optimization of GBWI. Combining these two technologies can meet the tank inerting requirements at different initial fuel loads and initial oxygen concentrations in the ullage, and the application range of GBWI is expanded. Compared with the OBIGGS, the optimized GBWI has smaller fuel compensation loss, so there are considerable advantages in applying the optimized GBWI to UAV inerting.