Analysis of Electric Transmission Failure Induced by Electrostatic Migration and Deposition of Abrasive Dusts in SADA
Abstract
:1. Introduction
2. Experimental Setup and Simulation Method
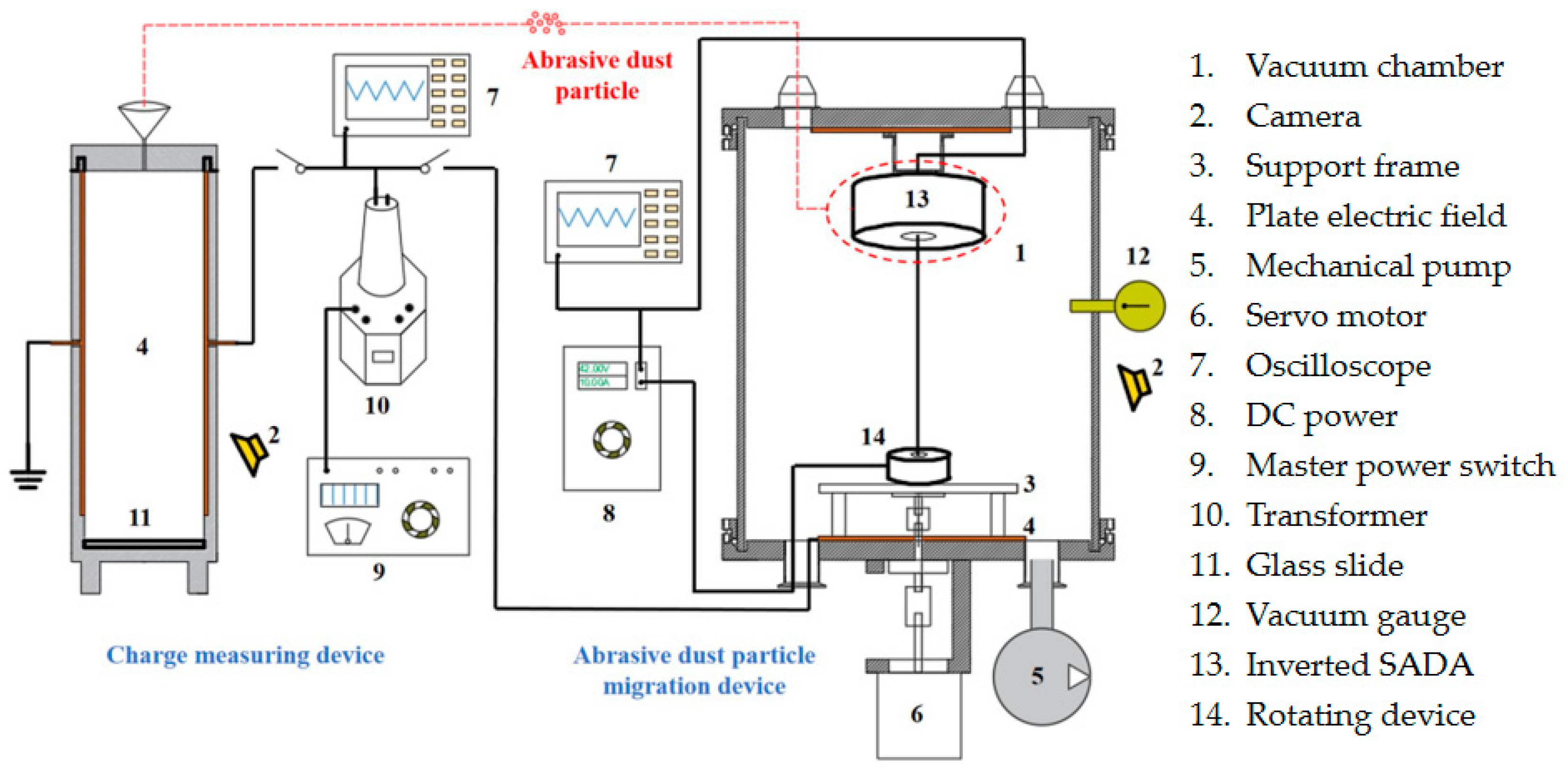
2.1. Experimental Setup
2.2. Simulation Method for Abrasive Dusts’ Migration
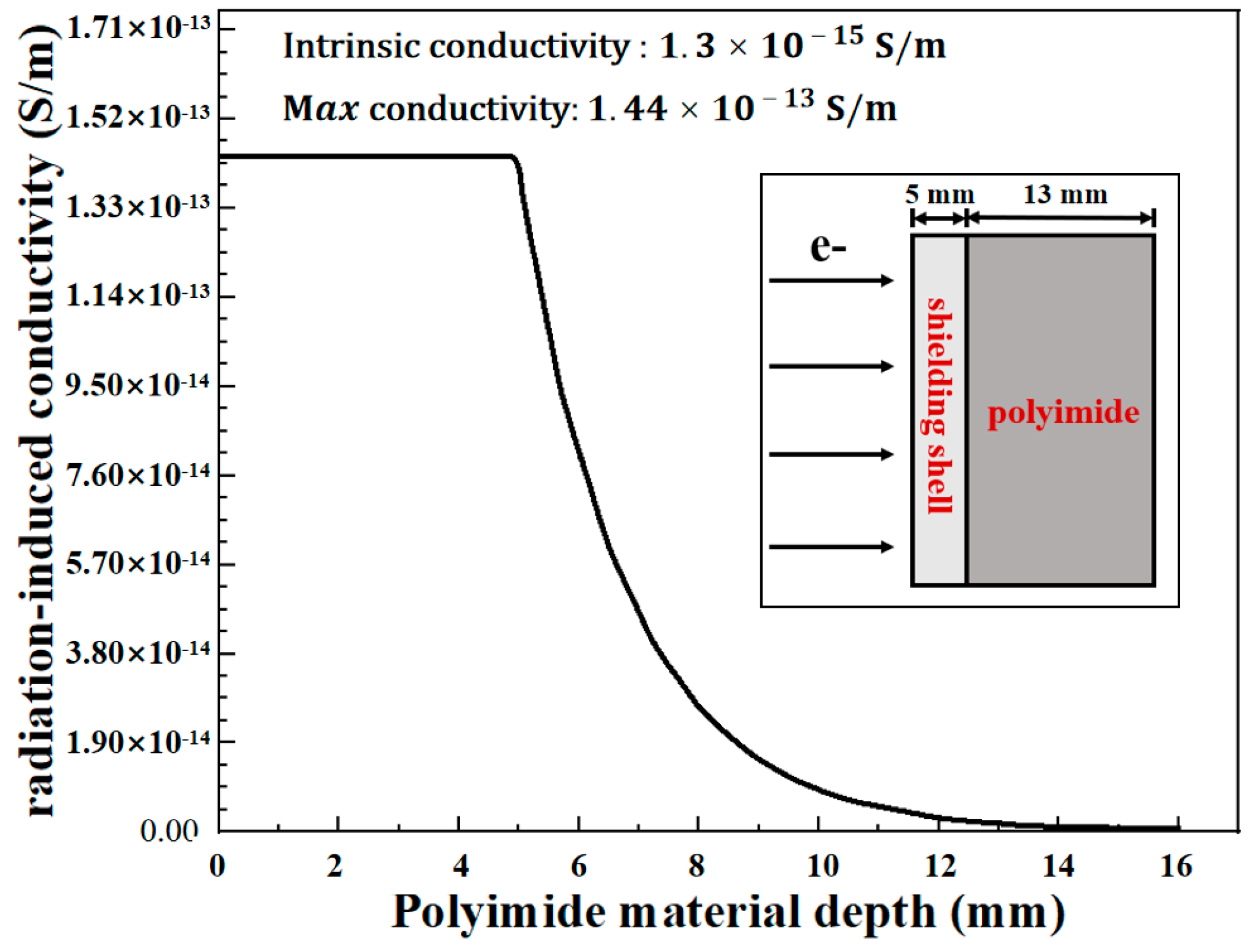
2.3. Space Irradiation Simulation Method
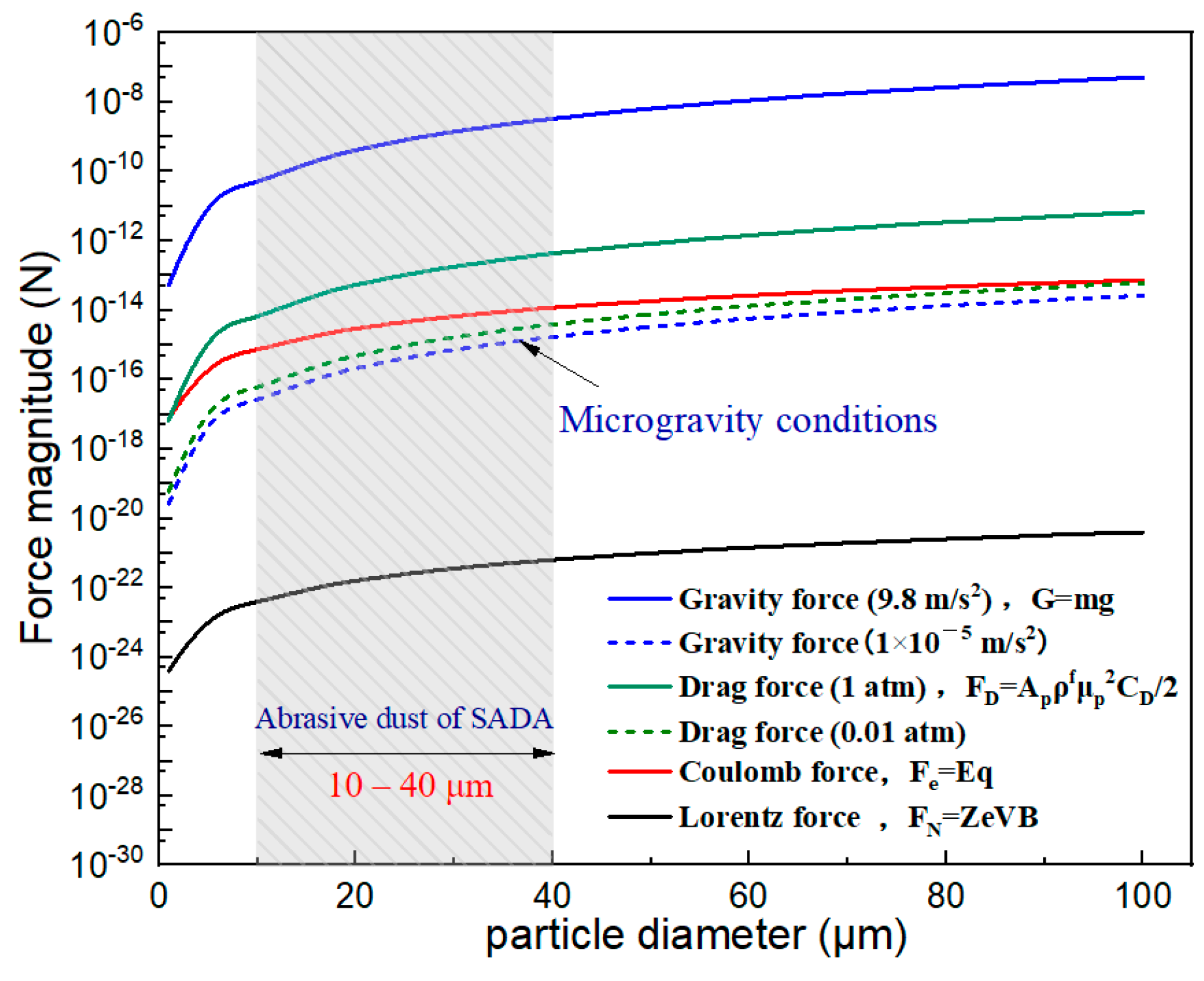
2.4. Force Analysis for Abrasive Dusts
3. Results and Discussion
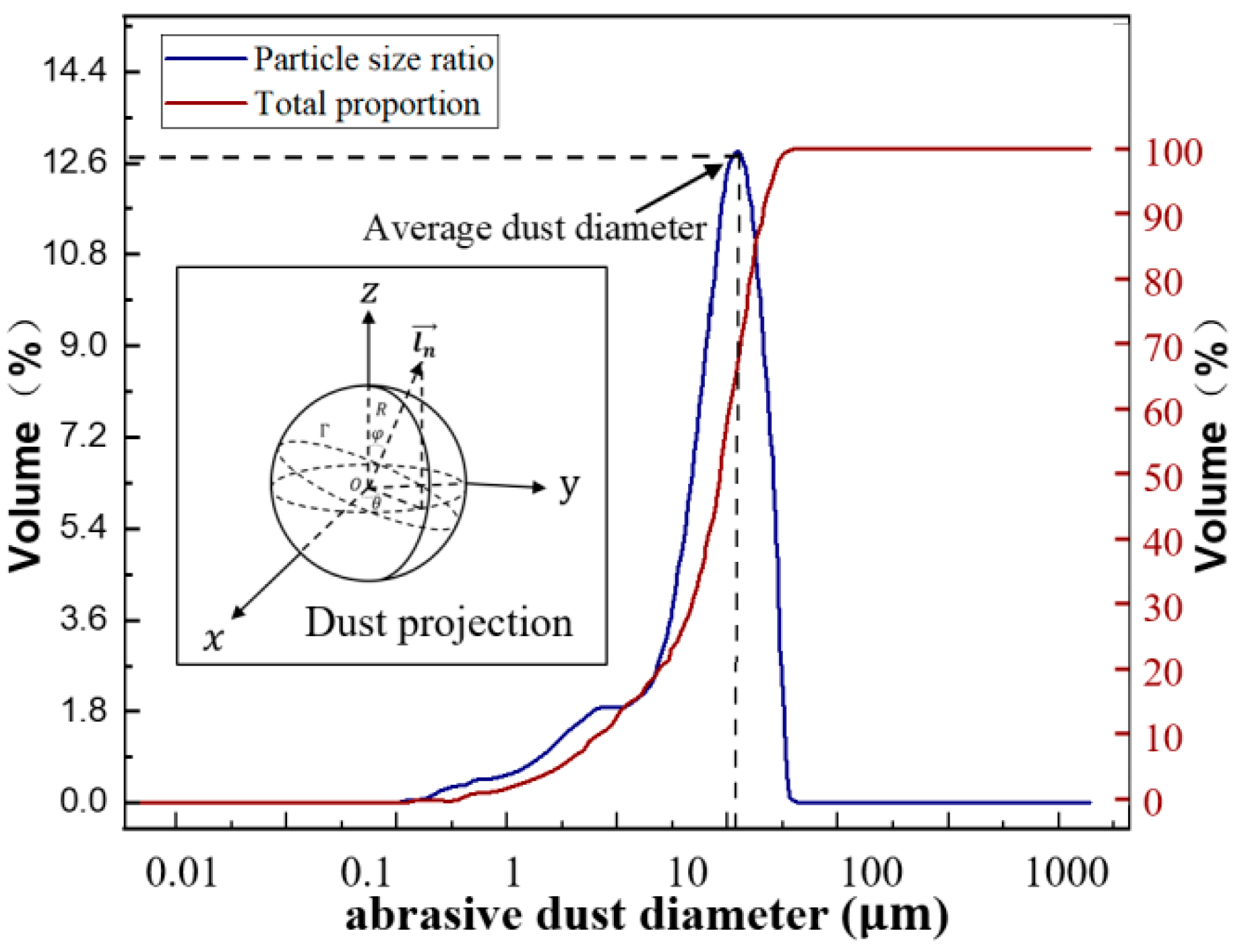
3.1. Experimental Characterization of Abrasive Dusts
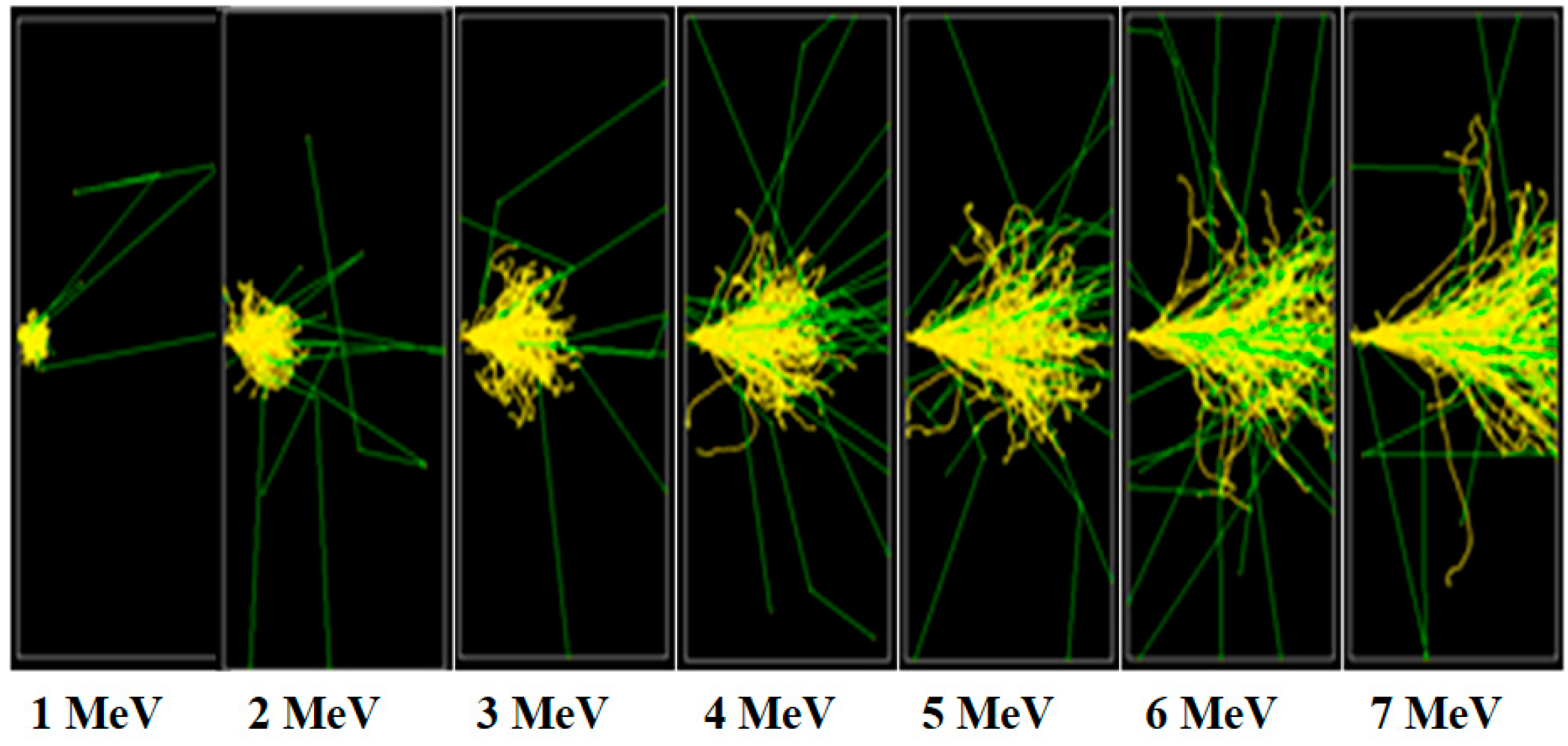
3.2. Damage Effect of Space Energetic Particles on SADA
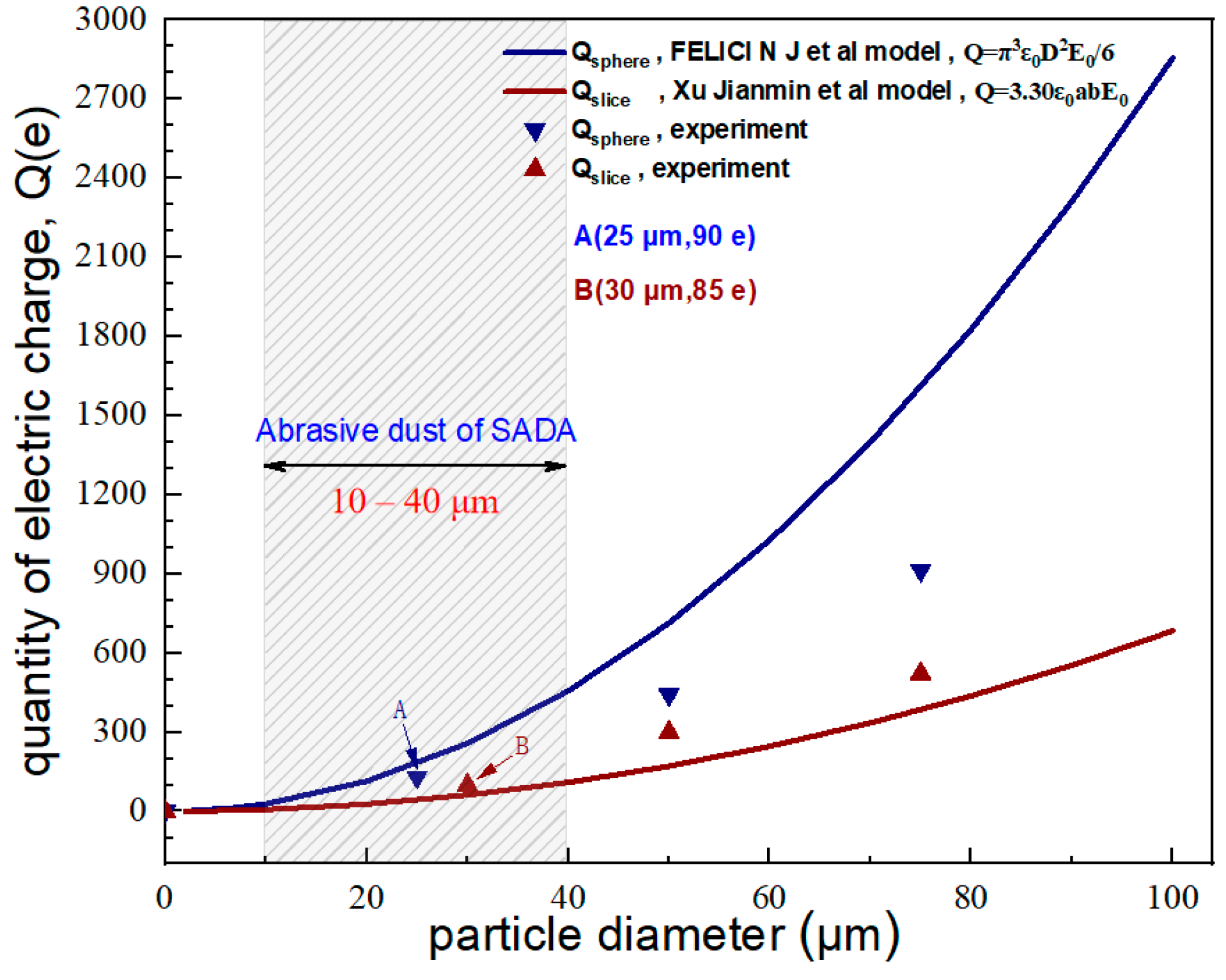
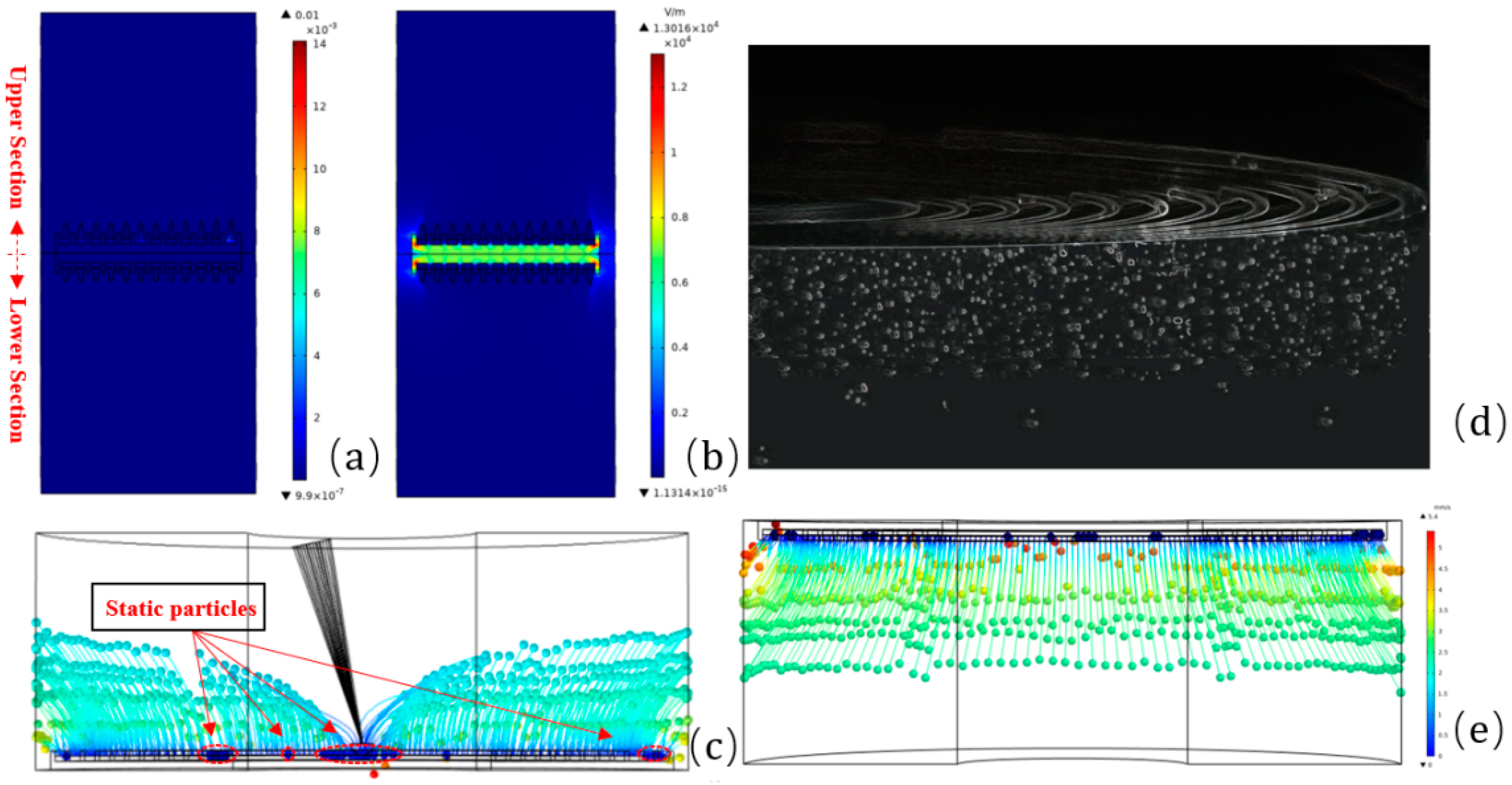
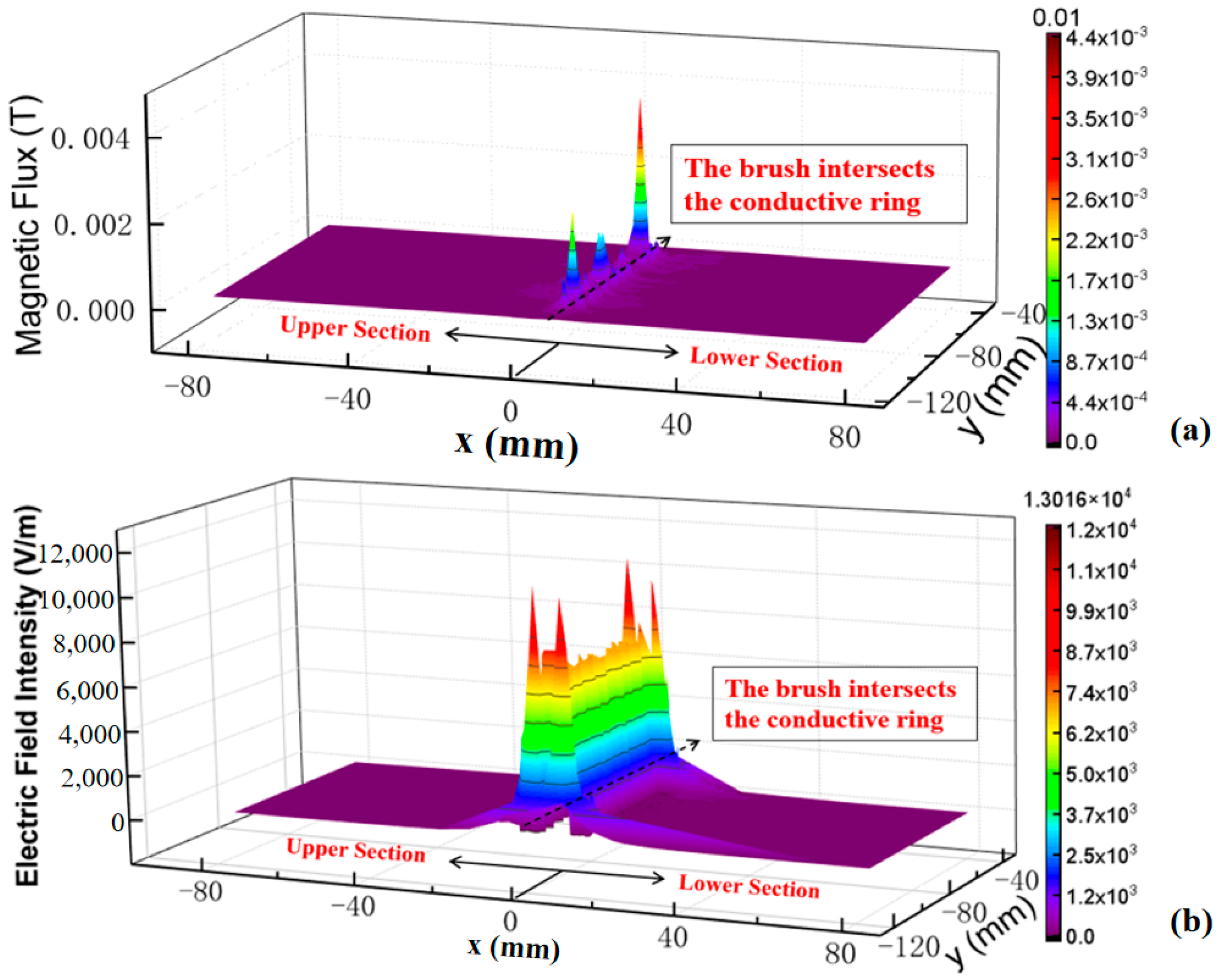
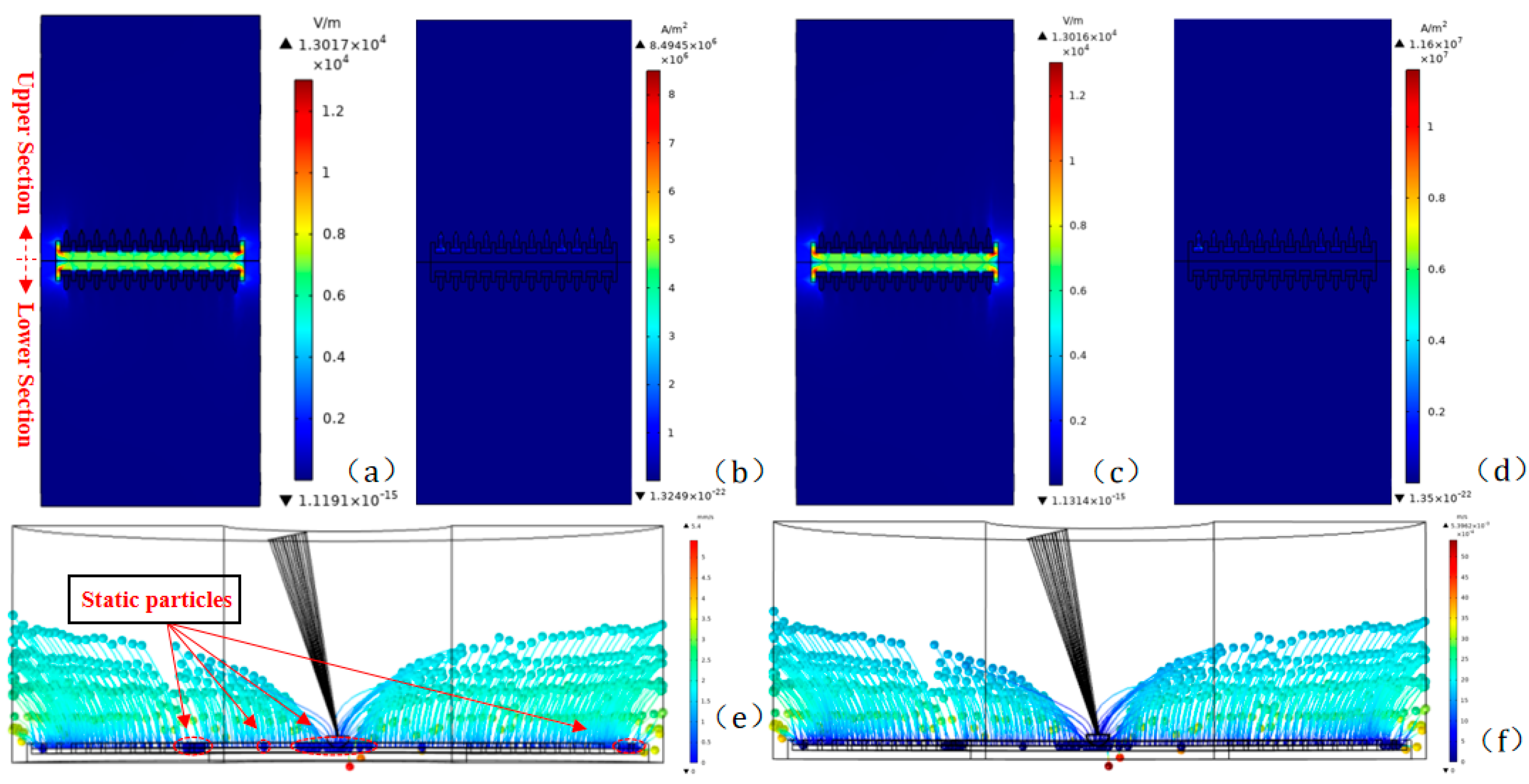
3.3. Model Validity
3.4. Migration and Deposition of Abrasive Dusts under Fault Conditions
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cao, Y.; Cao, D.; Wei, J.; Huang, W. Modeling for solar array drive assembly system and compensating for the rotating speed fluctuation. Aerosp. Sci. Technol. 2019, 84, 131–142. [Google Scholar] [CrossRef]
- Wang, X.; Min, D.; Pan, S.; Zheng, S.; Hou, X.; Wang, L.; Li, S. Coupling effect of electron irradiation and operating voltage on the deep dielectric charging characteristics of solar array drive assembly. IEEE Trans. Nucl. Sci. 2021, 68, 1399–1406. [Google Scholar] [CrossRef]
- Chen, J.P.; Cheng, W.; Wang, Y.F. Modeling and simulation of solar array drive assembly disturbance driving a flexible load. In Applied Mechanics and Materials; Trans Tech Publications: Zurich, Switzerland, 2014; Volume 565, pp. 67–73. [Google Scholar]
- Zhang, Y.; Meng, L.; Liu, M. Finite angle multichannel space slip ring design. IOP Conf. Ser. Earth Environ. Sci. 2019, 252, 022108. [Google Scholar] [CrossRef]
- Li, R.; Guo, F.; Yu, C.; He, Y.; Ye, Z.; Yuan, S. Development and validation of a mechatronic solar array drive assembly for mini/micro-satellites. Acta Astronaut. 2017, 134, 54–64. [Google Scholar] [CrossRef]
- Avino, F.; Martens, P.; Howling, A.A.; Bommottet, D.; Furno, I. Gas breakdown mitigation in satellite slip rings. Aerosp. Sci. Technol. 2019, 85, 229–233. [Google Scholar] [CrossRef]
- Zhao, X.; Nie, Y.; Chen, M.; Qin, H.; Xu, X. Analysis of contact characteristics of carbon brush/slip ring under eccentric oscillation of hydrogenerator rotor. Shock Vib. 2021, 2021, 1–12. [Google Scholar] [CrossRef]
- Greenwood, J.A.; Tripp, J.H. The contact of two nominally flat rough surfaces. Proc. Inst. Mech. Eng. 1970, 185, 625–633. [Google Scholar] [CrossRef]
- Zhang, J.; Ma, X.; Cheng, B.; Zhao, Y. Storage life modeling and analysis for contacting slip ring based on physics of failure. IEEE Trans. Compon. Packag. Manufact. Technol. 2017, 7, 1969–1980. [Google Scholar] [CrossRef]
- Grandin, M.; Wiklund, U. Wear and electrical performance of a slip-ring system with silver–graphite in continuous sliding against PVD coated wires. Wear 2016, 348–349, 138–147. [Google Scholar] [CrossRef]
- Dorsey, G. Fretting possibility in slip rings: A review. In Proceedings of the 2017 IEEE Holm Conference on Electrical Contacts, Denver, CO, USA, 10–13 September 2017; pp. 93–101. [Google Scholar]
- Zhang, D.R.; Jin, D. Slip-ring failure mechanism analysis and solution of airborne platform inertial navigation system. Chin. Inert. Technol. 2010, 18, 645–647. [Google Scholar]
- Song, K.; Ma, B.; Yang, G.; Jiang, J.; Wei, R.; Zhang, H.; Zhu, C. A Rotation-lightweight wireless power transfer system for solar wing driving. IEEE Trans. Power Electron. 2019, 34, 8816–8830. [Google Scholar] [CrossRef]
- Luza, L.M.; Wrobel, F.; Entrena, L.; Dilillo, L. Impact of atmospheric and space radiation on sensitive electronic devices. In Proceedings of the 2022 IEEE European Test Symposium (ETS), Barcelona, Spain, 23–27 May 2022; pp. 1–10. [Google Scholar]
- Lai, S.T. Fundamentals of Spacecraft Charging: Spacecraft Interactions with Space Plasmas; Princeton University Press: Princeton, NJ, USA, 2012; ISBN 978-1-4008-3909-4. [Google Scholar]
- Gupta, S.B.; Kalaria, K.R.; Vaghela, N.P.; Mukherjee, S.; Joshi, R.S.; Puthanveettil, S.E.; Shankaran, M.; Ekkundi, R.S. An overview of spacecraft charging research in india: Spacecraft plasma interaction experiments—SPIX-II. IEEE Trans. Plasma Sci. 2014, 42, 1072–1077. [Google Scholar] [CrossRef]
- Tafazoli, M. A Study of on-orbit spacecraft failures. Acta Astronaut. 2009, 64, 195–205. [Google Scholar] [CrossRef]
- Wang, J.; Li, Q.; Li, B.; Chen, C.; Liu, S.; Ji, H. Motion analysis of spherical metal particle in AC gas-insulated lines: Random effects and resistance of the SF6/N2 mixture. IEEE Trans. Dielect. Electr. Insul. 2016, 23, 2617–2625. [Google Scholar] [CrossRef]
- Khan, Y.; Sakai, K.; Okabe, S.; Suehiro, J.; Hara, M. Importance of the consideration of electrical gradient force in the deactivation of free conducting particle under dc voltage. In Proceedings of the IEEE/PES Transmission and Distribution Conference and Exhibition, Yokohama, Japan, 6–10 October 2002; pp. 904–908. [Google Scholar]
- Metwally, I.A.; A-Rahim, A.A. Dynamic analysis of motion of spherical metallic particles in non-uniform electric field. IEEE Trans. Dielect. Electr. Insul. 2002, 9, 282–293. [Google Scholar] [CrossRef]
- Yan, D.; Zhang, Z.; Gong, H.; Ya, Y. Effect of barbed tubular electrode corona discharge EHD flow on submicron particle collection in a wide-type ESP. J. Electrost. 2021, 109, 103545. [Google Scholar] [CrossRef]
- Nojima, K.; Zhang, X.; Sato, M.; Yasuoka, T.; Shiiki, M.; Takei, M.; Boggs, S.A. Forces affecting metallic particle motion in GIS. In Proceedings of the 2014 International Symposium on Electrical Insulating Materials, Niigata, Japan, 1–5 June 2014; pp. 132–135. [Google Scholar]
- Sakai, K.; Labrado Abella, D.; Khan, Y.; Suehiro, J.; Hara, M. Experimental studies of free conducting wire particle behavior between non-parallel plane electrodes with Ac voltages in air. IEEE Trans. Dielect. Electr. Insul. 2003, 10, 418–424. [Google Scholar] [CrossRef]
- Arif, S.; Branken, D.J.; Everson, R.C.; Neomagus, H.W.J.P.; Noras, M.A.; le Grange, L.A.; Arif, A. An experimentally validated computational model to predict the performance of a single-channel laboratory-scale electrostatic precipitator equipped with spiked and wire discharge electrodes. J. Electrost. 2021, 112, 103595. [Google Scholar] [CrossRef]
- Moradnouri, A.; Vakilian, M.; Hekmati, A.; Fardmanesh, M. HTS transformer’s partial discharges raised by floating particles and nitrogen bubbles. J. Supercond. Nov. Magn. 2020, 33, 3027–3034. [Google Scholar] [CrossRef]
- Drouin, D.; Couture, A.R.; Joly, D.; Tastet, X.; Aimez, V.; Gauvin, R. CASINO V2.42—A fast and easy-to-use modeling tool for scanning electron microscopy and microanalysis users. Scanning 2007, 29, 92–101. [Google Scholar] [CrossRef]
- Carriere, P.R.; Yue, S. Energy absorption during pulsed electron beam spot melting of 304 stainless steel: Monte-Carlo simulations and in-situ temperature measurements. Vacuum 2017, 142, 114–122. [Google Scholar] [CrossRef]
- Arat, K.T.; Hagen, C.W. Model sensitivity analysis of Monte-Carlo based SEM simulations. Results Phys. 2020, 19, 103545. [Google Scholar] [CrossRef]
- Hovington, P.; Drouin, D.; Gauvin, R. CASINO: A new Monte Carlo code in C language for electron beam interaction—Part I: Description of the program. Scanning 2006, 19, 1–14. [Google Scholar] [CrossRef]
- Pan, S.; Min, D.; Wang, X.; Hou, X.; Wang, L.; Li, S. Effect of electron irradiation and operating voltage on the deep dielectric charging characteristics of polyimide. IEEE Trans. Nucl. Sci. 2019, 66, 549–556. [Google Scholar] [CrossRef]
- Wu, Y.; Castle, G.S.P.; Inculet, I.I.; Petigny, S.; Swei, G. Induction charge on freely levitating particles. Powder Technol. 2003, 135, 59–64. [Google Scholar] [CrossRef]
- Li, J.; Lu, H.; Xu, Z.; Zhou, Y. A model for computing the trajectories of the conducting particles from waste printed circuit boards in corona electrostatic separators. J. Hazard. Mater. 2008, 151, 52–57. [Google Scholar] [CrossRef]
- Zalevsky, Z.; Rudnitsky, A.; Haran, A.; Zentner, A.; David, D.; Noter, Y. Irradiation test results of components selected from novel optical rotman lens configuration for space systems. Aerosp. Sci. Technol. 2011, 15, 261–268. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, T.; Ma, Z.; Jiang, J.; Chen, Q. Analysis of Electric Transmission Failure Induced by Electrostatic Migration and Deposition of Abrasive Dusts in SADA. Aerospace 2023, 10, 243. https://doi.org/10.3390/aerospace10030243
Wang T, Ma Z, Jiang J, Chen Q. Analysis of Electric Transmission Failure Induced by Electrostatic Migration and Deposition of Abrasive Dusts in SADA. Aerospace. 2023; 10(3):243. https://doi.org/10.3390/aerospace10030243
Chicago/Turabian StyleWang, Tongben, Zhifei Ma, Jingming Jiang, and Qi Chen. 2023. "Analysis of Electric Transmission Failure Induced by Electrostatic Migration and Deposition of Abrasive Dusts in SADA" Aerospace 10, no. 3: 243. https://doi.org/10.3390/aerospace10030243
APA StyleWang, T., Ma, Z., Jiang, J., & Chen, Q. (2023). Analysis of Electric Transmission Failure Induced by Electrostatic Migration and Deposition of Abrasive Dusts in SADA. Aerospace, 10(3), 243. https://doi.org/10.3390/aerospace10030243





