Scale Effect Assessment of Innovative 3D-Printed Honeycomb under Quasi-Static Compression
Abstract
1. Introduction
1.1. Lattice Structures
1.2. Honeycomb Lattices
1.3. Additive Manufacturing of Lattices
1.4. Scale Effect of 3D-Printed Lattices
2. Materials and Methods
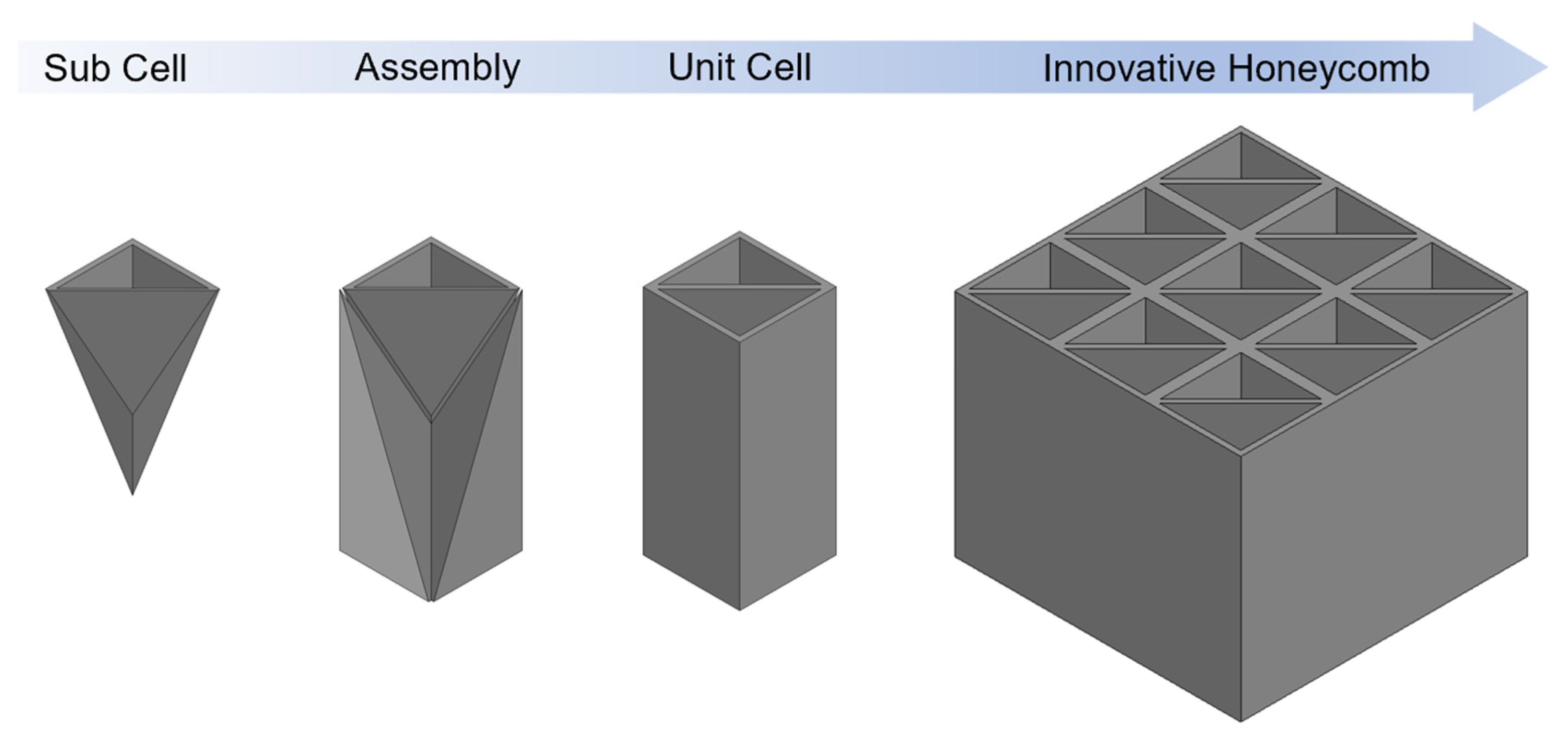
2.1. Cell Geometry Design
- For the novel geometry, it was important to have at least one cell surrounded by the other cells on all sides, to appreciate the effect of the interaction between different cells. The cell number chosen was 9 (3 × 3), which corresponds to the minimum number of cells that satisfies the condition mentioned above.
- For the standard geometry, the total number of cells is equivalent to 30, i.e., the number of cells arranged within a square that most closely approximates the number of sub-cells of the novel geometry sample (i.e., 9 × 4 = 36).
- The numerical values of the dimensional parameters of the two different samples, in both regular scale and large (double) scale, are detailed below and in Table 1.
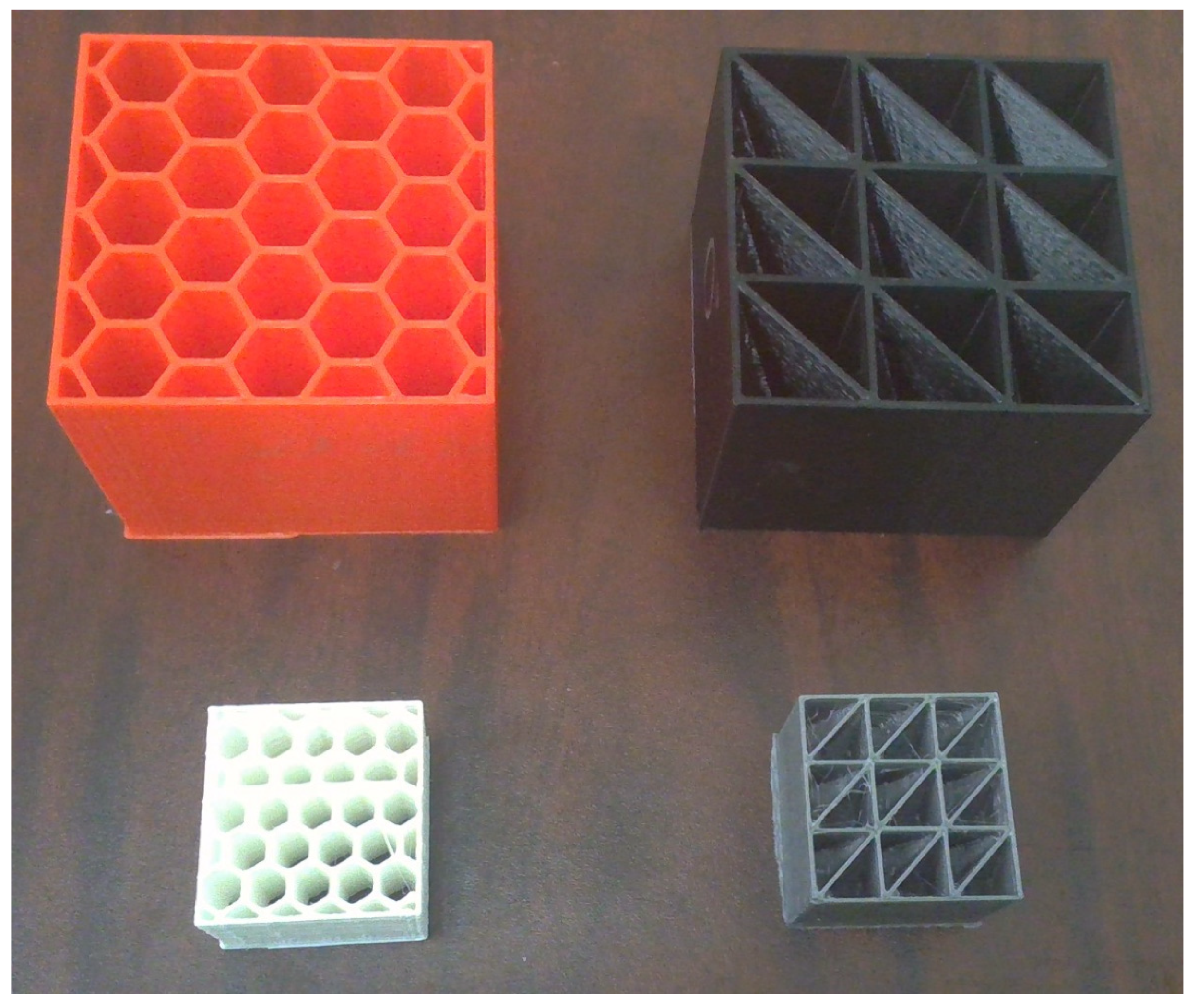
- In the regular scale, the external longitudinal dimensions were 38.1 mm × 38.1 mm × 31.8 mm, with wall thicknesses of 0.75 mm for the novel honeycomb and 0.72 mm for the standard honeycomb. The cell walls were manufactured with two filament layers and 100% infill.
- The larger scale (“double scale”) had the external longitudinal dimensions scaled up by 200%, i.e., 76.2 mm × 76.2 mm × 63.5 mm with wall thickness of 1.50 mm for the novel honeycomb and 1.44 mm for the standard honeycomb. The cell walls were manufactured with four filament layers and 100%-infill.
2.2. Materials and Fabrication
2.3. Experimental Setup
2.4. Finite Element Modeling
3. Results
- Hexagonal, regular scale, under axial load;
- Novel, regular scale, under axial load;
- Hexagonal, regular scale, under lateral load;
- Novel, regular scale, under lateral load;
- Hexagonal, large scale, under lateral load;
- Novel, large scale, under lateral load.
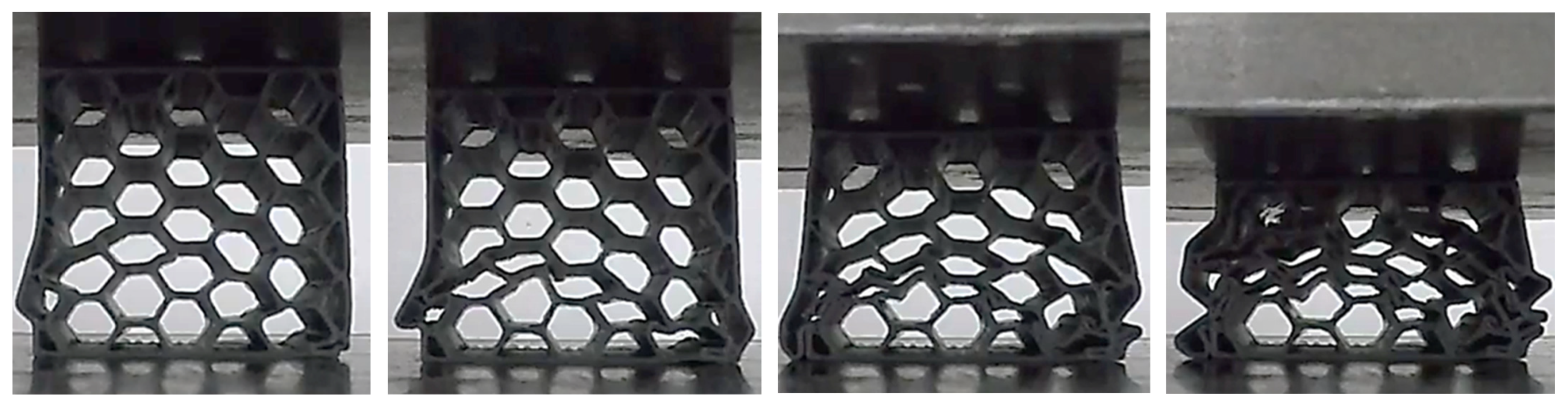
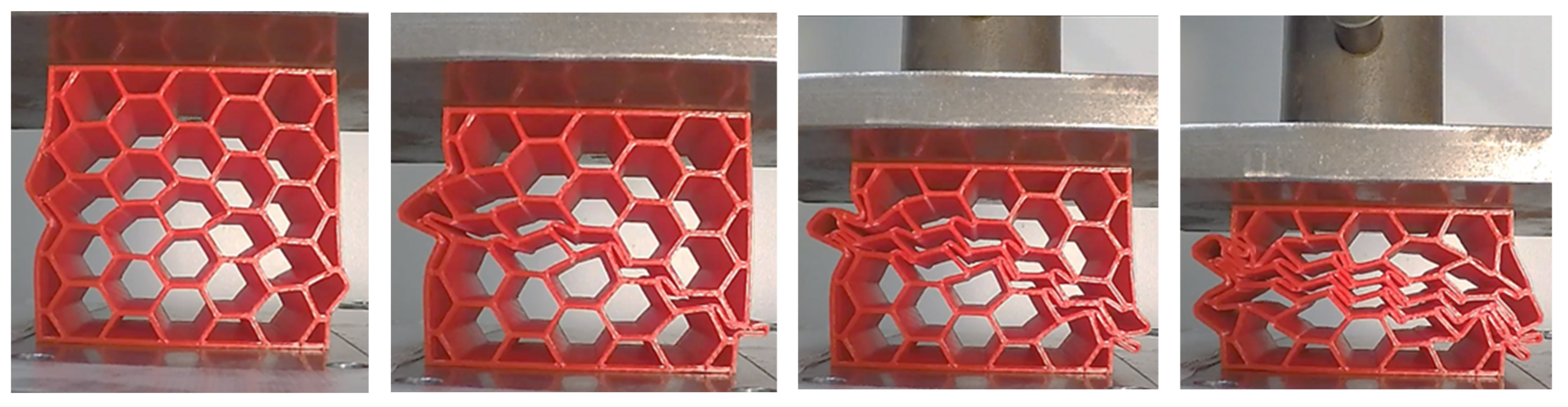
3.1. Regular-Scale Specimens with Novel Geometry
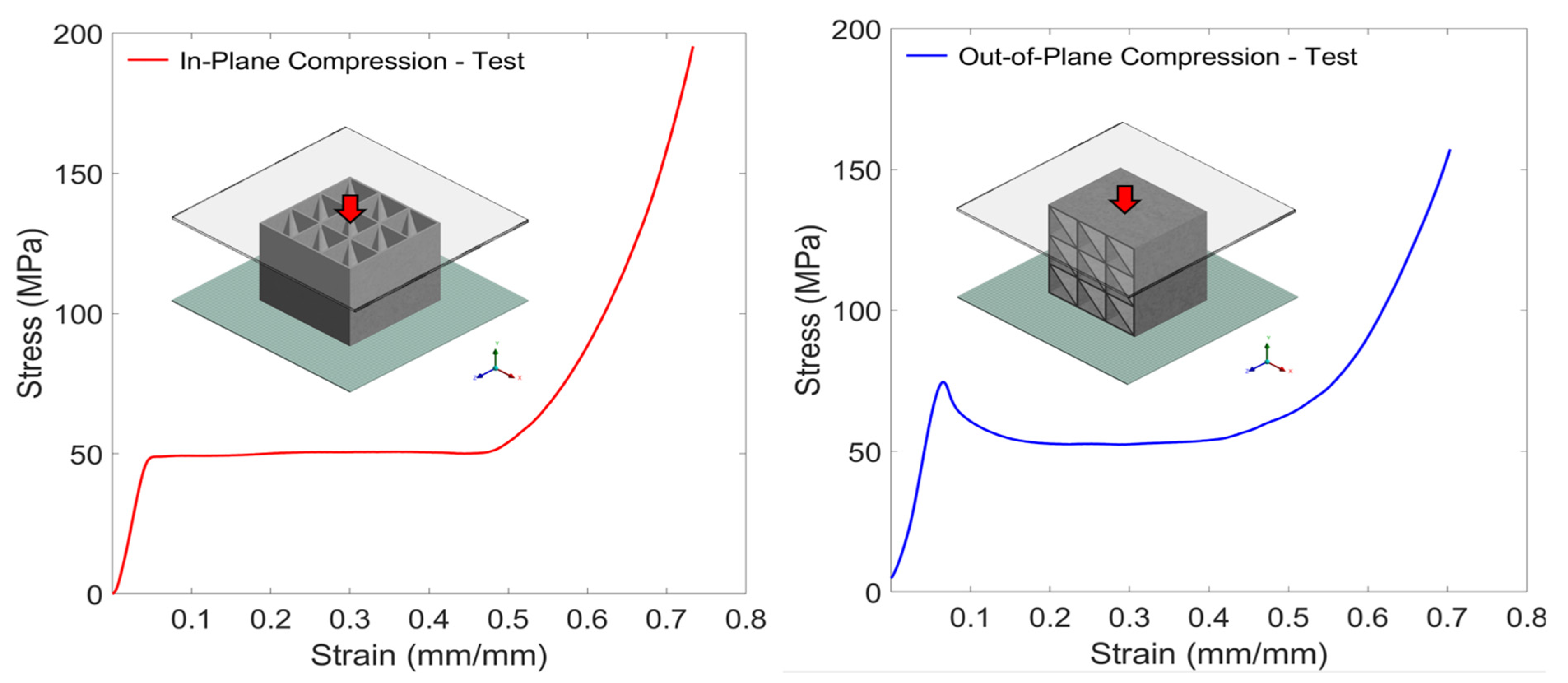
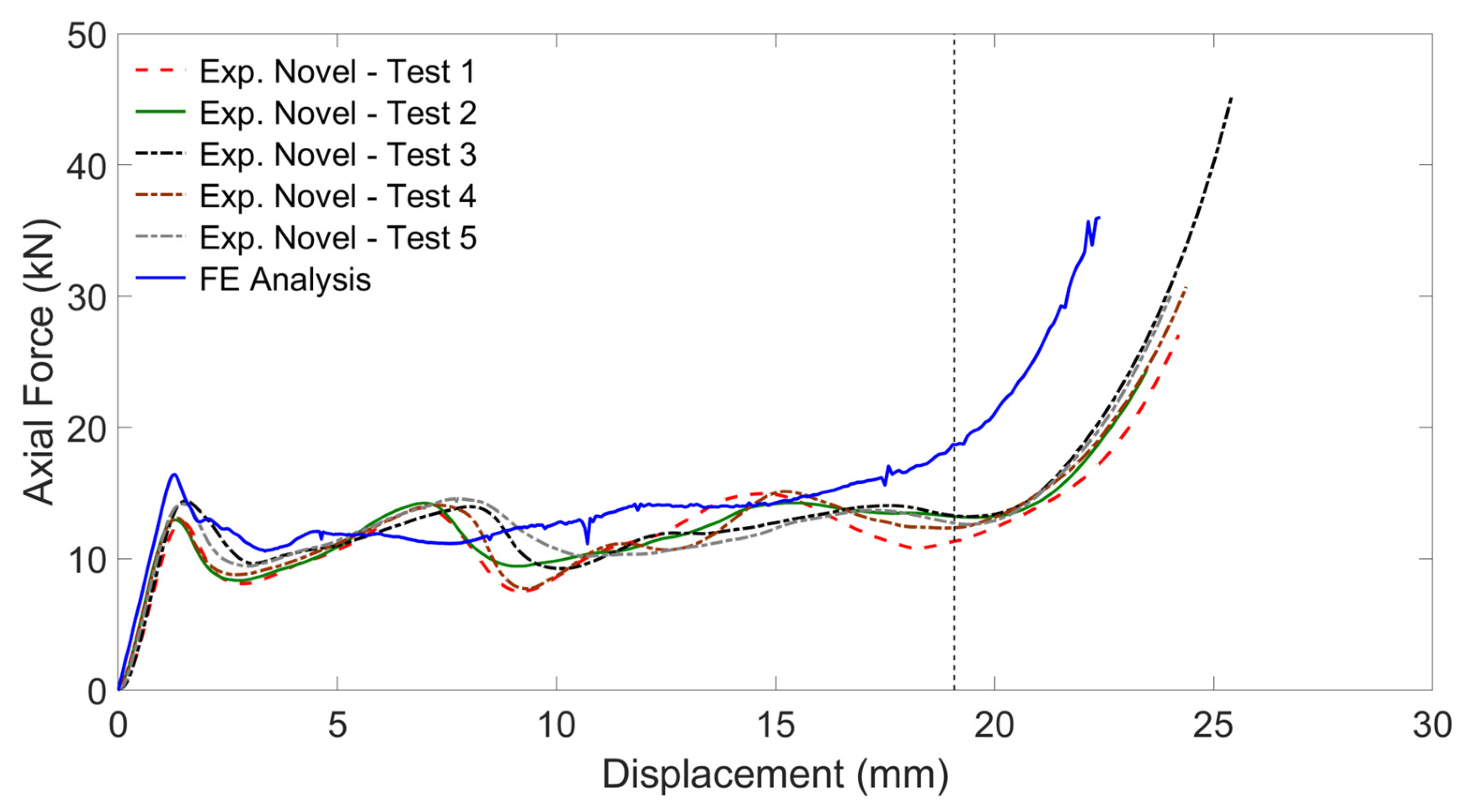
3.1.1. Under Axial Load
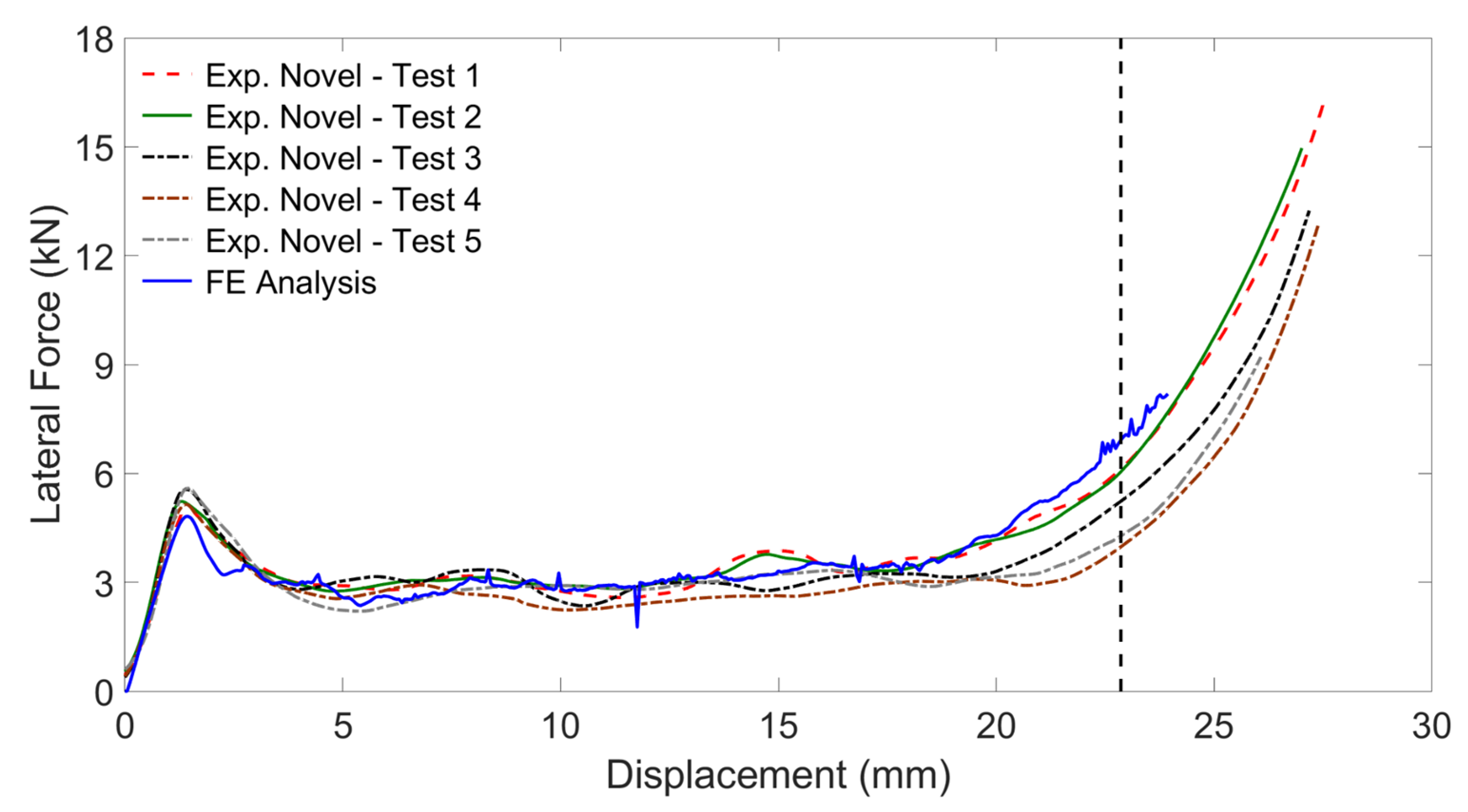
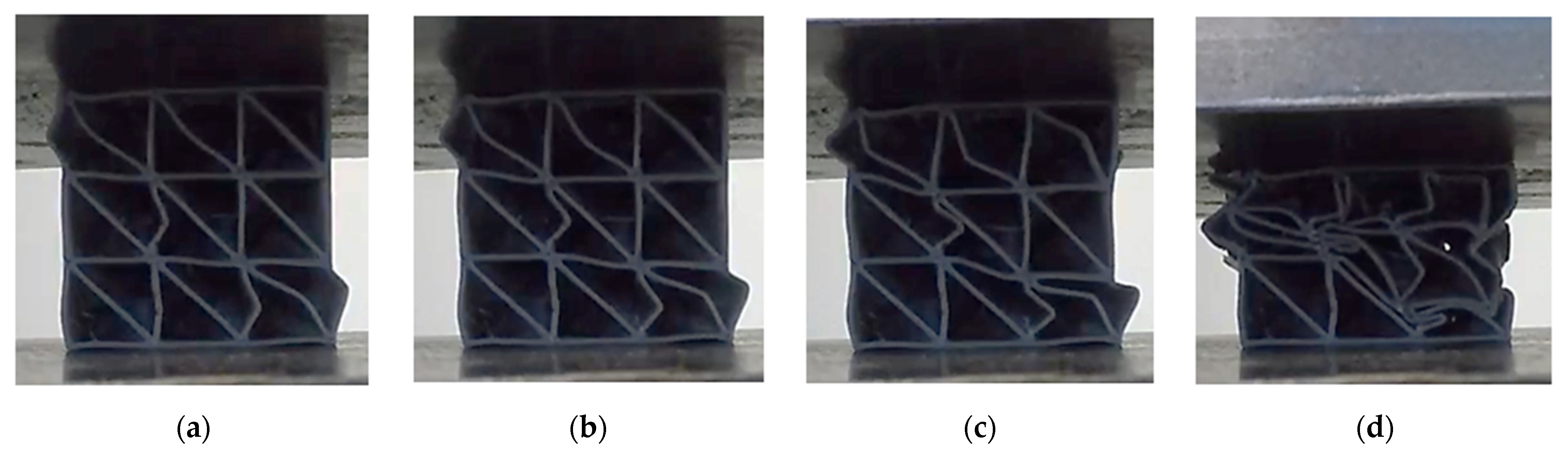
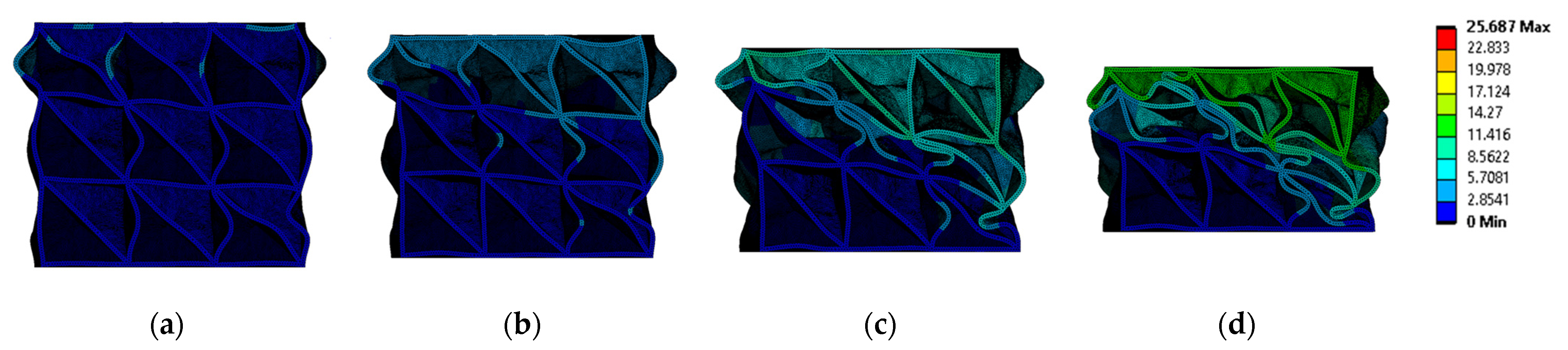
3.1.2. Under Lateral Load
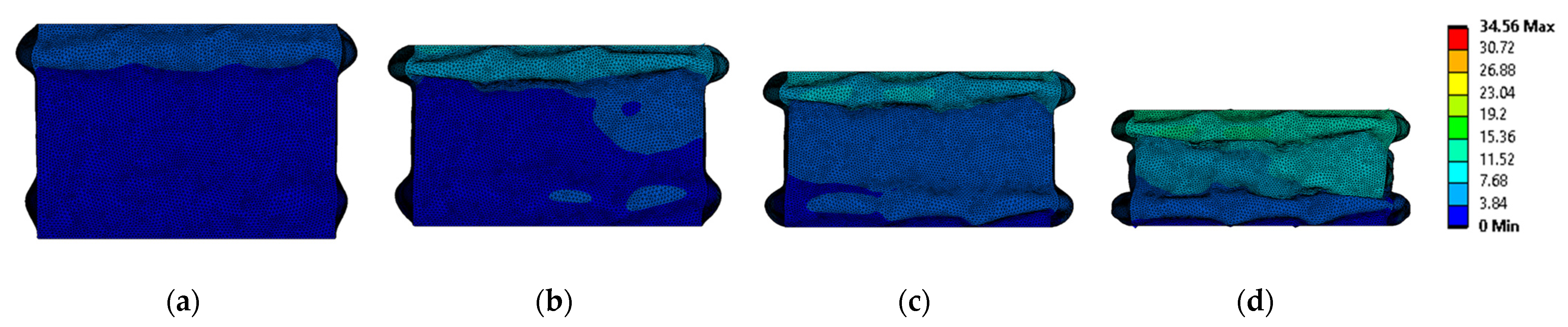
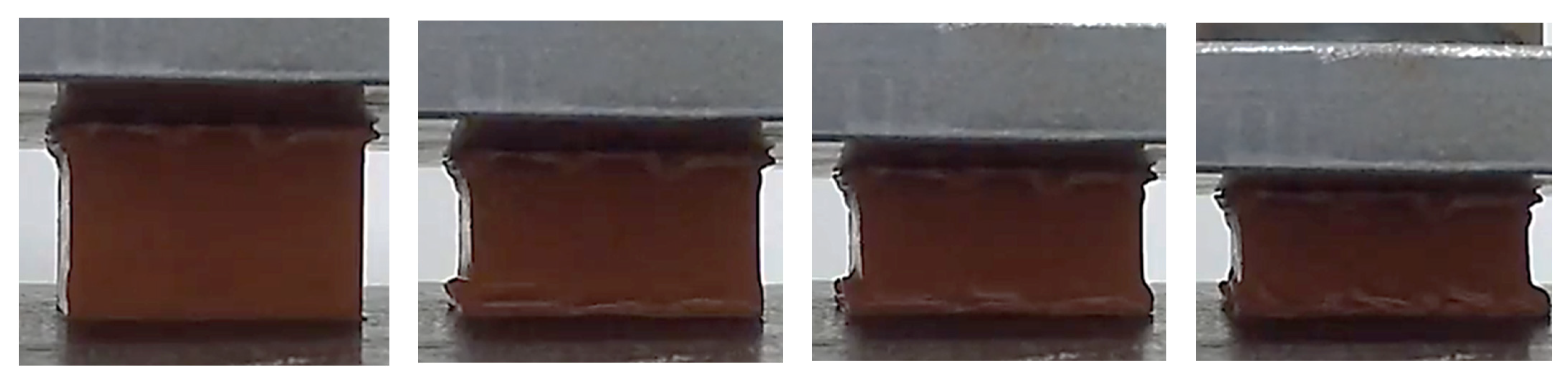
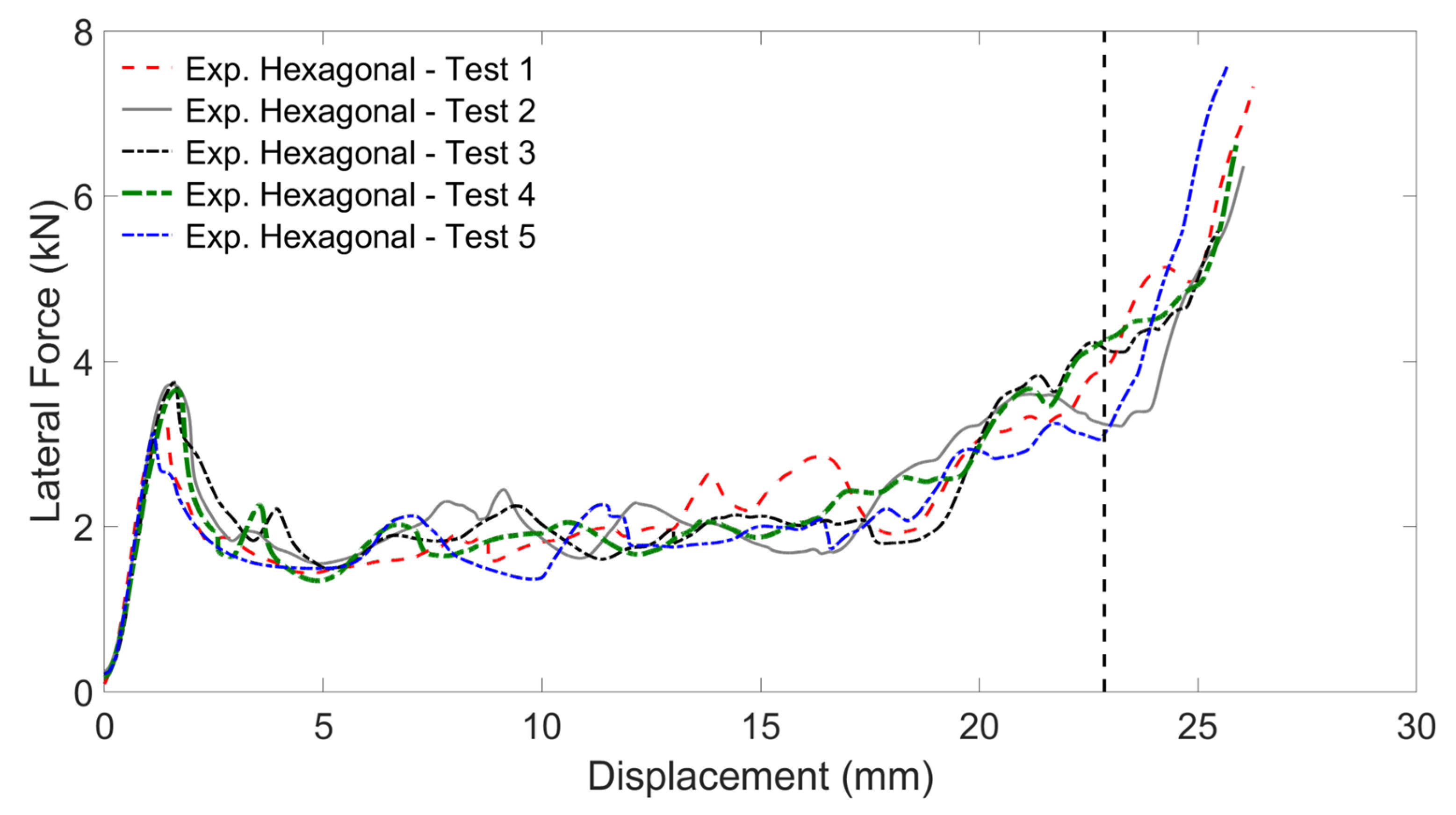
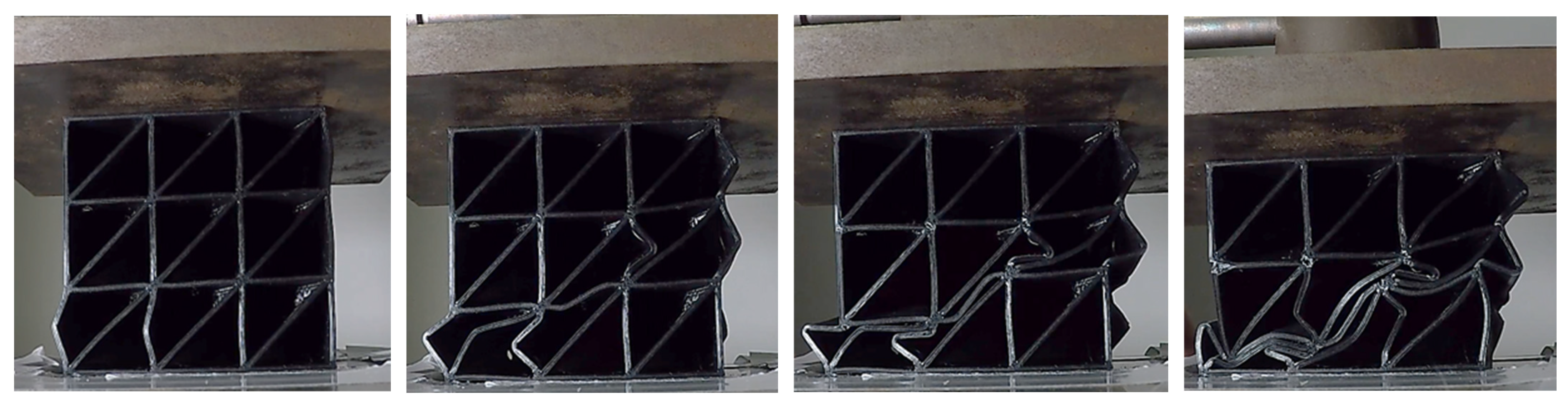
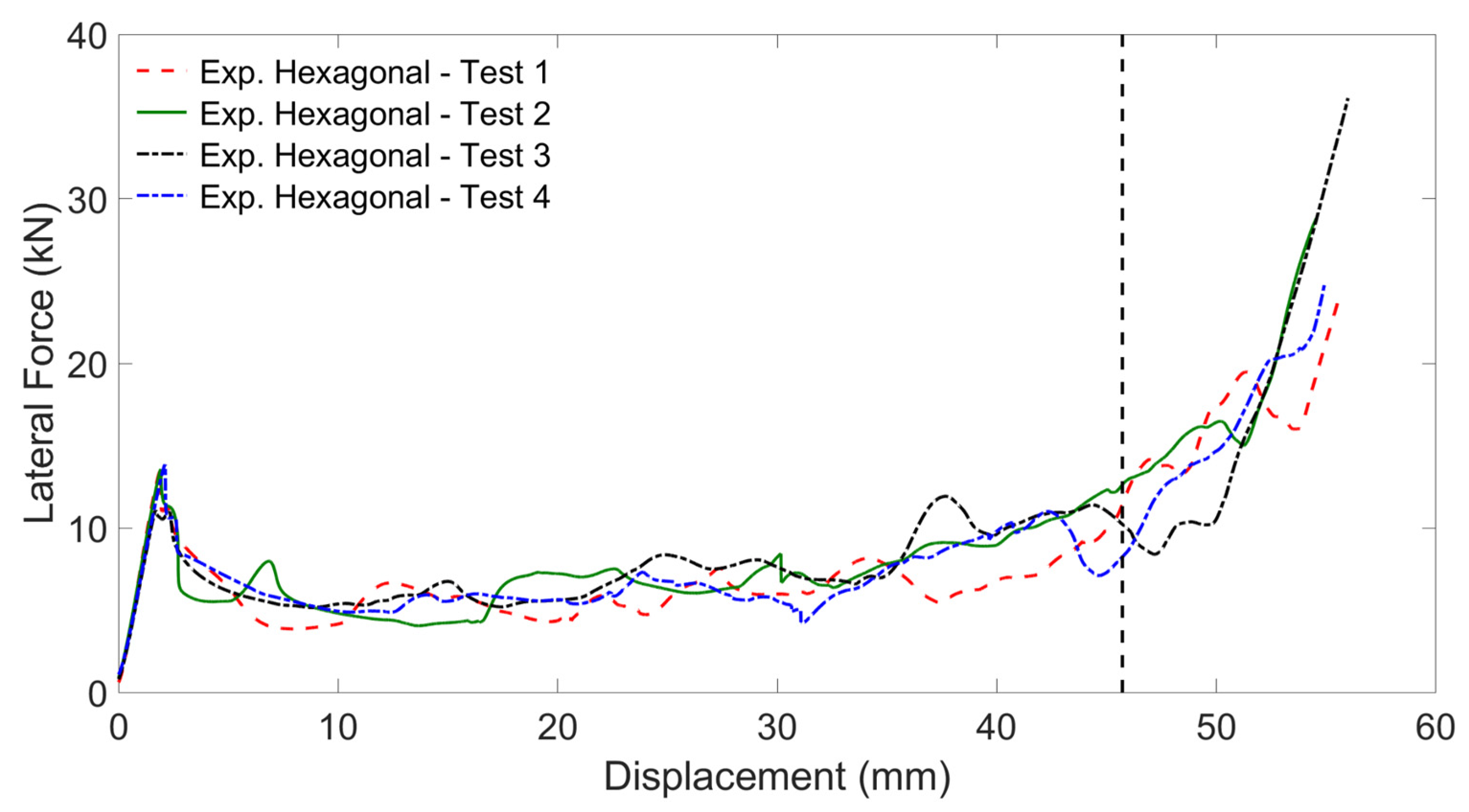
3.2. Regular-Scale Specimens with Hexagonal Geometry
3.2.1. Under Axial Load
3.2.2. Under Lateral Load
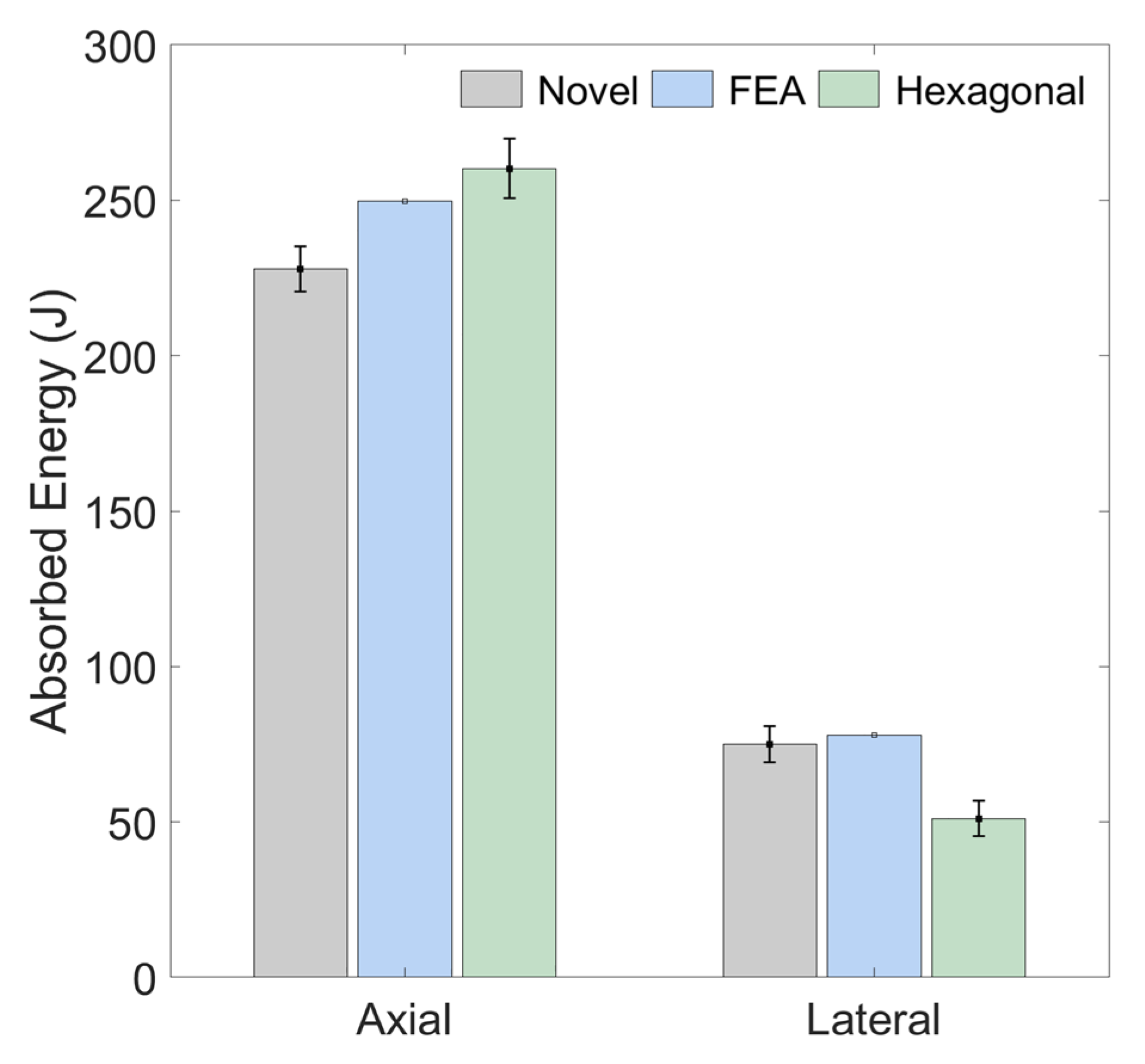
3.3. Overview of the Sample Geometry Comparison at Regular Scale
3.4. Large-Scale Specimens under Lateral Load
3.4.1. Large-Scale, Novel Geometry under Lateral Load
3.4.2. Large-Scale, Hexagonal Geometry under Lateral Load
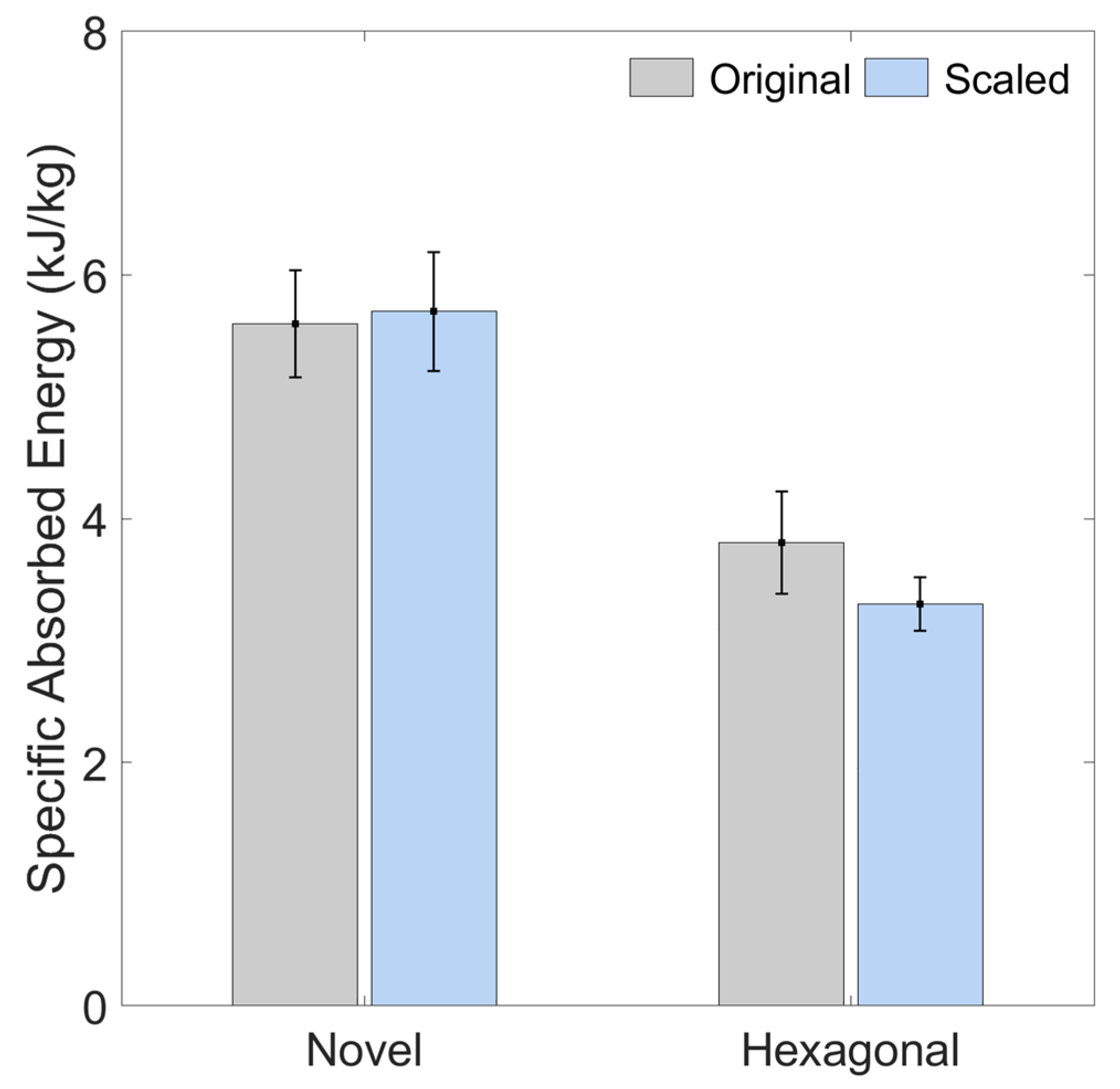
3.5. Scale Effect
4. Discussion
- The hexagonal geometry experiences a reduction, in terms of specific energy absorption per unit mass, equal to 12.24%.
- The novel geometry experiences an increase, in terms of specific energy absorption per unit mass, equal to 2.12%.
5. Conclusions
6. Patents
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Seilsepour, H.; Zarastvand, M.; Talebitooti, R. Acoustic insulation characteristics of sandwich composite shell systems with double curvature: The effect of nature of viscoelastic core. J. Vib. Control 2022, 29, 1076–1090. [Google Scholar] [CrossRef]
- Zarastvand, M.; Asadijafari, M.; Talebitooti, R. Acoustic wave transmission characteristics of stiffened composite shell systems with double curvature. Compos. Struct. 2022, 292, 115688. [Google Scholar] [CrossRef]
- Pan, C.; Han, Y.; Lu, J. Design and Optimization of Lattice Structures: A Review. Appl. Sci. 2020, 10, 6374. [Google Scholar] [CrossRef]
- Guide to Lattice Structures in Additive Manufacturing. nTopology, 28 April 2022. Available online: https://ntopology.com/blog/guide-to-lattice-structures-in-additive-manufacturing/ (accessed on 6 June 2022).
- Maskery, I.; Sturm, L.; Aremu, A.O.; Panesar, A.; Williams, C.B.; Tuck, C.J.; Wildman, R.D.; Ashcroft, I.; Hague, R.J.M. Insights into the mechanical properties of several triply periodic minimal surface lattice structures made by polymer additive manufacturing. Polymer 2018, 152, 62–71. [Google Scholar] [CrossRef]
- Qi, C.; Jiang, F.; Yang, S. Advanced honeycomb designs for improving mechanical properties: A review. Compos. Part B Eng. 2021, 227, 109393. [Google Scholar] [CrossRef]
- Compton, B.G.; Lewis, J.A. 3D-Printing of Lightweight Cellular Composites. Adv. Mater. 2014, 26, 5930–5935. [Google Scholar] [CrossRef] [PubMed]
- Hales, T.C. The Honeycomb Conjecture. Discret. Comput. Geom. 2001, 25, 1–22. [Google Scholar] [CrossRef]
- Bhate, D.; Penick, C.A.; Ferry, L.A.; Lee, C. Classification and Selection of Cellular Materials in Mechanical Design: Engineering and Biomimetic Approaches. Designs 2019, 3, 19. [Google Scholar] [CrossRef]
- Yin, H.; Zhang, W.; Zhu, L.; Meng, F.; Liu, J.; Wen, G. Review on lattice structures for energy absorption properties. Compos. Struct. 2023, 304, 116397. [Google Scholar] [CrossRef]
- Zeng, C.; Liu, L.; Bian, W.; Leng, J.; Liu, Y. Compression behavior and energy absorption of 3D printed continuous fiber reinforced composite honeycomb structures with shape memory effects. Addit. Manuf. 2021, 38, 101842. [Google Scholar] [CrossRef]
- Wickramasinghe, S.; Do, T.; Tran, P. FDM-Based 3D Printing of Polymer and Associated Composite: A Review on Mechanical Properties, Defects and Treatments. Polymers 2020, 12, 1529. [Google Scholar] [CrossRef] [PubMed]
- Hull, C.W.; Gabriel, S. Apparatus for Production of Three-Dimensional Objects by Stereolithography. U.S. Patent 4,575,330, 11 March 1986. [Google Scholar]
- Gwamuri, J.; Poliskey, J.E.; Pearce, J.M. Open Source 3-D Printers: An Appropriate Technology for Developing Communities. In Proceedings of the 7th International Conference on Appropriate Technology, Victoria Falls, Zimbabwe, 23–26 November 2016; Available online: https://hal.archives-ouvertes.fr/hal-02113460 (accessed on 17 July 2022).
- Flora, F.; Pinto, F.; Meo, M. Manufacturing and characterisation of a new thermal pre-stressed carbon fibre-reinforced lattice core for sandwich panels. J. Compos. Mater. 2022, 56, 1233–1254. [Google Scholar] [CrossRef]
- Liu, S. Harnessing Elastic Instabilities for Energy Transduction. Ph.D. Thesis, Michigan State University, East Lansing, MI, USA, 2019. [Google Scholar]
- Menegozzo, M.; Cecchini, A.; Just-Agosto, F.A.; Acevedo, D.S.; Velez, O.J.F.; Acevedo-Figueroa, I.; Ruiz, J.D.J. A 3D-Printed Honeycomb Cell Geometry Design with Enhanced Energy Absorption under Axial and Lateral Quasi-Static Compression Loads. Appl. Mech. 2022, 3, 296–312. [Google Scholar] [CrossRef]
- Savini, A.; Savini, G.G. A short history of 3D printing, a technological revolution just started. In Proceedings of the 2015 ICOHTEC/IEEE International History of High-Technologies and their Socio-Cultural Contexts Conference (HISTELCON), Tel Aviv, Israel, 18–19 August 2015; pp. 1–8. [Google Scholar] [CrossRef]
- Horvath, J. Mastering 3D Printing; Apress: Berkeley, CA, USA, 2014. [Google Scholar] [CrossRef]
- Winick, E. 3D Printing’s 30 Year History and Why It’s Popular Now. Medium, 26 January 2017. Available online: https://medium.com/@erinwinick/3d-printings-30-year-history-and-why-it-is-popular-now-5200ab21a7ed (accessed on 1 July 2022).
- All3DP. The Official History of the RepRap Project. All3DP, 8 April 2016. Available online: https://all3dp.com/history-of-the-reprap-project/ (accessed on 1 July 2022).
- DE Editors. Sub-$10,000 3D Printers Near Market. Digital Engineering, 27 September 2007. Available online: https://www.digitalengineering247.com/article/sub-10-000-3d-printers-near-market (accessed on 1 July 2022).
- Timeline of the 3D Printing History—ASME. Available online: https://www.asme.org/topics-resources/content/infographic-the-history-of-3d-printing (accessed on 1 July 2022).
- Kuncius, T.; Rimašauskas, M.; Rimašauskienė, R. Interlayer Adhesion Analysis of 3D-Printed Continuous Carbon Fibre-Reinforced Composites. Polymers 2021, 13, 1653. [Google Scholar] [CrossRef] [PubMed]
- Xu, Z.; Medori, E.; Sarasini, F.; Razavi, S.M.J. Quasi-static behavior of 3D printed lattice structures of various scales. Procedia Struct. Integr. 2021, 33, 578–585. [Google Scholar] [CrossRef]
- Aziz, A.R.; Zhou, J.; Thorne, D.; Cantwell, W.J. Geometrical Scaling Effects in the Mechanical Properties of 3D-Printed Body-Centered Cubic (BCC) Lattice Structures. Polymers 2021, 13, 3967. [Google Scholar] [CrossRef] [PubMed]
- Mueller, J.; Shea, K. Buckling, build orientation, and scaling effects in 3D printed lattices. Mater. Today Commun. 2018, 17, 69–75. [Google Scholar] [CrossRef]
- Andrew, J.J.; Ubaid, J.; Hafeez, F.; Schiffer, A.; Kumar, S. Impact performance enhancement of honeycombs through additive manufacturing-enabled geometrical tailoring. Int. J. Impact Eng. 2019, 134, 103360. [Google Scholar] [CrossRef]
- Nazir, A.; Gohar, A.; Lin, S.-C.; Jeng, J.-Y. Flexural Properties of Periodic Lattice Structured Lightweight Cantilever Beams Fabricated Using Additive Manufacturing: Experimental and Finite Element Methods. 3D Print. Addit. Manuf. 2022, 3dp.2022.0017. [Google Scholar] [CrossRef]
- Overture 3D. Available online: https://overture3d.com/ (accessed on 21 December 2022).
- Overture PLA 3D Printing Filament 1.75mm. Overture 3D. Available online: https://overture3d.com/products/overturepla (accessed on 21 December 2022).







| Dimensions | L (mm) | W (mm) | H (mm) | t (mm) | D (mm) | d (mm) | Mass (g) |
|---|---|---|---|---|---|---|---|
| Hexagonal | 38.1 | 38.1 | 31.8 | 0.72 | - | 7.6 | 13.3 |
| Novel | 38.1 | 38.1 | 31.8 | 0.75 | 12.7 | - | 13.3 |
| Hexagonal (Scaled) | 76.2 | 76.2 | 63.5 | 1.44 | - | 15.2 | 106.4 |
| Novel (Scaled) | 76.2 | 76.2 | 63.5 | 1.50 | 25.4 | - | 106.4 |
| Parameter | Setting | Parameter | Setting |
|---|---|---|---|
| Analysis type | Explicit-dynamic | Density | 1190 kg/m3 |
| Material model | Multilinear isotropic hardening | Contact type (axial/lateral) | Bonded/Frictional |
| Elastic modulus | 1.25 GPa | Hourglass control | Flanagan-Belytschko |
| Yield strength (axial/lateral) | 46/60 MPa | Stiffness coefficient | 0.05 |
| Poisson’s ratio | 0.3 | Viscous coefficient | 0.1 |
| Honeycomb Geometry | Load Direction | Energy Absorption (J) | Max. Elastic Reaction Force (kN) | Specific Energy Absorption (kJ/kg) |
|---|---|---|---|---|
| Novel | Axial | 227.9 (7.2) | 14.3 (0.5) | 17.1 (0.5) |
| Hexagonal | Axial | 260.2 (9.5) | 18.0 (0.7) | 19.5 (0.7) |
| Novel | Lateral | 75.0 (5.9) | 5.8 (0.6) | 5.6 (0.4) |
| Hexagonal | Lateral | 51.1 (5.6) | 4.3 (0.7) | 3.8 (0.4) |
| Honeycomb Geometry | Load Direction | Energy Absorption (J) | Max. Elastic Reaction Force (kN) | Specific Energy Absorption (kJ/kg) |
|---|---|---|---|---|
| Novel | Lateral | 75.0 (5.9) | 5.8 (0.6) | 5.6 (0.4) |
| Hexagonal | Lateral | 51.1 (5.6) | 4.3 (0.7) | 3.8 (0.4) |
| Novel (Scaled) | Lateral | 613.8 (52.2) | 22.7 (1.2) | 5.77 (0.4) |
| Hexagonal (Scaled) | Lateral | 358.0 (23.4) | 14.9 (2.5) | 3.37 (0.2) |
| Honeycomb Geometry. | Load Direction | Energy Absorption (J) | Max. Elastic Reaction Force (kN) | Specific Energy Absorption (kJ/kg) |
|---|---|---|---|---|
| Novel | Axial | 227.9 (7.2) | 14.3 (0.5) | 17.1 (0.5) |
| Hexagonal | Axial | 260.2 (9.5) | 18.0 (0.7) | 19.5 (0.7) |
| Novel | Lateral | 75.0 (5.9) | 5.8 (0.6) | 5.6 (0.4) |
| Hexagonal | Lateral | 51.1 (5.6) | 4.3 (0.7) | 3.8 (0.4) |
| Novel (Scaled) | Lateral | 613.8 (52.2) | 22.7 (1.2) | 5.77 (0.4) |
| Hexagonal (Scaled) | Lateral | 358.0 (23.4) | 14.9 (2.5) | 3.37 (0.2) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Menegozzo, M.; Cecchini, A.; Ogle, R.C.; Vaidya, U.K.; Acevedo-Figueroa, I.; Torres-Hernández, J.A. Scale Effect Assessment of Innovative 3D-Printed Honeycomb under Quasi-Static Compression. Aerospace 2023, 10, 242. https://doi.org/10.3390/aerospace10030242
Menegozzo M, Cecchini A, Ogle RC, Vaidya UK, Acevedo-Figueroa I, Torres-Hernández JA. Scale Effect Assessment of Innovative 3D-Printed Honeycomb under Quasi-Static Compression. Aerospace. 2023; 10(3):242. https://doi.org/10.3390/aerospace10030242
Chicago/Turabian StyleMenegozzo, Marco, Andrés Cecchini, Ryan Christian Ogle, Uday Kumar Vaidya, Isaac Acevedo-Figueroa, and Jaine A. Torres-Hernández. 2023. "Scale Effect Assessment of Innovative 3D-Printed Honeycomb under Quasi-Static Compression" Aerospace 10, no. 3: 242. https://doi.org/10.3390/aerospace10030242
APA StyleMenegozzo, M., Cecchini, A., Ogle, R. C., Vaidya, U. K., Acevedo-Figueroa, I., & Torres-Hernández, J. A. (2023). Scale Effect Assessment of Innovative 3D-Printed Honeycomb under Quasi-Static Compression. Aerospace, 10(3), 242. https://doi.org/10.3390/aerospace10030242






