Investigations of the Atomization Characteristics and Mechanisms of Liquid Jets in Supersonic Crossflow
Abstract
:1. Introduction
2. Model and Numerical Methods
2.1. Model
2.2. Numerical Methods
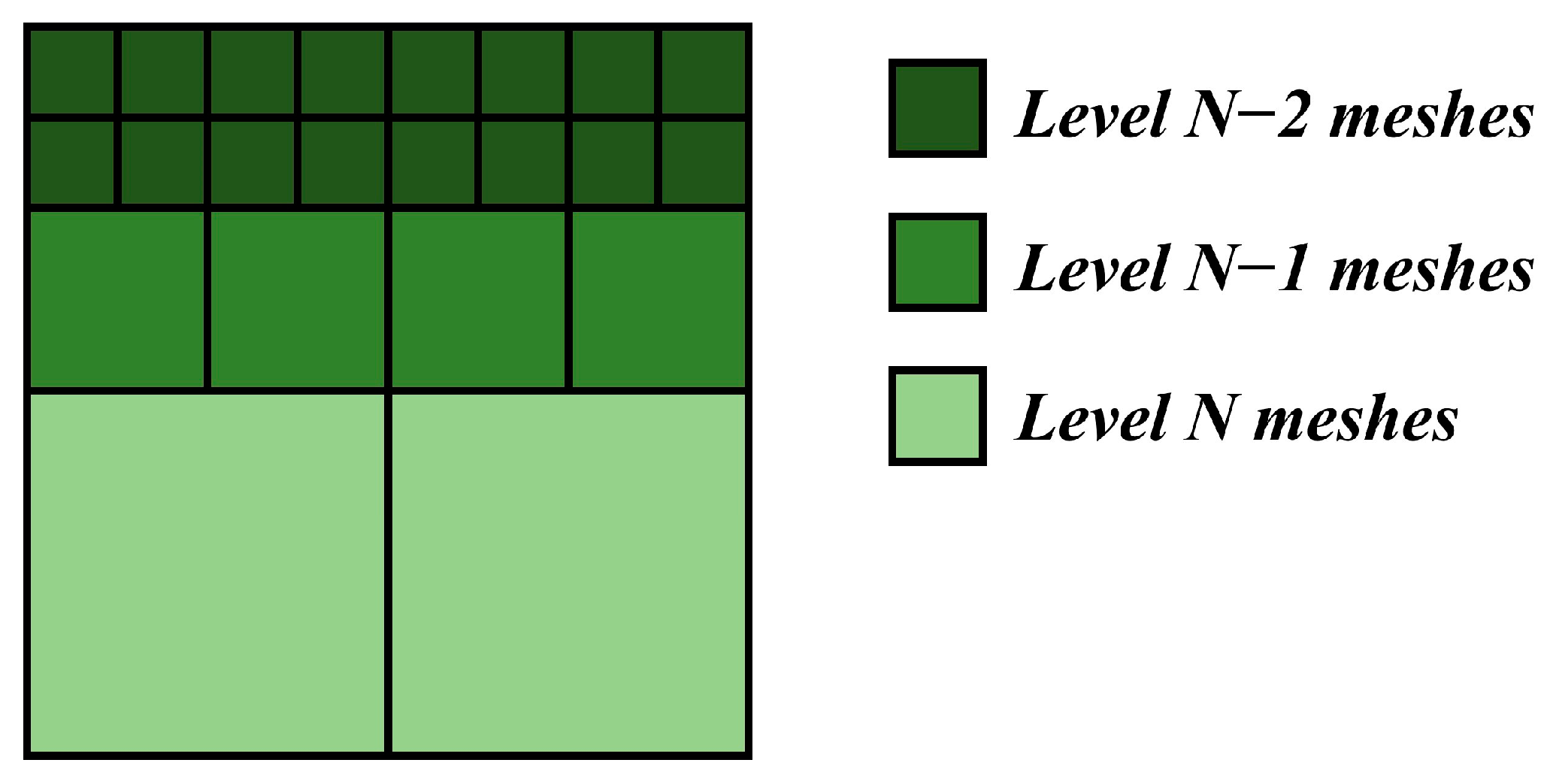
2.3. Verification of Mesh Independence and Accuracy
3. Results and Discussion
3.1. Breakup Process and Atomization Mechanism of Supersonic LJICs
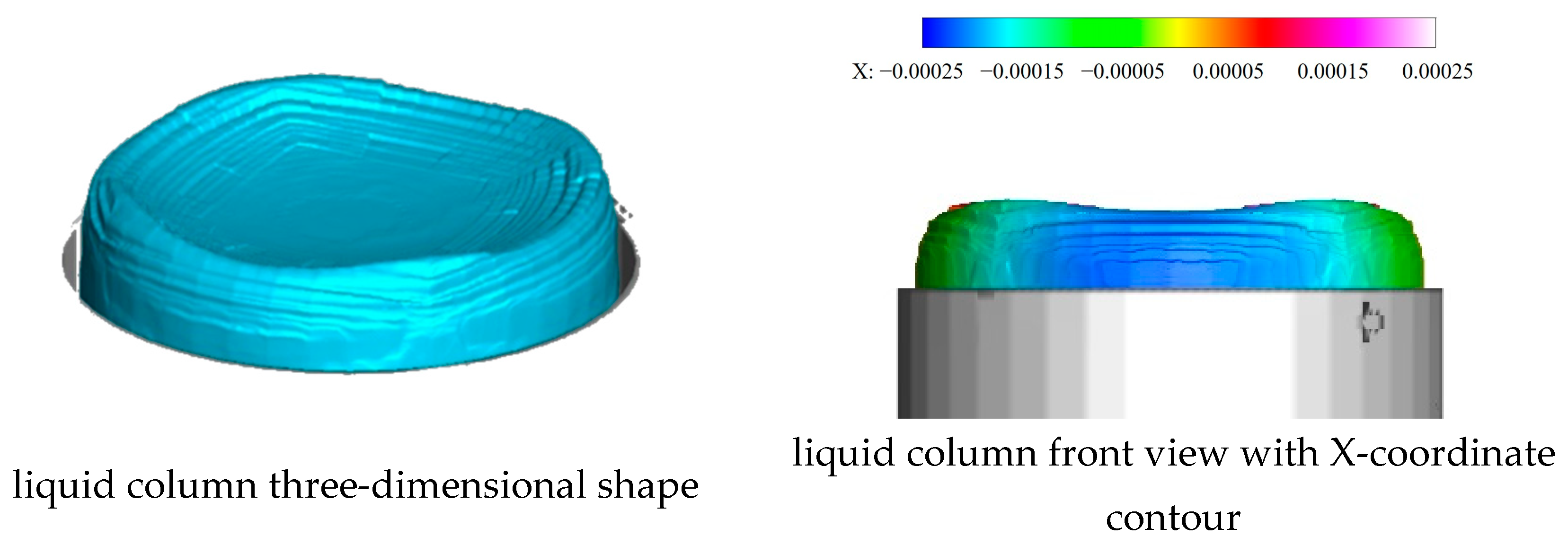
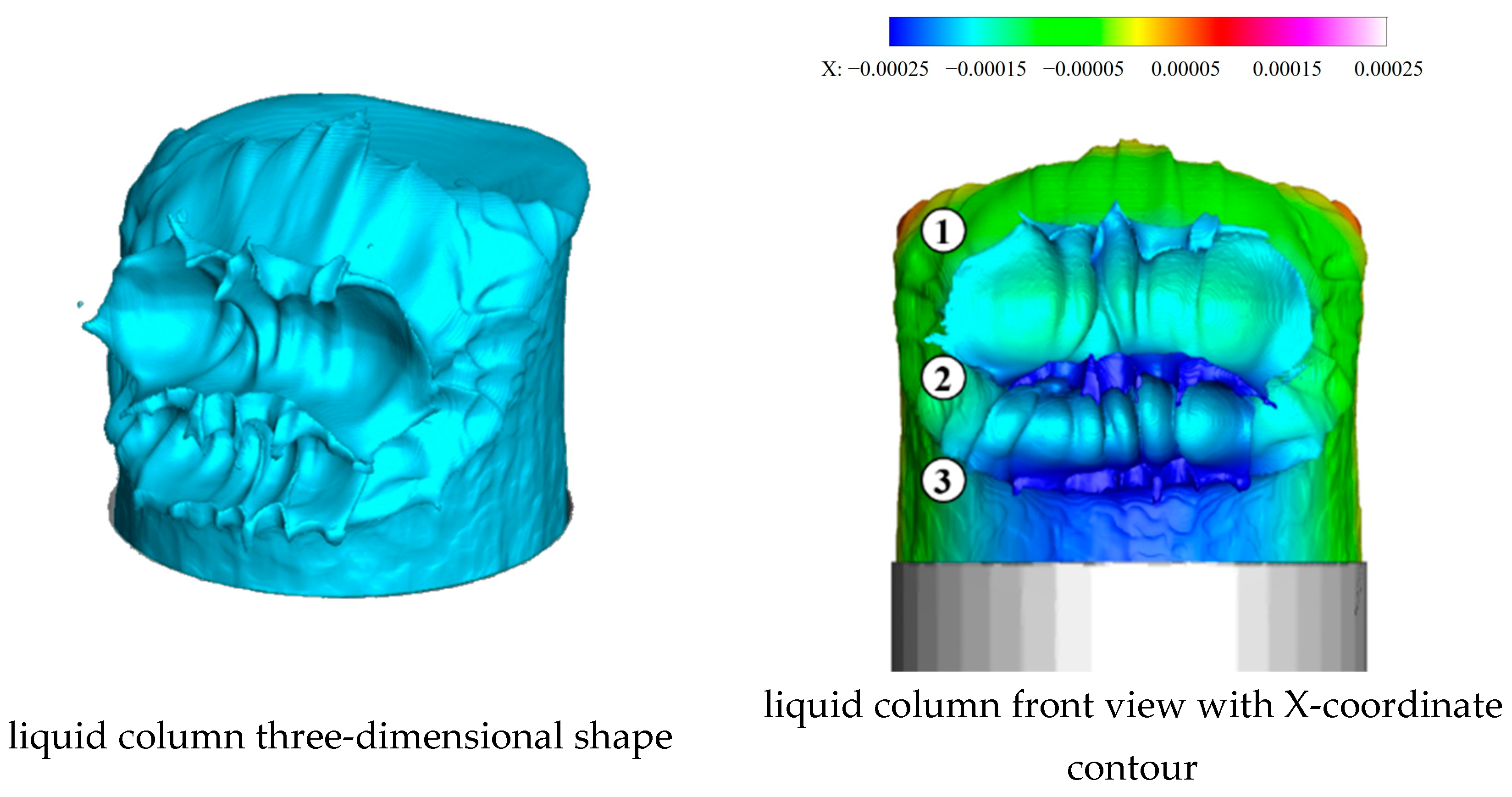
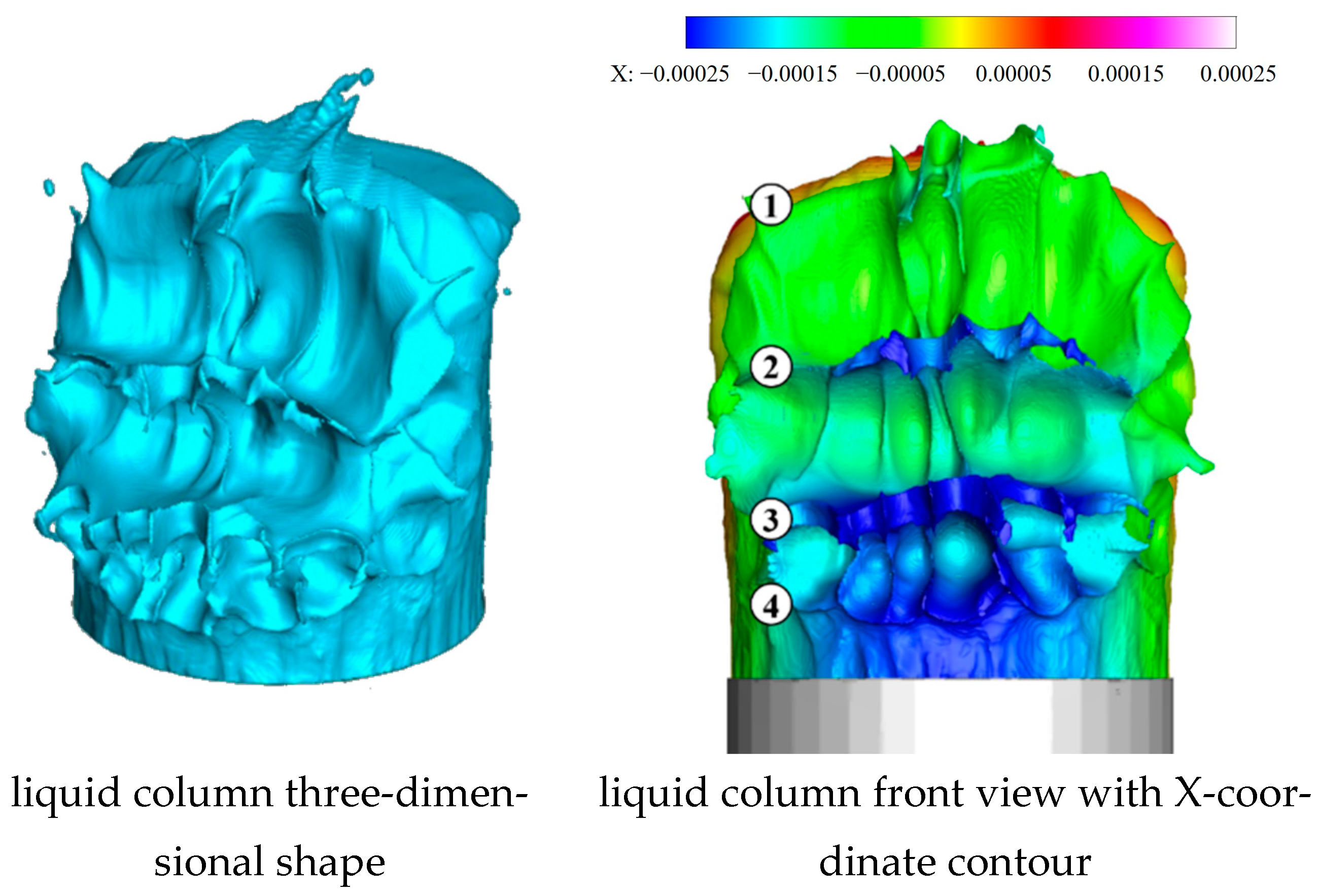
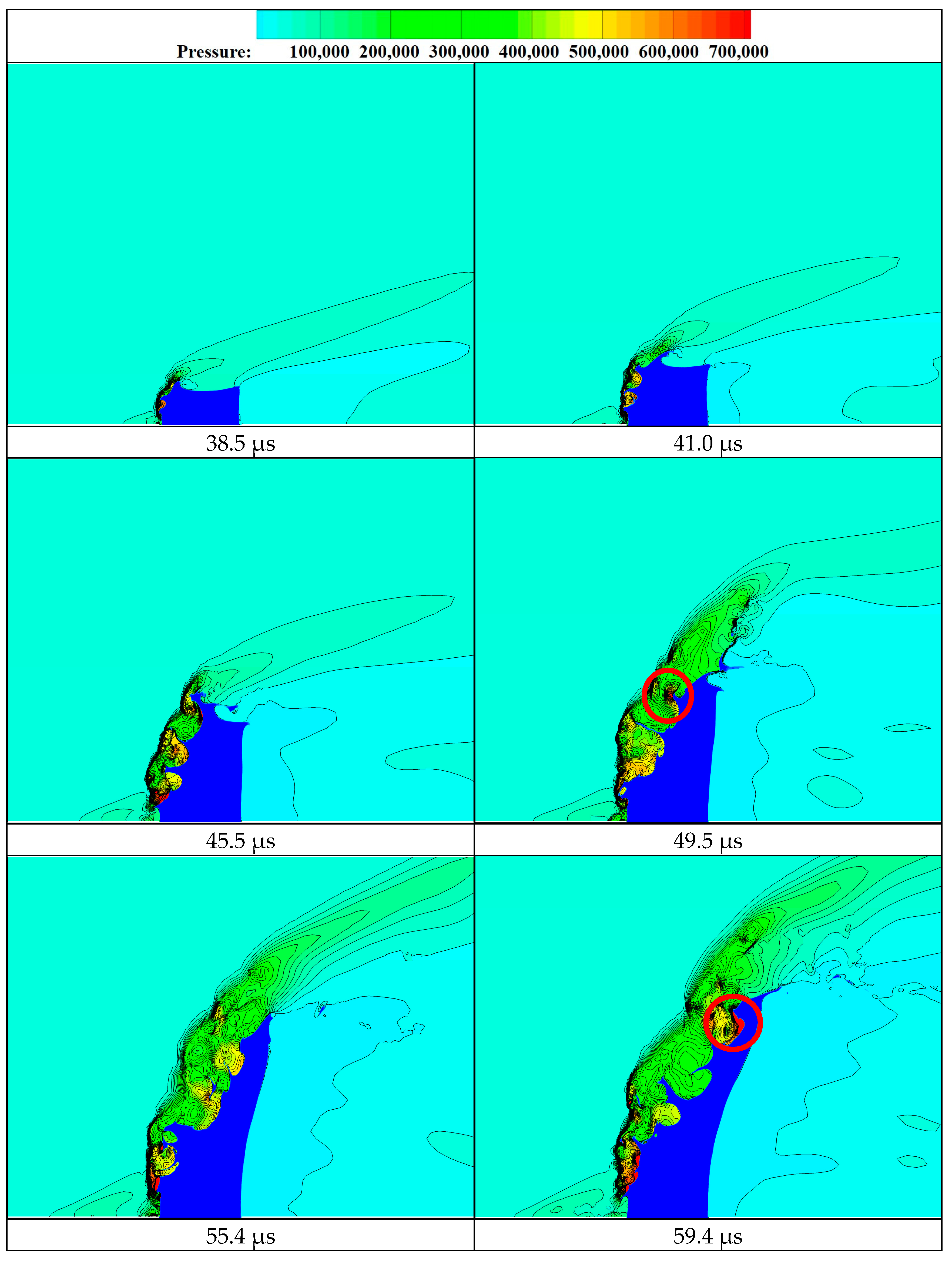
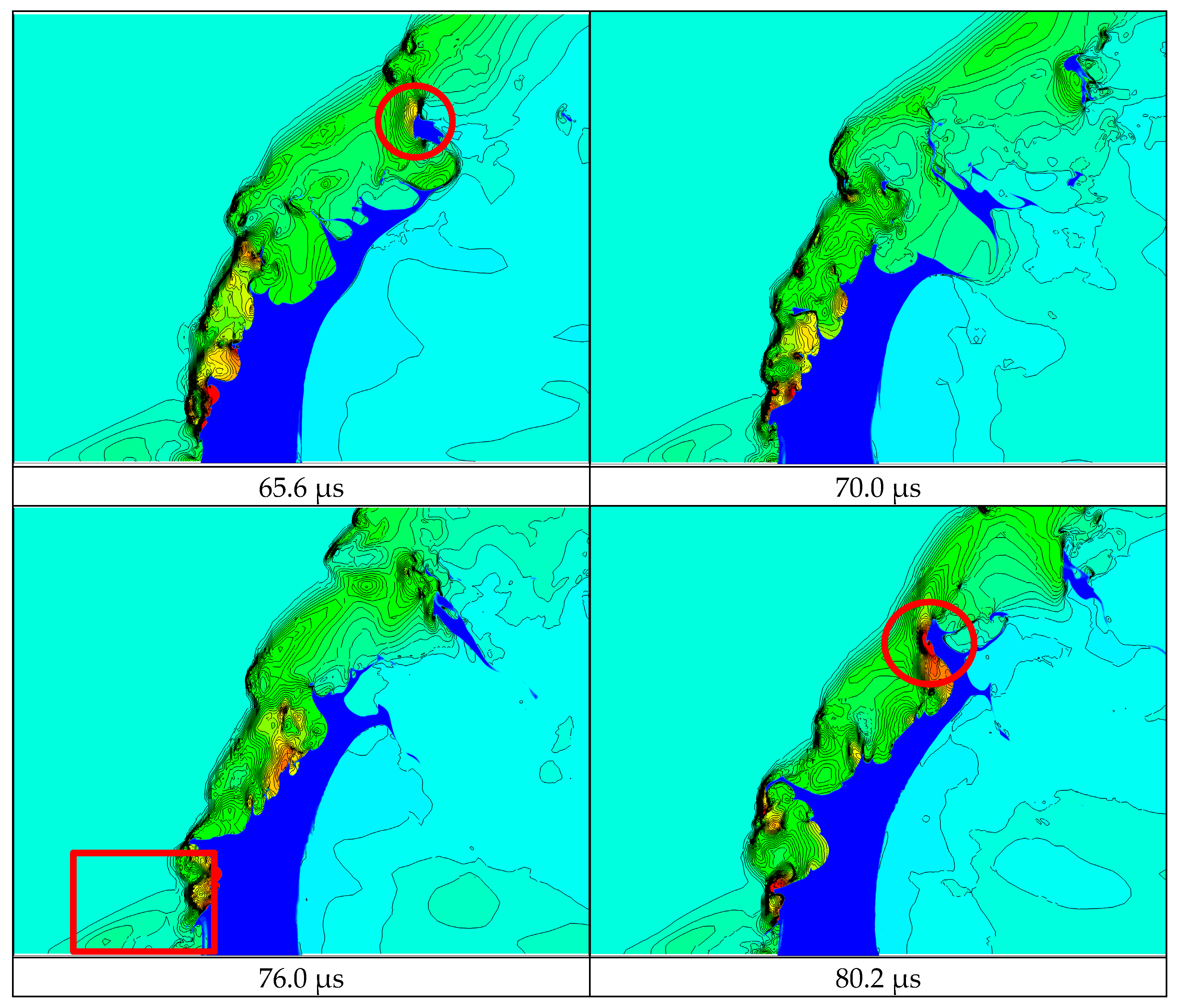
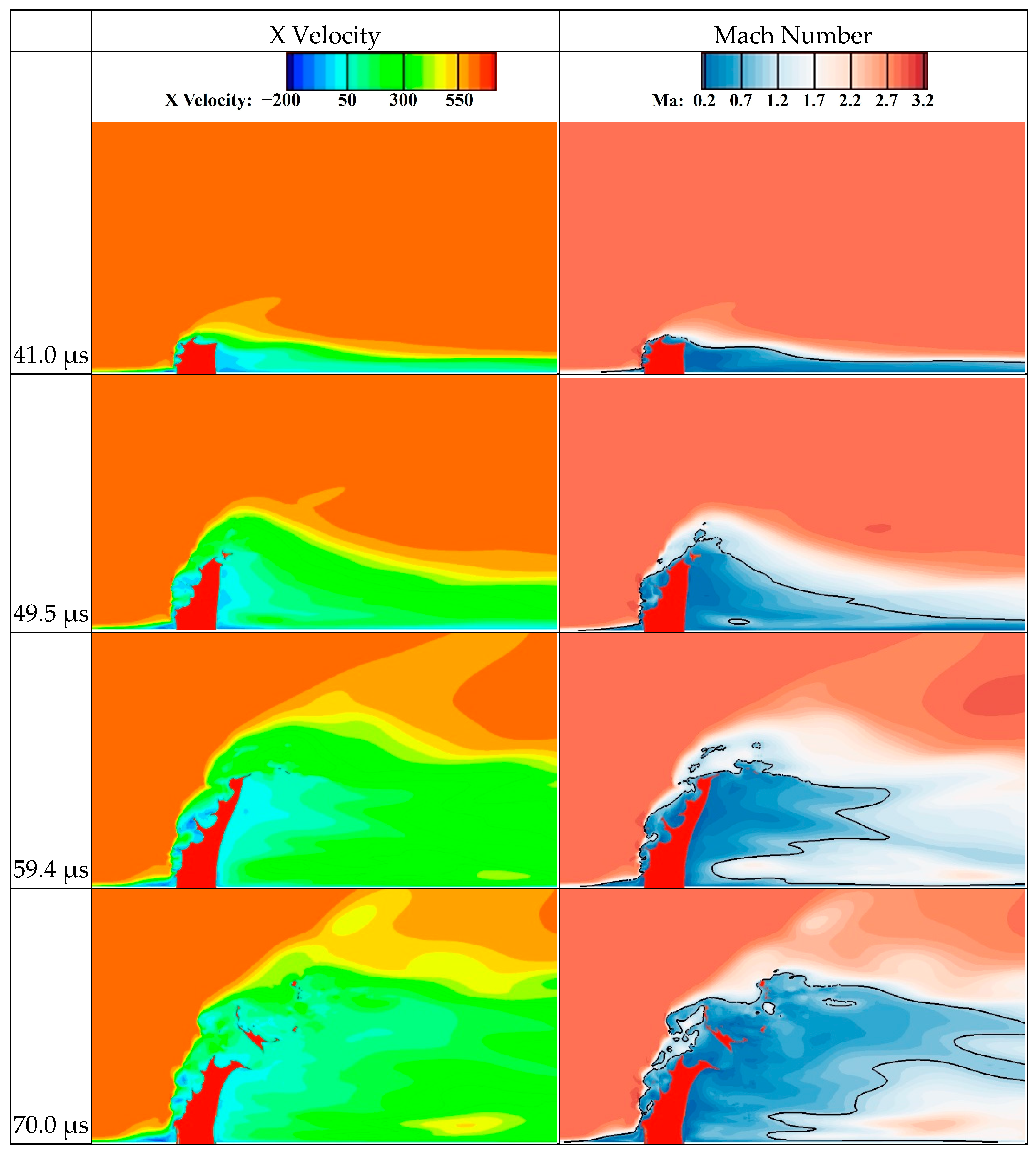
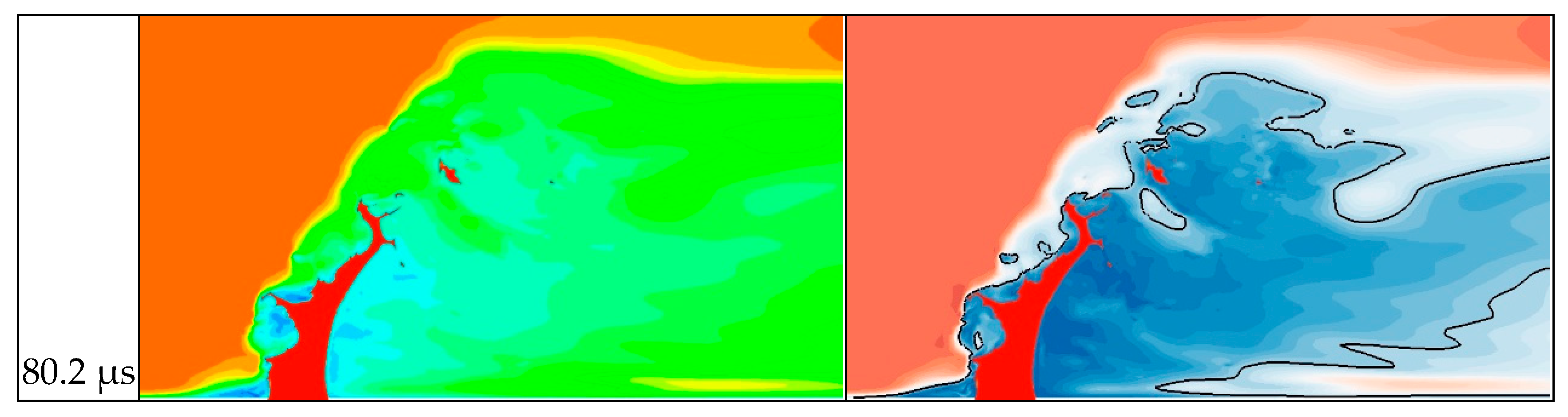
3.1.1. Breakup Process of Supersonic LJIC
3.1.2. Atomization Mechanism of Supersonic LJIC
3.2. Flow Field Analysis of Supersonic LJIC
3.2.1. Shock Wave System Analysis of Supersonic LJIC
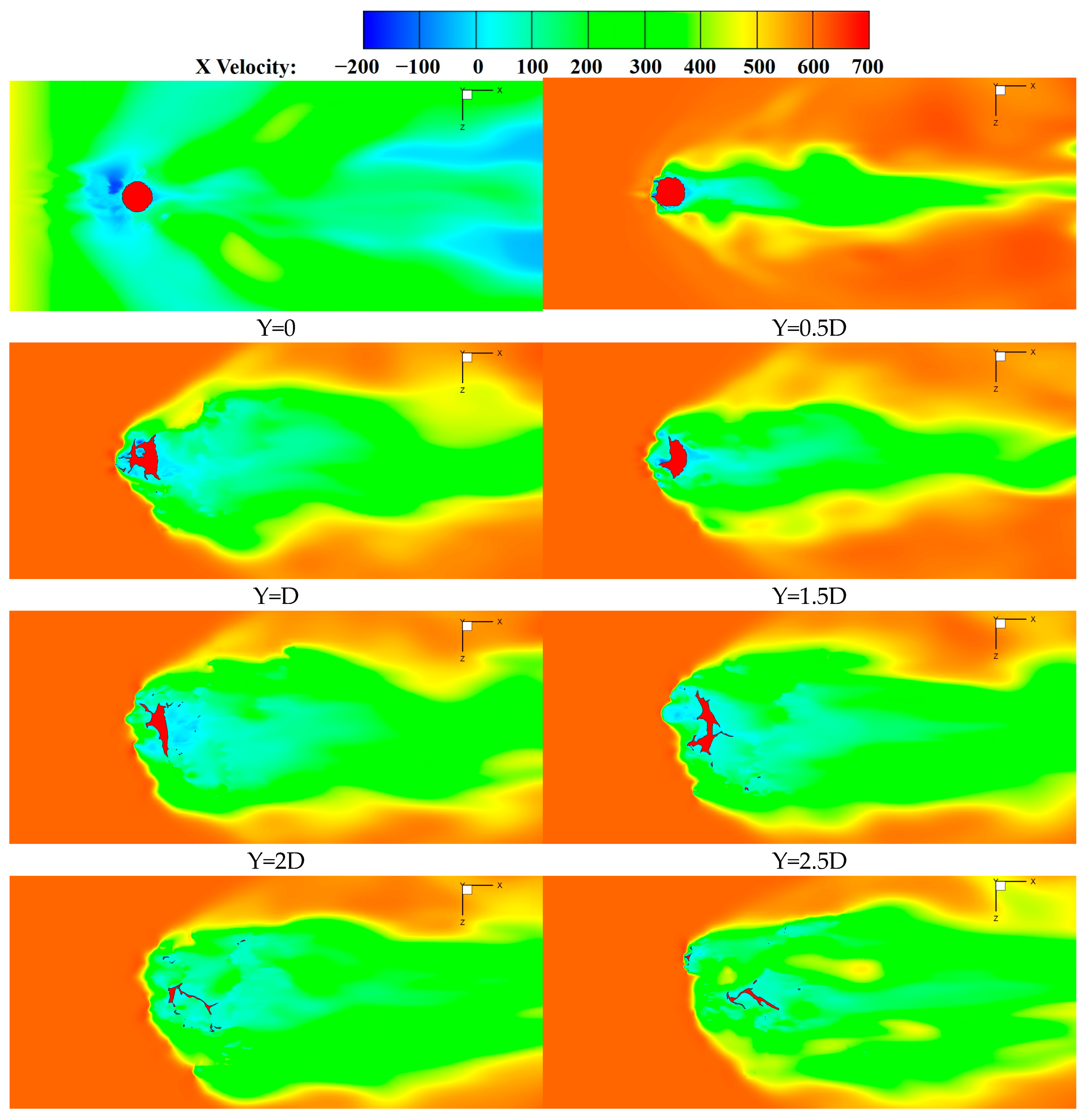
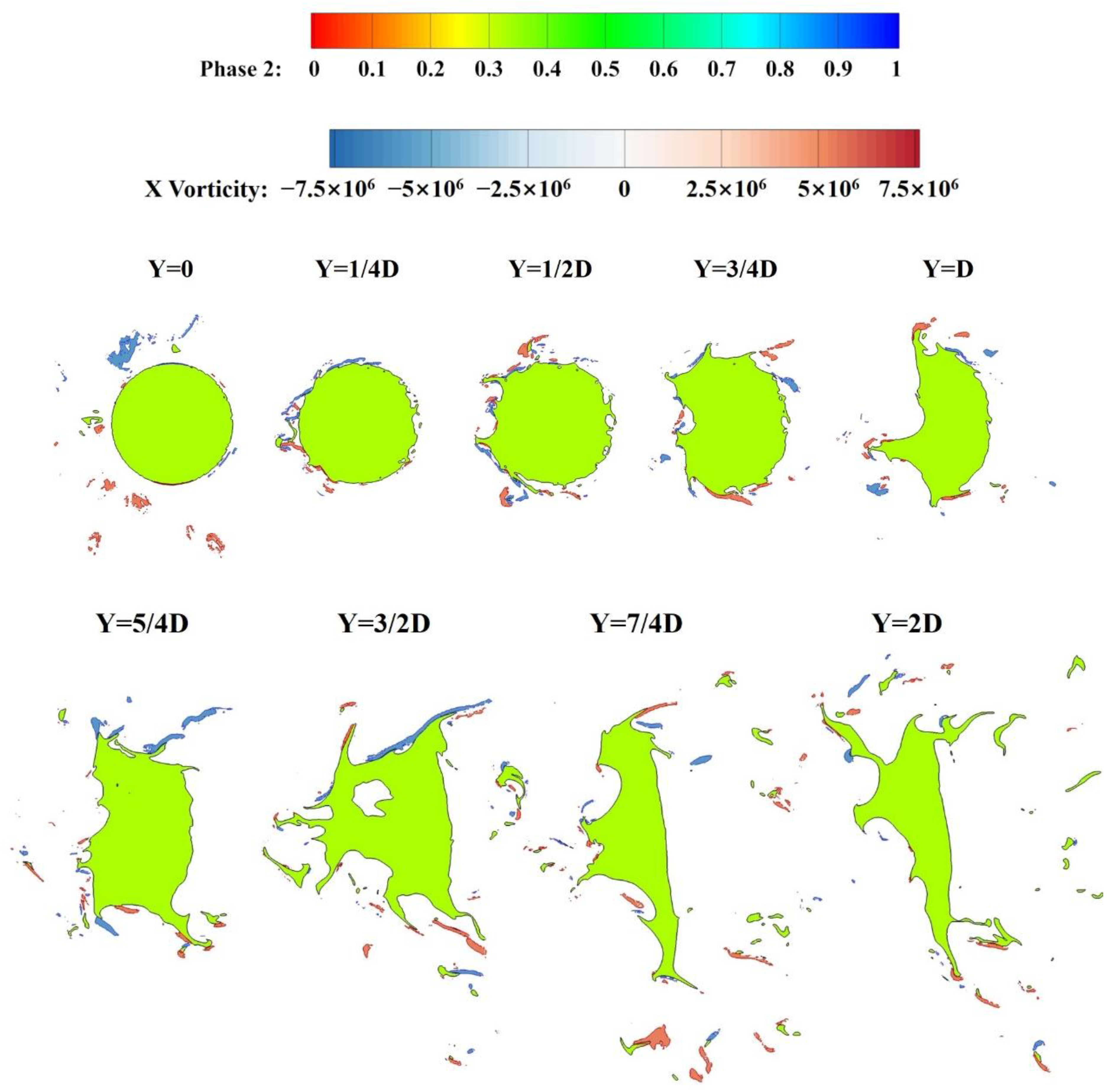
3.2.2. Vertical Flow Field Analysis of Supersonic LJIC
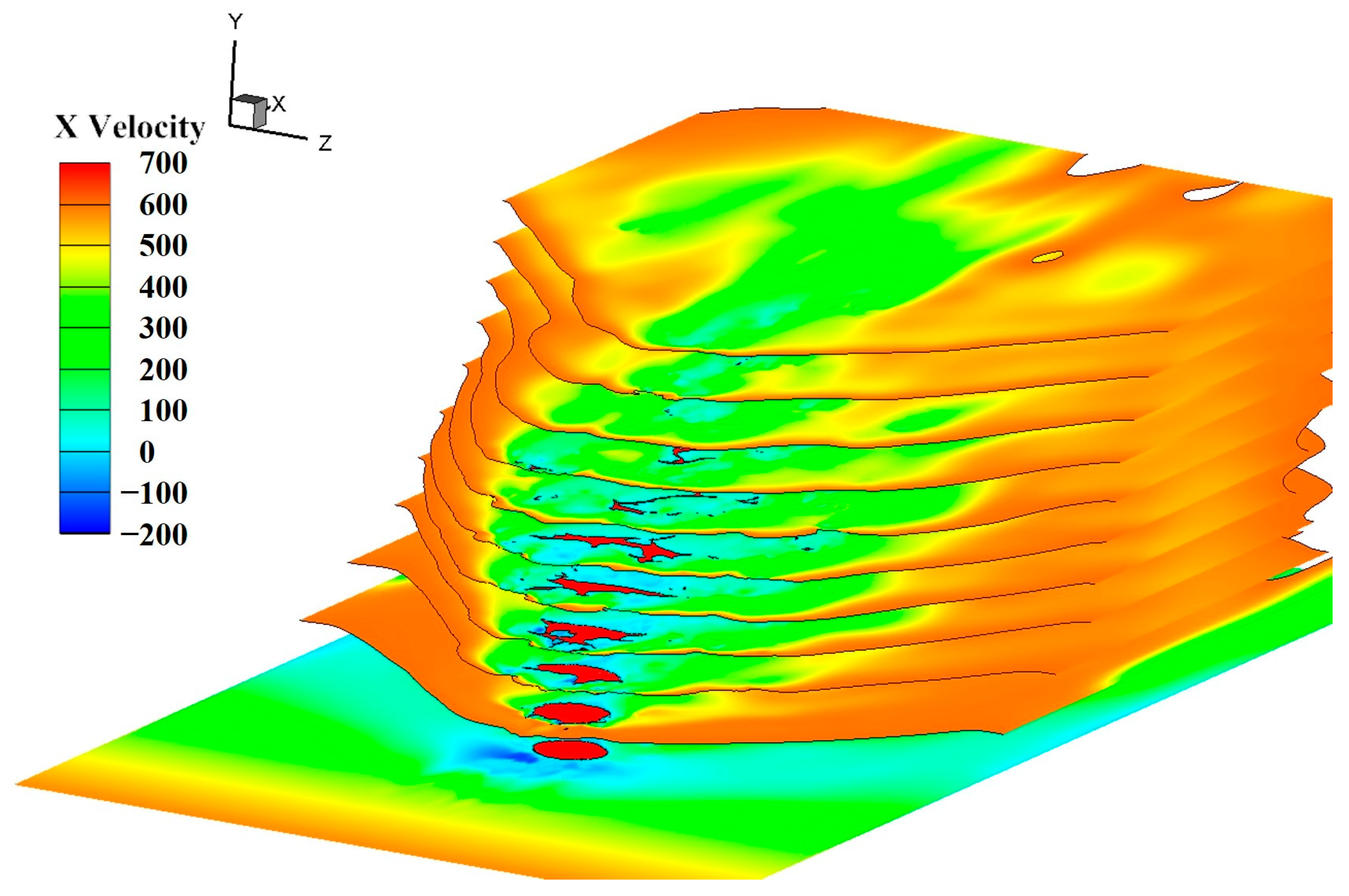
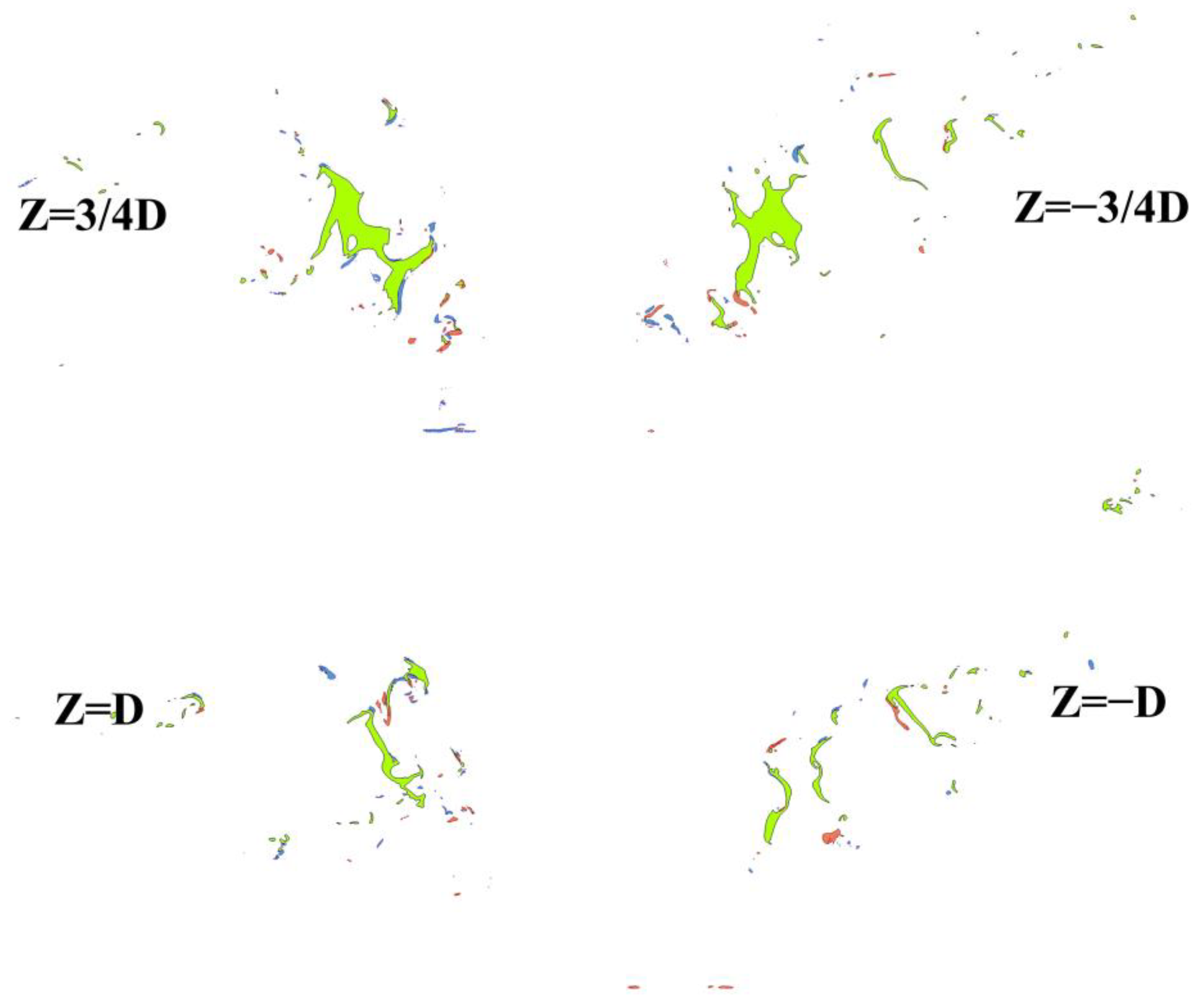
3.2.3. Spanwise Flow Field Analysis of Supersonic LJIC
3.3. Vortex Ring Flow Characteristics of Supersonic LJIC
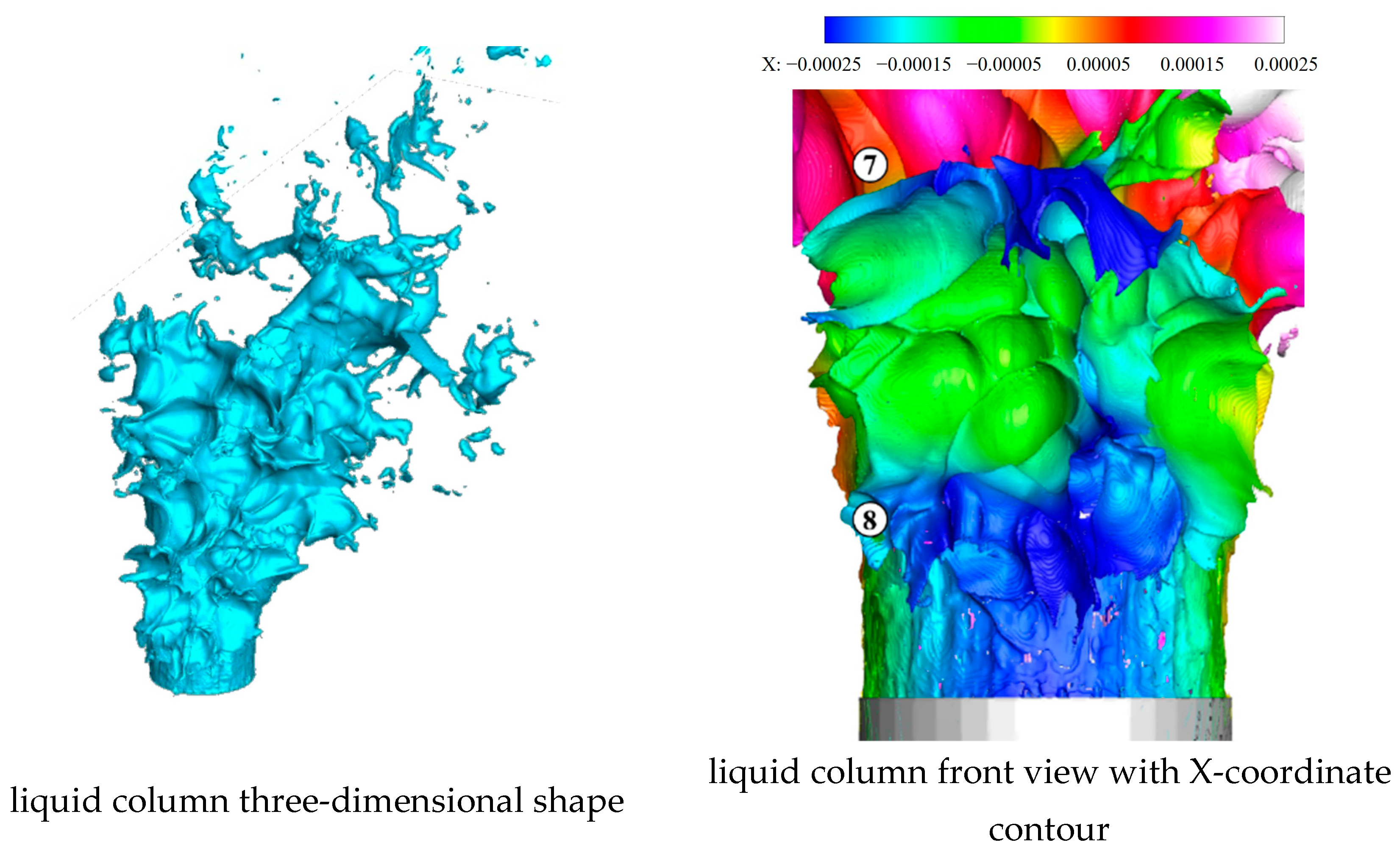
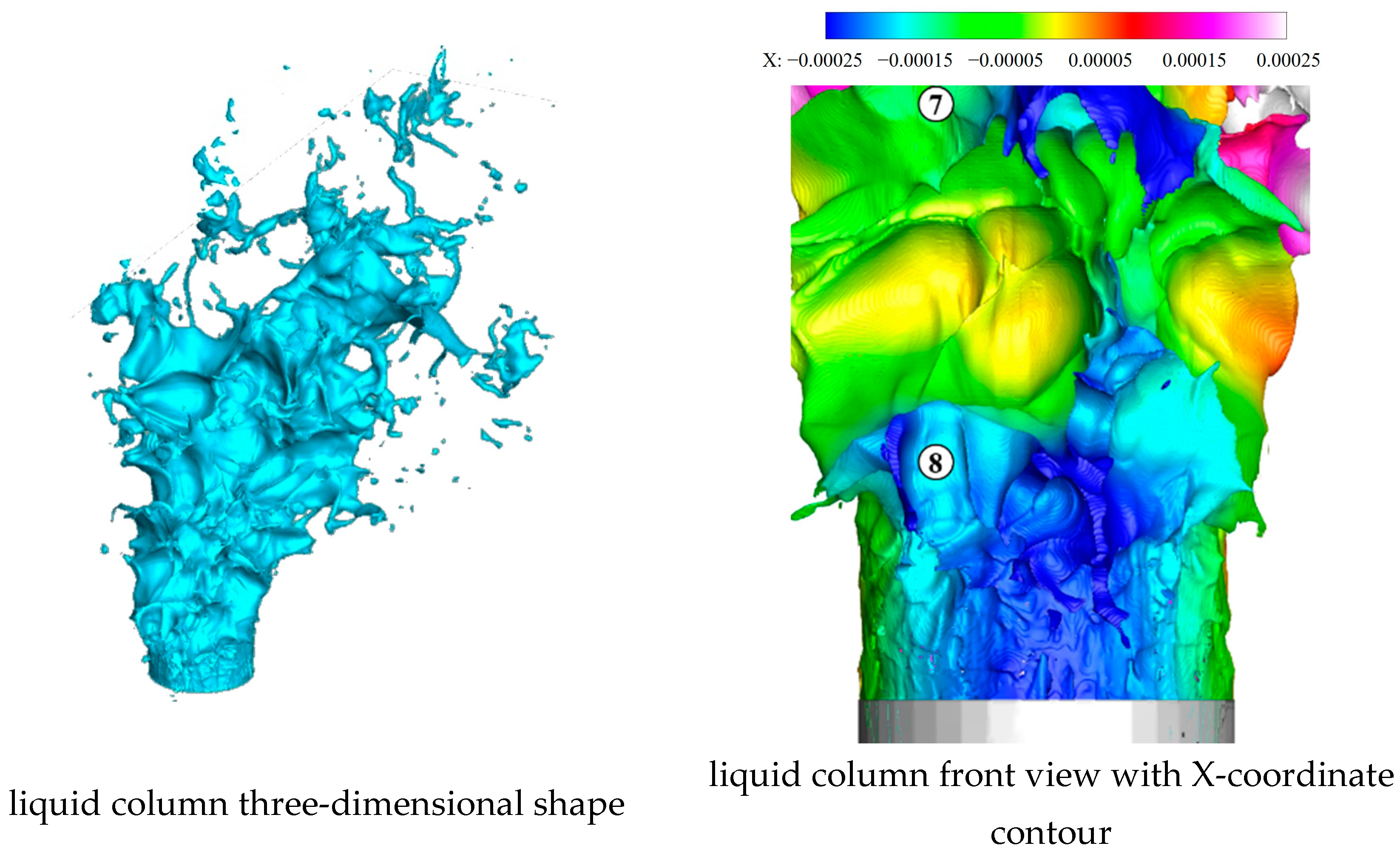
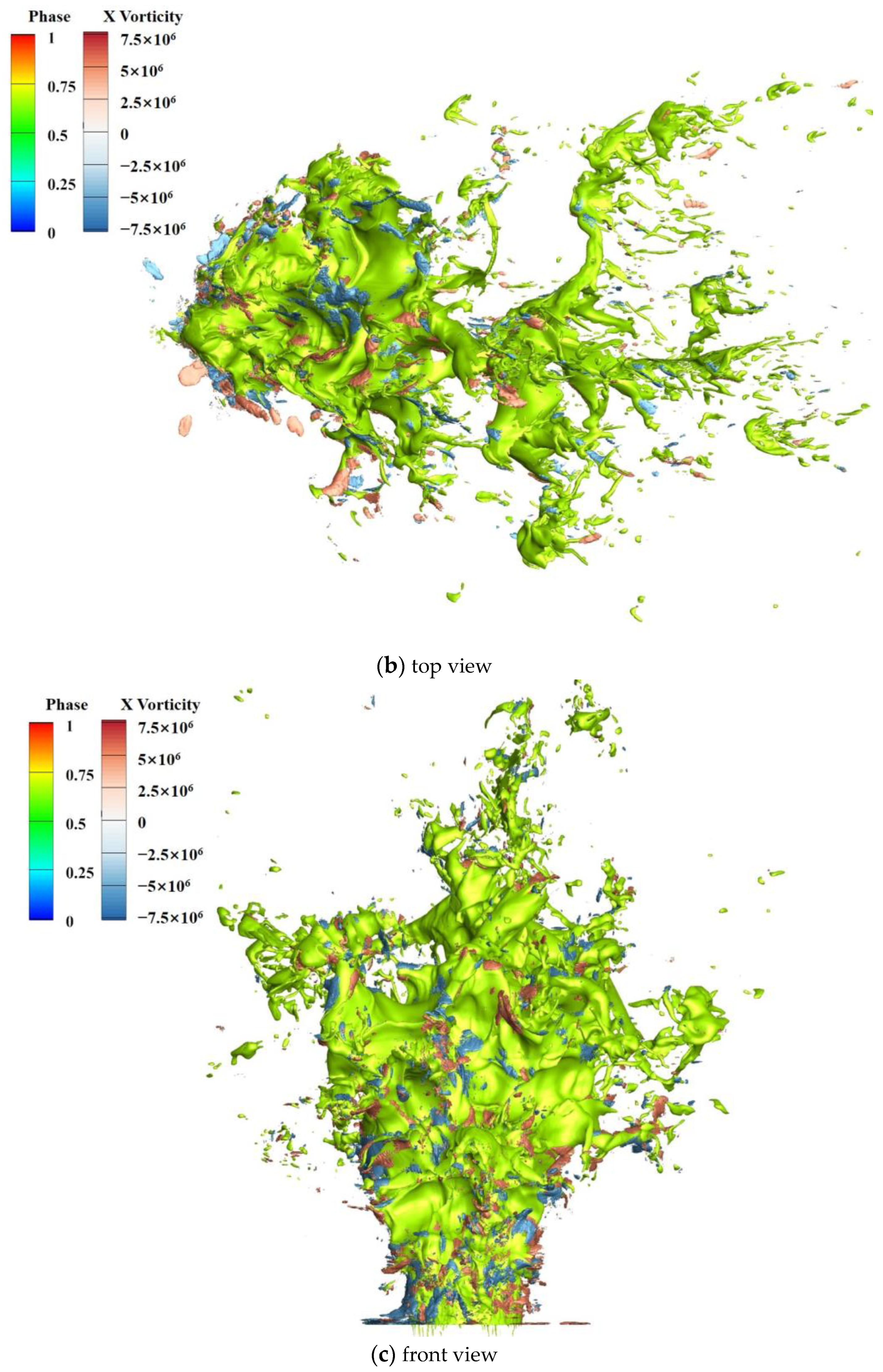
3.3.1. Three-Dimensional Vortex Ring of Supersonic LJIC
3.3.2. Vortex Ring Flow Characteristics of Supersonic LJIC
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Li, C.; Zhou, Y.; Chen, H.; Li, Q. Cross-sectional droplets distribution of a liquid jet in supersonic crossflow. Acta Astronaut. 2021, 186, 109–117. [Google Scholar] [CrossRef]
- Wang, Z.-G.; Wu, L.; Li, Q.; Li, C. Experimental investigation on structures and velocity of liquid jets in a supersonic crossflow. Appl. Phys. Lett. 2014, 105, 134102. [Google Scholar] [CrossRef]
- Li-Yin, W.; Zhen-Guo, W.; Qing-Lian, L.; Chun, L. Unsteady oscillation distribution model of liquid jet in supersonic crossflows. Acta Phys. Sin. 2016, 65, 186–194. [Google Scholar] [CrossRef]
- Wu, L.; Zhang, K.; Li, C.; Li, Q. Model for three-dimensional distribution of liquid fuel in supersonic cross-flows. J. Exp. Fluid Mech. 2018, 32, 20–30. [Google Scholar]
- Wu, L.; Wang, Z.-G.; Li, Q.; Zhang, J. Investigations on the droplet distributions in the atomization of kerosene jets in supersonic crossflows. Appl. Phys. Lett. 2015, 107, 104103. [Google Scholar] [CrossRef]
- Yang, H.; Li, F.; Sun, B. Trajectory analysis of fuel injection into supersonic cross flow based on schlieren method. Chin. J. Aeronaut. 2012, 25, 42–50. [Google Scholar] [CrossRef]
- Nambu, T.; Mizobuchi, Y. Detailed numerical simulation of primary atomization by crossflow under gas turbine engine com-bustor conditions. Proc. Combust. Inst. 2021, 38, 3213–3221. [Google Scholar] [CrossRef]
- Behzad, M.; Ashgriz, N.; Karney, B. Surface breakup of a non-turbulent liquid jet injected into a high pressure gaseous crossflow. Int. J. Multiph. Flow 2016, 80, 100–117. [Google Scholar] [CrossRef]
- Mukundan, A.A.; Tretola, G.; Ménard, T.; Herrmann, M.; Navarro-Martinez, S.; Vogiatzaki, K.; de Motta, J.C.B.; Berlemont, A. DNS and LES of primary atomization of turbulent liquid jet injection into a gaseous crossflow environment. Proc. Combust. Inst. 2021, 38, 3233–3241. [Google Scholar] [CrossRef]
- Xiao, F.; Dianat, M.; McGuirk, J.J. Large eddy simulation of liquid-jet primary breakup in air crossflow. AIAA J. 2013, 51, 2878–2893. [Google Scholar] [CrossRef]
- Wen, J.; Hu, Y.; Nakanishi, A.; Kurose, R. Atomization and evaporation process of liquid fuel jets in crossflows: A numerical study using Eulerian/Lagrangian method. Int. J. Multiph. Flow 2020, 129, 103331. [Google Scholar] [CrossRef]
- Jadidi, M.; Dolatabadi, A. On the trajectory of nonturbulent liquid jets in subsonic crossflows at different density ratios. Theor. Appl. Mech. Lett. 2018, 8, 277–283. [Google Scholar] [CrossRef]
- Zhao, J.; Yan, C.; Wu, L.; Lin, W.; Tong, Y.; Nie, W. Numerical simulation of single/double liquid jets in supersonic crossflows. Aerosp. Sci. Technol. 2022, 120, 107289. [Google Scholar] [CrossRef]
- Xiao, F.; Wang, Z.G.; Sun, M.B.; Liang, J.H.; Liu, N. Large eddy simulation of liquid jet primary breakup in supersonic air crossflow. Int. J. Multiph. Flow 2016, 87, 229–240. [Google Scholar] [CrossRef]
- Xiao, F.; Sun, M.B. Effects of Mach number on liquid jet primary breakup in gas crossflow. At. Sprays 2018, 28, 975–999. [Google Scholar] [CrossRef]
- Li, C.; Shen, C.; Li, Q.; Zhu, Y. Primary breakup process of liquid jet in supersonic crossflow. J. Natl. Univ. Def. Technol. 2019, 41, 73–78. [Google Scholar]
- Zhou, Y.; Li, Q.; Li, C. Study on Breaking Process of Liquid Jet in Supersonic Flow Based on Adaptive Mesh. J. Propuls. Technol. 2020, 41, 1571–1579. [Google Scholar]
- Li, P.; Wang, Z.; Sun, M.; Wang, H. Numerical simulation of the gas-liquid interaction of a liquid jet in supersonic crossflow. Acta Astronaut. 2017, 134, 333–344. [Google Scholar] [CrossRef]
- Li, P.; Wang, Z.; Bai, X.-S.; Wang, H.; Sun, M.; Wu, L.; Liu, C. Three-dimensional flow structures and droplet-gas mixing process of a liquid jet in supersonic crossflow. Aerosp. Sci. Technol. 2019, 90, 140–156. [Google Scholar] [CrossRef]
- Li, P.; Wang, Z.; Sun, M.; Wang, H. Numerical Simulation of the Gas-Liquid Interaction of Cross Liquid Jet in Supersonic Flow. J. Astronaut. 2016, 37, 209–215. [Google Scholar]
- Liu, W.L.; Zhu, L.; Qi, Y.Y.; Ge, J.R.; Luo, F.; Zou, H.R.; Wei, M.; Jen, T.C. Effects of injection pressure variation on mixing in a cold supersonic combustor with kerosene fuel. Acta Astronaut. 2017, 139, 67–76. [Google Scholar] [CrossRef]
- Zhu, L.; Luo, F.; Qi, Y.-Y.; Wei, M.; Ge, J.-R.; Liu, W.-L.; Li, G.-L.; Jen, T.-C. Effects of spray angle variation on mixing in a cold supersonic combustor with kerosene fuel. Acta Astronaut. 2018, 144, 1–11. [Google Scholar] [CrossRef]
- Zhu, L.; Qi, Y.Y.; Liu, W.L.; Xu, B.J.; Ge, J.R.; Xuan, X.C.; Jen, T.C. Numerical investigation of scale effect of various injection diameters on interaction in cold ker-osene-fueled supersonic flow. Acta Astronaut. 2016, 129, 111–120. [Google Scholar] [CrossRef]
- Zhou, Y.-Z.; Xiao, F.; Li, Q.-L.; Li, C.-Y. Simulation of elliptical liquid jet primary breakup in supersonic crossflow. Int. J. Aerosp. Eng. 2020, 2020, 1–12. [Google Scholar] [CrossRef]
- Niu, Y.Y.; Wu, C.H.; Huang, Y.H.; Chou, Y.J.; Kong, S.C. Evaluation of breakup models for liquid side jets in supersonic cross flows. Numer. Heat Transf. Part A Appl. 2020, 79, 353–369. [Google Scholar] [CrossRef]
- Almanzalawy, M.S.; Rabie, L.H.; Mansour, M.H. Modeling of an efficient airblast atomizer for liquid jet into a supersonic crossflow. Acta Astronaut. 2020, 177, 142–157. [Google Scholar] [CrossRef]
- Zhu, Y.H.; Xiao, F.; Li, Q.L.; Mo, R.; Li, C.; Lin, S. LES of primary breakup of pulsed liquid jet in supersonic crossflow. Acta Astronaut. 2019, 154, 119–132. [Google Scholar] [CrossRef]
- Liu, N.; Wang, Z.; Sun, M.; Deiterding, R.; Wang, H. Simulation of liquid jet primary breakup in a supersonic crossflow under Adaptive Mesh Refinement framework. Aerosp. Sci. Technol. 2019, 91, 456–473. [Google Scholar] [CrossRef]
- Allaire, G.; Clerc, S.; Kokhc, S. A five-equation model for the simulation of interfaces between compressible fluids. J. Comput. Phys. 2002, 181, 577–616. [Google Scholar] [CrossRef]
- Lin, S.; Shen, C.; Xiao, F.; Zhu, Y. Large Eddy Simulation of Primary Breakup of Transverse Pulsed Liquid Jet in Supersonic Flow. J. Combust. Sci. Technol. 2020, 26, 87–95. [Google Scholar]
- Hu, R.; Li, Q.; Li, C.; Li, C. Effects of an accompanied gas jet on transverse liquid injection in a supersonic crossflow. Acta Astronaut. 2019, 159, 440–451. [Google Scholar] [CrossRef]
- Zhao, J.; Lin, W.; Yan, C.; Zheng, Z.; Tong, Y.; Nie, W. Mixing enhancement mechanism of combined H2–Water jets in supersonic crossflows in a com-bustor with an expanded section. Int. J. Hydrog. Energy 2022, 47, 10747–10761. [Google Scholar] [CrossRef]
- Zhao, J.; Nie, W.; Tong, Y.; Ren, Y.; Zhu, Y.; Lin, W. Effects of Injection Angles on Liquid Jets in Supersonic Crossflows. J. Propuls. Technol. 2022, 43, 239–249. [Google Scholar]
- Almeida, H.; Sousa, J.M.M.; Costa, M. Effect of the liquid injection angle on the atomization of liquid jets in subsonic crossflows. At. Sprays 2014, 24, 81–96. [Google Scholar] [CrossRef]
- Beloki Perurena, J.; Asma, C.O.; Theunissen, R.; Chazot, O. Experimental investigation of liquid jet injection into Mach 6 hypersonic crossflow. Exp. Fluids 2009, 46, 403–417. [Google Scholar] [CrossRef]
- Olyaei, G.; Kebriaee, A. Experimental study of liquid jets injected in crossflow. Exp. Therm. Fluid Sci. 2020, 115, 110049. [Google Scholar] [CrossRef]
- Jadidi, M.; Sreekumar, V.; Dolatabadi, A. Breakup of elliptical liquid jets in gaseous crossflows at low Weber numbers. J. Vis. 2019, 22, 259–271. [Google Scholar] [CrossRef]
- Prakash, R.S.; Sinha, A.; Tomar, G.; Ravikrishna, R. Liquid jet in crossflow—Effect of liquid entry conditions. Exp. Therm. Fluid Sci. 2018, 93, 45–56. [Google Scholar] [CrossRef]
- Lee, I.C.; Kang, Y.S.; Moon, H.J.; Jang, S.P.; Kim, J.K.; Koo, J. Spray jet penetration and distribution of modulated liquid jets in subsonic cross-flows. J. Mech. Sci. Technol. 2010, 24, 1425–1431. [Google Scholar] [CrossRef]
- Lee, I.; Kang, Y.; Koo, J. Mixing characteristics of pulsed air-assist liquid jet into an internal subsonic cross-flow. J. Therm. Sci. 2010, 19, 136–140. [Google Scholar] [CrossRef]
- Sussman, M.; Puckett, E.G. A coupled level set and volume-of-fluid method for computing 3D and axisymmetric incompressible two-phase flows. J. Comput. Phys. 2000, 162, 301–337. [Google Scholar] [CrossRef]
- Xiao, F.; Wang, Z.G.; Sun, M.B.; Liang, J.H.; Liu, N. Large Eddy Simulation of Droplet Breakup in Supersonic Flow. J. Propuls. Technol. 2016, 37, 706–712. [Google Scholar]
- Smagorinsky, J. General circulation experiments with the primitive equations: I. The basic experiment. Mon. Weather Rev. 1963, 91, 99–164. [Google Scholar] [CrossRef]
- Deardorff, J.W. A numerical study of three-dimensional turbulent channel flow at large Reynolds numbers. J. Fluid Mech. 1970, 41, 453–480. [Google Scholar] [CrossRef]
- Scotti, A.; Meneveau, C.; Lilly, D.K. Generalized Smagorinsky model for anisotropic grids. Phys. Fluids A Fluid Dyn. 1993, 5, 2306–2308. [Google Scholar] [CrossRef]
- Moeng, C.H.; Wyngaard, J.C. Spectral analysis of large-eddy simulations of the convective boundary layer. J. Atmos. Sci. 1988, 45, 3573–3587. [Google Scholar] [CrossRef]
- Fuster, D.; Agbaglah, G.; Josserand, C.; Popinet, S.; Zaleski, S. Numerical simulation of droplets, bubbles and waves: State of the art. Fluid Dyn. Res. 2009, 41, 065001. [Google Scholar] [CrossRef]
- Sussman, M.; Almgren, A.S.; Bell, J.B.; Colellab, P.; Howell, L.H.; Welcome, M.L. An Adaptive Level Set Approach for Incompressible Two-Phase Flows. J. Comput. Phys. 1999, 148, 81–124. [Google Scholar] [CrossRef]
- Lebas, R.; Menard, T.; Beau, P.; Berlemont, A.; Demoulin, F. Numerical simulation of primary break-up and atomization: DNS and modelling study. Int. J. Multiph. Flow 2009, 35, 247–260. [Google Scholar] [CrossRef]












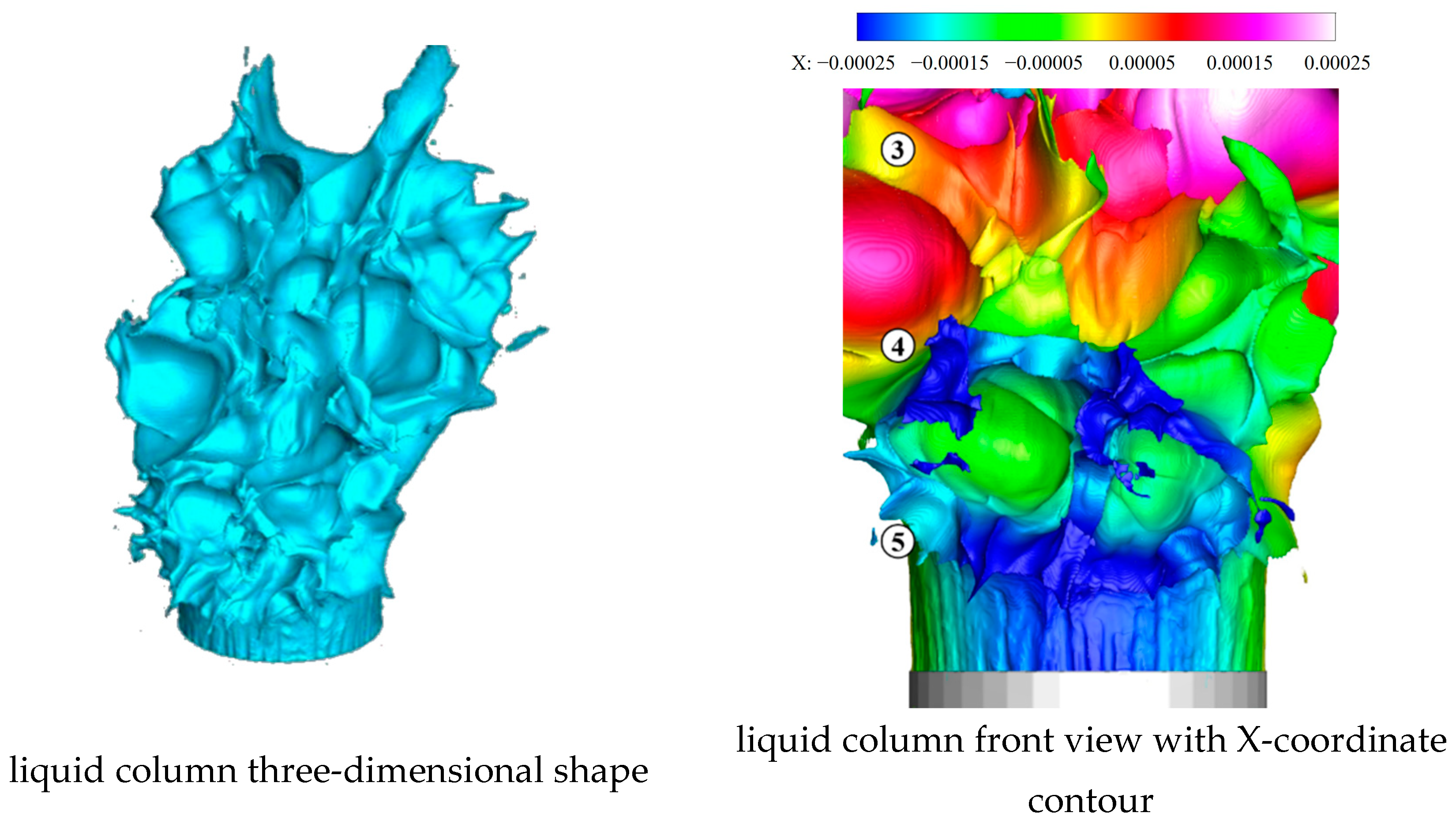
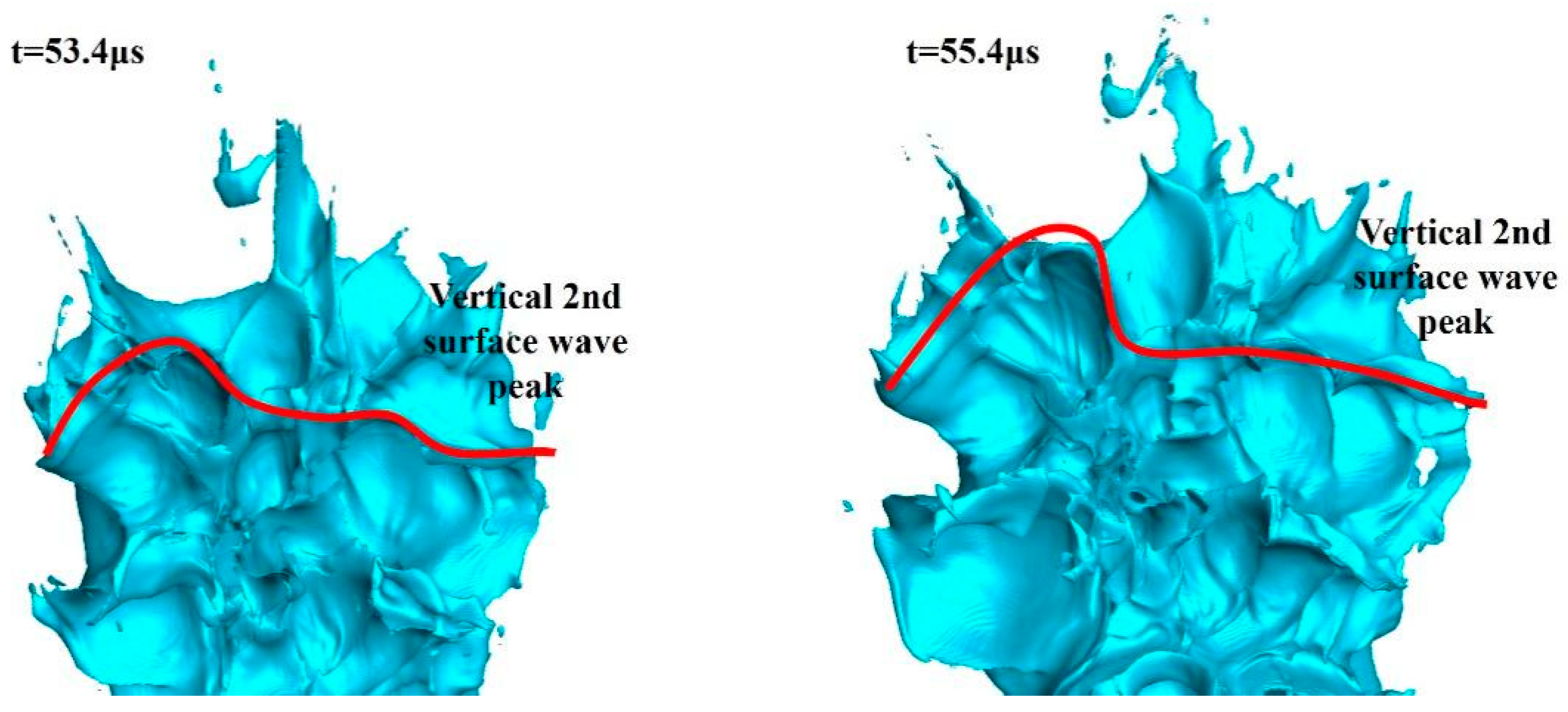

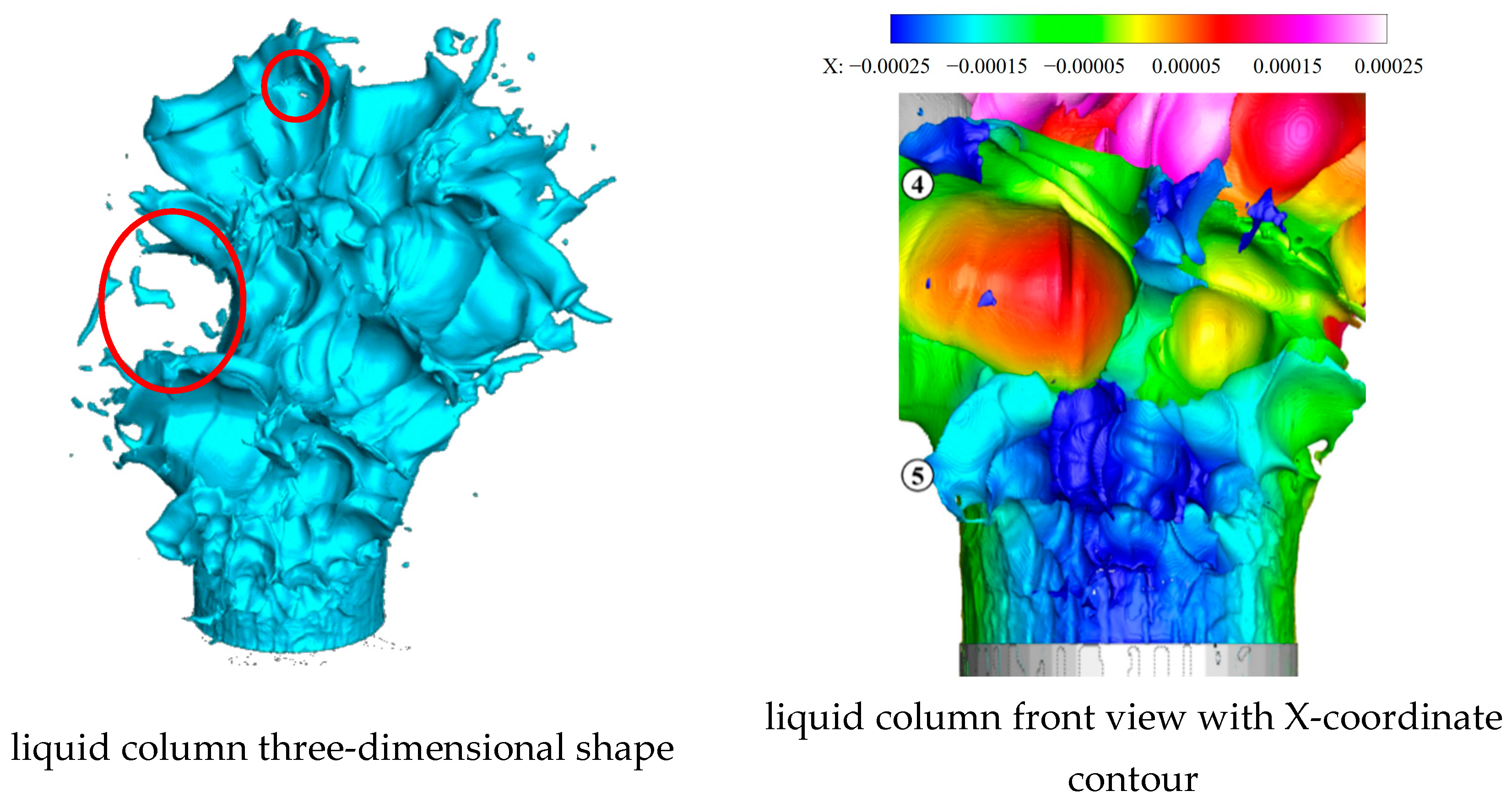

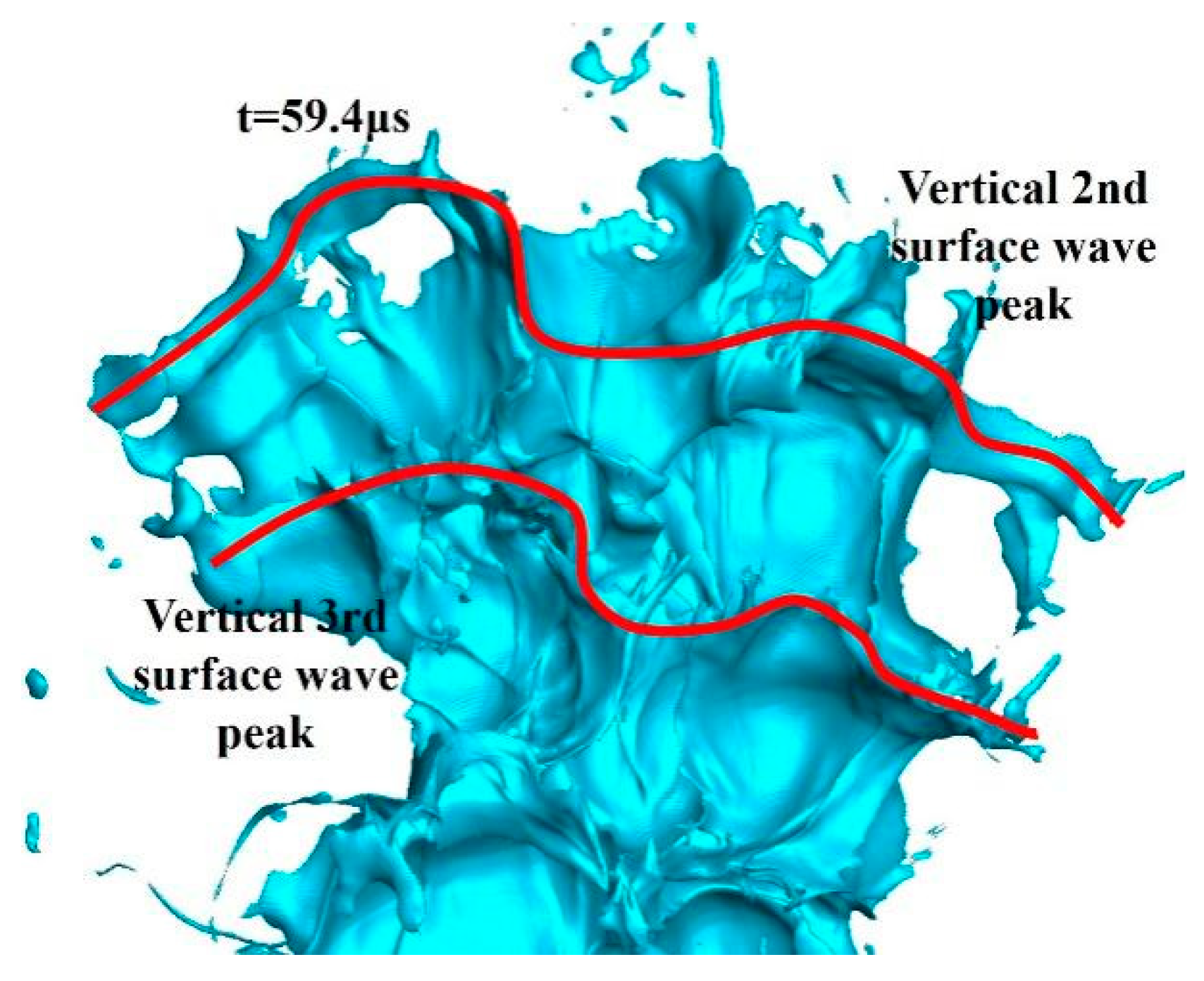
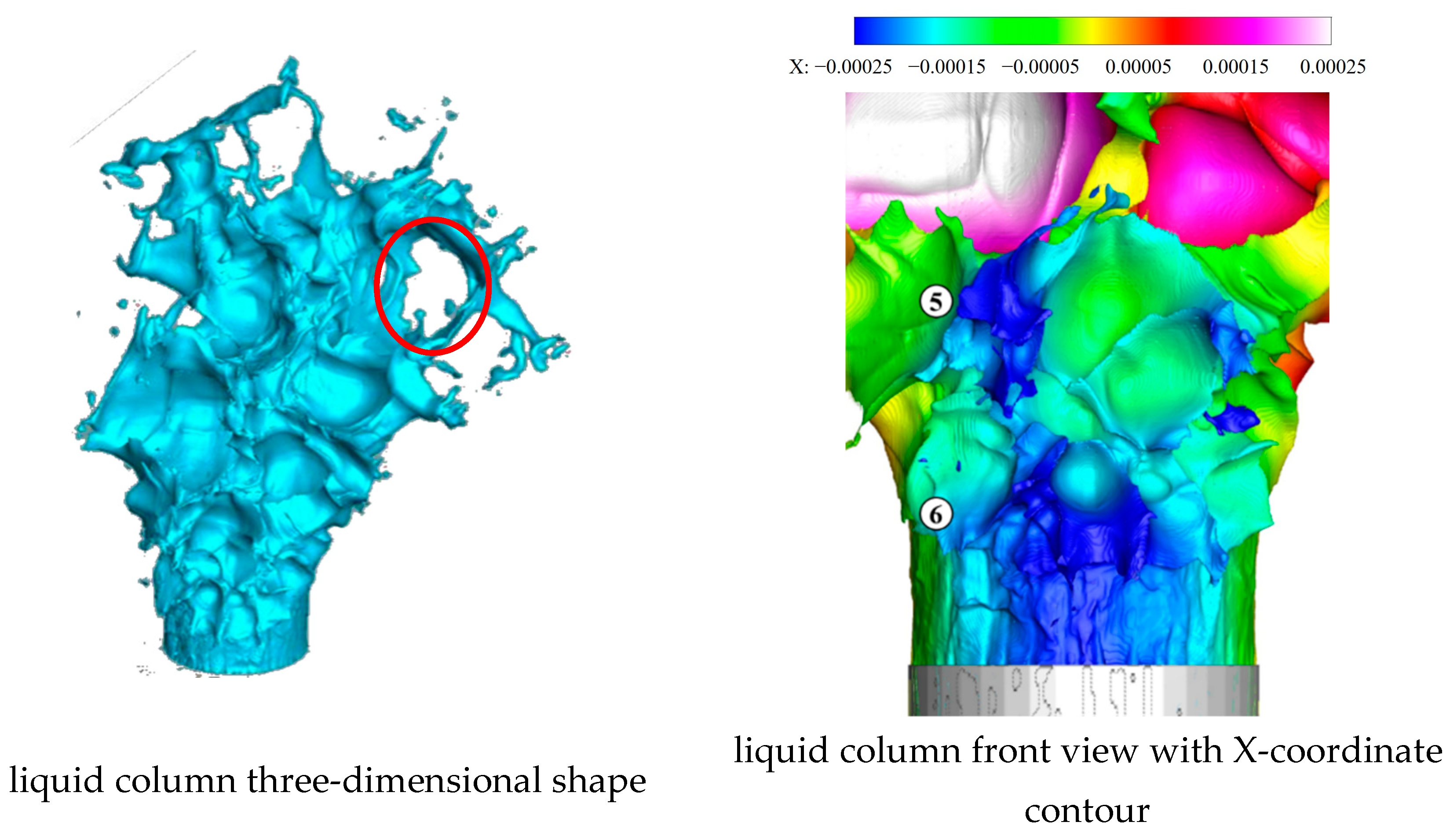

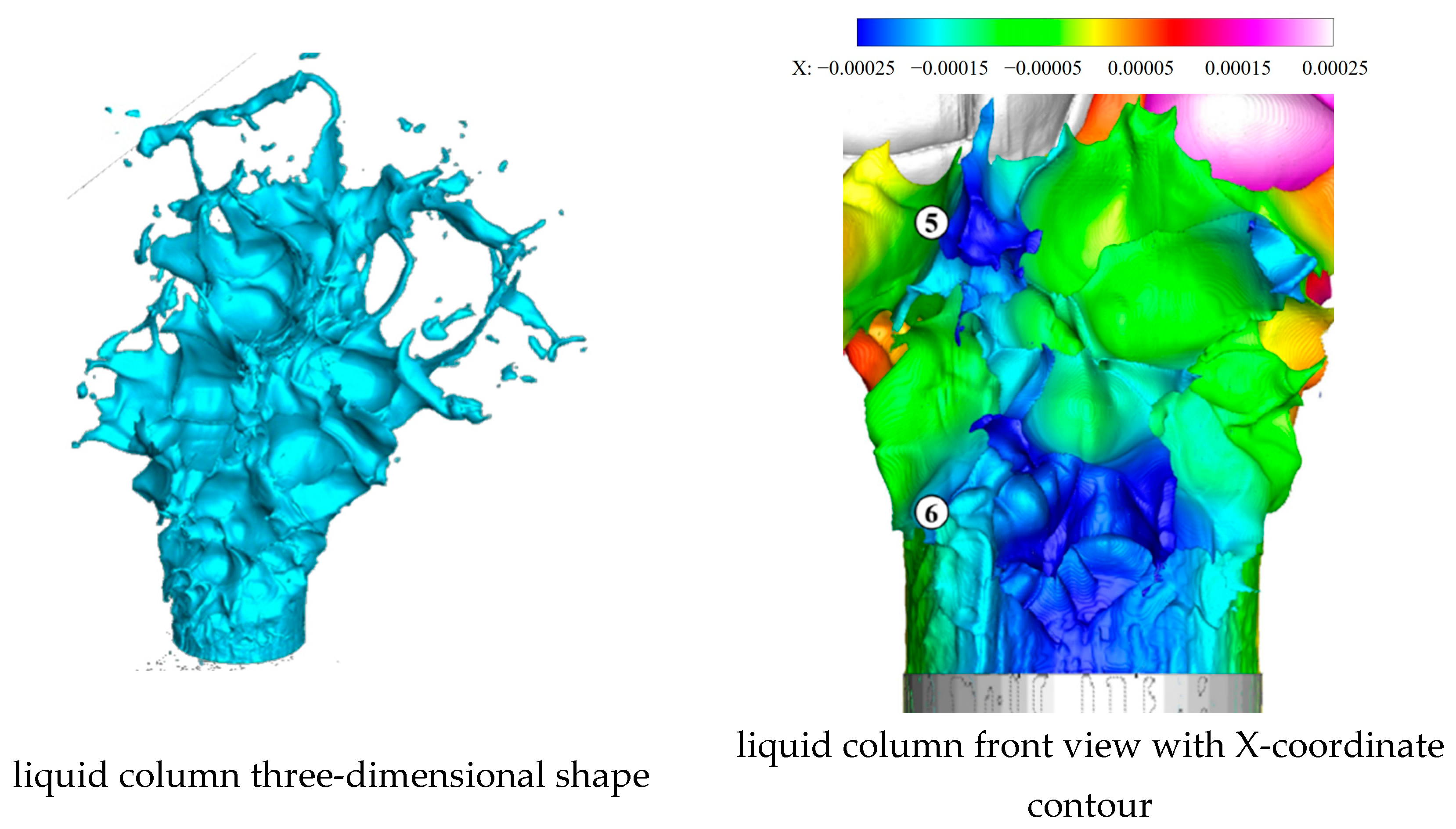
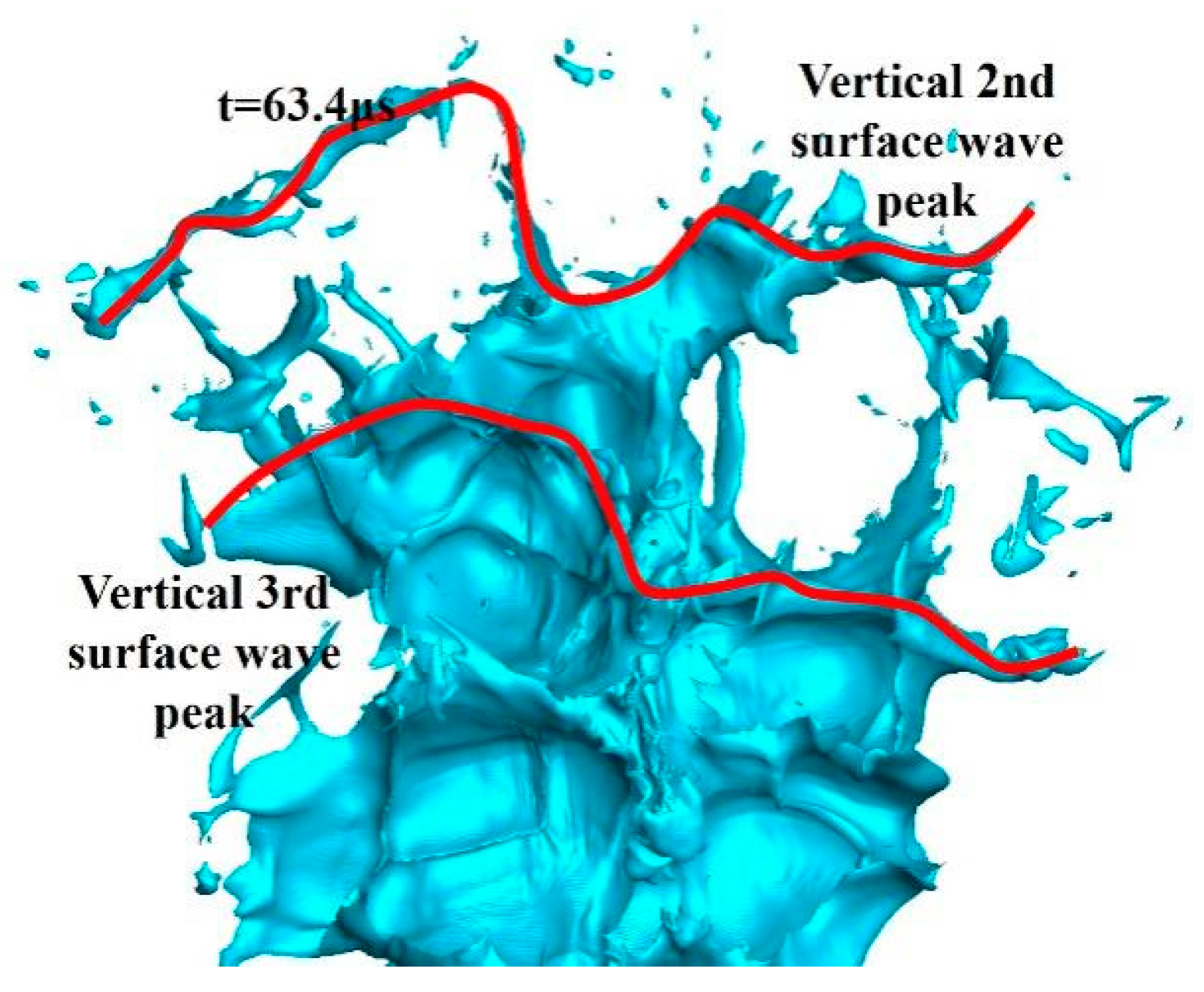
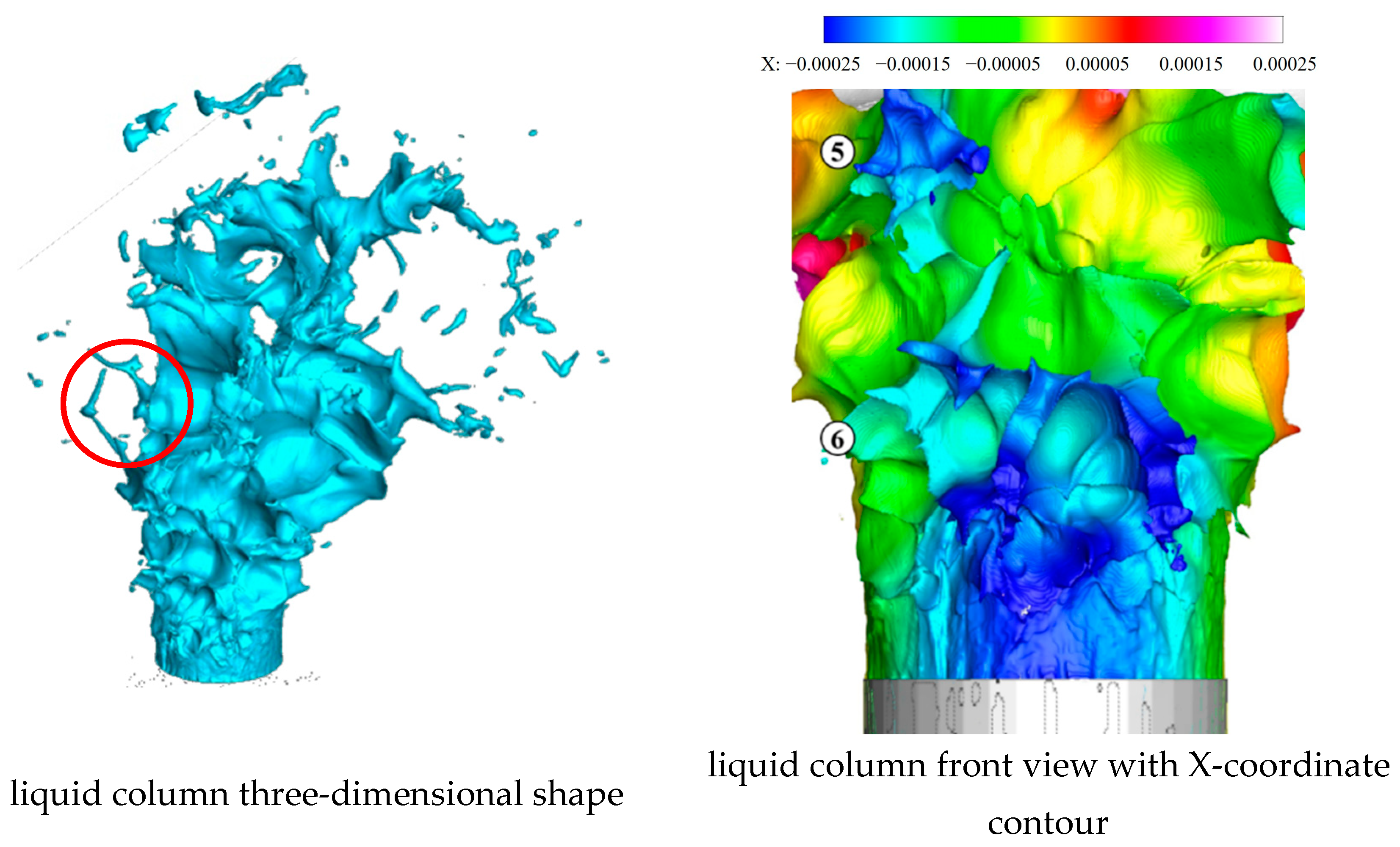
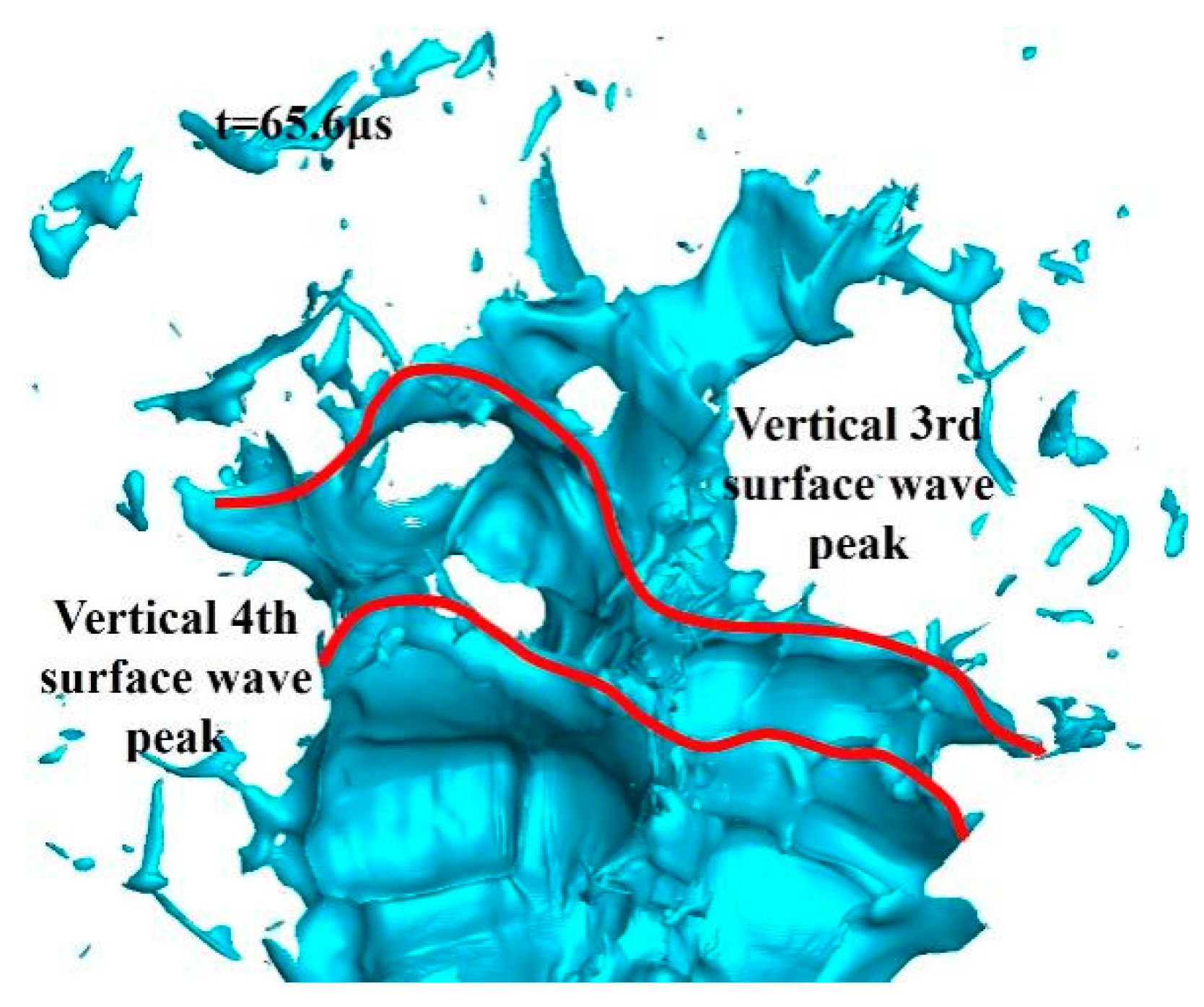
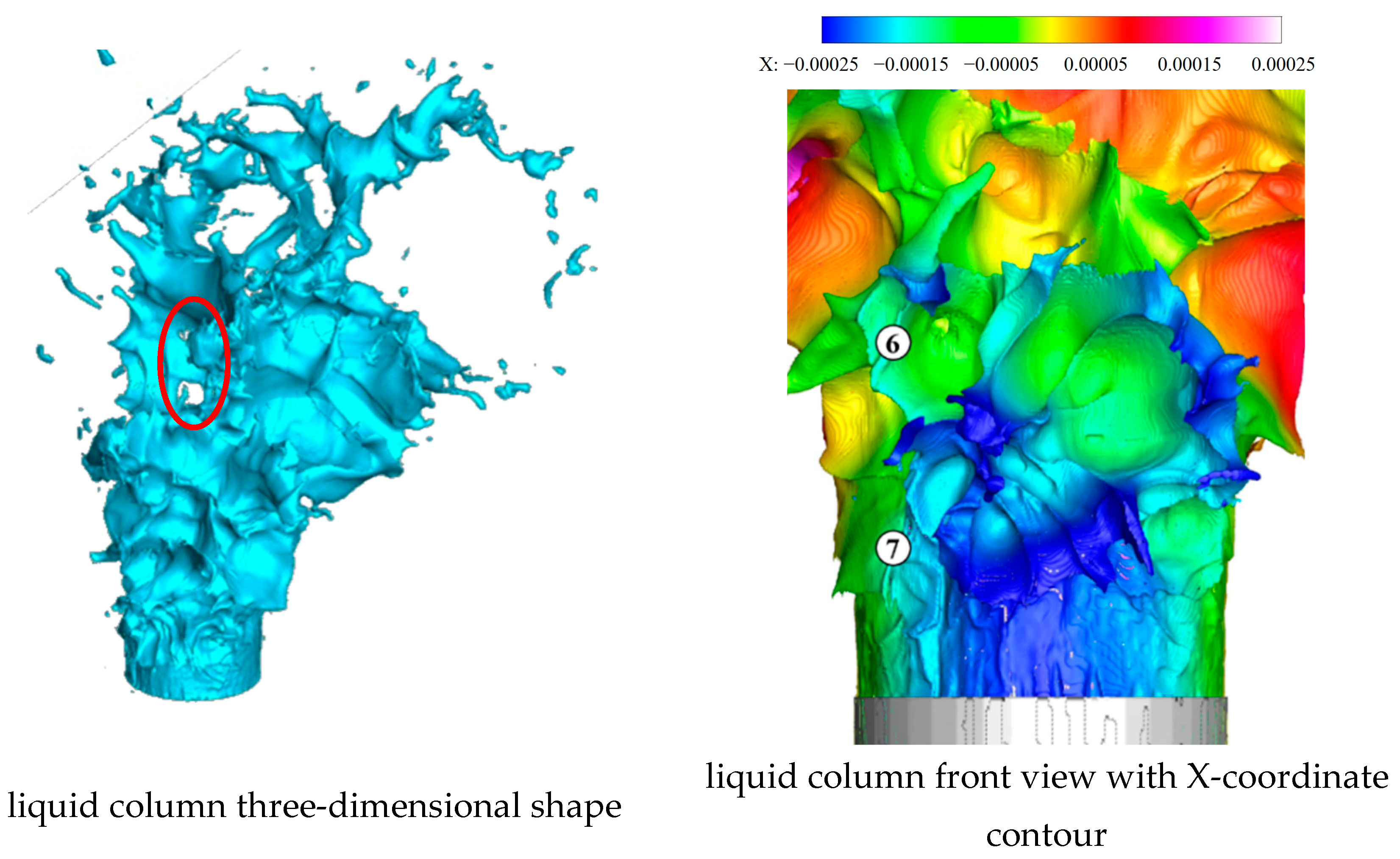

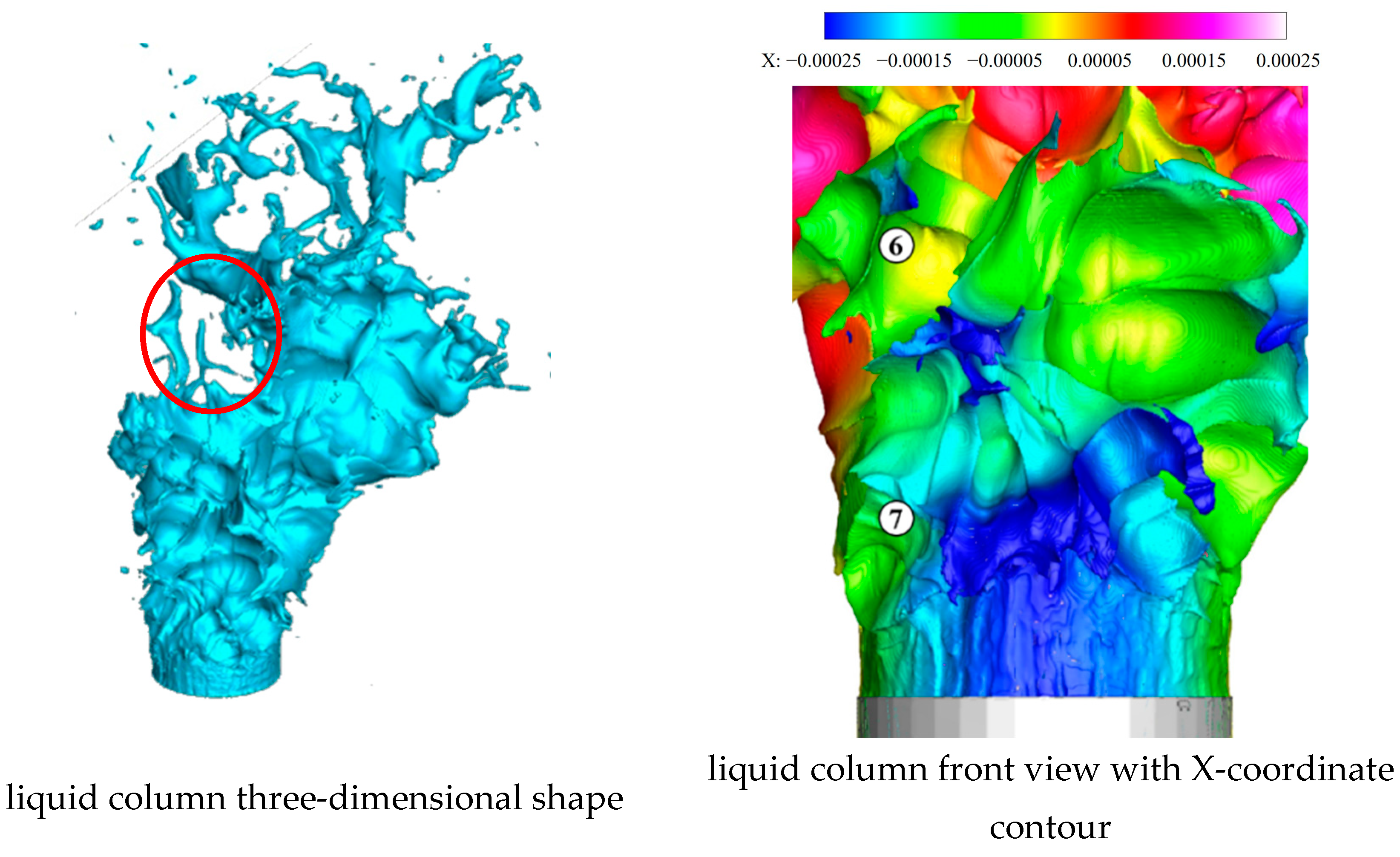




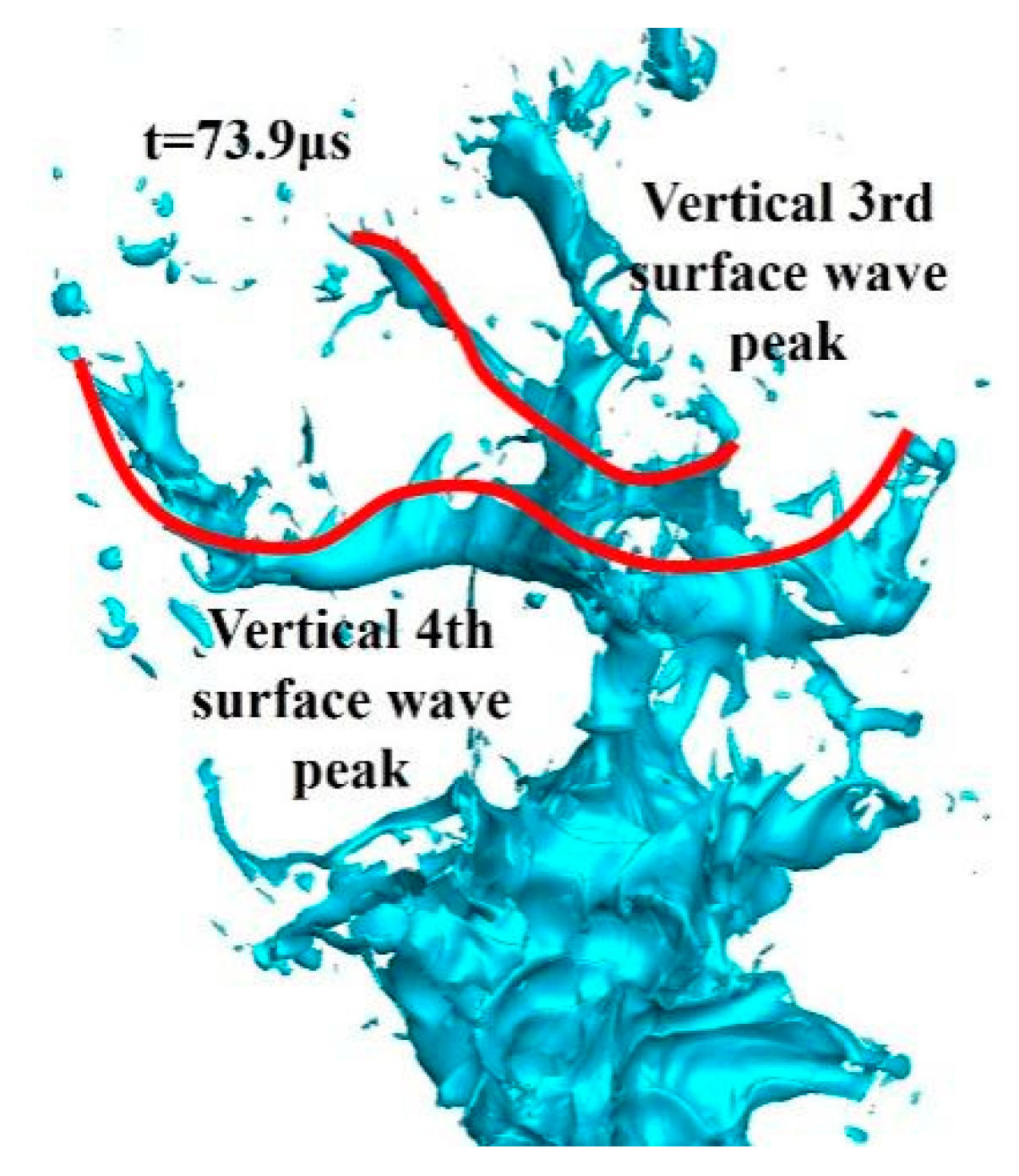










| Boundary | Type |
|---|---|
| Crossflow inlet | Pressure inlet |
| Liquid jet inlet | Velocity inlet |
| Wall | No slip condition |
| Symmetry | Symmetry |
| Outlet | Pressure outlet |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, D.; Chang, J.; Shan, H. Investigations of the Atomization Characteristics and Mechanisms of Liquid Jets in Supersonic Crossflow. Aerospace 2023, 10, 995. https://doi.org/10.3390/aerospace10120995
Zhou D, Chang J, Shan H. Investigations of the Atomization Characteristics and Mechanisms of Liquid Jets in Supersonic Crossflow. Aerospace. 2023; 10(12):995. https://doi.org/10.3390/aerospace10120995
Chicago/Turabian StyleZhou, Donglong, Jianlong Chang, and Huawei Shan. 2023. "Investigations of the Atomization Characteristics and Mechanisms of Liquid Jets in Supersonic Crossflow" Aerospace 10, no. 12: 995. https://doi.org/10.3390/aerospace10120995
APA StyleZhou, D., Chang, J., & Shan, H. (2023). Investigations of the Atomization Characteristics and Mechanisms of Liquid Jets in Supersonic Crossflow. Aerospace, 10(12), 995. https://doi.org/10.3390/aerospace10120995






