Numerical Simulation on Primary Breakup Characteristics of Liquid Jet in Oscillation Crossflow
Abstract
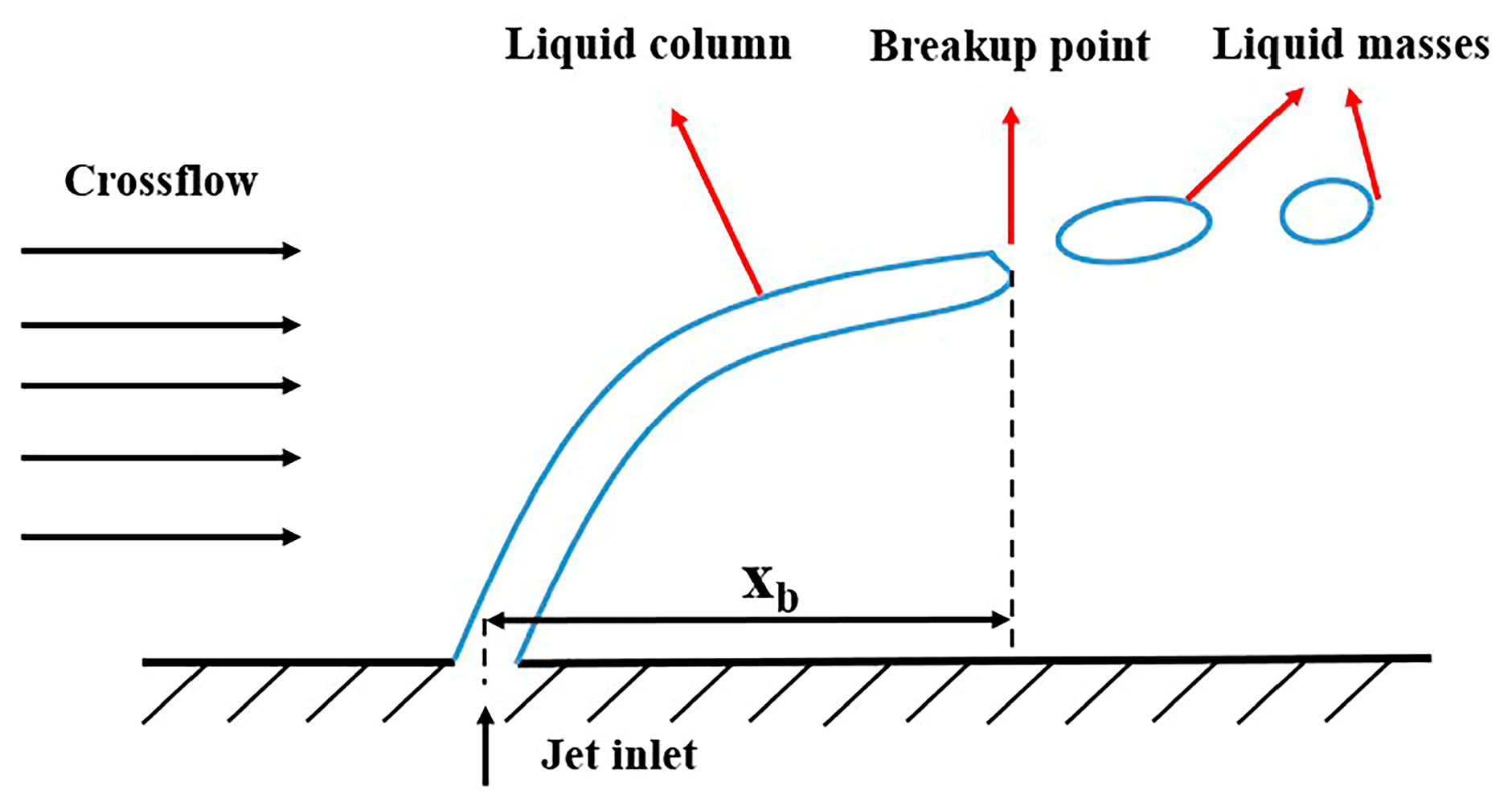
:1. Introduction
2. Mathematical Physical Model
2.1. Numerical Methods
2.2. Multiphase Flow Model
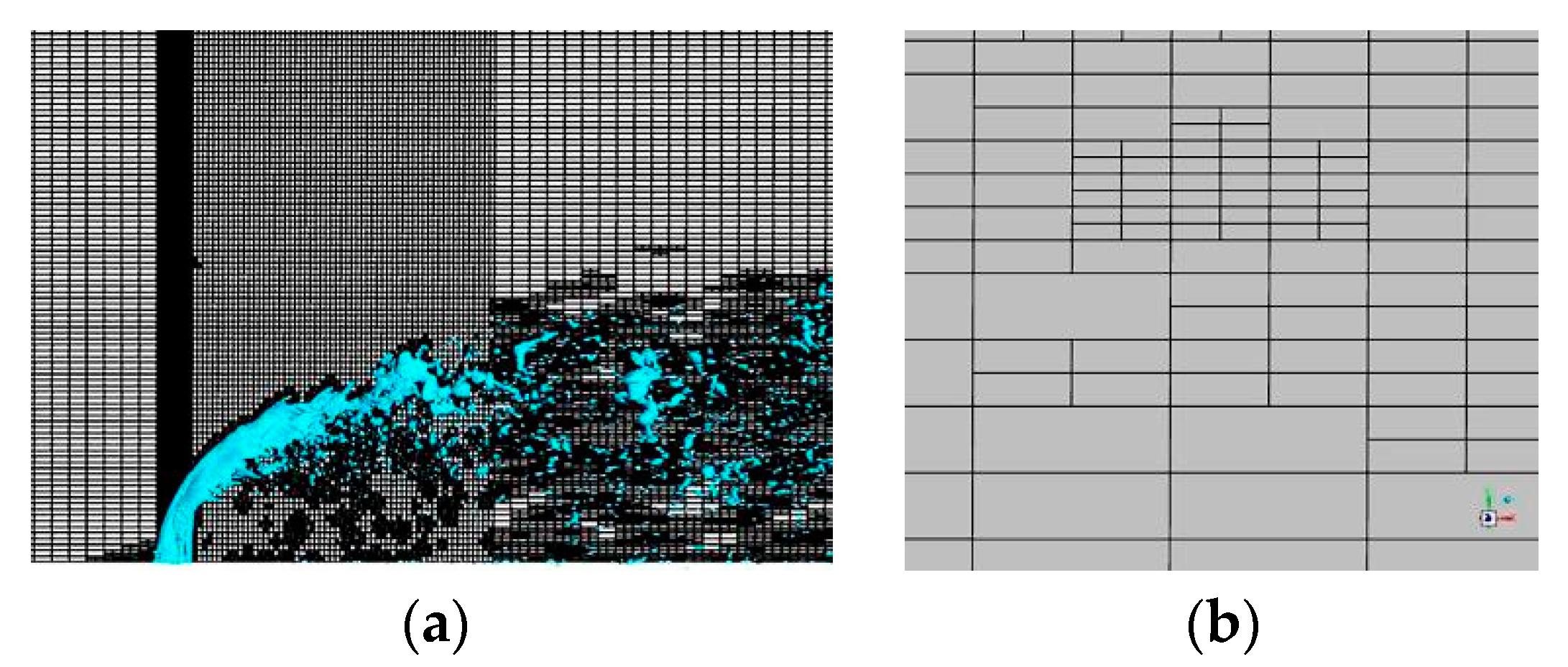
2.3. Computational Mesh
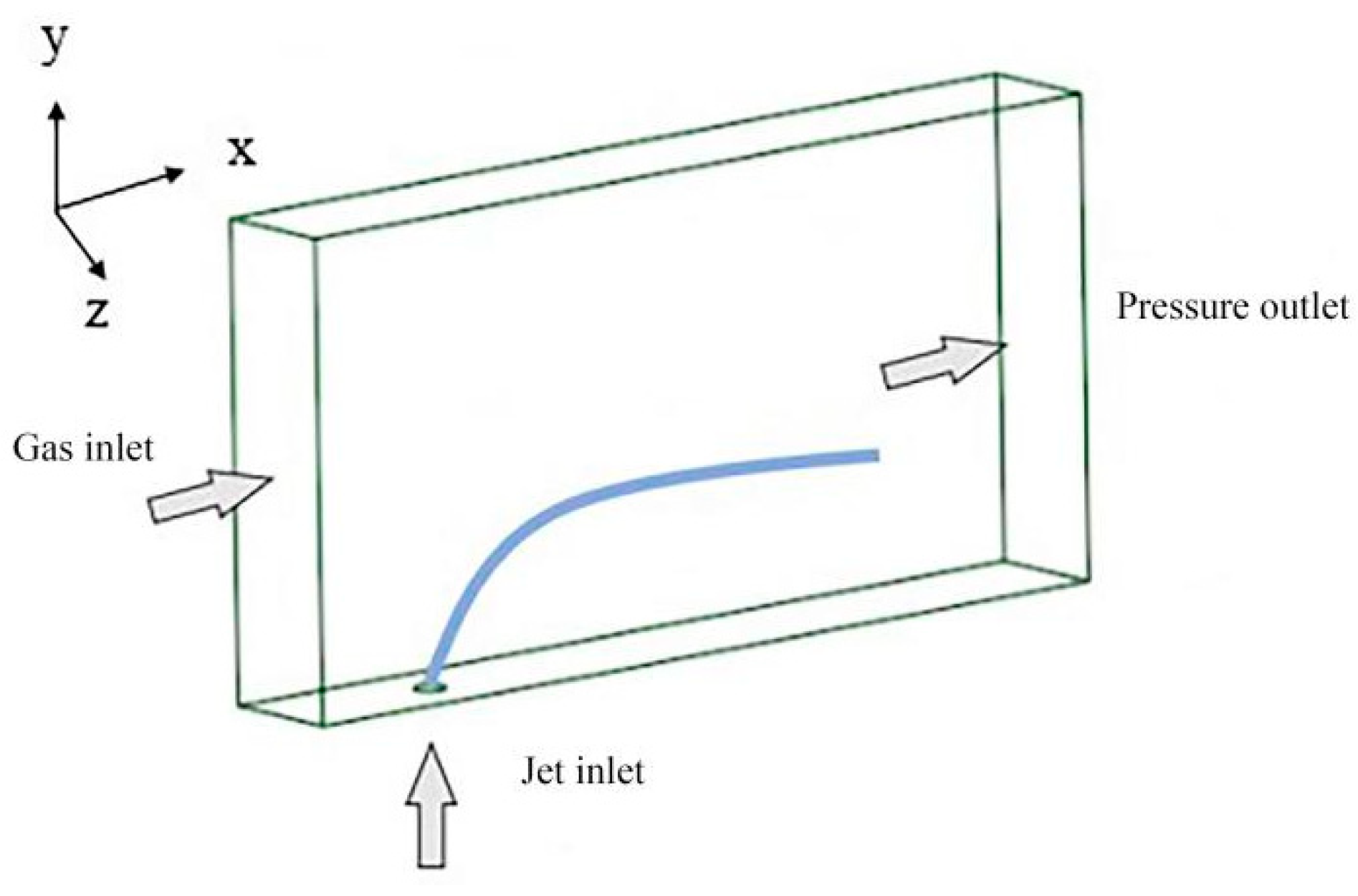
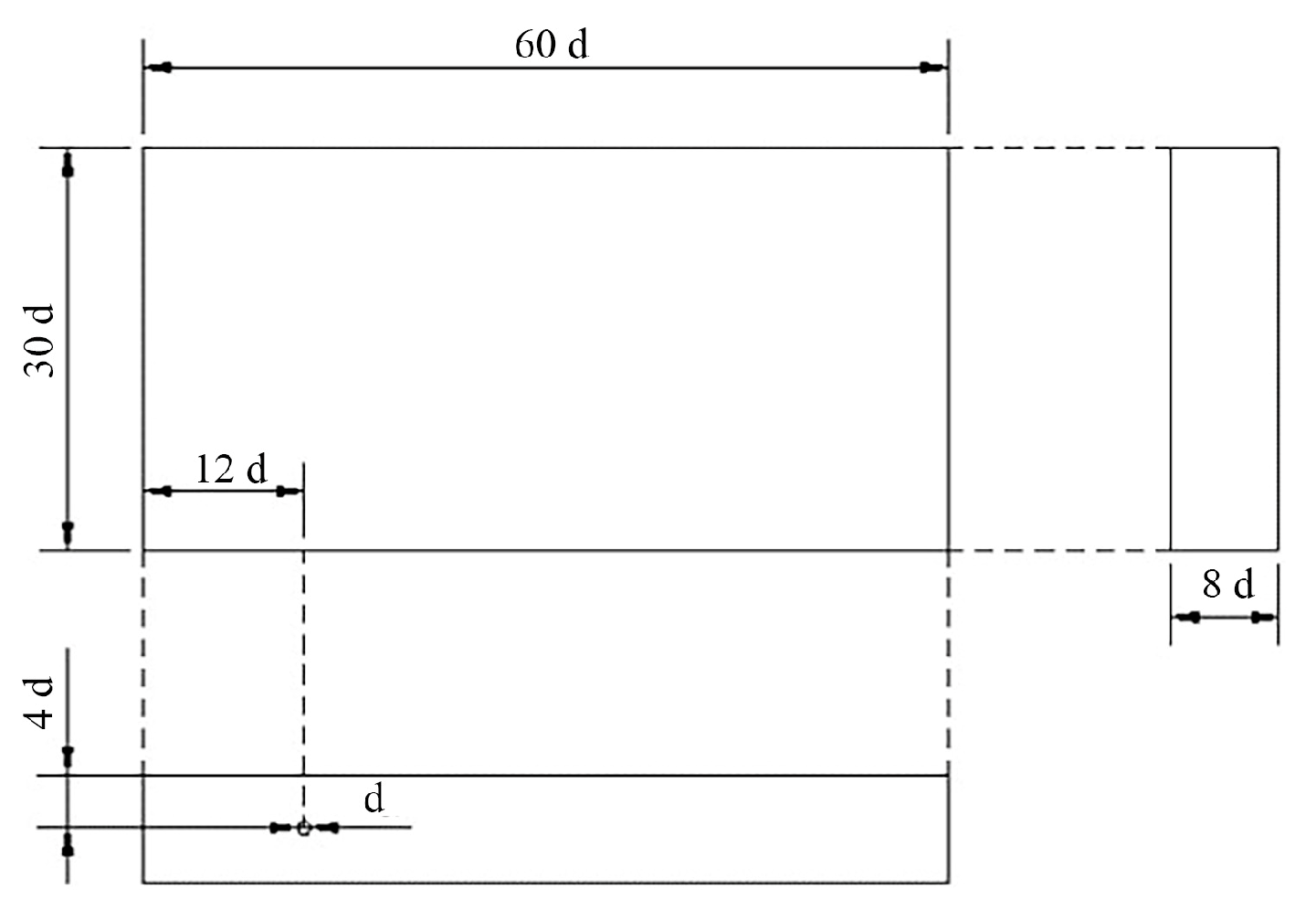
2.4. Physical Model and Boundary Conditions
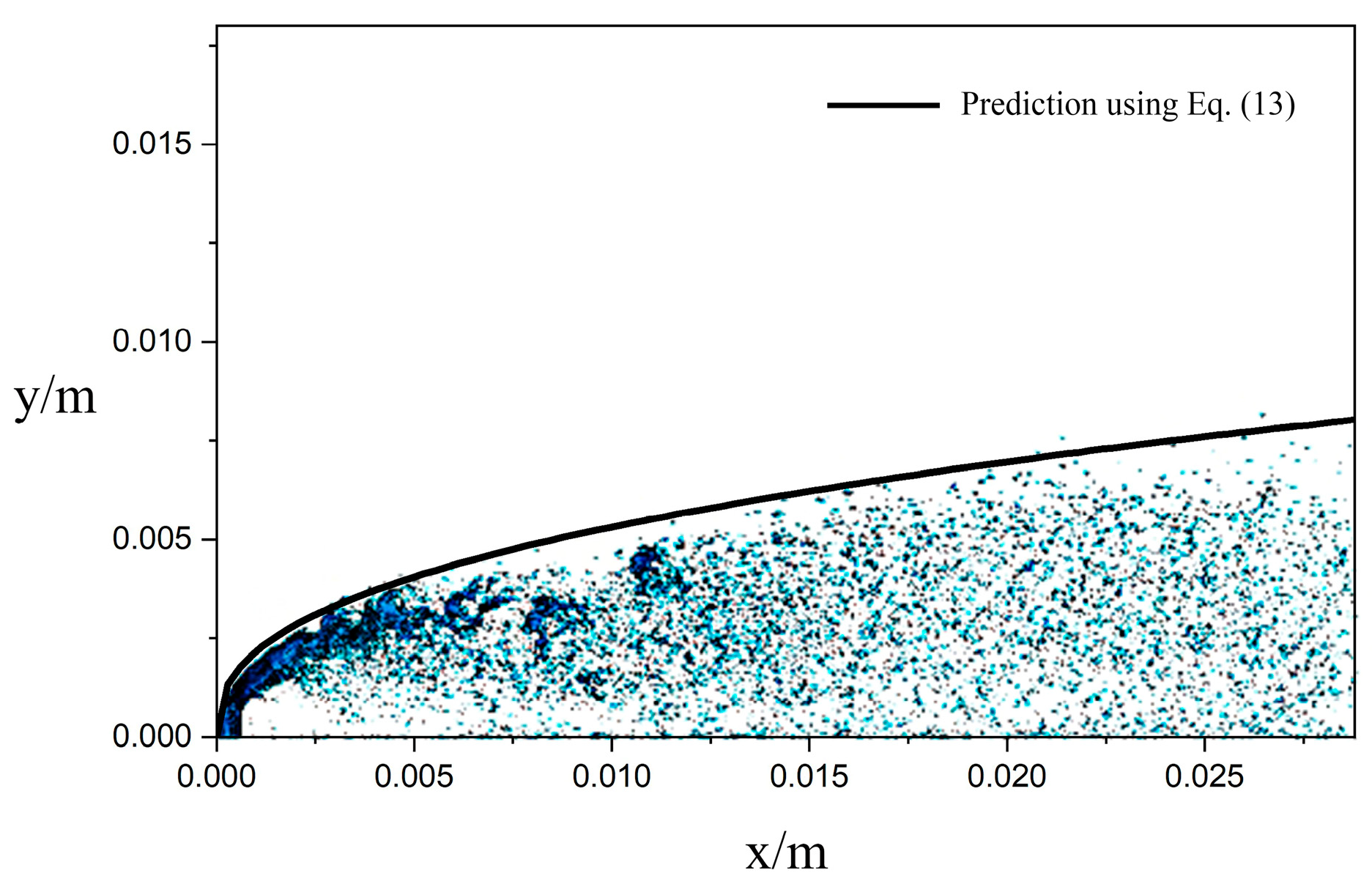
2.5. Verification of Calculation Results
3. Conclusions and Discussion
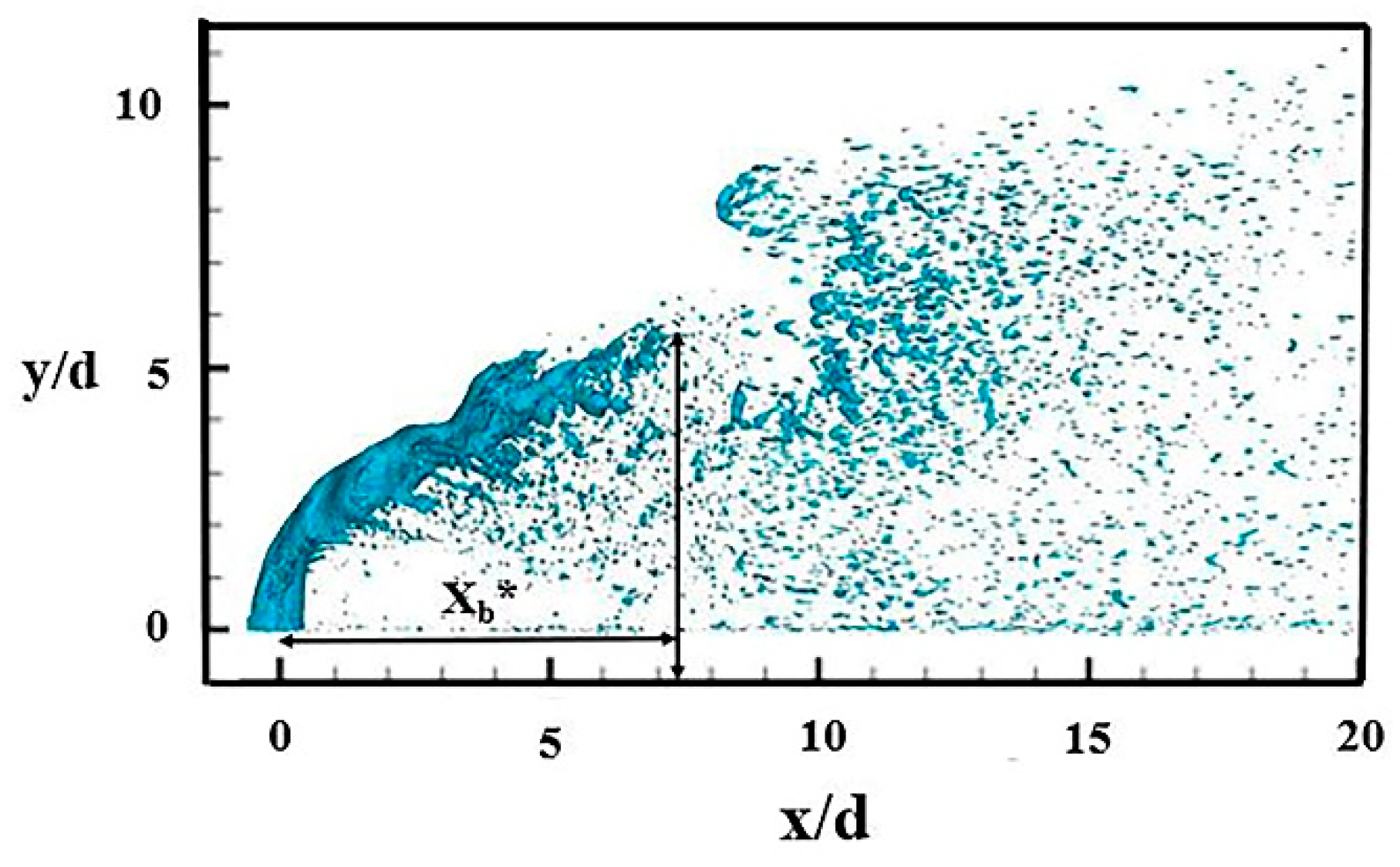
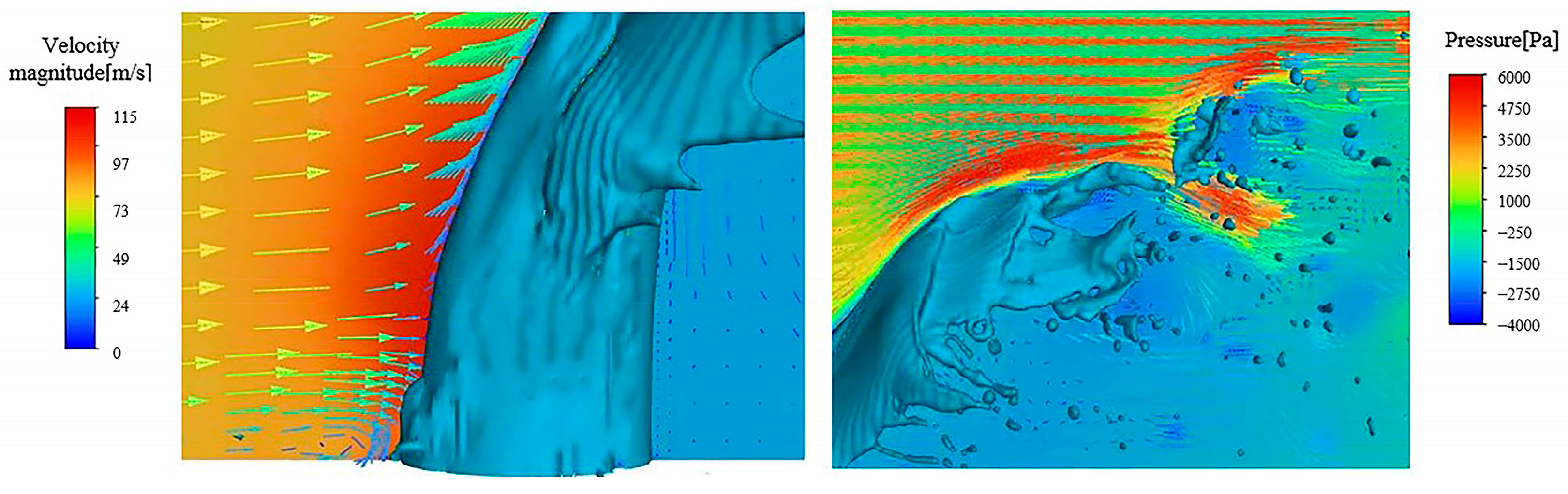
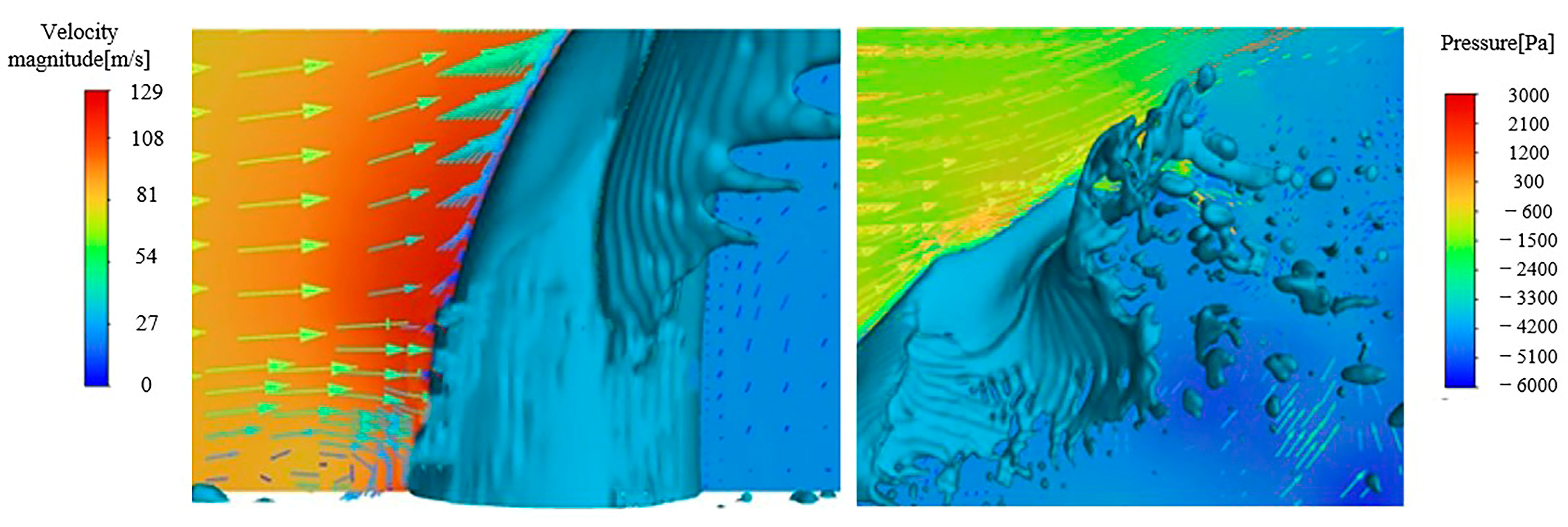
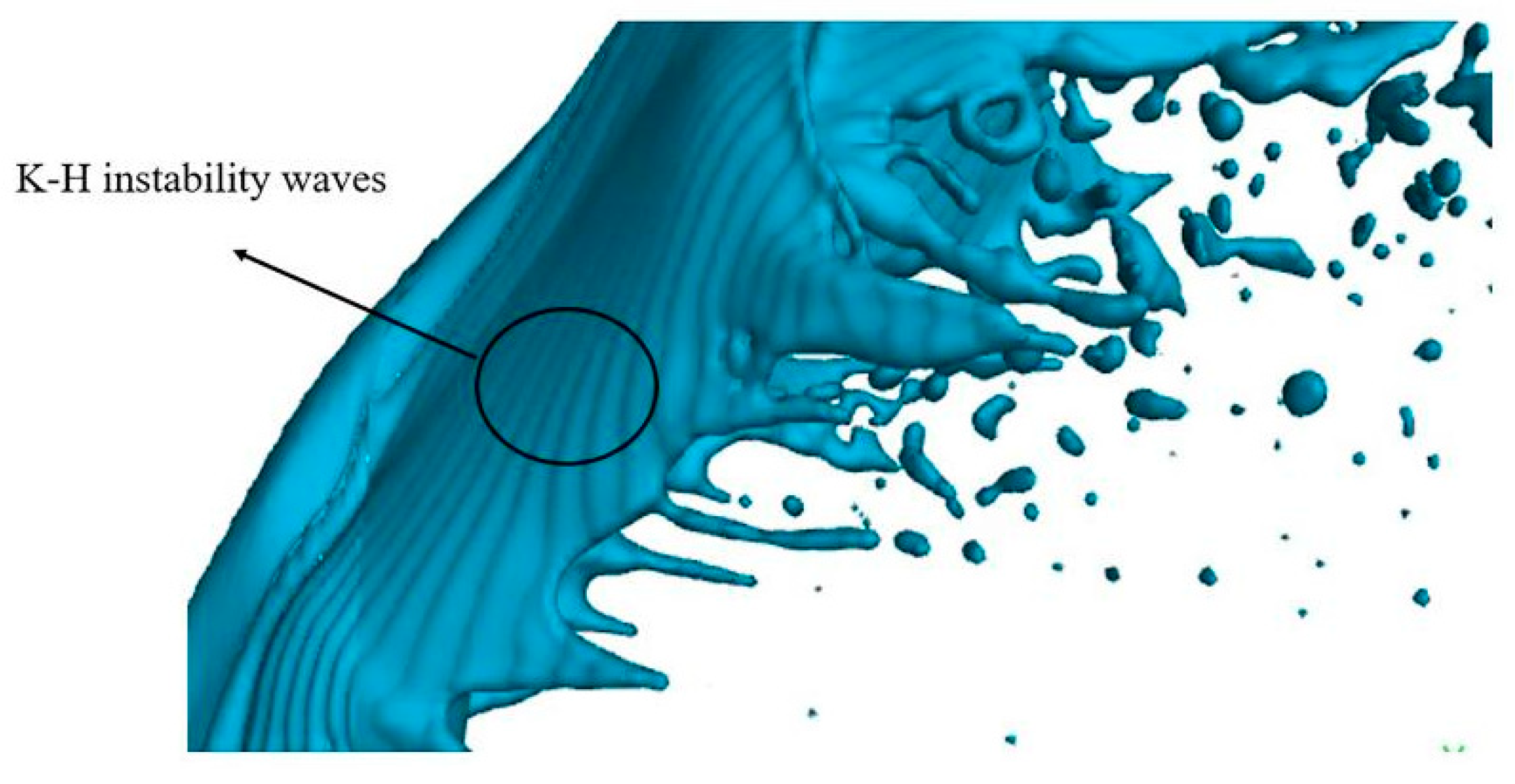
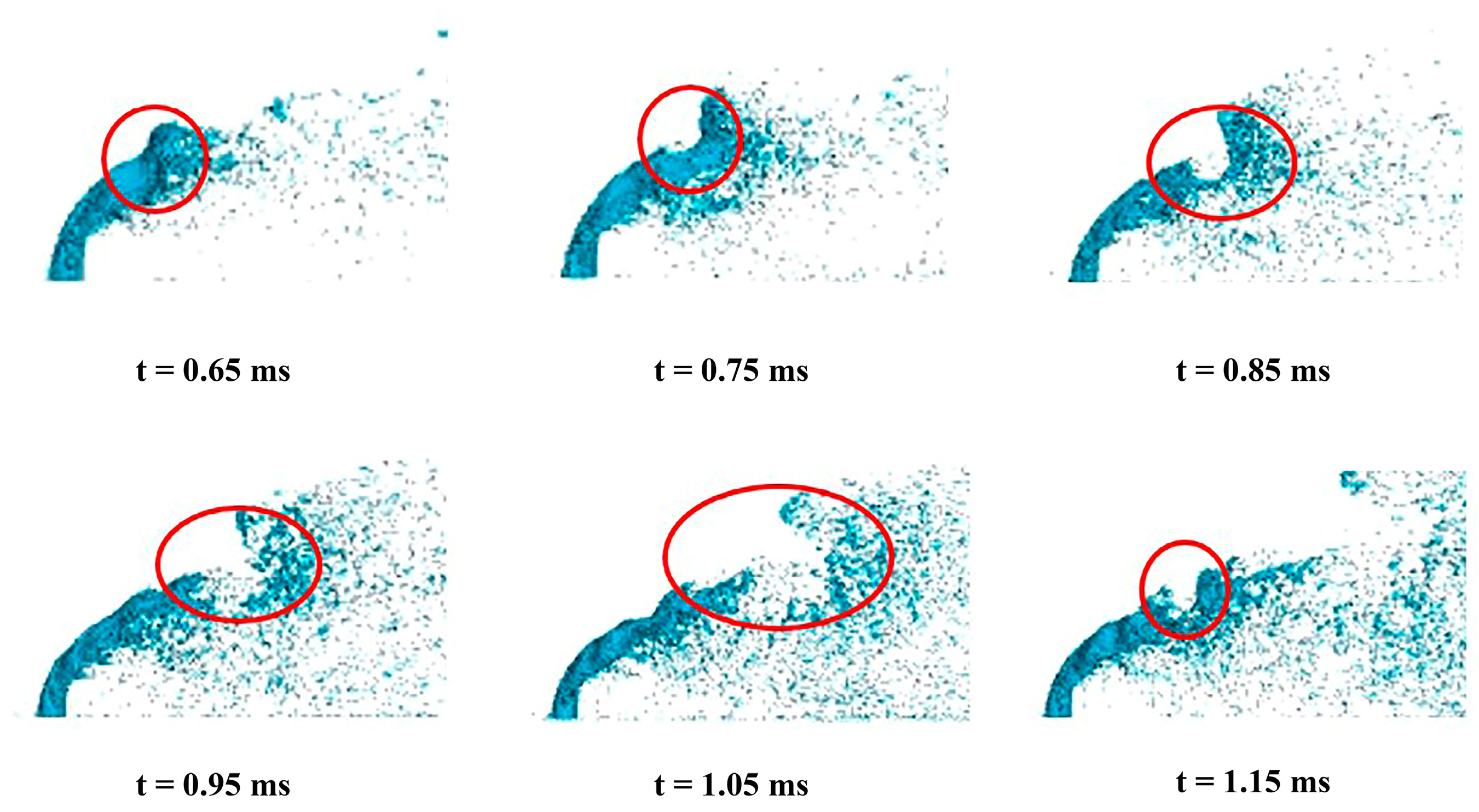
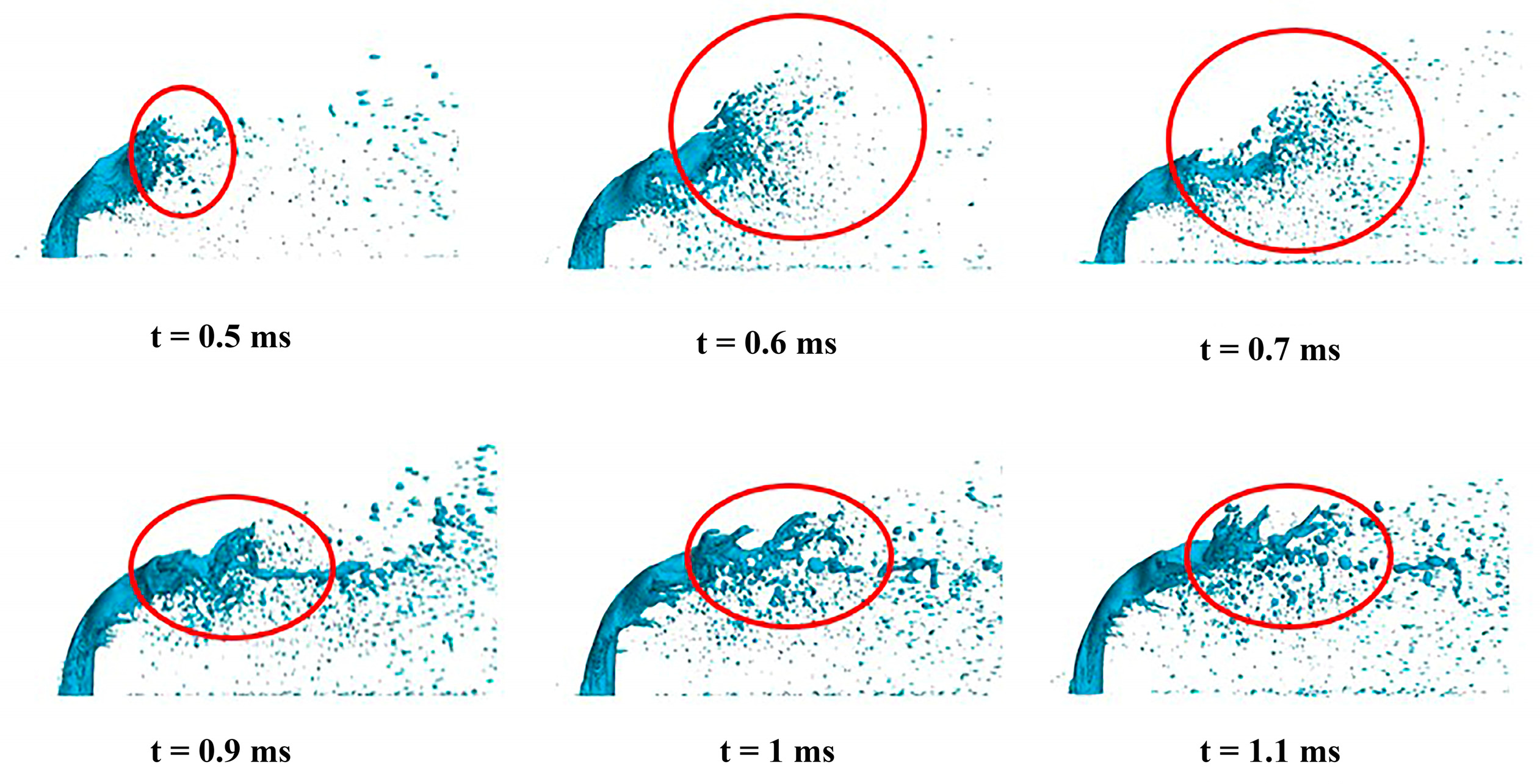
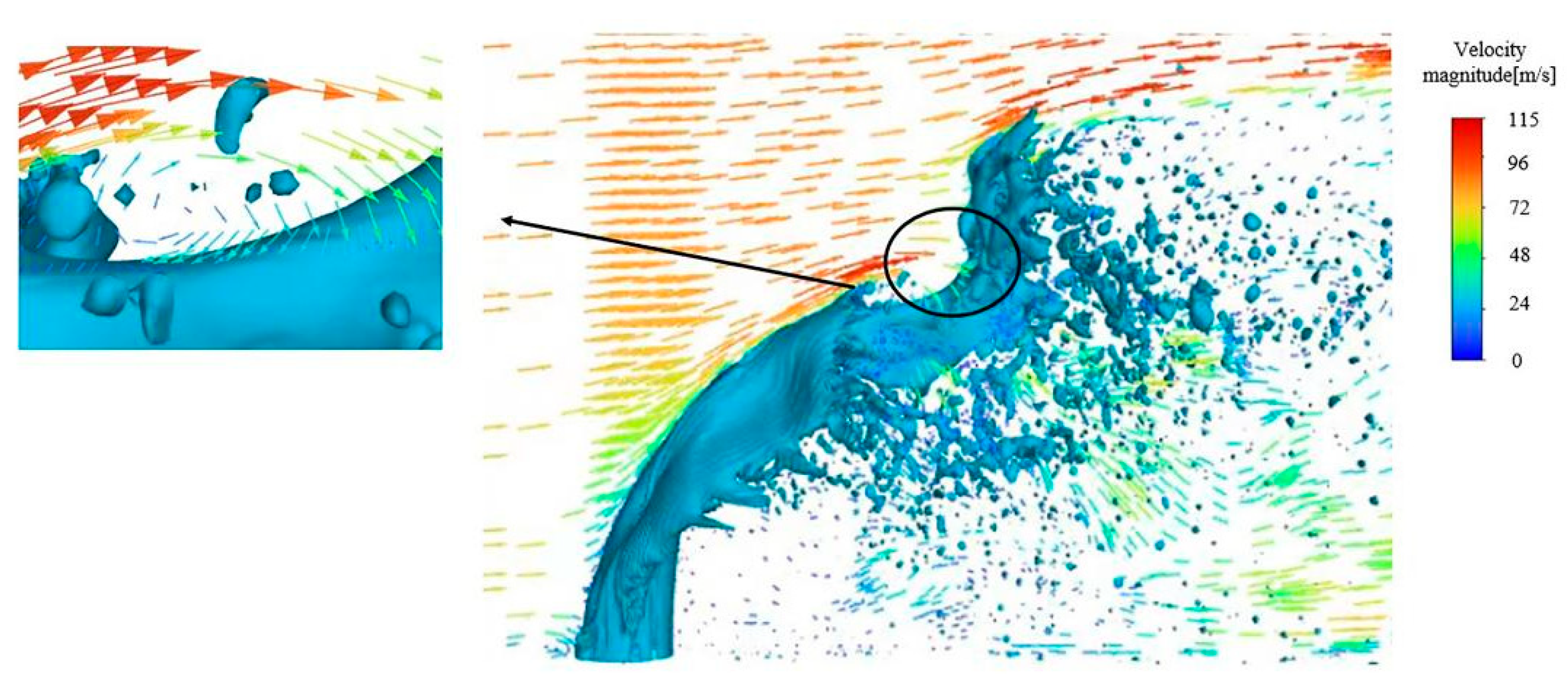
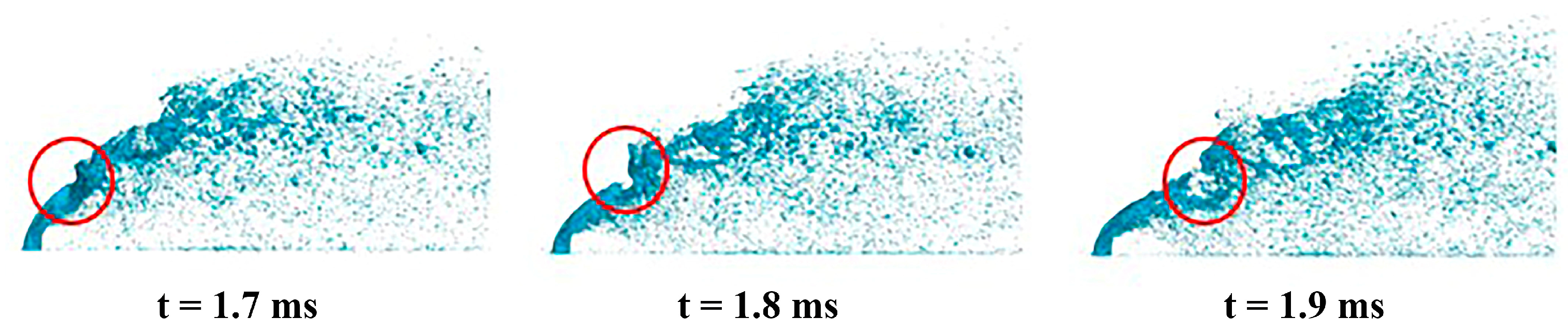
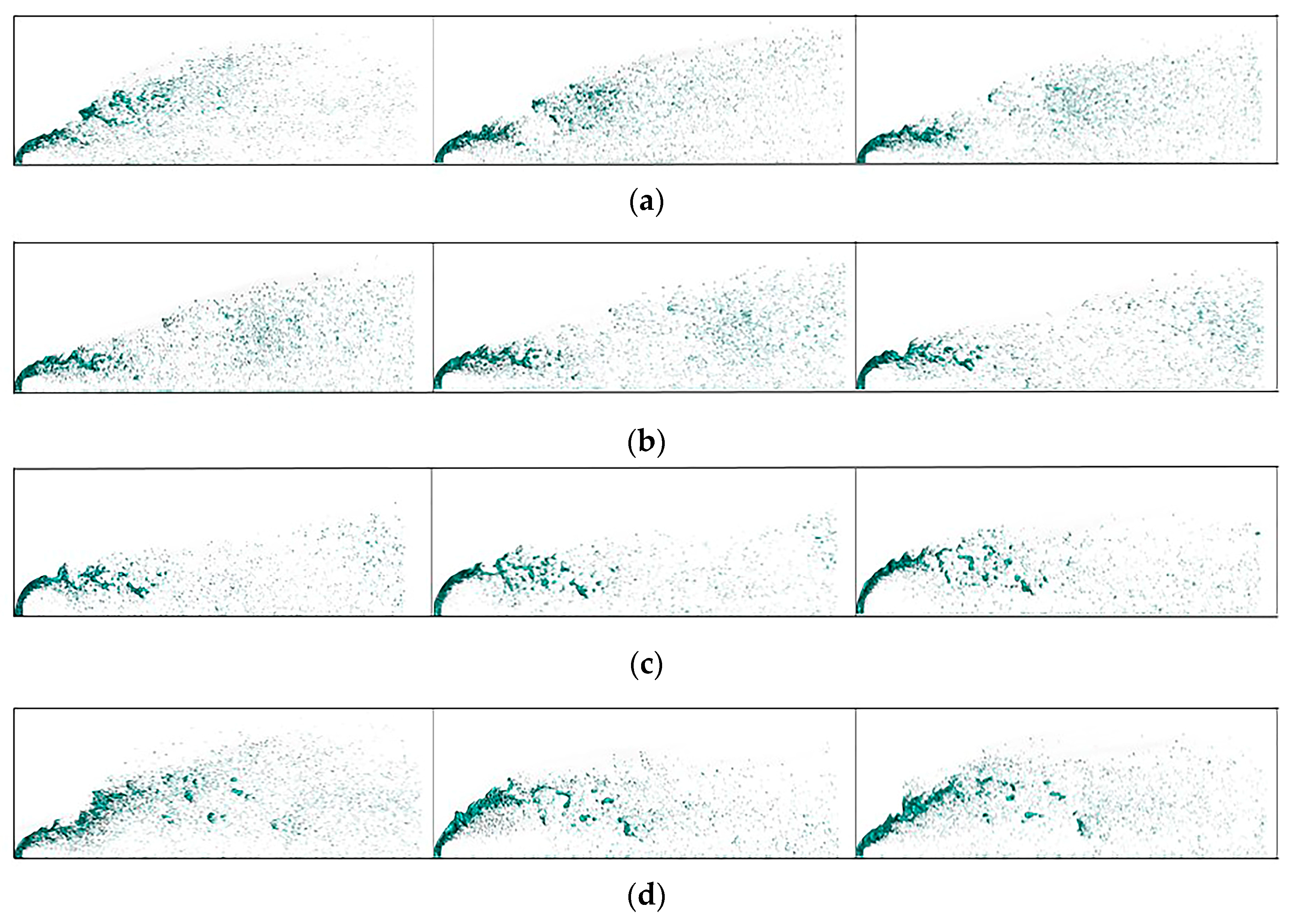
3.1. Jet Morphology
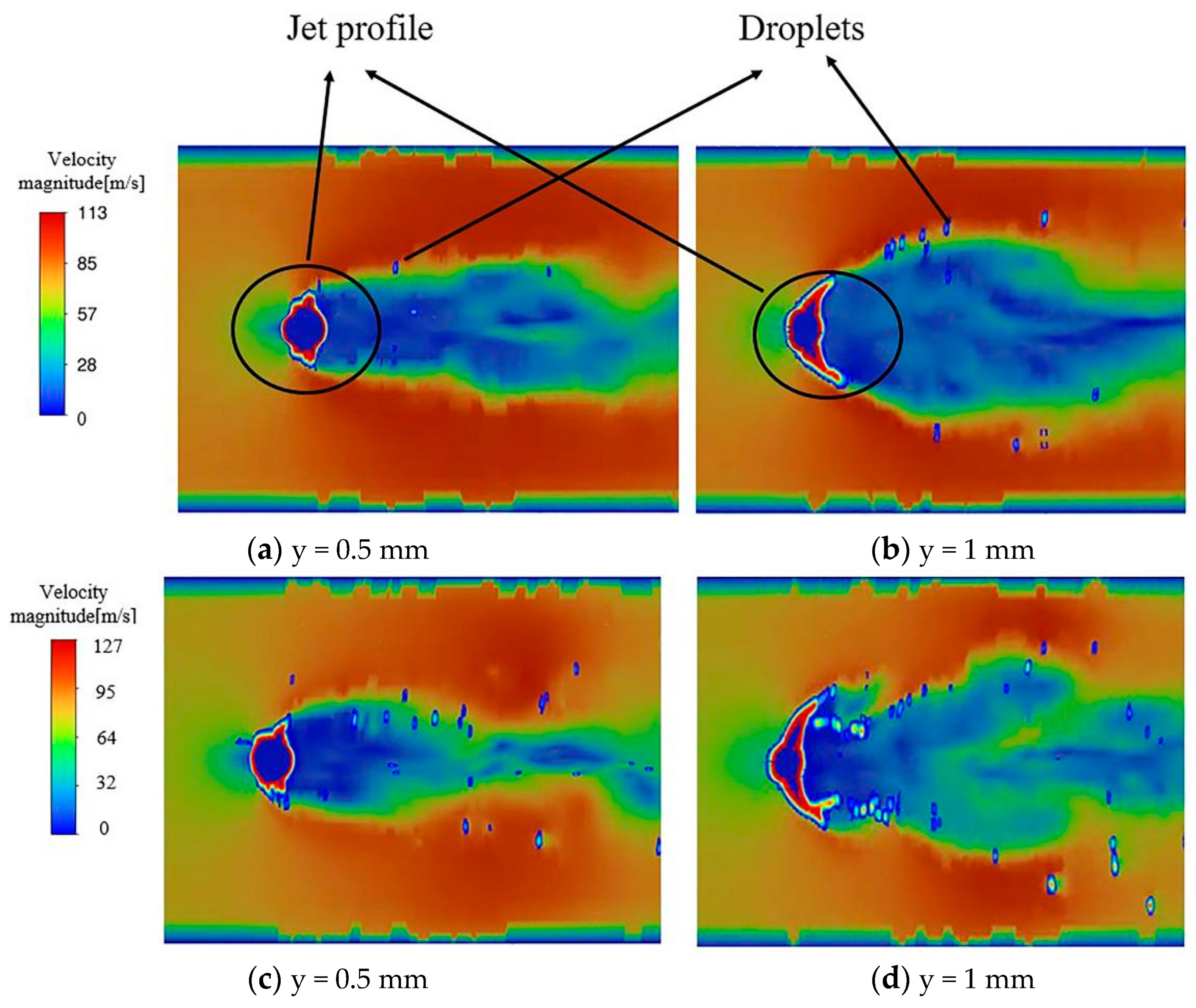
3.2. The Development of Oscillating Air Crossflow
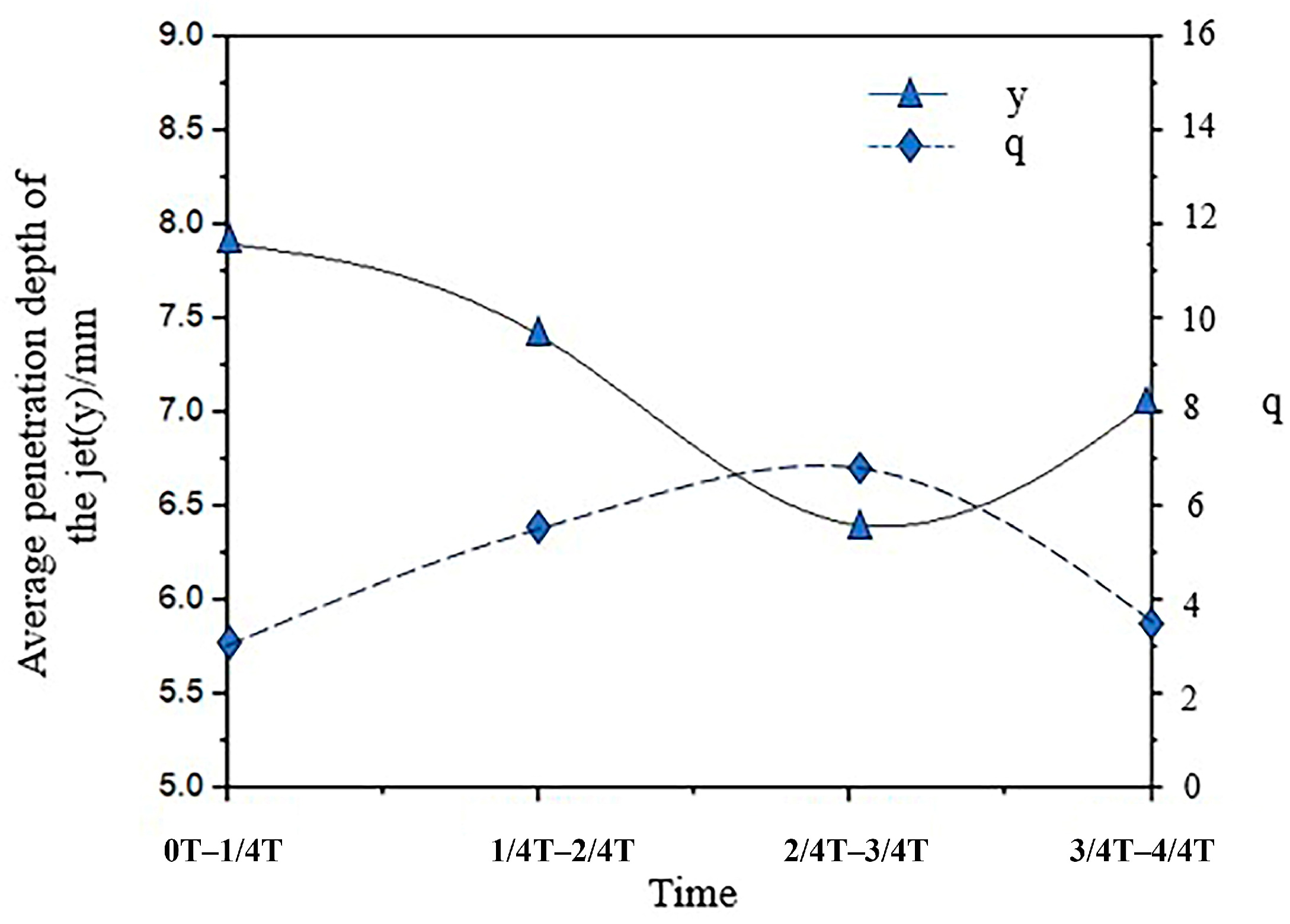
3.3. Penetration Depth
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Rezayat, S.; Farshchi, M.; Ghorbanhoseini, M. Primary breakup dynamics and spray characteristics of a rotary atomizer with radial-axial discharge channels. Int. J. Multiph. Flow 2019, 111, 315–338. [Google Scholar] [CrossRef]
- Mazallon, J.; Dai, Z.; Faeth, G. Aerodynamic primary breakup at the surface of nonturbulent round liquid jets in crossflow. In Proceedings of the 36th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 12–15 January 1998. [Google Scholar]
- Wu, P.K.; Kirkendall, K.A.; Fuller, R.P.; Nejad, A.S. Breakup Processes of Liquid Jets in Subsonic Crossflows. J. Propuls. Power 1997, 13, 64–73. [Google Scholar] [CrossRef]
- Schetz, J.A.; Padhye, A. Penetration and Breakup of Liquids in Subsonic Airstreams. AIAA J. 1977, 15, 1385–1390. [Google Scholar] [CrossRef]
- Lubarsky, E.; Shcherbik, D.; Bibik, O.; Gopala, Y.; Zinn, B.T. Fuel Jet in Cross Flow—Experimental Study of Spray Characteristics. In Advanced Fluid Dynamics; In Tech: London, UK, 2012. [Google Scholar]
- Xiao, F.; Sun, M.B. Effects of Mach number on liquid jet primary breakup in gas crossflow. At. Sprays 2018, 28, 975–999. [Google Scholar] [CrossRef]
- Zhao, J.; Ren, Y.; Tong, Y.; Lin, W.; Nie, W. Atomization of a liquid jet in supersonic crossflow in a combustion chamber with an expanded section. Acta Astronaut. 2021, 180, 35–45. [Google Scholar] [CrossRef]
- Sinha, A. Surface Waves on Liquid Jet in Crossflow: Effect of Injector Geometry. AIAA J. 2019, 57, 4577–4582. [Google Scholar] [CrossRef]
- Lee, S.; Kim, W.; Yoon, W. Spray formation by a swirl spray jet in low speed cross-flow. J. Mech. Sci. Technol. 2010, 24, 559–568. [Google Scholar] [CrossRef]
- Im, K.S.; Zhang, Z.C.; Cook, G.; Lai, M.C.; Chon, M.S. Simulation of Liquid and Gas Phase Characteristics of Aerated-Liquid Jets in Quiescent and Cross Flow Conditions. Int. J. Automot. Technol. 2019, 20, 207–213. [Google Scholar] [CrossRef]
- Lee, I.C.; Kang, Y.S.; Moon, H.J.; Jang, S.P.; Kim, J.K.; Koo, J. Spray jet penetration and distribution of modulated liquid jets in subsonic cross-flows. J. Mech. Sci. Technol. 2010, 24, 1425–1431. [Google Scholar] [CrossRef]
- Nygård, A.; Altimira, M.; Semlitsch, B.; Wittberg, L.P.; Fuchs, L. Analysis of Vortical Structures in Intermittent Jets. In Proceedings of the International Conference of Jets, Wakes and Separated Flows (ICJWSF2015), Stockholm, Sweden, 15–18 June 2015. [Google Scholar]
- Sharma, A.; Lee, J.G. Dynamics of Near-field and Far-field Spray Formed by Liquid Jet in Oscillating Crossflow. J. Int. Inst. Liq. At. Spray Syst. 2018, 28, 1–21. [Google Scholar] [CrossRef]
- Bunce, K.; Lee, J.; Santavicca, D. Characterization of Liquid Jets-In-Crossflow Under High Temperature, High Velocity Non-Oscillating and Oscillating Flow Conditions. In Proceedings of the 44th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 9–12 January 2006; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2006. [Google Scholar]
- Song, J.; Lee, J.G. Characterization of Spray Formed by Liquid Jet Injected Into Oscillating Air Crossflow. In Asme Turbo Expo: Turbine Technical Conference & Exposition; American Society of Mechanical Engineers: New York, NY, USA, 2015. [Google Scholar]
- Anderson, T.; Kendrick, D.; Cohen, J. Measurement of spray/acoustic coupling in gas turbine fuel injectors. In Proceedings of the 36th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 12–15 January 1998. [Google Scholar]
- Wen, J.; Hu, Y.; Kurose, R. Numerical simulation of kerosene jet in crossflow atomization and evaporation under the elevated pressure and oscillating air-flow condition. At. Sprays 2021, 31, 73–87. [Google Scholar] [CrossRef]
- Lee, I.; Kang, Y.; Koo, J. Mixing Characteristics of Pulsed Air-assist Liquid Jet into an Internal Subsonic Cross-flow. J. Therm. Sci. 2010, 19, 136–140. [Google Scholar] [CrossRef]
- Bodoc, V.; Desclaux, A.; Gajan, P.; Simon, F.; Illac, G. Characterization of Confined Liquid Jet Injected into Oscillating Air Crossflow. Flow Turbul. Combust. 2019, 116, 1–18. [Google Scholar] [CrossRef]
- Desclaux, A.; Thuillet, S.; Zuzio, D.; Senoner, J.M.; Sebbane, D.; Bodoc, V.; Gajan, P. Experimental and Numerical Characterization of a Liquid Jet Injected into Air Crossflow with Acoustic Forcing. Flow Turbul. Combust. 2020, 105, 1087–1117. [Google Scholar] [CrossRef]
- Brackbill, J.U.; Kothe, D.B. Dynamical modeling of surface tension. In Proceedings of the Third Microgravity Fluid Physics Conference, Cleveland, OH, USA, 13–15 June 1996. [Google Scholar]
- Ubbink, O.; Issa, R.I. A Method for Capturing Sharp Fluid Interfaces on Arbitrary Meshes. J. Comput. Phys. 1999, 153, 26–50. [Google Scholar] [CrossRef]
- Li, C.; Shen, C.B.; Li, Q.L. Primary breakup process of liquid jet in supersonic crossflow. J. Natl. Univ. Def. Technol. 2019, 41, 73–78. [Google Scholar]
- Hirt, C.W.; Nichols, B.D. Volume of fluid (VOF) method for the dynamics of free boundaries. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- Zhou, Y.; Li, Q.; Li, C. Study on Breaking Process of Liquid Jet in Supersonic Flow Based on Adaptive Mesh. J. Propuls. Technol. 2020, 41, 1571–1579. [Google Scholar]
- Zhang, B.; Cheng, P.; Li, Q.L.; Chen, H.Y.; Li, C.Y. Breakup process of liquid jet in gas film. Acta Phys. Sin. 2021, 70, 1–12. [Google Scholar] [CrossRef]
- Wang, Y.; Yan, Y.; Dang, L.; LI, J. Numerical investigation on atomization characteristics of liquid jet. J. Aerosp. Power 2016, 31, 2464–2471. [Google Scholar]
- Tachibana, S.; Saito, K.; Yamamoto, T.; Makida, M.; Kitano, T.; Kurose, R. Experimental and numerical investigation of thermo-acoustic instability in a liquid-fuel aero-engine combustor at elevated pressure: Validity of large-eddy simulation of spray combustion. Combust. Flame 2015, 162, 2621–2637. [Google Scholar] [CrossRef]
- Stenzler, J.N.; Lee, J.G.; Santavicca, D.A.; Lee, W. Penetration of Liquid Jets in a Crossflow. At. Sprays 2013, 16, 887–906. [Google Scholar] [CrossRef]
- Zandian, A.; Sirignano, W.A.; Hussain, F. Planar liquid jet: Early deformation and atomization cascades. Phys. Fluids 2017, 29, 062109. [Google Scholar] [CrossRef]
- Wan, Y.X.; Huang, Y.; Zhu, Y. Experiment on the breakup process of free round liquid jet. J. Aerodyn. 2008, 23, 208–214. [Google Scholar]
- Sallam, K.A.; Dai, Z.; Faeth, G.M. Liquid breakup at the surface of turbulent round liquid jets in still gases. Int. J. Multiph. Flow 2002, 28, 427–449. [Google Scholar] [CrossRef]



| Gas Inlet | Jet Inlet | |
|---|---|---|
| Medium | Air | Water |
| Density, ρ (kg/m3) | 1.225 | 998.2 |
| Kinematic viscosity, μ (Pa·s) | 1.7894 × 10−5 | 0.001003 |
| Liquid–gas momentum flux ratio (q) | 4.58 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, T.; Song, X.; Kai, X.; He, Y.; Li, R. Numerical Simulation on Primary Breakup Characteristics of Liquid Jet in Oscillation Crossflow. Aerospace 2023, 10, 991. https://doi.org/10.3390/aerospace10120991
Zhang T, Song X, Kai X, He Y, Li R. Numerical Simulation on Primary Breakup Characteristics of Liquid Jet in Oscillation Crossflow. Aerospace. 2023; 10(12):991. https://doi.org/10.3390/aerospace10120991
Chicago/Turabian StyleZhang, Tao, Xinyu Song, Xingping Kai, Yeguang He, and Rundong Li. 2023. "Numerical Simulation on Primary Breakup Characteristics of Liquid Jet in Oscillation Crossflow" Aerospace 10, no. 12: 991. https://doi.org/10.3390/aerospace10120991
APA StyleZhang, T., Song, X., Kai, X., He, Y., & Li, R. (2023). Numerical Simulation on Primary Breakup Characteristics of Liquid Jet in Oscillation Crossflow. Aerospace, 10(12), 991. https://doi.org/10.3390/aerospace10120991






