Numerical and Experimental Investigations to Assess the Impact of an Oil Jet Nozzle with Double Orifices on the Oil Capture Performance of a Radial Oil Scoop

Abstract

To study the influence of orifice spacing on the oil–air two-phase flow and the oil capture efficiency of an oil scoop in an under-race lubrication system, an experimental platform for under-race lubrication was built, and a calculation model for the oil–air two-phase flow field was established. The rationality of the experiment and the validity of the numerical model were verified by comparing the experimental and numerical results. The results showed that under the same oil supply pressure, the captured oil mass flow rate of the double-orifice structure was much higher than that of the single-orifice structure, though it was still less than twice that of the single-orifice structure. When applying a tandem layout structure of double orifices to an under-race lubrication system, the orifice spacing of the tandem layout structure should be determined based on a full evaluation of the influence of the orifice spacing and working condition parameters on the oil capture performance. Otherwise, it may lead to a decrease in oil capture efficiency, with the maximum reduction even reaching 12%.

1. Introduction

An aero-engine is a piece of high-speed rotating thermal machinery, and developments are being made to reduce their fuel consumption and increase their thrust weight ratio, reliability, and service life. As a key component of mechanical systems, rolling bearings need to operate stably in demanding working environments, such as those involving high speeds, high temperatures, and heavy loads. Efficient lubrication and cooling have become important conditions for ensuring the long service life and high reliability of bearings. The structure of direct jet lubrication is relatively simple, but some lubricant oil is atomized to a certain extent under high-speed operations. It is difficult for the lubricant oil to enter the bearing, so the lubrication and cooling of the contact area between the inner race and the rolling body is poor. Compared with jet lubrication, under-race lubrication has significant advantages in the lubrication and cooling of bearings; in particular, it effectively reduces the working temperature of the inner race of the bearing, facilitating the control of the working clearance of the bearing and significantly reducing the churning loss between the bearing components and the lubricant oil.

Existing studies have shown that under-race lubrication can meet the lubrication and cooling requirements of bearings with a DN value of 3 × 10⁶ mm·r/min, and that it is an effective way to solve the lubrication and cooling problems of high-speed bearings [1,2]. When under-race lubrication is used, bearing lubrication, friction heat generation, and temperature are no longer limiting factors for high-speed bearings [3,4]. The working process of under-race lubrication includes four stages: oil injection, oil capture, oil delivery, and lubrication and cooling. Due to structural and spatial limitations, it is difficult for the oil scoop to capture all the lubricant oil sprayed from the oil jet nozzle into the bearing, resulting in some rebound, fragmentation, and splash losses. Therefore, there is a problem with oil capture efficiency. Researchers have carried out a large number of numerical simulations and experimental studies on the internal flow and oil capture performance of under-race lubrication systems, aiming to reveal the complex oil–air two-phase flow mechanism of under-race lubrication and to find out the correlations between operating conditions, structural parameters, and oil capture performance.

Prasad et al. [5] carried out numerical simulations and experimental research on under-race lubrication systems in 2014, exploring the influences of working conditions and structural parameters on oil capture efficiency. Their results show that the oil capture efficiency increases as rotational speed increases, but decreases with further increases in speed after a certain speed is reached. Reducing the outer diameter of the oil scoop and increasing the oil flow rate and the axial width of the oil scoop improve the oil capture efficiency. Korsukova et al. [6] used a radial oil scoop containing six blades as their research object. They used the computational fluid dynamics (CFD) and smoothed particle hydrodynamics (SPH) methods to predict the oil capture efficiency of the oil scoop. The error between the oil capture efficiency values obtained using the two methods and the test results was less than 15% in the whole speed range. Prabhakar et al. [7] used the two-dimensional CFD method to carry out numerical calculation research on the oil–air two-phase flow in an under-race lubrication structure. Their results show that the pressure fluctuation near the tip of the blade causes the oil jet to deflect and form a plume. To reduce the oil loss caused by the plume, the direction of the oil jet injection was adjusted to the rotation direction of the oil scoop, and this almost eliminated the deflection of the oil jet and the plume, though the oil capture efficiency was reduced by 10%. Prabhakar and Abakr et al. [8] focused on the relationship between oil capture efficiency and rotational speed. Their results show that the oil loss caused by the centrifugal effect is related to the position of the oil jet, which impacts the oil scoop blade. The closer the impact point is to the tip of the oil scoop blade, the greater the oil loss. At higher rotational speeds, reducing the jet angle will cause the plume loss to become more significant. Simmons et al. [9] proposed a method to improve oil capture efficiency by using an oil jet. When the flow rate of the trailing-edge jet is large, part of the oil deflected by the trailing-edge jet can be re-captured by the oil scoop blade. When the flow rate of the trailing-edge jet is large, the leading-edge jet can buffer the trailing-edge jet. Paleo et al. [10] conducted experiments to compare the effects of single-jet, dual-jet, and tandem-jet systems on oil capture efficiency. The difference in oil capture efficiency between the dual-jet and tandem-jet systems was very small, though both exhibited higher oil capture efficiency than the single-jet system.

Prabhakar [11] found that the flow capacity of the oil capture passage increases as the outlet pressure decreases. When the pressure difference is 0.18 bar, the oil capture efficiency increases by 7%. As the rotational speed increases, it is necessary to reduce the number of oil scoop blades to achieve higher oil capture efficiency. Kruisbrink et al. [12] proposed the “splash criterion” and “capture criterion” to describe the operating conditions of the oil jet impacting the oil scoop blade. Their results indicate that the optimal oil capture efficiency is related to the splash criterion, and the trend of the optimal oil capture efficiency can be well described by the splash criterion. Korsukova and Morvan [13] found that when an oil jet impinges on the outer surface profile of an oil scoop blade tip, the oil capture efficiency significantly decreases. When the lubricant oil jet impacts the rear of the outer surface contour of the oil scoop blade, the oil capture efficiency will be improved. Lee et al. [14] compared the oil capture efficiency of radial oil scoops with straight and curved blades. They found that the oil capture efficiency values corresponding to the curved and straight blades showed consistent trends related to variations in rotational speed. Compared with straight blades, curved blades provide a very limited overall improvement in oil capture efficiency.

Jiang and Lyu et al. studied the oil capture performance of a radial oil scoop using the numerical method. It was found that the higher the rotational speed, the greater the wind resistance, which is not conducive to the oil scoop blade cutting the lubricant oil jet [15]. When the oil velocity was in the range of 10–30 m/s, the oil capture efficiency presented three kinds of changes in the process of gradually increasing the rotational speed: a monotonic decline, an initial increase and then a decrease, and a monotonic increase. However, the amount of captured oil, which is determined by the oil capture efficiency and the flow rate of the oil supply, increased monotonically under all operating conditions [16]. Qin et al. [17] experimentally studied the effects of rotational speed, oil jet nozzle diameter, and oil temperature on oil capture efficiency. It was found that increasing the diameter of the oil jet nozzle and increasing the oil temperature could improve the oil capture efficiency. They also proposed a radial oil scoop with curved blades and solved the curve equation of the inner surface of the scoop blade based on the principle of relative velocity optimization. Their numerical calculation results showed that the oil scoop with curved blades had a 30% higher oil capture efficiency than the original structure [18].

In summary, some progress has been made in the research on the oil capture efficiency of under-race lubrication systems. Studies have been conducted on the effect of an oil jet nozzle with double orifices on the oil capture performance of an under-race lubrication system, but the effects of orifice spacing on the oil–air two-phase flow inside the under-race lubrication structure and oil capture performance have not yet been evaluated. In this paper, an under-race lubrication test device was set up according to the structural characteristics and test requirements of an aero-engine. At the same time, an unsteady two-phase flow model was established to accurately capture the characteristics of the oil–air flow. A comparison and analysis of typical experimental data and numerical simulation results confirmed the rationality of the experimental scheme and the effectiveness of the numerical model. Furthermore, the variation trends of oil capture efficiency were obtained and summarized through experiments, and numerical simulations were conducted to reveal the underlying mechanisms behind the oil capture characteristics. This provides a research foundation and support for the optimization design of an under-race lubricated bearing.

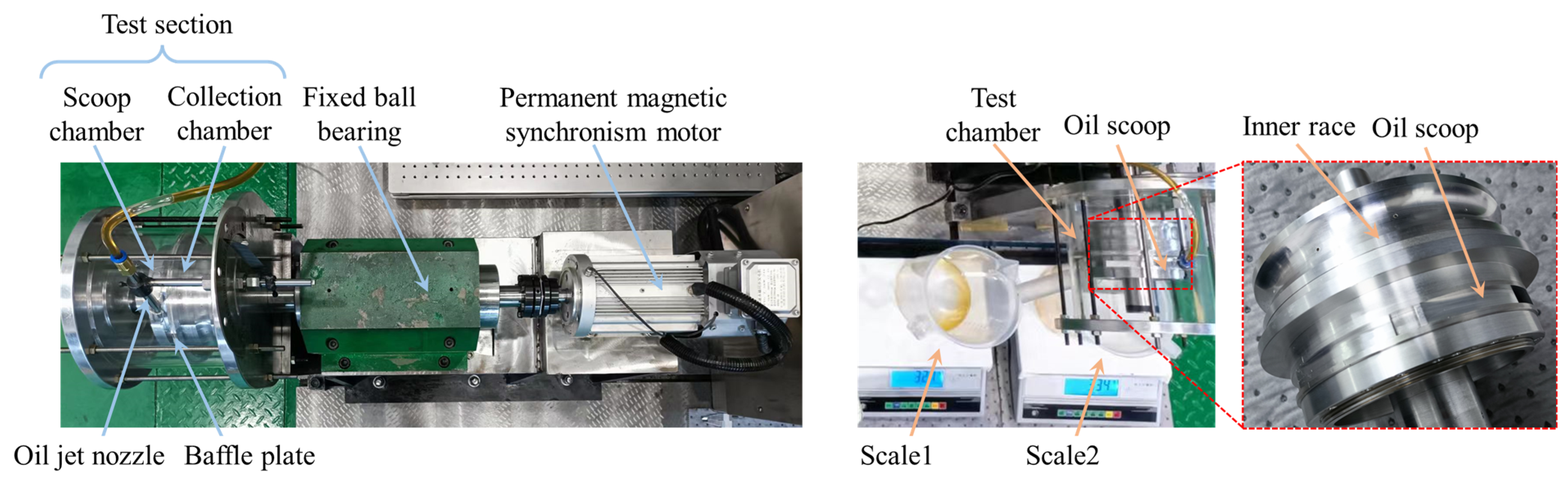
2. Experimental Setup

Figure 1 shows the experimental setup for the radial under-race lubrication system. In the experiment, the radial oil scoop and the inner race of the bearing are driven by a high-speed synchronous permanent magnet motor, and the rotational speed is adjusted through a frequency converter. During the test, the oil supply system pressurizes the lubricant oil and provides clean lubricant oil at an adjustable pressure and flow rate to the test chamber through the oil jet nozzle. The lubricant oil entering the bearing is completely separated from the lubricant oil not collected by the oil scoop through a baffle in the test chamber. The lubricant oil in the scoop chamber and the collection chamber flows through the return port to the oil collection cup, and the lubricant oil flow rate is calculated via real-time monitoring of the quality in the oil collection cup. All measurement parameters, such as rotational speed, oil supply pressure, oil temperature, oil supply flow rate, and captured oil mass, are recorded using the data collection system.

During the test, the parameters of each working condition are adjusted to ensure the stable operation of the system, and the changes in the oil return quality on both sides are recorded with the corresponding time. After the oil quality has maintained a linear increase, the oil return flow rate is recorded and the oil capture efficiency is calculated. The oil capture efficiency is calculated using the following formula:

$$\overline{\eta }=\frac{{\dot{m}}_c}{{\dot{m}}_{jet}}$$

(1)

where ${\dot{m}}_c$ is the oil flow rate captured by the oil scoop and ${\dot{m}}_{jet}$ is the oil supply flow rate sprayed out by the oil jet nozzle.

3. Mathematical Modeling

3.1. Computational Domain and Mesh

Complex oil–air two-phase flow phenomena, such as jet breakup and splashing, occur during the process of capturing lubricant oil with the radial oil scoop [6,15]. To accurately predict the two-phase flow using a three-dimensional numerical simulation, high mesh resolution and almost unacceptable computational costs are required. Korsukova and Morvan have compared semi-3D and two-dimensional numerical simulations, and their results show that both models can reflect typical two-phase flow characteristics inside an under-race lubrication system [13]. In addition, some studies have also proved that two-dimensional numerical simulations can reasonably predict the internal flow and oil collection performance of an under-race lubrication system [6,7,8,9,11]. To improve the calculation efficiency while ensuring prediction accuracy, a two-dimensional numerical calculation model was established for the middle section containing the main structural features of the oil jet nozzle and the radial oil scoop, as is shown in Figure 2. In contrast with the real structure, the oil captured by the radial oil scoop cannot flow along the axis. Therefore, an outlet was set at the blade root of each oil scoop blade, and it was assumed that all the oil flowing out of the outlet would be able to enter the bearing. In addition, an outlet was provided in the external static computational domain to provide a reasonable outlet flow boundary for the broken and splashed lubricant oil.

Figure 3 shows the two-dimensional computational mesh of the radial under-race lubrication system. Both the internal rotation domain and the external stationary computational domain are divided into a quadrilateral structured mesh. To accurately capture the flow details of the oil jet impinging on the surface of the oil scoop blade and along the wall surface, the grid of the boundary layer near the wall surface of the radial oil scoop is refined to ensure that the dimensionless grid height (y⁺) of the first layer is less than 5. At the same time, to reduce the computational errors caused by the use of interpolation methods for flow information transfer between different computational domains, the mesh size consistency on both sides of the interface is ensured as much as possible.

3.2. Two-Phase Flow and Turbulence Models

The lubricant oil is supplied to the oil scoop through the oil jet nozzle in the under-race lubrication structure, and the oil jet will deflect and break in the high-speed air flow near the oil scoop. The oil entering the oil scoop passage flows close to the wall under the action of high-speed rotating centrifugation. The position and shape of the oil–air interface always deforms, breaks, merges, etc. On the whole, a study of the oil–air two-phase flow in the under-race lubrication system needs to accurately capture the different motion patterns of the lubricant oil through phase interface tracking. The performance of the VOF method has been fully evaluated in the simulation of liquid jet breakup [19,20] and unsteady oil–gas two-phase flow in a bearing cavity [21,22].

The VOF method proposed by Hirt and Nichols [23] for tracking gas–liquid interfaces involves introducing the phase volume fraction to represent the percentage of each phase occupying the grid unit area or volume and calculating the position and direction of the gas–liquid interface using the phase volume fraction.

A gas–liquid interface exists within grid cells with a phase volume fraction of 0–1. For the calculation of the oil–air two-phase flow, without considering mass transfer, the continuity equation of the lubricant oil phase is as follows:

$$\frac{1}{{\rho }_{\mathrm{oil}}}\left(\frac{\partial }{\partial t}\left({\alpha }_{\mathrm{oil}}{\rho }_{\mathrm{oil}}\right)+\nabla \cdot \left({\alpha }_{\mathrm{oil}}{\rho }_{\mathrm{oil}}\overrightarrow{v}\right)\right)=0$$

(2)

where $\overrightarrow{v}$ represents the velocity and ${\alpha }_{\mathrm{oil}}$ and ${\rho }_{\mathrm{oil}}$ represent the volume fraction and density of the oil, respectively. In any grid cell, the sum of the volume fractions of each phase is 1, and the volume fraction of the air phase is calculated according to the following formula:

$${\alpha }_{\mathrm{oil}}+{\alpha }_{\mathrm{air}}=1$$

(3)

In the under-race lubrication system, the oil scoop and the main shaft rotate synchronously at high speed to form a strong rotational turbulent flow, which in turn affects the flow of the lubricant oil jet. Therefore, the accurate modeling of turbulent flow is the basis of accurately predicting the flow field inside an under-race lubrication system. Prabhakar et al. used the RNG k-ε turbulence model to predict the oil–air two-phase flow in a study of radial under-race lubrication and obtained results consistent with those obtained in their experiment [7,8]. The RNG k-ε turbulence model proposed by Yakhot and Orzag was selected after considering the required computer resources and the application range of the turbulence model. The transport equations for turbulent kinetic energy and turbulent energy dissipation rate are as follows [24]:

$$\frac{\partial }{\partial t}\left(\rho k\right)+\frac{\partial }{\partial x_i}\left(\rho k{u}_i\right)=\frac{\partial }{\partial x_j}\left({\alpha }_k{\mu }_{eff}\frac{\partial k}{\partial x_j}\right)+G_k-\rho \epsilon$$

(4)

$$\frac{\partial }{\partial t}\left(\rho \epsilon \right)+\frac{\partial }{\partial x_i}\left(\rho \epsilon u_i\right)=\frac{\partial }{\partial x_j}\left({\alpha }_{\epsilon }{\mu }_{eff}\frac{\partial \epsilon }{\partial x_j}\right)+\frac{C_{1\epsilon }\epsilon }{k}{G}_k-C_{2\epsilon }\rho \frac{{\epsilon }^2}{k}$$

(5)

where $G_k$ is the generation term of the turbulent kinetic energy caused by the average velocity gradient and ${\alpha }_k$ and ${\alpha }_{\epsilon }$ are the reciprocal of the Prandtl number corresponding to the turbulent kinetic energy and the turbulent energy dissipation rate, respectively. ${\mu }_{eff}$ is the effective viscosity, which can be expressed as follows:

$${\mu }_{eff}=\mu +{\mu }_t$$

(6)

$${\mu }_t=\rho C_{\mu }\frac{k^2}{\epsilon }$$

(7)

The value of $C_{\mu }$ derived using this equation is 0.0845, which is very close to the value determined by experience in the standard k-ε model. The other parameters in the above formula are calculated as follows:

$$\begin{array}{l}{C}_{1\epsilon }=1.42-\frac{\eta \left(1-{\eta }^{\prime }/{\eta }_0\right)}{1+\beta {{\eta }^{\prime }}^3}\\ {\eta }^{\prime }={\left(2E_{ij}\cdot E_{ij}\right)}^{1/2}\frac{k}{\epsilon }\\ E_{ij}=\frac{1}{2}\left(\frac{\partial u_i}{\partial x_j}+\frac{\partial u_j}{\partial x_i}\right)\\ C_{1\epsilon }=1.68,{\eta }_0=4.377,\beta =0.012\end{array}\}$$

(8)

The RNG k-ε turbulence model is suitable for fully developed turbulence at high Reynolds numbers. To accurately calculate the flow phenomenon of the oil jet impacting the oil scoop blade, a double-layer wall model is used in the near wall region. According to the turbulent Reynolds number, the near wall region can be divided into a viscous-affected region and a fully turbulent region. The turbulent Reynolds number is defined as follows [25]:

$$R{e}_y=\frac{\rho y\sqrt{k}}{\mu }$$

(9)

where $y$ is the distance between the center of the normal grid and the wall surface. In the fully turbulent region ($R{e}_y>200$), the RNG k-ε turbulence model is adopted. In the viscous-affected region ($R{e}_y\le 200$), the Wolfstein one-equation model is used for the solution.

3.3. Boundary Conditions and Fluid Properties

The outlet of the oil jet nozzle is set as the pressure inlet boundary condition, and the value of the oil supply pressure is 0.1–0.6 MPa. The volume fraction of lubricant oil at the nozzle inlet is 1. The oil scoop is set as a wall boundary condition without slip and penetration, and with a rotational speed range of 2517 r/min to 10,017 r/min. Both the internal and external calculation domain outlets are pressure outlet boundary conditions, and the gauge pressure is 0 Pa. The diameter of the nozzle orifice is 1.5 mm, and the variation range of the orifice spacing is 2.0–3.5 mm. At the initial calculation, the volume fraction of lubricant oil in the computational domain is 0. The air physical parameters are all taken at a room temperature of 25 °C and with a lubricant oil density of 965 kg/m³, a lubricant oil viscosity of 5 mm²/s, and a surface tension of 0.035 N/m.

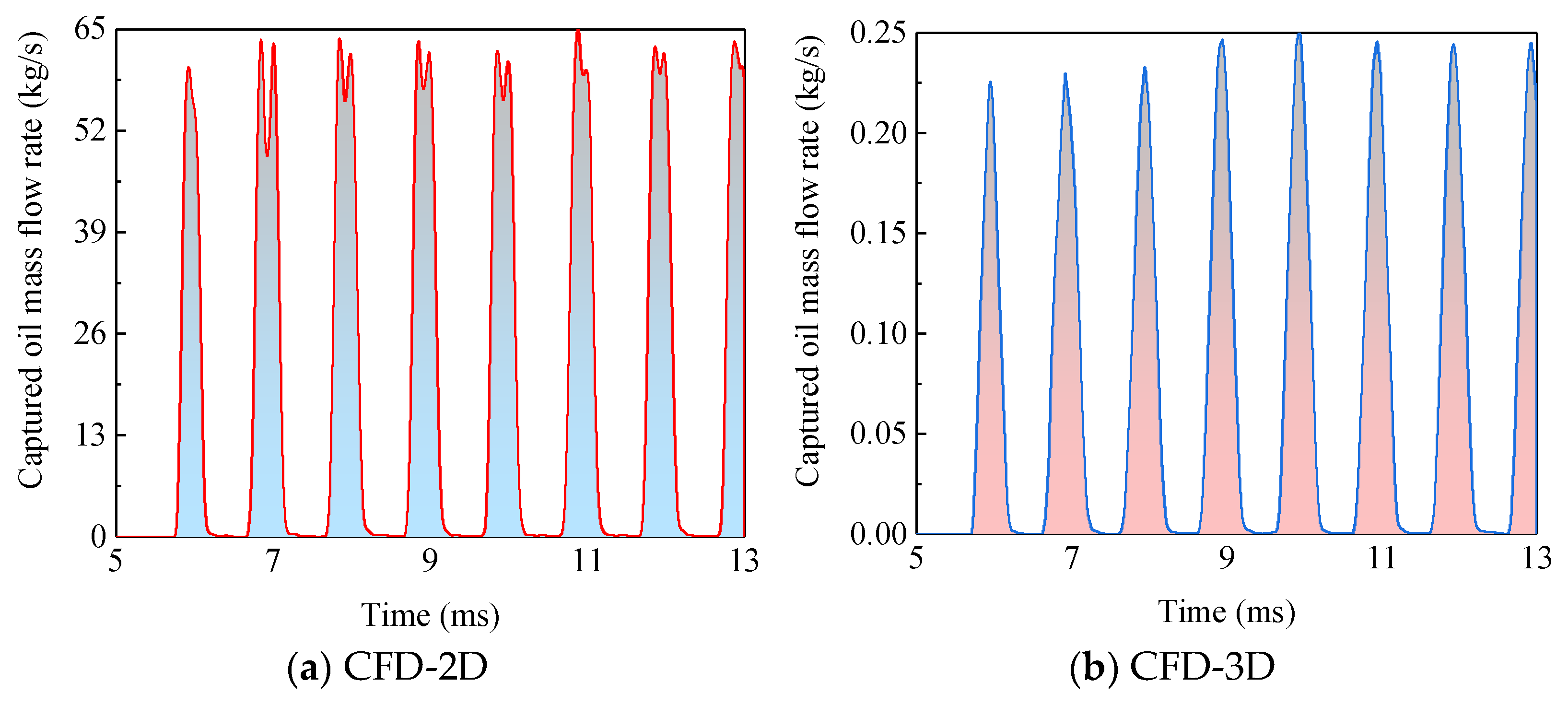
3.4. Model Validation

Figure 4 compares the variations in captured oil mass flow rate over time obtained from the 2D and 3D numerical simulations, where the height of the cross-section in the 2D numerical simulation is 1 m. It can be seen that the variation trends of the captured oil mass flow rate obtained using the 2D and 3D numerical simulations are similar, but the oil mass flow rate values are different. The sum of the total mass outflow is divided by the sum of the inflow of the oil jet nozzle to obtain the oil capture efficiency [8,16]. Figure 5 compares the oil capture efficiency obtained from the numerical simulations with the experimental results. The oil jet nozzle is a single-orifice structure, and the oil supply pressure ΔP = 0.2 MPa. The 2D and 3D numerical simulation results show a good consistency at high rotational speed, but the 3D simulation results are much higher than the 2D simulation results at low rotational speed. This is mainly due to the limited flow capacity of the oil passage at low rotational speed, and because the lubricant oil cannot flow out of the oil passage in the 2D computational domain in time. In the 3D numerical simulation, the diameter of the oil jet nozzle is smaller than the axial width of the oil passage, so there is no problem of insufficient flow capacity. The flow capacity of the oil passage increases continuously as the rotational speed increases, and the calculation results of the 2D and 3D numerical simulations are in good agreement. In general, the variation trends of the oil capture efficiency obtained from the numerical simulations are in good agreement with the experimental results, and the maximum calculation error is less than 15%. We thus verified that the numerical simulation model established in this paper can reliably calculate the oil–air two-phase flow and oil capture efficiency in an under-race lubrication system. The computational cost of a 2D numerical simulation is only 10% that of a 3D numerical simulation. Therefore, under the premise of ensuring the accuracy of numerical calculation, a 2D numerical simulation is used to predict the internal oil–air two-phase flow characteristics and oil capture performance of a radial oil scoop.

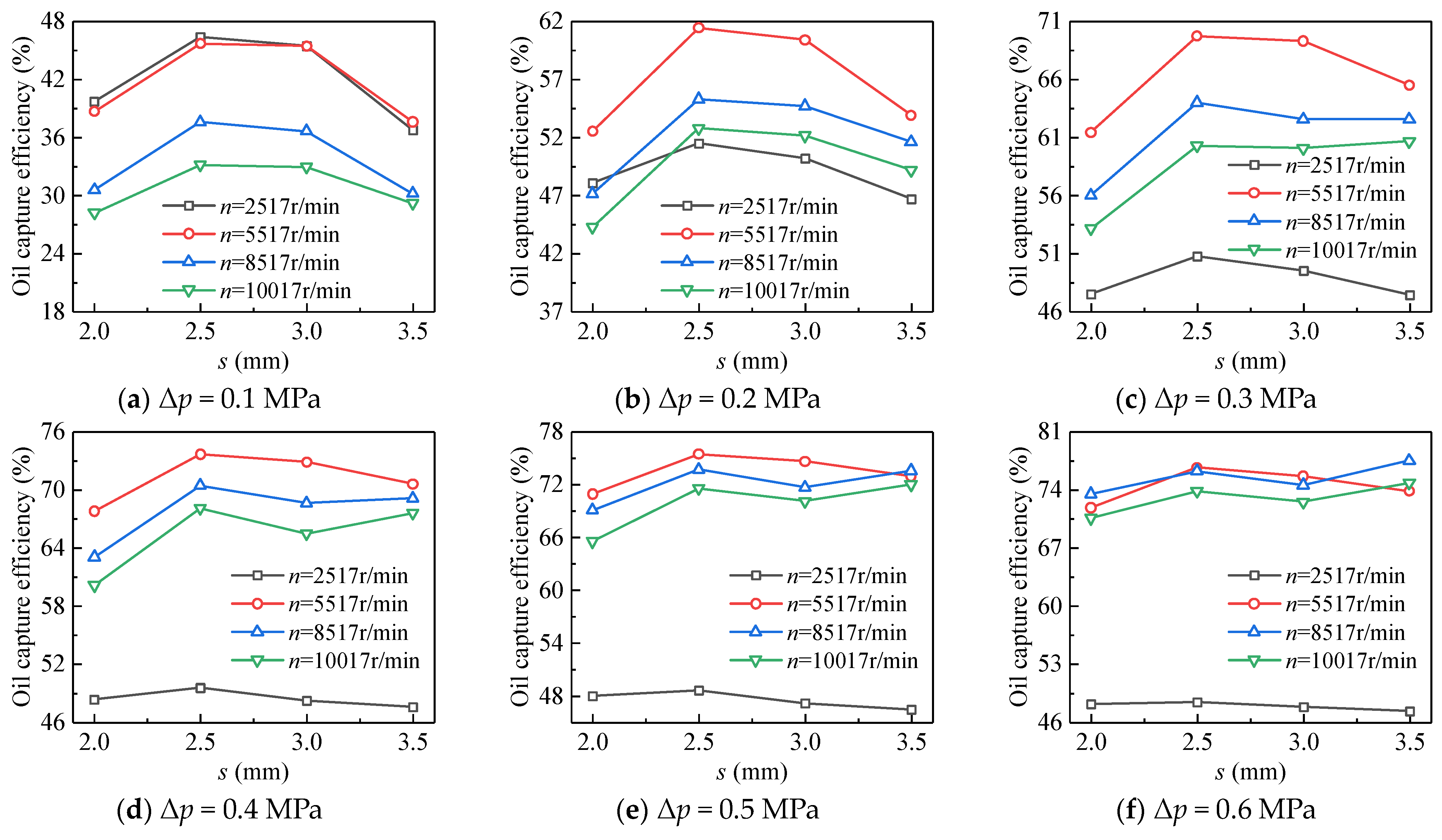
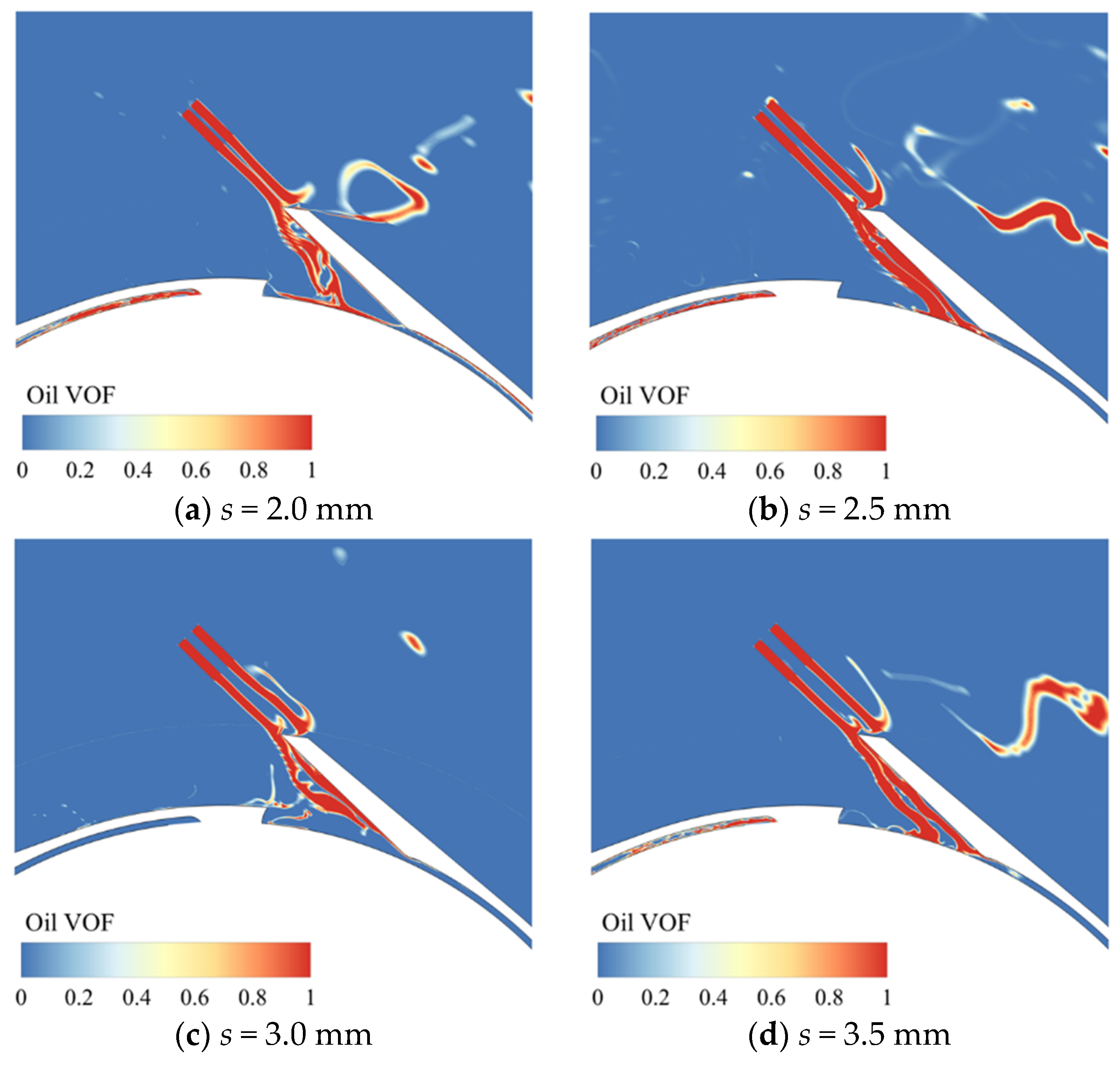
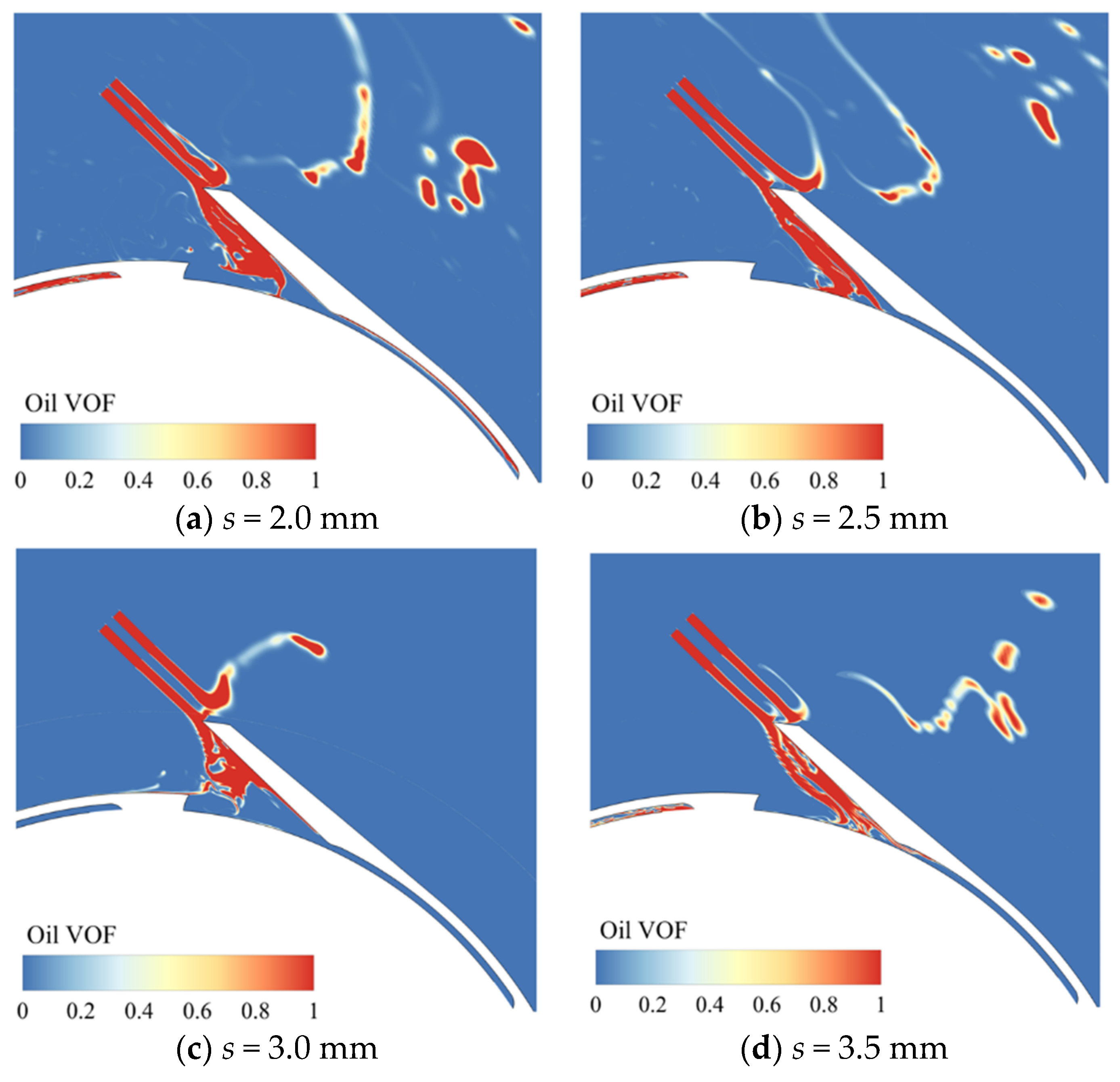
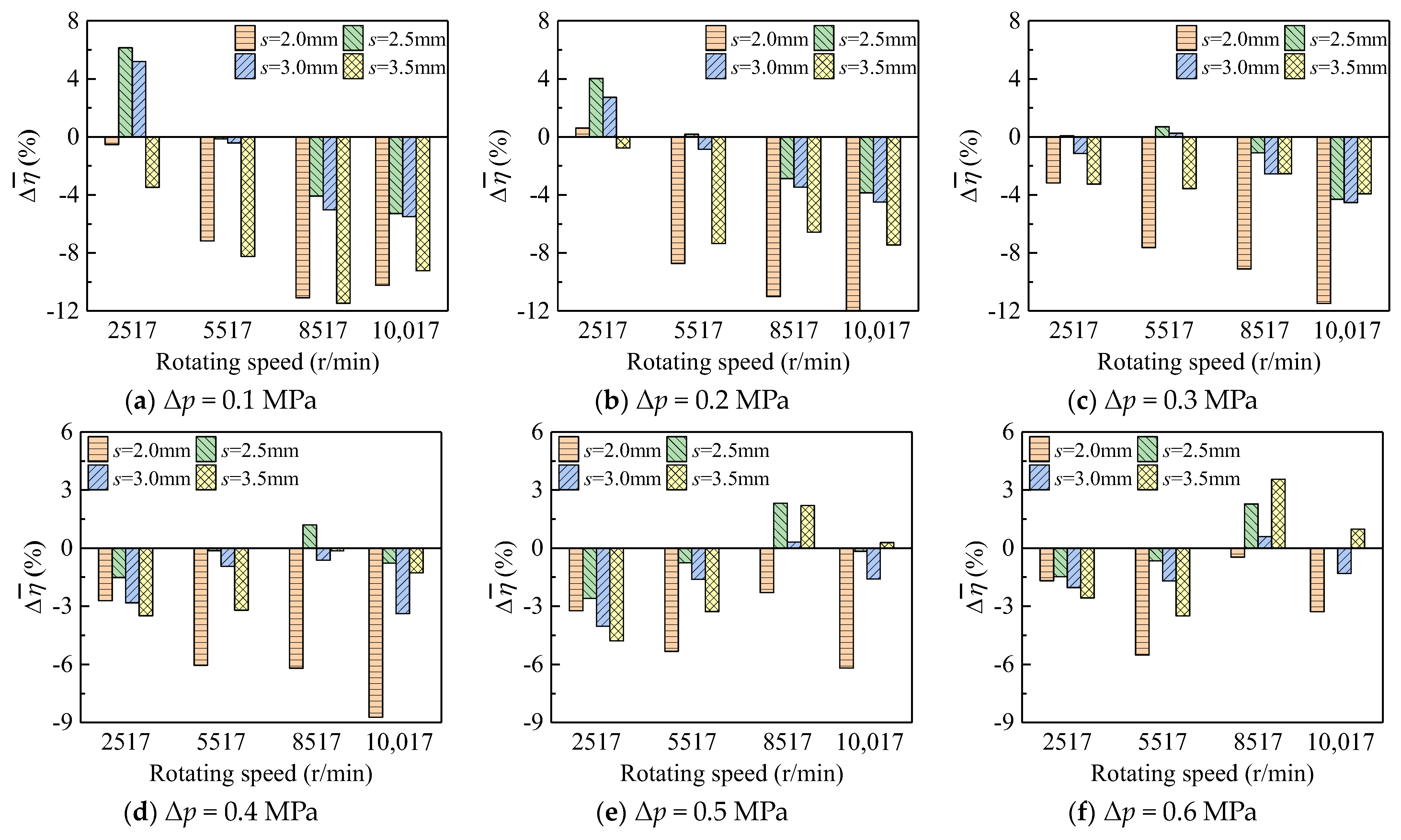
4. Results and Discussion

Figure 6 shows the relationship between oil capture efficiency and orifice spacing under different oil supply pressures in a double-orifice tandem layout structure. The results show that the effect of orifice spacing on oil capture efficiency is not entirely the same at different rotational speeds and oil supply pressures. When the oil supply pressure Δp < 0.2 MPa, the oil capture efficiency shows a trend of first increasing and then decreasing as the orifice spacing increases at all rotational speeds. The oil capture efficiency is relatively high when the orifice spacing is within the range of 2.5–3.0 mm. As the oil supply pressure increases, the trend of oil capture efficiency remains unchanged at lower rotational speeds (n ≤ 5517 r/min). However, at higher rotational speeds (n ≥ 8517 r/min), the oil capture efficiency corresponding to the orifice spacing s = 3.5 mm increases again, and even exceeds the oil capture efficiency corresponding to the orifice spacing s = 2.5 mm under some working conditions.

Previous studies have shown that under the same oil supply pressure, the oil supply flow rate of any orifice in a dual-orifice jet nozzle is smaller than that of a single orifice. The smaller the orifice spacing, the more significant the flow interference between the orifices, and the greater the difference in oil supply flow rate. Therefore, under the same oil supply pressure, the larger the orifice spacing, the higher the oil flow rate. To clarify the impact of orifice spacing on oil capture performance, Figure 7 shows the distribution of the oil–air two-phase flow when the oil scoop blade cuts the oil jet in different structures of the oil supply at pressure Δp = 0.1 MPa and rotational speed n = 2517 r/min. It can be seen that in the structure with orifice spacing s = 2.0 mm, two lubricant oil jets will create flow interference under the action of a jet stream before entering the oil passage. On the one hand, the breakup and splashing loss during the cutting of the oil jet by the oil scoop blade increases, and on the other hand, the oil entering the oil passage directly impacts the surface of the shaft, causing some of the lubricant oil to rebound and resulting in secondary loss of oil. When the orifice spacing increases to s = 2.5 mm, the interference effect of the two jets before entering the oil passage is significantly weakened, and the breakup and splashing loss of the rear jet under the obstruction of the front jet is reduced. The lubricant oil that enters the oil passage at the same time can smoothly flow into the oil delivery channel, which explains the increase in oil capture efficiency. After further increasing the orifice spacing, the amount of air entrained by the two lubricant oil jets entering the oil scoop increases, and the volume of the oil–air mixture increases. When the flow capacity of the oil passage is limited, the secondary loss of lubricant oil also slightly increases, leading to a gradual decrease in oil capture efficiency.

Figure 8 shows the two-phase distribution of oil and air when the oil supply pressure Δp = 0.4 MPa and the rotational speed n = 10,017 r/min and the oil scoop blade cuts the oil jet with different orifice spacing structures. It can be seen that the oil–air two-phase distribution is consistent with the phenomenon shown in Figure 7 when the orifice spacing is 2.0 mm < s < 3.0 mm. In the structures with orifice spacing s = 3.0 mm and s = 3.5 mm, the flow interference between the two lubricant oil jets tends to disappear, and the fragmentation splash loss that occurs when the oil scoop blade cuts the lubricant oil jet decreases. Even if there is air mixed in when the two jets enter the oil scoop, the flow capacity of the oil passage is significantly improved at high rotational speeds, and the secondary loss of the lubricant oil is also reduced. This phenomenon can be seen in Figure 8d. Therefore, when the rotational speed and oil supply pressure are high, the oil capture efficiency corresponding to the orifice spacing s = 3.5 mm shows a slight improvement.

Based on the oil capture efficiency of the d = 1.5 mm single-orifice nozzle, Figure 9 compares the trend of the oil capture efficiency changes of the double-orifice tandem layout structure to that of the single-orifice structure under different working conditions. The results show that the oil capture efficiency of the double-orifice structure is lower than that of the single-orifice structure under most operating conditions and under various oil supply pressures, with a maximum decrease of 12.0% in oil capture efficiency. The oil capture efficiency of the double-orifice structure is higher than that of the single-orifice structure under only a few operating conditions, with a maximum increase of only 6.0%, and this increased efficiency is mainly concentrated in structures with orifice spacing s = 2.5 mm and s = 3.0 mm. It is worth noting that as the oil supply pressure increases, the double-orifice structure brings about an increase in oil capture efficiency which corresponds to an increase in rotational speed. This further indicates a coupling relationship between the oil supply pressure and the rotational speed. If a double-orifice nozzle is used to improve the oil capture efficiency, it is necessary to match the design of the oil supply pressure, rotational speed, and orifice spacing.

Figure 10 compares the captured oil of a double-orifice tandem layout structure and that of a single-orifice structure under typical operating conditions. The horizontal line in the figure represents twice the captured oil of a single-orifice structure under the same oil supply pressure. It is found by comparison that although the oil capture efficiency of the double-orifice structure is higher than that of the single-orifice structure under individual working conditions, the amount of oil collected by almost all of the double-orifice structures is less than twice that collected by the single-orifice structure. The reason for this result is that the oil supply flow rate of the double-orifice structure is less than twice the oil supply flow rate of the single-orifice structure.

5. Conclusions

In this paper, by combining experimental data with numerical simulations, the effect of orifice spacing on the internal flow and oil capture efficiency of an under-race lubrication system was studied. The main conclusions are as follows:

(1)

The variation trend of the typical test data is consistent with that of the numerical simulation results, which confirms the rationality of the experimental scheme and the effectiveness of the numerical model.

(2)

A double-orifice structure can significantly increase the amount of captured oil compared to the single orifice structure, but it still captures less than twice the amount of oil captured by the single-orifice structure. At the same time, the degree to which a double-orifice tandem structure can improve the oil capture efficiency of a radial oil scoop is very limited, and the maximum increase is only 6%. It is worth noting that improper design of the orifice spacing will reduce the oil capture efficiency, with a maximum reduction of up to 12%.

(3)

When the structure design of the oil jet nozzle or the installation space is limited and it is necessary to choose the double-orifice tandem structure, the orifice spacing should be determined based on a full evaluation of the effect of the orifice spacing and working parameters on the oil capture performance.