Reactive Flow Dynamics of Low-Frequency Instability in a Scramjet Combustor
Abstract
:1. Introduction
2. Methodology
2.1. Two-Dimensional Turbulent Combustion Simuilation
2.2. Governing Equations
2.3. Numerical Approaches
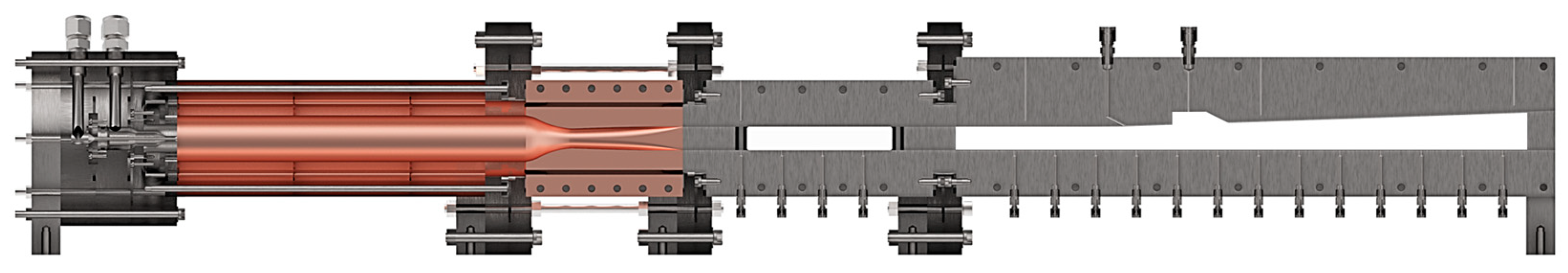
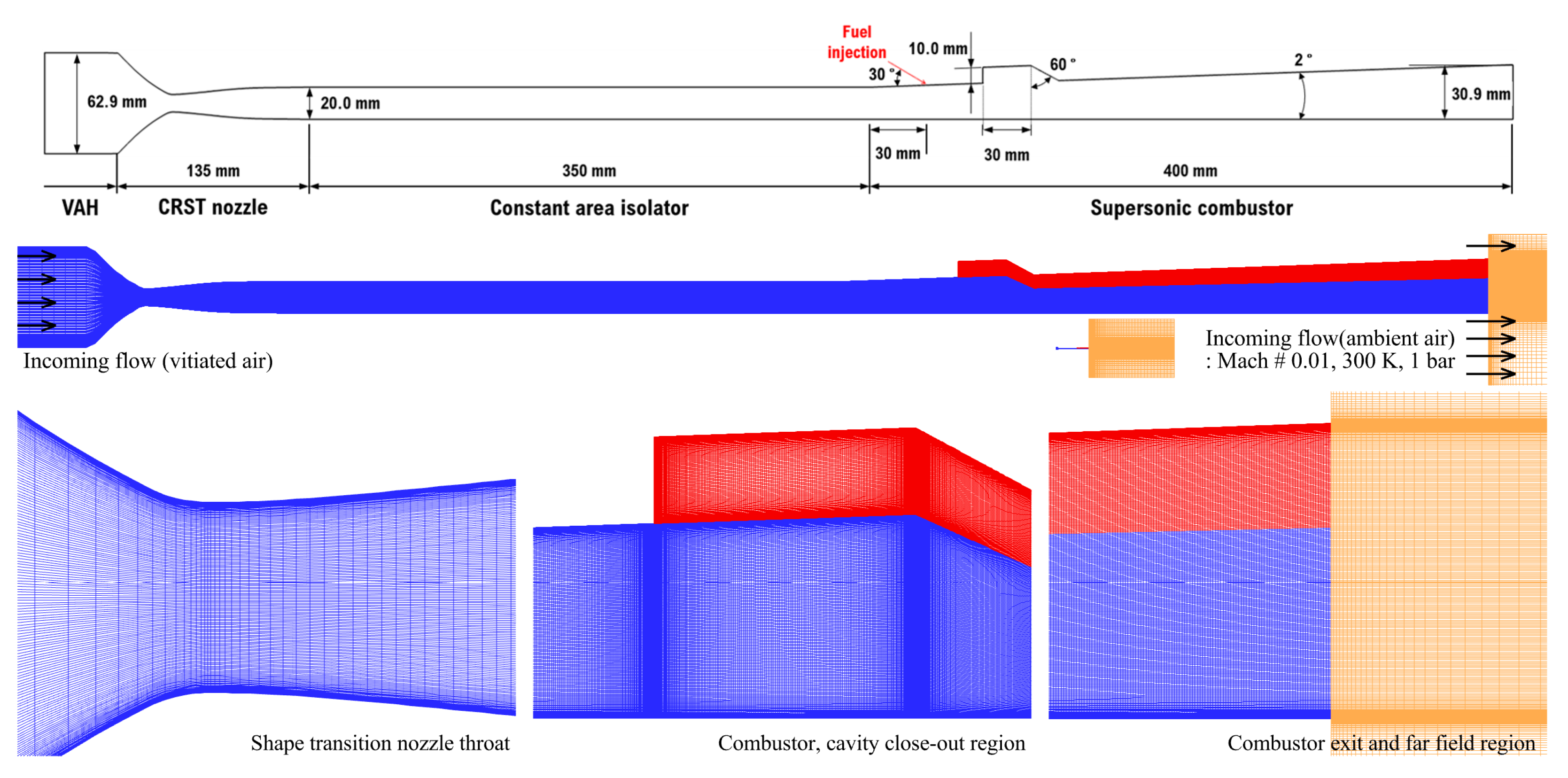
2.4. Model Supersonic Combustor and Operating Condition
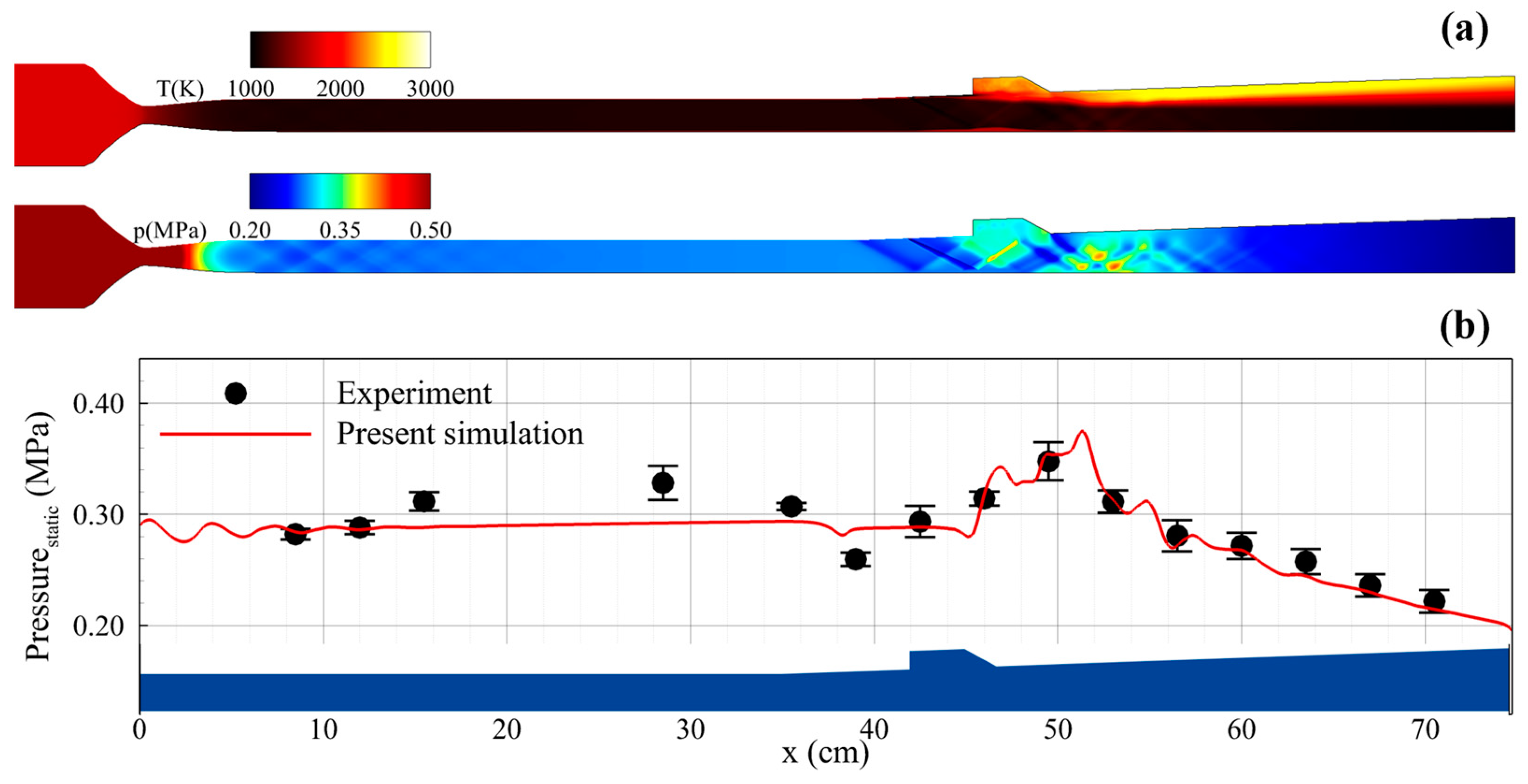
2.5. Validation
2.6. Formation and Stabilization of Supersonic Flow Field
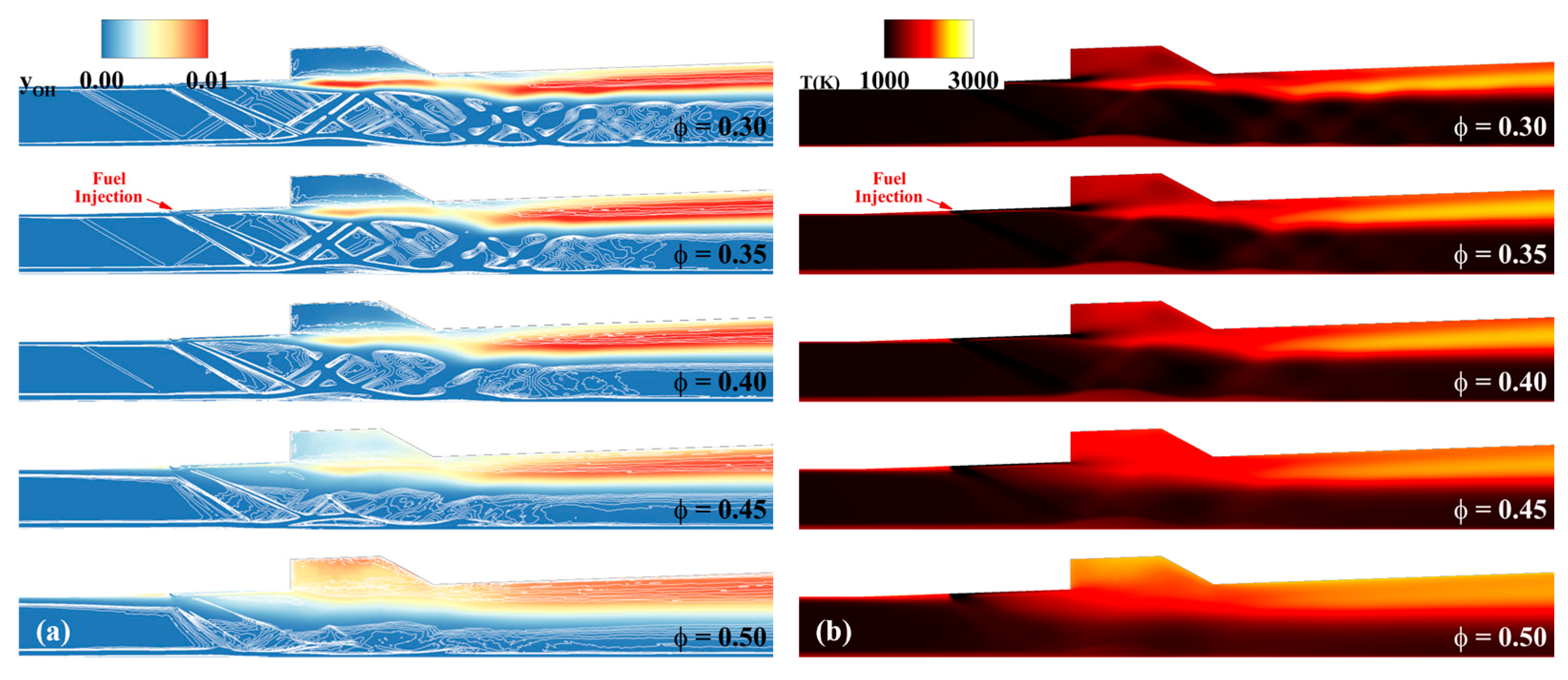
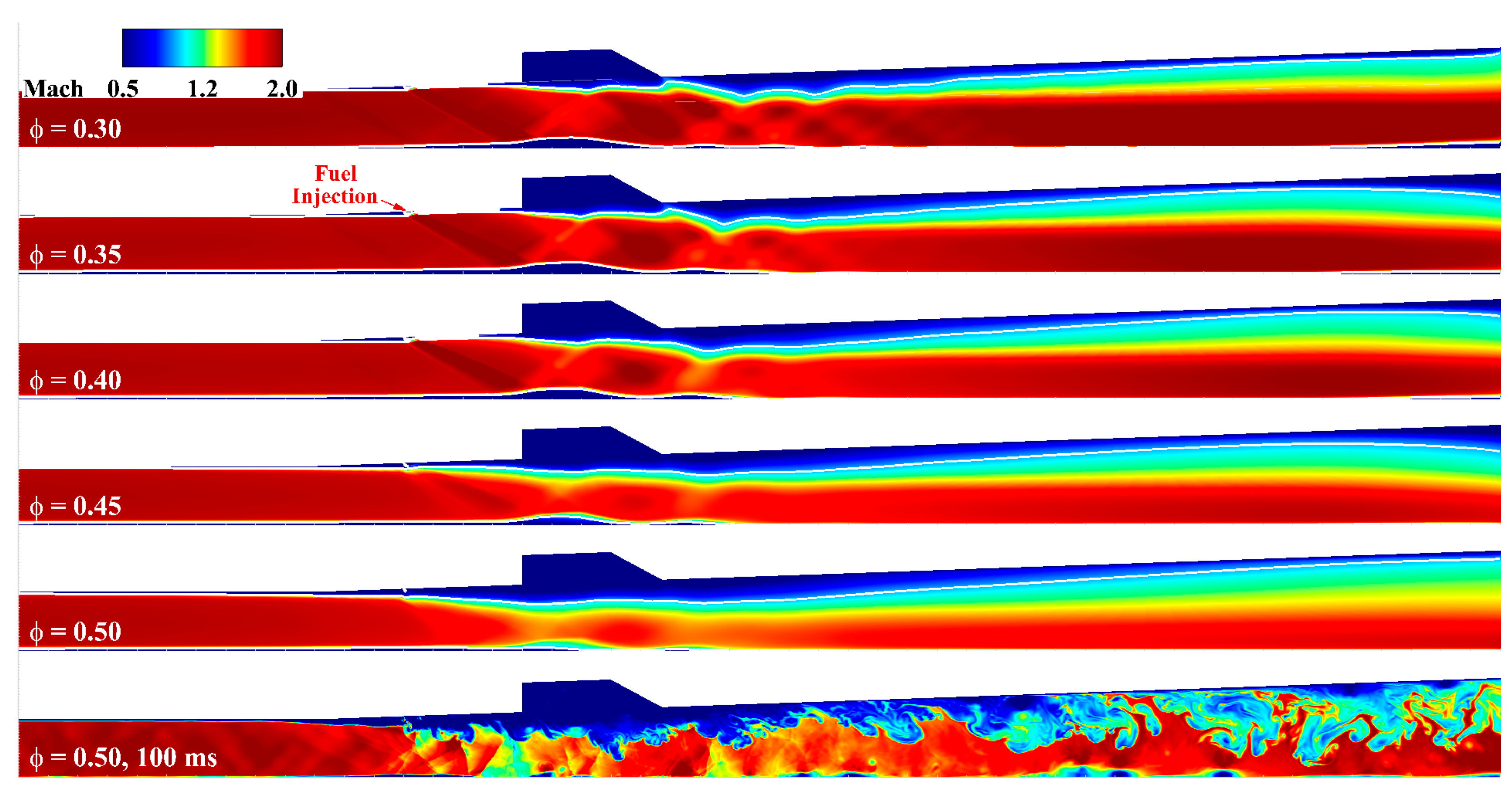
3. Combustion Characteristics
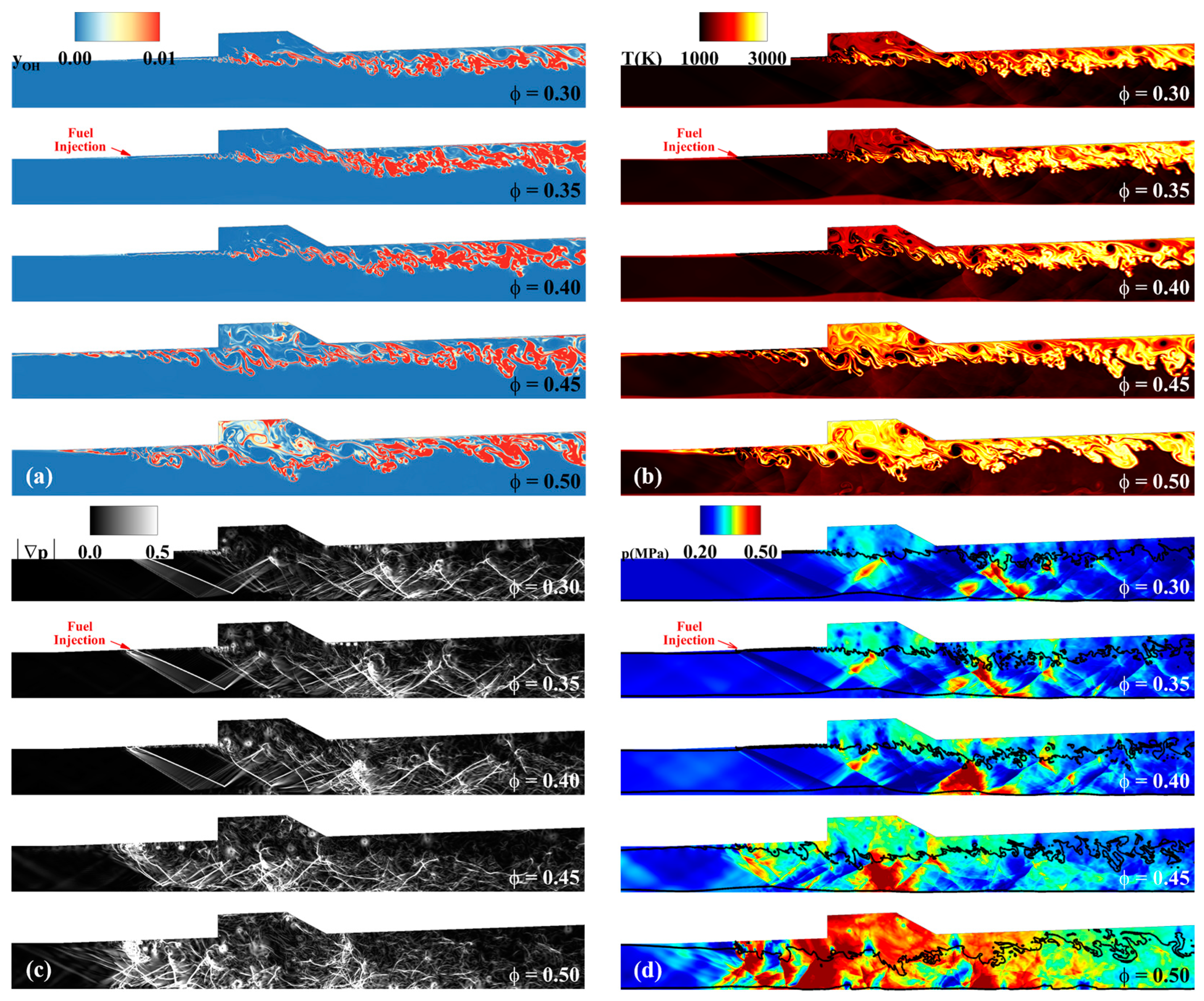
3.1. Combustion Modes
3.2. Operating Conditions
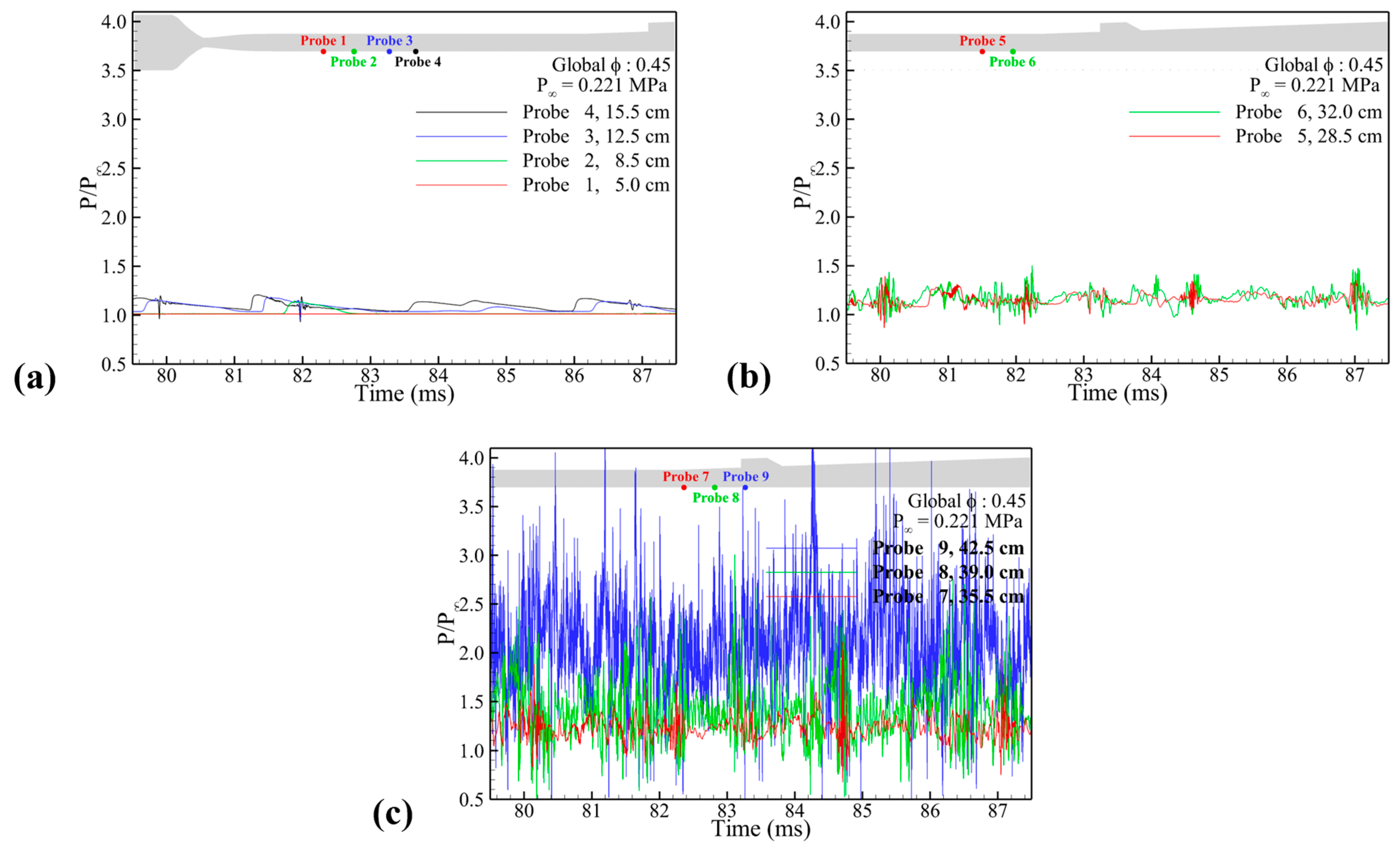
3.3. Fluctuation of Pressure and Temperature
4. Low-Frequency Combustion Instability
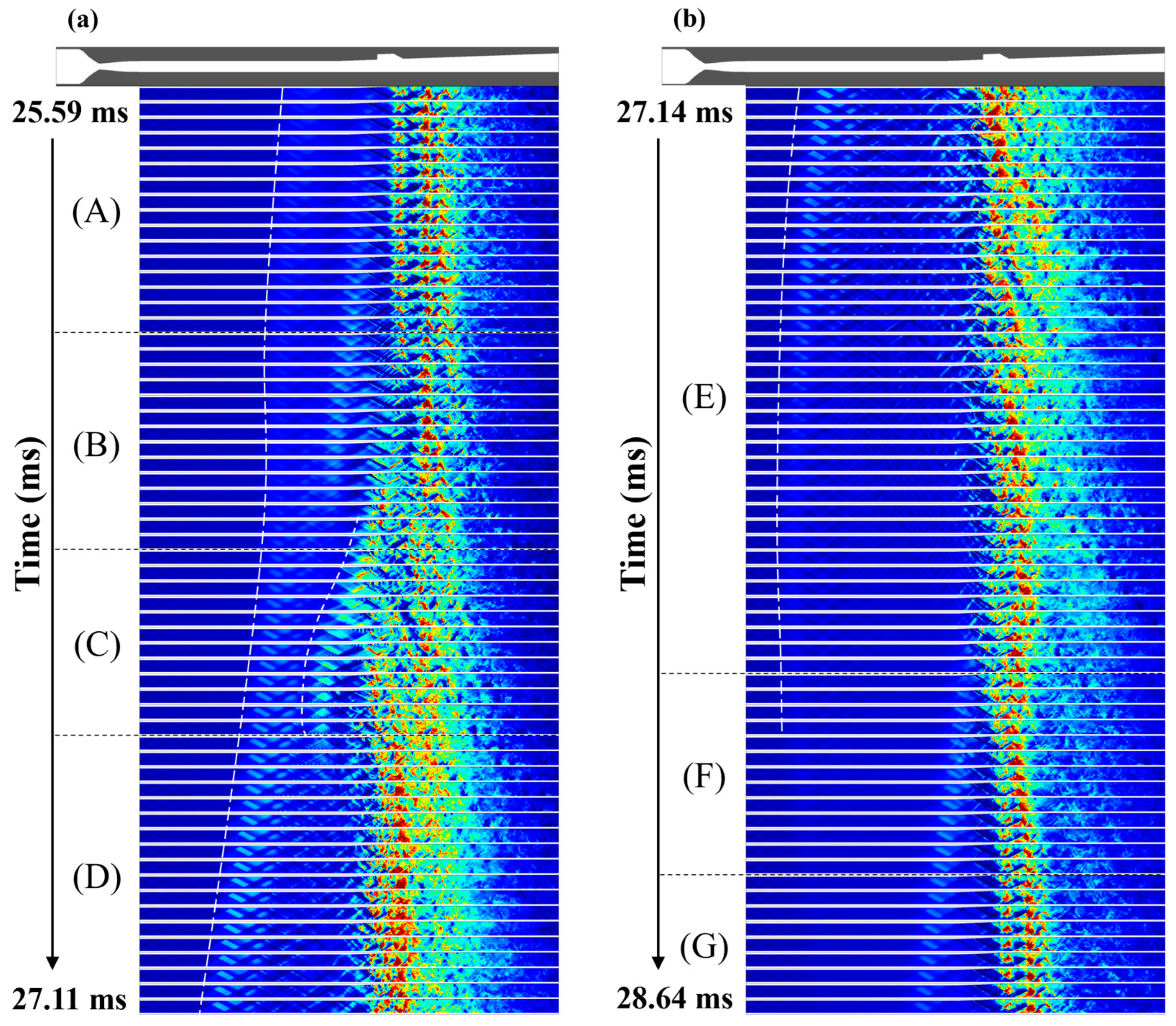
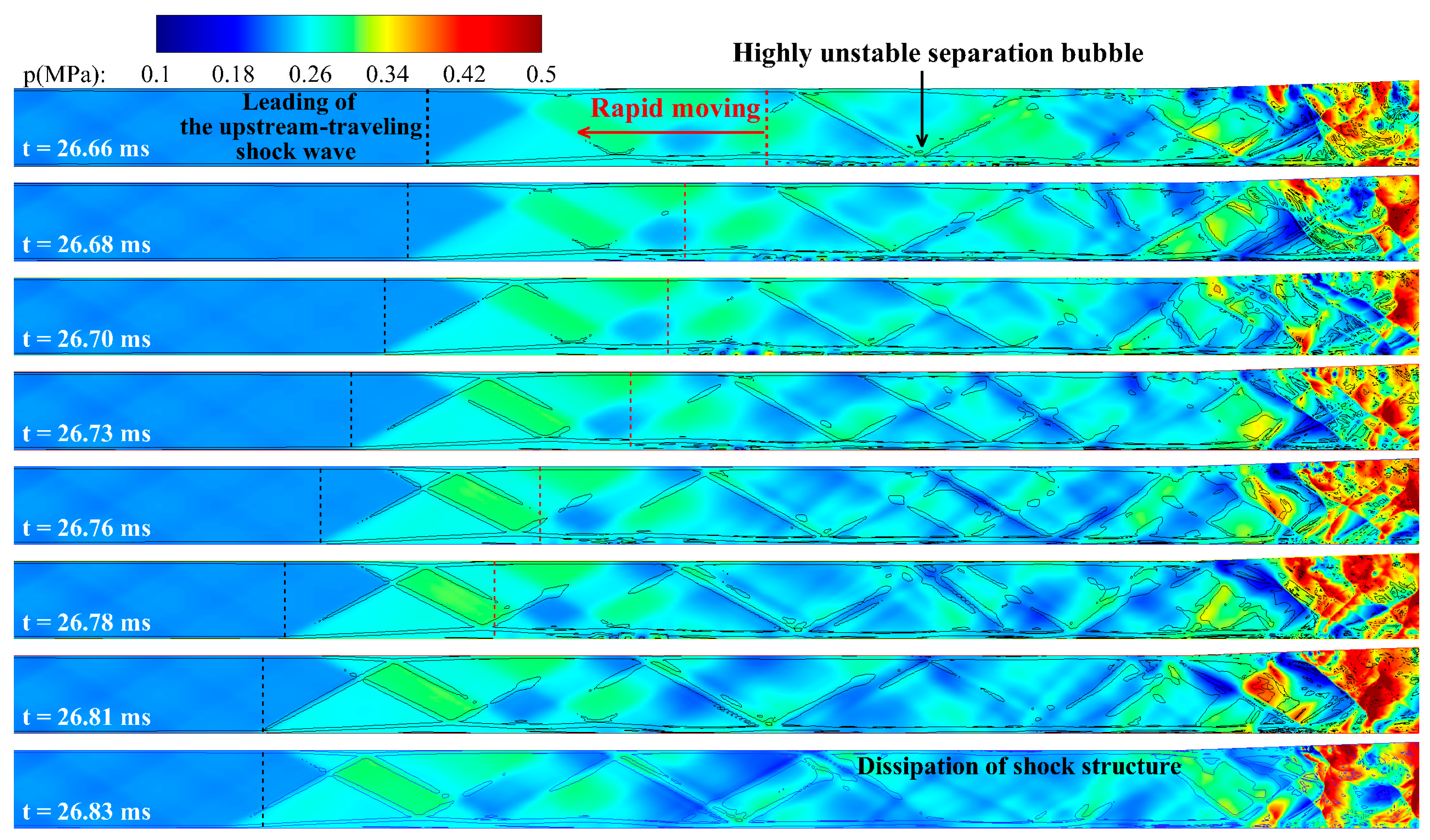
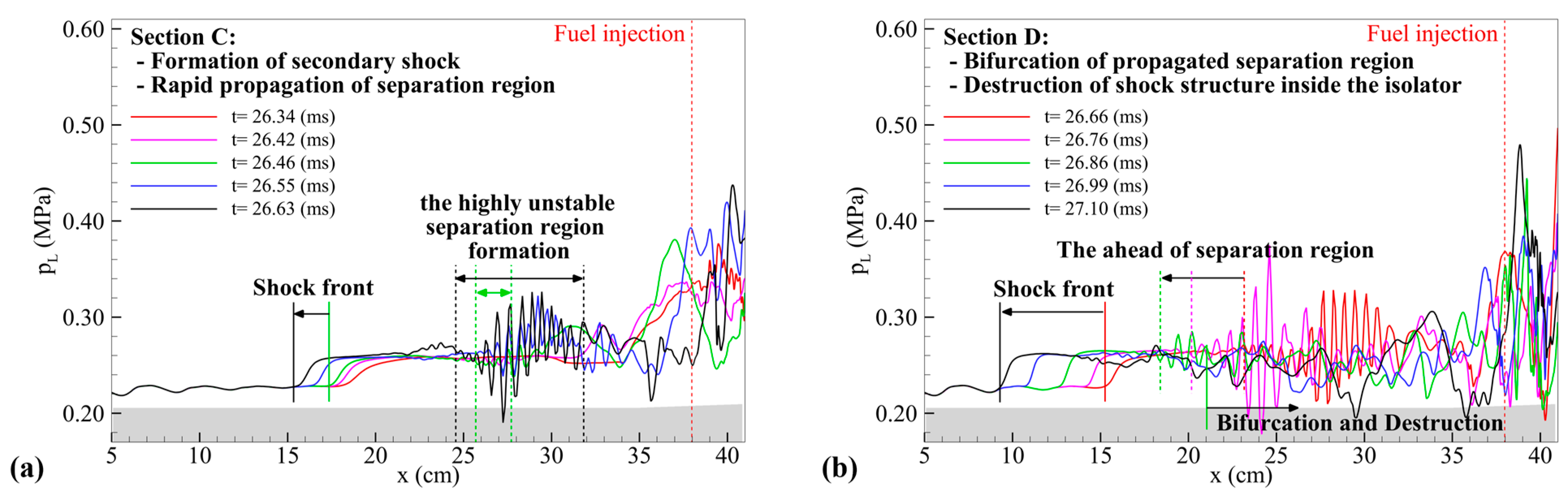
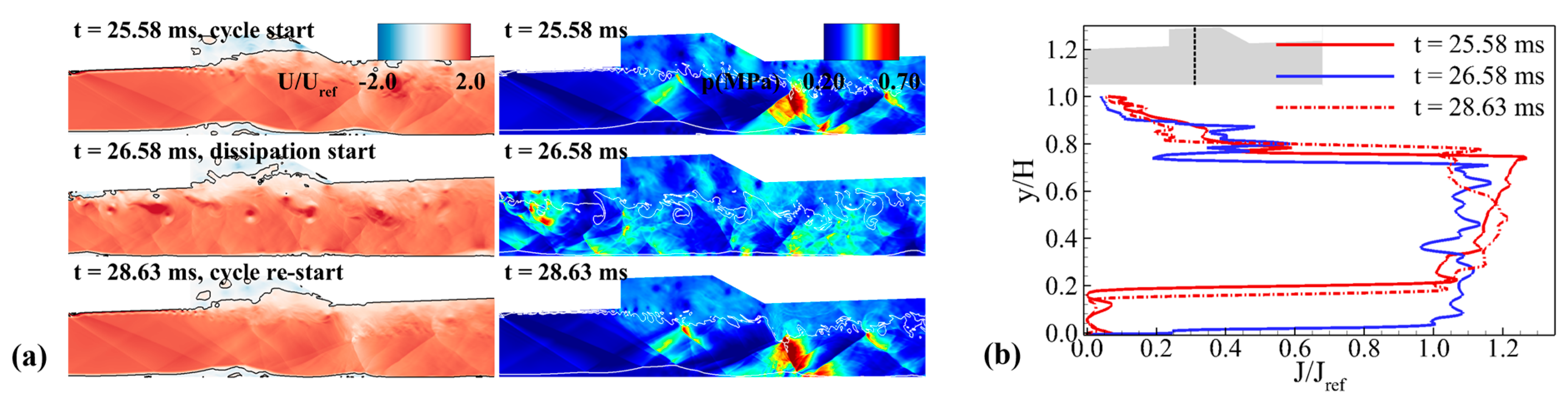
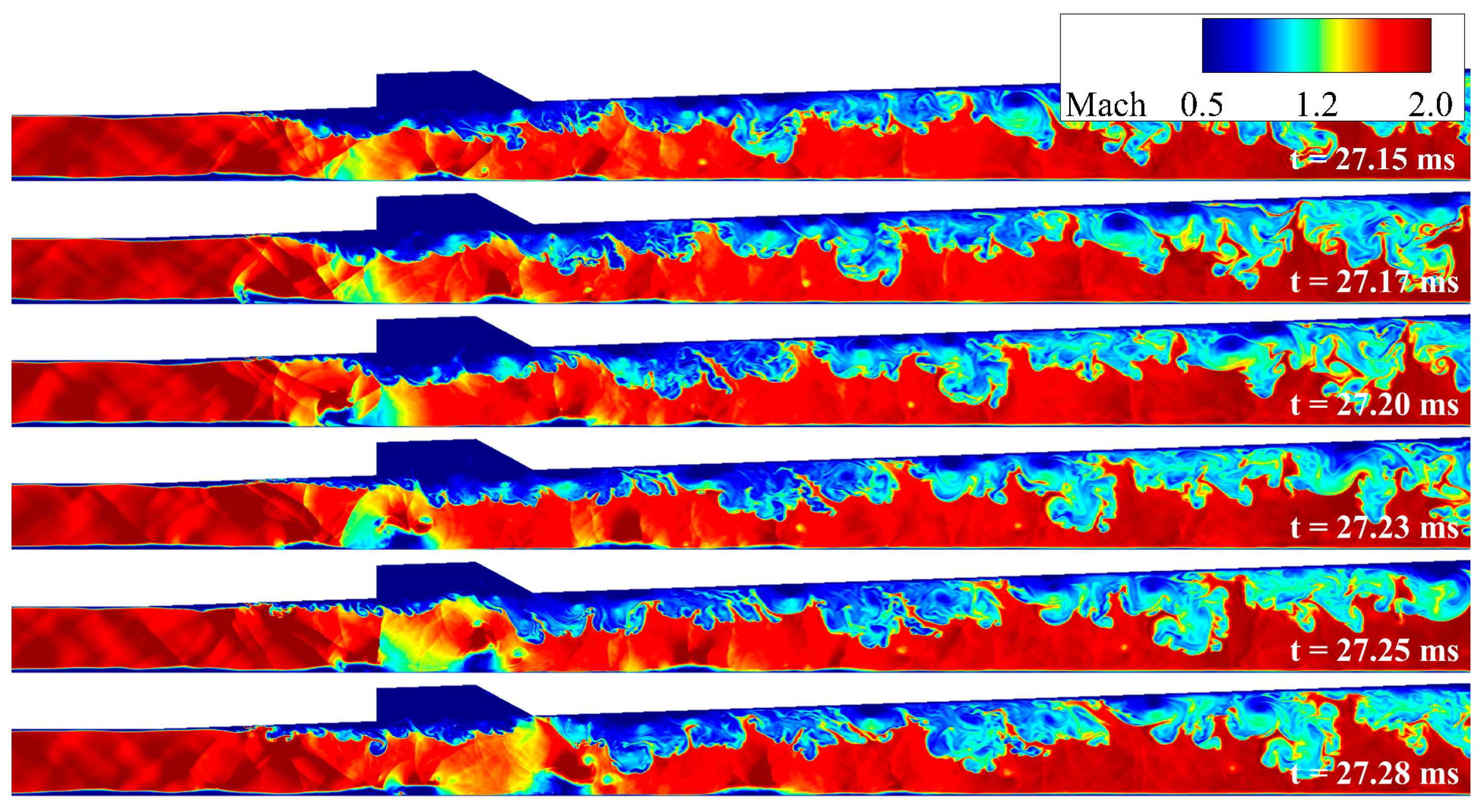
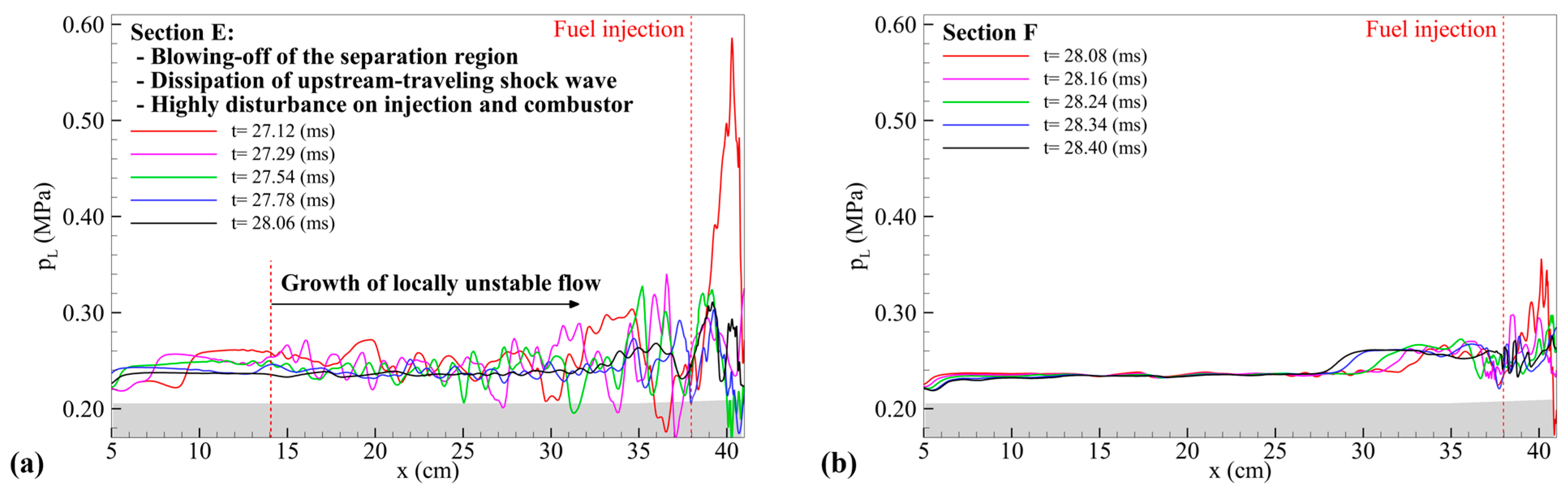
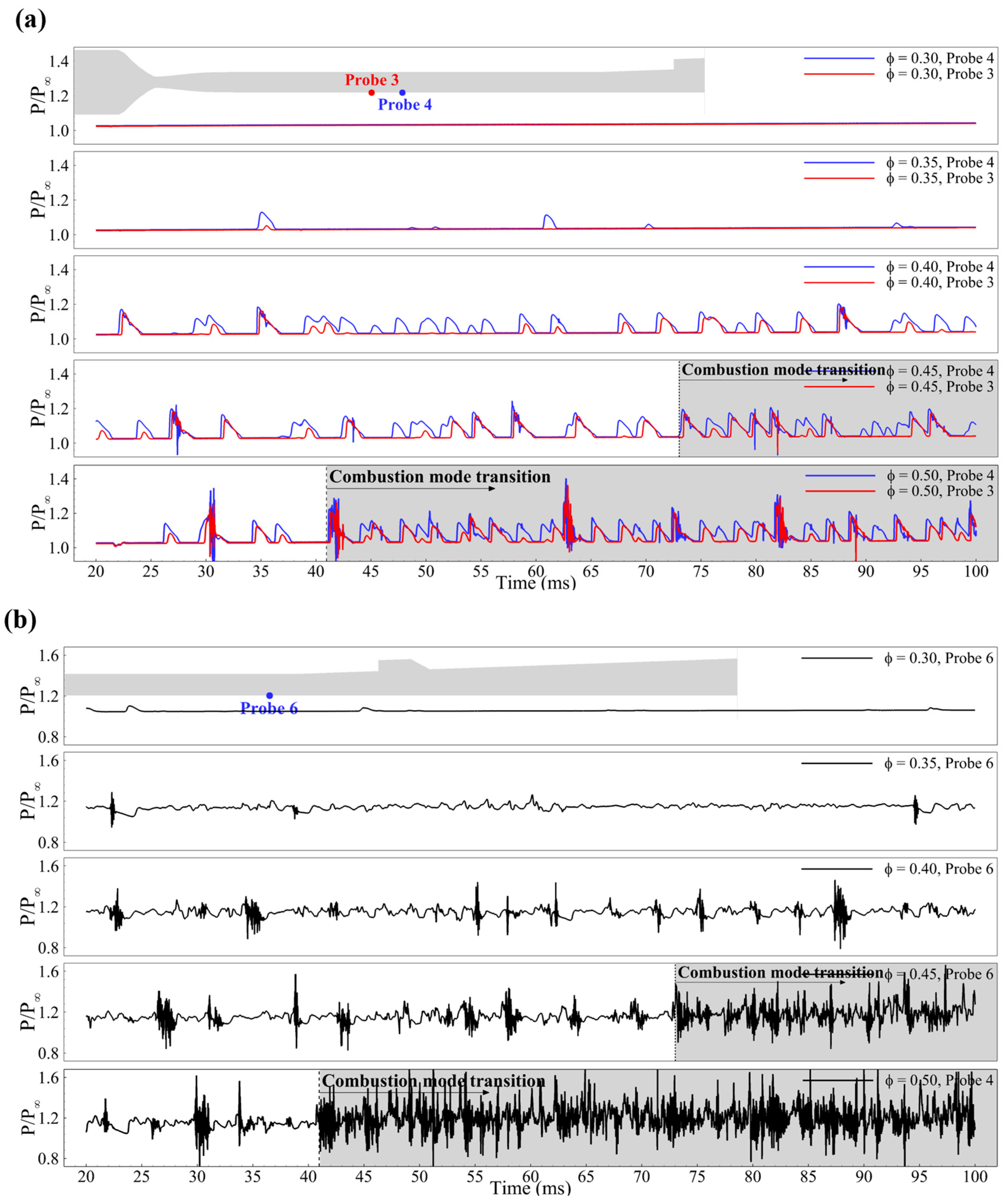
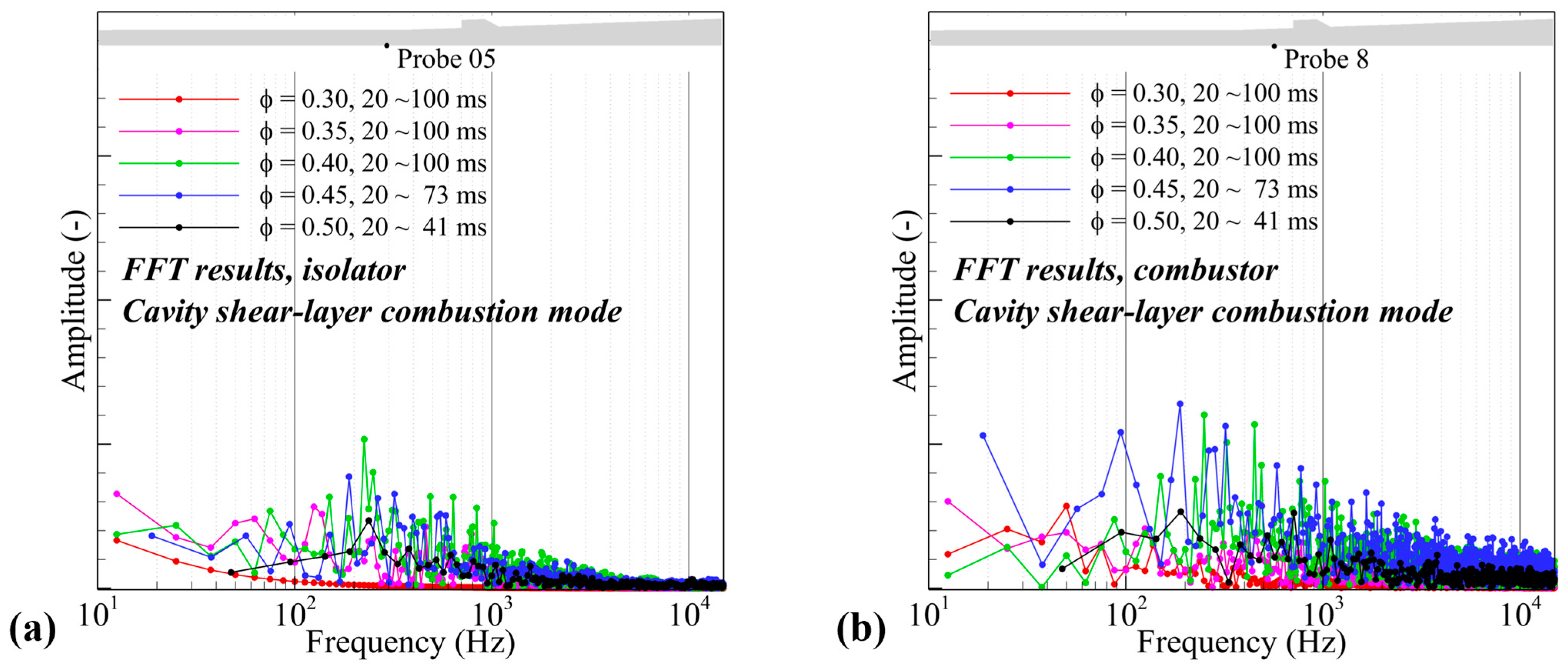
4.1. Dynamics of the Upstream-Traveling Shock Waves in the Cavity Shear-Layer Combustion Mode
4.2. Transition in the Combustion Mode
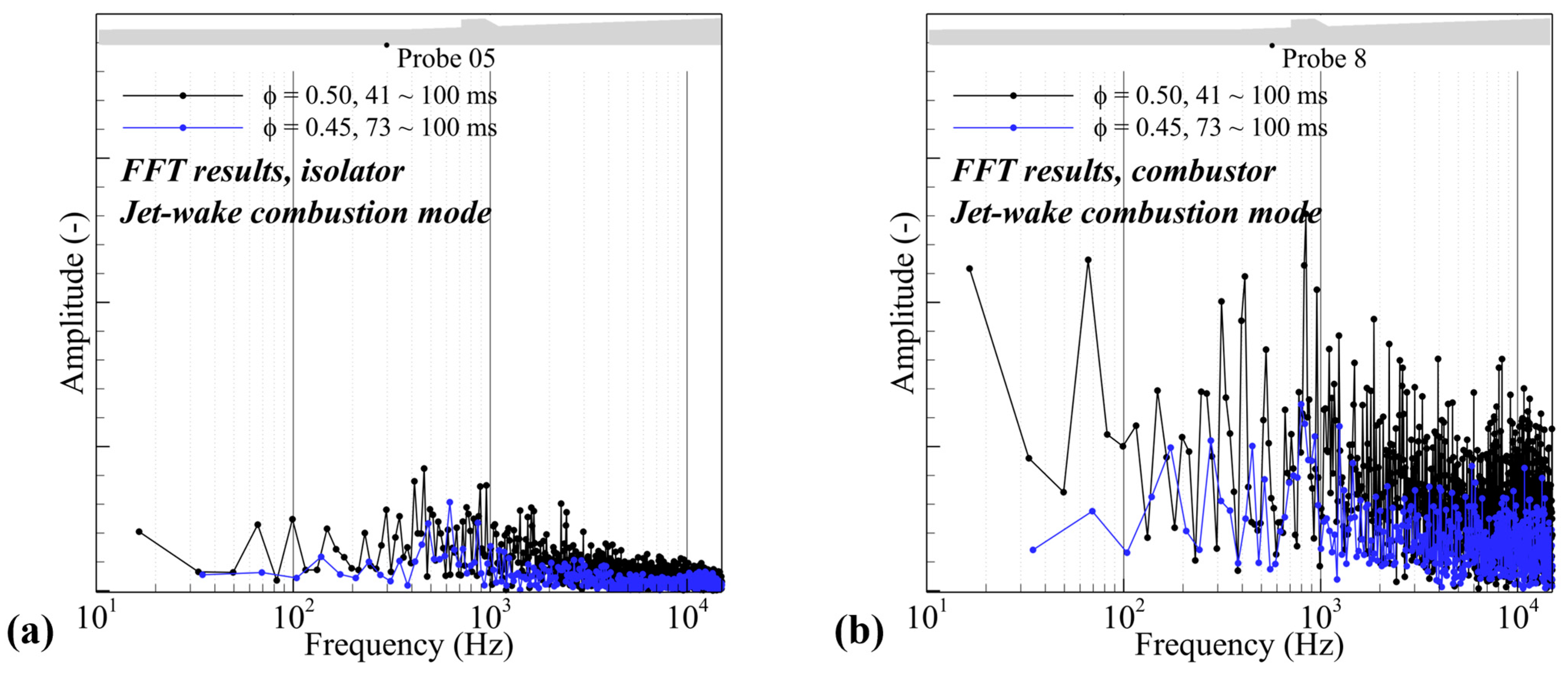
4.3. Dynamics of the Upstream-Traveling Shock Wave in the Jet-Wake-Type Combustion Mode
4.4. Factors Affecting the Dynamics of the Upstream-Traveling Shock Wave
4.5. Characteristics of the Combustion Instability
5. Conclusions
- Two different types of “upstream-traveling shock waves” were sequentially formed and interacted under the cavity shear-layer combustion mode, resulting in the generation of combustion instability. The period of each cycle of combustion instability is approximately 2.0–5.0 ms. In the jet-wake combustion mode, the “upstream-traveling shock wave” with a strong pressure gradient and relatively higher propagation speed with a period of approximately 2.0–2.5 ms was formed. Each shock wave has not behaved sequentially but overlaps.
- Combustion mode determined the propagation speed and occurrence interval of the “upstream-traveling shock wave,” and the equivalence ratio governed the location at which the shock reached the isolator and the accompanying pressure level.
- The instability frequency is identical at the isolator and the combustor. This finding confirms that the “upstream-traveling shock wave” is a primary factor generating the combustion instability in a scramjet combustor. The formation/dissipation of the “upstream-traveling shock wave” is caused by the coupled fluid-combustion dynamics of the isolator and the combustor. This indicates that the combustion instability of the scramjet engine is a problem generated at a macroscopic scale throughout the entire combustor.
- The range of the instability frequency was increased, even under the same equivalence ratio condition, once the combustion mode had changed from the cavity shear-layer mode to the jet-wake mode. These results reveal that the “instability frequency shifting” of the scramjet combustor is governed by the combustion mode rather than the increase/decrease in the equivalence ratio.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| AUSMDV | Advection Upstream Splitting Method with flux Difference splitting and flux Vector splitting |
| CRST | Circular-to-Rectangular Shape Transition |
| DCSC | Direct-Connect Scramjet Combustor |
| DES | Detached Eddy Simulation |
| DDES | Delayed Detached Eddy Simulation |
| DMD | Dynamic Mode Decomposition |
| EDC | Eddy Dissipation Concept |
| F/PVA | Flamelet Progress Variable Approach |
| FTT | Flow Through Time |
| GIS | Grid Induced Separation |
| HDCR | HIFiRE Direct Connect Rig |
| LES | Large Eddy Simulation |
| LU-SGS | Lower-Upper Symmetric Gauss-Seidel |
| MOC | Method Of Characteristics |
| MUSCL | Monotonic Upstream-Centered Scheme for Conservation Laws |
| oMLP | optimized Multi-Dimensional Limiting Process |
| PaSR | Partially Stirred Reactor |
| PNU-DCSC | Pusan National University’s Direct-Connect Scramjet Combustor |
| POD | Proper Orthogonal Decomposition |
| PNURPL | Pusan National University Rocket Propulsion Labortory |
| PPM | Piecewise Parabolic Method |
| SOD | Standing Oblique Detonation |
| UV’a SCF | University of Virginia’s scramjet combustion facility |
| RCM-1 | Rocket Combustor Model-1 |
| VAH | Vitiation Air Heater |
| WENO | Weighted Essentially Non-Oscillatory |
References
- Ma, F.; Li, J.; Yang, V.; Lin, K.-C.; Jackson, T. Thermoacoustic Flow Instability in a Scramjet Combustor. In Proceedings of the 41st AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Tucson, AZ, USA, 10–13 July 2005. [Google Scholar]
- Li, J.; Ma, F.; Yang, V.; Lin, K.-C.; Jackson, T. A Comprehensive Study of Combustion Oscillations in a Hydrocarbon-fueled Scramjet Engine. In Proceedings of the 45th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 8–11 January 2007. [Google Scholar]
- Choi, J.-Y.; Ma, F.; Yang, V. Dynamic Combustion Characteristics in Scramjet Combustors with Transverse Fuel Injection. In Proceedings of the 41st AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Tucson, AZ, USA, 10–13 July 2005. [Google Scholar]
- Choi, J.-Y.; Ma, F.; Yang, V. Combustion Oscillations in a Scramjet Engine Combustor with Transverse Fuel Injection. Proc. Combust. Inst. 2005, 30, 2851–2858. [Google Scholar] [CrossRef]
- Lin, K.-C.; Jackson, K.; Behdadnia, R.; Jackson, T.A.; Ma, F.; Yang, V. Acoustic Characterization of an Ethylene-fueled Scramjet Combustor with a Cavity Flameholder. J. Propuls. Power 2010, 26, 1161–1170. [Google Scholar] [CrossRef]
- Micka, D.J.; Driscoll, J.F. Combustion Characteristics of a Dual-mode Scramjet Combustor with Cavity Flameholder. Proc. Combust. Inst. 2009, 32, 2397–2404. [Google Scholar] [CrossRef]
- Micka, D.J.; Knaus, D.A.; Temme, J.; Driscoll, J.F. Passive Optical Combustion Sensors for Scramjet Engine Control. In Proceedings of the 51st AIAA/SAE/ASEE Joint Propulsion Conference, Orlando, FL, USA, 27–29 July 2015. [Google Scholar]
- Wang, Z.; Sun, M.; Wang, H.; Yu, J.; Liang, J.; Zhang, F. Mixing-related Low Frequency Oscillation of Combustion in an Ethylene-fueled Supersonic Combustor. Proc. Combust. Inst. 2015, 35, 2137–2144. [Google Scholar] [CrossRef]
- Nakaya, S.; Kinoshita, R.; Lee, J.; Ishikawa, H.; Tsue, M. Analysis of Supersonic Combustion Characteristics of Ethylene/Methane Fuel Mixture on High-Speed Measurements of CH* Chemiluminescence. Proc. Combust. Inst. 2019, 37, 3749–3756. [Google Scholar] [CrossRef]
- Nakaya, S.; Yamana, H.; Tsue, M. Experimental Investigation of Ethylene/air Combustion Instability in a Model Scramjet Combustor using Image-based Methods. Proc. Combust. Inst. 2021, 38, 3869–3880. [Google Scholar] [CrossRef]
- van der Lee, J.; Yokev, N.; Michaels, D. Combustion Instability Due to Combustion Mode Transition in a Cavity-Stabilized Scramjet. J. Propul Power 2022, 38, 945–956. [Google Scholar] [CrossRef]
- Zhao, G.; Sun, M.; Wu, J.; Cui, X.; Wang, H. Investigation of Flame Flashback Phenomenon in a Supersonic Crossflow with Ethylene Injection Upstream of Cavity Flameholder. Aerosp. Sci. Technol. 2019, 87, 190–206. [Google Scholar] [CrossRef]
- Jiang, Z.; Zhang, Z.; Liu, Y.; Wang, C.; Luo, C. Criteria for Hypersonic Airbreathing Propulsion and its Experimental Verification. Chin. J. Aeront. 2021, 34, 94–104. [Google Scholar] [CrossRef]
- Zettervall, N.; Nordin-Bates, K.; Ibron, C.; Fureby, C. LES of Combustion Dynamics in an Ethylene-Hydrogen-Air Ramjet. In Proceedings of the 33rd Congress of the International Council of the Aeronautical Science, Stockholm, Sweden, 4–9 September 2022. [Google Scholar]
- Wang, H.; Wang, Z.; Sun, M.; Qin, N. Combustion Characteristics in a Supersonic Combustor with Hydrogen Injection Upstream of Cavity Flameholder. Proc. Combust. Inst. 2013, 34, 2073–2082. [Google Scholar] [CrossRef]
- Bermejo-Moreno, I.; Larsson, J.; Bodart, J.; Vicquelin, R. Wall-modeled Large-eddy Simulations of the HIFiRE-2 Scramjet. Cent. Turbul. Res. Annu. Res. Briefs. 2013, 3–19. [Google Scholar]
- Johan, L.; Stuart, L.; Iván, B.-M.; Julien, B.; Sebastian, K.; Ronan, V. Incipient Thermal Choking and Stable Shock-train Formation in the Heat-release Region of a Scramjet Combustor. Part II: Large eddy simulations. Combust. Flame 2015, 162, 907–920. [Google Scholar]
- Potturi, A.S.; Edwards, J.R. Large-eddy/reynolds-averaged Navier–stokes Simulation of Cavity-stabilized Ethylene Combustion. Combust. Flame 2015, 162, 1176–1192. [Google Scholar] [CrossRef]
- Lacaze, G.; Vane, Z.P.; Oefelein, J.C. Large Eddy Simulation of the HIFiRE Direct Connect Rig Scramjet Combustor. In Proceedings of the 55th AIAA Aerospace Sciences Meeting, Grapevine, TX, USA, 9–13 January 2017. [Google Scholar]
- Nordin-Bates, K.; Fureby, C.; Karl, S.; Hannemann, K. Understanding Scramjet Combustion using LES of the HyShot II Combustor. Proc. Combust. Inst. 2017, 36, 2893–2900. [Google Scholar] [CrossRef]
- Ruan, J.L.; Domingo, P.; Ribert, G. Analysis of Combustion Modes in a Cavity Based Scramjet. Combust. Flame 2020, 215, 238–251. [Google Scholar] [CrossRef]
- Hash, C.A.; Drummond, P.M.; Edwards, J.R.; Kato, N.; Lee, T. Numerical Simulation of Stable and Unstable Ram-mode Operation of an Axisymmetric Ethylene-fueled Inlet-isolator-combustor Configuration. Combust. Flame 2022, 242, 112157–112173. [Google Scholar] [CrossRef]
- Cao, D.; Brod, H.E.; Yokev, N.; Michaels, D. Flame Stabilization and Local Combustion Modes in a Cavity-based Scramjet using Different Fuel Injection Schemes. Combust. Flame 2021, 233, 111562–111574. [Google Scholar] [CrossRef]
- Peterson, D.M.; Hassan, E.; Bornhoft, B. Improved Delayed Detached-eddy Simulation of a Round Supersonic Combustor with and without Periodic Boundary Conditions. In Proceedings of the AIAA SCITECH 2022 Forum, San Diego, CA, USA, 3–7 January 2022. [Google Scholar]
- Peterson, D.M. Simulation of a Round Supersonic Combustion using Wall-modeled Large-eddy Simulation and Partially Stirred Reactor Model. Proc. Combust. 2022, 39, 3137–3145. [Google Scholar] [CrossRef]
- Jeong, S.-M.; Lee, J.-H.; Choi, J.-Y. Numerical Investigation of Low-frequency Combustion Instability and Frequency Shifting in a Scramjet Combustor. Proc. Combust. 2022, 39, 3107–3116. [Google Scholar] [CrossRef]
- Hinterberger, C.; Fröhlich, J.; Rodi, W. Three-dimensional and Depth-averaged Large-eddy Simulations of Some Shallow Water Flows. J. Hydraul. Eng. 2007, 133, 857–872. [Google Scholar] [CrossRef]
- Norris, J.W.; Edward, J.R. Large-eddy Simulation of High-speed, Turbulent Diffusion Flames with Detailed Chemistry. In Proceedings of the 35th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 6–9 January 1997. [Google Scholar]
- Edwards, J.R.; Boles, J.A.; Baurle, R.A. Large-eddy/Reynolds-averaged Navier–Stokes Simulation of a Supersonic Reacting Wall Jet. Combust. Flame 2021, 159, 1127–1138. [Google Scholar] [CrossRef]
- Zhang, L.; Choi, J.-Y.; Yang, V. Supersonic Combustion and Flame Stabilization of Coflow Ethylene and Air with Splitter Plate. J. Propuls. Power 2015, 31, 1242–1255. [Google Scholar] [CrossRef]
- Garby, R.; Selle, L.; Poinsot, T. Large-Eddy Simulation of Combustion Instabilities in a Variable-length Combustor. Comptes Rendus Mécanique. 2013, 341, 220–229. [Google Scholar] [CrossRef]
- Jeong, S.-M.; Kim, J.-E.; Lee, J.-H.; Choi, J.-Y. Analysis on Flow-Field Characteristics of a Scramjet Combustor with respect to Equivalence Ratio using IDDES Simulation. In Proceedings of the AIAA SCITECH 2023 Forum, National Harbor, MD, USA, 23–27 January 2023. [Google Scholar]
- Won, S.-H.; Jeung, I.-S.; Parent, B.; Choi, J.-Y. Numerical Investigation of Transverse Hydrogen Jet into Supersonic Crossflow Using Detached-Eddy Simulation. AIAA J. 2010, 48, 1047–1058. [Google Scholar] [CrossRef]
- Pavalavanni, P.K.; Sohn, C.H.; Lee, B.J.; Choi, J.-Y. Revisiting unsteady shock-induced combustion with modern analysis techniques. Proc. Combust. 2019, 37, 3637–3644. [Google Scholar] [CrossRef]
- Choi, J.-Y.; Unnikrishnan, U.; Hwang, W.-S.; Jeong, S.-M.; Han, S.-H.; Kim, K.H.; Yang, V. Effect of Fuel Temperature on Flame Characteristics of Supersonic Turbulent Combustion. Fuel 2022, 329, 125310–125329. [Google Scholar] [CrossRef]
- Potturi, A.; Edwards, J.R. Investigation of Subgrid Closure Models for Finite-Rate Scramjet Combustion. In Proceedings of the 43rd AIAA Fluid Dynamics Conference, San Deigo, CA, USA, 24–27 June 2013. [Google Scholar]
- Fulton, J.A.; Edwards, J.R.; Cutler, A.; McDaniel, J.; Goyne, C. Turbulence/chemistry interactions in a ramp-stabilized supersonic hydrogen–air diffusion flame. Combust. Flame 2016, 174, 152–165. [Google Scholar] [CrossRef]
- Li, Z.; Ferrarotti, M.; Cuoci, A.; Parente, A. Finite-rate chemistry modelling of non-conventional combustion regimes using a Partially-Stirred Reactor closure: Combustion model formulation and implementation detail. Appl. Energy 2018, 225, 637–655. [Google Scholar] [CrossRef]
- Wang, N.; Yang, T.; Ren, Z. Active Subspace Variation and Modeling Uncertainty in a Supersonic Flame Simulation. AIAA J. 2021, 59, 1798–1807. [Google Scholar] [CrossRef]
- Boukharfane, R.; Techer, A.; Er-raiy, A. LES of Reacting Flow in a Hydrogen Jet into Supersonic Crossflow Combustor Using a New Turbulent Combustion Model. Int. J. Aeronaut. Space Sci. 2021, 25, 115–128. [Google Scholar] [CrossRef]
- Gonzalez-Juez, E.D.; Kerstein, A.R.; Ranjan, R.; Menon, S. Advances and Challenges in Modeling High-speed Turbulent Combustion in Propulsion Systems. Prog. Energy Combust. Sci. 2017, 60, 26–67. [Google Scholar] [CrossRef]
- Chemical-Kinetic Mechanisms for Combustion Applications, San Diego Mechanism, Combustion Research Group, UC San Diego. Available online: http://web.eng.ucsd.edu/mae/groups/combustion/index.html (accessed on 26 June 2014).
- Spalart, P.R.; Jou, W.H.; Strelets, M.; Allmaras, S.R. Comments on the Feasibility of LES for Wings, and on a Hybrid RANS/LES Approach. In Proceedings of the First AFOSR International Conference on DNS/LES, Ruston, LA, USA, 4–8 August 1997. [Google Scholar]
- Menter, F.R. Two-equation Eddy-viscosity Turbulence Models for Engineering Applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Abe, K. A Hybrid LES/RANS Approach using an Anisotropy-resolving Algebraic Turbulence Model. Int. J. Heat Fluid. Flow. 2005, 26, 204–222. [Google Scholar] [CrossRef]
- Strelets, M. Detached Eddy Simulation of Massively Separated Flows. In Proceedings of the 39th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 8–11 January 2001. [Google Scholar]
- Menter, F.R.; Kuntz, M.; Langtry, R. Ten Years of Industrial Experience with the SST Turbulence Model. Turbul. Heat Mass. Transf. 2003, 4, 625–632. [Google Scholar]
- Gritskevich, M.S.; Garbaruk, A.V.; Schutze, J.; Menter, F.R. Development of DDES and IDDES Formulations for the k-ω Shear Stress Transport Model. Flow. Turbul. Combust. 2012, 88, 431–449. [Google Scholar] [CrossRef]
- Harten, A. High Resolution Schemes for Hyperbolic Conservation Laws. J. Comput. Phys. 1997, 135, 260–278. [Google Scholar] [CrossRef]
- Liu, X.-D.; Osher, S.; Chan, T. Weighted Essentially Non-oscillatory Schemes. J. Comput. Phys. 1994, 115, 200–212. [Google Scholar] [CrossRef]
- Jiang, G.-S.; Shu, C.-W. Efficient Implementation of Weighted ENO Schemes. J. Comput. Phys. 1996, 126, 202–228. [Google Scholar] [CrossRef]
- Balsara, D.S.; Shu, C.-W. Monotonicity Preserving Weighted Essentially Non-oscillatory Schemes with Increasingly High Order of Accuracy. J. Comput. Phys. 2000, 160, 405–452. [Google Scholar] [CrossRef]
- Kim, K.H.; Kim, C. Accurate, Efficient and Monotonic Numerical Methods for Multi-dimensional Compressible Flows Part II: Multi-dimensional Limiting Process. J. Comput. Phys. 2005, 208, 570–615. [Google Scholar] [CrossRef]
- Kim, S.; Lee, S.; Kim, K.H. Wavenumber-extended High-order Oscillation Control Finite Volume Schemes for Multi-dimensional Aeroacoustic Computations. J. Comput. Phys. 2008, 227, 4089–4122. [Google Scholar] [CrossRef]
- Wada, Y.; Liou, M.-S. A Flux Splitting Scheme with High-resolution and Robustness for Discontinuities. In Proceedings of the 32nd Aerospace Science Meeting & Exhibit, Reno, NV, USA, 10–13 January 1994. [Google Scholar]
- Jang, K.-J.; Kim, J.-K.; Cho, D.-R.; Choi, J.-Y. Optimization of LU-SGS Code for the Acceleration on the Modern Microprocessors. Int. J. Aeronaut. Space Sci. 2013, 14, 112–121. [Google Scholar] [CrossRef]
- Marshall, W.; Pal, S.; Woodward, R.; Santoro, R. Benchmark Wall Heat Flux Data for a GO2/GH2 Single Element Combustor. In Proceedings of the 41st AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Tucson, AZ, USA, 10–13 July 2005. [Google Scholar]
- Sung, B.-K.; Choi, J.-Y. Design of a Mach 2 Shape Transition Nozzle for Lab-scale Direct-connect Supersonic Combustor. Aerosp. Sci. Technol. 2021, 117, 106906–106918. [Google Scholar] [CrossRef]
- Evans, J.S.; Schexnayder, C.J. Influence of Chemical Kinetics and Unmixedness on Burning in Supersonic Hydrogen Flames. AIAA J. 1979, 18, 188–193. [Google Scholar] [CrossRef]
- Möbus, H.; Gerlinger, P.; Brüggemann, D. Scalar and Joint Scalar-velocity-frequency Monte Carlo PDF Simulation of Supersonic Combustion. Combust. Flame. 2003, 132, 3–24. [Google Scholar] [CrossRef]
- Koo, H.; Donde, P.; Raman, V. A Quadrature-based LES/transported Probability Density Function Approach for Modeling Supersonic Combustion. Proc. Combust. Inst. 2011, 33, 2203–2210. [Google Scholar] [CrossRef]
- Wang, H.; Wang, Z.; Sun, M.; Wu, H. Combustion Modes of Hydrogen Jet Combustion in a Cavity-based Supersonic Combustor. Int. J. Hydrog. Energy 2013, 38, 12078–12089. [Google Scholar] [CrossRef]
- Wang, Z.; Wang, H.; Sun, M. Review of Cavity-stabilized Combustion for Scramjet Applications. Proc. Inst. Mech. Eng. Part. G. J. Aerosp. Eng. 2014, 228, 2718–2735. [Google Scholar] [CrossRef]
- Li, F.; Sun, M.; Zhu, J.; Cai, Z.; Wang, H.; Zhang, Y.; Sun, Y. Scaling Effects on Combustion Modes in a Single-side Expansion Kerosene-fueled Scramjet Combustor. Chin. J. Aeronaut. 2021, 34, 684–690. [Google Scholar] [CrossRef]
- Yokev, N.; Brod, H.E.; Cao, D.; Michaels, D. Impact of Fuel Injection Distribution on Flame Holding in a Cavity-Stabilized Scramjet. J. Propuls. Power. 2021, 37, 584–594. [Google Scholar] [CrossRef]
- Barnes, F.W.; Segal, C. Cavity-based Flameholding for Chemically-reacting Supersonic Flows. Prog. Aerosp. Sci. 2015, 76, 24–41. [Google Scholar] [CrossRef]
- Yamashita, H.; Shimada, M.; Takeno, T. A Numerical Study on Flame Stability at the Transition Point of Jet Diffusion Flames. Symp. Inst. Combust. 1996, 26, 27–34. [Google Scholar] [CrossRef]
- Im, S.; Do, H. Unstart Phenomena Induced by Flow Choking in Scramjet Inlet-isolators. Prog. Aerosp. Sci. 2018, 97, 1–21. [Google Scholar] [CrossRef]
- Nichols, J.W.; Larsson, J.; Bernardini, M.; Pirozzoli, S. Stability and Modal Analysis of Shock/boundary Layer Interactions. Theor. Comput. Fluid Dyn. 2017, 31, 33–50. [Google Scholar] [CrossRef]
- Pasquariello, V.; Hickel, S.; Adams, N.A. Unsteady Effects of Strong Shock-wave/boundary-layer Interaction at High Reynolds Number. J. Fluid Mech. 2017, 823, 617–657. [Google Scholar] [CrossRef]
- Meng, Y.; Sun, W.; Gu, H.; Chen, F.; Zhou, R. Supersonic Combustion Mode Analysis of a Cavity Based Scramjet. Aerospace 2022, 9, 826. [Google Scholar] [CrossRef]






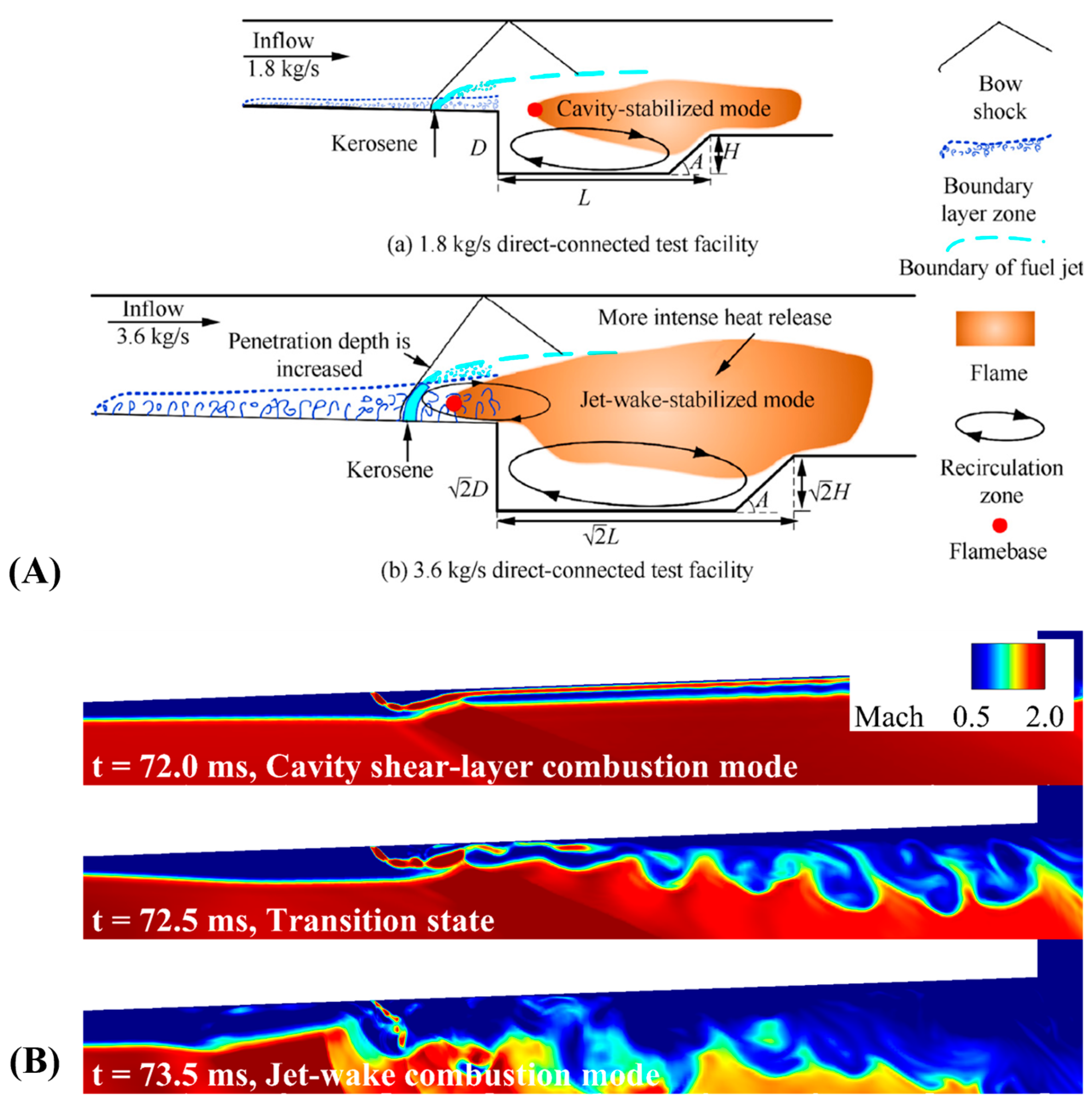
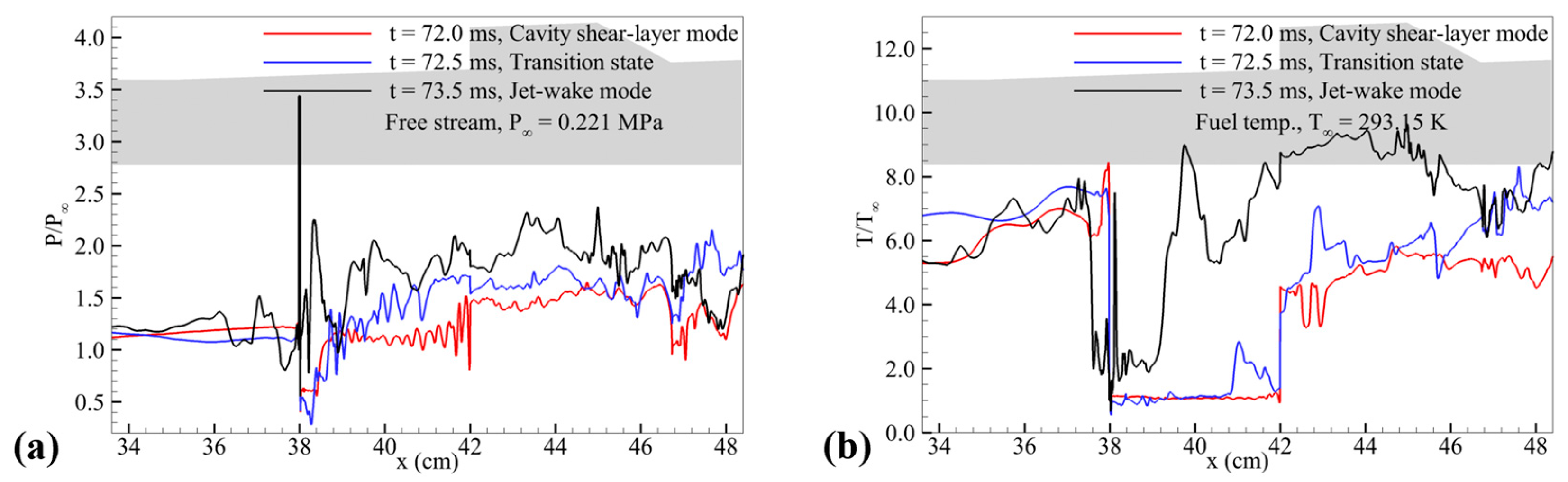

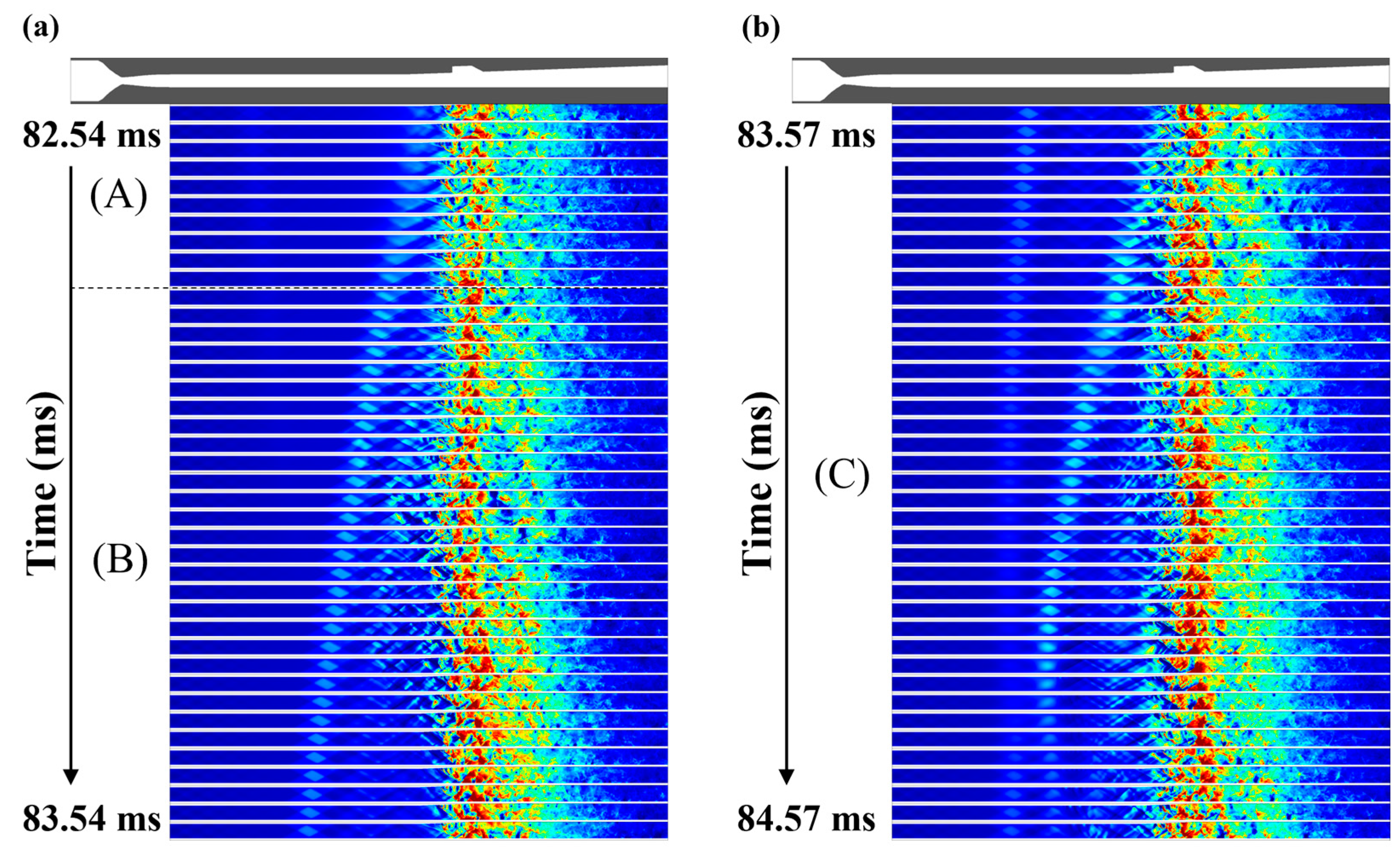
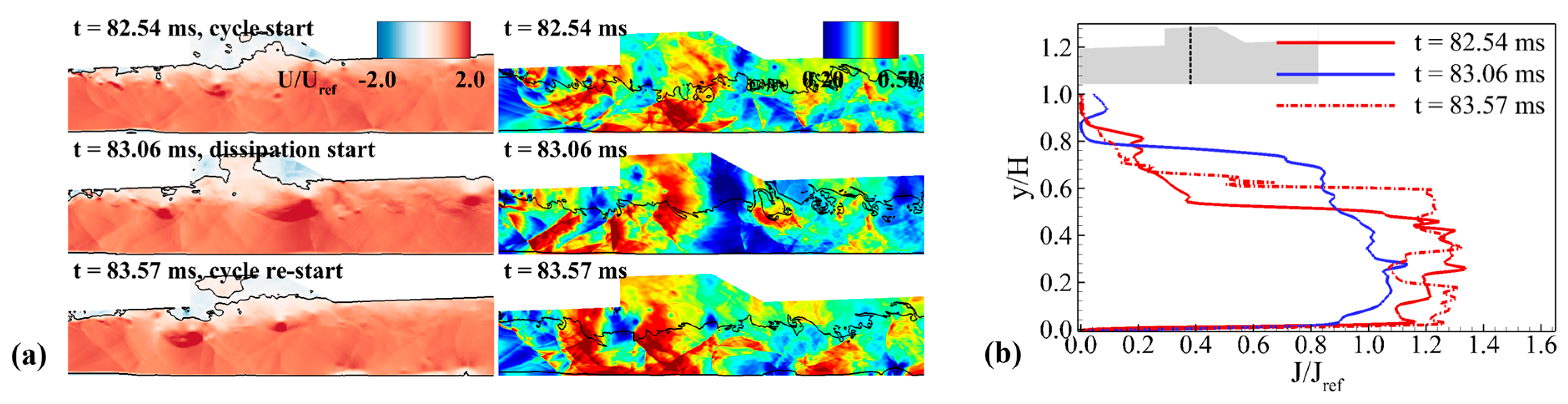
| VAH Downstream | Fuel Injection | ||||||
| 0.30 | 0.35 | 0.40 | 0.45 | 0.50 | |||
| Mach number | 0.01 | Sonic condition | |||||
| Temperature (T0) | 1675.0 K | 293.15 K | |||||
| Pressure (P0) | 17.25 bar | 5.0 bar | 5.9 bar | 6.7 bar | 7.6 bar | 8.4 bar | |
| Volume [%] | H2 | - | 100 | ||||
| N2 | 60.5 | - | |||||
| O2 | 21.1 | - | |||||
| H2O | 18.4 | - | |||||
| ϕ | Cavity Shear-Layer Comb. Mode (Hz) | Jet-Wake Comb. Mode (Hz) | ||
| Isolator | Combustor | Isolator | Combustor | |
| 0.40 | 222, 248, 492 | 250, 326, 444 | - | |
| 0.45 | 186, 266, 321 | 187, 285, 321 | 484, 615, 865 | 800, 835, 933 |
| 0.50 | 238, 384, 527 | 192, 381, 522 | 412, 460, 959 | 843, 821, 418 |
| Incoming Air (T0, Mach#) | Instability Frequency | Global ϕ | Combustion Mode | |
| Lin et al. [5] | 1016.6 K, Mach# 2.2 | 120 Hz | 0.62 | Did not mention |
| 260, 292 Hz | 0.80 | |||
| Micka et al. [7] | 1200 K, Mach# 2.2 | 200~250 Hz | 0.60 0.60 | Cavity shear-layer |
| 1400 K, Mach# 2.2 | 1000 Hz | Jet-wake | ||
| Nakaya et al. [9] | 50 K, Mach# 2.0 | 100~500 Hz | 0.07 | Cavity shear-layer |
| 100~1800 Hz | 0.15 | Jet-wake | ||
| 100~1800 Hz | 0.25 | Jet-wake | ||
| Meng et al. [71] | 1249 K, Mach # 2.5 | 150, 270, 480 Hz | 0.10 | Cavity shear-layer |
| 180, 300, 459 Hz | 0.14 | Cavity shear-layer | ||
| 300, 510 Hz | 0.20 | Jet-wake | ||
| Present | 1675 K, Mach# 2.0 | 187~321 Hz | 0.45 | Cavity shear-layer |
| 800~933 Hz | 0.45 | Jet-wake |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jeong, S.-M.; Han, H.-S.; Sung, B.-K.; Kim, W.; Choi, J.-Y. Reactive Flow Dynamics of Low-Frequency Instability in a Scramjet Combustor. Aerospace 2023, 10, 932. https://doi.org/10.3390/aerospace10110932
Jeong S-M, Han H-S, Sung B-K, Kim W, Choi J-Y. Reactive Flow Dynamics of Low-Frequency Instability in a Scramjet Combustor. Aerospace. 2023; 10(11):932. https://doi.org/10.3390/aerospace10110932
Chicago/Turabian StyleJeong, Seung-Min, Hyung-Seok Han, Bu-Kyeng Sung, Wiedae Kim, and Jeong-Yeol Choi. 2023. "Reactive Flow Dynamics of Low-Frequency Instability in a Scramjet Combustor" Aerospace 10, no. 11: 932. https://doi.org/10.3390/aerospace10110932
APA StyleJeong, S.-M., Han, H.-S., Sung, B.-K., Kim, W., & Choi, J.-Y. (2023). Reactive Flow Dynamics of Low-Frequency Instability in a Scramjet Combustor. Aerospace, 10(11), 932. https://doi.org/10.3390/aerospace10110932







