Impact-Rebound Momentum Excitation Based Inertial Parameters and State Estimation of Defunct Space Object
Abstract
:1. Introduction
2. Problem Analysis and System Modeling
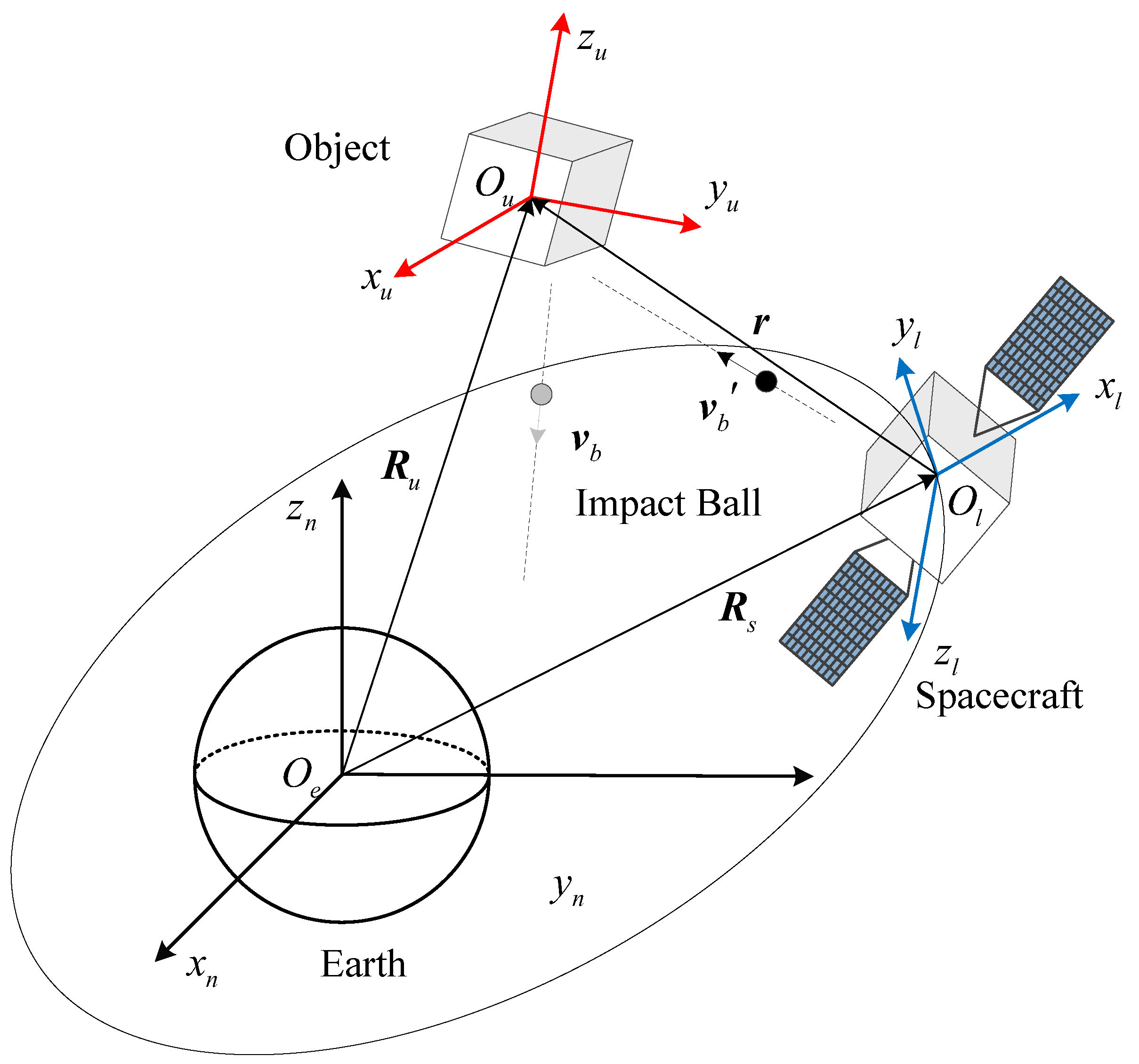
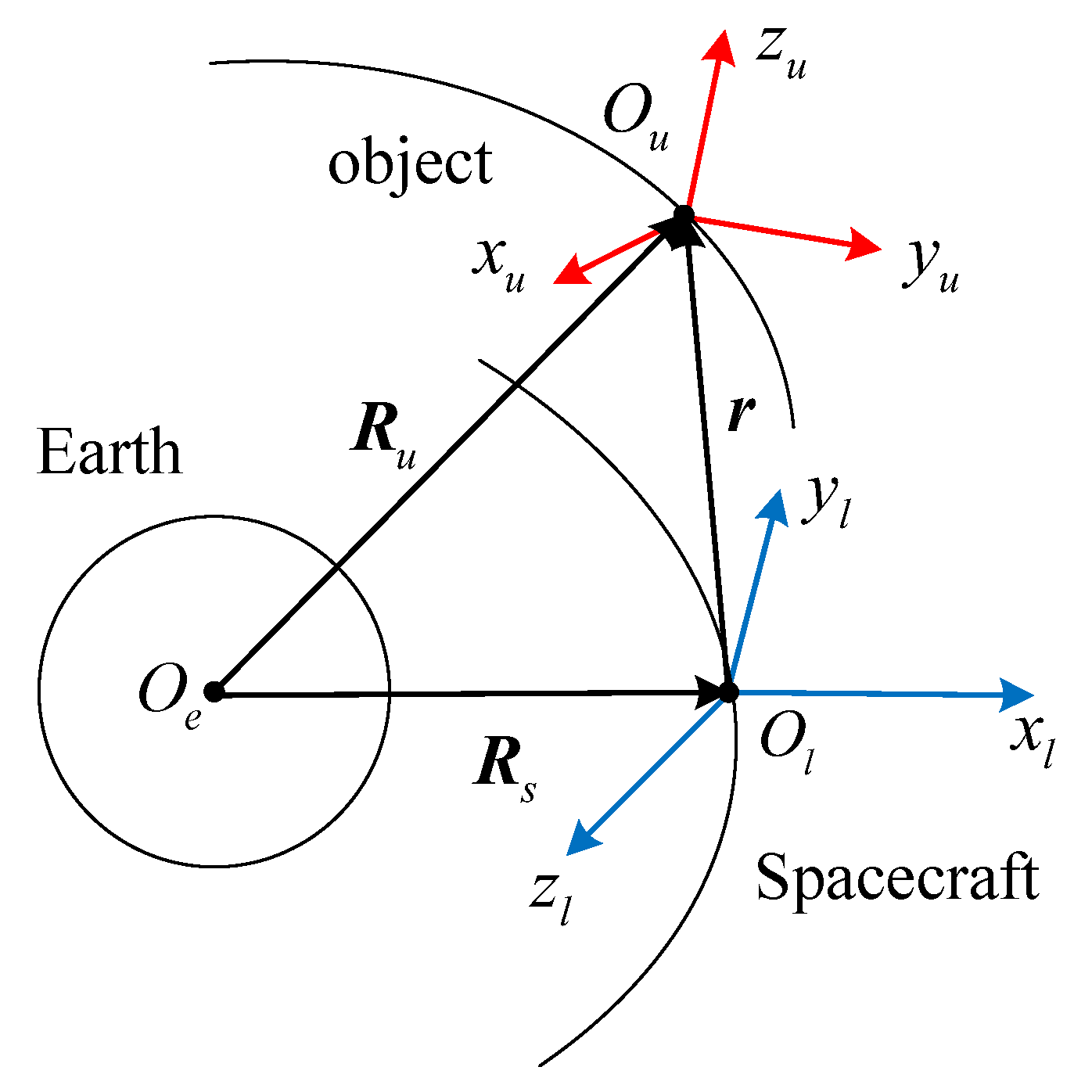
2.1. Coordinate Frames
2.2. Attitude Motion
2.3. Orbit Motion
3. Parameter Estimation Filter Design
3.1. Observation Equations Based on Measurements
3.2. Observation Equation Based on Angular Momentum Conservation Model
3.2.1. Before the Collision
3.2.2. After the Collision
3.3. Filter Design
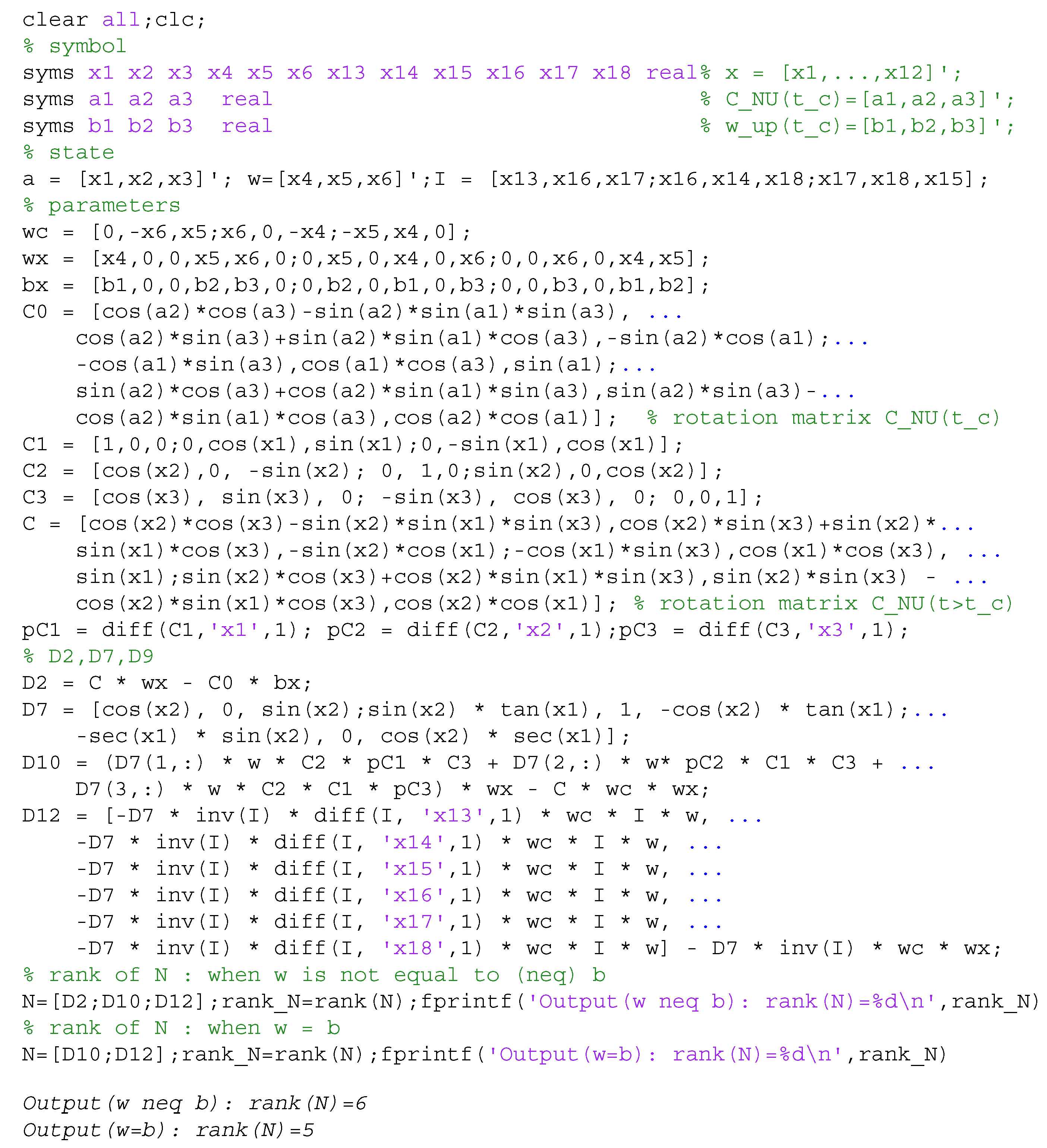
4. Observability Analysis
4.1. The Observability of Estimator after the Collision
4.2. The Observability of Estimator before the Collision
5. Numerical Simulations
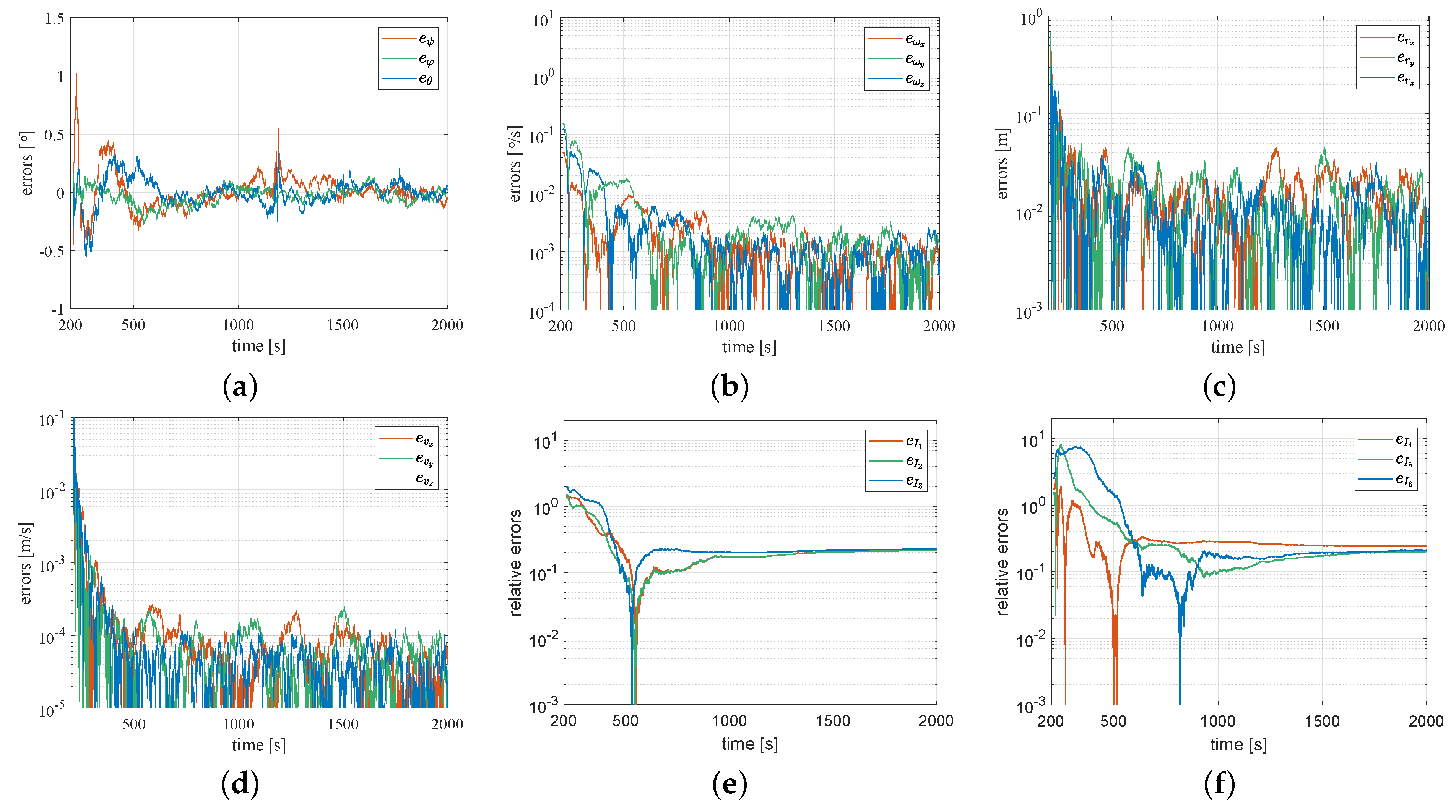
5.1. Estimation Results
5.1.1. Before the Collision
5.1.2. After the Collision
5.2. The Influence of Initial Values of Inertial Parameters
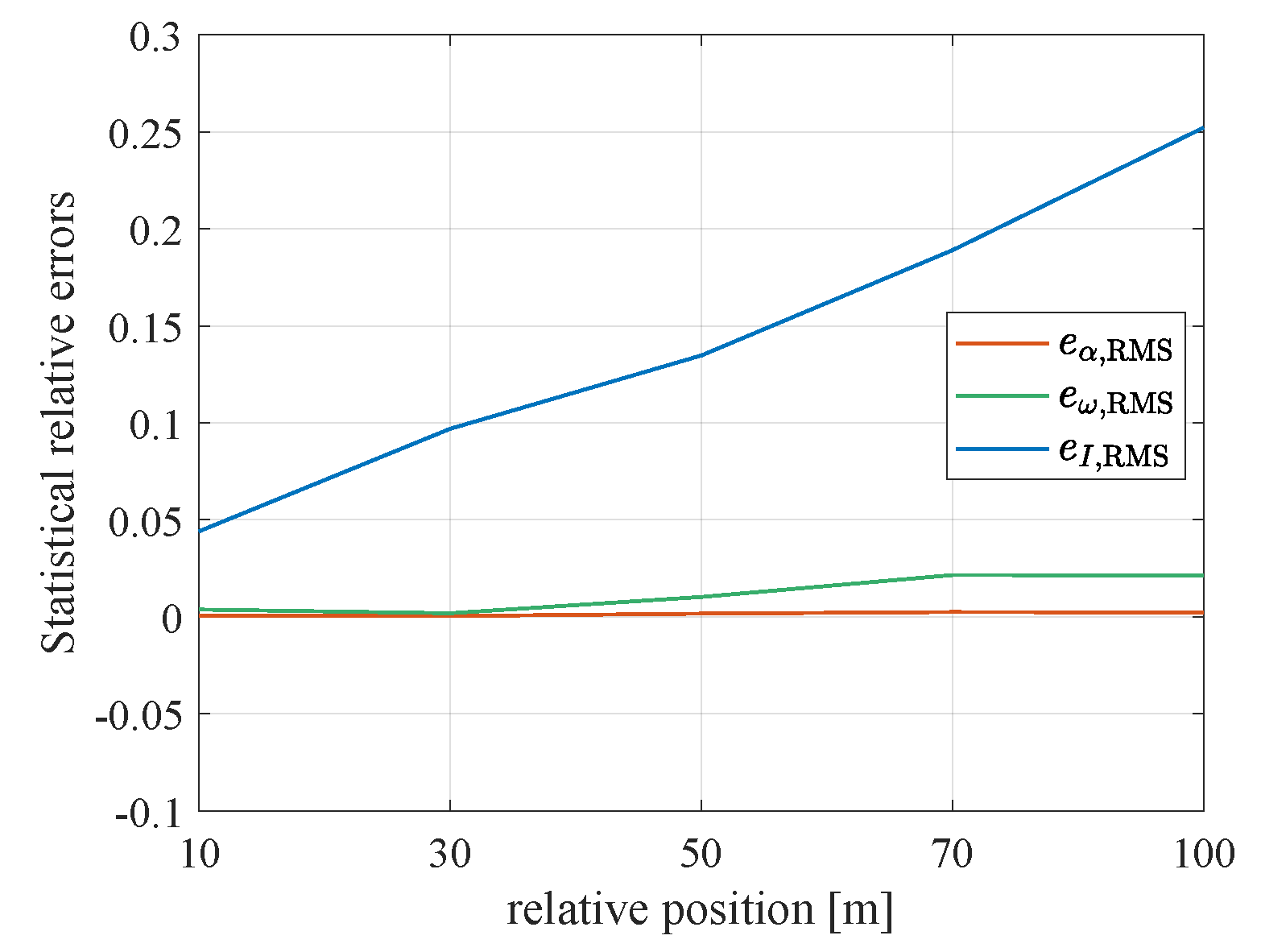
5.3. The Influence of Relative Distance
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. The Program of Verifying the Observability of the Estimation Model
References
- Dubowsky, S.; Papadopoulos, E. The kinematics, dynamics, and control of free-flying and free-floating space robotic systems. IEEE Trans. Robot. Autom. 1993, 9, 531–543. [Google Scholar] [CrossRef]
- Zhu, L.; Wang, S. Rotating Object Specific Tracking Based on Orbit-Attitude Coordinated Adaptive Control. J. Guid. Control Dyn. 2021, 44, 266–282. [Google Scholar] [CrossRef]
- Zhang, S.; Wang, S. Two-Craft Lorentz Force Formation Dynamics and Control. In Proceedings of the IEEE Chinese Guidance, Navigation and Control Conference, Nanjing, China, 12–14 August 2016. [Google Scholar] [CrossRef]
- Philip, N.; Ananthasayanam, M. Relative position and attitude estimation and control schemes for the final phase of an autonomous docking mission of spacecraft. Acta Astronaut. 2003, 52, 511–522. [Google Scholar] [CrossRef]
- Lichter, M.D.; Dubowsky, S. State, shape, and parameter estimation of space objects from range images. In Proceedings of the IEEE International Conference on Robotics and Automation, New Orleans, LA, USA, 26 April–1 May 2004. [Google Scholar] [CrossRef] [Green Version]
- Wilson, E.; Sutter, D.W.; Mah, R.W. MCRLS for On-line Spacecraft Mass-and Thruster-Property Identification. In Proceeding of the IASTED International Conference on Intelligent Systems and Control, Honolulu, HI, USA, 1–6 August 2004. [Google Scholar]
- Wilson, E.; Sutter, D.W.; Mah, R.W. Motion-Based Mass and Thruster-Property Identification for Thruster-Controlled Spacecraft. In Proceedings of the AIAA Infotech @Aerospace Conference, Arlington, VA, USA, 26–29 September 2005. [Google Scholar] [CrossRef]
- Sheinfeld, D.; Rock, S. Rigid Body Inertia Estimation with Applications to the Capture of a Tumbling Satellite. In Proceedings of the 19th AAS/AIAA Spaceflight Mechanics Meeting, Savannah, GA, USA, 8–12 February 2009. [Google Scholar]
- Benninghoff, H.; Boge, T. Rendezvous Involving a Non-Cooperative, Tumbling Target-Estimation of Moments of Inertia and Center of Mass of an Unknown Target. In Proceedings of the International Symposium on Space Flight Dynamics, Munich, Germany, 19–23 October 2015. [Google Scholar]
- Pesce, V.; Lavagna, M.; Bevilacqua, R. Stereovision-based pose and inertia estimation of unknown and uncooperative space objects. Adv. Space Res. 2017, 59, 236–251. [Google Scholar] [CrossRef]
- Hou, X.; Ma, C.; Wang, Z.; Yuan, J. Adaptive pose and inertial parameters estimation of free-floating tumbling space objects using dual vector quaternions. Adv. Mech. Eng. 2017, 9, 1–17. [Google Scholar] [CrossRef]
- Yuan, J.; Hou, X.; Sun, C.; Cheng, Y. Fault-tolerant pose and inertial parameters estimation of an uncooperative spacecraft based on dual vector quaternions. J. Aerosp. Eng. 2018, 233, 1250–1269. [Google Scholar] [CrossRef]
- Nocerino, A.; Opromolla, R.; Fasano, G.; Grassi, M. LIDAR-based multi-step approach for relative state and inertia parameters determination of an uncooperative target. Acta Astronaut. 2021, 181, 662–678. [Google Scholar] [CrossRef]
- Nocerino, A.; Opromolla, R.; Fasano, G.; Grassi, M. Experimental validation of inertia parameters and attitude estimation of uncooperative space targets using solid state LIDAR. In Proceedings of the 73rd International Astronautical Congress, Paris, France, 18–22 September 2022. [Google Scholar]
- Hillenbrand, U.; Lampariello, R. Motion and Parameter Estimation of a Free-Floating Space Object from Range Data for Motion Prediction. In Proceedings of the International Symposium on Artificial Intelligence, Robotics and Automation in Space, Munich, Germany, 5–8 September 2005. [Google Scholar]
- Zhou, B.; Cai, G.; Liu, Y.; Liu, P. Motion prediction of a non-cooperative space target. Adv. Space Res. 2018, 61, 207–222. [Google Scholar] [CrossRef]
- Murotsu, Y.; Tsujio, S.; Senda, K.; Mitsuya, A. System identification and resolved acceleration control of space robots by using experimental system. In Proceedings of the IEEE/RSJ International Workshop on Intelligent Robots and Systems, Osaka, Japan, 3–5 November 1991. [Google Scholar] [CrossRef]
- Murotsu, Y.; Senda, K.; Ozaki, M.; Tsujio, S. Parameter identification of unknown object handled by free-flying space robot. J. Guid. Control Dyn. 1994, 17, 488–494. [Google Scholar] [CrossRef]
- Yoshida, K.; Abiko, S. Inertia parameter identification for a free-flying space robot. In Proceedings of the AIAA Guidance, Navigation, and Control Conference, Monterey, CA, USA, 5–8 August 2002. [Google Scholar] [CrossRef]
- Nguyenhuynh, T.C.; Sharf, I. Adaptive Reactionless Motion and Parameter Identification in Postcapture of Space Debris. J. Guid. Control Dyn. 2013, 36, 404–414. [Google Scholar] [CrossRef]
- Felicetti, L.; Sabatini, M.; Pisculli, A.; Gasbarri, P.; Palmerini, G.B. Adaptive Thrust Vector Control during On-Orbit Servicing. In Proceedings of the AIAA SPACE 2014 Conference and Exposition, San Diego, CA, USA, 4–7 August 2014. [Google Scholar] [CrossRef]
- Meng, Q.; Liang, J.; Ma, O. Identification of all the inertial parameters of a non-cooperative object in orbit. Aerosp. Sci. Technol. 2019, 91, 571–582. [Google Scholar] [CrossRef]
- Meng, Q.; Zhao, C.; Ji, H.; Liang, J. Identify the Full Inertial Parameters of a Non-cooperative Target with Eddy Current Detumbling. Adv. Space Res. 2020, 66, 1792–1802. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, X.; Chen, P.; Cai, G. Inertia Parameter Identification for an Unknown Satellite in Precapture Scenario. Int. J. Aerosp. Eng. 2020, 2020, 7521434. [Google Scholar] [CrossRef]
- Xu, B.; Wang, S. Vision-Based Moment of Inertia Estimation of Non-Cooperative Space Object. In Proceedings of the International Symposium on Computational Intelligence and Design, Hangzhou, China, 9–10 December 2017. [Google Scholar] [CrossRef]
- Xu, B.; Wang, S.; Zhao, L. Mechanism of collision and adhesion between adhesive impact ball and spacecraft. In Proceedings of the 3rd International Conference on Applied Mechanics and Mechanical Engineering, Xi’an, China, 23–25 December 2022. [Google Scholar]
- Schaub, H.; Junkins, J. Analytical Mechanics of Space Systems, 4th ed.; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2018. [Google Scholar] [CrossRef]
- Fisher, J.; Vadali, S.R. Gyroless Attitude Control of Multi-Body Satellites Using an Unscented Kalman Filter. J. Guid. Control Dyn. 2008, 31, 245–251. [Google Scholar] [CrossRef]
- Soken, H.E.; Cilden, D.; Hajiyev, C. Attitude Estimation for Nanosatellites Using Singular Value Decomposition and Unscented Kalman Filter. In Proceedings of the International Symposium on Space Technology and Science, Hyogo, Japan, 4 July 2015. [Google Scholar]
- Hermann, R.; Krener, A. Nonlinear controllability and observability. IEEE Trans. Autom. Control 1977, 22, 728–740. [Google Scholar] [CrossRef] [Green Version]
- Kelly, J.; Sukhatme, G.S. Visual-Inertial Sensor Fusion: Localization, Mapping and Sensor-to-Sensor Self-calibration. Int. J. Robot. Res. 2010, 30, 56–79. [Google Scholar] [CrossRef]

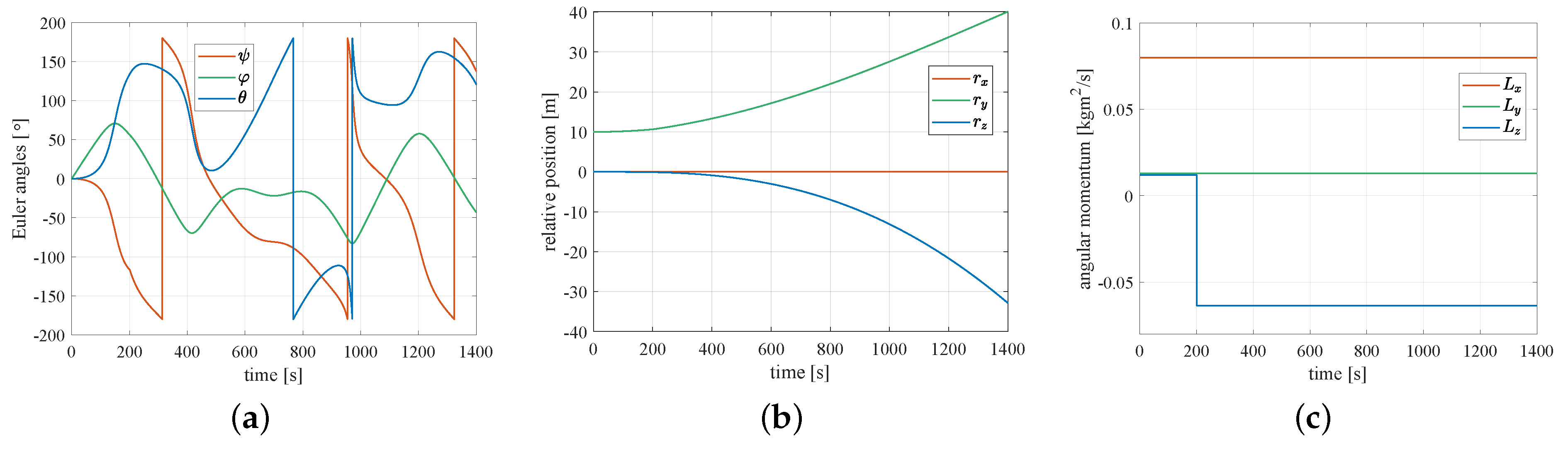
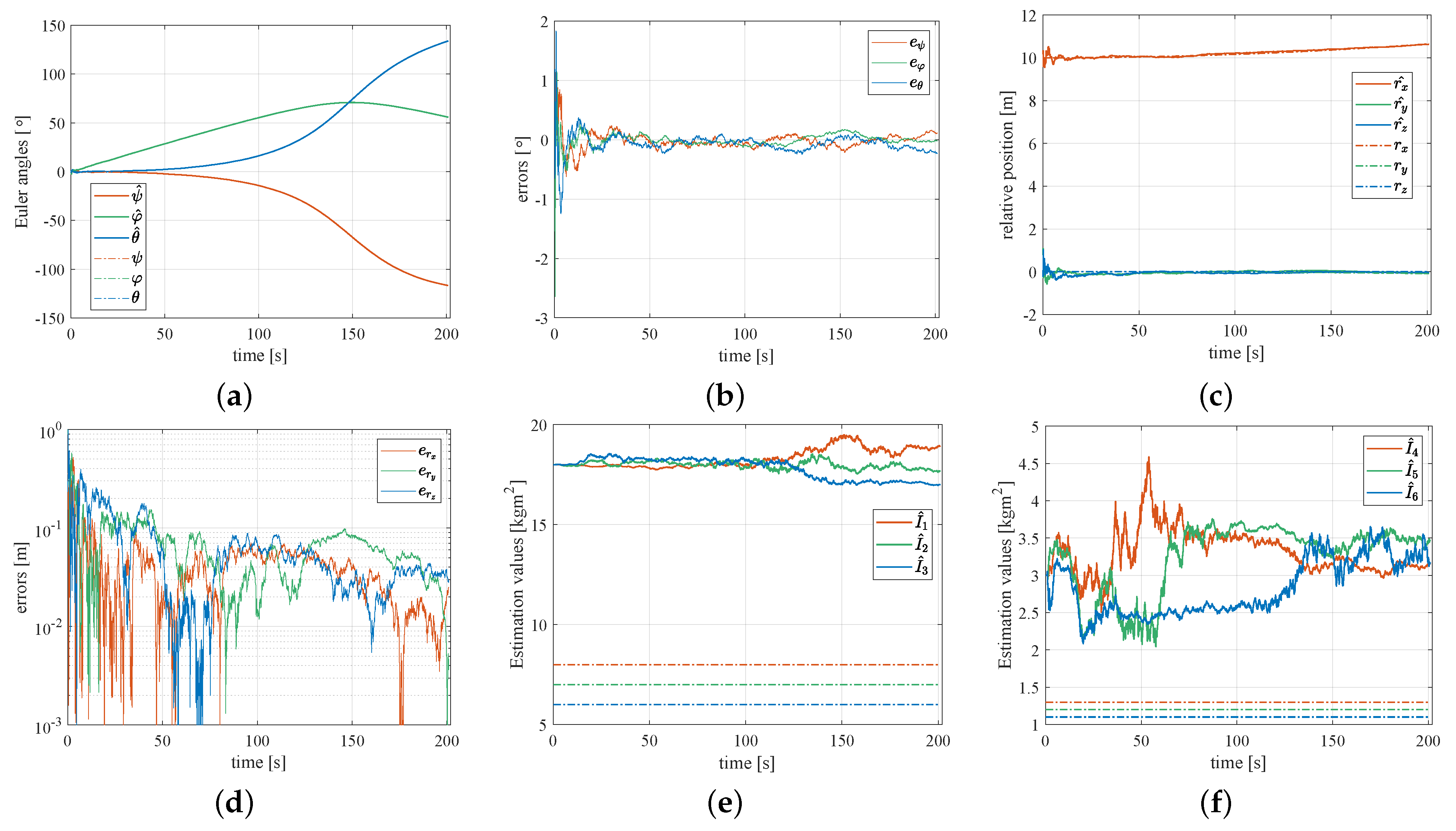
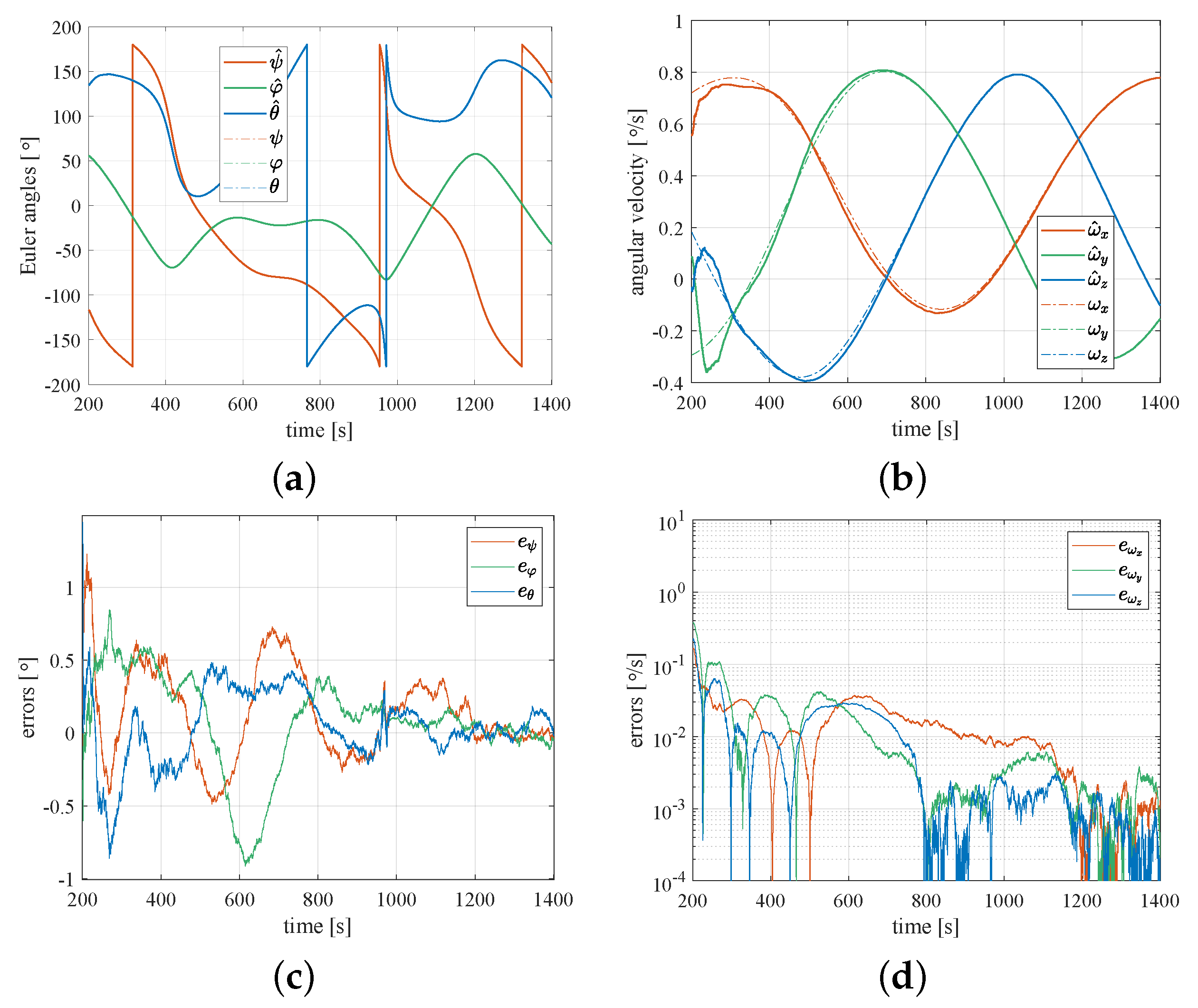

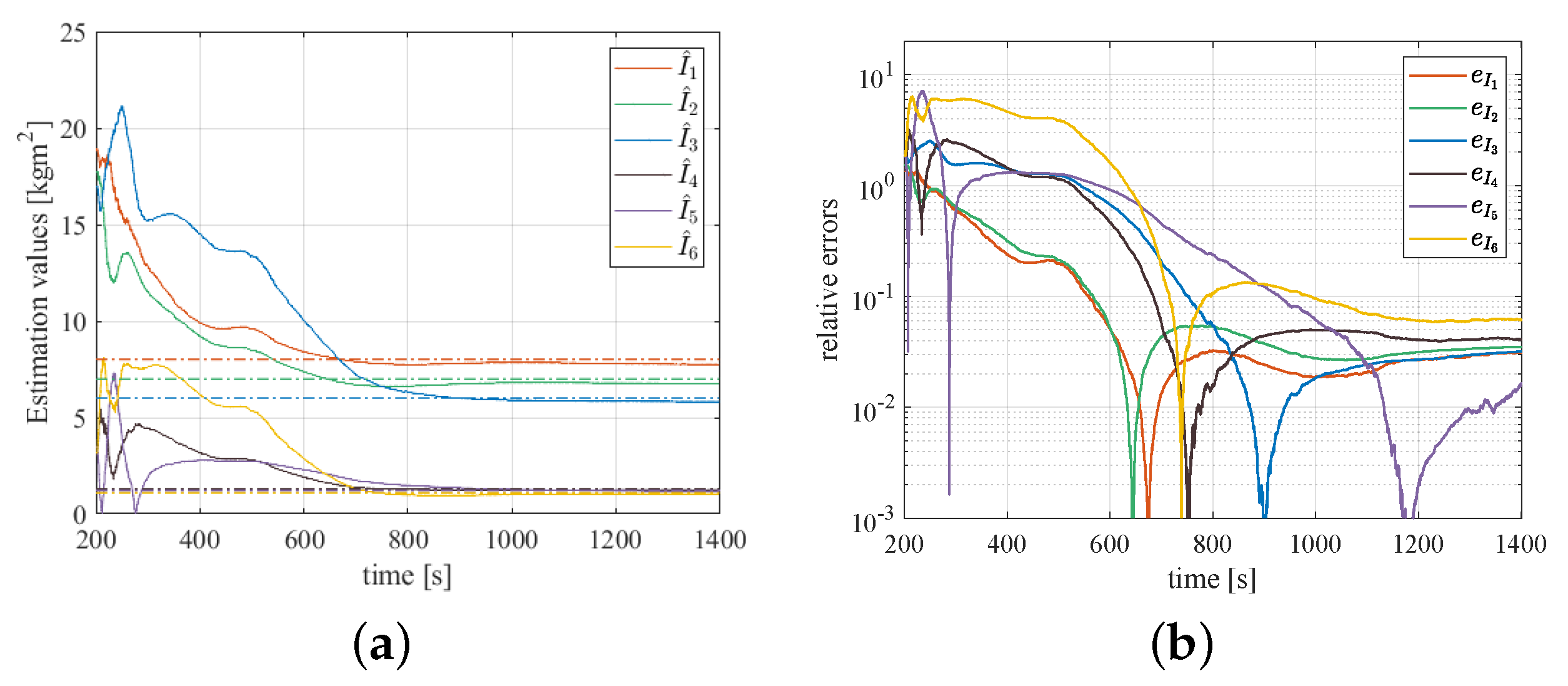
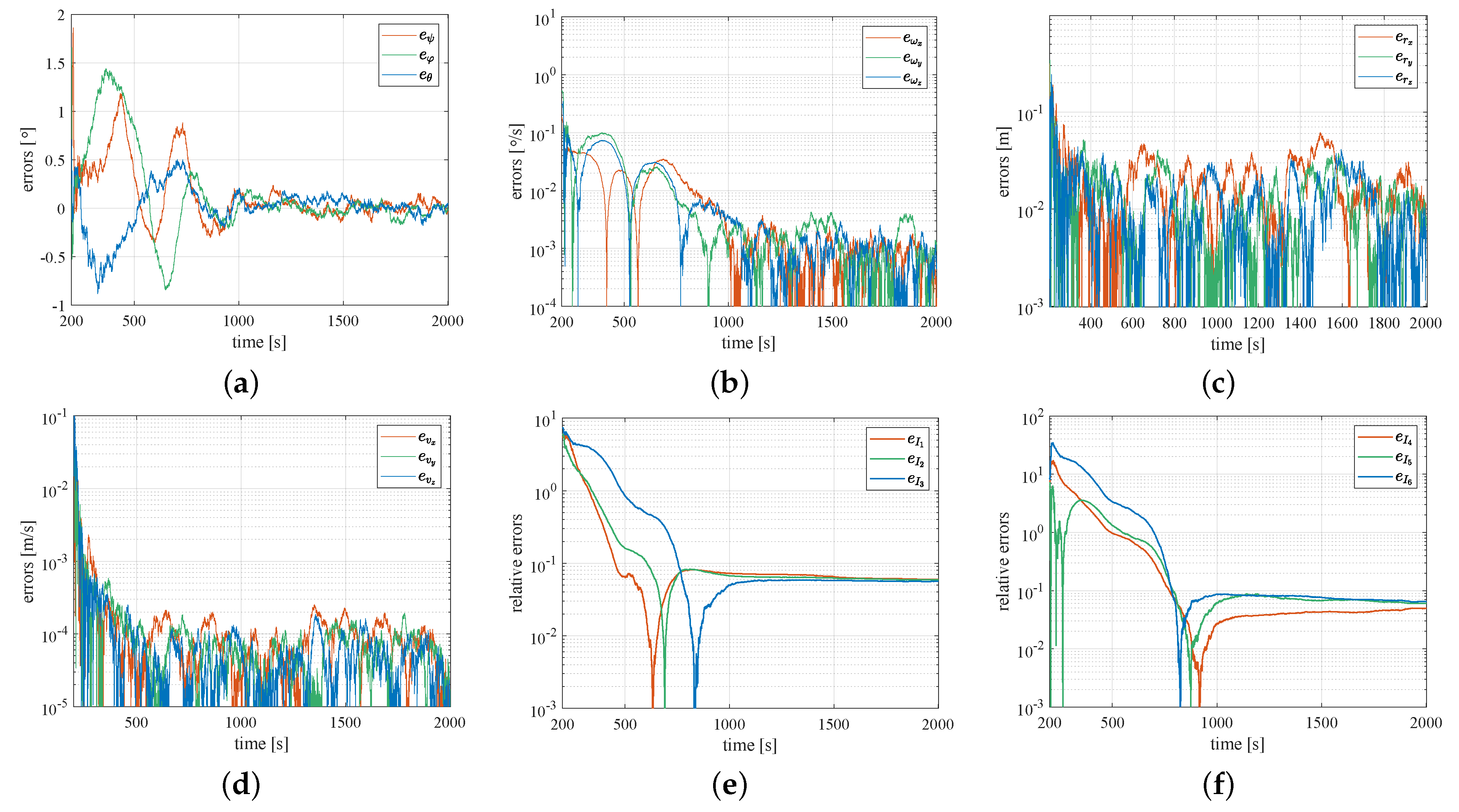
| Impact Ball | Object | |
|---|---|---|
| Euler angles (°) | ||
| Angular velocity (°/s) | ||
| Relative position (m) | ||
| Relative velocity (m/s) | ||
| Inertial tensor (kgm2) |
| Estimation Error | (°) | (°/s) | (m) | (m/s) | |
|---|---|---|---|---|---|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, B.; Wang, S.; Zhao, L.; Zhang, L. Impact-Rebound Momentum Excitation Based Inertial Parameters and State Estimation of Defunct Space Object. Aerospace 2023, 10, 38. https://doi.org/10.3390/aerospace10010038
Xu B, Wang S, Zhao L, Zhang L. Impact-Rebound Momentum Excitation Based Inertial Parameters and State Estimation of Defunct Space Object. Aerospace. 2023; 10(1):38. https://doi.org/10.3390/aerospace10010038
Chicago/Turabian StyleXu, Bingyu, Shuquan Wang, Liping Zhao, and Long Zhang. 2023. "Impact-Rebound Momentum Excitation Based Inertial Parameters and State Estimation of Defunct Space Object" Aerospace 10, no. 1: 38. https://doi.org/10.3390/aerospace10010038
APA StyleXu, B., Wang, S., Zhao, L., & Zhang, L. (2023). Impact-Rebound Momentum Excitation Based Inertial Parameters and State Estimation of Defunct Space Object. Aerospace, 10(1), 38. https://doi.org/10.3390/aerospace10010038






