The Moist Adiabat, Key of the Climate Response to Anthropogenic Forcing
Abstract
1. Introduction
1.1. State of the Art
1.2. The Response of the Climate System
2. Data
3. Method
3.1. Representation of the Surface Air Temperature (SAT) Anomalies
3.2. Representation of the Precipitations
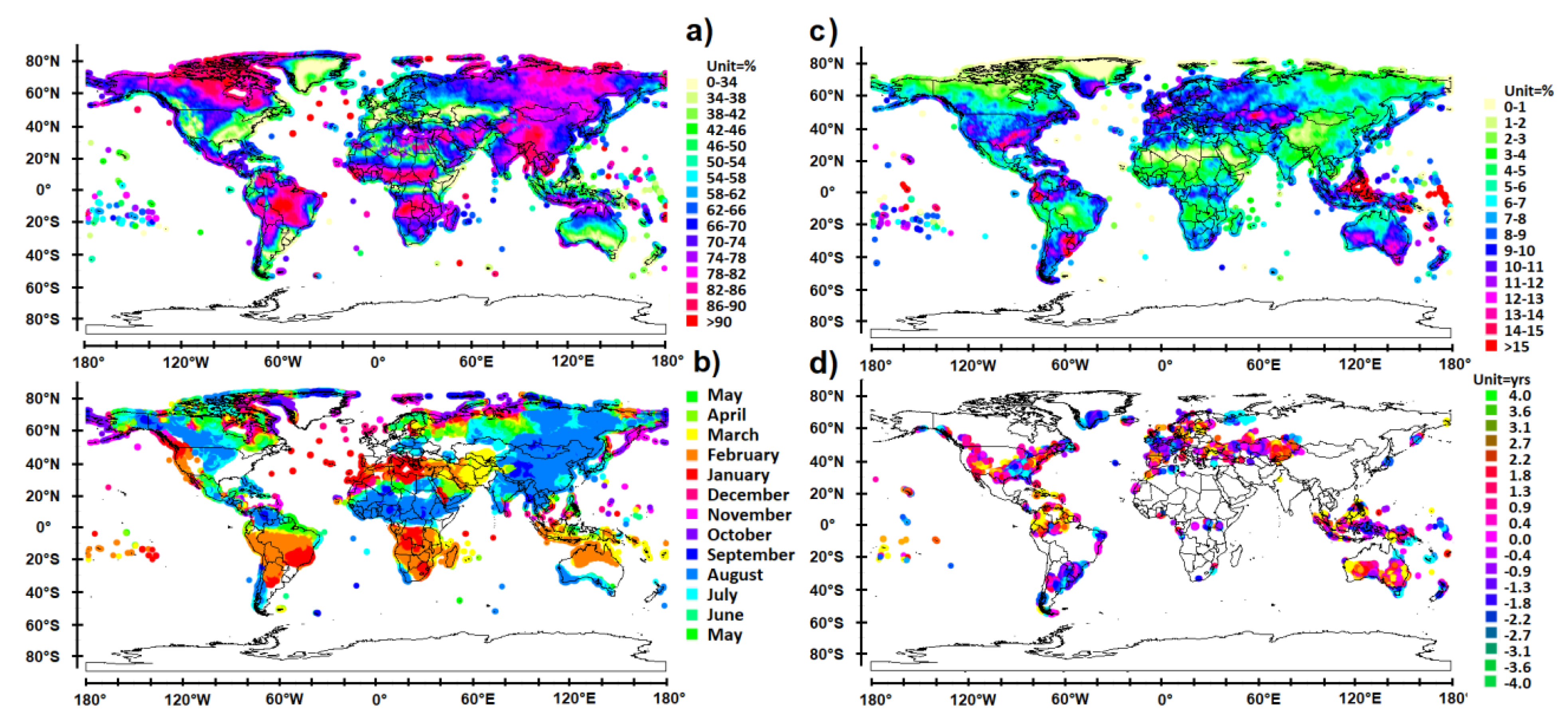
3.2.1. Regions Subject to Rainfall Oscillation
3.2.2. Regions Subject to Seasonal Rainfall
3.2.3. Complementarity of the Two Cycles
4. Results and Discussion
4.1. Evolution of the Moist Adiabat
4.2. Latitudinal Variations of SAT
4.3. Longitudinal Variations of SAT
4.4. Anthropogenic Forcing Efficiency
4.4.1. Seasonal Low-Pressure Systems
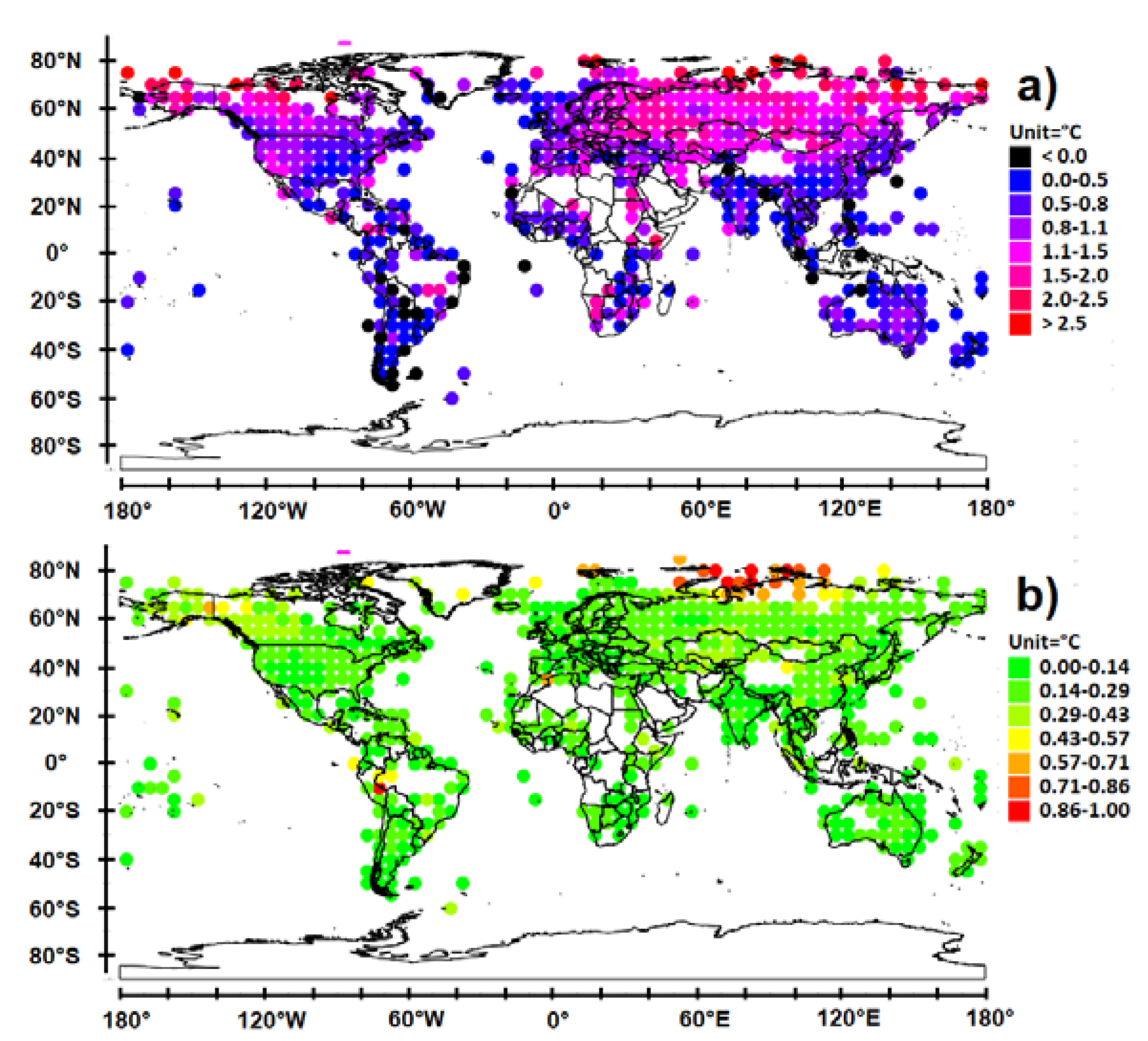
- The low SAT of regions most impacted by polar vortices are subject to a very high sensitivity to anthropogenic forcing > 2.0 °C/50 years from Figure 1 ( is increase in SAT resulting from the anthropogenic forcing).
- Large landmasses in temperate latitudes are significantly affected by anthropogenic forcing 1.1 °C/50 years < < 2.0 °C/50 years.
- The impact of anthropogenic forcing in the monsoon trough or the intertropical convergence zone is low within the tropical belt but increases significantly as the latitude increases as occurs in South America and central Africa, up to 35° S. It is −0.5 °C/50 years < < 0.8 °C/50 years within the tropical belt and 0.8 °C/50 years < < 1.5 °C/50 years at higher latitudes.
- Regions concerned by thermal lows are heavily impacted by anthropogenic forcing because in such dry regions the convective condensation level is elevated several kilometers so that the difference between SAT and may reach up to 35 °C. Consequently 1.1 °C/50 years < < 2.0 °C/50 years.
- Regions impacted by tropical cyclones are also affected by monsoon troughing so that the anthropogenic impact is the same in both cases −0.5 °C/50 years < < 0.8 °C/50 years within the tropical belt.
4.4.2. Subtropical Depressions and Extratropical Cyclones
4.4.3. The Case of the Antarctic
5. Conclusions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Radiative Forcing Efficiency
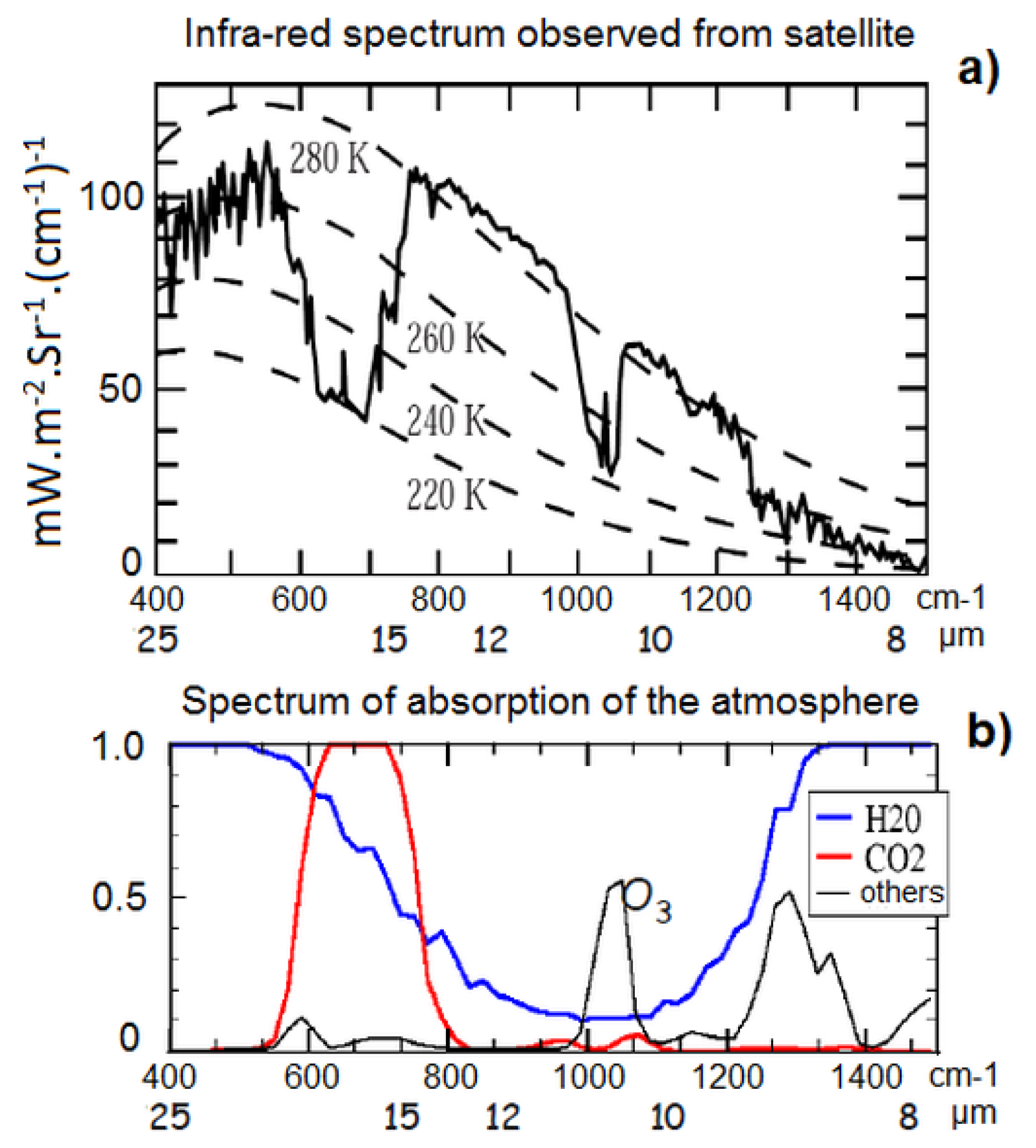
Appendix B. The Differential Fluxes Resulting from the SAT Anomaly
| SAT °C | ΔT °C | ||||||
|---|---|---|---|---|---|---|---|
| 12 | 0.14 | −45 | −0.09 | −50 | −0.46 | −15 | −0.30 |
Appendix C. The Moist Adiabatic Lapse Rate Versus Temperature
- wet adiabatic lapse rate, K/mEarth’s gravitational acceleration = 9.8076 m/s2heat of vaporization of water = 2501000 J/kgspecific gas constant of dry air = 287 J/kg·Kspecific gas constant of water vapor = 461.5 J/kg·Kthe dimensionless ratio of the specific gas constant of dry air to the specific gas constant for water vapor = 0.622the water vapor pressure of the saturated air
- ; is the temperature of the saturated air in °Cthe mixing ratio of the mass of water vapor to the mass of dry air [29]the pressure of the saturated airtemperature of the saturated air, Kthe specific heat of dry air at constant pressure, = 1003.5 J/kg·K
References
- Pinault, J.-L. Anthropogenic and natural radiative forcing: Positive feedbacks. J. Mar. Sci. Eng. 2018, 6, 146. [Google Scholar] [CrossRef]
- Stephens, G.L. Cloud feedbacks in the climate system: A critical review. J. Clim. 2005. [Google Scholar] [CrossRef]
- Stocker, T.F.; Clarke, G.K.C.; Treut, H.L.; Lindzen, R.S.; Meleshko, V.P.; Mugara, P.K.; Palmer, T.N.; Pierrehumbert, R.T.; Sellers, P.J.; Trenberth, K.E.; et al. Physical climate processes and feedbacks. In Climate Change 2001: The Scientific Basis, Contributions of Working Group I to the Third Assessment Report of the Intergovernmental Panel on Climate Change; Houghton, J.T., Ed.; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Benestad, R.E. A mental picture of the greenhouse effect. Theor. Appl. Climatol. 2017, 128, 679–688. [Google Scholar] [CrossRef]
- Hanel, R.A.; Schlachman, B.; Rogers, D.; Vanous, D. The Nimbus 4 Michelson interferometer. Appl. Opt. 1971, 10, 1376–1382. [Google Scholar] [CrossRef] [PubMed]
- Kyle, H.L.; Hickey, J.R.; Ardanuy, P.E.; Jacobwitz, H.; Arking, A.; Campbell, G.G.; House, F.B.; Maschhoff, R.; Smith, G.L.; Stowe, L.L.; et al. The nimbus earth radiation budget (ERB) experiment: 1975 to 1992. 1993. [Google Scholar] [CrossRef]
- Taylor, P.C.; Ellingson, R.G.; Cai, M. Seasonal variations of climate feedbacks in the NCAR CCSM3. J. Clim. 2011, 24, 3433–3444. [Google Scholar] [CrossRef]
- Betts, A.K. Saturation point analysis of moist convective overturning. J. Atm. Sci. 1982, 39, 1484–1505. [Google Scholar] [CrossRef]
- Jones, P.D.; Lister, D.H.; Osborn, T.J.; Harpham, C.; Salmon, M.; Morice, C.P. Hemispheric and large-scale land surface air temperature variations: An extensive revision and an update to 2010. J. Geophys. Res. 2012, 117, D05127. Available online: www.metoffice.gov.uk/hadobs/crutem4/data/ (accessed on 13 March 2020). [CrossRef]
- National Oceanic and Atmospheric Administration, Earth System Research Laboratory, Physical Sciences Division. Available online: https://www.esrl.noaa.gov/psd/ (accessed on 13 March 2020).
- National Oceanic and Atmospheric Administration. Available online: ftp://ftp.cpc.ncep.noaa.gov/precip/cmap/monthly/ (accessed on 13 March 2020).
- Pinault, J.-L. Resonantly forced Baroclinic waves in the oceans: Subharmonic modes. J. Mar. Sci. Eng. 2018, 6, 78. [Google Scholar] [CrossRef]
- Torrence, C.; Compo, G.P. A practical guide for wavelet analysis. Bull. Am. Meteorol. Soc. 1998, 79, 61–78. [Google Scholar] [CrossRef]
- Pinault, J.L. Global warming and rainfall oscillation in the 5–10 yr band in Western Europe and Eastern North America. Clim. Chang. 2012, 114, 621–650. [Google Scholar] [CrossRef]
- Gamage, N.; Blumen, W. Comparative analysis of low-level cold fronts: Wavelet, Fourier, and empirical orthogonal function decompositions. Mon. Weather Rev. 1993, 121, 2867–2878. [Google Scholar] [CrossRef]
- Pinault, J.-L. Regions subject to rainfall oscillation in the 5–10 year band. Climate 2018, 6, 2. [Google Scholar] [CrossRef]
- Gill, A.E. Atmosphere–Ocean Dynamics; International Geophysics Series, 30; Academic Press: Cambridge, MA, USA, 1982; p. 662. [Google Scholar]
- Polar Vortex. Glossary of Meteorology. American Meteorological Society. Available online: http://glossary.ametsoc.org/wiki/Polar_vortex (accessed on 13 March 2020).
- Intertropical Convergence Zone, Wikipedia. Available online: https://en.wikipedia.org/wiki/Intertropical_Convergence_Zone (accessed on 13 March 2020).
- Davis, M.E.; Thompson, L.G.; Yao, T.; Wang, N. Forcing of the Asian monsoon on the Tibetan Plateau: Evidence from high-resolution ice core and tropical coral records. J. Geophys. Res. 2005, 110. [Google Scholar] [CrossRef]
- Thermal Low. Glossary of Meteor. Available online: http://glossary.ametsoc.org/wiki/Thermal_low (accessed on 13 March 2020).
- Sun, W.-Y.; Sun, O.M. Revisiting the parcel method and CAPE. Dyn. Atmos. Ocean. 2019, 86, 134–152. [Google Scholar] [CrossRef]
- Total Solar Irradiance (TSI). Available online: https://www.ngdc.noaa.gov/stp/solar/solarirrad.html (accessed on 13 March 2020).
- Rothman, L.S.; Gordon, I.E.; Babikov, Y.; Barbe, A.; Benner, D.C.; Bernath, P.F.; Birk, M.; Bizzocchi, L.; Boudon, V.; Brown, L.R. The HITRAN2012 molecular spectroscopic database. J. Quant. Spectrosc. Radiat. Transf. 2013, 130, 4–50. [Google Scholar] [CrossRef]
- Pinault, J.-L. Modulated response of subtropical gyres: Positive feedback loop, subharmonic modes, resonant solar and orbital forcing. J. Mar. Sci. Eng. 2018, 6, 107. [Google Scholar] [CrossRef]
- Kallberg, P.; Berrisford, P.; Hoskins, B.; Simmons, A.; Uppala, S.; Lamy-Thepaut, S. Atlas of the Atmospheric General Circulation. ECMWF ERA-40 Project Report Series, No. 19; European Centre for Medium-Range Weather Forecasts: Shinfield, Reading, UK. Available online: http://www.ecmwf.int/publications (accessed on 13 March 2020).
- Saturation Adiabatic Lapse Rate. Glossary of Meteorology. American Meteorological Society. Available online: http://glossary.ametsoc.org/wiki/Saturation-adiabatic_lapse_rate (accessed on 13 March 2020).
- Alduchov, O.A.; Eskridge, R.E. Improved Magnus form approximation of saturation vapor pressure. J. Appl. Meteorol. 1996, 35, 601–609. [Google Scholar] [CrossRef]
- Mixing Ratio. Glossary of Meteorology. American Meteorological Society. Available online: http://glossary.ametsoc.org/wiki/Mixing_ratio (accessed on 13 March 2020).
- Emagram, Wikipedia. Available online: https://en.wikipedia.org/wiki/Emagram (accessed on 13 March 2020).



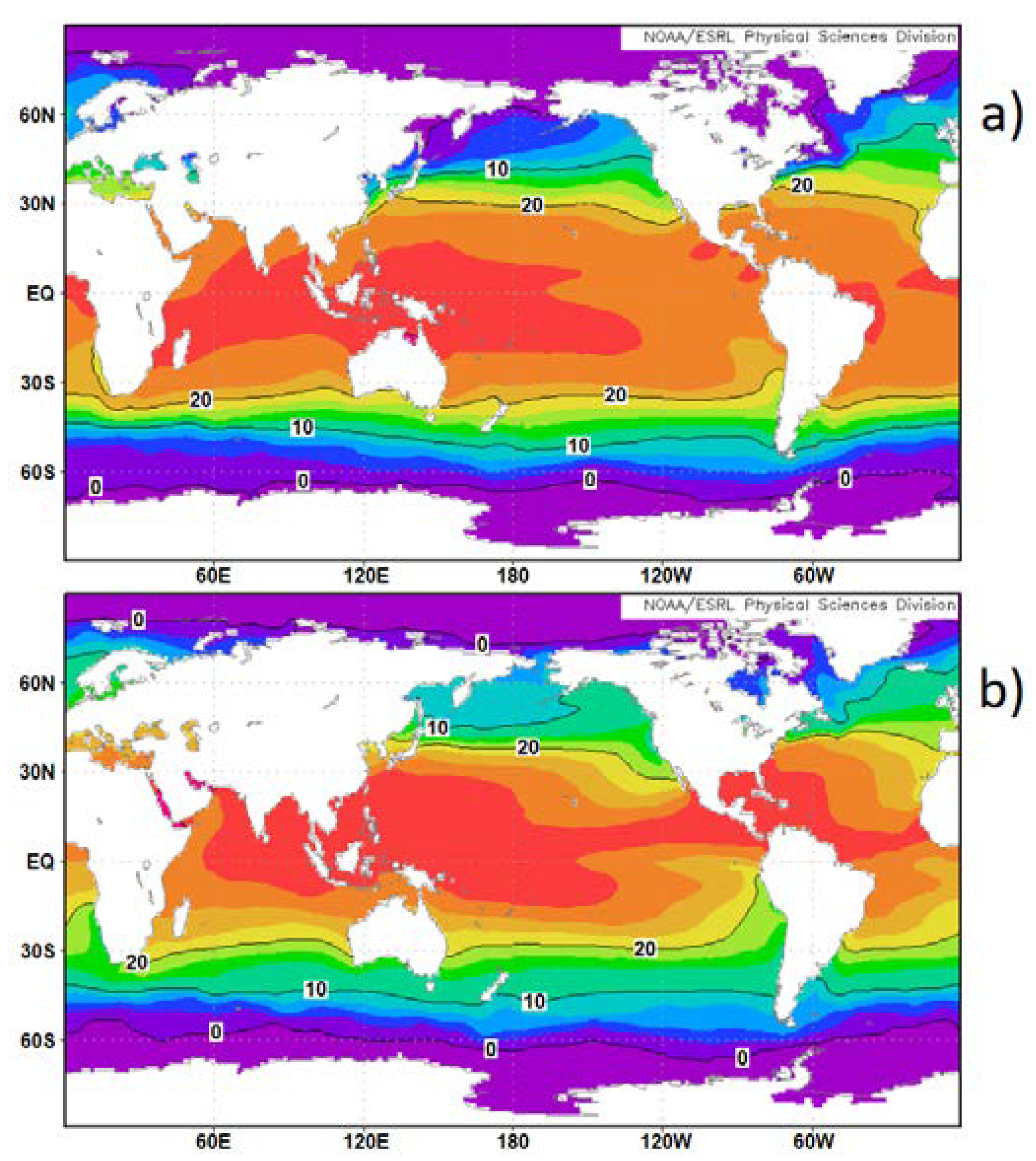
| Location of RRH Anomalies | Location of Related SST Anomalies |
|---|---|
| Southwestern North America | eastern antinode of the North Pacific extending along the western coast of the North America between 40° N and 60° N |
| Texas | along the subtropical gyre from the Baja California peninsula between 20° N and 30° N |
| Southeastern North America, Northeastern North America, southern tip of Greenland, Europe and Western-Central Asia | western antinode of the North Atlantic subtropical gyre that follows the North American coast from the Cape Hatteras to Newfoundland around 40° N. About the southeastern North America, the SST anomaly promotes the motion of tropical cyclones from the Gulf of Mexico or the Caribbean Sea. |
| Region of the Rio de la Plata | western antinode of the South Atlantic subtropical gyre nearly 30° S |
| South-Western and South-Eastern Australia | between 90° E and the western coast of Australia, and between 20° S and 40° S in latitude |
| Southeast Asia | Like what occurs in southeastern North America, here northeastward migration of tropical cyclones is related to SST anomalies in the Northwestern Pacific |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pinault, J.-L. The Moist Adiabat, Key of the Climate Response to Anthropogenic Forcing. Climate 2020, 8, 45. https://doi.org/10.3390/cli8030045
Pinault J-L. The Moist Adiabat, Key of the Climate Response to Anthropogenic Forcing. Climate. 2020; 8(3):45. https://doi.org/10.3390/cli8030045
Chicago/Turabian StylePinault, Jean-Louis. 2020. "The Moist Adiabat, Key of the Climate Response to Anthropogenic Forcing" Climate 8, no. 3: 45. https://doi.org/10.3390/cli8030045
APA StylePinault, J.-L. (2020). The Moist Adiabat, Key of the Climate Response to Anthropogenic Forcing. Climate, 8(3), 45. https://doi.org/10.3390/cli8030045





