Body Motion Sensor Analysis of Human-Induced Dynamic Load Factor (DLF) for Normal Walks on Slender Transparent Floors
Abstract
1. Introduction
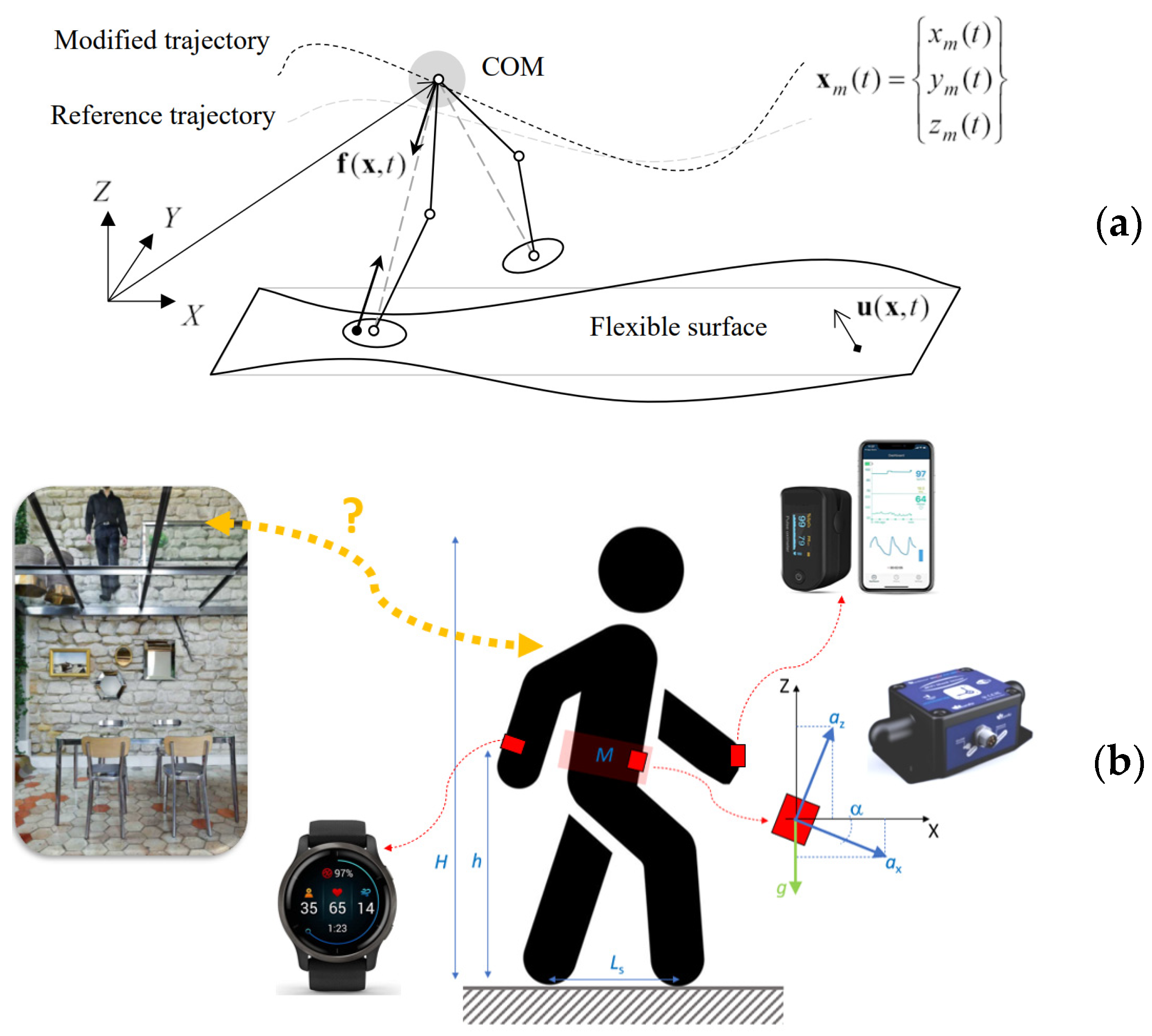
2. Theoretical Background and Experimental Methods
2.1. Deterministic Approach
2.2. Literature Experiments for DLF Estimation
2.3. Present Study
3. Experimental Investigation
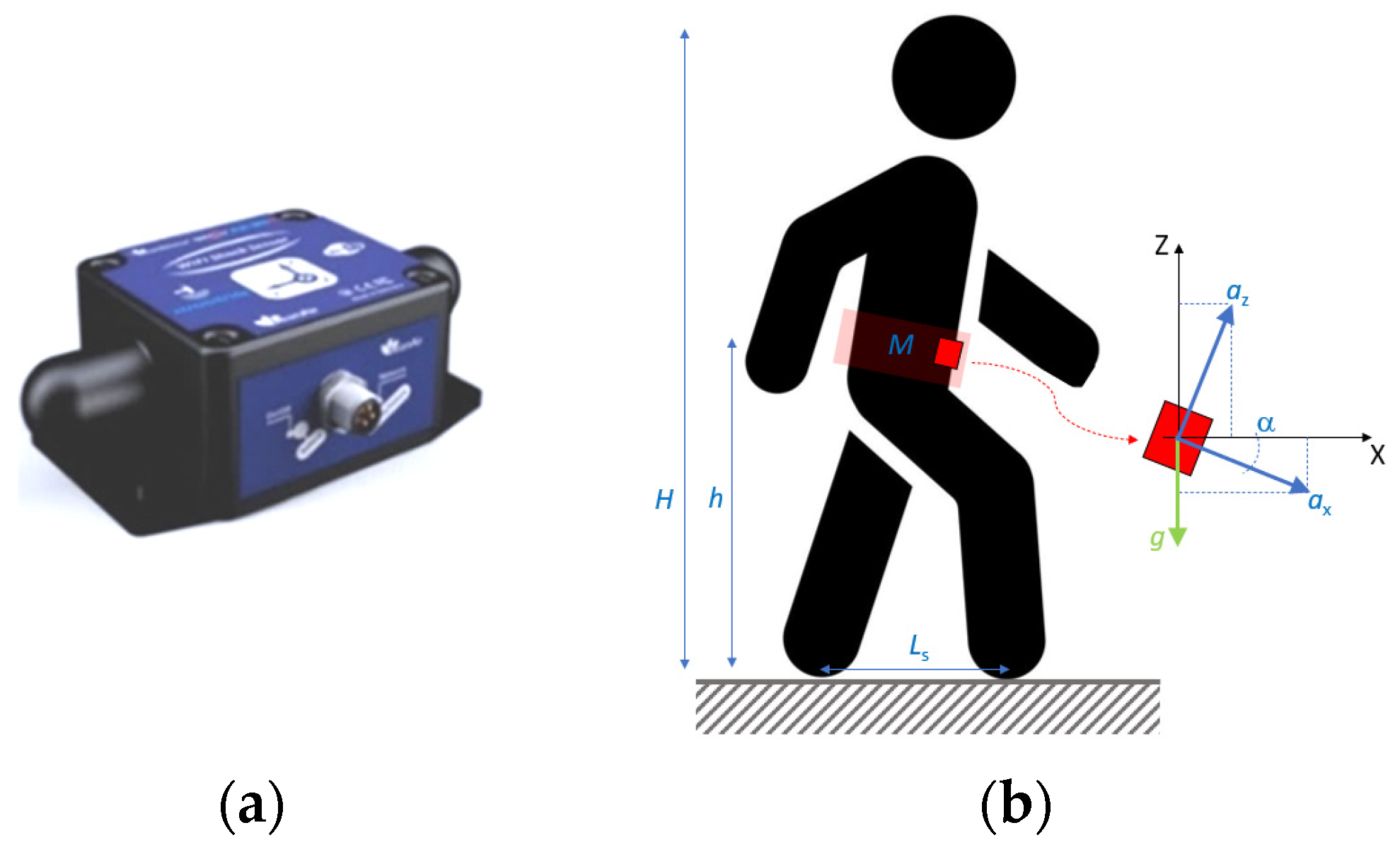
3.1. Setup
3.2. Instruments and Measured Body CoM Motion Records
4. Discussion of Experimental Results
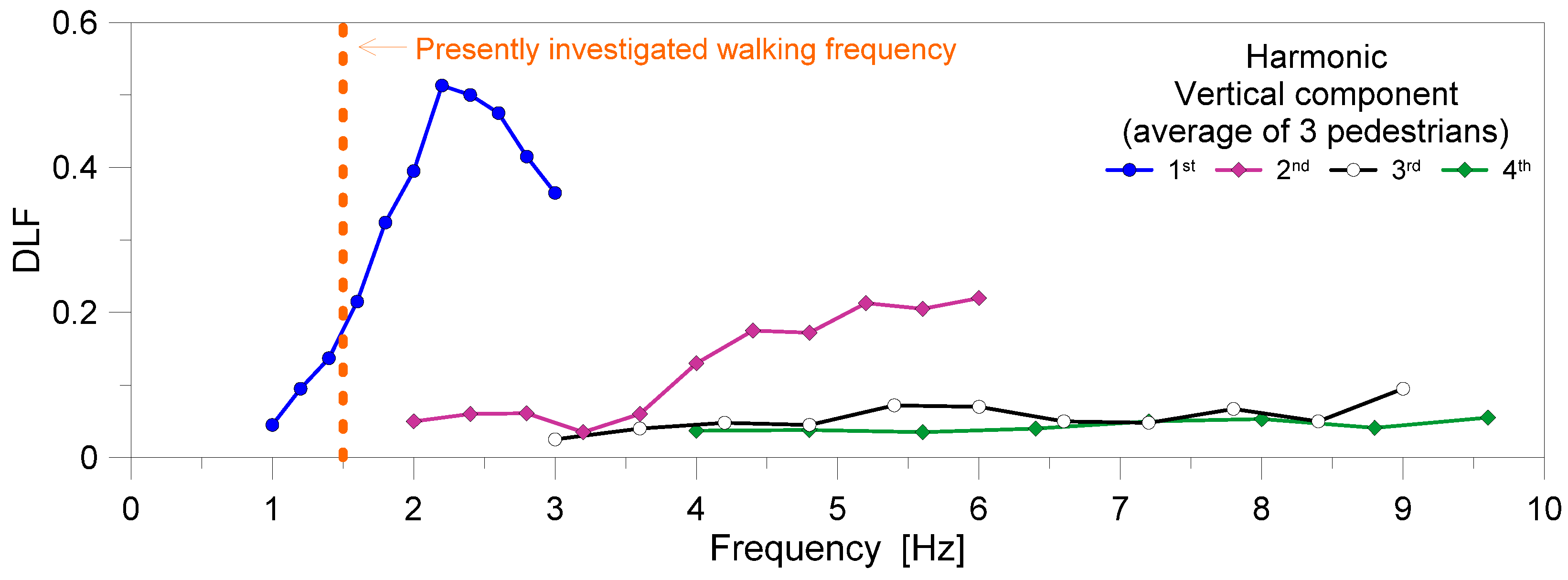
4.1. Frequency Domain Analysis
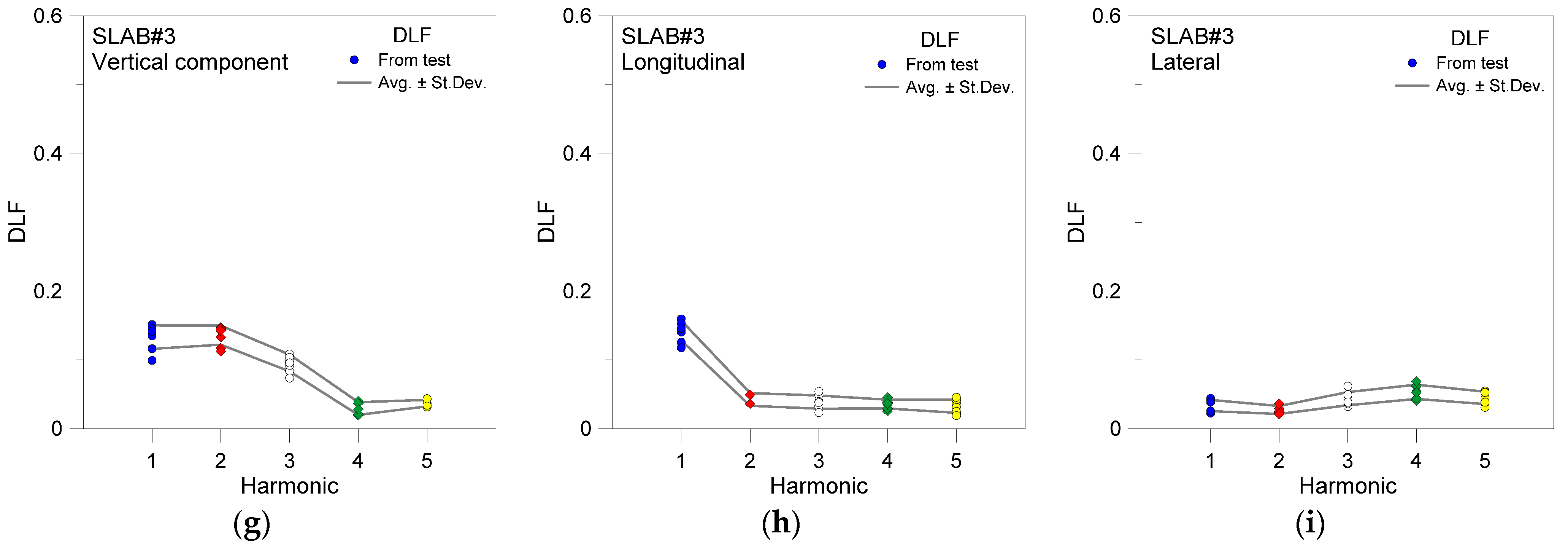
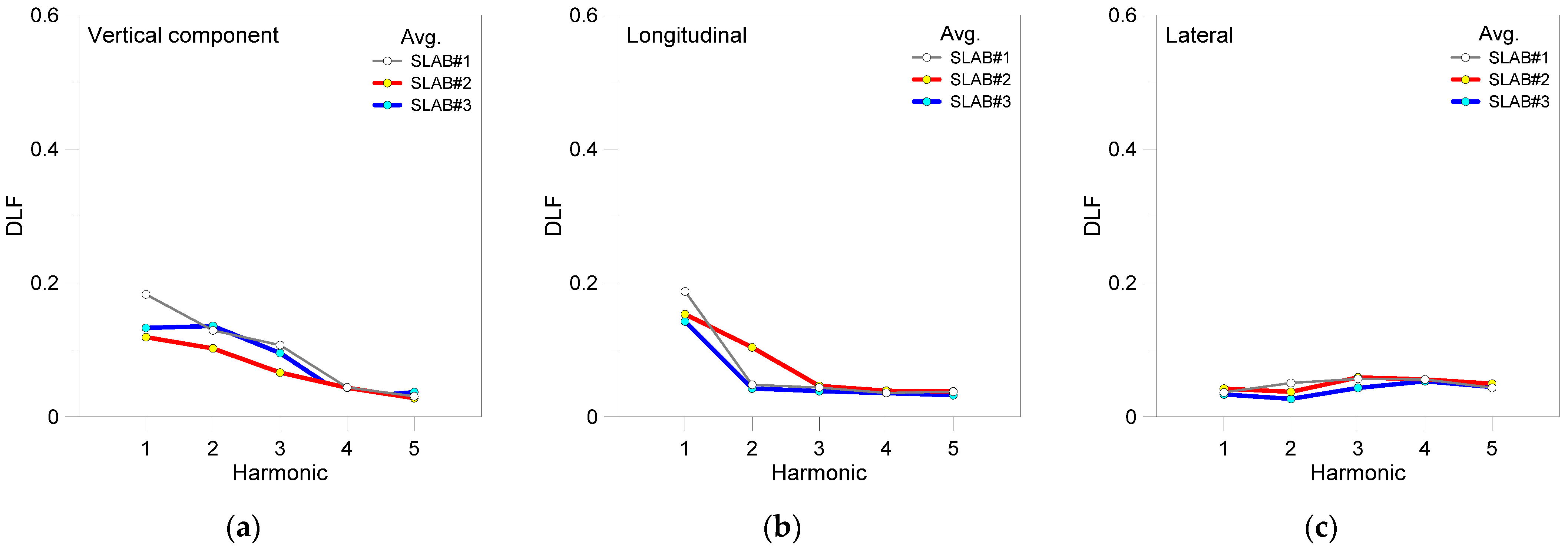
4.2. Average DLF for Vertical, Longitudinal, and Lateral Force Components
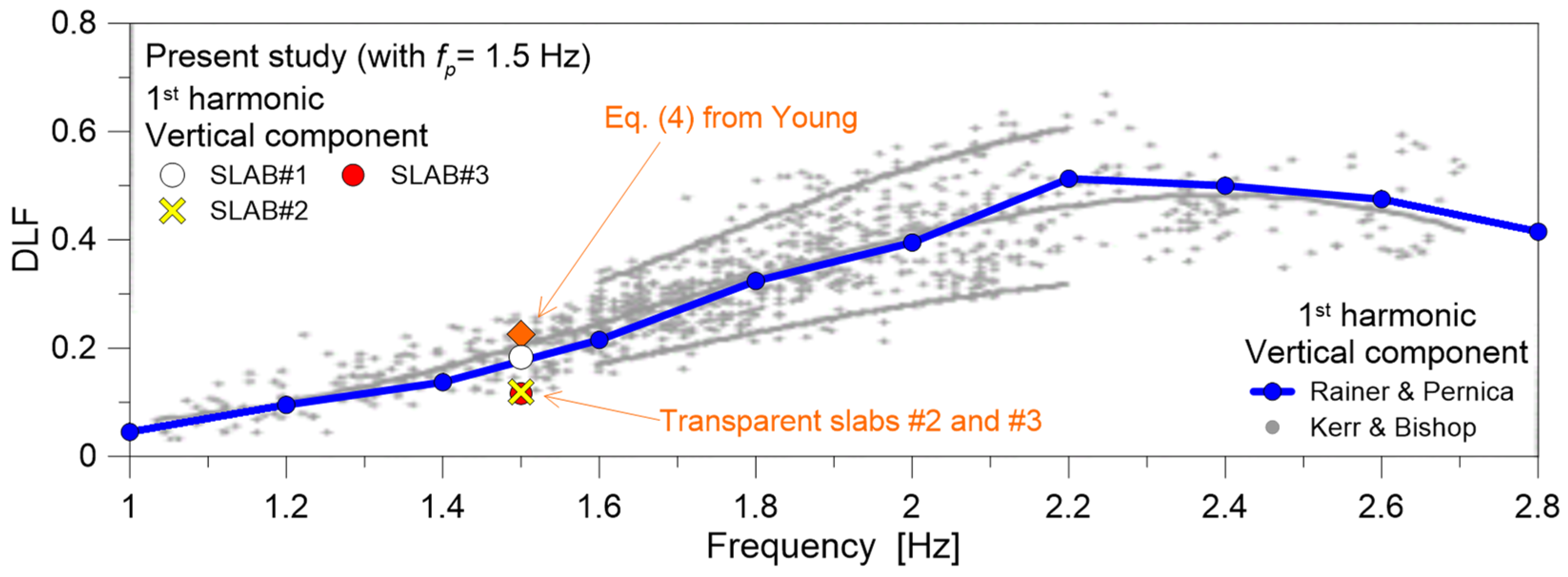
4.3. Average DLF for Vertical Force Component: Present and Literature Outcomes
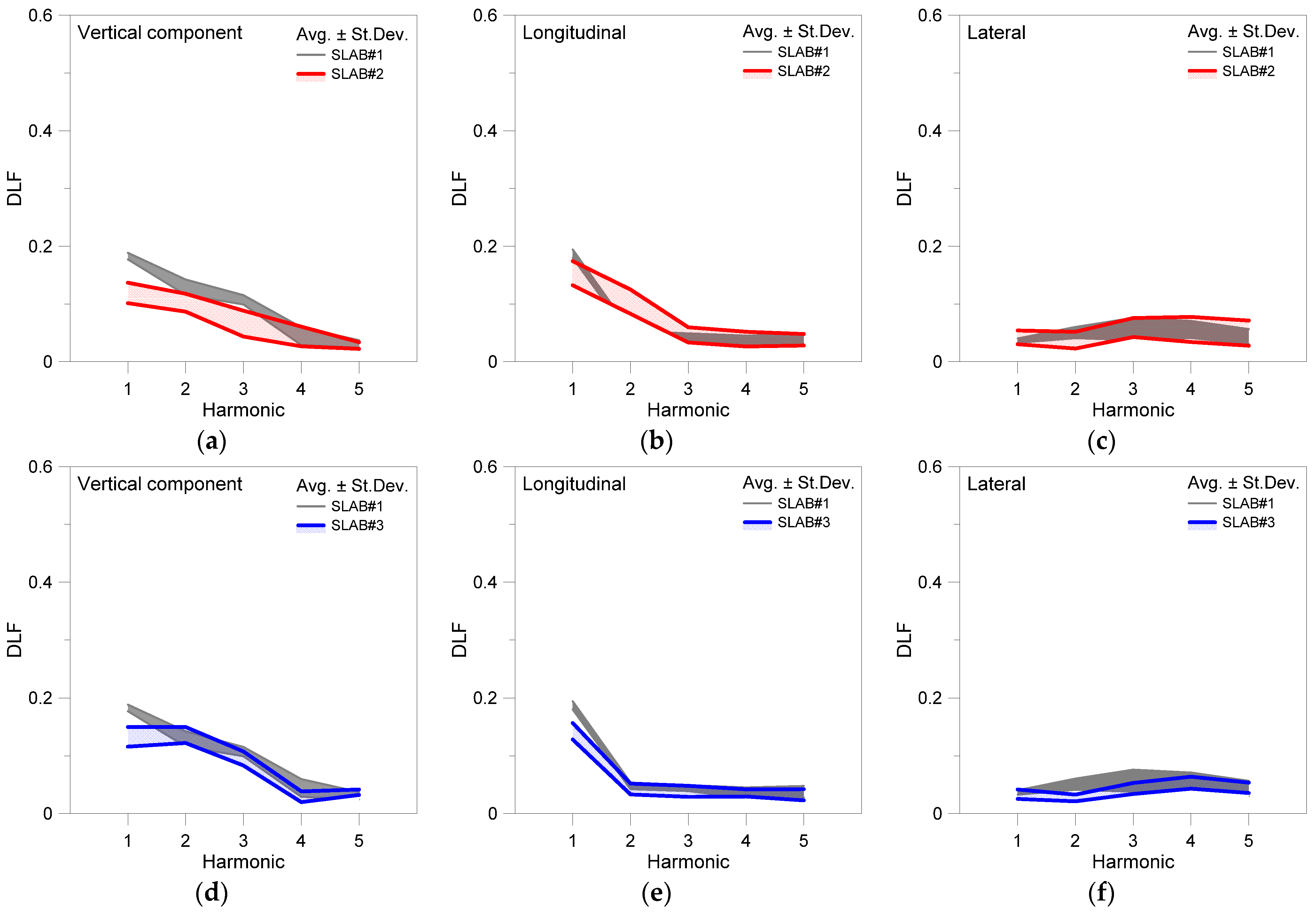
4.4. DLF Sensitivity to Floor Characteristics
4.5. Empirical DLF Models for Vertical Force Component
5. Conclusions and Future Developments
- the DLF corresponding to the first harmonic on the rigid, opaque SLAB#1 system was calculated with a very good match to literature data available for opaque slabs and floors characterized by high or low vibration frequency;
- on the other hand, a marked sensitivity (in terms of standard deviation) and low amplitude (compared to literature expectations) was found for DLF corresponding to the first harmonic for the pedestrian walking on transparent flexible floors. This was noted both for SLAB#2 (with high vibration frequency) and SLAB#3 (low frequency);
- for the transparent floor with low-frequency (as SLAB#3 in the current investigation), moreover, the DLF of second harmonic was found higher than the first one.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
List of Symbols
| av(t) | vertical component of human-induced acceleration |
| fi | i-th harmonic frequency, for Fourier decomposition |
| fp | walking frequency |
| f1,e | fundamental vibration frequency of floor/substructure |
| Fl(t) | longitudinal or lateral component of human-induced reaction force |
| Fv(t) | vertical component of human-induced reaction force |
| g | gravitational constant |
| i | order number of the harmonic, for Fourier decomposition |
| Hp | height of pedestrian |
| Mp | weight of pedestrian |
| Mstru | weight of floor/slab |
| n | total number of harmonics, for Fourier decomposition |
| p | number of pedestrians/floor occupants for each walking setup |
| αi | the Fourier’s coefficient for the i-th harmonic (also known to represent the dynamic load factor, DLF) |
| ϕi | the phase shift of the i-th harmonic, for Fourier decomposition |
Appendix A

References
- Bachmann, H.; Ammann, W. Vibrations in structures induced by man and machines. Can. J. Civ. Eng. 1987, 15, 1086–1087. [Google Scholar]
- Sedlacek, G.; Heinemeyer, C.; Butz, C.; Veiling, B.; Waarts, P.; Duin, F.; Hicks, S.; Devine, P.; Demarco, T. Generalisation of Criteria for Floor Vibrations for Industrial, Office, Residential and Public Building and Gymnasium Halls; European Commission: Luxembourg, 2006. [Google Scholar]
- Shahabpoor, E.; Pavic, A.; Racic, V. Interaction between Walking Humans and Structures in Vertical Direction: A Literature Review. Shock Vib. 2016, 2016, 1–22. [Google Scholar] [CrossRef]
- Cai, Y.; Gong, G.; Xia, J.; He, J.; Hao, J. Simulations of human-induced floor vibrations considering walking overlap. SN Appl. Sci. 2019, 2, 19. [Google Scholar] [CrossRef]
- da Silva, J.G.S.; Vellasco, P.D.S.; de Andrade, S.A.L.; de Lima, L.R.; Figueiredo, F.P. Vibration analysis of footbridges due to vertical human loads. Comput. Struct. 2007, 85, 1693–1703. [Google Scholar] [CrossRef]
- Racic, V.; Pavic, A.; Brownjohn, J. Experimental identification and analytical modelling of walking forces: Literature review. J. Sound Vib. 2009, 326, 1–49. [Google Scholar] [CrossRef]
- Rainer, J.H.; Pernica, G. Vertical dynamic forces from footsteps. Can. Acoust. Acoust. Can. 1986, 14, 12–21. [Google Scholar]
- Galbraith, F.W.; Barton, M.V. Ground loading from footsteps. J. Acoust. Soc. Am. 1970, 48, 1288–1292. [Google Scholar] [CrossRef]
- Ingólfsson, E.; Georgakis, C.; Ricciardelli, F.; Jönsson, J. Experimental identification of pedestrian-induced lateral forces on footbridges. J. Sound Vib. 2011, 330, 1265–1284. [Google Scholar] [CrossRef]
- Picozzi, V.; Avossa, A.M.; Ricciardelli, F. Probabilistic assessment of footbridge response to single walkers. Arch. Appl. Mech. 2022, 92, 1913–1927. [Google Scholar] [CrossRef]
- Pavic, A.; Reynolds, P. Vibration serviceability of long-span concrete building floors. Part 2: Review of mathematical modelling approaches. Shock Vib. Dig. 2002, 34, 279–297. [Google Scholar]
- Piccardo, G.; Tubino, F. Simplified procedures for vibration serviceability analysis of footbridges subjected to realistic walking loads. Comput. Struct. 2009, 87, 890–903. [Google Scholar] [CrossRef]
- Martinelli, L.; Racic, V.; Dal Lago, B.A.; Foti, F. Testing Walking-Induced Vibration of Floors Using Smartphones Recordings. Robotics 2020, 9, 37. [Google Scholar] [CrossRef]
- Bocian, M.; Brownjohn, J.M.W.; Racic, V.; Hester, D.; Quattrone, A.; Monnickendam, R. A framework for experimental determination of localised vertical pedestrian forces on full-scale structures using wireless attitude and heading reference systems. J. Sound Vib. 2016, 376, 217–243. [Google Scholar] [CrossRef]
- Van Nimmen, K.; Zhao, G.; Seyfarth, A.; Van den Broeck, P. A Robust Methodology for the Reconstruction of the Vertical Pedestrian-Induced Load from the Registered Body Motion. Vibration 2018, 1, 250–268. [Google Scholar] [CrossRef]
- Bedon, C. Experimental investigation on vibration sensitivity of an indoor glass footbridge to walking conditions. J. Build. Eng. 2020, 29, 101195. [Google Scholar] [CrossRef]
- Bedon, C. Diagnostic analysis and dynamic identification of a glass suspension footbridge via on-site vibration experiments and FE numerical modelling. Compos. Struct. 2019, 216, 366–378. [Google Scholar] [CrossRef]
- Bedon, C. Pilot experiments for multi-criteria human comfort-driven structural glass design assessment. Proc. Challenging Glass Conf. 2022, 8. [Google Scholar] [CrossRef]
- Bedon, C.; Fasan, M. Reliability of field experiments, analytical methods and pedestrian’s perception scales for the vibration serviceability assessment of an in-service glass walkway. Appl. Sci. 2019, 9, 1936. [Google Scholar] [CrossRef]
- Bedon, C.; Mattei, S. Facial Expression-Based Experimental Analysis of Human Reactions and Psychological Comfort on Glass Structures in Buildings. Buildings 2021, 11, 204. [Google Scholar] [CrossRef]
- Simonetti, E.; Bergamini, E.; Vannozzi, G.; Bascou, J.; Pillet, H. Estimation of 3D Body Center of Mass Acceleration and Instantaneous Velocity from a Wearable Inertial Sensor Network in Transfemoral Amputee Gait: A Case Study. Sensors 2021, 21, 3129. [Google Scholar] [CrossRef]
- Blachowski, B.; Holobut, P.; Ortiz, A.R.; Caicedo, J.M. Simple human-structure interaction model of walking on a flexible surface. In Proceedings of the ISMA2016—International Conference on Noise and Vibration, Leuven, Belgium, 19–21 September 2016. [Google Scholar]
- Gaile, L.; Radinsh, I. Human Induced Dynamic Loading on Stairs. Int. J. Civ. Env. Eng. 2012, 6, 530–536. [Google Scholar]
- Kerr, S.C.; Bishop, N.W.M. Human induced loading on flexible staircases. Eng. Struct. 2001, 23, 37–45. [Google Scholar] [CrossRef]
- Bougard, A. Human loading on staircases. Struct. Build. 2002, 152, 371–380. [Google Scholar] [CrossRef]
- Bishop, N.W.M.; Willford, M.; Pumphrey, R. Human induced loading of flexible staircases. Saf. Sci. 1995, 18, 261–276. [Google Scholar] [CrossRef]
- Bedon, C. Time-domain numerical analysis of single pedestrian random walks on laminated glass slabs in pre- or post-breakage regime. Eng. Struct. 2022, 260, 114250. [Google Scholar] [CrossRef]
- Živanović, S.; Pavić, A.; Reynolds, P. Vibration serviceability of footbridges under human-induced excitation: A literature review. J. Sound Vib. 2005, 279, 1–74. [Google Scholar] [CrossRef]
- Young, P. Improved Floor Vibration Prediction Methodologies. In Proceedings of Arup Vibration Seminar on Engineering for Structural Vibration—Current Developments in Research and Practice; Institution of Mechanical Engineers: London, UK, 2001. [Google Scholar]
- Bedon, C. Body CoM Acceleration for Rapid Analysis of Gait Variability and Pedestrian Effects on Structures. Buildings 2022, 12, 251. [Google Scholar] [CrossRef]
- Bedon, C.; Noè, S. Uncoupled Wi-Fi Body CoM Acceleration for the Analysis of Lightweight Glass Slabs under Random Walks. J. Sens. Actuator Netw. 2022, 11, 10. [Google Scholar] [CrossRef]
- BeanDevice® Wilow® User Manual—Wilow® Wireless Sensor, version 2.3.2; BenAir: Berlin, Germany, 2019.
- MATLAB (R2018a); Version 9.4 Natick; The MathWorks Inc.: Natick, MA, USA, 2018.





| Present Study | Rainer & Pernica [7] | Kerr & Bishop [24] | |
|---|---|---|---|
| Volunteer(s) | 1 | 3 | 40 |
| Pedestrian(s) p | 1 | 1 | 1 |
| Walking frequency fp (in Hz) | 1.5 | 1 ÷ 3 | 1 ÷ 3 |
| Substructure type | Rigid RC floor (laboratory) & two flexible glass systems (in-field) | Laboratory floor strip made of thick precast concrete panels sustained by steel trusses (17 m span) | Laboratory floor strip made of sandwich section (5 m span) |
| Test setup | Laboratory & in-field | Laboratory | Laboratory |
| Reaction force component | Vertical, longitudinal, lateral | Vertical | Vertical |
| Floor frequency (in Hz) | 80 (RC floor) & 7.28, 15.1 (glass systems) | 12 | 650 |
| Floor view | Opaque (laboratory) & transparent (in-field) | Opaque | Opaque |
| Instruments setup | Single body CoM sensor (MEMS accelerometer + inclinometer) | Two force transducers at floor strip mid-span | Force plate with 4 Kistler piezo-electric transducers |
| Material | Span | Width | Surface | Thickness | Mass | Frequency | |
|---|---|---|---|---|---|---|---|
| SLAB | [m] | [m] | [m2] | [m] | [kg] | [Hz] | |
| #1 | RC | 13 | 8.5 | 110.5 | 0.80 | 221,000 | >80 1 |
| #2 * | LG + steel | 2.65 | 1.65 | 4.37 | 0.04352 | 460 | 15.1 2 |
| #3 | LG + steel | 14.5 | 2.8 | 40.6 | 0.04352 | 4020 | 7.28 3 |
| Occupants | Density | Mass Ratio | Frequency Ratio | |
|---|---|---|---|---|
| SLAB | [p] | [p/m2] | Structure-to-Pedestrian | Structure-to-Pedestrian |
| #1 | 1 | 0.009 | 2763 | >53 |
| #2 | 1 | 0.228 | 5.75 | ≈10 |
| #3 | 1 | 0.026 | 134.13 | ≈4.85 |
| Harmonic Order | ||||||
|---|---|---|---|---|---|---|
| Vertical Component | Longitudinal | Lateral | ||||
| SLAB | 1st | 2nd | 1st | 2nd | 1st | 2nd |
| #1 | 0.121 | 0.088 | 0.134 | 0.029 | 0.026 | 0.034 |
| #2 | 0.086 | 0.064 | 0.087 | 0.039 | 0.021 | 0.032 |
| #3 | 0.081 | 0.096 | 0.090 | 0.027 | 0.019 | 0.026 |
| #1/#2 | 1.407 | 1.375 | 1.542 | 0.743 | 1.238 | 1.062 |
| #1/#3 | 1.482 | 0.916 | 1.488 | 1.074 | 1.368 | 1.307 |
| Average DLF | Standard Deviation (±) | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| SLAB | 1st | 2nd | 3rd | 4th | 5th | 1st | 2nd | 3rd | 4th | 5th |
| #1 | 0.183 | 0.129 | 0.107 | 0.044 | 0.031 | 0.0057 | 0.0132 | 0.081 | 0.0154 | 0.0063 |
| #2 | 0.119 | 0.102 | 0.066 | 0.044 | 0.028 | 0.0176 | 0.0155 | 0.0224 | 0.0170 | 0.0057 |
| #3 | 0.133 | 0.136 | 0.095 | 0.029 | 0.037 | 0.0171 | 0.0139 | 0.0121 | 0.0094 | 0.0047 |
| Average DLF | Standard Deviation (±) | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| SLAB | 1st | 2nd | 3rd | 4th | 5th | 1st | 2nd | 3rd | 4th | 5th |
| #1 | 0.187 | 0.048 | 0.044 | 0.036 | 0.038 | 0.0071 | 0.0057 | 0.0052 | 0.0093 | 0.0100 |
| #2 | 0.153 | 0.104 | 0.046 | 0.039 | 0.038 | 0.0210 | 0.0201 | 0.0132 | 0.0127 | 0.0099 |
| #3 | 0.142 | 0.042 | 0.038 | 0.036 | 0.032 | 0.0144 | 0.0093 | 0.0095 | 0.0063 | 0.0097 |
| Average DLF | Standard Deviation (±) | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| SLAB | 1st | 2nd | 3rd | 4th | 5th | 1st | 2nd | 3rd | 4th | 5th |
| #1 | 0.037 | 0.051 | 0.057 | 0.056 | 0.043 | 0.0037 | 0.0097 | 0.0192 | 0.0148 | 0.0132 |
| #2 | 0.042 | 0.037 | 0.059 | 0.056 | 0.050 | 0.0118 | 0.0146 | 0.0165 | 0.0218 | 0.0217 |
| #3 | 0.033 | 0.027 | 0.043 | 0.053 | 0.045 | 0.0082 | 0.0058 | 0.0096 | 0.0104 | 0.0091 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bedon, C.; Fasan, M.; Noè, S. Body Motion Sensor Analysis of Human-Induced Dynamic Load Factor (DLF) for Normal Walks on Slender Transparent Floors. J. Sens. Actuator Netw. 2022, 11, 81. https://doi.org/10.3390/jsan11040081
Bedon C, Fasan M, Noè S. Body Motion Sensor Analysis of Human-Induced Dynamic Load Factor (DLF) for Normal Walks on Slender Transparent Floors. Journal of Sensor and Actuator Networks. 2022; 11(4):81. https://doi.org/10.3390/jsan11040081
Chicago/Turabian StyleBedon, Chiara, Marco Fasan, and Salvatore Noè. 2022. "Body Motion Sensor Analysis of Human-Induced Dynamic Load Factor (DLF) for Normal Walks on Slender Transparent Floors" Journal of Sensor and Actuator Networks 11, no. 4: 81. https://doi.org/10.3390/jsan11040081
APA StyleBedon, C., Fasan, M., & Noè, S. (2022). Body Motion Sensor Analysis of Human-Induced Dynamic Load Factor (DLF) for Normal Walks on Slender Transparent Floors. Journal of Sensor and Actuator Networks, 11(4), 81. https://doi.org/10.3390/jsan11040081












