Order-to-Disorder and Disorder-to-Order Transitions of Proteins upon Binding to Phospholipid Membranes: Common Ground and Dissimilarities
Abstract
1. Introduction
- To what extent do hydrophobic interactions contribute to the binding to lipid membranes in addition to the already mentioned electrostatic interactions?
- Do the proteins undergo a conformational transition upon their binding to lipid membranes and how does the probability for this to occur depend on the lipid-to-protein ratio?
- Do the above proteins penetrate the lipid membrane upon binding by at least partially moving into the interior of corresponding lipid vesicles?
- To what extent does the binding to lipid membranes change the function of the protein?
2. Thermodynamics of Protein Binding to Membrane Surfaces
2.1. Basic Electrostatic Theory
2.2. Redistribution of Anionic Lipids After Protein Binding
2.3. Protein Binding and Membrane Penetration
3. Cytochrome c–Phospholipid Binding
3.1. Binding Isotherms
3.1.1. Variation in Cytochrome c Concentration
3.1.2. Variation of Lipid Concentration
3.1.3. Summary
3.2. Conformational Changes
3.3. Membrane Lipid–Lipid Demixing and Protein Penetration
3.4. Conformational Change and Peroxidase Activity
3.5. Summary
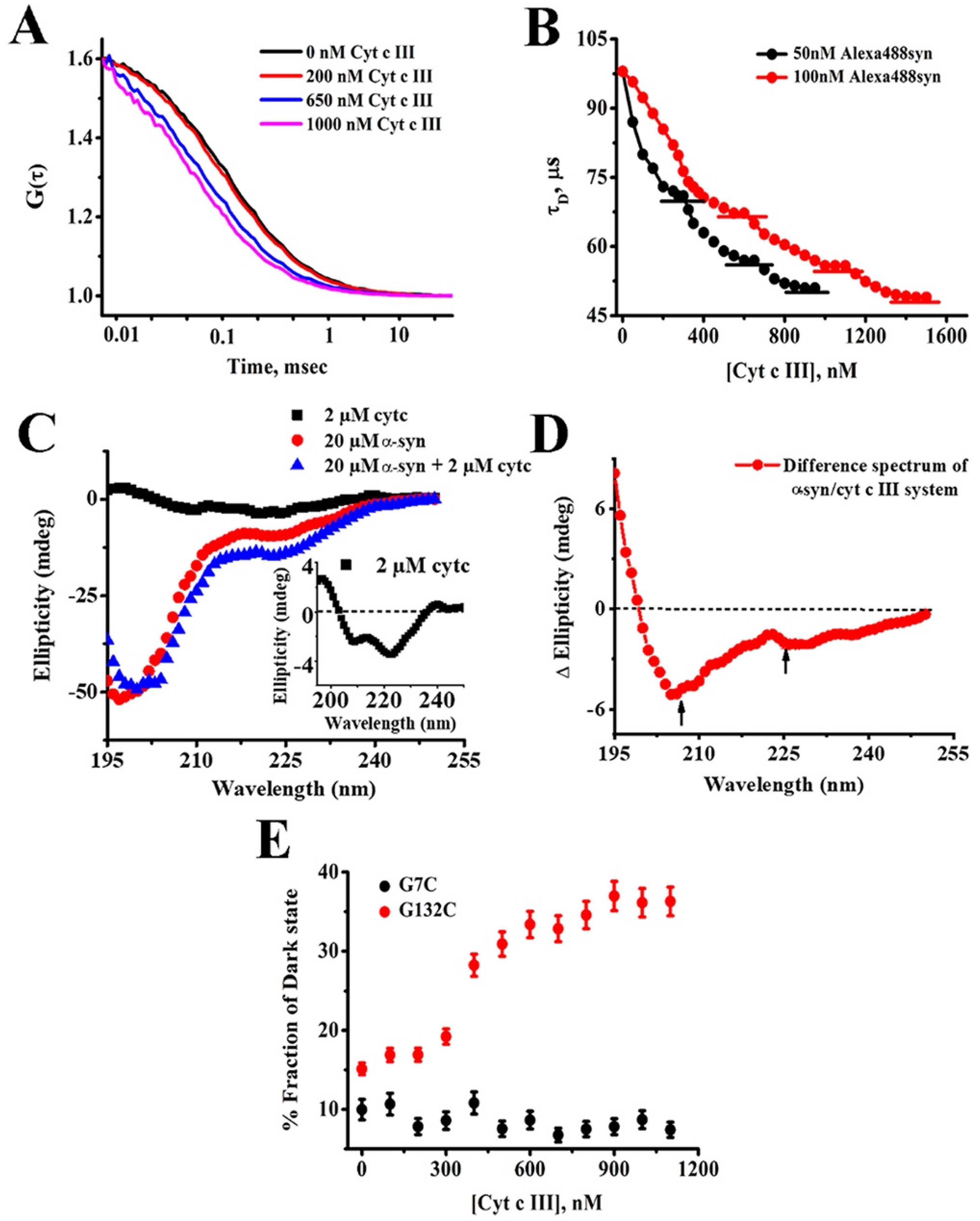
4. α-Synuclein–Phospholipid Binding
4.1. α-Synuclein in Solution
4.2. Binding and Structural Changes
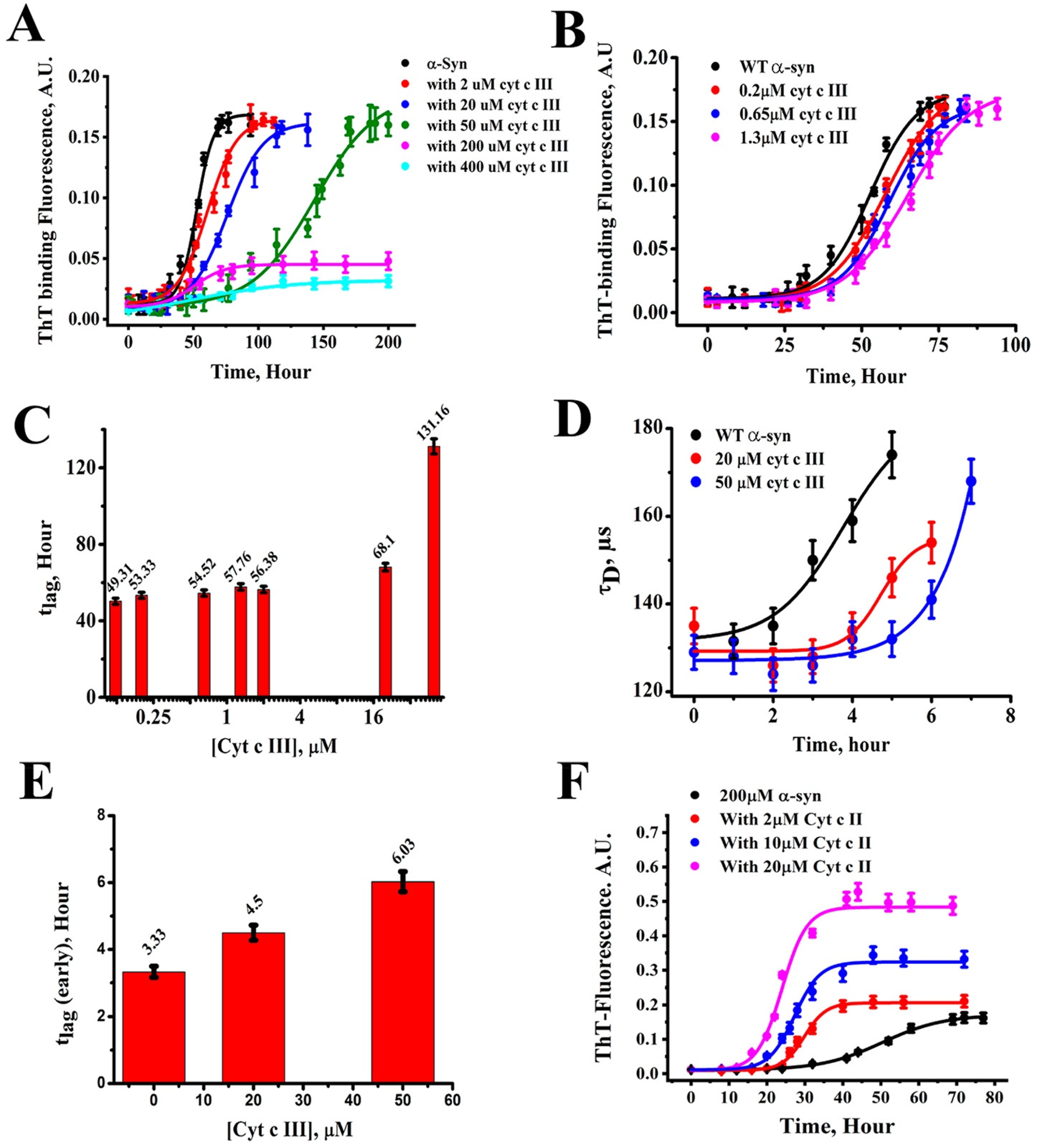
4.3. α-Synuclein Aggregation and Self-Assembly
5. Cytochrome c and α-Synuclein
6. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lewis, R.N.A.H.; McElhaney, R.N. The Physicochemical Properties of Cardiolipin Bilayers and Cardiolipin-Containing Lipid Membranes. Biochim. Biophys. Acta Biomembr. 2009, 1788, 2069–2079. [Google Scholar] [CrossRef] [PubMed]
- Ryan, T.; Bamm, V.V.; Stykel, M.G.; Coackley, C.L.; Humphries, K.M.; Jamieson-Williams, R.; Ambasudhan, R.; Mosser, D.D.; Lipton, S.A.; Harauz, G.; et al. Cardiolipin Exposure on the Outer Mitochondrial Membrane Modulates α-Synuclein. Nat. Commun. 2018, 9, 817. [Google Scholar] [CrossRef]
- Monje-Galvan, V.; Klauda, J.B. Peripheral Membrane Proteins: Tying the Knot between Experiment and Computation. Biochim. Biophys. Acta (BBA)—Biomembr. 2016, 1858, 1584–1593. [Google Scholar] [CrossRef] [PubMed]
- Hannibal, L.; Tomasina, F.; Capdevila, D.A.; Demicheli, V.; Tórtora, V.; Alvarez-Paggi, D.; Jemmerson, R.; Murgida, D.H.; Radi, R. Alternative Conformations of Cytochrome c: Structure, Function, and Detection. Biochemistry 2016, 55, 407–428. [Google Scholar] [CrossRef] [PubMed]
- Alvarez-Paggi, D.; Hannibal, L.; Castro, M.A.; Oviedo-Rouco, S.; Demicheli, V.; Tórtora, V.; Tomasina, F.; Radi, R.; Murgida, D.H. Multifunctional Cytochrome c: Learning New Tricks from an Old Dog. Chem. Rev. 2017, 117, 13382–13460. [Google Scholar] [CrossRef] [PubMed]
- Schweitzer-Stenner, R. Relating the Multi-Functionality of Cytochrome c to Membrane Binding and Structural Conversion. Biophys. Rev. 2018, 10, 1151–1185. [Google Scholar] [CrossRef]
- Vincent, J.S.; Kon, H.; Levin, I.W. Low-Temperature Electron Paramagnetic Resonance Study of the Ferricytochrome c-Cardiolipin Complex. Biochemistry 1987, 26, 2312–2314. [Google Scholar] [CrossRef]
- Das, T.; Eliezer, D. Membrane Interactions of Intrinsically Disordered Proteins: The Example of Alpha-Synuclein. Biochim. Biophys. Acta (BBA)—Proteins Proteom. 2019, 1867, 879–889. [Google Scholar] [CrossRef]
- Fauvet, B.; Mbefo, M.K.; Fares, M.B.; Desobry, C.; Michael, S.; Ardah, M.T.; Tsika, E.; Coune, P.; Prudent, M.; Lion, N.; et al. α-Synuclein in Central Nervous System and from Erythrocytes, Mammalian Cells, and Escherichia Coli Exists Predominantly as Disordered Monomer. J. Biol. Chem. 2012, 287, 15345–15364. [Google Scholar] [CrossRef]
- Hashimoto, M.; Takeda, A.; Hsu, L.J.; Takenouchi, T.; Masliah, E. Role of Cytochrome c as a Stimulator of α-Synuclein Aggregation in Lewy Body Disease. J. Biol. Chem. 1999, 274, 28849–28852. [Google Scholar] [CrossRef]
- Kumar, A.; Ganini, D.; Mason, R.P. Role of Cytochrome c in α-Synuclein Radical Formation: Implications of α-Synuclein in Neuronal Death in Maneb- and Paraquat-Induced Model of Parkinson’s Disease. Mol. Neurodegener. 2016, 11, 70. [Google Scholar] [CrossRef] [PubMed]
- Battistuzzi, G.; Borsari, M.; Cowan, J.A.; Ranieri, A.; Sola, M. Control of Cytochrome c Redox Potential: Axial Ligation and Protein Environment Effects. J. Am. Chem. Soc. 2002, 124, 5315–5324. [Google Scholar] [CrossRef] [PubMed]
- Stryer, L. Biochemistry, 5th ed.; W.H. Freeman: New York, NY, USA, 2002. [Google Scholar]
- Purring-Koch, C.; McLendon, G. Cytochrome c Binding to Apaf-1: The Effects of DATP and Ionic Strength. Proc. Natl. Acad. Sci. USA 2000, 97, 11928–11931. [Google Scholar] [CrossRef] [PubMed]
- Atlante, A.; Calissano, P.; Bobba, A.; Azzariti, A.; Marra, E.; Passarella, S. Cytochrome c Is Released from Mitochondria in a Reactive Oxygen Species (ROS)-Dependent Fashion and Can Operate as a ROS Scavenger and as a Respiratory Substrate in Cerebellar Neurons Undergoing Excitotoxic Death. J. Biol. Chem. 2000, 275, 37159–37166. [Google Scholar] [CrossRef]
- Paradies, G.; Petrosillo, G.; Pistolese, M.; Ruggiero, F.M. The Effect of Reactive Oxygen Species Generated from the Mitochondrial Electron Transport Chain on the Cytochrome c Oxidase Activity and on the Cardiolipin Content in Bovine Heart Submitochondrial Particles. FEBS Lett. 2000, 466, 323–326. [Google Scholar] [CrossRef]
- Banci, L.; Bertini, I.; Reddig, T.; Turano, P. Monitoring the Conformational Flexibility of Cytochrome c at Low Ionic Strength by 1H-NMR Spectroscopy. Eur. J. Biochem. 1998, 256, 271–278. [Google Scholar] [CrossRef]
- Soffer, J.B. The Folded, Partially Folded, Misfolded, and Unfolded Conformations of Cytochrome c Probed by Optical Spectroscopy. Ph.D. Thesis, Drexel University, Philadelphia, PA, USA, 2013. [Google Scholar]
- Uversky, V.N. Dancing Protein Clouds: The Strange Biology and Chaotic Physics of Intrinsically Disordered Proteins. J. Biol. Chem. 2016, 291, 6681–6688. [Google Scholar] [CrossRef]
- Uversky, V.N. Natively Unfolded Proteins: A Point Where Biology Waits for Physics. Protein Sci. 2002, 11, 739–756. [Google Scholar] [CrossRef]
- Uversky, V.N.; Li, J.; Fink, A.L. Evidence for a Partially Folded Intermediate in α-Synuclein Fibril Formation. J. Biol. Chem. 2001, 276, 10737–10744. [Google Scholar] [CrossRef]
- Makasewicz, K.; Linse, S.; Sparr, E. Interplay of α-Synuclein with Lipid Membranes: Cooperative Adsorption, Membrane Remodeling and Coaggregation. JACS Au 2024, 4, 1250–1262. [Google Scholar] [CrossRef]
- Sharma, M.; Burré, J. α-Synuclein in Synaptic Function and Dysfunction. Trends Neurosci. 2023, 46, 153–166. [Google Scholar] [CrossRef] [PubMed]
- Burré, J.; Sharma, M.; Tsetsenis, T.; Buchman, V.; Etherton, M.R.; Südhof, T.C. α-Synuclein Promotes SNARE-Complex Assembly in Vivo and in Vitro. Science (1979) 2010, 329, 1663–1667. [Google Scholar] [CrossRef] [PubMed]
- Vicario, M.; Cieri, D.; Brini, M.; Calì, T. The Close Encounter Between Alpha-Synuclein and Mitochondria. Front. Neurosci. 2018, 12, 388. [Google Scholar] [CrossRef] [PubMed]
- Dill, K.A.; Bromberg, S. Molecular Driving Forces. Statistical Thermodynamics in Chemistry and Biology; Garland Science: New York, NY, USA, 2002; ISBN 0-8153-2051-5. [Google Scholar]
- Heimburg, T.; Marsh, D. Protein Surface-Distribution and Protein-Protein Interactions in the Binding of Peripheral Proteins to Charged Lipid Membranes. Biophys. J. 1995, 68, 536–546. [Google Scholar] [CrossRef]
- Zuckermann, M.J.; Heimburg, T. Insertion and Pore Formation Driven by Adsorption of Proteins onto Lipid Bilayer Membrane-Water Interfaces. Biophys. J. 2001, 81, 2458–2472. [Google Scholar] [CrossRef]
- Heimburg, T.; Angerstein, B.; Marsh, D. Binding of Peripheral Proteins to Mixed Lipid Membranes: Effect of Lipid Demixing upon Binding. Biophys. J. 1999, 76, 2575–2586. [Google Scholar] [CrossRef]
- Minton, A.P. Adsorption of Globular Proteins on Locally Planar Surfaces. II. Models for the Effect of Multiple Adsorbate Conformations on Adsorption Equilibria and Kinetics. Biophys. J. 1999, 76, 176–187. [Google Scholar] [CrossRef]
- Chatelier, R.C.; Minton, A.P. Adsorption of Globular Proteins on Locally Planar Surfaces: Models for the Effect of Excluded Surface Area and Aggregation of Adsorbed Protein on Adsorption Equilibria. Biophys. J. 1996, 71, 2367–2374. [Google Scholar] [CrossRef]
- Gorbenko, G.P.; Molotkovsky, J.G.; Kinnunen, P.K.J. Cytochrome c Interaction with Cardiolipin/Phosphatidylcholine Model Membranes: Effect of Cardiolipin Protonation. Biophys. J. 2006, 90, 4093–4103. [Google Scholar] [CrossRef]
- Heimburg, T. Thermal Biophysics of Membranes; Wiley-VCH Verlag GmbH & Co: Weinheim, Germany, 2007. [Google Scholar]
- Helfand, E.; Frisch, H.L.; Lebowitz, J.L. Theory of the Two- and One-Dimensional Rigid Sphere Fluids. J. Chem. Phys. 1961, 34, 1037–1042. [Google Scholar] [CrossRef]
- Jähnig, F. Electrostatic Free Energy and Shift of the Phase Transition for Charged Lipid Membranes. Biophys. Chem. 1976, 4, 309–318. [Google Scholar] [CrossRef] [PubMed]
- Talbot, J. Molecular Thermodynamics of Binary Mixture Adsorption: A Scaled Particle Theory Approach. J. Chem. Phys. 1997, 106, 4696–4706. [Google Scholar] [CrossRef]
- Rytömaa, M.; Mustonen, P.; Kinnunen, P.K.J. Reversible, Nonionic, and PH-Dependent Association of Cytochrome c with Cardiolipin-Phosphatidylcholine Liposomes. J. Biol. Chem. 1992, 267, 22243–22248. [Google Scholar] [CrossRef] [PubMed]
- Rytömaa, M.; Kinnunen, P.K.J. Reversibility of the Binding of Cytochrome c to Liposomes. Implications for Lipid-Protein Interactions. J. Biol. Chem. 1995, 270, 3197–3202. [Google Scholar] [CrossRef]
- Rytömaa, M.; Kinnunen, P.K.J. Evidence for Two Distinct Acidic Phospholipid-Binding Sites in Cytochrome c. J. Biol. Chem. 1994, 269, 1770–1774. [Google Scholar] [CrossRef]
- Tuominen, E.K.J.; Wallace, C.J.A.; Kinnunen, P.K.J. Phospholipid-Cytochrome c Interaction. J. Biol. Chem. 2002, 277, 8822–8826. [Google Scholar] [CrossRef]
- Kalanxhi, E.; Wallace, C.J.A. Cytochrome c Impaled: Investigation of the Extended Lipid Anchorage of a Soluble Protein to Mitochondrial Membrane Models. Biochem. J. 2007, 407, 179–187. [Google Scholar] [CrossRef]
- Trusova, V.M.; Gorbenko, G.P.; Molotkovsky, J.G.; Kinnunen, P.K.J. Cytochrome C-Lipid Interactions: New Insights from Resonance Energy Transfer. Biophys. J. 2010, 99, 1754–1763. [Google Scholar] [CrossRef]
- Dragomir, I.; Hagarman, A.; Wallace, C.; Schweitzer-Stenner, R. Optical Band Splitting and Electronic Perturbations of the Heme Chromophore in Cytochrome c at Room Temperature Probed by Visible Electronic Circular Dichroism Spectroscopy. Biophys. J. 2007, 92, 095976. [Google Scholar] [CrossRef]
- Schweitzer-Stenner, R. Internal Electric Field in Cytochrome C Explored by Visible Electronic Circular Dichroism Spectroscopy. J. Phys. Chem. B 2008, 112, 10358–10366. [Google Scholar] [CrossRef]
- Schweitzer-Stenner, R. Probing the Versatility of Cytochrome c by Spectroscopic Means: A Laudatio on Resonance Raman Spectroscopy. J. Inorg. Biochem. 2024, 259, 112641. [Google Scholar] [CrossRef] [PubMed]
- Pielak, G.J.; Oikawa, K.; Kay, C.M.; Mauk, A.G.; Smith, M. Elimination of the Negative Soret Cotton Effect of Cytochrome c by Replacement of the Invariant Phenylalanine Using Site-Directed Mutagenesis. J. Am. Chem. Soc. 1986, 108, 2724–2727. [Google Scholar] [CrossRef]
- Sinibaldi, F.; Fiorucci, L.; Patriarca, A.; Lauceri, R.; Ferri, T.; Coletta, M.; Santucci, R. Insights into Cytochrome C-Cardiolipin Interaction. Role Played by Ionic Strength. Biochemistry 2008, 47, 6928–6935. [Google Scholar] [CrossRef] [PubMed]
- Pandiscia, L.A.; Schweitzer-Stenner, R. Coexistence of Native-Like and Non-Native Cytochrome c on Anionic Lipsomes with Different Cardiolipin Content. J. Phys. Chem. B 2015, 119, 12846–12859. [Google Scholar] [CrossRef] [PubMed]
- Pandiscia, L.A.; Schweitzer-Stenner, R. Coexistence of Native-like and Non-Native Partially Unfolded Ferricytochrome c on the Surface of Cardiolipin-Containing Liposomes. J. Phys. Chem. B 2015, 119, 1334–1349. [Google Scholar] [CrossRef]
- Steele, H.B.B.; Elmer-Dixon, M.M.; Rogan, J.T.; Ross, J.B.A.; Bowler, B.E. The Human Cytochrome c Domain-Swapped Dimer Facilitates Tight Regulation of Intrinsic Apoptosis. Biochemistry 2020, 59, 2055–2068. [Google Scholar] [CrossRef]
- Rezaei Sani, S.M.; Akhavan, M.; Jalili, S. Salt-Induced Effects on Natural and Inverse DPPC Lipid Membranes: Molecular Dynamics Simulation. Biophys. Chem. 2018, 239, 7–15. [Google Scholar] [CrossRef]
- Kawai, C.; Pessoto, F.S.; Rodrigues, T.; Mugnol, K.C.U.; Tórtora, V.; Castro, L.; Milícchio, V.A.; Tersariol, I.L.S.; Di Mascio, P.; Radi, R.; et al. PH-Sensitive Binding of Cytochrome c to the Inner Mitochondrial Membrane. Implications for the Participation of the Protein in Cell Respiration and Apoptosis. Biochemistry 2009, 48, 8335–8342. [Google Scholar] [CrossRef]
- Kawai, C.; Prado, F.M.; Nunes, G.L.C.; Di Mascio, P.; Carmona-Ribeiro, A.M.; Nantes, I.L. PH-Dependent Interaction of Cytochrome c with Mitochondrial Mimetic Membranes: The Role of an Array of Positively Charged Amino Acids. J. Biol. Chem. 2005, 280, 34709–34717. [Google Scholar] [CrossRef]
- Milorey, B.; Schweitzer-Stenner, R.; Kurbaj, R.; Malyshka, D. PH-Induced Switch between Different Modes of Cytochrome c Binding to Cardiolipin-Containing Liposomes. ACS Omega 2019, 4, 1386–1400. [Google Scholar] [CrossRef]
- Milorey, B.; Malyshka, D.; Schweitzer-Stenner, R. PH Dependence of Ferricytochrome c Conformational Transitions during Binding to Cardiolipin Membranes: Evidence for Histidine as the Distal Ligand at Neutral PH. J. Phys. Chem. Lett. 2017, 8, 1993–1998. [Google Scholar] [CrossRef] [PubMed]
- O’Brien, E.S.; Nucci, N.V.; Fuglestad, B.; Tommos, C.; Wand, A.J. Defining the Apoptopic Trigger: The Interaction of Cytochrome c and Cardiolipin. J. Biol. Chem. 2015, 290, 30879–30887. [Google Scholar] [PubMed]
- Elmer-Dixon, M.M.; Bowler, B.E. Site A-Mediated Partial Unfolding of Cytochrome c on Cardiolipin Vesicles Is Species-Dependent and Does Not Require Lys72. Biochemistry 2017, 56, 4830–4839. [Google Scholar] [CrossRef] [PubMed]
- Rosell, F.I.; Ferrer, J.C.; Mauk, A.G. Proton-Linked Protein Conformational Switching: Definition of the Alkaline Conformational Transition of Yeast Iso-1-Ferricytochrome c. J. Am. Chem. Soc. 1998, 120, 11234–11245. [Google Scholar] [CrossRef]
- Döpner, S.; Hildebrandt, P.; Resell, F.I.; Mauk, A.G. Alkaline Conformational Transitions of Ferricytochrome c Studied by Resonance Raman Spectroscopy. J. Am. Chem. Soc. 1998, 120, 11246–11255. [Google Scholar] [CrossRef]
- Hagarman, A.; Duitch, L.; Schweitzer-Stenner, R. The Conformational Manifold of Ferricytochromec Explored by Visible and Far-UV Electronic Circular Dichroism Spectroscopy. Biochemistry 2008, 47, 9667–9677. [Google Scholar] [CrossRef]
- Elmer-Dixon, M.M.; Bowler, B.E. Electrostatic Constituents of the Interaction of Cardiolipin with Site A of Cytochrome c. Biochemistry 2018, 57, 5683–5695. [Google Scholar] [CrossRef]
- Elmer-Dixon, M.M.; Hoody, J.; Steele, H.B.B.; Becht, D.C.; Bowler, B.E. Cardiolipin Preferentially Partitions to the Inner Leaflet of Mixed Lipid Large Unilamellar Vesicles. J. Phys. Chem. B 2019, 123, 9111–9122. [Google Scholar] [CrossRef]
- Elmer-Dixon, M.M.; Xie, Z.; Alverson, J.B.; Priestley, N.D.; Bowler, B.E. Curvature-Dependent Binding of Cytochrome c to Cardiolipin. J. Am. Chem. Soc. 2020, 142, 19532–19539. [Google Scholar] [CrossRef]
- Basova, L.V.; Kurnikov, I.V.; Wang, L.; Ritov, V.B.; Belikova, N.A.; Vlasova, I.I.; Pacheco, A.A.; Winnica, D.E.; Peterson, J.; Bayir, H.; et al. Cardiolipin Switch in Mitochondria: Sutting off the Reduction of Cytochrome and Turning on the Peroxidase Activity. Biochemistry 2007, 46, 3423–3434. [Google Scholar] [CrossRef]
- Kapralov, A.A.; Kurnikov, I.V.; Vlasova, I.I.; Belikova, N.A.; Tyurin, V.A.; Basova, L.V.; Zhao, Q.; Tyurina, Y.Y.; Jiang, J.; Bayir, H.; et al. The Hierarchy of Structural Transitions Induced in Cytochrome c by Anionic Phospholipids Determines Its Peroxidase Activation and Selective Peroxidation during Apoptosis in Cells. Biochemistry 2007, 46, 14232–14244. [Google Scholar] [CrossRef] [PubMed]
- Kapralov, A.A.; Yanamala, N.; Tyurina, Y.Y.; Castro, L.; Samhan-Arias, A.; Vladimirov, Y.A.; Maeda, A.; Weitz, A.A.; Peterson, J.; Mylnikov, D.; et al. Topography of Tyrosine Residues and Their Involvement in Peroxidation of Polyunsaturated Cardiolipin in Cytochrome c/Cardiolipin Peroxidase Complexes. Biochim. Biophys. Acta Biomembr. 2011, 1808, 2147–2155. [Google Scholar] [CrossRef] [PubMed]
- McClelland, L.J.; Steele, H.B.B.; Whitby, F.G.; Mou, T.-C.; Holley, D.; Ross, J.B.A.; Sprang, S.R.; Bowler, B.E. Cytochrome c Can Form a Well-Defined Binding Pocket for Hydrocarbons. J. Am. Chem. Soc. 2016, 138, 16770–16778. [Google Scholar] [CrossRef] [PubMed]
- Englander, S.W.; Mayne, L. The Case for Defined Protein Folding Pathways. Proc. Natl. Acad. Sci. USA 2017, 114, 8253–8258. [Google Scholar] [CrossRef] [PubMed]
- Hong, Y.; Muenzner, J.; Grimm, S.K.; Pletneva, E.V. Origin of the Conformational Heterogeneity of Cardiolipin-Bound Cytochrome c. J. Am. Chem. Soc. 2012, 134, 18713–18723. [Google Scholar] [CrossRef]
- Hanske, J.; Toffey, J.R.; Morenz, A.M.; Bonilla, A.J.; Schiavoni, K.H.; Pletneva, E.V. Conformational Properties of Cardiolipin-Bound Cytochrome c. Proc. Natl. Acad. Sci. USA 2012, 109, 125–130. [Google Scholar] [CrossRef]
- Muenzner, J.; Toffey, J.R.; Hong, Y.; Pletneva, E.V. Becoming a Peroxidase: Cardiolipin-Induced Unfolding of Cytochrome c. J. Phys. Chem. B 2013, 117, 12878–12886. [Google Scholar] [CrossRef]
- Malyshka, D.; Schweitzer-Stenner, R. Ferrocyanide-Mediated Photoreduction of Ferricytochrome C Utilized to Selectively Probe Non-Native Conformations Induced by Binding to Cardiolipin-Containing Liposomes. Chem. Eur. J. 2017, 23, 1151–1156. [Google Scholar] [CrossRef]
- Milazzo, L.; Tognaccini, L.; Howes, B.D.; Sinibaldi, F.; Piro, M.C.; Fittipaldi, M.; Baratto, M.C.; Pogni, R.; Santucci, R.; Smulevich, G. Unravelling the Non-Native Low-Spin State of the Cytochrome c-Cardiolipin Complex: Evidence of the Formation of a His-Ligated Species Only. Biochemistry 2017, 56, 1887–1898. [Google Scholar] [CrossRef]
- Mandal, A.; Hoop, C.L.; Delucia, M.; Kodali, R.; Kagan, V.E.; Ahn, J.; Van Der Wel, P.C.A. Structural Changes and Proapoptotic Peroxidase Activity of Cardiolipin-Bound Mitochondrial Cytochrome c. Biophys. J. 2015, 109, 1873–1884. [Google Scholar] [CrossRef]
- Li, M.; Sun, W.; Tyurin, V.A.; DeLucia, M.; Ahn, J.; Kagan, V.E.; van der Wel, P.C.A. Activation of Cytochrome C Peroxidase Function Through Coordinated Foldon Loop Dynamics upon Interaction with Anionic Lipids. J. Mol. Biol. 2021, 433, 167057. [Google Scholar] [CrossRef] [PubMed]
- Hu, W.; Kan, Z.-Y.; Mayne, L.; Englander, S.W. Cytochrome c Folds through Foldon-Dependent Native-like Intermediates in an Ordered Pathway. Proc. Nat. Acad. Sci. USA 2016, 113, 3809–3814. [Google Scholar] [CrossRef] [PubMed]
- Zhan, J.; Zeng, D.; Xiao, X.; Fang, Z.; Huang, T.; Zhao, B.; Zhu, Q.; Liu, C.; Jiang, B.; Zhou, X.; et al. Real-Time Observation of Conformational Changes and Translocation of Endogenous Cytochrome c within Intact Mitochondria. J. Am. Chem. Soc. 2024, 146, 4455–4466. [Google Scholar] [CrossRef] [PubMed]
- Paul, M.; Govind, C.; Karunakaran, V. Significance of the Double Bond in the Acyl Chain of Cardiolipin Revealed by the Partial Unfolding Dynamics of Cytochrome c Using Femtosecond Transient Absorption Spectroscopy. J. Phys. Chem. B 2024, 128, 11885–11892. [Google Scholar] [CrossRef] [PubMed]
- Hildebrandt, P.; Stockburger, M. Cytochrome c at Charged Interfaces. 2. Complexes with Negatively Charged Macromolecular Systems Studied by Resonance Raman Spectroscopy. Biochemistry 1989, 28, 6722–6728. [Google Scholar] [CrossRef]
- Wackerbarth, H.; Hildebrandt, P. Redox and Conformational Equilibria and Dynamics of Cytochrome c at High Electric Fields. ChemPhysChem 2003, 4, 714–724. [Google Scholar] [CrossRef]
- Hildebrandt, P.; Heimburg, T.; Marsh, D. Quantitative Conformational Analysis of Cytochrome c Bound to Phospholipid Vesicles Studied by Resonance Raman Spectroscopy. Eur. Biophys. J. 1990, 18, 193–201. [Google Scholar] [CrossRef]
- Oellerich, S.; Lecomte, S.; Paternostre, M.; Heimburg, T.; Hildebrandt, P. Peripheral and Integral Binding of Cytochrome c to Phospholipids Vesicles. J. Phys. Chem. B. 2004, 108, 3781–3878. [Google Scholar] [CrossRef]
- De Kruijff, B.; Cullis, P.R. Cytochrome c Specifically Induces Non-Bilayer Structures in Cardiolipin Containing Model Membranes. Biochim. Biophys. Acta 1980, 602, 470–499. [Google Scholar] [CrossRef]
- Mohammadyani, D.; Yanamala, N.; Samhan-Arias, A.K.; Kapralov, A.A.; Stepanov, G.; Nuar, N.; Planas-Iglesias, J.; Sanghera, N.; Kagan, V.E.; Klein-Seetharaman, J. Structural Characterization of Cardiolipin-Driven Activation of Cytochrome c into a Peroxidase and Membrane Perturbation. Biochim. Biophys. Acta (BBA)—Biomembr. 2018, 1860, 1057–1068. [Google Scholar] [CrossRef]
- Beales, P.A.; Bergstrom, C.L.; Geerts, N.; Groves, J.T.; Vanderlick, T.K. Single Vesicle Observations of the Cardiolipin-Cytochrome c Interaction: Induction of Membrane Morphology Changes. Langmuir 2011, 27, 6107–6115. [Google Scholar] [CrossRef] [PubMed]
- Bergstrom, C.L.; Beales, P.A.; Lv, Y.; Vanderlick, T.K.; Groves, J.T. Cytochrome c Causes Pore Formation in Cardiolipin-Containing Membranes. Proc. Natl. Acad. Sci. USA 2013, 110, 6269–6274. [Google Scholar] [CrossRef] [PubMed]
- Kitt, J.P.; Bryce, D.A.; Minteer, S.D.; Harris, J.M. Raman Spectroscopy Reveals Selective Interactions of Cytochrome c with Cardiolipin That Correlate with Membrane Permeability. J. Am. Chem. Soc. 2017, 139, 3851–3860. [Google Scholar] [CrossRef] [PubMed]
- Gajhede, M.; Schuller, D.; Henricksen, A.; Smith, A.; Poulos, T. Crystal Structure Determination of Classical Horseradish Peroxidase at 2.15A Resolution. Nat. Struct. Mol. Biol. 1997, 4, 1032–1039. [Google Scholar] [CrossRef] [PubMed]
- Kincaid, J.R.; Zheng, Y.; Al-Mustafa, J.; Czarnecki, K. Resonance Raman Spectra of Native and Mesoheme-Reconstituted Horseradish Peroxidase and Their Catalytic Intermediates. J. Biol. Chem. 1996, 271, 28805–28811. [Google Scholar] [CrossRef]
- Belikova, N.A.; Vladimirov, Y.A.; Osipov, A.N.; Kapralov, A.A.; Tyurin, V.A.; Potapovich, M.V.; Basova, L.V.; Peterson, J.; Kurnikov, I.V.; Kagan, V.E. Peroxidase Activity and Structural Transitions of Cytochrome c Bound to Cardiolipin-Containing Membranes. Biochemistry 2006, 45, 4998–5009. [Google Scholar] [CrossRef]
- Patriarca, A.; Polticelli, F.; Piro, M.C.; Sinibaldi, F.; Mei, G.; Bari, M.; Santucci, R.; Fiorucci, L. Conversion of Cytochrome c into a Peroxidase: Inhibitory Mechanisms and Implication for Neurodegenerative Diseases. Arch. Biochem. Biophys. 2012, 522, 62–69. [Google Scholar] [CrossRef]
- Shah, R.; Schweitzer-Stenner, R. Structural Changes of Horse Heart Ferricytochrome c Induced by Changes of Ionic Strength and Anion Binding. Biochemistry 2008, 47, 5250–5257. [Google Scholar] [CrossRef]
- Kurbaj, R.; Milorey, B.; Schweitzer-Stenner, R. Substrate Induced Conformational Changes of Liposomes Bound Cytochrome c. Biophys. J. 2019, 116, 519A. [Google Scholar] [CrossRef]
- McClelland, L.J.; Mou, T.-C.; Jeakins-Cooley, M.E.; Sprang, S.R.; Bowler, B.E. Structure of a Mitochondrial Cytochrome c Conformer Competent for Peroxidase Activity. Proc. Nat. Acad. Sci. USA 2014, 111, 6648–6653. [Google Scholar] [CrossRef]
- Kleckner, I.R.; Foster, M.P. An Introduction to NMR-Based Approaches for Measuring Protein Dynamics. Biochim. Biophys. Acta (BBA)—Proteins Proteom. 2011, 1814, 942–968. [Google Scholar] [CrossRef] [PubMed]
- Olafsson, G.; Sparr, E. Ionization Constants pKa of Cardiolipin. PLoS ONE 2013, 8, e73040. [Google Scholar] [CrossRef] [PubMed]
- Kooijman, E.E.; Swim, L.A.; Garber, Z.T.; Tyurina, Y.Y.; Bayir, H.; Kagan, V.R. Magic Angle Spinning 31NMR Spectroscopy Reveals Two Essentially Identical Ionization States for the Cardiolipin Phosphates in Phospholipid Liposomes. Biochim. Biophys. Acta 2017, 1859, 61–68. [Google Scholar] [CrossRef] [PubMed]
- Schwalbe, M.; Ozenne, V.; Bibow, S.; Jaremko, M.; Jaremko, L.; Gajda, M.; Jensen, M.R.; Biernat, J.; Becker, S.; Mandelkow, E.; et al. Predictive Atomic Resolution Descriptions of Intrinsically Disordered HTau40 and α-Synuclein in Solution from NMR and Small Angle Scattering. Structure 2014, 22, 238–249. [Google Scholar] [CrossRef]
- Schweitzer-Stenner, R. The Relevance of Short Peptides for an Understanding of Unfolded and Intrinsically Disordered Proteins. Phys. Chem. Chem. Phys. 2023, 25, 11908–11933. [Google Scholar] [CrossRef]
- Tanaka, S.; Scheraga, H.A. Statistical Mechanical Treatment of Protein Conformation. 4. A Four-State Model for Specific-Sequence Copolymers of Amino Acids. Macromolecules 1976, 9, 812–833. [Google Scholar] [CrossRef]
- Tanaka, S.; Scheraga, H.A. Statistical Mechanical Treatment of Protein Conformation. 5. A Multistate Model for Specific Sequence Copolymers of Amino Acids. Macromolecules 1977, 10, 9–20. [Google Scholar] [CrossRef]
- Khare, S.D.; Chinchilla, P.; Baum, J. Multifaceted Interactions Mediated by Intrinsically Disordered Regions Play Key Roles in Alpha Synuclein Aggregation. Curr. Opin. Struct. Biol. 2023, 80, 102579. [Google Scholar] [CrossRef]
- Davidson, W.S.; Jonas, A.; Clayton, D.F.; George, J.M. Stabilization of α-Synuclein Secondary Structure upon Binding to Synthetic Membranes. J. Biol. Chem. 1998, 273, 9443–9449. [Google Scholar] [CrossRef]
- Andersson, A.; Linse, S.; Sparr, E.; Fornasier, M.; Jönsson, P. The Density of Anionic Lipids Modulates the Adsorption of α-Synuclein onto Lipid Membranes. Biophys. Chem. 2024, 305, 107143. [Google Scholar] [CrossRef]
- Busch, D.J.; Houser, J.R.; Hayden, C.C.; Sherman, M.B.; Lafer, E.M.; Stachowiak, J.C. Intrinsically Disordered Proteins Drive Membrane Curvature. Nat. Commun. 2015, 6, 7875. [Google Scholar] [CrossRef] [PubMed]
- Hellstrand, E.; Grey, M.; Ainalem, M.-L.; Ankner, J.; Forsyth, V.T.; Fragneto, G.; Haertlein, M.; Dauvergne, M.-T.; Nilsson, H.; Brundin, P.; et al. Adsorption of α-Synuclein to Supported Lipid Bilayers: Positioning and Role of Electrostatics. ACS Chem. Neurosci. 2013, 4, 1339–1351. [Google Scholar] [CrossRef] [PubMed]
- Makasewicz, K.; Wennmalm, S.; Stenqvist, B.; Fornasier, M.; Andersson, A.; Jönsson, P.; Linse, S.; Sparr, E. Cooperativity of α-Synuclein Binding to Lipid Membranes. ACS Chem. Neurosci. 2021, 12, 2099–2109. [Google Scholar] [CrossRef] [PubMed]
- Adair, G.S.; Bock, A.V.; Field, H. THE HEMOGLOBIN SYSTEM. J. Biol. Chem. 1925, 63, 515–516. [Google Scholar] [CrossRef]
- Monod, J.; Wyman, J.; Changeux, J.-P. On the Nature of Allosteric Transitions: A Plausible Model. J. Mol. Biol. 1965, 12, 88–118. [Google Scholar] [CrossRef]
- Braun, A.R.; Lacy, M.M.; Ducas, V.C.; Rhoades, E.; Sachs, J.N. α-Synuclein’s Uniquely Long Amphipathic Helix Enhances Its Membrane Binding and Remodeling Capacity. J. Membr. Biol. 2017, 250, 183–193. [Google Scholar] [CrossRef]
- Viennet, T.; Wördehoff, M.M.; Uluca, B.; Poojari, C.; Shaykhalishahi, H.; Willbold, D.; Strodel, B.; Heise, H.; Buell, A.K.; Hoyer, W.; et al. Structural Insights from Lipid-Bilayer Nanodiscs Link α-Synuclein Membrane-Binding Modes to Amyloid Fibril Formation. Commun. Biol. 2018, 1, 44. [Google Scholar] [CrossRef]
- Denisov, I.G.; Sligar, S.G. Nanodiscs for Structural and Functional Studies of Membrane Proteins. Nat. Struct. Mol. Biol. 2016, 23, 481–486. [Google Scholar] [CrossRef]
- Pirc, K.; Ulrih, N.P. α-Synuclein Interactions with Phospholipid Model Membranes: Key Roles for Electrostatic Interactions and Lipid-Bilayer Structure. Biochim. Biophys. Acta (BBA)—Biomembr. 2015, 1848, 2002–2012. [Google Scholar] [CrossRef]
- Middleton, E.R.; Rhoades, E. Effects of Curvature and Composition on α-Synuclein Binding to Lipid Vesicles. Biophys. J. 2010, 99, 2279–2288. [Google Scholar] [CrossRef]
- Rhoades, E.; Ramlall, T.F.; Webb, W.W.; Eliezer, D. Quantification of α-Synuclein Binding to Lipid Vesicles Using Fluorescence Correlation Spectroscopy. Biophys. J. 2006, 90, 4692–4700. [Google Scholar] [CrossRef] [PubMed]
- Rocha, S.; Kumar, R.; Nordén, B.; Wittung-Stafshede, P. Orientation of α-Synuclein at Negatively Charged Lipid Vesicles: Linear Dichroism Reveals Time-Dependent Changes in Helix Binding Mode. J. Am. Chem. Soc. 2021, 143, 18899–18906. [Google Scholar] [CrossRef] [PubMed]
- Bernadó, P.; Bertoncini, C.W.; Griesinger, C.; Zweckstetter, M.; Blackledge, M. Defining Long-Range Order and Local Disorder in Native α-Synuclein Using Residual Dipolar Couplings. J. Am. Chem. Soc. 2005, 127, 17968–17969. [Google Scholar] [CrossRef] [PubMed]
- Li, B.; Ge, P.; Murray, K.A.; Sheth, P.; Zhang, M.; Nair, G.; Sawaya, M.R.; Shin, W.S.; Boyer, D.R.; Ye, S.; et al. Cryo-EM of Full-Length α-Synuclein Reveals Fibril Polymorphs with a Common Structural Kernel. Nat. Commun. 2018, 9, 3609. [Google Scholar] [CrossRef]
- Nelson, R.; Sawaya, M.R.; Balbirnie, M.; Madsen, A.; Riekel, C.; Grothe, R.; Eisenberg, D. Structure of the Cross-β Spine of Amyloid-like Fibrils. Nature 2005, 435, 773–778. [Google Scholar] [CrossRef]
- Schweitzer-Stenner, R. The Physics of Protein Structure and Dynamics. When and Why Proteins Fold and Don’t Fold; Elsevier: San Diego, DA, USA, 2024. [Google Scholar]
- Haque, F.; Pandey, A.P.; Cambrea, L.R.; Rochet, J.-C.; Hovis, J.S. Adsorption of α-Synuclein on Lipid Bilayers: Modulating the Structure and Stability of Protein Assemblies. J. Phys. Chem. B 2010, 114, 4070–4081. [Google Scholar] [CrossRef][Green Version]
- Galvagnion, C.; Buell, A.K.; Meisl, G.; Michaels, T.C.T.; Vendruscolo, M.; Knowles, T.P.J.; Dobson, C.M. Lipid Vesicles Trigger α-Synuclein Aggregation by Stimulating Primary Nucleation. Nat. Chem. Biol. 2015, 11, 229–234. [Google Scholar] [CrossRef]
- Gaspar, R.; Lund, M.; Sparr, E.; Linse, S. Anomalous Salt Dependence Reveals an Interplay of Attractive and Repulsive Electrostatic Interactions in α-Synuclein Fibril Formation. QRB Discov. 2020, 1, e2. [Google Scholar] [CrossRef]
- Kiskis, J.; Horvath, I.; Wittung-Stafshede, P.; Rocha, S. Unraveling Amyloid Formation Paths of Parkinson’s Disease Protein α-Synuclein Triggered by Anionic Vesicles. Q. Rev. Biophys. 2017, 50, e3. [Google Scholar] [CrossRef]
- Ghosh, S.; Mahapatra, A.; Chattopadhyay, K. Modulation of α-Synuclein Aggregation by Cytochrome c Binding and Hetero-Dityrosine Adduct Formation. ACS Chem. Neurosci. 2019, 10, 1300–1310. [Google Scholar] [CrossRef]
- Sinibaldi, F.; Howes, B.D.; Piro, M.C.; Polticelli, F.; Bombelli, C.; Ferri, T.; Coletta, M.; Smulevich, G.; Santucci, R. Extended Cardiolipin Anchorage to Cytochrome c: A Model for Protein-Mitochondrial Membrane Binding. J. Biol. Inorg. Chem. 2010, 15, 689–700. [Google Scholar] [CrossRef]
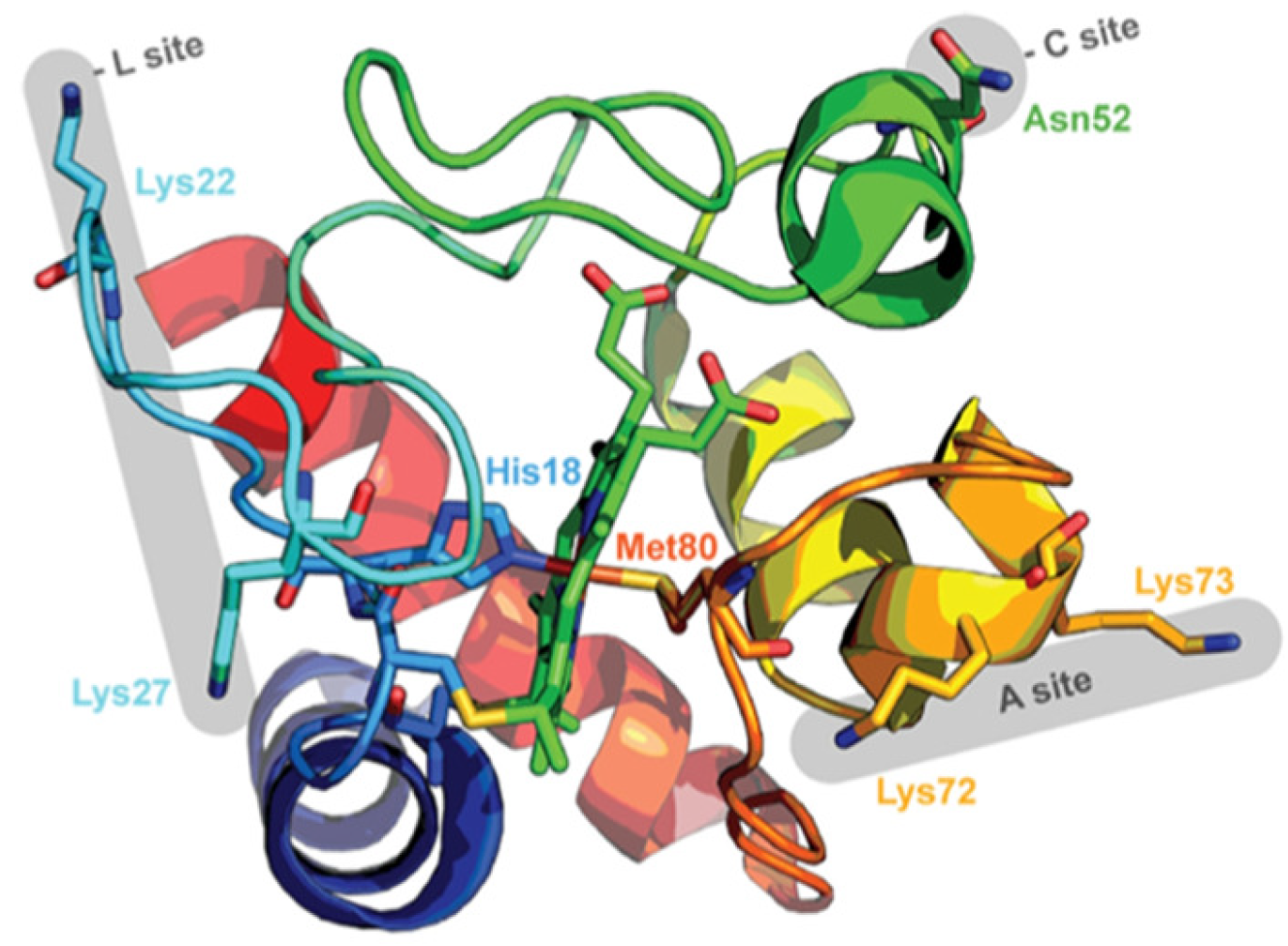
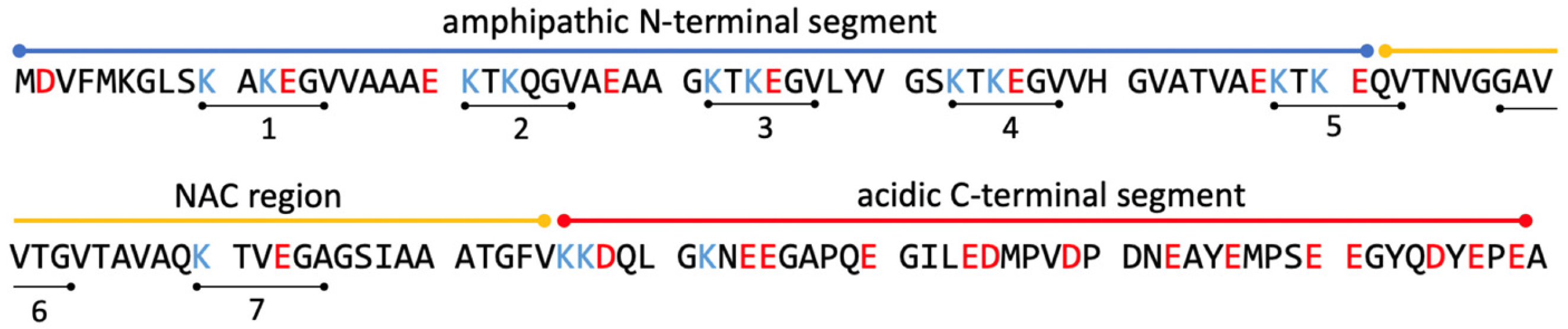
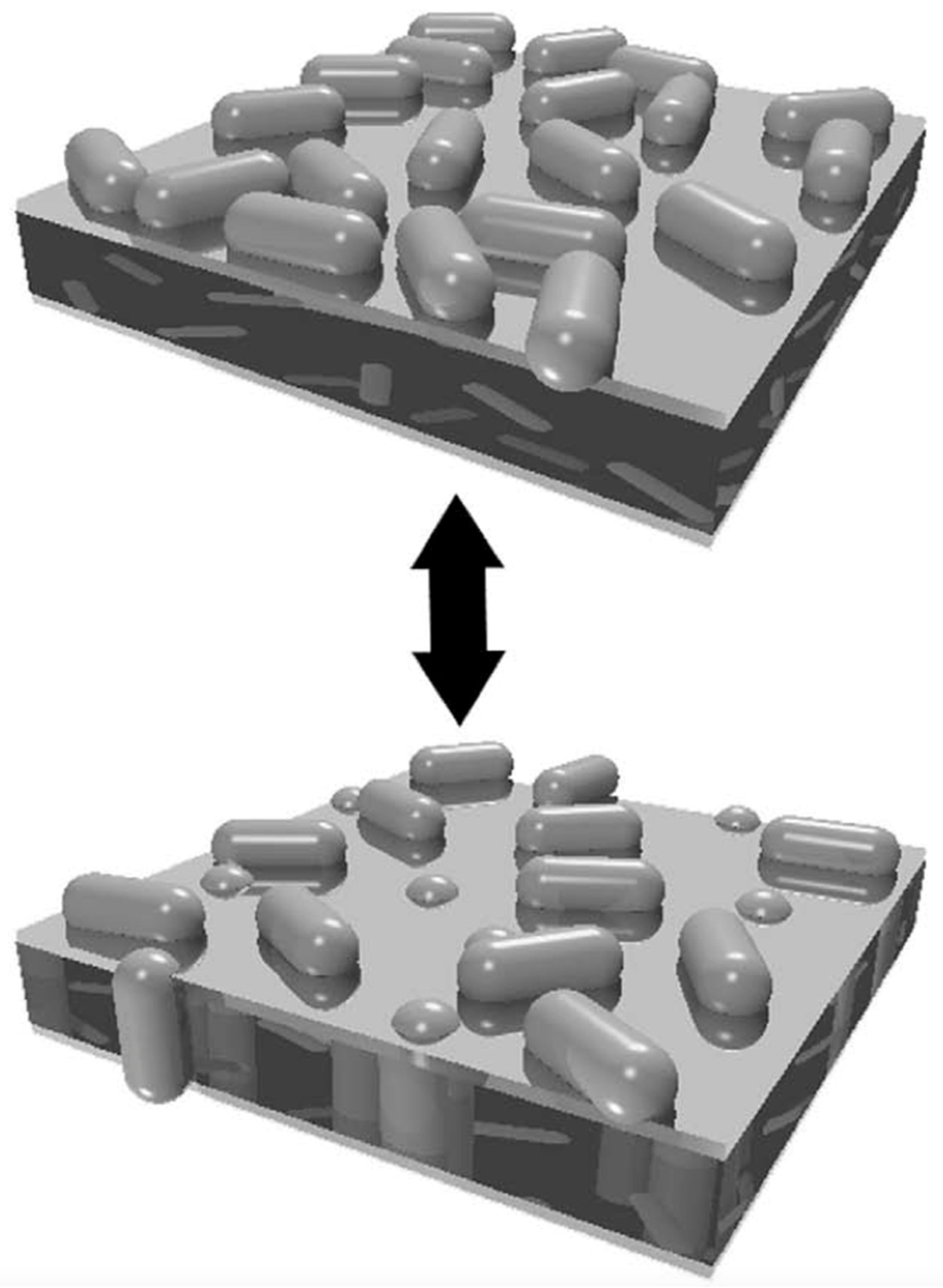
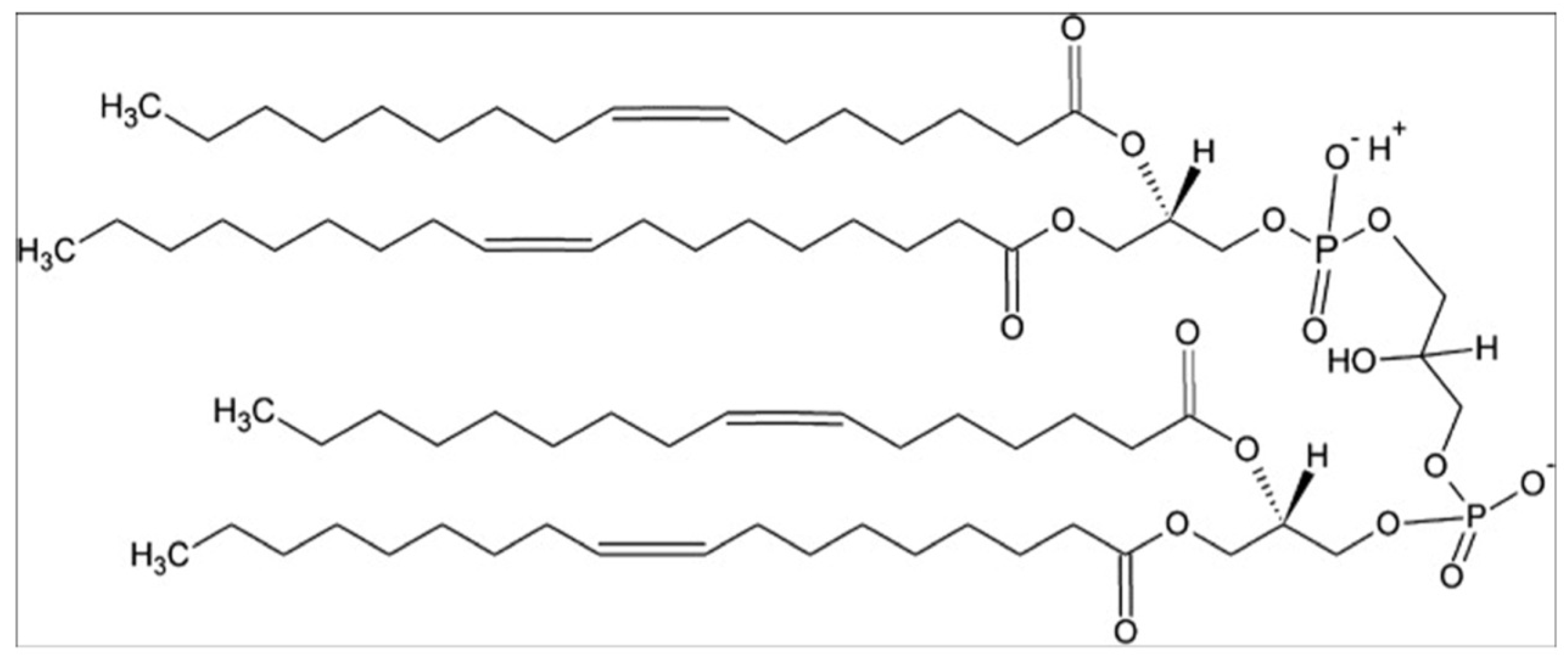
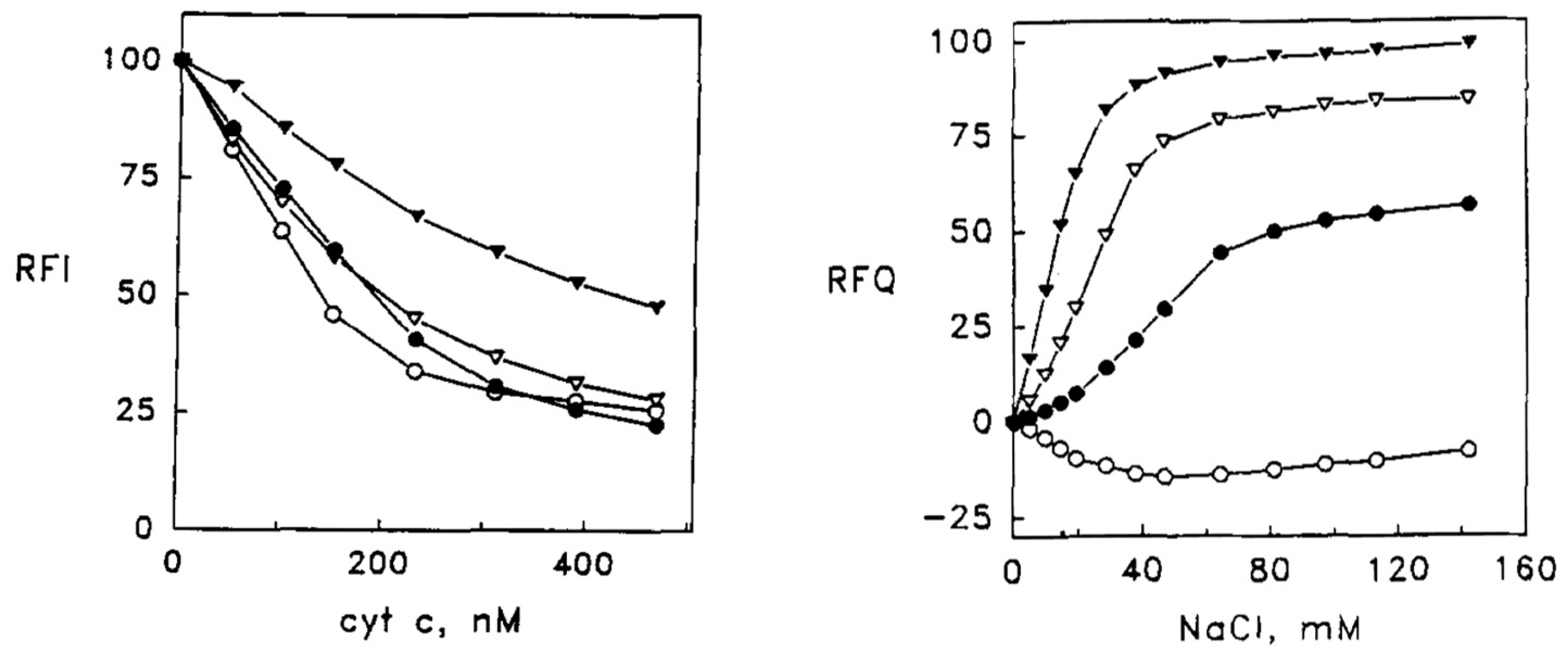
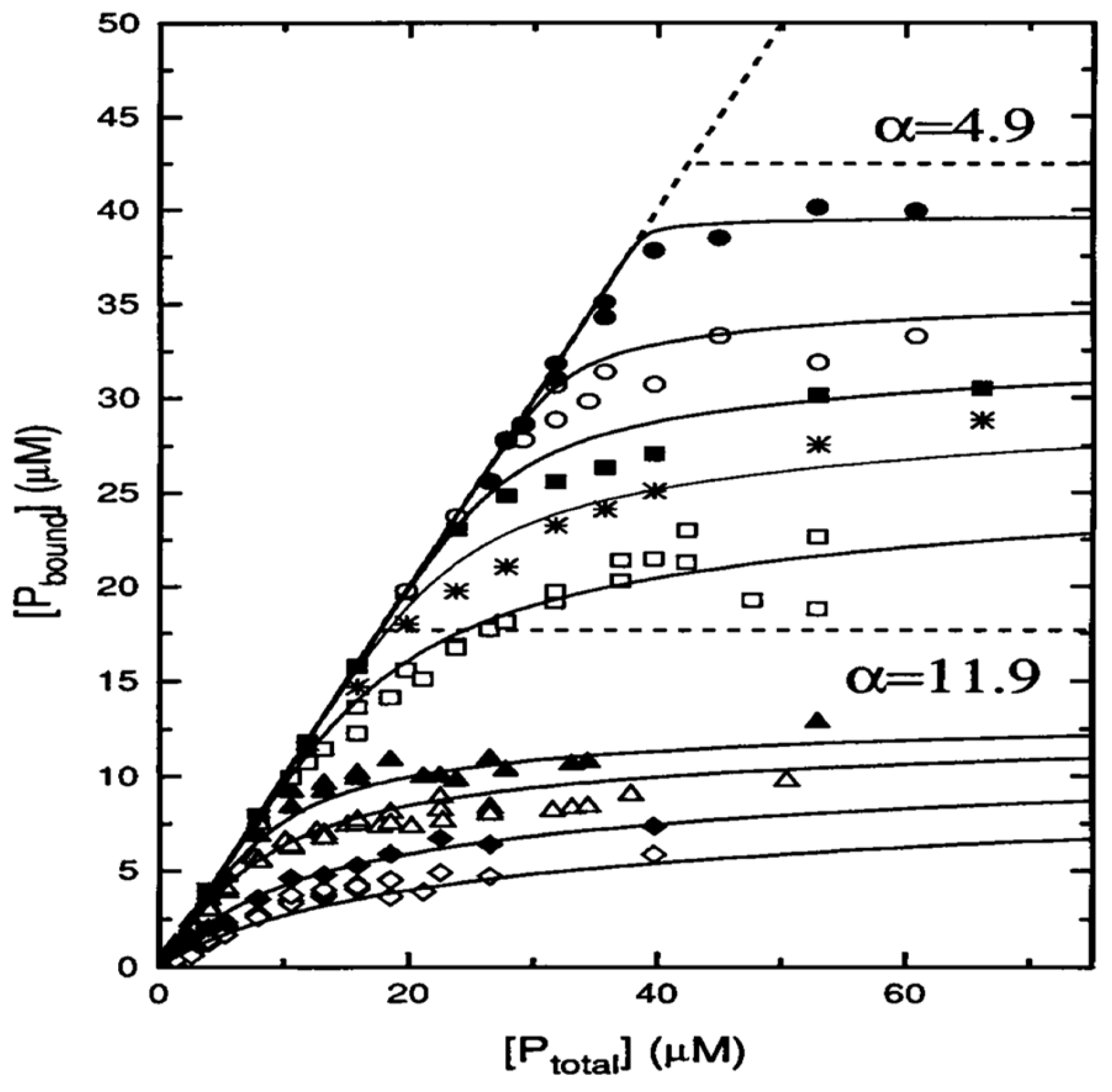
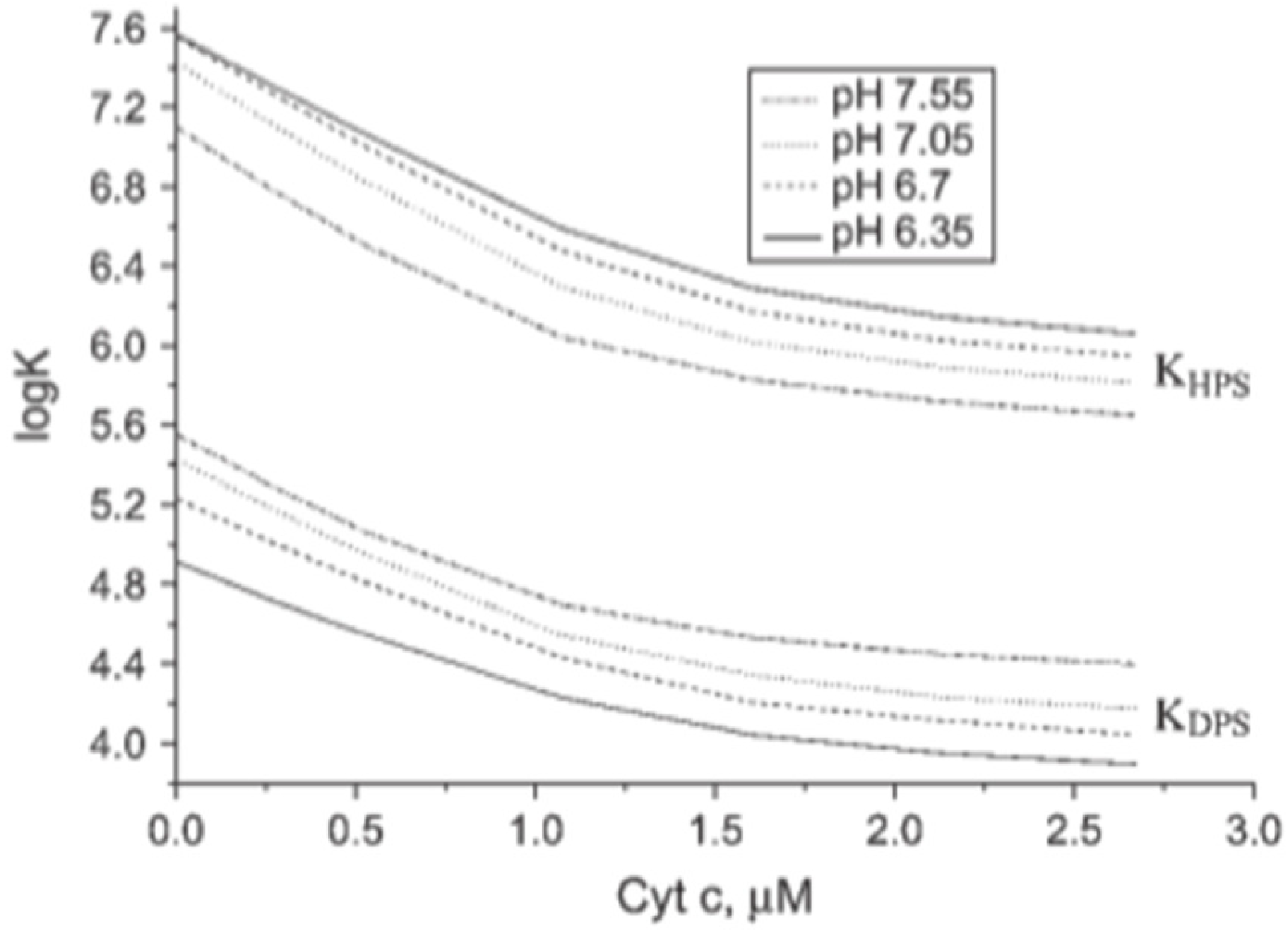
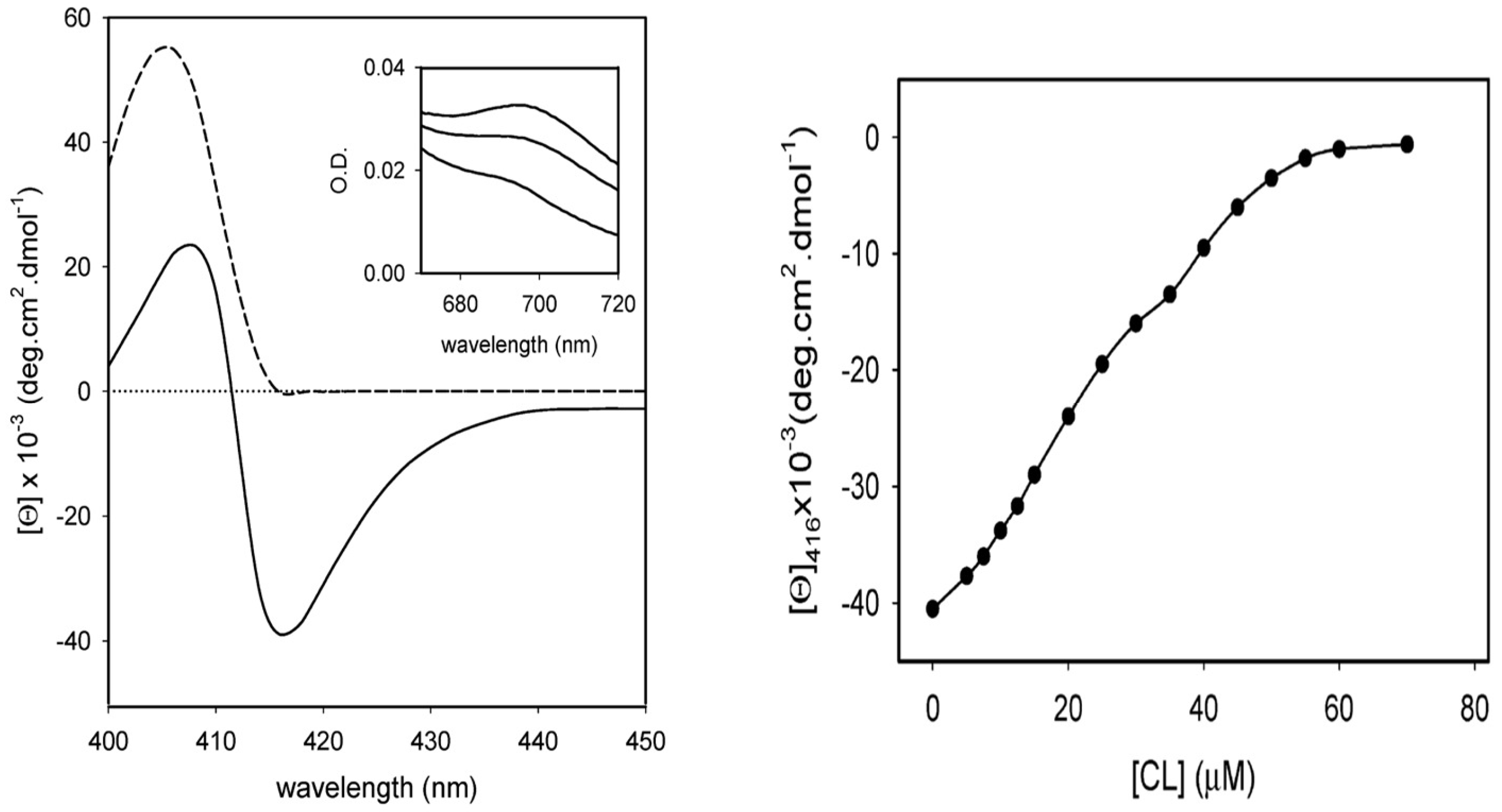


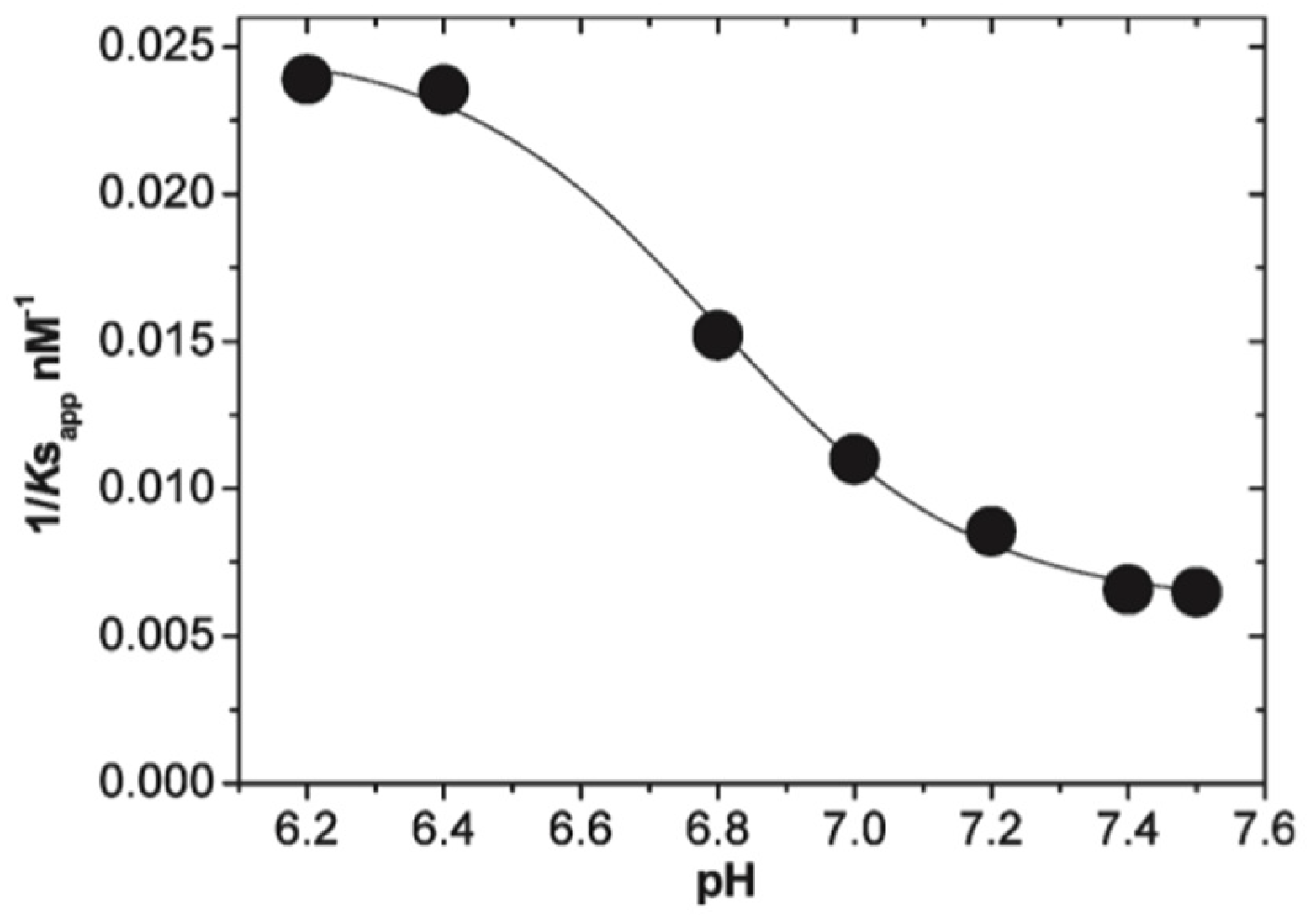
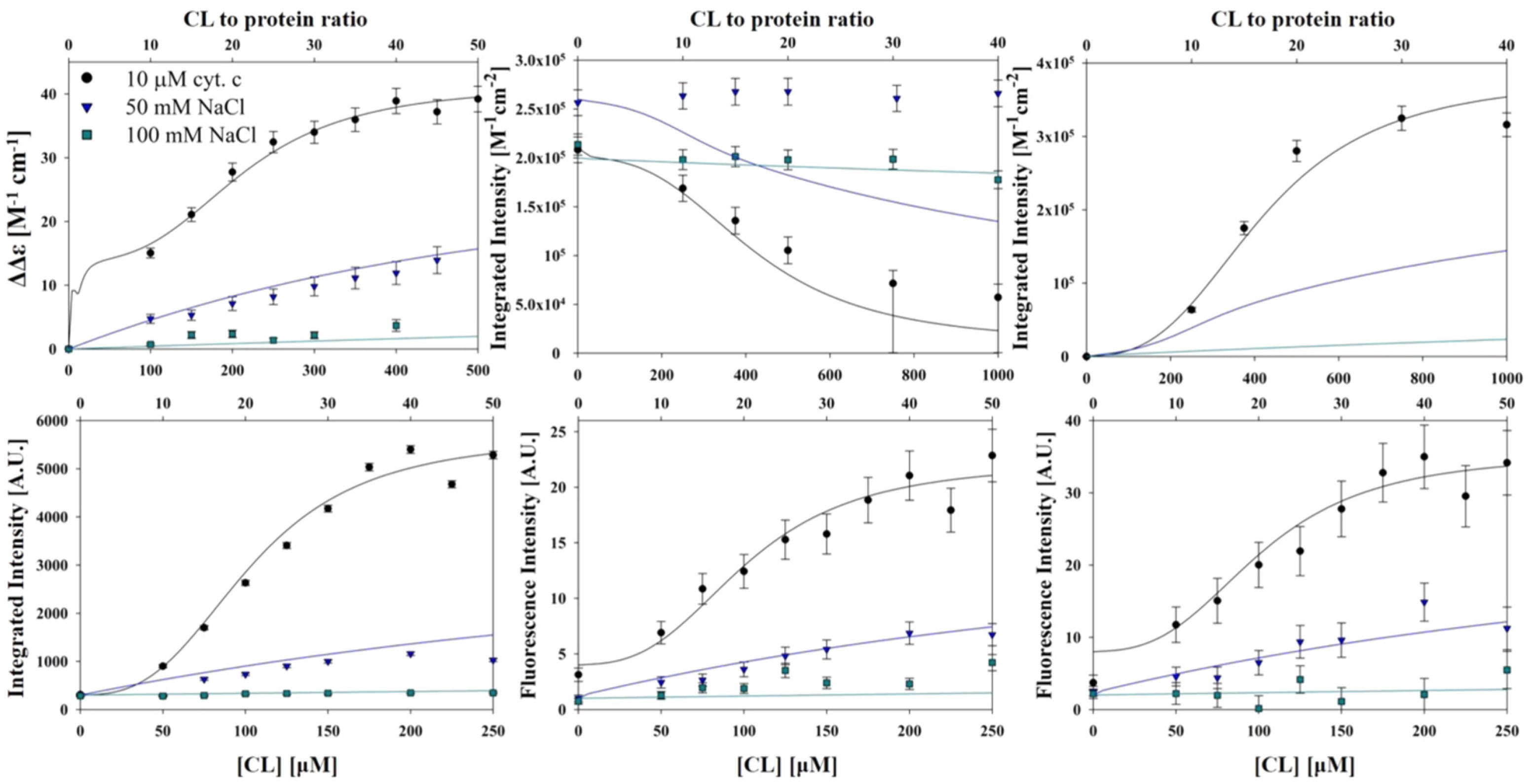





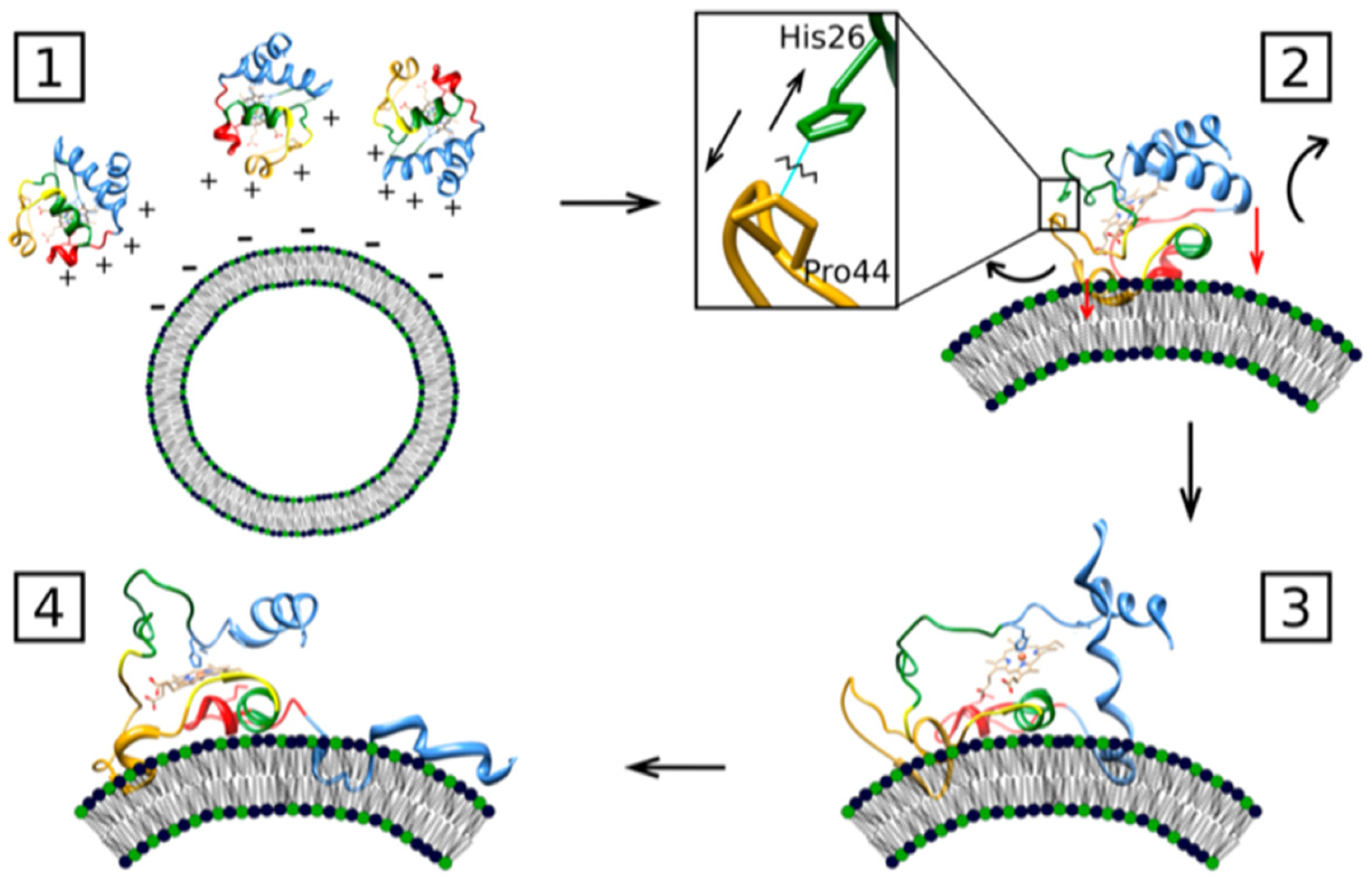
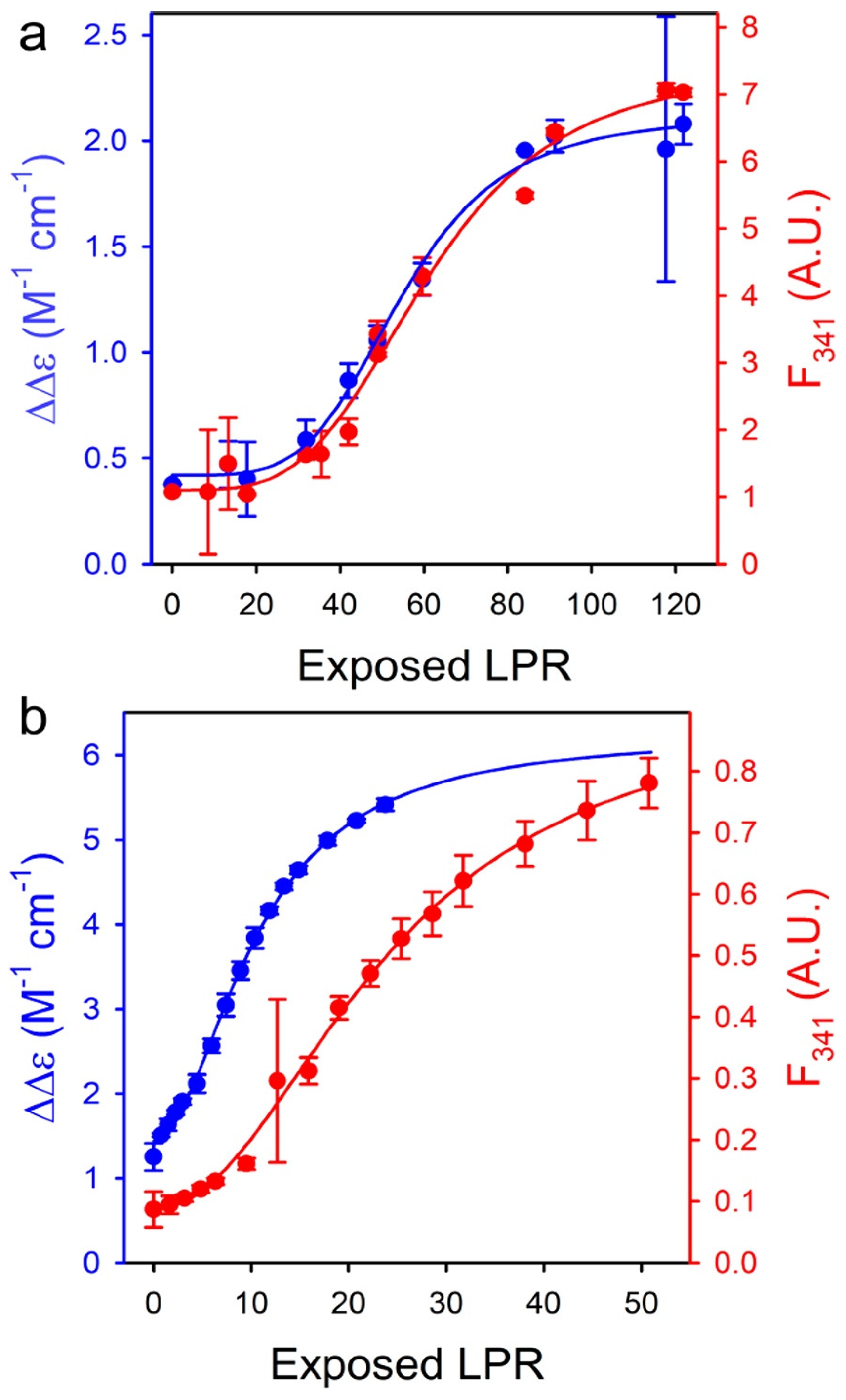

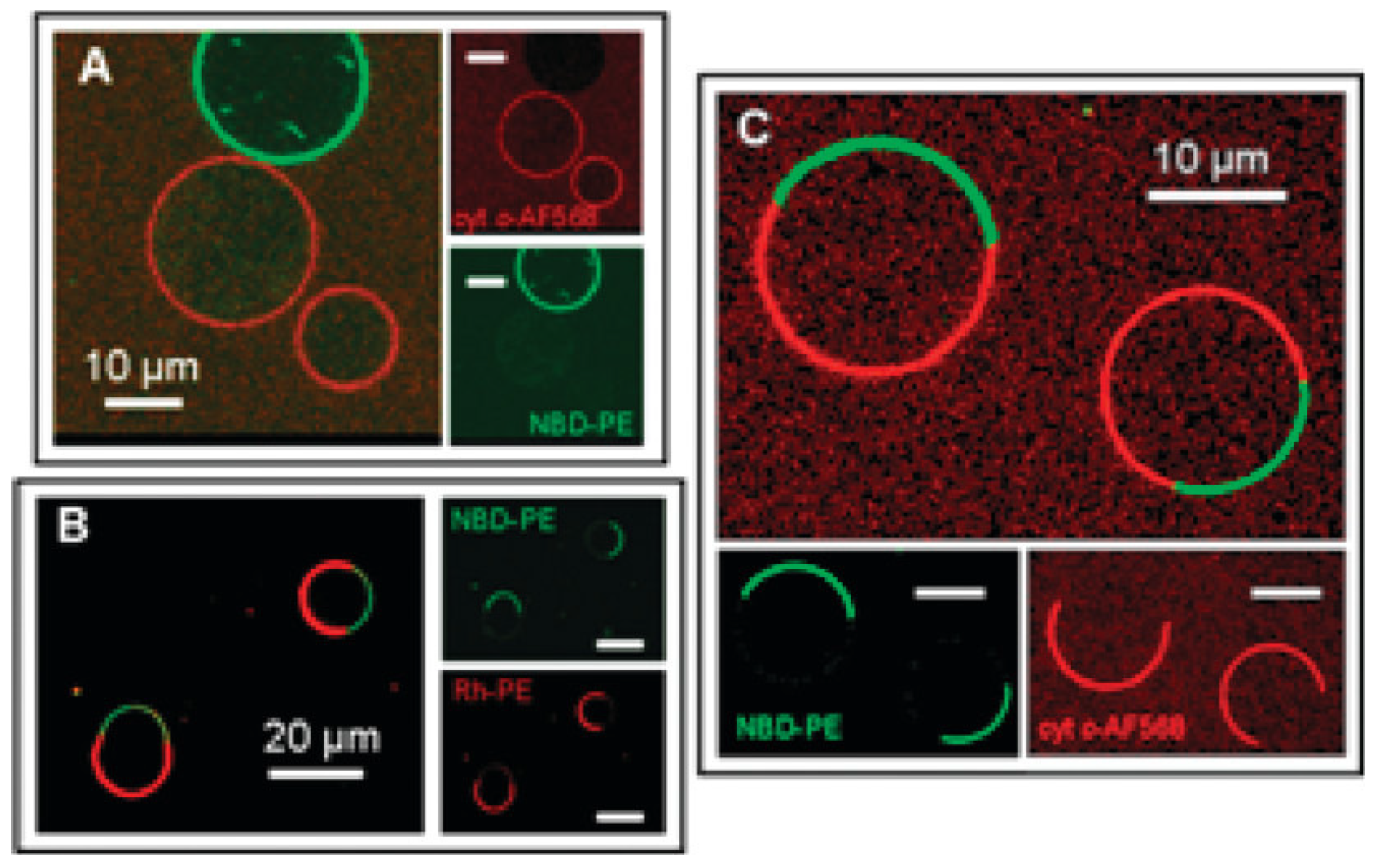
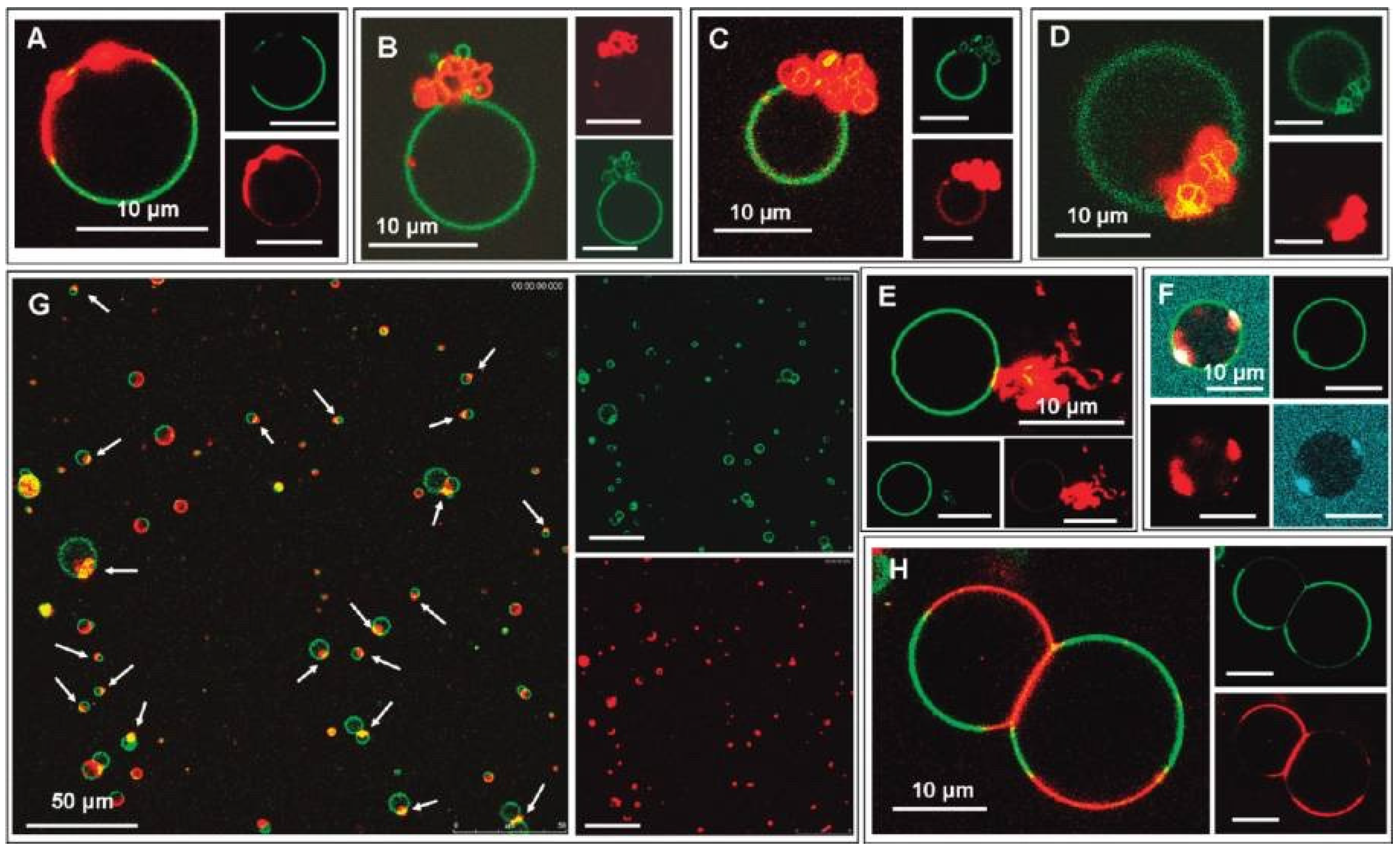

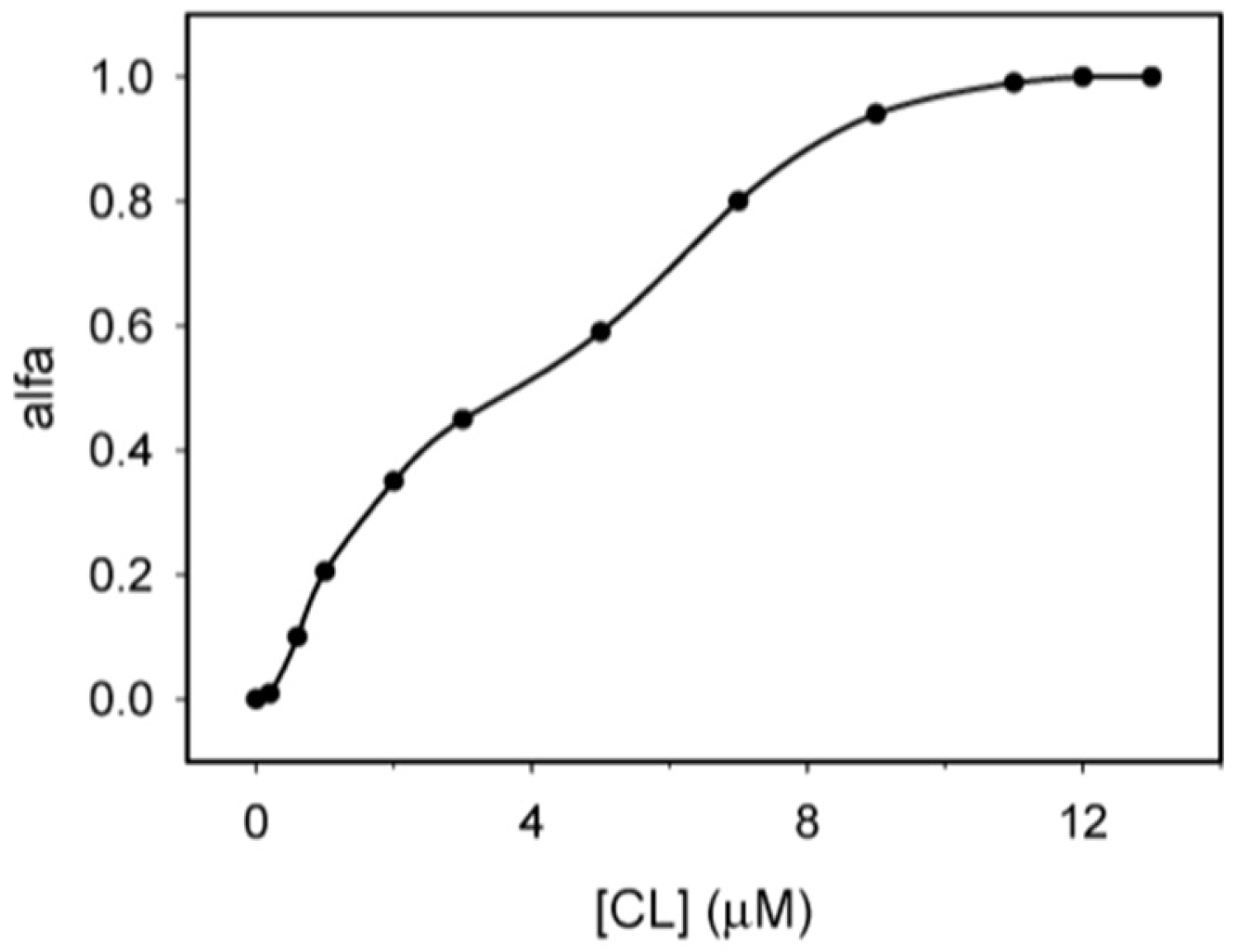
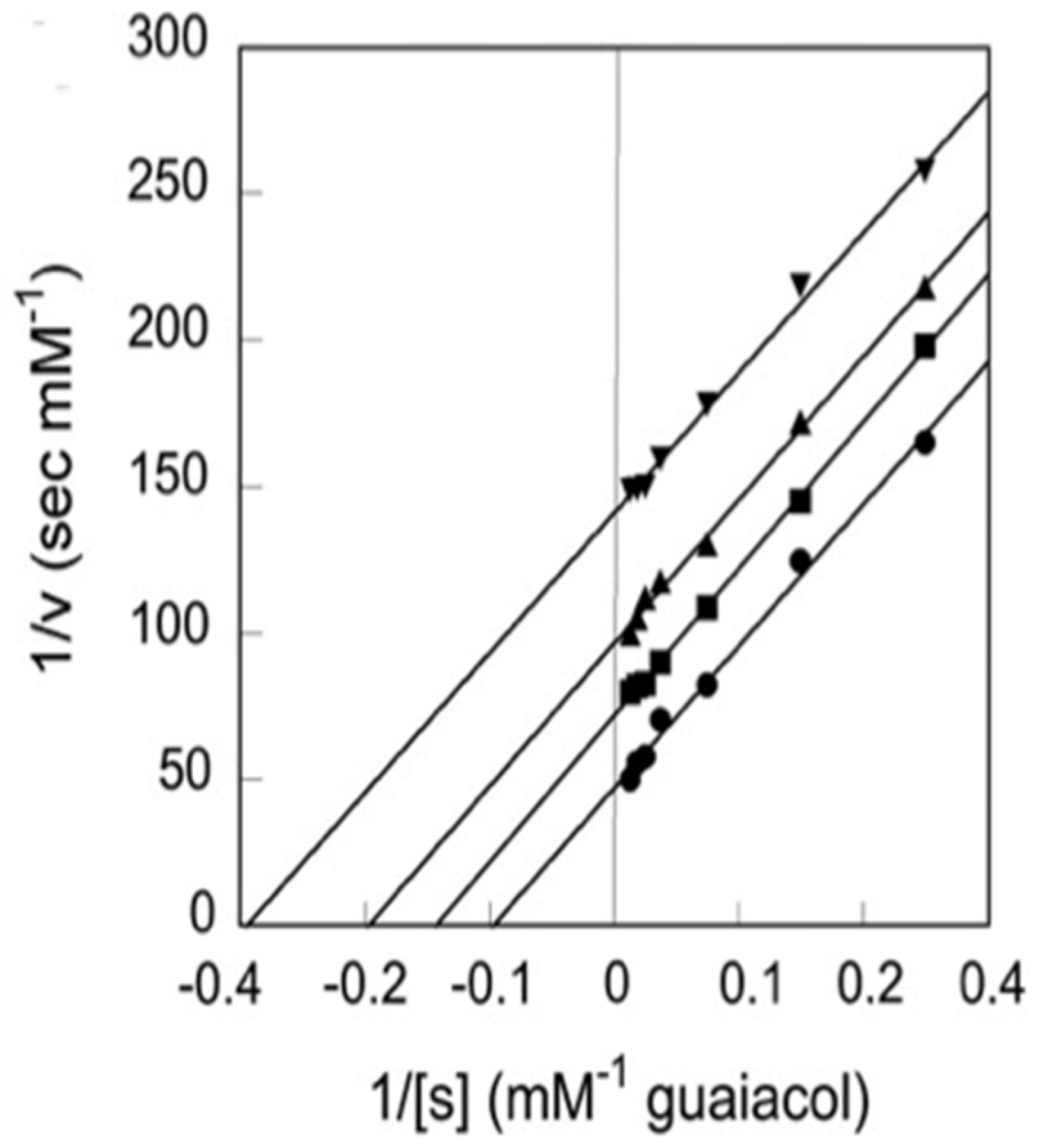
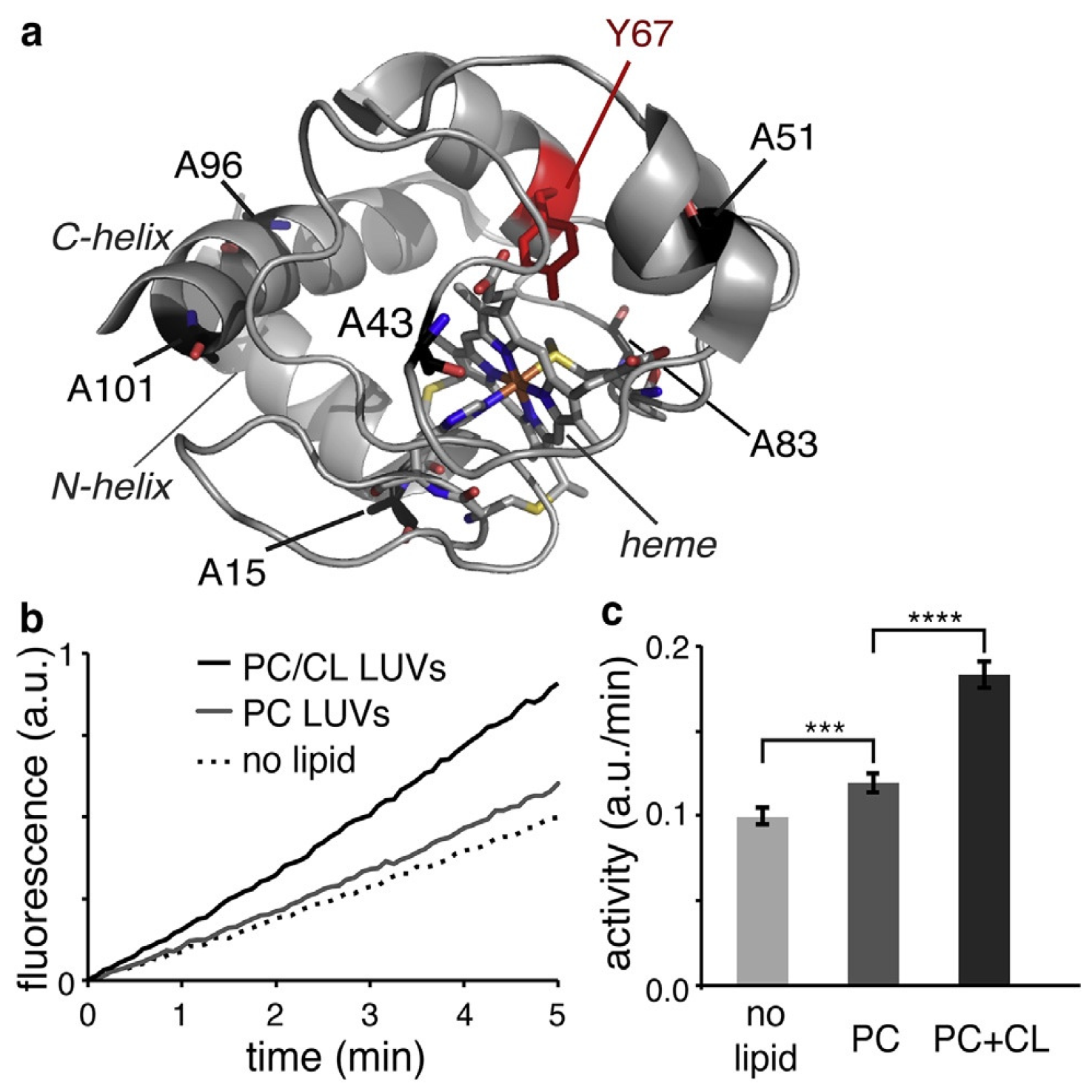
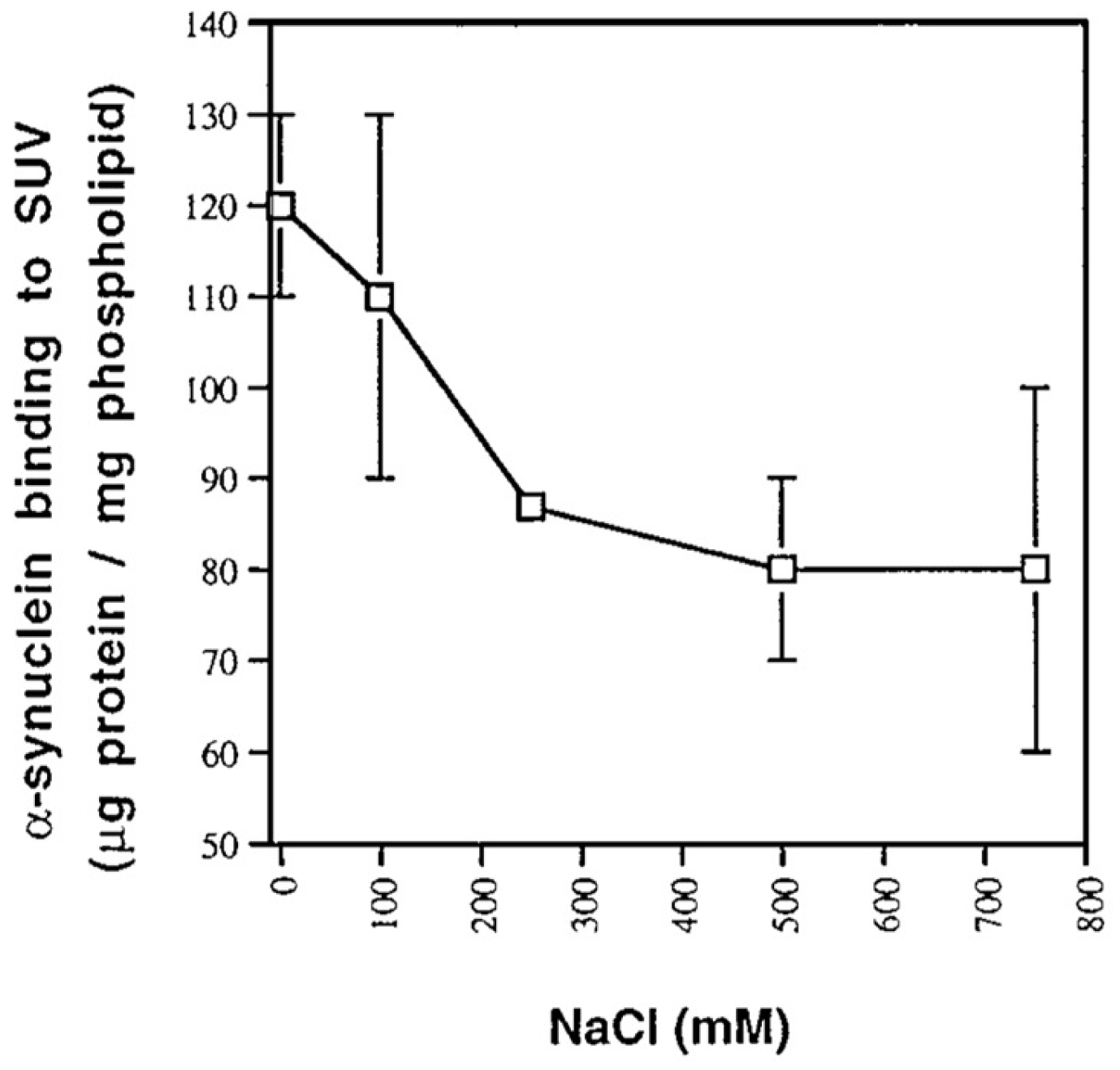
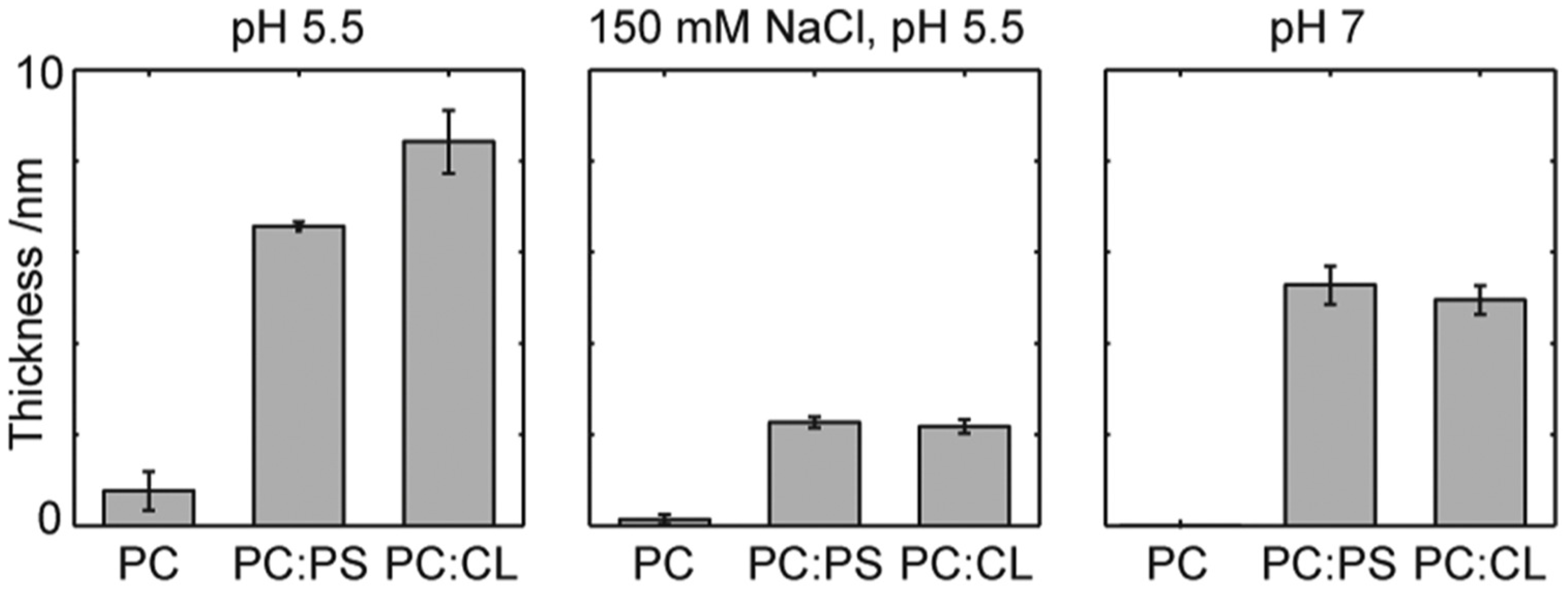
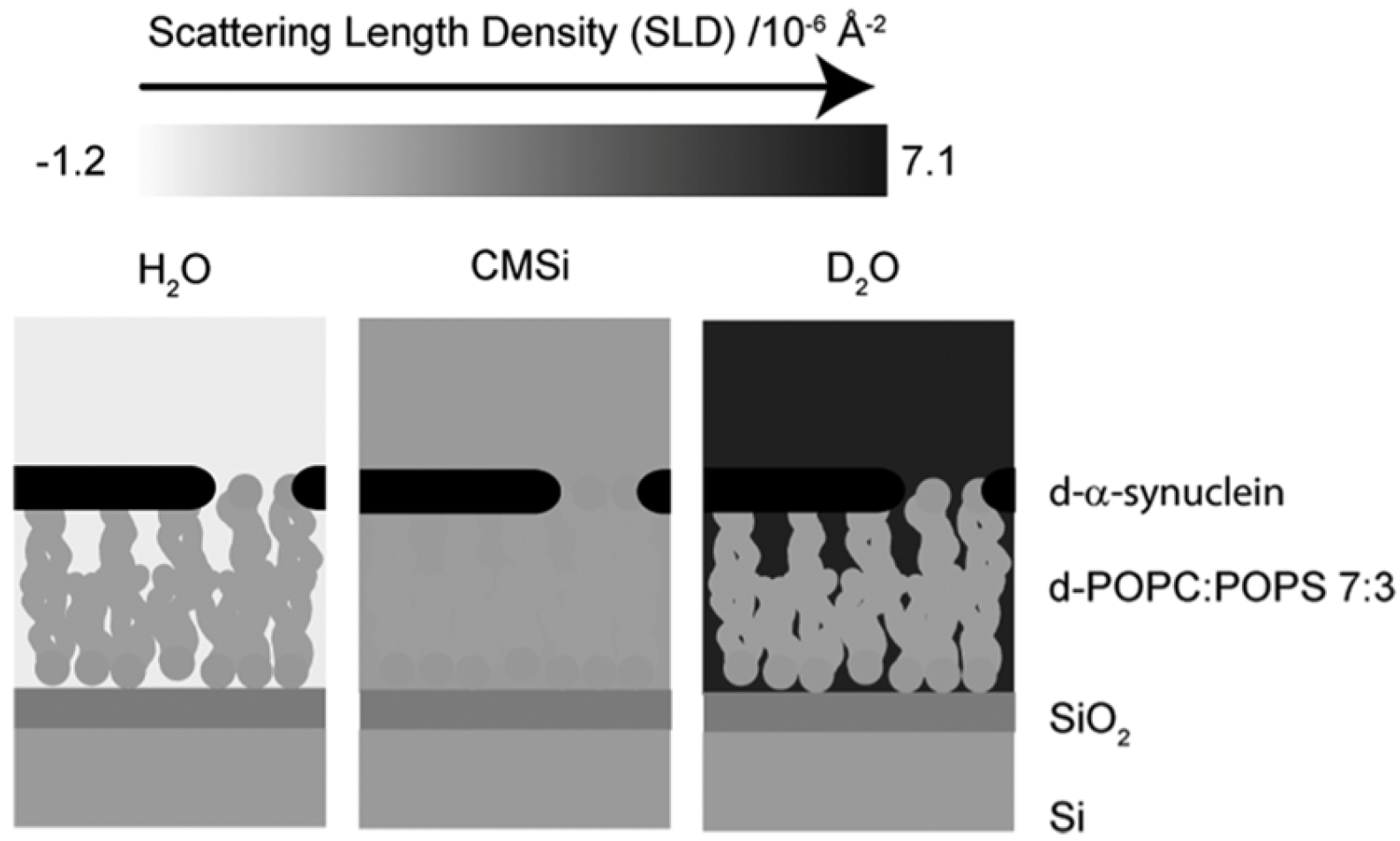

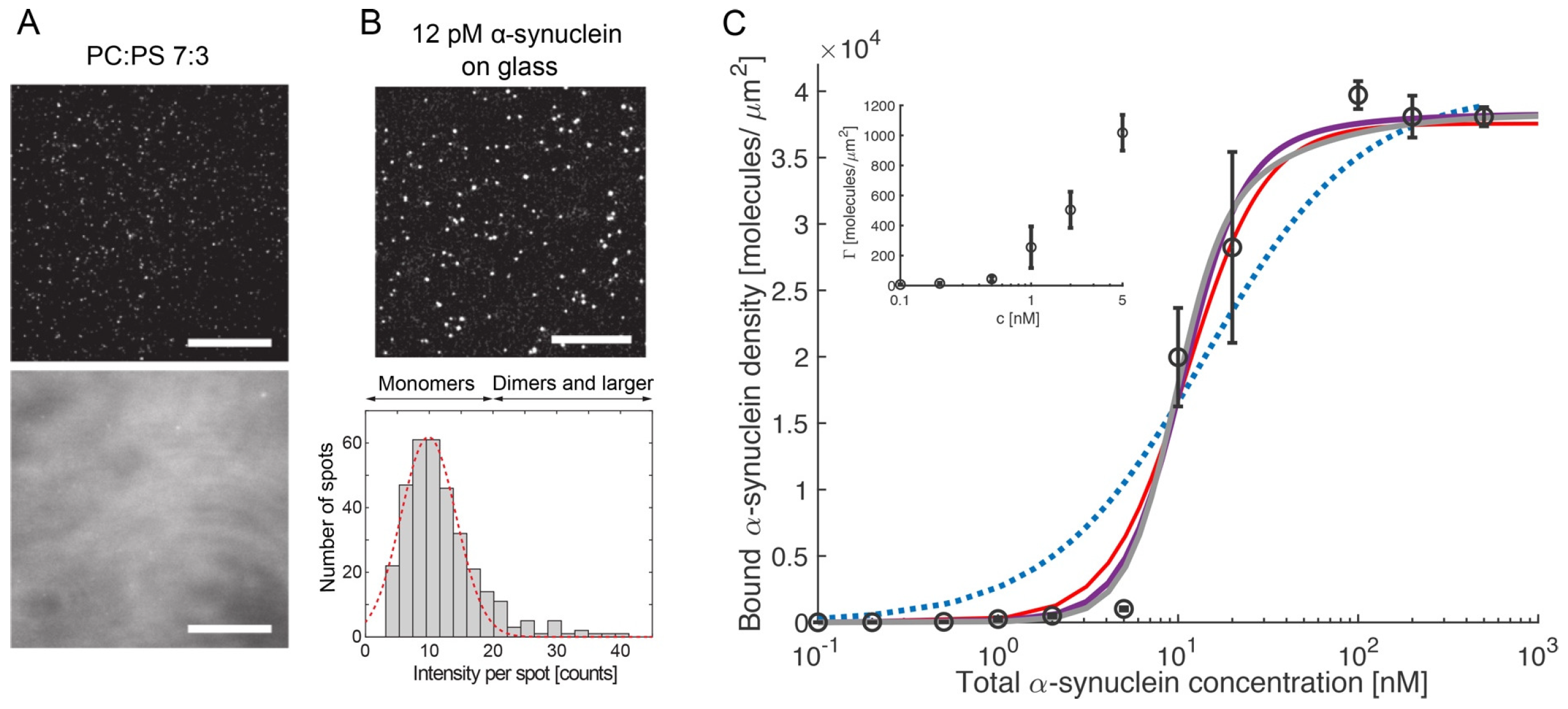
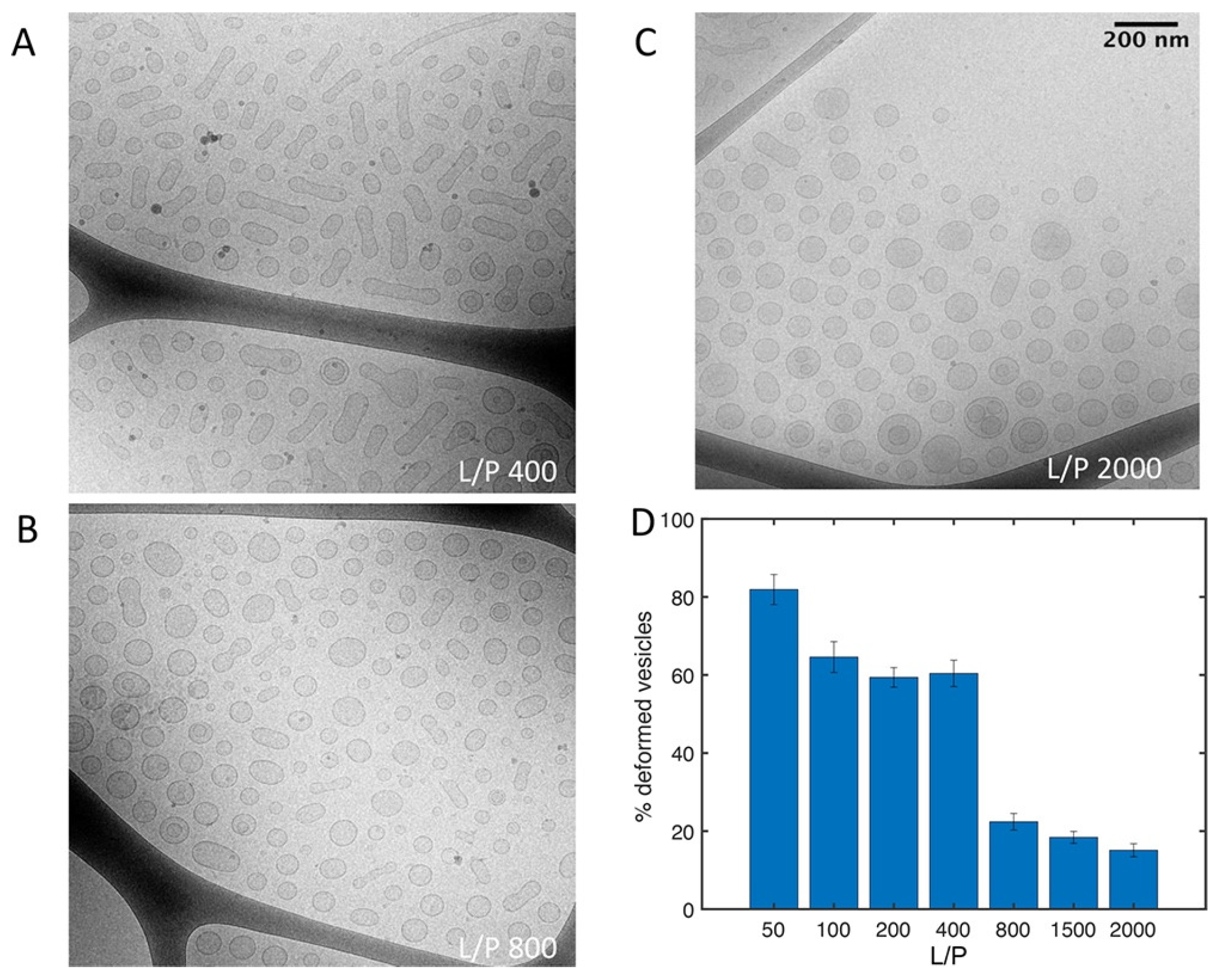
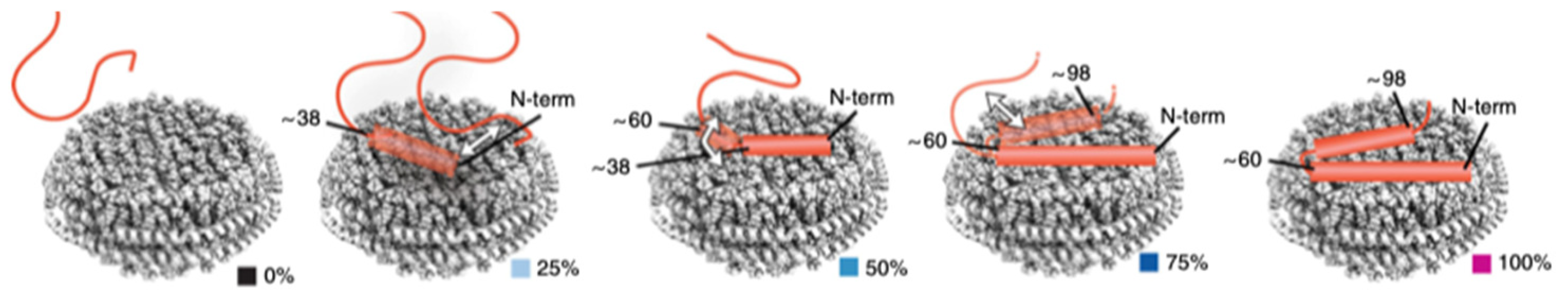
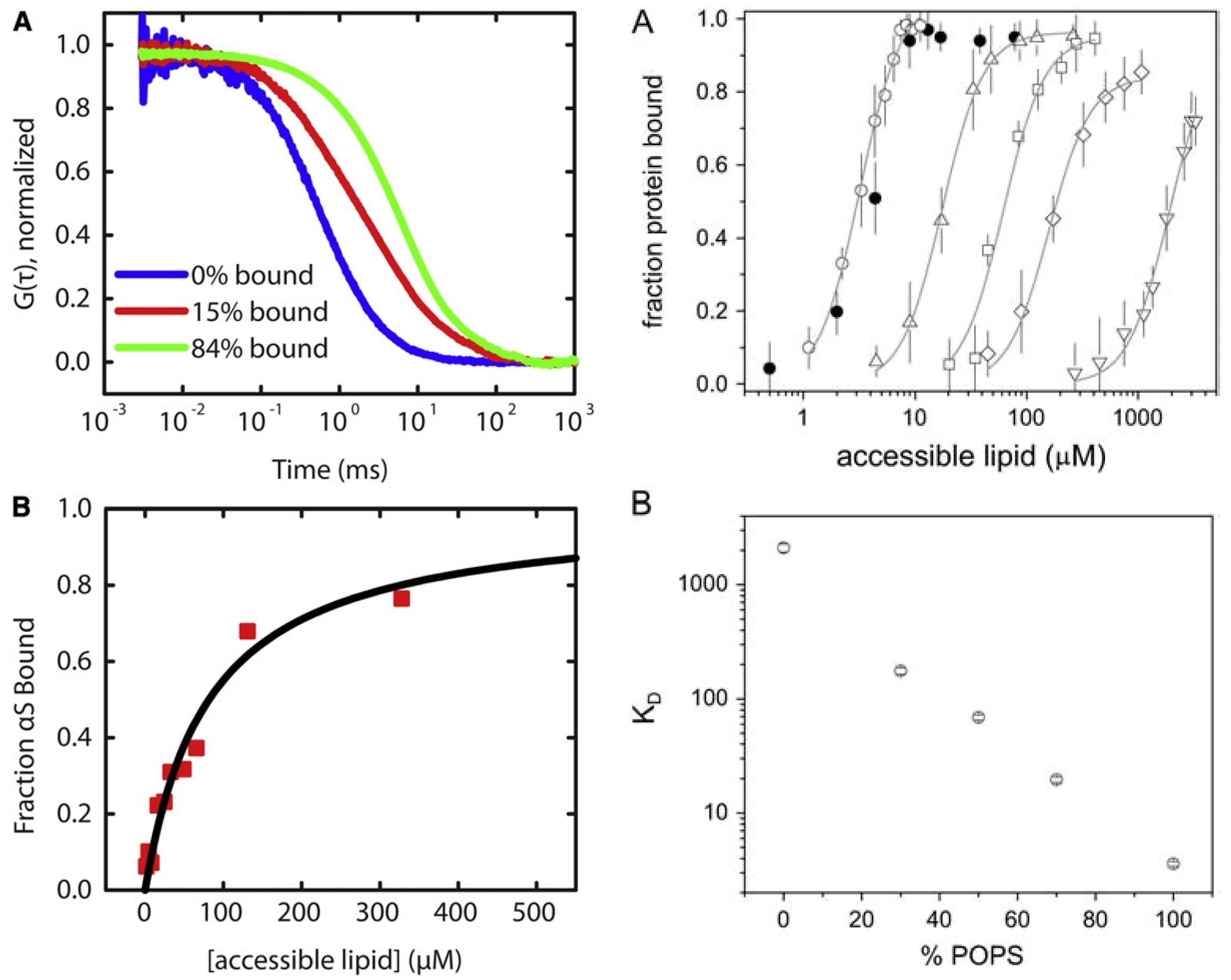

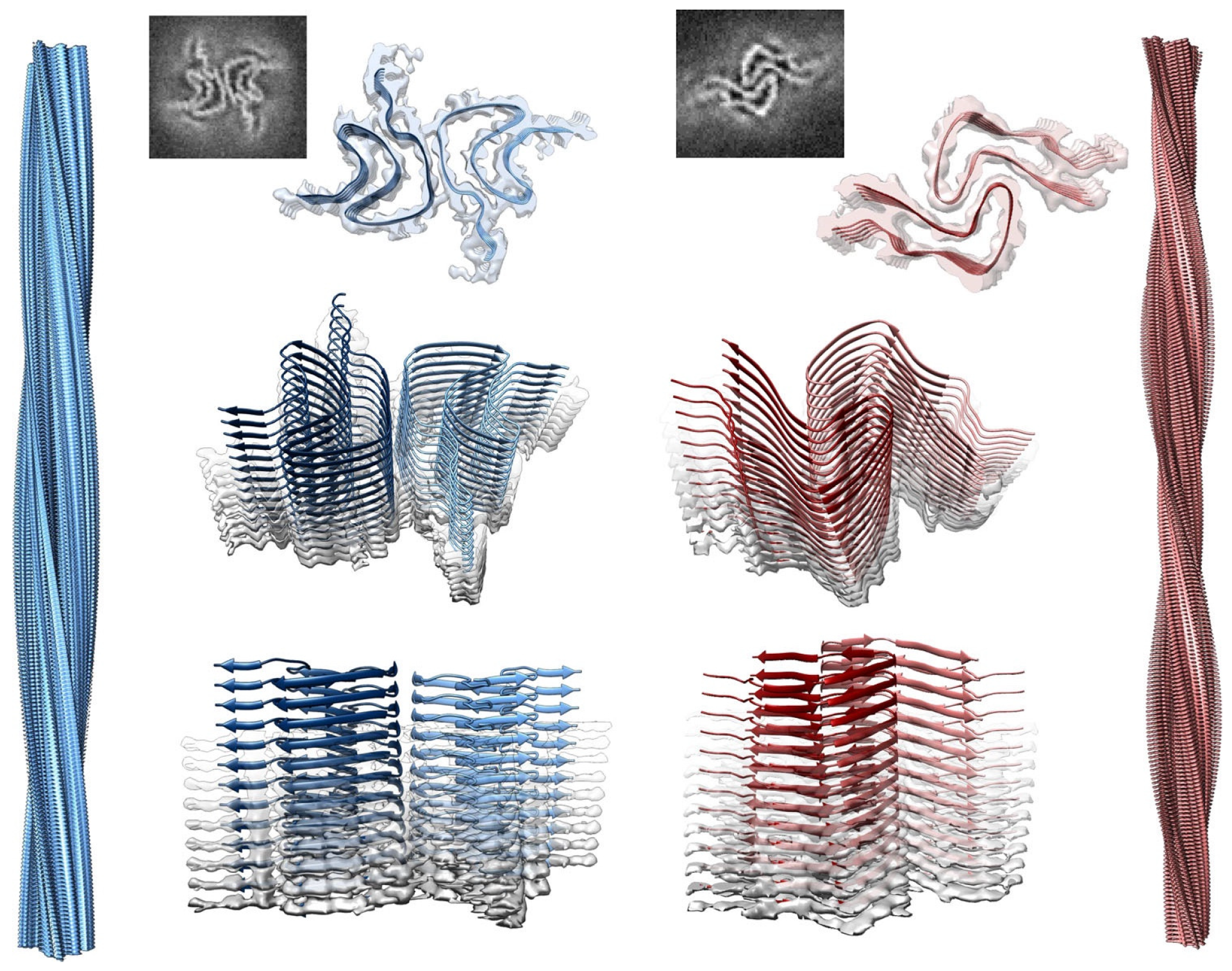
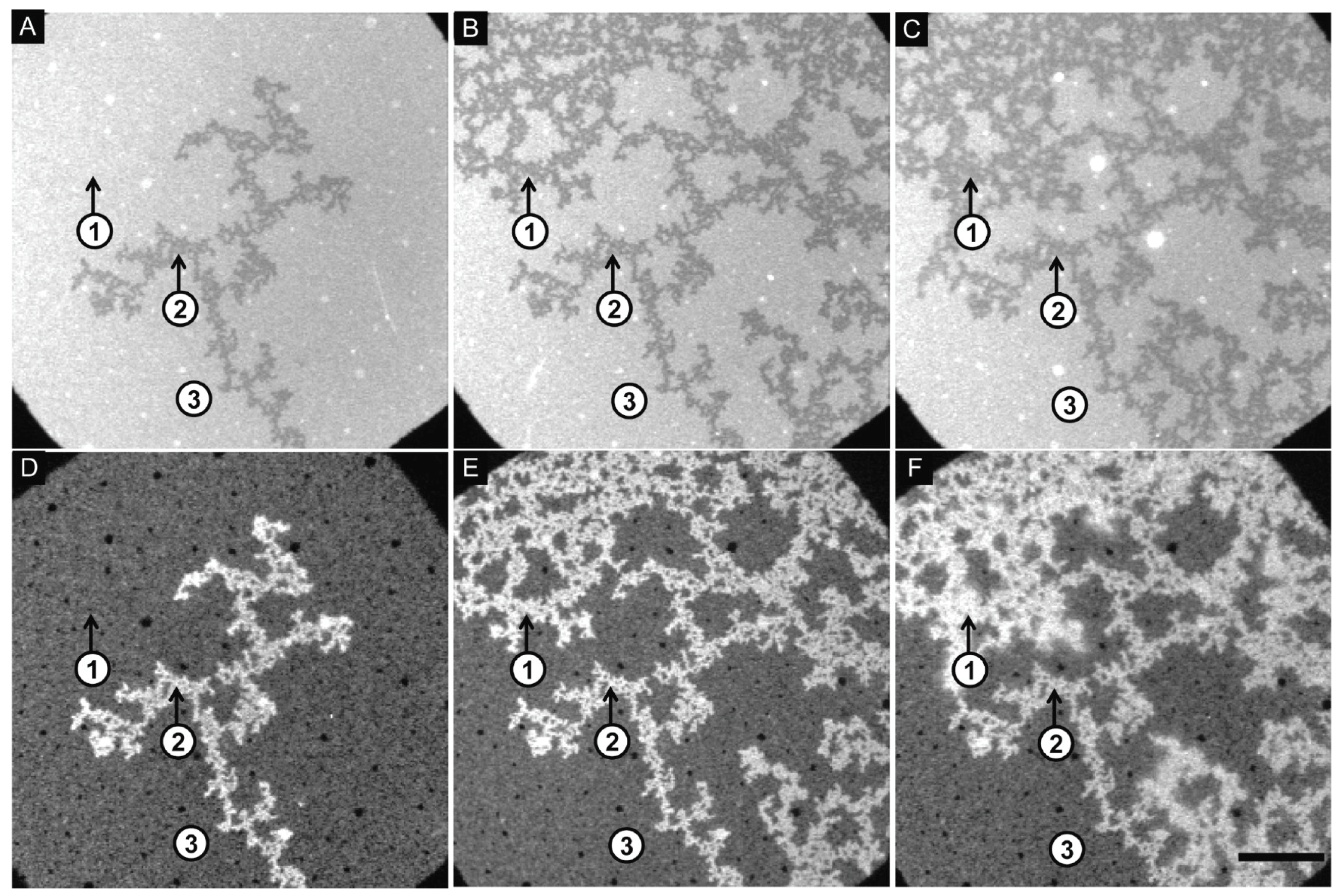
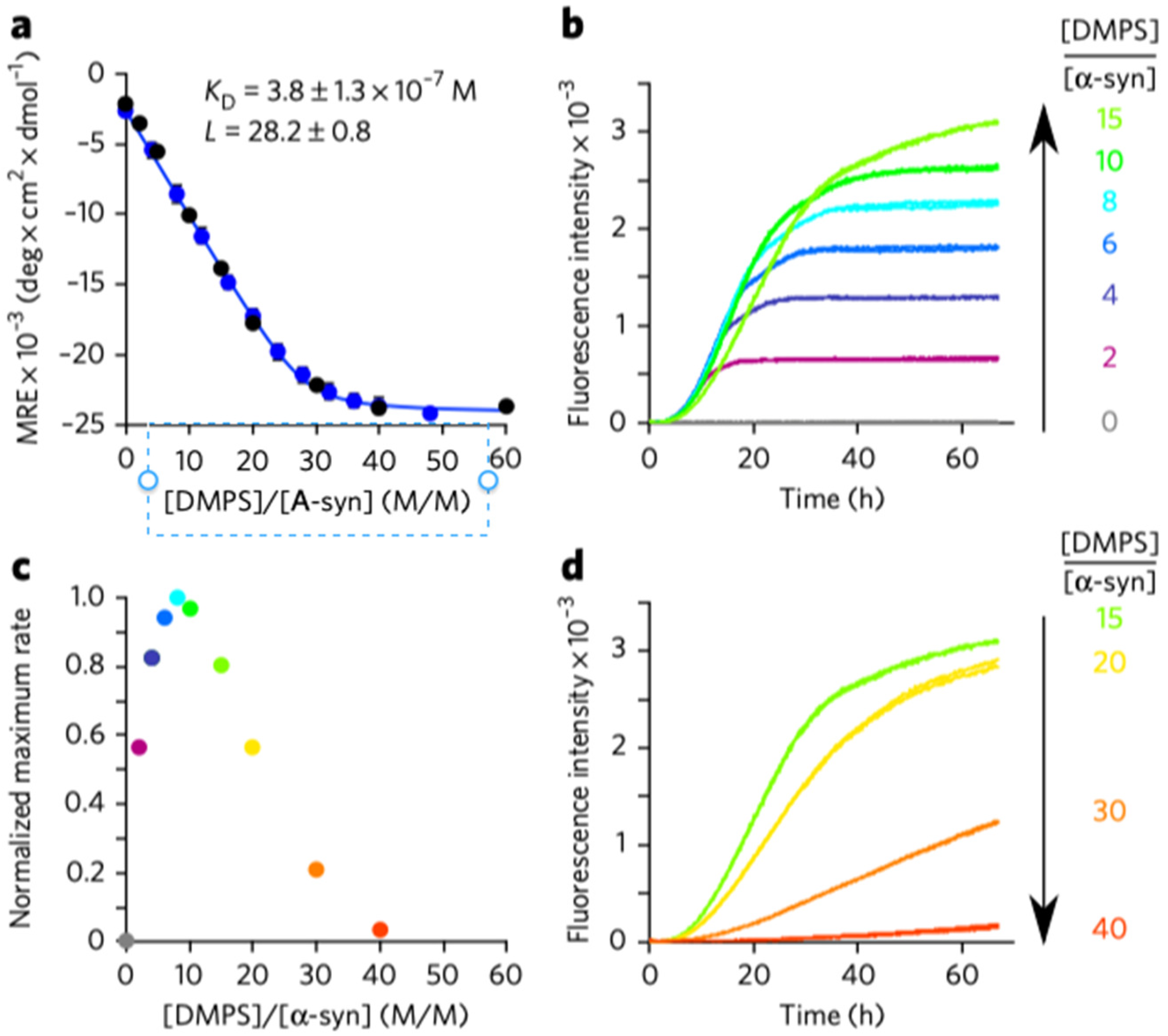

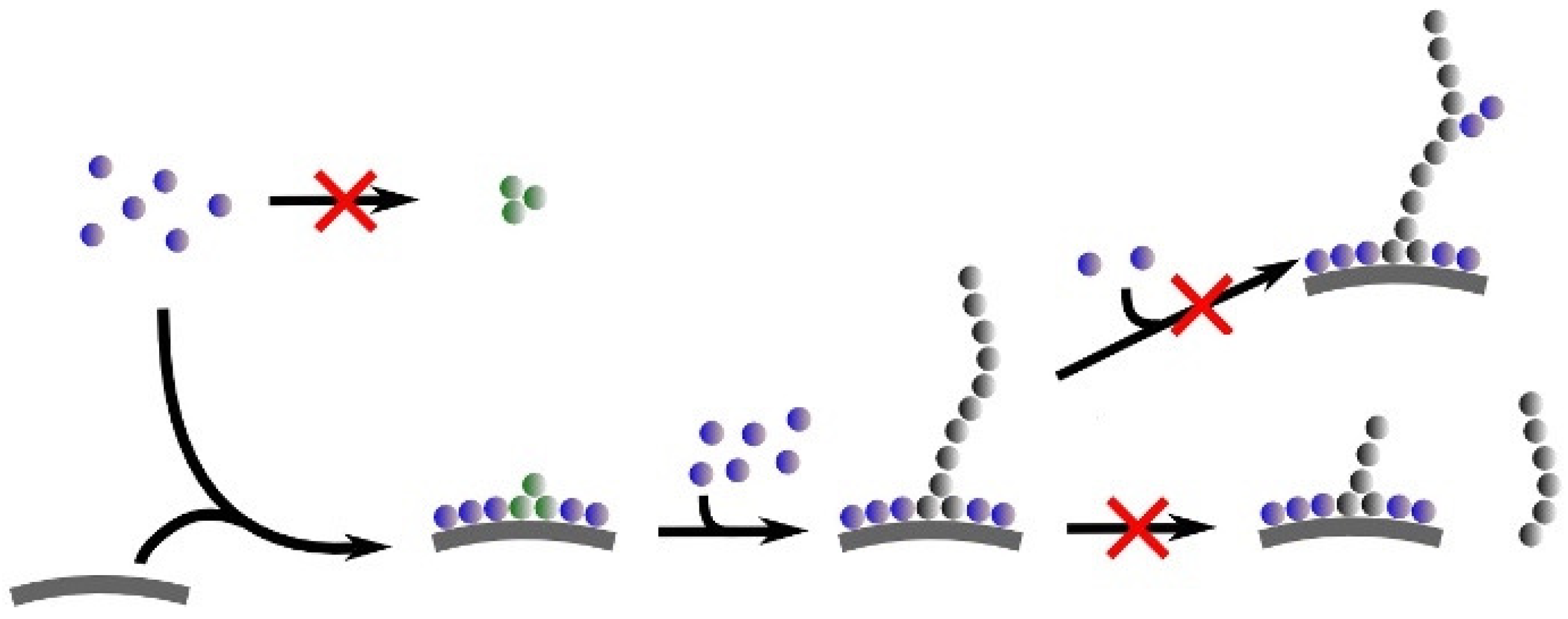
| Variant | Kd,app ([Cl]/[cyt]) Soret Band CD | n | Kd,app ([Cl]/[cyt]) Fluorescence | n |
|---|---|---|---|---|
| Yeast wild type | 10.2 | 2.2 | 23.4 | 2.3 |
| Yeast K72A | 9.8 | 2.0 | 26.2 | 2.3 |
| Human wild type | 8.6 | 1.3 | 36.0 | 2.2 |
| Huan K72A | 8.4 | 1.1 | 40.5 | 1.9 |
| Membrane Curvature | Kd [Exposed Lipid/Protein] Soret Band CD | Kd [Exposed Lipid/Protein] Fluorescence |
|---|---|---|
| concave | 55 | 61 |
| convex | 10.2 | 23.4 |
| Vesicle | Binding to SUV [μg Protein/mg Lipid] | Binding to LUV [μg Protein/mg Lipid]) |
|---|---|---|
| 1:1 (w/w) PC/PA | 120 | 40 |
| PC only | 2 | >1 |
| Mechanisms and Processes | Cytochrome c | α-Synuclein |
|---|---|---|
| Structural changes | Partial population of a partially unfolded state, increasing towards higher lipid/protein ratios. | Disorder to order (helical) transitions of the N-terminal segment of the protein (residues 1–100). Specifics depend on the lipid-to-protein ratio. |
| Binding sites | Four different binding sites have been identified. The relative contributions depend on solution conditions (pH an ionic strength). | Only the N-terminal segment is involved in the binding to anionic surfaces. |
| Changes to the membrane | Conflicting results ranging from slight membrane penetration to induced membrane curvature and even pore formation. | A high degree of curvature facilitates protein binding to anionic surfaces. |
| Electrostatic contributions | Electrostatic interactions are involved in cytochrome c binding via its A- and L-sites, but their contribution depends on the pH of the solution. | Electrostatic interactions (with anionic lipids) significantly increase the binding affinity. |
| Hydrophobic contributions | Hydrophobic interactions and H-bonding has been proposed for C-site binding and the corresponding involvement of lipid insertion. It is unclear to what extent it happens solely for C-site binding, which was suggested to occur at acidic pH. | While electrostatic interactions dominate, N-terminal binding also encompasses hydrophobic binding owing to its amphiphilic character. The addition of NaCl causes only a partial inhibition of protein binding. |
| Protein aggregation | Involvement of protein aggregation is unclear. It might occur at low lipid-to-protein ratios. Whether or not domain-swapped dimers are formed remains to be determined. | Protein aggregation and fibril formation is facilitated at low lipid/protein ratios. In the presence of cytochrome c and H2O2, dityrosine formation might add a covalent component. |
| Functional changes | Cytochrome becomes a peroxidase. The balancing between electron transfer and peroxidase activity is still not fully understood. | The normal function of the protein is not entirely clear, nor is its modification by membrane binding. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Schweitzer-Stenner, R. Order-to-Disorder and Disorder-to-Order Transitions of Proteins upon Binding to Phospholipid Membranes: Common Ground and Dissimilarities. Biomolecules 2025, 15, 198. https://doi.org/10.3390/biom15020198
Schweitzer-Stenner R. Order-to-Disorder and Disorder-to-Order Transitions of Proteins upon Binding to Phospholipid Membranes: Common Ground and Dissimilarities. Biomolecules. 2025; 15(2):198. https://doi.org/10.3390/biom15020198
Chicago/Turabian StyleSchweitzer-Stenner, Reinhard. 2025. "Order-to-Disorder and Disorder-to-Order Transitions of Proteins upon Binding to Phospholipid Membranes: Common Ground and Dissimilarities" Biomolecules 15, no. 2: 198. https://doi.org/10.3390/biom15020198
APA StyleSchweitzer-Stenner, R. (2025). Order-to-Disorder and Disorder-to-Order Transitions of Proteins upon Binding to Phospholipid Membranes: Common Ground and Dissimilarities. Biomolecules, 15(2), 198. https://doi.org/10.3390/biom15020198






