Quantum Confinement Effects of Thin Co3O4 Films
Abstract
:1. Introduction
2. Experimental Details
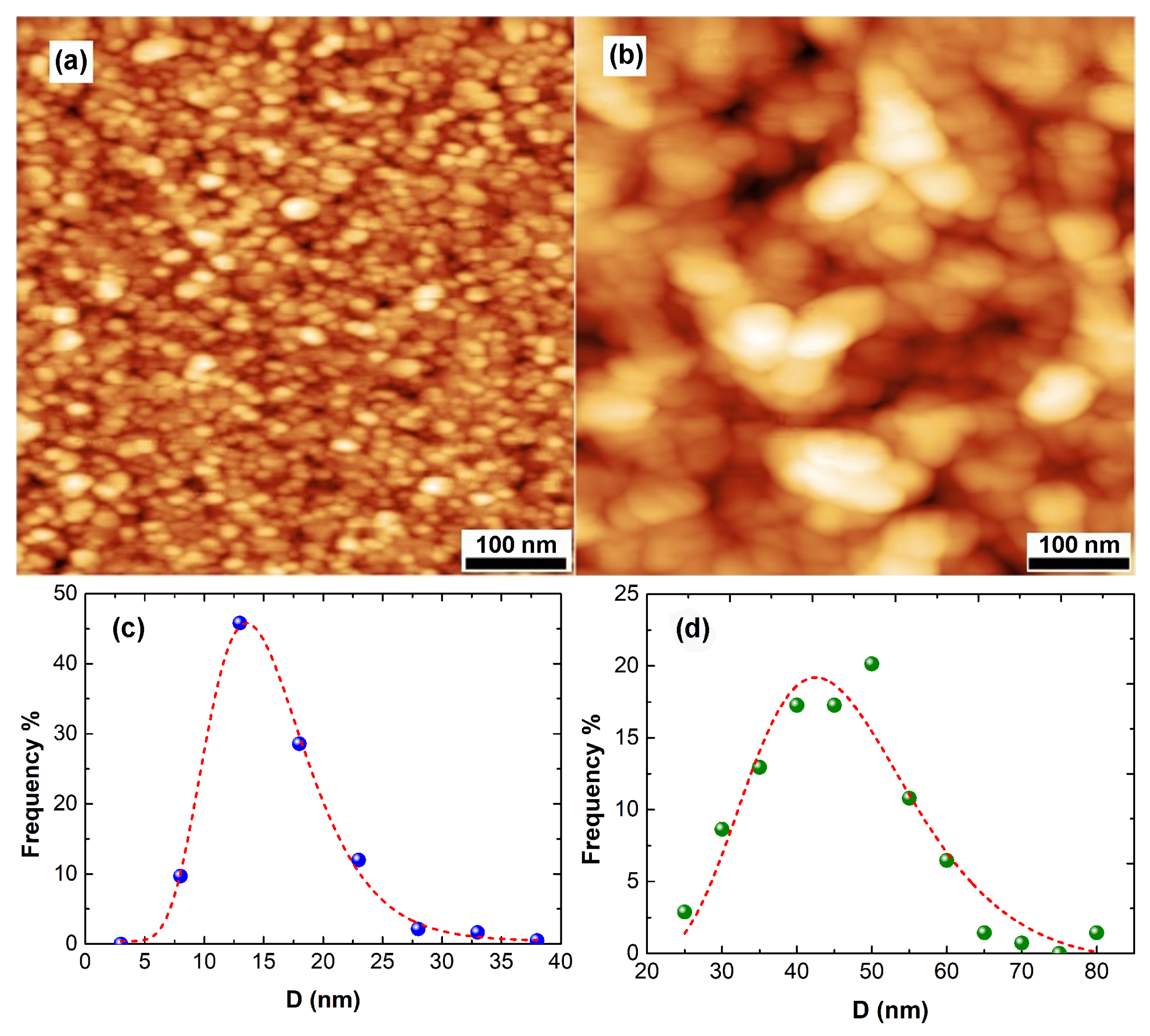
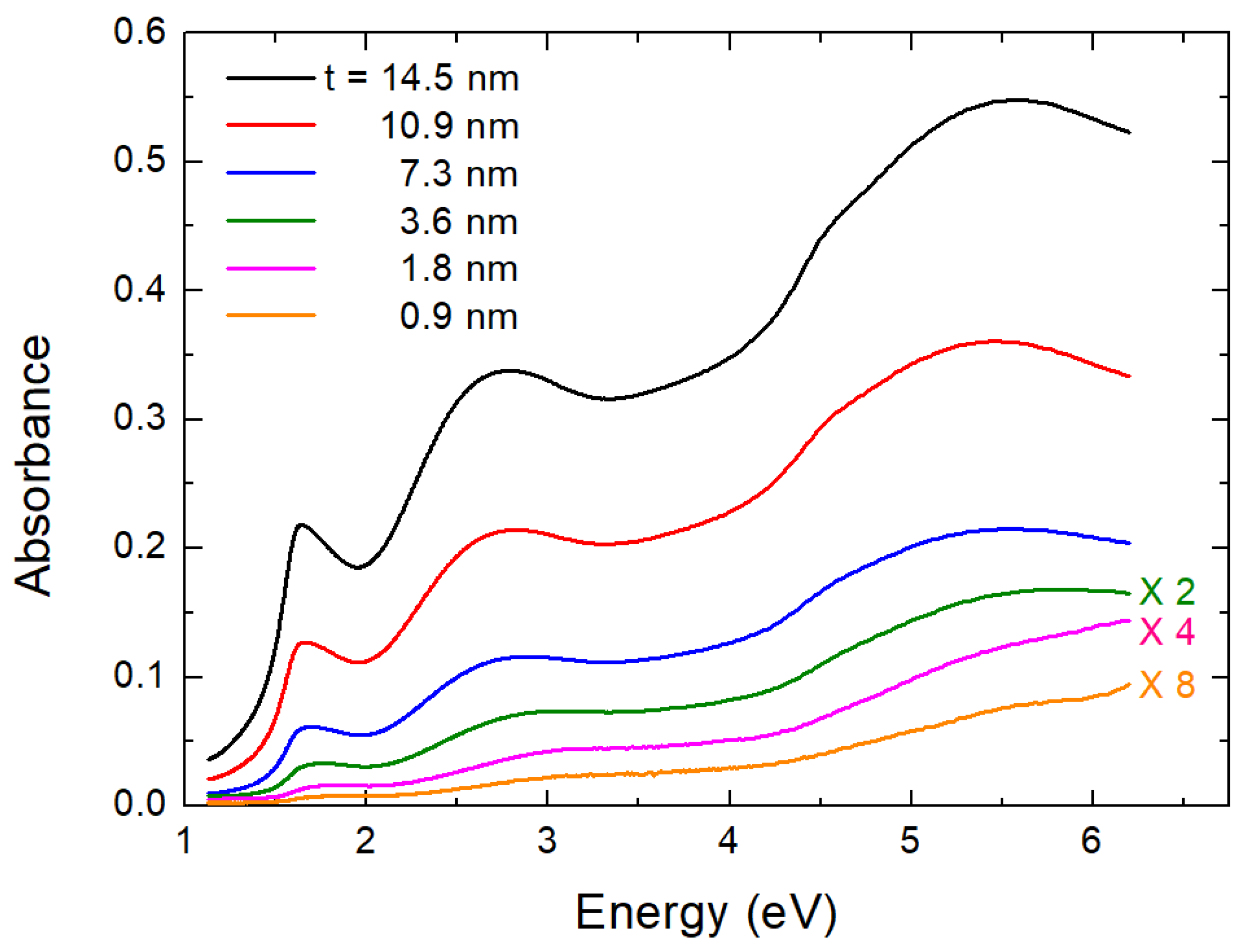
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Gou, W.; Zhou, X.; Li, J.; Ma, Y. Nanoporous Co3O4 plates as highly electroactive materials for electrochemical energy storage. Mater. Lett. 2016, 180, 207–211. [Google Scholar] [CrossRef]
- Feng, C.; Zhang, J.; Deng, Y.; Zhong, C.; Liu, L.; Hu, W. A review of electrolyte materials and compositions for electrochemical supercapacitors. Mater. Sci. Eng. B 2015, 199, 15–21. [Google Scholar] [CrossRef]
- Meng, T.; Xu, Q.; Wang, Z.; Li, Y.; Gao, Z.; Xing, X.; Ren, T. Co3O4 Nanorods with Self-assembled Nanoparticles in Queue for Supercapacitor. Electrochim. Acta 2015, 180, 104–111. [Google Scholar] [CrossRef]
- Li, Z.; Bui, P.; Kwak, D.; Akhtar, M.; Yang, O. Enhanced electrochemical activity of low temperature solution process synthesized Co3O4 nanoparticles for pseudo-supercapacitors applications. Ceram. Int. 2016, 42, 1879–1885. [Google Scholar] [CrossRef]
- Wang, Y.; Zhou, T.; Jiang, K.; Da, P.; Peng, Z.; Tang, J.; Kong, B.; Cai, W.; Yang, Z.; Zheng, G. Reduced Mesoporous Co3O4 Nanowires as Efficient Water Oxidation Electrocatalysts and Supercapacitor Electrodes. Adv. Energy Mater. 2014, 4, 1400692–1400696. [Google Scholar] [CrossRef]
- Geng, T.; Zhang, L.; Wang, H.; Zhang, K.; Zhou, X. Facile synthesis of porous Co3O4 nanoplates for supercapacitor applications. Bull. Mater. Sci. 2015, 38, 1171–1175. [Google Scholar] [CrossRef] [Green Version]
- Kandalkar, S.; Gunjakar, J.; Lokhande, C. Preparation of cobalt oxide thin films and its use in supercapacitor application. Appl. Surf. Sci. 2008, 254, 5540–5544. [Google Scholar] [CrossRef]
- Lokhande, C.D.; Dubal, D.P.; Joo, O.-S. Metal oxide thin film based supercapacitors. Curr. Appl. Phys. 2011, 11, 255–270. [Google Scholar] [CrossRef]
- Barreca, D.; Massignan, C.; Daolio, S.; Fabrizio, M.; Piccirillo, C.; Armelao, L.; Tondello, E. Composition and Microstructure of Cobalt Oxide Thin Films Obtained from a Novel Cobalt (II) Precursor by Chemical Vapor Deposition. Chem. Mater. 2001, 13, 588. [Google Scholar] [CrossRef]
- Švegl, F.; Orel, B.; Hutchins, M.G.; Kalcher, K. Spectroelectrochemical Investigations of Sol-Gel Derived Electrochromic Spinel Co3O4 Films. J. Electrochem. Soc. 1996, 143, 1532. [Google Scholar] [CrossRef]
- Barnasas, A.; Kanistras, N.; Ntagkas, A.; Anyfantis, D.I.; Stamatelatos, A.; Kapaklis, V.; Bouropoulos, N.; Mystiridou, E.; Poulopoulos, P.; Garoufalis, C.S. Quantum confinement effects of thin ZnO films by experiment and theory. Phys. E Low-Dimens. Syst. Nanostruct. 2020, 120, 114072. [Google Scholar] [CrossRef]
- Petitto, S.C.; Langell, M.A. Surface composition and structure of Co3O4 (110) and the effect of impurity segregation. J. Vac. Sci. Technol. A 2004, 22, 1690. [Google Scholar] [CrossRef] [Green Version]
- Vaz, C.A.F.; Wang, H.-Q.; Ahn, C.H.; Henrich, V.E.; Baykara, M.Z.; Schwendemann, T.C.; Pilet, N.; Albers, B.J.; Schwarz, U.D.; Zhang, L.H.; et al. Interface and electronic characterization of thin epitaxial films. Surf. Sci. 2009, 603, 291–297. [Google Scholar] [CrossRef] [Green Version]
- Cox, V.P.A. Transition Metal Oxides an Introduction to Their Electronic Structure and Properties; Oxford University Press: Oxford, UK, 1992; Volume 284. [Google Scholar]
- Lohaus, C.; Morasch, J.; Brötz, J.; Klein, A.; Jaegermann, W. Investigations on RF-magnetron sputtered Co3O4 thin films regarding the solar energy conversion properties. J. Phys. D Appl. Phys. 2016, 49, 155306. [Google Scholar] [CrossRef]
- Angelov, S.; Zhecheva, E.; Stoyanova, R.; Atanasov, M. Bulk Defects in CoO Pure and Slightly Doped with Lithium, Revealed by EPR of the Tetrahedral Co+ Ions. J. Phys. Ckm. Sd 1990, 51, 1157–1161. [Google Scholar]
- Kupfer, B.; Majhi, K.; Keller, D.A.; Bouhadana, Y.; Rühle, S.; Barad, H.N.; Anderson, A.Y.; Zaban, A. Thin Film Co3O4 /TiO2 Heterojunction Solar Cells. Adv. Energy Mater. 2015, 5, 11401007. [Google Scholar]
- Nam, H.-J.; Sasaki, T.; Koshizaki, N. Optical CO Gas Sensor Using a Cobalt Oxide Thin Film Prepared by Pulsed Laser Deposition under Various Argon Pressures. J. Phys. Chem. B 2006, 110, 23081. [Google Scholar] [CrossRef]
- Han, K.; Song, S.W.; Yoshimura, M. Room-Temperature Fabrication of Lithium Cobalt Oxide Thin-Film Electrodes by Lithium Hydroxide Solution Treatment. J. Am. Ceram. 2005, 81, 2465–2468. [Google Scholar] [CrossRef]
- Huang, B.; Cao, K.; Liu, X.; Qian, L.; Shan, B.; Chen, R. Tuning the morphology and composition of ultrathin cobalt oxide films via atomic layer deposition. RSC Adv. 2015, 5, 71816–71823. [Google Scholar] [CrossRef]
- Vaz, C.A.F.; Prabhakaran, D.; Altman, E.I.; Henrich, V.E. Experimental study of the interfacial cobalt oxide in Co3O4/a-Al2O3 (0001) epitaxial films. Phys. Rev. B-Condens. Matter Mater. Phys. 2009, 80, 1–7. [Google Scholar] [CrossRef] [Green Version]
- Baskoutas, S.; Poulopoulos, P.; Karoutsos, V.; Angelakeris, M.; Flevaris, N.K. Strong quantum confinement effects in thin zinc selenide films. Chem. Phys. Lett. 2006, 417, 461–464. [Google Scholar] [CrossRef]
- Rieth, M.; Schommers, W.; Baskoutas, S. Exact numerical solution of Schrodinger’s equation for a particle in an interaction potential of general shape. Int. J. Mod. Phys. B 1992, 16, 4081. [Google Scholar] [CrossRef]
- Baskoutas, S.; Terzis, A.F. Size-dependent band gap of colloidal quantum dots. J. Appl. Phys. 2006, 99, 013708. [Google Scholar]
- Baskoutas, S.; Terzis, A.F.; Schommers, W. Size-dependent exciton energy of narrow band gap colloidal quantum dots in the finite depth square-well effective mass approximation. J. Comp. Theor. Nanosci. 2006, 3, 269. [Google Scholar] [CrossRef]
- Karoutsos, V. Scanning probe microscopy: Instrumentation and applications on thin films and magnetic multilayers. J. Nanosci. Nanotechnol. 2009, 9, 6783–6798. [Google Scholar]
- Garoufalis, C.S.; Poulopoulos, P.; Bouropoulos, N.; Barnasas, A.; Baskoutas, S. Growth and optical properties of Fe2O3 thin films: A study of quantum confinement effects by experiment and theory. Phys. E Low-Dimens. Syst. Nanostruct. 2017, 89, 67. [Google Scholar] [CrossRef]
- JCPDS Card No. 211276. unpublished.
- Escobar, M.A.; Magana, L.F.; Valenzuela, R. Effect of the grain size distribution on the magnetization curve. J. Appl. Phys. 1985, 57, 2142–2147. [Google Scholar] [CrossRef]
- Martens, J.; Peeters, W.; Noort, H.V.; Erman, M. Optical, magneto-optical and mössbauer spectroscopy on Co3+ substituted cobalt ferrite Co2+ Fe2− xCo3+ xO4 (0 ≤ x ≤ 2). J. Phys. Chem. Solids 1985, 46, 411. [Google Scholar] [CrossRef]
- Yamamoto, H.; Tanaka, S.; Hirao, K. Effects of substrate temperature on nanostructure and band structure of Co3O4 sputtered thin films. J. Appl. Phys. 2003, 93, 4158. [Google Scholar] [CrossRef] [Green Version]
- Jiang, C.-M.; Baker, L.R.; Lucas, J.M.; Vura-Weis, J.; Alivisatos, A.P.; Leone, S.R. Characterization of Photo-Induced Charge Transfer and Hot Carrier Relaxation Pathways in Spinel Cobalt Oxide (Co3O4). J. Phys. Chem. C 2014, 118, 22774. [Google Scholar] [CrossRef] [Green Version]
- Waegele, M.M.; Doan, H.Q.; Cuk, T. Long-Lived Photoexcited Carrier Dynamics of d–d Excitations in Spinel Ordered, Co3O4. J. Phys. Chem. C 2014, 118, 3426. [Google Scholar] [CrossRef]
- Wheeler, D.A.; Wang, G.; Ling, Y.; Li, Y.; Zhang, J.Z. Nanostructured hematite: Synthesis, characterization, charge carrierdynamics, and photoelectrochemical properties. Energy Environ. Sci. 2012, 5, 6682. [Google Scholar] [CrossRef]
- Smart, T.J.; Pham, T.A.; Ping, Y.; Ogitsu, T. Optical absorption induced by small polaron formation in transition metal oxides: The case of Co3O4. Phys. Rev. Mater. 2019, 3, 102401(R). [Google Scholar] [CrossRef] [Green Version]
- Fox, M. Optical Properties of Solids, 2nd ed.; Oxford University Press: New York, NY, USA, 2010; ISBN 9780199573370. [Google Scholar]
- Garoufalis, C.S.; Barnasas, A.; Stamatelatos, A.; Karoutsos, V.; Grammatikopoulos, S.; Poulopoulos, P.; Baskoutas, S. A Study of Quantum Confinement Effects in Ultrathin NiO Films Performed by Experiment and Theory. Materials 2018, 11, 949. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Qiao, L.; Xiao, H.Y.; Meyer, H.; Sun, J.; Rouleau, C.M.; Puretzky, A.A.; Geohegan, D.B.; Ivanov, I.N.; Yoon, M.; Weber, W.J.; et al. Nature of the band gap and origin of the electro-/photo-activity of Co3O4. J. Mater. Chem. C 2013, 1, 4628. [Google Scholar] [CrossRef]
- Poulopoulos, P.; Wilhelm, F.; Wende, H.; Baberschke, K.; Benea, D.; Ebert, H.; Angelakeris, M.; Flevaris, N.K.; Rogalev, A.; Brookes, N.B. X-ray magnetic circular dichroic magnetometry on Ni/Pt multilayers. J. Appl. Phys. 2001, 89, 3874. [Google Scholar] [CrossRef]
- Blakemore, J.D.; Gray, H.B.; Winkler, J.R.; Müller, A.M. Co3O4 Nanoparticle Water-Oxidation Catalysts Made by Pulsed-Laser Ablation in Liquids. ACS Catal. 2013, 3, 2497–2500. [Google Scholar] [CrossRef]
- Lany, S. Semiconducting transition metal oxides. J. Phys. Condens. Matter 2015, 27, 283203. [Google Scholar] [CrossRef] [PubMed]
- Hanken, H. Kopplung nichtrelativistischer teilchen mit einem quantisierten feld. Nuovo Cimento 1956, 3, 1230. [Google Scholar] [CrossRef]
- Prabaharan, D.D.M.; Sadaiyandi, K.; Mahendran, M.; Sagadevan, S. Precipitation method and characterization of cobalt oxide nanoparticles. Appl. Phys. A 2017, 123, 264. [Google Scholar] [CrossRef]
- Li, Y. Investigation of Lattice Vibrations and Band Gaps of Cobalt Oxides by Fourier Transform Infrared, Raman Scattering and Photoluminescence Spectroscopy. Ph.D. Thesis, Department of Electrical Engineering, University of Houston, Houston, TX, USA, 2015. [Google Scholar]

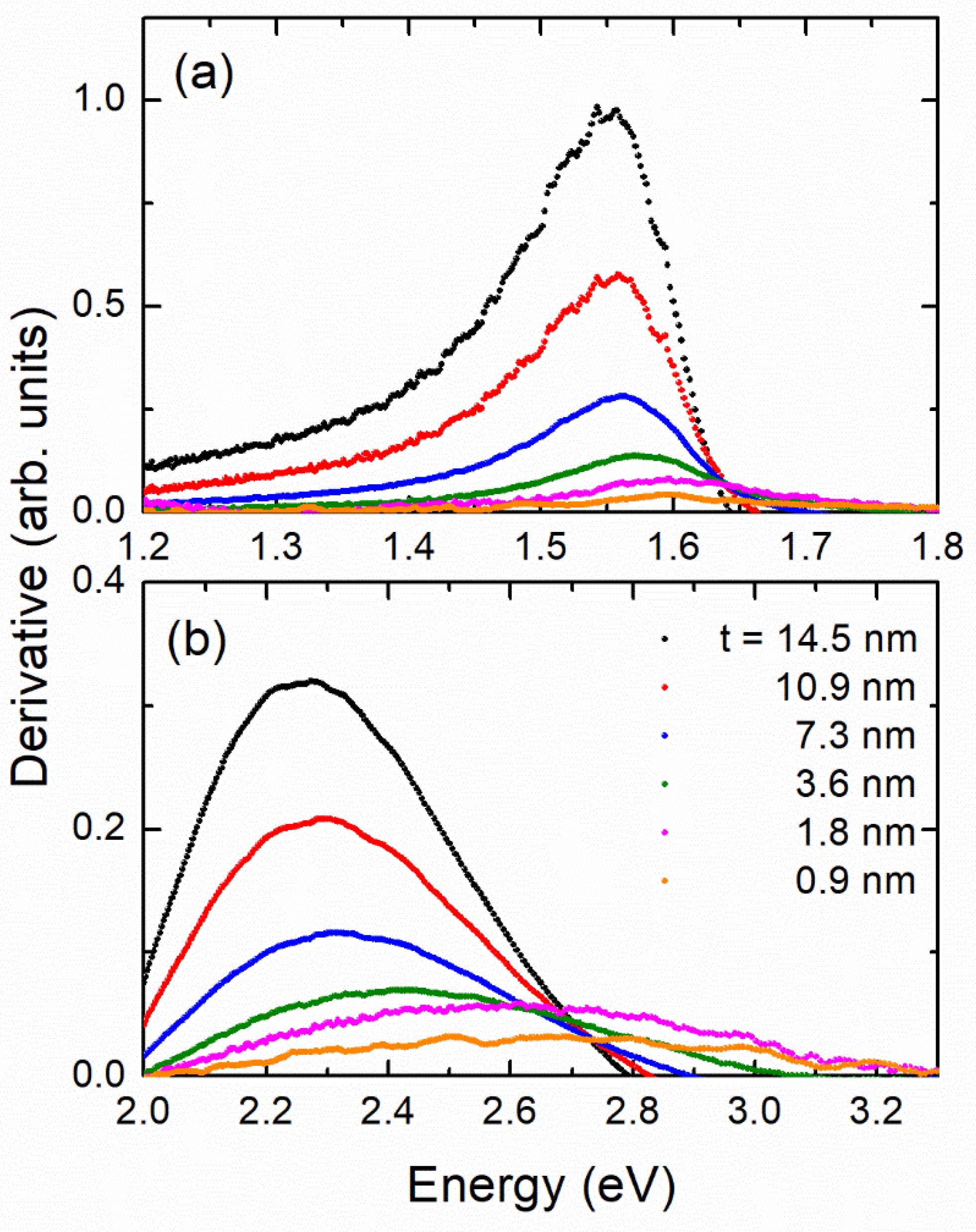

| t (Thickness) (nm) | (eV) | (eV) | t (nm) | (eV) |
|---|---|---|---|---|
| 14.5 | 1.55 | 2.27 | 14.0 | 1.55 |
| 10.9 | 1.56 | 2.29 | 10.0 | 1.55 |
| 7.3 | 1.56 | 2.32 | 8.0 | 1.55 |
| 3.6 | 1.58 | 2.43 | 5.0 | 1.56 |
| 1.8 | 1.60 | 2.60 | 3.0 | 1.58 |
| 0.9 | 1.61 | 2.69 | 2.0 | 1.60 |
| – | – | – | 1.5 | 1.64 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barnasas, A.; Garoufalis, C.S.; Anyfantis, D.I.; Bouropoulos, N.; Poulopoulos, P.; Hayrapetyan, D.B.; Baskoutas, S. Quantum Confinement Effects of Thin Co3O4 Films. Atoms 2021, 9, 70. https://doi.org/10.3390/atoms9040070
Barnasas A, Garoufalis CS, Anyfantis DI, Bouropoulos N, Poulopoulos P, Hayrapetyan DB, Baskoutas S. Quantum Confinement Effects of Thin Co3O4 Films. Atoms. 2021; 9(4):70. https://doi.org/10.3390/atoms9040070
Chicago/Turabian StyleBarnasas, Alexandros, Christos S. Garoufalis, Dimitrios I. Anyfantis, Nikolaos Bouropoulos, Panagiotis Poulopoulos, David B. Hayrapetyan, and Sotirios Baskoutas. 2021. "Quantum Confinement Effects of Thin Co3O4 Films" Atoms 9, no. 4: 70. https://doi.org/10.3390/atoms9040070
APA StyleBarnasas, A., Garoufalis, C. S., Anyfantis, D. I., Bouropoulos, N., Poulopoulos, P., Hayrapetyan, D. B., & Baskoutas, S. (2021). Quantum Confinement Effects of Thin Co3O4 Films. Atoms, 9(4), 70. https://doi.org/10.3390/atoms9040070












