A Note on the Opacity of the Sun’s Atmosphere

Abstract

The opacity of the atmosphere of the Sun is due to processes such as Thomson scattering, bound–bound transitions and photodetachment (bound–free) of hydrogen and positronium ions. The well-studied free–free transitions involving photons, electrons, and hydrogen atoms are re-examined, while free–free transitions involving positrons are considered for the first time. Cross sections, averaged over a Maxwellian velocity distribution, involving positrons are comparable to those involving electrons. This indicates that positrons do contribute to the opacity of the atmosphere of the Sun. Accurate results are obtained because definitive phase shifts are known for electron–hydrogen and positron–hydrogen scattering.

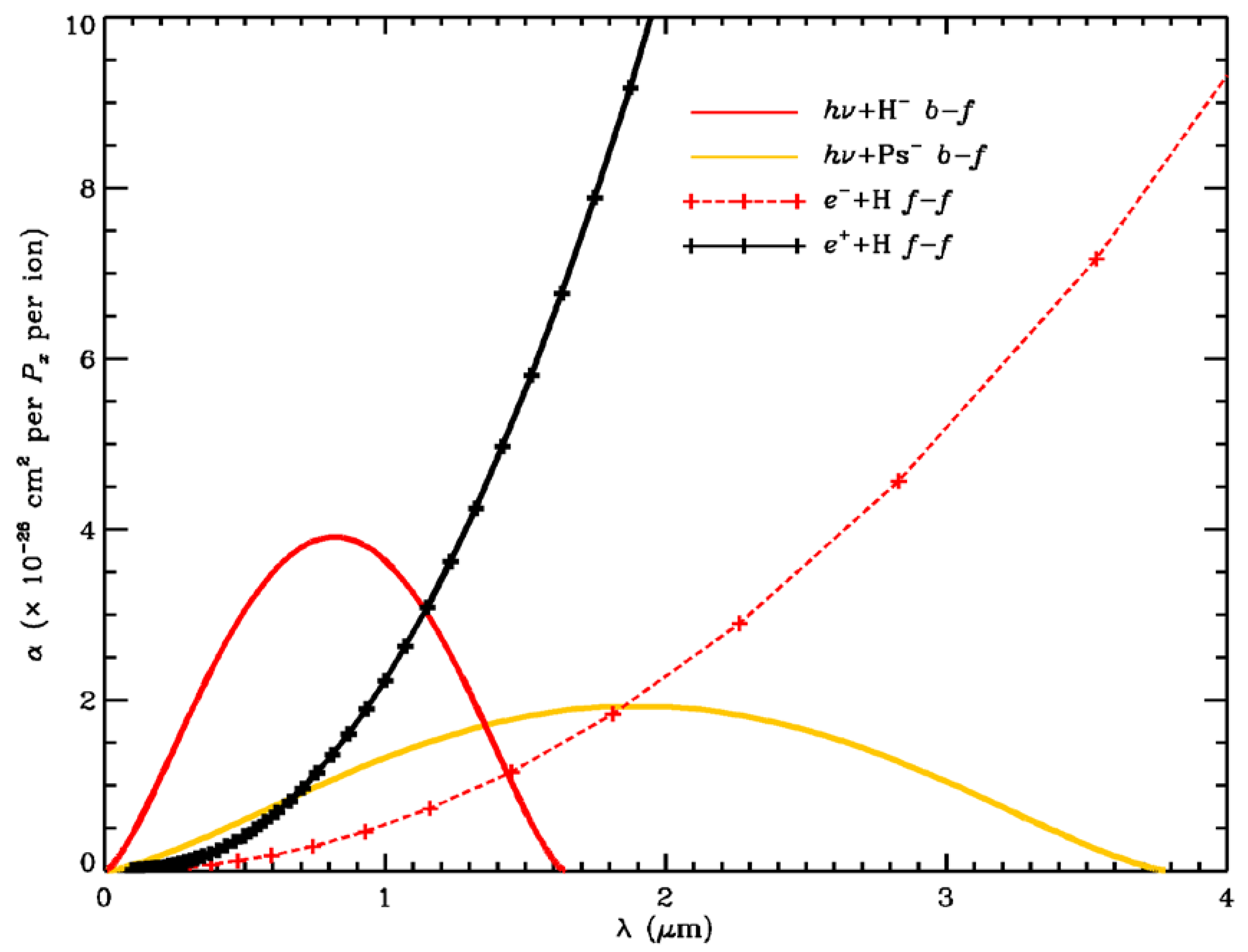
1. Introduction

The variation of the solar spectral irradiance with wavelength shows the effects of bound–bound, bound–free, and free–free opacity of many elements in the solar atmosphere. In 1923, using a classical approach, Kramers [1] showed that the free–free absorption coefficient is given by

$$k_{\nu }=Z^2\rho T^{-1/2}{\nu }^{-3}$$

(1)

The solar medium is opaque between 4000 and 25000 Å because of various processes, such as Thomson scattering, bound–bound transitions, photodetachment (bound–free) or free–free transitions. In 1939, Wildt [2] suggested that an important source of opacity in the solar atmosphere could be due to the photodetachment of negative hydrogen ions:

$$h\nu +H^{-}\to e+H$$

(2)

The bound–free transitions explain the opaqueness of the Sun’s atmosphere between 4000 and 16,000 Å; beyond this range, free–free transitions account for the continuous spectrum of the Sun

$$h\nu +e^{-}+H\to e^{-}+H$$

(3)

If an electron with energy $k_0^2$ absorbs photon energy and the final energy of the electron in the continuum is $k_1^2$, the change in energy is $\Delta k^2=|k_0^2-k_1^2|$. These transitions also explain the opacity of late-type stellar atmospheres. Cross sections for bound–free and free–free transitions have been calculated by Chandrasekhar and Elbert [3] and Chandrasekhar and Breen [4], respectively. The cross section for free–free transition, given by the latter [4] is

$$\sigma (k_0^2,\Delta k^2)=\frac{256{\pi }^2}{3}(\frac{2\pi e^2}{hc}){(\frac{h^2}{4{\pi }^{2}m{e}^2})}^5\frac{1}{k_0^2{k}_1{(\Delta k^2)}^3}M(k_0,k_1){\mathrm{cm}}^2$$

(4)

where various quantities have the usual meaning and

$$M(k,k_1)=|M(0,k^2|1,k_1{}^2){|}^2+|M(0,k_1{}^2|1,k^2){|}^2$$

(5)

$$M(0,k^2|1,k_1^2)=\frac{k_1^4}{16}(3{\sin }^2{\delta }_k^{-}+{\sin }^2{\delta }_k^{+})$$

(6)

The phase shifts ${\delta }_k^{-}$ and ${\delta }_k^{+}$ are the triplet and singlet phase shifts for the scattering of an electron from a hydrogen atom with momentum k. They were calculated using hybrid theory [5]. The present electron–hydrogen phase shifts are much more accurate compared to those used in earlier calculations for calculating cross sections for free–free transitions. The hybrid theory takes into account exchange, short-range correlations, and long-range correlations, at the same time. There are a number of earlier calculations. For example, the calculations in ref. [6] include only long-range correlations, while in ref. [7], only short-range correlations could be considered.

It has been known that there are positrons present in the Sun [8] and in interstellar space, as indicated by the detection of the 0.511 MeV line from the center of the galaxy due to the annihilation of the positron and electron pairs [9,10]. Positrons are produced due to various processes: when two protons collide, during the formation of ³He nuclei, the decay of radioactive nuclei, and the decay of positive pions to muons, which further decay into positrons [11]. Positrons produced by solar flares can reach the solar atmosphere and modulate the radiant flux passing through them during their lifetime.

Once positrons are available, the photodetachment of negative positronium ions

hν + Ps⁻ → e⁻ + Ps

(7)

and free–free transitions of positrons on H are possible:

$$h\nu +e^{+}+H\to e^{+}+H$$

(8)

For the latter, there is only one phase shift for a positron with an incident momentum k and Equation (6) takes the form

$$M(0,k^2|1,k_1^2)=\frac{k_1^4{\sin }^2({\delta }_k)}{4}$$

(9)

The positron–hydrogen phase shifts (δ_k) were calculated using hybrid theory [12]. An earlier calculation of reference [13] included only short-range correlations.

The cross section for photodetachment of H⁻ is given by Ohmura and Ohmura [14] as

$$\sigma (H^{-})=\frac{6.8475\times {10}^{-18}\gamma k^3}{(1-\gamma \rho ){({\gamma }^2+k^2)}^3}{\mathrm{cm}}^2$$

(10)

where $\gamma =0.2365833$ and $\rho =2.646$.

Photodetachment cross sections of negative positronium ions [15] were calculated using Ps⁻ wave functions of the form used by Ohmura and Ohmura [14] for the negative hydrogen ion. This cross section is written in the form

$$\sigma (P{s}^{-})=\frac{1.32\times {10}^{-18}{k}^3}{{({\gamma }^2+k^2)}^3}{\mathrm{cm}}^2$$

(11)

where $\gamma =0.12651775$, and because $1.5{\gamma }^2$ is the binding energy, this gives a value of 0.024010 Ry [15]. In the above equations, k is the momentum of the outgoing electron. A measurement of this cross section was reported in [16].

We list the cross sections for bound–free and free–free transitions for electrons and positrons in

Table 1. Comparison of bound–free (σ_bf) and free–free (σ_ff) cross sections (cm²) for electrons and positrons, T = 6300 K.

			Electrons			Positrons
Δk2	λ (Å)	σbf *	σff	σbf + σff	σbf	σff	σbf + σff
0.26	3505	2.29 (−17)	4.28 (−20)	2.29 (−17)	9.95 (−18)	4.14 (−21)	9.95 (−18)
0.12	7594	4.15 (−17)	1.88 (−19)	4.17 (−17)	3.17 (−17)	1.56 (−20)	3.17 (−17)
0.10	9113	4.13 (−17)	2.69 (−19)	4.16 (−17)	4.17 (−17)	2.15 (−20)	4.17 (−17)
0.06	15,188	7.05 (−18)	7.45 (−19)	7.80 (−18)	8.96 (−17)	5.38 (−20)	8.97 (−17)
0.04	22,783	0.00	1.68 (−18)	1.68 (−18)	1.65 (−16)	1.13 (−19)	1.65 (−16)
0.03	30,377	0.00	2.99 (−18)	2.99 (−18)	2.53 (−16)	1.96 (−19)	2.53 (−16)
0.02	45,565	0.00	6.74 (−18)	6.74 (−18)	4.64 (−16)	4.30 (−19)	4.64 (−16)
0.01	91,130	0.00	2.70 (−17)	2.70 (−17)	1.30 (−15)	1.68 (−18)	1.30 (−15)
0.005	182,260	0.00	1.08 (−16)	1.08 (−16)	3.63 (−15)	6.72 (−18)	3.64 (−15)
0.003	303,767	0.00	3.00 (−16)	3.00 (−16)	7.69 (−15)	1.87 (−17)	7.71 (−15)
0.001	911,300	0.00	2.70 (−15)	2.70 (−15)	3.55 (−14)	1.68 (−16)	3.57 (−14)

* The number in parentheses indicates the power of ten multiplying that entry.

(and show them in Figure 1), where we have assumed a temperature of 6300 K and used the H⁻/H ratio given by Wheeler and Wildt [17]. This temperature has been used for many years as representative of a typical cool star, including the Sun at an optical depth of approximately 1. We see that the contribution of positrons cannot be neglected in calculations of the opacity of the Sun’s atmosphere.

Our results are shown in Figure 1. An examination of Figure 1 shows several effects. The most noticeable effect of positrons on the emergent spectrum would be an increase in the brightness temperature of wavelengths longer than 1 μm. The positron–H free–free opacity is larger than the electron–H free–free opacity at those wavelengths. The second effect is when the Ps abundance becomes appreciable and a broad region of opacity appears between 0.1 and 4 μm. A third effect, positron–Ps free–free transitions, will be described in a future work.

2. Conclusions

In addition to bound–free transitions, free–free transitions are important in the solar as well as stellar atmospheres. We have calculated cross sections for these processes and have shown that the free–free transitions involving electrons dominate at wavelengths longer than 16,000 Å. The same processes are present when positrons are involved in transitions instead of electrons. Two observable quantities, the locations of the maximum in the bound–free opacity and the transition from dominance of bound–free to free–free opacity, are both located at longer wavelengths when positrons and the negative positronium ions are considered. The presence of these shifted features would be a unique marker of an object with a measurable number of positrons in its atmosphere. Processes involving positrons cannot be neglected and their contribution to opacity could be comparable to those involving electrons.