Automodel Solutions of Biberman-Holstein Equation for Stark Broadening of Spectral Lines

Abstract

The accuracy of approximate automodel solutions for the Green’s function of the Biberman-Holstein equation for the Stark broadening of spectral lines is analyzed using the distributed computing. The high accuracy of automodel solutions in a wide range of parameters of the problem is shown.

1. Introduction

The Stark broadening of spectral lines is known to produce the long-tailed spectral line shapes of atom and ion radiation in plasmas (see, e.g., [1,2,3,4,5,6,7,8]). In the broad range of conditions in plasmas and gases, where the complete redistribution (CRD) in photon frequency within the resonance line shape is applicable, the radiative transfer is described by the Biberman-Holstein equation for the density of excited atoms [9,10] and characterized by the infinite mean-squared displacement of the initial perturbation [11] and, respectively, by the irreducibility of the integral equation, in space variables, to a differential one. This makes the respective radiative transfer a nonlocal (superdiffusive) one [12,13] (for the deviation from the CRD and the limits of its applicability see, e.g., [14,15,16]).

The latter makes the numerical simulation of radiative transfer in resonance spectral lines a formidable task. The simple models based on the domination of the long-free-path flights of the photons were suggested [17] and developed for the quasi-steady-state transport problem, now known as the escape probability methods (see [18,19,20]).

For the time-dependent superdiffusive transport, recently, a wide class of non-steady-state superdiffusive transport on a uniform background with a power-law decay, at large distances, of the step-length probability distribution function (PDF) was shown [21] to possess an approximate automodel solution. The solution for the Green’s function was constructed using the scaling laws for the propagation front (i.e., time dependence of the relevant-to-superdiffusion average displacement of the carrier) and asymptotic solutions far beyond and far ahead the propagation front. These scaling laws were shown to be determined essentially by the long free-path carriers (Lévy flights [22,23,24,25,26]). The validity of the suggested automodel solution was proved by its comparison with numerical solutions in the one-dimensional (1D) case of the transport equation with a simple long-tailed PDF with various power-law exponents and in the case of the Biberman-Holstein equation of the 3D resonance radiative transfer for various (Doppler, Lorentz, Voigt, and Holtsmark) spectral line shapes. The analysis of the limits of applicability of the automodel solution in the above-mentioned cases was continued in [27]. The full-scale numerical analysis of the limits of applicability was done in [28], using the massive computations of the exact solution of the transport equation with a simple long-tailed PDF with various power-law exponents. The comparison of these results with automodel solutions has shown high accuracy of automodel solutions in a wide range of space-time variables and enabled us to identify the limits of applicability of the automodel solutions.

It is important to note that the success of automodel solutions [21] for a wide class of non-stationary superdiffusive transport was achieved due to identification of the scaling laws in the case of radiative transfer in the Biberman-Holstein model in [11,12,13,17,21,29,30,31,32,33].

The Stark broadening of spectral lines is, itself, a highly complicated problem (see, e.g., [34] for the efforts, based on the quantum kinetic theory approach to the line broadening, to eliminate the remaining discrepancy of theory and experiment). The allowance for radiative transfer effects under conditions of the Stark broadening of spectral lines substantially complicates the diagnostics of the medium’s parameters (see, e.g., the case of steady-state transport in inhomogeneous plasmas [35]). In light of these issues, the automodel solutions of the time-dependent Green’s function of the radiative transfer equation in homogeneous media, considered in the present paper, may serve as reliable benchmarks for radiative transfer models in various applications.

Here we extend the approach [28] to the case of the Green’s function of the 3D Biberman-Holstein equation for the Voigt spectral line shape, assuming the contribution of the impact Stark broadening to the Lorentz component of the Voigt line shape (Section 2.2.2). Additionally, the results for the case of Holtsmark line shape are presented (Section 2.2.3). The main equations are given in Section 2.1, and conclusions are made in Section 3.

2. Results

2.1. Main Equations

2.1.1. Biberman–Holstein Equation

The Biberman–Holstein equation [9,10] for radiative transfer in a uniform medium of two-level atoms/ions has been obtained from the system of equations for spatial density of excited atoms, F(r, t), and spectral intensity of resonance radiation. This system is reduced to a single equation for F(r, t), which appears to be an integral equation, non-reducible to a differential diffusion-type equation:

$$\frac{\partial F\left(r,t\right)}{\partial t}=\frac{1}{\tau }{\int }_{V}G\left(\left|r-r_1\right|\right)F\left(r_1,t\right)d{V}_1-\left(\frac{1}{\tau }+\sigma \right)F\left(r,t\right)+q\left(r,t\right),$$

(1)

where τ is the lifetime of the excited atomic state with respect to spontaneous radiative decay; σ is the rate of the collisional quenching of excitation; q is the source of excited atoms, different from populating by the absorption of resonant photons (e.g., collisional excitation). The kernel G is determined by the (normalized) emission spectral line shape ε_v and the absorption coefficient k_v. In homogeneous media, G depends on the distance between the points of emission and absorption of the photon:

$$G\left(r\right)=-\frac{1}{4\pi r^2}\frac{dT\left(r\right)}{dr}, T\left(r\right)=\underset{0}{\overset{\infty }{\int }}{\epsilon }_{\nu }\exp \left(-k_{\nu }r\right)d\nu .$$

(2)

The analytical solution of Equation (1) with a point instant source, q(r, t) = δ(r)δ(t), i.e., the Green’s function, was obtained in [11] with the help of the Fourier transform:

$$f\left(r,t\right)=-\frac{e^{-t\left(\frac{1}{\tau }+\sigma \right)}}{{\left(2\pi \right)}^{2}r}\frac{\partial }{\partial r}\left\{\underset{-\infty }{\overset{\infty }{\int }}{e}^{-ipr}\left[\exp \left\{\frac{t}{\tau }J\left(p\right)\right\}-1\right]dp+2\pi \delta \left(r\right)\right\},$$

(3)

where:

$$J\left(p\right)=\frac{1}{p}\underset{0}{\overset{\infty }{\int }}{\epsilon }_{\nu }{k}_{\nu }\mathrm{arctg}\frac{p}{k_{\nu }}d\nu .$$

(4)

The homogeneity of the medium implies that τ and σ are constants. The latter makes the role of quenching easily accounted for by the time exponent exp(–σt), so in what follows we omit the account of this process, i.e., in fact, for convenience we take σ = 0. Hereafter we use the dimensionless time, assuming the normalization by τ.

Equation (3) for r ≠ 0 may be transformed to take the following form:

$$f\left(r,t\right)=\frac{1}{{\left(2\pi \right)}^{2}r}\underset{-\infty }{\overset{\infty }{\int }}p\sin \left(pr\right)\left[exp\left\{t\left(J\left(p\right)-1\right)\right\}-exp\left\{-t\right\}\right]dp.$$

(5)

Let us consider two cases where the Stark broadening plays a dominant role in the resonance radiative transfer in the Biberman–Holstein model, namely, the case of the Voigt spectral line shape, assuming the contribution of the impact Stark broadening to the Lorentz component of the Voigt spectral line shape (Section 2.1.2), and the case of the static Stark broadening in the Holtsmark model (Section 2.1.3).

2.1.2. Exact Solution for Voigt Spectral Line Shape

The line shape ε_ν is taken in the form [36]:

$${\epsilon }_{\nu }\left(a\right)=C\prime \left(a\right)\frac{2\sqrt{\ln 2}}{\Delta {\nu }_D}W\left(a,\omega \left(\nu \right)\right),$$

(6)

where:

$$W\left(a,\omega \right)=\underset{-\infty }{\overset{+\infty }{\int }}\frac{e^{-y^2}dy}{a^2+{\left(\omega -y\right)}^2},$$

(7)

$$\omega =\frac{2\sqrt{\ln 2}\left({\nu }_0-\nu \right)}{\Delta {\nu }_D},$$

(8)

$$a=\frac{\Delta {\nu }_L}{\Delta {\nu }_D}\sqrt{\ln 2}.$$

(9)

Here $\Delta {\nu }_D$ is the full width at half maximum (FWHM) of the Doppler line shape, and $\Delta {\nu }_L$ is the FWHM of the Lorentz line shape. The coefficient $C^{\prime }\left(a\right)$ is determined by the normalization condition, ${\int }_0^{\infty }{\epsilon }_{\nu }d\nu =1$. For the Stark broadening of the spectral line shape one may use the well-known formulae for the impact broadening, e.g., in the monographs [1,2], and learn the progress in the recent surveys [37,38].

The respective absorption coefficient k_ν(a) has the form:

$$\begin{gathered} k_{\nu }\left(a\right)=k_0\frac{W\left(a,\omega \left(\nu \right)\right)}{W\left(a,0\right)}, \\ {k}_0=n_0\pi {\lambda }^2\frac{W\left(a,0\right)}{\tau }\frac{g_n}{g_0}, \end{gathered}$$

(10)

where n₀ is the density of absorbing atoms, λ, the wavelength of a photon, $g_i$, statistical weight of the i-th level. Using Equation (3.466.1) in [39], $W\left(a,0\right)$ may be expressed in the form:

$$W\left(a,0\right)=\underset{-\infty }{\overset{+\infty }{\int }}\frac{e^{-y^2}dy}{a^2+y^2}=\left[1-\mathrm{erf}\left(a\right)\right]\frac{\pi }{a}{e}^{a^2},$$

(11)

where erf(x) is the error function:

$$\mathrm{erf}\left(x\right)=\frac{2}{\sqrt{\pi }}\underset{0}{\overset{x}{\int }}{e}^{-t^2}dt.$$

(12)

Let us turn in Equation (5) to dimensionless variables ρ = k₀r and P = p/k₀, $\nu \prime \prime =2\sqrt{\ln 2}\left(\nu -{\nu }_0\right)/\mathsf{\Delta }{\nu }_D$ and use Equations (6) and (10) for ε_ν and k_ν. This gives:

$$G\left(\rho ;a\right)=-k_0^3\frac{1}{4\pi {\rho }^2}\frac{dT\left(\rho ;a\right)}{d\rho },$$

(13)

$$\mathrm{T}\left(\rho ;a\right)\equiv C\prime \left(a\right)\underset{-\infty }{\overset{\infty }{\int }}W\left(a,-\nu \prime \prime \right)\exp \left(-\frac{W\left(a,-\nu \prime \prime \right)}{W\left(a,0\right)}\rho \right)d{\nu }^{\prime },$$

(14)

$$f\left(\rho ,t;a\right)=k_0^3\frac{1}{{\left(2\pi \right)}^2\rho }\underset{-\infty }{\overset{\infty }{\int }}P\sin \left(P\rho \right)\left[\exp \left\{t\left(J\left(P;a\right)-1\right)\right\}-\exp \left\{-t\right\}\right] dP, \rho \ne 0,$$

(15)

$$J\left(P;a\right)=\frac{1}{P}\frac{C\prime \left(a\right)}{W\left(a,0\right)}\underset{-\infty }{\overset{\infty }{\int }}{\left[W\left(a,-\nu \prime \prime \right)\right]}^2\mathrm{arctg}\frac{PW\left(a,0\right)}{W\left(a,-\nu \prime \prime \right)}d\nu \prime \prime .$$

(16)

2.1.3. Exact Solution for Holtsmark Spectral Line Shape

For Holtsmark spectral line shape the functions ε_ν and k_ν, which enter the function J(p), for linear Stark effect may be expressed in the form (cf. [3]):

$${\epsilon }_{\nu }=\frac{1}{\Delta {\nu }_H}\mathcal{H}\left[\frac{\nu -{\nu }_0}{\Delta {\nu }_H}\right], \Delta {\nu }_H=C_2{F}_0, F_0=2.603e{N}^{2/3},$$

(17)

$$k_{\nu }=k_0\mathcal{H}\left[\frac{\nu -{\nu }_0}{\Delta {\nu }_H}\right],$$

(18)

where N is the density of the perturbing particles, and $\mathcal{H}\left(\beta \right)$ is the Holtsmark function:

$$\mathcal{H}\left(\beta \right)=\frac{2}{\pi }\beta \underset{0}{\overset{\infty }{\int }}x\sin \left(\beta x\right)e^{-x^{3/2}}dx.$$

Turning in Equation (5) to dimensionless variables ρ = k₀r and P = p/k₀, ${\nu }^{\prime }=\left(\nu -{\nu }_0\right)/\mathsf{\Delta }{\nu }_H$ and using Equations (17) and (18) for ε_ν and k_ν, we obtain:

$$f\left(\rho ,t\right)=-k_0^3\frac{1}{{\left(2\pi \right)}^2\rho }\frac{\partial }{\partial \rho }\underset{-\infty }{\overset{\infty }{\int }}\cos \left(P\rho \right)\left[\exp \left\{t\left(J\left(P\right)-1\right)\right\}- \exp \left\{-t\right\}\right]dP, \rho \ne 0,$$

(19)

$$J\left(P\right)=\frac{1}{P}\underset{-\infty }{\overset{\infty }{\int }}{\left[\mathcal{H}\left(\nu \prime \right)\right]}^{2}arctg\frac{P}{\mathcal{H}\left(\nu \prime \right)}d\nu \prime .$$

(20)

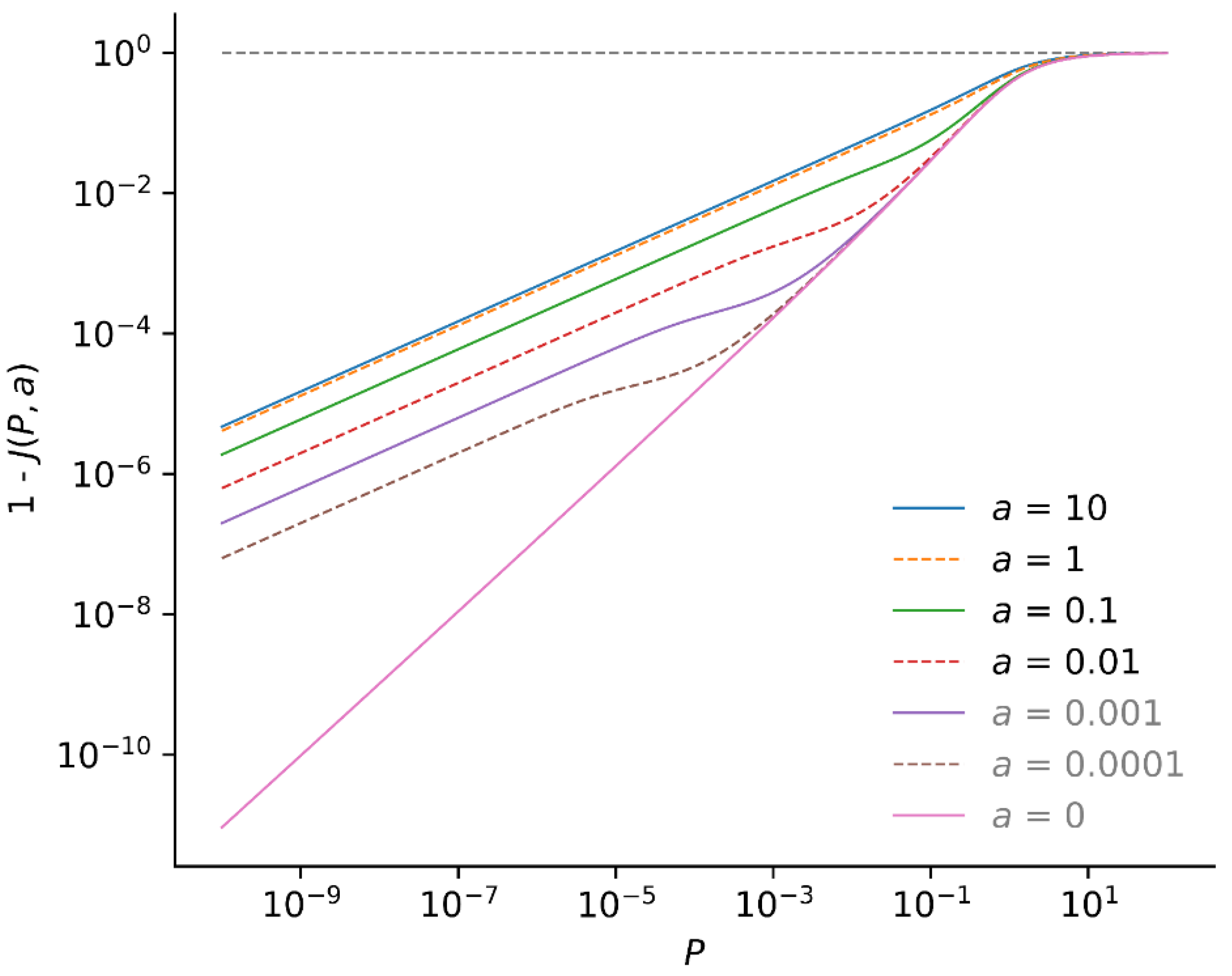
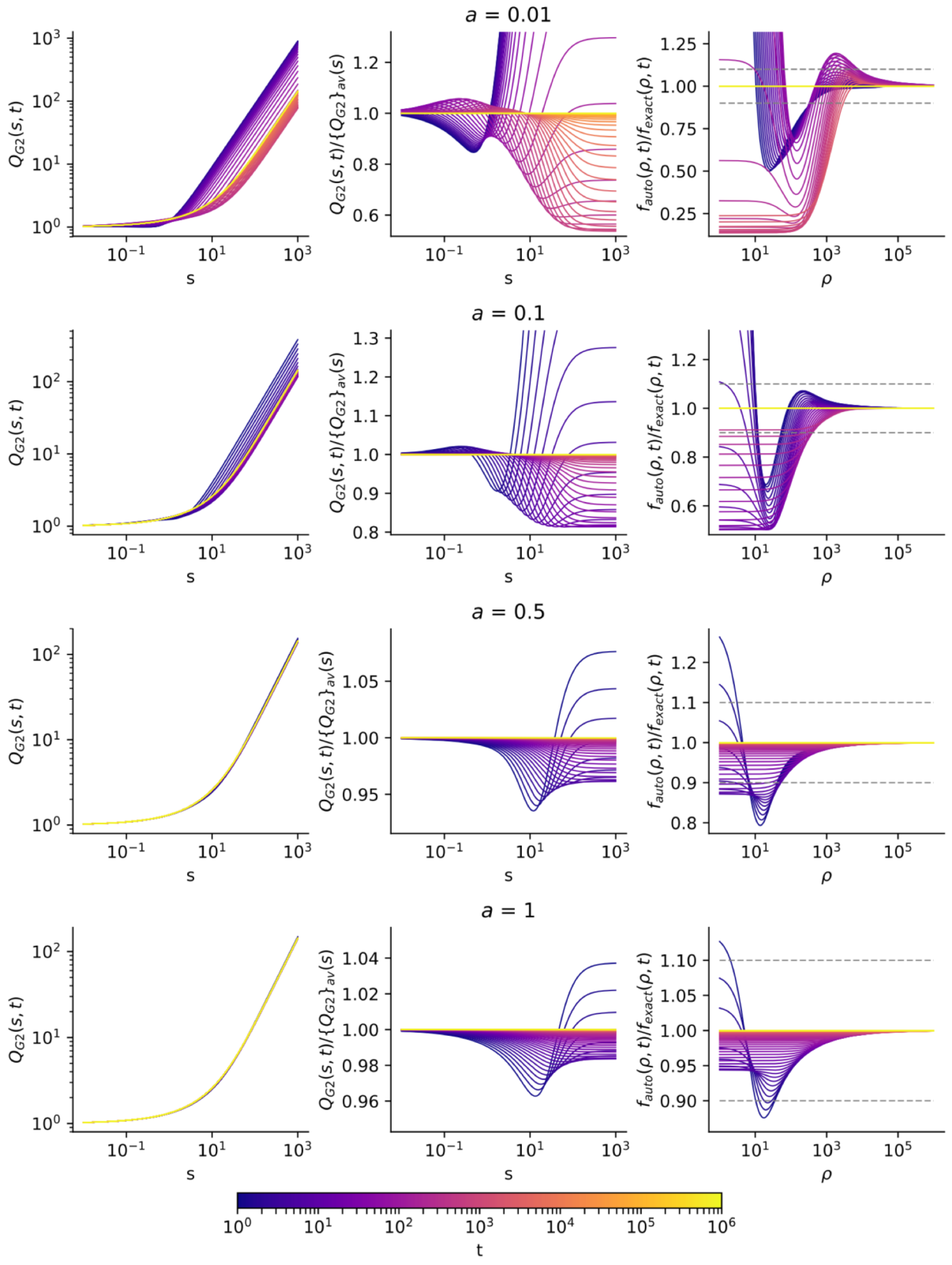
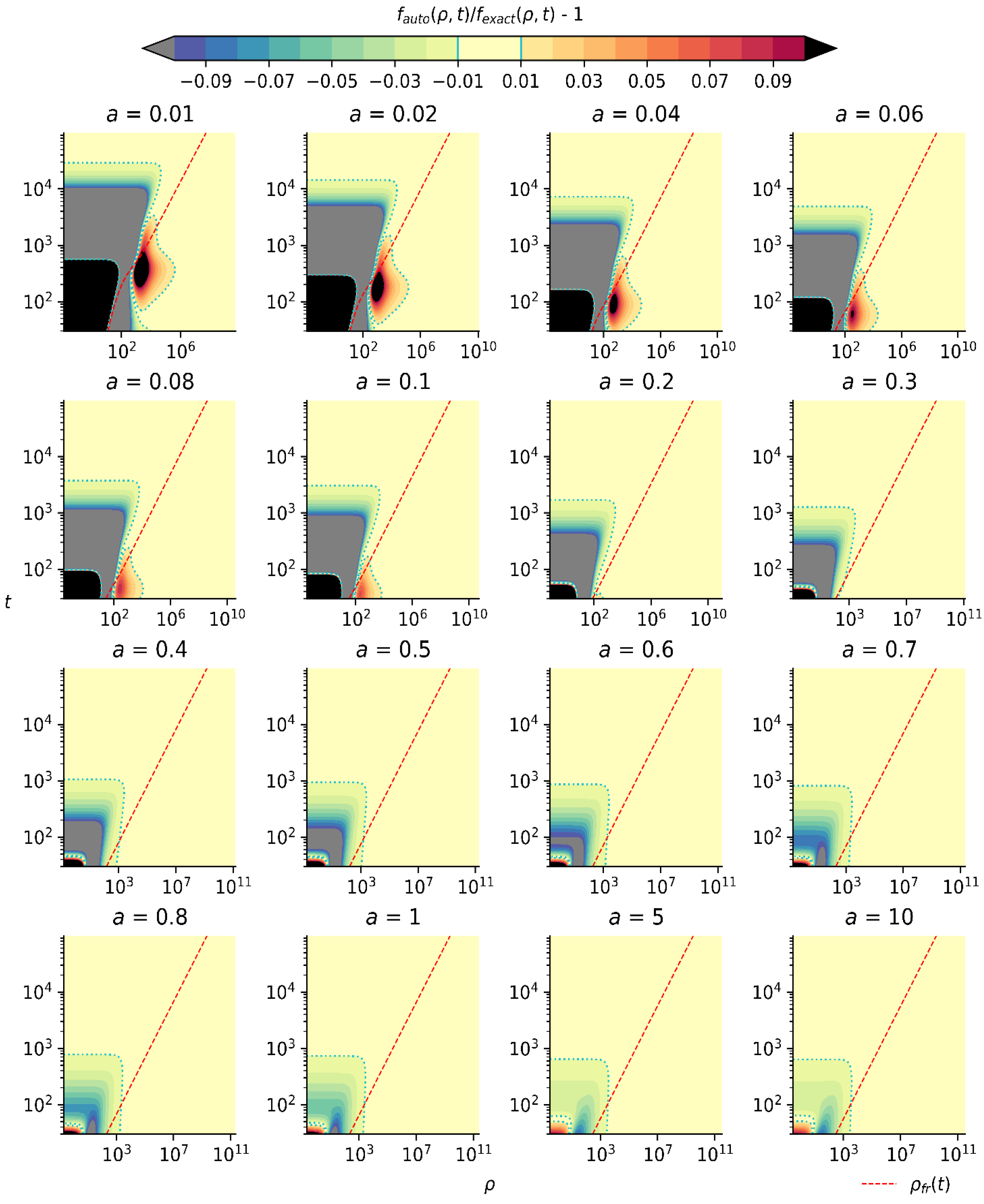
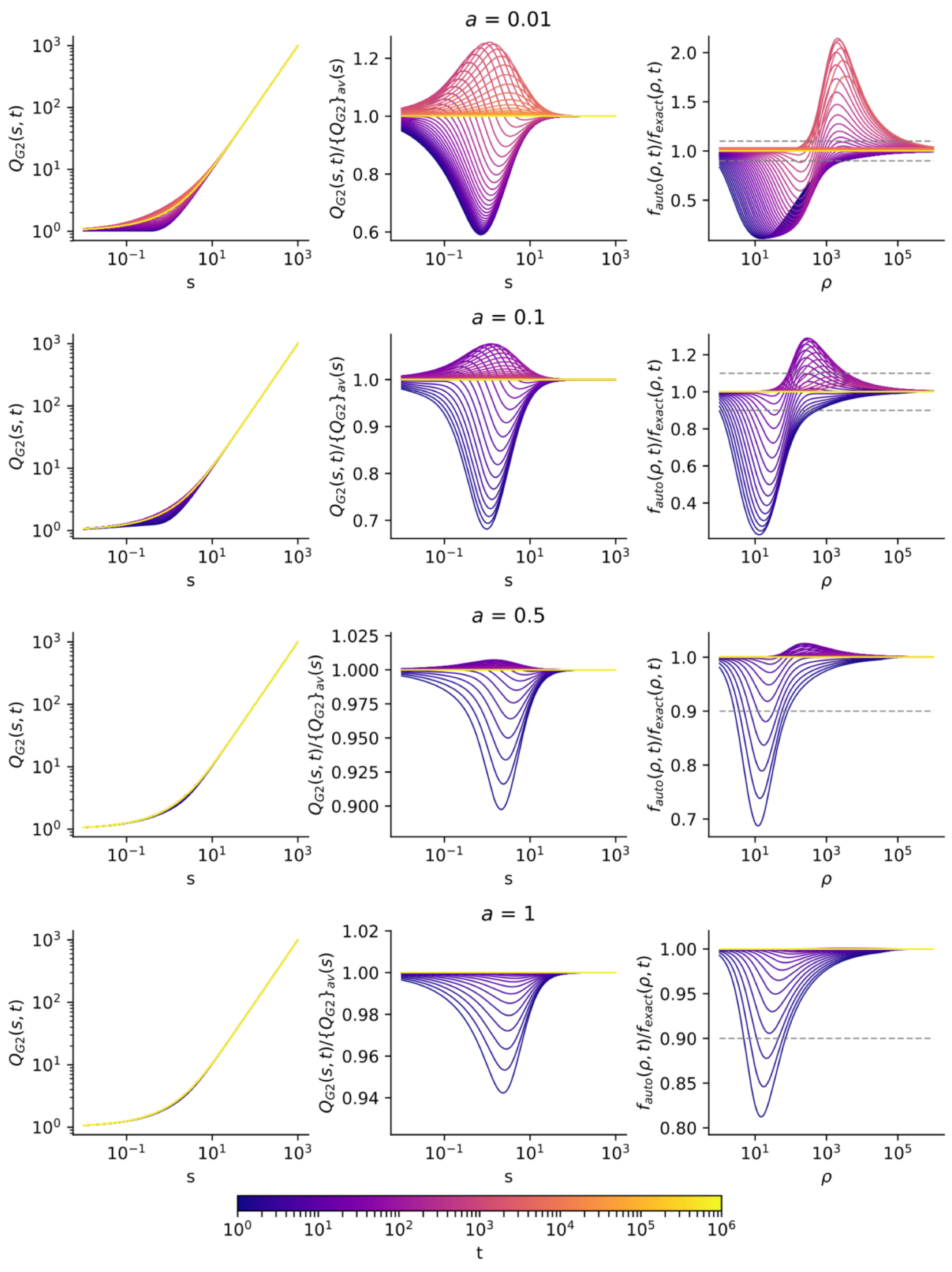
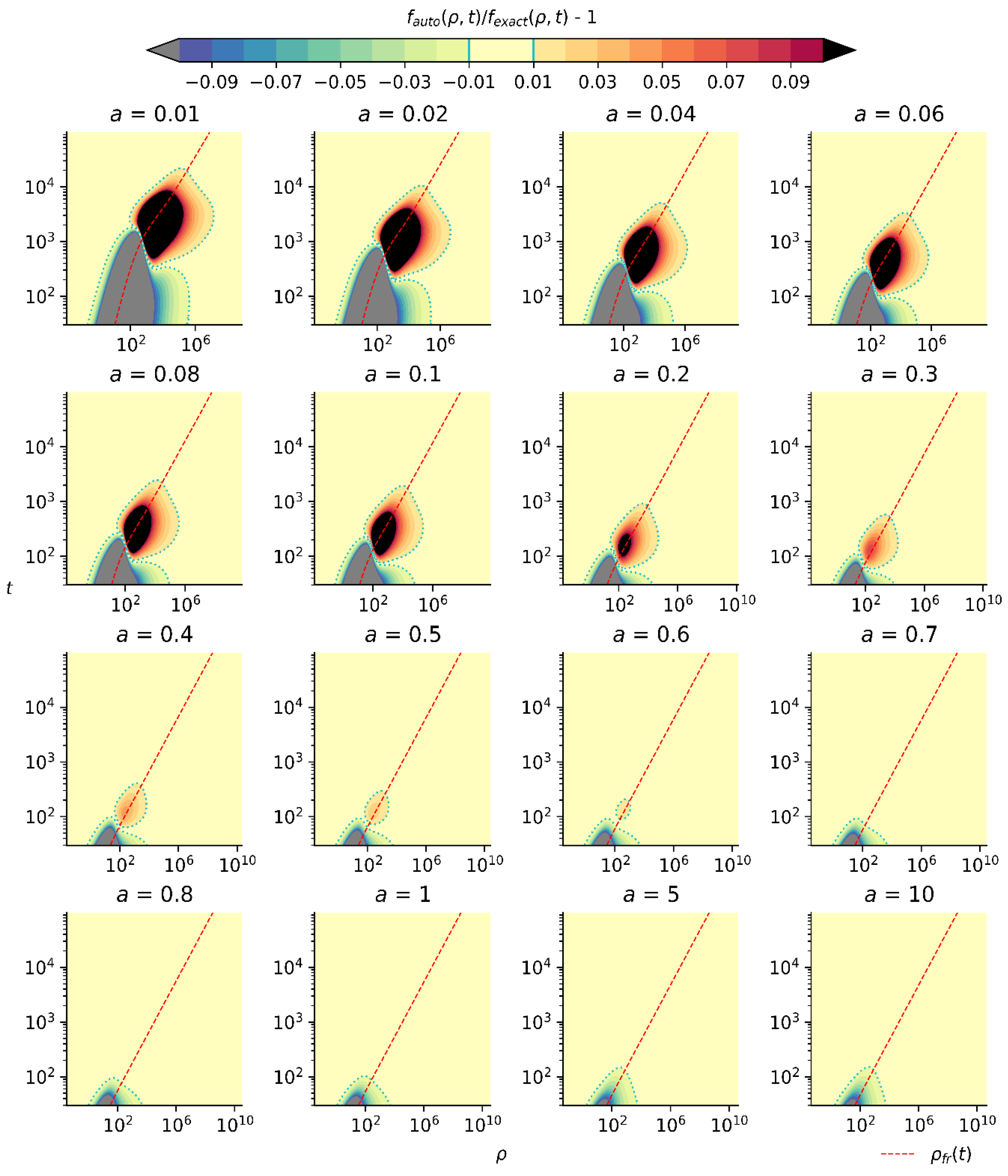
2.2. Approximate Automodel Solution and Verification for Accuracy

2.2.1. General Equations

The approximate automodel solution [21] has the form:

$$f_{\mathrm{auto}}\left(r,t\right)=tG\left(rg\left(\frac{r_{\mathrm{fr}}\left(t\right)}{r}\right)\right),$$

(21)

where G is the kernel of the Biberman-Holstein equation, g is an unknown function with the following asymptotics:

$$g\left(s\right)=1, s\ll 1,$$

(22)

$$g\left(s\right)\propto s, s\gg 1.$$

(23)

For the propagation front, ${\rho }_{\mathrm{fr}}\left(t\right)$, we used in [21,28] the function which appears to be close to the time dependence of the mean displacement:

$$\left(t+1\right)\mathrm{T}\left({\rho }_{\mathrm{fr}}\left(t\right)\right)=1, \rho =\left|x\right|.$$

(24)

Alternatively, one may use another function which appears to work better in the case of spectral line shape which is a convolution of two essentially different line shapes (e.g., for Voigt line shape):

$$f_{\mathrm{exact}}\left(0,t\right)=t G\left({\rho }_{\mathrm{fr}}\left(t\right)\right),$$

(25)

where:

$$f_{exact}\left(0,t\right)=k_0^3\frac{1}{{\left(2\pi \right)}^2}\underset{-\infty }{\overset{\infty }{\int }}{P}^2\left[\exp \left\{t\left(J\left(P\right)-1\right)\right\}- \exp \left\{-t\right\}\right]dP.$$

(26)

The relation between g and the exact solution of Equation (1), f_exact, is described by the following equation:

$$Q_G\left(\rho ,t\right)\equiv \frac{1}{\rho }{G}^{-1}\left(\frac{f_{\mathrm{exact}}\left(\rho ,t\right)}{t}\right),$$

(27)

where G⁻¹ is the function reciprocal to the G function, ρ ≡ k₀|r – r₀|, k₀ is the absorption coefficient for photons at the frequency, corresponding to the line shape center:

$$Q_G\left(\rho ,t\left(\rho ,s\right)\right)\equiv Q_{G1}\left(s,\rho \right)=g\left(s\right),$$

(28)

$$Q_G\left(\rho \left(t,s\right),t\right)\equiv Q_{G2}\left(s,t\right)=g\left(s\right),$$

(29)

where the functions t(ρ, s) and ρ(t, s) are determined by the relation:

$$s=\frac{{\rho }_{fr}\left(t\right)}{\rho }.$$

(30)

To prove the automodel solution one has to show weak dependence (independence) of Q_G1 and Q_G2 functions on, respectively, the space coordinate and time. The results of the validation of the automodel solution and the reconstruction of function g from comparison of function (21) with computations of the Green’s function for the Voigt and Holtsmark line shapes are given in what follows.

2.2.2. Automodel Solution for the Voigt Spectral Line Shape

The convolution of two line shapes with essentially different wings, an exponential one, for the Doppler case, and a power-law one, for the Lorentz case, makes the superdiffusive radiative transfer very sensitive to the contribution of the power-law wings of spectral line to the resulting long-tailed PDF. This is illustrated with the Figure 1 where the dependence of the exponent in the exact solution (Equation (15)) is shown. It is seen that even a small fraction of the Lorentz line shape in the Voigt line shape produces strong effects at large distances (for small values of p, respectively).

We illustrate the results of accuracy analysis with three figures. First, the functions Q_G2(s,t) (Equation (29)) are shown for different values of t in the range from t_min = 30 to t_max = 10⁶. Further, the normalized functions Q_G2(s,t)/{Q_G2}_av(s), where subscript av denotes averaging over time from t_min = 30 to t_max = 10⁸, and the relative errors of the automodel solution f_auto(ρ,t)/f_exact(ρ,t) are shown for the same range of time.

The results of analysis for various values of the Voigt parameter a are shown in Figure 2.

For applications it is of interest to determine the limits of applicability of the automodel solutions with a certain accuracy. To this end, in Figure 3 we show the level lines of the relative deviation of the automodel solution from the exact one, for the results from Figure 2.

It is seen that for the Lorentz-dominated line shapes, i.e., moderate and large values of the Voigt parameter a, the accuracy of automodel solution is high in almost the entire space of variables {ρ, t}, quite similar to the case [21,28] of the superdiffusive transport for a 1D model PDF with the power-law wings. However, for a small a, the accuracy dramatically falls down in the essential part of the space of variables {ρ, t}. This failure stimulated searching for improving the accuracy by using the propagation front (Equation (25)). The respective results are shown in Figure 4 and Figure 5.

Figure 3 and Figure 5 show that the automodel solution with the propagation front ρ = ρ_fr (Equation (25)) substantially improves the accuracy in the regions of the failure of the automodel solution with the propagation front (Equation (24)). This issue points to the possibility to further improve the accuracy by the variation of the definition of the propagation front and further verification of the accuracy of the respective automodel function via calculations of the exact solution in a limited part of the space of variables {ρ, t} (not in the entire space, only in the region around the propagation front).

2.2.3. Automodel Solution for the Holtsmark Spectral Line Shape

Here we append the results [21,27] of analyzing the automodel solution for the Holtsmark line shape with the results for the accuracy of automodel solution. Figure 6 shows the behavior of the automodel function Q_G2 (29) and the relative deviation of the automodel solution from the exact one in the range from t_min = 40 to t_max = 10³, with the time step equal to 20, for 40 ≤ t ≤ 500, and 50, for 500 < t ≤ 1000.

The results show that, similarly to the 1D transport for a model step-length PDF with power-law wings [21,28], the accuracy of automodel solution is reasonably good for the propagation front (Equation (24)).

3. Discussion

The history of the research on the superdiffusive transport suggests that it is not possible to guess the “hidden” self-similarity from the general form of the transport equation, including the Biberman-Holstein equation, or from its analytical solutions in certain cases like the analytic solution [11] of the Biberman-Holstein equation. The approximate automodel (self-similar) solution [21] has been found thanks to:

• a hint from physics (namely, analysis of the kinetics of elementary excitation carriers); and
• interpolation of asymptotic solutions and solving an inverse problem which requires numerical simulations to verify the accuracy of the automodel solution and determine the limits of its applicability.

Obtaining automodel (self-similar) solutions in the entire space of independent variables requires mass numerical simulations, however, their total volume is significantly reduced due to the self-similarity of the solution.

The Stark broadening of spectral lines, including the contribution of the impact of Stark broadening to the Lorentz component of the Voigt line shape and the Holsmark broadening, produce the step-length probability distribution function (PDF) which makes the transport superdiffusive. The accuracy of approximate automodel solutions for the Green's function of the Biberman-Holstein equation for the Stark broadening of spectral lines is analyzed using the distributed computing. The high accuracy of automodel solutions in a wide range of parameters of the problem is shown. Massive computing experiments are conducted to verify automodel solutions. The Everest distributed computing platform and the cluster at the NRC ‘Kurchatov Institute’ are used. The results, obtained with distributed computing, verified the high accuracy of automodel solutions in a wide range of space-time variables and enabled us to identify the limits of applicability of automodel solutions.

The sensitivity of the automodel solution to the definition of the front propagation scaling is shown for the line shape with two quite different line broadening mechanisms, and the improvement of the accuracy of the automodel solution is achieved via generalization of the definition of the propagation front.

The present progress in describing the radiative transfer for infinite velocity of carriers, Equation (1), may be extended to the case of a finite velocity [40,41] (which includes the case of the resonance radiative transfer in astrophysics), where the exact solution and the asymptotics were also obtained [42].

4. Materials and Methods

Distributed computations have been done on the cluster at NRC “Kurchatov Institute” (http://ckp.nrcki.ru/) by means of the Everest (http://everest.distcomp.org/), a computing platform for publication, execution, and composition of applications running across distributed computing resources [43]. A generic Everest application, the so-called Parameter Sweep, (http://everest.distcomp.org/docs/ps) [44], was used to run a number of independent tasks at the cluster via a special Everest agent installed on the cluster. The calculation of exact and automodel solutions for a single value of parameter a in the Voight line shape (6) was an independent task. The values of a were taken in the range from 0.01 to 10, evenly spaced on a log scale, that yields 28 tasks. Since the two different expressions for the propagation front were considered (see Equations (24) and (25)) the total number of independent tasks was 56.

In order to obtain functions g(s), the exact solutions f_exact(ρ,t) were calculated. The values of t were evenly spaced on a log scale in the range from t_min = 30 to t_max = 10⁸. The values of s were also evenly spaced on a log scale. Depending on the value of a, the lower limit for s was set in the range from 0.00003 (small a) to 0.005 (large a) while the upper limit was set to 1000 for all values of a. The respective values of ρ are defined by the t and s numerical meshes, using Equation (30).

All analytic expressions of Equations (6)–(30) have been implemented in the form of a Python program, using the NumPy and SciPy, well-known scientific libraries (www.scipy.org).

In addition, direct comparison of the automodel (Equation (21)) and exact (Equation (15)) solutions of the transport Equation (1), the range of variables in the two-dimensional space, {time, space coordinate}, where the automodel solution is accurate within certain error bars around the exact solution was identified.