New Los Alamos Opacity Calculations
Abstract
:1. Introduction
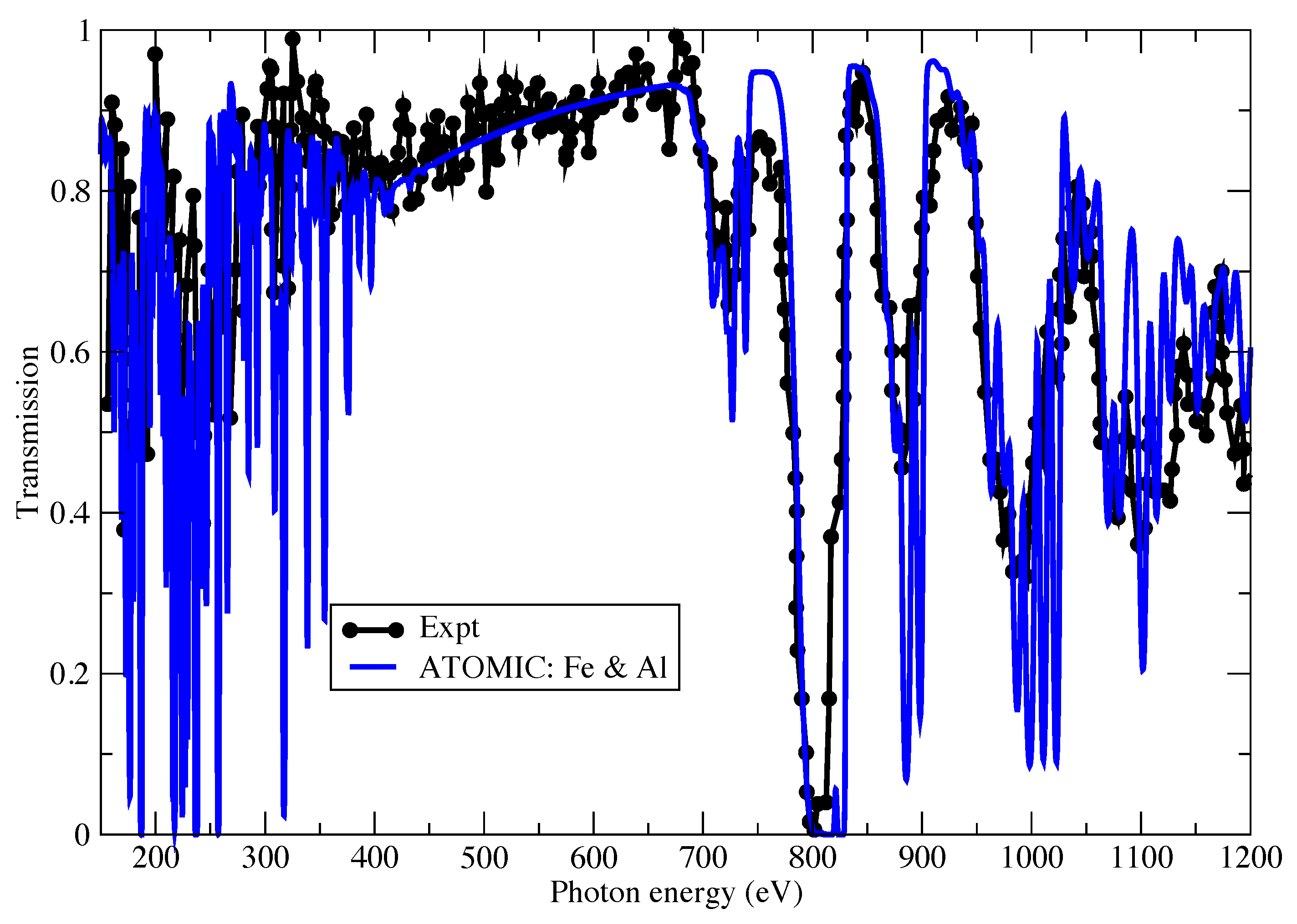
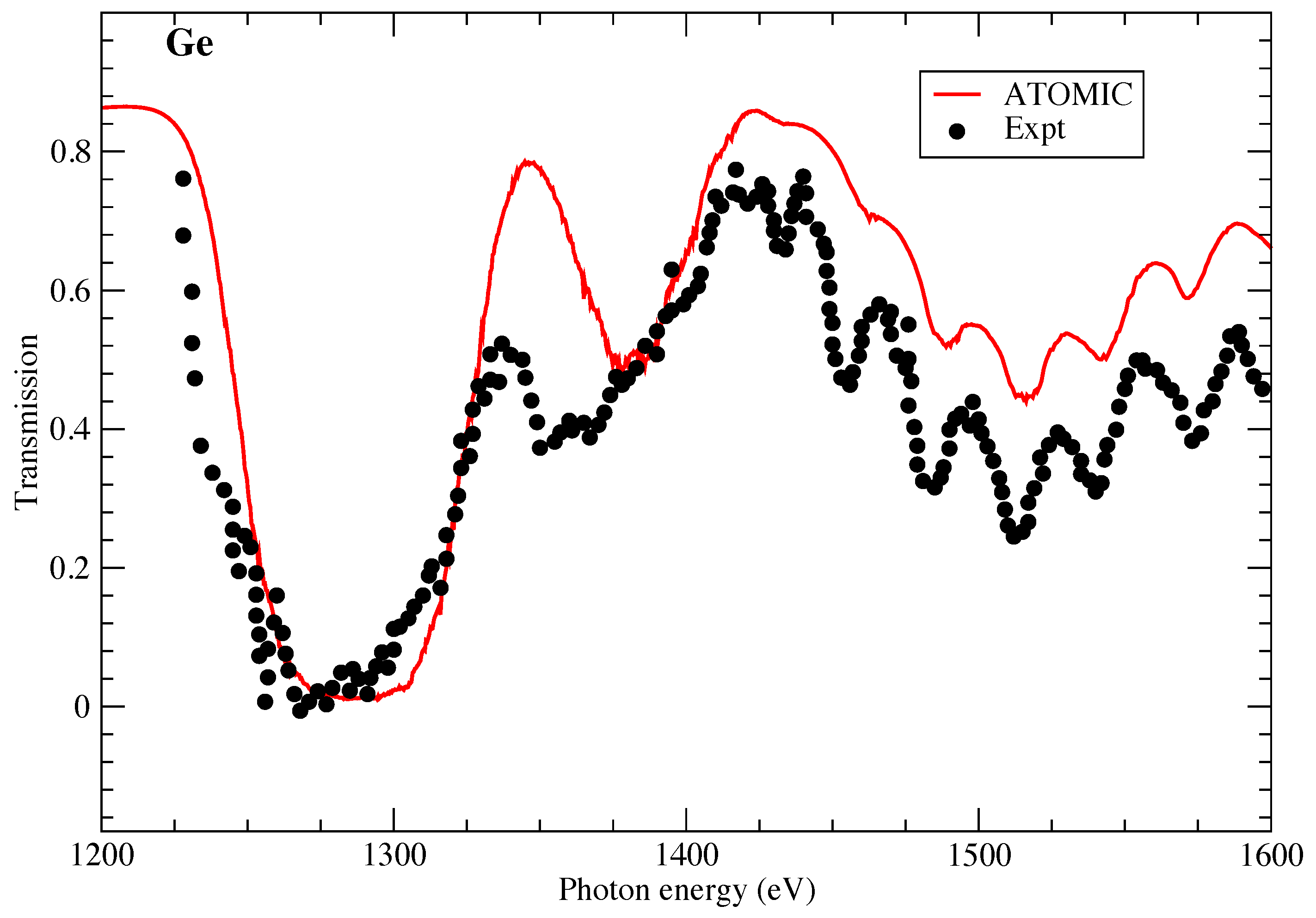
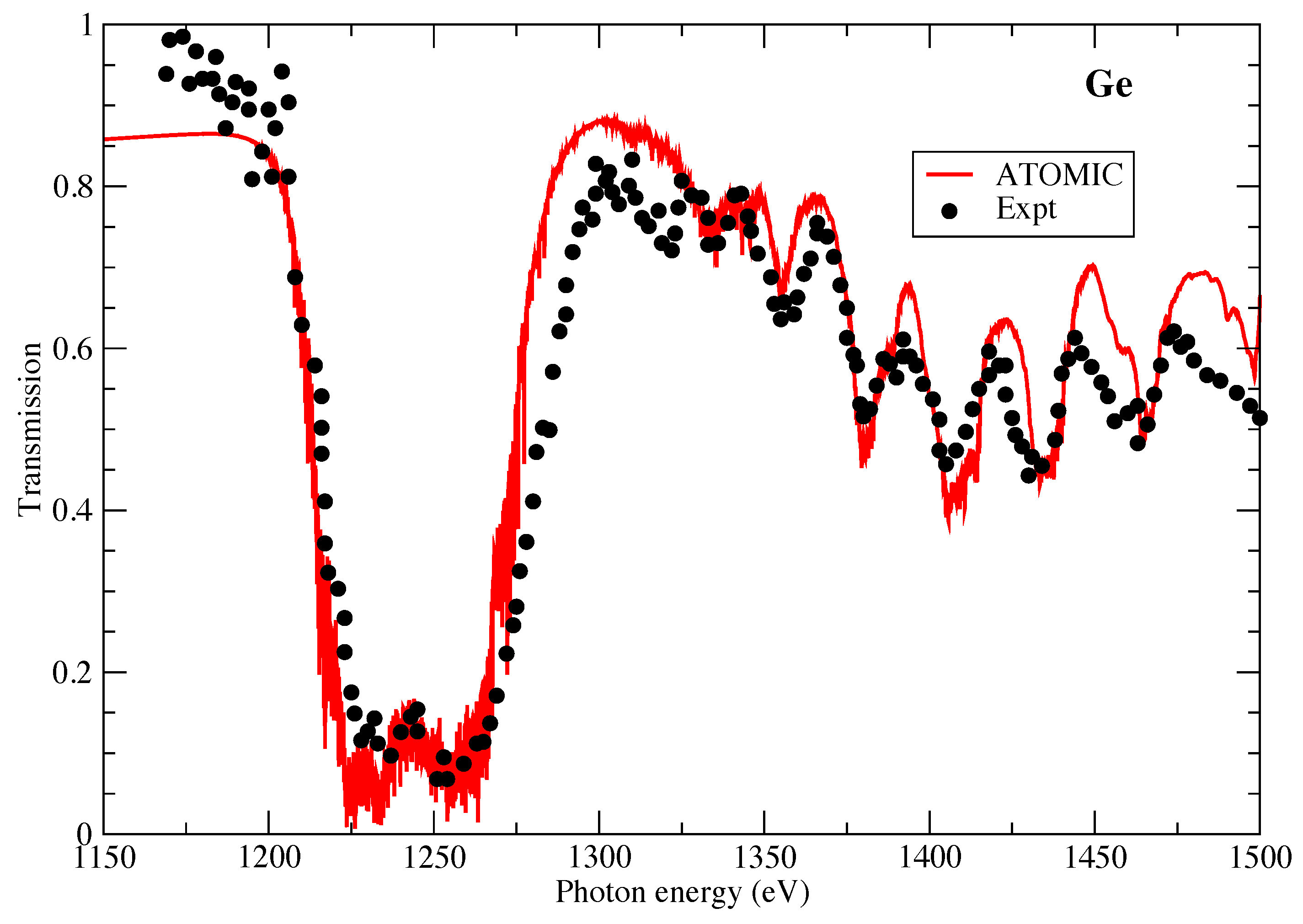
2. Results
3. Comparison with Experiment
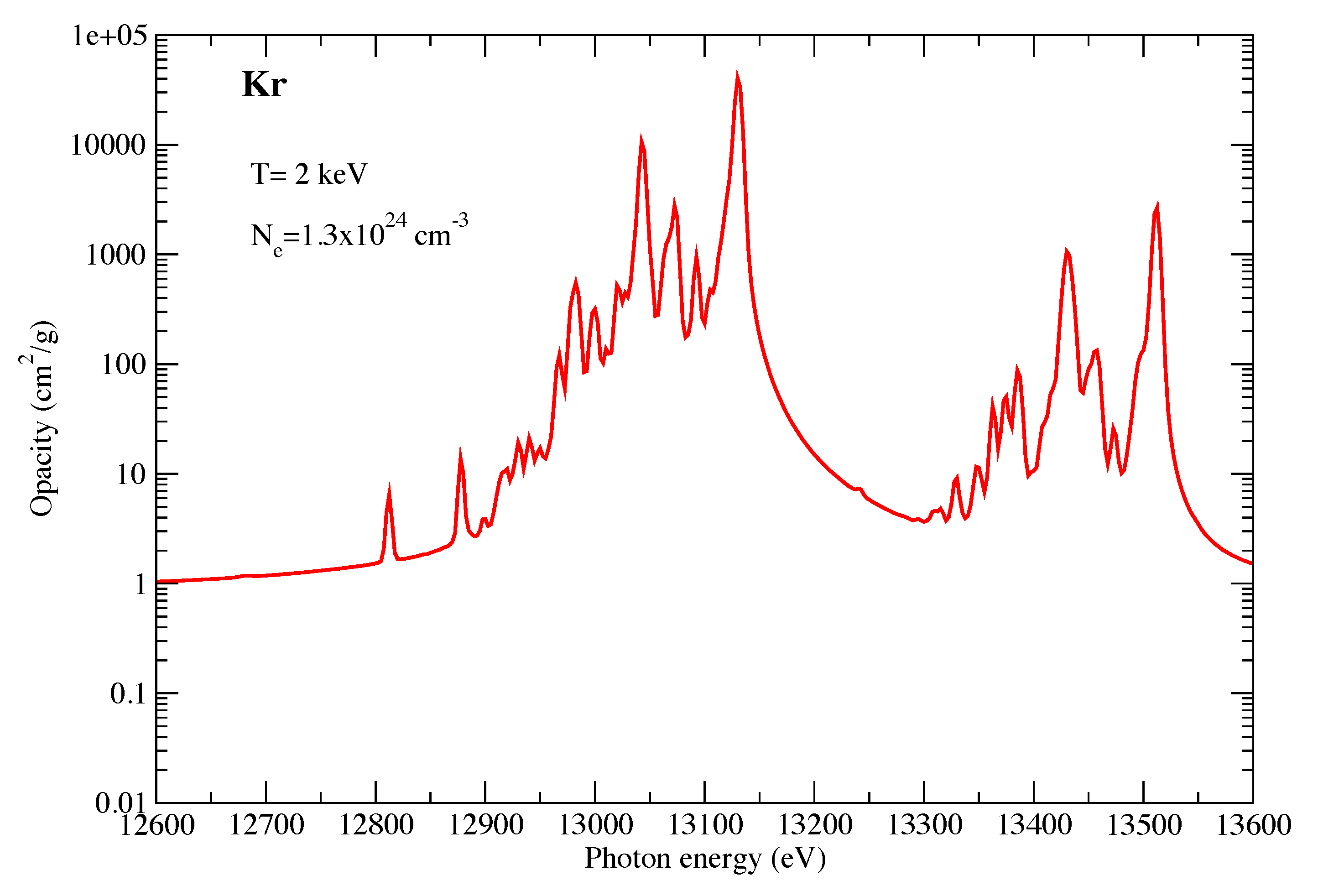
4. Kr Opacity
5. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Huebner, W.F.; Barfield, W.D. Opacity; Springer: New York, NY, USA, 2014. [Google Scholar]
- Magee, N.H.; Abdallah, J., Jr.; Clark, R.E.H.; Cohen, J.S.; Collins, L.A.; Csanak, G.; Fontes, C.J.; Gauger, A.; Keady, J.J.; Kilcrease, D.P.; et al. Atomic Structure Calculations and New Los Alamos Astrophysical Opacities. Astron. Soc. Pac. Conf. Ser. 1995, 78, 51. [Google Scholar]
- Neuforge-Verheecke, C.; Guzik, J.A.; Keady, J.J.; Magee, N.H.; Bradley, P.A.; Noels, A. Helioseismic Tests of the New Los Alamos LEDCOP Opacities. Astrophys. J. 2001, 561, 450–454. [Google Scholar] [CrossRef] [Green Version]
- Colgan, J.; Kilcrease, D.P.; Magee, N.H., Jr.; Sherrill, M.E.; Abdallah, J., Jr.; Hakel, P.; Fontes, C.J.; Guzik, J.A.; Mussack, K.A. A new generation of Los Alamos opacity tables. Astrophys. J. 2016, 817, 116. [Google Scholar] [CrossRef]
- Walczak, P.; Fontes, C.J.; Colgan, J.; Kilcrease, D.P.; Guzik, J.A. Wider pulsation instability regions for β Cephei and SPB stars calculated using new Los Alamos opacities. Astron. Astrophys. 2015, 580, L9. [Google Scholar] [CrossRef]
- Daszyńska-Daszkiewicz, J.; Pamyatnykh, A.A.; Walczak, P.; Colgan, J.; Fontes, C.J.; Kilcrease, D.P. Interpretation of the BRITE oscillation data of the hybrid pulsator ν Eridani: A call for the modification of stellar opacities. Mon. Not. R. Astron. Soc. 2016, 466, 2284. [Google Scholar] [CrossRef]
- Buldgen, G.; Salmon, S.J.A.J.; Godart, M.; Noels, A.; Scuflaire, R.; Dupret, M.A.; Reese, D.R.; Colgan, J.; Fontes, C.J.; Eggenberger, P.; et al. Inversions of the Ledoux discriminant: A closer look at the tachocline. Mon. Not. Roy. Astron. Soc. 2017, 472, L70. [Google Scholar] [CrossRef]
- Buldgen, G.; Salmon, S.J.A.J.; Noels, A.; Scuflaire, R.; Dupret, M.A.; Colgan, J.; Fontes, C.J.; Eggenberger, P.; Hakel, P.; Kilcrease, D.P.; et al. Seismic inversion of the solar entropy: A case for improving the Standard Solar Model. Astron. Astrophys. 2017, 607, A58. [Google Scholar] [CrossRef]
- Basu, S. Helioseismic tests of stellar equations of state and opacities. Astron. Soc. Pac. Conf. Ser. 2018, in press. [Google Scholar]
- Colgan, J.; Kilcrease, D.P.; Magee, N.H.; Abdallah, J.; Sherrill, M.E.; Fontes, C.J.; Hakel, P.; Guzik, J.A.; Mussack, K.A.; Walczak, P. A new generation of Los Alamos opacity tables. AIP Conf. Proc. 2017, 1811, 160002. [Google Scholar] [Green Version]
- Colgan, J.; Kilcrease, D.P.; Magee, N.H.; Sherrill, M.E.; Fontes, C.J.; Hakel, P. New Los Alamos Opacities. Astron. Soc. Pac. Conf. Ser. 2018, in press. [Google Scholar]
- Chen, H.; Ma, T.; Nora, R.; Barrios, M.A.; Scott, H.A.; Schneider, M.B.; Berzak Hopkins, L.; Casy, D.T.; Hammel, B.A.; Jarrott, L.C.; et al. On krypton-doped capsule implosion experiments at the National Ignition Facility. Phys. Plasmas 2017, 24, 072715. [Google Scholar] [CrossRef]
- Hakel, P.; Kyrala, G.A.; Bradley, P.A.; Krasheninnikova, N.S.; Murphy, T.J.; Schmitt, M.J.; Tregillis, I.L.; Kanzleieter, R.J.; Batha, S.H.; Fontes, C.J.; et al. X-ray spectroscopic diagnostics and modeling of polar-drive implosion experiments on the National Ignition Facility. Phys. Plasmas 2014, 21, 063306. [Google Scholar] [CrossRef]
- Baumgaertel, J.A.; Bradley, P.A.; Hsu, S.C.; Cobble, J.A.; Hakel, P.; Tregillis, I.L.; Krasheninnikova, N.S.; Murphy, T.J.; Schmitt, M.J.; Shah, R.C.; et al. Observation of early shell-dopant mix in OMEGA direct-drive implosions and comparisons with radiation-hydrodynamic simulations. Phys. Plasmas 2014, 21, 052706. [Google Scholar] [CrossRef]
- Sasaki, A.; Sunahara, A.; Furukawa, H.; Nishihara, K.; Fujioka, S.; Nishikawa, T.; Koike, F.; Ohashi, H.; Tanuma, H. Modeling of radiative properties of Sn plasmas for extreme-ultraviolet source. J. Appl. Phys. 2010, 107, 113303. [Google Scholar]
- Colgan, J.; Kilcrease, D.P.; Abdallah, J., Jr.; Sherrill, M.E.; Fontes, C.J.; Hakel, P.; Armstrong, G.S.J. Atomic structure considerations for the low-temperature opacity of Sn. High Energy Density Phys. 2017, 23, 133. [Google Scholar] [CrossRef]
- Fontes, C.J.; Zhang, H.L.; Abdallah, J., Jr.; Clark, R.E.H.; Kilcrease, D.P.; Colgan, J.; Cunningham, R.T.; Hakel, P.; Magee, N.H.; Sherrill, M.E. The Los Alamos suite of relativistic atomic physics codes. J. Phys. B 2015, 48, 144014. [Google Scholar] [CrossRef]
- Magee, N.H.; Abdallah, J.; Colgan, J.; Hakel, P.; Kilcrease, D.P.; Mazevet, S.; Sherrill, M.; Fontes, C.J.; Zhang, H.L. Los Alamos Opacities: Transition from LEDCOP to ATOMIC. AIP Conf. Proc. 2004, 730, 168–179. [Google Scholar]
- Hakel, P.; Sherrill, M.E.; Mazevet, S.; Abdallah, J., Jr.; Colgan, J.; Kilcrease, D.P.; Magee, N.H.; Fontes, C.J.; Zhang, H.L. The new Los Alamos Opacity Code ATOMIC. J. Quant. Spectrosc. Rad. Transf. 2006, 99, 265. [Google Scholar] [CrossRef]
- Abdallah, J.; Clark, R.E.H.; Peek, J.M.; Fontes, C.J. Kinetics calculations for near Ne-like ions. J. Quant. Spectrosc. Rad. Transf. 1994, 51, 1–8. [Google Scholar] [CrossRef]
- Cowan, R.D. The Theory of Atomic Structure and Spectra; University of California Press: Berkeley, CA, USA, 1981. [Google Scholar]
- Mazevet, S.; Abdallah, J., Jr. Mixed UTA and detailed line treatment for mid-Z opacity and spectral calculations. J. Phys. B 2006, 39, 3419. [Google Scholar] [CrossRef]
- Abdallah, J., Jr.; Kilcrease, D.P.; Magee, N.H.; Mazevet, S.; Hakel, P.; Sherrill, M.E. Spectral line strength binning method for opacity calculations. High Energy Dens. Phys. 2007, 3, 309. [Google Scholar] [CrossRef]
- Clark, R.E.H.; Abdallah, J., Jr.; Mann, J.B. Integral and differential cross sections for electron impact ionization. Astrophys. J. 1991, 381, 597. [Google Scholar] [CrossRef]
- Colgan, J.; Kilcrease, D.P.; Magee, N.H., Jr.; Abdallah, J., Jr.; Sherrill, M.E.; Fontes, C.J.; Zhang, H.L.; Hakel, P. Light element opacities of astrophysical interest from ATOMIC. High Energy Dens. Phys. 2015, 14, 33–37. [Google Scholar] [CrossRef] [Green Version]
- Bailey, J.E.; Rochau, G.A.; Iglesias, C.A.; Abdallah, J., Jr.; MacFarlane, J.J.; Golovkin, I.; Wang, P.; Mancini, R.C.; Lake, P.W.; Moore, T.C.; et al. Iron-Plasma Transmission Measurements at Temperatures Above 150 eV. Phys. Rev. Lett. 2007, 99, 265002. [Google Scholar] [CrossRef] [PubMed]
- Bailey, J.E.; Nagayama, T.; Loisel, G.P.; Rochau, G.A.; Blancard, C.; Colgan, J.; Cosse, Ph.; Faussurier, G.; Fontes, C.J.; Gilleron, F.; et al. A higher-than-predicted measurement of iron opacity at solar interior temperatures. Nature 2015, 517, 56–59. [Google Scholar] [CrossRef] [PubMed]
- Heeter, R.F.; Bailey, J.E.; Craxton, R.S.; Devolder, B.G.; Dodd, E.S.; Garcia, E.M.; Huffman, E.J.; Iglesias, C.A.; King, J.A.; Kline, J.L.; et al. Conceptual design of initial opacity experiments on the national ignition facility. J. Plasma Phys. 2017, 83, 595830103. [Google Scholar] [CrossRef]
- Zhang, J.; Li, H.; Zhao, Y.; Xiong, G.; Yuan, Z.; Zhang, H.; Yang, G.; Yang, J.; Liu, S.; Jiang, S.; et al. L- and M-shell absorption measurements of radiatively heated Fe plasma. Phys. Plasmas 2012, 19, 113302. [Google Scholar] [CrossRef]
- Foster, J.M.; Hoarty, D.J.; Smith, C.C.; Rosen, P.A.; Davidson, S.J.; Rose, S.J.; Perry, T.S.; Serduke, F.J.D. L-shell absorption spectrum of an open M-shell germanium plasma: Comparison of experimental data with a detailed configuration-accounting calculation. Phys. Rev. Lett. 1991, 67, 3255. [Google Scholar] [CrossRef] [PubMed]
- Perry, T.S.; Budil, K.S.; Cauble, R.; Ward, R.A.; Bach, D.R.; Iglesias, C.A.; Wilson, B.G.; Nash, J.K.; Smith, C.C.; Foster, J.M.; et al. Quantitative measurement of mid-Z opacities. J. Quant. Spectrosc. Rad. Transf. 1995, 54, 317. [Google Scholar] [CrossRef]
- Bailey, J.E.; Arnault, P.; Blenski, R.; Dejonghe, G.; Peyrusse, O.; MacFarlane, J.J.; Mancini, R.C.; Cuneo, M.E.; Nielsen, D.S.; Rochau, G.A. Opacity measurement of tamped NaBr samples heated by z-pinch X-rays. J. Quant. Spectrosc. Rad. Transf. 2003, 81, 31. [Google Scholar] [CrossRef]
- Kramida, A.; Ralchenko, Y.; Reader, J.; NIST ASD Team. NIST Atomic Spectra Database (ver. 5.5.2). 2018. Available online: http://physics.nist.gov/asd (accessed on 6 March 2018).

© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Colgan, J.; Kilcrease, D.P.; Magee, N.H.; Sherrill, M.E.; Fontes, C.J.; Hakel, P. New Los Alamos Opacity Calculations. Atoms 2018, 6, 32. https://doi.org/10.3390/atoms6020032
Colgan J, Kilcrease DP, Magee NH, Sherrill ME, Fontes CJ, Hakel P. New Los Alamos Opacity Calculations. Atoms. 2018; 6(2):32. https://doi.org/10.3390/atoms6020032
Chicago/Turabian StyleColgan, J., D. P. Kilcrease, N. H. Magee, M. E. Sherrill, C. J. Fontes, and P. Hakel. 2018. "New Los Alamos Opacity Calculations" Atoms 6, no. 2: 32. https://doi.org/10.3390/atoms6020032
APA StyleColgan, J., Kilcrease, D. P., Magee, N. H., Sherrill, M. E., Fontes, C. J., & Hakel, P. (2018). New Los Alamos Opacity Calculations. Atoms, 6(2), 32. https://doi.org/10.3390/atoms6020032




