The Application of the Cut-Off Coulomb Model Potential for the Calculation of Bound-Bound State Transitions
Abstract
:1. Introduction
2. Theory
2.1. The Approximation of the Cut-Off Coulomb Potential
2.2. The Calculated Quantities
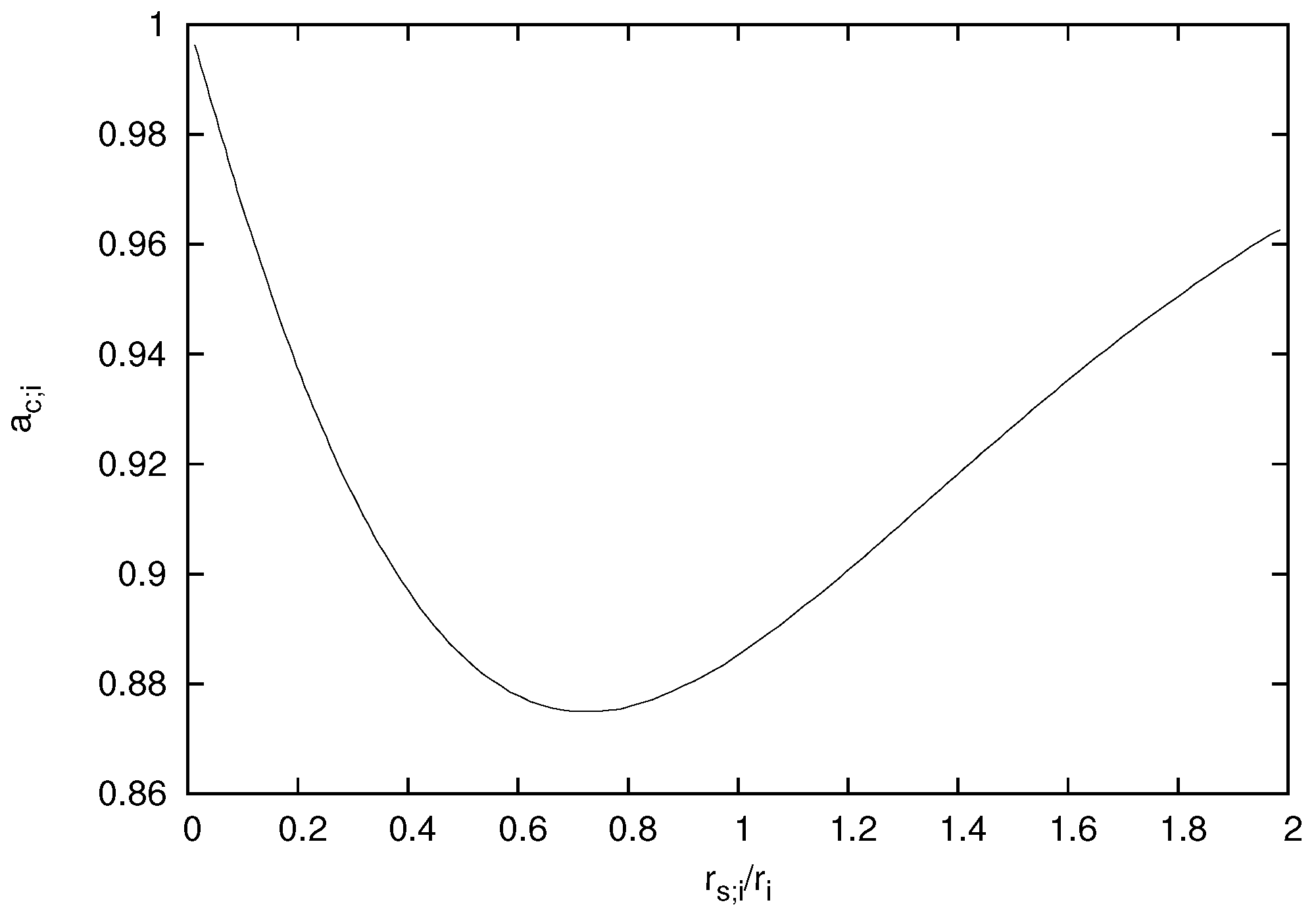
3. Results and Discussion
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Fortov, V.E.; Iakubov, I.T. The Physics of Non-Ideal Plasma; World Scientific: Singapore, 1999. [Google Scholar]
- Rogers, F.J.; Iglesias, C.A. Opacity of stellar matter. Space Sci. Rev. 1998, 85, 61–70. [Google Scholar] [CrossRef]
- Mihajlov, A.A.; Sakan, N.M.; Srećković, V.A.; Vitel, Y. Modeling of continuous absorption of electromagnetic radiation in dense partially ionized plasmas. J. Phys. A 2011, 44, 095502. [Google Scholar] [CrossRef]
- Mihajlov, A.A.; Ignjatović, L.M.; Srećković, V.A.; Dimitrijević, M.S.; Metropoulos, A. The non-symmetric ion-atom radiative processes in the stellar atmospheres. Mon. Not. R. Astron. Soc. 2013, 431, 589–599. [Google Scholar] [CrossRef]
- Mihajlov, A.A.; Djordjević, D.; Popović, M.M.; Meyer, T.; Luft, M.; Kraeft, W.D. Determination of the Electrical Conductivity of a Plasma on the Basis of the Coulomb cut-off Potential Model. Contrib. Plasma Phys. 1989, 29, 441–446. [Google Scholar] [CrossRef]
- Ignjatović, L.M.; Srećković, V.A.; Dimitrijević, M.S. The Screening Characteristics of the Dense Astrophysical Plasmas: The Three-Component Systems. Atoms 2017, 5, 42. [Google Scholar] [CrossRef]
- Mihajlov, A.A.; Sakan, N.M.; Srećković, V.A.; Vitel, Y. Modeling of the Continuous Absorption of Electromagnetic Radiation in Dense Hydrogen Plasma. Balt. Astron. 2011, 20, 604–608. [Google Scholar] [CrossRef]
- Mihajlov, A.A.; Srećković, V.A.; Sakan, N.M. Inverse Bremsstrahlung in Astrophysical Plasmas: The Absorption Coefficients and Gaunt Factors. J. Astrophys. Astron. 2015, 36, 635–642. [Google Scholar] [CrossRef]
- Sakan, N.M.; Srećković, V.A.; Mihajlov, A.A. The application of the cut-off Coulomb potential for the calculation of a continuous spectra of dense hydrogen plasma. Mem. Soc. Astron. Ital. Suppl. 2005, 7, 221–224. [Google Scholar]
- Mihajlov, A.A.; Vitel, Y.; Ignjatović, L.M. The new screening characteristics of strongly non-ideal and dusty plasmas. Part 1: Single-component systems. High Temp. 2008, 46, 737–745. [Google Scholar] [CrossRef]
- Mihajlov, A.A.; Vitel, Y.; Ignjatović, L.M. The new screening characteristics of strongly non-ideal and dusty plasmas. Part 2: Two-component systems. High Temp. 2009, 47, 1–12. [Google Scholar] [CrossRef]
- Mihajlov, A.; Vitel, Y.; Ignjatović, L.M. The new screening characteristics of strongly non-ideal and dusty plasmas. Part 3: Properties and applications. High Temp. 2009, 47, 147–157. [Google Scholar] [CrossRef]
- Demura, A. Physical models of plasma microfield. Int. J. Spectrosc. 2009, 2010, 671073. [Google Scholar] [CrossRef]
- Vitel, Y.; Gavrilova, T.; D’yachkov, L.; Kurilenkov, Y.K. Spectra of dense pure hydrogen plasma in Balmer area. J. Quant. Spectrosc. Radiat. Transf. 2004, 83, 387–405. [Google Scholar] [CrossRef]
- Griem, H.R. Principles of Plasma Spectroscopy; Cambridge University Press: Cambridge, UK, 2005; Volume 2. [Google Scholar]
- Konjević, N.; Ivković, M.; Sakan, N. Hydrogen Balmer lines for low electron number density plasma diagnostics. Spectrochim. Acta B 2012, 76, 16–26. [Google Scholar] [CrossRef]
- Sobelman, I.I. Atomic spectra and radiative transitions. In Springer Series in Chemical Physics; Springer: Berlin, Germany, 1979. [Google Scholar]
- Hoang-Binh, D. A program to compute exact hydrogenic radial integrals, oscillator strengths, and Einstein coefficients, for principal quantum numbers up to n ≈ 1000. Comput. Phys. Commun. 2005, 166, 191–196. [Google Scholar] [CrossRef]
- Hilborn, R.C. Einstein coefficients, cross sections, f values, dipole moments, and all that. Am. J. Phys. 1982, 50, 982–986. [Google Scholar] [CrossRef]
- Stambulchik, E.; Maron, Y. Plasma line broadening and computer simulations: A mini-review. High Energ. Dens. Phys. 2010, 6, 9–14. [Google Scholar] [CrossRef]
- Stambulchik, E.; Fisher, D.; Maron, Y.; Griem, H.; Alexiou, S. Correlation effects and their influence on line broadening in plasmas: Application to Hα. High Energ. Dens. Phys. 2007, 3, 272–277. [Google Scholar] [CrossRef]
- Talin, B.; Dufour, E.; Calisti, A.; Gigosos, M.A.; Gonzalez, M.A.; Gaztelurrutia, T.D.R.; Dufty, J.W. Molecular dynamics simulation for modeling plasma spectroscopy. J. Phys. A 2003, 36, 6049. [Google Scholar] [CrossRef]
- Ferri, S.; Calisti, A.; Mossé, C.; Rosato, J.; Talin, B.; Alexiou, S.; Gigosos, M.A.; González, M.A.; González-Herrero, D.; Lara, N.; et al. Ion Dynamics Effect on Stark-Broadened Line Shapes: A Cross-Comparison of Various Models. Atoms 2014, 2, 299–318. [Google Scholar] [CrossRef]
- Calisti, A.; Demura, A.V.; Gigosos, M.A.; González-Herrero, D.; Iglesias, C.A.; Lisitsa, V.S.; Stambulchik, E. Influence of microfield directionality on line shapes. Atoms 2014, 2, 259–276. [Google Scholar] [CrossRef]
- Alexiou, S.; Dimitrijević, M.S.; Sahal-Brechot, S.; Stambulchik, E.; Duan, B.; González-Herrero, D.; Gigosos, M.A. The second workshop on lineshape code comparison: Isolated lines. Atoms 2014, 2, 157–177. [Google Scholar] [CrossRef]
- Marinković, B.P.; Jevremović, D.; Srećković, V.A.; Vujčić, V.; Ignjatović, L.M.; Dimitrijević, M.S.; Mason, N.J. BEAMDB and MolD—Databases for atomic and molecular collisional and radiative processes: Belgrade nodes of VAMDC. Eur. Phys. J. D 2017, 71, 158. [Google Scholar] [CrossRef]
- Srećković, V.A.; Ignjatović, L.M.; Jevremović, D.; Vujčić, V.; Dimitrijević, M.S. Radiative and Collisional Molecular Data and Virtual Laboratory Astrophysics. Atoms 2017, 5, 31. [Google Scholar] [CrossRef]
- Sadykova, S.; Ebeling, W.; Valuev, I.; Sokolov, I. Electric Microfield Distributions in Li + Plasma With Account of the Ion Structure. Contrib. Plasma Phys. 2009, 49, 76–89. [Google Scholar] [CrossRef]
- Calisti, A.; Ferri, S.; Mossé, C.; Talin, B.; Gigosos, M.; González, M. Microfields in hot dense hydrogen plasmas. High Energ. Dens. Phys. 2011, 7, 197–202. [Google Scholar] [CrossRef]
- Kramida, A.; Ralchenko, Y.; Reader, J.; NIST ASD Team. NIST Atomic Spectra Database (Ver. 5.5); National Institute of Standards and Technology: Gaithersburg, MD, USA, 2017. Available online: https://physics.nist.gov/asd (accessed on 30 October 2017).
- Sakan, N.M. The Calculation of the Photo Absorption Processes in Dense Hydrogen Plasma with the Help of Cut-Off Coulomb Potential Model. J. Phys. Conf. Ser. 2010, 257, 012036. [Google Scholar] [CrossRef]
- Sahal-Bréchot, S.; Dimitrijević, M.; Moreau, N.; Nessib, N.B. The STARK-B database VAMDC node: A repository for spectral line broadening and shifts due to collisions with charged particles. Phys. Scr. 2015, 90, 054008. [Google Scholar] [CrossRef]
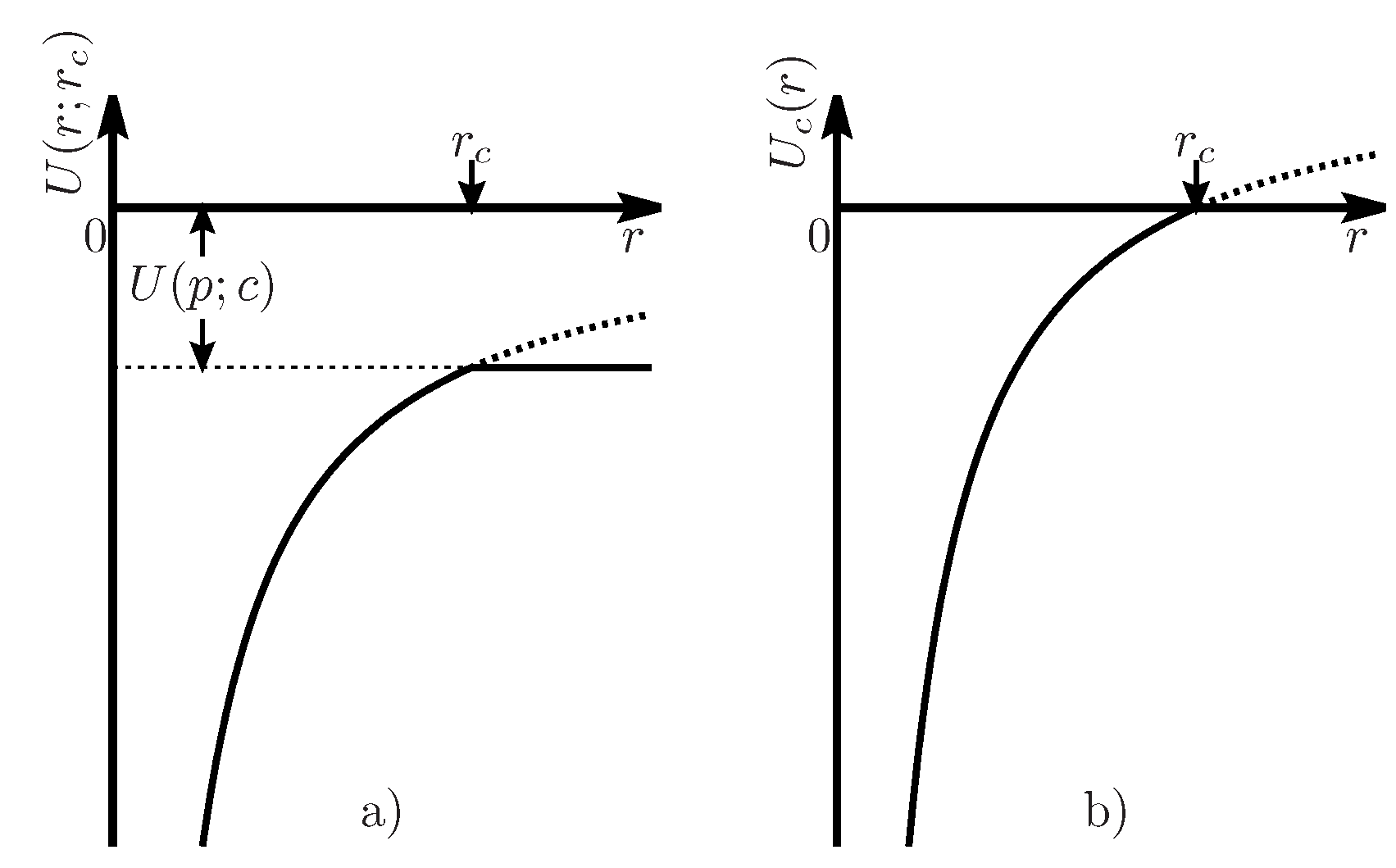
| Short Pulse | Long Pulse | Theory | NIST | ||
|---|---|---|---|---|---|
| |1,0> | |2,1> | 0.416197 | 0.416197 | 0.416200 | 0.416400 |
| |1,0> | |3,1> | 0.079102 | 0.079102 | 0.079101 | 0.079120 |
| |1,0> | |4,1> | 0.028923 | 0.028991 | 0.028991 | 0.029010 |
| |1,0> | |5,1> | 0.013728 | 0.013938 | 0.013950 | |
| |2,0> | |3,1> | 0.434865 | 0.434865 | 0.434870 | 0.435100 |
| |2,0> | |4,1> | 0.102533 | 0.102756 | 0.102760 | 0.102800 |
| |2,0> | |5,1> | 0.041425 | 0.041930 | 0.041950 | |
| |2,1> | |3,0> | 0.013589 | 0.013589 | 0.013590 | 0.013600 |
| |2,1> | |3,2> | 0.695785 | 0.695785 | 0.695780 | 0.696100 |
| |2,1> | |4,0> | 0.003035 | 0.003045 | 0.003045 | 0.003046 |
| |2,1> | |4,2> | 0.121659 | 0.121795 | 0.121800 | 0.102800 |
| |2,1> | |5,0> | 0.001191 | 0.001213 | 0.001214 | |
| |2,1> | |5,2> | 0.043962 | 0.044371 | 0.044400 | |
| |3,0> | |4,1> | 0.483750 | 0.484708 | 0.484710 | 0.484900 |
| |3,0> | |5,1> | 0.119310 | 0.121020 | 0.121100 | |
| |3,1> | |4,0> | 0.032165 | 0.032250 | 0.032250 | 0.032280 |
| |3,1> | |4,2> | 0.617675 | 0.618282 | 0.618290 | 0.618600 |
| |3,1> | |5,0> | 0.007299 | 0.007428 | 0.007433 | |
| |3,1> | |5,2> | 0.138013 | 0.139230 | 0.139300 | |
| |3,2> | |4,1> | 0.010971 | 0.010992 | 0.010992 | 0.011000 |
| |3,2> | |4,3> | 1.017260 | 1.017520 | 1.017500 | 1.018000 |
| |3,2> | |5,1> | 0.002180 | 0.002210 | 0.002211 | |
| |3,2> | |5,3> | 0.156046 | 0.156640 | 0.156700 | |
| |4,0> | |5,1> | 0.537527 | 0.544150 | 0.544400 | |
| |4,1> | |5,0> | 0.052123 | 0.052907 | 0.052940 | |
| |4,1> | |5,2> | 0.604678 | 0.609290 | 0.609700 | |
| |4,2> | |5,1> | 0.027498 | 0.027822 | 0.027840 | |
| |4,2> | |5,3> | 0.887328 | 0.890250 | 0.890600 | |
| |4,3> | |5,2> | 0.008809 | 0.008871 | 0.008877 | |
| |4,3> | |5,4> | 1.344790 | 1.345800 | 1.346000 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sakan, N.M.; Srećković, V.A.; Simić, Z.J.; Dimitrijević, M.S. The Application of the Cut-Off Coulomb Model Potential for the Calculation of Bound-Bound State Transitions. Atoms 2018, 6, 4. https://doi.org/10.3390/atoms6010004
Sakan NM, Srećković VA, Simić ZJ, Dimitrijević MS. The Application of the Cut-Off Coulomb Model Potential for the Calculation of Bound-Bound State Transitions. Atoms. 2018; 6(1):4. https://doi.org/10.3390/atoms6010004
Chicago/Turabian StyleSakan, Nenad M., Vladimir A. Srećković, Zoran J. Simić, and Milan S. Dimitrijević. 2018. "The Application of the Cut-Off Coulomb Model Potential for the Calculation of Bound-Bound State Transitions" Atoms 6, no. 1: 4. https://doi.org/10.3390/atoms6010004
APA StyleSakan, N. M., Srećković, V. A., Simić, Z. J., & Dimitrijević, M. S. (2018). The Application of the Cut-Off Coulomb Model Potential for the Calculation of Bound-Bound State Transitions. Atoms, 6(1), 4. https://doi.org/10.3390/atoms6010004







