Electron Impact Excitation and Dielectronic Recombination of Highly Charged Tungsten Ions
Abstract
:1. Introduction
2. Theoretical Background
2.1. Electron Impact Excitation and Dielectronic Recombination Cross-Sections
2.2. Degree of Linear Polarization of Radiated X-ray Emissions
2.3. Dielectronic Recombination Rate Coefficients
3. Results and Discussion
3.1. Excitation Energies and Electron-Impact Excitation Cross-Sections

| Ions | Upper Level → Lower level | Present | Theo. [71] | Theo. [72] | Exp. [73] |
|---|---|---|---|---|---|
| W | → | 2111.59 | 2112.57 | ||
| → | 2179.97 | 2181.36 | |||
| → | 2814.39 | 2816.1(3) | |||
| → | 2876.70 | 2878.2(3) | |||
| → | 3194.47 | 3196.8(3) | |||
| → | 3257.73 | 3259.9(3) | |||
| W | → | 2098.36 | 2094.9 | 2094.8(5) | |
| → | 2166.84 | 2169.8 | |||
| → | 2782.97 | 2783.1 | |||
| → | 2843.69 | 2844.7 | |||
| → | 3149.21 | 3151.9 | |||
| → | 3211.78 | 3214.7 | |||
| W | → | 2085.38 | 2082.6 | 2082.2(4) | |
| → | 2152.70 | 2159.0 | |||
| → | 2751.51 | 2750.2 | 2750.3(10) | ||
| → | 2813.45 | 2811.6 | |||
| → | 3104.45 | 3107.9 | 3107.8(5) | ||
| → | 3167.36 | 3171.0 | 3171.2(6) | ||
| W | → | 2067.96 | |||
| → | 2136.12 | 2140.3 | |||
| → | 2715.49 | 2718.1 | |||
| → | 2780.92 | 2781.1 | |||
| → | 3060.51 | 3062.1 | |||
| → | 3124.77 | 3126.7 | |||
| W | → | 2054.00 | |||
| → | 2119.98 | ||||
| → | 2683.09 | ||||
| → | 2748.89 | ||||
| → | 3016.27 | ||||
| → | 3080.31 |
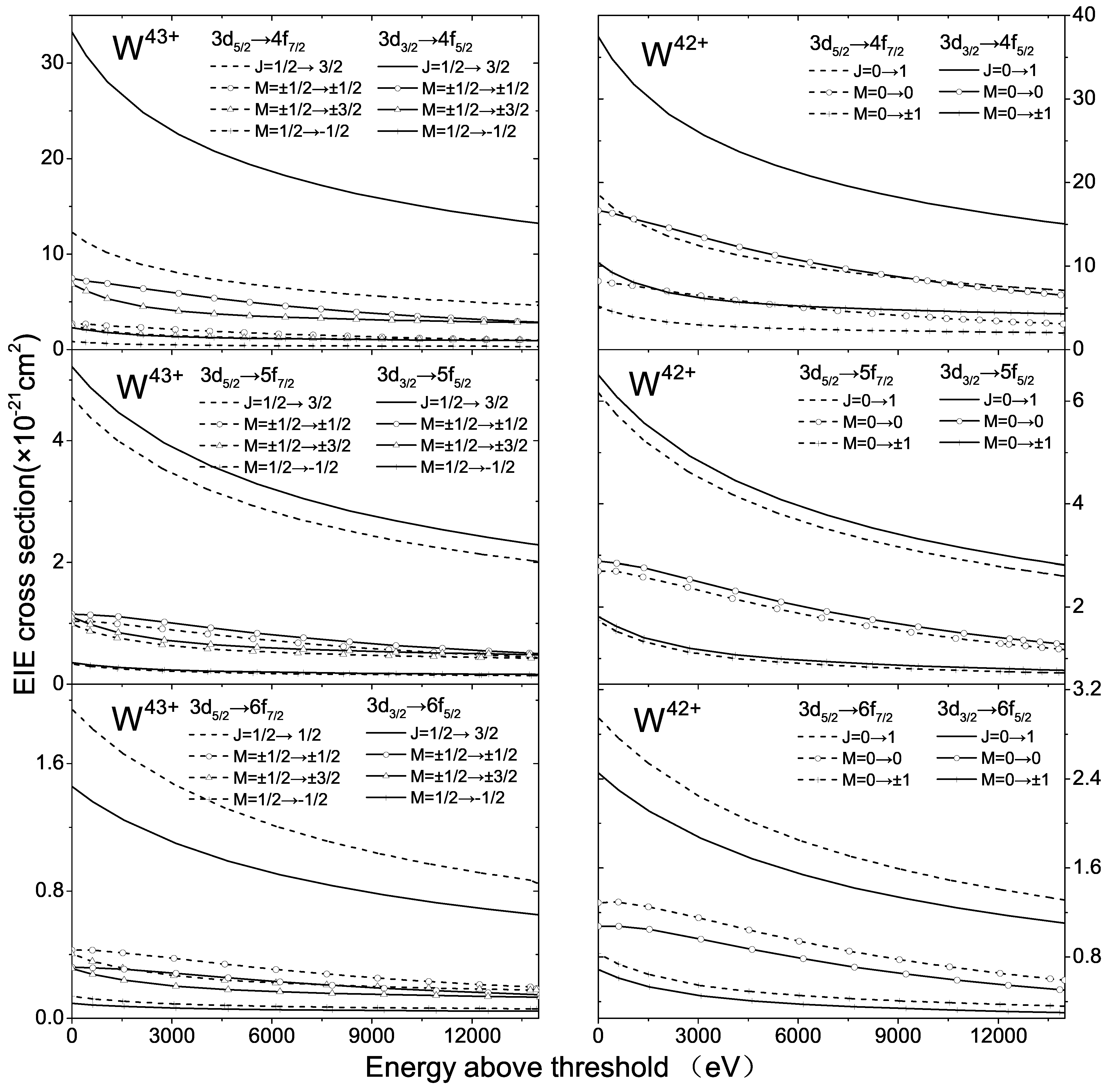
3.2. Resonant Energies and Resonant Electron Capture Cross-Sections
| Ions | Initial Level → Final Level | Magnetic Sub-level CS | Total CS | |||
|---|---|---|---|---|---|---|
| W | ||||||
| → | 363.89 | 21.1 | 0 | 42.2 | ||
| → | 425.39 | 28.0 | 0 | 56.0 | ||
| → | 732.42 | 5.12 | 0 | 10.2 | ||
| → | 794.99 | 5.97 | 0 | 11.9 | ||
| W | ||||||
| → | 398.44 | 11.8 | 0 | 0.26 | 12.1 | |
| → | 460.17 | 12.9 | 0 | 1.19 | 14.1 | |
| → | 754.40 | 3.02 | 0 | 0.10 | 3.12 | |
| → | 817.30 | 2.79 | 0 | 0.34 | 3.16 | |
| W | ||||||
| → | 513.23 | 1.06 | 0 | 2.12 | ||
| → | 575.84 | 0.64 | 0 | 1.28 | ||
| → | 856.78 | 0.10 | 0 | 0.20 | ||
| → | 921.03 | 0.01 | 0 | 0.02 | ||
| W | ||||||
| → | 536.81 | 2.53 | 0 | 1.11 | 3.64 | |
| → | 598.89 | 3.00 | 0 | 2.08 | 5.08 | |
| → | 868.14 | 0.69 | 0 | 0.56 | 1.24 | |
| → | 931.56 | 0.68 | 0 | 0.85 | 1.53 | |
3.3. Degrees of Linear Polarization of Subsequently Radiated X-Ray Emissions
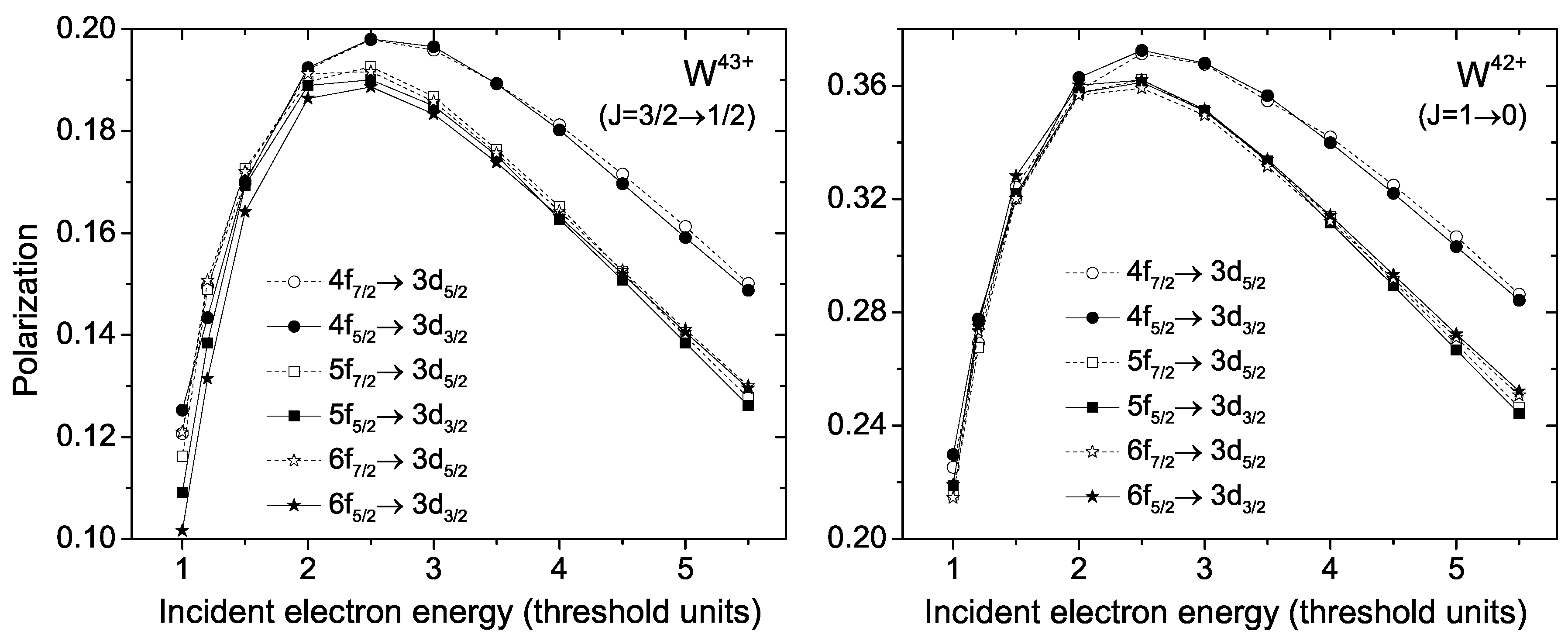
| Ions | Transitions | Polarization |
|---|---|---|
| W | → | 0.60 |
| → | 0.60 | |
| → | 0.60 | |
| → | 0.60 | |
| W | → | 0.98 |
| → | 0.91 | |
| → | 0.97 | |
| → | 0.89 | |
| W | → | 0.60 |
| → | 0.60 | |
| → | 0.60 | |
| → | 0.60 | |
| W | → | 0.64 |
| → | 0.49 | |
| → | 0.42 | |
| → | 0.23 |
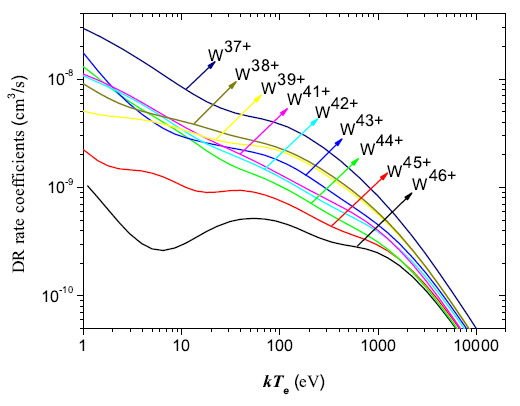
3.4. Dielectronic Recombination Rate Coefficients

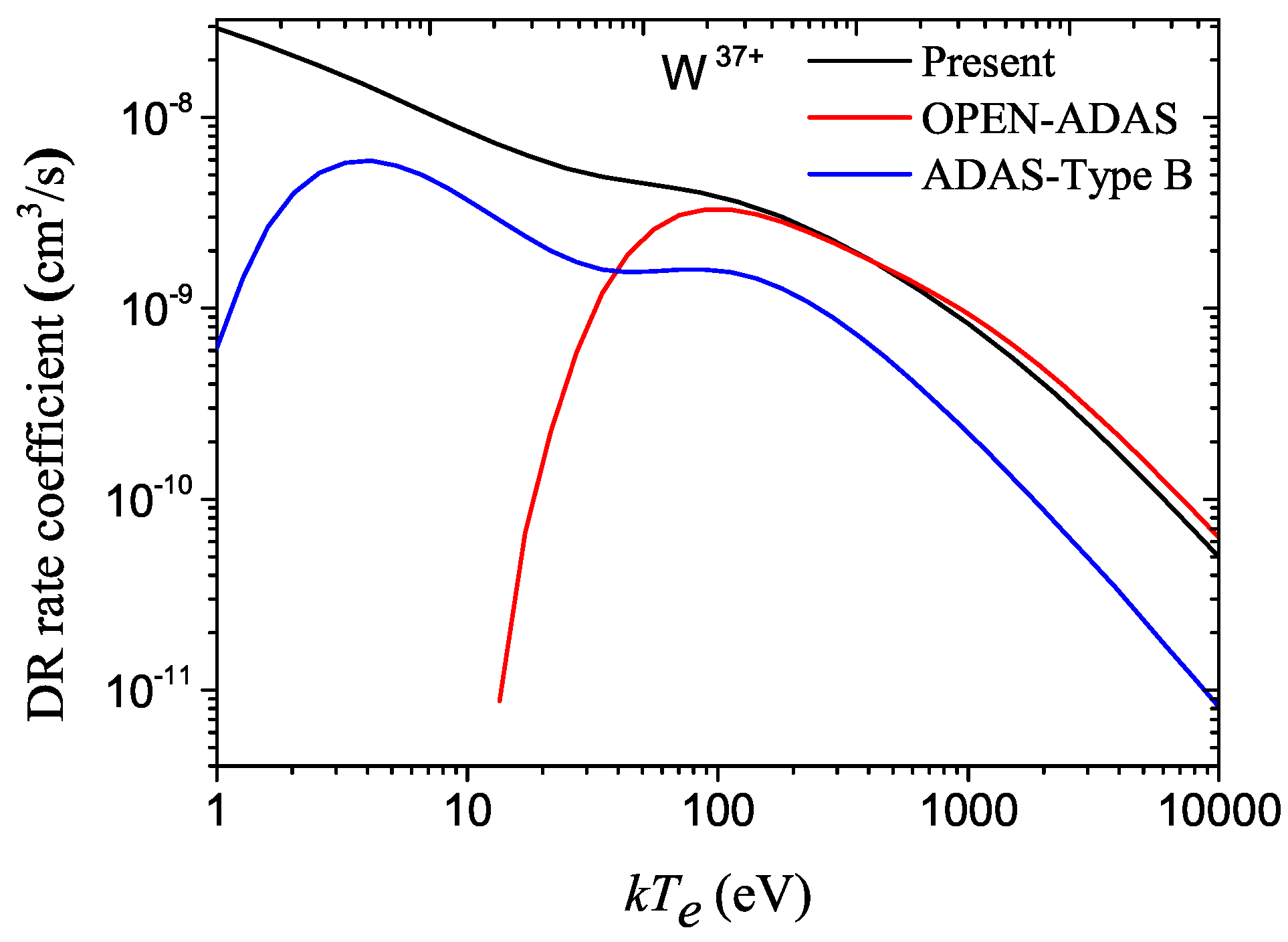
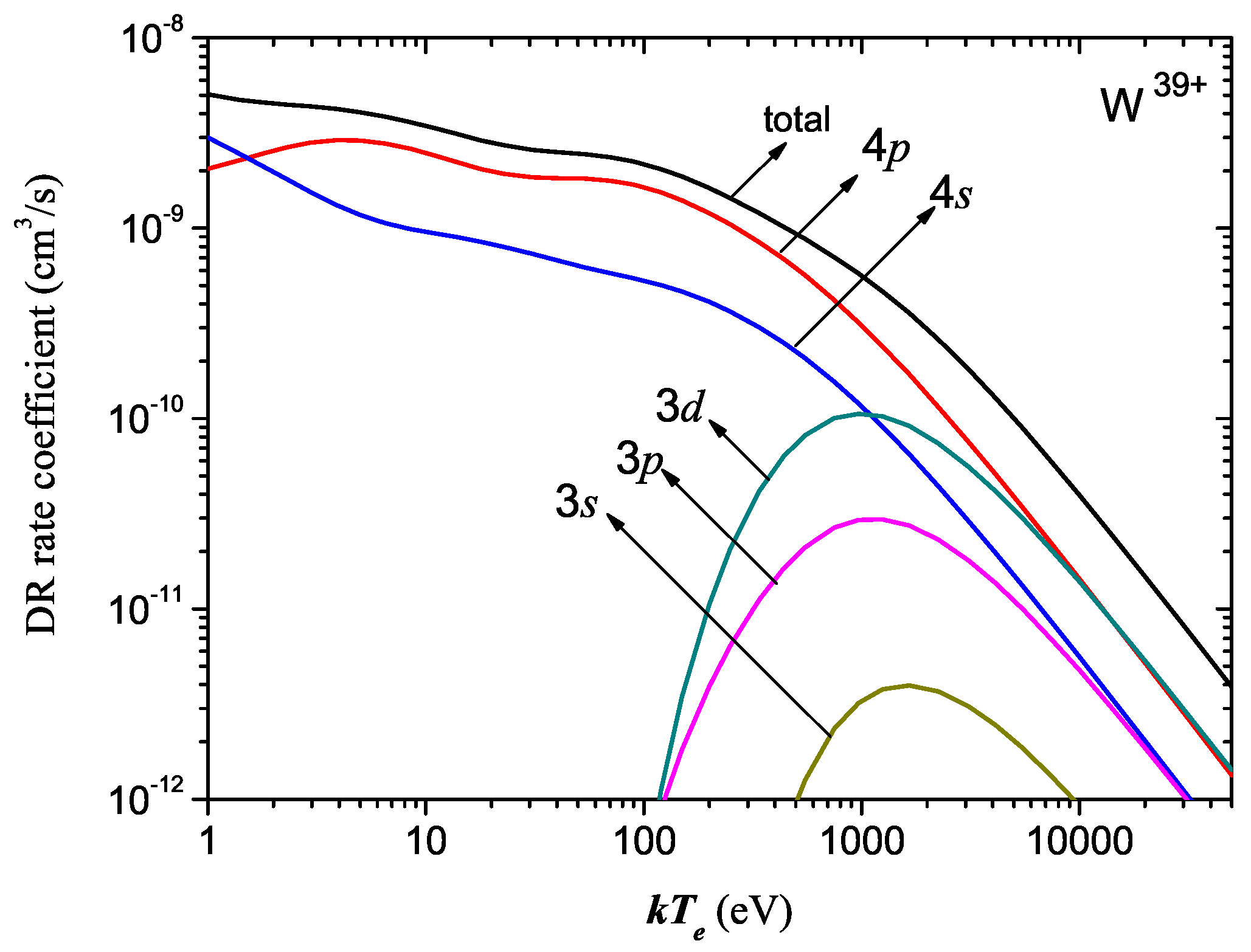
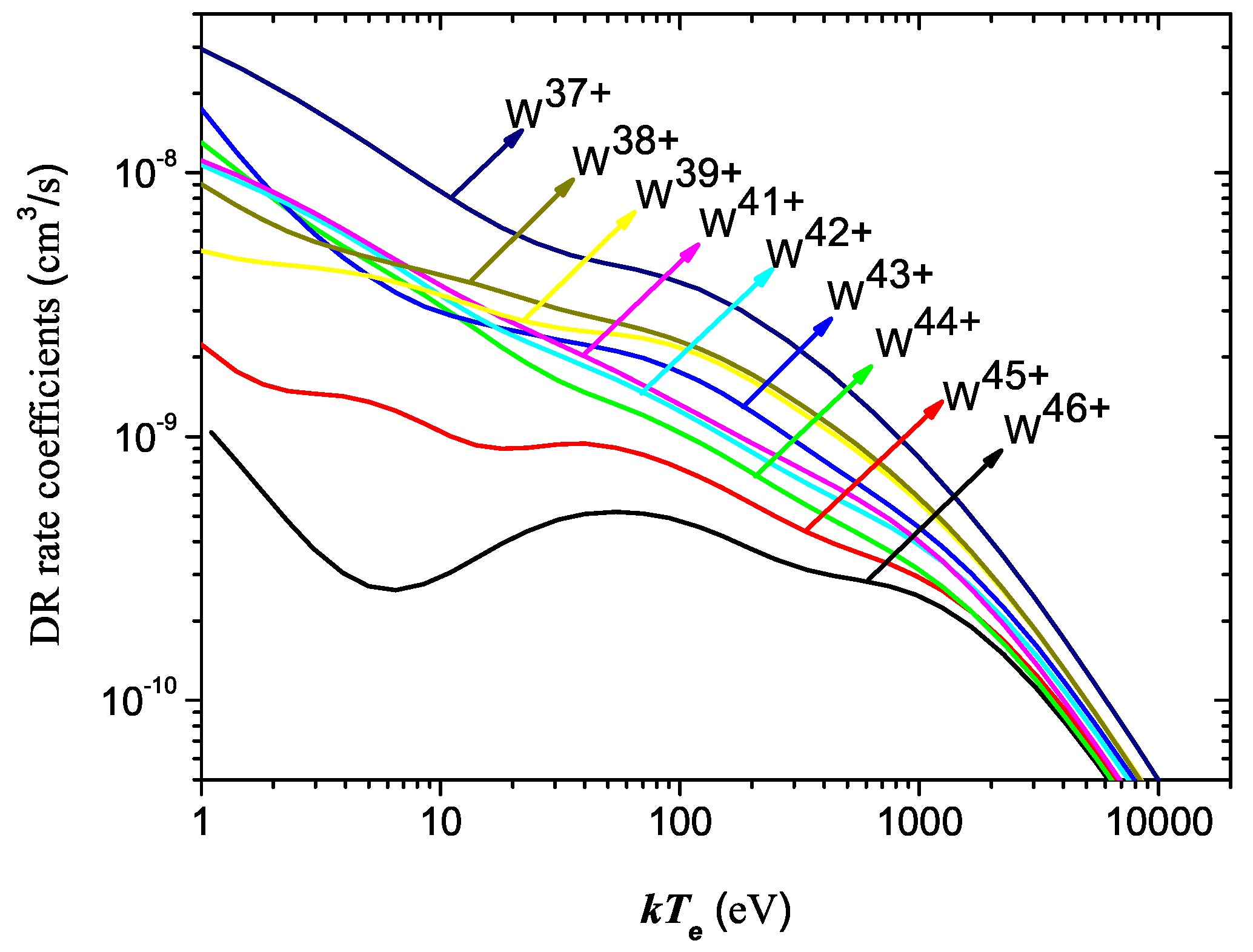
| Fitting | W | W | W | W | W | W | W | W | W |
|---|---|---|---|---|---|---|---|---|---|
| 1.4543[−8] | 2.6717[−7] | 1.1569[−6] | 4.1371[−7] | 1.6944[−6] | 1.6014[−7] | 6.0746[−7] | 1.9070[−7] | 3.9007[−7] | |
| 8.3243[0] | 7.0342[0] | 9.0374[1] | 7.7850[0] | 1.1615[2] | 5.0613[0] | 3.3713[1] | 6.5731[0] | 5.1508[0] | |
| 1.9915[−7] | 4.0559[−7] | 7.4592[−6] | 1.6914[−6] | 4.4742[−6] | 4.8517[−6] | 1.7700[−7] | 7.7977[−7] | 1.2141[−6] | |
| 4.2241[1] | 3.2974[1] | 7.1384[2] | 5.9659[1] | 3.4936[2] | 3.3090[2] | 6.9936[0] | 3.2360[1] | 3.5521[1] | |
| 6.7361[−7] | 2.6009[−6] | 2.5384[−7] | 4.3113[−6] | 6.2817[−7] | 1.6915[−6] | 5.7629[−6] | 2.9429[−6] | 5.0545[−6] | |
| 1.0717[2] | 1.0912[2] | 2.2324[1] | 1.8934[2] | 4.0746[1] | 1.0235[2] | 2.2787[2] | 1.0602[2] | 1.0896[2] | |
| 1.7027[−6] | 5.9321[−6] | 1.0583[−7] | 9.6379[−6] | 1.7999[−5] | 1.2484[−5] | 2.9373[−6] | 6.7028[−6] | 1.0545[−5] | |
| 2.7908[2] | 2.7452[2] | 3.8774[0] | 5.9801[2] | 2.1950[3] | 9.1731[2] | 9.8938[1] | 2.5447[2] | 2.4496[2] | |
| 2.0410[−5] | 2.1212[−5] | 2.3367[−6] | 2.8462[−5] | 1.7095[−7] | 1.4246[−5] | 2.2508[−5] | 1.2033[−5] | 1.5455[−5] | |
| 2.0661[3] | 9.0060[2] | 2.1982[2] | 1.7244[3] | 5.6003[0] | 1.9728[3] | 1.7514[3] | 6.7877[2] | 6.4479[2] | |
| 8.3537[−6] | 4.9140[−5] | 2.0007[−5] | 1.4323[−5] | 5.2788[−7] | 1.1814[−5] | 1.9456[−5] | 2.1248[−5] | ||
| 9.7091[2] | 1.9984[3] | 1.9094[3] | 1.0829[3] | 3.1933[1] | 6.5898[2] | 1.7055[3] | 1.6974[3] |
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Causey, R.; Wilson, K.; Venhaus, T.; Wampler, W.R. Tritium retention in tungsten exposed to intense fluxes of 100 eV tritons. J. Nucl. Mater. 1999, 266–269, 467–471. [Google Scholar] [CrossRef]
- Neu, R.; Asmussen, K.; Krieger, K.; Thoma, A.; Bosch, H.-S.; Deschka, S.; Dux, R.; Engelhardt, W.; Garcia-Rosales, C.; Gruber, O.; et al. The tungsten divertor experiment at ASDEX Upgrade. Plasma Phys. Control. Fus. 1996, 38, A165–A179. [Google Scholar] [CrossRef]
- Neu, R.; Bobkov, V.; Dux, R.; Fuchs, J.C.; Gruber, O.; Herrmann, A.; Kallenbach, A.; Maier, H.; Mayer, M.; Pütterich, T.; et al. Ten years of W programme in ASDEX Upgrade—Challenges and conclusions. Phys. Scr. 2009, 2009, 014038. [Google Scholar] [CrossRef]
- Rohde, V.; Balden, M.; Lunt, T.; the ASDEX Upgrade Team. Dust investigations at ASDEX Upgrade. Phys. Scr. 2009, 2009, 014024. [Google Scholar] [CrossRef]
- Riccardo, V.; Firdaouss, M.; Joffrin, E.; Matthews, G.; Mertens, P.H.; Thompson, V.; Villedieu, E. Operational limits for the ITER-like wall in JET. Phys. Scr. 2009, 2009, 014033. [Google Scholar] [CrossRef]
- Reinke, M.L.; Beiersdorfer, P.; Howard, N.T.; Magee, E.W.; Podpaly, Y.; Rice, J.E.; Terry, J.L. Vacuum ultraviolet impurity spectroscopy on the Alcator C-Mod tokamak. Rev. Sci. Instrum. 2010, 81, 10D736. [Google Scholar] [CrossRef] [PubMed]
- Neu, R.; Dux, R.; Kallenbach, A.; Pütterich, T.; Balden, M.; Fuchs, J.C.; Herrmann, A.; Maggi, C.F.; O’Mullane, M.; Pugno, R.; et al. Tungsten: An option for divertor and main chamber plasma facing components in future fusion devices. Nucl. Fus. 2005, 45, 209–218. [Google Scholar] [CrossRef]
- Skinner, C.H. Applications of EBIT to magnetic fusion diagnostics. Can. J. Phys. 2008, 86, 285–290. [Google Scholar] [CrossRef]
- Reader, J. Spectral data for fusion energy: From W to W. Phys. Scr. 2009, 2009, 014023. [Google Scholar] [CrossRef]
- Kramida, A.E.; Shirai, T. Energy levels and spectral lines of tungsten, W III through W LXXIV. At. Data Nucl. Data Tables 2009, 95, 305–474. [Google Scholar] [CrossRef]
- Kramida, A.E.; Shirai, T. Erratum to “Energy levels and spectral lines of tungsten, W III through W LXXIV” [Atomic Data and Nuclear Data Tables 95 (2009) 305–474]. At. Data Nucl. Data Tables 2009, 95, 1051. [Google Scholar] [CrossRef]
- Kramida, A. Recent progress in spectroscopy of tungsten. Can. J. Phys. 2011, 89, 551–570. [Google Scholar] [CrossRef]
- Clementson, J.; Beiersdorfer, P.; Brown, G.V.; Gu, M.F.; Lundberg, H.; Podpaly, Y.; Träbert, E. Tungsten spectroscopy at the Livermore electron beam ion trap facility. Can. J. Phys. 2011, 89, 571–580. [Google Scholar] [CrossRef]
- Müller, A. Fusion-related ionization and recombination data for tungsten ions in low to moderately high charge states. Atoms 2015, 3, 120–161. [Google Scholar] [CrossRef]
- Utter, S.B.; Beiersdorfer, P.; Träbert, E. Electron-beam ion-trap spectra of tungsten in the EUV. Can. J. Phys. 2002, 80, 1503–1515. [Google Scholar] [CrossRef]
- Neill, P.; Harris, C.; Safronova, A.S.; Hamasha, S.; Hansen, S.; Safronova, U.I.; Beiersdorfer, P. The study of X-ray M-shell spectra of W ions from the Lawrence Livermore National Laboratory Electron Beam Ion Trap. Can. J. Phys. 2004, 82, 931–942. [Google Scholar] [CrossRef]
- Pütterich, T.; Neu, R.; Biedermann, C.; Radtke, R.; ASDEX Upgrade Team. Disentangling the emissions of highly ionized tungsten in the range 4–14 nm. J. Phys. B 2005, 38, 3071–3082. [Google Scholar] [CrossRef]
- Ralchenko, Y.; Tan, J.N.; Gillaspy, J.D.; Pomeroy, J.M.; Silver, E. Accurate modeling of benchmark X-ray spectra from highly charged ions of tungsten. Phys. Rev. A 2006, 74, 042514. [Google Scholar] [CrossRef]
- Ralchenko, Y.; Reader, J.; Pomeroy, J.M.; Tan, J.N.; Gillaspy, J.D. Spectra of W39+–W47+ in the 12–20 nm region observed with an EBIT light source. J. Phys. B 2007, 40, 3861–3875. [Google Scholar] [CrossRef]
- Biedermann, C.; Radtke, R.; Seidel, R.; Pütterich, T. Spectroscopy of highly charged tungsten ions relevant to fusion plasmas. Phys. Scr. 2009, 2009, 014026. [Google Scholar] [CrossRef]
- Harte, C.S.; Suzuki, C.; Kato, T.; Sakaue, H.A.; Kato, D.; Sato, K.; Tamura, N.; Sudo, S.; D’Arcy, R.; Sokell, E.; et al. Tungsten spectra recorded at the LHD and comparison with calculations. J. Phys. B 2010, 43, 205004. [Google Scholar] [CrossRef]
- Fournier, K.B. Atomic data and spectral line intensities for highly ionized tungsten (Co-like W47+ to Rb-like W37+) in a high-temperature, low-density plasma. At. Data Nucl. Data Tables 1998, 68, 1–48. [Google Scholar] [CrossRef]
- Safronova, U.I.; Safronova, A.S. Wavelengths and transition rates for nl-n′l′ transitions in Be-, B-, Mg-, Al-, Ca-, Zn-, Ag- and Yb-like tungsten ions. J. Phys. B 2010, 43, 074026. [Google Scholar] [CrossRef]
- Quinet, P. Dirac-Fock calculations of forbidden transitions within the 3pk and 3dk ground configurations of highly charged tungsten ions (W47+–W61+). J. Phys. B 2011, 44, 195007. [Google Scholar] [CrossRef]
- Quinet, P. A theoretical survey of atomic structure and forbidden transitions in the 4pk and 4dk ground configurations of tungsten ions W29+ through W43+. J. Phys. B 2012, 45, 025003. [Google Scholar] [CrossRef]
- Loch, S.D.; Ludlow, J.A.; Pindzola, M.S.; Whiteford, A.D.; Griffin, D.C. Electron-impact ionization of atomic ions in the W isonuclear sequence. Phys. Rev. A 2005, 72, 052716. [Google Scholar] [CrossRef]
- Ballance, C.P.; Griffin, D.C. Relativistic radiatively damped R-matrix calculation of the electron-impact excitation of W46+. J. Phys. B 2006, 39, 3617–3628. [Google Scholar] [CrossRef]
- Ballance, C.P.; Griffin, D.C. Electron-impact excitation of W44+ and W45+. J. Phys. B 2007, 40, 247–258. [Google Scholar] [CrossRef]
- Behar, E.; Peleg, A.; Doron, R.; Mandelbaum, P.; Schwob, J.L. Dielectronic recombination of Ni-, Cu-, and Ar-like tungsten and Barium through the low inner-shell excited configurations including collision processes. J. Quant. Spectrosc. Radiat. Transf. 1997, 58, 449–469. [Google Scholar] [CrossRef]
- Takács, E.; Meyer, E.S.; Gillaspy, J.D.; Roberts, J.R.; Chantler, C.T.; Hudson, L.T.; Deslattes, R.D.; Brown, C.M.; Laming, J.M.; Dubau, J.; et al. Polarization measurements on a magnetic quadrupole line in Ne-like barium. Phys. Rev. A 1996, 54, 1342–1350. [Google Scholar] [CrossRef] [PubMed]
- Reed, K.J.; Chen, M.H. Relativistic effects on the polarization of line radiation emitted from He-like and H-like ions following electron-impact excitation. Phys. Rev. A 1993, 48, 3644–3651. [Google Scholar] [CrossRef] [PubMed]
- Fritzsche, S.; Surzhykov, A.; Stöhlker, T. Dominance of the Breit interaction in the X-ray emission of highly charged ions following dielectronic recombination. Phys. Rev. Lett. 2009, 103, 113001. [Google Scholar] [CrossRef] [PubMed]
- Hu, Z.M.; Han, X.Y.; Li, Y.M.; Kato, D.; Tong, X.M.; Nakamura, N. Experimental demonstration of the Breit interaction which dominates the angular distribution of X-ray emission in dielectronic recombination. Phys. Rev. Lett. 2012, 108, 073002. [Google Scholar] [CrossRef] [PubMed]
- Weber, G.; Bräuning, H.; Surzhykov, A.; Brandau, C.; Fritzsche, S.; Geyer, S.; Hagmann, S.; Hess, S.; Kozhuharov, C.; Märtin, R.; et al. Direct determination of the magnetic quadrupole contribution to the Lyman-α1 transition in a hydrogenlike ion. Phys. Rev. Lett. 2010, 105, 243002. [Google Scholar] [CrossRef] [PubMed]
- Wu, Z.W.; Kabachnik, N.M.; Surzhykov, A.; Dong, C.Z.; Fritzsche, S. Determination of small level splittings in highly charged ions via angle-resolved measurements of characteristic X-rays. Phys. Rev. A 2014, 90, 052515. [Google Scholar] [CrossRef]
- Wu, Z.W.; Jiang, J.; Dong, C.Z. Influence of Breit interaction on the polarization of radiation following inner-shell electron-impact excitation of highly charged berylliumlike ions. Phys. Rev. A 2011, 84, 032713. [Google Scholar] [CrossRef]
- Wu, Z.W.; Dong, C.Z.; Jiang, J. Degrees of polarization of the two strongest 5f→3d lines following electron-impact excitation and dielectronic recombination processes of Cu-like to Se-like gold ions. Phys. Rev. A 2012, 86, 022712. [Google Scholar] [CrossRef]
- Jiang, J.; Dong, C.Z.; Xie, L.Y.; Wang, J.G. Resonance electron-impact excitation and polarization of the magnetic quadrupole line of neonlike Ba46+ ions. Phys. Rev. A 2008, 78, 022709. [Google Scholar] [CrossRef]
- Schippers, S.; Bernhardt, D.; Müller, A.; Krantz, C.; Grieser, M.; Repnow, R.; Wolf, A.; Lestinsky, M.; Hahn, M.; Novotný, O.; et al. Dielectronic recombination of xenonlike tungsten ions. Phys. Rev. A 2011, 83, 012711. [Google Scholar] [CrossRef]
- Spruck, K.; Badnell, N.R.; Krantz, C.; Novotný, O.; Becker, A.; Bernhardt, D.; Grieser, M.; Hahn, M.; Repnow, R.; Savin, D.W.; et al. Recombination of W18+ ions with electrons: Absolute rate coefficients from a storage-ring experiment and from theoretical calculations. Phys. Rev. A 2014, 90, 032715. [Google Scholar] [CrossRef]
- Ballance, C.P.; Loch, S.D.; Pindzola, M.S.; Griffin, D.C. Dielectronic recombination of W35+. J. Phys. B 2010, 43, 205201. [Google Scholar] [CrossRef]
- Badnell, N.R.; Ballance, C.P.; Griffin, D.C.; O’Mullane, M. Dielectronic recombination of W20+ (4d104f8): Addressing the half-open f shell. Phys. Rev. A 2012, 85, 052716. [Google Scholar] [CrossRef]
- Dzuba, V.A.; Flambaum, V.V.; Gribakin, G.F.; Harabati, C. Chaos-induced enhancement of resonant multielectron recombination in highly charged ions: Statistical theory. Phys. Rev. A 2012, 86, 022714. [Google Scholar] [CrossRef]
- Dzuba, V.A.; Flambaum, V.V.; Gribakin, G.F.; Harabati, C.; Kozlov, M.G. Electron recombination, photoionization, and scattering via many-electron compound resonances. Phys. Rev. A 2013, 88, 062713. [Google Scholar] [CrossRef]
- Safronova, U.I.; Safronova, A.S.; Beiersdorfer, P. Dielectronic recombination of Rh-like Gd and W. Phys. Rev. A 2012, 86, 042510. [Google Scholar] [CrossRef]
- Ralchenko, Y.; Gillaspy, J.D. Anisotropic LMN dielectronic resonances from ratios of magnetic-dipole lines. Phys. Rev. A 2013, 88, 012506. [Google Scholar] [CrossRef]
- Li, B.W.; O’Sullivan, G.; Fu, Y.B.; Dong, C.Z. Dielectronic recombination of Rh-like Gd and W. Phys. Rev. A 2012, 85, 052706. [Google Scholar] [CrossRef]
- Li, M.J.; Fu, Y.B.; Su, M.G.; Dong, C.Z.; Koike, F. Dielectronic recombination of Br-Like tungsten ions. Plasma Sci. Technol. 2014, 16, 182–187. [Google Scholar] [CrossRef]
- Li, M.J.; Fu, Y.B.; Zhang, G.D.; Zhang, Y.Z.; Dong, C.Z.; Koike, F. Dielectronic recombination of the 4p and 4d open sub-shell tungsten ions. J. Phys. 2014, 488, 062022. [Google Scholar] [CrossRef]
- Wu, Z.W.; Zhang, Y.Z.; Fu, Y.B.; Surzhykov, A.; Fritzsche, S.; Dong, C.Z. Dielectronic recombination rate coefficients of initially rubidium-like tungsten. Eur. Phys. J. D 2015, 69, 140. [Google Scholar] [CrossRef]
- Pütterich, T.; Neu, R.; Dux, R.; Whiteford, A.D.; O’Mullane, M.G.; the ASDEX Upgrade Team. Modelling of measured tungsten spectra from ASDEX Upgrade and predictions for ITER. Plasma Phys. Control. Fus. 2008, 50, 085016. [Google Scholar] [CrossRef]
- Summers, H.P. The ADAS User Manual, Version 2.6, 2004. Available online: http://www.adas.ac.uk (accessed on 10 November 2015).
- Badnell, N.R.; O’Mullane, M.G.; Summers, H.P.; Altun, Z.; Bautista, M.A.; Colgan, J.; Gorczyca, T.W.; Mitnik, D.M.; Pindzola, M.S.; Zatsarinny, O. Dielectronic recombination data for dynamic finite-density plasmas I. Goals and methodology. Astron. Astrophys. 2003, 406, 1151–1165. [Google Scholar] [CrossRef]
- Burgess, A. A general formula for the estimation of dielectronic recombination coefficients in low-density plasmas. Astrophys. J. 1965, 141, 1588–1590. [Google Scholar] [CrossRef]
- Trzhaskovskaya, M.B.; Nikulin, V.K.; Clark, R.E.H. Radiative recombination rate coefficients for highly-charged tungsten ions. At. Data Nucl. Data Tables 2010, 96, 1–25. [Google Scholar] [CrossRef]
- Trzhaskovskaya, M.B.; Nikulin, V.K. Radiative recombination data for tungsten ions: I. W24+–W45+. At. Data Nucl. Data Tables 2013, 99, 249–311. [Google Scholar] [CrossRef]
- Trzhaskovskaya, M.B.; Nikulin, V.K. Radiative recombination data for tungsten ions: II. W47+–W71+. At. Data Nucl. Data Tables 2014, 100, 986–1058. [Google Scholar] [CrossRef]
- Trzhaskovskaya, M.B.; Nikulin, V.K. Radiative recombination data for tungsten ions: III. W14+–W23+. At. Data Nucl. Data Tables 2014, 100, 1156–1188. [Google Scholar] [CrossRef]
- Jiang, J.; Dong, C.Z.; Xie, L.Y.; Wang, J.G.; Yan, J.; Fritzsche, S. Relativistic distorted-wave calculations of electron impact excitation cross-sections of Be-like C2+ ions. Chin. Phys. Lett. 2007, 24, 691–694. [Google Scholar]
- Zhang, H.L.; Sampson, D.H.; Clark, R.E.H. Relativistic cross-sections for excitation of highly charged ions to specific magnetic sub-levels by an electron beam. Phys. Rev. A 1990, 41, 198–206. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.L.; Sampson, D.H. Resonance contributions to collision strengths for transitions between magnetic sub-levels in highly charged ions by impact with an electron beam. Phys. Rev. A 2002, 66, 042704. [Google Scholar] [CrossRef]
- Parpia, F.A.; Fischer, C.F.; Grant, I.P. GRASP92: A package for large-scale relativistic atomic structure calculations. Comput. Phys. Commun. 1996, 94, 249–271. [Google Scholar] [CrossRef]
- Fritzsche, S. The RATIP program for relativistic calculations of atomic transition, ionization and recombination properties. Comput. Phys. Commun. 2012, 183, 1525–1559. [Google Scholar] [CrossRef]
- Percival, I.C.; Seaton, M.J. The polarization of atomic line radiation excited by electron impact. Philos. Trans. R. Soc. Lond. Ser. A 1958, 251, 113–138. [Google Scholar] [CrossRef]
- Blum, K. Density Matrix Theory and Applications; Plenum: New York, NY, USA, 1981. [Google Scholar]
- Balashov, V.V.; Grum-Grzhimailo, A.N.; Kabachnik, N.M. Polarization and Correlation Phenomena in Atomic Collisions; Kluwer Academic: New York, NY, USA, 2000. [Google Scholar]
- Xie, L.Y.; Ma, X.Y.; Dong, C.Z.; Wu, Z.W.; Shi, Y.L.; Jiang, J. Polarization of the nf → 3d (n = 4, 5, 6) X-rays from tungsten ions following electron-impact excitation and dielectronic recombination processes. J. Quant. Spectrosc. Radiat. Transfer 2014, 141, 31–39. [Google Scholar] [CrossRef]
- Dubau, J.; Volonté, S. Dielectronic recombination and its applications in astronomy. Rep. Prog. Phys. 1980, 43, 199–251. [Google Scholar] [CrossRef]
- Gu, M.F. Dielectronic recombination rate coefficients for H-like through Ne-like isosequences of Mg, Si, S, Ar, Ca, Fe, and Ni. Astrophys. J. 2003, 590, 1131–1140. [Google Scholar] [CrossRef]
- Gu, M.F. The flexible atomic code. Can. J. Phys. 2008, 86, 675–689. [Google Scholar] [CrossRef]
- Clementson, J. Spectroscopic Investigations of Highly Charged Tungsten Ions—Atomic Spectroscopy and Fusion Plasma Diagnostics; Atomic Physics Division, Department of Physics, Lund University: Lund, Sweden, 2010. [Google Scholar]
- Das, T.; Sharma, L.; Srivastava, R. Electron impact excitation of the M-shell electrons from Zn-like through Co-like tungsten ions. Phys. Scr. 2012, 86, 035301. [Google Scholar] [CrossRef]
- Clementson, J.; Beiersdorfer, P.; Brown, G.V.; Gu, M.F. Spectroscopy of M-shell X-ray transitions in Zn-like through Co-like W. Phys. Scr. 2010, 81, 015301. [Google Scholar] [CrossRef]
- Zhang, H.L.; Sampson, D.H.; Fontes, C.J. Relativistic distorted-wave collision strengths and oscillator strengths for the 33 Ni-like ions with 60 ≤ Z ≤ 92. At. Data Nucl. Data Tables 1991, 48, 91–163. [Google Scholar] [CrossRef]
- Nakamura, N.; Kavanagh, A.P.; Watanabe, H.; Sakaue, H.A.; Li, Y.M.; Kato, D.; Currell, F.J.; Ohtani, S. Evidence for strong Breit interaction in dielectronic recombination of highly charged heavy ions. Phys. Rev. Lett. 2008, 100, 073203. [Google Scholar] [CrossRef] [PubMed]
- O’Mullane, M.G.; (Department of Physics, University of Strathclyde, Glasgow, UK). Private communication, 2015.
- Fu, Y.B.; Dong, C.Z.; Su, M.G.; Koike, F.; O’Sullivan, G.; Wang, J.G. Theoretical investigation of dielectronic recombination of Sn12+ ions. Phys. Rev. A 2011, 83, 062708. [Google Scholar] [CrossRef]
- Safronova, U.; Kato, T.; Ohira, M. Dielectronic recombination rate coefficients to the excited states of CIII from CIV. J. Quant. Spectrosc. Radiat. Transfer 1997, 58, 193–215. [Google Scholar] [CrossRef]
- Clark, R.E.H.; Reiter, D.H. Nuclear Fusion Research; Springer: New York, NY, USA, 2005. [Google Scholar]
- Zhang, H.; Li, Y.M.; Yan, J.; Wang, J.G. Dielectronic recombination processes through doubly excited states (4l4l′, 4l5l′) in Co-like Pd ions. Phys. Rev. A 2005, 71, 042705. [Google Scholar] [CrossRef]
- Meng, F.C.; Chen, C.Y.; Wang, Y.S.; Zou, Y.M. Total dielectronic recombination rate coefficient for Co-like tungsten. J. Quant. Spectrosc. Radiat. Transfer 2008, 109, 2000–2008. [Google Scholar] [CrossRef]
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, Z.; Fu, Y.; Ma, X.; Li, M.; Xie, L.; Jiang, J.; Dong, C. Electron Impact Excitation and Dielectronic Recombination of Highly Charged Tungsten Ions. Atoms 2015, 3, 474-494. https://doi.org/10.3390/atoms3040474
Wu Z, Fu Y, Ma X, Li M, Xie L, Jiang J, Dong C. Electron Impact Excitation and Dielectronic Recombination of Highly Charged Tungsten Ions. Atoms. 2015; 3(4):474-494. https://doi.org/10.3390/atoms3040474
Chicago/Turabian StyleWu, Zhongwen, Yanbiao Fu, Xiaoyun Ma, Maijuan Li, Luyou Xie, Jun Jiang, and Chenzhong Dong. 2015. "Electron Impact Excitation and Dielectronic Recombination of Highly Charged Tungsten Ions" Atoms 3, no. 4: 474-494. https://doi.org/10.3390/atoms3040474
APA StyleWu, Z., Fu, Y., Ma, X., Li, M., Xie, L., Jiang, J., & Dong, C. (2015). Electron Impact Excitation and Dielectronic Recombination of Highly Charged Tungsten Ions. Atoms, 3(4), 474-494. https://doi.org/10.3390/atoms3040474