Fusion-Related Ionization and Recombination Data for Tungsten Ions in Low to Moderately High Charge States
Abstract
:1. Introduction
- electron-impact single ionizationand
- electron-impact multiple ionization
- recombinationand time-reversed recombination, i.e.,
- (single) photoionizationand
- multiple ionization by one photon
2. Preparation of Ion-Target Beams
2.1. Ion Production and Associated Population of Excited States
2.2. Generating Beams of Multiply Charged Wq+ Ions
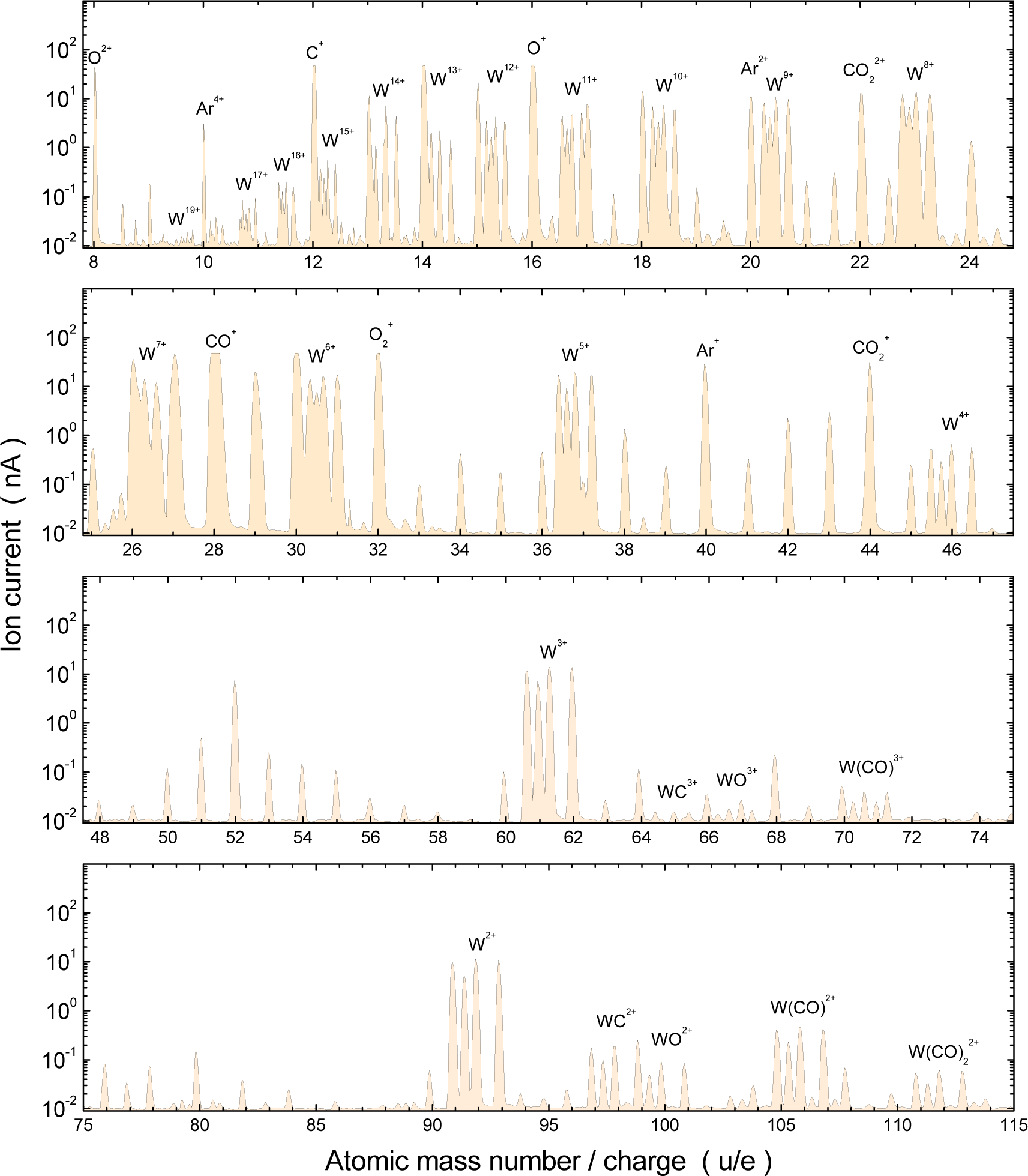
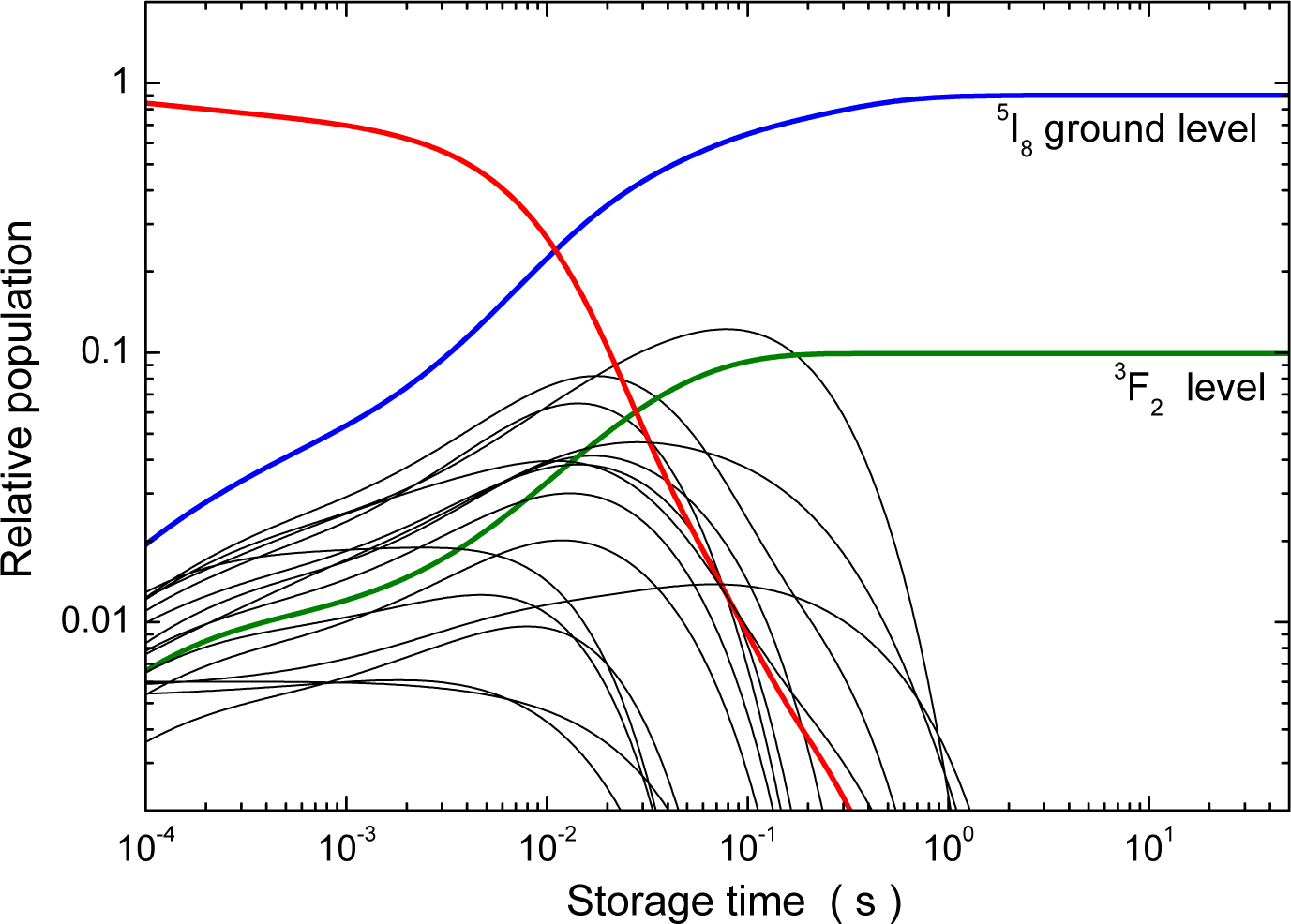
2.3. Assessment of the Presence of Metastable Excited States in Beams of Wq+ Ions Used for the Present Experiments
3. Overview of the Collisional Data Obtained for W Ions
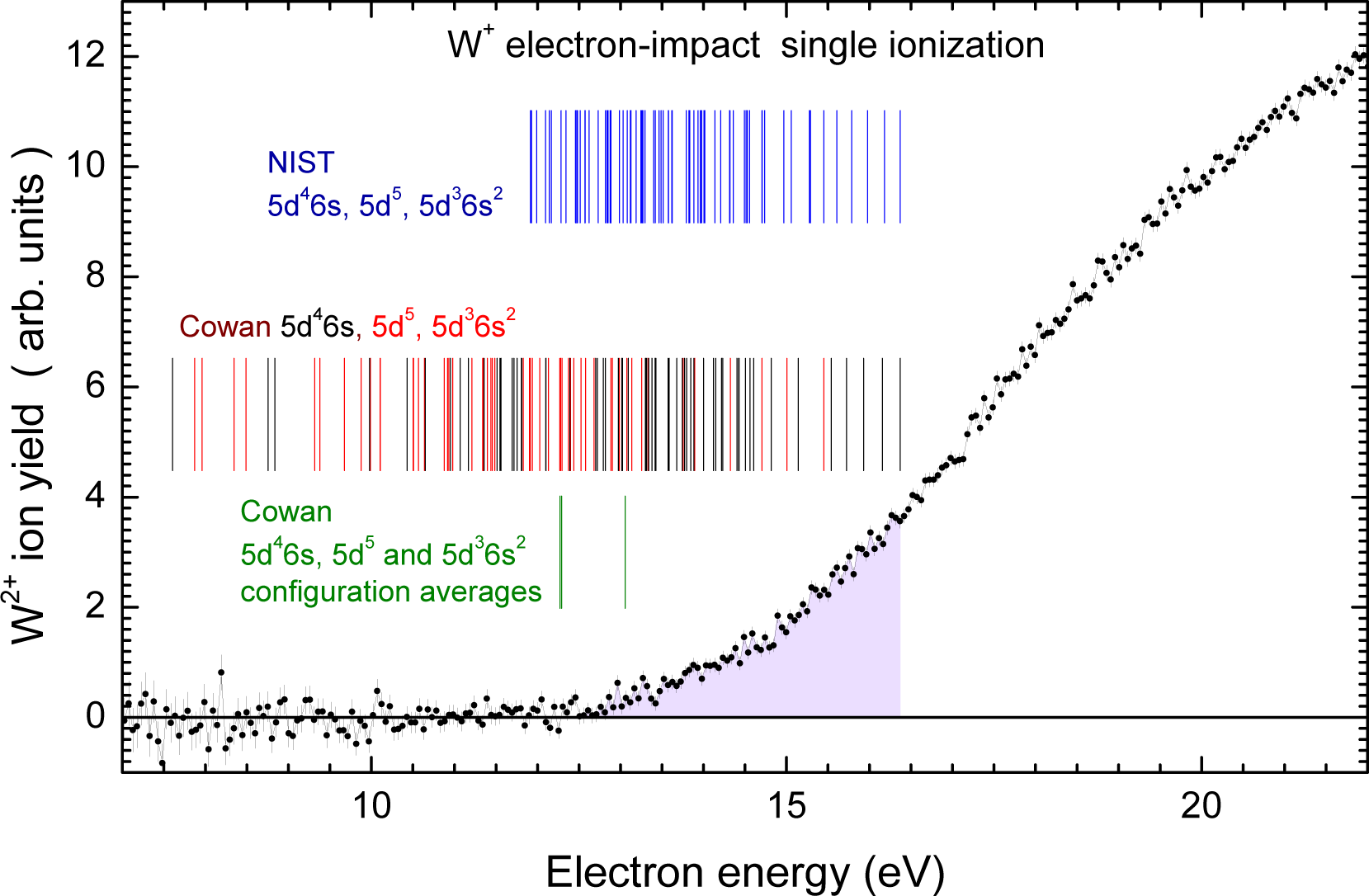
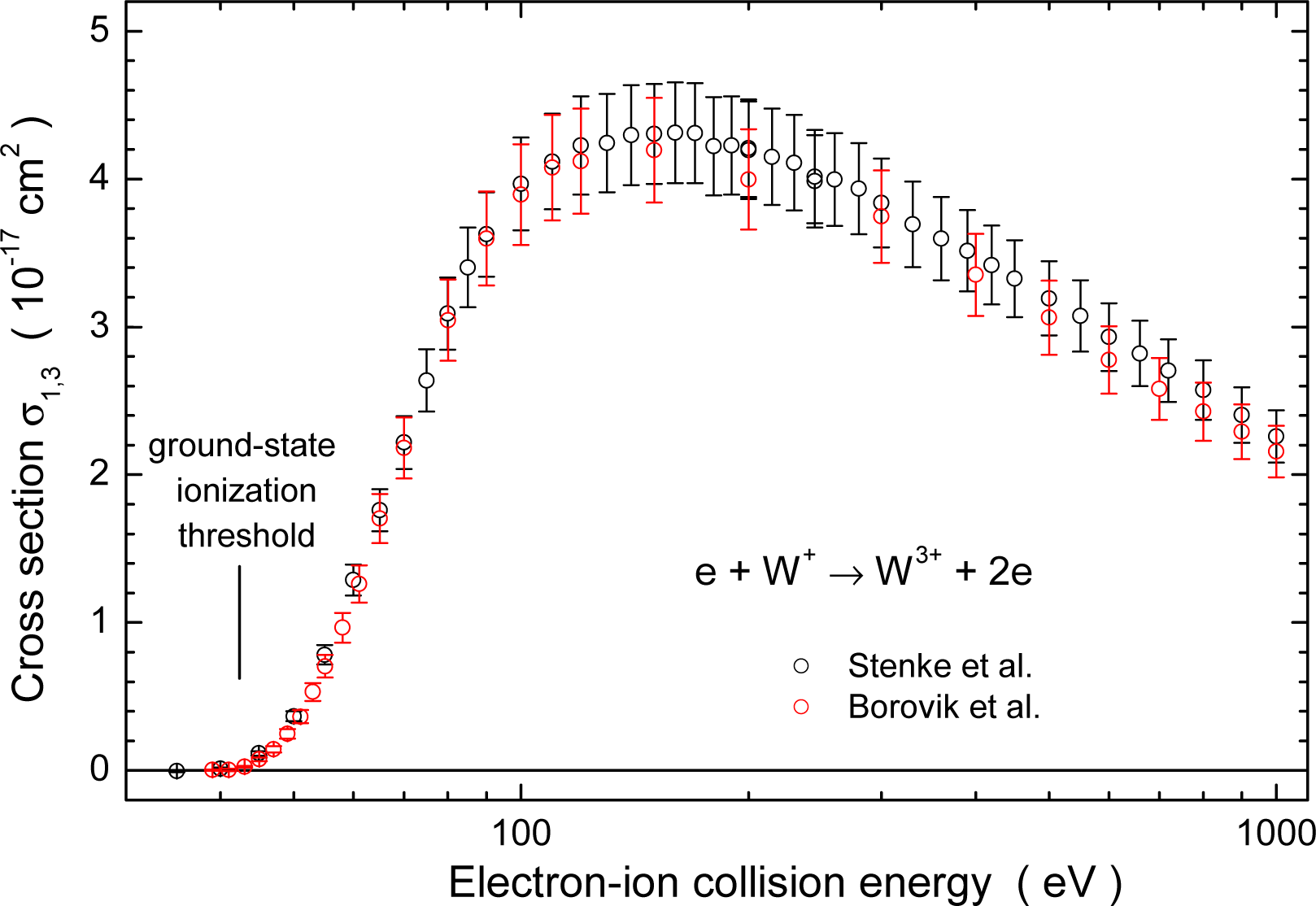
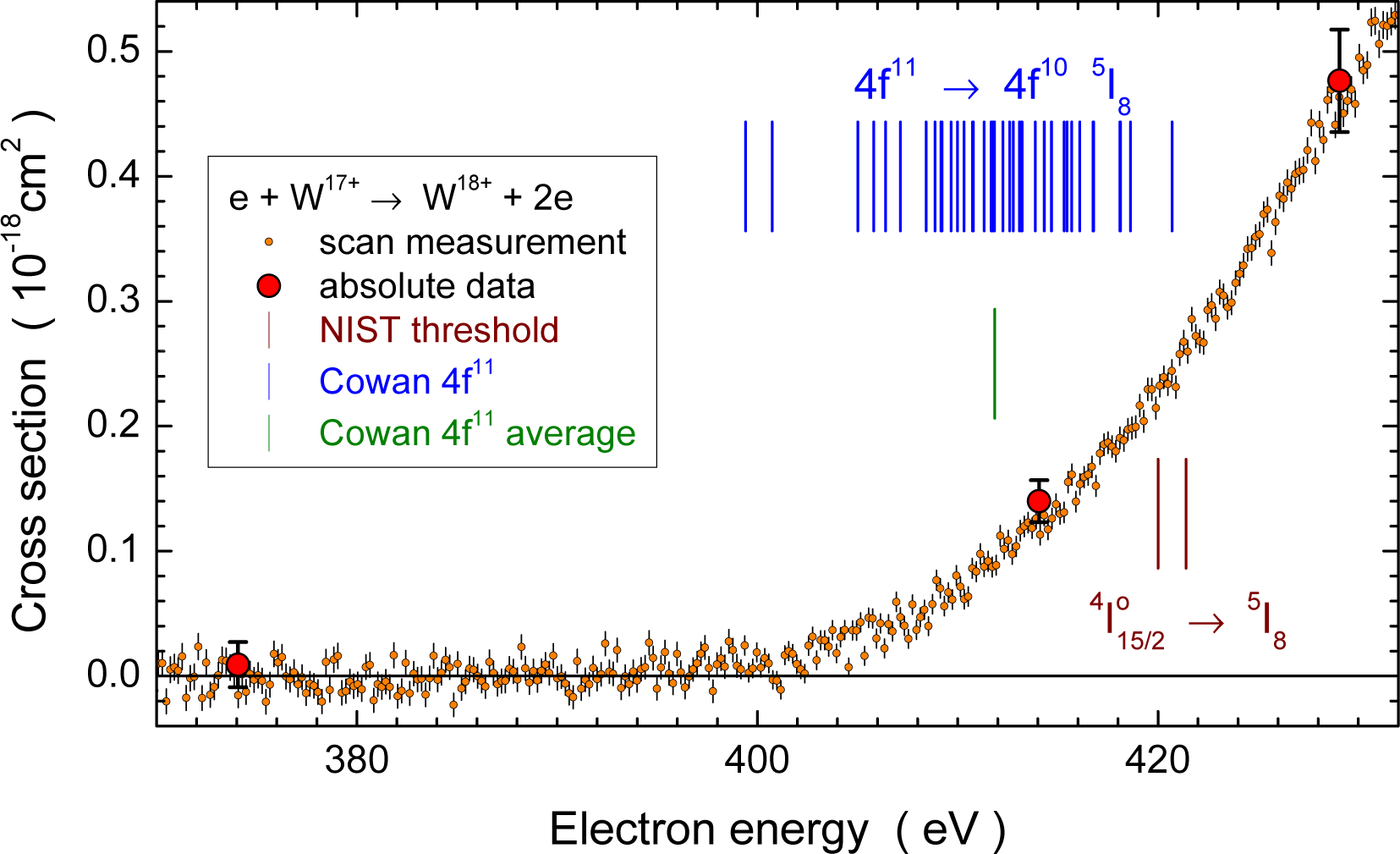
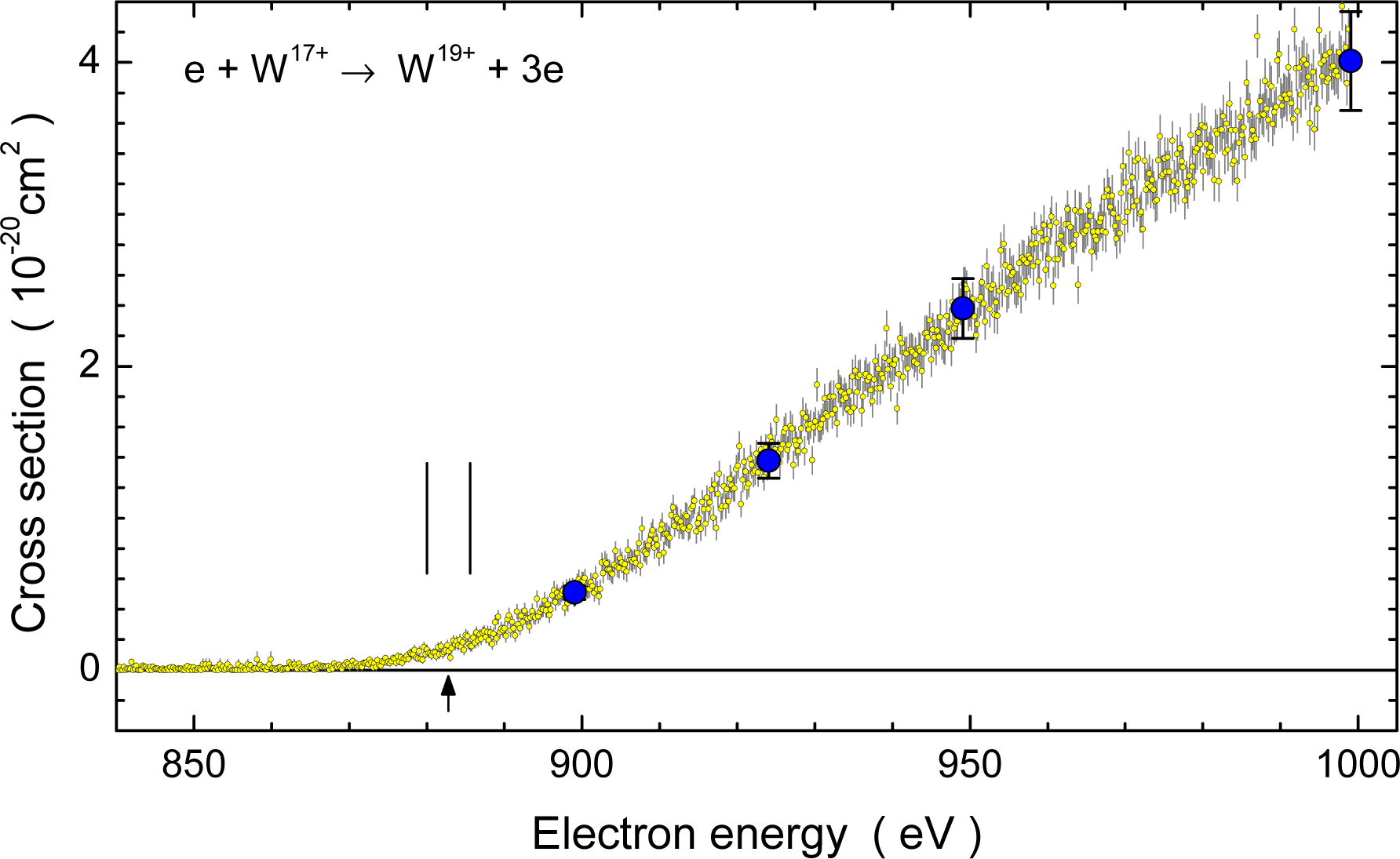
3.1. Electron-Impact Ionization
- Direct knock-off removal of an outer-shell electron, termed direct ionization (DI)
- Excitation of an inner-shell electron so that an autoionizing intermediate state is produced; by a subsequent Auger decay an electron is released from the atom or ion; the process is termed excitation-autoionization (EA)
- Dielectronic (radiationless) capture of the incident electron with simultaneous inner-shell excitation reducing the ion charge state by one unit; the resulting highly excited state may then decay by the emission of two electrons (either sequentially or simultaneously) and, thus, an ionized ion with a charge state one unit above that of the parent ion is produced. The whole process is resonant as a result of the first step, the dielectronic capture, which is essentially a time-reversed Auger-decay process
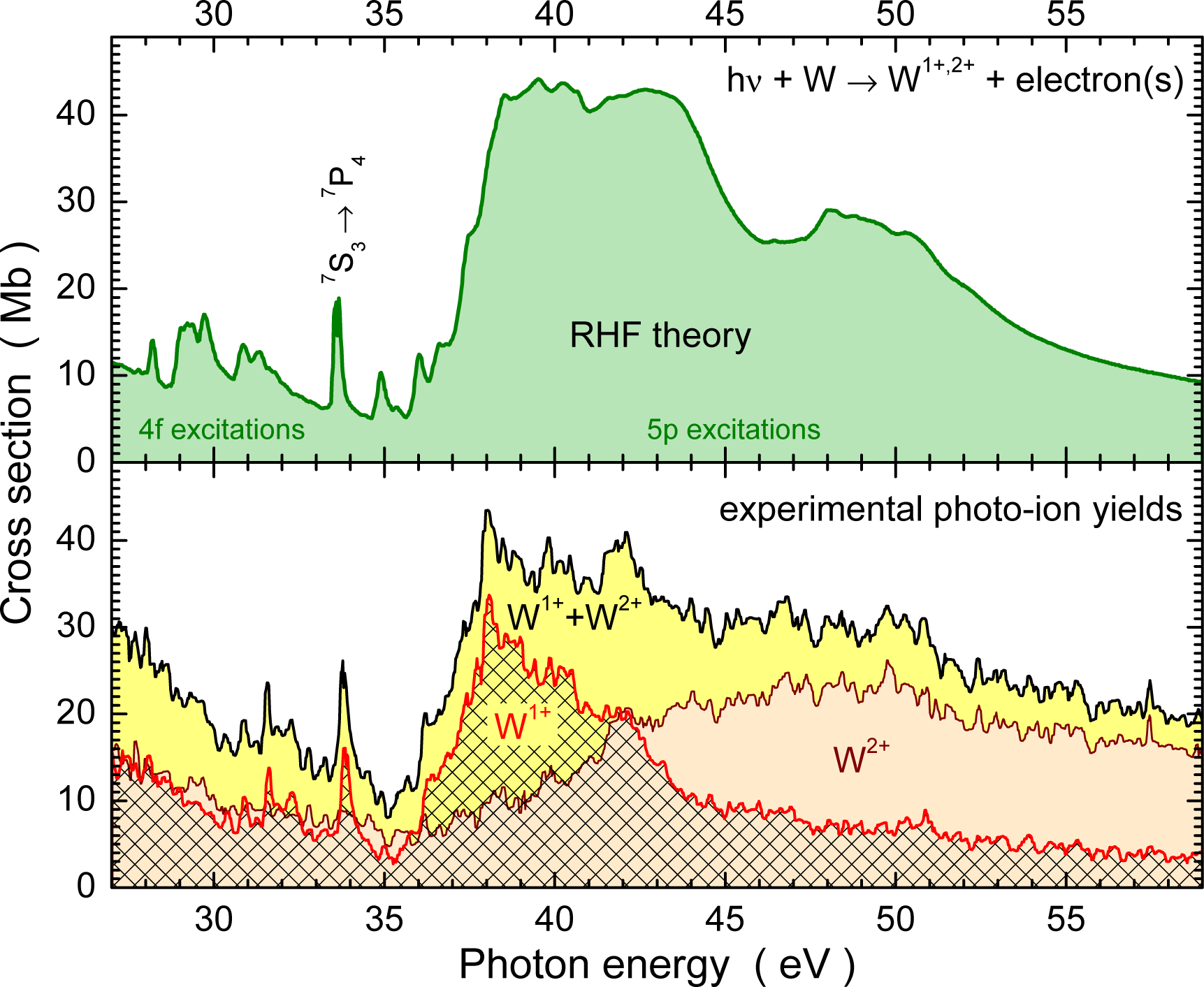
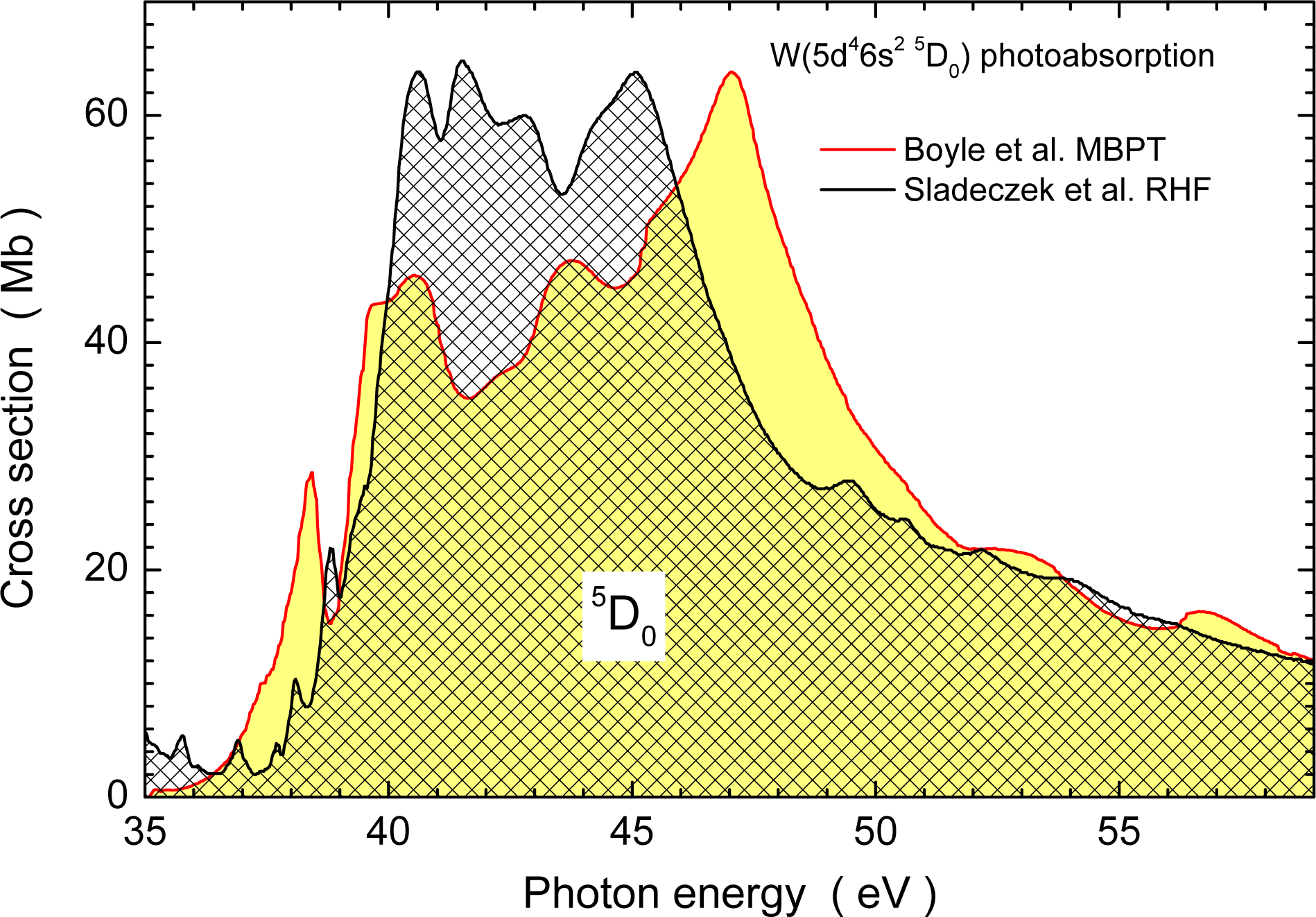
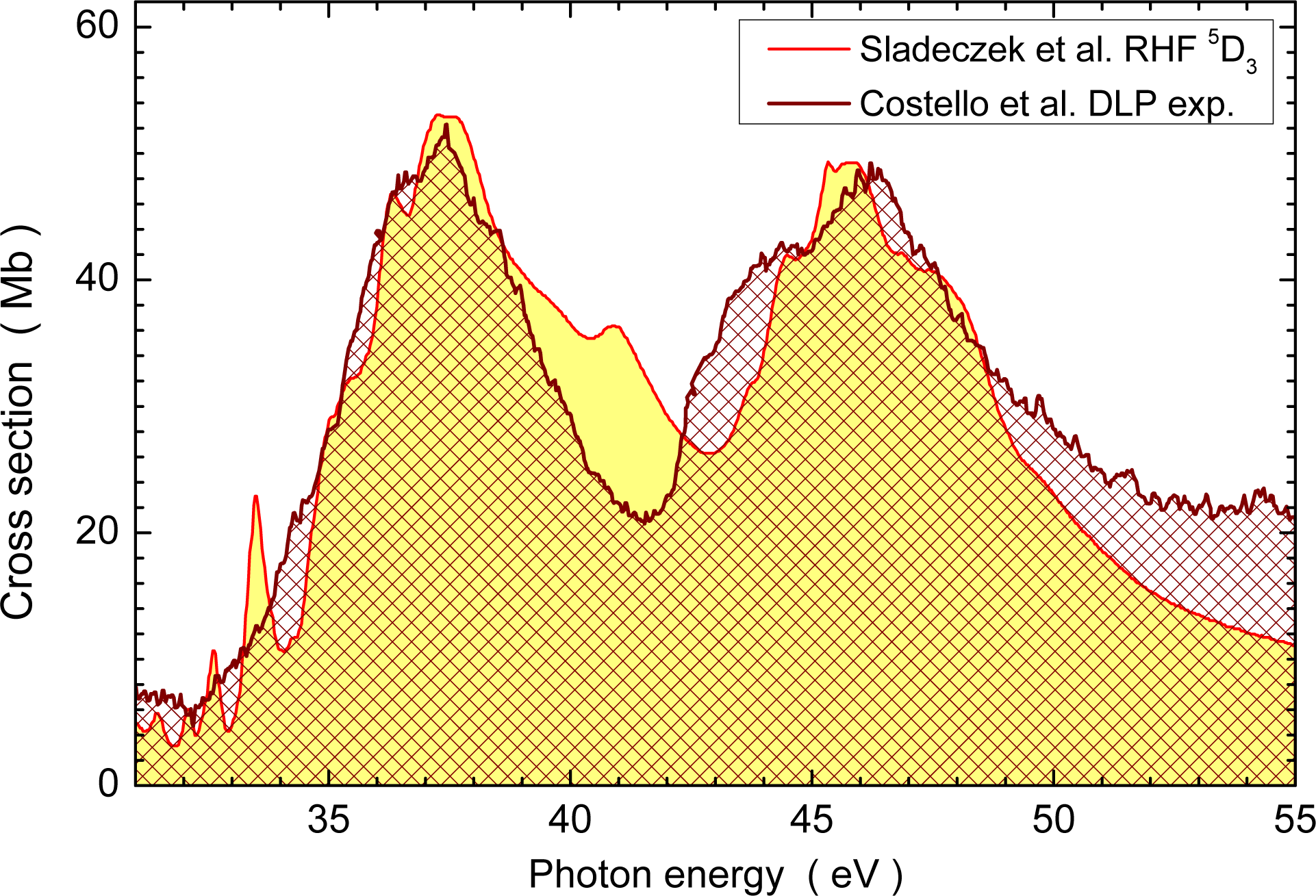
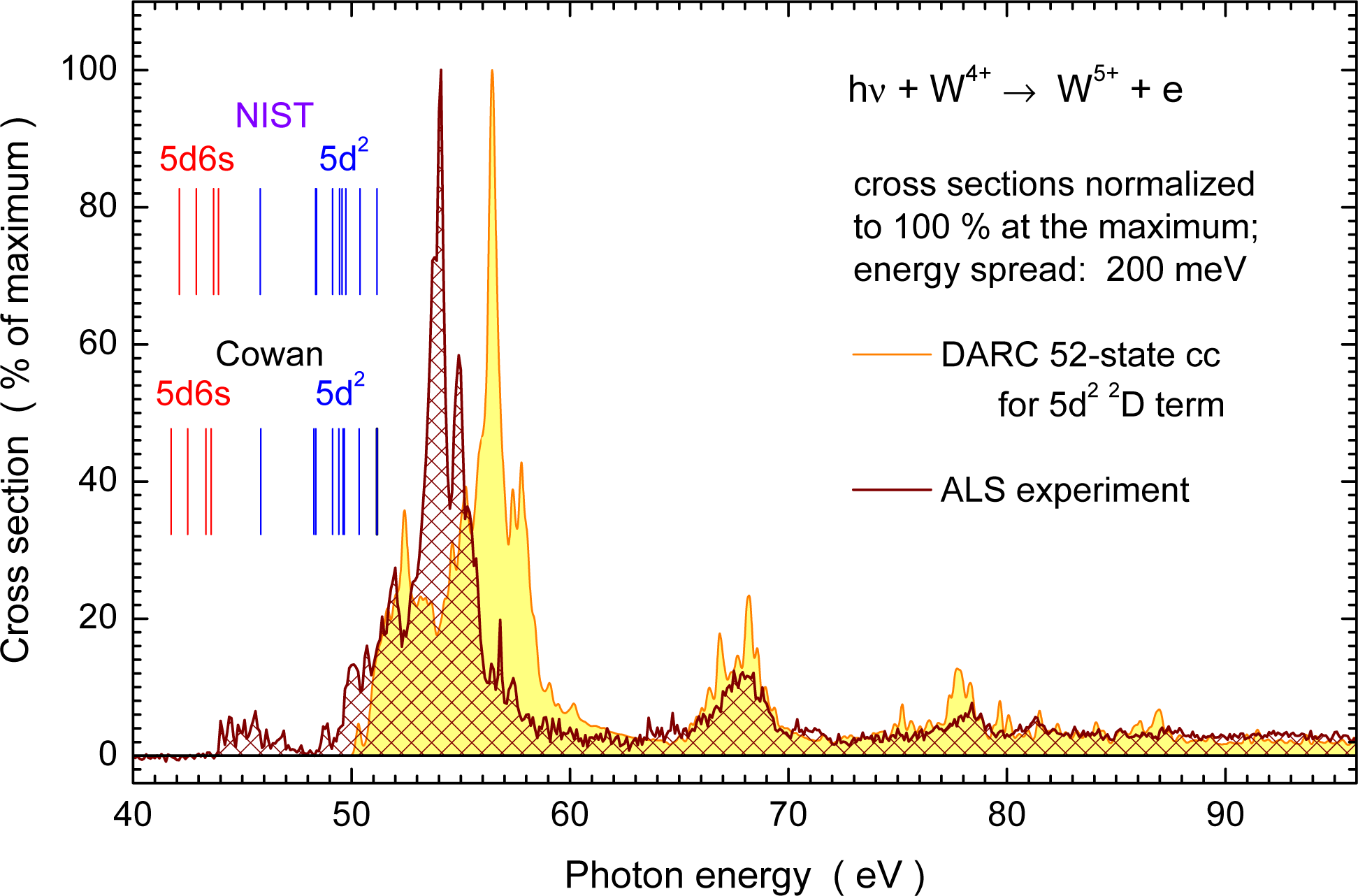
3.2. Photoionization
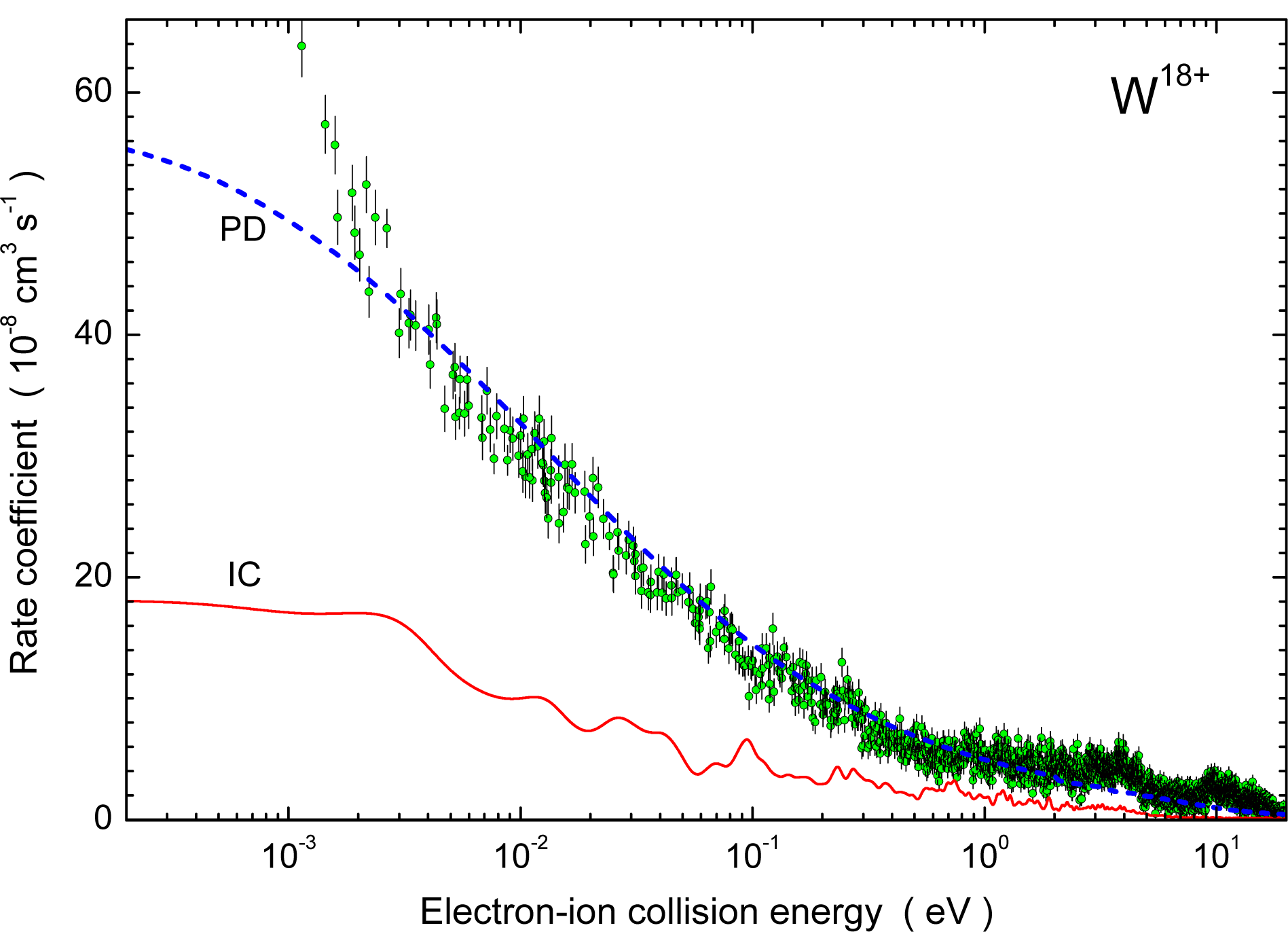
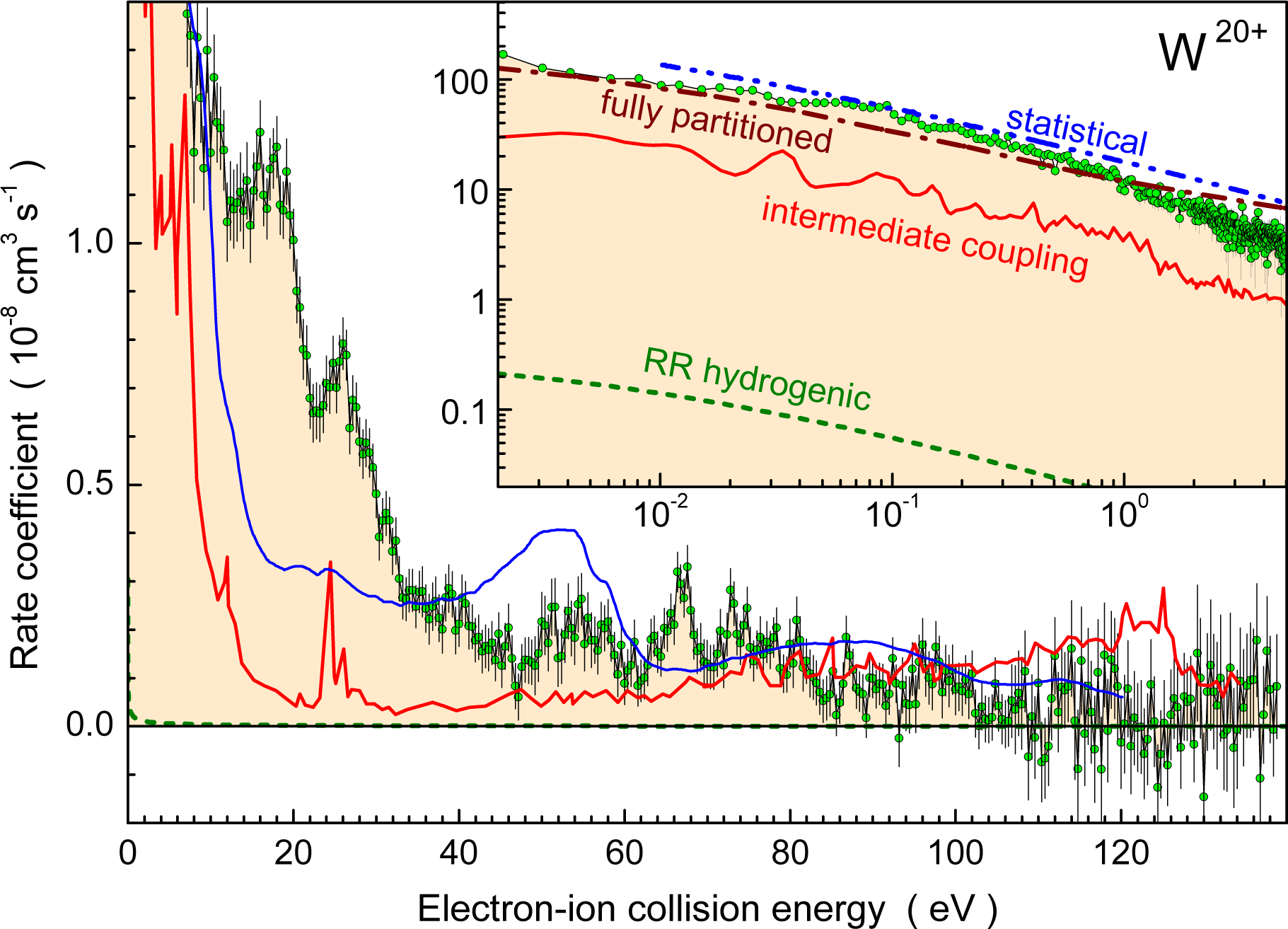
3.3. Electron-Ion Recombination
4. Conclusions
Acknowledgments
Conflicts of Interest
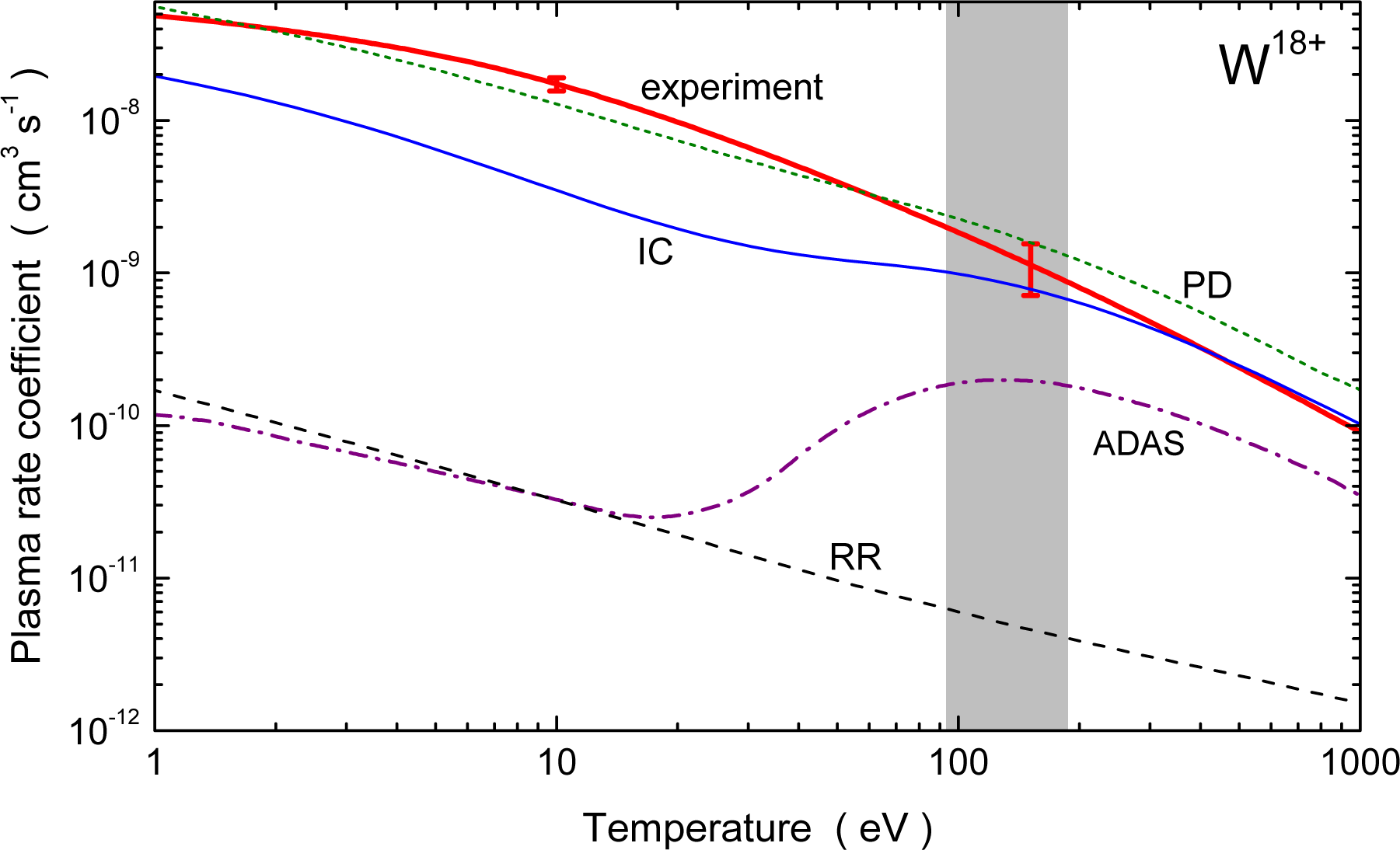
Appendix: Plasma Rate Coefficients
| Plasma temperature [eV] | |
|---|---|
| 1 | 4.88 × 10−8 |
| 1.5 | 4.36 × 10−8 |
| 2 | 3.98 × 10−8 |
| 3 | 3.42 × 10−8 |
| 5 | 2.70 × 10−8 |
| 7 | 2.22 × 10−8 |
| 10 | 1.74 × 10−8 |
| 15 | 1.26 × 10−8 |
| 20 | 9.78 × 10−9 |
| 30 | 6.66 × 10−9 |
| 50 | 3.96 × 10−9 |
| 70 | 2.76 × 10−9 |
| 100 | 1.85 × 10−9 |
| 150 | 1.15 × 10−9 |
| 200 | 8.04 × 10−10 |
| 300 | 4.78 × 10−10 |
| 500 | 2.40 × 10−10 |
| 700 | 1.51 × 10−10 |
| 1000 | 9.07 × 10−11 |
| Plasma temperature [eV] | |
|---|---|
| 1 | 1.37 × 10−7 |
| 1.5 | 1.03 × 10−7 |
| 2 | 8.34 × 10−8 |
| 3 | 6.09 × 10−8 |
| 5 | 4.01 × 10−8 |
| 7 | 3.02 × 10−8 |
| 10 | 2.23 × 10−8 |
| 15 | 1.55 × 10−8 |
| 20 | 1.19 × 10−8 |
| 30 | 8.02 × 10−9 |
| 50 | 4.70 × 10−9 |
| 70 | 3.23 × 10−9 |
| 100 | 2.11 × 10−9 |
| 150 | 1.26 × 10−9 |
| 200 | 8.65 × 10−10 |
| 300 | 4.98 × 10−10 |
| 500 | 2.42 × 10−10 |
| 700 | 1.49 × 10−10 |
| 1000 | 8.88 × 10−11 |
References
- Drawin, H.W. Atomic Physics and Thermonuclear Fusion Research. Phys. Scr. 1981, 24, 622–655. [Google Scholar]
- Neu, R.; Dux, R.; Geier, A.; Gruber, O.; Kallenbach, A.; Krieger, K.; Maier, H.; Pugno, R.; Rohde, V.; Schweizer, S.; ASDEX Upgrade Team. Tungsten as plasma-facing material in ASDEX Upgrade. Fusion Eng. Des. 2003, 65, 367–374. [Google Scholar]
- Neu, R.; Arnoux, G.; Beurskens, M.; Bobkov, V.; Brezinsek, S.; Bucalossi, J.; Calabro, G.; Challis, C.; Coenen, J.W.; de la Luna, E.; et al. First operation with the JET International Thermonuclear Experimental Reactor-like wall. Phys. Plasmas 2013, 20, 056111. [Google Scholar]
- Aymar, R.; Barabaschi, P.; Shimomura, Y. The ITER design. Plasma Phys. Control. Fusion 2002, 44, 519–565. [Google Scholar]
- O’Mullane, M.G.; Summers, H.P.; Whiteford, A.D.; Meigs, A.G.; Lawson, K.D.; Zastrow, K.D.; Barnsley, R.; Coffey, I.H. JET-EFDA Contributors. Atomic modeling and instrumentation for measurement and analysis of emission in preparation for the ITER-like wall in JET. Rev. Sci. Instrum. 2006, 77, 10F520. [Google Scholar]
- Summers, H.P.; Dickson, W.J.; O’Mullane, M.G.; Badnell, N.R.; Whiteford, A.D.; Brooks, D.H.; Lang, J.; Loch, S.D.; Griffin, D.C. Ionization State, Excited Populations Emission of Impurities in Dynamic Finite Density Plasmas: I. The Generalized Collisional-Radiative Model for Light Elements. Plasma Phys. Control. Fusion 2006, 48, 263–293. [Google Scholar]
- Post, D.; Abdallah, J.; Clark, R.E.H.; Putvinskaya, N. Calculations of energy losses due to atomic processes in tokamaks with applications to the International Thermonuclear Experimental Reactor divertor. Phys. Plasmas 1995, 2, 2328–2336. [Google Scholar]
- Flannery, M.R. Electron-ion and ion-ion recombination. In SpringerHandbook of Atomic, Molecular & Optical Physics; Drake, G.W., Ed.; Springer: New York, NY, USA, 2006; pp. 800–827. [Google Scholar]
- Pütterich, T.; Neu, R.; Dux, R.; Whiteford, A.D.; O’Mullane, M.G. the ASDEX Upgrade Team. Modelling of measured tungsten spectra from ASDEX Upgrade and predictions for ITER. Plasma Phys. Control. Fusion 2008, 50, 085016. [Google Scholar]
- Krücken, T.; Bergmann, K.; Juschkin, L.; Lebert, R. Fundamentals and limits for the EUV emission of pinch plasma sources for EUV lithography. J. Phys. D 2004, 37, 3213–3224. [Google Scholar]
- Summers, H.P.; Badnell, N.R.; O’Mullane, M.G.; Whiteford, A.D.; Bingham, R.; Kellett, B.J.; Lang, J.; Behringer, K.H.; Fantz, U.; Zastrow, K.D.; et al. Atomic data for modelling fusion and astrophysical plasmas. Plasma Phys. Control. Fusion 2002, 44, B323–B338. [Google Scholar]
- Schippers, S. Storage-ring ionization and recombination experiments with multiply charged ions relevant to astrophysical and fusion plasmas. J. Phys. Conf. Ser. 2012, 388, 012010. [Google Scholar]
- Bernhardt, D.; Becker, A.; Grieser, M.; Hahn, M.; Krantz, C.; Lestinsky, M.; Novotný, O.; Repnow, R.; Savin, D.W.; Spruck, K.; et al. Absolute rate coefficients for photorecombination and electron-impact ionization of magnesiumlike iron ions from measurements at a heavy-ion storage ring. Phys. Rev. A 2014, 90, 012702. [Google Scholar]
- Hahn, M.; Badnell, N.R.; Grieser, M.; Krantz, C.; Lestinsky, M.; Müller, A.; Novotný, O.; Repnow, R.; Schippers, S.; Wolf, A.; Savin, D.W. Electron-Ion Recombination of Fe12+ Forming Fe11+: Laboratory Measurements and Theoretical Calculations. Astrophys. J. 2014, 788, 46. [Google Scholar]
- Schippers, S. Electron-ion merged-beam experiments at heavy-ion storage rings. Nucl. Instrum. Meth. in Phys. Res. 2015, 350, 61–65. [Google Scholar]
- Müller, A. Electron-Ion Collisions: Fundamental Processes in the Focus of Applied Research. Adv. At. Mol. Opt. Phys. 2008, 55, 293–417. [Google Scholar]
- Müller, A. Resonance phenomena in electron-ion and photon-ion collisions. J. Phys. Conf. Ser. 2009, 194, 012002. [Google Scholar]
- Imai, A.M.; Iriki, Y.; Ohta, Y.; Majima, T.; Tsuchida, H.; Shibata, H.; Itoh, A. Single-electron-capture cross-section scaling for low-q heavy ions at low energy. J. Phys. Conf. Ser 2014, 488, 082010. [Google Scholar]
- Tolstikhina, I.Y.; Song, M.Y.; Imai, M.; Iriki, Y.; Itoh, A.; Kato, D.; Tawara, H.; Yoon, J.S.; Shevelko, V.P. Charge-changing collisions of tungsten and its ions with neutral atoms. J. Phys. B At. Mol. Opt. Phys. 2012, 45, 145201. [Google Scholar]
- Dipti; Das, T.; Sharma, L.; Srivastava, R. L-shell electron excitations of Mg-through O-like tungsten ions. Phys. Scr. 2014, 89, 085403. [Google Scholar]
- Ballance, C.P.; Loch, S.D.; Pindzola, M.S.; Griffin, D.C. Electron-impact excitation and ionization of W3+ for the determination of tungsten influx in a fusion plasma. J. Phys. B At. Mol. Opt. Phys. 2013, 46, 055202. [Google Scholar]
- Safronova, U.I.; Safronova, A.S.; Beiersdorfer, P. Relativistic many-body calculations of excitation energies, oscillator strengths, transition rates, and lifetimes in samariumlike ions. Phys. Rev. A 2013, 87, 032508. [Google Scholar]
- Kramida, A.E.; Shirai, T. Compilation of Wavelengths, Energy Levels, and Transition Probabilities for W I and W II. J. Phys. Chem. Ref. Data 2006, 35, 423. [Google Scholar]
- Kramida, A.E.; Shirai, T. Energy levels and spectral lines of tungsten, W III through W LXXIV. At. Data Nucl. Data Tables 2009, 95, 305–474. [Google Scholar]
- Kramida, A.E.; Shirai, T. Erratum to “Energy levels and spectral lines of tungsten, W III through W LXXIV” [Atomic Data and Nuclear Data Tables 95 (2009) 305–474]. At. Data Nucl. Data Tables 2009, 95, 1051. [Google Scholar]
- Kramida, A.E.; Ralchenko, Y.; Reader, J. NIST ASD Team, NIST Atomic Spectra Database (version 5.2); National Institute of Standards and Technology: Gaithersburg, MD, USA, 2014. [Google Scholar]
- Müller, A. Targets Consisting of Charged Particles. Nucl. Instrum. Methods A 1989, 282, 80–86. [Google Scholar]
- Müller, A.; Huber, K.; Tinschert, K.; Becker, R.; Salzborn, E. An improved crossed-beams technique for the measurement of absolute cross sections for electron impact ionisations of ions and its application to Ar+ ions. J. Phys. B At. Mol. Opt. Phys 1985, 18, 2993–3009. [Google Scholar]
- Phaneuf, R.A.; Havener, C.C.; Dunn, G.H.; Müller, A. Merged-beams experiments in atomic and molecular physics. Rep. Prog. Phys. 1999, 62, 1143–1180. [Google Scholar]
- Currell, F.J. Electron Beam Ion Traps their Use in the Study of Highly Charged Ions. In The Physics of Multiply and Highly Charged Ions, Volume 1; Currell, F., Ed.; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2003; pp. 39–75. [Google Scholar]
- Bernitt, S.; Brown, G.V.; Rudolph, J.K.; Steinbrügge, R.; Graf, A.; Leutenegger, M.; Epp, S.W.; Eberle, S.; Kubicek, K.; Mäckel, V.; et al. An unexpectedly low oscillator strength as the origin of the Fe XVII emission problem. Nature 2012, 492, 225. [Google Scholar]
- Rudolph, J.K.; Bernitt, S.; Epp, S.W.; Steinbrügge, R.; Beilmann, C.; Brown, G.V.; Eberle, S.; Graf, A.; Harman, Z.; Hell, N.; et al. X-ray resonant photoexcitation: Linewidths and energies of Kα transitions in highly charged Fe ions. Phys. Rev. Lett. 2013, 111, 103002. [Google Scholar]
- Steinbrügge, R.; Bernitt, S.; Epp, S.W.; Rudolph, J.K.; Beilmann, C.; Bekker, H.; Eberle, S.; Müller, A.; Versolato, O.O.; Wille, H.C.; et al. Absolute measurement of radiative and Auger rates of K-vacancy states in highly charged Fe ions. Phys. Rev. A 2015, 91, 032502. [Google Scholar]
- Trassl, R. ECR Ion Sources. In The Physics of Multiply and Highly Charged Ions; Volume 1, Currell, F., Ed.; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2003; pp. 3–37. [Google Scholar]
- Müller, A.; Wolf, A. Heavy ion storage rings. In Accelerator-Based Atomic Physics Techniques and Applications; Austin, J.C., Shafroth, S.M., Eds.; AIP Press: Woodbury, NJ, USA, 1997; p. 147. [Google Scholar]
- Linkemann, J.; Müller, A.; Kenntner, J.; Habs, D.; Schwalm, D.; Wolf, A.; Badnell, N.R.; Pindzola, M.S. Electron-impact ionization of Fe15+ ions: An ion storage ring cross section measurement. Phys. Rev. Lett. 1995, 74, 4173–4176. [Google Scholar]
- Hahn, M.; Bernhardt, D.; Grieser, M.; Krantz, C.; Lestinsky, M.; Müller, A.; Novotný, O.; Repnow, R.; Schippers, S.; Wolf, A.; Savin, D.W. Storage Ring Cross Section Measurements for Electron Impact Ionization of Fe11+ Forming Fe12+ and Fe13+. Astrophys. J. 2011, 729, 76. [Google Scholar]
- Brötz, F.; Trassl, R.; McCullough, R.W.; Arnold, W.; Salzborn, E. Design of Compact All-Permanent Magnet Electron Cyclotron Resonance (ECR) Ion Sources for Atomic Physics Experiments. Phys. Scr. 2001, 2001, 278–280. [Google Scholar]
- Trassl, R.; Thompson, W.R.; Broetz, F.; Pawlowsky, M.; McCullough, R.W.; Salzborn, E. Development of a 10 GHz “Multi-Mode” ECR Ion Source for the Production of Multiply Charged Ions from Metallic Elements. Phys. Scr. 1999, 1999, 504–506. [Google Scholar]
- Borovik, A., Jr.; Rausch, J.; Becker, A.; Spruck, K.; Schury, D.; Ebinger, B.; Gharaibeh, M.; Schippers, S.; Müller, A. Test experiments in preparation of studies on electron-impact ionization of tungsten ions, unpublished.
- Spruck, K.; Badnell, N.R.; Krantz, C.; Novotný, O.; Becker, A.; Bernhardt, D.; Grieser, M.; Hahn, M.; Repnow, R.; Savin, D.W.; et al. Recombination of W18+ ions with electrons: Absolute rate coefficients from a storage-ring experiment and from theoretical calculations. Phys. Rev. A 2014, 90, 032715. [Google Scholar]
- Badnell, N. A Breit-Pauli distorted wave implementation for AUTOSTRUCTURE. Comput. Phys. Commun. 2011, 182, 1528–1536. [Google Scholar]
- Cowan, R.D. The Theory of Atomic Structure and Spectra; University of California Press: Berkeley, CA, USA, 1981. [Google Scholar]
- Lestinsky, M.; Badnell, N.R.; Bernhardt, D.; Bing, D.; Grieser, M.; Hahn, M.; Hoffmann, J.; Jordon-Thaden, B.; Krantz, C.; Novotný, O.; et al. Electron-Ion Recombination of Mg6+ Forming Mg5+ and of Mg7+ Forming Mg6+: Laboratory Measurements and Theoretical Calculations. Astrophys. J. 2012, 758, 40. [Google Scholar]
- Stenke, M.; Aichele, K.; Harthiramani, D.; Hofmann, G.; Steidl, M.; Völpel, R.; Salzborn, E. Electron-impact single-ionization of singly and multiply charged tungsten ions. J. Phys. B At. Mol. Opt. Phys. 1995, 28, 2711–2721. [Google Scholar]
- Stenke, M.; Aichele, K.; Harthiramani, D.; Hofmann, G.; Steidl, M.; Völpel, R.; Shevelko, V.P.; Tawara, H.; Salzborn, E. Electron-impact multiple ionization of singly and multiply charged tungsten ions. J. Phys. B At. Mol. Opt. Phys. 1995, 28, 4853–4859. [Google Scholar]
- Montague, R.G.; Harrison, M.F.A. A measurement of the cross section for electron impact ionisation of singly charged tungsten ions. J. Phys. B At. Mol. Opt. Phys. 1984, 17, 2707–2711. [Google Scholar]
- Sladeczek, P.; Feist, H.; Feldt, M.; Martins, M.; Zimmermann, P. Photoionization Experiments with an Atomic Beam of Tungsten in the Region of the 5p and 4f Excitation. Phys. Rev. Lett. 1995, 75, 1483. [Google Scholar]
- Haensel, R.; Radler, K.; Sonntag, B.; Kunz, C. Optical absorption measurements of tantalum, tungsten, rhenium and platinum in the extreme ultraviolet. Solid State Commun. 1969, 7, 1495. [Google Scholar]
- Costello, J.T.; Kennedy, E.T.; Sonntag, B.F.; Cromer, C.L. XUV photoabsorption of laser-generated W and Pt vapours. J. Phys. B At. Mol. Opt. Phys. 1991, 24, 5063. [Google Scholar]
- Watanabe, H.; Nakamura, N.; Kato, D.; Nakano, T.; Ohtani, S. X-Ray Spectra from Neon-like Tungsten Ions in the Interaction with Electrons. J. Plasma, Fusion Res. 2007, 2, 27. [Google Scholar]
- Biedermann, C.; Radtke, R.; Seidel, R.; Behar, E. Dielectronic and radiative recombination of Si- to N-like tungsten ions. J. Phys. Conf. Ser. 2009, 163, 012034. [Google Scholar]
- Loch, S.D.; Ludlow, J.A.; Pindzola, M.S.; Whiteford, A.D.; Griffin, D.C. Electron-impact ionization of atomic ions in the W isonuclear sequence. Phys. Rev. A 2005, 72, 052716. [Google Scholar]
- Spruck, K.; Becker, A.; Borovik, A., Jr.; Gharaibeh, M.F.; Rausch, J.; Schippers, S.; Müller, A. Electron-impact ionization of multiply charged tungsten ions. J. Phys. Conf. Ser. 2014, 488, 062026. [Google Scholar]
- Rausch, J.; Becker, A.; Spruck, K.; Hellhund, J.; Borovik, A., Jr.; Huber, K.; Schippers, S.; Müller, A. Electron-impact single and double ionization of W17+. J. Phys. B At. Mol. Opt. Phys. 2011, 44, 165202. [Google Scholar]
- Zhang, D.H.; Kwon, D.H. Theoretical electron-impact ionization of W17+ forming W18+. J. Phys. B: At. Mol. Opt. Phys. 2014, 47, 075202. [Google Scholar]
- Gu, M.F. The flexible atomic code. Can. J. Phys. 2008, 86, 675–689. [Google Scholar]
- Jonauskas, V.; Kynienė, A.; Merkelis, G.; Gaigalas, G.; Kisielius, R.; Kučas, S.; Masys,, Š.; Radžiūtė, L.; Rynkun,, P. Contribution of high-nl shells to electron-impact ionization processes. Phys. Rev. A 2015, 91, 012715. [Google Scholar]
- Borovik, A., Jr.; Brandau, C.; Jacobi,, J.; Schippers,, S.; Müller,, A. Electron-impact single ionization of Xe10+ ions. J. Phys. B At. Mol. Opt. Phys 2011, 44, 205205. [Google Scholar]
- Pindzola, M.S.; Loch, S.D.; Borovik, A., Jr.; Gharaibeh, M.F.; Rudolph, J.K.; Schippers, S.; Müller, A. Electron-impact ionization of moderately charged xenon ions. J. Phys. B At. Mol. Opt. Phys. 2013, 46, 215202. [Google Scholar]
- Borovik, A., Jr.; Gharaibeh, M.F.; Schippers, S.; Müller, A. Plasma rate coefficients for electron-impact ionization of Xeq+ ions (q = 8,…,17). J. Phys. B At. Mol. Opt. Phys. 2015, 48, 035203. [Google Scholar]
- Borovik, A., Jr.; Rausch, J.; Rudolph, J.; Gharaibeh, M.; Schippers, S.; Müller, A. Electron impact ionization of xenon ions. J. Phys. Conf. Ser. 2009, 194, 062014. [Google Scholar]
- Trzhaskovskaya, M.; Nikulin, V.; Clark, R.E.H. Radiative recombination rate coefficients for highly-charged tungsten ions. At. Data Nucl. Data Tables 2010, 96, 1–25. [Google Scholar]
- Boyle, J.; Altun, Z.; Kelly, H.P. Photoionization cross-section calculation of atomic tungsten. Phys. Rev. A 1993, 47, 4811. [Google Scholar]
- Ballance, P.C.; McLaughlin, B.M. Photoionization of the valence shells of the neutral tungsten atom. J. Phys. B At. Mol. Opt. Phys. 2015, 48, 085201. [Google Scholar]
- Chang, J.J. The R-matrix theory of electron-atom scattering using the Dirac Hamiltonian. J. Phys. B At. Mol. Phys. 1975, 8, 2327. [Google Scholar]
- Norrington, P.H.; Grant, I.P. Low-energy electron scattering by Fe XXIII and Fe VII using the Dirac R-matrix method. J. Phys. B At. Mol. Phys. 1987, 20, 4869–4881. [Google Scholar]
- Ballance, C.P.; Griffin, D.C. Relativistic radiatively damped R-matrix calculation of the electron-impact excitation of W46+. J. Phys. B At. Mol. Opt. Phys. 2006, 39, 3617. [Google Scholar]
- Müller, A.; Schippers, S.; Esteves-Macaluso, D.; Habibi, M.; Aguilar, A.; Kilcoyne, A.L.D.; Phaneuf, R.A.; Ballance, C.P.; McLaughlin, B.M. Valence-shell photoionization of Ag-like Xe7+ ions: Experiment and theory. J. Phys. B At. Mol. Opt. Phys. 2014, 47, 215202. [Google Scholar]
- Habibi, M.; Esteves, D.A.; Phaneuf, R.A.; Kilcoyne, A.L.D.; Aguilar, A.; Cisneros, C. Photoionization cross sections for ions of the cerium isonuclear sequence. Phys. Rev. A 2009, 80, 033407. [Google Scholar]
- Müller, A.; Schippers, S.; Hellhund, J.; Kilcoyne, A.L.D.; Phaneuf, R.A.; Ballance, C.P.; McLaughlin, B.M. Single and multiple photoionization of Wq+ tungsten ions in charge states q=1,2,..,5: Experiment and theory. J. Phys. Conf. Ser 2014, 488, 022032. [Google Scholar]
- Li, M.; Fu, Y.; Su, M.; Dong, C.; Koike, F. Dielectronic Recombination of Br-Like Tungsten Ions. Plasma Sci. Technol 2014, 16, 182–187. [Google Scholar]
- Safronova, U.; Safronova, A.; Beiersdorfer, P. Excitation energies, radiative and autoionization rates, dielectronic satellite lines, and dielectronic recombination rates for excited states of Na-like W from Ne-like W. At. Data Nucl. Data Tables 2009, 95, 751–785. [Google Scholar]
- Behar, E.; Mandelbaum, P.; Schwob, J.L. Dielectronic recombination of Ne-like tungsten. Phys. Rev. A 1999, 59, 2787–2793. [Google Scholar]
- Badnell, N.R. Dielectronic recombination of Fe 13+: Benchmarking the M-shell. J. Phys. B At. Mol. Opt. Phys. 2006, 39, 4825–4852. [Google Scholar]
- Safronova, U.I.; Safronova, A.S. Dielectronic recombination of Er-like tungsten. Phys. Rev. A 2012, 85, 032507. [Google Scholar]
- Safronova, U.I.; Safronova, A.S.; Beiersdorfer, P. Excitation energies, radiative and autoionization rates, dielectronic satellite lines, and dielectronic recombination rates for excited states of Yb-like W. J. Phys. B At. Mol. Opt. Phys. 2012, 45, 085001. [Google Scholar]
- Schippers, S. Analytical Expression for the Convolution of a Fano Line Profile with a Gaussian. Int. Rev. At. Mol. Phys. 2011, 2, 151–156. [Google Scholar]
- Badnell, N.R.; Ballance, C.P.; Griffin, D.C.; O’Mullane, M. Dielectronic recombination of W20+(4d104f8): Addressing the half-open f shell. Phys. Rev. A 2012, 85, 052716. [Google Scholar]
- Müller, A.; Wolf, A. Production of Antihydrogen by Recombination of with e+: What Can We Learn from Electron-Ion Collision Studies? Hyperfine Interact 1997, 109, 233–267. [Google Scholar]
- Gao, H.; DeWitt, D.R.; Schuch, R.; Zong, W.; Asp, S.; Pajek, M. Observation of Enhanced Electron-Ion Recombination Rates at Very Low Energies. Phys. Rev. Lett. 1995, 75, 4381–4384. [Google Scholar]
- Gwinner, G.; Hoffknecht, A.; Bartsch, T.; Beutelspacher, M.; Eklöw, N.; Glans, P.; Grieser, M.; Krohn, S.; Lindroth, E.; Müller, A.; et al. Influence of Magnetic Fields on Electron-Ion Recombination at Very Low Energies. Phys. Rev. Lett. 2000, 84, 4822–4825. [Google Scholar]
- Hoffknecht, A.; Brandau, C.; Bartsch, T.; Böhme, C.; Knopp, H.; Schippers, S.; Müller, A.; Kozhuharov, C.; Beckert, K.; Bosch, F.; et al. Recombination of Bare Bi83+ Ions with Electrons. Phys. Rev. A 2001, 63, 012702. [Google Scholar]
- Hoffknecht, A.; Schippers, S.; Müller, A.; Gwinner, G.; Schwalm, D.; Wolf, A. Recombination of Bare Cl17+ Ions in an Electron Cooler. Phys. Scr. 2001, T92, 402–405. [Google Scholar]
- Shi, W.; Böhm, S.; Böhme, C.; Brandau, C.; Hoffknecht, A.; Kieslich, S.; Schippers, S.; Müller, A.; Kozhuharov, C.; Bosch, F.; et al. Recombination of U92+ Ions with Electrons. Eur. Phys. J. D 2001, 15, 145–154. [Google Scholar]
- Heerlein, C.; Zwicknagel, G.; Toepffer, C. Radiative Recombination Enhancement of Bare Ions in Storage Rings with Electron Cooling. Phys. Rev. Lett. 2002, 89, 083202. [Google Scholar]
- Hörndl, M.; Yoshida, S.; Wolf, A.; Gwinner, G.; Burgdörfer, J. Enhancement of Low Energy Electron-Ion Recombination in a Magnetic Field: Influence of Transient Field Effects. Phys. Rev. Lett. 2005, 95, 243201. [Google Scholar]
- Hörndl, M.; Yoshida, S.; Burgdörfer, J.; Gwinner, G.; Wolf, A. Enhanced electron-ion recombination in ion storage rings: Influence of transient field effects. Hyperfine Interact 2006, 173, 67–72. [Google Scholar]
- Müller, A. Plasma Rate Coefficients for Highly Charged Ion-Electron Collisions: New Experimental Access Via Ion Storage Rings. Int. J. Mass Spectrom 1999, 192, 9–22. [Google Scholar]
- Hoffknecht, A.; Uwira, O.; Frank, A.; Schennach, S.; Spies, W.; Wagner, M.; Schippers, S.; Müller, A.; Becker, R.; Kleinod, M.; et al. Recombination of Au25+ with Free Electrons at Very Low Energies. J. Phys. B At. Mol. Opt. Phys. 1998, 31, 2415–2428. [Google Scholar]
- Dzuba, V.A.; Flambaum, V.V.; Gribakin, G.F.; Harabati, C. Chaos-induced enhancement of resonant multielectron recombination in highly charged ions: Statistical theory. Phys. Rev. A 2012, 86, 022714. [Google Scholar]
- Dzuba, V.A.; Flambaum, V.V.; Gribakin, G.F.; Harabati, C.; Kozlov, M.G. Electron recombination, photoionization, and scattering via many-electron compound resonances. Phys. Rev. A 2013, 88, 062713. [Google Scholar]
- Schippers, S.; Bernhardt, D.; Müller, A.; Krantz, C.; Grieser, M.; Repnow, R.; Wolf, A.; Lestinsky, M.; Hahn, M.; Novotný, O.; Savin, D.W. Dielectronic recombination of xenonlike tungsten ions. Phys. Rev. A 2011, 83, 012711. [Google Scholar]
- Stobbe, M. Zur Quantenmechanik Photoelektrischer Prozesse. Ann. Phys. 1930, 7, 661–715. [Google Scholar]
- Müller, A.; Schennach, S.; Wagner, M.; Haselbauer, J.; Uwira, O.; Spies, W.; Jennewein, E.; Becker, R.; Kleinod, M.; Pröbstel, U.; et al. Recombination of free electrons with ions. Phys. Scr. 1991, T37, 62–65. [Google Scholar]
- Uwira, O.; Müller, A.; Spies, W.; Frank, A.; Linkemann, J.; Empacher, L.; Mokler, P.H.; Becker, R.; Kleinod, M.; Ricz, S. Recombination of U28+ Ions in a Dense, Cold Electron Target. Nucl. Instrum. Methods B 1995, 98, 162–164. [Google Scholar]
- Mitnik, D.M.; Pindzola, M.S.; Robicheaux, F.; Badnell, N.R.; Uwira, O.; Müller, A.; Frank, A.; Linkemann, J.; Spies, W.; Angert, N.; et al. Dielectronic Recombination of U28+ Atomic Ions. Phys. Rev. A 1998, 57, 4365–4372. [Google Scholar]
- Baird, S.; Bosser, J.; Carli, C.; Chanel, M.; Lefèvre, P.; Ley, R.; Maccaferri, R.; Maury, S.; Meshkov, I.; Möhl, D.; et al. Measurements of the Lifetime of Pb52+, Pb53+ and Pb54+ Beams at 4.2 MeV Per Nucleon Subject to Electron Cooling. Phys. Lett. B 1995, 361, 184. [Google Scholar]
- Uwira, O.; Müller, A.; Linkemann, J.; Bartsch, T.; Brandau, C.; Schmitt, M.; Wolf, A.; Schwalm, D.; Schuch, R.; Zong, W.; et al. Recombination Measurements at Low Energies with Au49+,50+,51+ at the TSR. Hyperfine Interact 1997, 108, 149–154. [Google Scholar]
- Lindroth, E.; Danared, H.; Glans, P.; Pesic, Z.; Tokman, M.; Vikor, G.; Schuch, R. QED Effects in Cu-Like Pb Recombination Resonances Near Threshold. Phys. Rev. Lett. 2001, 86, 5027–5030. [Google Scholar]
- Schippers, S.; Bernhardt, D.; Grieser, M.; Hahn, M.; Krantz, C.; Lestinsky, M.; Novotný, O.; Repnow, R.; Savin, D.W.; Wolf, A.; Müller, A. Recombination of Au20+ at low electron-ion collision energies. Phys. Scr. 2011, 2011, 014039. [Google Scholar]
- Gribakin, G.F.; Gribakina, A.A.; Flambaum, V.V. Quantum Chaos in Multicharged Ions and Statistical Approach to the Calculation of Electron-Ion Resonant Radiative Recombination. Aust. J. Phys. 1999, 52, 443–457. [Google Scholar]
- Flambaum, V.V.; Gribakina, A.A.; Gribakin, G.F.; Harabati, C. Electron Recombination with Multicharged Ions Via Chaotic Many-Electron States. Phys. Rev. A 2002, 66, 012713. [Google Scholar]
- Gribakin, G.F.; Sahoo, S. Mixing of dielectronic and multiply excited states in electron-ion recombination: A study of Au24+. J. Phys. B At. Mol. Opt. Phys. 2003, 36, 3349–3370. [Google Scholar]
- Ballance, C.P.; Griffin, D.C.; Loch, S.D.; Badnell, N.R. Dielectronic recombination of Au20+: A theoretical description of the resonances at low electron energies. J. Phys. B At. Mol. Opt. Phys. 2012, 45, 045001. [Google Scholar]
- Schippers, S.; Müller, A.; Gwinner, G.; Linkemann, J.; Saghiri, A.A.; Wolf, A. Storage Ring Measurement of the C IV Recombination Rate Coefficient. Astrophys. J 2001, 555, 1027–1037. [Google Scholar]
- Müller, A.; Borovik, A., Jr; Huber, K.; Schippers, S.; Fursa, D.V.; Bray, I. Double-K-vacancy states in electron-impact single ionization of metastable two-electron N5+(1s2s 3S1) ions. Phys. Rev. A 2014, 90, 010701(R). [Google Scholar]
- Müller, A.; Teng, H.; Hofmann, G.; Phaneuf, R.A.; Salzborn, E. Autoionizing Resonances in Electron-Impact Ionization of O5+ Ions. Phys. Rev. A 2000, 62, 062720. [Google Scholar]
- Müller, A.; Phaneuf, R.A.; Aguilar, A.; Gharaibeh, M.F.; Schlachter, A.S.; Alvarez, I.; Cisneros, C.; Hinojosa, G.; McLaughlin, B.M. Photoionization of C2+ Ions: Time-Reversed Recombination of C3+ with Electrons. J. Phys. B At. Mol. Opt. Phys. 2002, 35, L137–L143. [Google Scholar]
- Schippers, S.; Kieslich, S.; Müller, A.; Gwinner, G.; Schnell, M.; Wolf, A.; Bannister, M.; Covington, A.; Zhao, L.B. Interference Effects in the Photorecombination of Argonlike Sc3+ Ions: Storage Ring Experiment and Theory. Phys. Rev. A 2002, 65, 042723. [Google Scholar]
- Schippers, S.; Müller, A.; Ricz, S.; Bannister, M.E.; Dunn, G.H.; Bozek, J.D.; Schlachter, A.S.; Hinojosa, G.; Cisneros, C.; Aguilar, A.; et al. Experimental Link of Photoionization of Sc2+ to Photorecombination of Sc3+: An Application of Detailed Balance in a Unique Atomic System. Phys. Rev. Lett. 2002, 89, 193002. [Google Scholar]
- Müller, A.; Phaneuf, R.A.; Aguilar, A.; Gharaibeh, M.F.; Schlachter, A.S.; Alvarez, I.; Cisneros, C.; Hinojosa, G.; McLaughlin, B.M. Photoionization of C2+ ions. Nucl. Instrum. Methods B 2003, 205, 301–305. [Google Scholar]
- Müller, A.; Schippers, S.; Aguilar, A.; Alvarez, I.; Bannister, M.E.; Bozek, J.; Cisneros, C.; Covington, A.; Dunn, G.H.; Gharaibeh, M.F.; et al. Time-reversal studies in photorecombination and photoionization experiments with ion beams. In Application of Accelerators in Research and Industry; Duggan, J.L., Morgan, I.L., Eds.; American Institute of Physics; Melville, NY, USA, 2003; Volume 680, pp. 191–194. [Google Scholar]
- Schippers, S.; Müller, A.; Ricz, S.; Bannister, M.E.; Dunn, G.H.; Schlachter, A.S.; Hinojosa, G.; Cisneros, C.; Aguilar, A.; Covington, A.M.; et al. Photoionization of Sc2+ Ions by Synchrotron Radiation: Measurements and Absolute Cross Sections in the Photon Energy Range 23–68 eV. Phys. Rev. A 2003, 67, 032702. [Google Scholar]
- Schippers, S.; Müller, A.; Ricz, S.; Bannister, M.E.; Dunn, G.H.; Bozek, J.; Schlachter, A.S.; Hinojosa, G.; Cisneros, C.; Aguilar, A.; et al. Photoionization of Sc2+: Experimental Link with Photorecombination of Sc3+ by Application of Detailed Balance. Nucl. Instrum. Methods B 2003, 205, 297–300. [Google Scholar]
- Schippers, S.; Bartsch, T.; Brandau, C.; Gwinner, G.; Linkemann, J.; Müller, A.; Saghiri, A.A.; Wolf, A. Photorecombination of Ti4+ Ions: Search for Interference Effects, Recombination at Low Energies and Rate Coefficient in Plasmas. J. Phys. B At. Mol. Opt. Phys. 1998, 31, 4873–4886. [Google Scholar]
- Schippers, S.; Müller, A.; Phaneuf, R.A.; van Zoest, T.; Álvarez, I.; Cisneros, C.; Emmons, E.D.; Gharaibeh, M.F.; Hinojosa, G.; Schlachter, A.S.; Scully, S.W.J. Threshold truncation of a ‘giant’ dipole resonance in photoionization of Ti3+. J. Phys. B At. Mol. Opt. Phys. 2004, 37, L209–L216. [Google Scholar]
- Müller, A.; Schippers, S.; Phaneuf, R.A.; Scully, S.W.J.; Aguilar, A.; Covington, A.M.; Álvarez, I.; Cisneros, C.; Emmons, E.D.; Gharaibeh, M.F.; et al. K-shell photoionization of ground-state Li-like carbon ions [C3+]: Experiment, theory and comparison with time-reversed photorecombination. J. Phys. B At. Mol. Opt. Phys. 2009, 42, 235602. [Google Scholar]
- Müller, A.; Schippers, S.; Phaneuf, R.A.; Scully, S.W.J.; Aguilar, A.; Cisneros, C.; Gharaibeh, M.F.; Schlachter, A.S.; McLaughlin, B.M. K-shell photoionization of ground-state Li-like boron ions [B2+]: Experiment and Theory. J. Phys. B At. Mol. Opt. Phys. 2010, 43, 135602. [Google Scholar]
- Müller, A.; Schippers, S.; Phaneuf, R.A.; Scully, S.W.J.; Aguilar, A.; Cisneros, C.; Gharaibeh, M.F.; Schlachter, A.S.; McLaughlin, B.M. K -shell photoionization of Be-like boron (B+) ions: Experiment and theory. J. Phys. B At. Mol. Opt. Phys. 2014, 47, 135201. [Google Scholar]




© 2015 by the authors; licensee MDPI, Basel, Switzerland This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Müller, A. Fusion-Related Ionization and Recombination Data for Tungsten Ions in Low to Moderately High Charge States. Atoms 2015, 3, 120-161. https://doi.org/10.3390/atoms3020120
Müller A. Fusion-Related Ionization and Recombination Data for Tungsten Ions in Low to Moderately High Charge States. Atoms. 2015; 3(2):120-161. https://doi.org/10.3390/atoms3020120
Chicago/Turabian StyleMüller, Alfred. 2015. "Fusion-Related Ionization and Recombination Data for Tungsten Ions in Low to Moderately High Charge States" Atoms 3, no. 2: 120-161. https://doi.org/10.3390/atoms3020120
APA StyleMüller, A. (2015). Fusion-Related Ionization and Recombination Data for Tungsten Ions in Low to Moderately High Charge States. Atoms, 3(2), 120-161. https://doi.org/10.3390/atoms3020120





