Vibrational Excitation of HDO Molecule by Electron Impact
Abstract
1. Introduction
2. Theoretical Approach
2.1. HDO Molecule Properties
2.2. Fixed Nuclei Scattering Matrix
2.3. HDO Vibrational Dynamics
2.4. The Scattering Matrix for an Electron Colliding with HDO
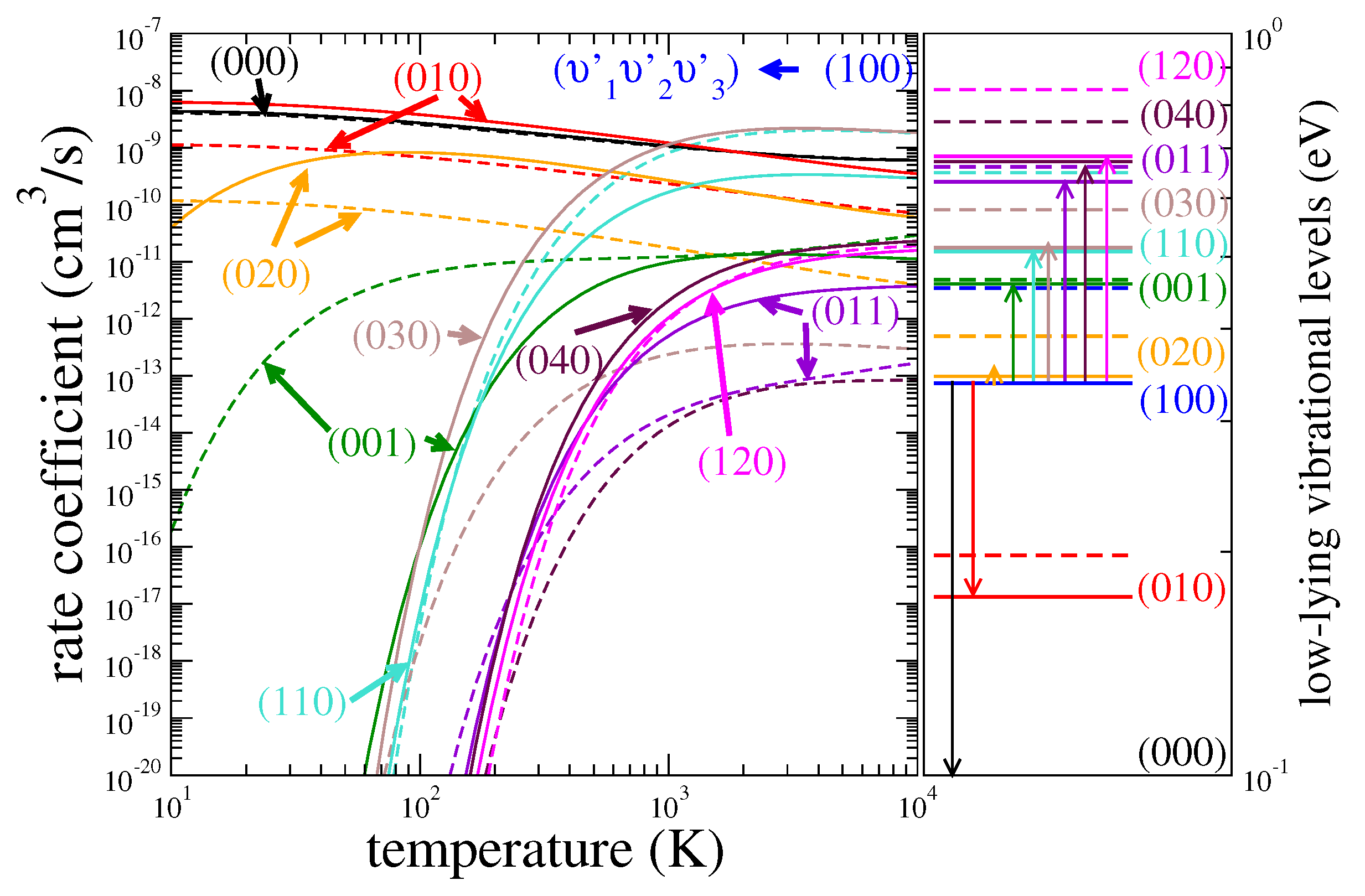
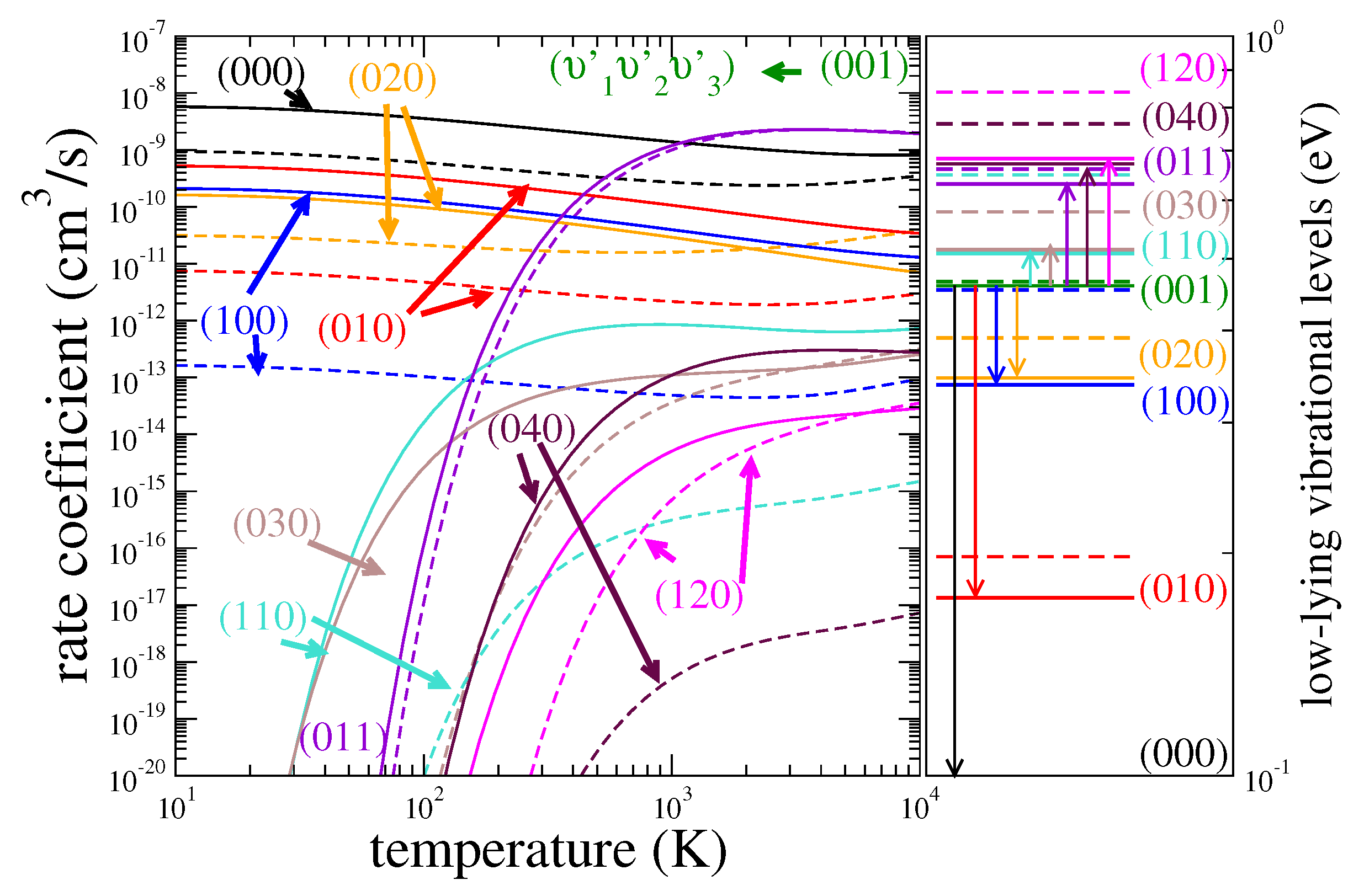
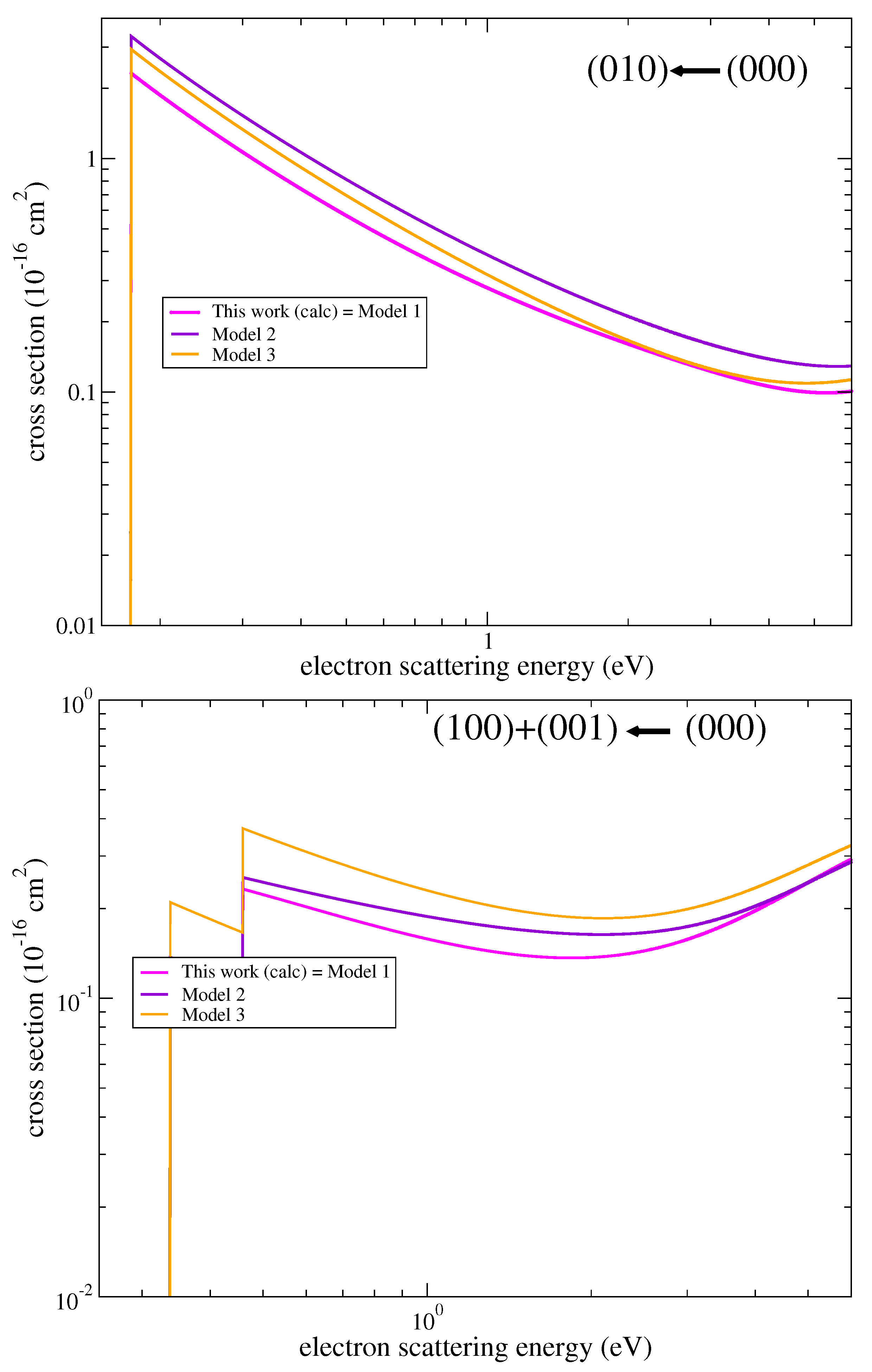
3. Results and Discussions
3.1. Cross Sections
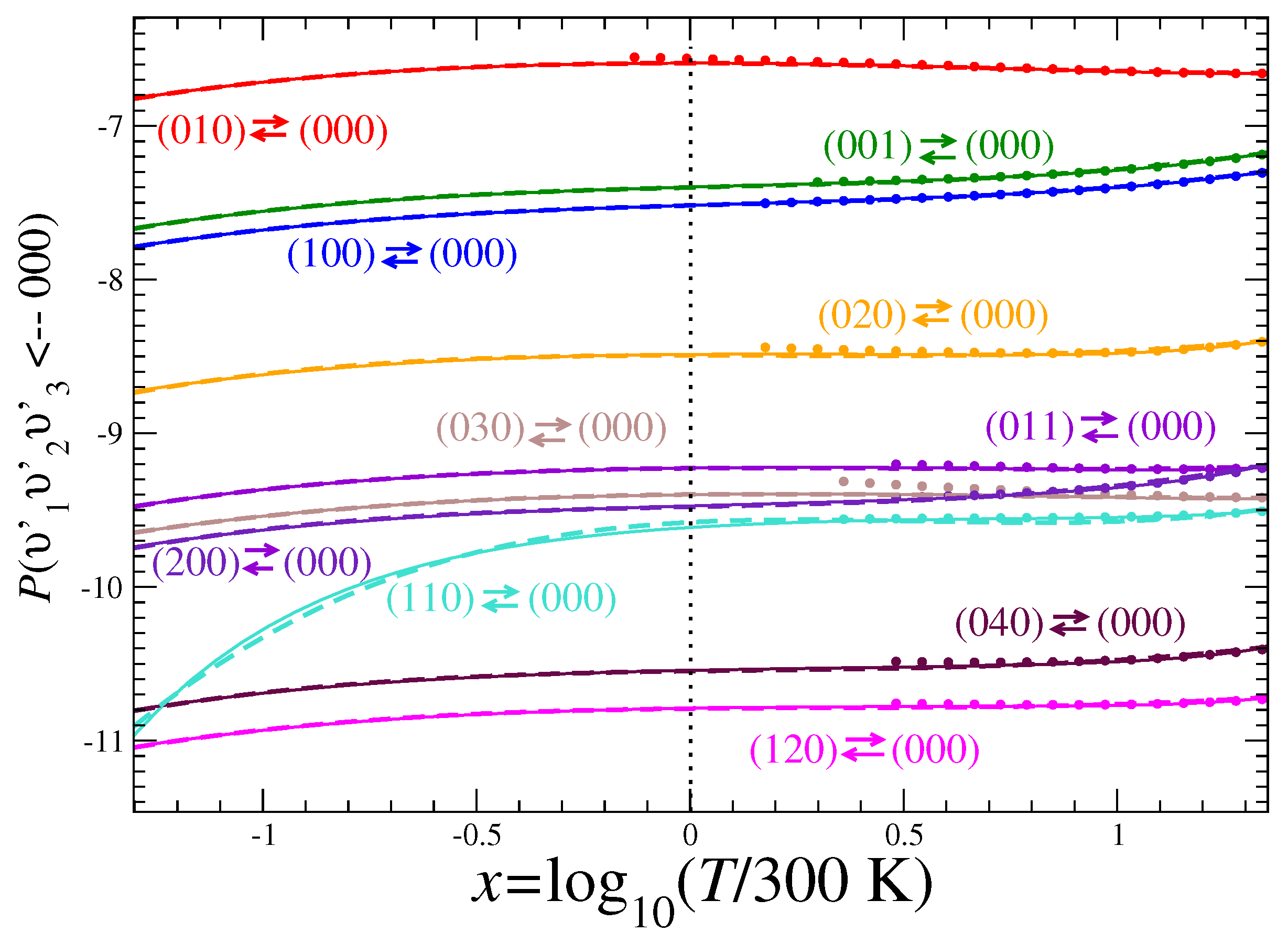
3.2. Rate Coefficients
3.3. Assessment of Uncertainties
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Fitting Parameters for the Calculated Rate Coefficients
| (K) | |||||
|---|---|---|---|---|---|
| 0.0 | −6.59 | −4.74 | −8.68 | 4.06 | |
| 0.0 | −7.52 | 7.82 | −1.95 | 6.13 | |
| 0.0 | −8.49 | 1.52 | −4.71 | 7.29 | |
| 0.0 | −7.40 | 6.87 | −1.79 | 6.69 | |
| 0.0 | −9.40 | 2.68 | −7.94 | 3.55 | |
| 0.0 | −9.58 | 1.47 | −3.72 | 2.30 | |
| 0.0 | −9.23 | 2.28 | −7.14 | 4.45 | |
| 0.0 | −9.48 | 6.05 | −5.46 | 8.16 | |
| 0.0 | −10.50 | 3.78 | −3.46 | 6.84 | |
| 0.0 | −10.80 | 2.84 | −5.52 | 5.54 | |
| 0.0 | −10.10 | 7.55 | −2.79 | 5.47 | |
| 0.0 | −9.90 | 7.30 | −4.94 | 3.72 | |
| 0.0 | −13.70 | 6.45 | −1.78 | 7.04 | |
| 0.0 | −11.8 | 2.60 | −1.37 | 9.12 | |
| 0.0 | −12.40 | 1.09 | −3.84 | 7.86 |
| (K) | |||||
|---|---|---|---|---|---|
| 2019.0 | −6.56 | −2.91 | −1.10 | 5.97 | |
| 0.0 | −7.40 | −5.67 | −8.73 | 4.05 | |
| 0.0 | −6.33 | −2.57 | −8.64 | 4.01 | |
| 0.0 | −8.47 | 9.79 | −7.82 | 4.31 | |
| 0.0 | −7.82 | 1.70 | −3.38 | 2.46 | |
| 0.0 | −7.68 | 7.07 | −2.48 | 5.96 | |
| 0.0 | −7.43 | 7.18 | −1.52 | 6.76 | |
| 0.0 | −9.75 | 3.28 | −7.19 | 3.88 | |
| 0.0 | −9.38 | 2.40 | −8.60 | 3.10 | |
| 0.0 | −9.08 | 2.86 | −6.18 | 4.95 | |
| 0.0 | −9.43 | 2.17 | −7.89 | 3.87 | |
| 0.0 | −9.11 | 2.60 | −6.53 | 4.81 | |
| 0.0 | −11.50 | 8.39 | 4.22 | 1.06 | |
| 0.0 | −9.54 | 6.36 | −1.14 | 8.38 | |
| 0.0 | −10.10 | 3.34 | −4.61 | 6.07 |
| (K) | |||||
|---|---|---|---|---|---|
| 3919.0 | −7.53 | 1.28 | −9.39 | 9.00 | |
| 1899.0 | −7.37 | −3.03 | −1.11 | 6.00 | |
| 0.0 | −7.97 | −1.50 | −1.11 | 6.36 | |
| 0.0 | −8.88 | −1.83 | −7.66 | 5.29 | |
| 0.0 | −7.41 | 1.10 | −4.01 | 2.19 | |
| 0.0 | −6.60 | −8.83 | −8.57 | 4.01 | |
| 0.0 | −9.34 | 3.48 | −6.70 | 5.56 | |
| 0.0 | −7.23 | 7.97 | −1.82 | 6.16 | |
| 0.0 | −8.52 | −1.68 | −7.02 | 6.21 | |
| 0.0 | −8.78 | 3.79 | −4.66 | 5.58 | |
| 0.0 | −7.72 | 6.64 | −2.04 | 6.59 | |
| 0.0 | −7.68 | 7.01 | −1.68 | 6.71 | |
| 0.0 | −9.73 | 2.40 | −8.48 | 3.20 | |
| 0.0 | −8.80 | 3.09 | −6.23 | 4.80 | |
| 0.0 | −10.90 | 3.15 | −3.96 | 6.57 |
| (K) | |||||
|---|---|---|---|---|---|
| 4003.0 | −8.45 | 4.68 | −2.45 | 1.75 | |
| 1983.0 | −6.29 | −3.97 | −9.80 | 5.55 | |
| 84.0 | −7.93 | −2.87 | 2.26 | −1.29 | |
| 0.0 | −9.00 | −2.94 | −1.04 | 3.46 | |
| 0.0 | −6.38 | 1.17 | −4.00 | 2.17 | |
| 0.0 | −6.55 | 1.22 | −8.55 | 3.94 | |
| 0.0 | −8.23 | 1.51 | −7.73 | 4.17 | |
| 0.0 | −8.79 | 4.42 | −3.51 | 6.36 | |
| 0.0 | −7.72 | 4.65 | −3.62 | 6.02 | |
| 0.0 | −7.66 | 5.73 | −2.10 | 6.92 | |
| 0.0 | −7.70 | 7.25 | −1.50 | 6.74 | |
| 0.0 | −7.81 | 7.60 | −1.13 | 6.88 | |
| 0.0 | −9.21 | 2.12 | −8.46 | 3.33 | |
| 0.0 | −10.80 | 9.58 | −1.28 | 5.91 | |
| 0.0 | −8.91 | 2.92 | −5.94 | 5.11 |
| (K) | |||||
|---|---|---|---|---|---|
| 5334.0 | −7.38 | 5.62 | −4.76 | 8.40 | |
| 3315.0 | −8.43 | 2.28 | −1.76 | 9.42 | |
| 1415.0 | −8.85 | −4.49 | −1.04 | 7.68 | |
| 1332.0 | −8.98 | −5.37 | −1.06 | 4.13 | |
| 0.0 | −10.30 | −5.07 | −3.57 | 3.04 | |
| 0.0 | −11.30 | 1.22 | 8.37 | 1.09 | |
| 0.0 | −6.58 | −4.62 | −8.67 | 4.07 | |
| 0.0 | −9.98 | 1.89 | −7.90 | 3.76 | |
| 0.0 | −10.40 | 3.89 | −8.60 | 3.83 | |
| 0.0 | −11.70 | 5.61 | −2.04 | 7.31 | |
| 0.0 | −7.68 | 7.22 | −1.80 | 6.52 | |
| 0.0 | −7.87 | 6.83 | −2.86 | 5.78 | |
| 0.0 | −11.90 | −3.78 | −5.58 | 8.42 | |
| 0.0 | −10.40 | 6.45 | −3.18 | 5.72 | |
| 0.0 | −11.40 | 7.66 | −2.23 | 5.96 |
| (K) | |||||
|---|---|---|---|---|---|
| 5899.0 | −9.62 | 2.54 | −3.35 | 1.55 | |
| 3880.0 | −7.81 | 1.31 | −1.66 | 1.31 | |
| 1980.0 | −7.42 | 8.23 | −2.05 | 8.87 | |
| 1896.0 | −6.39 | 9.84 | −2.02 | 8.34 | |
| 565.0 | −10.30 | −4.01 | −2.91 | 2.50 | |
| 0.0 | −7.46 | −1.49 | −1.12 | 6.22 | |
| 0.0 | −8.51 | −1.57 | −9.79 | 3.51 | |
| 0.0 | −9.68 | 4.65 | −7.19 | 3.38 | |
| 0.0 | −6.82 | 2.31 | −8.55 | 3.88 | |
| 0.0 | −6.23 | −3.01 | −8.57 | 3.97 | |
| 0.0 | −8.34 | 1.34 | −7.43 | 4.50 | |
| 0.0 | −8.94 | 3.21 | −7.44 | 3.65 | |
| 0.0 | −8.17 | 5.33 | −6.08 | 5.84 | |
| 0.0 | −7.60 | 8.32 | −1.69 | 6.10 | |
| 0.0 | −7.63 | 3.81 | −2.79 | 7.21 |
| (K) | |||||
|---|---|---|---|---|---|
| 5964.0 | −9.29 | −4.45 | −1.42 | 7.79 | |
| 3945.0 | −7.55 | −1.49 | 9.67 | 3.73 | |
| 2046.0 | −6.41 | −3.39 | 1.30 | −6.23 | |
| 1962.0 | −6.36 | −3.27 | 1.24 | −6.26 | |
| 630.0 | −11.10 | −3.49 | 4.98 | −1.59 | |
| 65.0 | −7.43 | −2.30 | 8.37 | −4.92 | |
| 0.0 | −9.71 | −1.19 | −3.22 | 1.24 | |
| 0.0 | −7.38 | −4.28 | −8.74 | 4.00 | |
| 0.0 | −6.13 | 3.15 | −8.58 | 3.94 | |
| 0.0 | −7.96 | −1.80 | −8.68 | 4.60 | |
| 0.0 | −10.20 | 7.22 | −5.19 | 3.76 | |
| 0.0 | −8.23 | 1.48 | −7.25 | 4.60 | |
| 0.0 | −7.61 | 2.58 | −4.73 | 6.02 | |
| 0.0 | −7.48 | 7.71 | −1.82 | 6.29 | |
| 0.0 | −8.24 | 8.01 | −1.81 | 6.14 |
| (K) | |||||
|---|---|---|---|---|---|
| 7323.0 | −9.23 | 1.63 | −2.99 | 1.33 | |
| 5303.0 | −7.35 | −6.01 | 4.11 | 6.25 | |
| 3404.0 | −9.29 | 1.76 | −2.05 | 1.30 | |
| 3320.0 | −8.18 | 1.14 | −1.56 | 8.56 | |
| 1988.0 | −6.47 | −1.74 | −9.82 | 3.04 | |
| 1424.0 | −8.41 | −2.02 | 2.70 | 5.97 | |
| 1358.0 | −9.54 | −3.62 | 1.67 | 1.58 | |
| 0.0 | −10.70 | −2.80 | −4.06 | 8.75 | |
| 0.0 | −10.10 | 1.32 | −7.85 | 3.39 | |
| 0.0 | −9.84 | −1.43 | −4.28 | 1.20 | |
| 0.0 | −6.84 | −3.66 | -8.68 | 4.02 | |
| 0.0 | −6.42 | −1.55 | −8.57 | 4.02 | |
| 0.0 | −9.77 | −8.72 | −8.83 | 4.18 | |
| 0.0 | −10.20 | 2.07 | −7.99 | 3.60 | |
| 0.0 | −11.30 | 7.89 | −9.20 | 7.24 |
| (K) | |||||
|---|---|---|---|---|---|
| 7717.0 | −9.35 | -2.31 | 2.05 | 2.61 | |
| 5698.0 | −9.77 | 1.65 | −2.53 | 1.05 | |
| 3799.0 | −7.18 | 3.36 | −2.62 | 7.41 | |
| 3715.0 | −8.76 | 5.81 | −1.40 | 1.19 | |
| 2383.0 | −9.95 | −1.88 | −9.87 | 5.24 | |
| 1818.0 | −9.66 | 3.91 | −1.05 | 5.33 | |
| 1753.0 | −7.33 | −7.90 | −6.55 | 4.50 | |
| 395.0 | −10.70 | −1.74 | 5.08 | 7.44 | |
| 0.0 | −7.73 | −1.34 | −1.07 | 6.04 | |
| 0.0 | −9.39 | −7.53 | −8.64 | 5.50 | |
| 0.0 | −8.41 | −1.47 | −8.63 | 4.43 | |
| 0.0 | −9.04 | −2.09 | −7.34 | 5.69 | |
| 0.0 | −7.12 | 1.08 | −8.57 | 3.93 | |
| 0.0 | −6.67 | −5.60 | −8.65 | 4.11 | |
| 0.0 | −8.58 | −4.43 | −8.58 | 4.14 |
| (K) | |||||
|---|---|---|---|---|---|
| 7798.0 | −10.40 | −9.53 | −3.15 | 8.97 | |
| 5779.0 | −9.35 | 8.33 | −2.20 | 8.62 | |
| 3879.0 | −8.44 | −2.06 | −2.58 | 1.68 | |
| 3796.0 | −7.62 | −9.95 | 2.05 | 5.92 | |
| 2464.0 | −10.30 | −1.21 | −4.51 | 4.22 | |
| 1899.0 | −6.73 | −1.42 | −1.12 | 2.91 | |
| 1834.0 | −6.02 | −1.86 | 1.79 | 2.26 | |
| 476.0 | −9.97 | −2.93 | 2.44 | −8.37 | |
| 81.0 | −7.65 | −3.84 | 5.15 | −2.94 | |
| 0.0 | −7.67 | −1.33 | −1.08 | 5.73 | |
| 0.0 | −9.82 | −1.20 | −7.77 | 9.36 | |
| 0.0 | −8.55 | −2.07 | −9.83 | 3.68 | |
| 0.0 | −5.96 | 2.88 | −8.54 | 3.89 | |
| 0.0 | −7.25 | 3.37 | −8.49 | 3.89 | |
| 0.0 | −9.24 | −4.27 | −5.06 | 8.34 |
| (K) | |||||
|---|---|---|---|---|---|
| 7922.0 | −10.80 | 6.96 | −1.87 | 1.13 | |
| 5903.0 | −9.04 | 8.26 | −2.27 | 1.24 | |
| 4003.0 | -8.73 | −9.34 | −7.01 | 7.80 | |
| 3919.0 | −7.56 | −6.47 | −1.50 | 9.16 | |
| 2588.0 | −11.70 | −8.99 | −1.11 | 1.41 | |
| 2023.0 | −6.14 | −1.56 | −6.57 | 2.98 | |
| 1958.0 | −7.84 | −2.08 | −3.95 | 4.28 | |
| 599.0 | −9.66 | -4.69 | 1.24 | 1.05 | |
| 205.0 | −9.32 | −2.67 | 1.66 | −5.33 | |
| 124.0 | −7.61 | −3.09 | 1.81 | −8.37 | |
| 0.0 | −8.53 | −2.02 | −1.00 | 3.46 | |
| 0.0 | −8.84 | 1.20 | −8.83 | 3.64 | |
| 0.0 | −7.57 | 9.46 | −8.38 | 3.75 | |
| 0.0 | −7.26 | −1.94 | −8.69 | 3.94 | |
| 0.0 | −6.12 | 8.98 | −8.53 | 3.95 |
| (K) | |||||
|---|---|---|---|---|---|
| 9230.0 | −9.84 | −1.01 | 1.61 | −4.73 | |
| 7211.0 | −9.47 | 2.39 | −3.53 | 1.37 | |
| 5312.0 | −7.73 | 1.14 | −9.55 | 9.54 | |
| 5228.0 | −7.66 | 2.04 | −1.11 | 7.45 | |
| 3896.0 | −7.67 | 9.01 | −8.64 | 9.79 | |
| 3331.0 | −8.34 | 8.75 | −2.17 | 1.08 | |
| 3266.0 | −10.20 | 5.44 | −5.29 | 3.84 | |
| 1908.0 | −6.80 | −5.36 | −8.89 | 5.31 | |
| 1513.0 | −8.42 | 2.85 | −1.58 | 7.71 | |
| 1432.0 | −9.82 | −3.42 | −3.10 | 2.17 | |
| 1308.0 | −8.48 | −1.00 | −5.44 | 2.67 | |
| 0.0 | −7.58 | −1.76 | −1.18 | 6.57 | |
| 0.0 | −9.72 | −9.64 | −8.63 | 3.76 | |
| 0.0 | −10.30 | −2.39 | −9.16 | 1.70 | |
| 0.0 | −9.61 | −1.31 | −6.50 | 9.83 |
| (K) | |||||
|---|---|---|---|---|---|
| 9283.0 | −9.88 | −3.79 | 3.64 | −7.03 | |
| 7264.0 | −9.02 | −2.36 | −1.73 | 1.07 | |
| 5364.0 | −7.53 | −1.91 | 1.22 | 4.36 | |
| 5280.0 | −7.66 | −1.98 | 1.50 | 3.59 | |
| 3949.0 | −7.74 | −1.49 | 8.54 | 3.92 | |
| 3384.0 | −8.81 | −1.54 | 5.43 | 2.97 | |
| 3318.0 | −8.08 | −1.74 | −4.00 | 6.75 | |
| 1960.0 | −6.24 | −3.24 | 1.17 | −2.69 | |
| 1565.0 | −8.84 | −3.78 | 1.36 | 1.98 | |
| 1485.0 | −8.35 | −3.96 | 1.66 | −2.91 | |
| 1361.0 | −8.66 | −3.65 | 1.84 | −3.57 | |
| 52.0 | −7.52 | −3.40 | 2.83 | −1.63 | |
| 0.0 | −9.32 | 7.34 | −8.42 | 3.38 | |
| 0.0 | −10.10 | −1.62 | 2.11 | 1.68 | |
| 0.0 | −9.99 | −1.10 | −5.21 | 1.01 |
| (K) | |||||
|---|---|---|---|---|---|
| 9626.0 | −13.50 | −2.82 | 2.45 | −8.39 | |
| 7607.0 | −11.10 | −8.69 | 9.51 | −1.87 | |
| 5707.0 | −9.69 | 6.52 | −2.09 | 8.55 | |
| 5623.0 | −9.17 | 6.66 | −2.13 | 8.89 | |
| 4292.0 | −11.80 | −2.64 | −3.28 | 2.36 | |
| 3727.0 | −8.06 | −1.25 | −6.63 | 8.97 | |
| 3662.0 | −7.54 | −6.98 | −4.73 | 8.08 | |
| 2303.0 | −9.68 | −1.41 | −4.94 | 4.96 | |
| 1909.0 | −7.02 | −1.71 | 7.77 | 2.49 | |
| 1828.0 | −5.86 | −1.67 | 8.27 | 2.38 | |
| 1704.0 | −7.47 | −1.63 | 2.50 | 1.45 | |
| 396.0 | −9.59 | −3.17 | 2.46 | −8.56 | |
| 343.0 | −9.23 | −2.50 | 2.13 | −8.14 | |
| 0.0 | −7.88 | −1.37 | −1.10 | 5.80 | |
| 0.0 | −8.09 | −1.13 | −1.08 | 5.11 |
| (K) | |||||
|---|---|---|---|---|---|
| 9707.0 | −11.40 | −8.75 | 7.08 | −1.09 | |
| 7688.0 | −9.39 | −2.84 | 2.59 | 1.31 | |
| 5789.0 | −8.76 | 6.86 | −2.11 | 1.17 | |
| 5705.0 | −10.70 | −2.56 | 6.10 | 3.88 | |
| 4373.0 | −10.40 | 6.02 | −4.26 | 7.32 | |
| 3808.0 | −7.55 | 1.50 | 1.15 | 6.08 | |
| 3743.0 | −7.41 | −2.19 | 3.35 | 7.07 | |
| 2385.0 | −10.10 | −1.17 | −9.40 | 2.53 | |
| 1990.0 | −6.56 | −1.79 | 1.02 | 3.07 | |
| 1909.0 | −7.15 | −1.70 | 1.17 | 2.29 | |
| 1785.0 | −7.16 | −1.62 | −5.08 | 2.90 | |
| 477.0 | −10.20 | −4.19 | 1.95 | 2.04 | |
| 424.0 | −9.97 | −4.69 | 2.04 | 1.40 | |
| 81.0 | −7.84 | −2.68 | 2.08 | −1.22 | |
| 0.0 | −7.76 | −1.10 | −9.94 | 5.51 |
| (K) | |||||
|---|---|---|---|---|---|
| 9854.0 | −12.20 | −4.74 | 2.44 | 2.25 | |
| 7835.0 | −10.00 | −2.75 | −1.05 | 9.97 | |
| 5935.0 | −10.80 | −1.08 | −3.92 | 9.31 | |
| 5851.0 | −8.83 | 1.80 | −1.83 | 1.17 | |
| 4520.0 | −11.30 | −6.57 | 4.13 | 5.19 | |
| 3955.0 | −7.54 | −5.39 | −7.11 | 1.16 | |
| 3890.0 | −8.13 | −9.82 | 7.36 | 4.69 | |
| 2531.0 | −11.20 | −6.30 | −1.97 | 1.12 | |
| 2137.0 | −8.44 | −2.38 | 3.64 | 2.42 | |
| 2056.0 | −9.08 | −2.68 | −6.19 | 1.12 | |
| 1932.0 | −5.98 | −2.39 | 5.88 | 1.16 | |
| 624.0 | −9.47 | −3.87 | 6.80 | 8.65 | |
| 571.0 | −9.80 | −4.89 | 2.15 | 3.94 | |
| 228.0 | −7.97 | −4.10 | 2.55 | −9.86 | |
| 147.0 | −7.66 | −3.84 | 3.20 | −1.42 |
References
- van Dishoeck, E.F.; Kristensen, L.E.; Mottram, J.C.; Benz, A.O.; Bergin, E.A.; Caselli, P.; Herpin, F.; Hogerheijde, M.R.; Johnstone, D.; Liseau, R.; et al. Water in star-forming regions: Physics and chemistry from clouds to disks as probed by Herschel spectroscopy. Astron. Astrophys. 2021, 648, A24. [Google Scholar] [CrossRef]
- Tobin, J.J.; van’t Hoff, M.L.R.; Leemker, M.; van Dishoeck, E.F.; Paneque-Carreño, T.; Furuya, K.; Harsono, D.; Persson, M.V.; Cleeves, L.I.; Sheehan, P.D.; et al. Deuterium-enriched water ties planet-forming disks to comets and protostars. Nature 2023, 615, 227–230. [Google Scholar] [CrossRef]
- Mahieux, A.; Viscardy, S.; Yelle, R.V.; Karyu, H.; Chamberlain, S.; Robert, S.; Piccialli, A.; Trompet, L.; Erwin, J.T.; Ubukata, S.; et al. Unexpected increase of the deuterium to hydrogen ratio in the Venus mesosphere. Proc. Natl. Acad. Sci. USA 2024, 121, e2401638121. [Google Scholar] [CrossRef] [PubMed]
- Tennyson, J.; Yurchenko, S.N.; Zhang, J.; Bowesman, C.A.; Brady, R.P.; Buldyreva, J.; Chubb, K.L.; Gamache, R.R.; Gorman, M.N.; Guest, E.R.; et al. The 2024 release of the ExoMol database: Molecular line lists for exoplanet and other hot atmospheres. J. Quant. Spectrosc. Radiat. Transfer 2024, 326, 109083. [Google Scholar] [CrossRef]
- Voronin, B.A.; Tennyson, J.; Tolchenov, R.N.; Lugovskoy, A.A.; Yurchenko, S.N. A high accuracy computed line list for the HDO molecule. Mon. Not. R. Astron. Soc. 2010, 402, 492–496. [Google Scholar] [CrossRef]
- Villanueva, G.L.; Mumma, M.J.; Bonev, B.P.; Novak, R.E.; Barber, R.J.; Disanti, M.A. Water in planetary and cometary atmospheres: H2O/HDO transmittance and fluorescence models. J. Quant. Spectrosc. Radiat. Transfer 2012, 113, 202–220. [Google Scholar] [CrossRef]
- García Muñoz, A.; Asensio Ramos, A.; Faure, A. NLTE modelling of water-rich exoplanet atmospheres. Cooling and heating rates. Icarus 2024, 415, 116080. [Google Scholar] [CrossRef]
- Faure, A.; Lique, F.; Loreau, J. The Effect of H2O and Electron Collisions on Rotational Populations of Cometary CO. In European Conference on Laboratory Astrophysics ECLA2020; The Interplay of Dust; Springer: Cham, Switzerland, 2023; pp. 281–286. [Google Scholar] [CrossRef]
- Faure, A.; Żółtowski, M.; Wiesenfeld, L.; Lique, F.; Bergeat, A. The rotational excitation of the water isotopologues by molecular hydrogen. Mon. Not. R. Astron. Soc. 2024, 527, 3087–3093. [Google Scholar] [CrossRef]
- Faure, A.; Gorfinkiel, J.D.; Tennyson, J. Electron-impact rotational excitation of water. Mon. Not. R. Astron. Soc. 2004, 347, 323–333. [Google Scholar] [CrossRef]
- García-Vázquez, R.M.; Bergeat, A.; Denis-Alpizar, O.; Faure, A.; Stoecklin, T.; Morales, S.B. Scattering resonances in the rotational excitation of HDO by Ne and normal-H2: Theory and experiment. Faraday Discuss. 2024, 251, 205–224. [Google Scholar] [CrossRef]
- Ayouz, M.; Faure, A.; Kokoouline, V. Theoretical study of the electron-induced vibrational excitation of H2O. Astron. Astrophys. 2024, 687, A3. [Google Scholar] [CrossRef]
- Song, M.Y.; Cho, H.; Karwasz, G.P.; Kokoouline, V.; Nakamura, Y.; Tennyson, J.; Faure, A.; Mason, N.J.; Itikawa, Y. Cross Sections for Electron Collisions with H2O. J. Phys. Chem. Ref. Data 2021, 50, 023103. [Google Scholar] [CrossRef]
- Werner, H.J.; Knowles, P.J.; Knizia, G.; Manby, F.R.; Schütz, M. Molpro: A general-purpose quantum chemistry program package. WIREs Comput. Mol. Sci. 2012, 2, 242–253. [Google Scholar] [CrossRef]
- Tennyson, J.; Brown, D.B.; Munro, J.J.; Rozum, I.; Varambhia, H.N.; Vinci, N. Quantemol-N: An expert system for performing electron molecule collision calculations using the R-matrix method. J. Phys. Conf. Ser. 2007, 86, 012001. [Google Scholar] [CrossRef]
- Ayouz, M.; Faure, A.; Tennyson, J.; Tudorovskaya, M.; Kokoouline, V. Cross sections and rate coefficients for vibrational excitation of H2O by electron impact. Atoms 2021, 9, 62. [Google Scholar] [CrossRef]
- Shimanouchi, T. Tables of Molecular Vibrational Frequencies: Part 6. J. Phys. Chem. Ref. Data 1973, 2, 121–162. [Google Scholar] [CrossRef]
- Császár, A.G.; Czakó, G.; Furtenbacher, T.; Tennyson, J.; Szalay, V.; Shirin, S.V.; Zobov, N.F.; Polyansky, O.L. On equilibrium structures of the water molecule. J. Chem. Phys. 2005, 122, 214305. [Google Scholar] [CrossRef]
- Tennyson, J. Electron–molecule collision calculations using the R-matrix method. Phys. Rep. 2010, 491, 29–76. [Google Scholar] [CrossRef]
- Carr, J.; Galiatsatos, P.; Gorfinkiel, J.; Harvey, A.; Lysaght, M.; Madden, D.; Mašín, Z.; Plummer, M.; Tennyson, J.; Varambhia, H. UKRmol: A low-energy electron- and positron-molecule scattering suite. Euro. Phys. J. D 2012, 66, 58. [Google Scholar] [CrossRef]
- Kokoouline, V.; Dulieu, O.; Kosloff, R.; Masnou-Seeuws, F. Mapped Fourier methods for long-range molecules: Application to perturbations in the Rb2 (0u+) photoassociation spectrum. J. Chem. Phys. 1999, 110, 9865. [Google Scholar] [CrossRef]
- Mizus, I.I.; Kyuberis, A.A.; Zobov, N.F.; Makhnev, V.Y.; Polyansky, O.L.; Tennyson, J. High-accuracy water potential energy surface for the calculation of infrared spectra. Philos. Trans. R. Soc. A 2018, 376. [Google Scholar] [CrossRef] [PubMed]
- Tennyson, J.; Bernath, P.F.; Brown, L.R.; Campargue, A.; Császár, A.G.; Daumont, L.; Gamache, R.R.; Hodges, J.T.; Naumenko, O.V.; Polyansky, O.L.; et al. IUPAC critical evaluation of the rotational—Vibrational spectra of water vapor. Part II: Energy levels and transition wavenumbers for HD16O, HD17O, and HD18O. J. Quant. Spectrosc. Radiat. Transfer 2010, 111, 2160–2184. [Google Scholar] [CrossRef]
- Zobov, N.F.; Koshelev, M.A.; Makarov, D.S.; Makhnev, V.Y.; Boyarkin, O.V.; Tyuterev, V.G.; Tennyson, J.; Polyansky, O.L. A global line list for HDO between 0 and 35000 cm−1 constructed using multiphoton spectra. J. Quant. Spectrosc. Radiat. Transfer 2021, 271, 107694. [Google Scholar] [CrossRef]
- Sironneau, V.T.; Hodges, J.T. Line shapes, positions and intensities of water transitions near 1.28 μm. J. Quant. Spectrosc. Radiat. Transfer 2015, 152, 1–15. [Google Scholar] [CrossRef]
- Khakoo, M.A.; Muse, J.; Campbell, C.; Lopes, M.C.A.; Silva, H.; Winstead, C.; McKoy, V. Low energy electron scattering from polyatomic targets. J. Phys. Conf. Ser. 2009, 194, 012027. [Google Scholar] [CrossRef]
- El-Zein, A.A.A.; Brunger, M.J.; Newell, W.R. Excitation of vibrational quanta in water by electron impact. J. Phys. B At. Mol. Opt. Phys. 2000, 33, 5033. [Google Scholar] [CrossRef]
- Shyn, T.W.; Cho, S.Y.; Cravens, T.E. Vibrational-excitation cross sections of water molecules by electron impact. Phys. Rev. A 1988, 38, 678–682. [Google Scholar] [CrossRef]
- Seng, G.; Linder, F. Vibrational excitation of polar molecules by electron impact. II. Direct and resonant excitation in H2O. J. Phys. B At. Mol. Phys. 1976, 9, 2539. [Google Scholar] [CrossRef]
- Nishimura, T.; Gianturco, F.A. Vibrational excitation of water by low-energy electron scattering: Calculations and experiments. Europhys. Lett. 2004, 65, 179–185. [Google Scholar] [CrossRef][Green Version]
- Curà k, R.; Cársky, P. Vibrationally inelastic electron scattering on polyatomic molecules by the discrete momentum representation (DMR) method. J. Phys. B At. Mol. Opt. Phys. 2003, 36, 2165. [Google Scholar] [CrossRef]
- Itikawa, Y.; Mason, N. Rotational excitation of molecules by electron collisions. Phys. Rep. 2005, 414, 1. [Google Scholar] [CrossRef]
- Yousfi, M.; Benabdessadok, M.D. Boltzmann equation analysis of electron-molecule collision cross sections in water vapor and ammonia. J. Appl. Phys. 1996, 80, 6619–6630. [Google Scholar] [CrossRef]
- Ayouz, M.A.; Buch, A. Theoretical Study of the Dissociative Recombination and Vibrational (De-)Excitation of HCNH+ and Its Isomers by Electron Impact. Atoms 2024, 12, 64. [Google Scholar] [CrossRef]
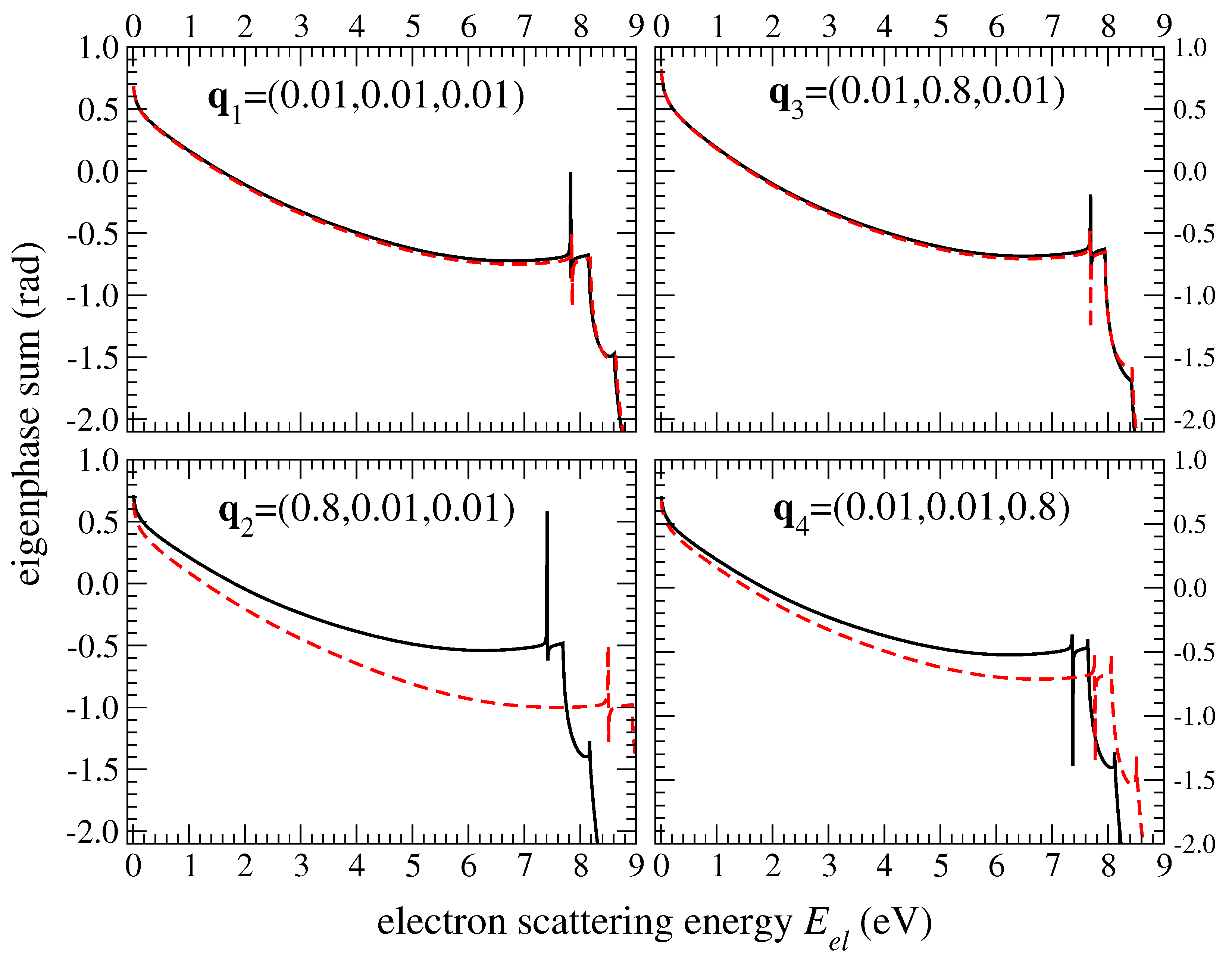
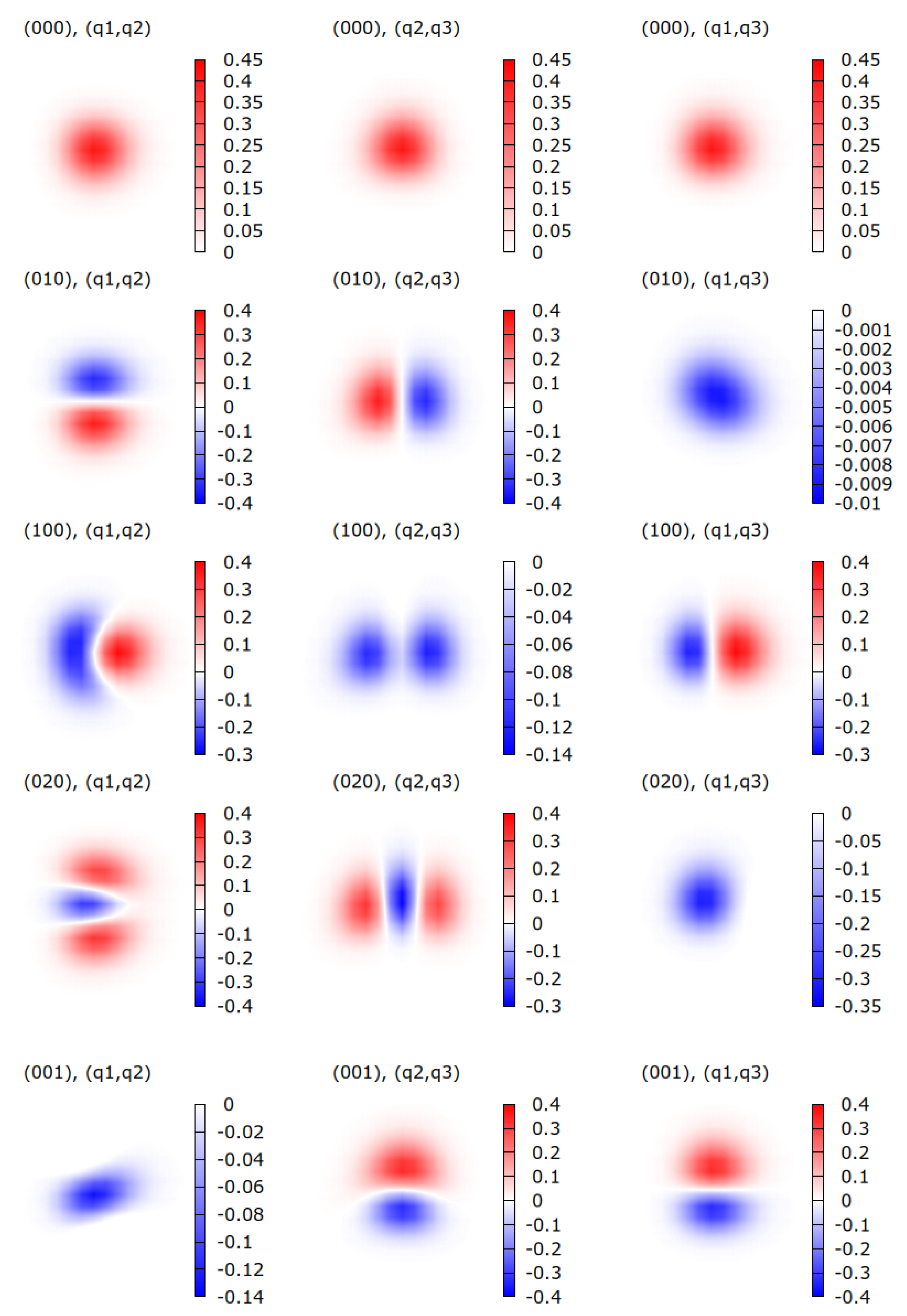
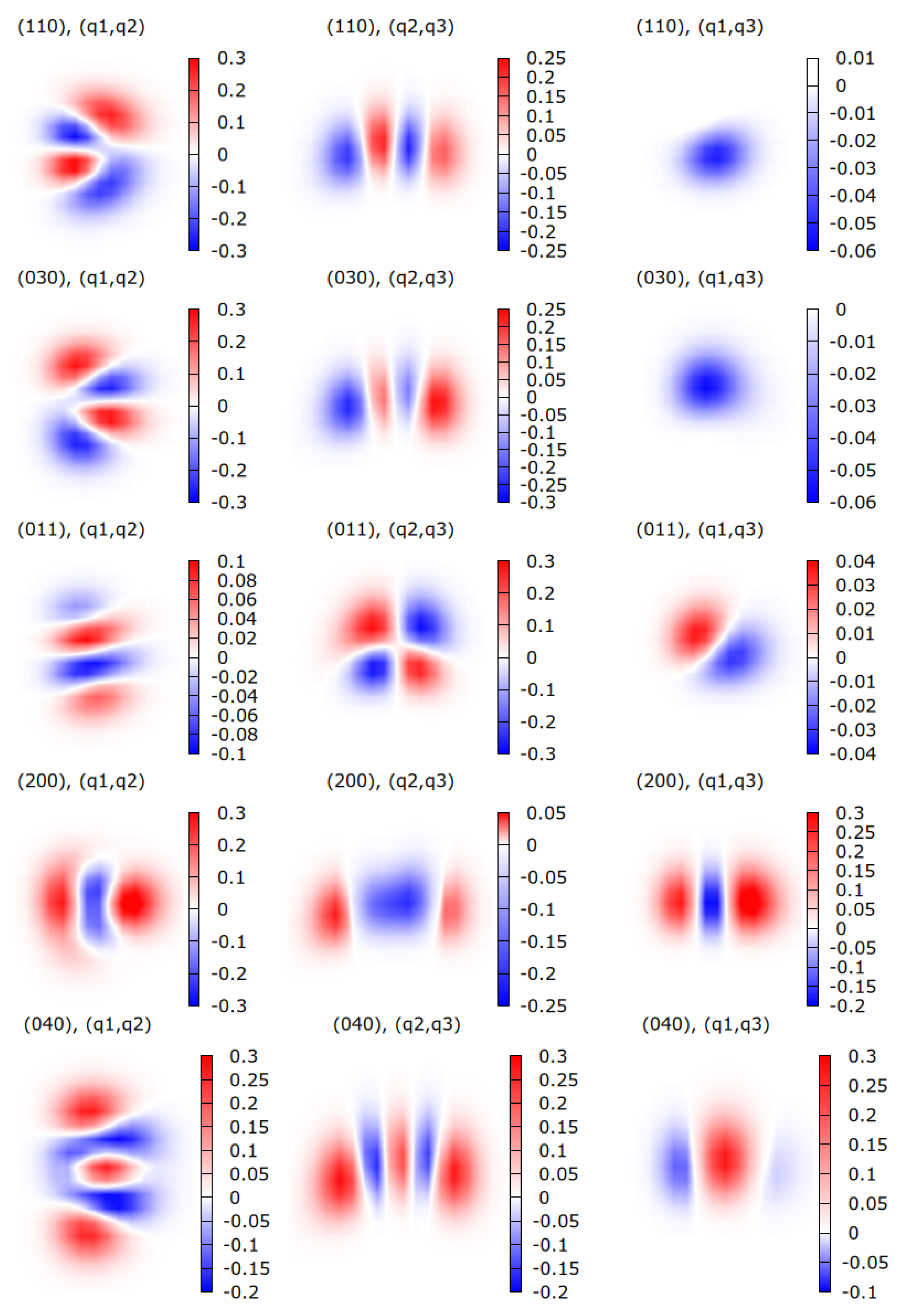
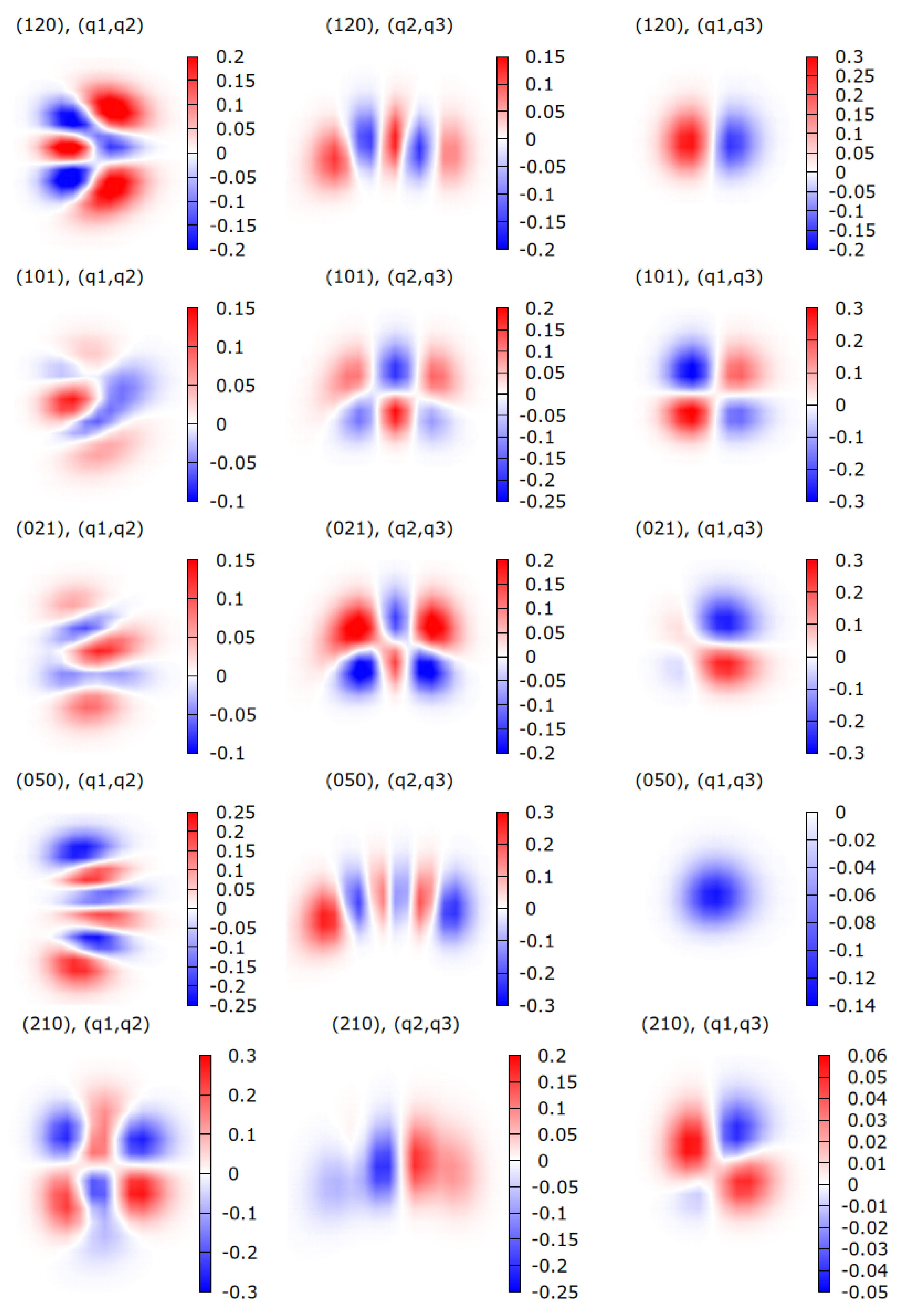
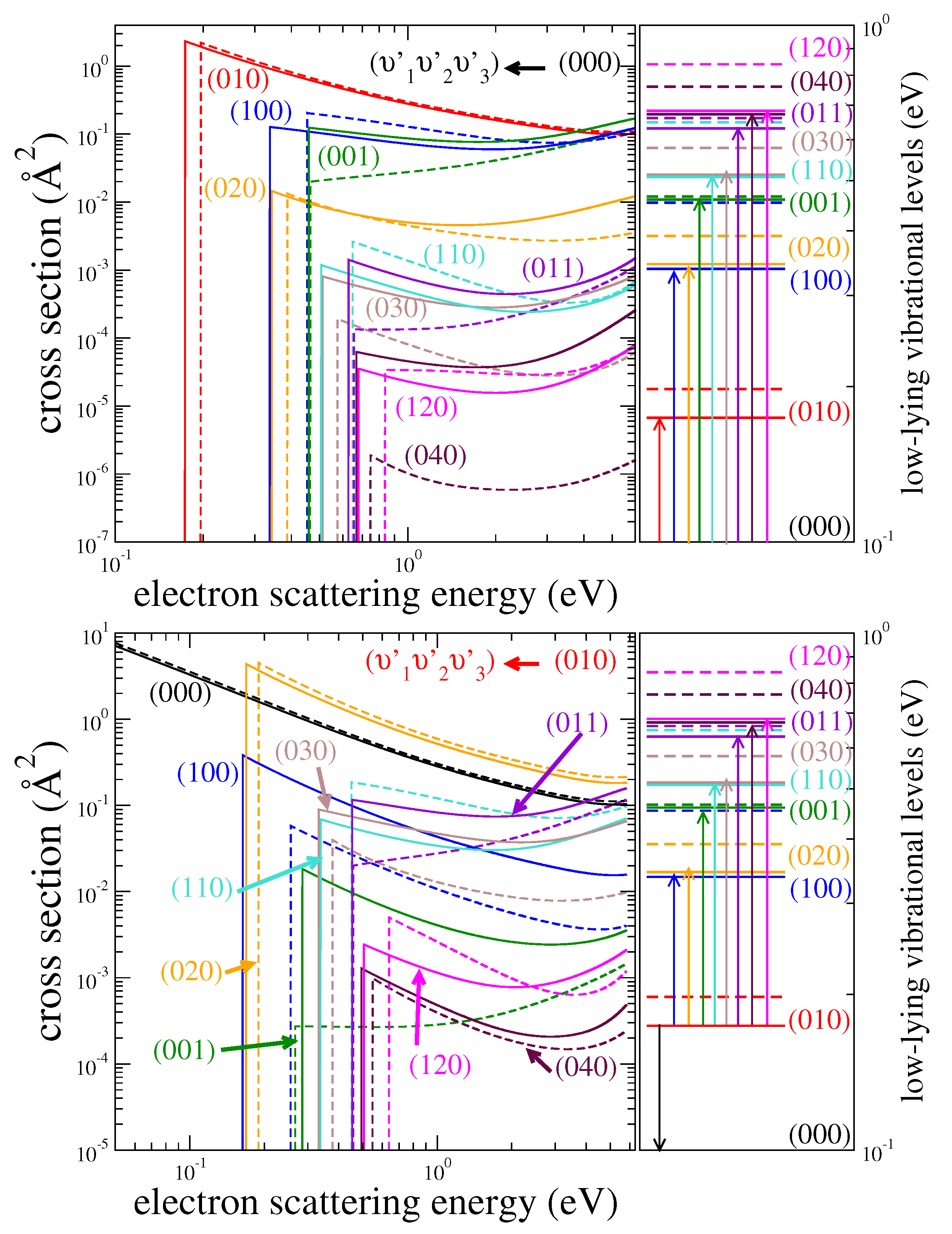
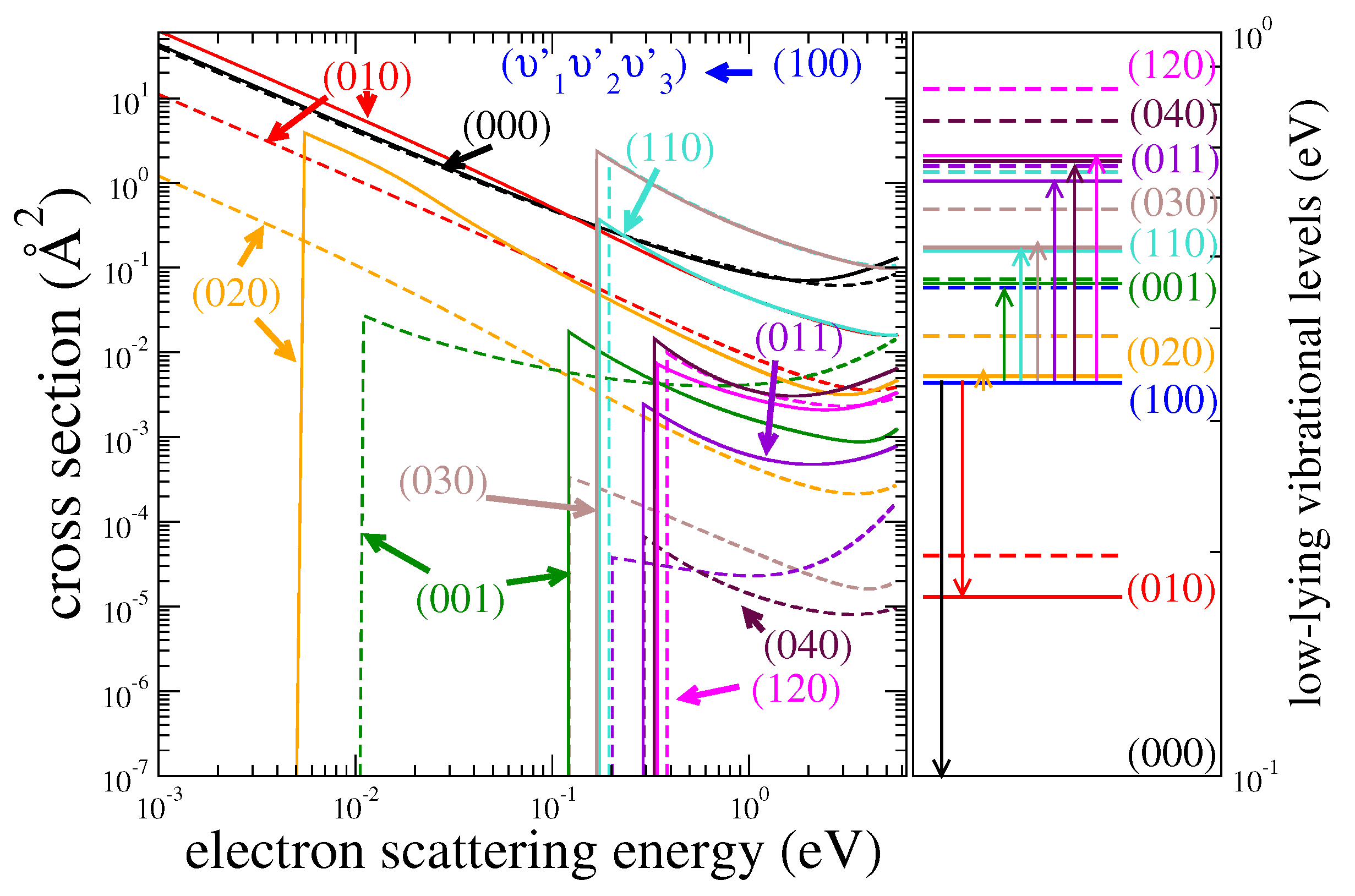

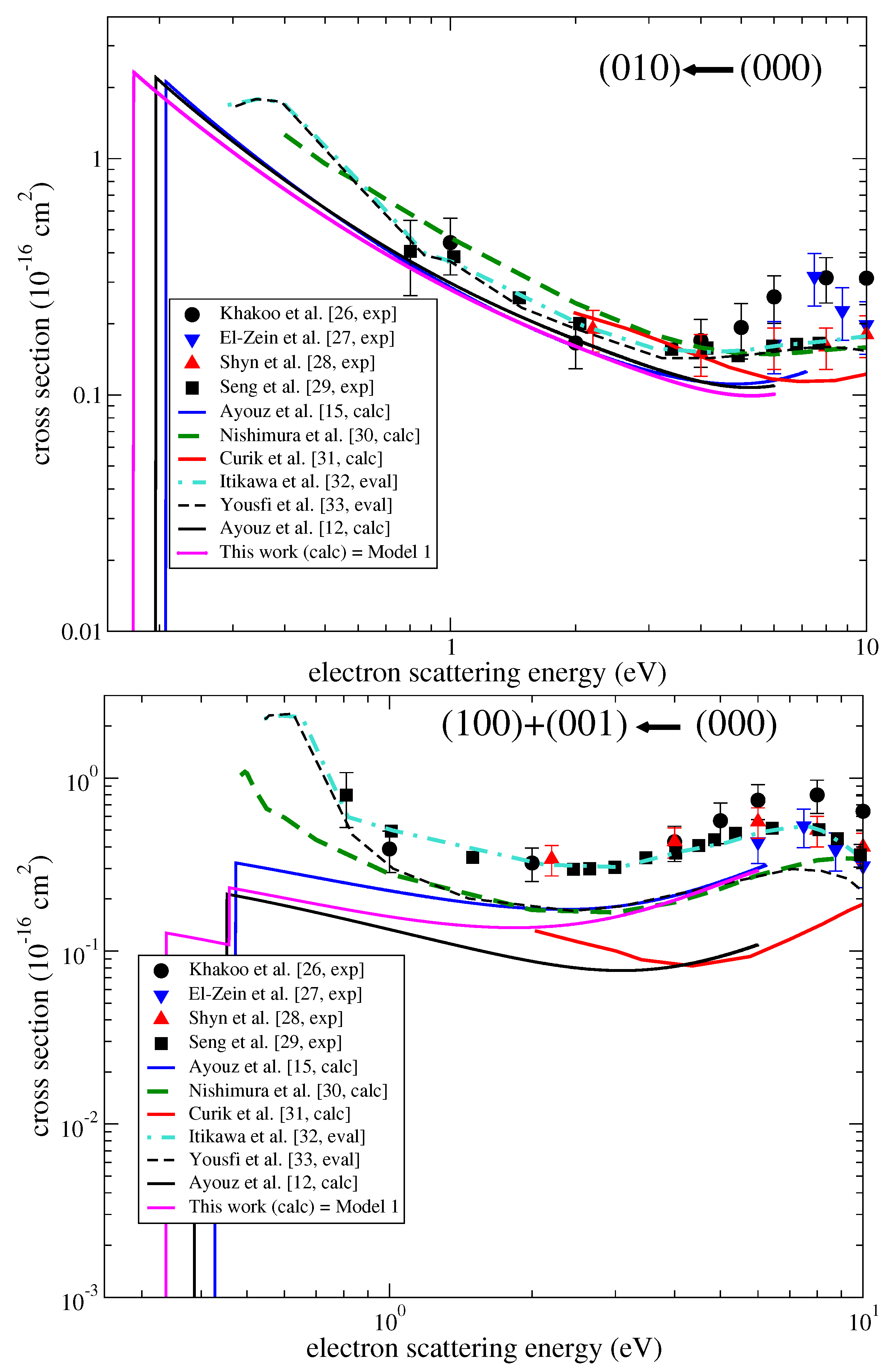
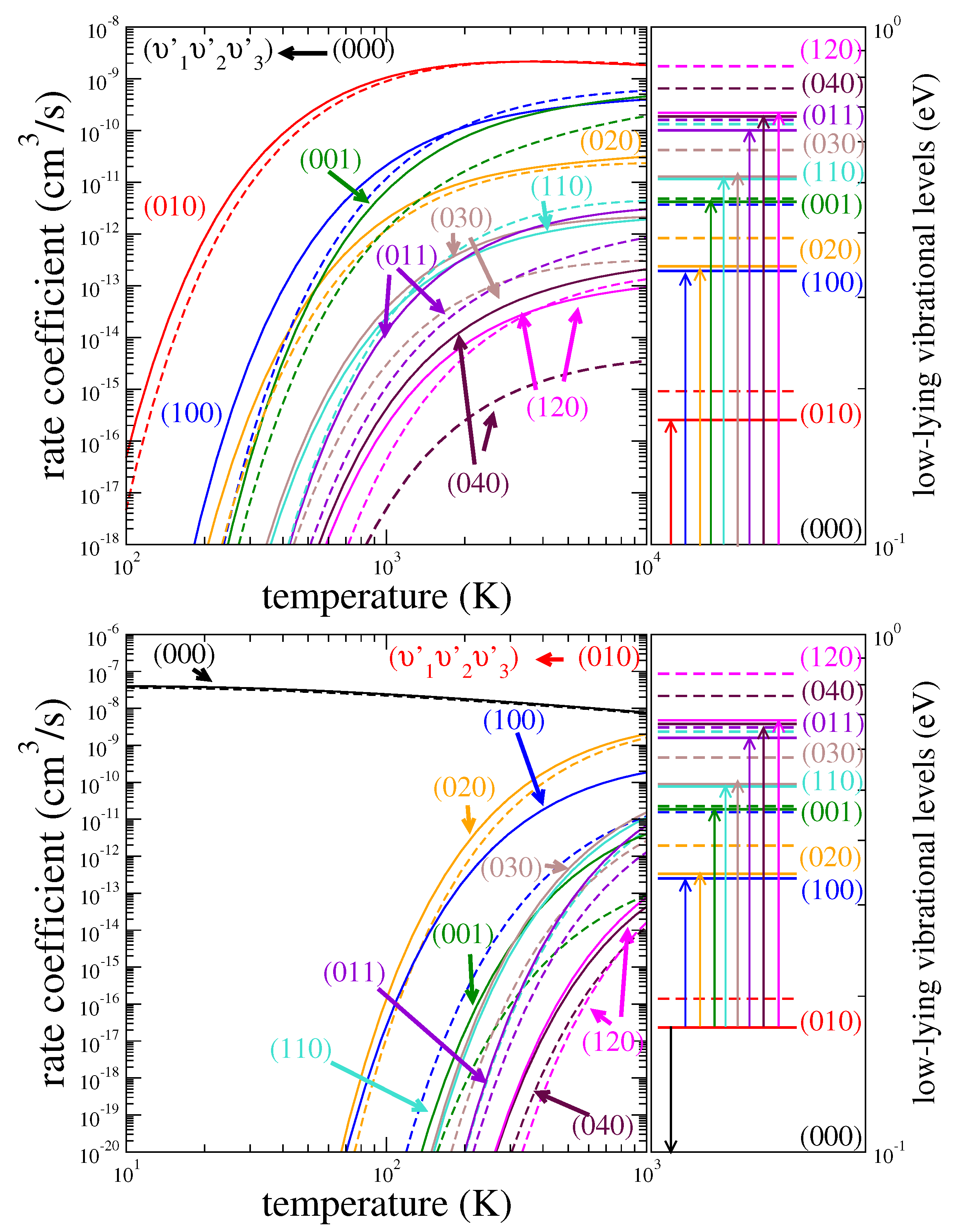
| HDO | H2O | ||||
|---|---|---|---|---|---|
| Mode (v) | This Study | Exp. [17] | Mode (v) | Calc. [12,16] | Exp. [17,18] |
Bending (010)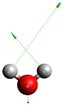 | 0.179 | 0.173 | Bending (010) 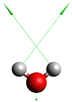 | 0.207 | 0.198 |
OD stretching (100)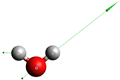 | 0.349 | 0.338 | Symmetric stretching (100) | 0.472 | 0.453 |
OH stretching (001)
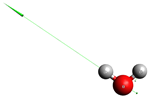 | 0.482 | 0.458 | Asymmetric stretching (001)
 | 0.488 | 0.466 |
| Bond lengths , (Å) | 0.958 | 0.956 | , (Å) | 0.958 | 0.958 |
| Bond angle (Degrees) | 104.36 | 105.20 | (Degrees) | 104.44 | 104.50 |
| HDO | H2O | ||||||
|---|---|---|---|---|---|---|---|
| () | This Work | Calc. [24] | Exp. [23] | Rel. Deviation (%) | Calc. [12] | Exp. [25] | |
| (000) | 0 | 0 | 0 | - | 0 | 0 | |
| (010) | 1394.4249 | 1403.50 | 1403.4837 (0.1740) | 0.65 | 1582.1016 | 1594.7463 | |
| (100) | 2720.0465 | 2723.71 | 2723.6797 (0.3376) | 0.13 | 3656.7438 | 3657.0533 | |
| (020) | 2764.8648 | 2782.02 | 2782.0111 (0.3449) | 0.62 | 3124.3086 | 3151.6298 | |
| (001) | 3700.3818 | 3707.47 | 3707.4667 (0.4596) | 0.19 | 3742.8112 | 3755.9285 | |
| (110) | 4078.2012 | 4099.98 | 4099.9559 (0.5083) | 0.53 | 5223.0359 | 5234.9756 | |
| (030) | 4123.3998 | 4145.48 | 4145.4731 (0.5139) | 0.53 | 4638.2210 | 4666.7905 | |
| (011) | 5061.2551 | 5089.55 | 5089.5398 (0.6310) | 0.56 | 5281.1118 | 5331.2673 | |
| (200) | 5357.8041 | 5363.84 | 5363.8244 (0.6650) | 0.11 | 7195.4949 | 7201.5399 | |
| (040) | 5385.3203 | 5420.00 | 5420.0414 (0.6719) | 0.64 | 6015.0987 | 6134.0150 | |
| (120) | 5474.3136 | - | 5506.1868 (0.6826) | 0.58 | 6744.5580 | 6775.0935 | |
| (101) | 6387.2463 | 6415.48 | 6415.4606 (0.7954) | 0.44 | 7239.0123 | 7249.8169 | |
| (021) | 6420.4831 | 6451.91 | 6451.8998 (0.7999) | 0.49 | 6781.2869 | 6871.5202 | |
| (050) | 6645.5504 | 6690.42 | 6690.4132 (0.8295) | 0.67 | 7642.5333 | 7542.3725 | |
| (210) | 6721.3248 | 6746.92 | 6746.9082 (0.8365) | 0.38 | 8737.1399 | 8761.5816 | |
| (130) | 6805.1825 | - | 6849 (0.8491) | 0.64 | 8253.4218 | 8273.9757 | |
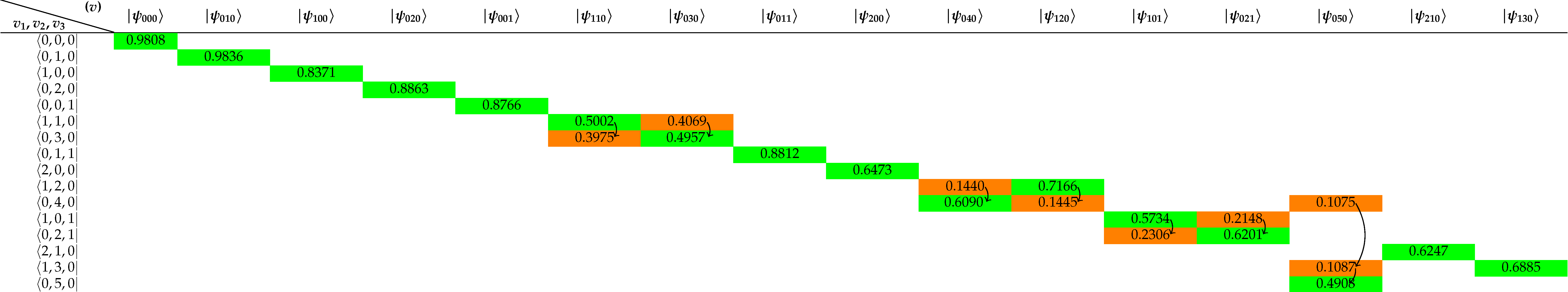 |
| Step | Input | Description | Output |
|---|---|---|---|
| (1) | Geometries of Figure 1 | Calculate the reactance matrix | |
| (2) | Diagonalize | Eigenvalues | |
| and the eigenvectors matrix | |||
| (3) | , diagonal matrix of | Build the matrix of scattering phaseshifts | |
| (4) | Elements of | Linear fit of | and |
| using | for each partial wave | ||
| (5) | Extrapolate | ||
| (6) | Diagonalize | Eigen phaseshifts | |
| and eigenvectors matrix | |||
| (7) | Diagonal matrix from | Build |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ayouz, M.A.; Faure, A.; Schneider, I.F.; Mezei, J.Z.; Kokoouline, V. Vibrational Excitation of HDO Molecule by Electron Impact. Atoms 2025, 13, 32. https://doi.org/10.3390/atoms13040032
Ayouz MA, Faure A, Schneider IF, Mezei JZ, Kokoouline V. Vibrational Excitation of HDO Molecule by Electron Impact. Atoms. 2025; 13(4):32. https://doi.org/10.3390/atoms13040032
Chicago/Turabian StyleAyouz, Mehdi Adrien, Alexandre Faure, Ioan F. Schneider, János Zsolt Mezei, and Viatcheslav Kokoouline. 2025. "Vibrational Excitation of HDO Molecule by Electron Impact" Atoms 13, no. 4: 32. https://doi.org/10.3390/atoms13040032
APA StyleAyouz, M. A., Faure, A., Schneider, I. F., Mezei, J. Z., & Kokoouline, V. (2025). Vibrational Excitation of HDO Molecule by Electron Impact. Atoms, 13(4), 32. https://doi.org/10.3390/atoms13040032








