Electron Scattering from Sevoflurane
Abstract
1. Introduction
2. Methodology
2.1. Single-Centre-Expansion Method
2.2. Ionization Cross Section: The BEB Model
3. Computational Details
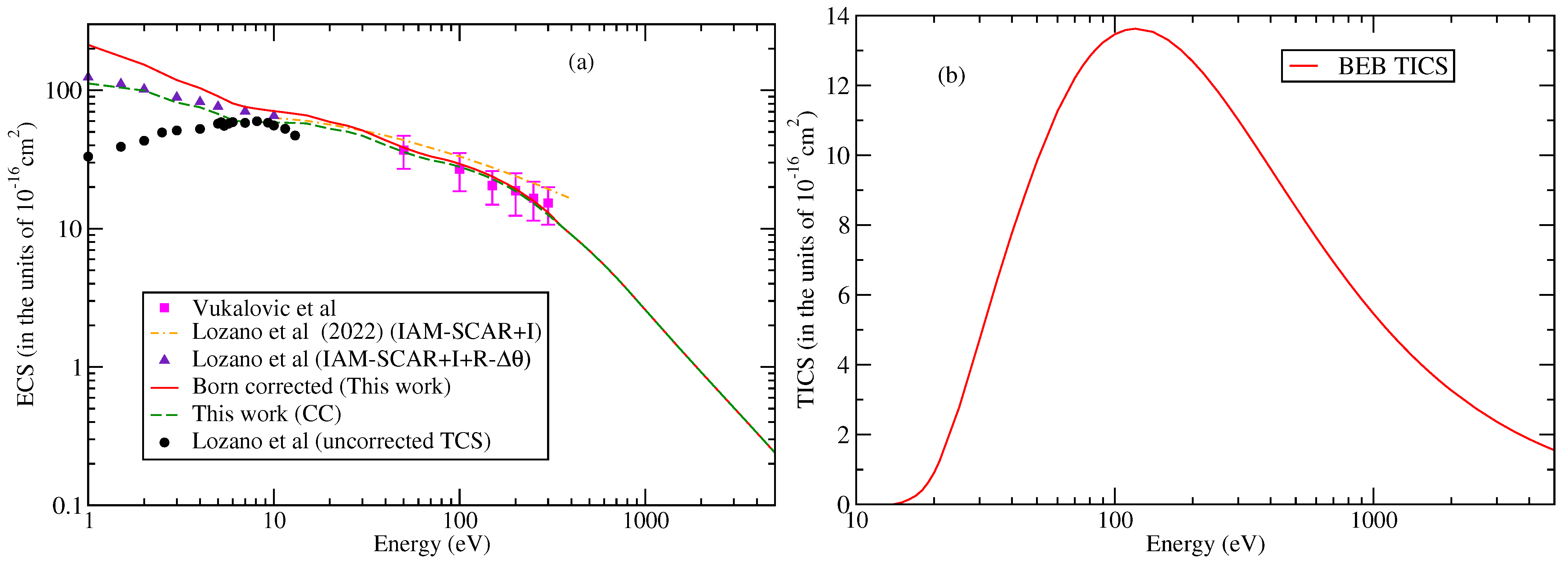
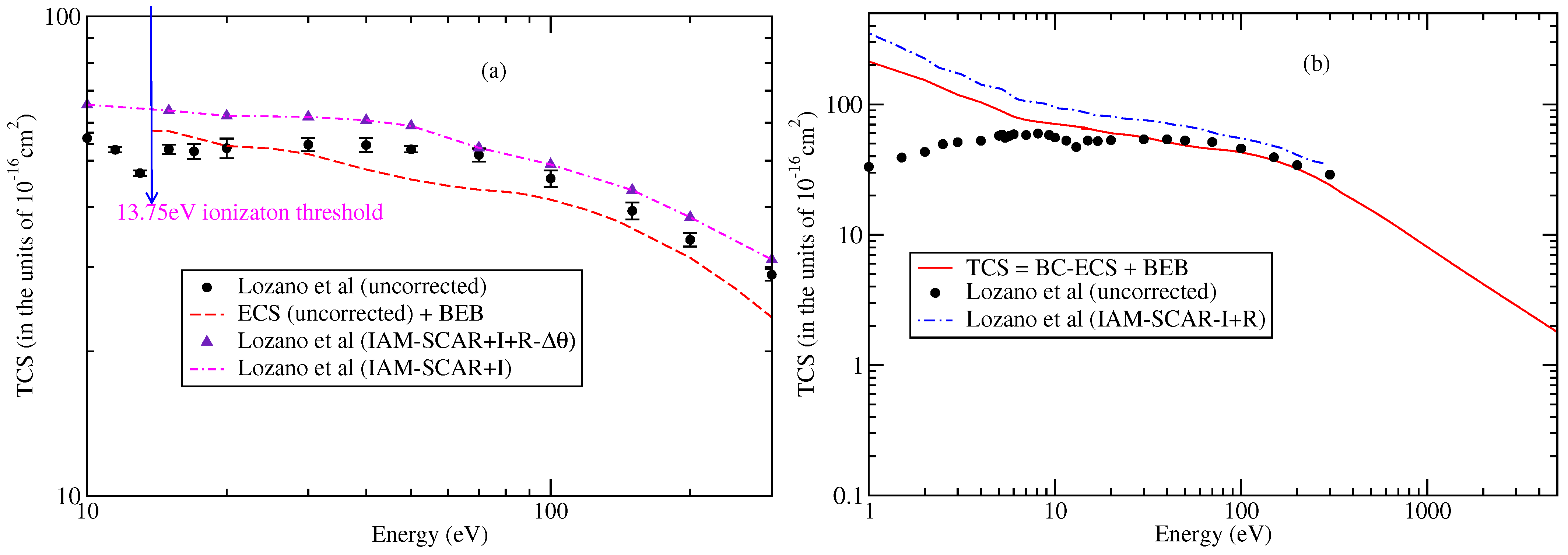
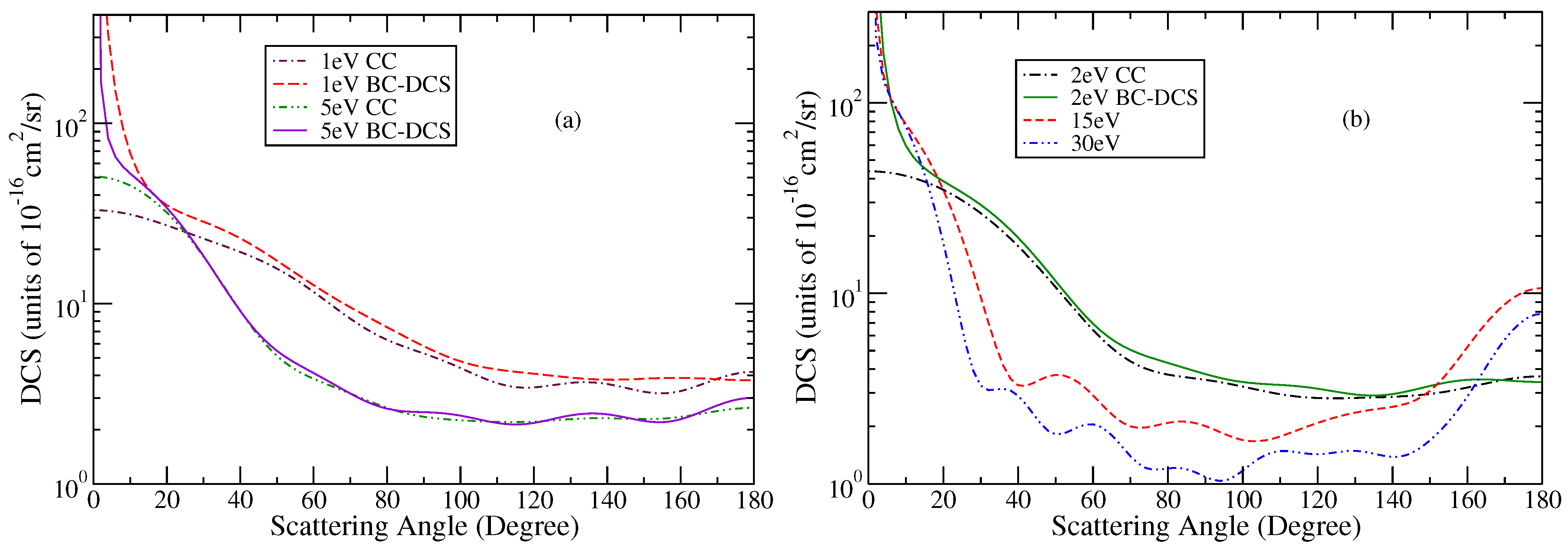
4. Results and Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| SCE | Single-centre-expansion |
| BEB | binary-encounter-Bethe |
| ECS | Elastic cross sections |
| TCS | Total cross sections |
| TICS | Total ionization cross sections |
| DCS | Differential cross sections |
| IR | Irreducible representation |
| MO | Molecular orbitals |
| eV | electron volt |
| i.e. | that is |
References
- Sanche, L. Low energy electron-driven damage in biomolecules. Eur. Phys. J. D 2005, 35, 367–390. [Google Scholar] [CrossRef]
- Campbell, L.; Brunger, M.J. Modelling of plasma processes in cometary and planetary atmospheres. Plasma Sources Sci. Technol. 2013, 22, 013002. [Google Scholar] [CrossRef]
- Makabe, T.; Petrović, Z.L. Plasma Electronics: Applications in Microelectronic Device Fabrication, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]
- Chu, P.K.; Lu, X. (Eds.) Low Temperature Plasma Technology: Methods and Applications; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Christophorou, L.G. (Ed.) Electron Molecule Interactions and Their Applications; Academic Press: Cambridge, MA, USA, 1984. [Google Scholar]
- Christophorou, L.G.; Olthoff, J.K. Fundamental Electron Interactions with Plasma Processing Gases; Springer: Boston, MA, USA, 2004. [Google Scholar]
- Janev, R.K. Atomic and Molecular Processes in Fusion Edge Plasmas; Plenum Press: New York, NY, USA, 1995. [Google Scholar]
- Starikovskiy, A. Physics and chemistry of plasma-assisted combustion. Philos. Trans. R. Soc. A 2015, 373, 20150074. [Google Scholar] [CrossRef]
- Ptasinska, S.; Varella, M.T.d.N.; Khakoo, M.A.; Slaughter, D.S.; Denifl, S. Electron scattering processes: Fundamentals, challenges, advances, and opportunities. Eur. Phys. J. D 2022, 76, 179. [Google Scholar] [CrossRef]
- Szmytkowski, C.; Możejko, P. Recent total cross section measurements in electron scattering from molecules. Eur. Phys. J. D 2020, 74, 90. [Google Scholar] [CrossRef]
- Hagelaar, G.J.M.; Pitchford, L.C. Solving the Boltzmann equation to obtain electron transport coefficients and rate coefficients for fluid models. Plasma Sources Sci. Technol. 2005, 14, 722. [Google Scholar] [CrossRef]
- Cooper, B.; Tudorovskaya, M.; Mohr, S.; O’Hare, A.; Hanicinec, M.; Dzarasova, A.; Gorfinkiel, J.D.; Benda, J.; Mašín, Z.; Al-Refaie, A.F.; et al. Quantemol Electron Collisions (QEC): An Enhanced Expert System for Performing Electron Molecule Collision Calculations Using the R-Matrix Method. Atoms 2019, 7, 97. [Google Scholar] [CrossRef]
- Mohr, S.; Tudorovskaya, M.; Hanicinec, M.; Tennyson, J. Targeted Cross-Section Calculations for Plasma Simulations. Atoms 2021, 9, 85. [Google Scholar] [CrossRef]
- Albert, D.; Antony, B.; Ba, Y.A.; Babikov, Y.L.; Bollard, P.; Boudon, V.; Delahaye, F.; Del Zanna, G.; Dimitrijević, M.S.; Drouin, B.J.; et al. A Decade with VAMDC: Results and Ambitions. Atoms 2020, 8, 76. [Google Scholar] [CrossRef]
- Bartschat, K.; Kushner, M.J. Electron collisions with atoms, ions, molecules, and surfaces: Fundamental science empowering advances in technology. Proc. Natl. Acad. Sci. USA 2016, 113, 7026–7034. [Google Scholar] [CrossRef]
- Bartschat, K.; Tennyson, J.; Zatsarinny, O. Quantum-Mechanical Calculations of Cross Sections for Electron Collisions with Atoms and Molecules. Plasma Process. Polym. 2017, 14, 1600093. [Google Scholar] [CrossRef]
- Zammit, M.C.; Fursa, D.V.; Savage, J.S.; Bray, I. Electron- and positron-molecule scattering: Development of the molecular convergent close-coupling method. J. Phys. B At. Mol. Opt. Phys. 2017, 50, 123001. [Google Scholar] [CrossRef]
- Sullivan, J.P.; Makochekanwa, C.; Jones, A.; Caradonna, P.; Slaughter, D.S.; Machacek, J.; McEachran, R.P.; Mueller, D.W.; Buckman, S.J. Forward angle scattering effects in the measurement of total cross sections for positron scattering. J. Phys. B At. Mol. Opt. Phys. 2011, 44, 035201. [Google Scholar] [CrossRef]
- Brunger, M.J.; Buckman, S.J.; Ratnavelu, K. Positron scattering from molecules: An experimental cross section compilation for positron transport studies and benchmarking theory. J. Phys. Chem. Ref. Data 2017, 46, 023102. [Google Scholar] [CrossRef]
- Kadokura, R.; Loreti, A.; Kövér, Á.; Faure, A.; Tennyson, J.; Laricchia, G. Angle-Resolved Electron Scattering from H2O near 0°. Phys. Rev. Lett. 2019, 123, 033401. [Google Scholar] [CrossRef]
- Tennyson, J. Electron-molecule collision calculations using the R-matrix method. Phys. Rep. 2010, 491, 29–76. [Google Scholar] [CrossRef]
- da Costa, R.F.; Varella, M.T.d.N.; Bettega, M.H.F.; Lima, M.A.P. Recent advances in the application of the Schwinger multichannel method with pseudopotentials to electron-molecule collisions. Eur. Phys. D 2015, 69, 159. [Google Scholar] [CrossRef]
- Schneider, B.I.; Rescigno, T.N. Complex Kohn variational method: Application to low-energy electron-molecule collisions. Phys. Rev. A 1988, 37, 3749. [Google Scholar] [CrossRef]
- Luthra, M.; Garkoti, P.; Goswami, K.; Bharadvaja, A.; Baluja, K.L. Electron impact cross-sections of tetraethyl silicate. Plasma Sources Sci. Technol. 2022, 31, 095013. [Google Scholar] [CrossRef]
- Kaur, S.; Arora, A.K.; Bharadvaja, A.; Baluja, K.L. Positron interactions with vinyl acetate from 0.1 eV to 5 keV. Phys. Scr. 2024, 99, 115411. [Google Scholar] [CrossRef]
- Prashant, A.; Luthra, M.; Goswami, K.; Bharadvaja, A.; Baluja, K.L. Positron Scattering from Pyrimidine. Atoms 2023, 11, 55. [Google Scholar] [CrossRef]
- Charlton, M.; Humberston, J.W. Positron Physics; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Tang, P.; Zubrycki, I.; Xu, Y. Ab Initio Calculation of structures and properties of halogenated general anesthetics: Halothane and sevoflurane. J. Comput. Chem. 2001, 22, 436–444. [Google Scholar] [CrossRef]
- Lesarri, A.; Vega-Toribio, A.; Suenram, R.D.; Brugh, D.J.; Grabow, J.-U. The conformational landscape of the volatile anesthetic sevoflurane. Phys. Chem. Chem. Phys. 2010, 12, 9624–9631. [Google Scholar] [CrossRef]
- The Toxin and Toxin Target Database (T3DB). Available online: https://go.drugbank.com/drugs/DB01236 (accessed on 10 February 2025).
- Jones, R.M. Desflurane and sevoflurane: Inhalation anaesthetics for this decade? Br. J. Anaesth. 1990, 65, 527–536. [Google Scholar] [CrossRef] [PubMed]
- Gaya da Costa, M.; Kalmar, A.F.; Struys, M.M.R.F. Inhaled Anesthetics: Environmental Role, Occupational Risk, and Clinical Use. J. Clin. Med. 2021, 10, 1306. [Google Scholar] [CrossRef]
- Langbein, T.; Sonntag, H.; Trapp, D.; Hoffmann, A.; Malms, W.; Röth, E.P.; Mörs, V.; Zellner, R. Volatile anaesthetics and the atmosphere: Atmospheric lifetimes and atmospheric effects of halothane, enflurane, isoflurane, desflurane and sevoflurane. Br. J. Anaesth. 1999, 82, 66–73. [Google Scholar] [PubMed]
- Lange, E.; Ferreira da Silva, F.; Jones, N.C.; Hoffmann, S.V.; Duflot, D.; Limão-Vieira, P.M. The lowest-lying electronic states of isoflurane and sevoflurane in the 5.0–10.8 eV energy range investigated by experimental and theoretical methods. Chem. Phys. Lett. 2019, 716, 42–48. [Google Scholar] [CrossRef]
- Lozano, A.I.; Ferreira da Silva, F.; Blanco, F.; Limão-Vieira, P.M.; García, G. Total electron scattering cross section from sevoflurane by 1–300 eV energy electron impact. Chem. Phys. Lett. 2018, 706, 533–537. [Google Scholar] [CrossRef]
- Blanco, F.; Rosada, J.; Illana, A.; García, G. Comparison of two screening corrections to the additivity rule for the calculation of electron scattering from polyatomic molecules. Phys. Lett. A 2010, 374, 4420–4424. [Google Scholar] [CrossRef]
- Blanco, F.; Ellis-Gibbings, L.; García, G. Screening corrections for the interference contributions to the electron and positron scattering cross sections from polyatomic molecules. Chem. Phys. Lett. 2016, 645, 71–75. [Google Scholar] [CrossRef]
- Dubuis, A.T.; Verkhovtsev, A.; Ellis-Gibbings, L.; Krupa, K.; Blanco, F.; Jones, D.B.; Brunger, M.J.; García, G. Total cross section of furfural by electron impact: Experiment and theory. Chem. Phys. Lett. 2017, 147, 054301. [Google Scholar] [CrossRef]
- Vukalović, J.; Maljković, J.B.; Blanco, F.; García, G.; Predojević, B.; Marinković, B.P. Absolute Differential Cross-Sections for Elastic Electron Scattering from Sevoflurane Molecule in the Energy Range from 50–300 eV. Int. J. Mol. Sci. 2022, 23, 21. [Google Scholar] [CrossRef]
- Gianturco, F.A.; Sanna, N. SCELIB: A parallel computational library of molecular properties in the single-center expansion approach. Comput. Phys. Commun. 2000, 128, 139–169. [Google Scholar] [CrossRef]
- Gianturco, F.A.; Thompson, D.G.; Jain, A.K. Computational Methods for Electron Molecule Collisions; Huo, W.M., Gianturco, F.A., Eds.; Plenum: New York, NY, USA, 1995. [Google Scholar]
- Gianturco, F.A.; Lucchese, R.R.; Sanna, N.; Talamo, A. A.; Lucchese, R.R.; Sanna, N.; Talamo, A. A Generalized Single Center Approach for Treating Electron Scattering from Polyatomic Molecules. In Electron Collisions with Molecules, Clusters and Surfaces; Ehrhardt, H., Morgan, L.A., Eds.; Plenum: New York, NY, USA, 1994. [Google Scholar]
- Gianturco, F.A.; Lucchese, R.R.; Sanna, N.J. Calculation of low-energy elastic electron scattering by CF4. Chem. Phys. 1994, 100, 6464–6471. [Google Scholar] [CrossRef]
- Joshipura, K.N.; Mason, N. Atomic-Molecular Ionization by Electron Scattering: Theory and Applications; Cambridge University Press: Cambridge, UK, 2019. [Google Scholar]
- Jain, A.K.; Baluja, K.L. Total (elastic plus inelastic) cross sections for electron scattering from diatomic and polyatomic molecules at 10–5000 eV: H2, Li2, HF, CH4, N2, CO, C2H2, HCN, O2, HCl, H2S, PH3, SiH4, and CO2. Phys. Rev. A 1992, 45, 202–218. [Google Scholar] [CrossRef]
- Kaur, S.; Baluja, K.L.; Tennyson, J. Electron-impact study of NeF using the R-matrix method. Phys. Rev. A 2008, 77, 032718. [Google Scholar] [CrossRef]
- Kim, Y.-K.; Rudd, M.E. Binary-encounter-dipole model for electron-impact ionization. Phys. Rev. A 1994, 50, 3954. [Google Scholar] [CrossRef] [PubMed]
- Perdew, J.P.; Zunger, A. Self-interaction correction to density-functional approximations for many-electron systems. Phys. Rev. B 1981, 23, 5048. [Google Scholar] [CrossRef]
- Hara, S. The Scattering of Slow Electrons by Hydrogen Molecules. J. Phys. Soc. Jpn. 1967, 22, 710–718. [Google Scholar] [CrossRef]
- Burke, P.G. R-Matrix Theory of Atomic Collisions: Application to Atomic, Molecular and Optical Processes; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Franz, J. Solution of coupled integral equations for quantum scattering in the presence of complex potentials. J. Math. Phys. 2015, 56, 012104. [Google Scholar] [CrossRef]
- Itikawa, Y. The Born closure approximation for the scattering amplitude of an electron-molecule collision. Theor. Chem. Acc. 2000, 105, 123–131. [Google Scholar] [CrossRef]
- Fabrikant, I.I. Long-range effects in electron scattering by polar molecules. J. Phys. B At. Mol. Opt. Phys. 2016, 49, 222005. [Google Scholar] [CrossRef]
- Gianturco, F.A.; Jain, A. The theory of electron scattering from polyatomic molecules. Phys. Rep. 1986, 143, 347–425. [Google Scholar] [CrossRef]
- GAUSSIAN 03. Gaussian, Inc.: Wallingford, UK, 2003.
- Sanna, N.; Baccarelli, I.; Morelli, G. SCELib3.0: The new revision of SCELib, the parallel computational library of molecular properties in the Single Center Approach. Comput. Phys. Commun. 2009, 180, 2544–2549. [Google Scholar] [CrossRef]
- Psi4: An Open-Source Ab Initio Electronic Structure Package Psi4 1.3.2 Release. Available online: https://psicode.org/ (accessed on 13 December 2024).
- Jain, A.; Thompson, D.G. A program to generate the symmetry-adapted rotational eigenfunctions and energy levels for asymmetric top molecules. Comput. Phys. Commun. 1983, 30, 301–309. [Google Scholar] [CrossRef]
- Sanna, N.; Gianturco, F.A. Differential cross sections for electron/positron scattering from polyatomic molecules. Comput. Phys. Commun. 1998, 114, 142–167. [Google Scholar] [CrossRef]
- Franz, J.; Gianturco, F.A.; Baccarelli, I. Low-energy positron scattering from gas-phase uracil. Eur. Phys. J. D 2014, 68, 183. [Google Scholar] [CrossRef]
- Meltzer, T.; Tennyson, J.; Mašin, Z.; Zammit, M.C.; Scarlett, L.H.; Fursa, D.V.; Bray, I. Benchmark calculations of electron impact electronic excitation of the hydrogen molecule. J. Phys. B At. Mol. Opt. Phys. 2020, 53, 145204. [Google Scholar] [CrossRef]
- Randi, P.A.S.; Moreira, G.M.; Bettega, M.H.F. Inelastic scattering by formamide: Elastic and electronically inelastic cross sections up to 179 energetically open states. Phys. Rev. A 2023, 107, 012806. [Google Scholar] [CrossRef]
- Mas̃in, Z.; Benda, J.; Harvey, A.G.; Al-Refaie, A.; Gorfinkiel, J.D.; Tennyson, J. UKRmol+: A suite for modelling electronic processes in molecules interacting with electrons, positrons and photons using the R-matrix method. Comp. Phys. Commun. 2020, 249, 107092. [Google Scholar] [CrossRef]

| Rotational Constants (MHz) | A | B | C | |
| This work (using Gaussian [55]) | 1044.556 | 915.365 | 608.529 | |
| Lessari et al. [29] | 1020.537 | 903.188 | 601.280 | |
| Dipole Moment (Debye, D) | ||||
| Correlated (8,9) model (This work) [57] | 0.28 | 2.39 | 0.13 | 2.408 |
| Lessari et al. [29] | 0.856 | 2.050 | 0.464 | 2.27 |
| 2.33 [28] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kaur, S.; Arora, A.K.; Baluja, K.L.; Bharadvaja, A. Electron Scattering from Sevoflurane. Atoms 2025, 13, 29. https://doi.org/10.3390/atoms13040029
Kaur S, Arora AK, Baluja KL, Bharadvaja A. Electron Scattering from Sevoflurane. Atoms. 2025; 13(4):29. https://doi.org/10.3390/atoms13040029
Chicago/Turabian StyleKaur, Savinder, Ajay Kumar Arora, Kasturi Lal Baluja, and Anand Bharadvaja. 2025. "Electron Scattering from Sevoflurane" Atoms 13, no. 4: 29. https://doi.org/10.3390/atoms13040029
APA StyleKaur, S., Arora, A. K., Baluja, K. L., & Bharadvaja, A. (2025). Electron Scattering from Sevoflurane. Atoms, 13(4), 29. https://doi.org/10.3390/atoms13040029






