Properties of a Static Dipolar Impurity in a 2D Dipolar BEC
Abstract
1. Introduction
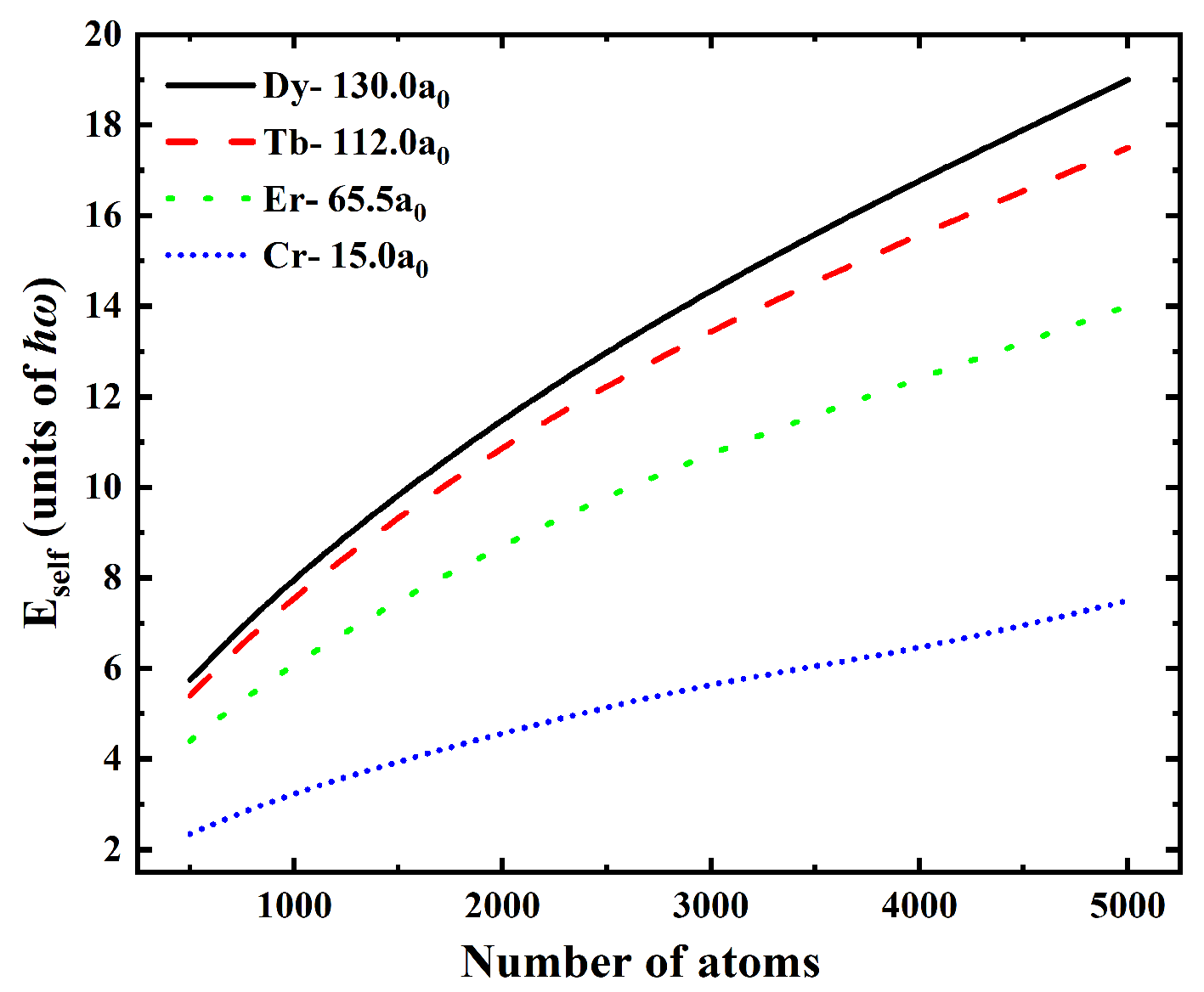
2. Results
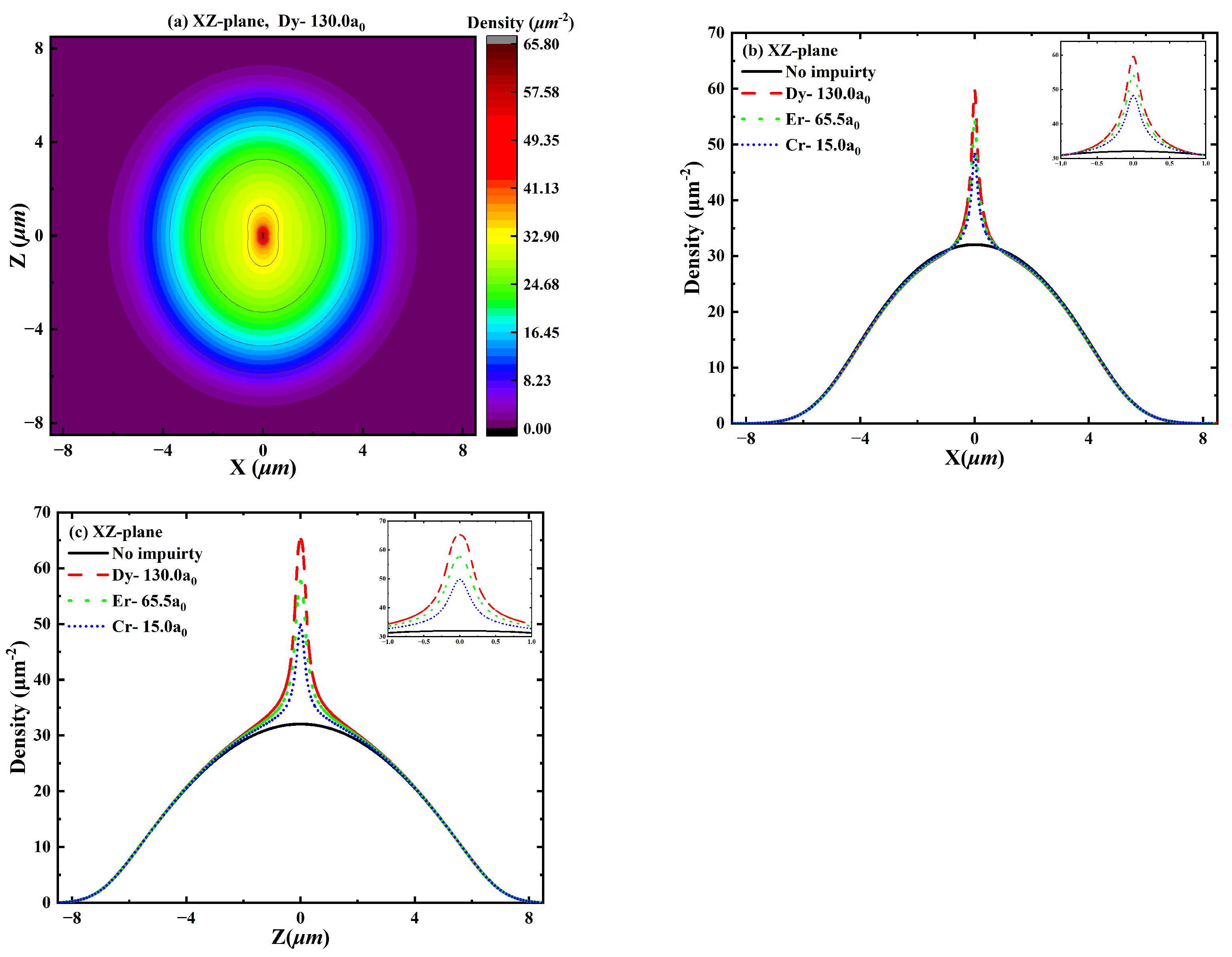
2.1. -Plane
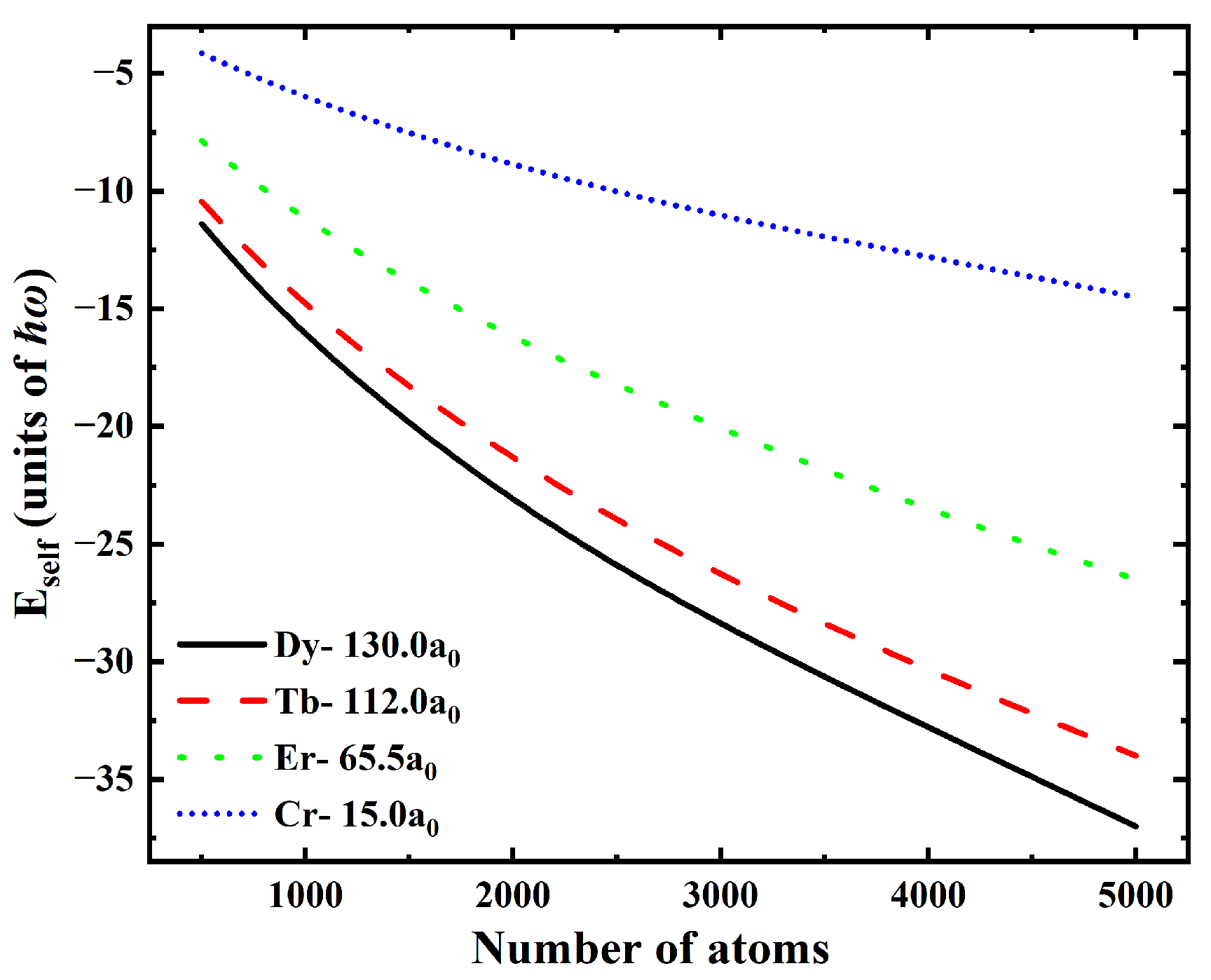
2.2. -Plane
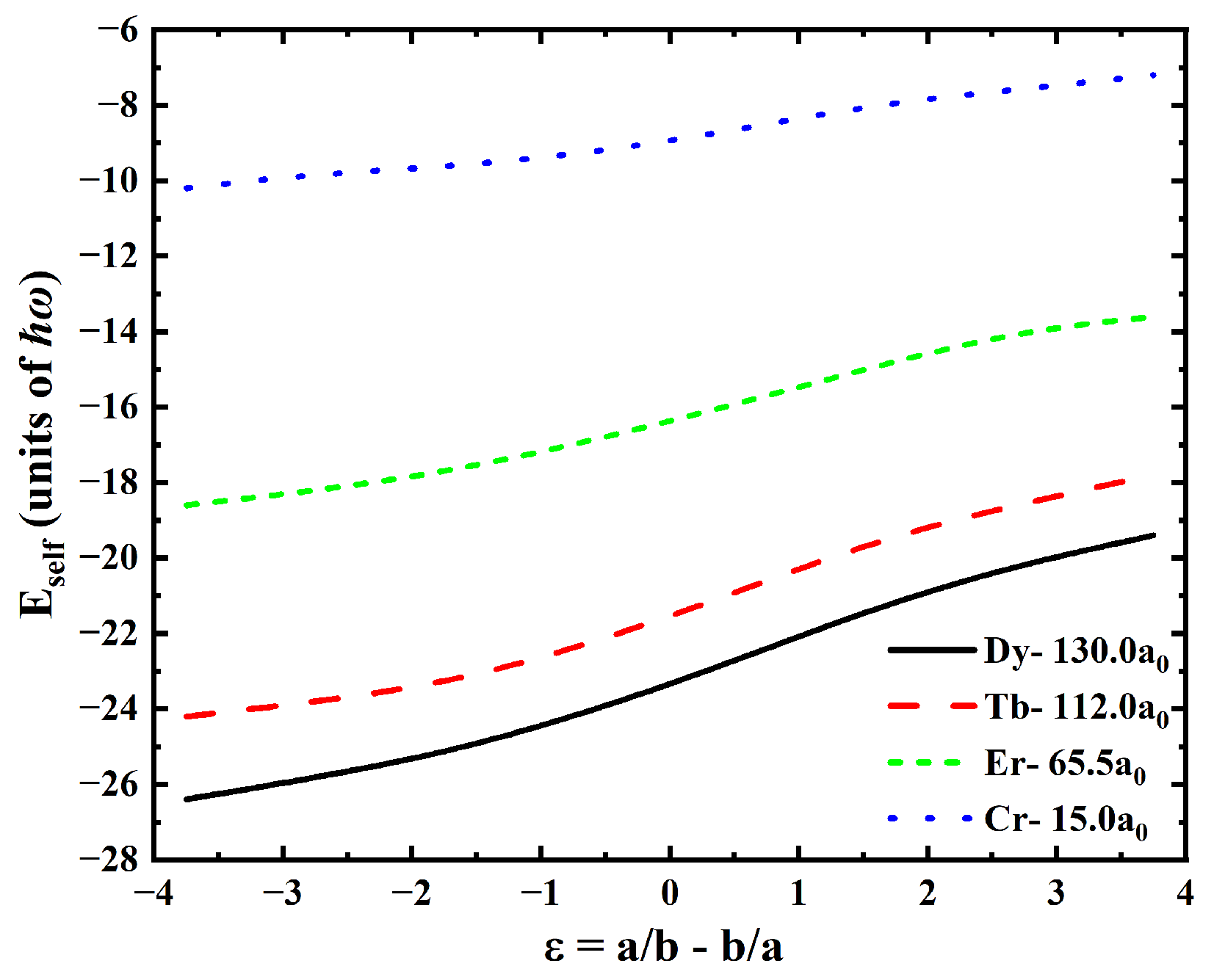
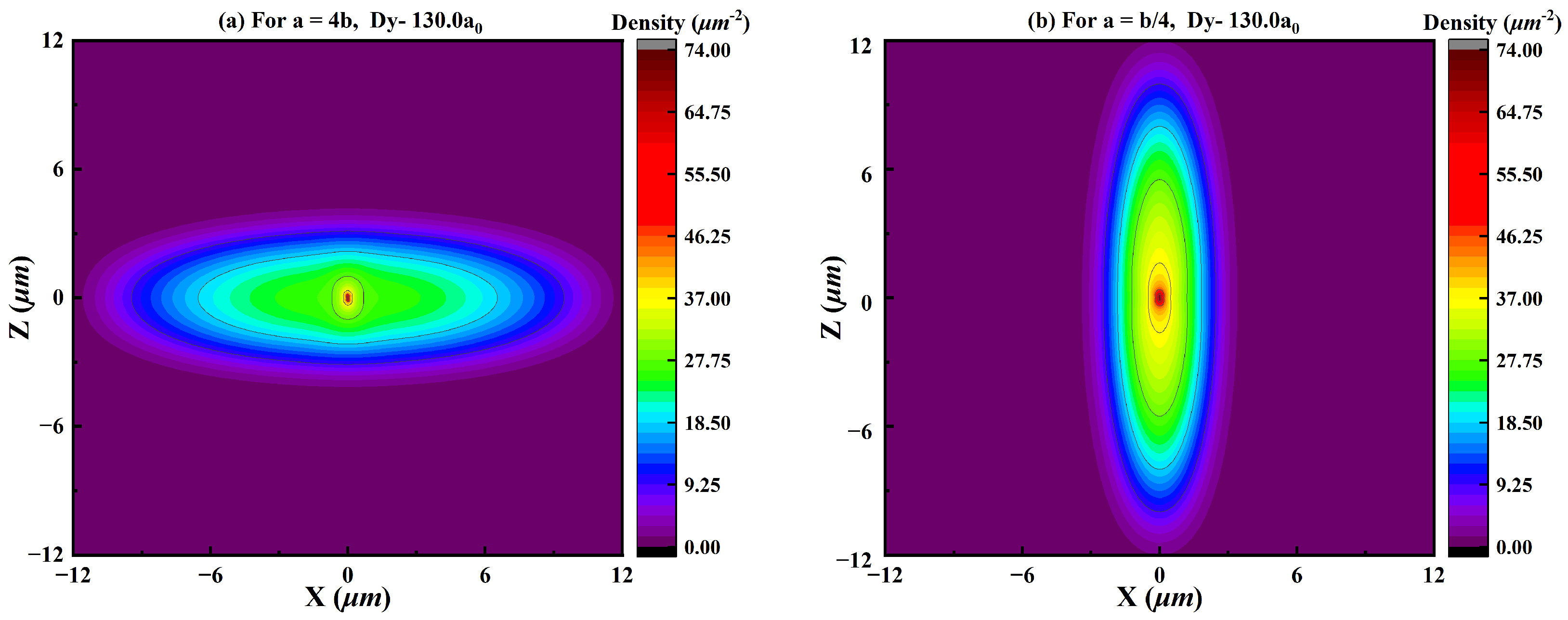
2.3. Anisotropic Trap
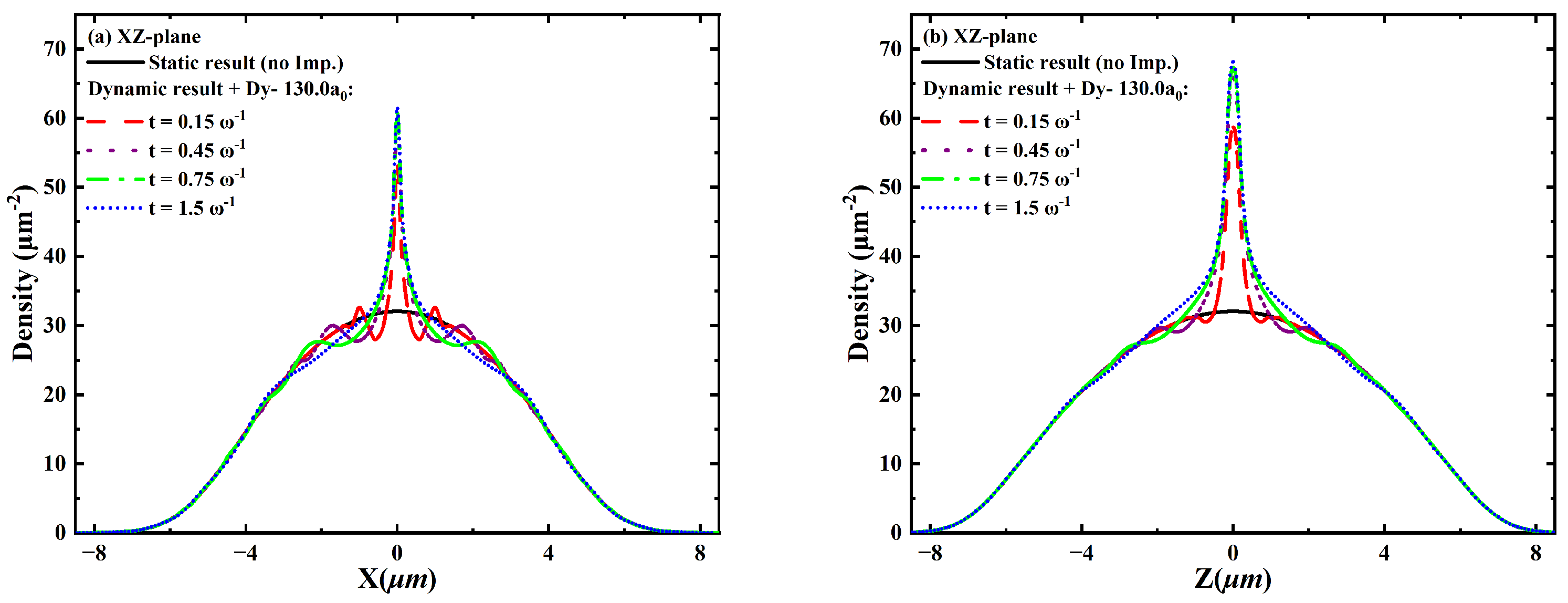
2.4. Time Dynamics
3. Methods
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Derivation of Our GPE
References
- Lahaye, T.; Menotti, C.; Santos, L.; Lewenstein, M.; Pfau, T. The physics of dipolar bosonic quantum gases. Rep. Prog. Phys. 2009, 72, 126401. [Google Scholar] [CrossRef]
- Chomaz, L.; Ferrier-Barbut, I.; Ferlaino, F.; Laburthe-Tolra, B.; Lev, B.L.; Pfau, T. Dipolar physics: A review of experiments with magnetic quantum gases. Rep. Prog. Phys. 2022, 86, 026401. [Google Scholar] [CrossRef] [PubMed]
- Griesmaier, A.; Werner, J.; Hensler, S.; Stuhler, J.; Pfau, T. Bose-Einstein condensation of chromium. Phys. Rev. Lett. 2005, 94, 160401. [Google Scholar] [CrossRef]
- Bigagli, N.; Yuan, W.; Zhang, S.; Bulatovic, B.; Karman, T.; Stevenson, I.; Will, S. Observation of Bose–Einstein condensation of dipolar molecules. Nature 2024, 631, 289–293. [Google Scholar] [CrossRef]
- Hu, M.G.; Van de Graaff, M.J.; Kedar, D.; Corson, J.P.; Cornell, E.A.; Jin, D.S. Bose polarons in the strongly interacting regime. Phys. Rev. Lett. 2016, 117, 055301. [Google Scholar] [CrossRef] [PubMed]
- Jørgensen, N.B.; Wacker, L.; Skalmstang, K.T.; Parish, M.M.; Levinsen, J.; Christensen, R.S.; Bruun, G.M.; Arlt, J.J. Observation of attractive and repulsive polarons in a Bose-Einstein condensate. Phys. Rev. Lett. 2016, 117, 055302. [Google Scholar] [CrossRef]
- Scazza, F.; Zaccanti, M.; Massignan, P.; Parish, M.M.; Levinsen, J. Repulsive Fermi and Bose polarons in quantum gases. Atoms 2022, 10, 55. [Google Scholar] [CrossRef]
- Mistakidis, S.I.; Volosniev, A.; Barfknecht, R.; Fogarty, T.; Busch, T.; Foerster, A.; Schmelcher, P.; Zinner, N. Few-body Bose gases in low dimensions—A laboratory for quantum dynamics. Phys. Rep. 2023, 1042, 1–108. [Google Scholar]
- Grusdt, F.; Mostaan, N.; Demler, E.; Ardila, L.A.P. Impurities and polarons in bosonic quantum gases: A review on recent progress. arXiv 2024, arXiv:2410.09413. [Google Scholar]
- Christensen, E.R.; Camacho-Guardian, A.; Bruun, G.M. Charged polarons and molecules in a Bose-Einstein condensate. Phys. Rev. Lett. 2021, 126, 243001. [Google Scholar] [CrossRef]
- Astrakharchik, G.E.; Ardila, L.A.P.; Schmidt, R.; Jachymski, K.; Negretti, A. Ionic polaron in a Bose-Einstein condensate. Commun. Phys. 2021, 4, 94. [Google Scholar] [CrossRef]
- Suchorowski, M.; Badamshina, A.; Lemeshko, M.; Tomza, M.; Volosniev, A.G. Quantum rotor in a two-dimensional mesoscopic Bose gas. arXiv 2024, arXiv:2407.06046. [Google Scholar] [CrossRef]
- Camargo, F.; Schmidt, R.; Whalen, J.D.; Ding, R.; Woehl, G., Jr.; Yoshida, S.; Burgdörfer, J.; Dunning, F.B.; Sadeghpour, H.R.; Demler, E.; et al. Creation of Rydberg polarons in a Bose gas. Phys. Rev. Lett. 2018, 120, 083401. [Google Scholar] [CrossRef]
- Volosniev, A.G.; Jensen, A.S.; Harshman, N.L.; Armstrong, J.R.; Zinner, N.T. A solvable model for decoupling of interacting clusters. Europhys. Lett. 2019, 125, 20003. [Google Scholar] [CrossRef]
- Mehboudi, M.; Lampo, A.; Charalambous, C.; Correa, L.A.; García-March, M.Á.; Lewenstein, M. Using polarons for sub-nk quantum nondemolition thermometry in a bose-einstein condensate. Phys. Rev. Lett. 2019, 122, 030403. [Google Scholar] [CrossRef] [PubMed]
- Bouton, Q.; Nettersheim, J.; Adam, D.; Schmidt, F.; Mayer, D.; Lausch, T.; Tiemann, E.; Widera, A. Single-atom quantum probes for ultracold gases boosted by nonequilibrium spin dynamics. Phys. Rev. X 2020, 10, 011018. [Google Scholar] [CrossRef]
- Zhang, Y.; Ong, W.; Arakelyan, I.; Thomas, J. Polaron-to-Polaron Transitions in the Radio-Frequency Spectrum of a Quasi-Two-Dimensional Fermi Gas. Phys. Rev. Lett. 2012, 108, 235302. [Google Scholar] [CrossRef] [PubMed]
- Ong, W.; Cheng, C.; Arakelyan, I.; Thomas, J. Spin-imbalanced quasi-two-dimensional Fermi gases. Phys. Rev. Lett. 2015, 114, 110403. [Google Scholar] [CrossRef]
- Koschorreck, M.; Pertot, D.; Vogt, E.; Fröhlich, B.; Feld, M.; Köhl, M. Attractive and repulsive Fermi polarons in two dimensions. Nature 2012, 485, 619–622. [Google Scholar] [CrossRef]
- Ardila, L.P.; Astrakharchik, G.; Giorgini, S. Strong coupling Bose polarons in a two-dimensional gas. Phys. Rev. Res. 2020, 2, 023405. [Google Scholar] [CrossRef]
- Nakano, Y.; Parish, M.M.; Levinsen, J. Variational approach to the two-dimensional Bose polaron. Phys. Rev. A 2024, 109, 013325. [Google Scholar] [CrossRef]
- Cárdenas-Castillo, L.F.; Camacho-Guardian, A. Strongly interacting Bose polarons in two-dimensional atomic gases and quantum fluids of polaritons. Atoms 2022, 11, 3. [Google Scholar] [CrossRef]
- Massignan, P.; Schmidt, R.; Astrakharchik, G.E.; İmamoglu, A.; Zwierlein, M.; Arlt, J.J.; Bruun, G.M. Polarons in atomic gases and two-dimensional semiconductors. arXiv 2025, arXiv:2501.09618. [Google Scholar]
- Kain, B.; Ling, H.Y. Polarons in a dipolar condensate. Phys. Rev. A 2014, 89, 023612. [Google Scholar] [CrossRef]
- Ardila, L.A.P.; Pohl, T. Ground-state properties of dipolar Bose polarons. J. Phys. B At. Mol. Opt. Phys. 2018, 52, 015004. [Google Scholar] [CrossRef]
- Volosniev, A.G.; Bighin, G.; Santos, L.; Peña Ardila, L.A. Non-equilibrium dynamics of dipolar polarons. SciPost Phys. 2023, 15, 232. [Google Scholar] [CrossRef]
- Sánchez-Baena, J.; Ardila, L.A.P.n.; Astrakharchik, G.E.; Mazzanti, F. Universal properties of dipolar Bose polarons in two dimensions. Phys. Rev. A 2024, 110, 023317. [Google Scholar] [CrossRef]
- Shukla, N.; Volosniev, A.G.; Armstrong, J.R. Anisotropic potential immersed in a dipolar Bose-Einstein condensate. Phys. Rev. A 2024, 110, 053317. [Google Scholar] [CrossRef]
- Gross, E. Motion of foreign bodies in boson systems. Ann. Phys. 1962, 19, 234–253. [Google Scholar] [CrossRef]
- Volosniev, A.G.; Hammer, H.W. Analytical approach to the Bose-polaron problem in one dimension. Phys. Rev. A 2017, 96, 031601. [Google Scholar] [CrossRef]
- Hryhorchak, O.; Panochko, G.; Pastukhov, V. Mean-field study of repulsive 2D and 3D Bose polarons. J. Phys. B At. Mol. Opt. Phys. 2020, 53, 205302. [Google Scholar] [CrossRef]
- Jager, J.; Barnett, R.; Will, M.; Fleischhauer, M. Strong-coupling Bose polarons in one dimension: Condensate deformation and modified Bogoliubov phonons. Phys. Rev. Res. 2020, 2, 033142. [Google Scholar] [CrossRef]
- Drescher, M.; Salmhofer, M.; Enss, T. Theory of a resonantly interacting impurity in a Bose-Einstein condensate. Phys. Rev. Res. 2020, 2, 032011. [Google Scholar] [CrossRef]
- Guenther, N.E.; Schmidt, R.; Bruun, G.M.; Gurarie, V.; Massignan, P. Mobile impurity in a Bose-Einstein condensate and the orthogonality catastrophe. Phys. Rev. A 2021, 103, 013317. [Google Scholar] [CrossRef]
- Włodzyński, D. Several fermions strongly interacting with a heavy mobile impurity in a one-dimensional harmonic trap. Phys. Rev. A 2022, 106, 033306. [Google Scholar] [CrossRef]
- Lee, T.D.; Low, F.; Pines, D. The Motion of Slow Electrons in a Polar Crystal. Phys. Rev. 1953, 90, 297. [Google Scholar] [CrossRef]
- Girardeau, M.D. Reduction of a quantum n-body problem to an (n − 1)-body problem. Phys. Rev. A 1983, 28, 3635. [Google Scholar] [CrossRef]
- Catani, J.; Lamporesi, G.; Naik, D.; Gring, M.; Inguscio, M.; Minardi, F.; Kantian, A.; Giamarchi, T. Quantum dynamics of impurities in a one-dimensional Bose gas. Phys. Rev. A 2012, 85, 023623. [Google Scholar] [CrossRef]
- Kumar, R.K.; Young-S, L.E.; Vudragović, D.; Balaž, A.; Muruganandam, P.; Adhikari, S.K. Fortran and C programs for the time-dependent dipolar Gross—Pitaevskii equation in an anisotropic trap. Comput. Phys. Commun. 2015, 195, 117–128. [Google Scholar] [CrossRef]
- Chin, C.; Grimm, R.; Julienne, P.; Tiesinga, E. Feshbach resonances in ultracold gases. Rev. Mod. Phys. 2010, 82, 1225–1286. [Google Scholar] [CrossRef]
- Böttcher, F.; Schmidt, J.N.; Hertkorn, J.; Ng, K.S.H.; Graham, S.D.; Guo, M.; Langen, T.; Pfau, T. New states of matter with fine-tuned interactions: Quantum droplets and dipolar supersolids. Rep. Prog. Phys. 2020, 84, 012403. [Google Scholar] [CrossRef] [PubMed]
- Fischer, U.R. Stability of quasi-two-dimensional Bose-Einstein condensates with dominant dipole-dipole interactions. Phys. Rev. A—At. Mol. Opt. Phys. 2006, 73, 031602. [Google Scholar] [CrossRef]
- Paredes, R.; Bruun, G.; Camacho-Guardian, A. Interactions mediated by atoms, photons, electrons, and excitons. Phys. Rev. A 2024, 110, 030101. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shukla, N.; Armstrong, J.R. Properties of a Static Dipolar Impurity in a 2D Dipolar BEC. Atoms 2025, 13, 24. https://doi.org/10.3390/atoms13030024
Shukla N, Armstrong JR. Properties of a Static Dipolar Impurity in a 2D Dipolar BEC. Atoms. 2025; 13(3):24. https://doi.org/10.3390/atoms13030024
Chicago/Turabian StyleShukla, Neelam, and Jeremy R. Armstrong. 2025. "Properties of a Static Dipolar Impurity in a 2D Dipolar BEC" Atoms 13, no. 3: 24. https://doi.org/10.3390/atoms13030024
APA StyleShukla, N., & Armstrong, J. R. (2025). Properties of a Static Dipolar Impurity in a 2D Dipolar BEC. Atoms, 13(3), 24. https://doi.org/10.3390/atoms13030024





