Statistics of Quantum Numbers for Non-Equivalent Fermions in Single-j Shells

Abstract

This work addresses closed-form expressions for the distributions $P\left(M\right)$ of the magnetic quantum numbers M and $Q\left(J\right)$ of total angular momentum J for non-equivalent fermions in single-j orbits. Such quantities play an important role in both nuclear and atomic physics, through the shell models. Using irreducible representations of the rotation group, different kinds of formulas are presented, involving multinomial coefficients, generalized Pascal triangle coefficients, or hypergeometric functions. Special cases are discussed, and the connections between $P\left(M\right)$ (and therefore $Q\left(J\right)$) and mathematical functions such as elementary symmetric, cyclotomic, and Jacobi polynomials are outlined.

1. Introduction

The problem of determining the number of states corresponding to a given $(J,M)$ pair, where J represents the magnitude of the total angular momentum operator and M is the eigenvalue (in units of ℏ) of its projection along the z-axis ($-J\le M\le J$) in the case of indistinguishable particles, was first addressed by Bethe in 1936 for nuclear systems [1]. The total angular momentum of N fermions

$$\overrightarrow{J}=\sum _{i=1}^N\overrightarrow{j}\left[i\right]$$

(1)

is the addition of the intrinsic angular momenta $\overrightarrow{j}\left[i\right]$ of each fermion. The one-electron states are defined by $\left|j\right[i\left]m\right[i]⟩$, where $-j\left[i\right]\le m\left[i\right]\le j\left[i\right]$. Multielectron states of the ion are represented by $|JM⟩$. The notations $Q\left(J\right)$ and $P\left(M\right)$ are introduced to represent the number of levels and states, respectively, with the same value of J (or M) in common. The study of the distribution of quantum number J in an atomic system is somewhat cumbersome, in particular because J is not the eigenvalue of any simple operator. Furthermore, there is no simple rule on J to account for the Pauli exclusion principle in configurations with more than two equivalent fermions. It is usually more convenient to study the distribution of the projection M of J, because M is the eigenvalue of the one-fermion operator $J_z$ and is thus additive with respect to the magnetic quantum numbers of all the electrons:

$$M=\sum _{i=1}^{N}m\left[i\right].$$

The Pauli exclusion principle can be handled through the combinatorics, by searching for all the possibilities to populate the one-electron states (Figure 1 shows an example of a state of a single-j shell ${(9/2)}^4$ corresponding to $M=-3$). For a general configuration made of r shells

$$j_1^{N_1}{j}_2^{N_2}\cdots j_r^{N_r},$$

(2)

the total degeneracy is

$$g=\prod _{k=1}^r\left({\displaystyle \genfrac{}{}{0pt}{}{2j_k+1}{N_k}}\right).$$

Figure 1. Example of a state of a single-j shell ${(9/2)}^4$ corresponding to $M=m\left[1\right]+m\left[2\right]+m\left[3\right]+m\left[4\right]=-9/2-5/2+3/2+5/2=-3$.

The single-j shell (corresponding to $r=1$ and $j_1=j$) is a model system and a cornerstone of nuclear structure studies [2]. Many aspects of this paradigm have been investigated, such as symmetries [3], two-body random Hamiltonians [4,5], isospin relations, and the J-pairing interaction (see, for instance, Refs. [6,7]). The enumeration of M-states and J-levels has been the subject of numerous studies over the years within the context of nuclear physics. For example, in a study on the quantum Hall effect [8], Ginocchio and Haxton derived a simple formula for $Q\left(0\right)$ in the case of $j^4$, which is also equal to $Q\left(j\right)$ for $j^3$. Zhao and Arima found empirical formulas for $Q\left(J\right)$ for three, four, and five particles [9] for specific J values. Zamick and Escuderos interpreted the Ginocchio–Haxton formula using a combinatorial approach for $J=j$ with $n=3$[10], and Talmi derived a recursion relation for $Q\left(J\right)$ of n fermions in a j orbit in terms of $k\le n$ fermions in a $(j-1)$ orbit [11]. In Refs. [12,13,14], the authors extended the studies for $n=3$ and $n=4$ to determine the number of states with a given spin J and isospin T. The number of states for a given spin was found to be closely related to the sum rules of many six-j and nine-j symbols, as well as coefficients of fractional parentage [15,16,17,18,19,20]. In particular, Zamick and Escuderos identified a relationship between coefficients of fractional parentage obtained from the principal-parent method and from a seniority classification. They applied this relationship to Redmond’s recursion relation formula [21,22], transformed it to the seniority scheme, and used it to determine the number of spin-J states for $J=j$ (a result previously obtained by Zhao and Arima [9]). Bao et al. derived recursive formulas by induction with respect to n and j and applied them to systems of two, three, and five identical particles [23]. A couple of years ago, we proposed new recursion relations for $P\left(M\right)$ obtained using generating functions [24,25]. We also studied the statistical properties of the distributions using the cumulants [26,27], as well as the odd–even staggering (the number of odd-J states is larger than the number of even-J states) [25]. In addition, it is worth mentioning that we obtained analytical expressions of the total number of J levels for identical particles in a single-j shell using coefficients of fractional parentage [28] for $j^3$ and $j^4$.

The enumeration of M-states and J-levels is also of fundamental and practical interest for atomic physics and the statistical modeling of hot-plasma atomic spectral properties, such as emission and absorption spectra (see for instance [29,30] and references therein) or the Auger effect [31]. It is not so common for the same model to apply to both nuclear and atomic physics.

Obtaining general analytical expressions for $P\left(M\right)$ and $Q\left(J\right)$ is a very difficult task. In Ref. [32], we derived analytical expressions in the case of three, four, and five fermions (i.e., configurations $j^3$, $j^4$ and $j^5$) using recursion relations. Very recently, we developed a general method yielding closed-form expressions of $P\left(M\right)$, whatever the number of fermions (electrons in the case of atomic physics, neutrons and protons in the framework of nuclear physics), as linear combinations of piecewise polynomials and congruences, and presented illustrations of up to six fermions [33]. The distributions $P\left(M\right)$ and $Q\left(J\right)$ for four fermions in a shell $j=9/2$ are displayed in Figure 2 and Figure 3, respectively. The corresponding analytical expressions for $j^4$, obtained in Ref. [32], are recalled in Appendix A.

Figure 2. Distribution $P\left(M\right)$ of magnetic quantum number M (angular momentum projection) for $j=9/2$ and $N=4$.

Figure 3. Distribution $Q\left(J\right)$ of total angular momentum J for $j=9/2$ and $N=4$.

In the present work, we focus on a particular case, a configuration made of n single-j shells, with each shell being populated with a single fermion and each having its own value of j. In such a situation, we could derive two analytical expressions, the first one involving multinomial coefficients and the second one, more compact and more satisfactory from a practical point of view, based on generalized Pascal triangles, i.e., products of simple binomial coefficients.

In Section 2, the distributions of angular momentum J and its projection M in a general configuration made of single-j shells are introduced, the Sunko algorithm is explained and illustrated [34,35], and useful expressions in terms of Gaussian polynomials (or q−binomial coefficients) [36], symmetric functions and cyclotomic polynomials [37] are outlined. In Section 3, integral expressions of $P\left(M\right)$ and $Q\left(J\right)$ in the case of a configuration made of non-equivalent fermions in single-j orbits (corresponding to $j_k=j$ and $N_k=1$, $\forall k\in [1,r]$ in Equation (2)) [38,39,40] are derived in the framework of group theory, using irreducible representations of $\mathrm{SO}\left(3\right)$ (or of the Lie group $\mathrm{SU}\left(2\right)$; see Appendix B), and exact expressions involving multinomial coefficients are deduced. In Section 4, exact expressions in terms of generalized Pascal triangle coefficients are given. Special cases for $j=1/2$ are presented in Section 5 in terms of hypergeometric functions, and sum rules over angular momentum J are given in Section 6. Expressions for the total number of levels in a configuration and possibilities offered by the Molien functions are explained in Appendices Appendix C and Appendix D, respectively.

2. Characterization of the Magnetic Quantum Number Distribution

2.1. Generalities

Since the quantum number J is the eigenvalue of no simple operator, its mathematical study is tedious. Therefore, it is more appropriate to study the distribution of the magnetic quantum number M. The number $Q\left(J\right)$ of levels with angular momentum J in a configuration can be obtained from the M values by means of

$$Q\left(J\right)=\sum _{M=J}^{J+1}{(-1)}^{J-M}P\left(M\right)=P\left(M=J\right)-P\left(M=J+1\right),$$

(3)

where P represents the distribution of the angular momentum projection M. Let us consider a system of N identical fermions in a configuration consisting of a single orbital j (which is a half-integer) of degeneracy $g=2j+1$, with $m_i$ being the angular momentum projection of electron state i ($m_1=-j,m_2=-j+1,m_3=-j+2,\cdots ,m_{g-1}=j-1,m_g=j$). The following constraint must be satisfied:

$$N=n_1+\cdots +n_g=\sum _{i=1}^g{n}_i,$$

where $n_i$ is the number of fermions in state i ($n_i=0$ or 1 $\forall i$). $P\left(M\right)$ is the number of N-electron states such as

$$M=n_1{m}_1+\cdots +n_g{m}_g=\sum _{i=1}^g{n}_i{m}_i.$$

The latter condition can be formulated in an alternative way: if we have N fermions of spin j, the number of possible states with total projection M is equal to the number of all arrangements of length $2j+1$ for which

$$m_1+m_2+\cdots m_N=M,$$

(4)

where $m_i$ ($m_i<m_{i+1}$) are the single-particle projections ranging from $-j$ to j. The maximum total angular momentum is

$$J_{\max }=\sum _{m=j-N+1}^{j}m=(2j+1-N)N/2$$

and the minimum angular momentum $J_{\min }$ is 0 if N is even and $1/2$ if N is odd.

2.2. Binomial Coefficients and the Sunko Algorithm

Let us consider a single-j shell populated by N fermions (in other words, a configuration $j^N$). In this case, $P\left(M\right)$ is in fact the coefficient of $q^M$ in

$$q^{-{\displaystyle \frac{N}{2}}(2j+1-N)}{\left[\begin{array}{c}2j+1\\ N\end{array}\right]}_q=q^{-J_{\max }}{\left[\begin{array}{c}2j+1\\ N\end{array}\right]}_q,$$

where

$${\left[\begin{array}{c}2j+1\\ N\end{array}\right]}_q={\displaystyle \frac{\left(q^{2j+1}-1\right)\left(q^{2j+1}-q\right)\left(q^{2j+1}-q^2\right)\cdots \left(q^{2j+1}-q^{N-1}\right)}{\left(q^N-1\right)\left(q^N-q\right)\left(q^N-q^2\right)\cdots \left(q^N-q^{N-1}\right)}}.$$

(5)

One also has (see Ref. [26], Equations (3.1)–(3.4))

$$P\left(M\right)={\displaystyle \frac{1}{(J_{\max }+M)!}}{\left.{\displaystyle \frac{d^{J_{\max }+M}}{d{q}^{J_{\max }+M}}}\left[{\displaystyle \frac{\left(q^{2j+1}-1\right)\left(q^{2j}-1\right)\cdots \left(q^{2j+2-N}-1\right)}{\left(q^N-1\right)\left(q^{N-1}-1\right)\cdots \left(q-1\right)}}\right]\right|}_{q=0},$$

(6)

which amounts to the derivative of a rational fraction. Since Equation (6) is not very convenient in practice, Sunko and Srvtan suggested writing (omitting the factor $q^{-J_{\max }}$ and replacing $P\left(M\right)$ by $P\left(q\right)$) [34,35]

$$P\left(q\right)=\sum _{m\ge 0}{c}_m{q}^m=exp\left[\sum _{k\ge 1}{\displaystyle \frac{1}{k}}{p}_k{q}^k\right],$$

where the $c_m$ and $p_k$ are connected by the determinantal identity [41]

$$c_m={\displaystyle \frac{1}{m!}}\left|\begin{array}{ccccccc}{p}_1& -1& 0& 0& 0& \cdots & 0\\ p_2& p_1& -2& 0& & \cdots & 0\\ p_3& p_2& p_1& -3& 0& \cdots & 0\\ ⋮& ⋮& ⋮& ⋮& ⋮& ⋮& ⋮\\ ⋮& ⋮& ⋮& ⋮& p_1& (-m+2)& 0\\ p_{m-1}& p_{m-2}& p_{m-3}& \cdots & p_2& p_1& (-m+1)\\ p_m& p_{m-1}& p_{m-2}& \cdots & p_3& p_2& p_1\end{array}\right|,$$

(7)

or equivalently

$$m{c}_m=p_1{c}_{m-1}+p_1{c}_{m-1}+\cdots +p_{m-1}{c}_1+p_m.$$

The point is that it is easy to obtain a closed-form expression for the $p_k$s of a Gaussian polynomial because its logarithm is a simple expression. Using the expansion of $ln(1-x)$, one obtains (setting $p=2j+1$ and $r=N$)

$$p_m=\sum _{\begin{array}{c}s=1,\\ s|m\end{array}}^{min(r,p-r)}s-\sum _{\begin{array}{c}s=max(r,p-r)+1,\\ s|m\end{array}}^{p}s,$$

where $s|m$ means that s is divided by m, and we have

$$c_m={\displaystyle \frac{1}{m}}\left(p_m+\sum _{q=1}^{m-1}{c}_q{p}_{m-q}\right)$$

(8)

with $c_0=1$ and $c_k=0$ for $k<0$. If we consider the example ${(7/2)}^3$: $j=7/2$ and $N=3$, corresponding to $p=2j+1=8$ and $r=N=3$, the maximum value of J is $J_{\max }=15/2$ and therefore

$$p_m=\sum _{\begin{array}{c}s=1,\\ s|m\end{array}}^{3}s-\sum _{\begin{array}{c}s=6,\\ s|m\end{array}}^{8}s.$$

The parameters $p_k$ and $c_k$ are given in Table 1 and the values of $Q\left(J\right)$ are in Table 2. For instance, if $k=4$,

$$p_4=\sum _{\begin{array}{c}s=1,\\ s|4\end{array}}^{3}s-\sum _{\begin{array}{c}s=6,\\ s|4\end{array}}^{8}s.$$

(9)

Table 1. Parameters $p_k$ and $c_k$ required by Sunko’s method in the ${(7/2)}^3$ case.

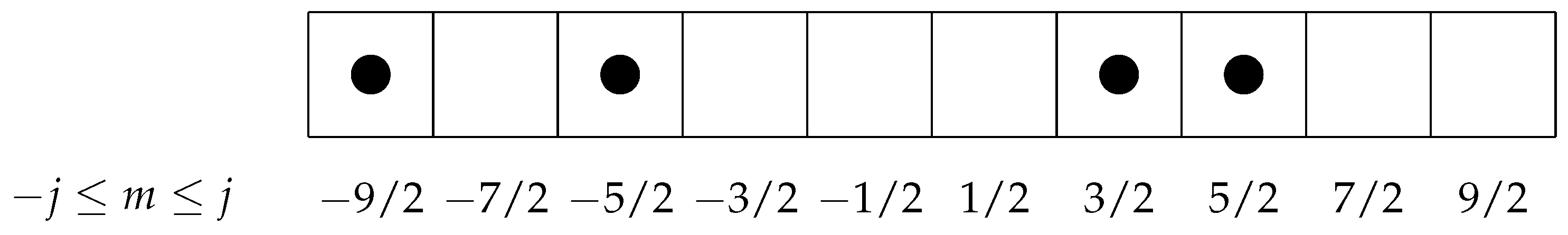
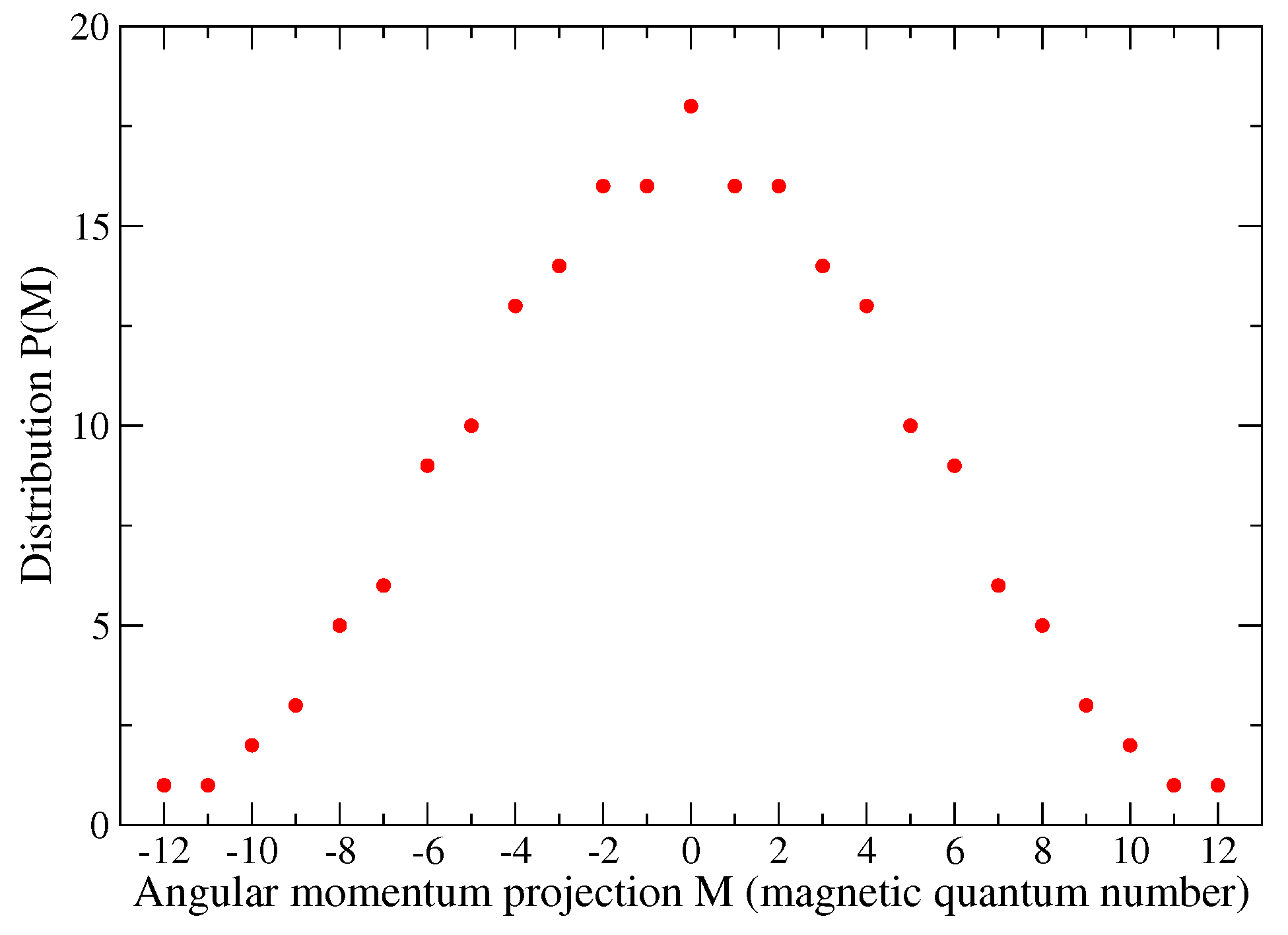
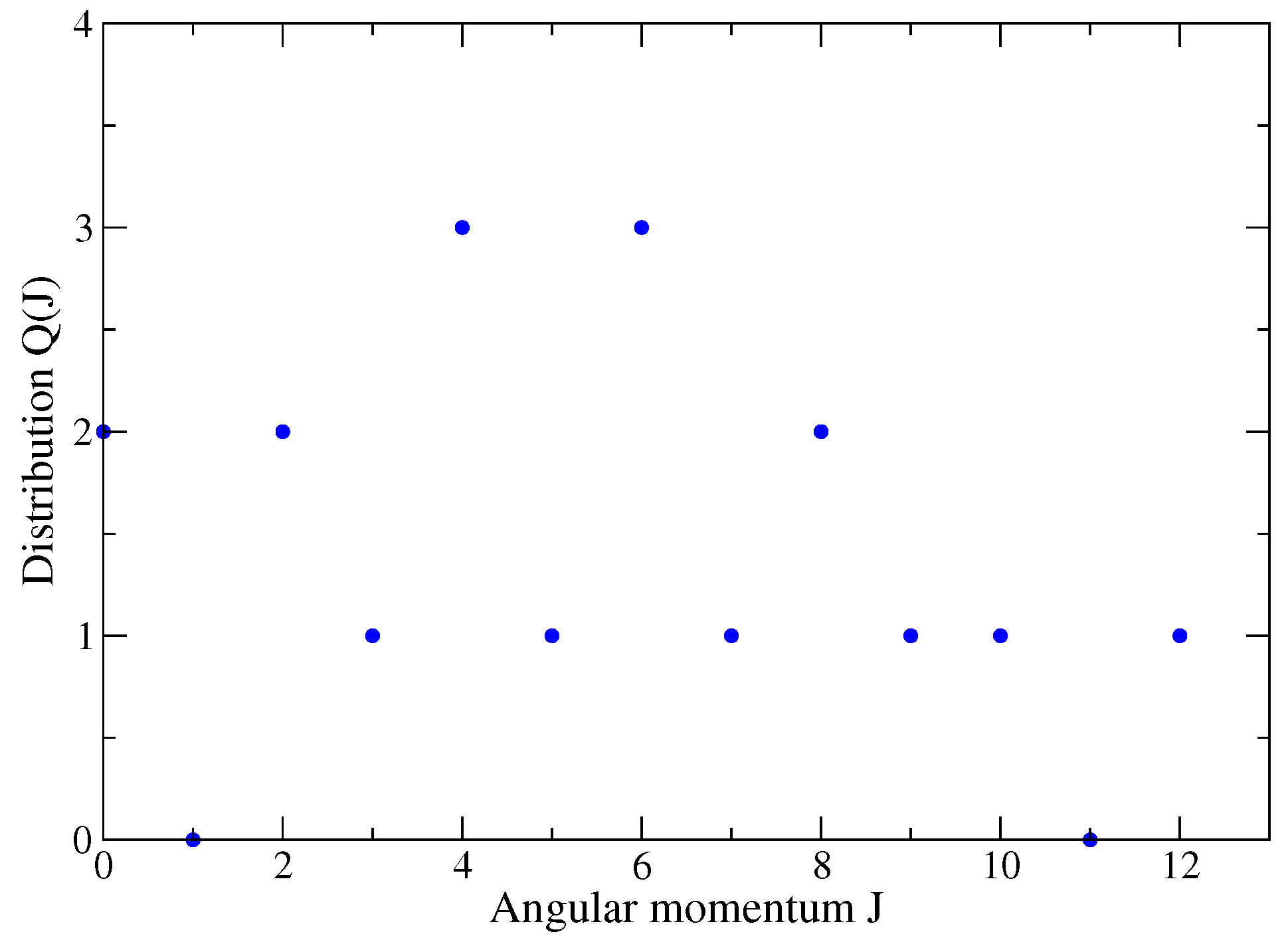
k	0	1	2	3	4	5	6	7
$p_k$	/	1	3	4	3	1	0	−6
$c_k$	1	1	2	3	4	5	6	6

Table 2. Distribution of $Q\left(J\right)$ in the ${(7/2)}^3$ case.

J	1/2	3/2	5/2	7/2	9/2	11/2	13/2	15/2
$Q\left(J\right)$	$c_7-c_6$	$c_6-c_5$	$c_5-c_4$	$c_4-c_3$	$c_3-c_2$	$c_2-c_1$	$c_1-c_0$	$c_0$
$Q\left(J\right)$	0	1	1	1	1	1	0	1

As concerns the first sum in Equation (9), the only divisors of 4 between 1 and 3 are 1 and 2. This sum is equal to 1 + 2 = 3. The second sum in Equation (9) is equal to 0, because there are no divisors of 4 between 6 and 8. Thus, $p_4=3-0=3$. In the same way, for $k=7$, one has to calculate

$$p_7=\sum _{\begin{array}{c}s=1,\\ s|7\end{array}}^{3}s-\sum _{\begin{array}{c}s=6,\\ s|7\end{array}}^{8}s.$$

(10)

As concerns the first sum in Equation (10), the only divisor of 7 between 1 and 3 is 1. This sum is equal to 1. The second sum in Equation (10) is equal to 7, because the only divisor of 7 between 6 and 8 is 7. Thus, $p_7=1-7=-6$. Once the $p_k$ is known, the $c_k$ can be obtained by the recurrence relation (8).

Considering the example ${(7/2)}^3{(5/2)}^2{(1/2)}^1{(9/2)}^2$, one has $J_{\max }=20$ and

$$p_m=\sum _{\begin{array}{c}s=1,\\ s|m\end{array}}^{3}s-\sum _{s=6,s|m}^{8}s+\sum _{\begin{array}{c}s=1,\\ s|m\end{array}}^{2}s-\sum _{\begin{array}{c}s=5,\\ s|m\end{array}}^{6}s+\sum _{\begin{array}{c}s=1,\\ s|m\end{array}}^{1}s-\sum _{\begin{array}{c}s=2,\\ s|m\end{array}}^{2}s+\sum _{\begin{array}{c}s=1,\\ s|m\end{array}}^{2}s-\sum _{\begin{array}{c}s=9,\\ s|m\end{array}}^{10}s.$$

The parameters $p_k$ and $c_k$ are listed in Table 3 and Table 4. The corresponding values of $Q\left(J\right)$ are displayed in Table 5 and Table 6.

Table 3. Parameters $p_k$ and $c_k$ required by Sunko’s method in the ${(7/2)}^3{(5/2)}^2{(1/2)}^1{(9/2)}^2$ case for $k=0$ to 10.

k	0	1	2	3	4	5	6	7	8	9	10
$p_k$	/	4	8	7	8	−1	−1	−3	0	−2	−7
$c_k$	1	4	12	29	62	119	211	349	545	808	1144

Table 4. Parameters $p_k$ and $c_k$ required by Sunko’s method in the ${(7/2)}^3{(5/2)}^2{(1/2)}^1{(9/2)}^2$ case for $k=11$ to 20.

k	11	12	13	14	15	16	17	18	19	20
$p_k$	4	−1	4	1	2	0	4	−10	4	−7
$c_k$	1553	2028	2554	3108	3661	4179	4628	4975	5195	5270

Table 5. Distribution $Q\left(J\right)$ in the ${(7/2)}^3{(5/2)}^2{(1/2)}^1{(9/2)}^2$ case for $J=0$ to 10.

J	0	1	2	3	4	5	6	7	8	9	10
$Q\left(J\right)$	$c_{20}$	$c_{19}$	$c_{18}$	$c_{17}$	$c_{16}$	$c_{15}$	$c_{14}$	$c_{13}$	$c_{12}$	$c_{11}$	$c_{10}$
	$-c_{19}$	$-c_{18}$	$-c_{17}$	$-c_{16}$	$-c_{15}$	$-c_{14}$	$-c_{13}$	$-c_{12}$	$-c_{11}$	$-c_{10}$	$-c_9$
$Q\left(J\right)$	75	220	347	449	518	553	554	526	475	409	336

Table 6. Distribution $Q\left(J\right)$ in the ${(7/2)}^3{(5/2)}^2{(1/2)}^1{(9/2)}^2$ case for $k=11$ to 20.

J	11	12	13	14	15	16	17	18	19	20
$Q\left(J\right)$	$c_9$	$c_8$	$c_7$	$c_6$	$c_5$	$c_4$	$c_3$	$c_2$	$c_1$	$c_0$
	$-c_8$	$-c_7$	$-c_6$	$-c_5$	$-c_4$	$-c_3$	$-c_2$	$-c_1$	$-c_0$
$Q\left(J\right)$	263	196	138	92	57	33	17	8	3	1

The last example ${(7/2)}^3{(3/2)}^2{(5/2)}^1{(9/2)}^2$ corresponds to $J_{\max }=43/2$ and

$$p_m=\sum _{\begin{array}{c}s=1,\\ s|m\end{array}}^{3}s-\sum _{\begin{array}{c}s=6,\\ s|m\end{array}}^{8}s+\sum _{\begin{array}{c}s=1,\\ s|m\end{array}}^{2}s-\sum _{\begin{array}{c}s=3,\\ s|m\end{array}}^{4}s+\sum _{\begin{array}{c}s=1,\\ s|m\end{array}}^{2}s-\sum _{\begin{array}{c}s=5,\\ s|m\end{array}}^{6}s+\sum _{\begin{array}{c}s=1,\\ s|m\end{array}}^{2}s-\sum _{\begin{array}{c}s=9,\\ s|m\end{array}}^{10}s,$$

yielding the $p_k$ and $c_k$ parameters of Table 7, Table 8 and Table 9. The $Q\left(J\right)$ values are in Table 10 and Table 11.

Table 7. Parameters $p_k$ and $c_k$ required by Sunko’s method in the ${(7/2)}^3{(3/2)}^2{(5/2)}^1{(9/2)}^2$ case for $k=0$ to 10.

k	0	1	2	3	4	5	6	7	8	9	10
$p_k$	/	4	12	4	8	−1	0	−3	0	−5	−3
$c_k$	1	4	14	36	84	171	324	565	932	1449	2153

Table 8. Parameters $p_k$ and $c_k$ required by Sunko’s method in the ${(7/2)}^3{(3/2)}^2{(5/2)}^1{(9/2)}^2$ case for $k=11$ to 15.

k	11	12	13	14	15
$p_k$	4	−4	4	5	−1
$c_k$	3054	4168	5472	6943	8515

Table 9. Parameters $p_k$ and $c_k$ required by Sunko’s method in the ${(7/2)}^3{(3/2)}^2{(5/2)}^1{(9/2)}^2$ case for $k=16$ to 21.

k	16	17	18	19	20	21
$p_k$	0	4	−9	4	−7	−3
$c_k$	10,123	11,668	13,062	14,204	15,018	15,440

Table 10. Distribution $Q\left(J\right)$ in the ${(7/2)}^3{(3/2)}^2{(5/2)}^1{(9/2)}^2$ case for $J=1/2$ to 21/2.

J	1/2	3/2	5/2	7/2	9/2	11/2	13/2	15/2	17/2	19/2	21/2
$Q\left(J\right)$	$c_{21}$	$c_{20}$	$c_{19}$	$c_{18}$	$c_{17}$	$c_{16}$	$c_{15}$	$c_{14}$	$c_{13}$	$c_{12}$	$c_{11}$
	$-c_{20}$	$-c_{19}$	$-c_{18}$	$-c_{17}$	$-c_{16}$	$-c_{15}$	$-c_{14}$	$-c_{13}$	$-c_{12}$	$-c_{11}$	$-c_{10}$
$Q\left(J\right)$	422	814	1142	1394	1545	1608	1572	1471	1304	1114	901

Table 11. Distribution $Q\left(J\right)$ in the ${(7/2)}^3{(3/2)}^2{(5/2)}^1{(9/2)}^2$ case for $J=23/2$ to 43/2.

J	23/2	25/2	27/2	29/2	31/2	33/2	35/2	37/2	39/2	41/2	43/2
$Q\left(J\right)$	$c_{10}$	$c_9$	$c_8$	$c_7$	$c_6$	$c_5$	$c_4$	$c_3$	$c_2$	$c_1$	$c_0$
	$-c_9$	$-c_8$	$-c_7$	$-c_6$	$-c_5$	$-c_4$	$-c_3$	$-c_2$	$-c_1$	$-c_0$
$Q\left(J\right)$	704	517	367	241	153	87	48	22	10	3	1

This algorithm does not yield an analytic expression of $P\left(M\right)$ or $Q\left(J\right)$, but is used as our reference calculation.

2.3. Connection with Elementary Symmetric Functions

Since we have

$$\prod _{i=1}^{2j+1}\left(1+q^{i}t\right)=\sum _{k=0}^{2j+1}{t}^k{e}_k\left(q,q^2,q^3,\cdots ,q^{2j+1}\right),$$

where $e_k$ is the kth elementary symmetric function, e.g.,

$$e_2(x_1,x_2,x_3)=x_1{x}_2+x_2{x}_3+x_1{x}_3$$

and

$$\prod _{i=1}^{2j+1}\left(1+q^{i}t\right)=\sum _{k=0}^{2j+1}{t}^k{q}^{k(k+1)/2}{\left[\begin{array}{c}2j+1\\ k\end{array}\right]}_q,$$

we obtain

$$q^{k(k+1)/2}{\left[\begin{array}{c}2j+1\\ k\end{array}\right]}_q=e_k\left(q,q^2,q^3,\cdots ,q^{2j+1}\right),$$

i.e., $P\left(M\right)$ is the coefficient of $q^M$ in

$$q^{-N(j+1)}{e}_N\left(q,q^2,q^3,\cdots ,q^{2j+1}\right).$$

2.4. Link with Cyclotomic Polynomials

The binomial coefficient can be expressed in terms of cyclotomic polynomials ${\Phi }_n$ as

$${\left[\begin{array}{c}n\\ k\end{array}\right]}_q=\prod _{d=2}^n{\Phi }_d{\left(q\right)}^{\lfloor n/d\rfloor -\lfloor k/d\rfloor -\lfloor (n-k)/d\rfloor },$$

where $\lfloor x\rfloor$ denotes the integer part of x and

$${\Phi }_n\left(q\right)=\prod _{\begin{array}{c}1\le k<n,\\ (k,n)=1\end{array}}\left(q-{\zeta }^k\right)\mathrm{with}\zeta =e^{{\displaystyle \frac{2i\pi }{n}}},$$

where $(k,n)=1$ means that k and n are relatively prime to each other. One also has

$${\Phi }_n\left(x\right)=\prod _{d|n}{\left(x^d-1\right)}^{\mu \left({\displaystyle \frac{n}{d}}\right)},$$

where $\mu$ represents the Möbius function:

$$\mu \left(n\right)=\left\{\begin{array}{c}1\mathrm{if}n=1,\hfill \\ {(-1)}^k\mathrm{if}n\mathrm{square}\mathrm{free}\mathrm{and}k\mathrm{number}\mathrm{of}\mathrm{prime}\mathrm{divisors}\mathrm{of}n,\hfill \\ 0\mathrm{otherwise}.\hfill \end{array}\right.$$

Using the formula for the multiple derivative of a product, we have

$${\displaystyle \frac{{\partial }^n}{\partial x^n}}\left(\prod _{i=1}^k{f}_i\right)=\sum _{j_1+j_2+\cdots +j_k=n}\left(\begin{array}{c}n\\ j_1,j_2,\cdots ,j_k\end{array}\right)\prod _{i=1}^k{\displaystyle \frac{{\partial }^{j_i}}{\partial x^{j_i}}}{f}_i,$$

(11)

where

$$\left(\begin{array}{c}n\\ j_1,j_2,\cdots ,j_k\end{array}\right)={\displaystyle \frac{n!}{j_1!j_2!\cdots j_k!}}$$

is the usual multinomial coefficient. We can write

$$\begin{array}{cccc}\hfill & {\displaystyle \frac{{\partial }^p}{\partial q^p}}\prod _{\begin{array}{c}1\le k<n,\\ (k,n)=1\end{array}}\left(q-{\zeta }^k\right)\hfill & \hfill =\sum _{{\ell }_1+{\ell }_2+\cdots +{\ell }_{n-1}=p}\left(\begin{array}{c}p\\ {\ell }_1,{\ell }_2,\cdots ,{\ell }_{n-1}\end{array}\right)& \prod _{i=1}^{n-1}{\displaystyle \frac{{\partial }^{{\ell }_i}}{\partial q^{{\ell }_i}}}\left(q-{\zeta }^k\right),\hfill \end{array}$$

with

$${\displaystyle \frac{{\partial }^{{\ell }_i}}{\partial q^{{\ell }_i}}}\left(q-{\zeta }^k\right)=\left[{\ell }_i+\left(1-{\ell }_i\right)\left(q-{\zeta }^k\right)\right]\theta \left(1-{\ell }_i\right).$$

One has in particular

$$x^n-1=\prod _{d|n}{\Phi }_d\left(x\right),$$

yielding for instance $x^{16}-1={\Phi }_1\left(x\right){\Phi }_2\left(x\right){\Phi }_4\left(x\right){\Phi }_8\left(x\right){\Phi }_{16}\left(x\right)$.

3. Expressions Involving Multinomial Coefficients

3.1. Integral Representation from Group Theory

Let us consider ${\mathcal{D}}^{\left(j\right)}$ a representation of dimension $2j+1$ of the group $\mathrm{SU}\left(2\right)$, and $\mathcal{U}\left(\theta \right)$ an element of $\mathrm{SU}\left(2\right)$ corresponding to the rotation of angle $\theta$ around axis (Oz). The quantity

$${\chi }^j\left(\mathcal{U}\right)=\mathrm{Tr}{\mathcal{D}}^{\left(j\right)}=\sum _{m=-j}^j{\mathcal{D}}_{mm}^j\left(\mathcal{U}\right)=\sum _{m=-j}^j⟨jm|{\mathcal{D}}^{\left(j\right)}|jm⟩,$$

where $\mathcal{D}$ is the Wigner function, is called the characteristic function or the character of the irreducible representation of rank j [42,43,44,45]. In contrast to ${\mathcal{D}}_{m{m}^{\prime }}^j\left(\mathcal{U}\right)$, the function ${\chi }^j\left(\mathcal{U}\right)$ is invariant under rotations of the coordinate system. ${\chi }^j\left(\mathcal{U}\right)$ is entirely determined by the rotation angle $\theta$ and is independent of the rotation axis: ${\chi }^j\left(\mathcal{U}\right)\equiv {\chi }^j\left(\theta \right)$. $\theta$ is related to the Euler angles by

$$cos\left({\displaystyle \frac{\theta }{2}}\right)=cos\left({\displaystyle \frac{\beta }{2}}\right)cos\left({\displaystyle \frac{\alpha +\gamma }{2}}\right).$$

There are many possible expressions for the latter quantity (by an integral representation, etc.); in particular, it can be formulated in terms of ${}_{2}F_1$, the Gauss hypergeometric function, and Jacobi $P_{2j}^{(1/2,1/2)}$[46,47], Gegenbauger $C_{2j}^1$[48,49] or Chebyshev polynomials of the second kind $U_{2j}$ [50]:

$$\begin{array}{ccc}\hfill {\chi }^j\left(\theta \right)& =& (2j+1) {}_{2}F_1\left[\begin{array}{c}-j,j+1\\ 3/2\end{array};{sin}^2\left({\displaystyle \frac{\theta }{2}}\right)\right]=(2j+1) {}_{2}F_1\left[\begin{array}{c}-2j,2(j+1)\\ 3/2\end{array};{sin}^2\left({\displaystyle \frac{\theta }{2}}\right)\right]\hfill \\ & =& U_{2j}\left[cos\left({\displaystyle \frac{\theta }{2}}\right)\right]=C_{2j}^1\left[cos\left({\displaystyle \frac{\theta }{2}}\right)\right]={\displaystyle \frac{(4j+2)!!}{2(4j+1)!!}}{P}_{2j}^{(1/2,1/2)}\left[cos\left({\displaystyle \frac{\theta }{2}}\right)\right],\hfill \end{array}$$

where $m!!=m(m-2)(m-3)\cdots$. One has

$${\chi }^j\left(\theta \right)=\sum _{m=-j}^j{e}^{-im\theta }={\displaystyle \frac{sin\left[(j+1/2)\theta \right]}{sin(\theta /2)}},$$

and the characters obey the orthogonality relation

$${\int }_0^{2\pi }d\theta {sin}^2\left({\displaystyle \frac{\theta }{2}}\right){\chi }^{j_1}\left(\theta \right){\chi }^{j_2}\left(\theta \right)=\pi {\delta }_{j_1,j_2},$$

where ${\delta }_{j_1,j_2}$ represents the Kroneckers symbol and

$${\int }_0^{2\pi }d\theta {sin}^2\left({\displaystyle \frac{\theta }{2}}\right){\chi }^{j_1}\left(\theta \right){\chi }^{j_2}\left(\theta \right){\chi }^{j_3}\left(\theta \right)=\pi \left\{j_1,j_2,j_3\right\},$$

(12)

where $\left\{j_1,j_2,j_3\right\}=1$ if $j_1+j_2+j_3$ is an integer and $|j_1-j_2|\le j_3\le j_1+j_2$, and 0 otherwise. Among particular values, one has ${\chi }^j\left(0\right)=2j+1$, ${\chi }^j\left(2\pi \right)={(-1)}^{2j}(2j+1)$ and ${\chi }^j\left(\pi \right)=0$ if J is a half-integer and ${(-1)}^j$ if J is an integer. It is worth noting that the three coordinates of one vector span the irreducible representation (1) of $\mathrm{SO}\left(3\right)$ and the $3N$ coordinates of the N vectors span the reducible representation $\left(1\right)\oplus \cdots \oplus \left(1\right)$ N times. In fact, the group $\mathrm{SO}\left(3\right)$ acts diagonally on the direct sum of the various symmetric tensor powers of this representation or, equivalently, on polynomials in the coordinates. The Molien generating function associated with a given irreducible representation j is the power series in a variable $\lambda$ where the coefficient of ${\lambda }^n$ is the multiplicity of j in the $\mathrm{SO}\left(3\right)$-module of n-degree polynomials in the coordinates (see Appendix D and references therein).

In contrast to the ${\mathcal{D}}_{m{m}^{\prime }}^j$, the characters ${\chi }^j\left(\mathcal{U}\right)$ are real. The characters which depend on the combined rotations ${\mathcal{U}}_1{\mathcal{U}}_2\cdots {\mathcal{U}}_n$ do not change under cyclic permutations of the rotations ${\chi }^j({\mathcal{U}}_1{\mathcal{U}}_2\cdots {\mathcal{U}}_n)={\chi }^j({\mathcal{U}}_2\cdots {\mathcal{U}}_n{\mathcal{U}}_1$), and therefore ${\chi }^j\left({\mathcal{U}}_1{\mathcal{U}}_2\right)={\chi }^j\left({\mathcal{U}}_2{\mathcal{U}}_1\right)$ in spite of the non-commutativity of ${\mathcal{U}}_1$ and ${\mathcal{U}}_2$.

For n non-equivalent fermions in shells j (i.e., a configuration $P_{{\otimes }_{n}j}$ as defined in the introduction), let us consider the representation

$$\underset{n\mathrm{times}}{\underbrace{{\mathcal{D}}^{\left(j\right)}\otimes {\mathcal{D}}^{\left(j\right)}\otimes \cdots \otimes {\mathcal{D}}^{\left(j\right)}}}.$$

According to a property of the tensorial product,

$$\mathrm{Tr}\left\{\underset{n\mathrm{times}}{\underbrace{{\mathcal{D}}^{\left(j\right)}\otimes {\mathcal{D}}^{\left(j\right)}\otimes \cdots \otimes {\mathcal{D}}^{\left(j\right)}}}\right\}=\underset{n\mathrm{times}}{\underbrace{\left(\mathrm{Tr}{\mathcal{D}}^{\left(j\right)}\right)\left(\mathrm{Tr}{\mathcal{D}}^{\left(j\right)}\right)\cdots \left(\mathrm{Tr}{\mathcal{D}}^{\left(j\right)}\right)}}$$

and the character ${\chi }_{\left(n\right)}$ of such a representation is therefore

$${\chi }_{\left(n\right)}\left(\theta \right)={\left[{\chi }^j\left(\theta \right)\right]}^n={\left({\displaystyle \frac{sin\left[\left(j+\frac{1}{2}\right)\theta \right]}{sin\left[\frac{\theta }{2}\right]}}\right)}^n.$$

All rotations through the same angle $\theta$ about different axes through the same center have the same character. From a group theory point of view, they belong to the same class. The characters of different irreducible representations (called the irreducible characters) are orthogonal over the range $(0,\pi )$ with the weight function $(1-cos\theta )$. Indeed,

$${\displaystyle \frac{2}{\pi }}{\int }_0^{\pi }{\chi }^j\left(\theta \right){\chi }^{j^{\prime }}\left(\theta \right){sin}^2\left({\displaystyle \frac{\theta }{2}}\right)d\theta ={\delta }_{j,j^{\prime }}.$$

(13)

The multiplicity $Q_{{\otimes }_{n}j}\left(J\right)$ which we wish to determine appears in the decomposition

$${\chi }_{\left(n\right)}\left(\theta \right)=\sum _{J=J_0}^{nj}{Q}_{{\otimes }_{n}j}\left(J\right){\chi }^J\left(\theta \right),$$

where $J_0$ = 0 or 1/2 depending upon whether $nj$ is an integer or half-integer and J moves in steps of 1 from $J_0$ to $nj$. $Q_{{\otimes }_{n}j}\left(J\right)$ denotes the distribution $Q\left(J\right)$ for n non-equivalent fermions in single-j shells, i.e., for a configuration

$$\underset{n\mathrm{times}}{\underbrace{j^1{j}^1\cdots j^1}}.$$

(14)

The orthogonality (Equation (13)) of the irreducible characters then results in an integral representation for the multiplicity. Mikhailov has given an analytic expression for the multiplicity $Q_{{\otimes }_{n}j}\left(J\right)$ of occurrence of any angular momentum J in the decomposition of the direct product of n identical angular momenta j [42]. He guessed the expression by examining special cases for low values of n. However, a proof based on combinatorics has been published, to our knowledge, only for $J\ge s$. In the following, we shall present another method of arriving at this expression which does not distinguish between the two cases $J\ge j$ and $J<j$.

The Haar measure enables one to integrate over the elements $\mathcal{U}$ of the group $\mathrm{SU}\left(2\right)$, which enables one to extend to a compact Lie group the notion, usual for finite groups, of summation over elements of that group. Such a measure reads

$$d\mu \left(\theta \right)=2(1-cos\theta )d\theta d^2\overrightarrow{n},$$

(15)

where $d^2\overrightarrow{n}$ is the integration measure over unit sphere $S^2$. $\theta$ varies between 0 and $4\pi$. One finds then that the volume of the group $\mathrm{SU}\left(2\right)$ is $16{\pi }^2$. One can show that

$$\int {\displaystyle \frac{d\mu \left(\theta \right)}{16{\pi }^2}}{\chi }^{j\ast }\left(\theta \right){\chi }^{j^{\prime }}\left(\theta \right)={\delta }_{j,j^{\prime }}$$

or

$${\int }_0^{2\pi }(1-cos\theta ){\chi }^{j\ast }\left(\theta \right){\chi }^{j^{\prime }}\left(\theta \right)d\theta =2\pi {\delta }_{j,j^{\prime }}.$$

In order to obtain $Q_{{\otimes }_{n}j}\left(J\right)$, one has to project such a character on the character ${\chi }^J$ of ${\mathcal{D}}^{\left(J\right)}$, which yields

$$Q_{{\otimes }_{n}j}\left(J\right)=\int {\displaystyle \frac{d\mu \left(\theta \right)}{16{\pi }^2}}{\chi }_{\left(n\right)}\left(\theta \right){\chi }^J\left(\theta \right),$$

or, setting $\varphi =\theta /2$,

$$Q_{{\otimes }_{n}j}\left(J\right)={\displaystyle \frac{2}{\pi }}{\int }_0^{\pi }{\left({\displaystyle \frac{sin\left[\left(2j+1\right)\varphi \right]}{sin\varphi }}\right)}^{n}sin\left[(2J+1)\varphi \right]sin\varphi d\varphi ,$$

which is also equal to

$$Q_{{\otimes }_{n}j}\left(J\right)={\displaystyle \frac{1}{\pi }}{\int }_0^{2\pi }{\left({\displaystyle \frac{sin\left[\left(2j+1\right)\varphi \right]}{sin\varphi }}\right)}^{n}sin\left[(2J+1)\varphi \right]sin\varphi d\varphi ,$$

and the integral representation of the distribution of angular momentum projection M reads

$$P_{{\otimes }_{n}j}\left(M\right)={\displaystyle \frac{1}{\pi }}{\int }_0^{\pi }{\left({\displaystyle \frac{sin\left[(2j+1)\varphi \right]}{sin\varphi }}\right)}^{n}cos\left(2M\varphi \right)d\varphi ,$$

where $P_{{\otimes }_{n}j}\left(J\right)$ denotes the distribution $P\left(M\right)$ for n non-equivalent electrons in single-j shells, i.e., for a configuration of Equation (14). Thus, for the simple case $j=1/2$, one has

$$Q_{{\otimes }_n{\displaystyle \frac{1}{2}}}\left(J\right)={\displaystyle \frac{2^{n+1}}{\pi }}{\int }_0^{\pi }{cos}^n\varphi sin\left[(2J+1)\varphi \right]sin\varphi d\varphi ,$$

which is in fact equal to

$$Q_{{\otimes }_n{\displaystyle \frac{1}{2}}}\left(J\right)={\displaystyle \frac{(2J+1)n!}{\left(\frac{n}{2}+J+1\right)!\left(\frac{n}{2}-J\right)!}}.$$

(16)

We also have a connection to the Vilenkin function ${\mathcal{P}}_{mn}^j$ [51,52,53]:

$$\sum _{m=-j}^j{\mathcal{P}}_{mm}^j\left[cos\left(\theta \right)\right]={\displaystyle \frac{sin\left[\left(j+\frac{1}{2}\right)\theta \right]}{sin\left(\frac{\theta }{2}\right)}}=U_{2j}\left[sin\left({\displaystyle \frac{\theta }{2}}\right)\right]$$

related to the Jacobi polynomials $P_n^{(\alpha ,\beta )}$ through

$${\mathcal{P}}_{mm}^j\left[cos\left(\theta \right)\right]={\displaystyle \frac{1}{2^m}}{\left(1+cos\theta \right)}^m{P}_{j-m}^{(0,2m)}.$$

3.2. Expression Using Multinomial Coefficients: A New Sum Rule

Using the Moivre formula, it can be easily proven that

$$sin\left[(2j+1)\varphi \right]=\sum _{0\le 2p+1\le 2j+1}{(-1)}^p\left(\begin{array}{c}2j+1\\ 2p+1\end{array}\right){cos}^{2j-2p}\varphi {sin}^{2p+1}\varphi .$$

(17)

Following the above-mentioned procedure, it is possible to derive a general formula for $Q_{{\otimes }_{n}j}\left(J\right)$, involving multinomial coefficients. One also has

$${\left({\displaystyle \frac{sin\left[(2j+1)\varphi \right]}{sin\varphi }}\right)}^n={\left(\sum _{p=0}^{j-1/2}{a}_p\left(j\right)\right)}^n,$$

i.e.,

$${\left({\displaystyle \frac{sin\left[(2j+1)\varphi \right]}{sin\varphi }}\right)}^n=\sum _{k_0+k_1+k_2+\cdots +k_{j-1/2}=n}\left(\begin{array}{c}n\\ k_0,k_1,k_2,\cdots ,k_{j-1/2}\end{array}\right)a_0^{k_0}{a}_1^{k_1}\cdots a_{j-1/2}^{k_{j-1/2}},$$

with

$$a_p\left(j\right)={(-1)}^p\left(\begin{array}{c}2j+1\\ 2p+1\end{array}\right){cos}^{2j-2p}\varphi {sin}^{2p}\varphi .$$

Since j is a half-integer, we can replace Equation (17) by

$$sin\left[(2j+1)\varphi \right]=\sum _{p=0}^{j-1/2}{(-1)}^p\left(\begin{array}{c}2j+1\\ 2p+1\end{array}\right){cos}^{2j-2p}\varphi {sin}^{2p+1}\varphi .$$

We also have

$$sin\left[(2J+1)\varphi \right]=\sum _{r=0}^B{(-1)}^r\left(\begin{array}{c}2J+1\\ 2r+1\end{array}\right){cos}^{2J-2r}\varphi {sin}^{2r+1}\varphi ,$$

where $B=J-1/2$ if J is a half-integer and $B=J$ if J is an integer, i.e.,

$$B=J+{\displaystyle \frac{{(-1)}^{2J}-1}{4}}.$$

We can therefore write

$$\begin{array}{ccc}\hfill Q_{{\otimes }_{n}j}\left(J\right)& =& {\displaystyle \frac{2}{\pi }}\sum _{k_0+k_1+k_2+\cdots +k_{j-1/2}=n}\sum _{r=0}^B\left(\begin{array}{c}n\\ k_0,k_1,k_2,\cdots ,k_{j-1/2}\end{array}\right){(-1)}^r\left(\begin{array}{c}2J+1\\ 2r+1\end{array}\right)\hfill \\ & & \times {\int }_0^{\pi }\prod _{p=0}^{j-1/2}{(-1)}^{p{k}_p}{\left[\left(\begin{array}{c}2j+1\\ 2p+1\end{array}\right){cos}^{2j-2p}\varphi {sin}^{2p}\varphi \right]}^{k_p}{cos}^{2J-2r}\varphi {sin}^{2r+2}\varphi d\varphi ,\hfill \\ & \end{array}$$

i.e.,

$$\begin{array}{ccc}\hfill Q_{{\otimes }_{n}j}\left(J\right)& =& \sum _{k_0+k_1+k_2+\cdots +k_{j-1/2}=n}\sum _{r=0}^B\left(\begin{array}{c}n\\ k_0,k_1,k_2,\cdots ,k_{j-1/2}\end{array}\right){(-1)}^r\left(\begin{array}{c}2J+1\\ 2r+1\end{array}\right)\hfill \\ & & \times {(-1)}^{{\sum }_{s=0}^{j-1/2}s{k}_s}\left[\prod _{p=0}^{j-1/2}{\left(\begin{array}{c}2j+1\\ 2p+1\end{array}\right)}^{k_p}\right]\hfill \\ & & \times {\int }_0^{\pi }{\left(cos\varphi \right)}^{2J-2r+{\sum }_{s=0}^{j-1/2}(2j-2s)k_s}{\left(sin\varphi \right)}^{2r+2+2{\sum }_{s=0}^{j-1/2}s{k}_s}d\varphi .\hfill \end{array}$$

We then use

$${\int }_0^{\pi }{cos}^a\varphi {sin}^b\varphi d\varphi ={\displaystyle \frac{1+{(-1)}^a}{2}}{\displaystyle \frac{\Gamma \left(\frac{a+1}{2}\right)\Gamma \left(\frac{b+1}{2}\right)}{\Gamma \left(\frac{a+b+2}{2}\right)}},$$

where $\Gamma$ is the usual Gamma function, with

$$a=2J-2r+\sum _{s=0}^{j-1/2}(2j-2s)k_s=2\left(J-r+\sum _{s=0}^{j-1/2}(j-s)k_s\right)$$

and

$$b=2r+2+2\sum _{s=0}^{j-1/2}s{k}_s=2\left(r+1+\sum _{s=0}^{j-1/2}s{k}_s\right).$$

We have

$$\begin{array}{ccc}\hfill {\displaystyle \frac{a+1}{2}}& =& J-r+\sum _{s=0}^{j-1/2}(j-s)k_s+{\displaystyle \frac{1}{2}}=J-r+j\sum _{s=0}^{j-1/2}{k}_s-\sum _{s=0}^{j-1/2}s{k}_s+{\displaystyle \frac{1}{2}}\hfill \\ & =& J-r+jn-\sum _{s=0}^{j-1/2}s{k}_s+{\displaystyle \frac{1}{2}},\hfill \end{array}$$

because ${\sum }_{s=0}^{j-1/2}{k}_s=n$. In the same way,

$${\displaystyle \frac{b+1}{2}}=r+1+\sum _{s=0}^{j-1/2}s{k}_s+{\displaystyle \frac{1}{2}}\mathrm{and}{\displaystyle \frac{a+b+2}{2}}=J+jn+2.$$

We obtain therefore finally

$$\begin{array}{ccc}\hfill Q_{{\otimes }_{n}j}\left(J\right)& =& \sum _{{\sum }_{s=0}^{j-1/2}{k}_s=n}\sum _{r=0}^B\left(\begin{array}{c}n\\ k_0,k_1,k_2,\cdots ,k_{j-1/2}\end{array}\right){(-1)}^r\left(\begin{array}{c}2J+1\\ 2r+1\end{array}\right)\hfill \\ & & \times {(-1)}^{{\sum }_{s=0}^{j-1/2}s{k}_s}\left[\prod _{p=0}^{j-1/2}{\left(\begin{array}{c}2j+1\\ 2p+1\end{array}\right)}^{k_p}\right]{\displaystyle \frac{1+{(-1)}^{2\left(J-r+{\sum }_{s=0}^{j-1/2}(j-s)k_s\right)}}{2}}\hfill \\ & & \times {\displaystyle \frac{\Gamma \left(J-r+jn-{\sum }_{s=0}^{j-1/2}s{k}_s+\frac{1}{2}\right)\Gamma \left(r+1+{\sum }_{s=0}^{j-1/2}s{k}_s+\frac{1}{2}\right)}{\Gamma \left(J+jn+2\right)}}.\hfill \end{array}$$

and a similar formula can of course be derived for $P_{{\otimes }_{n}j}\left(M\right)$.

4. Expression in Terms of Pascal Triangles

For the simple configuration $j^1$, we have

$$\sum _M{P}_j\left(M\right)z^M=z^{-j}+\cdots +z^j.$$

In a general way, the so-called generalized Pascal triangle ${\mathcal{T}}_{m,n,q}$ coefficients are given by [54]:

$${(1+t+t^2+\cdots +t^m)}^n=\sum _q{\mathcal{T}}_{m,n,q}{t}^q$$

(18)

and since

$$\begin{array}{ccc}\hfill {(1+t+t^2+\cdots +t^m)}^n& =& {\left({\displaystyle \frac{1-t^{m+1}}{1-t}}\right)}^n\hfill \\ & =& {\left(1-t^{m+1}\right)}^n\sum _q\left(\begin{array}{c}q+n-1\\ q\end{array}\right)t^q\hfill \\ & =& \sum _{k=0}^n\left(\begin{array}{c}n\\ k\end{array}\right){(-t^{m+1})}^k\sum _q\left(\begin{array}{c}q+n-1\\ q\end{array}\right)t^q\hfill \\ & =& \sum _{k=0}^n\sum _q{(-1)}^k\left(\begin{array}{c}n\\ k\end{array}\right)\left(\begin{array}{c}q+n-1\\ q\end{array}\right)t^{mk+k+q}\hfill \\ & =& \sum _q\left\{\sum _{k=0}^n{(-1)}^k\left(\begin{array}{c}n\\ k\end{array}\right)\left(\begin{array}{c}q-mk-k+n-1\\ q-k-mk\end{array}\right)\right\}{t}^q,\hfill \\ & \end{array}$$

we obtain

$${\mathcal{T}}_{m,n,q}=\sum _{k=0}^n{(-1)}^k\left(\begin{array}{c}n\\ k\end{array}\right)\left(\begin{array}{c}q-mk-k+n-1\\ q-k-mk\end{array}\right).$$

In the framework of the one-dimensional isotropic Heisenberg magnet, the quantum inverse scattering method has led to an algebraic version of the classical substitution method of Bethe (the so-called Bethe ansatz) for the determination of the trace of the monodromy matrix. These eigenvectors were referred to as Bethe vectors [55]. The completeness of the multiplet system constructed from the Bethe vectors was proven by Kirrilov for the Heisenberg model of arbitrary spin. Kirrilov [56] found that the number of Bethe vectors with fixed ℓ for the Heisenberg model of spin S reads (N being the total number of possible states):

$${\mathcal{Z}}_1(N,S|\ell )=\sum _j{(-1)}^j\left(\begin{array}{c}N\\ j\end{array}\right)\left(\begin{array}{c}N+2+\ell -(2S+1)j\\ N-2\end{array}\right),$$

which is also a generalized Pascal triangle coefficient. Therefore, in the case of a configuration made of n shells $j^1$ (i.e., n non-equivalent j fermions), then

$$\sum _{M=-jn}^{jn}{P}_{{\otimes }_{n}j}\left(M\right)z^M={\left(z^{-j}+\cdots +z^j\right)}^n,$$

and in that case, we obtain

$$P_{{\otimes }_{n}j}\left(M\right)=\sum _{k=0}^{min\left(n,\lfloor {\displaystyle \frac{jn+M}{2j+1}}\rfloor \right)}{(-1)}^k\left(\begin{array}{c}n\\ k\end{array}\right)\left(\begin{array}{c}(j+1)n+M-(2j+1)k-1\\ n-1\end{array}\right).$$

Using

$$\left(\begin{array}{c}s\\ t\end{array}\right)=\left(\begin{array}{c}s-1\\ t\end{array}\right)+\left(\begin{array}{c}s-1\\ t-1\end{array}\right),$$

one finds, for $Q\left(J\right)$,

$$Q_{{\otimes }_{n}j}\left(J\right)=\sum _{k=0}^{min\left(n,\lfloor {\displaystyle \frac{jn+J+1}{2j+1}}\rfloor \right)}{(-1)}^{k+1}\left(\begin{array}{c}n\\ k\end{array}\right)\left(\begin{array}{c}(j+1)n+J-(2j+1)k-1\\ n-2\end{array}\right).$$

Such coefficients were also encountered in multiphoton processes, for the proportion of neutral atoms in a statistical description of multiple ionization [57], or in the determination of the number of configurations in atomic physics [58].

Case of Different Angular Momenta

Let us consider a system of n non-equivalent fermions [59,60], each in a single-$j_i$ shell (of degeneracy $g_i=2j_i+1$, $i=1,\cdots ,n$). In the following, such a configuration

$$j_1^1{j}_2^1\cdots j_n^1$$

(19)

will be denoted: $j_1\otimes j_2\otimes \cdots \otimes j_{n-1}\otimes j_n$ (abbreviated $j_1\otimes j_n$ in the following). The number of J levels of such a configuration can be obtained as [61]

$$\begin{array}{ccc}\hfill Q_{j_1\otimes \cdots \otimes j_n}\left(J\right)& =& \sum _{M=J}^{J+1}{(-1)}^{J-M}{P}_{j_1\otimes \cdots \otimes j_n}\left(M\right)\hfill \\ & =& P_{j_1\otimes \cdots \otimes j_n}\left(J\right)-P_{j_1\otimes \cdots \otimes j_n}(J+1),\hfill \end{array}$$

(20)

where $P_{j_1\otimes \cdots \otimes j_n}\left(M\right)$ represents the distribution of the angular momentum projection M, i.e., the number of states of a given value of M. The number of such states for a configuration $j_1\otimes j_2\otimes \cdots \otimes j_{n-1}\otimes j_n$ is equal to the number of times that a given representation $\mathcal{U}$ appears in the product $\mathcal{U}\otimes {\mathcal{U}}_1\otimes {\mathcal{U}}_2\otimes \cdots {\mathcal{U}}_{n-1}\otimes {\mathcal{U}}_n$ [51,52]. Such a quantity reads

$$P_{j_1\otimes \cdots \otimes j_n}\left(M\right)=\int {\chi }^{\ast }\left(\mathcal{U}\right)\chi \left({\mathcal{U}}_1\right)\dots \chi \left({\mathcal{U}}_n\right)d\mu$$

where $\mu$ is a relevant measure (such as the Haar measure; see Equation (15)). The latter expression can be put in the form

$$P_{j_1\otimes \cdots \otimes j_n}\left(M\right)={\displaystyle \frac{1}{\pi }}{\int }_0^{2\pi }{\chi }^M\left(2\theta \right){\chi }^{j_1}\left(2\theta \right)\dots {\chi }^{j_n}\left(2\theta \right){sin}^2\left(\theta \right)d\theta .$$

For a configuration made of n non-equivalent fermions with respective angular momenta $j_1,\cdots ,j_n$, one has

$$\sum _M{P}_{j_1\otimes \cdots \otimes j_n}\left(M\right)z^M=\prod _{i=1}^n\left(z^{-j_i}+\cdots +z^{j_i}\right),$$

i.e.,

$$P_{j_1\otimes \cdots \otimes j_n}\left(M\right)=\sum _{\begin{array}{c}{\sum }_{i=1}^n(2j_i+1)k_i\le j_i+M\\ 0\le k_i\le 1\end{array}}{(-1)}^k\left(\begin{array}{c}n+\sum _{i=1}^n{j}_i+M-\sum _{i=1}^n(2j_i+1)k_i-1\\ n-1\end{array}\right)\prod _{i=1}^n\left(\begin{array}{c}1\\ k_i\end{array}\right)$$

and

$$Q_{j_1\otimes \cdots \otimes j_n}\left(J\right)=\sum _{\begin{array}{c}{\sum }_{i=1}^n(2j_i+1)k_i\le j_i+J\\ 0\le k_i\le 1\end{array}}{(-1)}^{k+1}\left(\begin{array}{c}n+\sum _{i=1}^n{j}_i+J-\sum _{i=1}^n(2j_i+1)k_i-1\\ n-2\end{array}\right)\prod _{i=1}^n\left(\begin{array}{c}1\\ k_i\end{array}\right).$$

In a more general case of a configuration made of $n_1$ non-equivalent $j_1$ fermions, $n_2$ non-equivalent $j_2$ fermions, …, $n_{\sigma }$ non-equivalent $j_{\sigma }$ fermions, we have, with $n={\sum }_{\alpha =1}^{\sigma }{n}_{\alpha }$,

$$\begin{array}{ccc}\hfill P_{\left\{j_{\alpha }^{\otimes n_{\alpha }}\right\}}\left(M\right)& =& \sum _{\begin{array}{c}\left\{s_{\alpha }\right\}/{\sum }_{\alpha =1}^{\sigma }(2j_{\alpha }+1)s_{\alpha }\le {\sum }_{\alpha =1}^{\sigma }{n}_{\alpha }{j}_{\alpha }+M;\\ 0\le s_{\alpha }\le n_{\alpha }\end{array}}{(-1)}^{s_1+s_2+\cdots +s_{\sigma }}\hfill \\ & & \times \left(\begin{array}{c}n+\sum _{\alpha =1}^{\sigma }{n}_{\alpha }{j}_{\alpha }+M-1-\sum _{\alpha =1}^{\sigma }(2j_{\alpha }+1)s_{\alpha }\\ n-1\end{array}\right)\left(\begin{array}{c}{n}_1\\ s_1\end{array}\right)\cdots \left(\begin{array}{c}{n}_{\sigma }\\ s_{\sigma }\end{array}\right)\hfill \end{array}$$

and

$$\begin{array}{ccc}\hfill Q_{\left\{j_{\alpha }^{\otimes n_{\alpha }}\right\}}\left(J\right)& =& \sum _{\begin{array}{c}\left\{s_{\alpha }\right\}/{\sum }_{\alpha =1}^{\sigma }(2j_{\alpha }+1)s_{\alpha }\le {\sum }_{\alpha =1}^{\sigma }{n}_{\alpha }{j}_{\alpha }+J;\\ 0\le s_{\alpha }\le n_{\alpha }\end{array}}{(-1)}^{s_1+s_2+\cdots +s_{\sigma }+1}\hfill \\ & & \times \left(\begin{array}{c}n+\sum _{\alpha =1}^{\sigma }{n}_{\alpha }{j}_{\alpha }+J-1-\sum _{\alpha =1}^{\sigma }(2j_{\alpha }+1)s_{\alpha }\\ n-2\end{array}\right)\left(\begin{array}{c}{n}_1\\ s_1\end{array}\right)\cdots \left(\begin{array}{c}{n}_{\sigma }\\ s_{\sigma }\end{array}\right),\hfill \end{array}$$

where the notation $\left\{j_{\alpha }^{\otimes n_{\alpha }}\right\}$ means $\underset{n_1\mathrm{times}}{\underbrace{j_1\otimes j_1}}\underset{n_2\mathrm{times}}{\underbrace{j_2\otimes j_2}}\cdots \underset{n_{\sigma }\mathrm{times}}{\underbrace{j_{\sigma }\otimes j_{\sigma }}}$.

5. Special Cases

The formulas for $Q\left(J\right)$ obtained above can be simplified in particular cases. Making the change of variables $t={cos}^2\theta$, one has

$$Q_{{\otimes }_{n}j}\left(J\right)={\displaystyle \frac{2}{\pi }}{\int }_0^1{U}_{2J}\left(\sqrt{t}\right){\left[U_{2j}\left(\sqrt{t}\right)\right]}^n\sqrt{{\displaystyle \frac{1-t}{t}}}dt,$$

which enables one to express $Q_{{\otimes }_{n}j}\left(J\right)$ in terms of roots of Chebyshev polynomials [62]. For a half-integer j,

$$U_{2j}(cos\theta )=4^j\sqrt{t}\prod _{l=1}^{j-1/2}\left[t-r_l\left(j\right)\right]$$

with

$$r_q\left(p\right)={cos}^2\left({\displaystyle \frac{q\pi }{2p+1}}\right).$$

Similarly, for a half-integer J (corresponding to an odd number n of j shells),

$$U_{2J}(cos\theta )=4^J\sqrt{t}\prod _{l=1}^{J-1/2}\left[t-r_l\left(J\right)\right],$$

and for integer J (corresponding to an even number n of j shells):

$$U_{2J}(cos\theta )=4^J\prod _{l=1}^J\left[t-r_l\left(J\right)\right].$$

Therefore, one has, in the case of an even number of fermions $n=2m$,

$$Q_{{\otimes }_{n}j}\left(J\right)={\displaystyle \frac{2}{\pi }}{4}^{J+2mj}{\int }_0^1\left\{\prod _{k=1}^J\left[t-r_k\left(J\right)\right]\right\}{\left\{\prod _{l=1}^{j-1/2}\left[t-r_l\left(j\right)\right]\right\}}^{2m}{t}^m\sqrt{{\displaystyle \frac{1-t}{t}}}dt,$$

with the usual convention that the empty product is 1. It is possible, using these formulas, to obtain analytical expressions of $Q\left(J\right)$ for $j=1/2$ and $j=3/2$, $n=2m$, and where m is an integer. For $j=1/2$, the distribution of J values reads

$$Q_{{\otimes }_{2m}{\displaystyle \frac{1}{2}}}\left(J\right)={\displaystyle \frac{2}{\pi }}{4}^{J+m}{\int }_0^1\left\{\prod _{k=1}^J\left[t-{cos}^2\left({\displaystyle \frac{k\pi }{2J+1}}\right)\right]\right\}{t}^m\sqrt{{\displaystyle \frac{1-t}{t}}}dt,$$

which is equal to (see Equation (16))

$$Q_{{\otimes }_{2m}{\displaystyle \frac{1}{2}}}\left(J\right)=(2J+1){\displaystyle \frac{\left(2m\right)!}{(m-J)!(m+J+1)!}}.$$

In the case $j=3/2$, one has

$$Q_{{\otimes }_{2m}{\displaystyle \frac{3}{2}}}\left(J\right)={\displaystyle \frac{2}{\pi }}{4}^{3m+1}{\int }_0^1\left\{\prod _{k=1}^J\left[t-{cos}^2\left({\displaystyle \frac{k\pi }{2J+1}}\right)\right]\right\}{\left[t-{\displaystyle \frac{1}{2}}\right]}^{2m}{t}^m\sqrt{{\displaystyle \frac{1-t}{t}}}dt$$

and specifically for $J=1$,

$$Q_{{\otimes }_{2m}{\displaystyle \frac{3}{2}}}\left(1\right)={\displaystyle \frac{2}{\pi }}{4}^{3m+1}{\int }_0^1\left(t-{\displaystyle \frac{1}{4}}\right){\left(t-{\displaystyle \frac{1}{2}}\right)}^{2m}{t}^m\sqrt{{\displaystyle \frac{1-t}{t}}}dt$$

or also

$$Q_{{\otimes }_{2m}{\displaystyle \frac{3}{2}}}\left(1\right)={\displaystyle \frac{2}{\pi }}{4}^{3m+1}{\int }_0^1\left(t+{\displaystyle \frac{1}{2}}\right){\left(t-{\displaystyle \frac{1}{2}}\right)}^{2m+1}{t}^m\sqrt{{\displaystyle \frac{1-t}{t}}}dt$$

which turns out to be equal to, using a computer algebra system,

$$\begin{array}{cc}\hfill Q_{{\otimes }_{2m}{\displaystyle \frac{3}{2}}}\left(1\right)& ={\displaystyle \frac{2^{3+6m}}{\pi }}\left\{-{\displaystyle \frac{{(-1)}^{2m}{2}^{-5/2-3m}\Gamma (1/2+m)\Gamma (1+2m)}{3\Gamma (3/2+3m)}}\right.\hfill \\ \hfill & \times \left[3 {}_{2}F_1\left(\begin{array}{c}-1/2,1/2+m\\ 3/2+3m\end{array};1\right)-2 {}_{2}F_1\left(\begin{array}{c}-1/2,3/2+m\\ 5/2+3m\end{array};1/2\right)\right]\hfill \\ \hfill & +{\displaystyle \frac{2^{-5/2-3m}\Gamma (-3/2-3m)\Gamma (1+2m)}{\Gamma (-1/2-m)}}\hfill \\ \hfill & \times \left[-3 {}_{2}F_1\left(\begin{array}{c}-1/2,1/2+m\\ 3/2+3m\end{array};1/2\right)+2 {}_{2}F_1\left(\begin{array}{c}-1/2,3/2+m\\ 5/2+3m\end{array};1/2\right)\right]\hfill \\ \hfill & +{\displaystyle \frac{\sqrt{\pi }\Gamma (1/2+3m)}{8\Gamma (3+3m)}}\left[2(1+6m) {}_{2}F_1\left(\begin{array}{c}-2-3m,-2m\\ -1/2-3m\end{array};1/2\right)\right.\hfill \\ \hfill & \left.\left.-(2+3m) {}_{2}F_1\left(\begin{array}{c}-1-3m,-2m\\ 1/2-3m\end{array};1/2\right)\right]\right\}.\hfill \end{array}$$

As an example, for $m=3$, we obtain

$$Q_{{\otimes }_6{\displaystyle \frac{3}{2}}}\left(1\right)=90$$

(21)

For higher values of J, the Computer Algebra System Mathematica [63] can give expressions involving hypergeometric functions ${}_{2}F_1$, but the latter become more and more cumbersome as J increases.

6. Sum Rules

The total number of states yields the following trivial sum rule:

$$\sum _{J=0}^{nj}(2J+1)Q_{{\otimes }_{n}j}\left(J\right)={(2j+1)}^n.$$

It is instructive to study the so-called odd–even staggering [27], i.e., the difference between the number of odd values of J minus the number of even values of J. Such a study can be easily performed using the sum rule

$$\sum _{J=0}^{nj}\mathcal{F}(2J+1,x)Q_{{\otimes }_{n}j}\left(J\right)={\left[\mathcal{F}(2j+1,x)\right]}^n,$$

where

$$\mathcal{F}(k,x)=x^{-{\displaystyle \frac{(k-1)}{2}}}+x^{-{\displaystyle \frac{(k-3)}{2}}}+\cdots +x^{{\displaystyle \frac{(k-3)}{2}}}+x^{{\displaystyle \frac{(k-1)}{2}}}={\displaystyle \frac{x^{k/2}-x^{-k/2}}{x^{1/2}-x^{-1/2}}}$$

(22)

yielding

$$\sum _{J=0}^{nj}{(-1)}^J{Q}_{{\otimes }_{n}j}\left(J\right)={(-1)}^{nj}$$

if it is an integer, and 0 otherwise. Polychronakos and Sfetsos derived the following sum [64]:

$$\sum _{J=0}^{nj}J(J+1)(2J+1)Q_{{\otimes }_{n}j}\left(J\right)=j(j+1)n{(2j+1)}^n.$$

Other sum rules can be obtained by differentiating Relation (22) with respect to x and taking the limit $x\to 1$. The odd derivative of the order $k+1$ gives the same result as the even derivative of order k. The first sum rules are

$$\sum _{J=0}^{nj}J(J+1)(2J+1)(J^2+J+18)Q_{{\otimes }_{n}j}\left(J\right)=j(j+1)(j^2+j+18)n{(2j+1)}^n,$$

$$\begin{array}{cc}\hfill & \sum _{J=0}^{nj}J(J+1)(2J+1)\left[600+J(J+1)(118+J(J+1\left)\right)\right]Q_{{\otimes }_{n}j}\left(J\right)\hfill \\ \hfill & =j(j+1)\left[600+j(j+1)(118+j(j+1\left)\right)\right]n{(2j+1)}^n\hfill \end{array}$$

and

$$\begin{array}{cc}\hfill & \sum _{J=0}^{nj}J(J+1)(2J+1)\left\{35280+J(J+1)\left[11772+J(J+1)(412+J(J+1\left)\right)\right]\right\}{Q}_{{\otimes }_{n}j}\left(J\right)\hfill \\ \hfill & =j(j+1)\left\{35280+j(j+1)\left[11772+j(j+1)(412+j(j+1\left)\right)\right]\right\}n{(2j+1)}^n.\hfill \end{array}$$

In addition, knowing the distribution $P\left(M\right)$ and evaluating it at the minimum value of M gives the total number of J levels (see Appendix C).

7. Conclusions

We have presented analytical formulas for the number of levels in the case of an arbitrary number of non-equivalent fermions in single-j orbits. We obtained expressions in terms of Gaussian polynomials, symmetric functions, and cyclotomic polynomials. From integral representations, derived within the framework of group theory and using irreducible representations of $SO\left(3\right)$, we deduced exact expressions of $P\left(M\right)$ and $Q\left(J\right)$ involving multinomial coefficients. We also obtained expressions in terms of generalized Pascal triangle coefficients, and presented special cases for $j=3/2$ in terms of hypergeometric functions, as well as sum rules for $Q\left(J\right)$. Formulas for the total number of levels in a configuration were also given, and the possibilities offered by the Molien functions were evoked. The formulas presented here apply to both atomic and nuclear physics. This also illustrates that the two disciplines can benefit greatly from each other.