Theoretical Investigation on Vortex Electron Impact Excitation of a Mg Atom Confined in a Solid-State Environment
Abstract
1. Introduction
2. Theory
2.1. Scattering Amplitude
2.2. Excitation Probabilities and Alignment Parameters
2.3. Angular Distribution of Subsequent Decay
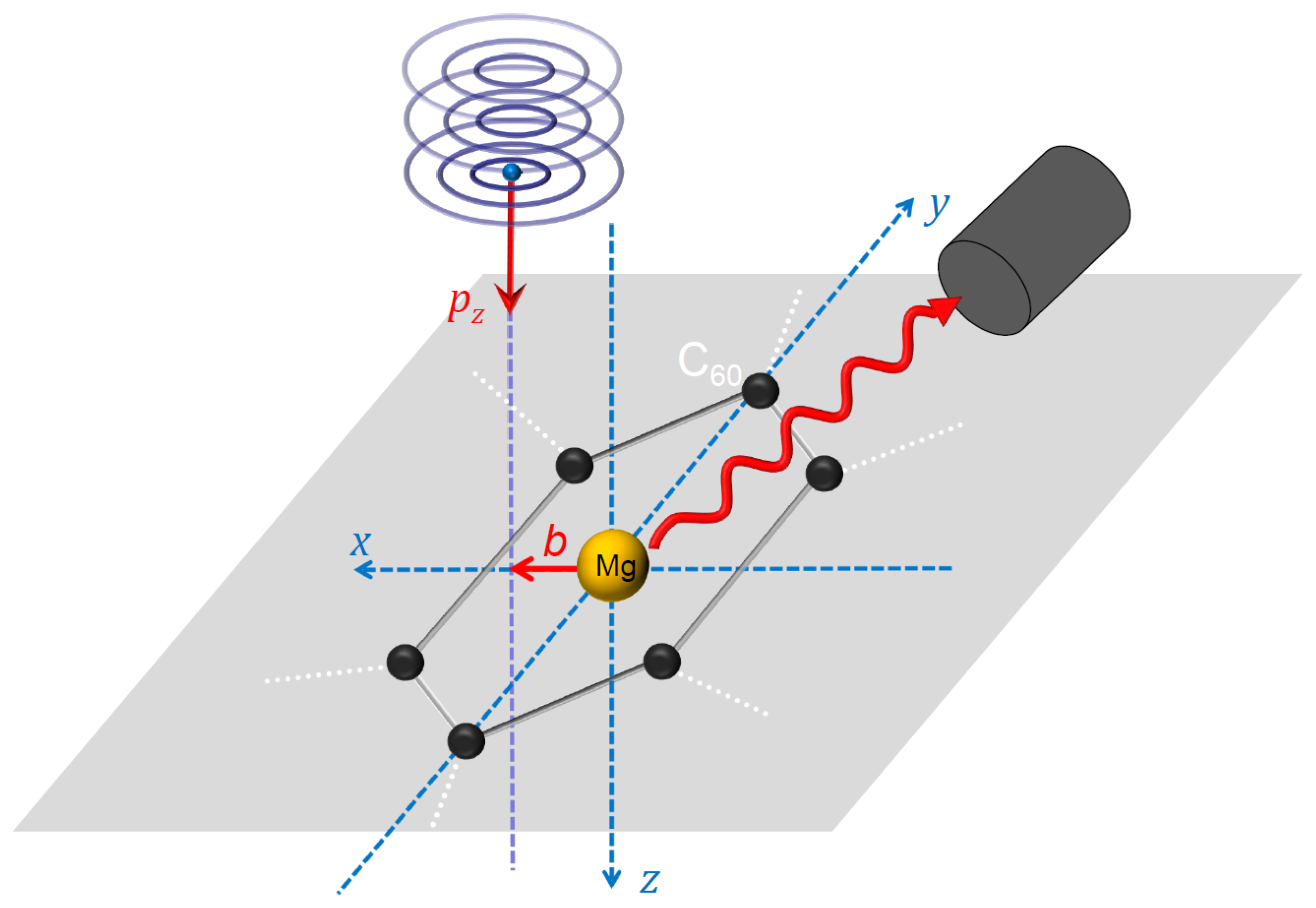
3. Calculation Details
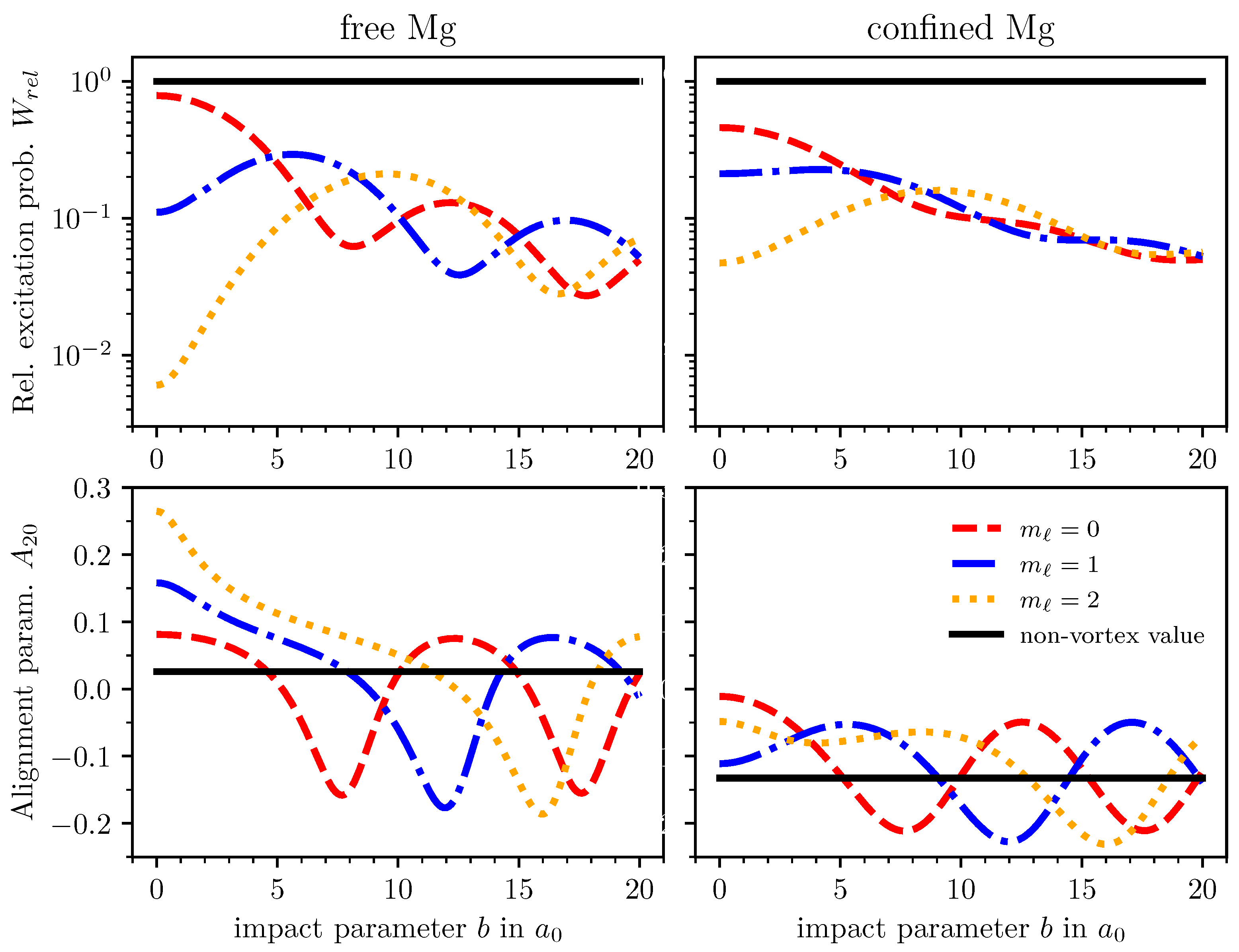
4. Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Uchida, M.; Tonomura, A. Generation of electron beams carrying orbital angular momentum. Nature 2010, 464, 737–739. [Google Scholar] [CrossRef]
- Verbeeck, J.; Tian, H.; Schattschneider, P. Production and application of electron vortex beams. Nature 2010, 467, 301–304. [Google Scholar] [CrossRef] [PubMed]
- Bliokh, K.Y.; Ivanov, I.P.; Guzzinati, G.; Clark, L.; Van Boxem, R.; Béché, A.; Juchtmans, R.; Alonso, M.A.; Schattschneider, P.; Nori, F.; et al. Theory and applications of free-electron vortex states. Phys. Rep. 2017, 690, 1–70. [Google Scholar] [CrossRef]
- Lloyd, S.; Babiker, M.; Thirunavukkarasu, G.; Yuan, J. Electron vortices: Beams with orbital angular momentum. Rev. Mod. Phys. 2017, 89, 035004. [Google Scholar] [CrossRef]
- Larocque, H.; Kaminer, I.; Grillo, V.; Leuchs, G.; Padgett, M.J.; Boyd, R.W.; Segev, M.; Karimi, E. ‘Twisted’ electrons. Contemp. Phys. 2018, 59, 126–144. [Google Scholar] [CrossRef]
- Pavlov, I.; Chaikovskaia, A.; Karlovets, D. Generation of vortex electrons by atomic photoionization. Phys. Rev. A 2024, 110, L031101. [Google Scholar] [CrossRef]
- Bu, Z.; Ji, L.; Geng, X.; Liu, S.; Lei, S.; Shen, B.; Li, R.; Xu, Z. Generation of quantum vortex electrons with intense laser pulses. Adv. Sci. 2024, 11, 2404564. [Google Scholar] [CrossRef]
- Tavabi, A.H.; Larocque, H.; Lu, P.H.; Duchamp, M.; Grillo, V.; Karimi, E.; Dunin-Borkowski, R.E.; Pozzi, G. Generation of electron vortices using nonexact electric fields. Phys. Rev. Res. 2020, 2, 013185. [Google Scholar] [CrossRef]
- Schattschneider, P.; Schachinger, T.; Stöger-Pollach, M.; Löffler, S.; Steiger-Thirsfeld, A.; Bliokh, K.Y.; Nori, F. Imaging the dynamics of free-electron Landau states. Nat. Commun. 2014, 5, 4586. [Google Scholar] [CrossRef] [PubMed]
- Schattschneider, P.; Löffler, S.; Stöger-Pollach, M.; Verbeeck, J. Is magnetic chiral dichroism feasible with electron vortices? Ultramicroscopy 2014, 136, 81–85. [Google Scholar] [CrossRef] [PubMed]
- Pohl, D.; Schneider, S.; Rusz, J.; Rellinghaus, B. Electron vortex beams prepared by a spiral aperture with the goal to measure EMCD on ferromagnetic films via STEM. Ultramicroscopy 2015, 150, 16–22. [Google Scholar] [CrossRef]
- Schachinger, T.; Löffler, S.; Steiger-Thirsfeld, A.; Stöger-Pollach, M.; Schneider, S.; Pohl, D.; Rellinghaus, B.; Schattschneider, P. EMCD with an electron vortex filter: Limitations and possibilities. Ultramicroscopy 2017, 179, 15–23. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Asenjo-Garcia, A.; García de Abajo, F. Dichroism in the interaction between vortex electron beams, plasmons, and molecules. Phys. Rev. Lett. 2014, 113, 066102. [Google Scholar] [CrossRef]
- Verbeeck, J.; Schattschneider, P.; Lazar, S.; Stöger-Pollach, M.; Löffler, S.; Steiger-Thirsfeld, A.; Van Tendeloo, G. Atomic scale electron vortices for nanoresearch. Appl. Phys. Lett. 2011, 99, 203109. [Google Scholar] [CrossRef]
- Rusz, J.; Idrobo, J.C.; Bhowmick, S. Achieving atomic resolution magnetic dichroism by controlling the phase symmetry of an electron probe. Phys. Rev. Lett. 2014, 113, 145501. [Google Scholar] [CrossRef]
- Van Boxem, R.; Partoens, B.; Verbeeck, J. Rutherford scattering of electron vortices. Phys. Rev. A 2014, 89, 032715. [Google Scholar] [CrossRef]
- Juchtmans, R.; Béché, A.; Abakumov, A.; Batuk, M.; Verbeeck, J. Using electron vortex beams to determine chirality of crystals in transmission electron microscopy. Phys. Rev. B 2015, 91, 094112. [Google Scholar] [CrossRef]
- Maiorova, A.; Fritzsche, S.; Müller, R.; Surzhykov, A. Elastic scattering of twisted electrons by diatomic molecules. Phys. Rev. A 2018, 98, 042701. [Google Scholar] [CrossRef]
- Serbo, V.; Ivanov, I.; Fritzsche, S.; Seipt, D.; Surzhykov, A. Scattering of twisted relativistic electrons by atoms. Phys. Rev. A 2015, 92, 012705. [Google Scholar] [CrossRef]
- Karlovets, D.V.; Kotkin, G.; Serbo, V.; Surzhykov, A. Scattering of twisted electron wave packets by atoms in the Born approximation. Phys. Rev. A 2017, 95, 032703. [Google Scholar] [CrossRef]
- Ivanov, I.; Seipt, D.; Surzhykov, A.; Fritzsche, S. Elastic scattering of vortex electrons provides direct access to the Coulomb phase. Phys. Rev. D 2016, 94, 076001. [Google Scholar] [CrossRef]
- Ivanov, V.; Chaikovskaia, A.; Karlovets, D. Studying highly relativistic vortex-electron beams by atomic scattering. Phys. Rev. A 2023, 108, 062803. [Google Scholar] [CrossRef]
- Kosheleva, V.; Zaytsev, V.; Surzhykov, A.; Shabaev, V.; Stöhlker, T. Elastic scattering of twisted electrons by an atomic target: Going beyond the Born approximation. Phys. Rev. A 2018, 98, 022706. [Google Scholar] [CrossRef]
- Ivanov, I.P. Promises and challenges of high-energy vortex states collisions. Prog. Part. Nucl. Phys. 2022, 127, 103987. [Google Scholar] [CrossRef]
- Harris, A.; Fritzsche, S. A distorted-wave approach to the elastic scattering of twisted electrons. arXiv 2024, arXiv:2411.14558. [Google Scholar]
- Harris, A.; Plumadore, A.; Smozhanyk, Z. Ionization of hydrogen by electron vortex beam. J. Phys. B At. Mol. Opt. Phys. 2019, 52, 094001. [Google Scholar] [CrossRef]
- Van Boxem, R.; Partoens, B.; Verbeeck, J. Inelastic electron-vortex-beam scattering. Phys. Rev. A 2015, 91, 032703. [Google Scholar] [CrossRef]
- Strnat, S.; Sommerfeldt, J.; Sahoo, A.K.; Sharma, L.; Surzhykov, A. Inelastic scattering of vortex electrons beyond the Born approximation. arXiv 2024, arXiv:2412.08246. [Google Scholar]
- Dunlap, B.I.; Ballester, J.L.; Schmidt, P.P. Interactions between fullerene (C60) and endohedral alkali atoms. J. Phys. Chem. 1992, 96, 9781–9787. [Google Scholar] [CrossRef]
- Hasoğlu, M.F.; Zhou, H.L.; Manson, S.T. Correlation study of endohedrally confined alkaline-earth-metal atoms (A@C60). Phys. Rev. A 2016, 93, 022512. [Google Scholar] [CrossRef]
- Kilcoyne, A.; Aguilar, A.; Müller, A.; Schippers, S.; Cisneros, C.; Alna’Washi, G.; Aryal, N.; Baral, K.; Esteves, D.; Thomas, C.; et al. Confinement resonances in photoionization of Xe@ C 60+. Phys. Rev. Lett. 2010, 105, 213001. [Google Scholar] [CrossRef]
- Allen, L.J.; D’Alfonso, A.J.; Freitag, B.; Klenov, D.O. Chemical mapping at atomic resolution using energy-dispersive x-ray spectroscopy. MRS Bull. 2012, 37, 47–52. [Google Scholar] [CrossRef]
- Zuo, T.; McEachran, R.; Stauffer, A. Relativistic distorted-wave calculation of electron impact excitation of xenon. J. Phys. B At. Mol. Opt. Phys. 1991, 24, 2853. [Google Scholar] [CrossRef]
- Chauhan, R.K.; Srivastava, R.; Stauffer, A. Electron impact excitation of the 41P1 state of calcium. J. Phys. B At. Mol. Opt. Phys. 2005, 38, 2385. [Google Scholar] [CrossRef]
- Sharma, L. Electron Induced Processes in Atomic Systems. Ph.D. Thesis, IIT Roorkee, Roorkee, India, 2008. [Google Scholar]
- Sharma, L.; Surzhykov, A.; Srivastava, R.; Fritzsche, S. Electron-impact excitation of singly charged metal ions. Phys. Rev. A 2011, 83, 062701. [Google Scholar] [CrossRef]
- Zaytsev, V.A.; Serbo, V.G.; Shabaev, V.M. Radiative recombination of twisted electrons with bare nuclei: Going beyond the Born approximation. Phys. Rev. A 2017, 95, 012702. [Google Scholar] [CrossRef]
- Karlovets, D.; Kotkin, G.; Serbo, V. Scattering of wave packets on atoms in the Born approximation. Phys. Rev. A 2015, 92, 052703. [Google Scholar] [CrossRef]
- Balashov, V.V.; Grum-Grzhimailo, A.N.; Kabachnik, N.M. Polarization and Correlation Phenomena in Atomic Collisions; Kluwer Academic: Dordrecht, The Netherlands, 2000. [Google Scholar]
- Itikawa, Y. Distorted-wave methods in electron-impact excitation of atoms and ions. Phys. Rep. 1986, 143, 69–108. [Google Scholar] [CrossRef]
- Mahato, D.; Sharma, L.; Baral, S.; Saha, S.; Jose, J.; Srivastava, R. Study of electron impact elastic scattering from Kr@ C60 and Xe@ C60 using a fully relativistic approach. J. Phys. B At. Mol. Opt. Phys. 2022, 55, 165201. [Google Scholar] [CrossRef]
- Bharti, S.; Sharma, L.; Sahoo, B.; Malkar, P.; Srivastava, R. Application of relativistic coupled cluster theory to elastic scattering of electrons from confined Ca atoms. J. Phys. B At. Mol. Opt. Phys. 2019, 52, 185003. [Google Scholar] [CrossRef]
- Dubey, K.A.; Jose, J. Effect of charge transfer on elastic scattering of electron from Ar@ C60: Dirac partial wave calculation. Eur. Phys. J. Plus 2021, 136, 713. [Google Scholar] [CrossRef]
- Knyazev, B.A.; Serbo, V. Beams of photons with nonzero projections of orbital angular momenta: New results. Physics-Uspekhi 2018, 61, 449. [Google Scholar] [CrossRef]
- Hayrapetyan, A.G.; Matula, O.; Aiello, A.; Surzhykov, A.; Fritzsche, S. Interaction of relativistic electron-vortex beams with few-cycle laser pulses. Phys. Rev. Lett. 2014, 112, 134801. [Google Scholar] [CrossRef] [PubMed]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Strnat, S.; Sahoo, A.K.; Sharma, L.; Sommerfeldt, J.; Park, D.; Bick, C.; Surzhykov, A. Theoretical Investigation on Vortex Electron Impact Excitation of a Mg Atom Confined in a Solid-State Environment. Atoms 2025, 13, 23. https://doi.org/10.3390/atoms13030023
Strnat S, Sahoo AK, Sharma L, Sommerfeldt J, Park D, Bick C, Surzhykov A. Theoretical Investigation on Vortex Electron Impact Excitation of a Mg Atom Confined in a Solid-State Environment. Atoms. 2025; 13(3):23. https://doi.org/10.3390/atoms13030023
Chicago/Turabian StyleStrnat, Sophia, Aloka K. Sahoo, Lalita Sharma, Jonas Sommerfeldt, Daesung Park, Christian Bick, and Andrey Surzhykov. 2025. "Theoretical Investigation on Vortex Electron Impact Excitation of a Mg Atom Confined in a Solid-State Environment" Atoms 13, no. 3: 23. https://doi.org/10.3390/atoms13030023
APA StyleStrnat, S., Sahoo, A. K., Sharma, L., Sommerfeldt, J., Park, D., Bick, C., & Surzhykov, A. (2025). Theoretical Investigation on Vortex Electron Impact Excitation of a Mg Atom Confined in a Solid-State Environment. Atoms, 13(3), 23. https://doi.org/10.3390/atoms13030023






