Natural Orbitals and Targeted Non-Orthogonal Orbital Sets for Atomic Hyperfine Structure Multiconfiguration Calculations †
Abstract
1. Introduction
2. Theory
2.1. The MCDHF and RCI Methods
2.2. Hyperfine Structure
2.3. Transformation to Natural Orbitals
3. Selection of CSF Expansions and Generation of Orbital Sets
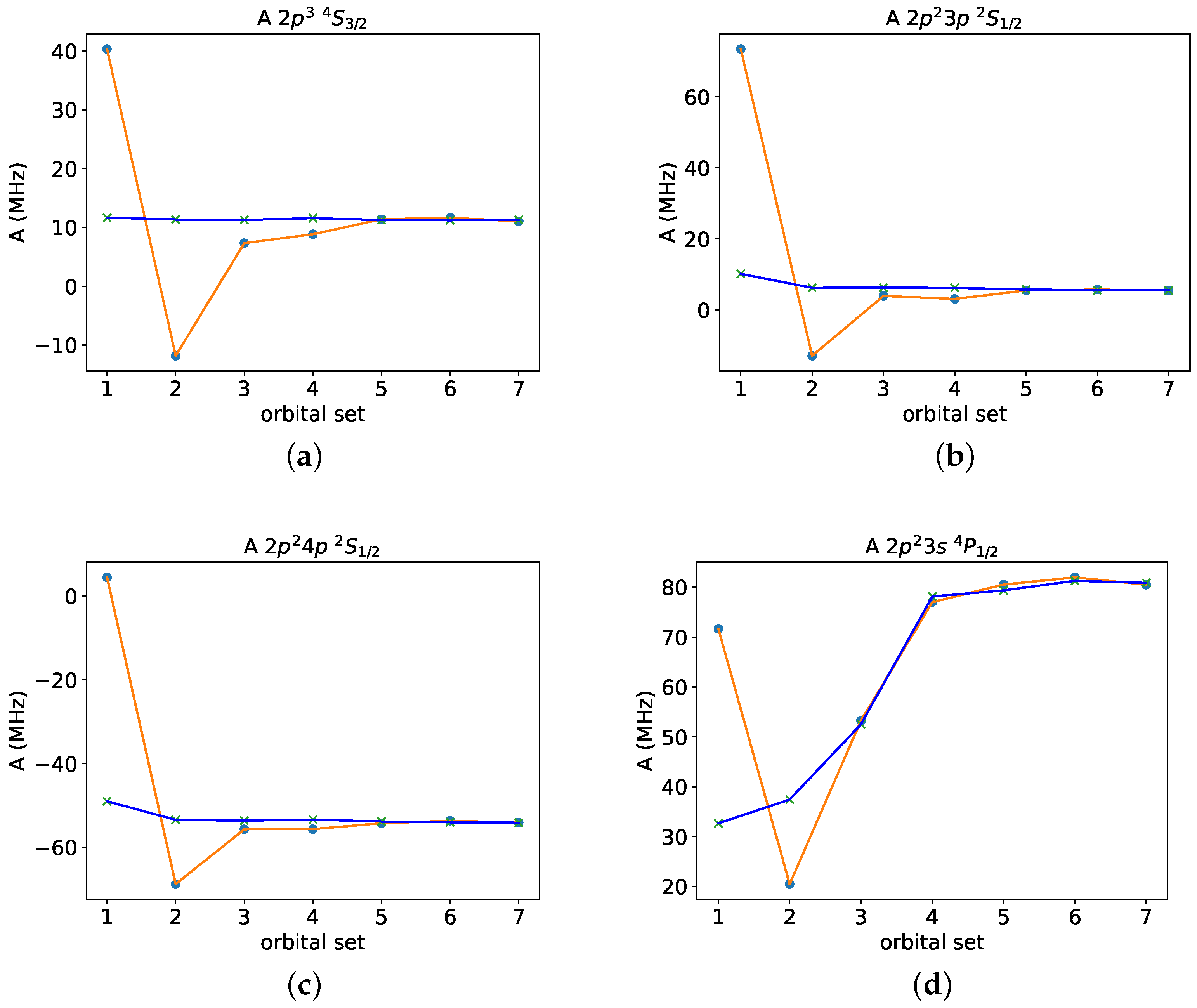
4. Calculations of Hyperfine Interaction Constants in Different Orbital Sets
4.1. Hyperfine Interaction Constants from Energy-Driven Calculations
4.2. Polarization Orbitals Merged with the Orbitals from Energy-Driven Calculations
4.3. Higher-Order Correlation Effects and Transformation to Natural Orbitals
5. Conclusions and Outlook
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kurucz, R. Atomic data for interpreting stellar spectra: Isotopic and hyperfine data. Phys. Scr. 1993, T47, 110. [Google Scholar] [CrossRef]
- Leckrone, D.S.; Johansson, S.; Kalus, G.; Wahlgren, G.M.; Brage, T.; Proffitt, C.R. Abundance and Isotopic Anomalies of Thallium in the Atmosphere of the HgMn Star χ LUPI. Astrophys. J. 1996, 462, 937. [Google Scholar] [CrossRef]
- Brage, T.; Judge, P.G.; Proffitt, C.R. Determination of Hyperfine-Induced Transition Rates from Observations of a Planetary Nebula. Phys. Rev. Lett. 2002, 89, 281101. [Google Scholar] [CrossRef] [PubMed]
- Mårtensson-Pendrill, A.-M.; Gustavsson, M. The Atomic Nucleus. In Handbook of Molecular Physics and Quantum Chemistry; Willey: Hoboken, NJ, USA, 2003; Volume 1, pp. 477–484. ISBN 0 471 62374 1. [Google Scholar]
- Yordanov, D.T.; Balabanski, D.L.; Bieroń, J.; Bissell, M.L.; Blaum, K.; Budinčević, I.; Fritzsche, S.; Frömmgen, N.; Georgiev, G.; Geppert, C.; et al. Spins, Moments, and Isomers of 107–129Cd. Phys. Rev. Lett. 2013, 110, 192501. [Google Scholar] [CrossRef] [PubMed]
- Cubiss, J.; Barzakh, A.; Seliverstov, M.; Andreyev, A.; Andel, B.; Antalic, S.; Ascher, P.; Atanasov, D.; Beck, D.; Bieroń, J.; et al. Charge radii and electromagnetic moments of 195–211At. Phys. Rev. C 2018, 97, 054327. [Google Scholar] [CrossRef]
- Li, F.C.; Tang, Y.B.; Qiao, H.X.; Shi, T.Y. Ab initio calculations of the hyperfine structure of Ra+ and evaluations of the electric quadrupole moment Q of the 209,211,221,223Ra nuclei. J. Phys. At. Mol. Opt. Phys. 2021, 54, 145004. [Google Scholar] [CrossRef]
- de Groote, R.; Moreno, J.; Dobaczewski, J.; Koszorús, Á.; Moore, I.; Reponen, M.; Sahoo, B.; Yuan, C. Precision measurement of the magnetic octupole moment in 45Sc as a test for state-of-the-art atomic- and nuclear-structure theory. Phys. Lett. B 2022, 827, 136930. [Google Scholar] [CrossRef]
- Zhang, T.X.; Zhang, Y.H.; Li, C.B.; Shi, T.Y. Theoretical study of the hyperfine interaction constants, Landé’ g-factors, and electric quadrupole moments for the low-lying states of the 61Niq+ (q = 11, 12, 14, and 15) ions. Chin. Phys. B 2021, 30, 013101. [Google Scholar] [CrossRef]
- Kimura, N.; Priti; Kono, Y.; Pipatpakorn, P.; Soutome, K.; Numadate, N.; Kuma, S.; Azuma, T.; Nakamura, N. Hyperfine-structure-resolved laser spectroscopy of many-electron highly charged ions. Commun. Phys. 2023, 6, 8. [Google Scholar] [CrossRef]
- Sobolewski, Ł.M.; Rathi, S.; Sharma, L.; Windholz, L.; Kwela, J. Laser optogalvanic spectroscopy of lead lines—Isotope shifts and hyperfine structure studies. J. Quant. Spectrosc. Radiat. Transf. 2024, 316, 108901. [Google Scholar] [CrossRef]
- Bieroń, J.; Froese Fischer, C.; Fritzsche, S.; Gaigalas, G.; Grant, I.P.; Indelicato, P.; Jönsson, P.; Pyykkö, P. Ab initio MCDHF calculations of electron-nucleus interactions. Phys. Scr. 2015, 90, 054011. [Google Scholar] [CrossRef]
- Verdebout, S.; Rynkun, P.; Jönsson, P.; Gaigalas, G.; Froese Fischer, C.; Godefroid, M. A partitioned correlation function interaction approach for describing electron correlation in atoms. J. Phys. B At. Mol. Opt. Phys. 2013, 46, 085003. [Google Scholar] [CrossRef]
- Li, Y.; Jönsson, P.; Godefroid, M.; Gaigalas, G.; Bieroń, J.; Marques, J.P.; Indelicato, P.; Chen, C. Independently Optimized Orbital Sets in GRASP—The Case of Hyperfine Structure in Li I. Atoms 2023, 11, 4. [Google Scholar] [CrossRef]
- Godefroid, M.R.; Van Meulebeke, G.; Jönsson, P.; Froese Fischer, C. Large-scale MCHF calculations of hyperfine structures in nitrogen and oxygen. Z. Phys. D—Atoms Mol. Clust. 1997, 42, 193–201. [Google Scholar] [CrossRef]
- Jennerich, R.; Keiser, A.; Tate, D. Hyperfine structure and isotope shifts in near-infrared transitions of atomic nitrogen. Eur. Phys. J. D 2006, 40, 81–89. [Google Scholar] [CrossRef]
- Carette, T.; Nemouchi, M.; Jönsson, P.; Godefroid, M. Saturation spectra of low lying states of Nitrogen: Reconciling experiment with theory. Eur. Phys. J. D At. Mol. Opt. Plasma Phys. 2010, 60, 231–242. [Google Scholar] [CrossRef]
- Jönsson, P.; Carette, T.; Nemouchi, M.; Godefroid, M. Ab initio calculations of 14N and 15N hyperfine structures. J. Phys. B At. Mol. Opt. Phys. 2010, 43, 115006. [Google Scholar] [CrossRef]
- Ahrendsen, K.J.; Maruko, C.; Albert-Aranovich, K.R.; Berfield-Brewer, Q.; Esseln, A.; Guo, L.; Ishimwe, A.E.; Kuzniar, Y.; McKenna, A.E.; Villarreal, K.J.S.; et al. Absolute frequency measurement of the 2p2(3P)3s2P − 2p2(3P)3p2Do transitions in neutral 14N. Phys. Rev. A 2023, 108, 042815. [Google Scholar] [CrossRef]
- Jönsson, P.; Godefroid, M.; Gaigalas, G.; Ekman, J.; Grumer, J.; Li, W.; Li, J.; Brage, T.; Grant, I.P.; Bieroń, J.; et al. GRASP Manual for Users. Atoms 2023, 11, 68. [Google Scholar] [CrossRef]
- Schiffmann, S.; Godefroid, M.; Ekman, J.; Jönsson, P.; Froese Fischer, C. Natural orbitals in multiconfiguration calculations of hyperfine-structure parameters. Phys. Rev. A 2020, 101, 062510. [Google Scholar] [CrossRef]
- Li, Y.T.; Wang, K.; Si, R.; Godefroid, M.; Gaigalas, G.; Chen, C.Y.; Jönsson, P. Reducing the computational load—Atomic multiconfiguration calculations based on configuration state function generators. Comput. Phys. Commun. 2023, 283, 108562. [Google Scholar] [CrossRef]
- Froese Fischer, C.; Godefroid, M.; Brage, T.; Jönsson, P.; Gaigalas, G. Advanced multiconfiguration methods for complex atoms: I. Energies and wave functions. J. Phys. B At. Mol. Opt. Phys. 2016, 49, 182004. [Google Scholar] [CrossRef]
- Grant, I. Relativistic Quantum Theory of Atoms and Molecules: Theory and Computation; Springer Science and Business Media, LLC: New York, NY, USA, 2007. [Google Scholar]
- Jönsson, P.; Godefroid, M.; Gaigalas, G.; Ekman, J.; Grumer, J.; Li, W.; Li, J.; Brage, T.; Grant, I.P.; Bieroń, J.; et al. An Introduction to Relativistic Theory as Implemented in GRASP. Atoms 2023, 11, 7. [Google Scholar] [CrossRef]
- Gaigalas, G. A Program Library for Computing Pure Spin–Angular Coefficients for One- and Two-Particle Operators in Relativistic Atomic Theory. Atoms 2022, 10, 129. [Google Scholar] [CrossRef]
- Schwartz, C. Theory of hyperfine structure. Phys. Rev. 1955, 97, 380. [Google Scholar] [CrossRef]
- Lindgren, I.; Rosén, A. Relativistic self-consistent-field calculations with application to atomic hyperfine interaction. Case Stud. At. Phys. 1974, 3, 93–196. [Google Scholar]
- Stone, N. Table of nuclear magnetic dipole and electric quadrupole moments. At. Data Nucl. Data Tables 2005, 90, 75–176. [Google Scholar] [CrossRef]
- Löwdin, P.O. Quantum Theory of Many-Particle Systems. I. Physical Interpretations by Means of Density Matrices, Natural Spin-Orbitals, and Convergence Problems in the Method of Configurational Interaction. Phys. Rev. 1955, 97, 1474–1489. [Google Scholar] [CrossRef]
- Bytautas, L.; Ivanic, J.; Ruedenberg, K. Split-localized orbitals can yield stronger configuration interaction convergence than natural orbitals. J. Chem. Phys. 2003, 119, 1474–1489. [Google Scholar] [CrossRef]
- Schiffmann, S.; Li, J.; Ekman, J.; Gaigalas, G.; Godefroid, M.; Jönsson, P.; Bieroń, J. Relativistic radial electron density functions and natural orbitals from GRASP2018. Comput. Phys. Commun. 2022, 278, 108403. [Google Scholar] [CrossRef]
- Layzer, D. On a screening theory of atomic spectra. Ann. Phys. 1959, 8, 271. [Google Scholar] [CrossRef]
- Lindgren, I. Effective operators in the atomic hyperfine interaction. Rep. Prog. Phys. 1984, 47, 345. [Google Scholar] [CrossRef]
- Engels, B.; Peyerimhoff, S.D.; Davidson, E.R. Calculation of hyperfine coupling constants. Mol. Phys. 1987, 62, 109–127. [Google Scholar] [CrossRef]
- Froese Fischer, C.; Gaigalas, G.; Jönsson, P.; Bieroń, J. GRASP2018—A Fortran 95 version of the general relativistic atomic structure package. Comput. Phys. Commun. 2019, 237, 184–187. [Google Scholar] [CrossRef]
- Graspg—An extension to Grasp2018 based on Configuration State Function Generators. Comput. Phys. Commun. manuscript in preparation.
- Dyall, K.; Grant, I.; Johnson, C.; Parpia, F.; Plummer, E. GRASP: A general-purpose relativistic atomic structure program. Comput. Phys. Commun. 1989, 55, 425–456. [Google Scholar] [CrossRef]
- Sundholm, D.; Olsen, J. Large multiconfigurational Hartree-Fock calculations on the hyperfine structure of Li(2S) and Li(2P). Phys. Rev. A 1990, 42, 2614–2621. [Google Scholar] [CrossRef]
- Sundholm, D.; Olsen, J. “Atomic” determination of the 23Na, 25Mg, and 27Al nuclear quadrupole moments: How accurate are the “muonic” values? Phys. Rev. Lett. 1992, 68, 927–930. [Google Scholar] [CrossRef]
- Hirsch, J.M.; Zimmerman, G.H.; Larson, D.J.; Ramsey, N.F. Precision measurement of the hyperfine structure and gJ factor of atomic nitrogen 14. Phys. Rev. A 1977, 16, 484–487. [Google Scholar] [CrossRef]

| Label | Orbitals |
|---|---|
| set 1 | |
| set 2 | |
| set 3 | |
| set 4 | |
| set 5 | |
| set 6 | |
| set 7 |
| Label | Orbitals |
|---|---|
| set 1 | |
| set 2 | |
| set 3 | |
| set 4 | |
| set 5 | |
| set 6 | |
| set 7 |
| spectroscopic | odd states | even states | ||||
| orbitals | ||||||
| 0.228 | – | – | 0.228 | – | – | |
| 1.262 | 1.273 | – | 1.251 | 1.278 | – | |
| – | 6.818 | – | 6.952 | – | 10.39 | |
| – | 14.88 | – | 11.15 | – | – | |
| correlation orbital set | ||||||
| set 1 | 1.769 | 2.373 | 1.427 | 2.061 | 1.912 | 1.452 |
| set 2 | 0.994 | 1.024 | 1.040 | 0.955 | 0.950 | 1.053 |
| set 3 | 1.711 | 1.403 | 1.330 | 1.359 | 1.406 | 1.387 |
| set 4 | 3.501 | 2.706 | 3.868 | 2.368 | 3.442 | 1.777 |
| set 5 | 0.969 | 0.970 | 0.986 | 0.931 | 0.823 | 3.636 |
| set 6 | 4.223 | 6.623 | 0.927 | 7.193 | 1.158 | 0.708 |
| set 7 | 0.711 | 0.715 | 3.705 | 0.783 | 6.121 | 0.733 |
| polarization orbitals | ||||||
| 0.999 | – | 0.778 | 1.105 | – | 0.785 | |
| 3.842 | – | 0.818 | 0.804 | – | 0.816 | |
| polarization orbitals | ||||||
| 2.394 | – | 1.512 | 2.911 | – | 1.536 | |
| 4.421 | – | 4.287 | 3.230 | – | 1.182 | |
| State | J P | Set 1 | Set 2 | Set 3 | Set 4 | Set 5 | Set 6 | Set 7 | Exp. | MCHF |
|---|---|---|---|---|---|---|---|---|---|---|
| 3/2 - | 40.35 | −11.84 | 7.331 | 8.838 | 11.40 | 11.65 | 11.04 | 10.4509 | ||
| 5/2 - | 117.2 | 112.7 | 114.5 | 116.1 | 115.9 | 115.9 | 115.9 | |||
| 3/2 - | 59.29 | 72.17 | 67.10 | 67.41 | 66.13 | 66.19 | 66.17 | |||
| 1/2 - | 294.3 | 332.2 | 320.5 | 324.0 | 321.8 | 321.1 | 321.8 | |||
| 3/2 - | 73.36 | 58.09 | 63.10 | 64.12 | 64.46 | 64.65 | 64.43 | |||
| 1/2 - | 73.47 | −12.92 | 3.931 | 3.105 | 5.489 | 5.730 | 5.484 | |||
| 1/2 - | 42.31 | 85.03 | 76.99 | 76.37 | 75.38 | 75.01 | 75.09 | 69.76(90) | 74.15 | |
| 3/2 - | 37.96 | 28.93 | 30.69 | 31.02 | 31.16 | 31.38 | 31.26 | 30.3(15) | 31.71 | |
| 5/2 - | 48.19 | 31.94 | 35.15 | 35.60 | 35.94 | 36.22 | 36.07 | 36.6(11) | 36.76 | |
| 7/2 - | 68.28 | 50.09 | 53.88 | 54.23 | 54.72 | 54.96 | 54.84 | 55.2(11) | 55.63 | |
| 1/2 - | 1.375 | −72.31 | −58.48 | −56.97 | −55.58 | −54.54 | −54.91 | −50.78(17) | −52.25 | |
| 3/2 - | 70.89 | 39.73 | 45.91 | 46.35 | 47.14 | 47.41 | 47.29 | 46.2(15) | 51.04 | |
| 5/2 - | 51.41 | 25.31 | 30.48 | 30.95 | 31.54 | 31.90 | 31.76 | 31.93(86) | 33.16 | |
| 3/2 - | 43.68 | 23.31 | 9.971 | 11.23 | 12.69 | 13.18 | 13.07 | |||
| 3/2 - | 58.23 | 51.77 | 72.10 | 72.85 | 72.41 | 71.63 | 71.62 | |||
| 5/2 - | 82.04 | 66.07 | 69.31 | 69.26 | 69.69 | 69.89 | 69.80 | |||
| 1/2 - | 122.7 | 151.1 | 145.5 | 145.9 | 145.4 | 146.2 | 146.3 | |||
| 3/2 - | 74.44 | 47.74 | 53.10 | 53.20 | 53.82 | 53.97 | 53.85 | |||
| 1/2 - | 72.28 | −16.06 | −0.08142 | −1.692 | 0.2150 | 0.3925 | −0.1555 | |||
| 1/2 - | 31.76 | 74.09 | 66.03 | 65.53 | 64.49 | 64.11 | 64.24 | |||
| 3/2 - | 28.99 | 20.20 | 21.64 | 21.36 | 21.47 | 21.56 | 21.45 | |||
| 5/2 - | 39.05 | 22.71 | 25.59 | 25.26 | 25.56 | 25.68 | 25.53 | |||
| 7/2 - | 67.53 | 49.21 | 52.90 | 52.80 | 53.31 | 53.45 | 53.34 | |||
| 1/2 - | 4.462 | −68.78 | −55.68 | −55.64 | −54.24 | −53.66 | −54.07 | |||
| 3/2 - | 73.67 | 42.53 | 48.15 | 46.96 | 47.72 | 47.34 | 47.20 | |||
| 5/2 - | 57.41 | 31.38 | 36.42 | 36.50 | 37.14 | 37.35 | 37.22 | |||
| 3/2 - | 55.21 | 72.13 | 69.78 | 68.92 | 68.67 | 67.47 | 67.55 | |||
| 5/2 - | 83.69 | 68.58 | 72.20 | 72.37 | 72.90 | 73.00 | 72.92 | |||
| 3/2 - | 45.89 | 1.977 | 11.55 | 13.77 | 15.04 | 16.13 | 15.90 | |||
| 1/2 - | 138.5 | 171.0 | 168.5 | 171.0 | 171.1 | 171.8 | 172.1 | |||
| 3/2 - | 77.82 | 53.14 | 58.39 | 57.88 | 58.51 | 57.91 | 57.78 |
| State | J P | Set 1 | Set 2 | Set 3 | Set 4 | Set 5 | Set 6 | Set 7 | Exp. | MCHF |
|---|---|---|---|---|---|---|---|---|---|---|
| 1/2 + | 71.65 | 20.48 | 53.27 | 76.00 | 80.54 | 81.98 | 80.51 | 112.3(13) | 100.21 | |
| 3/2 + | 52.23 | 28.82 | 43.04 | 52.98 | 54.92 | 55.59 | 54.91 | 68.33(69) | 62.46 | |
| 5/2 + | 116.9 | 99.51 | 110.9 | 118.8 | 120.2 | 120.7 | 120.2 | 129.52(84) | 124.84 | |
| 1/2 + | 47.24 | 71.56 | 64.82 | 65.16 | 63.85 | 64.07 | 64.28 | |||
| 3/2 + | 124.0 | 97.45 | 104.9 | 104.1 | 105.4 | 105.3 | 10.50 | |||
| 5/2 + | 344.1 | 361.3 | 360.3 | 354.9 | 350.8 | 350.3 | 350.2 | |||
| 3/2 + | 355.5 | 368.7 | 371.0 | 365.0 | 361.4 | 360.5 | 360.7 | |||
| 1/2 + | 782.0 | 810.6 | 818.8 | 808.4 | 801.5 | 799.4 | 800.0 | |||
| 5/2 + | 130.5 | 134.3 | 135.1 | 135.2 | 135.5 | 135.8 | 135.7 | |||
| 3/2 + | 146.4 | 150.5 | 148.6 | 148.6 | 148.0 | 147.9 | 147.9 | |||
| 1/2 + | 19.61 | −47.67 | −32.29 | −37.49 | −34.22 | −34.08 | −35.06 | |||
| 3/2 + | 25.31 | −4.749 | 2.476 | 0.4206 | 1.824 | 1.931 | 1.488 | |||
| 5/2 + | 94.72 | 72.02 | 79.28 | 78.34 | 79.43 | 79.47 | 79.07 | |||
| 1/2 + | 31.53 | 54.29 | 49.66 | 51.58 | 49.49 | 49.78 | 49.80 | |||
| 3/2 + | 117.5 | 92.85 | 103.9 | 105.0 | 104.7 | 104.4 | 103.3 | |||
| 3/2 + | −1.712 | −32.21 | −30.62 | −35.77 | −31.49 | −31.78 | −31.90 | |||
| 1/2 + | −115.4 | −93.35 | −102.0 | −102.5 | −102.8 | −102.6 | −101.9 | |||
| 3/2 + | 14.85 | 38.14 | 34.50 | 37.09 | 34.50 | 35.32 | 35.93 | |||
| 5/2 + | 21.56 | 24.68 | 24.19 | 24.39 | 24.63 | 24.61 | 24.59 | |||
| 7/2 + | 30.59 | 23.23 | 25.44 | 25.06 | 25.97 | 25.85 | 25.66 | |||
| 5/2 + | 31.71 | 38.24 | 35.44 | 9.579 | 28.50 | 27.93 | 22.38 | |||
| 5/2 + | 7.241 | −24.46 | −21.45 | 0.8877 | −17.02 | −16.59 | −11.41 | |||
| 3/2 + | −5.061 | −47.84 | −43.43 | −42.84 | −41.73 | −42.03 | −42.24 | |||
| 1/2 + | −16.48 | −88.26 | −35.85 | −6.957 | −5.629 | −6.556 | −5.808 | |||
| 7/2 + | 60.53 | 46.81 | 50.66 | 49.96 | 50.57 | 50.51 | 50.27 | |||
| 1/2 + | 222.2 | 266.3 | 217.2 | 184.4 | 184.0 | 184.7 | 183.4 | |||
| 3/2 + | 75.66 | 79.77 | 81.81 | 77.55 | 78.02 | 78.07 | 77.69 | |||
| 5/2 + | 47.82 | 36.13 | 40.88 | 40.11 | 40.50 | 40.46 | 40.25 | |||
| 7/2 + | 28.54 | 11.15 | 15.51 | 14.71 | 15.34 | 15.24 | 15.06 | |||
| 3/2 + | 49.17 | 64.39 | 60.97 | 61.75 | 61.00 | 61.13 | 61.40 | |||
| 5/2 + | 31.68 | 14.83 | 19.38 | 18.74 | 19.74 | 19.60 | 19.42 |
| Expansion | Odd States | Even States |
|---|---|---|
| set 7 | 10,883,618 | 9,333,523 |
| set 7 ∪ pol. sets | 10,901,511 | 9,347,089 |
| State | J P | Set 1 | Set 2 | Set 3 | Set 4 | Set 5 | Set 6 | Set 7 | TQ | TQ + NO | Exp. | MCHF |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 3/2 - | 11.66 | 11.35 | 11.27 | 11.58 | 11.26 | 11.22 | 11.29 | 10.49 | 10.77 | 10.4509 | 10.395 | |
| 5/2 - | 111.8 | 117.4 | 115.3 | 116.7 | 115.9 | 115.9 | 115.9 | 115.2 | 115.3 | |||
| 3/2 - | 63.38 | 67.57 | 66.08 | 66.75 | 66.26 | 66.14 | 66.13 | 65.97 | 66.00 | |||
| 1/2 - | 309.1 | 325.5 | 320.0 | 323.4 | 321.5 | 321.6 | 321.7 | 320.5 | 320.7 | |||
| 3/2 - | 62.36 | 65.08 | 64.10 | 64.95 | 64.47 | 64.48 | 64.50 | 63.67 | 63.79 | |||
| 1/2 - | 10.17 | 6.227 | 6.269 | 6.212 | 5.751 | 5.575 | 5.486 | 7.147 | 6.663 | |||
| 1/2 - | 72.06 | 74.51 | 74.87 | 74.58 | 74.99 | 74.98 | 75.01 | 74.26 | 74.44 | 69.76(90) | 74.15 | |
| 3/2 - | 31.51 | 31.21 | 31.35 | 31.28 | 31.28 | 31.29 | 31.25 | 31.60 | 31.57 | 30.3(15) | 31.71 | |
| 5/2 - | 36.74 | 36.12 | 36.24 | 36.18 | 36.12 | 36.14 | 36.09 | 36.61 | 36.54 | 36.6(11) | 36.76 | |
| 7/2 - | 55.27 | 54.74 | 54.92 | 54.95 | 54.71 | 54.91 | 54.87 | 55.39 | 55.28 | 55.2(11) | 55.63 | |
| 1/2 - | −50.54 | −54.56 | −54.65 | −54.22 | −54.70 | −54.71 | −54.80 | −53.24 | −53.60 | −50.78(17) | −52.25 | |
| 3/2 - | 48.42 | 47.21 | 47.34 | 47.53 | 47.42 | 47.36 | 47.33 | 47.92 | 47.73 | 46.2(15) | 51.04 | |
| 5/2 - | 32.63 | 31.60 | 31.74 | 31.95 | 31.86 | 31.83 | 31.80 | 32.38 | 32.22 | 31.93(86) | 33.16 | |
| 3/2 - | 15.52 | 13.16 | 13.29 | 13.55 | 13.22 | 13.13 | 13.08 | 14.12 | 13.96 | |||
| 3/2 - | 70.44 | 72.83 | 72.84 | 72.17 | 72.25 | 71.57 | 71.57 | 70.63 | 70.69 | |||
| 5/2 - | 69.95 | 69.80 | 69.83 | 69.92 | 69.85 | 69.87 | 69.83 | 70.27 | 70.16 | |||
| 1/2 - | 142.6 | 144.1 | 143.9 | 145.0 | 145.1 | 146.4 | 146.3 | 146.9 | 147.0 | |||
| 3/2 - | 54.50 | 54.21 | 54.25 | 54.30 | 54.15 | 53.93 | 53.89 | 54.29 | 54.12 | |||
| 1/2 - | 7.448 | 2.200 | 1.834 | 1.062 | 0.5278 | 0.02129 | −0.1153 | 2.625 | 2.153 | |||
| 1/2 - | 61.85 | 64.54 | 64.63 | 63.99 | 64.32 | 64.23 | 64.24 | 62.51 | 62.65 | |||
| 3/2 - | 22.42 | 22.04 | 22.05 | 21.57 | 21.55 | 21.52 | 21.48 | 21.49 | 21.32 | |||
| 5/2 - | 27.15 | 26.32 | 26.30 | 25.74 | 25.66 | 25.63 | 25.59 | 25.83 | 25.58 | |||
| 7/2 - | 53.97 | 53.32 | 53.50 | 53.43 | 53.31 | 53.38 | 53.35 | 54.07 | 53.96 | |||
| 1/2 - | −48.99 | −53.44 | −53.62 | −53.37 | −53.83 | −54.01 | −54.11 | −51.56 | −51.84 | |||
| 3/2 - | 49.34 | 47.89 | 47.98 | 47.76 | 47.70 | 47.18 | 47.18 | 47.83 | 47.73 | |||
| 5/2 - | 37.96 | 36.79 | 36.95 | 37.34 | 37.27 | 37.20 | 37.16 | 38.41 | 38.42 | |||
| 3/2 - | 66.12 | 69.31 | 69.68 | 68.40 | 68.59 | 67.48 | 67.54 | 65.52 | 65.65 | |||
| 5/2 - | 71.69 | 72.37 | 72.91 | 72.95 | 73.01 | 72.94 | 72.93 | 73.27 | 73.18 | |||
| 3/2 - | 17.73 | 15.21 | 15.37 | 16.04 | 15.70 | 16.00 | 15.89 | 18.17 | 18.01 | |||
| 1/2 - | 159.2 | 166.2 | 168.3 | 170.4 | 170.9 | 172.0 | 172.1 | 171.1 | 171.2 | |||
| 3/2 - | 58.17 | 59.13 | 59.44 | 58.78 | 58.71 | 57.80 | 57.79 | 57.95 | 57.84 |
| State | J P | Set 1 | Set 2 | Set 3 | Set 4 | Set 5 | Set 6 | Set 7 | TQ | TQ + NO | Exp. | MCHF |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1/2 + | 32.66 | 37.44 | 52.53 | 78.15 | 79.37 | 81.29 | 80.87 | 94.71 | 97.87 | 112.3(13) | 100.21 | |
| 3/2 + | 33.43 | 35.98 | 42.57 | 53.94 | 54.40 | 55.27 | 55.08 | 61.20 | 62.58 | 68.33(69) | 62.46 | |
| 5/2 + | 102.7 | 105.7 | 110.6 | 119.6 | 119.8 | 120.5 | 120.2 | 125.2 | 126.3 | 129.52(84) | 124.84 | |
| 1/2 + | 62.25 | 64.39 | 64.62 | 64.23 | 64.30 | 64.33 | 64.16 | 63.65 | 63.80 | |||
| 3/2 + | 104.9 | 104.2 | 104.8 | 105.1 | 105.0 | 105.0 | 105.2 | 105.9 | 105.8 | |||
| 5/2 + | 358.7 | 367.0 | 360.3 | 354.7 | 350.6 | 350.1 | 350.2 | 344.1 | 342.7 | |||
| 3/2 + | 372.1 | 375.4 | 370.9 | 364.9 | 361.1 | 360.4 | 360.8 | 353.7 | 352.2 | |||
| 1/2 + | 822.1 | 826.5 | 819.0 | 808.1 | 801.1 | 799.1 | 800.3 | 785.0 | 782.2 | |||
| 5/2 + | 131.6 | 134.6 | 134.9 | 135.2 | 135.5 | 135.8 | 135.7 | 136.3 | 136.5 | |||
| 3/2 + | 145.2 | 150.1 | 148.6 | 148.6 | 148.0 | 147.9 | 147.9 | 147.1 | 146.7 | |||
| 1/2 + | −23.62 | −31.15 | −32.82 | −35.04 | −35.37 | −34.80 | −34.70 | −31.91 | −31.76 | |||
| 3/2 + | 4.536 | 2.300 | 2.091 | 1.540 | 1.315 | 1.600 | 1.656 | 2.896 | 3.023 | |||
| 5/2 + | 78.43 | 78.30 | 79.10 | 79.27 | 78.99 | 79.20 | 79.07 | 80.39 | 80.45 | |||
| 1/2 + | 44.66 | 47.70 | 49.49 | 50.73 | 49.91 | 50.01 | 49.69 | 48.06 | 47.51 | |||
| 3/2 + | 97.52 | 99.82 | 103.6 | 106.1 | 104.3 | 104.1 | 103.4 | 103.8 | 102.4 | |||
| 3/2 + | −18.45 | −25.77 | −30.84 | −34.71 | −31.98 | −32.09 | −31.75 | −30.13 | −28.86 | |||
| 1/2 + | −98.12 | −99.42 | −101.9 | −103.4 | −102.4 | −102.4 | −102.0 | −102.8 | −102.1 | |||
| 3/2 + | 28.17 | 32.78 | 34.66 | 36.22 | 35.40 | 35.57 | 35.81 | 35.41 | 35.43 | |||
| 5/2 + | 23.08 | 23.91 | 24.17 | 24.29 | 24.67 | 24.63 | 24.59 | 24.63 | 24.68 | |||
| 7/2 + | 24.87 | 25.12 | 25.34 | 25.35 | 25.83 | 25.76 | 25.71 | 26.20 | 26.28 | |||
| 5/2 + | 36.01 | 36.50 | 35.47 | 9.842 | 28.58 | 27.95 | 22.38 | 19.45 | 20.54 | |||
| 5/2 + | −6.712 | −19.03 | −21.64 | 1.233 | −17.38 | −16.78 | −11.41 | −7.861 | −8.905 | |||
| 3/2 + | −15.17 | −42.66 | −43.61 | −41.87 | −42.17 | −42.31 | −42.11 | −40.81 | −40.89 | |||
| 1/2 + | 2.199 | −81.80 | −35.97 | −4.722 | −6.624 | −7.182 | −5.495 | −0.8566 | −1.526 | |||
| 7/2 + | 50.74 | 50.38 | 50.57 | 50.49 | 50.32 | 50.35 | 50.34 | 50.97 | 50.96 | |||
| 1/2 + | 189.6 | 266.1 | 217.3 | 182.9 | 184.6 | 185.1 | 183.2 | 179.7 | 180.4 | |||
| 3/2 + | 62.48 | 83.24 | 81.77 | 77.84 | 77.88 | 77.98 | 77.73 | 77.72 | 77.81 | |||
| 5/2 + | 35.54 | 40.21 | 40.77 | 40.67 | 40.23 | 40.30 | 40.25 | 40.89 | 40.88 | |||
| 7/2 + | 16.49 | 15.39 | 15.36 | 15.37 | 15.03 | 15.05 | 15.15 | 15.73 | 15.70 | |||
| 3/2 + | 59.89 | 60.98 | 61.16 | 61.20 | 61.26 | 61.29 | 61.31 | 60.75 | 60.70 | |||
| 5/2 + | 19.79 | 19.04 | 19.22 | 19.39 | 19.44 | 19.41 | 19.41 | 20.18 | 20.13 |
| Spectroscopic | Odd States | Even States | ||||
|---|---|---|---|---|---|---|
| Orbitals | ||||||
| 0.226 | – | – | 0.227 | – | – | |
| 1.262 | 1.275 | – | 1.249 | 1.268 | – | |
| – | 7.782 | – | 5.241 | – | 10.37 | |
| – | 13.98 | – | 12.64 | – | – | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, M.; Li, Y.; Godefroid, M.; Gaigalas, G.; Li, J.; Bieroń, J.; Chen, C.; Wang, J.; Jönsson, P. Natural Orbitals and Targeted Non-Orthogonal Orbital Sets for Atomic Hyperfine Structure Multiconfiguration Calculations. Atoms 2024, 12, 30. https://doi.org/10.3390/atoms12060030
Ma M, Li Y, Godefroid M, Gaigalas G, Li J, Bieroń J, Chen C, Wang J, Jönsson P. Natural Orbitals and Targeted Non-Orthogonal Orbital Sets for Atomic Hyperfine Structure Multiconfiguration Calculations. Atoms. 2024; 12(6):30. https://doi.org/10.3390/atoms12060030
Chicago/Turabian StyleMa, Mingxuan, Yanting Li, Michel Godefroid, Gediminas Gaigalas, Jiguang Li, Jacek Bieroń, Chongyang Chen, Jianguo Wang, and Per Jönsson. 2024. "Natural Orbitals and Targeted Non-Orthogonal Orbital Sets for Atomic Hyperfine Structure Multiconfiguration Calculations" Atoms 12, no. 6: 30. https://doi.org/10.3390/atoms12060030
APA StyleMa, M., Li, Y., Godefroid, M., Gaigalas, G., Li, J., Bieroń, J., Chen, C., Wang, J., & Jönsson, P. (2024). Natural Orbitals and Targeted Non-Orthogonal Orbital Sets for Atomic Hyperfine Structure Multiconfiguration Calculations. Atoms, 12(6), 30. https://doi.org/10.3390/atoms12060030









