Excitation of Helium by Proton and Antiproton Impact
Abstract
1. Introduction
2. Theory
2.1. Perturbational Method
2.2. The Time-Dependent Close-Coupling Method
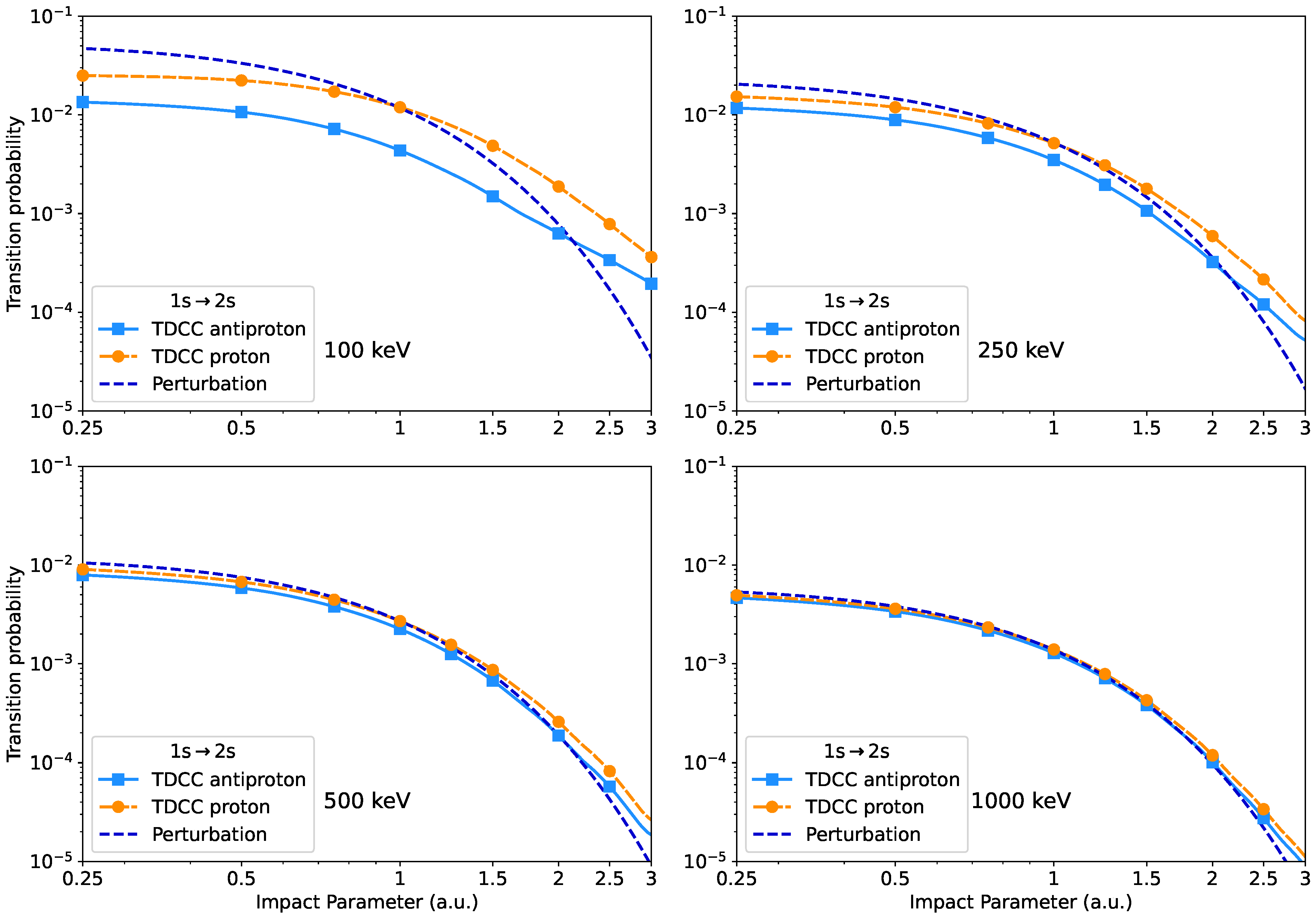
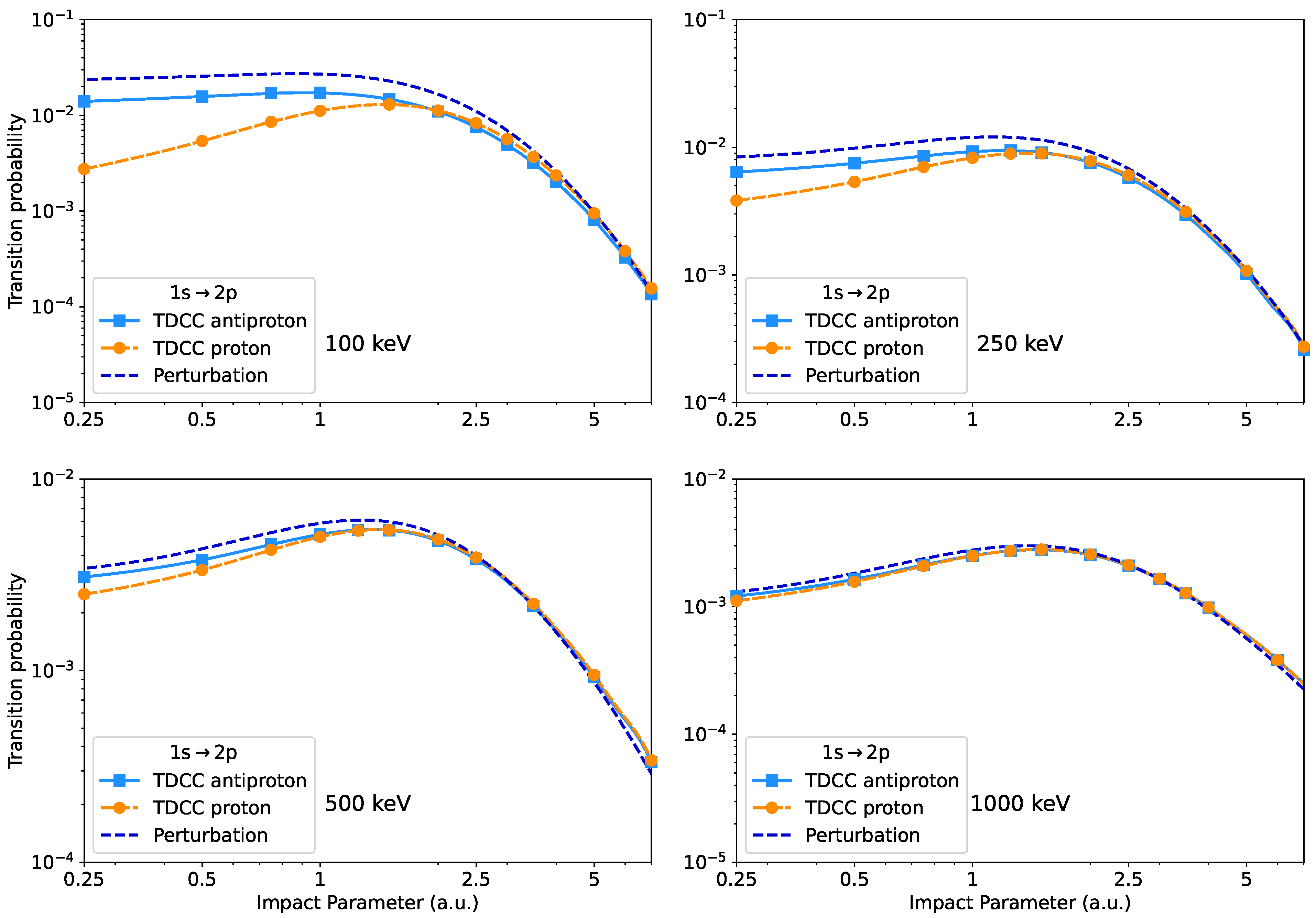
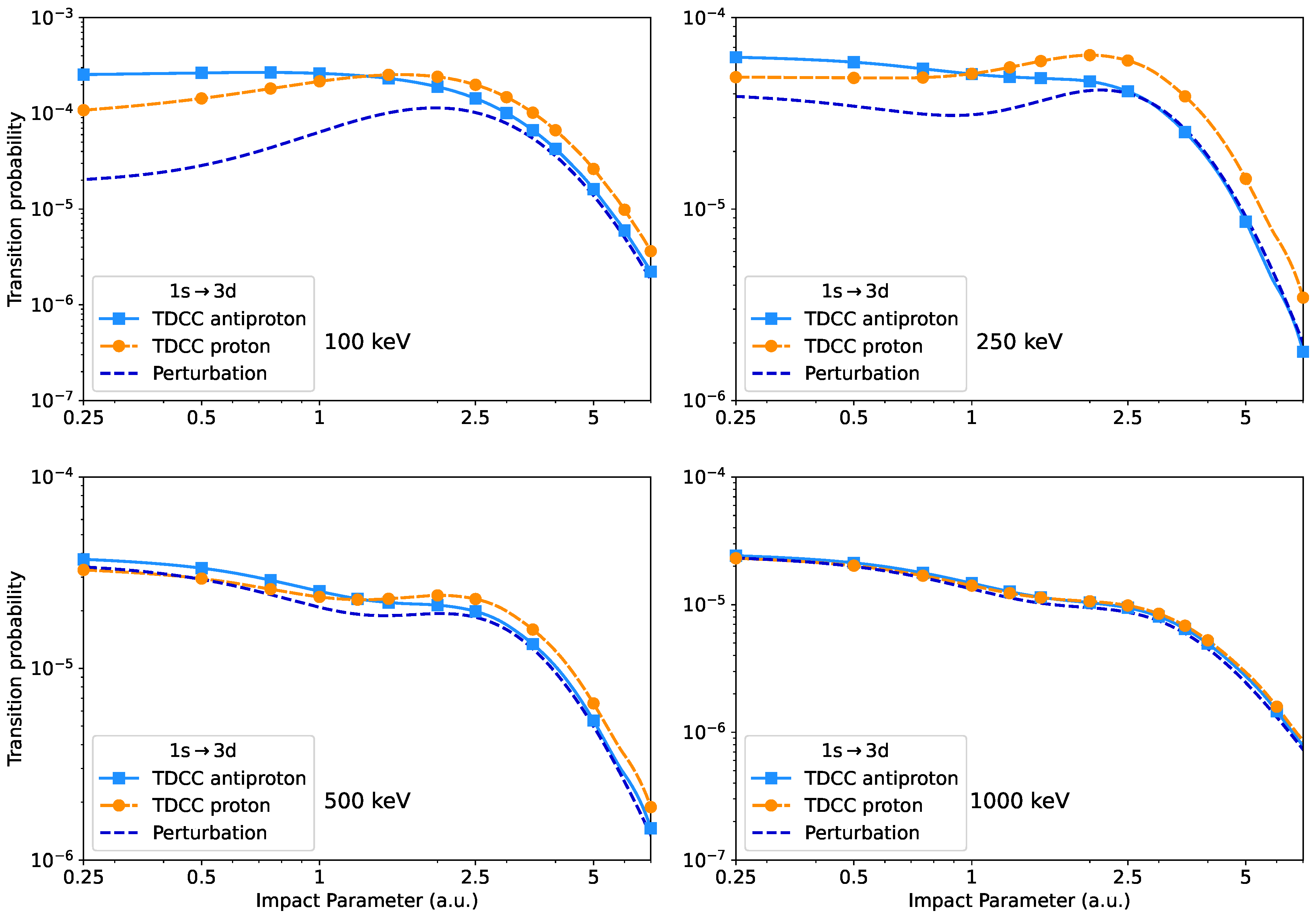
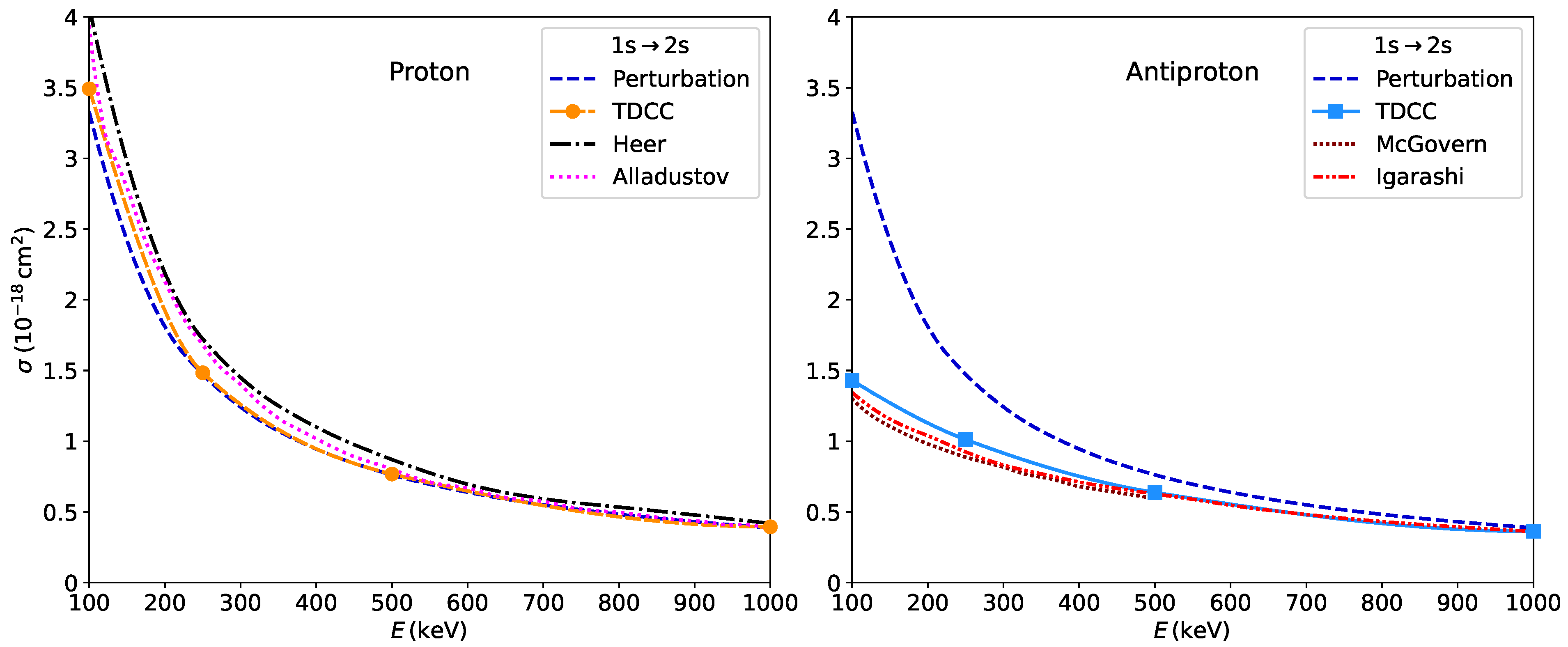
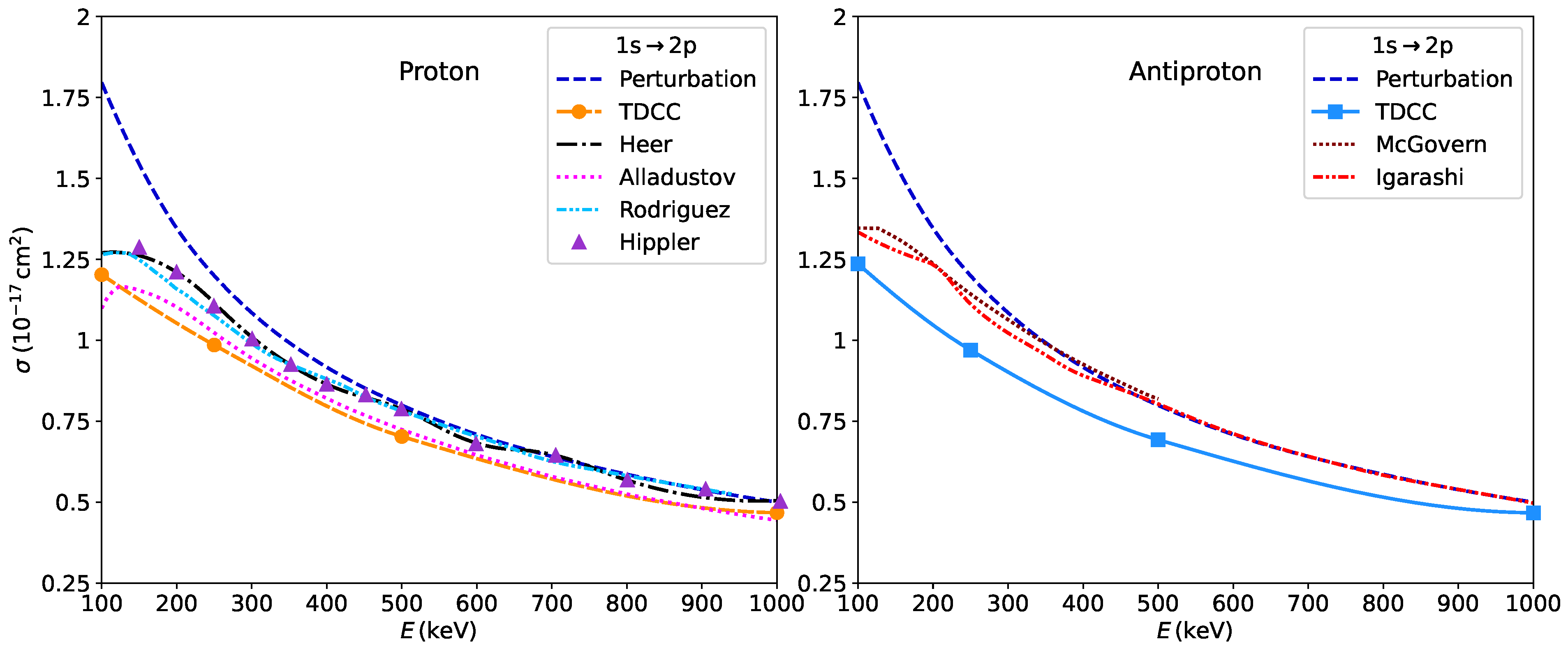
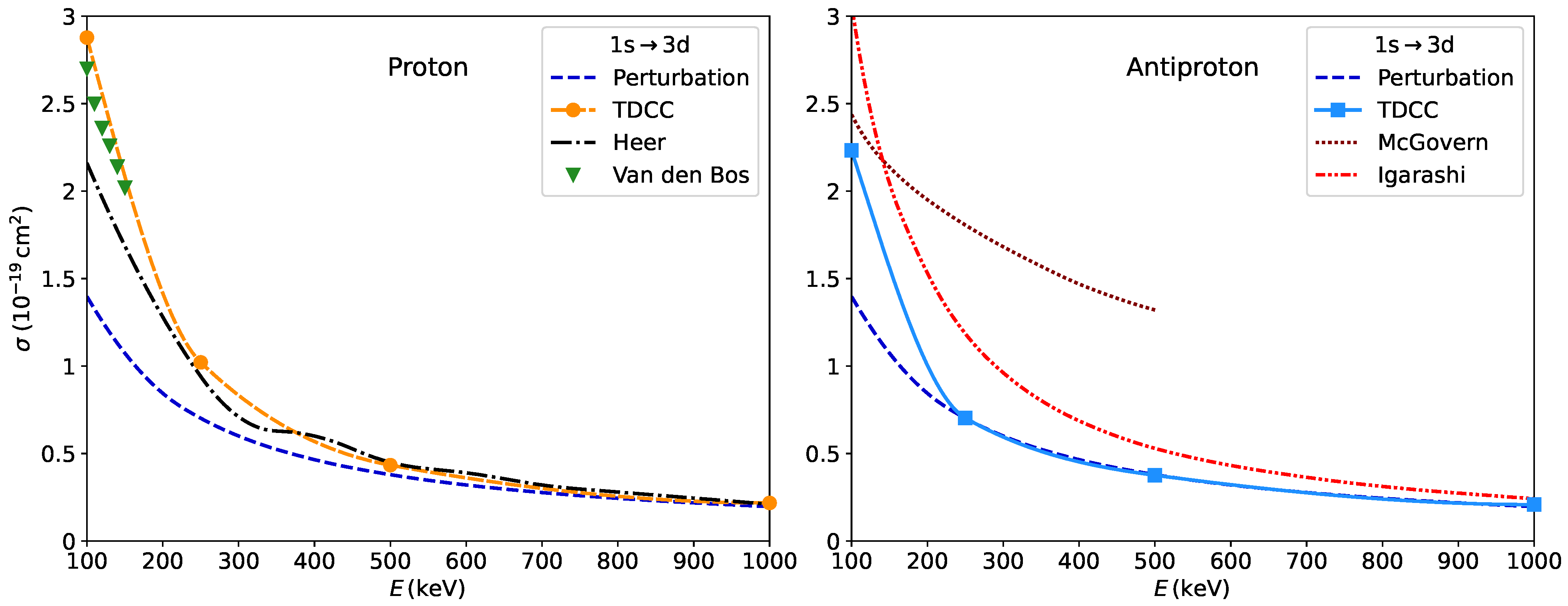
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Thomas, E.W.; Bent, G.D. Formation of Excited States in a Helium Target by the Impact of 0.15- to 1.0-MeV Protons and Deuterons. I. Experimental. Phys. Rev. 1967, 164, 143–150. [Google Scholar] [CrossRef]
- Thomas, E.W. Cross Sections for the Formation of Excited States in a Helium Target by the Impact of 0.15- to 1.0-MeV Protons and Deuterons. II. Comparison with Theory. Phys. Rev. 1967, 164, 151–155. [Google Scholar] [CrossRef]
- Fritsch, W. Helium Excitation in Heavy Particle Collisions. In Atomic and Plasma–Material Interaction Data for Fusion; IAEA: Vienna, Austria, 1992; Volume 3, pp. 41–46. [Google Scholar]
- De Heer, F.; Hoekstra, R.; Summers, H.P.; Undertaking, J.J. New Assessment of Cross-Section Data for Helium Excitation by Protons. In Atomic and Plasma–Material Interaction Data for Fusion; IAEA: Vienna, Austria, 1992; Volume 3, pp. 47–50. [Google Scholar]
- Andersen, L.H.; Hvelplund, P.; Knudsen, H.; Moller, S.P.; Sorensen, A.H.; Elsener, K.; Rensfelt, K.-G.; Uggerhoj, E. Multiple ionization of He, Ne, and Ar by fast protons and antiprotons. Phys. Rev. A 1987, 36, 3612–3629. [Google Scholar] [CrossRef] [PubMed]
- Reading, J.F.; Ford, A.L. The forced impulse method applied to the double ionisation of helium by collision with high-energy protons, antiprotons and alpha particles. J. Phys. B Atom. Mol. Phys. 1987, 20, 3747–3769. [Google Scholar] [CrossRef]
- Knudsen, H.; Reading, J. Ionization of atoms by particle and antiparticle impact. Phys. Rep. 1992, 212, 107–222. [Google Scholar] [CrossRef]
- Nagy, L. Two-electron processes in fast collisions with charged particles. Nucl. Instrum. Methods Phys. Res. B 1997, 124, 271–280. [Google Scholar] [CrossRef]
- Andersen, L.H.; Hvelplund, P.; Knudsen, H.; Mo, S.P.; Pedersen, J.O.P.; Tang-Petersen, S.; Uggerho, E.; Elsener, K.; Morenzoni, E. Single ionization of helium by 40–3000-keV antiprotons. Phys. Rev. A 1990, 41, 6536–6539. [Google Scholar] [CrossRef]
- Hvelplund, P.; Knudsen, H.; Mikkelsen, U.; Morenzoni, E.; Moller, S.P.; Uggerhoj, E.; Worm, T. Ionization of helium and molecular hydrogen by slow antiprotons. J. Phys. B At. Mol. Opt. Phys. 1994, 27, 925. [Google Scholar] [CrossRef]
- Knudsen, H.; Kristiansen, H.-P.E.; Thomsen, H.D.; Uggerhøj, U.I.; Ichioka, T.; Møller, S.P.; Hunniford, C.A.; McCullough, R.W.; Charlton, M.; Kuroda, N.; et al. Ionization of Helium and Argon by Very Slow Antiproton Impact. Phys. Rev. Lett. 2008, 101, 043201. [Google Scholar] [CrossRef]
- Knudsen, H.; Kristiansen, H.-P.E.; Thomsen, H.D.; Uggerhøj, U.I.; Ichioka, T.; Møller, S.P.; Hunniford, C.A.; McCullough, R.W.; Charlton, M.; Kuroda, N.; et al. On the double ionization of helium by very slow antiproton impact. Nucl. Instrum. Methods Phys. Res. Sect. B 2009, 267, 244. [Google Scholar] [CrossRef]
- Khayyat, K.; Weber, T.; Dörner, R.; Achler, M.; Mergel, V.; Spielberger, L.; Jagutzki, O.; Meyer, U.; Ullrich, J.; Moshammer, R.; et al. Differential cross sections in antiproton- and proton-helium collisions. J. Phys. B At. Mol. Opt. Phys. 1999, 32, L73. [Google Scholar] [CrossRef]
- Kirchner, T.; Knudsen, H. Current status of antiproton impact ionization of atoms and molecules: Theoretical and experimental perspectives. J. Phys. B At. Mol. Opt. Phys. 2011, 44, 122001. [Google Scholar] [CrossRef]
- Pindzola, M.S.; Lee, T.G.; Colgan, J. Antiproton-impact ionization of H, He and Li. J. Phys. B At. Mol. Opt. Phys. 2011, 44, 205204. [Google Scholar] [CrossRef]
- Guan, X.; Bartschat, K. Complete Breakup of the Helium Atom by Proton and Antiproton Impact. Phys. Rev. Lett. 2009, 103, 213201. [Google Scholar] [CrossRef]
- Abdurakhmanov, I.B.; Kadyrov, A.S.; Fursa, D.V.; Bray, I.; Stelbovics, A.T. Convergent close-coupling calculations of helium single ionization by antiproton impact. Phys. Rev. A 2011, 84, 062708. [Google Scholar] [CrossRef]
- Foster, M.; Colgan, J.; Pindzola, M.S. Fully Correlated Electronic Dynamics for Antiproton Impact Ionization of Helium. Phys. Rev. Lett. 2008, 100, 033201. [Google Scholar] [CrossRef]
- Borbély, S.; Feist, J.; Tőkési, K.; Nagele, S.; Nagy, L.; Burgdörfer, J. Ionization of helium by slow antiproton impact: Total and differential cross sections. Phys. Rev. A 2014, 90, 052706. [Google Scholar] [CrossRef]
- Borbély, S.; Tong, X.-M.; Nagele, S.; Feist, J.; Březinová, I.; Lackner, F.; Nagy, L.; Tőkési, K.; Burgdörfer, J. Electron correlations in the antiproton energy-loss distribution in He. Phys. Rev. A 2018, 98, 012707. [Google Scholar] [CrossRef]
- Rodríguez, V.D.; Ramírez, C.A.; Rivarola, R.D.; Miraglia, J.E. Helium excitation by protons and highly-charged-ion impact. Phys. Rev. A 1997, 55, 4201–4208. [Google Scholar] [CrossRef]
- Alladustov, S.U.; Abdurakhmanov, I.B.; Kadyrov, A.S.; Bray, I.; Bartschat, K. Wave-packet continuum-discretization approach to proton collisions with helium. Phys. Rev. A 2019, 99, 052706. [Google Scholar] [CrossRef]
- Spicer, K.H.; Plowman, C.T.; Abdurakhmanov, I.B.; Kadyrov, A.S.; Bray, I.; Alladustov, S.U. Differential study of proton-helium collisions at intermediate energies: Elastic scattering, excitation, and electron capture. Phys. Rev. A 2021, 104, 032818. [Google Scholar] [CrossRef]
- Hall, K.A.; Reading, J.F.; Ford, A.L. Excitation and ionization of atomic hydrogen by antiprotons. J. Phys. B 1996, 29, 6123. [Google Scholar] [CrossRef]
- Kirchner, T.; Lüdde, H.J.; Kroneisen, O.J.; Dreizler, R.M. New trends in the description of ion–atom collisions by time-dependent quantum methods. Nucl. Instrum. Methods Phys. Res. B 1999, 154, 46–53. [Google Scholar] [CrossRef]
- Igarashi, A.; Ohsaki, A.; Nakazaki, S. Single ionization of helium by antiproton impact. Phys. Rev. A 2000, 62, 052722. [Google Scholar] [CrossRef]
- McGovern, M.; Assafrão, D.; Mohallem, J.R.; Whelan, C.T.; Walters, H.R.J. Differential and total cross sections for antiproton-impact ionization of atomic hydrogen and helium. Phys. Rev. A 2009, 79, 042707. [Google Scholar] [CrossRef]
- Nagy, L.; Tóth, I.; Campeanu, R.I. Electron and Positron Impact Ionization of Molecules. Atoms 2024, 12, 38. [Google Scholar] [CrossRef]
- Schneider, B.I.; Collins, L.A. The discrete variable method for the solution of the time-dependent Schrödinger equation. J. Non-Cryst. Solids 2005, 351, 1551–1558. [Google Scholar] [CrossRef]
- Rescigno, T.N.; McCurdy, C.W. Numerical grid methods for quantum-mechanical scattering problems. Phys. Rev. A 2000, 62, 032706. [Google Scholar] [CrossRef]
- Park, T.J.; Light, J.C. Unitary quantum time evolution by iterative Lanczos reduction. J. Chem. Phys. 1986, 85, 5870–5876. [Google Scholar] [CrossRef]
- Schneider, B.I.; Feist, J.; Nagele, S.; Pazourek, R.; Hu, S.; Collins, L.A.; Burgdörfer, J. Recent Advances in Computational Methods for the Solution of the Time-Dependent Schrödinger Equation for the Interaction of Short, Intense Radiation with One and Two Electron Systems. In Quantum Dynamic Imaging: Theoretical and Numerical Methods; Bandrauk, A.D., Ivanov, M., Eds.; Springer: New York, NY, USA, 2011; pp. 149–208. [Google Scholar]
- Hernandez, V.; Roman, J.E.; Vidal, V. SLEPc: A scalable and flexible toolkit for the solution of eigenvalue problems. ACM Trans. Math. Softw. 2005, 31, 351–362. [Google Scholar] [CrossRef]
- Nesbet, R.K.; Watson, R.E. Approximate Wave Functions for the Ground State of Helium. Phys. Rev. 1958, 110, 1073–1076. [Google Scholar] [CrossRef]
- Bransden, B.H.; Joachain, C.J. Physics of Atoms and Molecules; Longman & Scientific Technical: New York, NY, USA, 1983; pp. 139, 275, 285. [Google Scholar]
- Bohr, N., II. On the theory of the decrease of velocity of moving electrified particles on passing through matter. Philos. Mag. 1913, 25, 10–31. [Google Scholar] [CrossRef]
- Hippler, R.; Schartner, K.H. Absolute cross sections for the excitation of n1P-levels of helium by proton impact (150–1000 keV). J. Phys. B Atom. Mol. Phys. 1974, 7, 618. [Google Scholar] [CrossRef]
- Van den Bos, J.; Winter, G.J.; De Heer, F.J. Excitation of helium by protons in the 1–150 keV region. Physica 1968, 40, 357–384. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bálint, Z.; Borbély, S.; Nagy, L. Excitation of Helium by Proton and Antiproton Impact. Atoms 2024, 12, 57. https://doi.org/10.3390/atoms12110057
Bálint Z, Borbély S, Nagy L. Excitation of Helium by Proton and Antiproton Impact. Atoms. 2024; 12(11):57. https://doi.org/10.3390/atoms12110057
Chicago/Turabian StyleBálint, Zsuzsánna, Sándor Borbély, and Ladislau Nagy. 2024. "Excitation of Helium by Proton and Antiproton Impact" Atoms 12, no. 11: 57. https://doi.org/10.3390/atoms12110057
APA StyleBálint, Z., Borbély, S., & Nagy, L. (2024). Excitation of Helium by Proton and Antiproton Impact. Atoms, 12(11), 57. https://doi.org/10.3390/atoms12110057






