Towards an Intrinsic Doppler Correction for X-ray Spectroscopy of Stored Ions at CRYRING@ESR

Abstract

We report on a new experimental approach for the Doppler correction of X-rays emitted by heavy ions, using novel metallic magnetic calorimeter detectors which uniquely combine a high spectral resolution with a broad bandwidth acceptance. The measurement was carried out at the electron cooler of CRYRING@ESR at GSI, Darmstadt, Germany. The X-ray emission associated with the radiative recombination of cooler electrons and stored hydrogen-like uranium ions was investigated using two novel microcalorimeter detectors positioned under 0${}^{\circ }$ and 180${}^{\circ }$ with respect to the ion beam axis. This new experimental setup allowed the investigation of the region of the N, M → L transitions in helium-like uranium with a spectral resolution unmatched by previous studies using conventional semiconductor X-ray detectors. When assuming that the rest-frame energy of at least a few of the recorded transitions is well-known from theory or experiments, a precise measurement of the Doppler shifted line positions in the laboratory system can be used to determine the ion beam velocity using only spectral information. The spectral resolution achievable with microcalorimeter detectors should, for the first time, allow intrinsic Doppler correction to be performed for the precision X-ray spectroscopy of stored heavy ions. A comparison with data from a previous experiment at the ESR electron cooler, as well as the conventional method of conducting Doppler correction using electron cooler parameters, will be discussed.

1. Introduction

One of the frontiers of quantum electrodynamics (QED) is the study of electrons in extreme electromagnetic fields [1,2]. In this context, few-electron ions of the heaviest elements, such as U${}^{91+}$ and U${}^{90+}$, may serve as the ultimate test bed for precision measurements at the highest field strengths [3,4]. Experimental access to the binding energies of few-electron heavy ions is usually provided by the X-ray spectroscopy of characteristic transitions, in particular the K${}_{\alpha }$ ($n=2\to n=1$) transitions, which occur between the most strongly bound states [5]. For such studies, storage rings equipped with electron cooler devices are the facilities of choice, e.g., the Experimental Storage Ring (ESR) [6] or the CRYRING@ESR [7,8], both located at GSI, Darmstadt. Electron cooling exploits the Coulomb interaction between stored ions and a cold electron beam to reduce the emittance as well as the kinetic energy dispersion [9] of the ion beam, thus reducing the Doppler broadening of the spectral lines emitted by the ions. Moreover, this process results in matching the ion beam velocity to the velocity of the electron beam, resulting in a better control of the Doppler shift. In addition, storage rings enable the deceleration of the ions to perform measurements at velocities significantly below the production threshold of the respective charge states and thus with a reduced Doppler shift. Finally, the high rates of repetition in the order of 1 MHz with which the stored ions are passed through an in-ring target result in a much higher luminosity compared with single-pass setups. This enables the use of dilute gas targets [10] or free-electron targets which provide single-collision conditions, thus yielding X-ray spectra undistorted by multiple-collision effects.

However, for the interpretation of the X-ray spectra obtained at storage rings, the correction of the Doppler shift, i.e., the transformation of the observed line energies $E_{\gamma ,\mathrm{lab}}$ from the laboratory system into the emitter frame, i.e., $E_{\gamma ,\mathrm{emit}}$, of the moving ions, is of paramount importance. The relativistic Doppler shift depends on the ion velocity $\beta$ and the observation angle $\theta$ (being defined with respect to the ion beam axis) and can be expressed as

$$E_{\gamma ,\mathrm{emit}}=E_{\gamma ,\mathrm{lab}}·{\displaystyle \frac{1-\beta \cos \left(\theta \right)}{\sqrt{1-{\beta }^2}}}.$$

(1)

As a consequence, the uncertainty of the reconstructed rest-frame transition energy $\Delta E_{\gamma }$ caused by the Doppler correction is related to the uncertainty of the velocity $\Delta \beta$, as well as to an imperfect knowledge of the observation angle expressed as $\Delta \theta$. The Doppler shift as well as the contribution of $\Delta \beta$ and $\Delta \theta$ to $\Delta E_{\gamma }$ is depicted in Figure 1 for the case of a photon with $E_{\gamma ,\mathrm{emit}}=100$ keV energy in the rest frame. In this figure, kinetic ion beam energies of 400 MeV/u, 50 MeV/u, and 10 MeV/u were chosen, covering the energy range accessible for heavy, highly charged ions in the storage rings ESR and CRYRING@ESR. The geometrical uncertainty $\Delta \theta$ is limited by the challenges in determining and monitoring the exact positions of an in-ring X-ray source and the surrounding spectrometer systems for a non-permanent experimental setup. On the other hand, the velocity uncertainty $\Delta \beta$ is tied to the knowledge of the effective acceleration potential inside the electron cooler, which determines the electron beam velocity that is in turn imprinted on the ion beam. For the uncertainty of the observation angle and the ion beam velocity, typical values of $0.{01}^{\circ }$ and $1\times {10}^{-5}$, respectively, were chosen. Note that for a typical distance of 1 m between the source and the detector, an angular uncertainty of $0.{01}^{\circ }$ corresponds to a uncertainty of the relative positions of approximately 0.2 mm. Moreover, at a low ion-beam energy of 10 MeV/u, the stated velocity uncertainty translates into an uncertainty of the acceleration voltage $\Delta U$ of slightly below 1 V. This is already very challenging to achieve given the fact that the externally applied high voltage, even if known precisely, needs to be corrected for effects such as the electron beam’s space-charge potential, as well as the contact potential between the cathode and the collector electrode.

According to Figure 1, the choice of a specific observation angle implies a trade-off between the imperfect knowledge of the ion beam velocity and the geometry of the experimental setup, i.e., the observation angle. In particular, as the gradient of the angular-dependent Doppler shift vanishes at $0^{\circ }$ and ${180}^{\circ }$, these angles are insensitive to a minor misalignment $\Delta \theta$ of the detector with respect to the ion beam axis. On the other hand, the uncertainty contribution due to $\Delta \beta$ reaches its maximum values at these observation angles. Conversely, there is a ’magic angle’ where the $\Delta \beta$ contribution vanishes, while the sensitivity of the reconstructed rest-frame photon energy on the observation angle is maximally pronounced. This angle is located at ${90}^{\circ }$ in the limit of $\beta \to 0$ and is shifted into the forward direction for increasing beam velocities.

Ongoing efforts in the field of hard X-ray spectroscopy aim to push the accuracy of L → K transition energy measurements in systems with high atomic numbers Z beyond the limit necessary for accessing higher-order QED effects on the ground state energy, the evaluation of which has been recently completed for a hydrogen-like system as a result of very extensive theoretical work [11]. For the highest quasi-stable system, namely uranium, this requires an uncertainty below 1 eV at a transition energy of roughly 100 keV, i.e., $\frac{\Delta E_{\gamma }}{E_{\gamma }}<{10}^{-5}$. Thus, the typical uncertainties of the reconstructed photon energy depicted in Figure 1 are too large to achieve this goal.

However, when assuming that the recorded spectrum also contains lines whose transition energies are precisely known from theory or experiment, an estimation of the Doppler factor solely from the recorded spectra is possible. This allows the positions of the lines of interest to be transformed into the emitter frame, without the need for auxiliary measurements to characterize the experimental setup. In previous studies relying on conventional semiconductor detectors, their limited spectral resolution prevented the use of this method, referred to in the following as intrinsic Doppler correction. On the other hand, with crystal spectrometers such as the FOCAL setup, a high resolution is achieved but only in a small energy window around the line of interest [12], such that lines suitable for the aforementioned Doppler correction method were not recorded. In this report, we present first results from a recent measurement using novel microcalorimeter detectors, which uniquely combine a high spectral resolution with a broad bandwidth acceptance. These findings are likely to pave the way towards a Doppler correction relying only on the X-ray spectra emitted by fast-moving, highly charged ions.

2. Experimental Results and Discussion

The data presented in this work were obtained in a recent experiment at the electron cooler of the recently commissioned CRYRING@ESR, where two novel microcalorimeters for precision X-ray spectroscopy were positioned at 0${}^{\circ }$ and 180${}^{\circ }$ with respect to the ion beam axis [13]. A beam of hydrogen-like uranium (U${}^{91+}$) ions was stored at a kinetic energy of 10.225 MeV/u and the interaction of these ions with the electron beam in the cooler device was investigated. The experiment was conducted with varying ion beam intensities averaging to approximately $1\times {10}^6$ ions per injection and a week of continuous measurement time. The electron cooler was operated with an electron density of approximately $1·{10}^7{\mathrm{cm}}^{-3}$. These free electrons can recombine with the stored ions via a process referred to as radiative recombination (RR) [14]. At relative velocities close to zero, as is the case in an electron cooler, the RR process favors the recombination of electrons into Rydberg states, i.e., high quantum numbers $n,l$, which subsequently decay to the ground state via radiative cascades. Due to the selection rules in combination with the high orbital angular momentum of the initially populated states, most of the recombined electrons pass through a state with the highest orbital angular momentum for a given shell at some point during their cascade. Therefore, the formation of an U${}^{90+}$ ion in the cooler section typically results in the emission of numerous characteristic photons along a yrast decay pattern [15,16].

This radiation was recorded using the aforementioned microcalorimeter detectors of the maXs-type (Micro-Calorimeter Arrays for High-Resolution X-ray Spectroscopy) [17,18]. These are based on the metallic magnetic calorimeter (MMC) technology, which features a unique combination of a high spectral resolution with a broad bandwidth acceptance [19]. To be more specific, each detector featured an 8 × 8 array of gold absorbers with an area of 1.25 mm × 1.25 mm each and a thickness of 50 $\mathsf{\mu }$m. The necessary operation temperature below 20 mK was achieved using a ${}^3$He/${}^4$He dilution refrigerator cryostat. 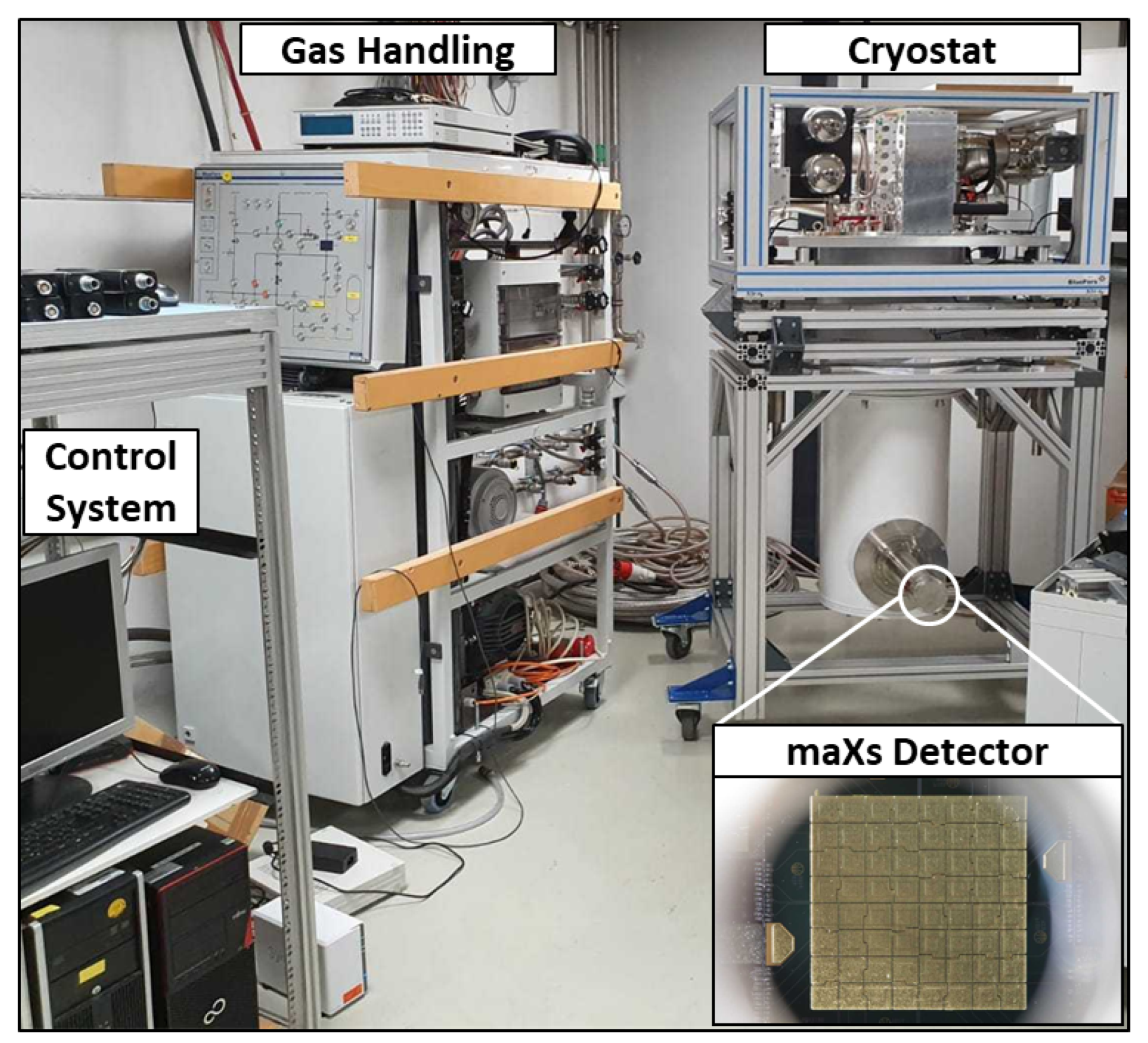These maXs-100 detector systems were designed for a spectral resolution $\Delta E_{\mathrm{FWHM}}<50$ eV in a broad photon energy range from a few keV to above 100 keV. A photograph of a maXs system operating at a test stand at the GSI facility is depicted in Figure 2. While in this environment the design value for the spectra resolution was reached, the preliminary analysis suggests that only a resolution of 80 to 90 eV was achieved at the setup at CRYRING@ESR. This spectral resolution, however, is still a major improvement compared to typical resolutions of Ge(i) detectors of several hundreds of eV. We attribute this to an imperfect isolation from vibrations, which can deteriorate the cooling power of the cryostats as well as the coupling of electromagnetic interferences into the superconducting quantum interference device (SQUID) readout electronics, resulting in a higher noise level of the detector signal. For a more detailed description of the experimental setup, the reader is referred to [13].

In the following, the discussion is restricted to the preliminary data recorded by the detector under 0${}^{\circ }$. Here, the blue shift of the observed spectrum results in a larger separation of neighboring transition lines; thus, the requirements on the spectral resolution for a meaningful analysis of individual lines are loosened. For the 180${}^{\circ }$ data, work on raw data analysis routines, aiming at an improvement in resolution, is still ongoing. 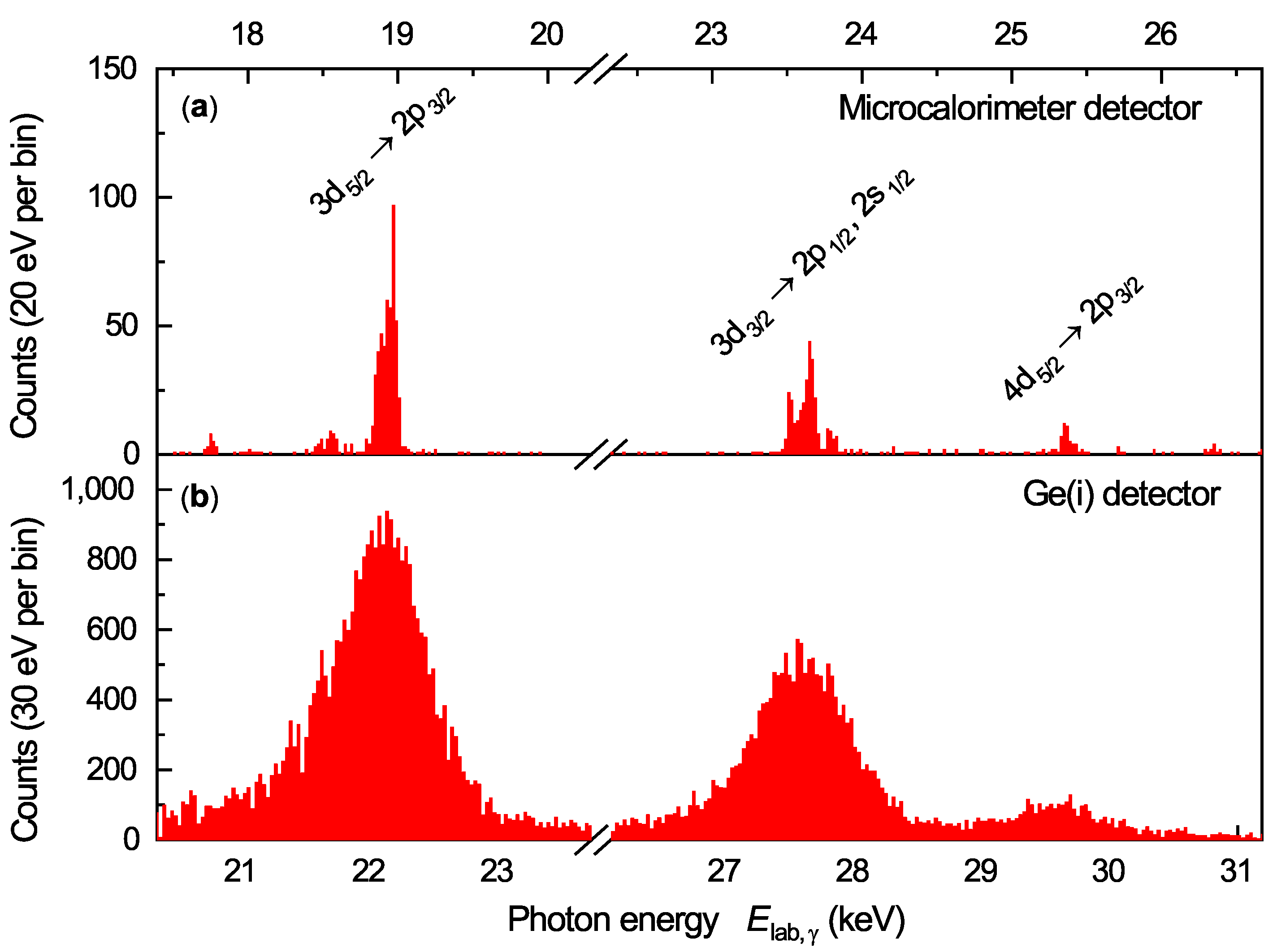In Figure 3, the spectral region of N, M → L transitions in U${}^{90+}$ is presented in comparison to a similar spectrum obtained with a Ge(i) X-ray detector at the electron cooler of the ESR [3] at an ion beam energy of 43.59 MeV/u. As can be clearly seen, the significantly improved spectral resolution of the maXs detector system enables numerous transitions of U${}^{90+}$ to be resolved at the same time, which were merged into single peaks in the previous system of measurement. Furthermore, the superior signal to background ratio of the spectrum recorded by the maXs detector is also noteworthy. The lower background level is due to the much smaller volume of the 64 absorber pixels (in total, approximately 0.005 cm${}^3$) of the maXs detector compared with a typical semiconductor crystal, which results in a correspondingly lower event rate caused by cosmic radiation and other types of background radiation. In contrast, the total number of detected photons is much smaller in the present experiment, which can be attributed to the relatively small active area of 1 cm${}^2$ of the maXs-100 detector, as well as to various starting difficulties in the operation of a new experimental setup at an only recently commissioned facility. Nevertheless, the high quality of the new spectrum will enable a much more detailed analysis than was possible with semiconductor detectors, the spectral resolution of which is innately limited to values $\gg 100$ eV in the energy region of interest. Namely, we will use the resolved N, M → L transitions to infer the relative populations of the excited states, which can then be compared to a model of the initial population by the RR process and subsequent decay cascades. In this direction, a rigorous analysis of the data obtained in this recent experiment is still ongoing.

Finally, we would like to point out the potential of such high-resolution spectra for a self-consistent intrinsic correction of the Doppler shift. To be more specific, X-rays emitted by fast-moving, heavy ions, with the combination of a high spectral resolution and a broad bandwidth acceptance, enable the usage of lines with well-known energies to determine the Doppler shift of the recorded spectrum. This alternative method for Doppler correction avoids the necessity of auxiliary measurements to determine the exact detector position and/or the ion beam velocity, which are typically associated with sizeable uncertainties, as explained above.

To assess the potential benefits of such an approach, we used a simulation to investigate the expected statistical uncertainty of the re-scaling of the energy axis to correct for the Doppler effect, determined based on the observed line positions of the N, M → L transitions depicted in Figure 3 versus their theory values. The assumption that at least some of these energies are precisely known is clearly justified for hydrogen-like systems as the contributions due to QED and the nuclear size vanish for high $n,l$ states, but less so for helium-like systems due to the complexity of the electron–electron interaction. However, as the following discussion is only intended for illustrative purposes, a detailed investigation of theoretical uncertainties of the relevant L, N, and M shell-binding energies in U${}^{90+}$ is beyond the scope of the present work.

In the simulation, we aimed to use a Doppler correction to reconstruct the rest-frame energy of a hypothetical L → K transition in a high-Z system. For this transition of interest, we assumed an energy of 100 keV in the rest frame, as was already carried out to produce the data shown in Figure 1. A range of possible detector resolutions $\Delta E_{\mathrm{FWHM}}$ and numbers of recorded photons $N_{\mathrm{photon}}$ across all relevant N, M → L transitions was considered. Note that as the achievable resolution is limited by the heat capacity, and thus the volume of the absorber, a better detector resolution will generally come at the expense of a decrease in active area and/or absorption efficiency, resulting in a lower number of recorded photons. This trade-off is exemplified by the fact that for 6 keV photons, a MMC detector system achieved a resolution of below 2 eV FWHM [19], but the absorber pixels used in this study were not suitable for the stopping of photons in the higher energy range, as was relevant for the present work. Therefore, we restricted the simulation to a realistic range of detector resolutions, from 10 eV to 100 eV FWHM. For the number of recorded photons available for the determination of the Doppler factor, we considered a range between 500 and 10,000. This is in line with our estimation that incremental improvements in the experimental setup and the accelerator performance compared with the present first-of-its-kind measurement will allow us to increase the number of recorded photons in the N, M → L range (approximately 800 at present) by a factor of 5 to 10.

For each setting of ($N_{\mathrm{photon}}$, $\Delta E_{\mathrm{FWHM}}$), we employed a Monte Carlo method to generate 500 synthetic N, M → L spectra. The N, M → L transition energies were calculated using the Flexible Atomic Code (FAC) [20,21] and the line intensities were predicted using a detailed model of the initial population by the RR process in combination with the subsequent decay cascades [16]. When setting the kinetic energy of the ion beam to 10.225 MeV/u, i.e., identical to the recent measurement, the simulation yields spectra very similar to the experimental data. For each of these synthetic spectra, the Doppler shift was extracted by comparing the recorded line positions to the theory values. This factor was then used to transform the energy of the hypothetical line of interest from the laboratory frame to the emitter frame. The 1$\sigma$ deviation of the reconstructed rest-frame photon energy to the real energy of 100 keV is presented in Figure 4. These values represent the purely statistical uncertainty of the described intrinsic Doppler correction method, assuming a perfect calibration of the energy axis of the detector and precise knowledge of the N, M → L transition energies. For reference, the position of the present data in this matrix is indicated by the red circle. It is found that for a reasonable number of a few thousand detected N, M → L photons and a spectral resolution of better than 50 eV, an intrinsic Doppler correction with a statistical uncertainty of less than 1 eV for L → K transitions in high-Z systems is feasible.

3. Summary and Outlook

We report the preliminary data of a precision X-ray spectroscopy experiment using novel microcalorimeter detectors at the electron cooler of the CRYRING@ESR at GSI. In this experiment, a stored beam of hydrogen-like uranium interacted with cooler electrons, resulting in the formation of helium-like uranium ions. The new experimental setup enabled us to record N, M → L transitions with a high spectral resolution unmatched by previous measurements relying on semiconductor detectors. The combination of a high spectral resolution with a broad bandwidth acceptance offers unique possibilities for the spectroscopy of fast-moving heavy ions. Namely, transitions with well-known energies can be utilized to precisely determine the Doppler shift from the recorded spectra, without having to rely on external information on the detector position and/or the ion beam velocity. This information can then be used to transform the positions of lines of interest into the emitter frame. We illustrate such scenarios using simulated data.

Moreover, when the spectra are recorded exactly under 0${}^{\circ }$, as well as 180${}^{\circ }$, this general method for Doppler correction, valid for arbitrary observation angles, can be replaced by a more simple method, already employed in laser spectroscopy measurements (see, e.g., [22]). Combining Formula (1) for each detector as

$$\sqrt{E_{\gamma ,\mathrm{lab}}\left(0\right)·E_{\gamma ,\mathrm{lab}}\left(180\right)}=E_{\gamma ,\mathrm{emit}}$$

(2)

results in a cancellation of the velocity-dependent Doppler factor. The treatment of this special case will be the subject of an upcoming study, once the data for both detectors are analyzed.