Studying the Spectral Energy Distributions Emanating from Regular Galactic XRBs
Abstract
1. Introduction
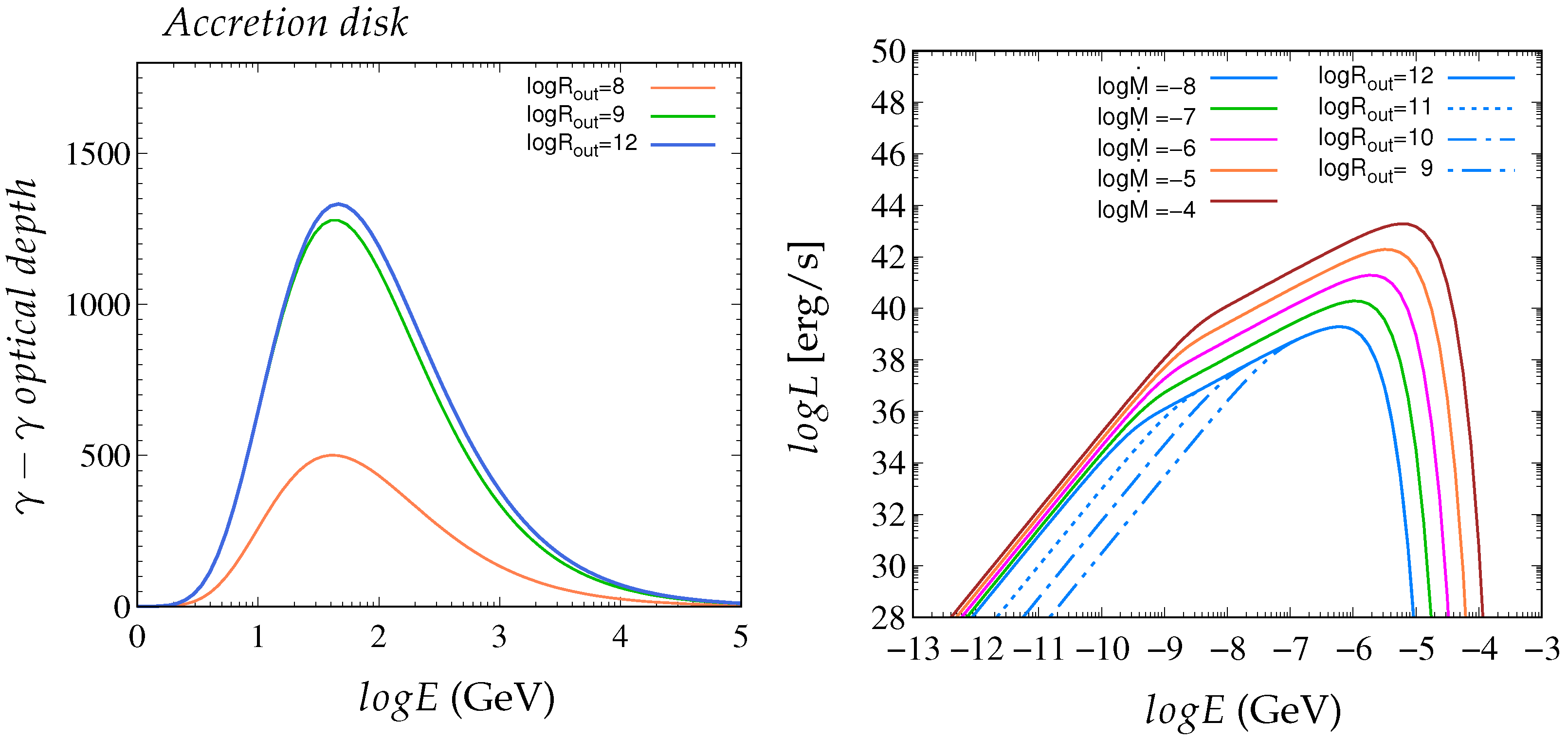
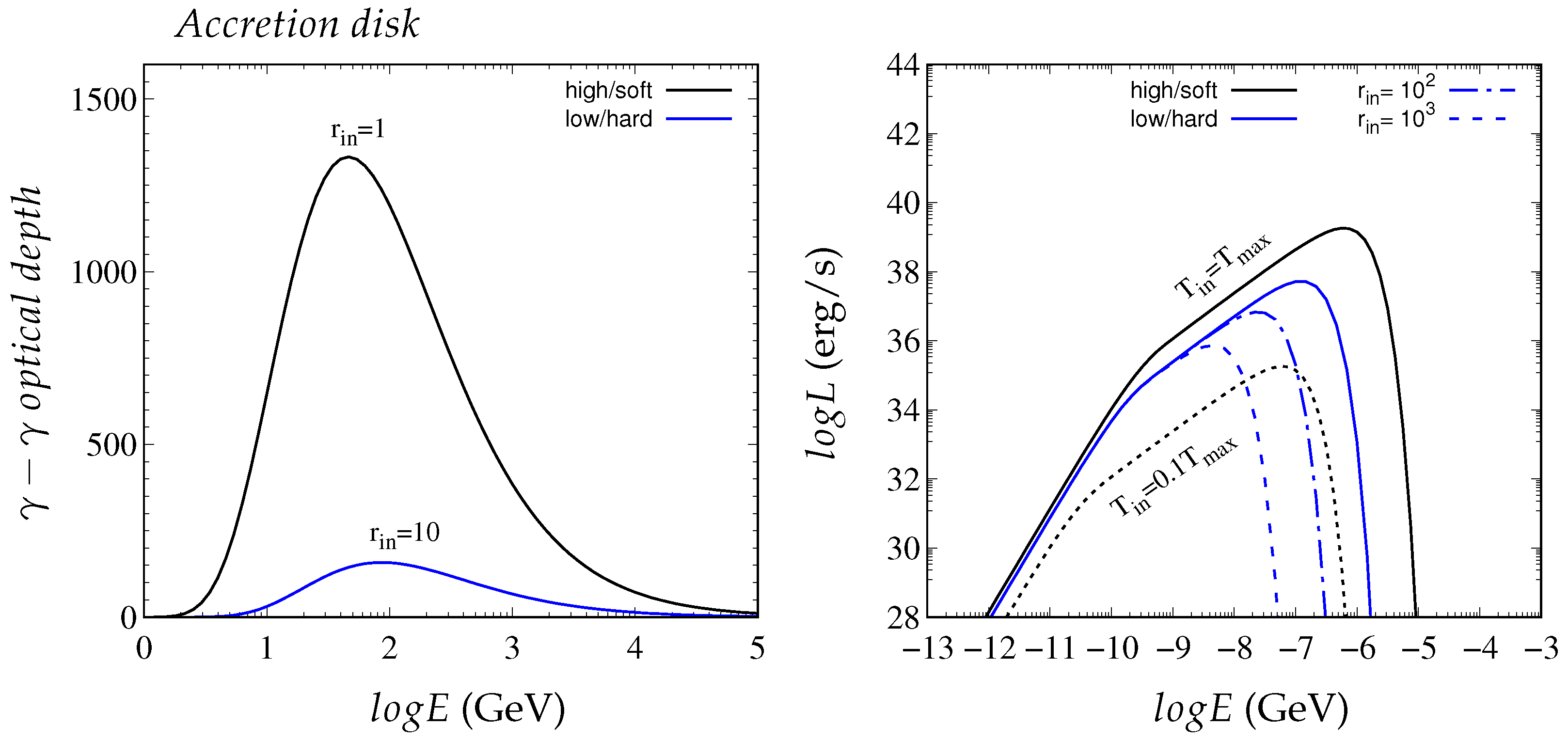
2. Accretion Disk Luminosity
3. Secondary Stellar Component
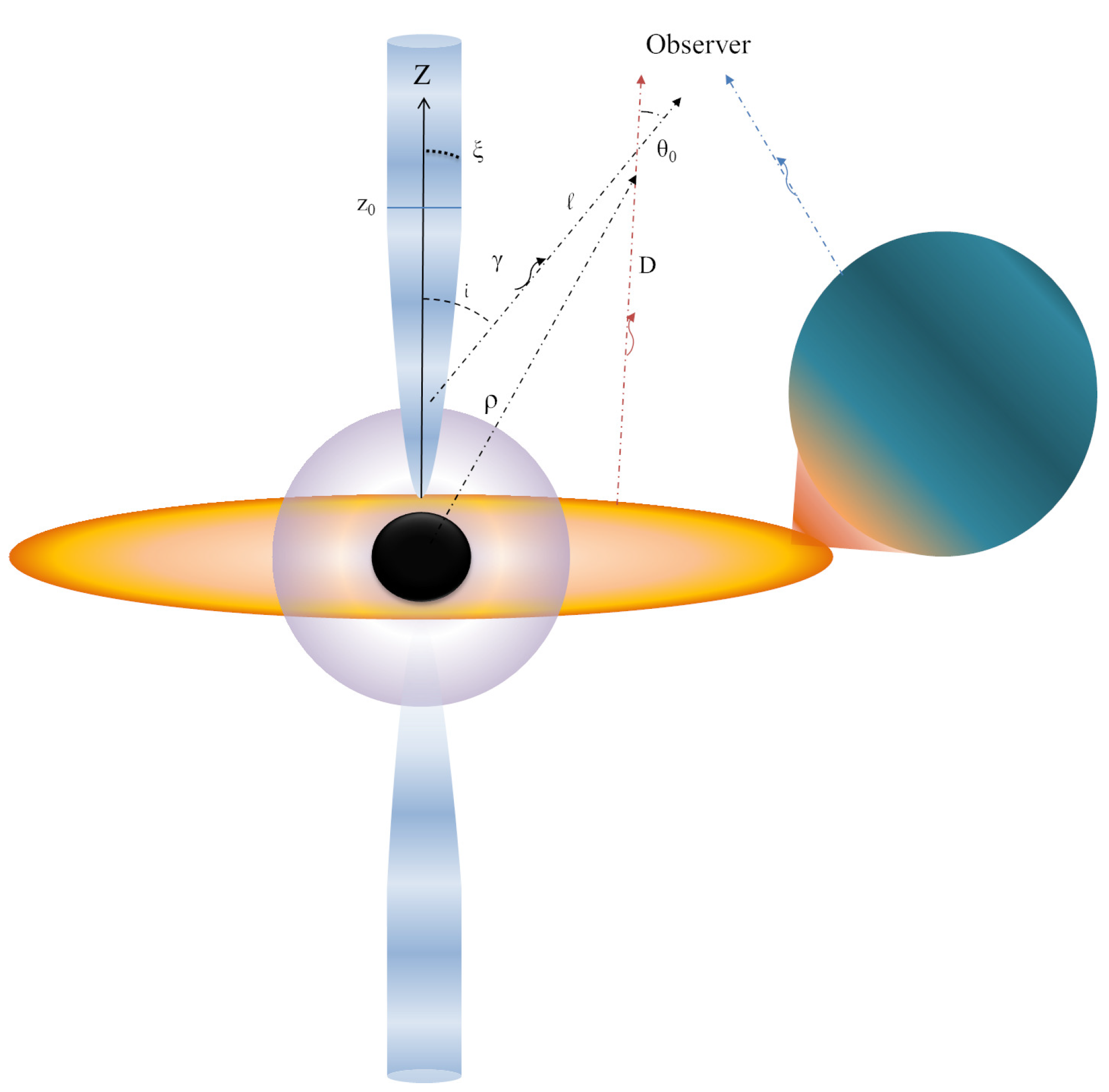
4. Jet Emission
4.1. Leptonic Mechanisms
4.1.1. Synchrotron Emission
4.1.2. Inverse Comptonization
4.2. Hadron Interactions
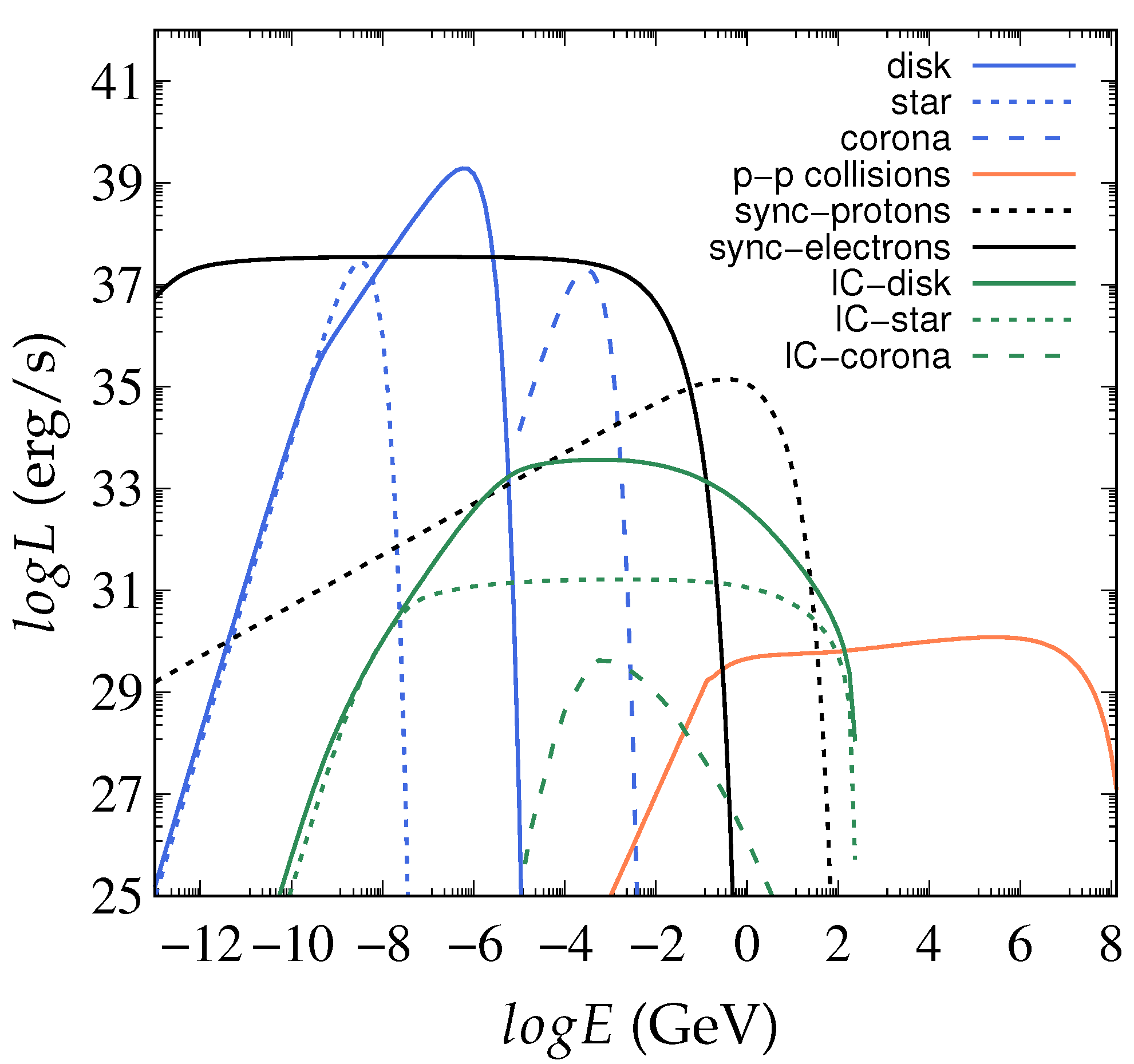
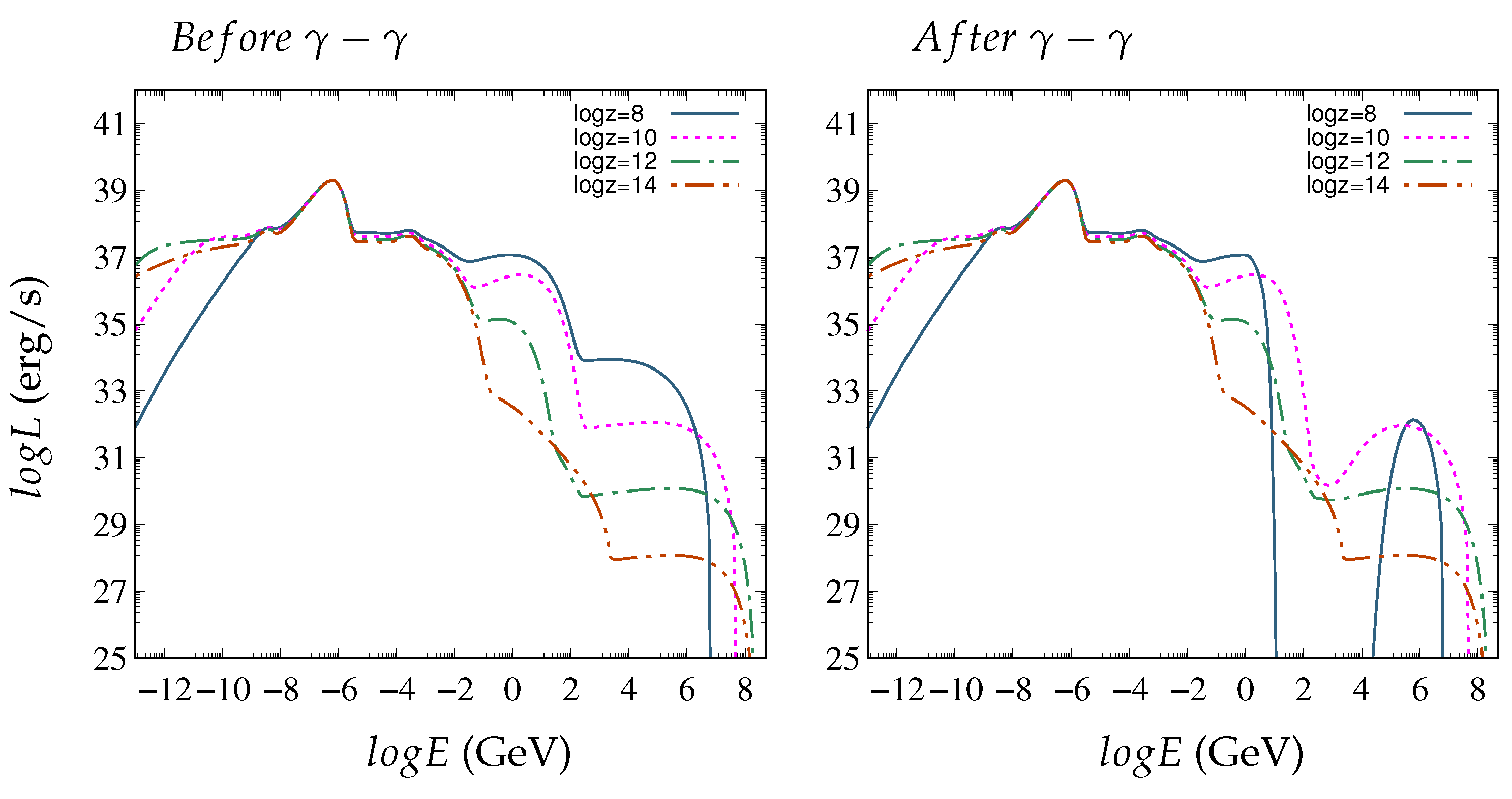
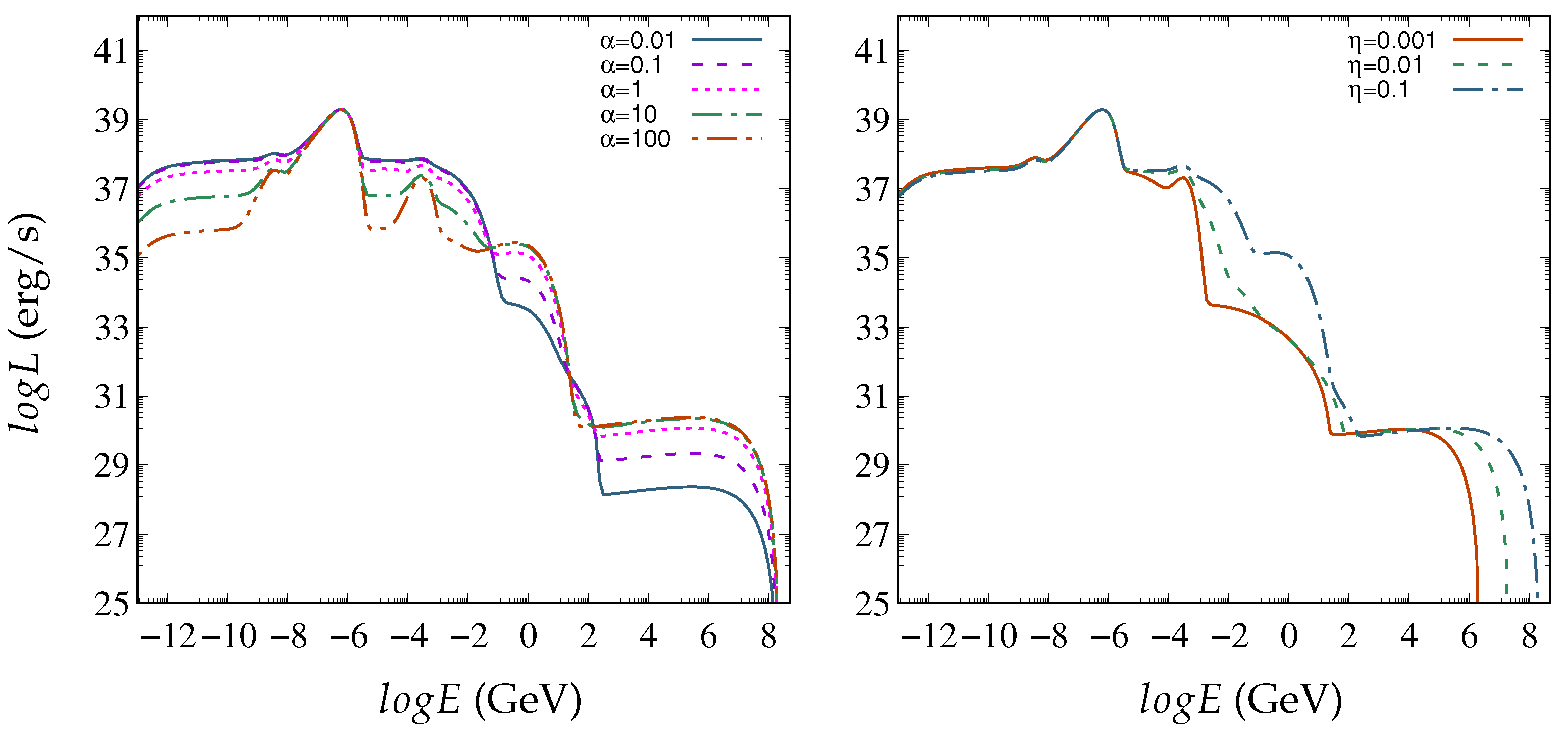
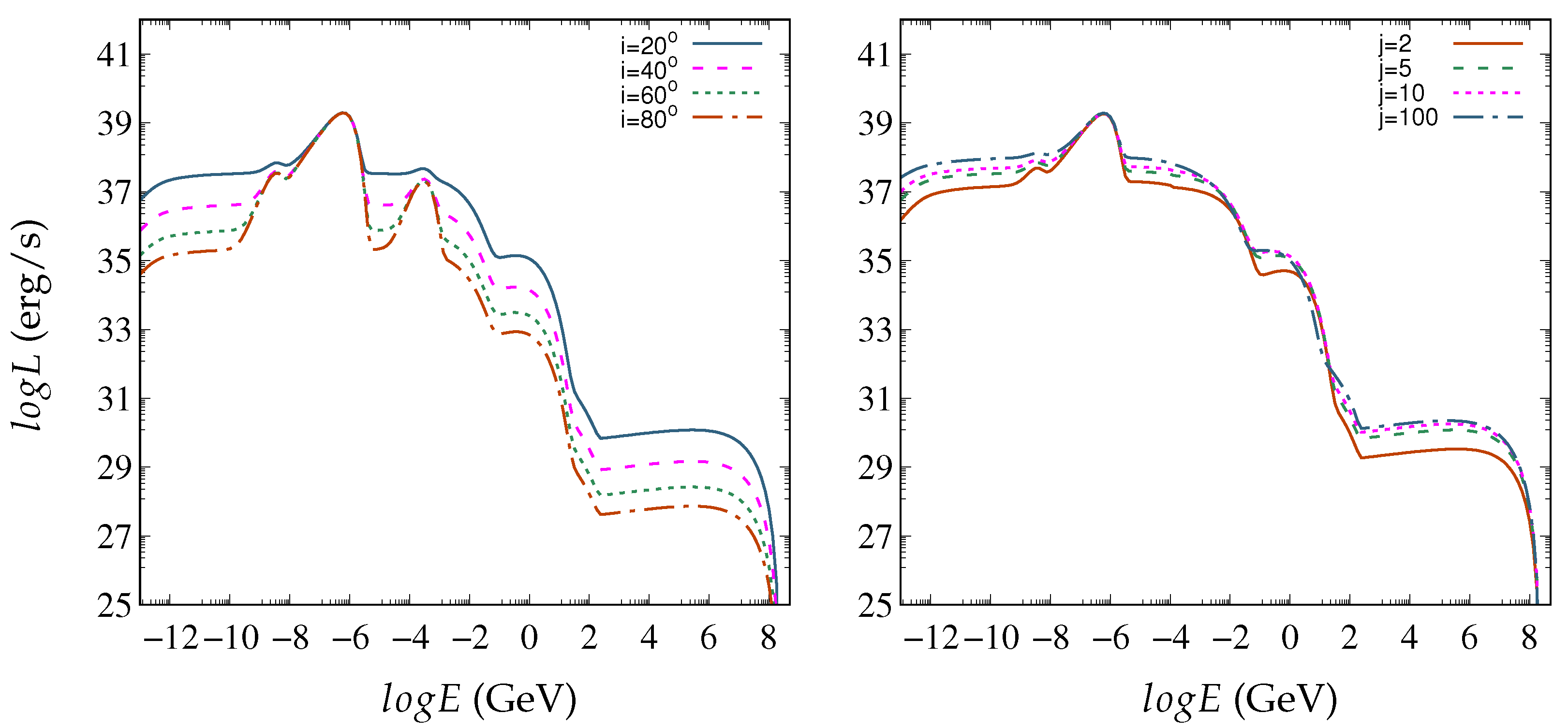
5. Results and Discussion
6. Summary and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Blandford, R.D.; Znajek, R.L. Electromagnetic extraction of energy from Kerr black holes. Mon. Not. R. Astron. Soc 1977, 179, 433–456. [Google Scholar] [CrossRef]
- Blumenthal, G.R.; Gould, R.J. Bremsstrahlung, Synchrotron Radiation, and Compton Scattering of High-Energy Electrons Traversing Dilute Gases. Rev. Mod. Phys. 1970, 42, 237–271. [Google Scholar] [CrossRef]
- Atoyan, A.M.; Aharonian, F.A. Modelling of the non-thermal flares in the Galactic microquasar GRS 1915+105. Mon. Not. R. Astron. Soc. 1999, 302, 253–276. [Google Scholar] [CrossRef]
- Markoff, S.; Falcke, H.; Fender, R. A jet model for the broadband spectrum of XTE J1118+480—Synchrotron emission from radio to X-rays in the Low/Hard spectral state. Astron. Astrophys. 2001, 372, L25–L28. [Google Scholar] [CrossRef]
- Bosch-Ramon, V.; Romero, G.E.; Paredes, J.M. A broadband leptonic model for gamma-ray emitting microquasars. Astron. Astrophys. 2006, 447, 263–276. [Google Scholar] [CrossRef]
- Khangulyan, D.; Aharonian, F.; Bosch-Ramon, V. On the formation of TeV radiation in LS 5039. Mon. Not. R. Astron. Soc. 2007, 383, 467–478. [Google Scholar] [CrossRef]
- Smponias, T.; Kosmas, T.S. Dynamical and radiative simulations of γ-ray jets in microquasars. Mon. Not. R. Astron. Soc. 2013, 438, 1014–1026. [Google Scholar] [CrossRef]
- Kosmas, O.; Smponias, T. Simulations of Gamma-Ray Emission from Magnetized Microquasar Jets. Adv. High Energy Phys. 2018, 2018, 9602960. [Google Scholar] [CrossRef]
- Romero, G.E.; Torres, D.F.; Kaufman Bernadó, M.M.; Mirabel, I.F. Hadronic gamma-ray emission from windy microquasars. Astron. Astrophys. 2003, 410, L1–L4. [Google Scholar] [CrossRef]
- Romero, G.E.; Okazaki, A.T.; Orellana, M.; Owocki, S.P. Accretion vs. colliding wind models for the gamma-ray binary LS I +61 303: An assessment. Astron. Astrophys. 2007, 474, 15–22. [Google Scholar] [CrossRef]
- Reynoso, M.M.; Romero, G.E. Magnetic field effects on neutrino production in microquasars. Astron. Astrophys. 2009, 493, 1–11. [Google Scholar] [CrossRef]
- King, A.R. Accretion rates and beaming in ultraluminous X-ray sources. Mon. Not. R. Astron. Soc. Lett. 2008, 385, L113–L115. [Google Scholar] [CrossRef]
- Abramowicz, M.A.; Fragile, P.C. Foundations of Black Hole Accretion Disk Theory. Living Rev. Relativ. 2013, 16, 1. [Google Scholar] [CrossRef]
- Shakura, N.I.; Sunyaev, R.A. Black holes in binary systems. Observational appearance. Astron. Astrophys. 1973, 24, 337–355. [Google Scholar]
- Narayan, R.; Yi, I. Advection-dominated Accretion: Underfed Black Holes and Neutron Stars. Astrophys. J. 1995, 452, 710. [Google Scholar] [CrossRef]
- Bardeen, J.M.; Press, W.H.; Teukolsky, S.A. Rotating Black Holes: Locally Nonrotating Frames, Energy Extraction, and Scalar Synchrotron Radiation. Astrophys. J. 1972, 178, 347–370. [Google Scholar] [CrossRef]
- Papavasileiou, T.; Kosmas, O.; Sinatkas, I. Simulations of Neutrino and Gamma-Ray Production from Relativistic Black-Hole Microquasar Jets. Galaxies 2021, 9, 67. [Google Scholar] [CrossRef]
- Papavasileiou, T.; Kosmas, O.; Sinatkas, I. Relativistic Magnetized Astrophysical Plasma Outflows in Black-Hole Microquasars. Symmetry 2022, 14, 485. [Google Scholar] [CrossRef]
- Papavasileiou, T.V.; Papadopoulos, D.A.; Kosmas, T.S. Astrophysical magnetohydrodynamical outflows in the extragalactic binary system LMC X-1. J. Phys. Conf. Ser. 2021, 1730, 012138. [Google Scholar] [CrossRef]
- Abdo, A.A.; Ackermann, M.; Ajello, M.; Atwood, W.B.; Axelsson, M.; Baldini, L.; Ballet, J.; Barbiellini, G.; Bastieri, D.; Baughman, B.M.; et al. Fermi/LAT observations of LS 5039. Astrophys. J. 2009, 706, L56. [Google Scholar] [CrossRef]
- Pepe, C.; Vila, G.S.; Romero, G.E. Lepto-hadronic model for the broadband emission of Cygnus X-1. Astron. Astrophys. 2015, 584, A95. [Google Scholar] [CrossRef]
- Pringle, J.E. Accretion discs in astrophysics. Annu. Rev. Astron. Astrophys. 1981, 19, 137–162. [Google Scholar] [CrossRef]
- Cerutti, B.; Dubus, G.; Malzac, J.; Szostek, A.; Belmont, R.; Zdziarski, A.A.; Henri, G. Absorption of high-energy gamma rays in Cygnus X-3. Astron. Astrophys. 2011, 529, A120. [Google Scholar] [CrossRef]
- Gould, R.G.; Schréder, G.P. Pair Profuction in Photon-Photon Collisions. Phys. Rev. 1967, 155, 1404. [Google Scholar] [CrossRef]
- Böttcher, M.; Dermer, C.D. Photon-Photon Absorption of Very High Energy Gamma Rays from Microquasars: Application to LS 5039. Astrophys. J. 2005, 634, L81–L84. [Google Scholar] [CrossRef]
- Reynoso, M.M.; Carulli, A.M. On the possibilities of high-energy neutrino production in the jets of microquasar SS433 in light of new observational data. Astropart. Phys. 2019, 109, 25–32. [Google Scholar] [CrossRef]
- Gliozzi, M.; Bodo, G.; Ghisellini, G. The bulk kinetic power of the jets of GRS 1915+105. Mon. Not. R. Astron. Soc. 1999, 303, L37–L40. [Google Scholar] [CrossRef]
- Cao, X.; Lai, D. Jet production in black hole X-ray binaries and active galactic nuclei: Mass feeding and advection of magnetic fields. Mon. Not. R. Astron. Soc. 2019, 485, 1916–1923. [Google Scholar] [CrossRef]
- Kelner, S.R.; Aharonian, F.A.; Bugayov, V.V. Energy spectra of gamma rays, electrons, and neutrinos produced at proton-proton interactions in the very high energy regime. Phys. Rev. D 2006, 74, 034018. [Google Scholar] [CrossRef]
- Fender, R.P.; Pooley, G.G.; Durouchoux, P.; Tilanus, R.P.J.; Brocksopp, C. The very flat radio-millimetre spectrum of Cygnus X-1. Mon. Not. R. Astron. Soc. 2000, 312, 853–858. [Google Scholar] [CrossRef]
- Heinz, S.; Begelman, M.C. Analysis of the Synchrotron Emission from the M87 Jet. Astrophys. J. 1997, 490, 653. [Google Scholar] [CrossRef]
- Shang, H.; Lizano, S.; Glassgold, A.; Shu, F. Free-free Radio Emission from Young Stellar Objects. Astrophys. J. 2004, 612, L69–L72. [Google Scholar] [CrossRef]
- Ohmura, T.; Machida, M.; Nakamura, K.; Kudoh, Y.; Asahina, Y.; Matsumoto, R. Two-Temperature Magnetohydrodynamics Simulations of Propagation of Semi-Relativistic Jets. Galaxies 2019, 7, 14. [Google Scholar] [CrossRef]
- Albert, J.; Aliu, E.; Anderhub, H.; Antoranz, P.; Armada, A.; Asensio, M.; Baixeras, C.; Barrio, J.A.; Bartelt, M.; Bartko, H.; et al. Observation of Gamma Rays from the Galactic Center with the MAGIC Telescope. Astrophys. J. 2006, 638, L101. [Google Scholar] [CrossRef]
- Bolmont, J.; Corona, P.; Gauron, P.; Ghislain, P.; Goffin, C.; Guevara Riveros, L.; Huppert, J.F.; Martineau-Huynh, O.; Nayman, P.; Parraud, J.M.; et al. The camera of the fifth H.E.S.S. telescope. Part I: System description. Nucl. Instrum. Methods Phys. Res. A 2014, 761, 46–57. [Google Scholar] [CrossRef]
- Acero, F.; Ackermann, M.; Ajello, M.; Albert, A.; Atwood, W.B.; Axelsson, M.; Baldini, L.; Ballet, J.; Barbiellini, G.; Bastieri, D.; et al. Fermi large area telescope third source catalog. Astrophys. J. Suppl. Ser. 2015, 218, 23. [Google Scholar] [CrossRef]
- Acciari, V.A.; Aliu, E.; Arlen, T.; Aune, T.; Beilicke, M.; Benbow, W.; Bradbury, S.M.; Buckley, J.H.; Bugaev, V.; Byrum, K.; et al. Veritas observations of the TeV binary LS I +61° 303 during 2008–2010. Astrophys. J. 2011, 738, 3. [Google Scholar] [CrossRef]
- Vila, G.S.; Romero, G.E.; Casco, N.A. An inhomogeneous lepto-hadronic model for the radiation of relativistic jets—Application to XTE J1118+480. Astron. Astrophys. 2012, 538, A97. [Google Scholar] [CrossRef]
- Kantzas, D.; Markoff, S.; Beuchert, T.; Lucchini, M.; Chhotray, A.; Ceccobello, C.; Tetarenko, A.J.; Miller-Jones, J.C.A.; Bremer, M.; Garcia, J.A.; et al. A new lepto-hadronic model applied to the first simultaneous multiwavelength data set for Cygnus X–1. Mon. Not. R. Astron. Soc. 2020, 500, 2112–2126. [Google Scholar] [CrossRef]
- Papavasileiou, T.V.; Kosmas, O.T.; Sinatkas, I. Prediction of gamma-ray emission from Cygnus X-1, SS 433, and GRS 1915+105 after absorption. Astron. Astophys. 2023, 673, A162. [Google Scholar] [CrossRef]
- Schüssler, F.; Bordas, P.; Chadwick, P.M.; Dickinson, H.; Ernenwein, J.P. Simultaneous H.E.S.S. and RXTE observations of the microquasars GRS 1915+105, Circinus X-1 and V4641 Sgr. arXiv 2015, arXiv:1509.03039. [Google Scholar] [CrossRef]
- Ahnen, M.; Ansoldi, S.; Antonelli, L.; Arcaro, C. Search for very high-energy gamma-ray emission from the microquasar Cygnus X-1 with the MAGIC telescopes. Mon. Not. R. Astron. Soc. 2017, 472, 3474–3485. [Google Scholar] [CrossRef]
- Ahnen, M.L.; Ansoldi, S.; Antonelli, L.A.; Arcaro, C.; Babic, A.; Banerjee, B.; Bangale, P.; Barres de Almeida, U.; Barrio, J.A.; Becerra González, J.; et al. Constraints on particle acceleration in SS433/W50 from MAGIC and H.E.S.S. observations. Astron. Astrophys. 2018, 612, A14. [Google Scholar] [CrossRef]
- Maitra, D.; Markoff, S.; Brocksopp, C.; Noble, M.; Nowak, M.; Wilms, J. Constraining jet/disc geometry and radiative processes in stellar black holes XTE J1118+480 and GX 339–4. Mon. Not. R. Astron. Soc. 2009, 398, 1638–1650. [Google Scholar] [CrossRef]
- Esin, A.A.; McClintock, J.E.; Narayan, R. Advection-Dominated Accretion and the Spectral States of Black Hole X-Ray Binaries: Application to Nova Muscae 1991. Astrophys. J. 1997, 489, 865. [Google Scholar] [CrossRef]
- Gierliński, M.; Zdziarski, A.A.; Poutanen, J.; Coppi, P.S.; Ebisawa, K.; Johnson, W.N. Radiation mechanisms and geometry of Cygnus X-1 in the soft state. Month. Not. R. Astron. Soc. 1999, 309, 496–512. [Google Scholar] [CrossRef]
- Done, C.; Gierliński, M.; Kubota, A. Modelling the behaviour of accretion flows in X-ray binaries. Astron. Astrophys. Rev. 2007, 15, 1–66. [Google Scholar] [CrossRef]
- Makishima, K.; Takahashi, H.; Yamada, S.; Done, C.; Kubota, A.; Dotani, T.; Ebisawa, K.; Itoh, T.; Kitamoto, S.; Negoro, H.; et al. Suzaku Results on Cygnus X-1 in the Low/Hard State. Publ. Astron. Soc. Jpn. 2008, 60, 585–604. [Google Scholar] [CrossRef]


| Free Parameter | Symbol | Value | Units |
|---|---|---|---|
| Acceleration efficiency | - | ||
| Jet portion of relativistic matter | - | ||
| Hadron to lepton ratio | 1 | - | |
| Acceleration zone length factor | j | 5 | - |
| Acceleration region start | cm | ||
| XRB Parameter | Symbol | Value | Units |
| Black hole mass | 10 | ||
| Secondary stellar mass | 20 | ||
| Distance to Earth | d | 2 | kpc |
| Orbital period | 6 | days | |
| Stellar luminosity | |||
| Stellar temperature | K | ||
| Initial secondary stellar phase | 0 | ||
| XRB inclination | i | 20 | |
| Jet bulk velocity | c | ||
| Jet Lorentz factor | - | ||
| Magnetic field strength | G | ||
| Jet’s half-opening angle | 2 | ||
| Mass accretion rate | /yr | ||
| Disk maximum temperature | K | ||
| Disk innermost radius | cm | ||
| Disk outermost radius | cm | ||
| Binary separation | s | cm |
| Parameter | Case A | Case B |
|---|---|---|
| 100 | ||
| j | 100 | 2 |
| i |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Papavasileiou, T.; Kosmas, O.; Sinatkas, I. Studying the Spectral Energy Distributions Emanating from Regular Galactic XRBs. Universe 2023, 9, 312. https://doi.org/10.3390/universe9070312
Papavasileiou T, Kosmas O, Sinatkas I. Studying the Spectral Energy Distributions Emanating from Regular Galactic XRBs. Universe. 2023; 9(7):312. https://doi.org/10.3390/universe9070312
Chicago/Turabian StylePapavasileiou, Theodora, Odysseas Kosmas, and Ioannis Sinatkas. 2023. "Studying the Spectral Energy Distributions Emanating from Regular Galactic XRBs" Universe 9, no. 7: 312. https://doi.org/10.3390/universe9070312
APA StylePapavasileiou, T., Kosmas, O., & Sinatkas, I. (2023). Studying the Spectral Energy Distributions Emanating from Regular Galactic XRBs. Universe, 9(7), 312. https://doi.org/10.3390/universe9070312






