Inflation and Primordial Black Holes
Abstract
1. Introduction
- Primordial black holes: history of the concept
- The structure of this review
- Conventions
2. PBH Formation in the Early Universe
2.1. PBH Formation as a Causal Process
2.2. The Relevant Quantities for PBH Abundance
- The threshold for collapse
- The mass of PBHs
- PBH abundance
- Collapse fraction of PBHs at formation
2.3. Relating PBH Properties to Primordial Scalar Fluctuations
- Collapse fraction vs. curvature perturbation
- The case of non-Gaussian curvature fluctuations
2.4. Brief Summary and the Path Ahead
3. Enhancement of Scalar Perturbations during Single-Field Inflation
3.1. The Dynamics of Curvature Perturbation
3.2. Enhancement through the Resurrection of the Decaying Mode
- The idea
- Model building parameterization of the non-attractor phase
- Phase I. The initial phase of inflationary evolution is characterized by a standard slow-roll (SR) regime, where and at the pivot scale () (assuming that modes at the pivot scale exit the horizon at the beginning of evolution ()) in order to match Planck observations [4];
- Phase II. As the scalar field starts to traverse the flat plateau-like region in its potential, its dynamics eventually enter the non-attractor era, lasting a given number of e-folds of evolution. This phase is characterized by a large negative , during which the first slow-roll parameter () decays exponentially:
- Phase III. The final phase of evolution ensures a graceful exit from the non-attractor phase into a final slow-roll epoch, leading to the end of inflation. Since decays quickly in the non-attractor era, this final phase is characterized by a hierarchy between the slow-roll parameters:where . We typically require a large positive to bring back from its tiny values at the end of the non-attractor era, towards the value () needed to conclude inflation. To capture this behavior accurately, we split the final phase of evolution into two parts, parameterizing asThe relevant parameter choices to model the dynamics can be found in the third column of Table 2.We note that our choice of in the initial stage of the Phase III and in Phase II is not a coincidence; in most of the single-field modes, there exists a correspondence that relates the values in Phase II and Phase III, i.e., , which is a consequence of Wands’ duality [218]. We will elaborate the consequence of this correspondence in the context of the power spectrum below, in particular for modes that exit the horizon as the background evolves from Phase II to Phase III.
- Numerical analysis
- The features of the spectrum: analytic considerations
- Stochastic inflation and quantum diffusion
3.3. Growth in the Power Spectrum When the Decaying Modes Are Slacking
- Slow-roll violation without triggering decaying modes
- An explicit realization
3.4. Brief Summary
4. Enhanced Primordial Power Spectrum in Multifield Models
Main References: In the discussion presented in Section 4.1, we benefit from the works presented in [79,80,81,82,85,86], while the material presented in Section 4.2 is based on [72,73].
4.1. Enhanced Scalar Perturbations from Axion-Gauge Field Dynamics
- Amplification of gauge field fluctuations
- 1.
- Section 4.1.1 As a first possibility, we identify the axion () with that drives inflation: . Then, the order parameter () controlling the gauge-field production increases over time, generating scalar perturbations through an inverse vector decay: . We refer to this scenario as “Smooth Axion Inflation”; models that can be considered in this category are studied in [79,80,81,82]. Such scenarios suffer from dynamical instabilities associated with large back-reaction effects from the gauge fields on the axion evolution.
- 2.
- Section 4.1.2 In certain axion-inflation models, the axion potential has special features located far away from the field range corresponding to scales affecting CMB physics. A sudden increase in the axion inflation velocity occurs at their location, with enhanced scalar perturbations amplified at small PBH scales through inverse vector decay. This possibility was first discussed in [81], while further developments were studied in [83,86]. We refer to scenarios producing PBHs by exploiting localized particle production as “Bumpy Axion Inflation”, and we analyze how they address the instabilities mentioned above.
- 3.
- Section 4.1.3 A final possibility corresponds to gauge field production in a hidden sector through the dynamics of an axion spectator field. This case also allows one to address the aforementioned dynamical instabilities, given that the back-reaction effects from the vector sector can be placed under control. Depending on the shape of the spectator axion potential, such a scenario can lead to localized peaks in the scalar curvature power spectrum [81,85]. We refer to this scenario as “Spectator axion-gauge field dynamics”.
4.1.1. Smooth Axion Inflation
- Background evolution in a concrete example
- Scalar fluctuations sourced by gauge fields
- Cautionary remarks: an instability in the inflation dynamics
4.1.2. Bumpy Axion Inflation
4.1.3. Spectator Axion-Gauge Field Dynamics
4.2. Strong Turns in the Multiscalar Field Space
- Broad turns
- Sudden turns
5. Outlook
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Background Cosmology: A Mini Review
Appendix B. A Simple Estimate for the Threshold of Collapse
Appendix C. Solving the Mukhanov–Sasaki Equation: Numerical Procedure
Appendix D. Details of the Axion-Gauge Field Dynamics
Appendix D.1. Gauge Field Production by Rolling Scalars
Appendix D.2. Scalars Sourced by Vector Fields and the Direct Coupling Case: χ=ϕ
Appendix D.3. Scalars Sourced by Vector Fields and the Indirect Coupling Case: χ = σ
Appendix E. Curvature Perturbation
| 1 | See, e.g., the impact of extended mass functions on the PBH abundance constraints [27]. |
| 2 | |
| 3 | |
| 4 | PBHs could also form in the post-inflationary universe through the collapse of cosmic strings [106,107,108] and domain walls [109,110,111,112], phase transitions [113,114], bubble collisions [115,116] or scalar-field fragmentation via instabilities [117,118]. We note that PBH formation in the post-inflationary universe can be triggered by bubble nucleation during inflation or instabilities generated in the final stage of inflation commonly referred as (p)reheating [119,120,121,122]. We will not dwell on these possibilities here; for a partial list of recent works in this line of research, see [123,124,125]. |
| 5 | A detailed discussion on these topics can be found in Chapter 4 of Baumann’s book [128]. |
| 6 | Throughout this work, we assume an efficient reheating process at the end of inflation such that the universe becomes radiation-dominated shortly after inflation terminates. For a collage of interesting physics that might arise through the reheating stage and alternative post-inflationary histories, see the recent review [131]. |
| 7 | A simple analytic argument that leads to this result is presented in Appendix B. |
| 8 | |
| 9 | Strictly speaking, is only satisfied when species are in thermal equilibrium at the same temperature. For a comprehensive overview of the thermal history of the universe after inflation, see Chapter 3 of [128]. |
| 10 | We note that another common convention is to count e-folds with respect to the end of inflation, denoting the end point as . |
| 11 | In contrast to the original approach by Press and Schechter [145], we do not take into account a symmetry factor of two on the right-hand side of (13), which accounts for all the mass in the universe, since it is not clear whether such a factor makes sense when considering non-symmetric PDFs of (e.g., non-Gaussian cases). Furthermore, the error introduced by omitting this factor is comparable to the other uncertainties in the computation of , such as the fraction of the horizon mass that collapses to form a PBH (see, e.g., [146,147,148]). |
| 12 | |
| 13 | |
| 14 | Note that the power spectrum is the variance of curvature perturbation per logarithmic interval in k, i.e., . Therefore, the approximate signs (≃) in the expressions in (26) and (30) can be turned into an equality if we consider the values defined in those expressions as the collapse fraction per in the spectrum, namely . |
| 15 | One can also introduce a time-dependent mass in the action (35) which may arise through broken spatial translations as in solid [179] and supersolid [180,181] inflation. Another possibility is to include higher derivative terms in the quadratic action to modify the dispersion relation of curvature perturbation (see, e.g., [182]) as in ghost inflation [183]. We will not consider these possibilities here. For a discussion of PBH formation in solid and ghost inflation, see Sections 4 and 6 of [64,184]. |
| 16 | In fact, if the time evolution of the pump field is known, up to second order in the gradient expansion, we can generate a solution for the curvature perturbation by replacing in the last integral of Equation (39) with the leading growing-mode solution of the homogeneous part of Equation (38), which we can identify as . |
| 17 | In particular, the standard decaying mode is given by the last term in (39), as it decays slowly, i.e., , compared to the second term. |
| 18 | Here, we assume that field rolls down on its potential from large to small values () with before it encounters the feature required for the enhancement. Since during the feature, there must be a point in the potential where the second derivative of the field vanishes (). |
| 19 | |
| 20 | Note that evaluating the power spectrum at the end of inflation is necessary when modes evolve outside the horizon, as in the example background we are focusing on in this section. |
| 21 | In fact, the general procedure outlined in Appendix C can be generalized to accurately solve the Mukhanov–Sasaki equation using a broad class of single-field models of inflation. In the context of phenomenological models we discuss in this and the next section, jupyter notebook files that compute the power spectrum is are available at https://github.com/oozsoy/SingleFieldINF_Powerspec_PBH (accessed on 24 January 2023). We acknowledge the use of the following Python libraries: matplotlib [219], numpy [220], scipy [221], pandas [222] along with jupyter notebooks [223]. |
| 22 | The distinct behavior of the cosmological correlators around the dip feature may also be probed by the 21 cm signal of the Hydrogen atom [230]. |
| 23 | Notice that an exponential tail in the PDF of curvature fluctuations, similar to the tailarising in the context of a stochastic approach to inflation, has been recently shown [256] to be a property of all single-field models with potential up to quadratic. Such a feature is physically caused by the logarithmic relation between curvature fluctuation and the (Gaussian) inflation field fluctuations (see [256] for details). |
| 24 | Notice that if the background dynamics have localized features, phases of slow-roll violation might occur for some time interval, and this exact identification ceases to be valid. In particular, in this case, might lose its monotonic nature for some time interval, leading to multiple horizon crossings for a range of modes, resulting in oscillations of the spectrum [64]. For the time being, we set a discussion on this possibility aside and continue identifying the quantity as an effective horizon for the slow-roll violating scenarios we are interested in. |
| 25 | Some other notable models we do not cover in this context utilize (i) dissipative dynamics as in warm inflation-type scenarios discussed in [264,265,266], (ii) the conversion of resonantly produced spectator fluctuations to curvature perturbation during [75] or after inflation, e.g., in curvaton-type scenarios discussed in [267,268]. |
| 26 | |
| 27 | Be aware that despite the terminology we adopt, the gauge fields () do not need to correspond to Standard Model photons. |
| 28 | At large CMB scales described by modes leaving the horizon in the early stages of inflation, the value of the effective coupling () is restricted by existing information and constraints on the scalar power spectrum and non-Gaussianity [282,283,288]. Depending on priors and on the shape of the inflation potential, these constraints give [289]. |
| 29 | In fact, the potential (78) is not unique for the purpose of generating a localized particle production scenario. In this context, any potential that exhibits (a) step-like feature(s) is sufficient (see e.g., [83,296]. However, the choice of the potential in (78) does provide some useful analytic control over the background evolution of the axion and the resulting amplification of the power spectrum, as discussed in [86]. |
| 30 | As discussed in [86], this assumption is actually self-consistent due to the localized nature of the particle production. |
| 31 | |
| 32 | In this work, by an appropriate choice of initial conditions and model parameters, we assume that traverses two step-like features on its potential before it settles to its global minimum. |
| 33 | The roll of towards the global minimum can be captured by modifying the monomial term as so that the axion potential (85) interpolates between and from large to small field () values, respectively. |
| 34 | For M2, this translates into a single step-like region in the potential (see Figure 15). |
| 35 | In the comoving gauge with which we are operating, the field fluctuations are proportional to entropy fluctuation (), while the spatial part of the metric takes the form . |
| 36 | Recall from our discussion above that entropic mass crossing at corresponds to the peak of the power spectrum. |
| 37 | |
| 38 | More realistic models of the turn are expected to have a smooth time dependence of such as the Gaussian profile we considered earlier. As shown by the numerical evaluations in [312], the choice of top-hat profile does not introduce any qualitative difference but appears to be a convenient choice for analytic manipulations. For a detailed study of sudden turn trajectories in conjunction with PBH formation, see [315]. |
| 39 | We also note that the presence of very light entropy mode (for the case) leads to a non-negligible contribution to the power spectrum for scales that cross the horizon before the turn, i.e., for . In particular, the analytic expression (101) does not perform well on these scales. For a more complete analytic formula including numerical analysis, see [312]. |
| 40 | Note that for flat spatial hypersurfaces (), we can define new coordinates as , and to turn the metric into a form that is commonly used in the literature (). |
| 41 | Indeed, CMB data inform us that the spatial geometry of our universe is flat on large cosmological scales (see (A18)). On the other hand, since it dilutes slowly () compared to radiation and matter, we would expect it to dominate the energy density before DED, but this did not happen. This implies that the initial value of the curvature must either be tuned to be extremely small or that it should relax to small values through a dynamical mechanism. Inflation could be also a solution to this puzzle because during such an exponential expansion, any initially large curvature would be inflated away. |
| 42 | The red-shift parameter can be defined as the fractional shift in the physical wavelength () of a photon emitted at a distant point and time (t) in the universe until today, i.e., . |
| 43 | Unless modes undergo resonance and are excited deep inside the horizon, the choice of initial conditions in Equations (A34) and (A35) provide an accurate prescription for the initialization of the numerical evaluation. For the models we consider in Section 3.2 and Section 3.3, this is indeed the case. For a model that leads to excited states inside the horizon, see [328,329]. |
| 44 | We note that at linear order in fluctuations, the Coulomb gauge condition () is equivalent to setting the temporal component of the gauge field to zero, i.e., . This can be seen by explicitly expanding the gauge field action (A42) to second order in and , then solving for the non-dynamical mode (see also the discussion in Section 3 of [331]). |
| 45 | This regime is not interesting from a phenomenological point of view, as the gauge field production will be very weak in this case. |
| 46 | We note that also takes up a contribution bilinear in gauge fields proportional to the absolute value of the Poynting vector (). We expect this contribution to be negligible at the end of inflation (i.e., at the time for we are interested in the correlators of ) because the gauge field production saturates on superhorizon scales, and the corresponding electromagnetic fields decay as . See, e.g., the discussion presented in [85,339] within similar contexts. |
References
- Guth, A.H. The Inflationary Universe: A Possible Solution to the Horizon and Flatness Problems. Phys. Rev. 1981, D23, 347–356. [Google Scholar] [CrossRef]
- Linde, A.D. A New Inflationary Universe Scenario: A Possible Solution of the Horizon, Flatness, Homogeneity, Isotropy and Primordial Monopole Problems. Phys. Lett. 1982, B108, 389–393. [Google Scholar] [CrossRef]
- Penzias, A.A.; Wilson, R.W. A Measurement of excess antenna temperature at 4080-Mc/s. Astrophys. J. 1965, 142, 419–421. [Google Scholar] [CrossRef]
- Akrami, Y.; Arroja, F.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Ballardini, M.; Banday, A.J.; Barreiro, R.B.; Bartolo, N.; Basak, S.; et al. Planck 2018 results. X. Constraints on inflation. Astron. Astrophys. 2020, 641, A10. [Google Scholar] [CrossRef]
- Akrami, Y.; Arroja, F.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Ballardini, M.; Banday, A.J.; Barreiro, R.B.; Bartolo, N.; Basak, S.; et al. Planck 2018 results. IX. Constraints on primordial non-Gaussianity. Astron. Astrophys. 2020, 641, A9. [Google Scholar] [CrossRef]
- Bertone, G.; Hooper, D. History of dark matter. Rev. Mod. Phys. 2018, 90, 045002. [Google Scholar] [CrossRef]
- Aghanim, N.; Arroja, F.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Ballardini, M.; Banday, A.J.; Barreiro, R.B.; Bartolo, N.; Basak, S.; et al. Planck 2018 results. VI. Cosmological parameters. Astron. Astrophys. 2021, 641, A6, Erratum in Astron. Astrophys. 2021, 652, C4. [Google Scholar] [CrossRef]
- Bergström, L. Nonbaryonic dark matter: Observational evidence and detection methods. Rep. Prog. Phys. 2000, 63, 793. [Google Scholar] [CrossRef]
- Bertone, G.; Hooper, D.; Silk, J. Particle dark matter: Evidence, candidates and constraints. Phys. Rep. 2005, 405, 279–390. [Google Scholar] [CrossRef]
- Zel’dovich, Y.B.; Novikov, I.D. The Hypothesis of Cores Retarded during Expansion and the Hot Cosmological Model. Sov. Astron. AJ (Engl. Transl.) 1967, 10, 602. [Google Scholar]
- Hawking, S. Gravitationally collapsed objects of very low mass. Mon. Not. R. Astron. Soc. 1971, 152, 75. [Google Scholar] [CrossRef]
- Carr, B.J.; Hawking, S.W. Black holes in the early Universe. Mon. Not. R. Astron. Soc. 1974, 168, 399–415. [Google Scholar] [CrossRef]
- Carr, B.J. The Primordial black hole mass spectrum. Astrophys. J. 1975, 201, 1–19. [Google Scholar] [CrossRef]
- Chapline, G.F. Cosmological effects of primordial black holes. Nature 1975, 253, 251–252. [Google Scholar] [CrossRef]
- Carr, B.J.; Rees, M.J. Can pregalactic objects generate galaxies? Mon. Not. R. Astron. Soc. 1984, 206, 801–818. [Google Scholar] [CrossRef]
- Aubourg, E.; Bareyre, P.; Brehin, S.; Gros, M.; Lachieze-Rey, M.; Laurent, B.; Lesquoy, E.; Magneville, C.; Milsztajn, A.; Moscoso, L.; et al. Evidence for gravitational microlensing by dark objects in the galactic halo. Nature 1993, 365, 623–625. [Google Scholar] [CrossRef]
- Tisserand, P.; Le Guillou, L.; Afonso, C.; Albert, J.N.; Andersen, J.; Ansari, R.; Aubourg, É.; Bareyre, P.; Beaulieu, J.P.; Charlot, X.; et al. Limits on the Macho Content of the Galactic Halo from the EROS-2 Survey of the Magellanic Clouds. Astron. Astrophys. 2007, 469, 387–404. [Google Scholar] [CrossRef]
- Wyrzykowski, L.; Kozlowski, S.; Skowron, J.; Udalski, A.; Szymanski, M.K.; Kubiak, M.; Pietrzynski, G.; Soszynski, I.; Szewczyk, O.; Ulaczyk, K.; et al. The OGLE View of Microlensing towards the Magellanic Clouds. III. Ruling out sub-solar MACHOs with the OGLE-III LMC data. Mon. Not. R. Astron. Soc. 2011, 413, 493. [Google Scholar] [CrossRef]
- Wyrzykowski, L.; Kozlowski, S.; Skowron, J.; Udalski, A.; Szymanski, M.K.; Kubiak, M.; Pietrzynski, G.; Soszynski, I.; Szewczyk, O.; Ulaczyk, K.; et al. The OGLE View of Microlensing towards the Magellanic Clouds. IV. OGLE-III SMC Data and Final Conclusions on MACHOs. Mon. Not. R. Astron. Soc. 2011, 416, 2949. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abernathy, M.R.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; et al. Observation of Gravitational Waves from a Binary Black Hole Merger. Phys. Rev. Lett. 2016, 116, 061102. [Google Scholar] [CrossRef]
- Bird, S.; Cholis, I.; Muñoz, J.B.; Ali-Haïmoud, Y.; Kamionkowski, M.; Kovetz, E.D.; Raccanelli, A.; Riess, A.G. Did LIGO detect dark matter? Phys. Rev. Lett. 2016, 116, 201301. [Google Scholar] [CrossRef] [PubMed]
- Clesse, S.; García-Bellido, J. The clustering of massive Primordial Black Holes as Dark Matter: Measuring their mass distribution with Advanced LIGO. Phys. Dark Univ. 2017, 15, 142–147. [Google Scholar] [CrossRef]
- Sasaki, M.; Suyama, T.; Tanaka, T.; Yokoyama, S. Primordial Black Hole Scenario for the Gravitational-Wave Event GW150914. Phys. Rev. Lett. 2018, 117, 061101, Erratum in Phys. Rev. Lett. 2018, 121, 059901. [Google Scholar] [CrossRef]
- Niikura, H.; Takada, M.; Yasuda, N.; Lupton, R.H.; Sumi, T.; More, S.; Kurita, T.; Sugiyama, S.; More, A.; Oguri, M.; et al. Microlensing constraints on primordial black holes with Subaru/HSC Andromeda observations. Nat. Astron. 2019, 3, 524–534. [Google Scholar] [CrossRef]
- Katz, A.; Kopp, J.; Sibiryakov, S.; Xue, W. Femtolensing by Dark Matter Revisited. J. Cosmol. Astropart. Phys. 2018, 2018, 005. [Google Scholar] [CrossRef]
- Montero-Camacho, P.; Fang, X.; Vasquez, G.; Silva, M.; Hirata, C.M. Revisiting constraints on asteroid-mass primordial black holes as dark matter candidates. J. Cosmol. Astropart. Phys. 2019, 2019, 031. [Google Scholar] [CrossRef]
- Carr, B.; Raidal, M.; Tenkanen, T.; Vaskonen, V.; Veermäe, H. Primordial black hole constraints for extended mass functions. Phys. Rev. D 2017, 96, 023514. [Google Scholar] [CrossRef]
- Khlopov, M.Y. Primordial Black Holes. Res. Astron. Astrophys. 2010, 10, 495–528. [Google Scholar] [CrossRef]
- García-Bellido, J. Massive Primordial Black Holes as Dark Matter and their detection with Gravitational Waves. J. Phys. Conf. Ser. 2017, 840, 012032. [Google Scholar] [CrossRef]
- Sasaki, M.; Suyama, T.; Tanaka, T.; Yokoyama, S. Primordial black holes: Perspectives in gravitational wave astronomy. Class. Quant. Grav. 2018, 35, 063001. [Google Scholar] [CrossRef]
- Carr, B.; Kuhnel, F. Primordial Black Holes as Dark Matter: Recent Developments. Ann. Rev. Nucl. Part. Sci. 2020, 70, 355–394. [Google Scholar] [CrossRef]
- Carr, B.; Kohri, K.; Sendouda, Y.; Yokoyama, J. Constraints on primordial black holes. Rep. Prog. Phys. 2021, 84, 116902. [Google Scholar] [CrossRef]
- Green, A.M.; Kavanagh, B.J. Primordial Black Holes as a dark matter candidate. J. Phys. G 2021, 48, 043001. [Google Scholar] [CrossRef]
- Escrivà, A.; Kuhnel, F.; Tada, Y. Primordial Black Holes. arXiv 2022, arXiv:2211.05767. [Google Scholar]
- Page, D.N. Particle Emission Rates from a Black Hole: Massless Particles from an Uncharged, Nonrotating Hole. Phys. Rev. D 1976, 13, 198–206. [Google Scholar] [CrossRef]
- Papanikolaou, T.; Vennin, V.; Langlois, D. Gravitational waves from a universe filled with primordial black holes. J. Cosmol. Astropart. Phys. 2021, 2021, 053. [Google Scholar] [CrossRef]
- Papanikolaou, T. Gravitational waves induced from primordial black hole fluctuations: The effect of an extended mass function. J. Cosmol. Astropart. Phys. 2022, 2022, 089. [Google Scholar] [CrossRef]
- Ivanov, P.; Naselsky, P.; Novikov, I. Inflation and primordial black holes as dark matter. Phys. Rev. 1994, D50, 7173–7178. [Google Scholar] [CrossRef]
- Starobinsky, A.A. Spectrum of adiabatic perturbations in the universe when there are singularities in the inflation potential. JETP Lett. 1992, 55, 489–494. [Google Scholar]
- Dimopoulos, K. Ultra slow-roll inflation demystified. Phys. Lett. 2017, B775, 262–265. [Google Scholar] [CrossRef]
- Leach, S.M.; Liddle, A.R. Inflationary perturbations near horizon crossing. Phys. Rev. 2001, D63, 043508. [Google Scholar] [CrossRef]
- Leach, S.M.; Sasaki, M.; Wands, D.; Liddle, A.R. Enhancement of superhorizon scale inflationary curvature perturbations. Phys. Rev. 2001, D64, 023512. [Google Scholar] [CrossRef]
- Kinney, W.H. Horizon crossing and inflation with large eta. Phys. Rev. 2005, D72, 023515. [Google Scholar] [CrossRef]
- Martin, J.; Motohashi, H.; Suyama, T. Ultra Slow-Roll Inflation and the non-Gaussianity Consistency Relation. Phys. Rev. 2013, D87, 023514. [Google Scholar] [CrossRef]
- Motohashi, H.; Starobinsky, A.A.; Yokoyama, J. Inflation with a constant rate of roll. J. Cosmol. Astropart. Phys. 2015, 2015, 018. [Google Scholar] [CrossRef]
- Garcia-Bellido, J.; Ruiz Morales, E. Primordial black holes from single field models of inflation. Phys. Dark Univ. 2017, 18, 47–54. [Google Scholar] [CrossRef]
- Ezquiaga, J.M.; Garcia-Bellido, J.; Ruiz Morales, E. Primordial Black Hole production in Critical Higgs Inflation. Phys. Lett. B 2018, 776, 345–349. [Google Scholar] [CrossRef]
- Germani, C.; Prokopec, T. On primordial black holes from an inflection point. Phys. Dark Univ. 2017, 18, 6–10. [Google Scholar] [CrossRef]
- Ballesteros, G.; Taoso, M. Primordial black hole dark matter from single field inflation. Phys. Rev. 2018, D97, 023501. [Google Scholar] [CrossRef]
- Hertzberg, M.P.; Yamada, M. Primordial Black Holes from Polynomial Potentials in Single Field Inflation. Phys. Rev. D 2017, 97, 083509. [Google Scholar] [CrossRef]
- Cicoli, M.; Diaz, V.A.; Pedro, F.G. Primordial Black Holes from String Inflation. J. Cosmol. Astropart. Phys. 2018, 2018, 034. [Google Scholar] [CrossRef]
- Özsoy, O.; Parameswaran, S.; Tasinato, G.; Zavala, I. Mechanisms for Primordial Black Hole Production in String Theory. J. Cosmol. Astropart. Phys. 2018, 2018, 005. [Google Scholar] [CrossRef]
- Mishra, S.S.; Sahni, V. Primordial Black Holes from a tiny bump/dip in the inflation potential. J. Cosmol. Astropart. Phys. 2020, 2020, 007. [Google Scholar] [CrossRef]
- Dimopoulos, K.; Markkanen, T.; Racioppi, A.; Vaskonen, V. Primordial Black Holes from Thermal Inflation. J. Cosmol. Astropart. Phys. 2019, 2019, 046. [Google Scholar] [CrossRef]
- Ballesteros, G.; Rey, J.; Taoso, M.; Urbano, A. Primordial black holes as dark matter and gravitational waves from single-field polynomial inflation. J. Cosmol. Astropart. Phys. 2020, 2020, 025. [Google Scholar] [CrossRef]
- Inomata, K.; McDonough, E.; Hu, W. Amplification of primordial perturbations from the rise or fall of the inflation. J. Cosmol. Astropart. Phys. 2022, 2022, 031. [Google Scholar] [CrossRef]
- Garcia-Bellido, J.; Linde, A.D.; Wands, D. Density perturbations and black hole formation in hybrid inflation. Phys. Rev. D 1996, 54, 6040–6058. [Google Scholar] [CrossRef]
- Kawasaki, M.; Sugiyama, N.; Yanagida, T. Primordial black hole formation in a double inflation model in supergravity. Phys. Rev. D 1998, 57, 6050–6056. [Google Scholar] [CrossRef]
- Yokoyama, J. Chaotic new inflation and formation of primordial black holes. Phys. Rev. D 1998, 58, 083510. [Google Scholar] [CrossRef]
- Kawaguchi, T.; Kawasaki, M.; Takayama, T.; Yamaguchi, M.; Yokoyama, J. Formation of intermediate-mass black holes as primordial black holes in the inflationary cosmology with running spectral index. Mon. Not. R. Astron. Soc. 2008, 388, 1426–1432. [Google Scholar] [CrossRef]
- Kohri, K.; Lyth, D.H.; Melchiorri, A. Black hole formation and slow-roll inflation. J. Cosmol. Astropart. Phys. 2008, 2008, 038. [Google Scholar] [CrossRef]
- Frampton, P.H.; Kawasaki, M.; Takahashi, F.; Yanagida, T.T. Primordial Black Holes as All Dark Matter. J. Cosmol. Astropart. Phys. 2010, 2010, 023. [Google Scholar] [CrossRef]
- Drees, M.; Erfani, E. Running Spectral Index and Formation of Primordial Black Hole in Single Field Inflation Models. J. Cosmol. Astropart. Phys. 2012, 2012, 035. [Google Scholar] [CrossRef]
- Ballesteros, G.; Beltran Jimenez, J.; Pieroni, M. Black hole formation from a general quadratic action for inflationary primordial fluctuations. J. Cosmol. Astropart. Phys. 2019, 2019, 016. [Google Scholar] [CrossRef]
- Kamenshchik, A.Y.; Tronconi, A.; Venturi, G. DBI inflation and warped black holes. J. Cosmol. Astropart. Phys. 2022, 2022, 051. [Google Scholar] [CrossRef]
- Cai, Y.F.; Tong, X.; Wang, D.G.; Yan, S.F. Primordial Black Holes from Sound Speed Resonance during Inflation. Phys. Rev. Lett. 2018, 121, 081306. [Google Scholar] [CrossRef]
- Chen, C.; Ma, X.H.; Cai, Y.F. Dirac-Born-Infeld realization of sound speed resonance mechanism for primordial black holes. Phys. Rev. D 2020, 102, 063526. [Google Scholar] [CrossRef]
- Baumann, D.; McAllister, L. Inflation and String Theory; Cambridge Monographs on Mathematical Physics, Cambridge University Press: Cambridge, UK, 2015. [Google Scholar] [CrossRef]
- Randall, L.; Soljacic, M.; Guth, A.H. Supernatural inflation: Inflation from supersymmetry with no (very) small parameters. Nucl. Phys. B 1996, 472, 377–408. [Google Scholar] [CrossRef]
- Clesse, S.; García-Bellido, J. Massive Primordial Black Holes from Hybrid Inflation as Dark Matter and the seeds of Galaxies. Phys. Rev. D 2015, 92, 023524. [Google Scholar] [CrossRef]
- Brown, A.R. Hyperbolic Inflation. Phys. Rev. Lett. 2018, 121, 251601. [Google Scholar] [CrossRef] [PubMed]
- Palma, G.A.; Sypsas, S.; Zenteno, C. Seeding primordial black holes in multifield inflation. Phys. Rev. Lett. 2020, 125, 121301. [Google Scholar] [CrossRef]
- Fumagalli, J.; Renaux-Petel, S.; Ronayne, J.W.; Witkowski, L.T. Turning in the landscape: A new mechanism for generating Primordial Black Holes. arXiv 2020, arXiv:2004.08369. [Google Scholar] [CrossRef]
- Braglia, M.; Hazra, D.K.; Finelli, F.; Smoot, G.F.; Sriramkumar, L.; Starobinsky, A.A. Generating PBHs and small-scale GWs in two-field models of inflation. J. Cosmol. Astropart. Phys. 2020, 2020, 001. [Google Scholar] [CrossRef]
- Zhou, Z.; Jiang, J.; Cai, Y.F.; Sasaki, M.; Pi, S. Primordial black holes and gravitational waves from resonant amplification during inflation. Phys. Rev. D 2020, 102, 103527. [Google Scholar] [CrossRef]
- Iacconi, L.; Assadullahi, H.; Fasiello, M.; Wands, D. Revisiting small-scale fluctuations in α-attractor models of inflation. J. Cosmol. Astropart. Phys. 2021, 2022, 007. [Google Scholar] [CrossRef]
- Kallosh, R.; Linde, A. Dilaton-Axion Inflation with PBHs and GWs. J. Cosmol. Astropart. Phys. 2022, 2022, 037. [Google Scholar] [CrossRef]
- Kawai, S.; Kim, J. Primordial black holes and gravitational waves from nonminimally coupled supergravity inflation. Phys. Rev. 2022, 107, 043523. [Google Scholar] [CrossRef]
- Linde, A.; Mooij, S.; Pajer, E. Gauge field production in supergravity inflation: Local non-Gaussianity and primordial black holes. Phys. Rev. D 2013, 87, 103506. [Google Scholar] [CrossRef]
- Bugaev, E.; Klimai, P. Axion inflation with gauge field production and primordial black holes. Phys. Rev. D 2014, 90, 103501. [Google Scholar] [CrossRef]
- Garcia-Bellido, J.; Peloso, M.; Unal, C. Gravitational waves at interferometer scales and primordial black holes in axion inflation. J. Cosmol. Astropart. Phys. 2016, 2016, 031. [Google Scholar] [CrossRef]
- Domcke, V.; Muia, F.; Pieroni, M.; Witkowski, L.T. PBH dark matter from axion inflation. J. Cosmol. Astropart. Phys. 2017, 2017, 048. [Google Scholar] [CrossRef]
- Cheng, S.L.; Lee, W.; Ng, K.W. Primordial black holes and associated gravitational waves in axion monodromy inflation. J. Cosmol. Astropart. Phys. 2018, 2018, 001. [Google Scholar] [CrossRef]
- Kawasaki, M.; Nakatsuka, H.; Obata, I. Generation of Primordial Black Holes and Gravitational Waves from Dilaton-Gauge Field Dynamics. J. Cosmol. Astropart. Phys. 2020, 2020, 007. [Google Scholar] [CrossRef]
- Özsoy, O. Synthetic Gravitational Waves from a Rolling Axion Monodromy. J. Cosmol. Astropart. Phys. 2021, 2021, 040. [Google Scholar] [CrossRef]
- Özsoy, O.; Lalak, Z. Primordial black holes as dark matter and gravitational waves from bumpy axion inflation. J. Cosmol. Astropart. Phys. 2021, 2021, 040. [Google Scholar] [CrossRef]
- Ananda, K.N.; Clarkson, C.; Wands, D. The Cosmological gravitational wave background from primordial density perturbations. Phys. Rev. 2007, D75, 123518. [Google Scholar] [CrossRef]
- Baumann, D.; Steinhardt, P.J.; Takahashi, K.; Ichiki, K. Gravitational Wave Spectrum Induced by Primordial Scalar Perturbations. Phys. Rev. 2007, D76, 084019. [Google Scholar] [CrossRef]
- Kohri, K.; Terada, T. Semianalytic calculation of gravitational wave spectrum nonlinearly induced from primordial curvature perturbations. Phys. Rev. 2018, D97, 123532. [Google Scholar] [CrossRef]
- Nakama, T.; Silk, J.; Kamionkowski, M. Stochastic gravitational waves associated with the formation of primordial black holes. Phys. Rev. D 2017, 95, 043511. [Google Scholar] [CrossRef]
- Cai, R.G.; Pi, S.; Sasaki, M. Gravitational Waves Induced by non-Gaussian Scalar Perturbations. Phys. Rev. Lett. 2019, 122, 201101. [Google Scholar] [CrossRef] [PubMed]
- Unal, C. Imprints of Primordial Non-Gaussianity on Gravitational Wave Spectrum. Phys. Rev. D 2019, 99, 041301. [Google Scholar] [CrossRef]
- Yuan, C.; Chen, Z.C.; Huang, Q.G. Log-dependent slope of scalar induced gravitational waves in the infrared regions. Phys. Rev. D 2020, 101, 043019. [Google Scholar] [CrossRef]
- Cai, R.G.; Pi, S.; Sasaki, M. Universal infrared scaling of gravitational wave background spectra. Phys. Rev. D 2020, 102, 083528. [Google Scholar] [CrossRef]
- Özsoy, O.; Tasinato, G. On the slope of the curvature power spectrum in non-attractor inflation. J. Cosmol. Astropart. Phys. 2020, 2020, 048. [Google Scholar] [CrossRef]
- Pi, S.; Sasaki, M. Gravitational Waves Induced by Scalar Perturbations with a Lognormal Peak. J. Cosmol. Astropart. Phys. 2020, 2020, 037. [Google Scholar] [CrossRef]
- Yuan, C.; Huang, Q.G. Gravitational waves induced by the local-type non-Gaussian curvature perturbations. Phys. Lett. B 2021, 821, 136606. [Google Scholar] [CrossRef]
- Ragavendra, H.V. Accounting for scalar non-Gaussianity in secondary gravitational waves. Phys. Rev. D 2022, 105, 063533. [Google Scholar] [CrossRef]
- Amaro-Seoane, P.; Audley, H.; Babak, S.; Baker, J.; Barausse, E.; Bender, P.; Berti, E.; Binetruy, P.; Born, M.; Bortoluzzi, D.; et al. Laser Interferometer Space Antenna. arXiv 2017, arXiv:1702.00786. [Google Scholar]
- Barausse, E.; Berti, E.; Hertog, T.; Hughes, S.A.; Jetzer, P.; Pani, P.; Sotiriou, T.P.; Tamanini, N.; Witek, H.; Yagi, K.; et al. Prospects for Fundamental Physics with LISA. Gen. Relat. Grav. 2020, 52, 81. [Google Scholar] [CrossRef]
- Lentati, L.; Taylor, S.R.; Mingarelli, C.M.; Sesana, A.; Sanidas, S.A.; Vecchio, A.; Caballero, R.N.; Lee, K.J.; Van Haasteren, R.; Babak, S.; et al. European Pulsar Timing Array Limits On An Isotropic Stochastic Gravitational-Wave Background. Mon. Not. R. Astron. Soc. 2015, 453, 2576–2598. [Google Scholar] [CrossRef]
- Arzoumanian, Z.; Baker, P.T.; Blumer, H.; Bécsy, B.; Brazier, A.; Brook, P.R.; Burke-Spolaor, S.; Chatterjee, S.; Chen, S.; Cordes, J.M.; et al. The NANOGrav 12.5 yr Data Set: Search for an Isotropic Stochastic Gravitational-wave Background. Astrophys. J. Lett. 2020, 905, L34. [Google Scholar] [CrossRef]
- Seto, N.; Kawamura, S.; Nakamura, T. Possibility of direct measurement of the acceleration of the universe using 0.1-Hz band laser interferometer gravitational wave antenna in space. Phys. Rev. Lett. 2001, 87, 221103. [Google Scholar] [CrossRef] [PubMed]
- Kawamura, S.; Ando, M.; Seto, N.; Sato, S.; Musha, M.; Kawano, I.; Yokoyama, J.I.; Tanaka, T.; Ioka, K.; Akutsu, T.; et al. Current status of space gravitational wave antenna DECIGO and B-DECIGO. Prog. Theor. Exp. Phys. 2021, 2021, 05A105. [Google Scholar] [CrossRef]
- Domènech, G. Scalar Induced Gravitational Waves Review. Universe 2021, 7, 398. [Google Scholar] [CrossRef]
- Polnarev, A.; Zembowicz, R. Formation of Primordial Black Holes by Cosmic Strings. Phys. Rev. D 1991, 43, 1106–1109. [Google Scholar] [CrossRef] [PubMed]
- Caldwell, R.R.; Casper, P. Formation of black holes from collapsed cosmic string loops. Phys. Rev. D 1996, 53, 3002–3010. [Google Scholar] [CrossRef]
- Helfer, T.; Aurrekoetxea, J.C.; Lim, E.A. Cosmic String Loop Collapse in Full General Relativity. Phys. Rev. D 2019, 99, 104028. [Google Scholar] [CrossRef]
- Rubin, S.G.; Khlopov, M.Y.; Sakharov, A.S. Primordial black holes from nonequilibrium second order phase transition. Grav. Cosmol. 2000, 6, 51–58. [Google Scholar]
- Garriga, J.; Vilenkin, A.; Zhang, J. Black holes and the multiverse. J. Cosmol. Astropart. Phys. 2016, 2016, 064. [Google Scholar] [CrossRef]
- Deng, H.; Garriga, J.; Vilenkin, A. Primordial black hole and wormhole formation by domain walls. J. Cosmol. Astropart. Phys. 2017, 2017, 050. [Google Scholar] [CrossRef]
- Kusenko, A.; Sasaki, M.; Sugiyama, S.; Takada, M.; Takhistov, V.; Vitagliano, E. Exploring Primordial Black Holes from the Multiverse with Optical Telescopes. Phys. Rev. Lett. 2020, 125, 181304. [Google Scholar] [CrossRef] [PubMed]
- Kodama, H.; Sasaki, M.; Sato, K. Abundance of Primordial Holes Produced by Cosmological First Order Phase Transition. Prog. Theor. Phys. 1982, 68, 1979. [Google Scholar] [CrossRef]
- Jedamzik, K. Primordial black hole formation during the QCD epoch. Phys. Rev. D 1997, 55, 5871–5875. [Google Scholar] [CrossRef]
- Moss, I.G. Singularity formation from colliding bubbles. Phys. Rev. D 1994, 50, 676–681. [Google Scholar] [CrossRef]
- Kitajima, N.; Takahashi, F. Primordial Black Holes from QCD Axion Bubbles. J. Cosmol. Astropart. Phys. 2020, 2020, 060. [Google Scholar] [CrossRef]
- Cotner, E.; Kusenko, A. Primordial black holes from supersymmetry in the early universe. Phys. Rev. Lett. 2017, 119, 031103. [Google Scholar] [CrossRef]
- Cotner, E.; Kusenko, A.; Sasaki, M.; Takhistov, V. Analytic Description of Primordial Black Hole Formation from Scalar Field Fragmentation. J. Cosmol. Astropart. Phys. 2019, 2019, 077. [Google Scholar] [CrossRef]
- Kofman, L.; Linde, A.D.; Starobinsky, A.A. Reheating after inflation. Phys. Rev. Lett. 1994, 73, 3195–3198. [Google Scholar] [CrossRef]
- Shtanov, Y.; Traschen, J.H.; Brandenberger, R.H. Universe reheating after inflation. Phys. Rev. D 1995, 51, 5438–5455. [Google Scholar] [CrossRef]
- Kofman, L.; Linde, A.D.; Starobinsky, A.A. Towards the theory of reheating after inflation. Phys. Rev. 1997, D56, 3258–3295. [Google Scholar] [CrossRef]
- Amin, M.A.; Hertzberg, M.P.; Kaiser, D.I.; Karouby, J. Nonperturbative Dynamics Of Reheating After Inflation: A Review. Int. J. Mod. Phys. D 2014, 24, 1530003. [Google Scholar] [CrossRef]
- Ashoorioon, A.; Rostami, A.; Firouzjaee, J.T. Examining the end of inflation with primordial black holes mass distribution and gravitational waves. Phys. Rev. D 2021, 103, 123512. [Google Scholar] [CrossRef]
- Martin, J.; Papanikolaou, T.; Vennin, V. Primordial black holes from the preheating instability in single-field inflation. J. Cosmol. Astropart. Phys. 2020, 2020, 024. [Google Scholar] [CrossRef]
- Auclair, P.; Vennin, V. Primordial black holes from metric preheating: Mass fraction in the excursion-set approach. J. Cosmol. Astropart. Phys. 2021, 2021, 038. [Google Scholar] [CrossRef]
- Byrnes, C.T.; Cole, P.S. Lecture notes on inflation and primordial black holes. arXiv 2021, arXiv:2112.05716. [Google Scholar]
- Franciolini, G. Primordial Black Holes: From Theory to Gravitational Wave Observations. Ph.D. Thesis, University of Geneva, Department of Theoretical Physics, Geneva, Switzerland, 2021. [Google Scholar] [CrossRef]
- Baumann, D. Cosmology; Cambridge University Press: Cambridge, UK, 2022. [Google Scholar] [CrossRef]
- Sasaki, M. Large Scale Quantum Fluctuations in the Inflationary Universe. Prog. Theor. Phys. 1986, 76, 1036. [Google Scholar] [CrossRef]
- Mukhanov, V.F.; Feldman, H.A.; Brandenberger, R.H. Theory of cosmological perturbations. Part 1. Classical perturbations. Part 2. Quantum theory of perturbations. Part 3. Extensions. Phys. Rep. 1992, 215, 203–333. [Google Scholar] [CrossRef]
- Allahverdi, R.; Amin, M.A.; Berlin, A.; Bernal, N.; Byrnes, C.T.; Delos, M.S.; Erickcek, A.L.; Escudero, M.; Figueroa, D.G.; Freese, K.; et al. The First Three Seconds: A Review of Possible Expansion Histories of the Early Universe. arXiv 2020, arXiv:2006.16182. [Google Scholar] [CrossRef]
- Harada, T.; Yoo, C.M.; Kohri, K. Threshold of primordial black hole formation. Phys. Rev. 2014, D88, 084051, Erratum in Phys. Rev. 2014, D89, 029903. [Google Scholar] [CrossRef]
- Niemeyer, J.C.; Jedamzik, K. Dynamics of primordial black hole formation. Phys. Rev. D 1999, 59, 124013. [Google Scholar] [CrossRef]
- Nakama, T.; Harada, T.; Polnarev, A.G.; Yokoyama, J. Identifying the most crucial parameters of the initial curvature profile for primordial black hole formation. J. Cosmol. Astropart. Phys. 2014, 2014, 037. [Google Scholar] [CrossRef]
- Musco, I. Threshold for primordial black holes: Dependence on the shape of the cosmological perturbations. Phys. Rev. D 2019, 100, 123524. [Google Scholar] [CrossRef]
- Musco, I.; De Luca, V.; Franciolini, G.; Riotto, A. Threshold for primordial black holes. II. A simple analytic prescription. Phys. Rev. D 2021, 103, 063538. [Google Scholar] [CrossRef]
- Musco, I.; Papanikolaou, T. Primordial black hole formation for an anisotropic perfect fluid: Initial conditions and estimation of the threshold. Phys. Rev. D 2022, 106, 083017. [Google Scholar] [CrossRef]
- Papanikolaou, T. Toward the primordial black hole formation threshold in a time-dependent equation-of-state background. Phys. Rev. D 2022, 105, 124055. [Google Scholar] [CrossRef]
- Shibata, M.; Sasaki, M. Black hole formation in the Friedmann universe: Formulation and computation in numerical relativity. Phys. Rev. D 1999, 60, 084002. [Google Scholar] [CrossRef]
- Germani, C.; Musco, I. Abundance of Primordial Black Holes Depends on the Shape of the Inflationary Power Spectrum. Phys. Rev. Lett. 2019, 122, 141302. [Google Scholar] [CrossRef] [PubMed]
- Escrivà, A. PBH Formation from Spherically Symmetric Hydrodynamical Perturbations: A Review. Universe 2022, 8, 66. [Google Scholar] [CrossRef]
- Escrivà, A.; Germani, C.; Sheth, R.K. Universal threshold for primordial black hole formation. Phys. Rev. D 2020, 101, 044022. [Google Scholar] [CrossRef]
- Escrivà, A.; Germani, C.; Sheth, R.K. Analytical thresholds for black hole formation in general cosmological backgrounds. J. Cosmol. Astropart. Phys. 2021, 2021, 030. [Google Scholar] [CrossRef]
- Akiyama, K.; Alberdi, A.; Alef, W.; Algaba, J.C.; Anantua, R.; Asada, K.; Azulay, R.; Bach, U.; Baczko, A.K.; Ball, D.; et al. First Sagittarius A* Event Horizon Telescope Results. I. The Shadow of the Supermassive Black Hole in the Center of the Milky Way. Astrophys. J. Lett. 2022, 930, L12. [Google Scholar] [CrossRef]
- Press, W.H.; Schechter, P. Formation of galaxies and clusters of galaxies by selfsimilar gravitational condensation. Astrophys. J. 1974, 187, 425–438. [Google Scholar] [CrossRef]
- Evans, C.R.; Coleman, J.S. Observation of critical phenomena and selfsimilarity in the gravitational collapse of radiation fluid. Phys. Rev. Lett. 1994, 72, 1782–1785. [Google Scholar] [CrossRef] [PubMed]
- Niemeyer, J.C.; Jedamzik, K. Near-critical gravitational collapse and the initial mass function of primordial black holes. Phys. Rev. Lett. 1998, 80, 5481–5484. [Google Scholar] [CrossRef]
- Koike, T.; Hara, T.; Adachi, S. Critical behavior in gravitational collapse of radiation fluid: A Renormalization group (linear perturbation) analysis. Phys. Rev. Lett. 1995, 74, 5170–5173. [Google Scholar] [CrossRef]
- Germani, C.; Sheth, R.K. Nonlinear statistics of primordial black holes from Gaussian curvature perturbations. Phys. Rev. D 2020, 101, 063520. [Google Scholar] [CrossRef]
- De Luca, V.; Franciolini, G.; Kehagias, A.; Peloso, M.; Riotto, A.; Ünal, C. The Ineludible non-Gaussianity of the Primordial Black Hole Abundance. J. Cosmol. Astropart. Phys. 2019, 2019, 048. [Google Scholar] [CrossRef]
- Young, S.; Musco, I.; Byrnes, C.T. Primordial black hole formation and abundance: Contribution from the non-linear relation between the density and curvature perturbation. J. Cosmol. Astropart. Phys. 2019, 2019, 012. [Google Scholar] [CrossRef]
- Young, S.; Byrnes, C.T.; Sasaki, M. Calculating the mass fraction of primordial black holes. J. Cosmol. Astropart. Phys. 2014, 2014, 045. [Google Scholar] [CrossRef]
- Byrnes, C.T.; Copeland, E.J.; Green, A.M. Primordial black holes as a tool for constraining non-Gaussianity. Phys. Rev. 2012, D86, 043512. [Google Scholar] [CrossRef]
- Young, S.; Byrnes, C.T. Primordial black holes in non-Gaussian regimes. J. Cosmol. Astropart. Phys. 2013, 2013, 052. [Google Scholar] [CrossRef]
- Passaglia, S.; Hu, W.; Motohashi, H. Primordial black holes and local non-Gaussianity in canonical inflation. Phys. Rev. D 2019, 99, 043536. [Google Scholar] [CrossRef]
- Biagetti, M.; De Luca, V.; Franciolini, G.; Kehagias, A.; Riotto, A. The formation probability of primordial black holes. Phys. Lett. B 2021, 820, 136602. [Google Scholar] [CrossRef]
- Atal, V.; Germani, C. The role of non-gaussianities in Primordial Black Hole formation. Phys. Dark Univ. 2019, 24, 100275. [Google Scholar] [CrossRef]
- Taoso, M.; Urbano, A. Non-gaussianities for primordial black hole formation. J. Cosmol. Astropart. Phys. 2021, 2021, 016. [Google Scholar] [CrossRef]
- Young, S. Peaks and primordial black holes: The effect of non-Gaussianity. J. Cosmol. Astropart. Phys. 2022, 2022, 037. [Google Scholar] [CrossRef]
- Ferrante, G.; Franciolini, G.; Iovino, A.J.; Urbano, A. Primordial non-gaussianity up to all orders: Theoretical aspects and implications for primordial black hole models. Phys. Rev. D 2023, 107, 043520. [Google Scholar] [CrossRef]
- Gow, A.D.; Assadullahi, H.; Jackson, J.H.P.; Koyama, K.; Vennin, V.; Wands, D. Non-perturbative non-Gaussianity and primordial black holes. arXiv 2022, arXiv:2211.08348. [Google Scholar]
- Lyth, D.H. The hybrid inflation waterfall and the primordial curvature perturbation. J. Cosmol. Astropart. Phys. 2012, 2012, 022. [Google Scholar] [CrossRef]
- Musco, I.; Miller, J.C. Primordial black hole formation in the early universe: Critical behaviour and self-similarity. Class. Quant. Grav. 2013, 30, 145009. [Google Scholar] [CrossRef]
- Byrnes, C.T.; Hindmarsh, M.; Young, S.; Hawkins, M.R.S. Primordial black holes with an accurate QCD equation of state. J. Cosmol. Astropart. Phys. 2018, 2018, 041. [Google Scholar] [CrossRef]
- Carr, B.; Clesse, S.; García-Bellido, J.; Kühnel, F. Cosmic conundra explained by thermal history and primordial black holes. Phys. Dark Univ. 2021, 31, 100755. [Google Scholar] [CrossRef]
- Ali-Haïmoud, Y. Correlation Function of High-Threshold Regions and Application to the Initial Small-Scale Clustering of Primordial Black Holes. Phys. Rev. Lett. 2018, 121, 081304. [Google Scholar] [CrossRef]
- Young, S.; Byrnes, C.T. Initial clustering and the primordial black hole merger rate. J. Cosmol. Astropart. Phys. 2020, 2020, 004. [Google Scholar] [CrossRef]
- De Luca, V.; Desjacques, V.; Franciolini, G.; Riotto, A. The clustering evolution of primordial black holes. J. Cosmol. Astropart. Phys. 2020, 2020, 028. [Google Scholar] [CrossRef]
- De Luca, V.; Franciolini, G.; Riotto, A.; Veermäe, H. Ruling Out Initially Clustered Primordial Black Holes as Dark Matter. Phys. Rev. Lett. 2022, 129, 191302. [Google Scholar] [CrossRef]
- Ali-Haïmoud, Y.; Kovetz, E.D.; Kamionkowski, M. Merger rate of primordial black-hole binaries. Phys. Rev. D 2017, 96, 123523. [Google Scholar] [CrossRef]
- Ballesteros, G.; Serpico, P.D.; Taoso, M. On the merger rate of primordial black holes: Effects of nearest neighbours distribution and clustering. J. Cosmol. Astropart. Phys. 2018, 2018, 043. [Google Scholar] [CrossRef]
- Vaskonen, V.; Veermäe, H. Lower bound on the primordial black hole merger rate. Phys. Rev. D 2020, 101, 043015. [Google Scholar] [CrossRef]
- De Luca, V.; Franciolini, G.; Riotto, A. On the Primordial Black Hole Mass Function for Broad Spectra. Phys. Lett. B 2020, 807, 135550. [Google Scholar] [CrossRef]
- Moradinezhad Dizgah, A.; Franciolini, G.; Riotto, A. Primordial Black Holes from Broad Spectra: Abundance and Clustering. J. Cosmol. Astropart. Phys. 2019, 2019, 001. [Google Scholar] [CrossRef]
- De Luca, V.; Riotto, A. A note on the abundance of primordial black holes: Use and misuse of the metric curvature perturbation. Phys. Lett. B 2022, 828, 137035. [Google Scholar] [CrossRef]
- Young, S. Constraining the Early Universe with Primordial Black Holes. Ph.D. Thesis, University of Sussex, Sussex, UK, 2016. [Google Scholar]
- Garcia-Bellido, J.; Peloso, M.; Unal, C. Gravitational Wave signatures of inflationary models from Primordial Black Hole Dark Matter. J. Cosmol. Astropart. Phys. 2017, 2017, 013. [Google Scholar] [CrossRef]
- Lyth, D.H.; Riotto, A. Particle physics models of inflation and the cosmological density perturbation. Phys. Rep. 1999, 314, 1–146. [Google Scholar] [CrossRef]
- Endlich, S.; Nicolis, A.; Wang, J. Solid Inflation. J. Cosmol. Astropart. Phys. 2013, 2013, 011. [Google Scholar] [CrossRef]
- Cannone, D.; Tasinato, G.; Wands, D. Generalised tensor fluctuations and inflation. J. Cosmol. Astropart. Phys. 2015, 2015, 029. [Google Scholar] [CrossRef]
- Bartolo, N.; Cannone, D.; Ricciardone, A.; Tasinato, G. Distinctive signatures of space-time diffeomorphism breaking in EFT of inflation. J. Cosmol. Astropart. Phys. 2016, 2016, 044. [Google Scholar] [CrossRef]
- Ashoorioon, A.; Rostami, A.; Firouzjaee, J.T. EFT compatible PBHs: Effective spawning of the seeds for primordial black holes during inflation. J. High Energy Phys. 2021, 2021, 087. [Google Scholar] [CrossRef]
- Arkani-Hamed, N.; Creminelli, P.; Mukohyama, S.; Zaldarriaga, M. Ghost inflation. J. Cosmol. Astropart. Phys. 2004, 2004, 001. [Google Scholar] [CrossRef]
- Ballesteros, G.; Céspedes, S.; Santoni, L. Large power spectrum and primordial black holes in the effective theory of inflation. J. High Energy Phys. 2022, 2022, 074. [Google Scholar] [CrossRef]
- Drees, M.; Xu, Y. Overshooting, Critical Higgs Inflation and Second Order Gravitational Wave Signatures. Eur. Phys. J. C 2021, 81, 182. [Google Scholar] [CrossRef]
- Cheong, D.Y.; Lee, S.M.; Park, S.C. Primordial black holes in Higgs-R2 inflation as the whole of dark matter. J. Cosmol. Astropart. Phys. 2021, 2021, 032. [Google Scholar] [CrossRef]
- Rasanen, S.; Tomberg, E. Planck scale black hole dark matter from Higgs inflation. J. Cosmol. Astropart. Phys. 2019, 2019, 038. [Google Scholar] [CrossRef]
- Dalianis, I.; Kehagias, A.; Tringas, G. Primordial black holes from α-attractors. J. Cosmol. Astropart. Phys. 2019, 2019, 037. [Google Scholar] [CrossRef]
- Dalianis, I.; Tringas, G. Primordial black hole remnants as dark matter produced in thermal, matter, and runaway-quintessence postinflationary scenarios. Phys. Rev. D 2019, 100, 083512. [Google Scholar] [CrossRef]
- Cicoli, M.; Pedro, F.G.; Pedron, N. Secondary GWs and PBHs in string inflation: Formation and detectability. J. Cosmol. Astropart. Phys. 2022, 2022, 030. [Google Scholar] [CrossRef]
- Armendariz-Picon, C.; Damour, T.; Mukhanov, V.F. k-inflation. Phys. Lett. B 1999, 458, 209–218. [Google Scholar] [CrossRef]
- Garriga, J.; Mukhanov, V.F. Perturbations in k-inflation. Phys. Lett. 1999, B458, 219–225. [Google Scholar] [CrossRef]
- Solbi, M.; Karami, K. Primordial black holes and induced gravitational waves in k-inflation. J. Cosmol. Astropart. Phys. 2021, 2021, 056. [Google Scholar] [CrossRef]
- Solbi, M.; Karami, K. Primordial black holes formation in the inflationary model with field-dependent kinetic term for quartic and natural potentials. Eur. Phys. J. C 2021, 81, 884. [Google Scholar] [CrossRef]
- Teimoori, Z.; Rezazadeh, K.; Rasheed, M.A.; Karami, K. Mechanism of primordial black holes production and secondary gravitational waves in α-attractor Galileon inflationary scenario. J. Cosmol. Astropart. Phys. 2021, 2021, 018. [Google Scholar] [CrossRef]
- Ahmed, W.; Junaid, M.; Zubair, U. Primordial black holes and gravitational waves in hybrid inflation with chaotic potentials. Nucl. Phys. B 2022, 984, 115968. [Google Scholar] [CrossRef]
- Kamenshchik, A.Y.; Tronconi, A.; Vardanyan, T.; Venturi, G. Non-Canonical Inflation and Primordial Black Holes Production. Phys. Lett. B 2019, 791, 201–205. [Google Scholar] [CrossRef]
- Horndeski, G.W. Second-order scalar-tensor field equations in a four-dimensional space. Int. J. Theor. Phys. 1974, 10, 363–384. [Google Scholar] [CrossRef]
- Kobayashi, T.; Yamaguchi, M.; Yokoyama, J. Generalized G-inflation: Inflation with the most general second-order field equations. Prog. Theor. Phys. 2011, 126, 511–529. [Google Scholar] [CrossRef]
- Frolovsky, D.; Ketov, S.V.; Saburov, S. Formation of primordial black holes after Starobinsky inflation. Mod. Phys. Lett. A 2022, 37, 2250135. [Google Scholar] [CrossRef]
- Fu, C.; Wu, P.; Yu, H. Primordial Black Holes from Inflation with Nonminimal Derivative Coupling. Phys. Rev. D 2019, 100, 063532. [Google Scholar] [CrossRef]
- Heydari, S.; Karami, K. Primordial black holes in nonminimal derivative coupling inflation with quartic potential and reheating consideration. Eur. Phys. J. C 2022, 82, 83. [Google Scholar] [CrossRef]
- Kawai, S.; Kim, J. Primordial black holes from Gauss-Bonnet-corrected single field inflation. Phys. Rev. D 2021, 104, 083545. [Google Scholar] [CrossRef]
- Langlois, D.; Noui, K. Degenerate higher derivative theories beyond Horndeski: Evading the Ostrogradski instability. J. Cosmol. Astropart. Phys. 2016, 2016, 034. [Google Scholar] [CrossRef]
- Crisostomi, M.; Koyama, K.; Tasinato, G. Extended Scalar-Tensor Theories of Gravity. J. Cosmol. Astropart. Phys. 2016, 2016, 044. [Google Scholar] [CrossRef]
- Ben Achour, J.; Crisostomi, M.; Koyama, K.; Langlois, D.; Noui, K.; Tasinato, G. Degenerate higher order scalar-tensor theories beyond Horndeski up to cubic order. J. High Energy Phys. 2016, 2016, 100. [Google Scholar] [CrossRef]
- Motohashi, H.; Hu, W. Primordial Black Holes and Slow-Roll Violation. Phys. Rev. 2017, D96, 063503. [Google Scholar] [CrossRef]
- Pi, S.; Wang, J. Primordial Black Hole Formation in Starobinsky’s Linear Potential Model. arXiv 2022, arXiv:2209.14183. [Google Scholar]
- Inoue, S.; Yokoyama, J. Curvature perturbation at the local extremum of the inflation’s potential. Phys. Lett. B 2002, 524, 15–20. [Google Scholar] [CrossRef]
- Tzirakis, K.; Kinney, W.H. Inflation over the hill. Phys. Rev. 2007, D75, 123510. [Google Scholar] [CrossRef]
- Motohashi, H.; Mukohyama, S.; Oliosi, M. Constant Roll and Primordial Black Holes. J. Cosmol. Astropart. Phys. 2020, 2020, 002. [Google Scholar] [CrossRef]
- Byrnes, C.T.; Cole, P.S.; Patil, S.P. Steepest growth of the power spectrum and primordial black holes. J. Cosmol. Astropart. Phys. 2019, 2019, 028. [Google Scholar] [CrossRef]
- Ragavendra, H.V.; Saha, P.; Sriramkumar, L.; Silk, J. Primordial black holes and secondary gravitational waves from ultraslow roll and punctuated inflation. Phys. Rev. D 2021, 103, 083510. [Google Scholar] [CrossRef]
- Ng, K.W.; Wu, Y.P. Constant-rate inflation: Primordial black holes from conformal weight transitions. J. High Energy Phys. 2021, 2021, 076. [Google Scholar] [CrossRef]
- Karam, A.; Koivunen, N.; Tomberg, E.; Vaskonen, V.; Veermäe, H. Anatomy of single-field inflationary models for primordial black holes. J. Cosmol. Astropart. Phys. 2023, 2023, 013. [Google Scholar] [CrossRef]
- Franciolini, G.; Urbano, A. Primordial black hole dark matter from inflation: The reverse engineering approach. Phys. Rev. D 2022, 106, 123519. [Google Scholar] [CrossRef]
- Cole, P.S.; Gow, A.D.; Byrnes, C.T.; Patil, S.P. Steepest growth re-examined: Repercussions for primordial black hole formation. arXiv 2022, arXiv:2204.07573. [Google Scholar]
- Wands, D. Duality invariance of cosmological perturbation spectra. Phys. Rev. 1999, D60, 023507. [Google Scholar] [CrossRef]
- Hunter, J.D. Matplotlib: A 2D Graphics Environment. Comput. Sci. Eng. 2007, 9, 90–95. [Google Scholar] [CrossRef]
- Harris, C.R.; Millman, K.J.; van der Walt, S.J.; Gommers, R.; Virtanen, P.; Cournapeau, D.; Wieser, E.; Taylor, J.; Berg, S.; Smith, N.J.; et al. Array programming with NumPy. Nature 2020, 585, 357–362. [Google Scholar] [CrossRef]
- Virtanen, P.; Gommers, R.; Oliphant, T.E.; Haberland, M.; Reddy, T.; Cournapeau, D.; Burovski, E.; Peterson, P.; Weckesser, W.; Bright, J.; et al. SciPy 1.0: Fundamental algorithms for scientific computing in Python. Nat. Methods 2020, 17, 261–272. [Google Scholar] [CrossRef] [PubMed]
- McKinney, W. Data Structures for Statistical Computing in Python. In Proceedings of the 9th Python in Science Conference, Austin, TX, USA, 28 June–3 July 2010; pp. 56–61. [Google Scholar] [CrossRef]
- Kluyver, T.; Ragan-Kelley, B.; Pérez, F.; Granger, B.; Bussonnier, M.; Frederic, J.; Kelley, K.; Hamrick, J.; Grout, J.; Corlay, S.; et al. Jupyter Notebooks ? A publishing format for reproducible computational workflows. In Positioning and Power in Academic Publishing: Players, Agents and Agendas; Loizides, F., Scmidt, B., Eds.; IOS Press: Amsterdam, The Netherlands, 2016; pp. 87–90. [Google Scholar]
- Carrilho, P.; Malik, K.A.; Mulryne, D.J. Dissecting the growth of the power spectrum for primordial black holes. Phys. Rev. D 2019, 100, 103529. [Google Scholar] [CrossRef]
- Liu, J.; Guo, Z.K.; Cai, R.G. Analytical approximation of the scalar spectrum in the ultraslow-roll inflationary models. Phys. Rev. D 2020, 101, 083535. [Google Scholar] [CrossRef]
- Tasinato, G. An analytic approach to non-slow-roll inflation. Phys. Rev. D 2021, 103, 023535. [Google Scholar] [CrossRef]
- Özsoy, O.; Tasinato, G. CMB μT cross correlations as a probe of primordial black hole scenarios. Phys. Rev. D 2021, 104, 043526. [Google Scholar] [CrossRef]
- Özsoy, O.; Tasinato, G. Consistency conditions and primordial black holes in single field inflation. Phys. Rev. D 2022, 105, 023524. [Google Scholar] [CrossRef]
- Zegeye, D.; Inomata, K.; Hu, W. Spectral distortion anisotropy from inflation for primordial black holes. Phys. Rev. D 2022, 105, 103535. [Google Scholar] [CrossRef]
- Balaji, S.; Ragavendra, H.V.; Sethi, S.K.; Silk, J.; Sriramkumar, L. Observing Nulling of Primordial Correlations via the 21-cm Signal. Phys. Rev. Lett. 2022, 129, 261301. [Google Scholar] [CrossRef]
- Morse, M.J.P.; Kinney, W.H. Large-η constant-roll inflation is never an attractor. Phys. Rev. 2018, D97, 123519. [Google Scholar] [CrossRef]
- Suyama, T.; Tada, Y.; Yamaguchi, M. Revisiting non-Gaussianity in non-attractor inflation models in the light of the cosmological soft theorem. Prog. Theor. Exp. Phys. 2021, 2021, 073E02. [Google Scholar] [CrossRef]
- Davies, M.W.; Carrilho, P.; Mulryne, D.J. Non-Gaussianity in inflationary scenarios for primordial black holes. J. Cosmol. Astropart. Phys. 2022, 2022, 019. [Google Scholar] [CrossRef]
- Starobinsky, A.A. Stochastic de Sitter (inflationary) stage in the early universe. Lect. Notes Phys. 1986, 246, 107–126. [Google Scholar] [CrossRef]
- Nambu, Y.; Sasaki, M. Stochastic Stage of an Inflationary Universe Model. Phys. Lett. B 1988, 205, 441–446. [Google Scholar] [CrossRef]
- Kandrup, H.E. Stochastic inflation as a time dependent random walk. Phys. Rev. D 1989, 39, 2245. [Google Scholar] [CrossRef]
- Nambu, Y. Stochastic Dynamics of an Inflationary Model and Initial Distribution of Universes. Prog. Theor. Phys. 1989, 81, 1037. [Google Scholar] [CrossRef]
- Starobinsky, A.A.; Yokoyama, J. Equilibrium state of a selfinteracting scalar field in the De Sitter background. Phys. Rev. 1994, D50, 6357–6368. [Google Scholar] [CrossRef]
- Finelli, F.; Marozzi, G.; Starobinsky, A.A.; Vacca, G.P.; Venturi, G. Generation of fluctuations during inflation: Comparison of stochastic and field-theoretic approaches. Phys. Rev. D 2009, 79, 044007. [Google Scholar] [CrossRef]
- Burgess, C.P.; Holman, R.; Tasinato, G.; Williams, M. EFT Beyond the Horizon: Stochastic Inflation and How Primordial Quantum Fluctuations Go Classical. J. High Energy Phys. 2015, 2015, 090. [Google Scholar] [CrossRef]
- Vennin, V.; Starobinsky, A.A. Correlation Functions in Stochastic Inflation. Eur. Phys. J. C 2015, 75, 413. [Google Scholar] [CrossRef]
- Burgess, C.P.; Holman, R.; Tasinato, G. Open EFTs, IR effects \& late-time resummations: Systematic corrections in stochastic inflation. J. High Energy Phys. 2016, 2016, 153. [Google Scholar] [CrossRef]
- Ando, K.; Vennin, V. Power spectrum in stochastic inflation. J. Cosmol. Astropart. Phys. 2021, 2021, 057. [Google Scholar] [CrossRef]
- Biagetti, M.; Franciolini, G.; Kehagias, A.; Riotto, A. Primordial Black Holes from Inflation and Quantum Diffusion. J. Cosmol. Astropart. Phys. 2018, 2018, 032. [Google Scholar] [CrossRef]
- Ezquiaga, J.M.; García-Bellido, J. Quantum diffusion beyond slow-roll: Implications for primordial black-hole production. J. Cosmol. Astropart. Phys. 2018, 2018, 018. [Google Scholar] [CrossRef]
- Ballesteros, G.; Rey, J.; Taoso, M.; Urbano, A. Stochastic inflationary dynamics beyond slow-roll and consequences for primordial black hole formation. J. Cosmol. Astropart. Phys. 2020, 2020, 043. [Google Scholar] [CrossRef]
- Cruces, D.; Germani, C.; Prokopec, T. Failure of the stochastic approach to inflation beyond slow-roll. J. Cosmol. Astropart. Phys. 2019, 2019, 048. [Google Scholar] [CrossRef]
- Firouzjahi, H.; Nassiri-Rad, A.; Noorbala, M. Stochastic Ultra Slow Roll Inflation. J. Cosmol. Astropart. Phys. 2019, 2019, 040. [Google Scholar] [CrossRef]
- Pattison, C.; Vennin, V.; Assadullahi, H.; Wands, D. Stochastic inflation beyond slow roll. J. Cosmol. Astropart. Phys. 2019, 2019, 031. [Google Scholar] [CrossRef]
- Vennin, V. Stochastic Inflation and Primordial Black Holes. Habilitation Thesis, Universite Paris-Saclay, Bures-sur-Yvette, France, 2020. [Google Scholar]
- Rigopoulos, G.; Wilkins, A. Inflation is always semi-classical: Diffusion domination overproduces Primordial Black Holes. J. Cosmol. Astropart. Phys. 2021, 2021, 027. [Google Scholar] [CrossRef]
- Pattison, C.; Vennin, V.; Assadullahi, H.; Wands, D. Quantum diffusion during inflation and primordial black holes. J. Cosmol. Astropart. Phys. 2017, 2017, 046. [Google Scholar] [CrossRef]
- Ezquiaga, J.M.; García-Bellido, J.; Vennin, V. The exponential tail of inflationary fluctuations: Consequences for primordial black holes. J. Cosmol. Astropart. Phys. 2020, 2020, 029. [Google Scholar] [CrossRef]
- Figueroa, D.G.; Raatikainen, S.; Rasanen, S.; Tomberg, E. Non-Gaussian Tail of the Curvature Perturbation in Stochastic Ultraslow-Roll Inflation: Implications for Primordial Black Hole Production. Phys. Rev. Lett. 2021, 127, 101302. [Google Scholar] [CrossRef]
- Figueroa, D.G.; Raatikainen, S.; Rasanen, S.; Tomberg, E. Implications of stochastic effects for primordial black hole production in ultra-slow-roll inflation. J. Cosmol. Astropart. Phys. 2022, 2022, 027. [Google Scholar] [CrossRef]
- Pi, S.; Sasaki, M. Logarithmic Duality of the Curvature Perturbation. arXiv 2022, arXiv:2211.13932. [Google Scholar]
- Cai, R.G.; Guo, Z.K.; Liu, J.; Liu, L.; Yang, X.Y. Primordial black holes and gravitational waves from parametric amplification of curvature perturbations. J. Cosmol. Astropart. Phys. 2020, 2020, 013. [Google Scholar] [CrossRef]
- Ballesteros, G.; Rey, J.; Rompineve, F. Detuning primordial black hole dark matter with early matter domination and axion monodromy. J. Cosmol. Astropart. Phys. 2020, 2020, 014. [Google Scholar] [CrossRef]
- Kristiano, J.; Yokoyama, J. Why Must Primordial Non-Gaussianity Be Very Small? Phys. Rev. Lett. 2022, 128, 061301. [Google Scholar] [CrossRef]
- Meng, D.S.; Yuan, C.; Huang, Q.G. One-loop correction to the enhanced curvature perturbation with local-type non-Gaussianity for the formation of primordial black holes. Phys. Rev. D 2022, 106, 063508. [Google Scholar] [CrossRef]
- Kristiano, J.; Yokoyama, J. Ruling Out Primordial Black Hole Formation From Single-Field Inflation. arXiv 2022, arXiv:2211.03395. [Google Scholar]
- Inomata, K.; Braglia, M.; Chen, X. Questions on calculation of primordial power spectrum with large spikes: The resonance model case. J. Cosmol. Astropart. Phys. 2023, 2023, 011. [Google Scholar] [CrossRef]
- Riotto, A. The Primordial Black Hole Formation from Single-Field Inflation is Not Ruled Out. arXiv 2023, arXiv:2301.00599. [Google Scholar]
- Arya, R. Formation of Primordial Black Holes from Warm Inflation. J. Cosmol. Astropart. Phys. 2020, 2020, 042. [Google Scholar] [CrossRef]
- Bastero-Gil, M.; Díaz-Blanco, M.S. Gravity waves and primordial black holes in scalar warm little inflation. J. Cosmol. Astropart. Phys. 2021, 2021, 052. [Google Scholar] [CrossRef]
- Ballesteros, G.; García, M.A.G.; Rodríguez, A.P.; Pierre, M.; Rey, J. Primordial black holes and gravitational waves from dissipation during inflation. J. Cosmol. Astropart. Phys. 2022, 2022, 006. [Google Scholar] [CrossRef]
- Pi, S.; Sasaki, M. Primordial Black Hole Formation in Non-Minimal Curvaton Scenario. arXiv 2021, arXiv:2112.12680. [Google Scholar]
- Meng, D.S.; Yuan, C.; Huang, Q.G. Primordial black holes generated by the non-minimal spectator field. arXiv 2022, arXiv:2212.03577. [Google Scholar]
- Wilczek, F. Problem of Strong P and T Invariance in the Presence of Instantons. Phys. Rev. Lett. 1978, 40, 279–282. [Google Scholar] [CrossRef]
- Weinberg, S. A New Light Boson? Phys. Rev. Lett. 1978, 40, 223–226. [Google Scholar] [CrossRef]
- Peccei, R.D.; Quinn, H.R. CP Conservation in the Presence of Instantons. Phys. Rev. Lett. 1977, 38, 1440–1443. [Google Scholar] [CrossRef]
- Peccei, R.D.; Quinn, H.R. Constraints Imposed by CP Conservation in the Presence of Instantons. Phys. Rev. D 1977, 16, 1791–1797. [Google Scholar] [CrossRef]
- Svrcek, P.; Witten, E. Axions In String Theory. J. High Energy Phys. 2006, 2006, 051. [Google Scholar] [CrossRef]
- Marsh, D.J.E. Axion Cosmology. Phys. Rep. 2016, 643, 1–79. [Google Scholar] [CrossRef]
- Freese, K.; Frieman, J.A.; Olinto, A.V. Natural inflation with pseudo—Nambu-Goldstone bosons. Phys. Rev. Lett. 1990, 65, 3233–3236. [Google Scholar] [CrossRef] [PubMed]
- McAllister, L.; Silverstein, E.; Westphal, A. Gravity Waves and Linear Inflation from Axion Monodromy. Phys. Rev. 2010, D82, 046003. [Google Scholar] [CrossRef]
- Silverstein, E.; Westphal, A. Monodromy in the CMB: Gravity Waves and String Inflation. Phys. Rev. D 2008, 78, 106003. [Google Scholar] [CrossRef]
- McAllister, L.; Silverstein, E.; Westphal, A.; Wrase, T. The Powers of Monodromy. J. High Energy Phys. 2014, 2014, 123. [Google Scholar] [CrossRef]
- Flauger, R.; McAllister, L.; Silverstein, E.; Westphal, A. Drifting Oscillations in Axion Monodromy. J. Cosmol. Astropart. Phys. 2017, 2017, 055. [Google Scholar] [CrossRef]
- Anber, M.M.; Sorbo, L. N-flationary magnetic fields. J. Cosmol. Astropart. Phys. 2006, 2006, 018. [Google Scholar] [CrossRef]
- Anber, M.M.; Sorbo, L. Naturally inflating on steep potentials through electromagnetic dissipation. Phys. Rev. 2010, D81, 043534. [Google Scholar] [CrossRef]
- Barnaby, N.; Peloso, M. Large Nongaussianity in Axion Inflation. Phys. Rev. Lett. 2011, 106, 181301. [Google Scholar] [CrossRef]
- Barnaby, N.; Namba, R.; Peloso, M. Phenomenology of a Pseudo-Scalar Inflaton: Naturally Large Nongaussianity. J. Cosmol. Astropart. Phys. 2011, 2011, 009. [Google Scholar] [CrossRef]
- Pajer, E.; Peloso, M. A review of Axion Inflation in the era of Planck. Class. Quant. Grav. 2013, 30, 214002. [Google Scholar] [CrossRef]
- Peloso, M.; Sorbo, L.; Unal, C. Rolling axions during inflation: Perturbativity and signatures. J. Cosmol. Astropart. Phys. 2016, 2016, 001. [Google Scholar] [CrossRef]
- Özsoy, O. On Synthetic Gravitational Waves from Multi-field Inflation. J. Cosmol. Astropart. Phys. 2018, 2018, 062. [Google Scholar] [CrossRef]
- Barnaby, N.; Pajer, E.; Peloso, M. Gauge Field Production in Axion Inflation: Consequences for Monodromy, non-Gaussianity in the CMB, and Gravitational Waves at Interferometers. Phys. Rev. 2012, D85, 023525. [Google Scholar] [CrossRef]
- Meerburg, P.D.; Pajer, E. Observational Constraints on Gauge Field Production in Axion Inflation. J. Cosmol. Astropart. Phys. 2013, 2013, 017. [Google Scholar] [CrossRef]
- Ade, P.A.R.; Aghanim, N.; Arnaud, M.; Arroja, F.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Ballardini, M.; Banday, A.J.; Barreiro, R.B.; et al. Planck 2015 results. XX. Constraints on inflation. Astron. Astrophys. 2016, 594, A20. [Google Scholar]
- Caravano, A.; Komatsu, E.; Lozanov, K.D.; Weller, J. Lattice Simulations of Axion-U(1) Inflation. arXiv 2022, arXiv:2204.12874. [Google Scholar]
- Cheng, S.L.; Lee, W.; Ng, K.W. Numerical study of pseudoscalar inflation with an axion-gauge field coupling. Phys. Rev. D 2016, 93, 063510. [Google Scholar] [CrossRef]
- Dall’Agata, G.; González-Martín, S.; Papageorgiou, A.; Peloso, M. Warm dark energy. J. Cosmol. Astropart. Phys. 2020, 2020, 032. [Google Scholar] [CrossRef]
- Domcke, V.; Guidetti, V.; Welling, Y.; Westphal, A. Resonant backreaction in axion inflation. J. Cosmol. Astropart. Phys. 2020, 2020, 009. [Google Scholar] [CrossRef]
- Gorbar, E.V.; Schmitz, K.; Sobol, O.O.; Vilchinskii, S.I. Gauge-field production during axion inflation in the gradient expansion formalism. Phys. Rev. D 2021, 104, 123504. [Google Scholar] [CrossRef]
- Peloso, M.; Sorbo, L. Instability in axion inflation with strong backreaction from gauge modes. J. Cosmol. Astropart. Phys. 2023, 2023, 038. [Google Scholar] [CrossRef]
- Cheng, S.L.; Lee, W.; Ng, K.W. Production of high stellar-mass primordial black holes in trapped inflation. J. High Energy Phys. 2017, 2017, 008. [Google Scholar] [CrossRef]
- Kallosh, R.; Linde, A.; Vercnocke, B. Natural Inflation in Supergravity and Beyond. Phys. Rev. 2014, D90, 041303. [Google Scholar] [CrossRef]
- Kobayashi, T.; Oikawa, A.; Otsuka, H. New potentials for string axion inflation. Phys. Rev. 2016, D93, 083508. [Google Scholar] [CrossRef]
- Cabo Bizet, N.; Loaiza-Brito, O.; Zavala, I. Mirror quintic vacua: Hierarchies and inflation. J. High Energy Phys. 2016, 2016, 082. [Google Scholar] [CrossRef]
- Parameswaran, S.; Tasinato, G.; Zavala, I. Subleading Effects and the Field Range in Axion Inflation. J. Cosmol. Astropart. Phys. 2016, 2016, 008. [Google Scholar] [CrossRef]
- Bhattacharya, S.; Zavala, I. Sharp turns in axion monodromy: Primordial black holes and gravitational waves. arXiv 2022, arXiv:2205.06065. [Google Scholar]
- Namba, R.; Peloso, M.; Shiraishi, M.; Sorbo, L.; Unal, C. Scale-dependent gravitational waves from a rolling axion. J. Cosmol. Astropart. Phys. 2016, 2016, 041. [Google Scholar] [CrossRef]
- Ferreira, R.Z.; Sloth, M.S. Universal Constraints on Axions from Inflation. J. High Energy Phys. 2014, 2014, 139. [Google Scholar] [CrossRef]
- Bassett, B.A.; Tsujikawa, S.; Wands, D. Inflation dynamics and reheating. Rev. Mod. Phys. 2006, 78, 537–589. [Google Scholar] [CrossRef]
- Groot Nibbelink, S.; van Tent, B.J.W. Density perturbations arising from multiple field slow roll inflation. arXiv 2000, arXiv:hep-ph/0011325. [Google Scholar]
- Groot Nibbelink, S.; van Tent, B.J.W. Scalar perturbations during multiple field slow-roll inflation. Class. Quant. Grav. 2002, 19, 613–640. [Google Scholar] [CrossRef]
- Sasaki, M.; Stewart, E.D. A General analytic formula for the spectral index of the density perturbations produced during inflation. Prog. Theor. Phys. 1996, 95, 71–78. [Google Scholar] [CrossRef]
- Langlois, D.; Renaux-Petel, S. Perturbations in generalized multi-field inflation. J. Cosmol. Astropart. Phys. 2008, 2008, 017. [Google Scholar] [CrossRef]
- Garcia-Saenz, S.; Renaux-Petel, S.; Ronayne, J. Primordial fluctuations and non-Gaussianities in sidetracked inflation. J. Cosmol. Astropart. Phys. 2018, 2018, 057. [Google Scholar] [CrossRef]
- Garcia-Saenz, S.; Renaux-Petel, S. Flattened non-Gaussianities from the effective field theory of inflation with imaginary speed of sound. J. Cosmol. Astropart. Phys. 2018, 2018, 005. [Google Scholar] [CrossRef]
- Bjorkmo, T.; Ferreira, R.Z.; Marsh, M.C.D. Mild Non-Gaussianities under Perturbative Control from Rapid-Turn Inflation Models. J. Cosmol. Astropart. Phys. 2019, 2019, 036. [Google Scholar] [CrossRef]
- Fumagalli, J.; Renaux-Petel, S.; Witkowski, L.T. Oscillations in the stochastic gravitational wave background from sharp features and particle production during inflation. J. Cosmol. Astropart. Phys. 2021, 2021, 030. [Google Scholar] [CrossRef]
- Chluba, J.; Hamann, J.; Patil, S.P. Features and New Physical Scales in Primordial Observables: Theory and Observation. Int. J. Mod. Phys. D 2015, 24, 1530023. [Google Scholar] [CrossRef]
- Slosar, A.; Chen, X.; Dvorkin, C.; Green, D.; Meerburg, P.D.; Silverstein, E.; Wallisch, B. Scratches from the Past: Inflationary Archaeology through Features in the Power Spectrum of Primordial Fluctuations. Bull. Am. Astron. Soc. 2019, 51, 98. [Google Scholar]
- Anguelova, L. On Primordial Black Holes from Rapid Turns in Two-field Models. J. Cosmol. Astropart. Phys. 2021, 2021, 004. [Google Scholar] [CrossRef]
- Fumagalli, J.; Garcia-Saenz, S.; Pinol, L.; Renaux-Petel, S.; Ronayne, J. Hyper-Non-Gaussianities in Inflation with Strongly Nongeodesic Motion. Phys. Rev. Lett. 2019, 123, 201302. [Google Scholar] [CrossRef]
- Ferreira, R.Z. Non-Gaussianities in models of inflation with large and negative entropic masses. J. Cosmol. Astropart. Phys. 2020, 2020, 034. [Google Scholar] [CrossRef]
- Wu, K.K.S.; Lahav, O.; Rees, M.J. The large-scale smoothness of the Universe. Nature 1999, 397, 225–230. [Google Scholar] [CrossRef]
- Yadav, J.; Bharadwaj, S.; Pandey, B.; Seshadri, T.R. Testing homogeneity on large scales in the Sloan Digital Sky Survey Data Release One. Mon. Not. R. Astron. Soc. 2005, 364, 601–606. [Google Scholar] [CrossRef]
- Friedmann, A. On the Possibility of a world with constant negative curvature of space. Z. Phys. 1924, 21, 326–332. [Google Scholar] [CrossRef]
- Lemaitre, G. The expanding universe. Ann. Soc. Sci. Brux. A 1933, 53, 51–85. [Google Scholar] [CrossRef]
- Robertson, H.P. Kinematics and World-Structure. Astrophys. J. 1935, 82, 284–301. [Google Scholar] [CrossRef]
- Walker, A.G. On Milne’s Theory of World-Structure. Proc. Lond. Math. Soc. 1937, 42, 90–127. [Google Scholar] [CrossRef]
- Baumann, D. Inflation. In Proceedings of the Theoretical Advanced Study Institute in Elementary Particle Physics: Physics of the Large and the Small, Boulder, CO, USA, 1–26 June 2009; pp. 523–686. [Google Scholar] [CrossRef]
- Kolb, E.W.; Turner, M.S. The Early Universe; CRC Press: Boca Raton, FL, USA, 1990; Volume 69. [Google Scholar] [CrossRef]
- Mukhanov, V. Physical Foundations of Cosmology; Cambridge University Press: Oxford, UK, 2005. [Google Scholar]
- Lyth, D.H.; Malik, K.A.; Sasaki, M. A General proof of the conservation of the curvature perturbation. J. Cosmol. Astropart. Phys. 2005, 2005, 004. [Google Scholar] [CrossRef]
- Flauger, R.; McAllister, L.; Pajer, E.; Westphal, A.; Xu, G. Oscillations in the CMB from Axion Monodromy Inflation. J. Cosmol. Astropart. Phys. 2010, 2010, 009. [Google Scholar] [CrossRef]
- Flauger, R.; Pajer, E. Resonant Non-Gaussianity. J. Cosmol. Astropart. Phys. 2011, 2011, 017. [Google Scholar] [CrossRef]
- Maldacena, J.M. Non-Gaussian features of primordial fluctuations in single field inflationary models. J. High Energy Phys. 2003, 2003, 013. [Google Scholar] [CrossRef]
- Adshead, P.; Giblin, J.T.; Scully, T.R.; Sfakianakis, E.I. Gauge-preheating and the end of axion inflation. J. Cosmol. Astropart. Phys. 2015, 2015, 034. [Google Scholar] [CrossRef]
- Barnaby, N.; Moxon, J.; Namba, R.; Peloso, M.; Shiu, G.; Zhou, P. Gravity waves and non-Gaussian features from particle production in a sector gravitationally coupled to the inflaton. Phys. Rev. 2012, D86, 103508. [Google Scholar] [CrossRef]
- Sorbo, L. Parity violation in the Cosmic Microwave Background from a pseudoscalar inflaton. J. Cosmol. Astropart. Phys. 2011, 2011, 003. [Google Scholar] [CrossRef]
- Cook, J.L.; Sorbo, L. Particle production during inflation and gravitational waves detectable by ground-based interferometers. Phys. Rev. D 2012, 85, 023534. [Google Scholar] [CrossRef]
- Özsoy, O. Parity violating non-Gaussianity from axion-gauge field dynamics. Phys. Rev. D 2021, 104, 123523. [Google Scholar] [CrossRef]
- Campeti, P.; Özsoy, O.; Obata, I.; Shiraishi, M. New constraints on axion-gauge field dynamics during inflation from Planck and BICEP/Keck data sets. J. Cosmol. Astropart. Phys. 2022, 2022, 039. [Google Scholar] [CrossRef]
- Malik, K.A.; Wands, D. Cosmological perturbations. Phys. Rep. 2009, 475, 1–51. [Google Scholar] [CrossRef]
- Notari, A.; Tywoniuk, K. Dissipative Axial Inflation. J. Cosmol. Astropart. Phys. 2016, 2016, 038. [Google Scholar] [CrossRef]
- Caprini, C.; Guzzetti, M.C.; Sorbo, L. Inflationary magnetogenesis with added helicity: Constraints from non-gaussianities. Class. Quantum Gravity 2018, 35, 124003. [Google Scholar] [CrossRef]
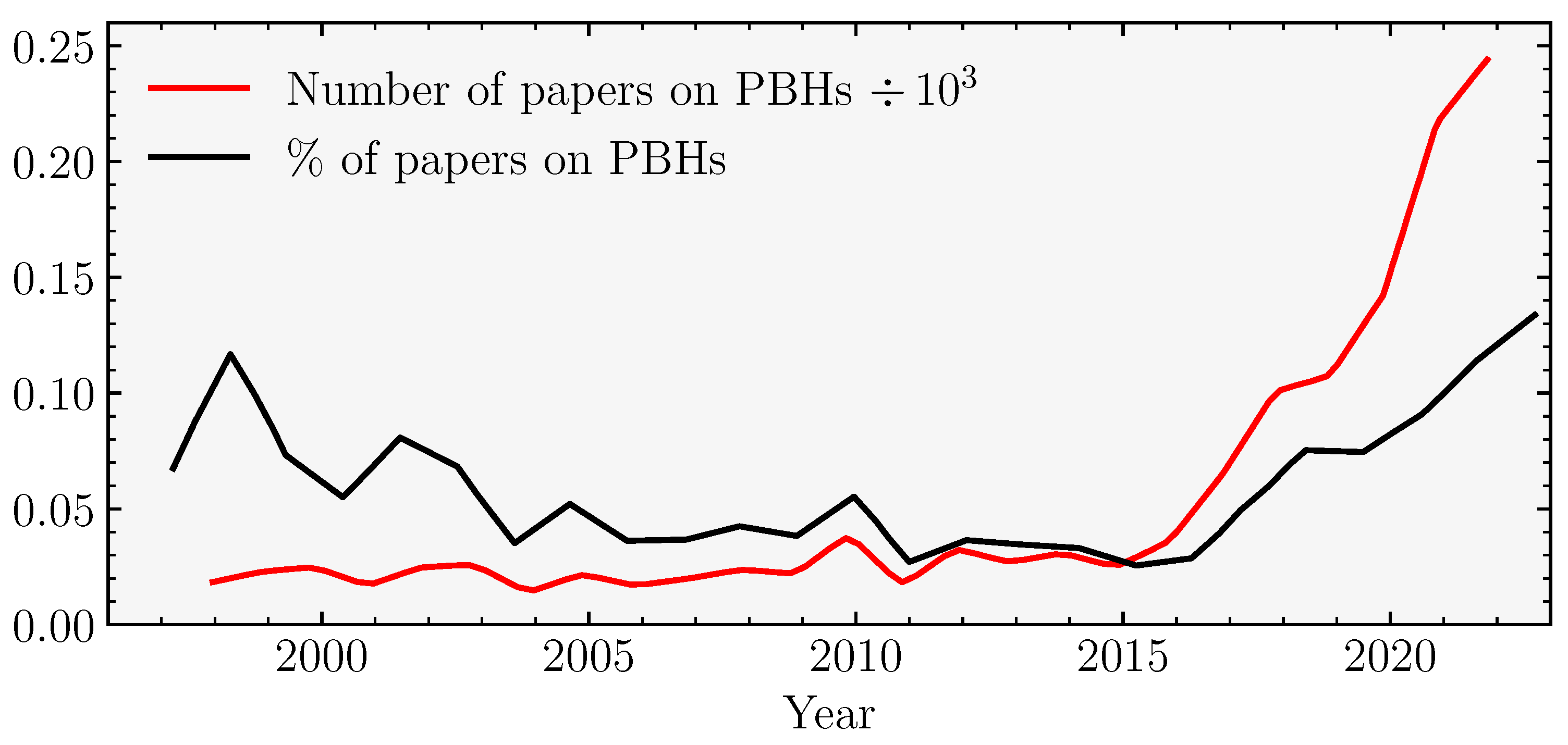
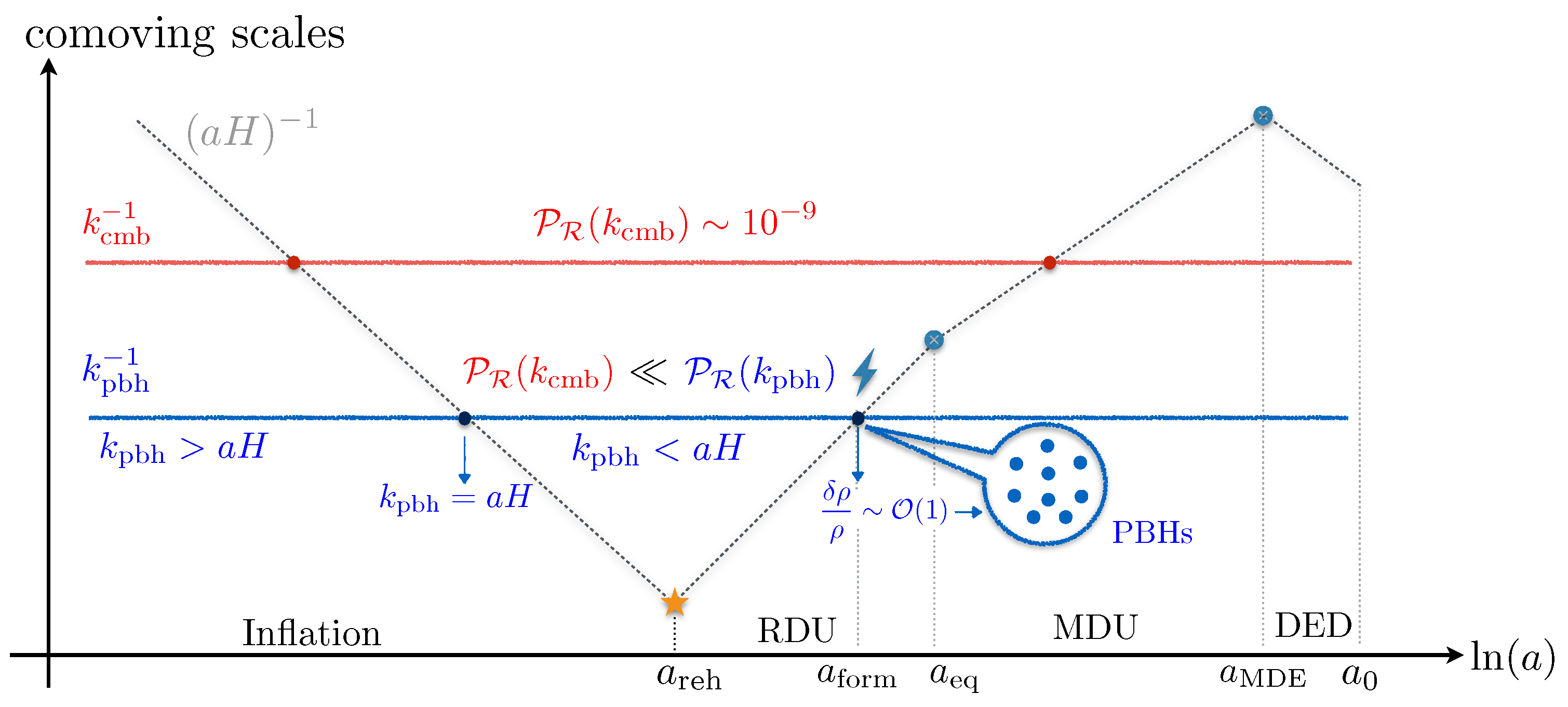
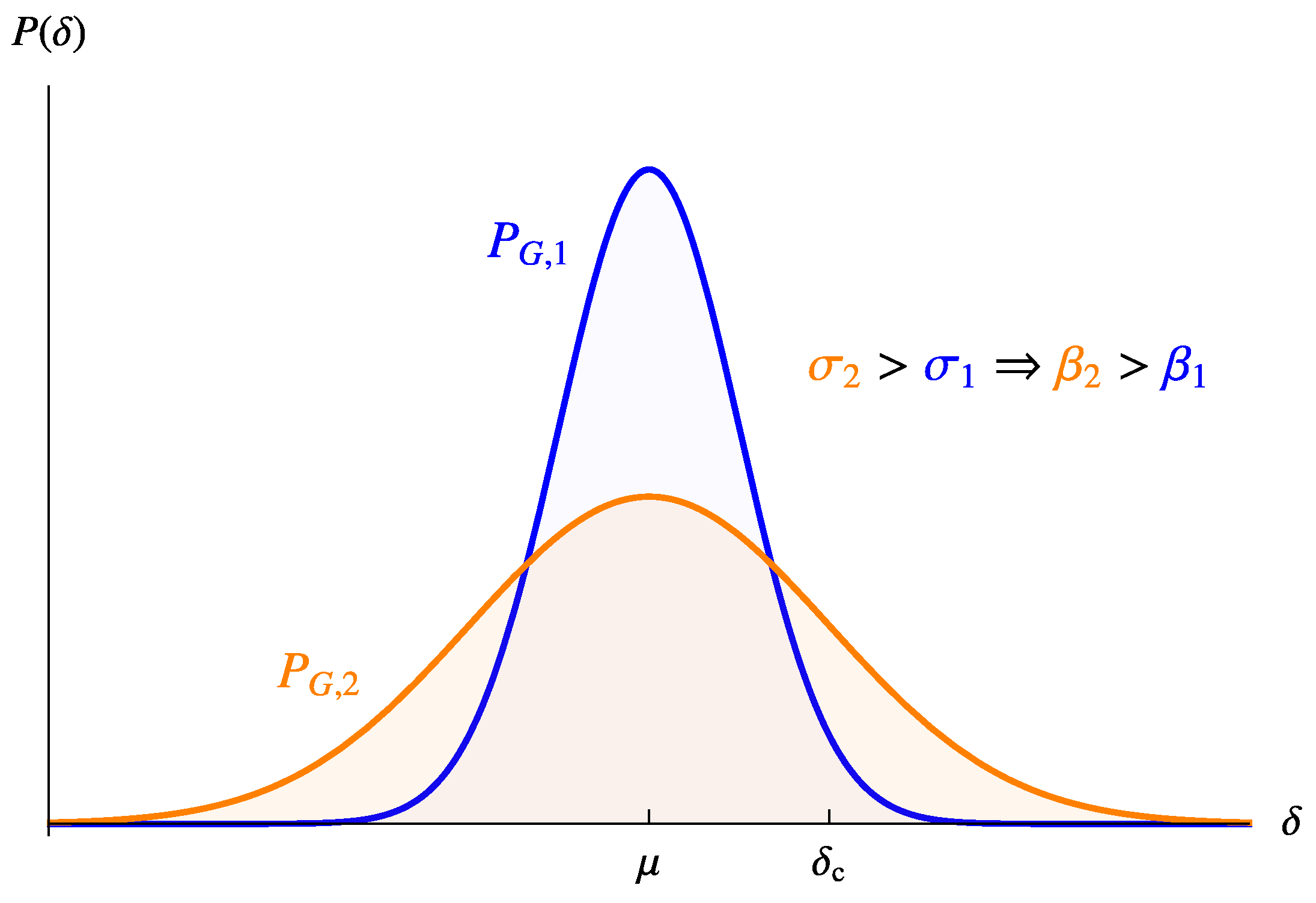
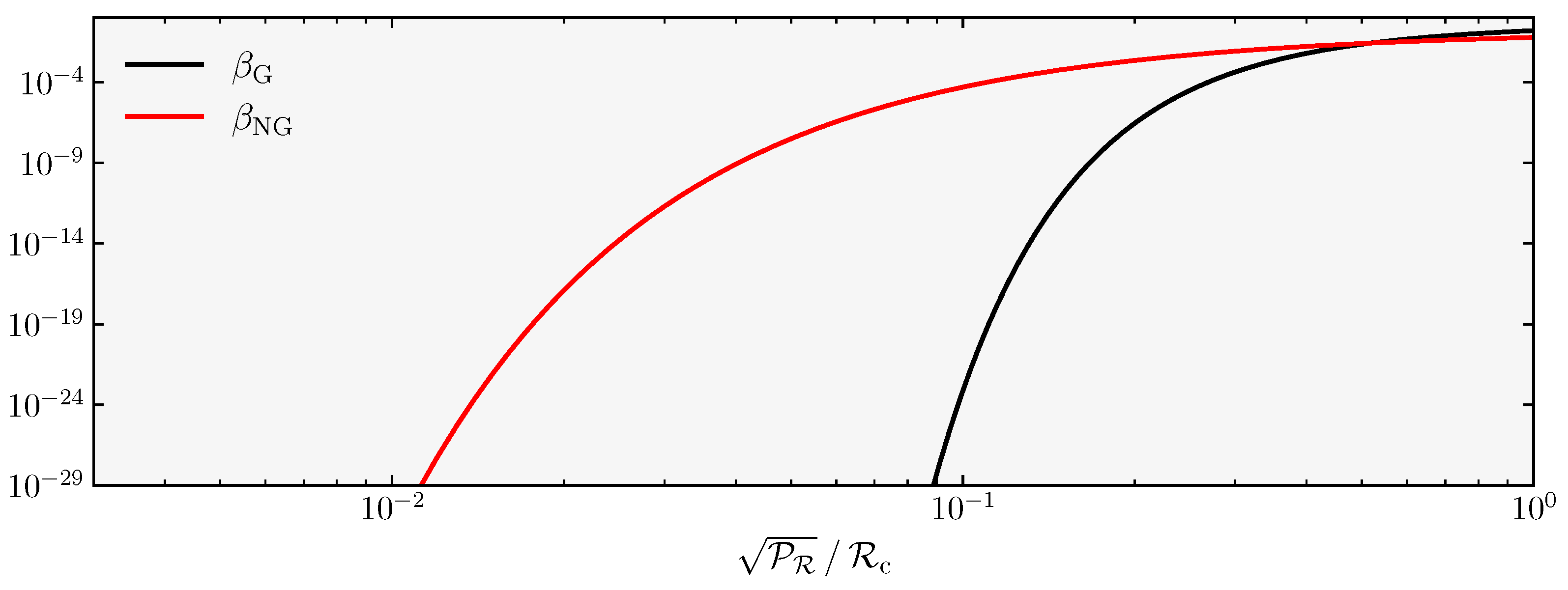







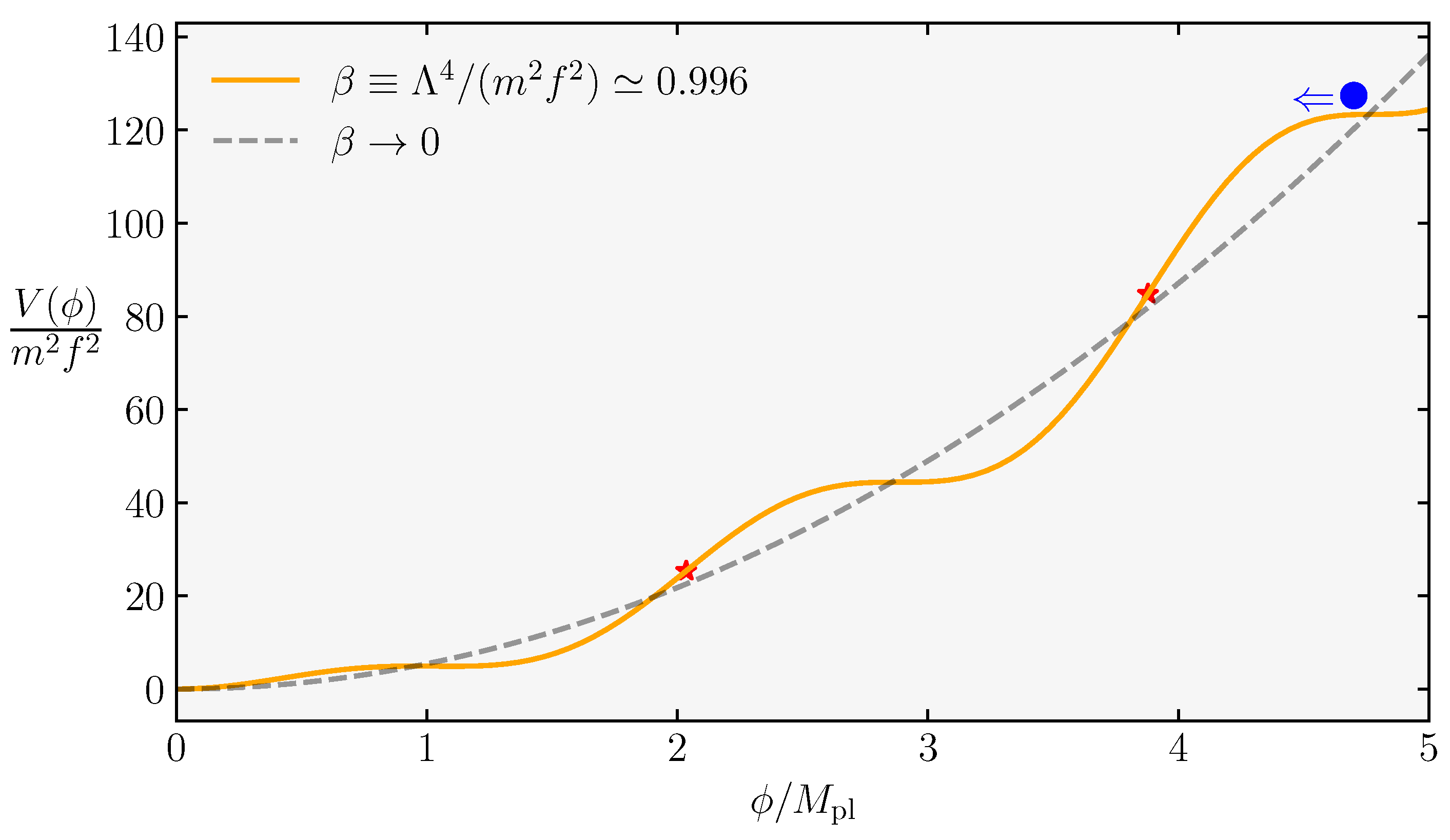
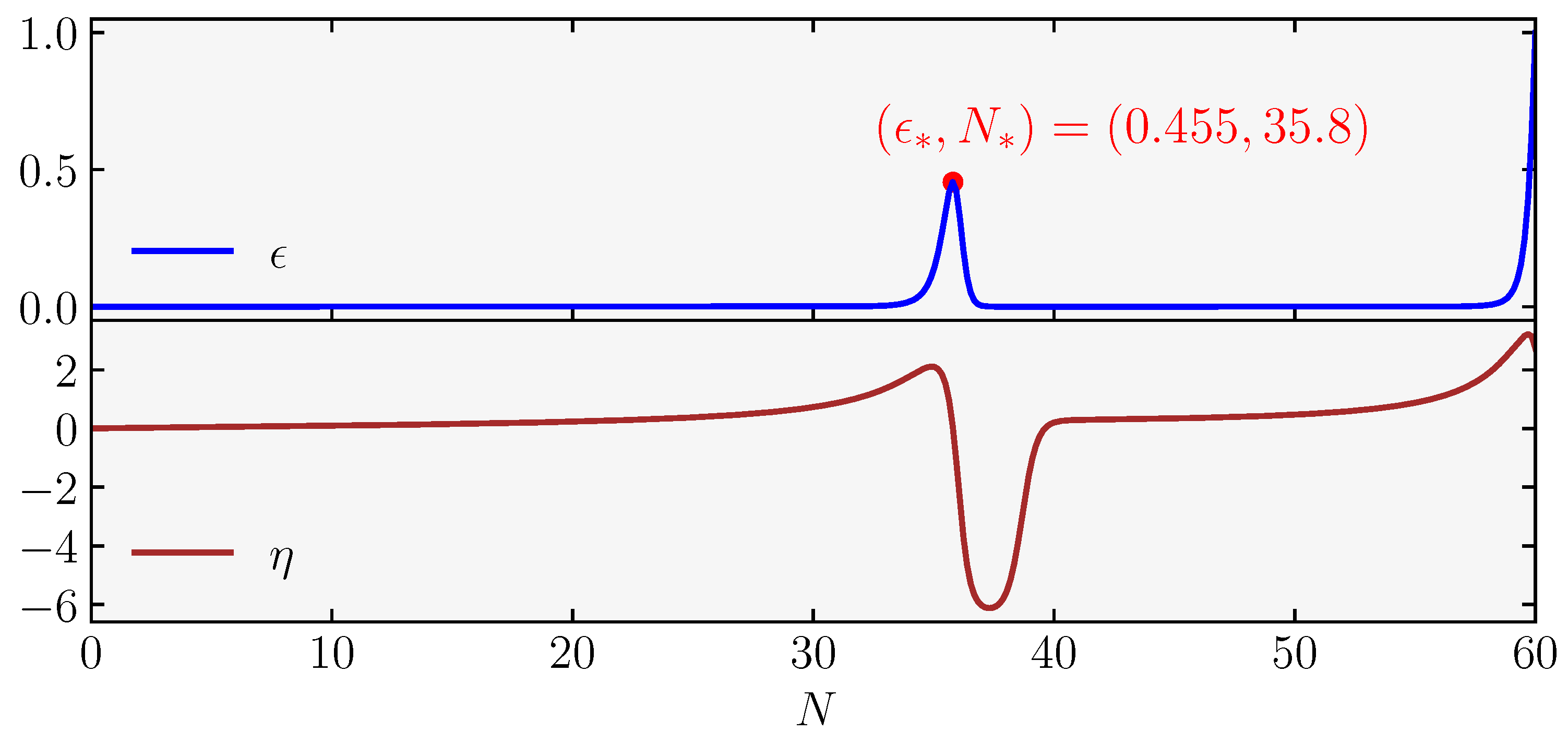

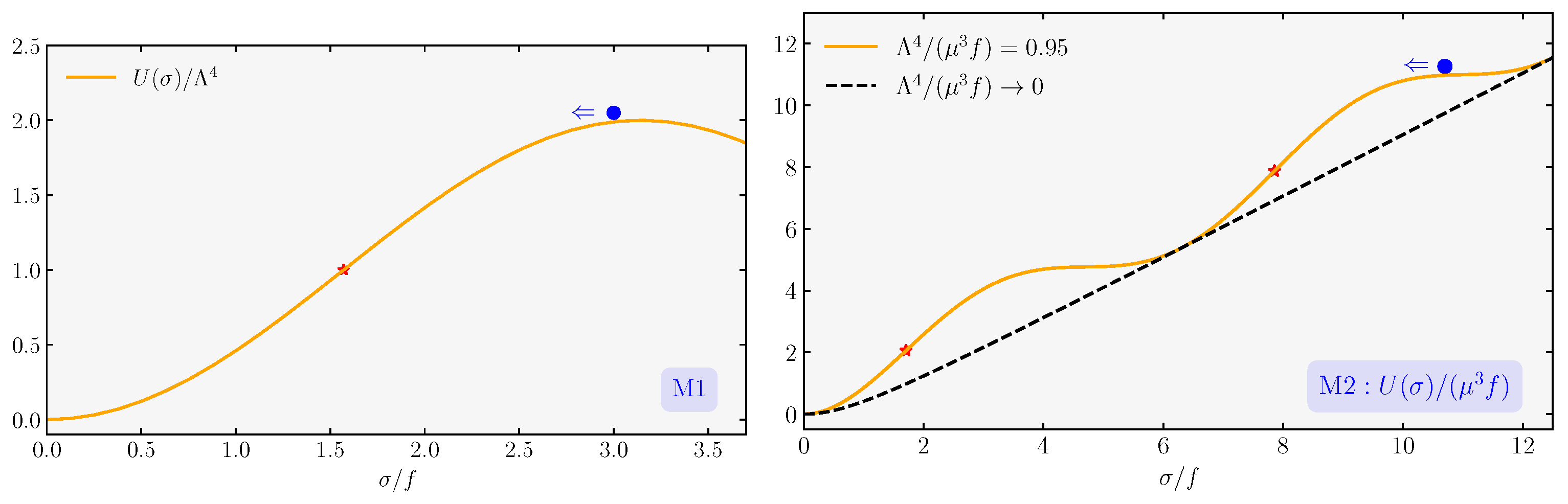

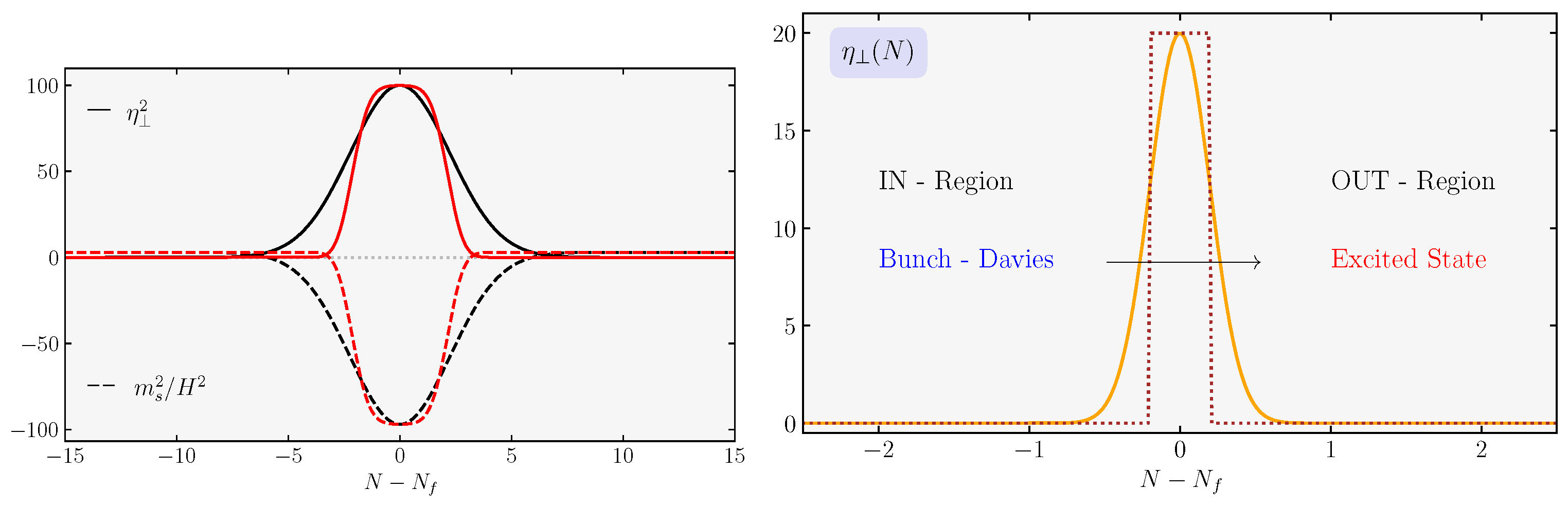
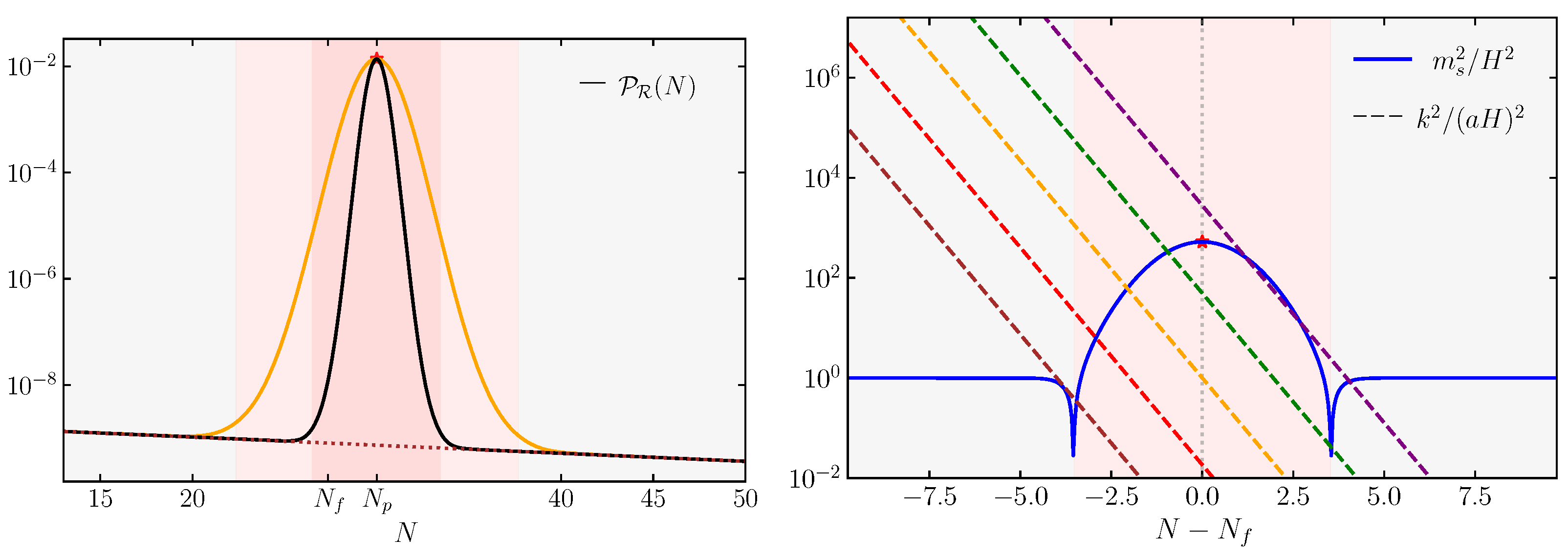

| M | ||||
|---|---|---|---|---|
| 14 | ||||
| Phase I | Phase II | Phase III | ||
|---|---|---|---|---|
| 0.30 | 3.00 | |||
| 35.7 | 55.0 | |||
| 55.0 | 65.0 | |||
| 1.00 | 2.00 | |||
| X | ||||
|---|---|---|---|---|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Özsoy, O.; Tasinato, G. Inflation and Primordial Black Holes. Universe 2023, 9, 203. https://doi.org/10.3390/universe9050203
Özsoy O, Tasinato G. Inflation and Primordial Black Holes. Universe. 2023; 9(5):203. https://doi.org/10.3390/universe9050203
Chicago/Turabian StyleÖzsoy, Ogan, and Gianmassimo Tasinato. 2023. "Inflation and Primordial Black Holes" Universe 9, no. 5: 203. https://doi.org/10.3390/universe9050203
APA StyleÖzsoy, O., & Tasinato, G. (2023). Inflation and Primordial Black Holes. Universe, 9(5), 203. https://doi.org/10.3390/universe9050203







